Study of Stress and Wear Behavior of Internal Components in Hydrogen-Based Shaft Furnaces Based on the Discrete Element Method (DEM) Model
Abstract
1. Introduction
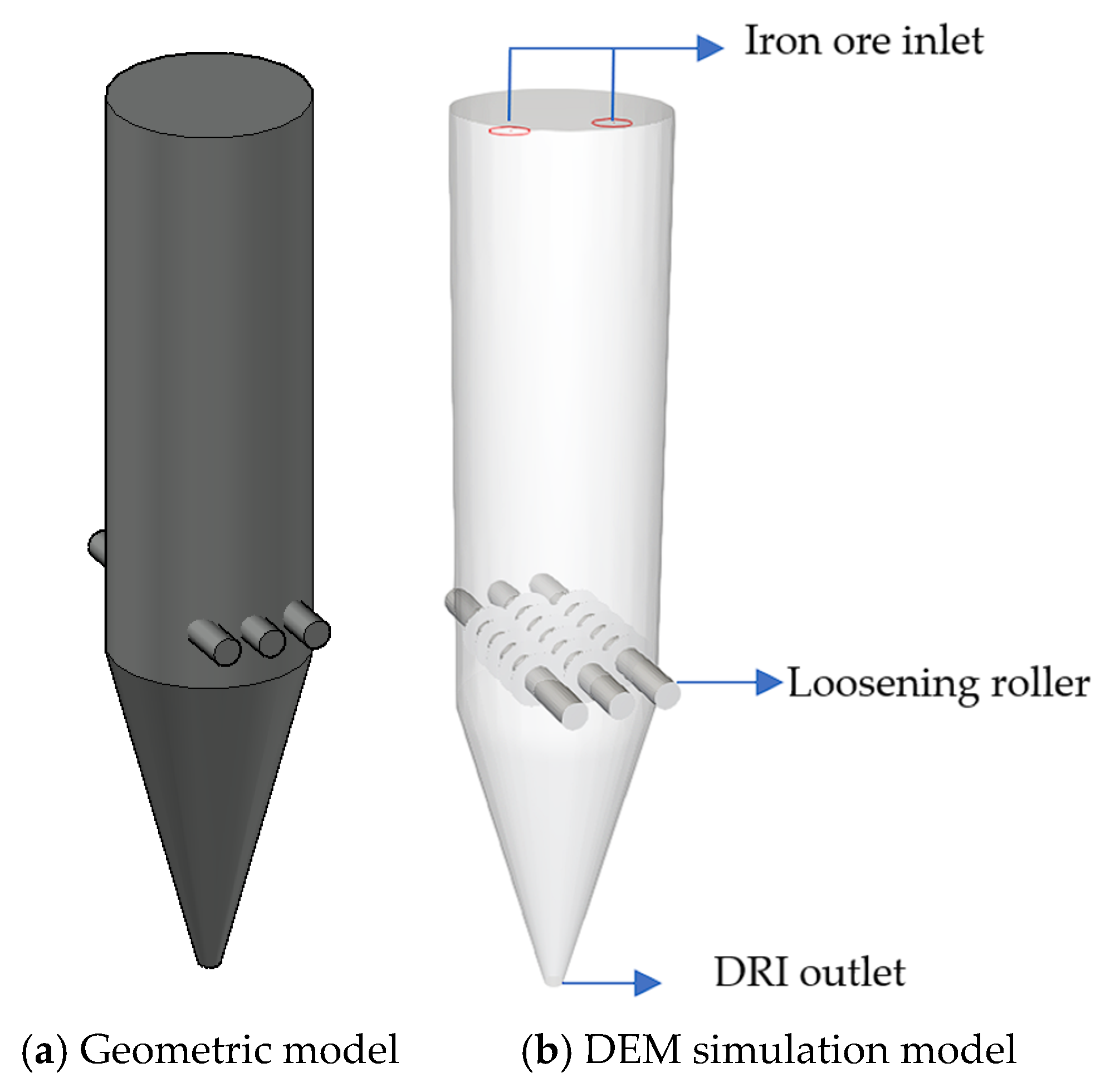
2. Discrete Element Method (DEM) Model
3. Model Parameters and Simulation Solution
- (1)
- The gas flow inside the hydrogen-based shaft furnace is in a steady state;
- (2)
- Each iron ore pellet has the same size;
- (3)
- Only consider the wear and tear of the shaft furnace wall caused by the ore pellet, without taking into account the deformation of the ore pellet;
- (4)
- Only consider the impact of ball ore movement on the erosion and wear of furnace walls and linings, ignoring the influence of temperature;
- (5)
- The loose roller has its own cooling effect and can operate normally.
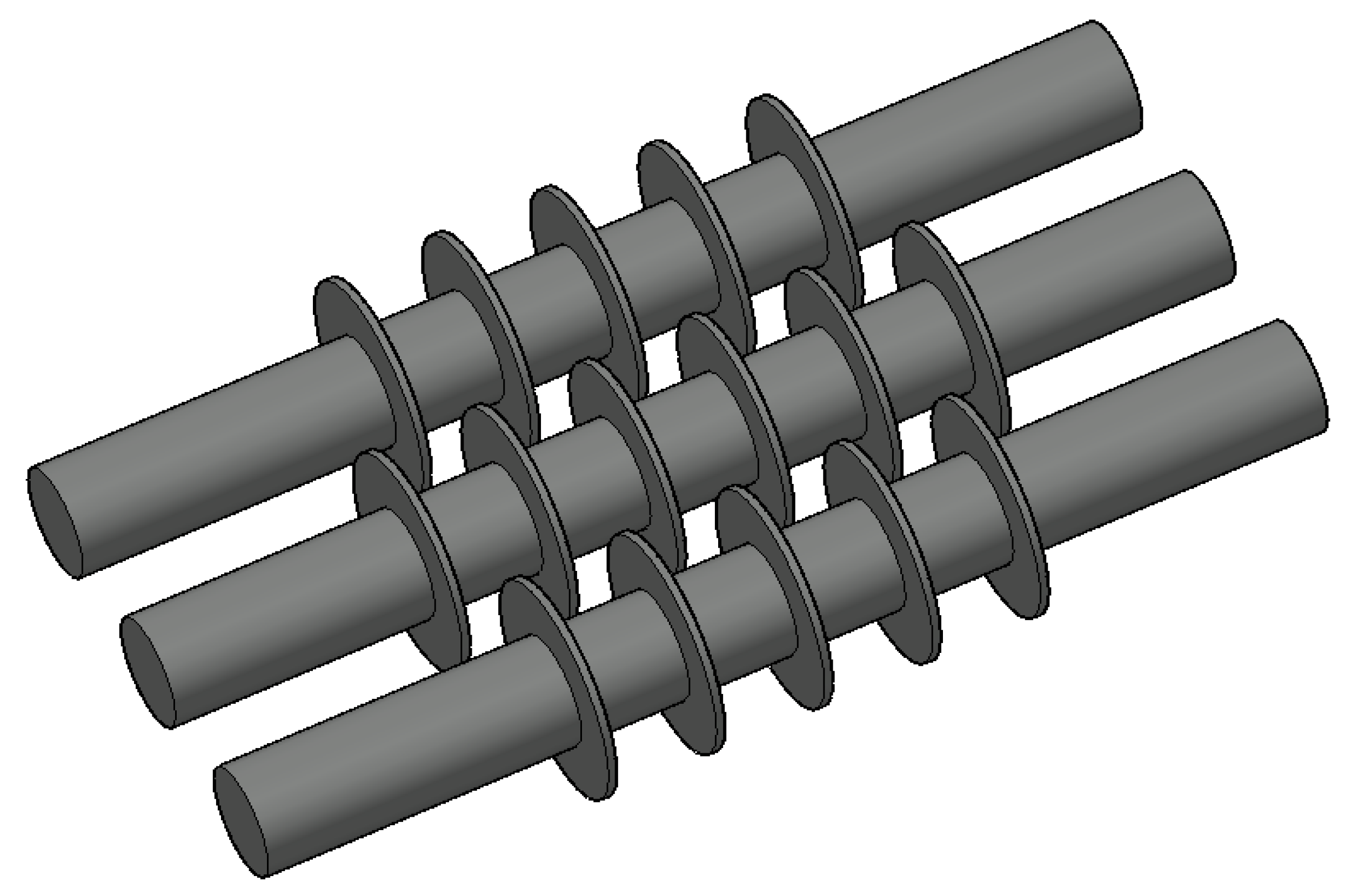
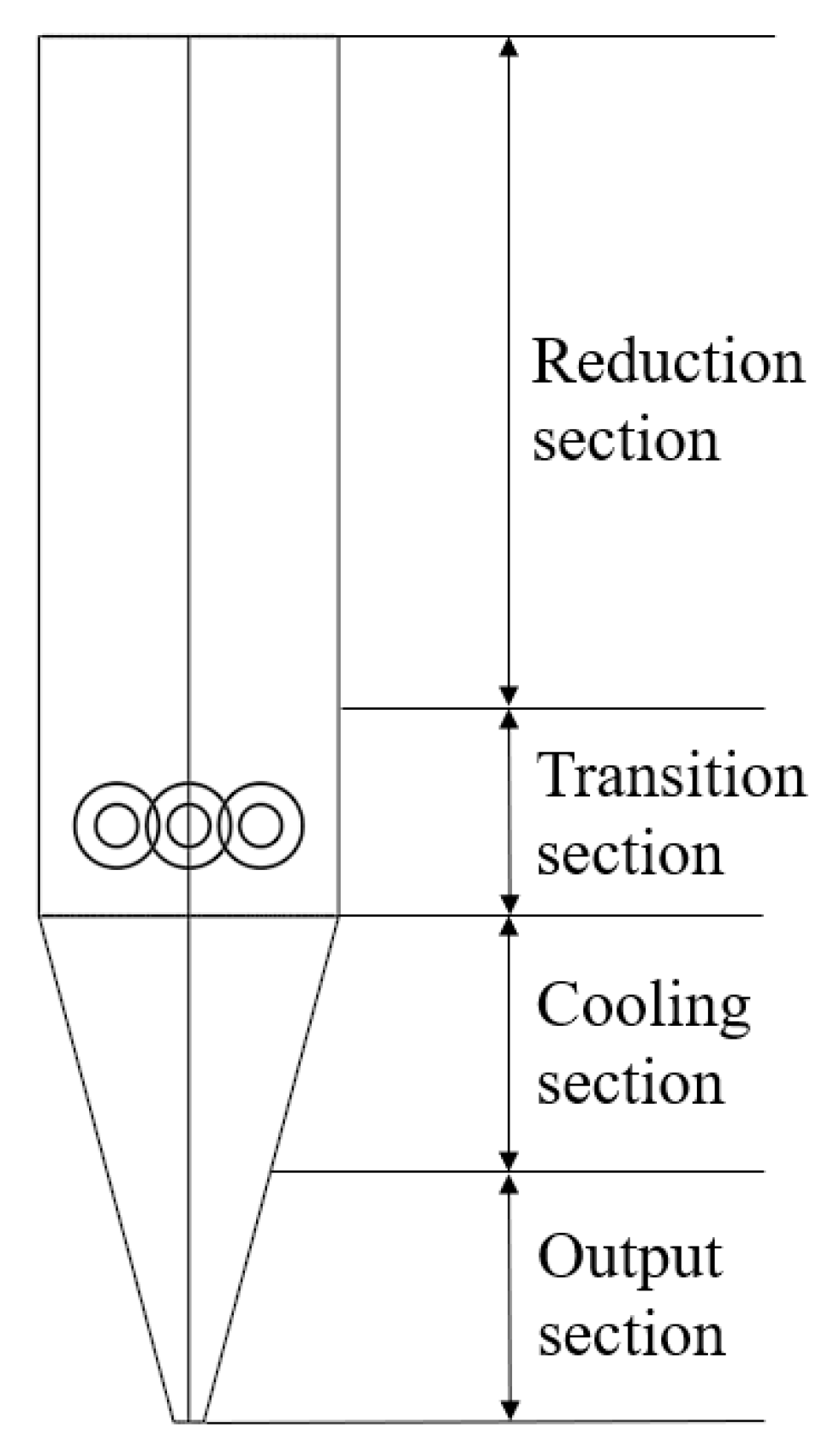
3.1. Model Parameter
3.2. Simulated Conditions
3.3. Numerical Solution
4. Results and Discussion
4.1. Analysis of Stress and Wear on the Wall of Hydrogen-Based Shaft Furnace
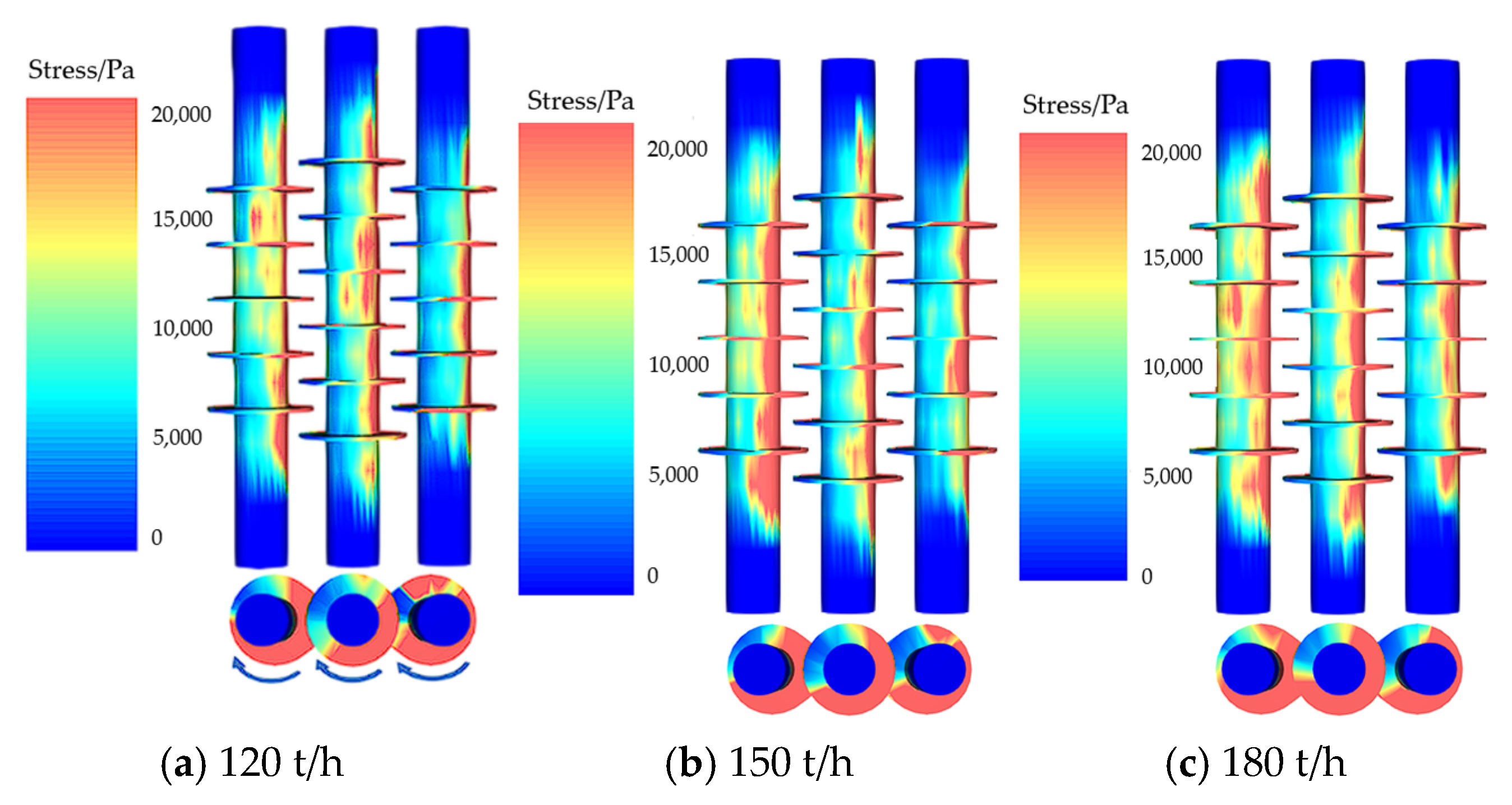
4.1.1. Stress Analysis of Iron Ore Pellets on Furnace Wall
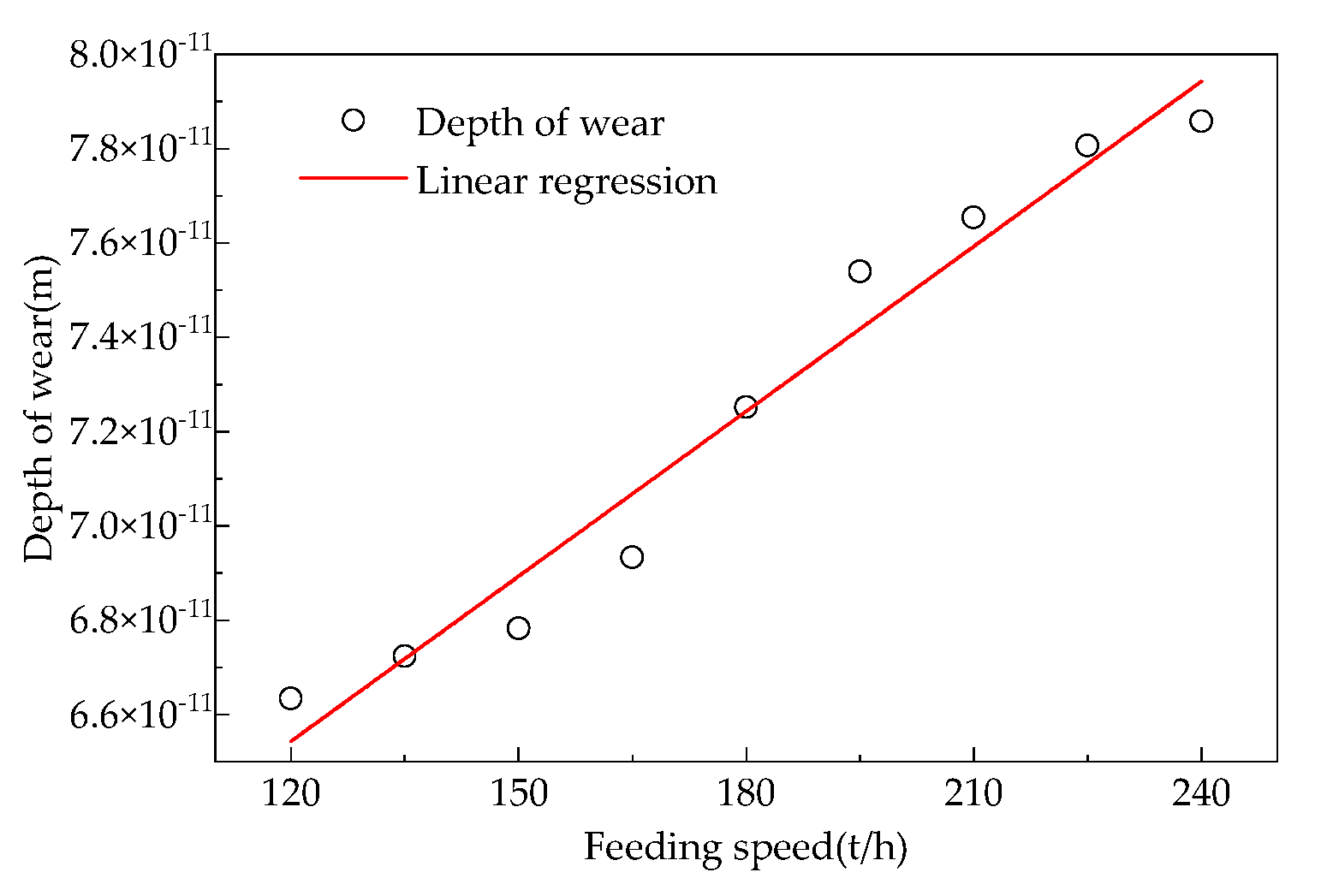
4.1.2. Wear Analysis of Furnace Wall
4.2. Stress Analysis of Iron Ore Pellets
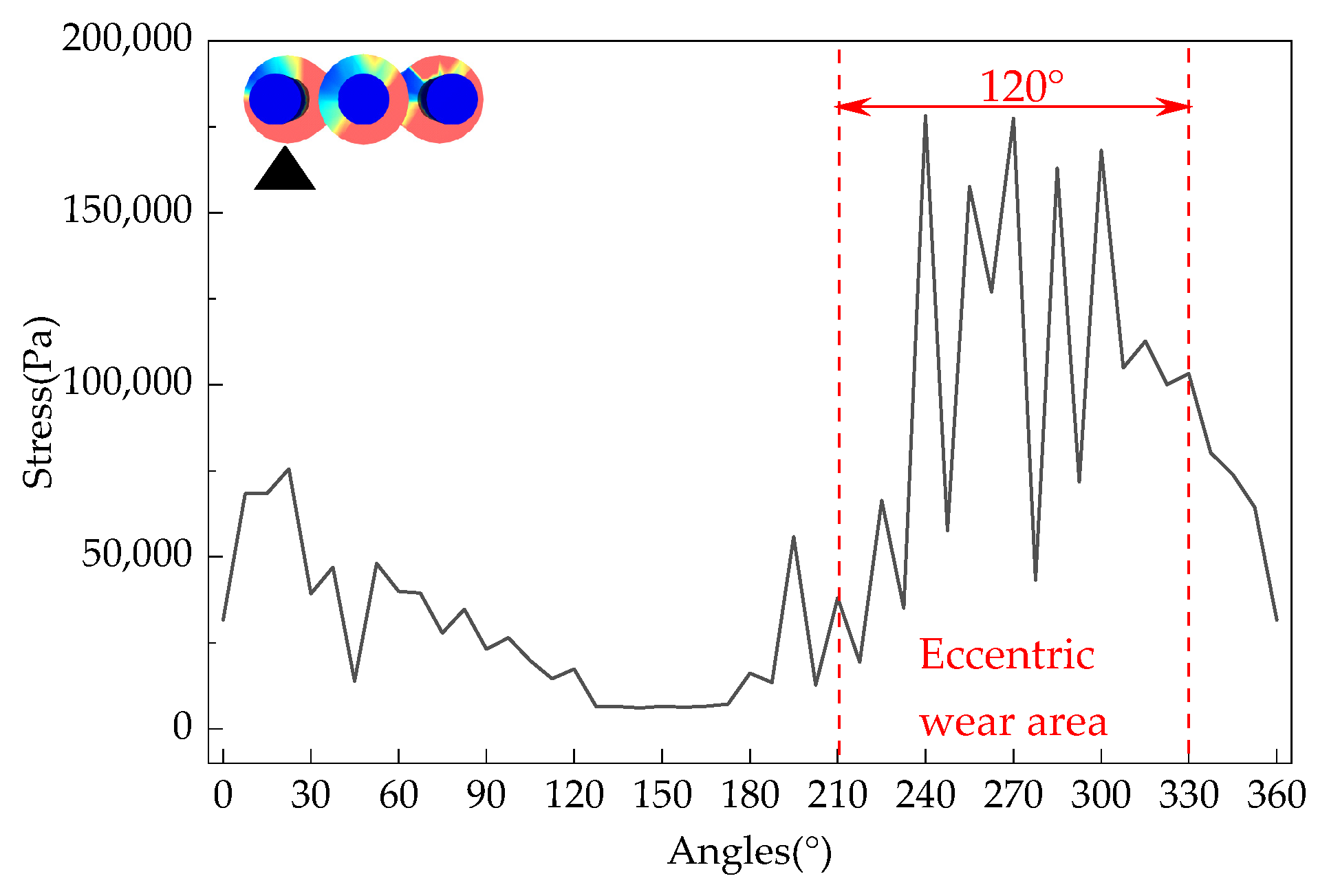
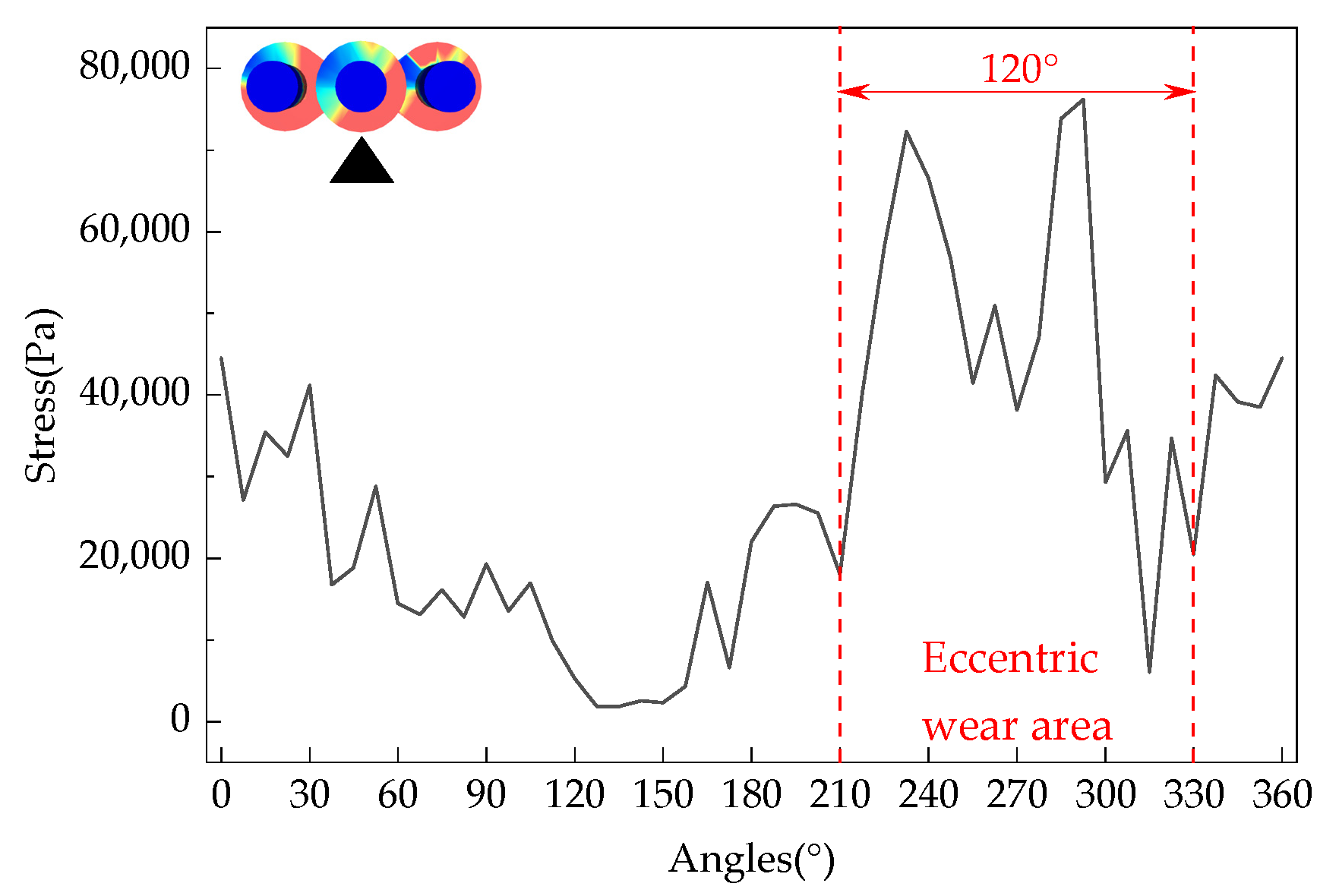
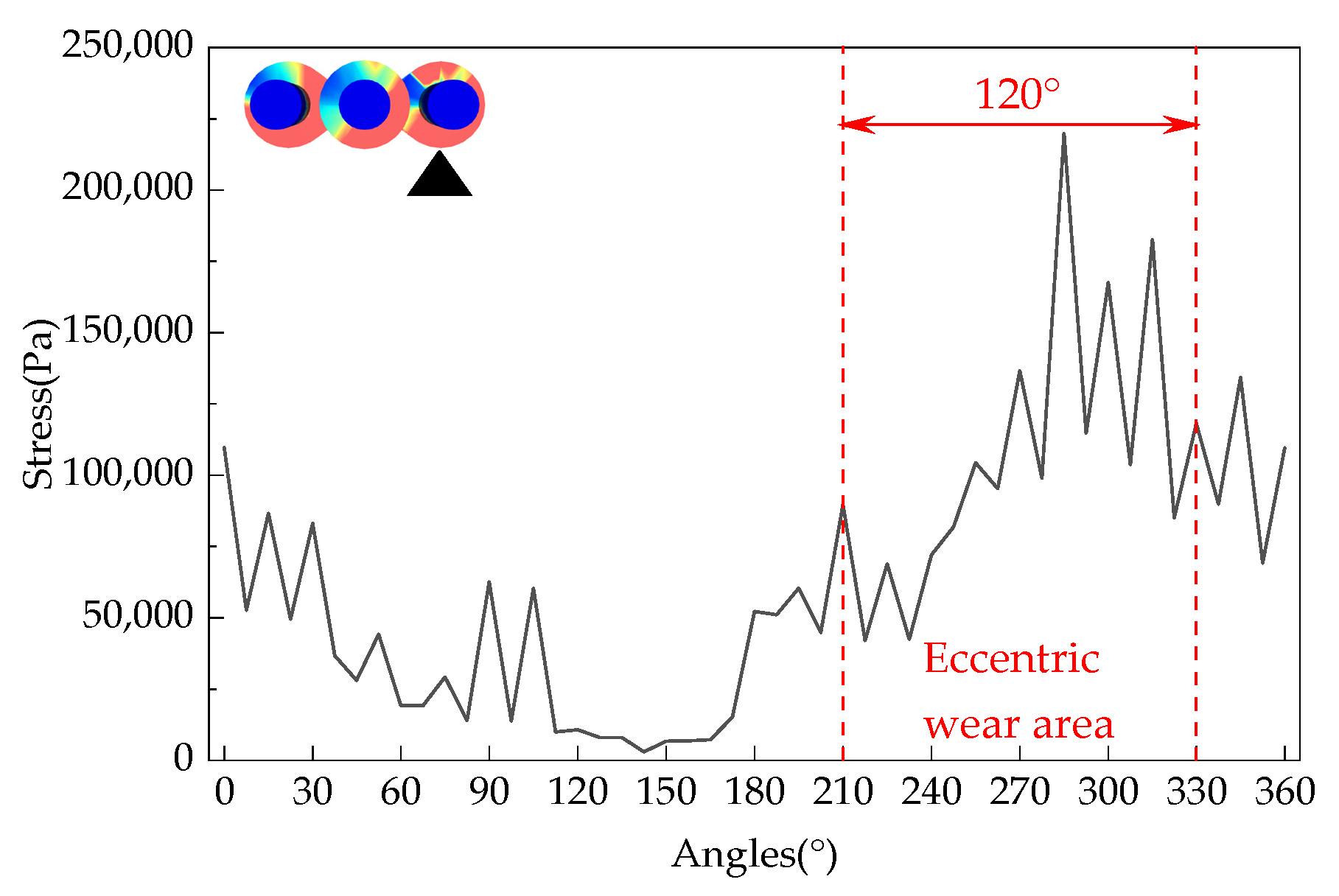
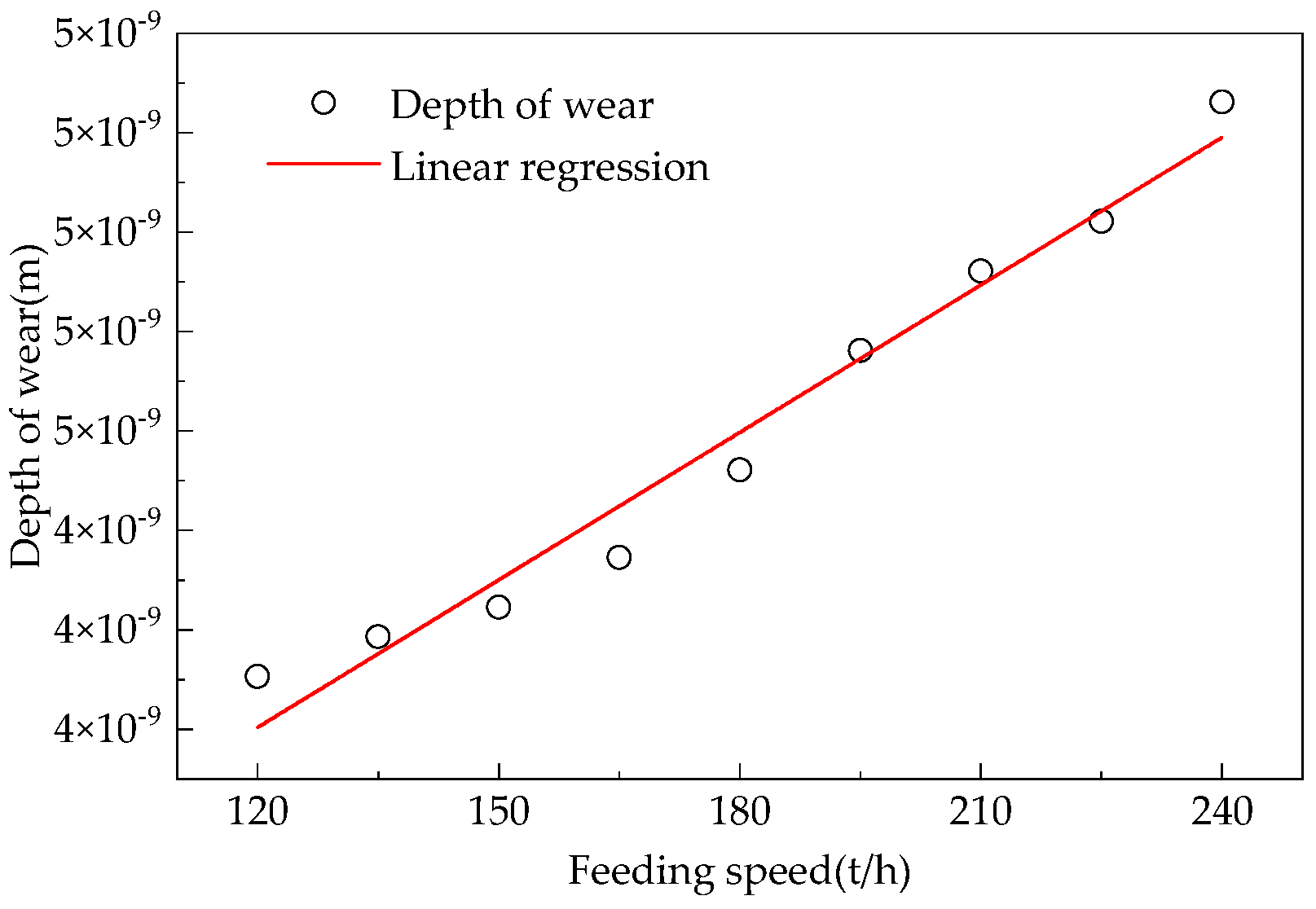
4.3. Analysis of Force and Wear on Loose Rollers
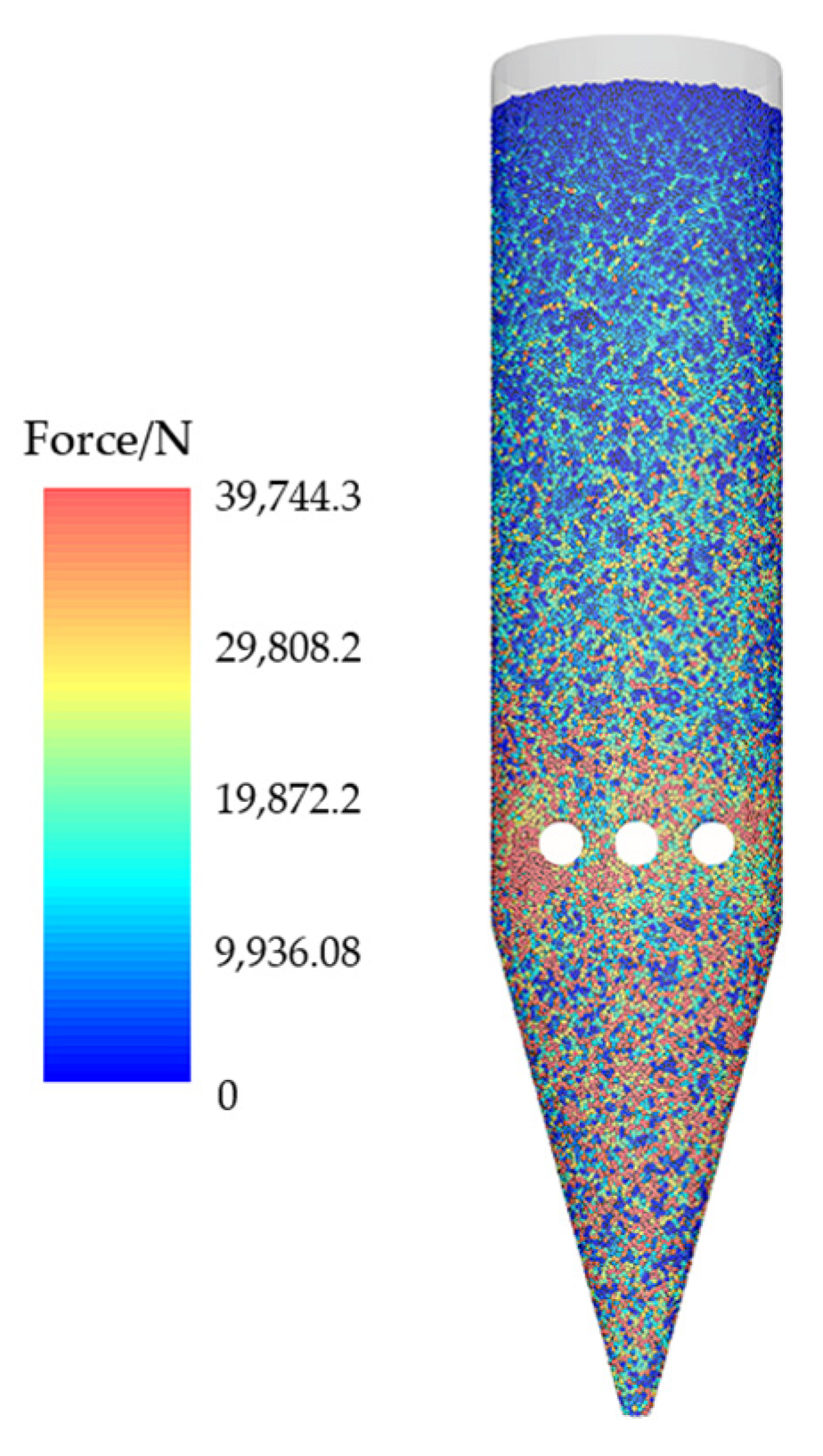
4.3.1. Force Analysis of Loose Rollers
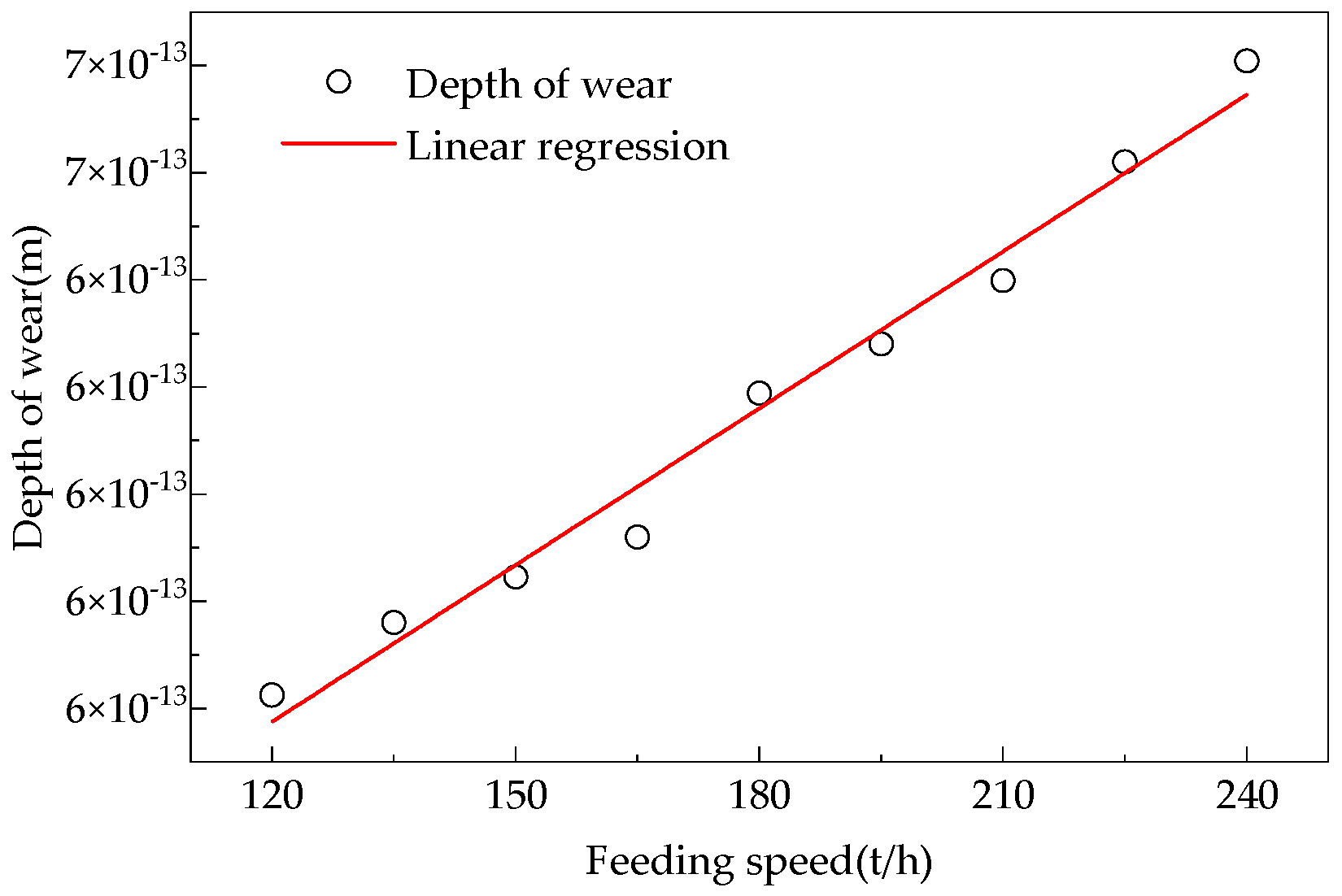
4.3.2. Wear Analysis of Loose Rollers
5. Conclusions
- (1)
- The maximum stress on the furnace wall in the output section of the hydrogen-based shaft furnace is 50,291.1 Pa when the feeding rate is 180 t/h. For every 10 t/h increase in feeding speed, the maximum stress on the furnace wall increases by 3052.9 Pa.
- (2)
- The wear coefficient of the refractory material in the reduction section is 4.88 × 10−16, and the wear coefficient of the steel shell in the cooling section is 1.17 × 10−13. The degree of wear on the cooling section is much greater than that on the reduction section.
- (3)
- Near the loosening roller, the maximum force on the DRI particles reached 39,744.3 N. At the core, 2.26 m below the material level in the reduction section, the maximum force on the pellet reaches 39,379.5 N.
- (4)
- According to the loose roller eccentric wear Formula (23) obtained in this article and the provided parameters, the degree of loose roller wear can be calculated. During the rotation of the loose roller, uneven force gradually causes irregular shape changes, resulting in more severe eccentric wear due to deformation.
- (5)
- There is a phenomenon of stress concentration in the inner shaft and fan blades of the loose roller, and the shear stress of the right loose roller reaches its maximum value of 219,896.1 Pa. The monthly wear of the loose roller is about 0.601 mm.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Q.; Shen, J.L.; Xu, L.S. Carbon peak and low-carbon transition path of China’s iron and steel industry. Iron Steel 2021, 56, 152–163. [Google Scholar]
- Shangguan, F.Q.; Liu, Z.D.; Yin, R.Y. Study on implementation path of “carbon peak” and “carbon neutrality” in steel industry in China. China Metall. 2021, 31, 15–20. [Google Scholar]
- He, L.L.; Zhang, H. China’s Energy Transformation Path Under “Double Carbon” Goal; Shanghai Energy Saving: Shanghai, China, 2023; pp. 1285–1294. [Google Scholar]
- Pan, Z.B. Development status and prospect of Chinese low-carbon ironmaking technology under carbon neutrality background. Sinter. Pelletizing 2024, 9, 5–41. [Google Scholar]
- Yu, Z.; Wang, Z.; Ma, L.; Ma, M. Energy-saving targets and carbon neutrality: A perspective on carbon emissions and carbon substitution in 288 Chinese cities. J. Environ. Manag. 2024, 356, 120523. [Google Scholar] [CrossRef]
- Lu, X.G.; Yu, W.; Zhu, K.; Li, G.; Ye, S. Development and key problems of hydrogen metallurgy. Chin. J. Nat. 2022, 44, 251–266. [Google Scholar]
- Jiang, Z.H.; Yang, C.; Zhu, H.C.; Lu, H. Research status and prospect of hydrogen metallurgy steelmaking technology. Iron Steel 2024, 59, 140–155. [Google Scholar]
- Zhang, J.L.; Zong, Y.B.; Li, K.J.; Li, Z.; Yang, T. Progress and future perspective of low-carbon ironmaking process in the world. Iron Steel 2024, 59, 45–55+155. [Google Scholar]
- Han, X.; Zhang, C.D.; Li, L.J.; Tian, Z.; Wang, X. Research status and prospects of hydrogen based shaft furnace direct reduction technology. Hebei Metall. 2024, 4, 1–5+11. [Google Scholar] [CrossRef]
- Shao, Y.J.; Xu, L.; Liu, X.P.; Cheng, H. Discussion on solution of “carbon neutrality” in China’s steel production. China Metall. 2022, 32, 1–8. [Google Scholar]
- Wang, X.D.; Hou, C.J.; Zhong, J.H. The development status of hydrogen industry and its application practice in China’s iron and steel industry. Hebei Metall. 2024, 32, 1–8. [Google Scholar]
- Chai, X.C.; Yue, Q.; Zhang, Y.J.; Wang, Q. Numerical simulation analysis of reduction domain in hydrogen metallurgy shaft furnace. Iron Steel 2022, 57, 138–147. [Google Scholar]
- Du, Y.M.; Guo, H.; You, G.; Mao, S.; Cao, W. Research progress of green and low-carbon hydrogen metallurgy technology. Min. Eng. 2024, 22, 62–65+69. [Google Scholar]
- Lu, L.J.; Wang, H.F.; Wang, F.; Qiu, J.; Ping, X. Development status and application of hydrogen metallurgy technology. Iron Steel 2024, 59, 183–196. [Google Scholar]
- Zhang, R.; Zhao, X.; Liu, Y.; Zhang, Q. Development pathway and influencing factors of hydrogen energy storage accommodating renewable energy growth. J. Energy Storage 2024, 87, 111441. [Google Scholar] [CrossRef]
- Bai, C.C.; Shi, X.F.; Wang, M.Y.; Yu, H.; Hu, C. Numerical simulation analysis of direct reduction of gas-based shaft furnace. Iron Steel 2024, 59, 41–48. [Google Scholar]
- Sun, F.R. Hydrogen based shaft furnaces assist traditional metallurgical industries in carbon reduction and upgrading. Shanxi Metall. 2023, 46, 89–91. [Google Scholar]
- Liu, J.H. Optimization of pellet production process for hydrogen-based shaft furnaces. Hebei Metall. 2024, 44–48. [Google Scholar] [CrossRef]
- Lu, P. Hydrogen-based shaft furnace production practice for increasing output and reducing consumption. Hebei Metall. 2024, 47–51. [Google Scholar] [CrossRef]
- Yu, Z.J. Process Features of ZXHT Hydrogen-based Shaft Furnace. Ironmaking 2024, 43, 65–68. [Google Scholar]
- Chen, T.R.; Wangz, M.; Qin, H.B.; Liang, K.; Li, X. Research progress on hydrogen metallurgy process and refractories for hydrogen-based shaft furnaces. Refractories 2024, 58, 263–269. [Google Scholar]
- Zhang, H.; Liu, M.L.; Huang, Z.Y.; Bo, H. DEM simulation and analysis of collision between single coarse particle and wall. At. Energy Sci. Technol. 2017, 51, 2212–2217. [Google Scholar]
- Xu, Q.; Ye, J.H. Structural contact-collision behavior analysis based on bar system discrete element method. J. Vib. Shock 2023, 42, 103–110. [Google Scholar]
- Huang, L.J.; Liu, X.D.; Su, S.Q. Numerical simulation on breaking behavior of spheroidal particles impact based on discrete element method. China Powder Sci. Technol. 2014, 20, 60–63. [Google Scholar]
- Kuang, D.-M.; Long, Z.-L.; Zhao, T.; Luo, B.; Ogwu, I.; Kuang, F.-L. Modeling the single particle crushing behavior by random discrete element method. Constr. Build. Mater. 2024, 411, 134519. [Google Scholar] [CrossRef]
- You, Y.; Zhou, H.; Zhang, T.; Luo, Z.; Zhou, Z. Modeling of solid flow in COREX shaft furnace with AGD introduced by discrete element method. J. Mater. Metall. 2016, 15, 6–11. [Google Scholar]
- Zhang, Q.X. Analysis of Potato Collision Problem and Research and Development of Key Components of Separator Based on Discrete Element. Master’s Thesis, Shandong University of Technology, Zibo, China, 2022. [Google Scholar]
- Ma, K.; Deng, J.; Wang, G.; Zhou, Q.; Xu, J. Utilization and impacts of hydrogen in the ironmaking processes: A review from lab-scale basics to industrial practices. Int. J. Hydrogen Energy 2021, 46, 26646. [Google Scholar] [CrossRef]
- Zhou, H.; Luo, Z.; Zou, Z.; Zhang, T.; You, Y. Experimental study on burden descending behavior in COREX shaft furnace with AGD beams. Steel Res. Int. 2015, 86, 1073–1081. [Google Scholar] [CrossRef]
- Zhang, B.L. DEM Modeling of the Flow Characteristics of Granular Materials in Moving Beds. Master’s Thesis, China University of Petroleum, Beijing, China, 2023. [Google Scholar]
- Shen, Q.J.; Dong, S.J.; Zhang, D.C.; Guo, H.; Song, Y. DEM modeling of resonant motion of particles inside moving bed. Chin. J. Process Eng. 2023, 23, 826–836. [Google Scholar]
- Liu, J.D. DEM Modeling of the Discharge Features of Moving Bed. Master’s Thesis, University of Science and Technology Liaoning, Anshan, China, 2022. [Google Scholar]
- Li, H.F.; Zhou, H.; Luo, Z.G.; Zhang, T.; You, Y.; Zou, Z.S. Three-dimensional discrete element method simulation of the effect of bottom structure on solid flow in COREX shaft furnace. Can. Metall. Q. 2016, 55, 112–123. [Google Scholar] [CrossRef]
- Du, B.B.; Wu, S.L.; Zhou, H.; Kou, M. Effect of scaffolding on solid flow in COREX shaft furnace by discrete element simulation method. Iron Steel 2020, 55, 12–19. [Google Scholar]
- Ma, S.H.; Ji, Z.J.; Cai, X.C.; Ji, M. Research on the bearing wear characteristics of wind turbine based on finite element analysis and Archard model. J. Qinghai Univ. 2024, 42, 70–77+106. [Google Scholar]
- Gao, J.F.; Zhao, J.Y.; Feng, Y.L.; Yang, L.; Li, W. Research of wear behavior of discontinuous contact floating ball seal structure based on modified Archard wear model. Fluid Mach. 2024, 52, 20–27. [Google Scholar] [CrossRef]
- Zhou, S.X.; Lei, Z.Y.; Qin, Z.; Niu, L.; Bai, X. Optimization of Disc Braking Correction Indexes Based on Archard Wear Theory. China Railw. Sci. 2022, 43, 129–138. [Google Scholar]
- Yang, X.J.; Zhu, X.L. Study on wear of warm upsetting mold for non-standard pin shaft based on revised Archard wear theory. Forg. Stamp. Technol. 2021, 46, 32–37. [Google Scholar]
- Fan, W.; Shui, P.; Xie, C.D.; Gao, L. Research and Optimization of Wear Evolution Law of High Pressure Roller Mill Based on Archard Model and DEM Simulation. Metal Mine 2024, 173–180. [Google Scholar]
- Xie, W.X.; Su, Q.X.; Guo, L.Q.; Sun, Z.; Zhang, L.; Gu, W.; Zhang, X. Analysis on wear law of friction pair of oil-well pump barrel-plunger based on, ANSYS. China Pet. Mach. 2022, 50, 115–121. [Google Scholar]
- Wei, D.; Wei, P.T.; Liu, H.J. Wear behavior and simulation analysis of micro gear tooth surface. J. Chongqing Univ. 2022, 45, 22–30. [Google Scholar]








| Part | Parameters | Value |
|---|---|---|
| Shaft furnace | Upper diameter/m | 5.0 |
| Lower diameter/m | 0.5 | |
| Height of reduction/m | 11.6 | |
| Height of transition/m | 3.0 | |
| Height of cooling/m | 4.2 | |
| Height of output/m | 4.2 | |
| Loosening roller | Inner shaft diameter/m | 0.7 |
| Fan diameter/m | 1.4 |
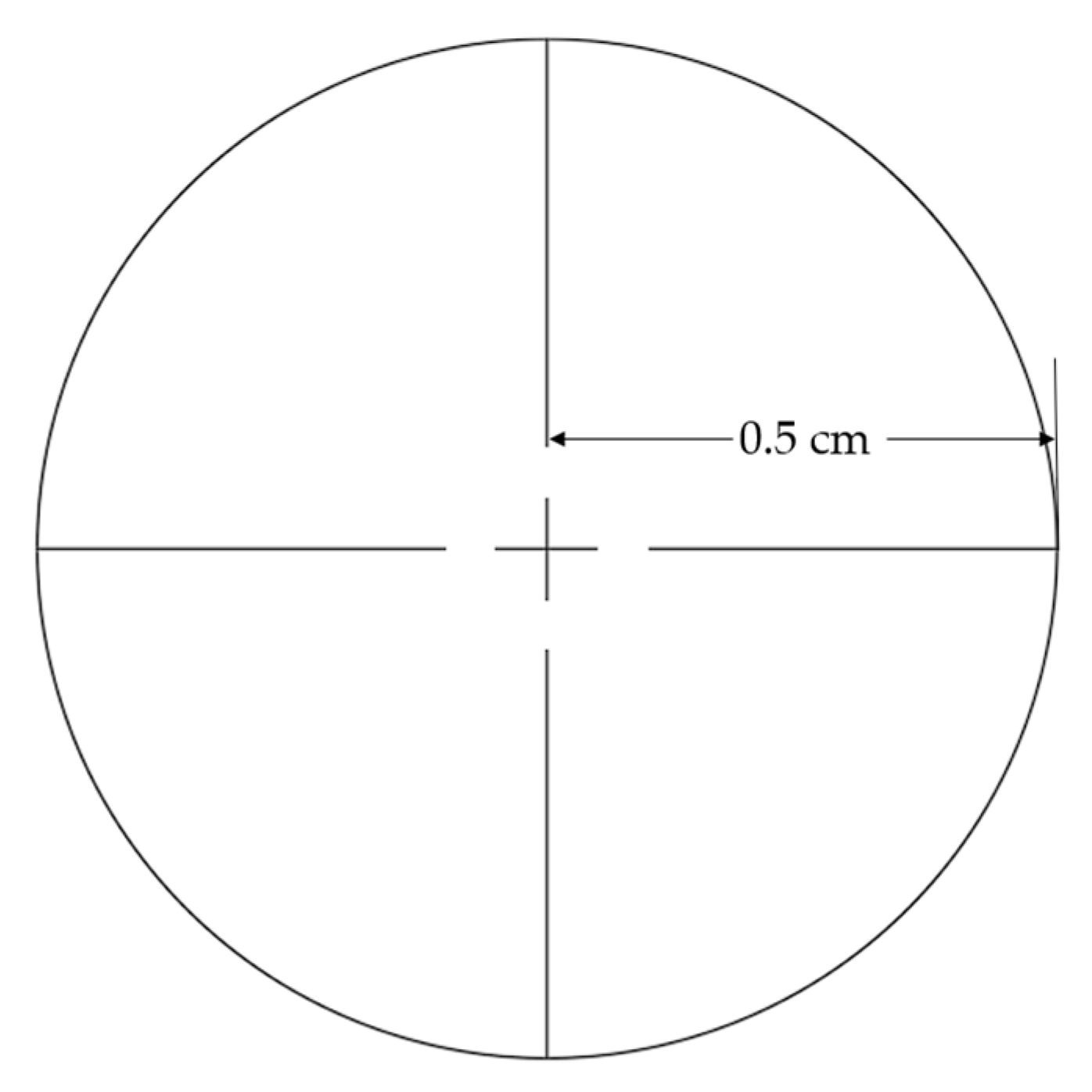
| Iron ore/DRI Particles | Information |
|---|---|
| Shape | Sphere |
| Diameter | 1 cm |
| Density | 2200 kg/m3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ling, H.; Jin, Y.; Huang, Z.; Liu, Z.; Lin, P. Study of Stress and Wear Behavior of Internal Components in Hydrogen-Based Shaft Furnaces Based on the Discrete Element Method (DEM) Model. Processes 2025, 13, 857. https://doi.org/10.3390/pr13030857
Ling H, Jin Y, Huang Z, Liu Z, Lin P. Study of Stress and Wear Behavior of Internal Components in Hydrogen-Based Shaft Furnaces Based on the Discrete Element Method (DEM) Model. Processes. 2025; 13(3):857. https://doi.org/10.3390/pr13030857
Chicago/Turabian StyleLing, Hongzhi, Yan Jin, Zhengchao Huang, Ziyu Liu, and Peng Lin. 2025. "Study of Stress and Wear Behavior of Internal Components in Hydrogen-Based Shaft Furnaces Based on the Discrete Element Method (DEM) Model" Processes 13, no. 3: 857. https://doi.org/10.3390/pr13030857
APA StyleLing, H., Jin, Y., Huang, Z., Liu, Z., & Lin, P. (2025). Study of Stress and Wear Behavior of Internal Components in Hydrogen-Based Shaft Furnaces Based on the Discrete Element Method (DEM) Model. Processes, 13(3), 857. https://doi.org/10.3390/pr13030857






