Study on the Dynamic Modeling of Two-Phase Flow and Lubrication Characteristics of Toothless Stirring Oil Pans
Abstract
1. Introduction
2. High-Speed Gear Lubrication Oil Field Mathematical Analysis Model
2.1. Flow-Field Control Equations and VOF Model
2.2. Turbulence Model
2.3. Dynamic Mesh Technology
2.4. Gearbox Dynamics Solution Strategy
3. Numerical Model of the Gear Lubricating Oil Field
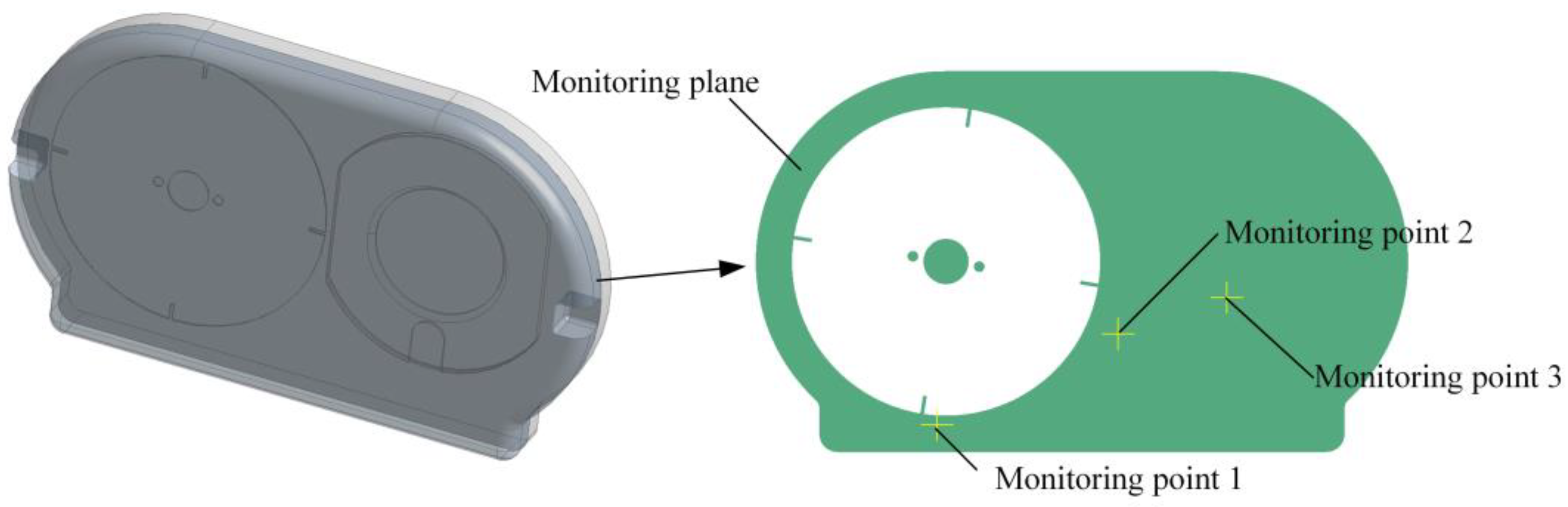
3.1. Geometric Model and Numerical Model
3.2. Initial Conditions and Boundary Conditions
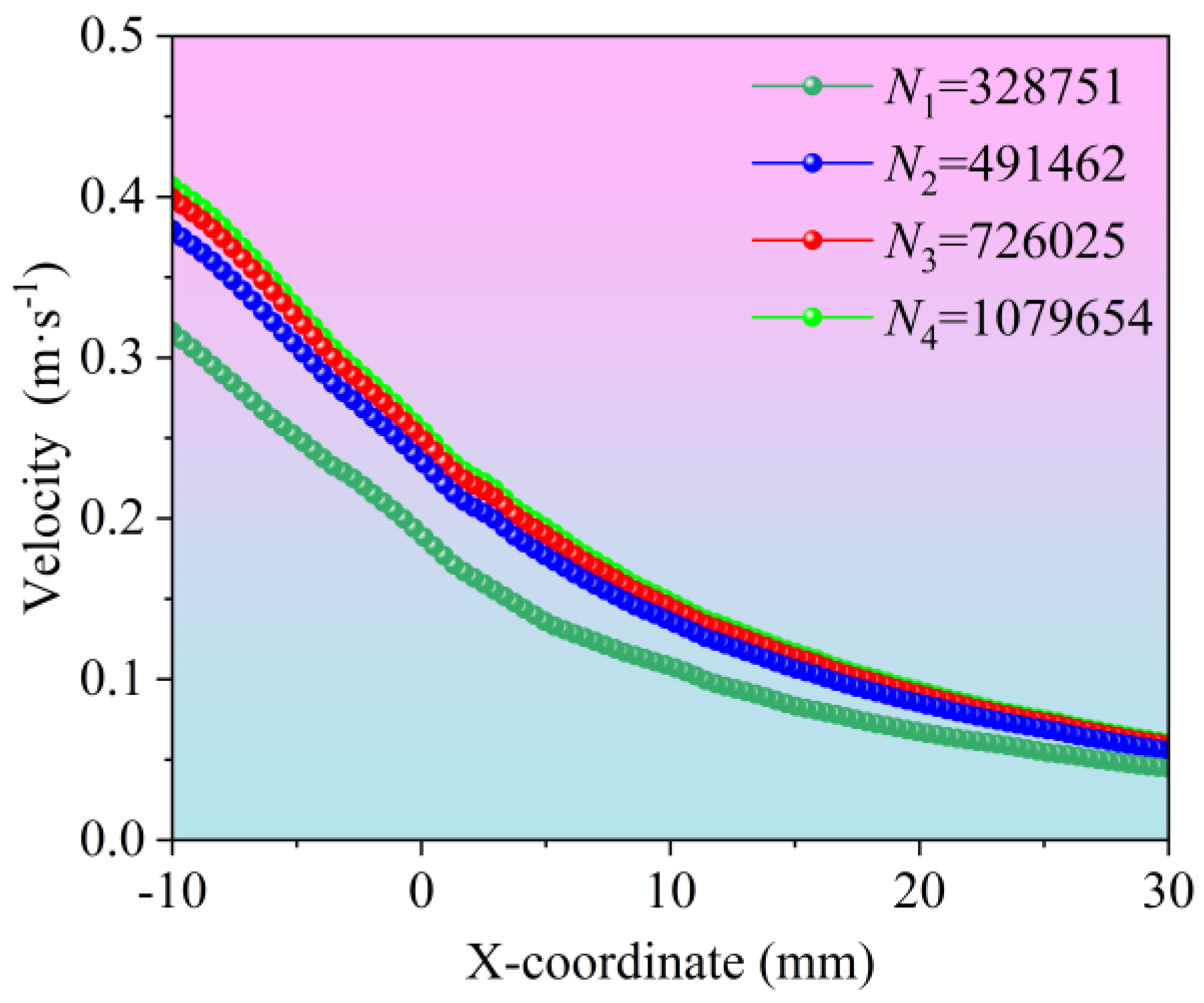
3.3. Grid Independence Verification
4. Numerical Simulation of Gear Lubricating Oil Field
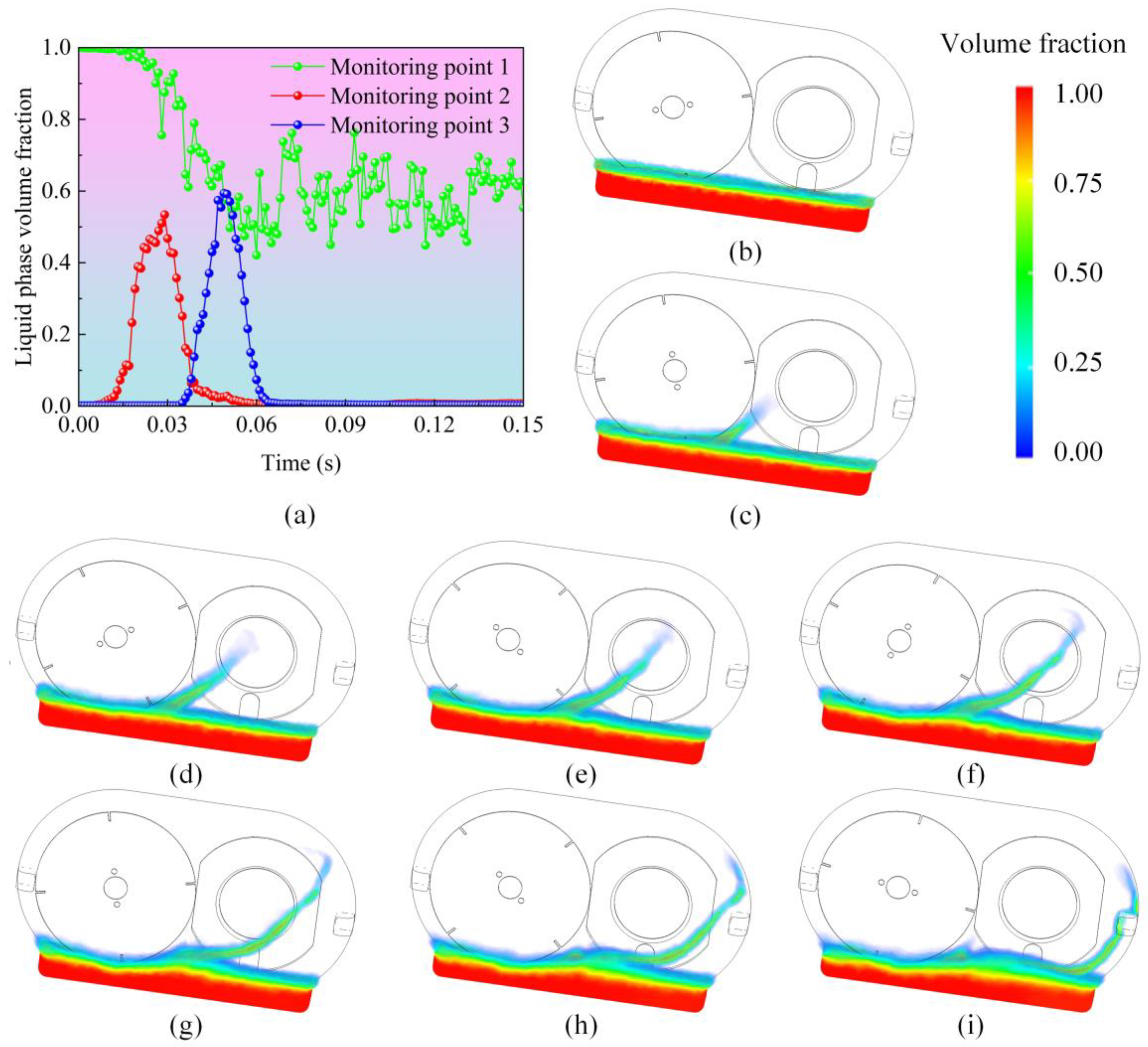
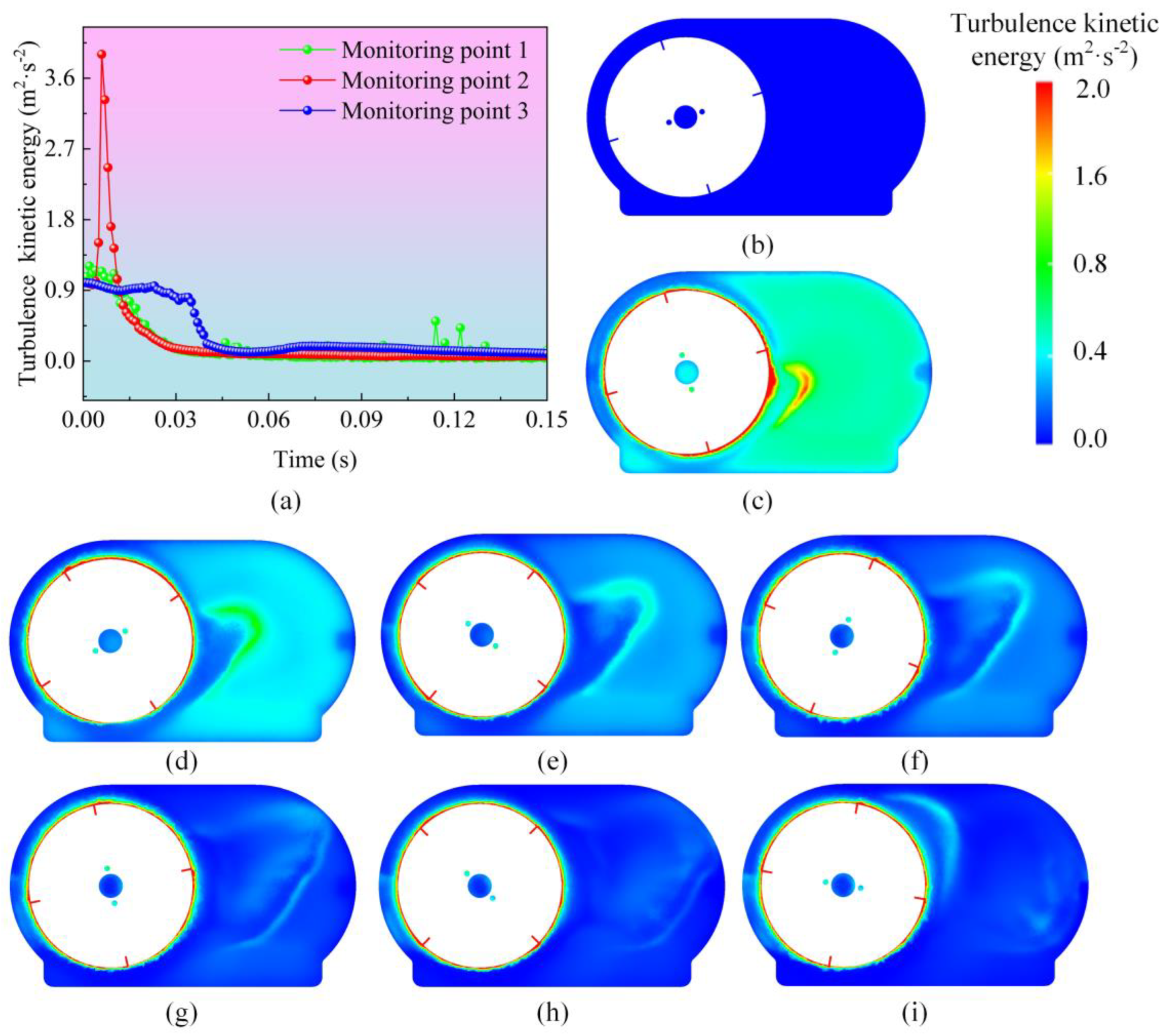
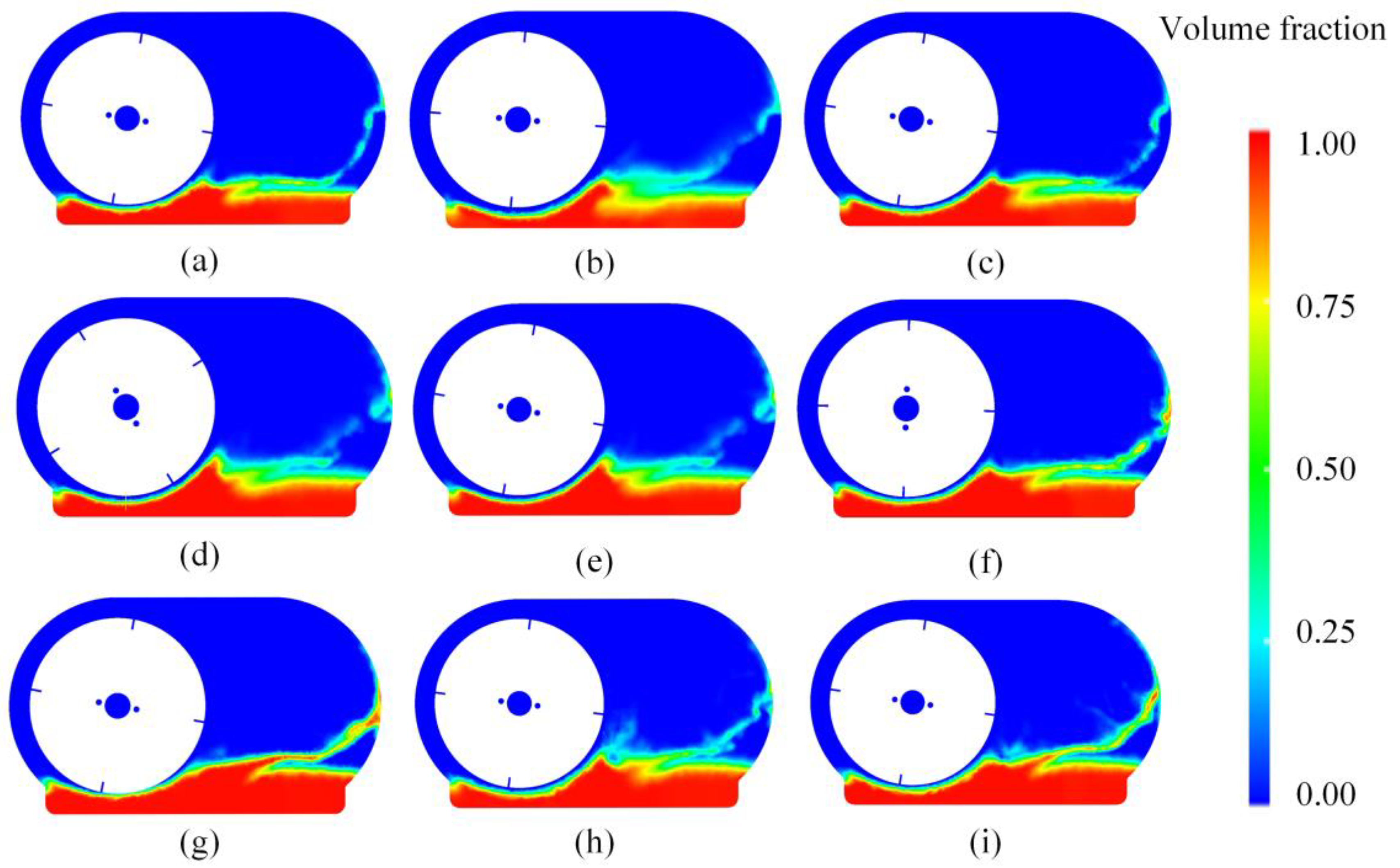
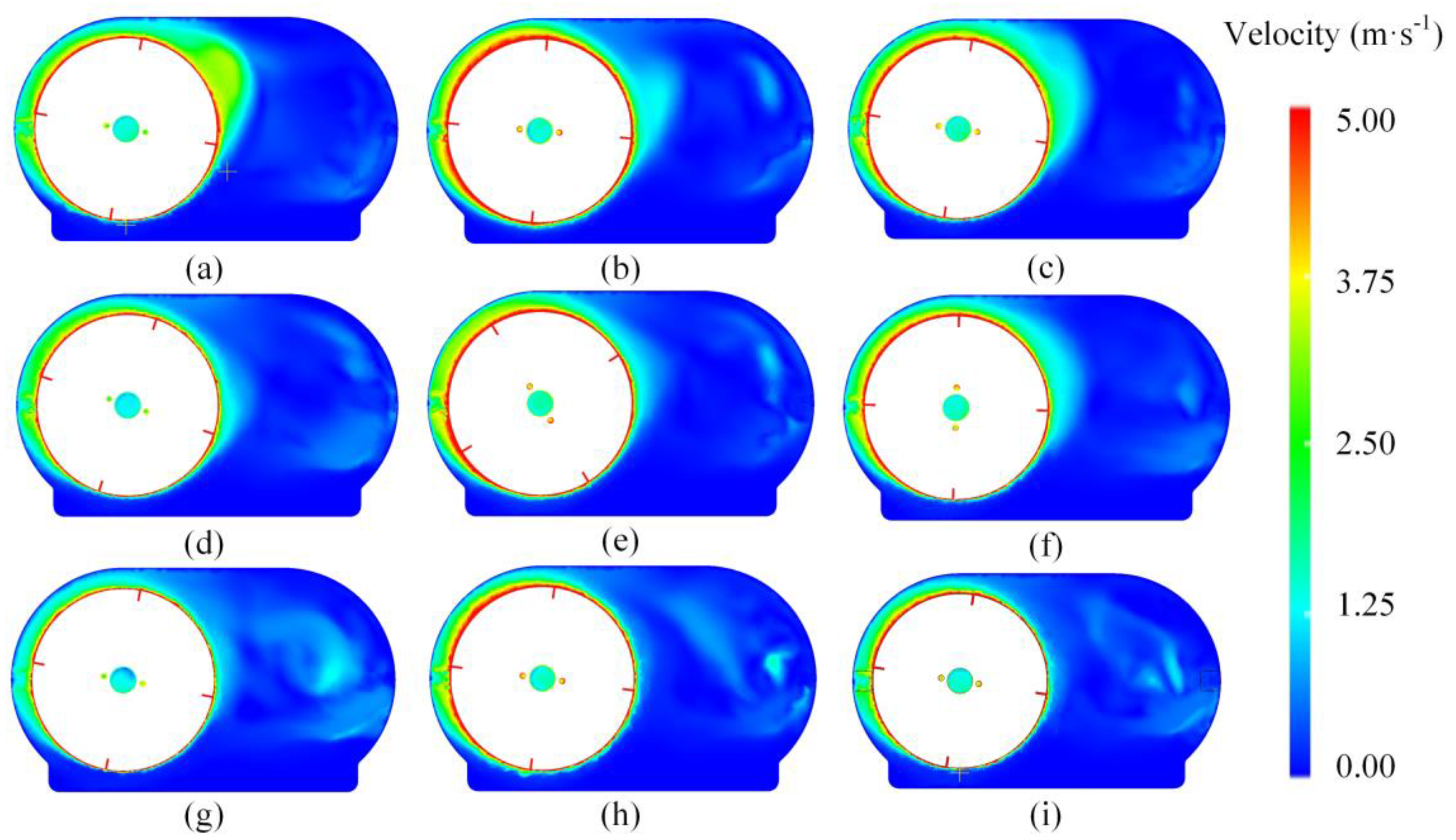
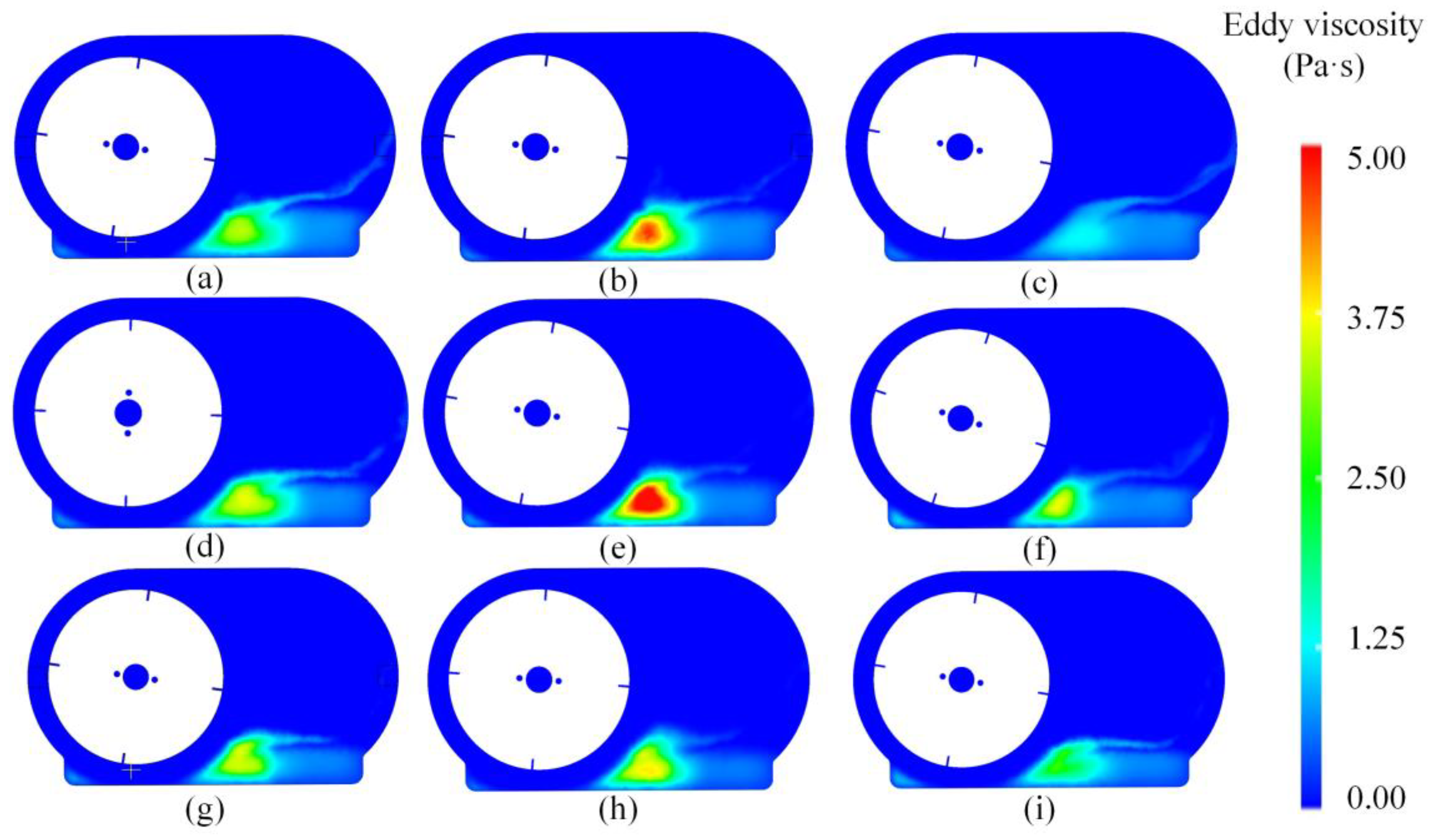
4.1. Lubricating Oil Distribution in High-Speed Gearbox
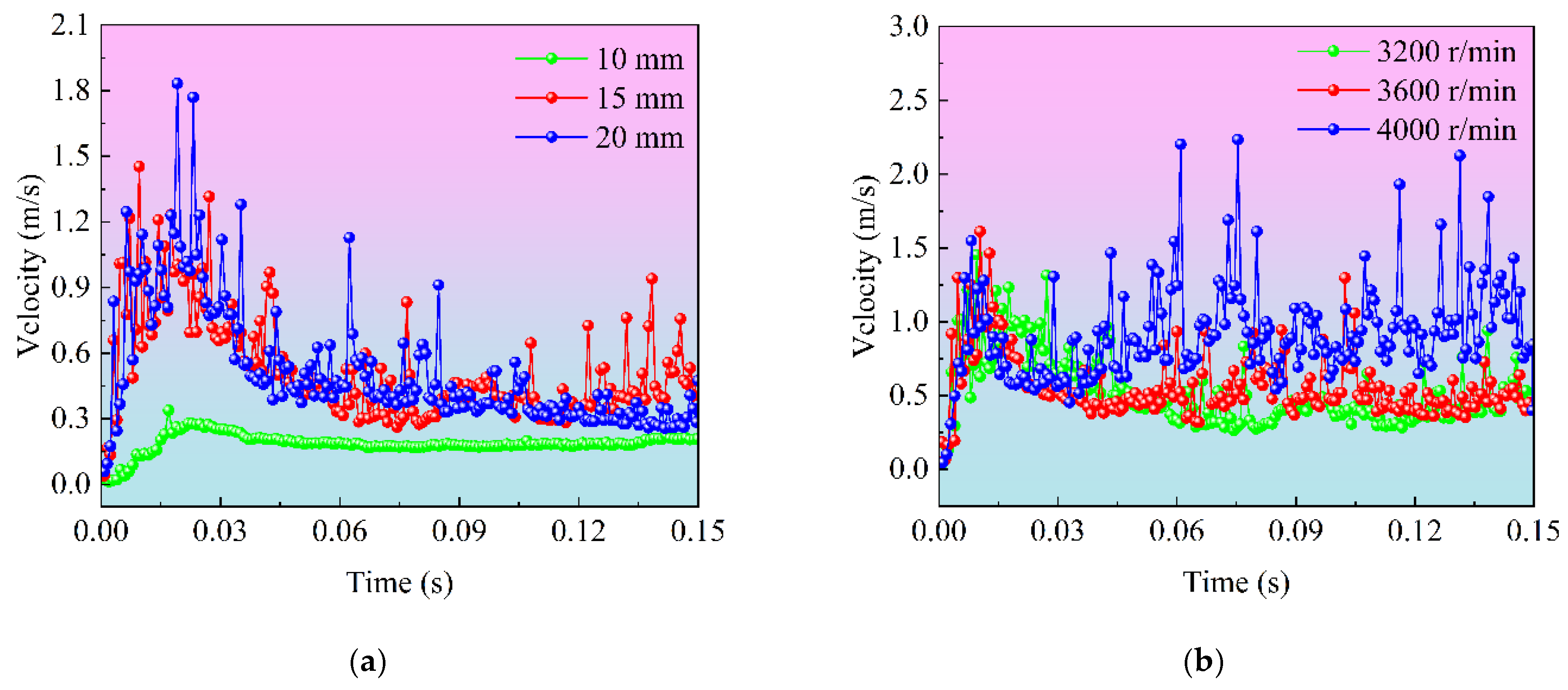
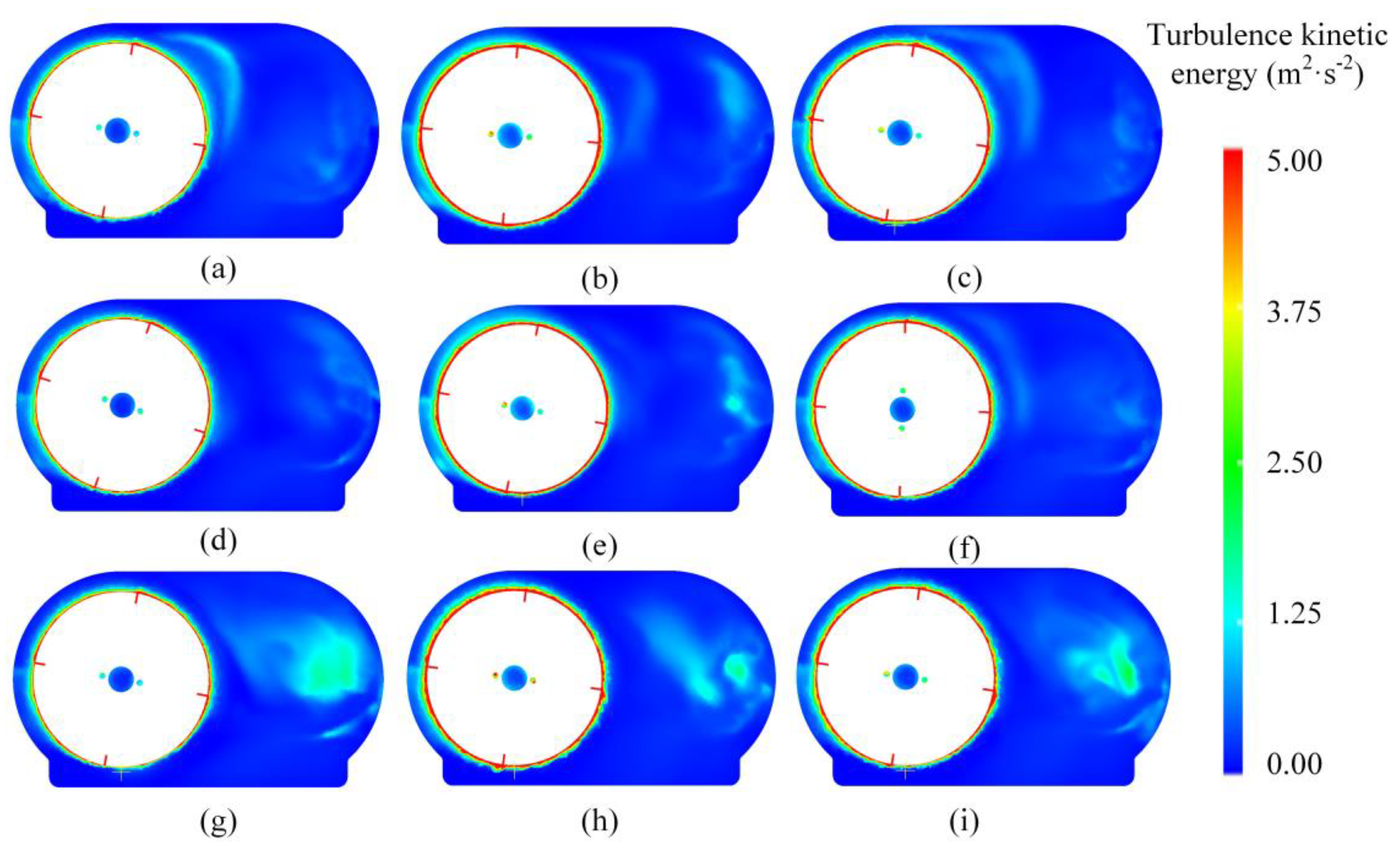
4.2. Different Oil Distribution at Various Rotational Speeds
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Hu, S.; Gong, W.; Gui, P. Numerical study on the churning power loss of spiral bevel gears at splash lubrication system. Lubr. Sci. 2024, 36, 259–276. [Google Scholar] [CrossRef]
- Jia, F.; Wang, B.; Fu, Y. A Novel Prediction Model for Churning Power Loss of Spur Gear. Lubr. Sci. 2024, 36, 645–655. [Google Scholar] [CrossRef]
- Li, Q.H.; Xu, P.; Li, L.; Xu, W.X.; Tan, D.P. Investigation on the Lubrication Heat Transfer Mechanism of the Multilevel Gearbox by the Lattice Boltzmann Method. Processes 2024, 12, 381. [Google Scholar] [CrossRef]
- Zheng, G.A.; Xu, P.; Li, L. Investigate on the Fluid Dynamics and Heat Transfer Behavior in an Automobile Gearbox based on the LBM-LES Model. Lubricants 2025, in press. [Google Scholar]
- Ji, R.Q.; Shen, Q.T.; Zhang, L.; Zeng, X.; Qi, H. Novel photocatalysis-assisted mechanical polishing of laser cladding cobalt-based alloy using TiO₂ nanoparticles. Powder Technol. 2024, 444, 119990. [Google Scholar] [CrossRef]
- Shore, J.F.; Kolekar, A.S.; Ren, N.; Kadiric, A. An Investigation Into the Influence of Viscosity on Gear Churning Losses by Considering the Effective Immersion Depth. Tribol. Trans. 2023, 66, 906–919. [Google Scholar] [CrossRef]
- Lin, H.; Shen, Q.T.; Ma, M.; Ji, R.Q.; Guo, H.J.; Qi, H.; Xing, W.; Tang, H.P. 3D Printing of Porous Ceramics for Enhanced Thermal Insulation Properties. Adv. Sci. 2024, 2412554. [Google Scholar] [CrossRef]
- Li, L.; Xu, W.X.; Tan, Y.F.; Yang, Y.S.; Yang, J.G.; Tan, D.P. Fluid-induced vibration evolution mechanism of multiphase free sink vortex and the multi-source vibration sensing method. Mech. Syst. Signal Process. 2023, 189, 110058. [Google Scholar] [CrossRef]
- Yang, X.; Zhang, Z.Y.; Zhang, T.C.; Song, F.; Yao, X.L.; Xiao, B.; Lin, P.; Qi, H.; Liu, S.F.; Tang, H.P. Multi-build orientation effects on microstructural evolution and mechanical behavior of truly as-built selective laser melting Ti6Al4V alloys. J. Mater. Res. Technol. 2024, 30, 3967–3976. [Google Scholar] [CrossRef]
- Shao, S.; Zhang, K.; Yao, Y.; Liu, Y.; Yang, J.; Xin, Z.; He, K. A Study on the Lubrication Characteristics and Parameter Influence of a High-Speed Train Herringbone Gearbox. Lubricants 2024, 12, 270. [Google Scholar] [CrossRef]
- Menon, M.; Schifko, M.; Peng, C.; Chitneedi, B.K.; Borra, R. Obtaining Precise Churning Loss for a Gearbox Using Advanced Smoothed Particle Hydrodynamics. SAE Tech. Pap. Ser 2019, 1, 0348. [Google Scholar]
- Lu, F.; Wang, M.; Bao, H.; Huang, W.; Zhu, R. Churning power loss of the intermediate gearbox in a helicopter under splash lubrication. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2022, 236, 49–58. [Google Scholar] [CrossRef]
- Su, J.; Li, S.; Hu, B.; Yin, L.; Zhou, C.; Wang, H.; Hou, S. Innovative insights into nanofluid-enhanced gear lubrication: Computational and experimental analysis of churn mechanisms. Tribol. Int. 2024, 199, 109949. [Google Scholar] [CrossRef]
- Shen, L.; Zhu, Y.; Shao, S.; Zhou, H.; Wang, Z. Research on Splash Lubrication Characteristics of a Spiral Bevel Gearbox Based on the MPS Method. Lubricants 2023, 11, 520. [Google Scholar] [CrossRef]
- Quiban, R.; Changenet, C.; Marchesse, Y.; Ville, F. Experimental investigations about the power loss transition between churning and windage for spur gears. J. Tribol. 2021, 143, 024501. [Google Scholar] [CrossRef]
- Hildebrand, L.; Genuin, S.; Lohner, T.; Stahl, K. Numerical analysis of the heat transfer of gears under oil dip lubrication. Tribol. Int. 2024, 195, 109652. [Google Scholar] [CrossRef]
- Boni, J.B.; Changenet, C.; Ville, F. Analysis of flow regimes and associated sources of dissipation in splash lubricated planetary gear sets. J. Tribol. 2021, 143, 111805. [Google Scholar] [CrossRef]
- Hu, X.; Jiang, Y.; Luo, C.; Feng, L.; Dai, Y. Churning power losses of a gearbox with spiral bevel geared transmission. Tribol. Int. 2019, 129, 398–406. [Google Scholar] [CrossRef]
- Mastrone, M.N.; Hartono, E.A.; Chernoray, V.; Concli, F. Oil distribution and churning losses of gearboxes: Experimental and numerical analysis. Tribol. Int. 2020, 151, 106496. [Google Scholar] [CrossRef]
- Laruelle, S.; Fossier, C.; Changenet, C.; Ville, F.; Koechlin, S. Experimental investigations and analysis on churning losses of splash lubricated spiral bevel gears. Mech. Ind. 2017, 18, 412. [Google Scholar] [CrossRef]
- Lu, F.; Wang, M.; Liu, W.; Bao, H.; Zhu, R. CFD-based calculation method of convective heat transfer coefficient of spiral bevel gear in intermediate gearbox under splash lubrication. Ind. Lubr. Tribol. 2021, 73, 470–476. [Google Scholar] [CrossRef]
- Liu, H.; Jurkschat, T.; Lohner, T.; Stahl, K. Detailed investigations on the oil flow in dip-lubricated gearboxes by the finite volume CFD method. Lubricants 2018, 6, 47. [Google Scholar] [CrossRef]
- Keller, M.C.; Kromer, C.; Cordes, L.; Schwitzke, C.; Bauer, H.J. CFD study of oil-jet gear interaction flow phenomena in spur gears. Aeronaut. J. 2020, 124, 1301–1317. [Google Scholar] [CrossRef]
- Mastrone, M.N.; Concli, F. CFD simulation of grease lubrication: Analysis of the power losses and lubricant flows inside a back-to-back test rig gearbox. J. Non-Newton. Fluid Mech. 2021, 297, 104652. [Google Scholar] [CrossRef]
- Dai, Y.; Jia, J.; Ouyang, B.; Bian, J. Determination of an Optimal Oil Jet Nozzle Layout for Helical Gear Lubrication: Mathematical Modeling, Numerical Simulation, and Experimental Validation. Complexity 2020, 2020, 1–18. [Google Scholar] [CrossRef]
- Singh, B.; Choudhary, V.P.; Kumar, A.; Chopra, C. CFD Simulation of Transmission for Lubrication Oil Flow Validation and Churning Loss Reduction. SAE Tech. Pap. Ser 2020, 1, 1089. [Google Scholar]
- Hosain, L.; Fdhila, R.B. Literature Review of Accelerated CFD Simulation Methods towards Online Application. Energy Procedia 2015, 75, 3307–3314. [Google Scholar] [CrossRef]
- Guo, X.M.; Yang, M.Y.; Li, F.Q.; Zhu, Z.C.; Cui, B.L. Investigation on Cryogenic Cavitation Characteristics of an Inducer Considering Thermodynamic Effects. Energies 2024, 17, 3627. [Google Scholar] [CrossRef]
- Ge, J.Q.; Lin, Y.H.; Qi, H.; Li, Y.T.; Li, X.L.; Li, C.; Li, Z.A.; Xu, K.Q. The impact of ultrasonic-induced jet morphology on polishing efficiency. Int. J. Mech. Sci. 2024, 284, 109764. [Google Scholar] [CrossRef]
- Li, Z.; Wang, C.Y.; Li, L.; Wu, J.F.; Yin, Z.C.; Tan, D.P. Numerical investigation of mesoscale multiphase mass transport mechanism in fibrous porous media. Eng. Appl. Comput. Fluid Mech. 2024, 18, 2363246. [Google Scholar] [CrossRef]
- Li, L.; Xu, P.; Xu, W.X.; Lu, B.; Wang, C.Y.; Tan, D.P. Multi-field coupling vibration patterns of the multiphase sink vortex and distortion recognition method. Mech. Syst. Signal Process. 2024, 219, 111624. [Google Scholar] [CrossRef]
- Rusche, H. Computational Fluid Dynamics of Dispersed Two-Phase Flows at High Phase Fractions. Ph.D. Thesis, Imperial College of Science, Technology and Medicine, University of London, London, UK, 2002. [Google Scholar]
- Lin, Q.; Li, Q.H.; Xu, P.; Zheng, R.Y.; Bao, J.J.; Li, L.; Tan, D.P. Transport mechanism and optimization design of the LBM-LES coupling-based two-phase flow in static mixers. J. Mar. Sci. Eng. 2025, in press. [Google Scholar]
- Tong, W.J.; Li, L. Analysis of Flow Field and Machining Parameters in RUREMM for High-precision Micro-texture Fabrication on SS304 Surfaces. Sci. Prog. 2025, in press. [Google Scholar]
- Alom, N.; Saha, U.K.; Dewan, A. In the quest of an appropriate turbulence model for analyzing the aerodynamics of a conventional Savonius (S-type) wind rotor. J. Renew. Sustain. Energy 2021, 13, 023301. [Google Scholar] [CrossRef]
- Velásquez, L.; Rubio-Clemente, A.; Chica, E. Numerical and Experimental Analysis of Vortex Profiles in Gravitational Water Vortex Hydraulic Turbines. Energies 2024, 17, 3543. [Google Scholar] [CrossRef]
- Wang, C.Y.; Li, Z.; Xu, P.; Hou, Y.Q.; Tan, D.P.; Li, L. Collision modelling approach and transient response mechanism of ring-ribbed cylindric shells for underwater vehicles. Appl. Math. Model. 2025, 141, 115923. [Google Scholar] [CrossRef]
- Bumrungthaichaichan, E. A note of caution on numerical scheme selection: Evidence from cyclone separator CFD simulations with appropriate near-wall grid sizes. Powder Technol. 2023, 427, 118713. [Google Scholar] [CrossRef]
- Li, L.; Lu, B.; Xu, W.X.; Wang, C.Y.; Wu, J.F.; Tan, D.P. Dynamic behaviors of multiphase vortex-induced vibration for hydropower energy conversion. Energy 2024, 308, 132897. [Google Scholar] [CrossRef]
- Tan, Y.F.; Ni, Y.S.; Wu, J.F.; Li, L.; Tan, D.P. Machinability evolution of gas–liquid–solid three-phase rotary abrasive flow finishing. Int. J. Adv. Manuf. Technol. 2023, 131, 2145–2164. [Google Scholar] [CrossRef]
- Li, L.; Xu, P.; Li, Q.H.; Zheng, R.Y.; Xu, X.M.; Wu, J.F.; He, B.Y.; Bao, J.J.; Tan, D.P. A coupled LBM-LES-DEM particle flow modeling for microfluidic chip and ultrasonic-based particle aggregation control method. Appl. Math. Model. 2025, in press. [Google Scholar] [CrossRef]
- Wu, J.F.; Xu, P.; Li, L.; Li, Z.; Qi, H.; Wang, C.Y.; Zhang, Y.K.; Xie, Y.S.; Tan, D.P. Multiphase dynamic interfaces and abrasive transport dynamics for abrasive flow machining in shear thickening transition states. Powder Technol. 2024, 446, 120150. [Google Scholar] [CrossRef]
- Lin, H.; Ma, M.; Qi, H.; Wang, X.; Xing, Z.; Alowasheeir, A.; Tang, H.; Chan Jun, S.; Yamauchi, Y.; Liu, S. 3D-Printed photocatalysts for revolutionizing catalytic conversion of solar to chemical energy. Prog. Mater. Sci. 2025, 151, 101427. [Google Scholar] [CrossRef]
- Li, L.; Li, Q.H.; Ni, Y.S.; Wang, C.Y.; Tan, Y.F.; Tan, D.P. Critical penetrating vibration evolution behaviors of the gas-liquid coupled vortex flow. Energy 2024, 292, 130236. [Google Scholar] [CrossRef]
- Yang, X.; Song, F.; Zhang, T.; Yao, X.; Wang, W.; Zhang, Z.; Hou, Y.; Qi, H.; Tang, H. Surface enhancement by micro-arc oxidation induced TiO2 ceramic coating on additive manufacturing Ti-6Al-4V. Chin. J. Mech. Eng. 2024, in press. [Google Scholar]
- Wu, J.F.; Li, L.; Li, Z.; Wang, T.; Tan, Y.F.; Tan, D.P. Mass transfer mechanism of multiphase shear flows and interphase optimization solving method. Energy 2024, 292, 130475. [Google Scholar] [CrossRef]
- Li, L.; Tan, Y.F.; Xu, W.X.; Ni, Y.S.; Yang, J.G.; Tan, D.P. Fluid-induced transport dynamics and vibration patterns of multiphase vortex in the critical transition states. Int. J. Mech. Sci. 2023, 252, 108376. [Google Scholar] [CrossRef]
- Weaver, D.S.; Mišković, S. CFD-DEM validation and simulation of gas–liquid–solid three-phase high-speed jet flow. Chem. Eng. Res. Des. 2024, 201, 561–578. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, M.; Luo, Z.; Yan, Z. Simultaneous modeling of powder rigid motion and molten pool evolution for powder-based additive manufacturing. Powder Technol. 2023, 415, 118118. [Google Scholar] [CrossRef]
- Bilandi, R.N.; Mancini, S.; Dashtimanesh, A.; Tavakoli, S. A revisited verification and validation analysis for URANS simulation of planing hulls in calm water. Ocean Eng. 2024, 293, 116589. [Google Scholar] [CrossRef]
- He, L.; Liu, Z.; Zhao, Y. Study on a semi-resolved CFD-DEM method for rod-like particles in a gas-solid fluidized bed. Particuology 2024, 87, 20–36. [Google Scholar] [CrossRef]
- Yi, H.; Kim, M.; Lee, D.; Park, J. Applications of computational fluid dynamics for mine ventilation in mineral development. Energies 2022, 15, 8405. [Google Scholar] [CrossRef]
- Fernandes, C.M.; Rocha, D.M.; Martins, R.C.; Magalhães, L.; Seabra, J.H. Finite element method model to predict bulk and flash temperatures on polymer gears. Tribol. Int. 2018, 120, 255–268. [Google Scholar] [CrossRef]
- Li, L.; Gu, Z.H.; Xu, W.X.; Tan, Y.F.; Fan, X.H.; Tan, D.P. Mixing mass transfer mechanism and dynamic control of gas-liquid-solid multiphase flow based on VOF-DEM coupling. Energy 2023, 272, 127015. [Google Scholar] [CrossRef]
- Tong, W.; Li, L. Experimental Research of Ultrasonic Cavitation Evolution Mechanism and Model Optimization of RUREMM on Cylindrical Surface. Processes 2024, 12, 0884. [Google Scholar] [CrossRef]
- Zhang, Y.K.; Li, Z.; Li, L.; Wang, C.Y.; Wu, J.F.; Xie, Y.S.; Yin, Z.C.; Tan, D.P. Deposition mechanism of microscopic impacting droplets on flexible porous substrates. Int. J. Mech. Sci. 2025, 288, 110050. [Google Scholar] [CrossRef]
- Tan, Y.F.; Ni, Y.S.; Xu, W.X.; Xie, Y.S.; Li, L.; Tan, D.P. Key technologies and development trends of the soft abrasive flow finishing method. J. Zhejiang Univ.-Sci. A 2023, 24, 1043–1064. [Google Scholar] [CrossRef]
- Qi, H.; Zhang, Z.; Huang, M.; Song, C.; Yang, X.; Tang, H. Enhancing the mechanical behaviors of 18Ni300 steel through microstructural evolution in electron beam powder bed fusion. J. Mater. Res. Technol. 2025, in press. [Google Scholar]
- Marchesse, Y.; Changenet, C.; Ville, F. Drag Power Loss Investigation in Cylindrical Roller Bearings Using CFD Approach. Tribol. Trans 2019, 62, 403–411. [Google Scholar] [CrossRef]
- Xu, W.X.; Xu, P.; Yang, Y.; Tan, D.P.; Li, L. The utilization and advancement of laser ultrasound testing in the assessment of aerospace composite characteristics: A review. Chin. J. Aeronaut. 2025, in press. [Google Scholar]
- Gu, Y.H.; Li, L.; Zheng, G.A. Study on the dynamic characteristics of the gear lubrication flow field with baffles and optimization design strategies. Lubricants 2025, in press. [Google Scholar]
- Li, L.; Xu, P.; Li, Q.H.; Yin, Z.C.; Zheng, R.Y.; Wu, J.F.; Bao, J.J.; Qi, H.; Tan, D.P. Multi-field coupling mixing transfer mechanism of the microreactor and the ultrasonic control method. Powder Technol. 2025, 454, 120731. [Google Scholar] [CrossRef]
- Roda-Casanova, V.; Gonzalez-Perez, I. Investigation of the effect of contact pattern design on the mechanical and thermal behaviors of plastic-steel helical gear drives. Mech. Mach. Theory 2021, 164, 104401. [Google Scholar] [CrossRef]
- Wang, T.; Tan, D.P.; Hou, Y.Q.; Wang, C.Y.; Cheng, J.W.; Song, W.L. Analytical and experimental investigation of vibration response for the cracked fluid-filled thin cylindrical shell under transport condition. Appl. Math. Model. 2025, 142, 115969. [Google Scholar] [CrossRef]
- Fu, D.N.; Sheng, J.; Wang, L.J.; Zhang, X.J.; Yang, R.D.; Li, X.K.; Wang, Y. In situ silver-loaded cellulose for high-strength antibacterial composite air filtration paper. Cellulose 2025, in press. [Google Scholar] [CrossRef]
- Zheng, G.A.; Xu, P.; Wang, T.; Yan, Q. Study on the bubble collapse characteristics and heat transfer mechanism of the microchannel reactor. Processes 2025, 13, 281. [Google Scholar] [CrossRef]
- Patil, H.; Patel, A.K.; Pant, H.J.; Vinod, A. CFD simulation model for mixing tank using multiple reference frame (MRF) impeller rotation. ISH J. Hydraul. Eng. 2021, 27, 200–209. [Google Scholar] [CrossRef]
- Zheng, G.A.; Weng, X.X.; Wang, T.; Xu, P.; Xu, W.X.; Li, L.; Xu, X.F.; Tan, D.P. Piezoelectric ultrasonic coupling-based polishing of micro-tapered holes with abrasive flow. J. Zhejiang Univ.-Sci. A 2025, in press. [Google Scholar]
- Lee, M.; Park, G.; Park, C.; Kim, C. Improvement of grid independence test for computational fluid dynamics model of building based on grid resolution. Adv. Civ. Eng. 2020, 2020, 8827936. [Google Scholar] [CrossRef]
- Xu, P.; Li, Q.H.; Wang, C.Y.; Li, L.; Tan, D.P.; Wu, H.P. Interlayer healing mechanism of multipath deposition 3D printing models and interlayer strength regulation method. J. Manuf. Process. 2025, in press. [Google Scholar]
- Kim, R.W.; Lee, I.B.; Kwon, K.S. Evaluation of wind pressure acting on multi-span greenhouses using CFD technique, Part 1: Development of the CFD model. Biosyst. Eng. 2017, 164, 235–256. [Google Scholar] [CrossRef]
- Peng, J.; Sun, W.; Han, H.; Xie, L. CFD modeling and simulation of the hydrodynamics characteristics of coarse coal particles in a 3D liquid-solid fluidized bed. Minerals 2021, 11, 569. [Google Scholar] [CrossRef]





| Parameter | Value |
|---|---|
| Lubricating oil density (kg/m3) | 880 |
| Lubricating oil dynamic viscosity (Pa·s) | 0.06 |
| Lubricating oil height h (mm) | 10, 15, 20 |
| Air density (kg/m3) | 1.225 |
| Air dynamic viscosity (Pa·s) | 1.7894 × 10−5 |
| Rotation speed n (r × min−1) | 3200, 3600, 4000 |
| Time step ∆t (s) | 2 × 10−5 |
| Number of time steps | 7500 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Y.; Ge, M.; Zheng, G. Study on the Dynamic Modeling of Two-Phase Flow and Lubrication Characteristics of Toothless Stirring Oil Pans. Processes 2025, 13, 829. https://doi.org/10.3390/pr13030829
Huang Y, Ge M, Zheng G. Study on the Dynamic Modeling of Two-Phase Flow and Lubrication Characteristics of Toothless Stirring Oil Pans. Processes. 2025; 13(3):829. https://doi.org/10.3390/pr13030829
Chicago/Turabian StyleHuang, Yiming, Man Ge, and Gaoan Zheng. 2025. "Study on the Dynamic Modeling of Two-Phase Flow and Lubrication Characteristics of Toothless Stirring Oil Pans" Processes 13, no. 3: 829. https://doi.org/10.3390/pr13030829
APA StyleHuang, Y., Ge, M., & Zheng, G. (2025). Study on the Dynamic Modeling of Two-Phase Flow and Lubrication Characteristics of Toothless Stirring Oil Pans. Processes, 13(3), 829. https://doi.org/10.3390/pr13030829




