The Oil/Water Two-Phase Flow Behavior of Dual-Porosity Carbonates
Abstract
1. Introduction
2. Materials and Methods
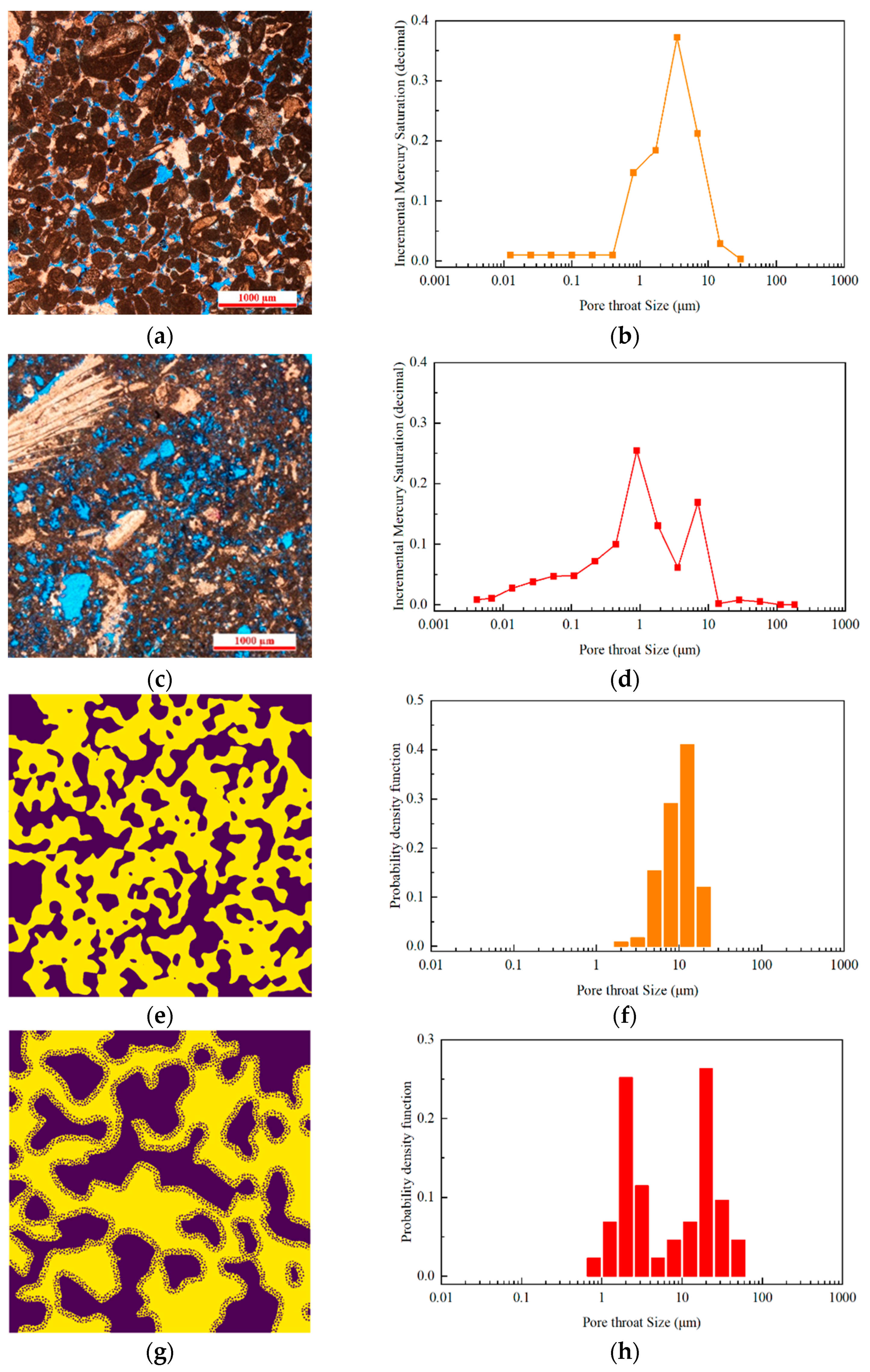
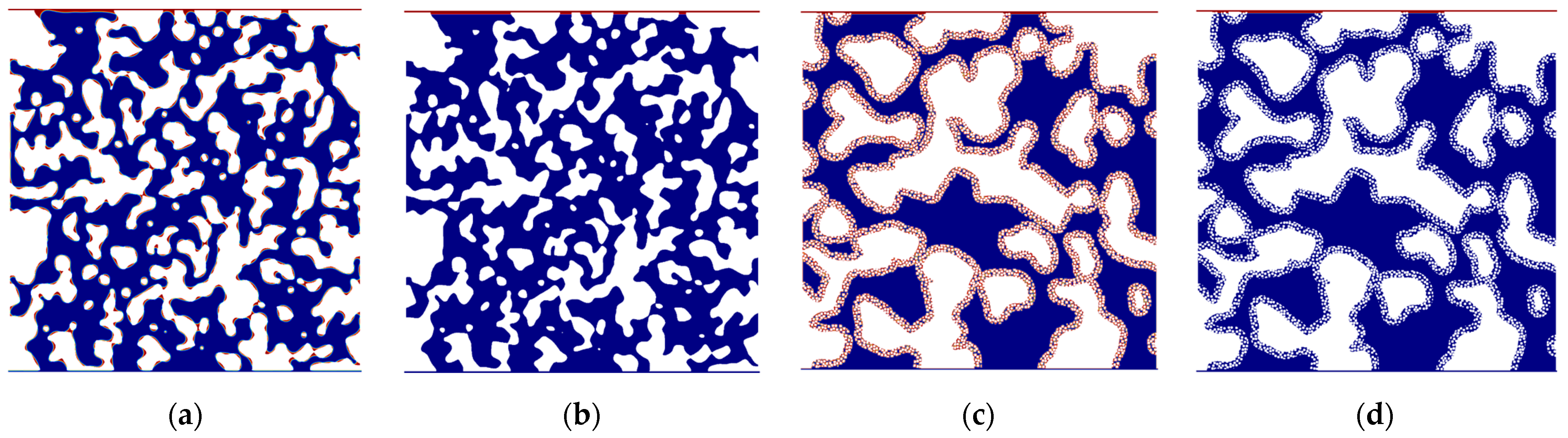
2.1. Porous Medium Generation
2.2. Lattice Boltzmann Method
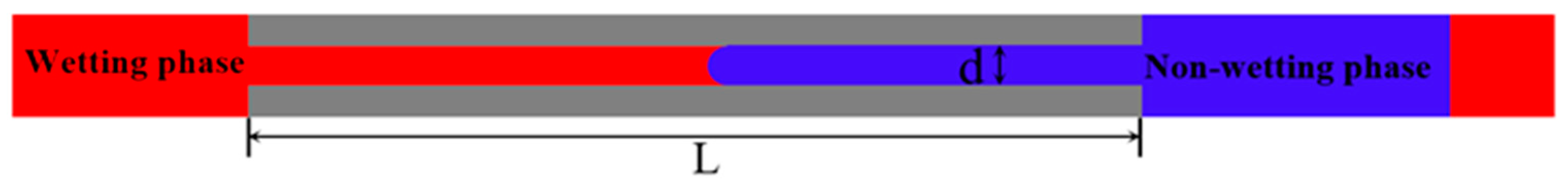
2.3. Simulation Set-Up
3. Results and Discussion
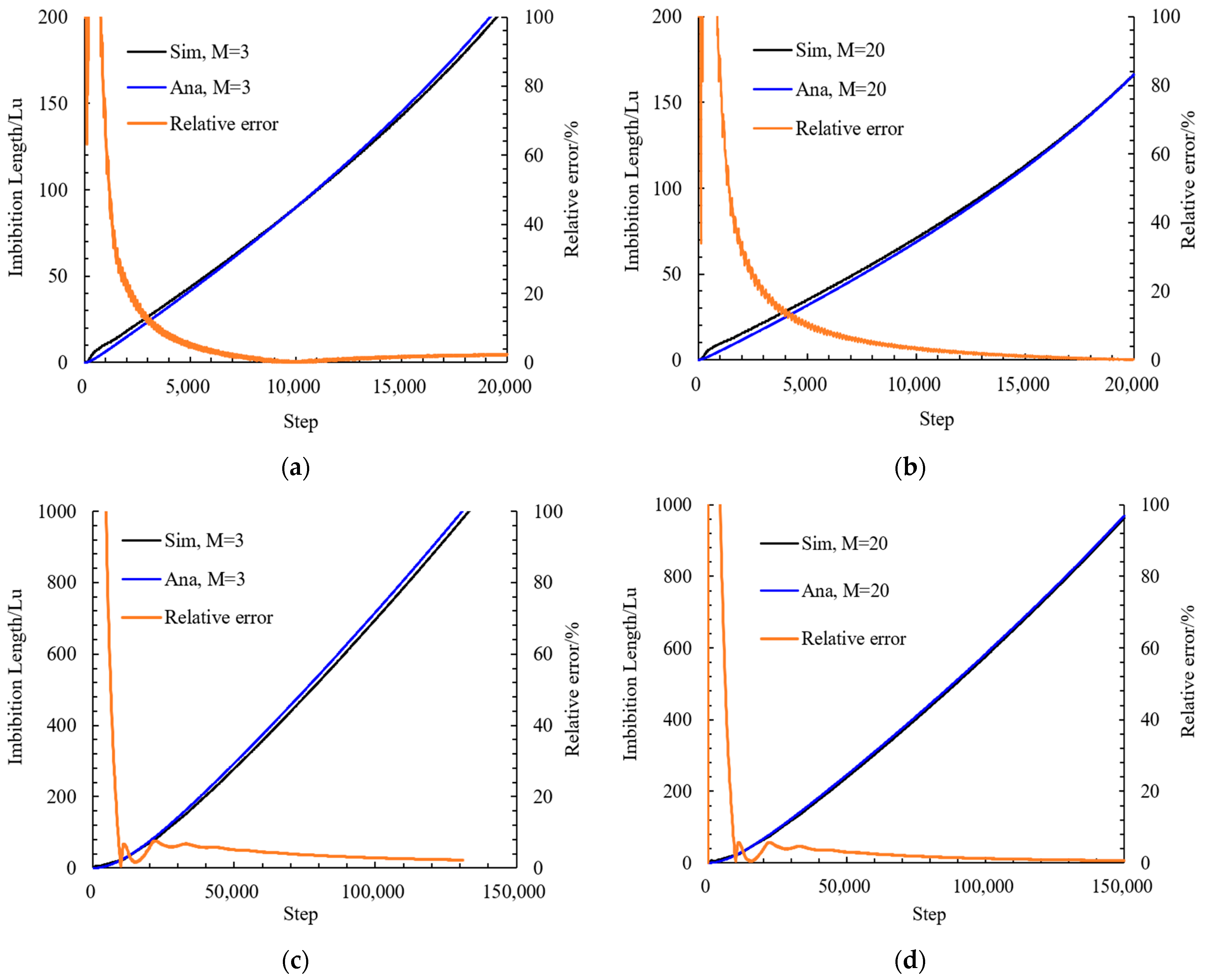
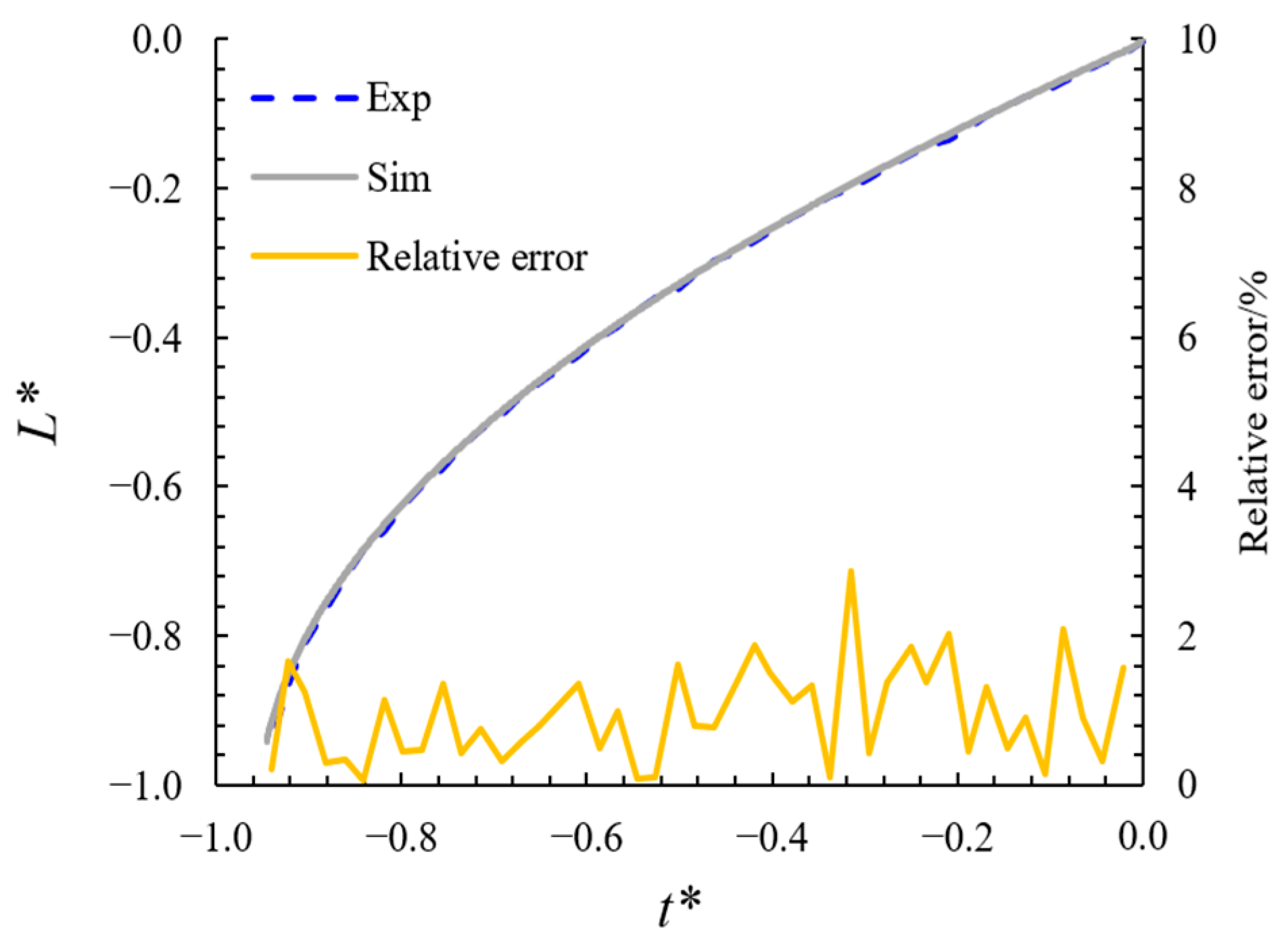
3.1. Model Validation
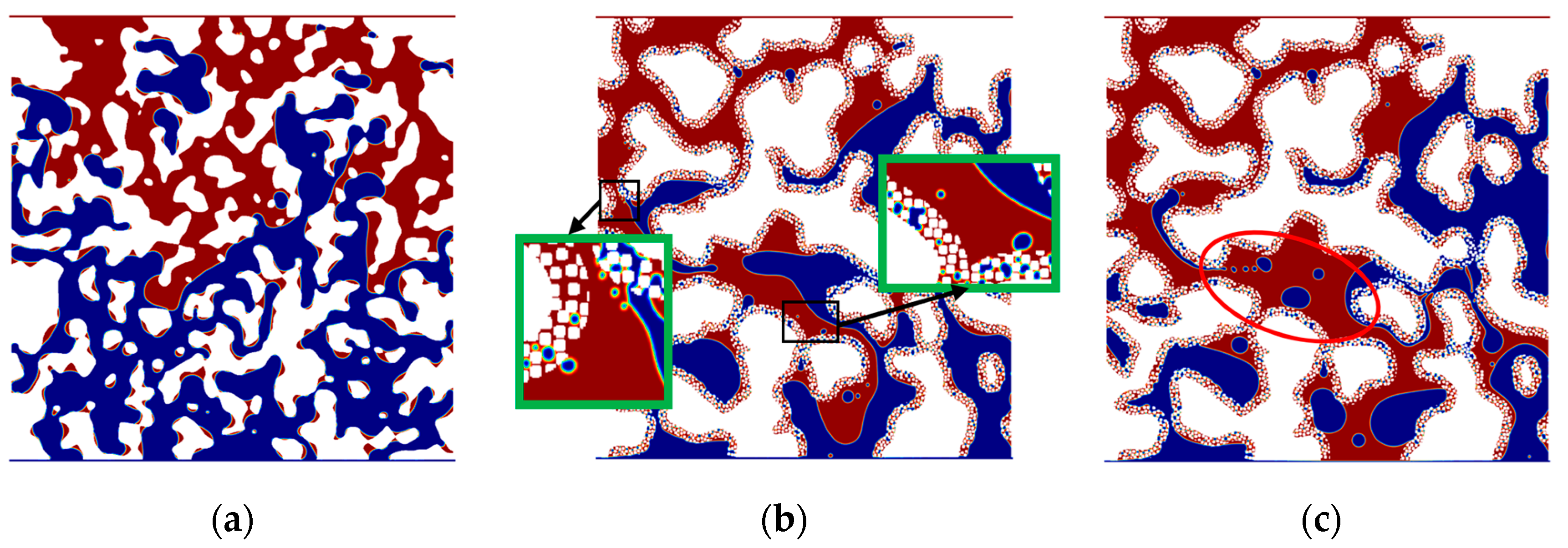
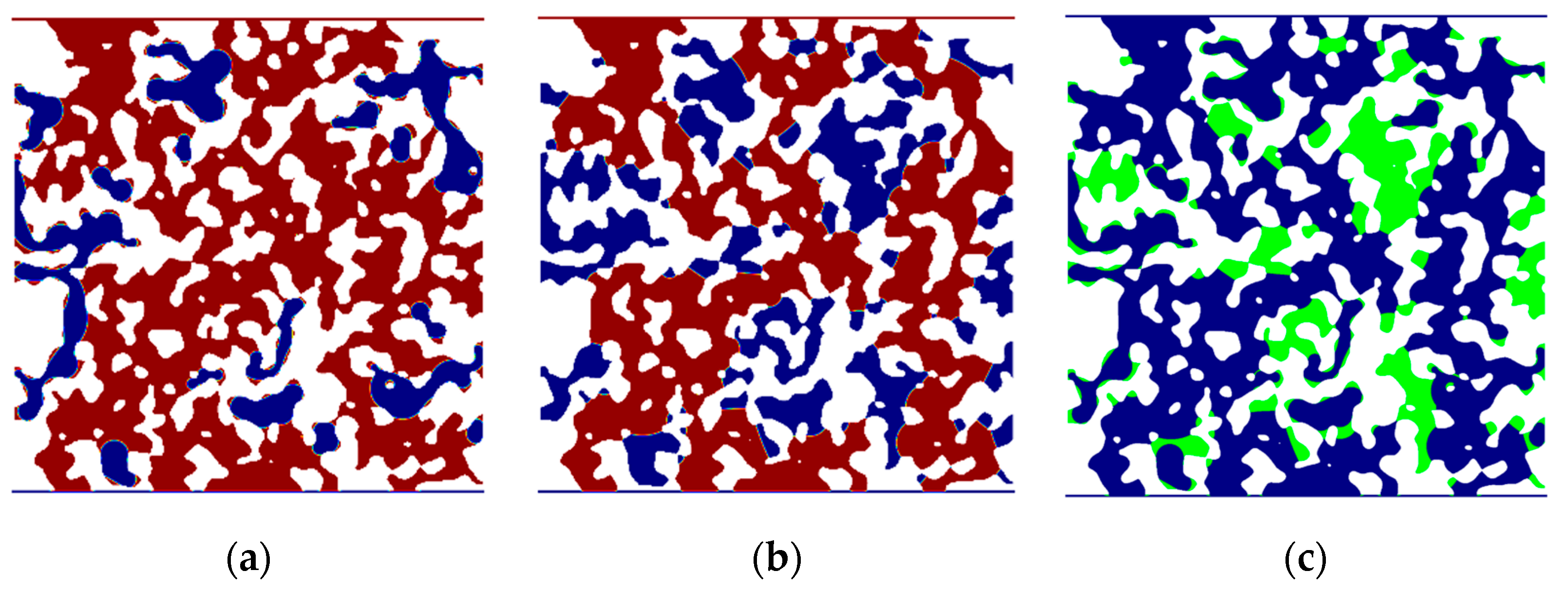
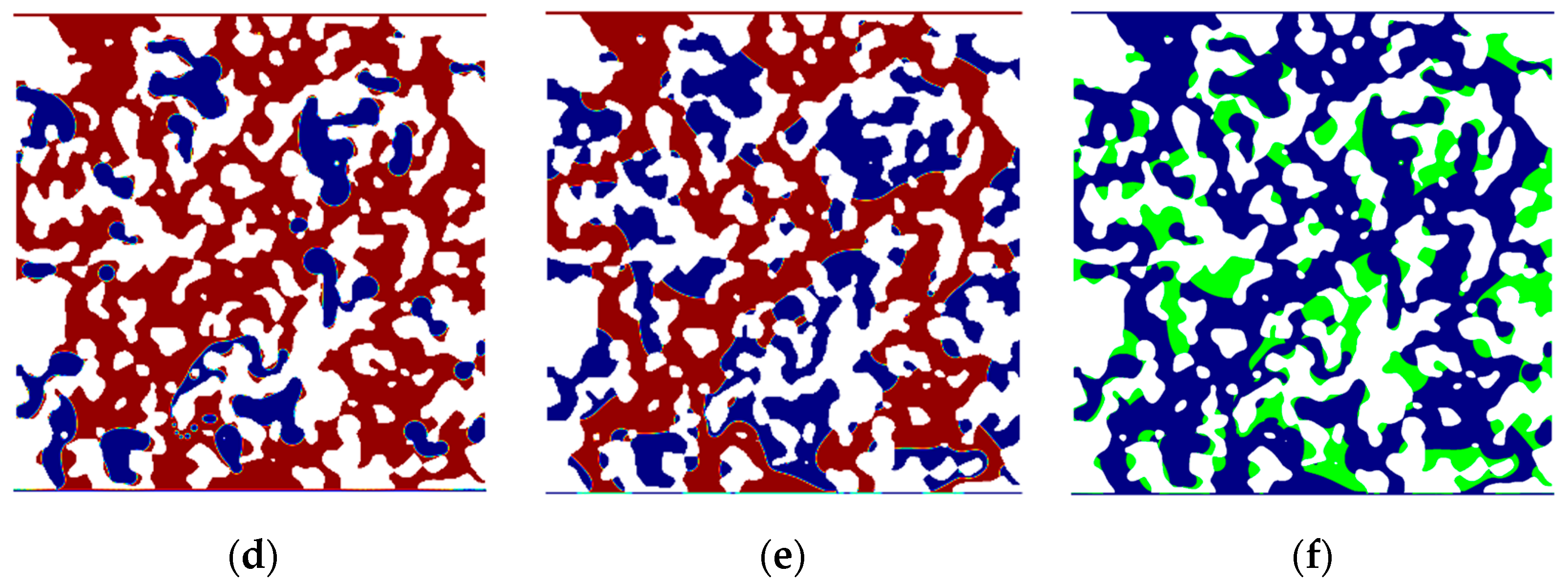
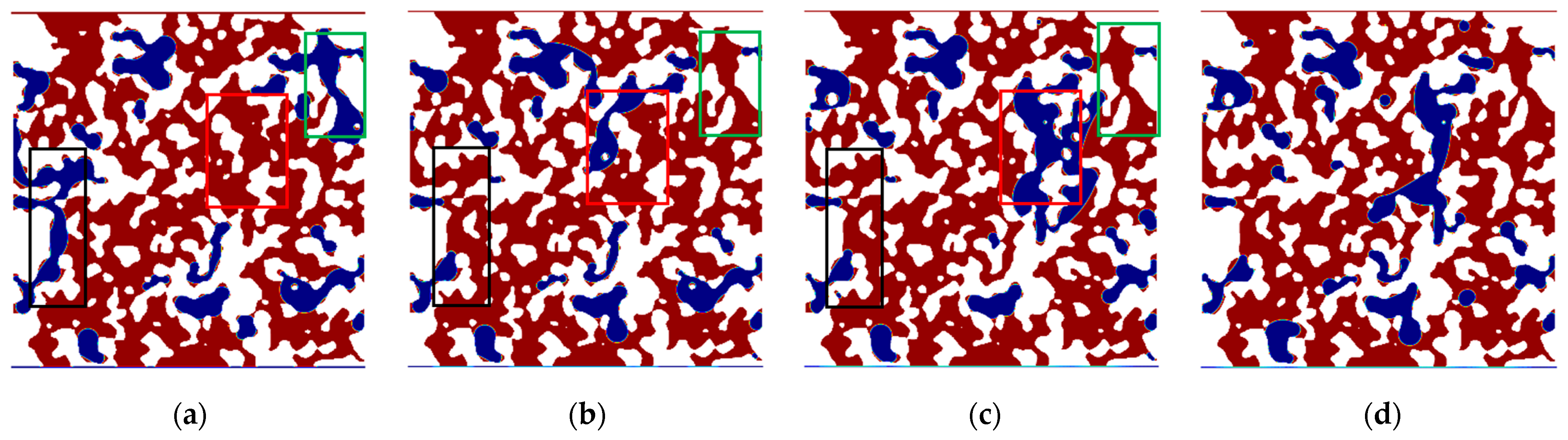
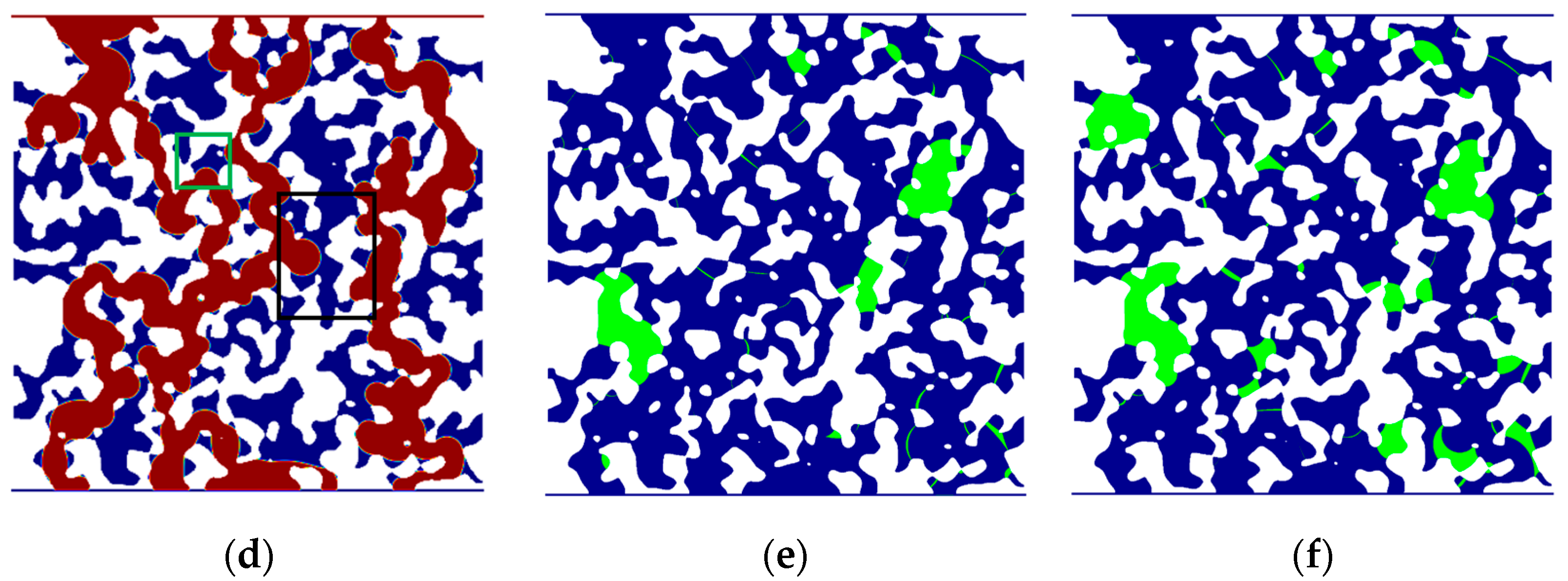
3.2. The Interface Evolution Pattern
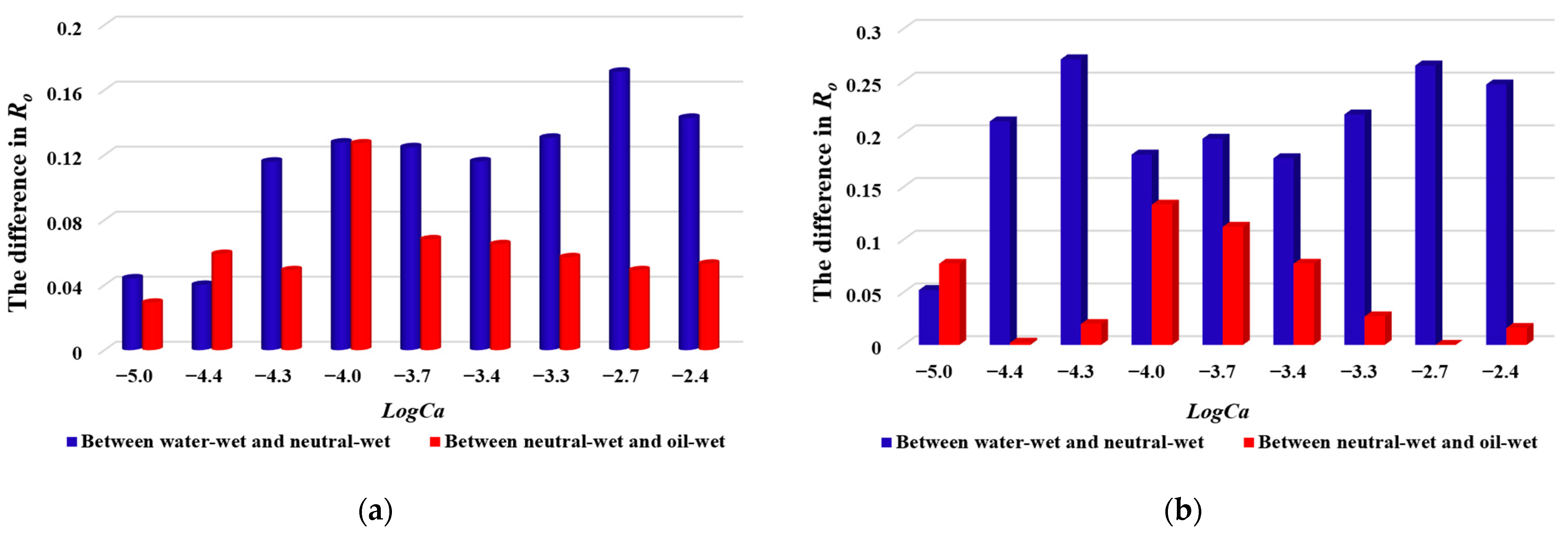
3.3. Effect of Wettability on Oil Displacement Efficiency
3.4. Effect of Ca on Oil Displacement Efficiency
3.5. Effect of Viscosity Ratio on Oil Displacement Efficiency
4. Conclusions
- In dual-porosity porous media under water-wet conditions and low capillary numbers, micrite particle blockage significantly alters flow paths, affecting interface progression and oil entrapment. At high capillary numbers, the imbibition effect and oil fragmentation caused by micrite particles generate more unstable interfaces. Under neutral-wet and oil-wet conditions, when capillary forces dominate, oil displacement within the macropores of dual-porosity porous media becomes more favorable.
- Under identical conditions, single-porosity porous media exhibit higher oil displacement efficiency than dual-porosity porous media. Both structures show the highest efficiency under water-wet conditions, while neutral-wet and oil-wet conditions lead to lower oil displacement efficiency. Dual-porosity porous media are more significantly influenced by the capillary resistance of micropore throats, which hinders the mobilization of oil in micropores and inaccessible macropores. As the capillary number increases, the difference in oil displacement efficiency between neutral-wet and oil-wet conditions diminishes. This indicates that wettability modification and improved waterflooding techniques can help mitigate the challenges posed by capillary resistance in dual-porosity carbonate reservoirs, ultimately improving oil recovery.
- Dual-porosity porous media demonstrates a distinct non-monotonic variation in oil displacement efficiency as a function of the capillary number. The efficiency initially increases, followed by a gradual decline, and then rises again. In non-water-wet porous media, the initial increase is primarily controlled by viscous forces, while the subsequent decline is dominated by capillary forces. Understanding this non-monotonic behavior can aid in designing injection parameters that balance viscous and capillary forces, enabling more effective oil recovery in complex carbonate systems.
- Dual-porosity porous media demonstrate a smaller reduction in oil displacement efficiency as viscosity ratios increase compared to single-porosity porous media. Under high capillary numbers, the decline in oil displacement efficiency is more pronounced in oil-wet conditions than in water-wet conditions.
- In summary, this study emphasizes the role of micrite particles and capillary resistance in oil displacement efficiency in dual-porosity media, aiding the optimization of waterflooding and wettability alteration techniques. The numerical simulation framework also supports efficient development of complex carbonate reservoirs and paves the way for future experimental validation and field applications.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Manrique, E.J.; Muci, V.E.; Gurfinkel, M.E. EOR Field Experiences in Carbonate Reservoirs in the United States. SPE Reserv. Eval. Eng. 2007, 10, 667–686. [Google Scholar] [CrossRef]
- Song, X.; Li, Y. Optimum Development Options and Strategies for Water Injection Development of Carbonate Reservoirs in the Middle East. Pet. Explor. Dev. 2018, 45, 723–734. [Google Scholar] [CrossRef]
- Xu, Z.-X.; Li, S.-Y.; Li, B.-F.; Chen, D.-Q.; Liu, Z.-Y.; Li, Z.-M. A Review of Development Methods and EOR Technologies for Carbonate Reservoirs. Pet. Sci. 2020, 17, 990–1013. [Google Scholar] [CrossRef]
- Moore, C.H.; Wade, W.J. Carbonate Reservoirs: Porosity and Diagenesis in a Sequence Stratigraphic Framework; Newnes: Oxford, UK, 2013; ISBN 0-444-53832-1. [Google Scholar]
- Dernaika, M.; Mansour, B.; Al-Jallad, O.; Koronfol, S. Overview of Carbonate Rock Types in the Middle East. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 21 March 2019; SPE: Manama, Bahrain, 2019; p. D042S098R002. [Google Scholar]
- El Husseiny, A.; Vanorio, T. Porosity-Permeability Relationship in Dual-Porosity Carbonate Analogs. Geophysics 2017, 82, MR65–MR74. [Google Scholar] [CrossRef]
- Miller, K.; Vanorio, T.; Yang, S.; Xiao, X. A Scale-Consistent Method for Imaging Porosity and Micrite in Dual-Porosity Carbonate Rocks. Geophysics 2019, 84, MR115–MR127. [Google Scholar] [CrossRef]
- Hu, J.; Yang, S.; Jiang, Y.; Deng, H.; Wang, M.; You, L.; Li, Q.; Bai, H.; Shen, B. Experimental Study on Edge Water Invasion of Strongly Heterogeneous Carbonate Gas Reservoirs Based on NMR Technology. Processes 2024, 12, 1361. [Google Scholar] [CrossRef]
- Zhao, L.; Nasser, M.; Han, D. Quantitative Geophysical Pore-type Characterization and Its Geological Implication in Carbonate Reservoirs. Geophys. Prospect. 2013, 61, 827–841. [Google Scholar] [CrossRef]
- Li, H.-B.; Zhang, J.-J.; Cai, S.-J.; Pan, H.-J. A Two-Step Method to Apply Xu–Payne Multi-Porosity Model to Estimate Pore Type from Seismic Data for Carbonate Reservoirs. Pet. Sci. 2020, 17, 615–627. [Google Scholar] [CrossRef]
- Zhang, H.; Peng, W.; Hao, P.; Du, M. A Heterogeneous Model for Simulating Fluid Flow in Naturally Fractured-Vuggy Carbonate Reservoirs. Int. J. Oil Gas Coal Technol. 2019, 20, 397. [Google Scholar] [CrossRef]
- Kang, L.; Guo, W.; Zhang, X.; Liu, Y.; Gao, J.; Shao, Z.; Li, M.; Sun, Y. Two-Phase Flow Model and Productivity Evaluation of Gas and Water for Dual-Medium Carbonate Gas Reservoirs. Front. Earth Sci. 2022, 9, 823764. [Google Scholar] [CrossRef]
- Li, J.; Liu, Y.; Gao, Y.; Cheng, B.; Meng, F.; Xu, H. Effects of Microscopic Pore Structure Heterogeneity on the Distribution and Morphology of Remaining Oil. Pet. Explor. Dev. 2018, 45, 1112–1122. [Google Scholar] [CrossRef]
- Guo, C.; Wang, X.; Wang, H.; He, S.; Liu, H.; Zhu, P. Effect of Pore Structure on Displacement Efficiency and Oil-Cluster Morphology by Using Micro Computed Tomography (μCT) Technique. Fuel 2018, 230, 430–439. [Google Scholar] [CrossRef]
- Namaee-Ghasemi, A.; Ayatollahi, S.; Mahani, H. Insights into the Effects of Pore Structure, Time Scale, and Injection Scenarios on Pore-Filling Sequence and Oil Recovery by Low-Salinity Waterflooding Using a Mechanistic DLVO-Based Pore-Scale Model. SPE J. 2023, 28, 1760–1776. [Google Scholar] [CrossRef]
- Riaz, A.; Tang, G.-Q.; Tchelepi, H.A.; Kovscek, A.R. Forced Imbibition in Natural Porous Media: Comparison between Experiments and Continuum Models. Phys. Rev. E 2007, 75, 036305. [Google Scholar] [CrossRef]
- Liu, H.; Sun, S.; Wu, R.; Wei, B.; Hou, J. Pore-Scale Modeling of Spontaneous Imbibition in Porous Media Using the Lattice Boltzmann Method. Water Resour. Res. 2021, 57, e2020WR029219. [Google Scholar] [CrossRef]
- Qin, C.; Wang, X.; Hefny, M.; Zhao, J.; Chen, S.; Guo, B. Wetting Dynamics of Spontaneous Imbibition in Porous Media: From Pore Scale to Darcy Scale. Geophys. Res. Lett. 2022, 49, e2021GL097269. [Google Scholar] [CrossRef]
- Wennberg, O.P.; Casini, G.; Jonoud, S.; Peacock, D.C.P. The Characteristics of Open Fractures in Carbonate Reservoirs and Their Impact on Fluid Flow: A Discussion. Pet. Geosci. 2016, 22, 91–104. [Google Scholar] [CrossRef]
- Qi, C.; Liu, Y.; Dong, F.; Liu, X.; Yang, X.; Shen, Y.; Huang, H. Study on Heterogeneity of Pore Throats at Different Scales and Its Influence on Seepage Capacity in Different Types of Tight Carbonate Reservoirs. Geofluids 2020, 2020, 1–7. [Google Scholar] [CrossRef]
- Soulaine, C.; Gjetvaj, F.; Garing, C.; Roman, S.; Russian, A.; Gouze, P.; Tchelepi, H.A. The Impact of Sub-Resolution Porosity of X-Ray Microtomography Images on the Permeability. Transp. Porous Med. 2016, 113, 227–243. [Google Scholar] [CrossRef]
- Carrillo, F.J.; Soulaine, C.; Bourg, I.C. The Impact of Sub-Resolution Porosity on Numerical Simulations of Multiphase Flow. Adv. Water Resour. 2022, 161, 104094. [Google Scholar] [CrossRef]
- Suo, S.; Liu, M.; Gan, Y. Fingering Patterns in Hierarchical Porous Media. Phys. Rev. Fluids 2020, 5, 034301. [Google Scholar] [CrossRef]
- Gao, Y.; Qaseminejad Raeini, A.; Blunt, M.J.; Bijeljic, B. Pore Occupancy, Relative Permeability and Flow Intermittency Measurements Using X-Ray Micro-Tomography in a Complex Carbonate. Adv. Water Resour. 2019, 129, 56–69. [Google Scholar] [CrossRef]
- Spurin, C.; Bultreys, T.; Bijeljic, B.; Blunt, M.J.; Krevor, S. Intermittent Fluid Connectivity during Two-Phase Flow in a Heterogeneous Carbonate Rock. Phys. Rev. E 2019, 100, 043103. [Google Scholar] [CrossRef]
- Wang, D.; Liu, F.; Sun, J.; Li, Y.; Wang, Q.; Jiao, Y.; Song, K.; Wang, S.; Ma, R. Lattice-Boltzmann Simulation of Two-Phase Flow in Carbonate Porous Media Retrieved from Computed Microtomography. Chem. Eng. Sci. 2023, 270, 118514. [Google Scholar] [CrossRef]
- Liu, Y.; Min, J.; Zhang, X. A Novel Micro-Continuum Lattice Boltzmann Approach for Multiscale Modeling Immiscible Two-Phase Flow in Porous Media. Phys. Fluids 2024, 36, 083345. [Google Scholar] [CrossRef]
- Chen, L.; Kang, Q.; Mu, Y.; He, Y.-L.; Tao, W.-Q. A Critical Review of the Pseudopotential Multiphase Lattice Boltzmann Model: Methods and Applications. Int. J. Heat Mass Transf. 2014, 76, 210–236. [Google Scholar] [CrossRef]
- Khadri, S.O.; Hussein, I.A.; Sadooni, F.; Shirif, E. CFD Estimation of Gas Production in Tight Carbonates Using Single and Dual-Porosity Models. Sci. Rep. 2023, 13, 22668. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Liu, P.; Zhou, C.; Bate, B.; Chen, Y. Numerical Pore-Scale Investigation of Two-Phase Displacement with Non-Newtonian Defending Fluid. Phys. Fluids 2024, 36, 093105. [Google Scholar] [CrossRef]
- Raeini, A.Q.; Blunt, M.J.; Bijeljic, B. Modelling Two-Phase Flow in Porous Media at the Pore Scale Using the Volume-of-Fluid Method. J. Comput. Phys. 2012, 231, 5653–5668. [Google Scholar] [CrossRef]
- Ambekar, A.S.; Mattey, P.; Buwa, V.V. Pore-Resolved Two-Phase Flow in a Pseudo-3D Porous Medium: Measurements and Volume-of-Fluid Simulations. Chem. Eng. Sci. 2021, 230, 116128. [Google Scholar] [CrossRef]
- Jettestuen, E.; Helland, J.O.; Prodanović, M. A Level Set Method for Simulating Capillary-controlled Displacements at the Pore Scale with Nonzero Contact Angles. Water Resour. Res. 2013, 49, 4645–4661. [Google Scholar] [CrossRef]
- Shi, H.; Zhu, Q.; Chen, Z.; Li, J.; Feng, D.; Zhang, S.; Ye, J.; Wu, K. Pore-Scale Modeling of Water–Gas Flow in Heterogeneous Porous Media. Phys. Fluids 2023, 35, 072114. [Google Scholar] [CrossRef]
- Li, B.; Yu, H.; Ji, D.; Wang, F.; Lei, Z.; Wu, H. Pore-Scale Imbibition Patterns in Layered Porous Media with Fractures. Phys. Fluids 2024, 36, 012120. [Google Scholar] [CrossRef]
- Taghilou, M.; Rahimian, M.H. Investigation of Two-Phase Flow in Porous Media Using Lattice Boltzmann Method. Comput. Math. Appl. 2014, 67, 424–436. [Google Scholar] [CrossRef]
- Wu, Y.; Gui, N.; Yang, X.; Tu, J.; Jiang, S. Improved Stability Strategies for Pseudo-Potential Models of Lattice Boltzmann Simulation of Multiphase Flow. Int. J. Heat Mass Transf. 2018, 125, 66–81. [Google Scholar] [CrossRef]
- Ezzatneshan, E.; Goharimehr, R. A Pseudopotential Lattice Boltzmann Method for Simulation of Two-Phase Flow Transport in Porous Medium at High-Density and High–Viscosity Ratios. Geofluids 2021, 2021, 1–18. [Google Scholar] [CrossRef]
- Leclaire, S.; Parmigiani, A.; Malaspinas, O.; Chopard, B.; Latt, J. Generalized Three-Dimensional Lattice Boltzmann Color-Gradient Method for Immiscible Two-Phase Pore-Scale Imbibition and Drainage in Porous Media. Phys. Rev. E 2017, 95, 033306. [Google Scholar] [CrossRef]
- Lafarge, T.; Boivin, P.; Odier, N.; Cuenot, B. Improved Color-Gradient Method for Lattice Boltzmann Modeling of Two-Phase Flows. Phys. Fluids 2021, 33, 082110. [Google Scholar] [CrossRef]
- Inamuro, T.; Konishi, N.; Ogino, F. A Galilean Invariant Model of the Lattice Boltzmann Method for Multiphase Fluid Flows Using Free-Energy Approach. Comput. Phys. Commun. 2000, 129, 32–45. [Google Scholar] [CrossRef]
- Huang, H.; Zheng, H.; Lu, X.; Shu, C. An Evaluation of a 3D Free-energy-based Lattice Boltzmann Model for Multiphase Flows with Large Density Ratio. Numer. Methods Fluids 2010, 63, 1193–1207. [Google Scholar] [CrossRef]
- Mirhoseini, M.; Banaee, A.; Jalali, A. A Novel Phase-Field Lattice Boltzmann Framework for Diffusion-Driven Multiphase Evaporation. Phys. Fluids 2024, 36, 083314. [Google Scholar] [CrossRef]
- Wang, M.; Wu, K.; Chen, Z.; Li, J.; Feng, D. Classification and Evaluation of Microscopic Pore Structure in Carbonate Rocks by Integrating MICP-Based Dynamic Information. In Proceedings of the SPE Western Regional Meeting, Palo Alto, CA, USA, 18 April 2024; SPE: Palo Alto, CA, USA, 2024; p. D031S015R007. [Google Scholar]
- Bauer, D.; Youssef, S.; Fleury, M.; Bekri, S.; Rosenberg, E.; Vizika, O. Improving the Estimations of Petrophysical Transport Behavior of Carbonate Rocks Using a Dual Pore Network Approach Combined with Computed Microtomography. Transp. Porous Med. 2012, 94, 505–524. [Google Scholar] [CrossRef]
- Kim, K.; Lee, Y.; Hwang, S.; Seo, J.; Sung, W. Improved Capillary Pressure Model Considering Dual-Pore Geometry System in Carbonate Reservoirs. J. Pet. Sci. Eng. 2011, 78, 601–608. [Google Scholar] [CrossRef]
- Gostick, J.; Khan, Z.; Tranter, T.; Kok, M.; Agnaou, M.; Sadeghi, M.; Jervis, R. PoreSpy: A Python Toolkit for Quantitative Analysis of Porous Media Images. J. Open Source Softw. 2019, 4, 1296. [Google Scholar] [CrossRef]
- Tarjus, G.; Schaaf, P.; Talbot, J. Random Sequential Addition: A Distribution Function Approach. J. Stat. Phys. 1991, 63, 167–202. [Google Scholar] [CrossRef]
- Liu, H.; Valocchi, A.J.; Werth, C.; Kang, Q.; Oostrom, M. Pore-Scale Simulation of Liquid CO2 Displacement of Water Using a Two-Phase Lattice Boltzmann Model. Adv. Water Resour. 2014, 73, 144–158. [Google Scholar] [CrossRef]
- Zou, Q.; He, X. On Pressure and Velocity Boundary Conditions for the Lattice Boltzmann BGK Model. Phys. Fluids 1997, 9, 1591–1598. [Google Scholar] [CrossRef]
- Fulcher, R.A.; Ertekin, T.; Stahl, C.D. Effect of Capillary Number and Its Constituents on Two-Phase Relative Permeability Curves. J. Pet. Technol. 1985, 37, 249–260. [Google Scholar] [CrossRef]
- Guo, H.; Song, K.; Hilfer, R. A Critical Review of Capillary Number and Its Application in Enhanced Oil Recovery. In Proceedings of the SPE Improved Oil Recovery Conference, Tulsa, OK, USA, 31 August–4 September 2020; SPE: Tulsa, OK, USA, 2020; p. D031S046R001. [Google Scholar]
- Washburn, E.W. The Dynamics of Capillary Flow. Phys. Rev. 1921, 17, 273–283. [Google Scholar] [CrossRef]
- Diotallevi, F.; Biferale, L.; Chibbaro, S.; Lamura, A.; Pontrelli, G.; Sbragaglia, M.; Succi, S.; Toschi, F. Capillary Filling Using Lattice Boltzmann Equations: The Case of Multi-Phase Flows. Eur. Phys. J. Spec. Top. 2009, 166, 111–116. [Google Scholar] [CrossRef]
- André, J.; Okumura, K. Capillary Replacement in a Tube Prefilled with a Viscous Fluid. Langmuir 2020, 36, 10952–10959. [Google Scholar] [CrossRef] [PubMed]
- Dasgupta, S.; Katava, M.; Faraj, M.; Auth, T.; Gompper, G. Capillary Assembly of Microscale Ellipsoidal, Cuboidal, and Spherical Particles at Interfaces. Langmuir 2014, 30, 11873–11882. [Google Scholar] [CrossRef] [PubMed]
- Xiao, R.; Gupta, R.; Glotzbach, R.C.; Sinha, S.; Teletzke, G.F. Evaluation of Low-Salinity Waterflooding in Middle East Carbonate Reservoirs Using a Novel, Field-Representative Coreflood Method. J. Pet. Sci. Eng. 2018, 163, 683–690. [Google Scholar] [CrossRef]
- Wang, M.; Li, Y.; Wu, K.; Chen, Z.; Li, J.; Feng, D.; Zhu, Q.; Guo, S. Quantitative Characterization of Microscopic Pore Structure and Oil Recovery Evaluation in Porous Carbonate Reservoirs. Pet. Geol. Recovery Effic. 2024, 31, 96–107. [Google Scholar] [CrossRef]









| Physical Quantity | Lattice Units | Physical Units |
|---|---|---|
| Length | 1 lu | 1 × 10−6 m |
| Mass | 1 mu | 1 × 10−15 kg |
| Time | 1 ts | 5 × 10−8 s |
| Porous Media | Initial Sw 1 | Top Inlet | Bottom Outlet | Solid Wall, Left and Right Sides | Contact Angle |
|---|---|---|---|---|---|
| Single-porosity | 0.13 (water-wet) | Non-equilibrium bounce-back scheme (fixed velocity) | Non-equilibrium bounce-back scheme (fixed pressure) | Halfway bounce-back boundary | Virtual density scheme |
| 0 (non-water-wet) | |||||
| Dual-porosity | 0.3 (water-wet) | ||||
| 0 (non-water-wet) |
| Number | Injection Rate (m/s) | Interfacial Tension (mN/m) | Contact Angle (°) | LogCa | Viscosity Ratio |
|---|---|---|---|---|---|
| 1 | 0.01 | 1 | 30 | −2.4 | 3, 10, 20 |
| 2 | 0.01 | 1 | 90 | −2.4 | 3 |
| 3 | 0.01 | 1 | 150 | −2.4 | 10, 20 |
| 4 | 0.01 | 10 | 30 | −3.3 | 3 |
| 5 | 0.01 | 10 | 90 | −3.3 | 3 |
| 6 | 0.01 | 10 | 150 | −3.3 | 3 |
| 7 | 0.01 | 40 | 30 | −4 | 3, 10, 20 |
| 8 | 0.01 | 40 | 90 | −4 | 3 |
| 9 | 0.01 | 40 | 150 | −4 | 3, 10, 20 |
| 10 | 0.005 | 1 | 30 | −2.7 | 3 |
| 11 | 0.005 | 1 | 90 | −2.7 | 3 |
| 12 | 0.005 | 1 | 150 | −2.7 | 3 |
| 13 | 0.005 | 10 | 30 | −3.7 | 3 |
| 14 | 0.005 | 10 | 90 | −3.7 | 3 |
| 15 | 0.005 | 10 | 150 | −3.7 | 3 |
| 16 | 0.005 | 40 | 30 | −4.3 | 3, 10, 20 |
| 17 | 0.005 | 40 | 90 | −4.3 | 3 |
| 18 | 0.001 | 40 | 150 | −4.3 | 3, 10, 20 |
| 19 | 0.001 | 1 | 30 | −3.4 | 3 |
| 20 | 0.001 | 1 | 90 | −3.4 | 3 |
| 21 | 0.001 | 1 | 150 | −3.4 | 3 |
| 22 | 0.001 | 10 | 30 | −4.4 | 3 |
| 23 | 0.001 | 10 | 90 | −4.4 | 3 |
| 24 | 0.001 | 10 | 150 | −4.4 | 3 |
| 25 | 0.001 | 40 | 30 | −5 | 3 |
| 26 | 0.001 | 40 | 90 | −5 | 3 |
| 27 | 0.001 | 40 | 150 | −5 | 3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, M.; Wu, K.; Zhu, Q.; Wang, T.; Dai, W. The Oil/Water Two-Phase Flow Behavior of Dual-Porosity Carbonates. Processes 2025, 13, 713. https://doi.org/10.3390/pr13030713
Wang M, Wu K, Zhu Q, Wang T, Dai W. The Oil/Water Two-Phase Flow Behavior of Dual-Porosity Carbonates. Processes. 2025; 13(3):713. https://doi.org/10.3390/pr13030713
Chicago/Turabian StyleWang, Muyuan, Keliu Wu, Qingyuan Zhu, Tianduoyi Wang, and Weixin Dai. 2025. "The Oil/Water Two-Phase Flow Behavior of Dual-Porosity Carbonates" Processes 13, no. 3: 713. https://doi.org/10.3390/pr13030713
APA StyleWang, M., Wu, K., Zhu, Q., Wang, T., & Dai, W. (2025). The Oil/Water Two-Phase Flow Behavior of Dual-Porosity Carbonates. Processes, 13(3), 713. https://doi.org/10.3390/pr13030713








