Mechanical Analysis of Lined Pipe System Under Temperature–Pressure Coupling in Elastic Laying
Abstract
1. Introduction
2. Numerical Modeling
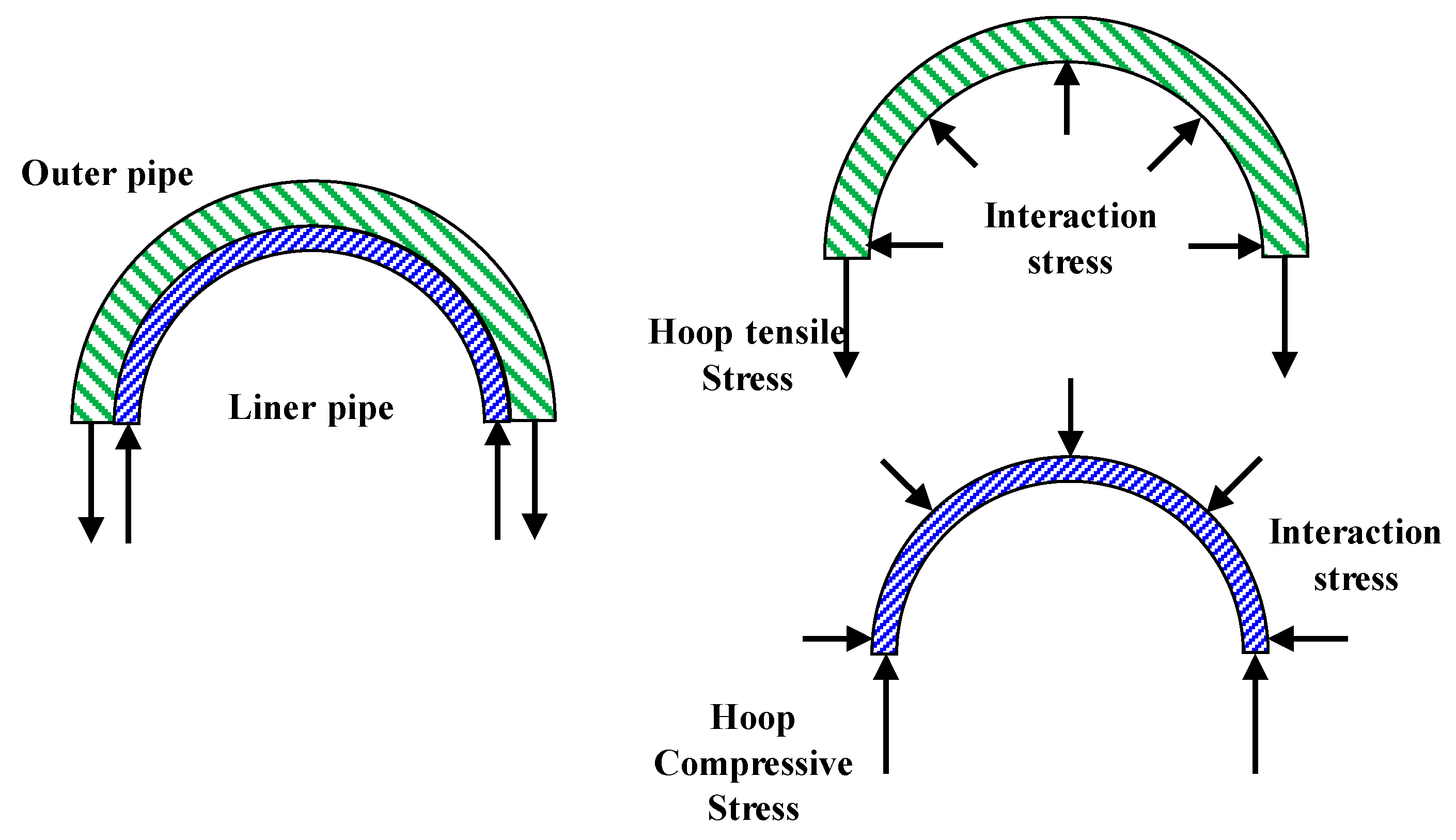
2.1. Contact Modeling
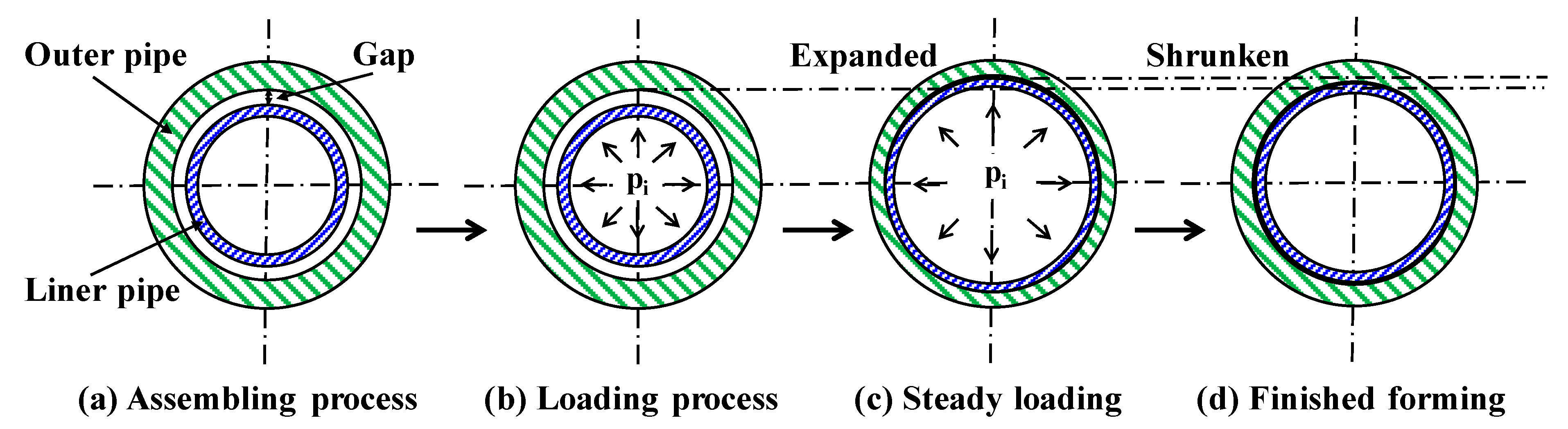
- (a)
- Assembling process. The stainless steel liner and the carbon steel outer pipe are assembled together on the same axis, and the outer diameter of the liner pipe is slightly smaller than the inner diameter of the outer pipe, which ensures a reasonable initial gap before forming.
- (b)
- Loading process. The inner wall of the liner pipe is loaded with hydraulic pressure to make the liner pipe expand radially, eliminate the gap between the two pipes, and make the outer wall of the liner pipe fit with the inner wall of the outer pipe.
- (c)
- Steady loading. When the hydraulic loading reaches the design value, the liner pipe has entered the plastic expansion, the outer pipe is still in the stage of elastic expansion, and the pressure is maintained to make the liner pipe and the outer pipe fully fit.
- (d)
- Finished forming. After unloading the pressure, both the outer pipe and the liner shrink elastically. Due to the residual plastic deformation in the liner, the outer pipe will grip the liner so that an initial tightness is formed between the outer pipe and the liner after hydraulic forming.
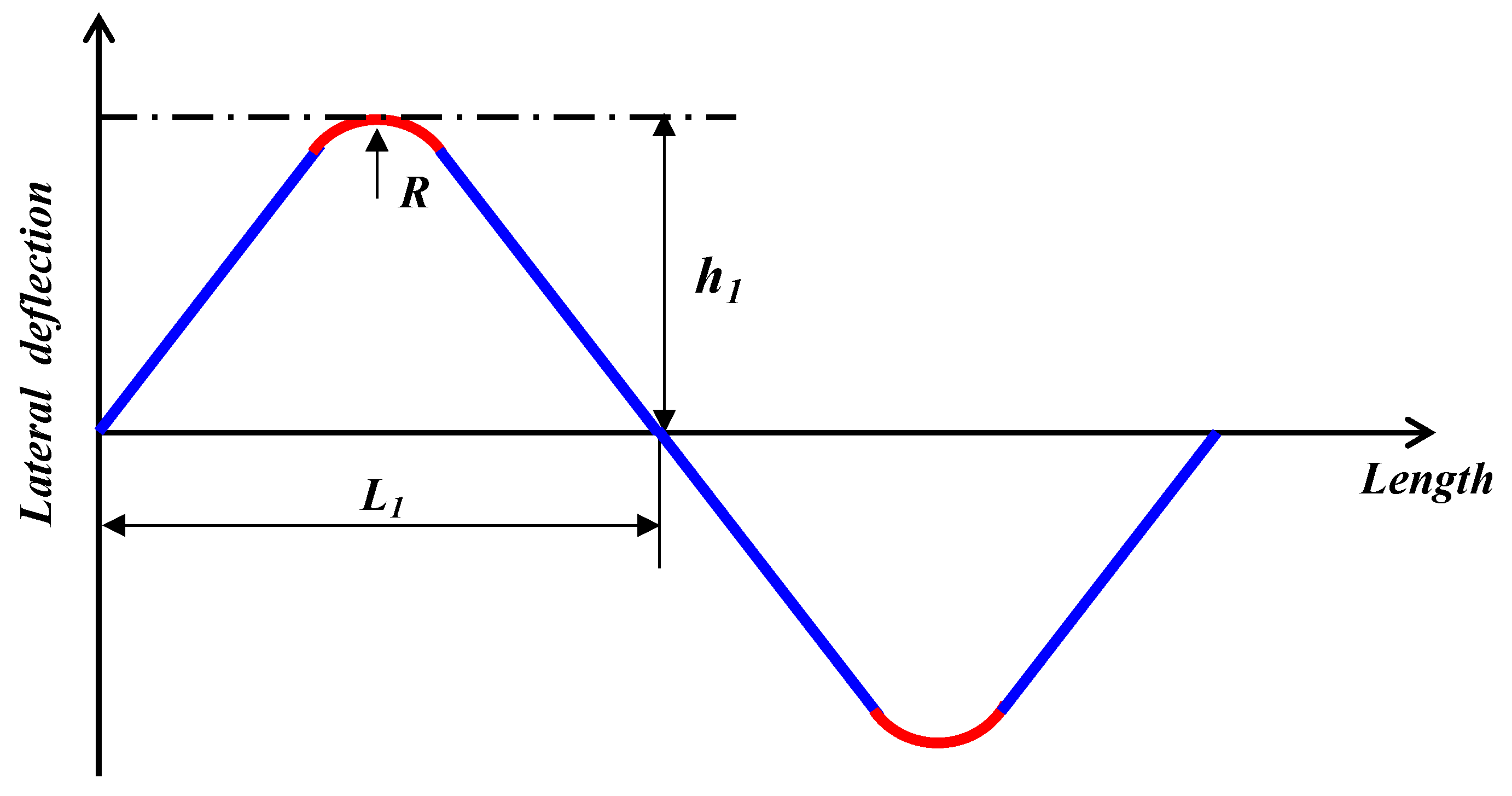
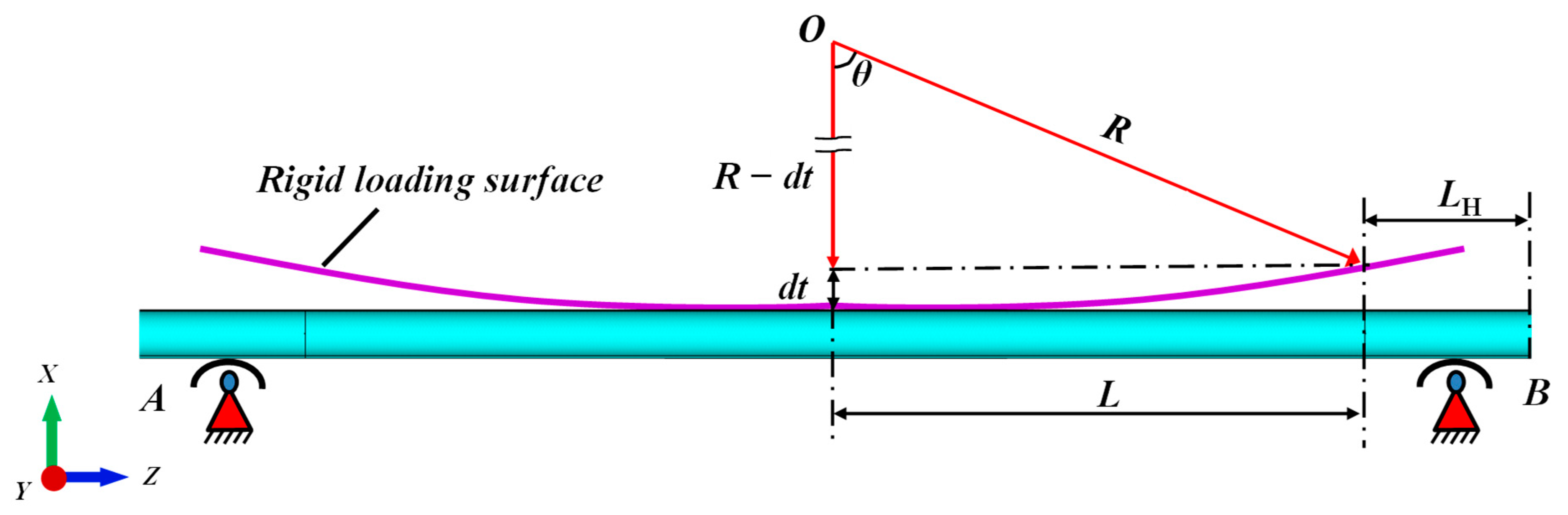
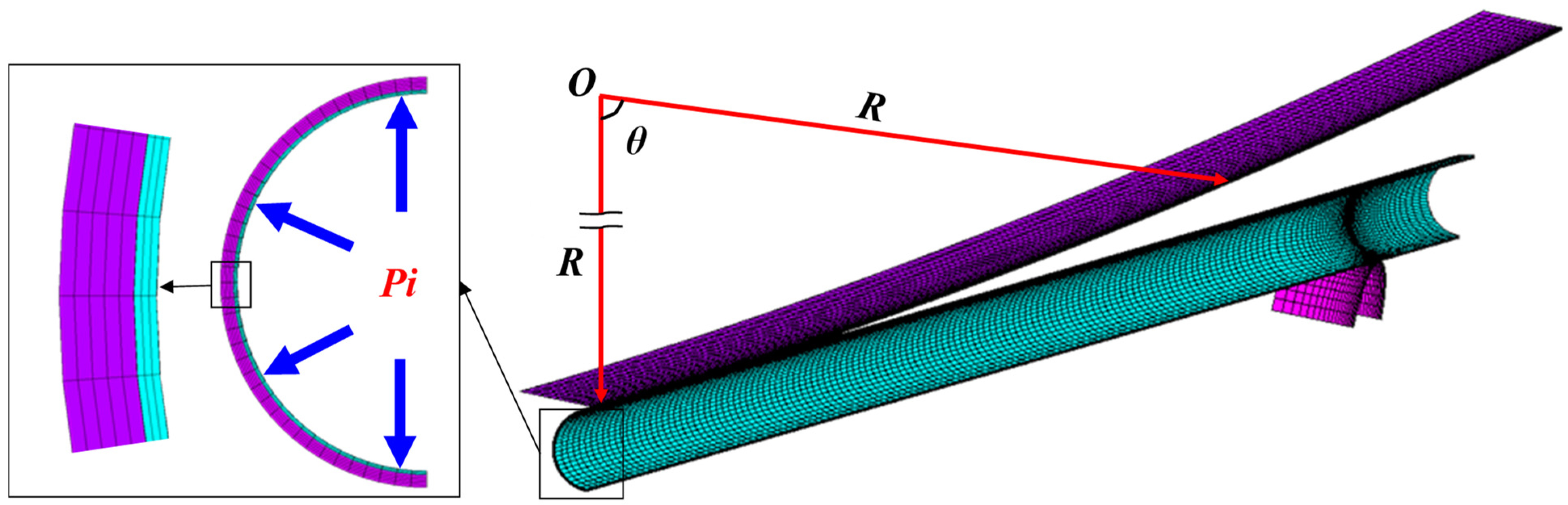
2.2. Elastic Laying Model
3. Results and Discussion
3.1. Stress Analysis
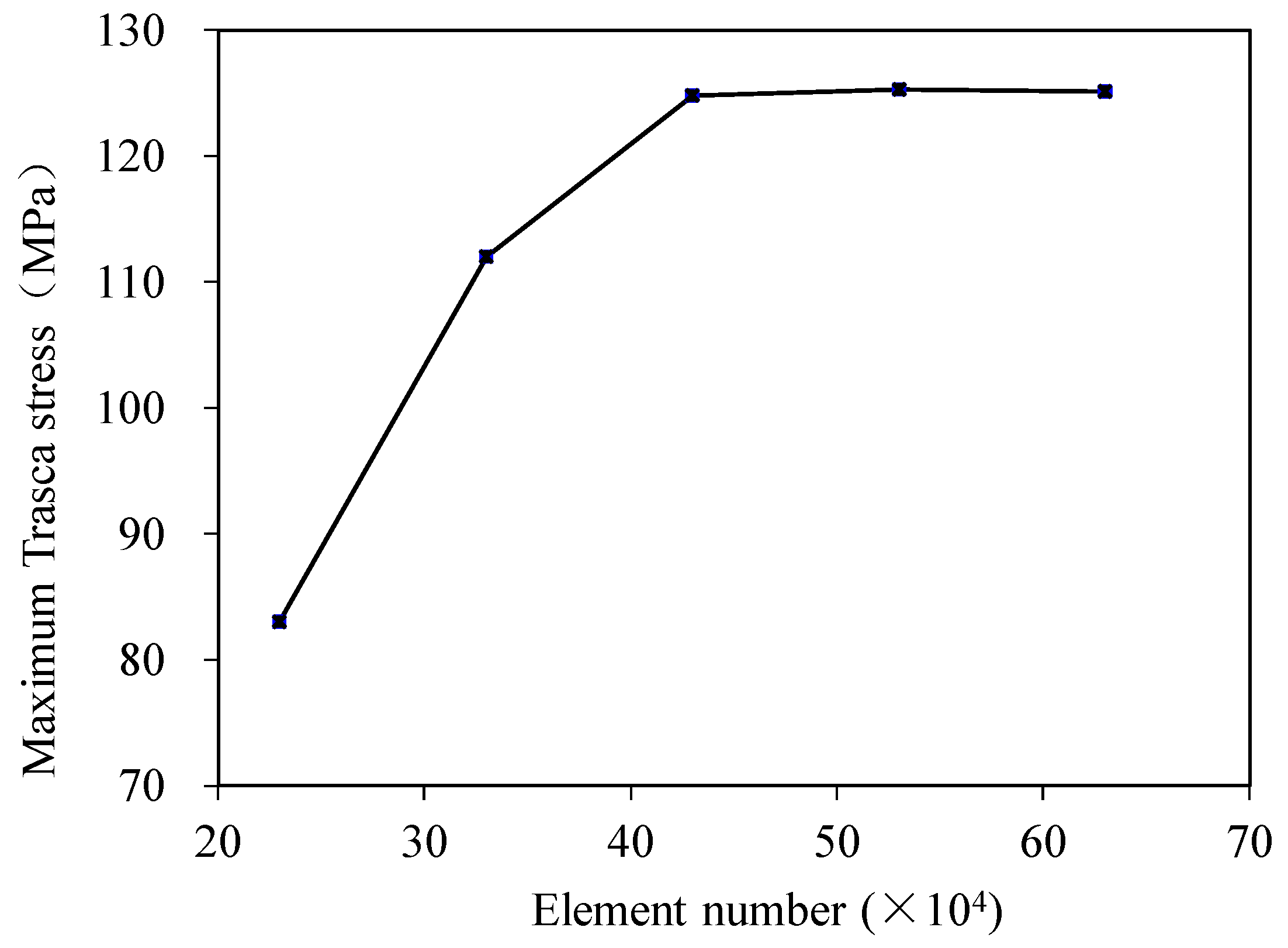
3.1.1. Grid Independence Verification
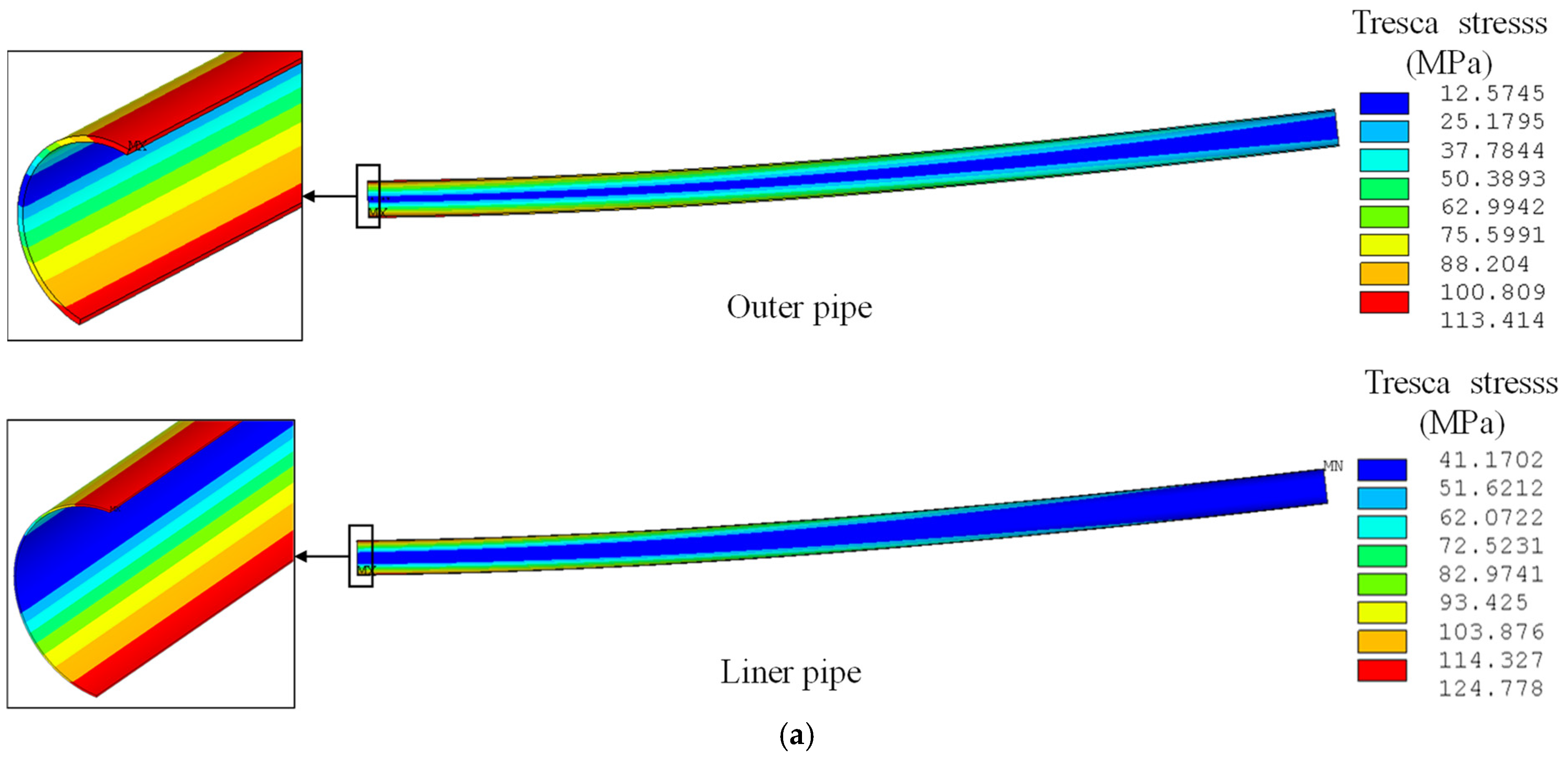
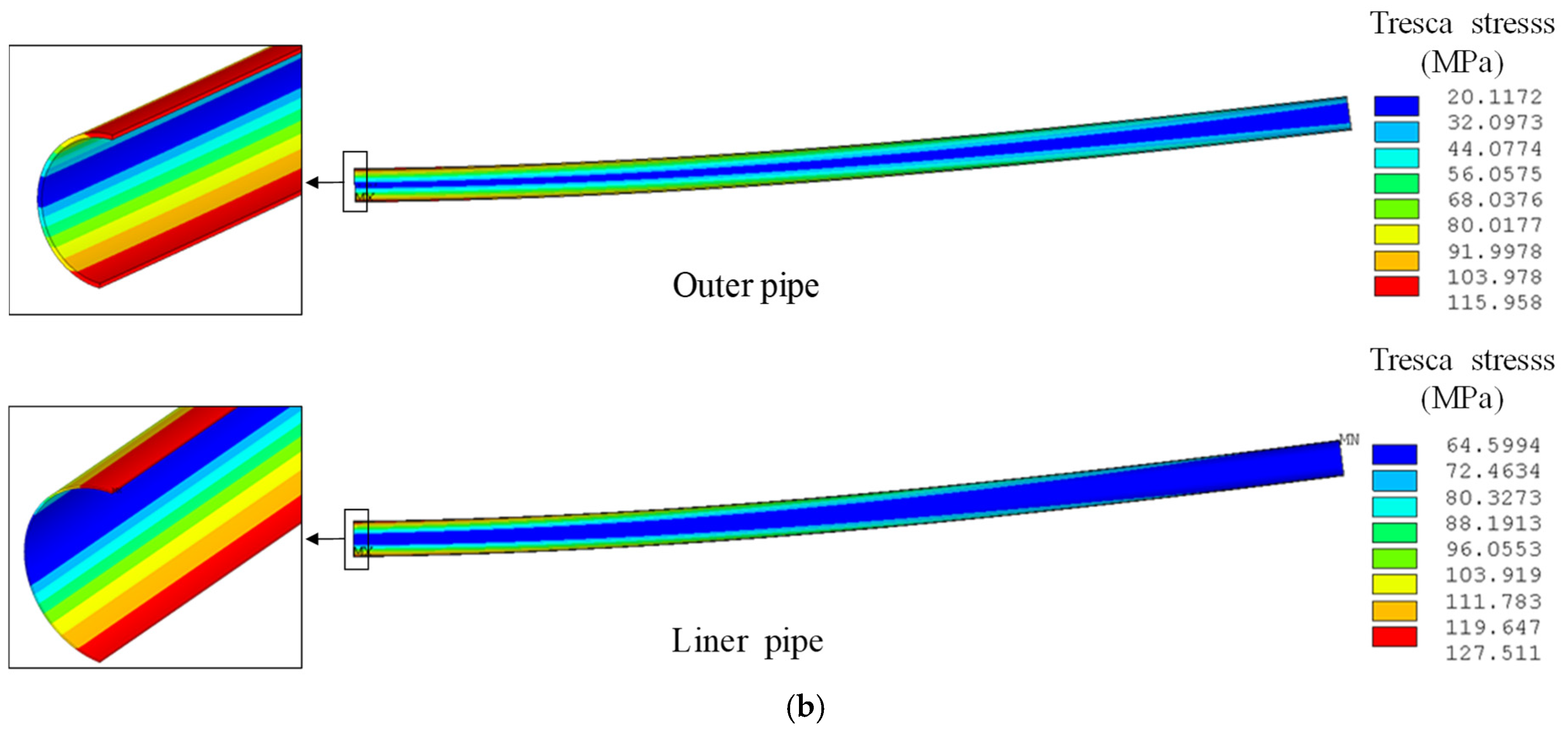
3.1.2. Variation in Temperature
3.1.3. Variation in Pressure
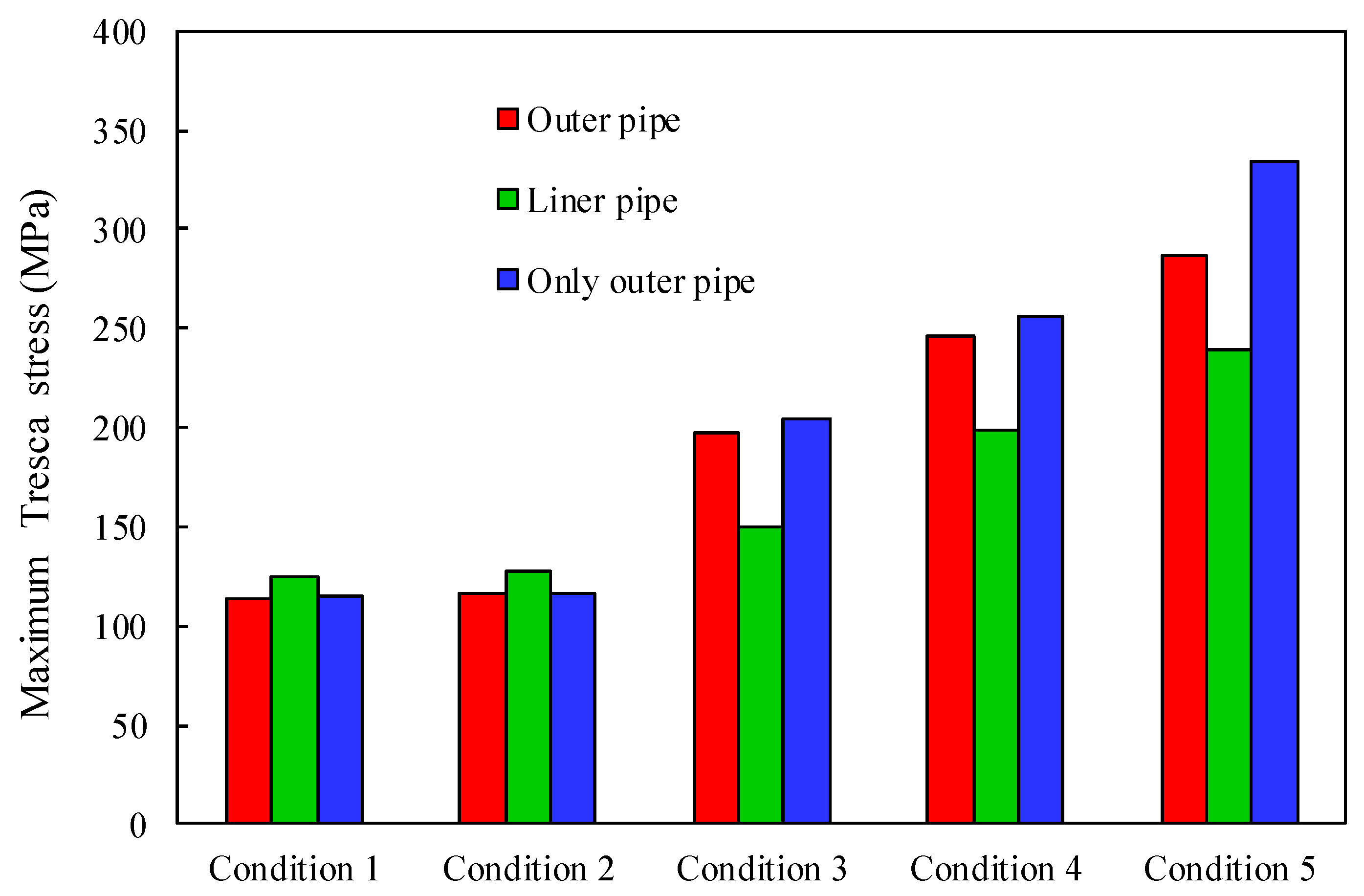
3.1.4. The Outer Pipe Model Error Analysis
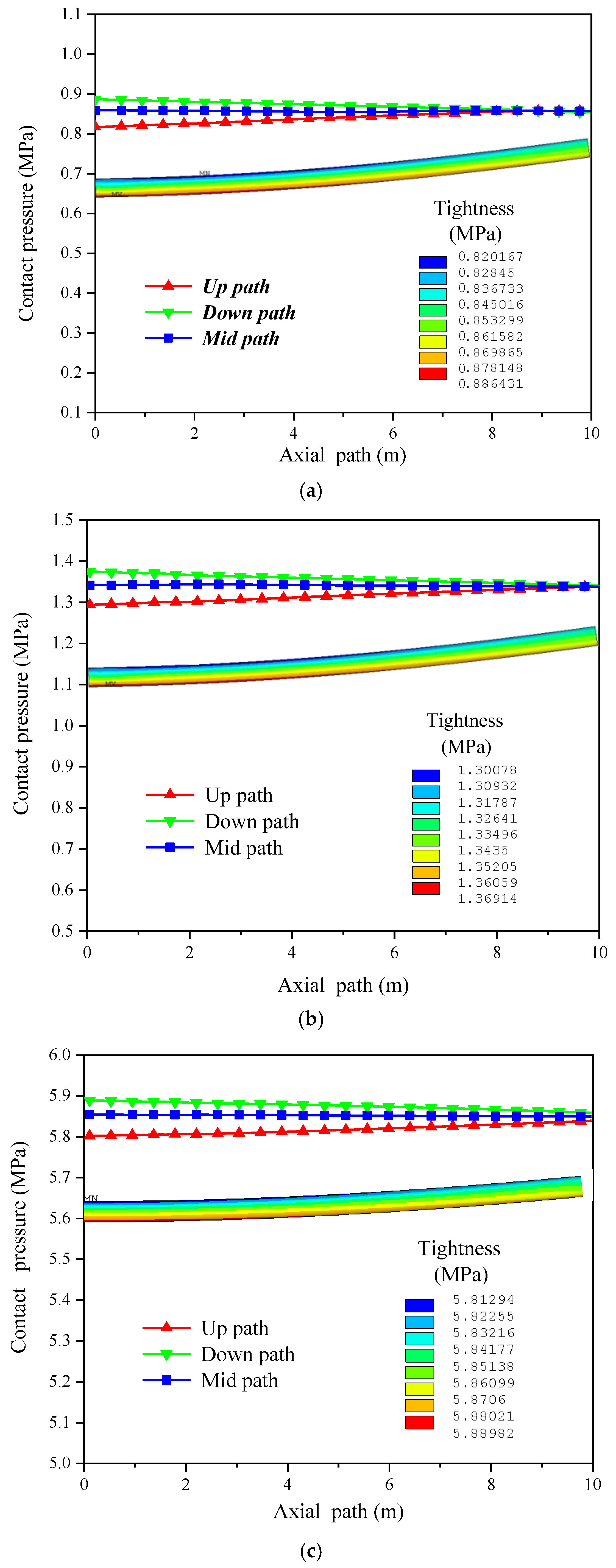
3.2. Tightness Analysis
4. Conclusions
- (1)
- Liner stress and yield strength: Under high-pressure conditions (14 MPa), the liner pipe’s stress levels approach its yield strength (241 MPa), indicating a potential risk of plastic deformation. This highlights the need for the careful design of laying radii to prevent liner failure under extreme operational conditions.
- (2)
- Bonding strength and internal pressure: Internal pressure significantly enhances the bonding strength between the liner and outer pipes, reducing the risk of delamination. The tightness between the two pipes increases with pressure, ensuring a stable interface under operational loads.
- (3)
- Limitations of simplified models: Simplified single-layer models, which ignore the liner’s mechanical contribution, underestimate the stress interactions between the liner and outer pipes. This can lead to design errors, particularly under high-pressure conditions, where the liner’s pressure-bearing effect becomes significant.
- (4)
- Design implications: This study emphasizes the importance of considering multi-field coupling effects in the design and installation of lined pipe systems. Designers should account for both the liner and outer pipe stresses under extreme conditions to ensure the pipeline’s long-term integrity.
- (5)
- Although this study based on numerical modeling provides a solid foundation for designing experimental setups, the results can guide the selection of test parameters, such as temperature, pressure, and bending radius, to ensure that the experiments are representative of real-world operational conditions.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, L.; Yu, T.; Xia, J.; Gao, Y.; Han, B.; Gao, Z. Failure analysis of L415/316L composite pipe welded joint. Eng. Fail. Anal. 2024, 158, 107981. [Google Scholar] [CrossRef]
- Gu, T.; Zhang, Q.; Lian, Z.; Yu, H.; Chen, J. Research and application of equivalent pipe model in stress analysis of lined pipe systems. Int. J. Press. Vessel. Pip. 2021, 192, 104418. [Google Scholar] [CrossRef]
- Ding, X.-D.; Wang, S.-Q.; Liu, W.-C.; Ye, X.-H. A comparative study on the post-buckling behavior of reinforced thermoplastic pipes (RTPs) under external pressure considering progressive failure. China Ocean Eng. 2024, 38, 233–246. [Google Scholar] [CrossRef]
- Yuan, L.; Li, Z.; Luo, Q.; Wang, C. Experimental and theoretical studies on the lateral collapse of mechanically lined pipes. Mar. Struct. 2023, 90, 103404. [Google Scholar] [CrossRef]
- Yuan, L.; Wang, C.; Luo, Q.; Chen, N. Collapse pressure prediction of mechanically lined pipes using FEM and machine learning techniques. Ocean Eng. 2023, 268, 113418. [Google Scholar] [CrossRef]
- Qu, Y.; Chen, N.-Z.; Yuan, L. Cohesive zone model based reliability analysis for a sandwich pipe. Ocean Eng. 2023, 270, 113550. [Google Scholar] [CrossRef]
- Yuan, L.; Zhou, J.; Yu, Z.; Xu, W. Numerical investigation of buckling behavior of dented lined pipe under bending. Int. J. Press. Vessel. Pip. 2023, 205, 104997. [Google Scholar] [CrossRef]
- GB 50251-2015; Code for Design of Gas Transmission Pipeline Engineering. Beijing China Planning Publishing House: Beijing, China, 2015.
- Gavriilidis, I.; Stamou, A.G.; Palagas, C.; Dourdounis, E.; Voudouris, N.; Tazedakis, A.; Karamanos, S.A. Heat treatment effects on collapse of JCO-E steel pipes under external pressure: Experiments and numerical predictions. Mar. Struct. 2024, 93, 103536. [Google Scholar] [CrossRef]
- Yuan, L.; Kyriakides, S. Liner buckling during reeling of lined pipe. Int. J. Solids Struct. 2020, 185–186, 1–13. [Google Scholar] [CrossRef]
- Yuan, L.; Kyriakides, S. Liner wrinkling and collapse of girth-welded bi-material pipe under bending. Appl. Ocean Res. 2015, 50, 209–216. [Google Scholar] [CrossRef]
- Yuan, L.; Kyriakides, S. Hydraulic expansion of lined pipe for offshore pipeline applications. Appl. Ocean Res. 2021, 108, 102523. [Google Scholar] [CrossRef]
- Gu, T.; Lian, Z.; Dou, Y.; Wu, Z.; Li, G. Simulation and experimental study on liner collapse of lined composite pipe. Int. J. Press. Vessel. Pip. 2024, 207, 105106. [Google Scholar] [CrossRef]
- Hilberink, A. Mechanical Behaviour of Lined Pipe; Delft University of Technology: Delft, The Netherlands, 2011. [Google Scholar]
- Wang, F.-C.; Li, W.; Han, L.-H. Interaction behavior between outer pipe and liner within offshore lined pipeline under axial compression. Ocean Eng. 2019, 175, 103–112. [Google Scholar] [CrossRef]
- Wang, X.; Li, P.; Wang, R. Study on hydro-forming technology of manufacturing bimetallic CRA-lined pipe. Int. J. Mach. Tools Manuf. 2005, 45, 373–378. [Google Scholar] [CrossRef]
- Focke, E.S.; Gresnigt, A.M.; Hilberink, A. Local Buckling of Tight Fit Liner Pipe. J. Press. Vessel Technol. 2011, 133, 011207. [Google Scholar] [CrossRef]
- Zheng, M.; Gao, H.; Teng, H.; Hu, J.; Tian, Z.; Zhao, Y. A Simplified Approach for the Hydro-Forming Process of Bi-Metallic Composite Pipe. Arch. Metall. Mater. 2017, 62, 879–883. [Google Scholar] [CrossRef][Green Version]
- Kalaki, A.; Eskandarzade, M.; Barghani, S.; Mohammadpour, M. Experimental and numerical evaluation of influencing parameters on the manufacturing of lined pipes. Int. J. Press. Vessel. Pip. 2019, 169, 71–76. [Google Scholar] [CrossRef]
- Guo, X.; Sun, W.; Becker, A.; Morris, A.; Pavier, M.; Flewitt, P.; Tierney, M.; Wales, C. Thermal and stress analyses of a novel coated steam dual pipe system for use in advanced ultra-supercritical power plant. Int. J. Press. Vessel. Pip. 2019, 176, 103933. [Google Scholar] [CrossRef]
- Balakrishnan, S.; Veerappan, A.R.; Shanmugam, S. B2 stress index for structurally distorted pipe bends under in-plane opening bending moment. Int. J. Press. Vessel. Pip. 2019, 176, 103957. [Google Scholar] [CrossRef]
- Phan, H.C.; Le, T.-T.; Bui, N.D.; Duong, H.T.; Pham, T.D. An empirical model for bending capacity of defected pipe combined with axial load. Int. J. Press. Vessel. Pip. 2021, 191, 104368. [Google Scholar] [CrossRef]




| Description | Outer Diameter (mm) | Wall Thickness (mm) | Yield Strength (MPa) | Ultimate Strength (MPa) | Poisson’s Ratio | Young Modulus (GPa) |
|---|---|---|---|---|---|---|
| Inner pipe | 303.8 | 3 | 241 | 586 | 0.3 | 196 |
| Outer pipe | 323.8 | 10 | 413 | 547 | 0.3 | 203 |
| Temperature /°C | Thermal Expansion Coefficient α/×10−6 (1/°C) | Elastic Modulus E/GPa | ||
|---|---|---|---|---|
| Outer (α1) | Liner (α2) | Outer (E1) | Liner (E2) | |
| −29 | 11.250 | 13.095 | 205 | 199 |
| 21 | 11.520 | 13.500 | 203 | 196 |
| 38 | 11.644 | 13.583 | 202 | 195 |
| 93 | 12.060 | 13.860 | 198 | 192 |
| Description | Temperature /°C | Pressure /MPa | Corresponding Operation Conditions |
|---|---|---|---|
| Condition 1 | 20 | 0 | Installation |
| Condition 2 | 80 | 0 | Stop operation and relieve pressure |
| Condition 3 | 80 | 6 | Low-pressure operation |
| Condition 4 | 80 | 10 | Mid-pressure operation |
| Condition 5 | 80 | 14 | Design condition |
| Description | Lined Pipe Model/(MPa) | Outer Pipe Model /(MPa) | Errors (%) | |
|---|---|---|---|---|
| Outer Pipe | Liner Pipe | |||
| Condition 1 | 113.41 | 124.77 | 114.51 | 0.97 |
| Condition 2 | 115.96 | 127.51 | 115.78 | −0.16 |
| Condition 3 | 196.97 | 149.16 | 204.60 | 3.87 |
| Condition 4 | 246.28 | 198.13 | 256.37 | 4.10 |
| Condition 5 | 286.51 | 238.81 | 334.17 | 16.63 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Zhang, W.; Gu, T.; Liu, J.; Peng, J.; Dou, Y. Mechanical Analysis of Lined Pipe System Under Temperature–Pressure Coupling in Elastic Laying. Processes 2025, 13, 691. https://doi.org/10.3390/pr13030691
Liu J, Zhang W, Gu T, Liu J, Peng J, Dou Y. Mechanical Analysis of Lined Pipe System Under Temperature–Pressure Coupling in Elastic Laying. Processes. 2025; 13(3):691. https://doi.org/10.3390/pr13030691
Chicago/Turabian StyleLiu, Junyan, Wei Zhang, Tianping Gu, Ju Liu, Jianxin Peng, and Yihua Dou. 2025. "Mechanical Analysis of Lined Pipe System Under Temperature–Pressure Coupling in Elastic Laying" Processes 13, no. 3: 691. https://doi.org/10.3390/pr13030691
APA StyleLiu, J., Zhang, W., Gu, T., Liu, J., Peng, J., & Dou, Y. (2025). Mechanical Analysis of Lined Pipe System Under Temperature–Pressure Coupling in Elastic Laying. Processes, 13(3), 691. https://doi.org/10.3390/pr13030691






