High-Accuracy Simulation of Polymer Flooding Based on Weighted Essentially Non-Oscillatory (WENO) Scheme
Abstract
1. Introduction
2. Methodology
2.1. Polymer-Flooding Model
2.2. WENO Calculation of Governing Equations
3. Numerical Examples
3.1. Example 1
3.2. Example 2
3.3. Example 3
4. Conclusions
- (1)
- The WENO method is employed to achieve high-order estimations of the saturation function and the polymer concentration function. Moreover, a sequential solution scheme for the hyperbolic mass conservation equation of the aqueous phase and the mass conservation equation of the polymer component in polymer-flooded reservoirs is derived.
- (2)
- Three numerical test cases are implemented by comparing the results obtained from the WENO scheme in this work and those from the traditional first-order upwind difference method commonly used in current commercial simulators. It is found that the computational accuracy of the WENO results is far higher than that of the traditional method, especially in the prediction of the water-flood front and the polymer concentration front. This can significantly improve the prediction accuracy of the water cut and polymer production concentration in production wells.
- (3)
- The results of the numerical test cases also show that, with the same level of accuracy, the number of grids required by the WENO method is far less than that required by the traditional method. Correspondingly, its computational efficiency is over multiple times that of the traditional method.
- (4)
- A sensitivity analysis of the parameters related to the viscosity of the polymer solution was conducted. The results indicate that the WENO method presented in this paper can accurately simulate the production performance of polymer-flooded reservoirs.
- (5)
- Integrating the high-precision simulation method for polymer flooding in one-dimensional channels in this paper into the INSIM model to conduct simulations of full three-dimensional reservoir blocks and comparing and validating the simulation results with experimental data to modify the model equations for polymer flooding are valuable future works.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Soeder, D.J. The successful development of gas and oil resources from shales in North America. J. Pet. Sci. Eng. 2018, 163, 399–420. [Google Scholar] [CrossRef]
- Seyyedattar, M.; Zendehboudi, S.; Butt, S. Technical and non-technical challenges of development of offshore petroleum reservoirs: Characterization and production. Nat. Resour. Res. 2020, 29, 2147–2189. [Google Scholar] [CrossRef]
- Seright, R.S.; Wang, D. Polymer flooding: Current status and future directions. Pet. Sci. 2023, 20, 910–921. [Google Scholar] [CrossRef]
- Hassan, A.M.; Al-Shalabi, E.W.; Alameri, W.; Kamal, M.S.; Patil, S.; Hussain, S.M.S. Manifestations of surfactant-polymer flooding for successful field applications in carbonates under harsh conditions: A comprehensive review. J. Pet. Sci. Eng. 2023, 220, 111243. [Google Scholar] [CrossRef]
- Pogaku, R.; Mohd Fuat, N.H.; Sakar, S.; Cha, Z.W.; Musa, N.; Awang Tajudin, D.N.A.; Morris, L.O. Polymer flooding and its combinations with other chemical injection methods in enhanced oil recovery. Polym. Bull. 2018, 75, 1753–1774. [Google Scholar] [CrossRef]
- Saboorian-Jooybari, H.; Dejam, M.; Chen, Z. Heavy oil polymer flooding from laboratory core floods to pilot tests and field applications: Half-century studies. J. Pet. Sci. Eng. 2016, 142, 85–100. [Google Scholar] [CrossRef]
- Ibiam, E.; Geiger, S.; Demyanov, V.; Arnold, D. Optimization of polymer flooding in a heterogeneous reservoir considering geological and history matching uncertainties. SPE Reserv. Eval. Eng. 2021, 24, 19–36. [Google Scholar] [CrossRef]
- Alhotan, M.M.; Batista Fernandes, B.R.; Delshad, M.; Sepehrnoori, K. A Systemic Comparison of Physical Models for Simulating Surfactant–Polymer Flooding. Energies 2023, 16, 5702. [Google Scholar] [CrossRef]
- Ferrari, S.; Rossi, R.; Di Bernardino, A. A review of laboratory and numerical techniques to simulate turbulent flows. Energies 2022, 15, 7580. [Google Scholar] [CrossRef]
- Urrea-Quintero, J.H.; Marino, M.; Wick, T.; Nackenhorst, U. A comparative analysis of transient finite-strain coupled diffusion-deformation theories for hydrogels. Arch. Comput. Methods Eng. 2024, 31, 3767–3800. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, Z.; Wu, Y. Advection–Diffusion lattice Boltzmann method with and without dynamical filter. Front. Phys. 2022, 10, 875628. [Google Scholar] [CrossRef]
- Alves, M.A.; Oliveira, P.J.; Pinho, F.T. Numerical methods for viscoelastic fluid flows. Annu. Rev. Fluid Mech. 2021, 53, 509–541. [Google Scholar] [CrossRef]
- Battiston, F.; Amico, E.; Barrat, A.; Bianconi, G.; Ferraz de Arruda, G.; Franceschiello, B.; Iacopini, I.; Kéfi, S.; Latora, V.; Moreno, Y.; et al. The physics of higher-order interactions in complex systems. Nat. Phys. 2021, 17, 1093–1098. [Google Scholar] [CrossRef]
- Du, J.; Shu, C.W.; Zhong, X. An improved simple WENO limiter for discontinuous Galerkin methods solving hyperbolic systems on unstructured meshes. J. Comput. Phys. 2022, 467, 111424. [Google Scholar] [CrossRef]
- Wang, Z.; Zhu, J.; Tian, L.; Yang, Y.; Zhao, N. An efficient fifth-order finite difference multi-resolution WENO scheme for inviscid and viscous flow problems. Comput. Fluids 2021, 230, 105138. [Google Scholar] [CrossRef]
- Rao, X. The First Application of Quantum Computing Algorithm in Streamline-Based Simulation of Water-Flooding Reservoirs. In Proceedings of the Abu Dhabi International Petroleum Exhibition and Conference, Abu Dhabi, United Arab Emirates, 4–7 November 2024; SPE: Richardson, TX, USA, 2024; p. D011S003R006. [Google Scholar]
- Yee, H.C.; Sweby, P.K.; Sjögreen, B.; Kotov, D.V. A nonlinear approach in the quantification of numerical uncertainty by high-order methods for compressible turbulence with shocks. Fluids 2024, 9, 250. [Google Scholar] [CrossRef]
- Lin, G.; Liu, J.; Sadre-Marandi, F. A comparative study on the weak Galerkin, discontinuous Galerkin, and mixed finite element methods. J. Comput. Appl. Math. 2015, 273, 346–362. [Google Scholar] [CrossRef]
- Ali, I.; Saleem, M.T.; Din, A.U. Special functions and its application in solving two dimensional hyperbolic partial differential equation of telegraph type. Symmetry 2023, 15, 847. [Google Scholar] [CrossRef]
- Shen, H. A class of ENO schemes with adaptive order for solving hyperbolic conservation laws. Comput. Fluids 2023, 266, 106050. [Google Scholar] [CrossRef]
- Ye, C.C.; Wan, Z.H.; Sun, D.J. An alternative formulation of targeted ENO scheme for hyperbolic conservation laws. Comput. Fluids 2022, 238, 105368. [Google Scholar] [CrossRef]
- Rao, X.; He, X.; Kwak, H.; Hoteit, H. A Hybrid Method Combining Mimetic Finite Difference and Discontinuous Galerkin for Two-Phase Reservoir Flow Problems. Int. J. Numer. Methods Fluids 2024. [Google Scholar] [CrossRef]
- Luna, P.; Hidalgo, A. Numerical approach of a coupled pressure-saturation model describing oil-water flow in porous media. Commun. Appl. Math. Comput. 2023, 5, 946–964. [Google Scholar] [CrossRef]
- Garcia, R.D.O.; Silveira, G.P. Numerical Solutions of the Classical and Modified Buckley-Leverett Equations Applied to Two-Phase Fluid Flow. Open J. Fluid Dyn. 2024, 14, 184–204. [Google Scholar] [CrossRef]
- Liu, Y.; Yao, C. Development of an efficient numerical model for two-phase flows in air-lift pumps and its application to deep-sea mining. Ocean Eng. 2023, 281, 114897. [Google Scholar] [CrossRef]
- Zhao, H.; Kang, Z.; Zhang, X.; Sun, H.; Cao, L.; Reynolds, A.C. INSIM: A data-driven model for history matching and prediction for waterflooding monitoring and management with a field application. In Proceedings of the SPE Reservoir Simulation Conference, Houston, TX, USA, 23–25 February 2015; SPE: Richardson, TX, USA, 2015; p. D021S007R004. [Google Scholar]
- Zhao, H.; Rao, X.; Liu, D.; Xu, Y.; Zhan, W.; Peng, X. A flownet-based method for history matching and production prediction of shale or tight reservoirs with fracturing treatment. SPE J. 2022, 27, 2793–2819. [Google Scholar] [CrossRef]
- Zhong, H.; Liu, H.; Cui, T.; Chen, Z.; Shen, L.; Yang, B.; He, R.; Guo, X. Numerical simulations of polymer flooding process in porous media on distributed-memory parallel computers. J. Comput. Phys. 2020, 400, 108995. [Google Scholar] [CrossRef]
- Firozjaii, A.M.; Saghafi, H.R. Review on chemical enhanced oil recovery using polymer flooding: Fundamentals, experimental and numerical simulation. Petroleum 2020, 6, 115–122. [Google Scholar] [CrossRef]
- Jiang, G.S.; Shu, C.W. Efficient implementation of weighted ENO schemes. J. Comput. Phys. 1996, 126, 202–228. [Google Scholar] [CrossRef]
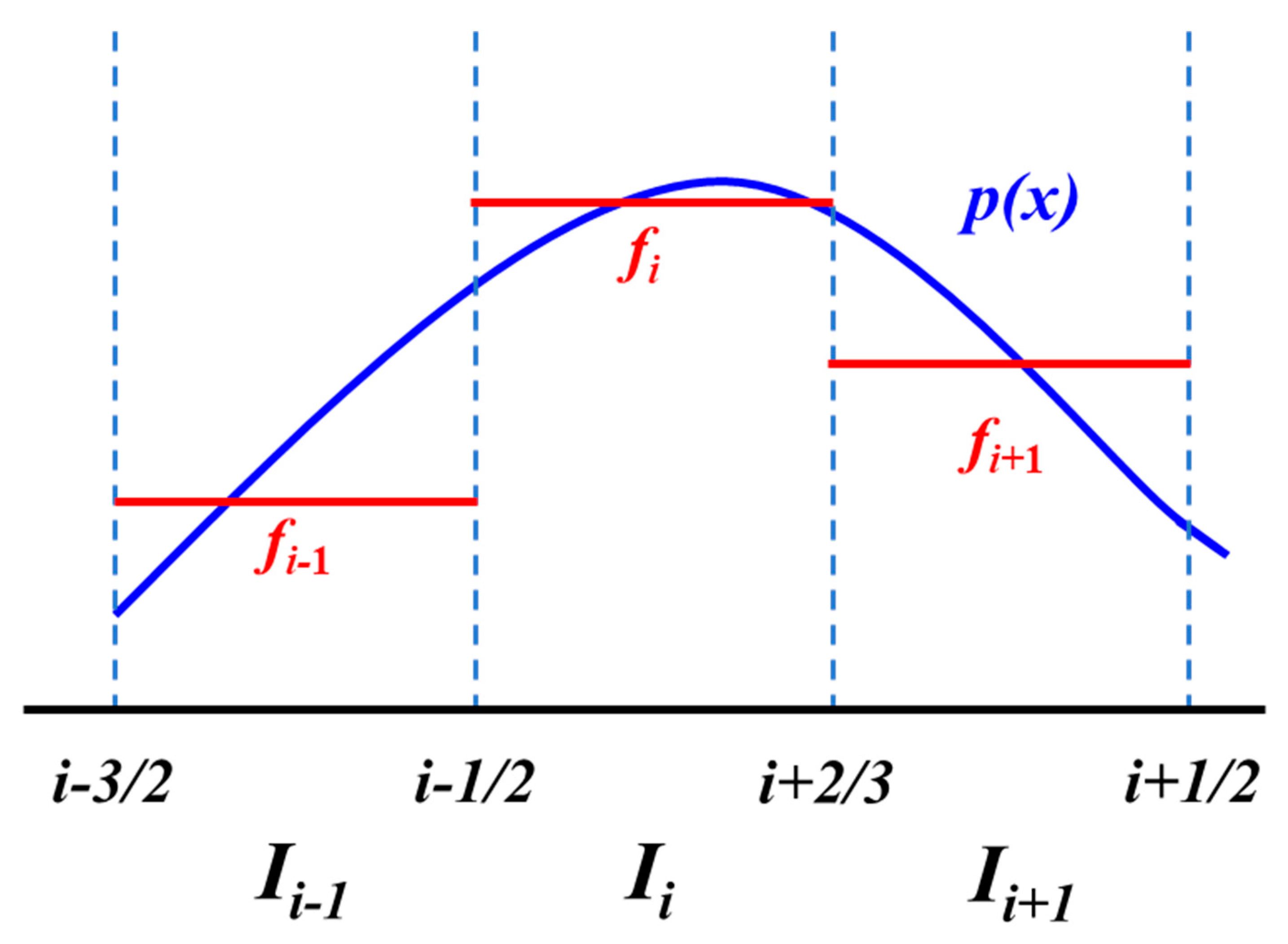

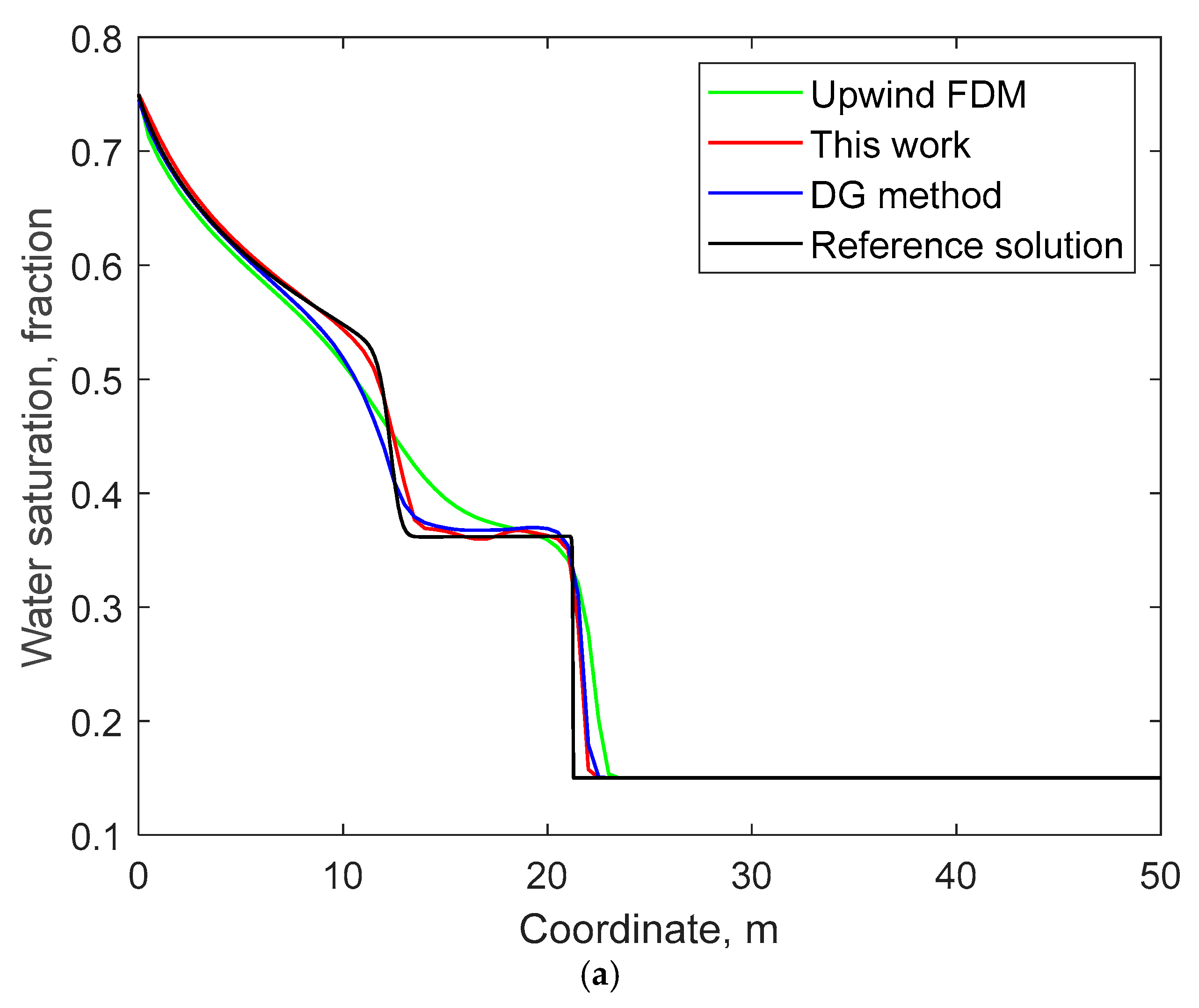
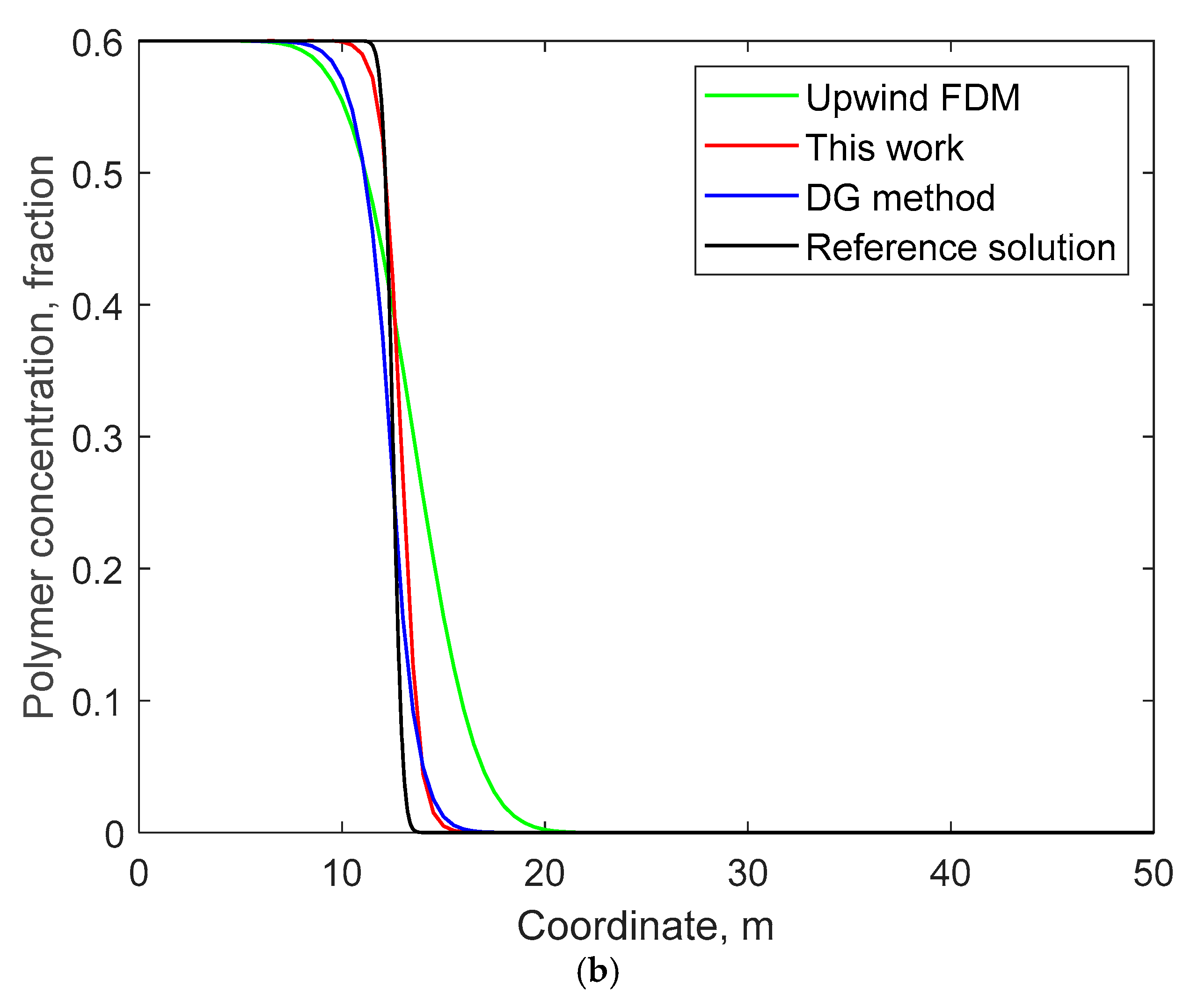
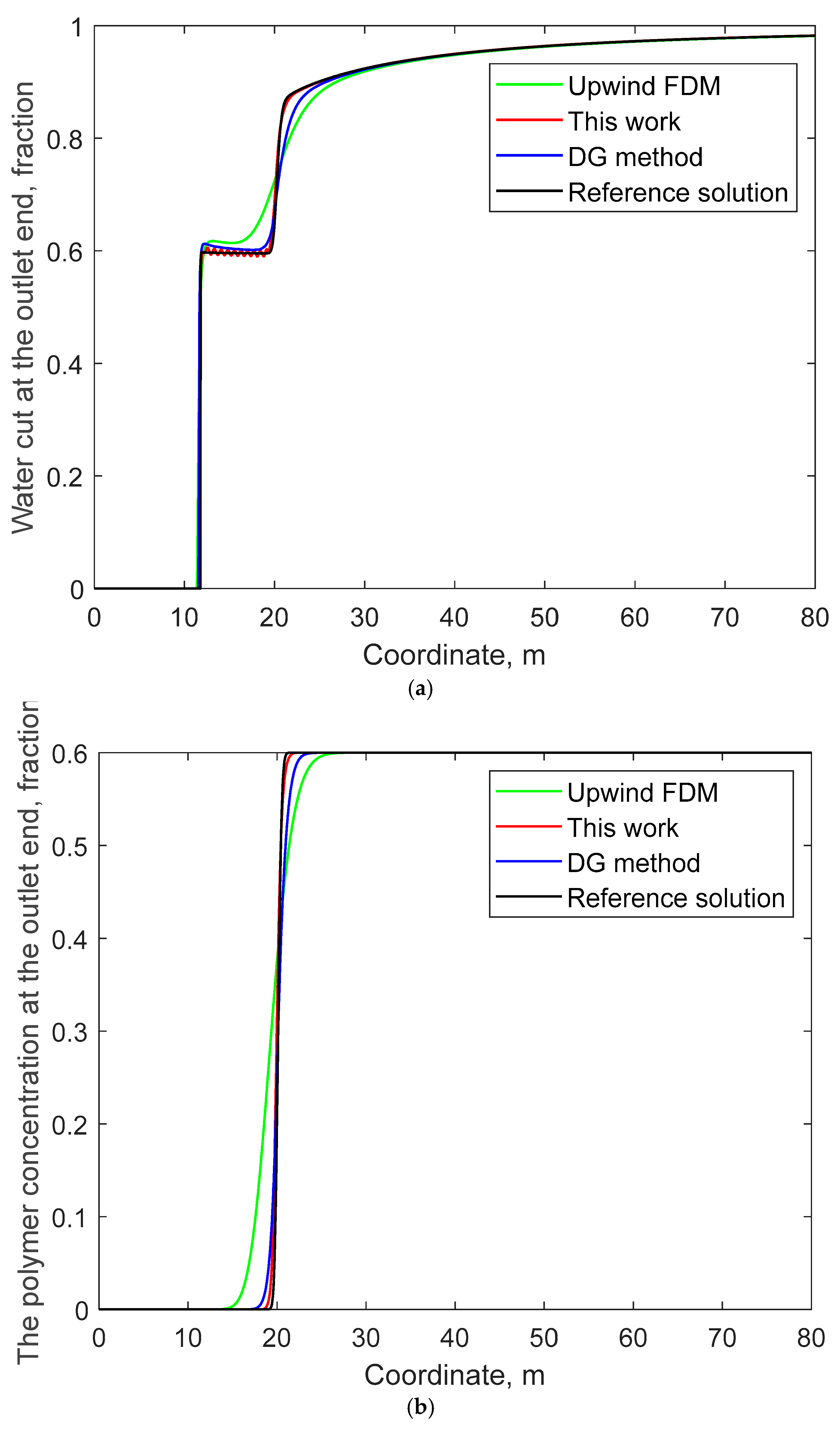


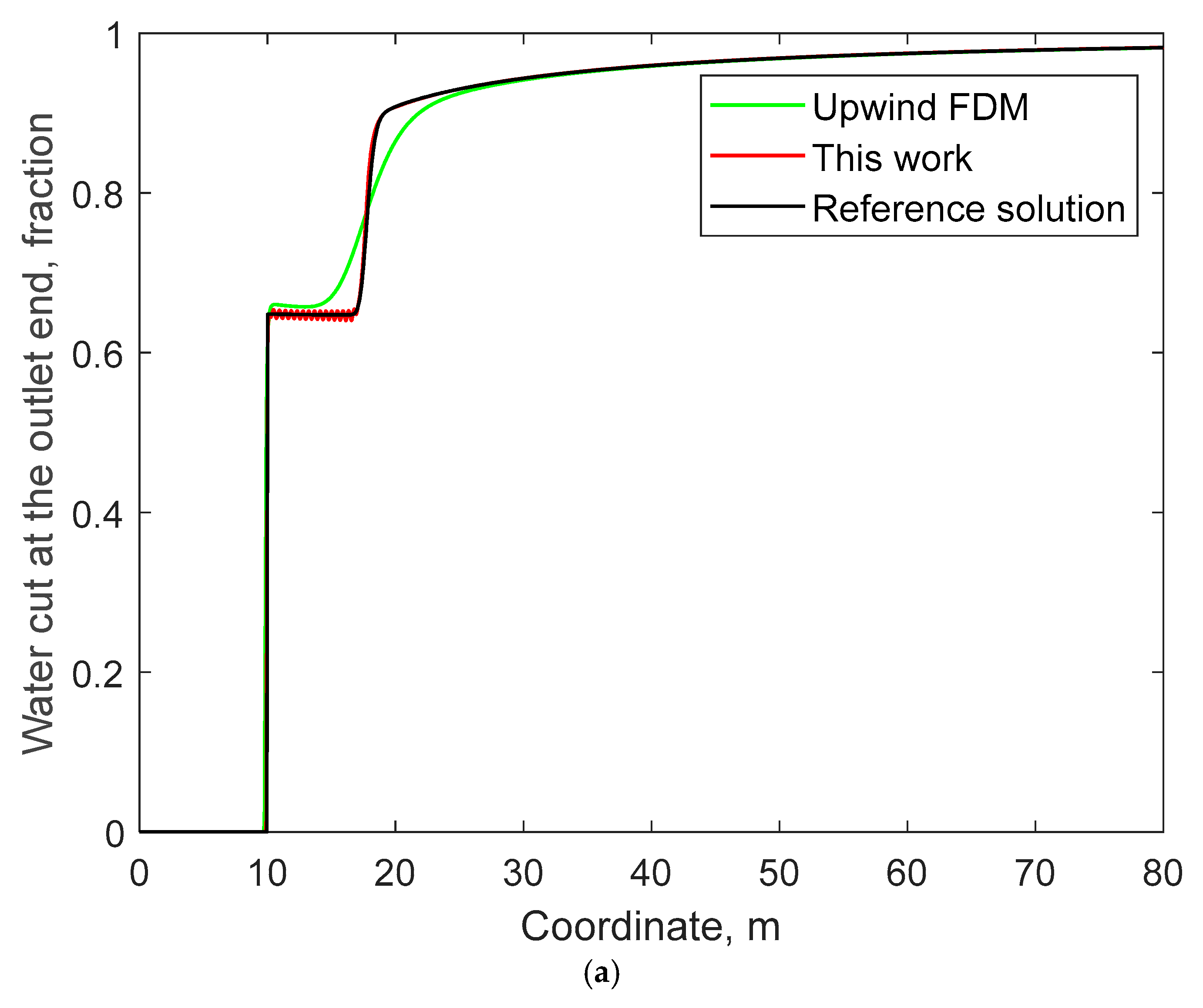
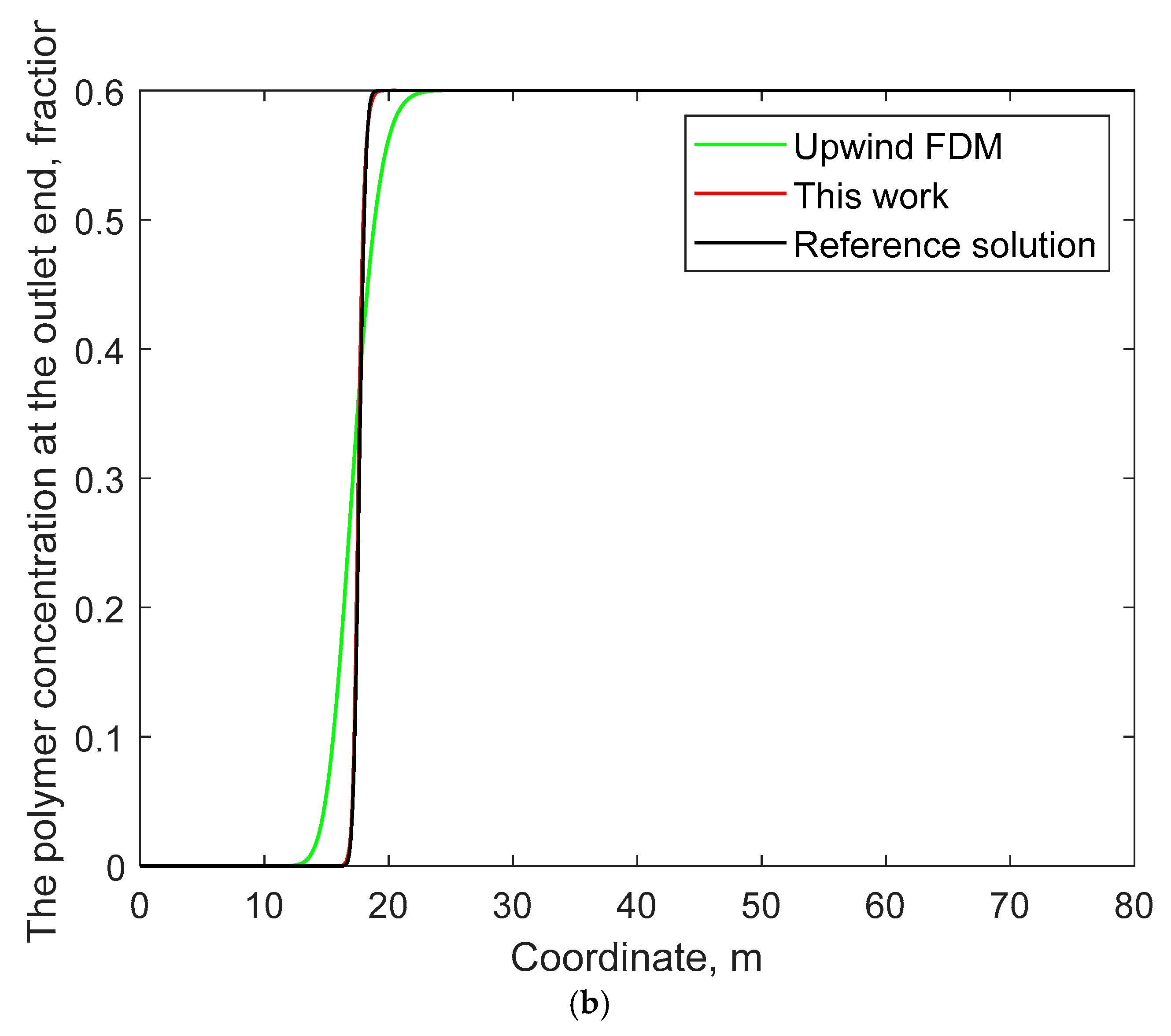
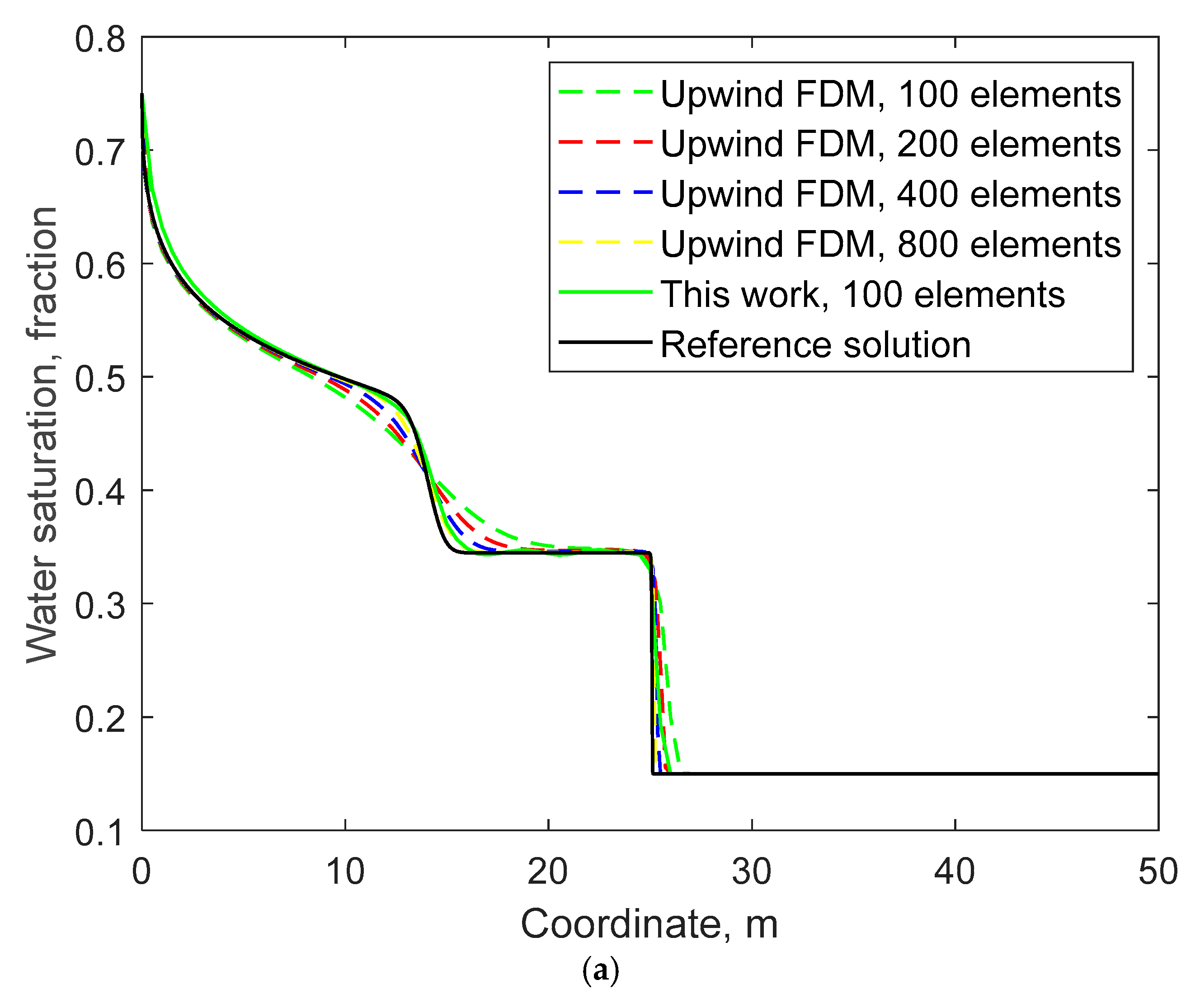
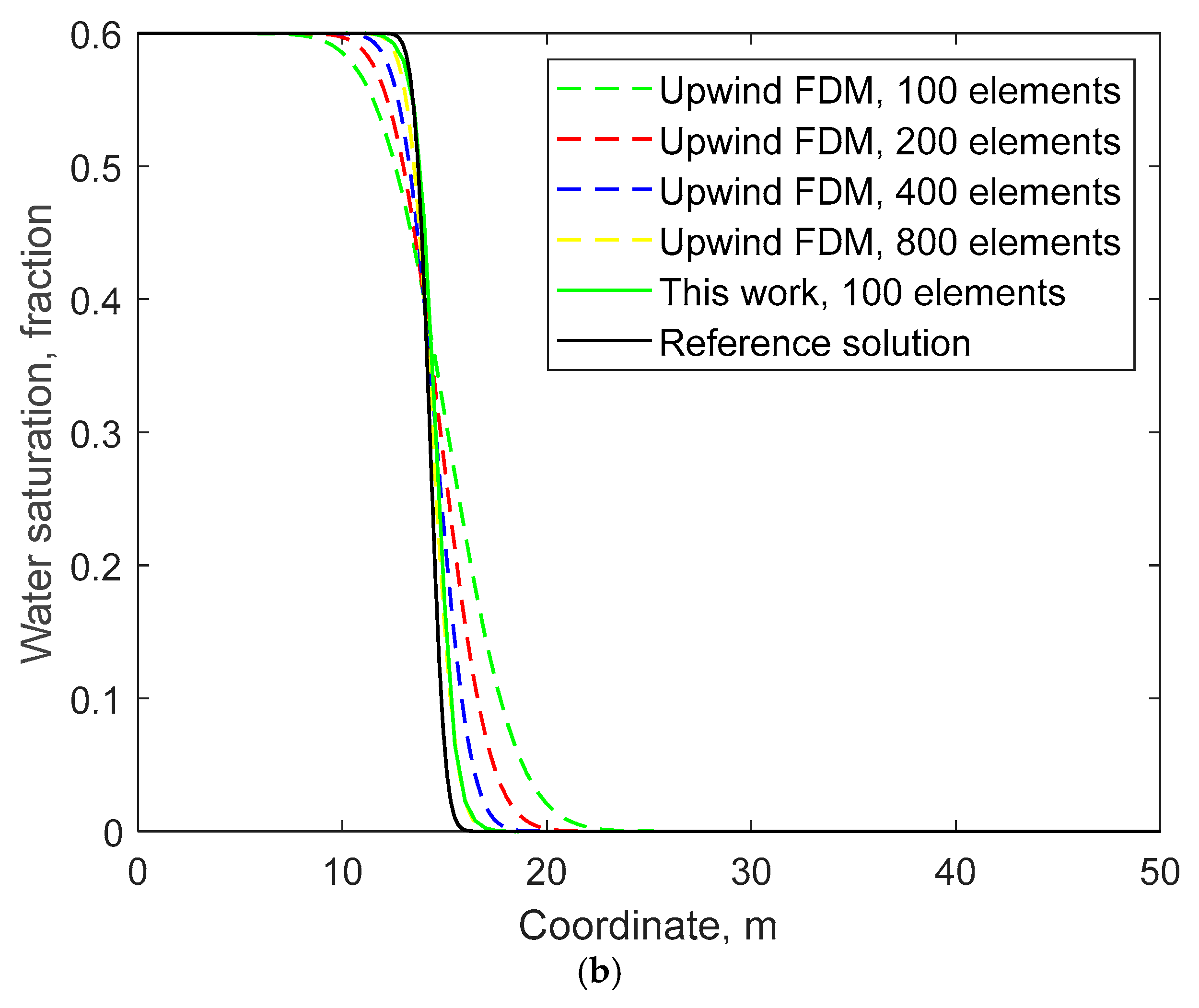

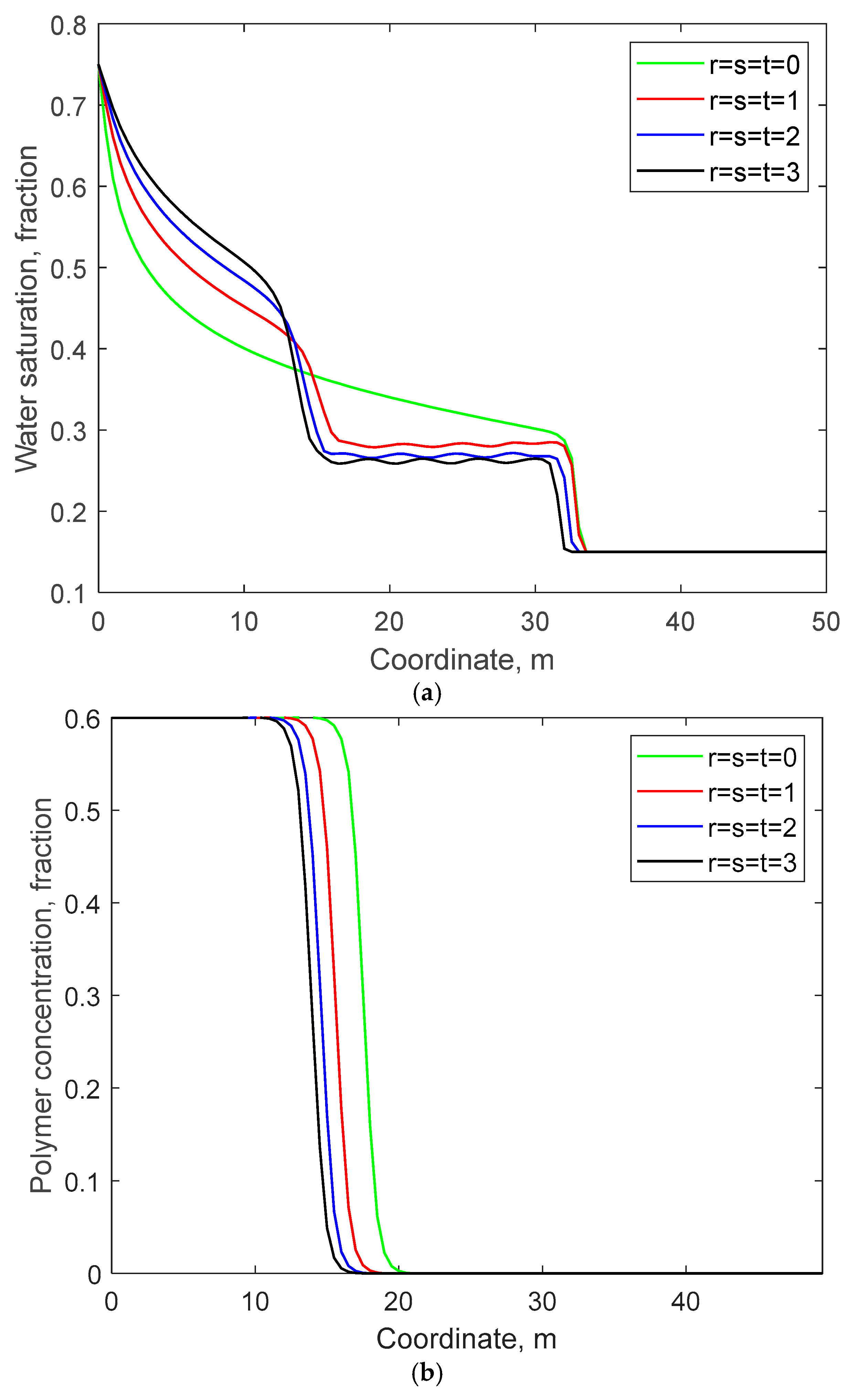
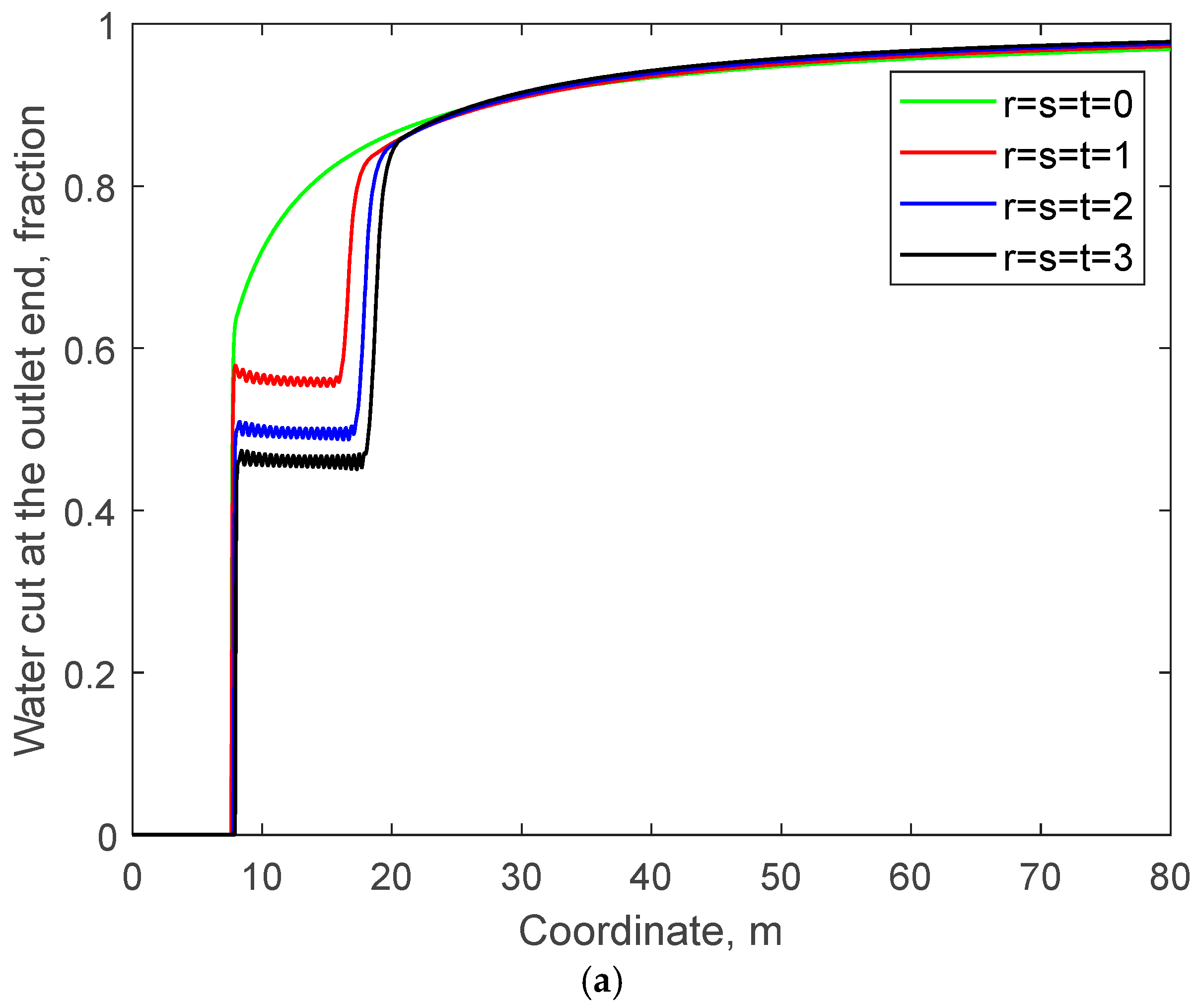
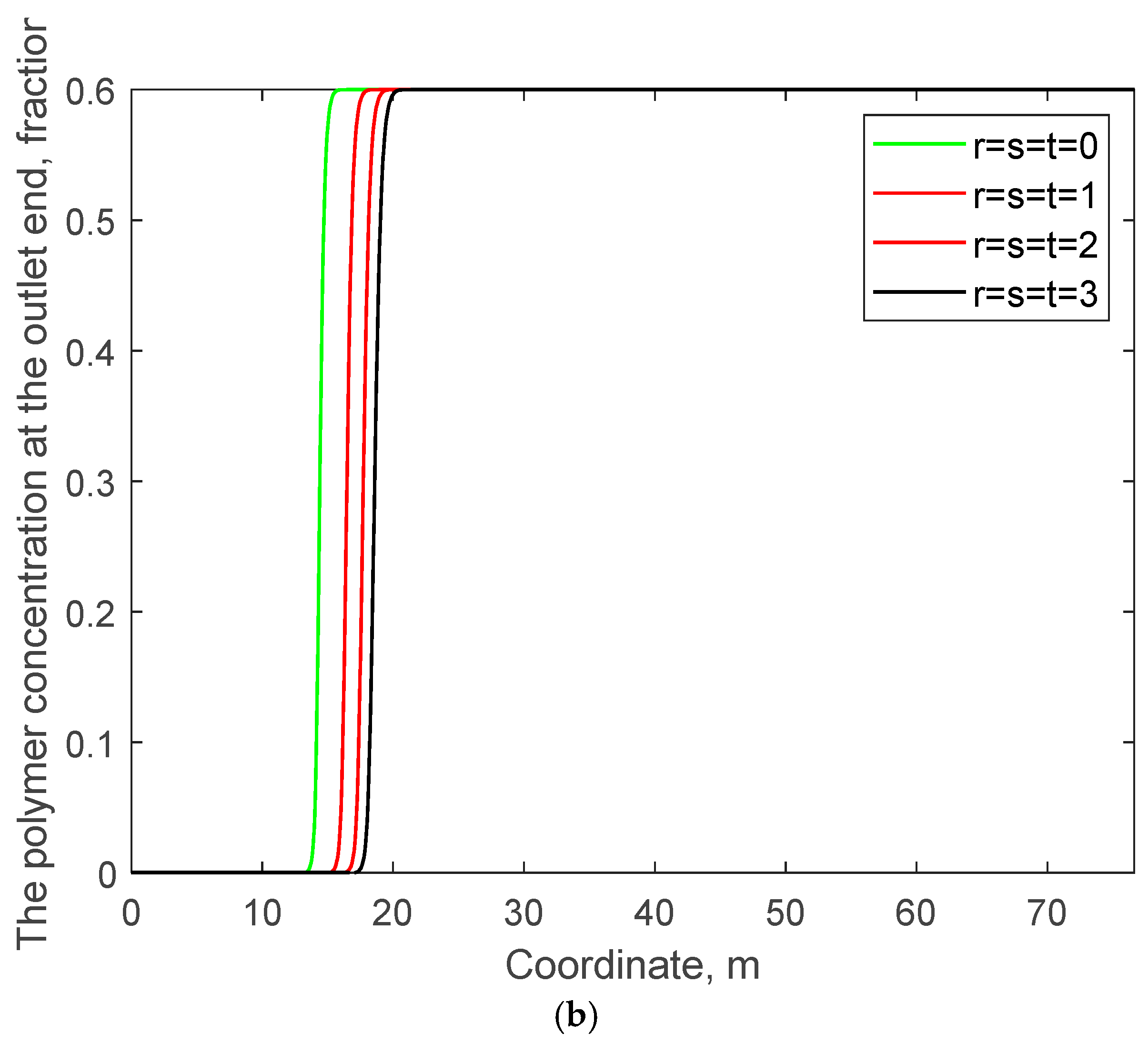
| Parameter | Value |
|---|---|
| Simulation duration, day | 10 |
| One-dimensional channel length, m | 50 |
| Grid length, m | 0.5 |
| Time step size, day | 0.001 |
| Channel flow rate, m3/day | 30 |
| Channel cross-sectional area, m2 | 100 |
| Upwind FDM | This Work | DG Method | Reference Solution | |
|---|---|---|---|---|
| Water | 11.318 days | 11.600 days | 11.556 days | 11.7832 days |
| polymer | 13.450 days | 18.427 days | 16.846 days | 19.055 days |
| Methods | This Work, 100 Elements | Upwind FDM, 100 Elements | Upwind FDM, 200 Elements | Upwind FDM, 400 Elements | Upwind FDM, 800 Elements |
|---|---|---|---|---|---|
| L2 error, Sw | 0.00727 | 0.02101 | 0.01724 | 0.01384 | 0.01061 |
| L2 error, Cp | 0.02512 | 0.06251 | 0.04758 | 0.03427 | 0.02220 |
| Methods | This Work, 100 Elements | Upwind FDM, 100 Elements | Upwind FDM, 200 Elements | Upwind FDM, 400 Elements | Upwind FDM, 800 Elements |
|---|---|---|---|---|---|
| CPU time, second | 5.6830 | 3.8906 | 7.1562 | 17.3125 | 36.8281 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, J.; Zhang, Z.; Zhang, X.; Rao, X. High-Accuracy Simulation of Polymer Flooding Based on Weighted Essentially Non-Oscillatory (WENO) Scheme. Processes 2025, 13, 671. https://doi.org/10.3390/pr13030671
Wei J, Zhang Z, Zhang X, Rao X. High-Accuracy Simulation of Polymer Flooding Based on Weighted Essentially Non-Oscillatory (WENO) Scheme. Processes. 2025; 13(3):671. https://doi.org/10.3390/pr13030671
Chicago/Turabian StyleWei, Jun, Zhijun Zhang, Xinlong Zhang, and Xiang Rao. 2025. "High-Accuracy Simulation of Polymer Flooding Based on Weighted Essentially Non-Oscillatory (WENO) Scheme" Processes 13, no. 3: 671. https://doi.org/10.3390/pr13030671
APA StyleWei, J., Zhang, Z., Zhang, X., & Rao, X. (2025). High-Accuracy Simulation of Polymer Flooding Based on Weighted Essentially Non-Oscillatory (WENO) Scheme. Processes, 13(3), 671. https://doi.org/10.3390/pr13030671





