Extended Finite Element Method for Analyzing Hydraulic Fracturing of Rock Cracks Under Compression
Abstract
1. Introduction
2. The Hydraulic Fracturing Model and Contact Model of the Extended Finite Element Method
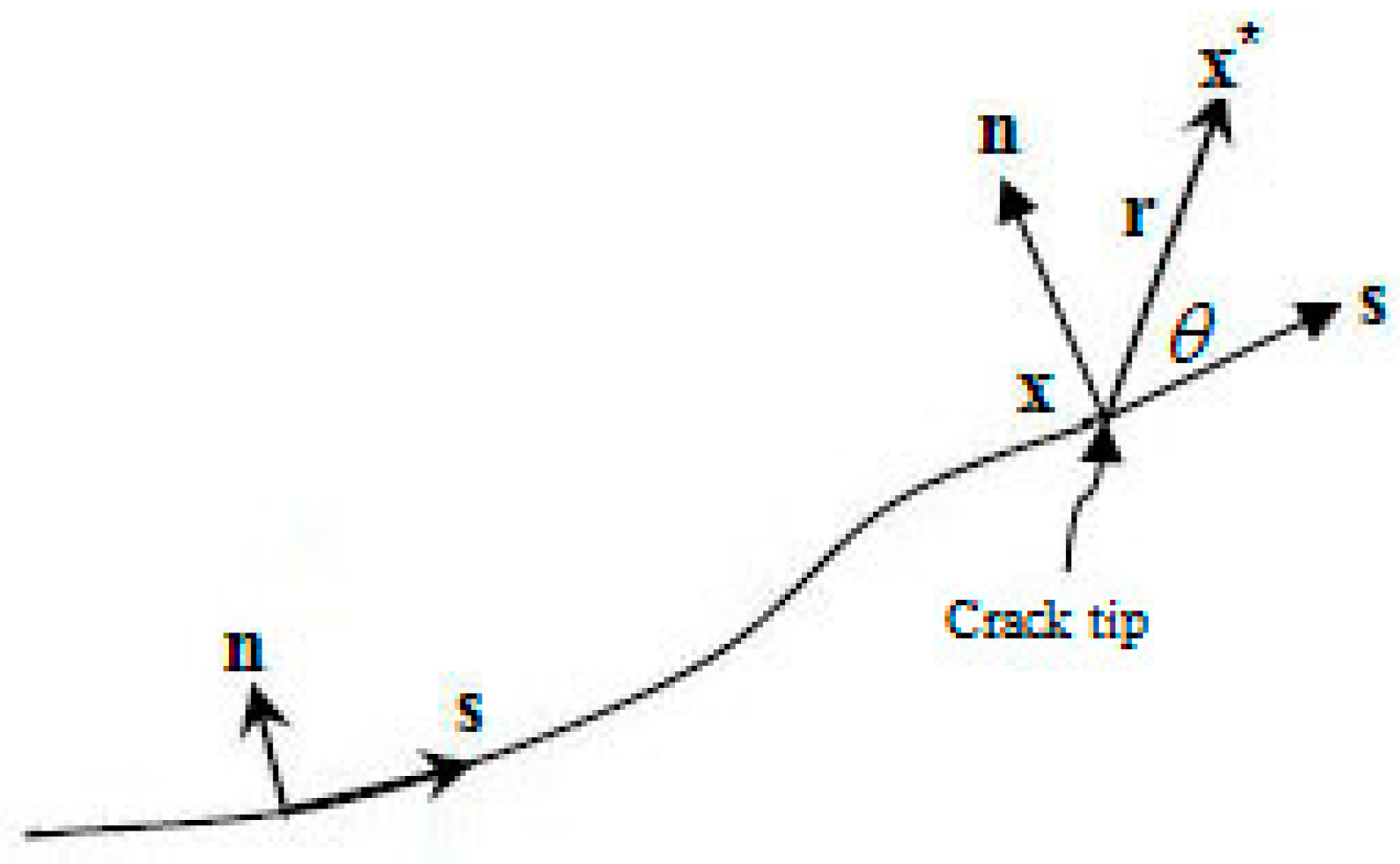
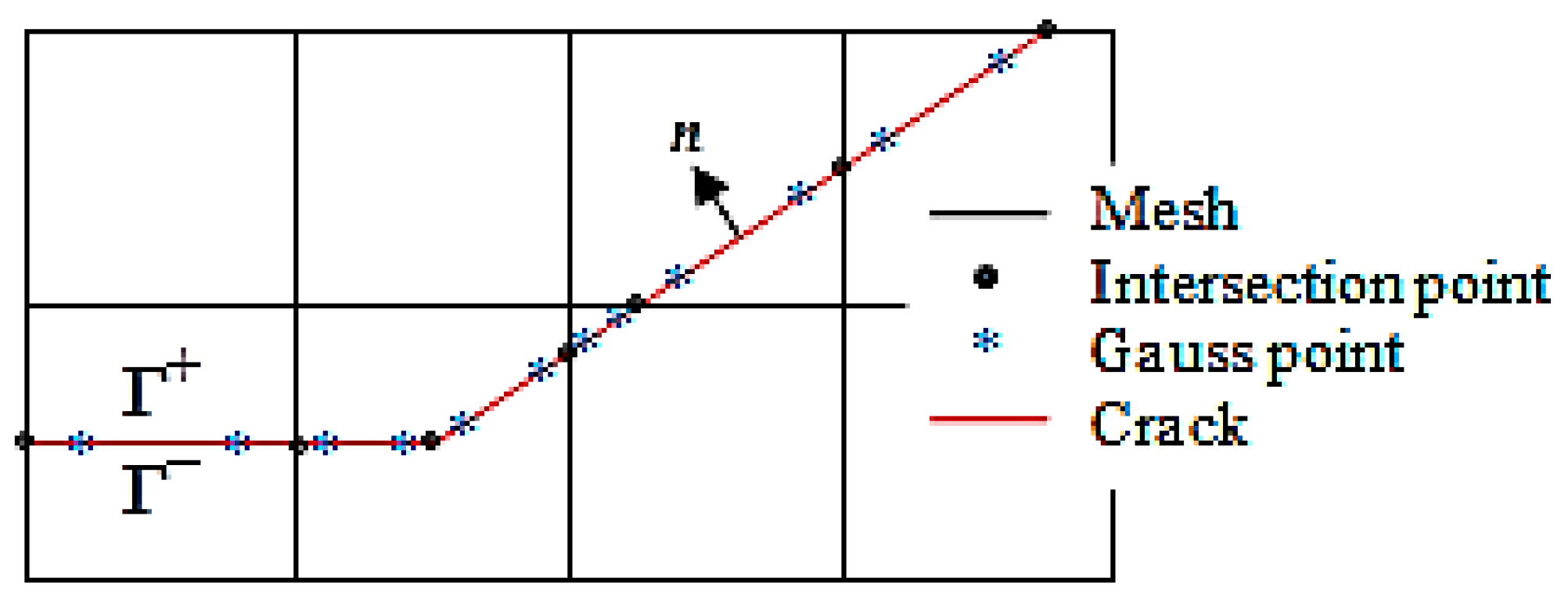
2.1. The Approximate Displacement Function of the Extended Finite Element Method
2.2. Discrete Equations for Hydraulic Fracturing
2.3. Contact Model
3. Stress Intensity Factor Calculation and Crack Propagation Criterion
3.1. Calculation of Stress Intensity Factor
3.2. Crack Propagation Criterion
4. Numerical Examples
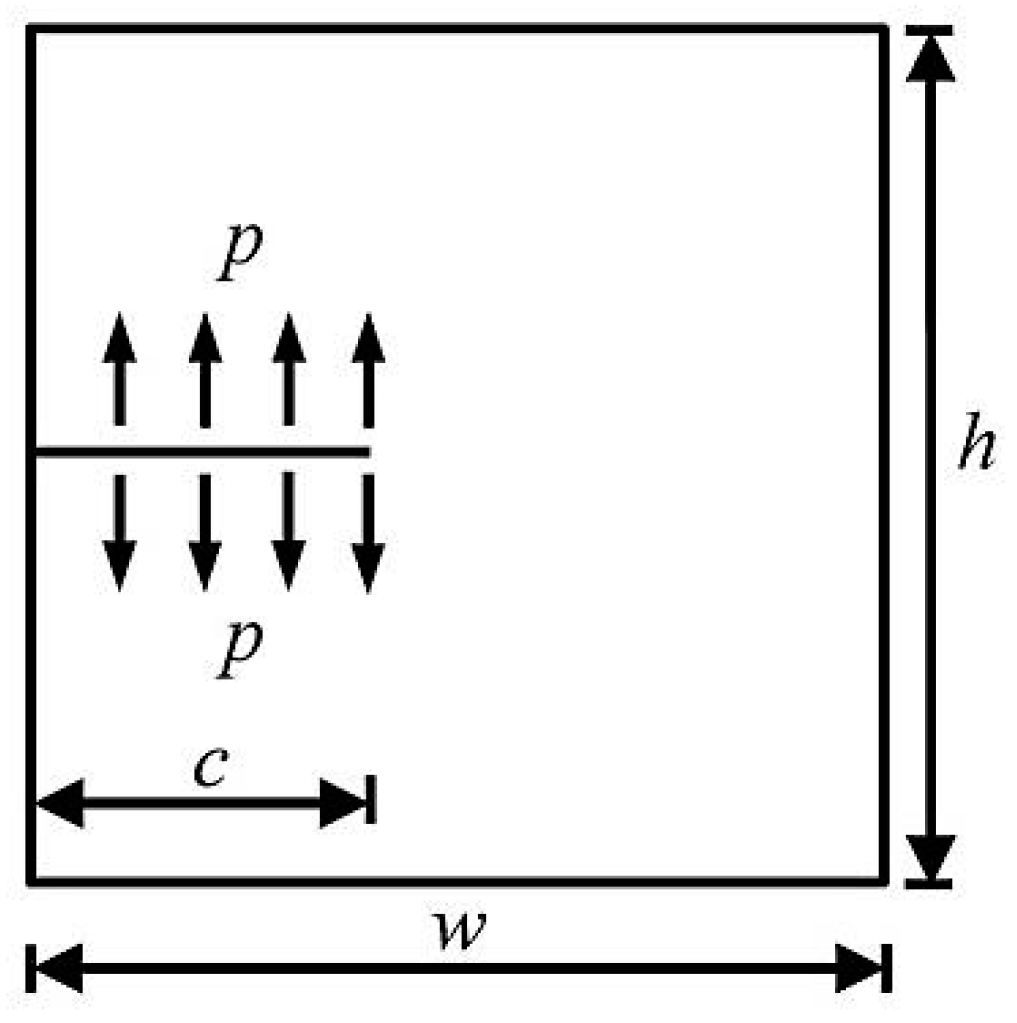
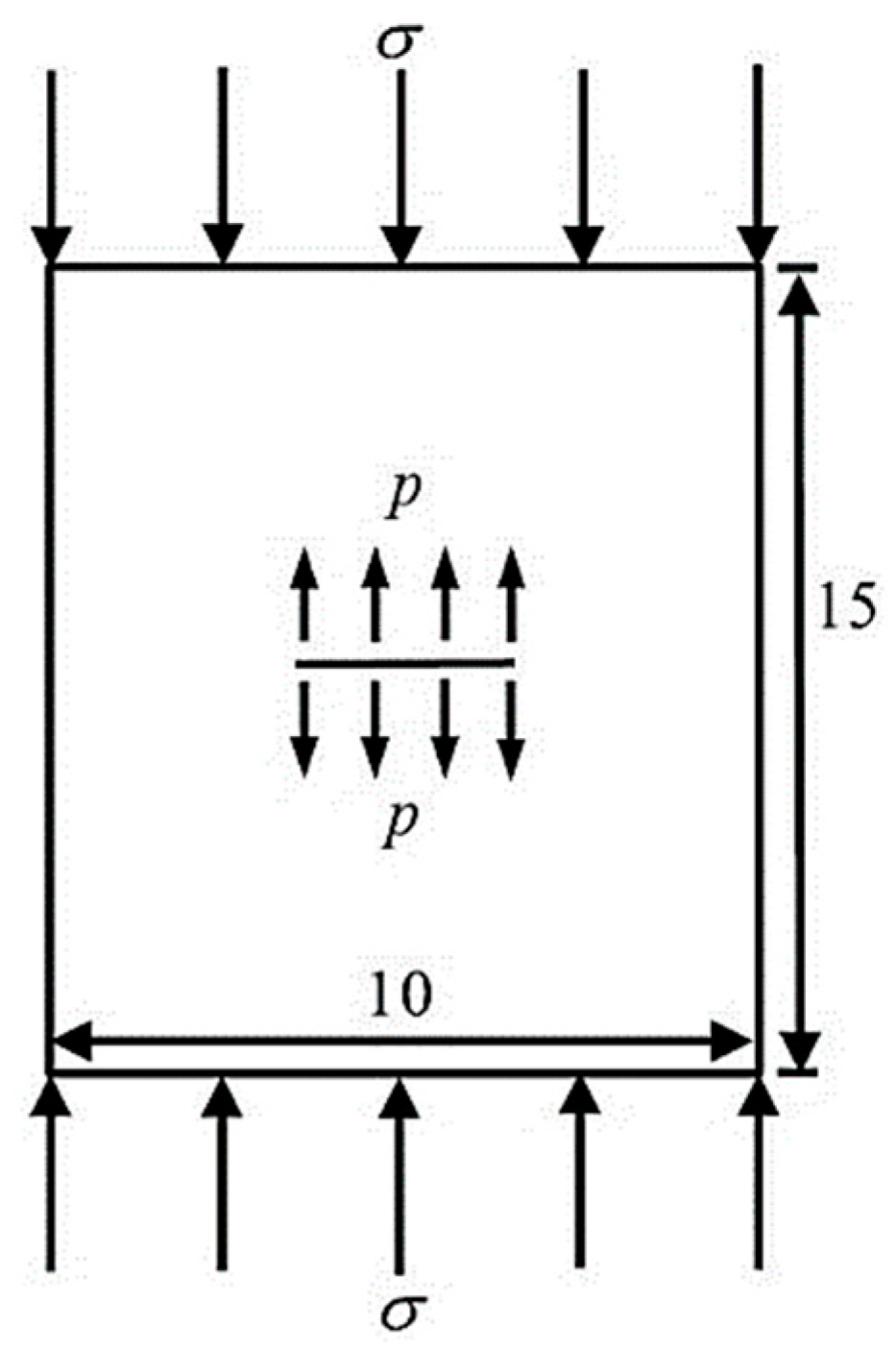
4.1. A Plate with a Single-Edge Crack
4.2. A Plate with a Through Crack
4.3. A Plate with a Central Crack
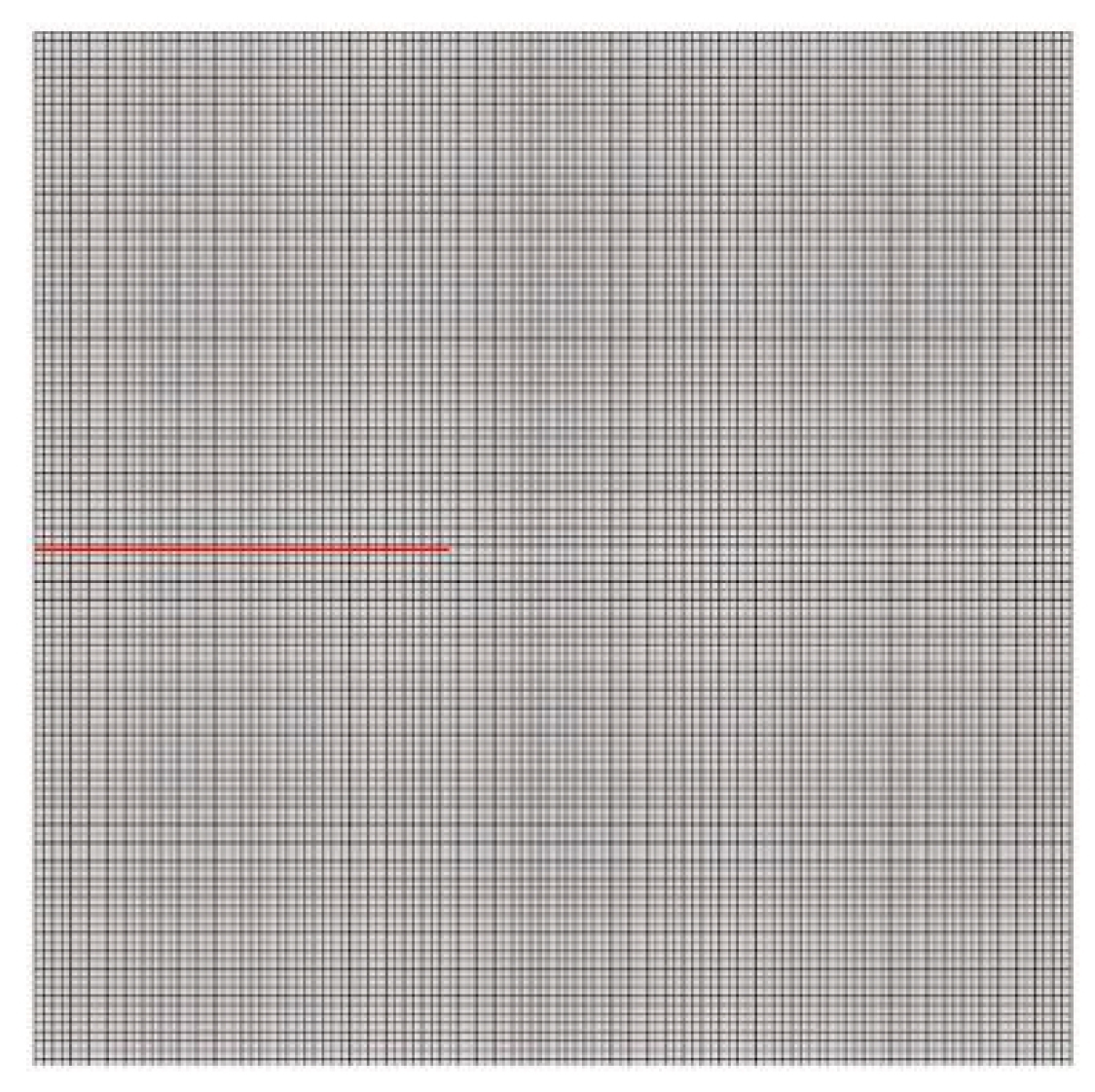
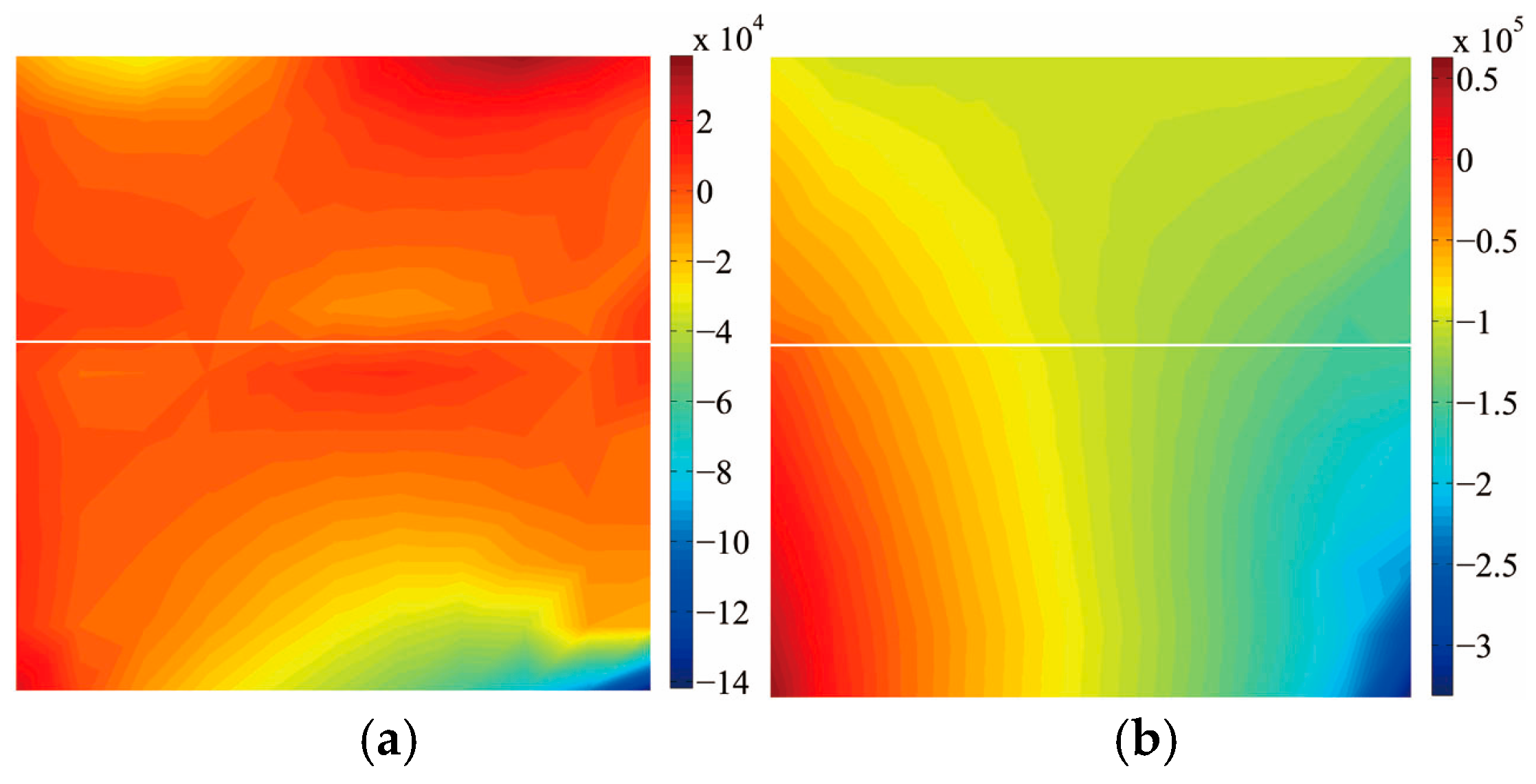
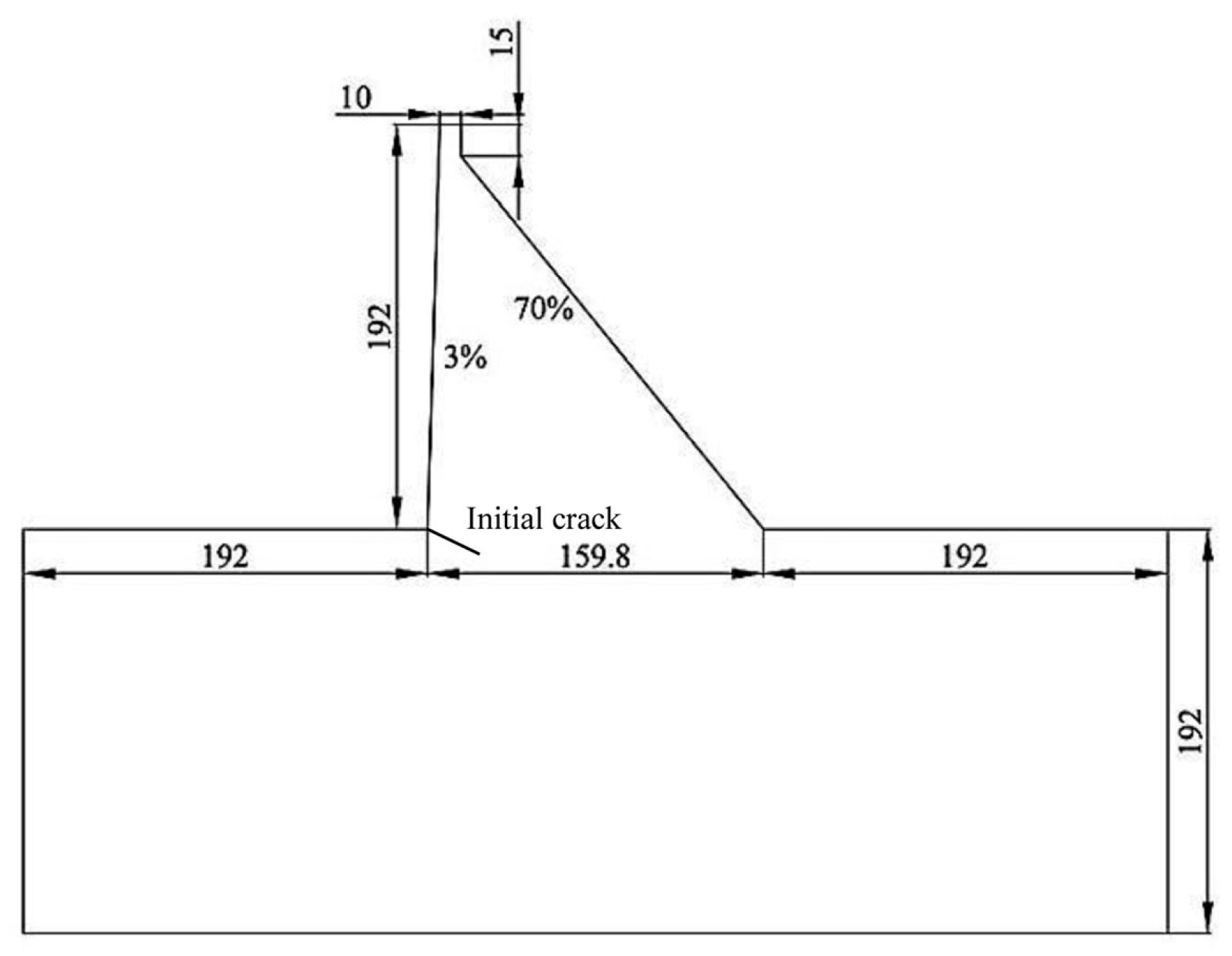
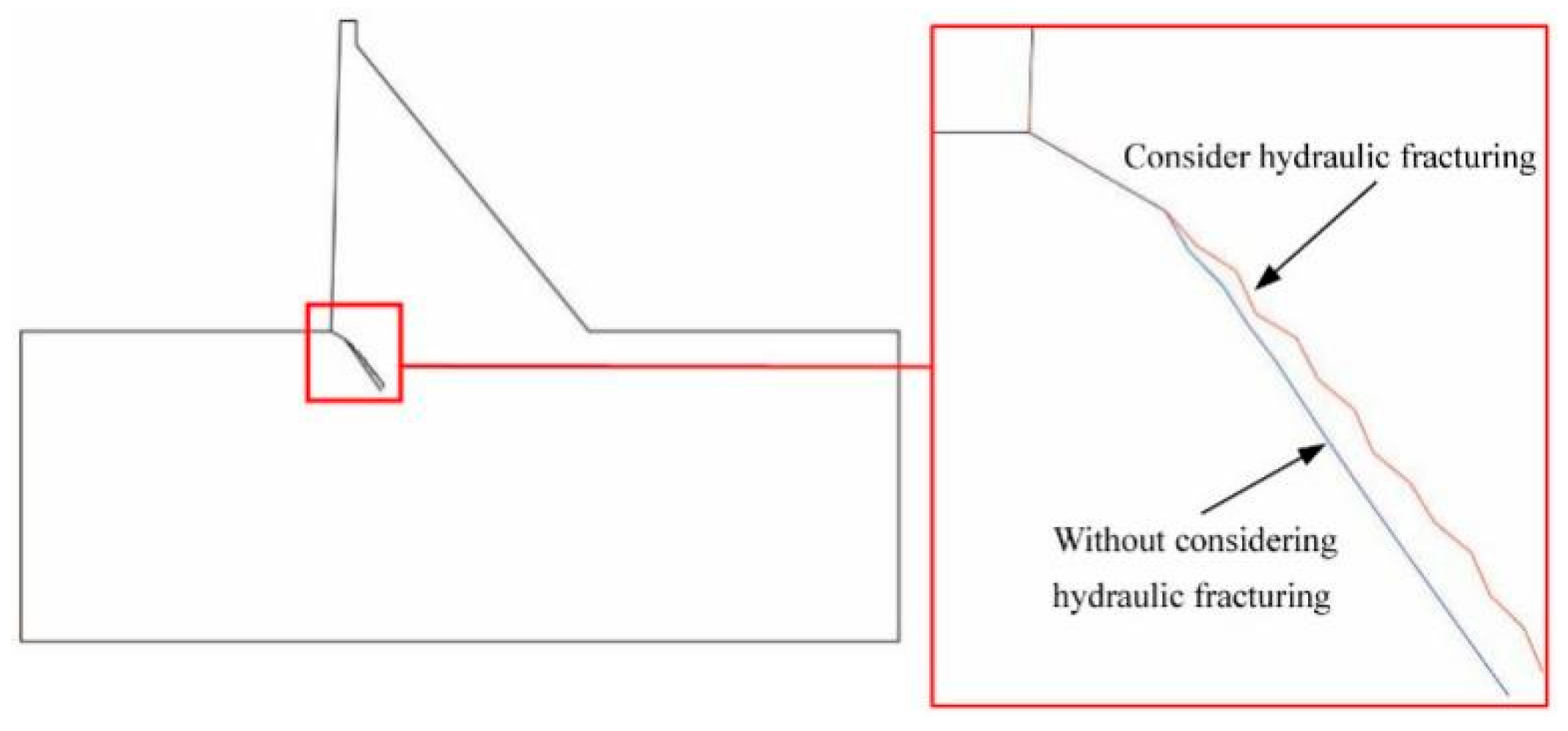
4.4. A Gravity Dam with an Initial Crack
5. Conclusions
- (1)
- The values of the stress-intensity factors calculated by the method in this paper are consistent with the exact solutions, indicating that the method in this paper is accurate in calculating the stress-intensity factors when the crack surface is subjected to the action of uniformly distributed water pressures. Moreover, as the number of elements increases, the computational error gradually decreases.
- (2)
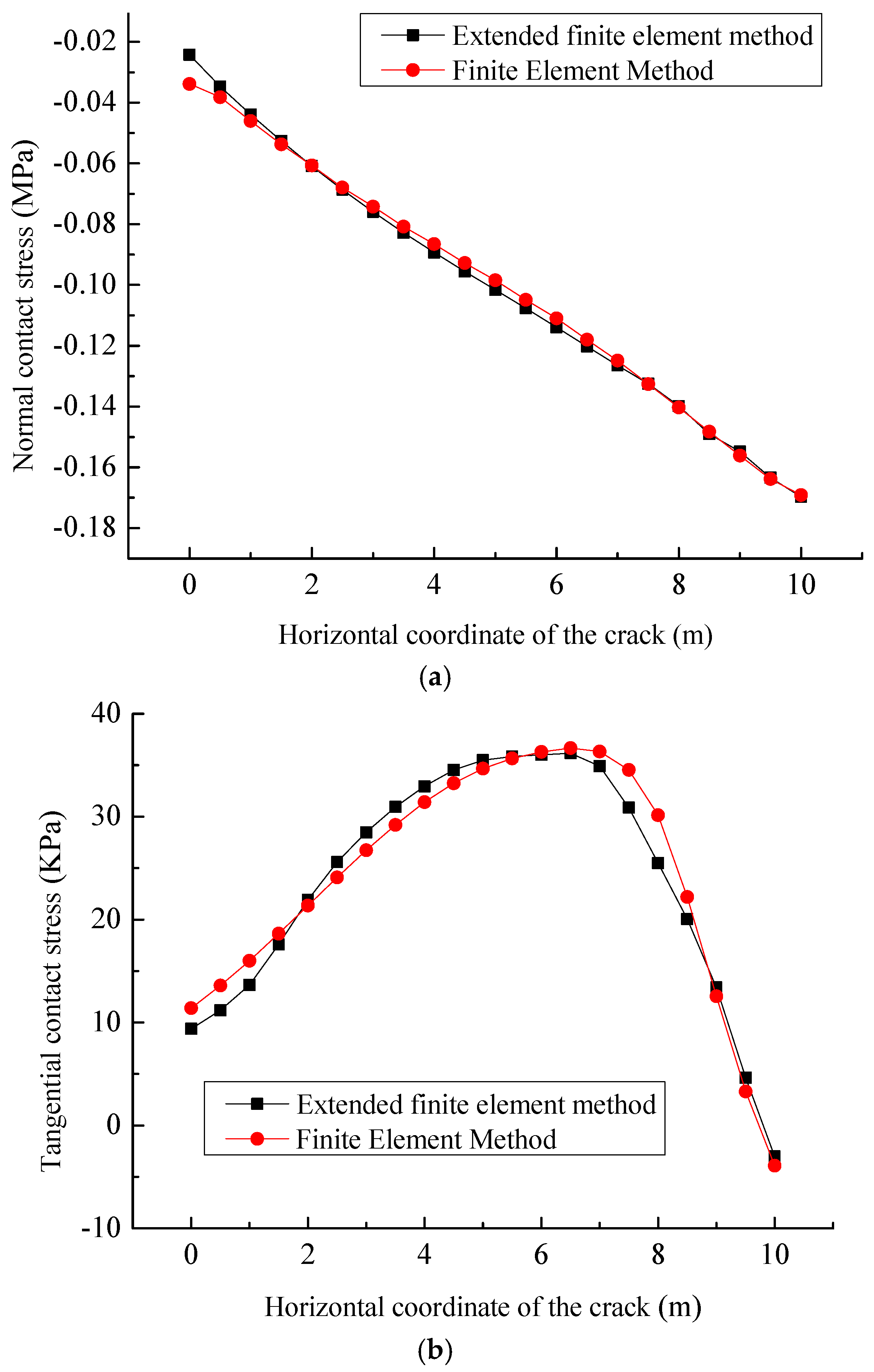
- The contact algorithm in this paper can effectively prevent the crack surfaces from interpenetrating. Its results are consistent with the calculation results of the finite-element penalty function method, indicating that the contact algorithm in this paper is accurate.
- (3)
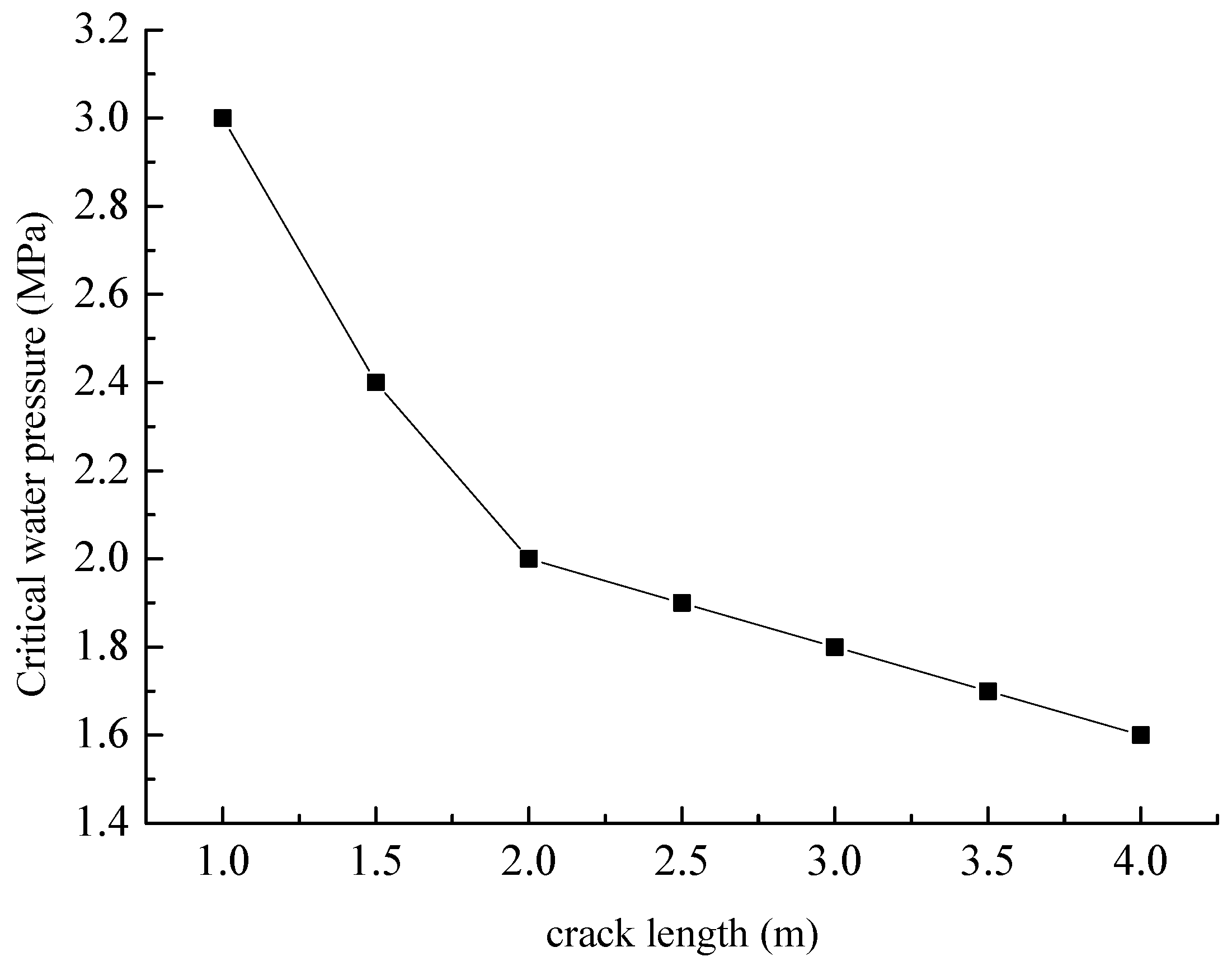
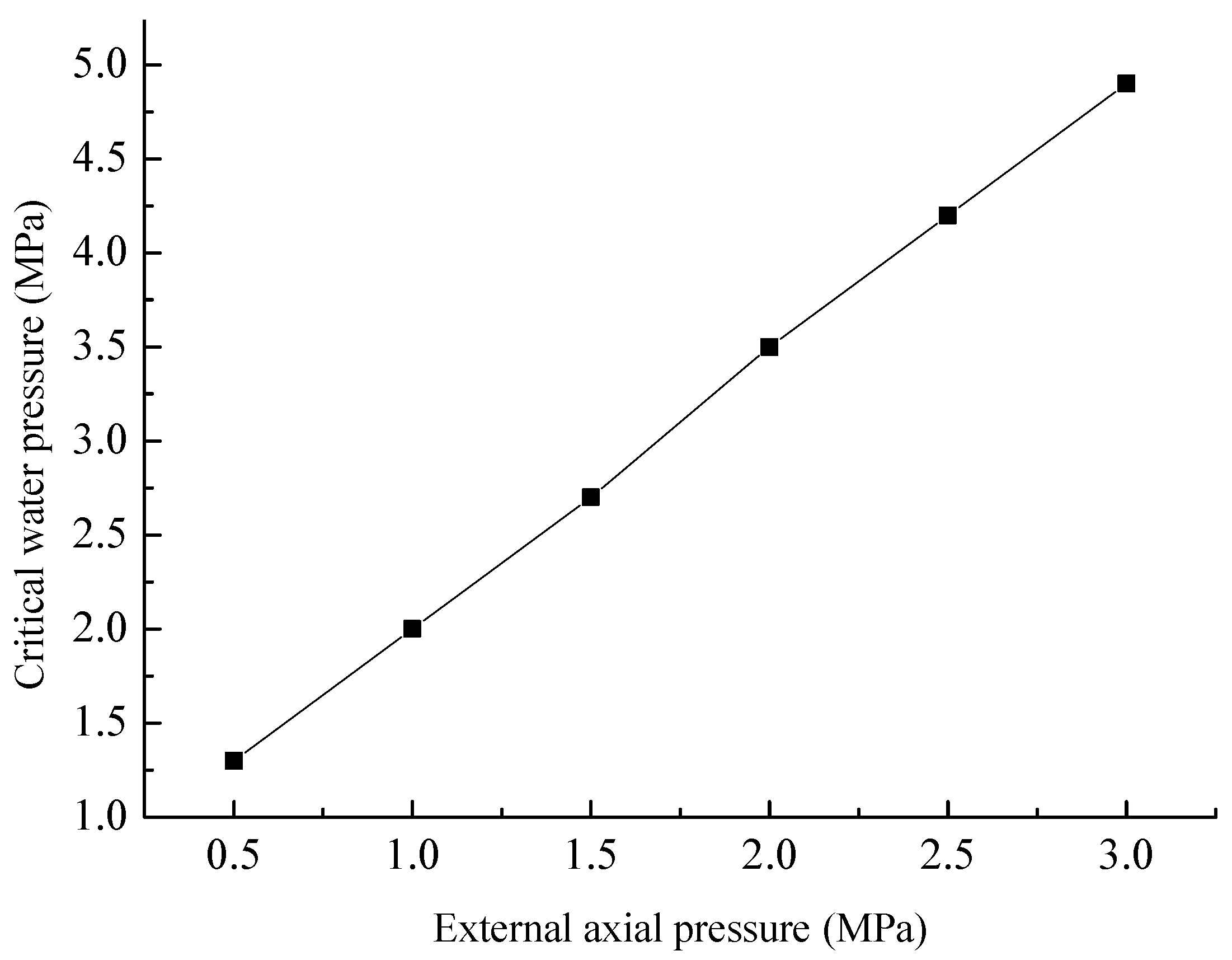
- Under the combined action of the external axial pressure and the internal water pressure, the stress intensity factor at the crack tip of the rock specimen increases as the crack length increases. The critical water pressure decreases as the crack length increases; the critical water pressure increases as the external axial pressure increases. The axial pressure has an inhibitory effect on crack initiation, and the critical water pressure will increase.
- (4)
- Hydraulic fracturing will increase the mode I stress-intensity factor at the crack tip and reduce the stability of the cracks at the foundation of the gravity dam.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Andree, G. Brittle Failure of Rock Materials Test Results and Constitutive Models; A. A. Balkema: Rotterdam, The Netherlands, 1995; pp. 1–5. [Google Scholar]
- Xu, Y.Q.; Zhan, D.Y. An Introduction to Rock Mass Hydraulics; Southwest Jiaotong University Press: Chengdu, China, 1995. [Google Scholar]
- Ahmad, D.; Parancheerivilakkathil, M.S.; Kumar, A.; Goswami, M.; Ajaj, R.M.; Patra, K.; Jawaid, M.; Volokh, K.; Zweiri, Y. Recent developments of polymer-based skins for morphing wing applications. Polym. Test. 2024, 135, 108463. [Google Scholar] [CrossRef]
- Qing, L.B.; Yu, K.L.; Xu, D.Q. Numerical analysis of macro-meso fractures in concrete gravity dams using extended finite element method. J. Hydroelectr. Eng. 2017, 36, 94–102. [Google Scholar]
- Shen, M.; Li, G.S. Extended finite element modeling of hydraulic fracture propagation. Eng. Mech. 2014, 31, 123–128. [Google Scholar]
- Shi, L.Y.; Li, J.; Xu, X.R.; Yu, T.T. Influence of hydraulic fracturing on natural fracture in rock mass. Rock Soil Mech. 2016, 37, 3003–3010. [Google Scholar]
- Zhang, J.N.; Yu, H.; Xu, W.L.; Lv, C.S.; Micheal, M.M.; Shi, F.; Wu, H.A. A hybrid numerical approach for hydraulic fracturing in a naturally fractured formation combining the XFEM and phase-field model. Eng. Fract. Mech. 2022, 271, 108621. [Google Scholar] [CrossRef]
- Lecampion, B. An extended finite element method for hydraulic fracture problems. Commun. Numer. Meth. Eng. 2009, 25, 121–133. [Google Scholar] [CrossRef]
- Shi, F.; Wang, D.B.; Li, H. An XFEM-based approach for 3D hydraulic fracturing simulation considering crack front segmentation. J. Pet. Sci. Eng. 2022, 214, 110518. [Google Scholar] [CrossRef]
- Zeng, Q.D.; Yao, J.; Shao, J.F. An extended fnite element solution for hydraulic fracturing with thermo-hydro-elastic–plastic coupling. Comput. Methods Appl. Mech. Engrg. 2020, 364, 112967. [Google Scholar] [CrossRef]
- Moës, N.; Dolbow, J.; Belytschko, T. A finite element method for crack growth without remeshing. Int. J. Numer. Methods Eng. 1999, 46, 131–150. [Google Scholar] [CrossRef]
- Fleming, M.; Chu, Y.A.; Moran, B.; Belytschko, T. Enriched element-free Galerkin methods for crack tip fields. Int. J. Numer. Methods Eng. 1997, 40, 1483–1504. [Google Scholar] [CrossRef]
- Zheng, A.X.; Luo, X.Q.; Chen, Z.H. Hydraulic fracturing coupling model of rock mass based on extended finite element method. Rock Soil Mech. 2019, 40, 799–808. [Google Scholar]
- Zheng, A.X.; Luo, X.Q. A mathematical programming approach for frictional contact problems with the extended finite element method. Arch. Appl. Mech. 2016, 86, 599–616. [Google Scholar] [CrossRef]
- Wang, J.; Shi, J.Q. Calculation of stress intensity factor for crack in a finite plate. Chin. J. Rock Mech. Eng. 2005, 24, 963–968. [Google Scholar]
- Xu, L.Q.; Tao, Y.C.; Liu, D.T. Hydraulic fracture test of rock-like materials under uniaxial compression. Adv. Sci. Technol. Water Resour. 2018, 38, 28–34. [Google Scholar]
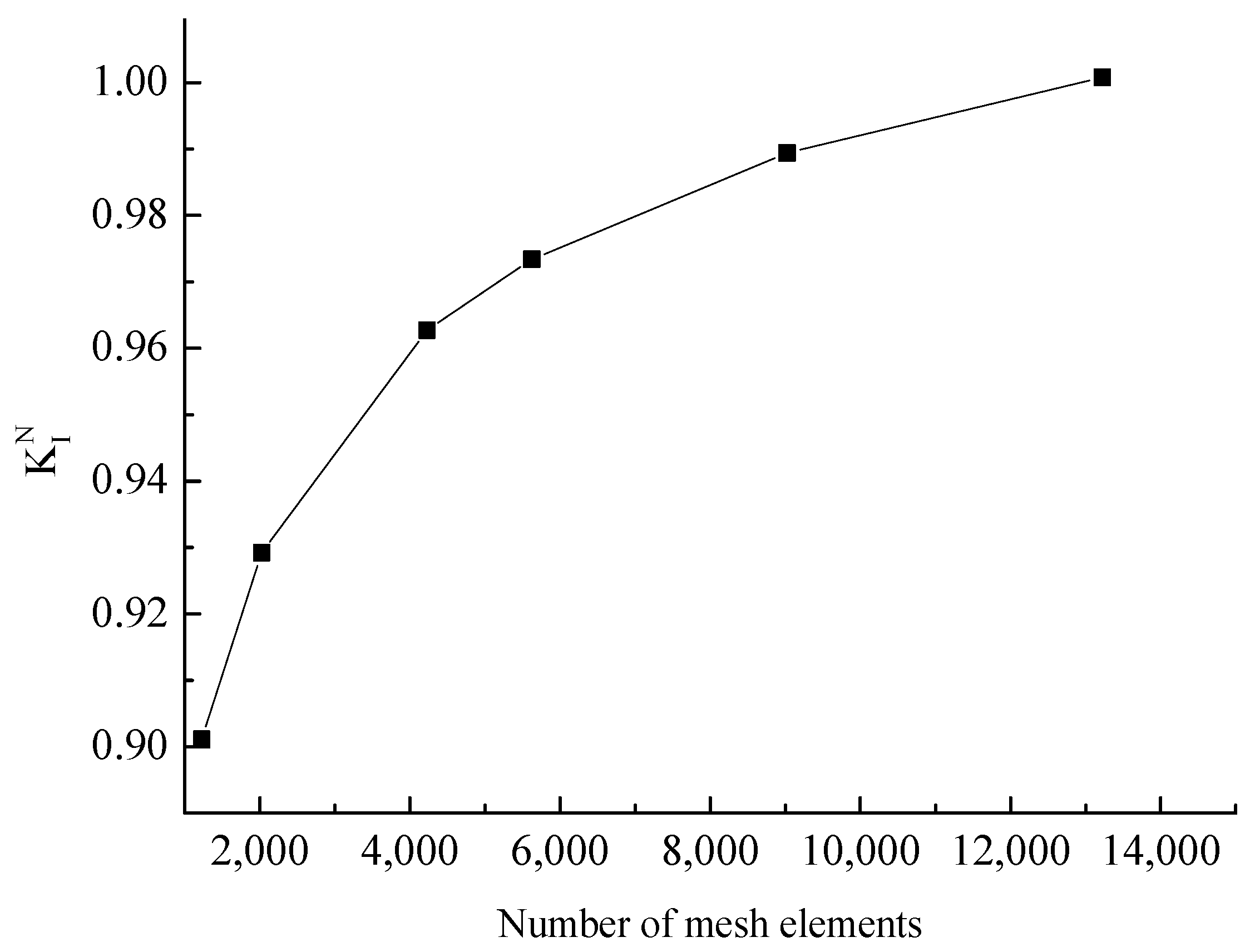










| The Number of Mesh Elements | ||||||
| 1225 | 2025 | 4225 | 5625 | 9025 | 13,225 | |
| 0.9011 | 0.9292 | 0.9627 | 0.9734 | 0.9894 | 1.0008 | |
| /N | /N | /m | /m | |
|---|---|---|---|---|
| 1 | −1.6978 × 104 | 2.8712 × 104 | 2.1843 × 10-7 | −2.8033 × 10-15 |
| 2 | −1.3372 × 104 | 4.0254 × 104 | 2.9343 × 10-7 | −2.6368 × 10-15 |
| 3 | −1.4746 × 104 | 4.7598 × 104 | 3.4758 × 10-7 | −2.5171 × 10-15 |
| 4 | −1.8951 × 104 | 5.7070 × 104 | 4.2055 × 10-7 | −2.3471 × 10-15 |
| 5 | −2.1670 × 104 | 6.3780 × 104 | 4.5915 × 10-7 | −2.2239 × 10-15 |
| 6 | −2.4894 × 104 | 7.2643 × 104 | 4.9167 × 10-7 | −2.0582 × 10-15 |
| 7 | −2.6968 × 104 | 7.8693 × 104 | 5.0011 × 10-7 | −1.9333 × 10-15 |
| 8 | −2.9409 × 104 | 8.6359 × 104 | 4.9064 × 10-7 | −1.7677 × 10-15 |
| 9 | −3.0967 × 104 | 9.2017 × 104 | 4.6729 × 10-7 | −1.6463 × 10-15 |
| 10 | −3.2781 × 104 | 9.9811 × 104 | 4.1295 × 10-7 | −1.4754 × 10-15 |
| 11 | −3.3636 × 104 | 1.0452 × 105 | 3.5693 × 10-7 | −1.3540 × 10-15 |
| 12 | −3.4159 × 104 | 1.0958 × 105 | 2.5821 × 10-7 | −1.1857 × 10-15 |
| 13 | −3.5201 × 104 | 1.1714 × 105 | 1.7515 × 10-7 | −1.0625 × 10-15 |
| 14 | −4.3069 × 104 | 1.3107 × 105 | 4.6932 × 10-8 | −8.9208 × 10-16 |
| 15 | −4.3905 × 104 | 1.2898 × 105 | 5.4210 × 10-19 | −7.6978 × 10-16 |
| 16 | −2.6140 × 104 | 1.1299 × 105 | 5.5904 × 10-19 | −6.0238 × 10-16 |
| 17 | −2.0941 × 104 | 1.4269 × 105 | 4.5740 × 10-19 | −4.6339 × 10-16 |
| 18 | −2.4505 × 104 | 2.3981 × 105 | 3.3881 × 10-20 | −2.4817 × 10-16 |
| 19 | −1.9113 × 104 | 2.2663 × 105 | −6.2680 × 10-20 | −1.4095 × 10-16 |
| 20 | −8.1822 × 102 | 9.3493 × 104 | 2.8799 × 10-20 | −6.2992 × 10-17 |
| Section | Elastic Modulus/GPa | Poisson’s Ratio | ) | ) |
|---|---|---|---|---|
| Dam body | 22 | 0.167 | 24 | 21,500 |
| Dam foundation | 24 | 0.2 | 28 | 22,800 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, A. Extended Finite Element Method for Analyzing Hydraulic Fracturing of Rock Cracks Under Compression. Processes 2025, 13, 514. https://doi.org/10.3390/pr13020514
Zheng A. Extended Finite Element Method for Analyzing Hydraulic Fracturing of Rock Cracks Under Compression. Processes. 2025; 13(2):514. https://doi.org/10.3390/pr13020514
Chicago/Turabian StyleZheng, Anxing. 2025. "Extended Finite Element Method for Analyzing Hydraulic Fracturing of Rock Cracks Under Compression" Processes 13, no. 2: 514. https://doi.org/10.3390/pr13020514
APA StyleZheng, A. (2025). Extended Finite Element Method for Analyzing Hydraulic Fracturing of Rock Cracks Under Compression. Processes, 13(2), 514. https://doi.org/10.3390/pr13020514





