Characterization of Damage and Infiltration Modeling of Coal-Slurry Consolidation Mechanics Under Loaded Conditions
Abstract
1. Introduction
2. Model Building
2.1. Model Assumption
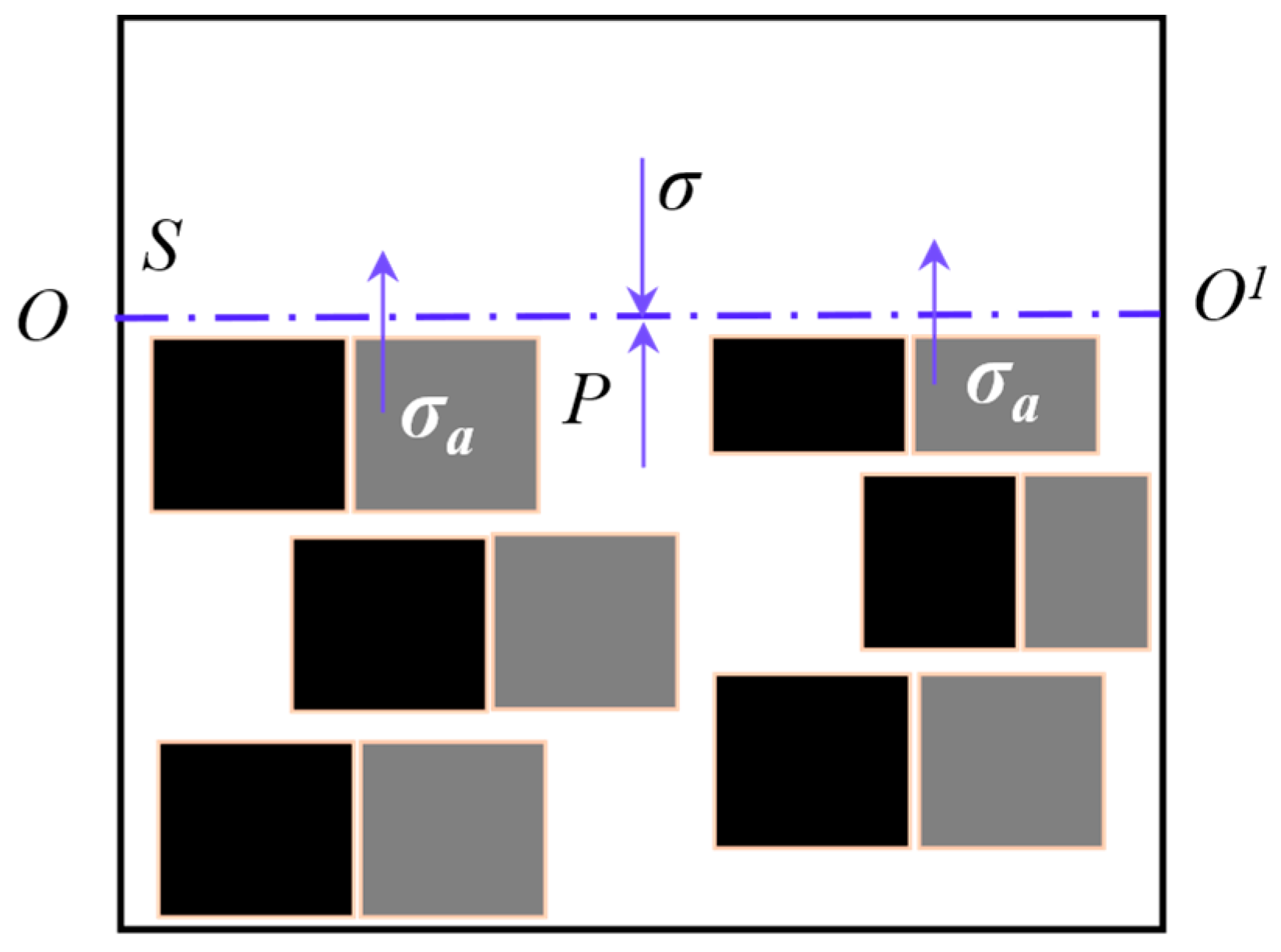
2.2. Deformation of Coal-Slurry Cementation Under Effective Stresses
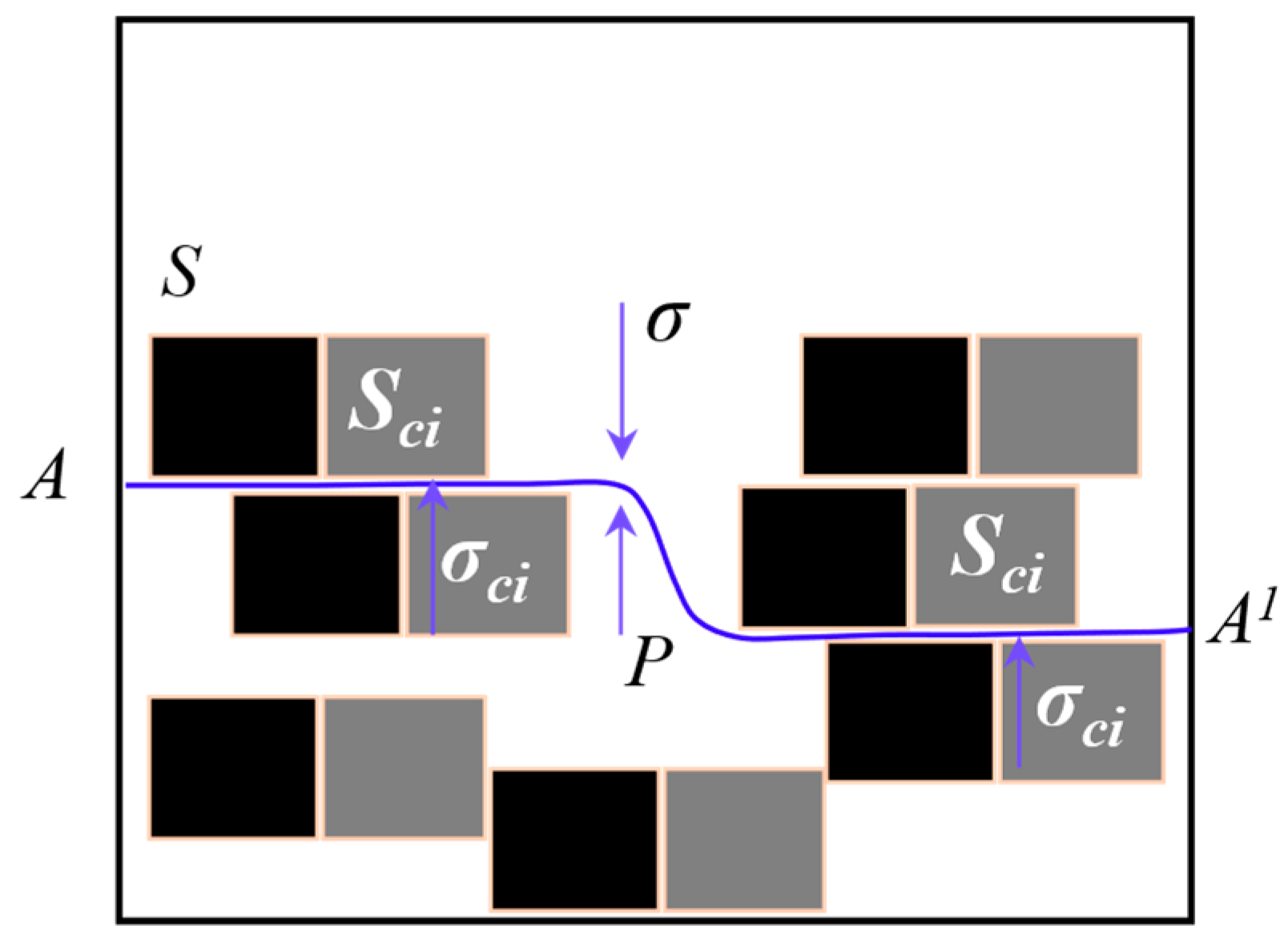
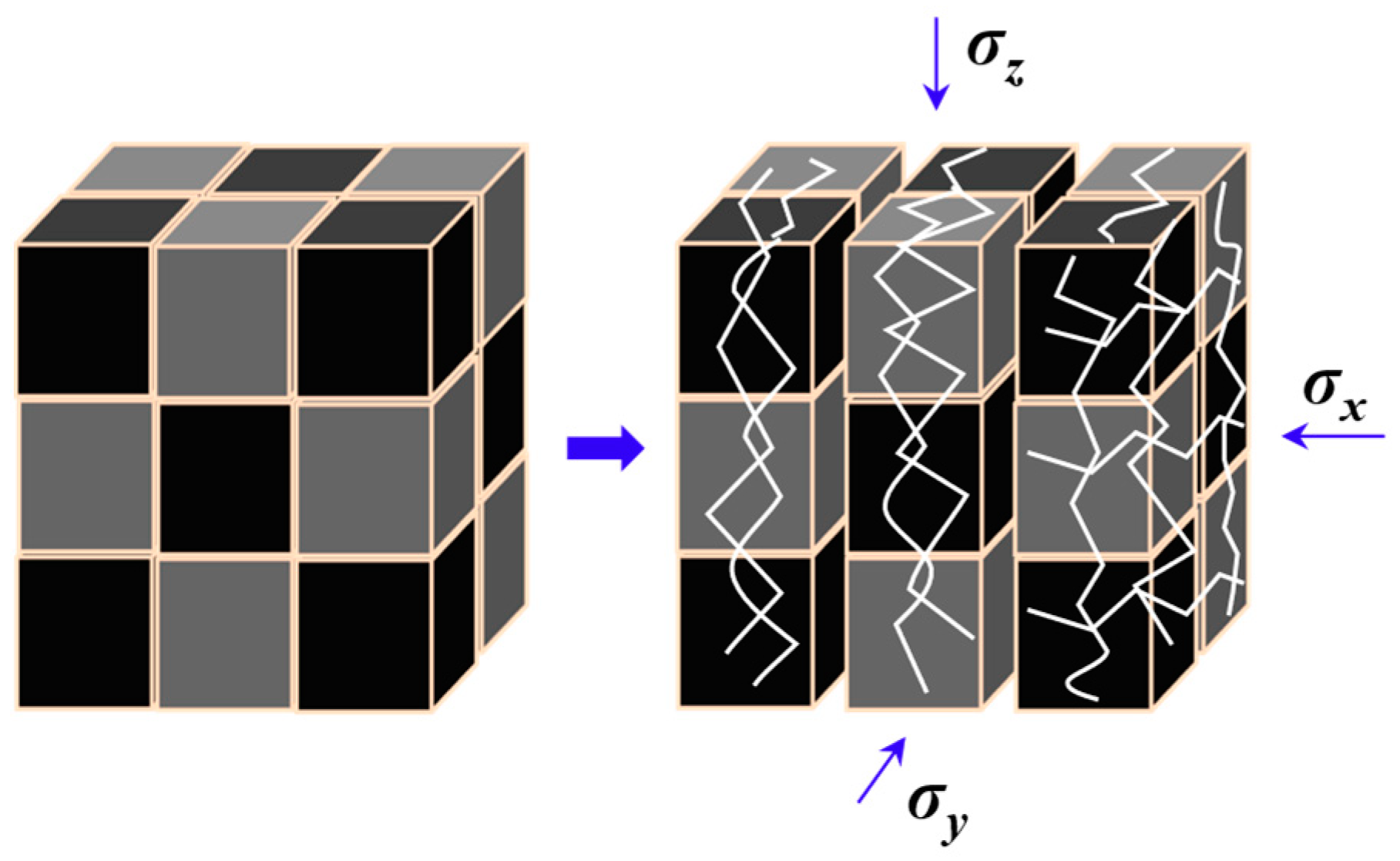
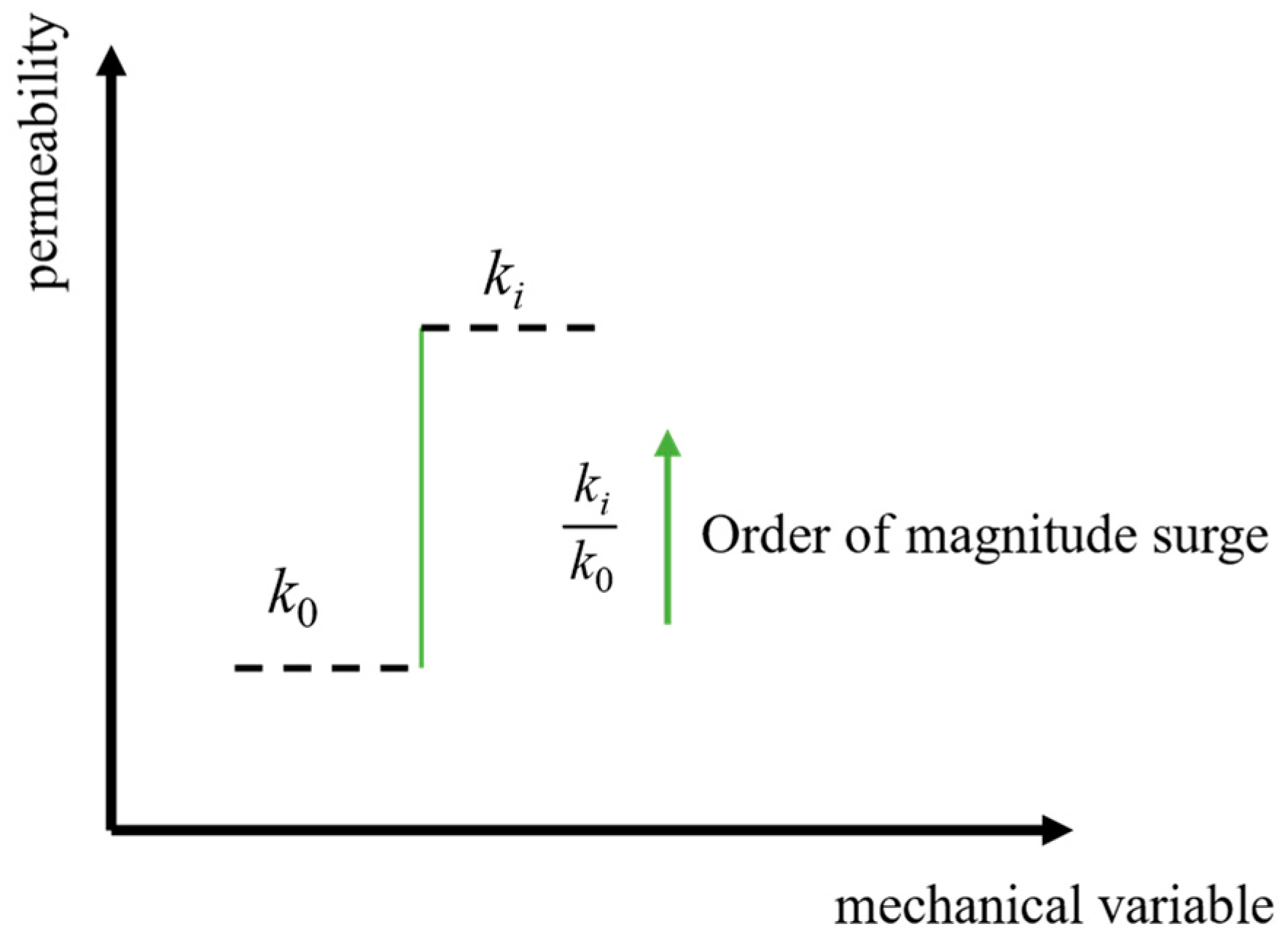
2.3. Modeling the Permeability of Damaged Coal-Slurry Consolidates
3. Coal-Slurry Cementation Seepage Test
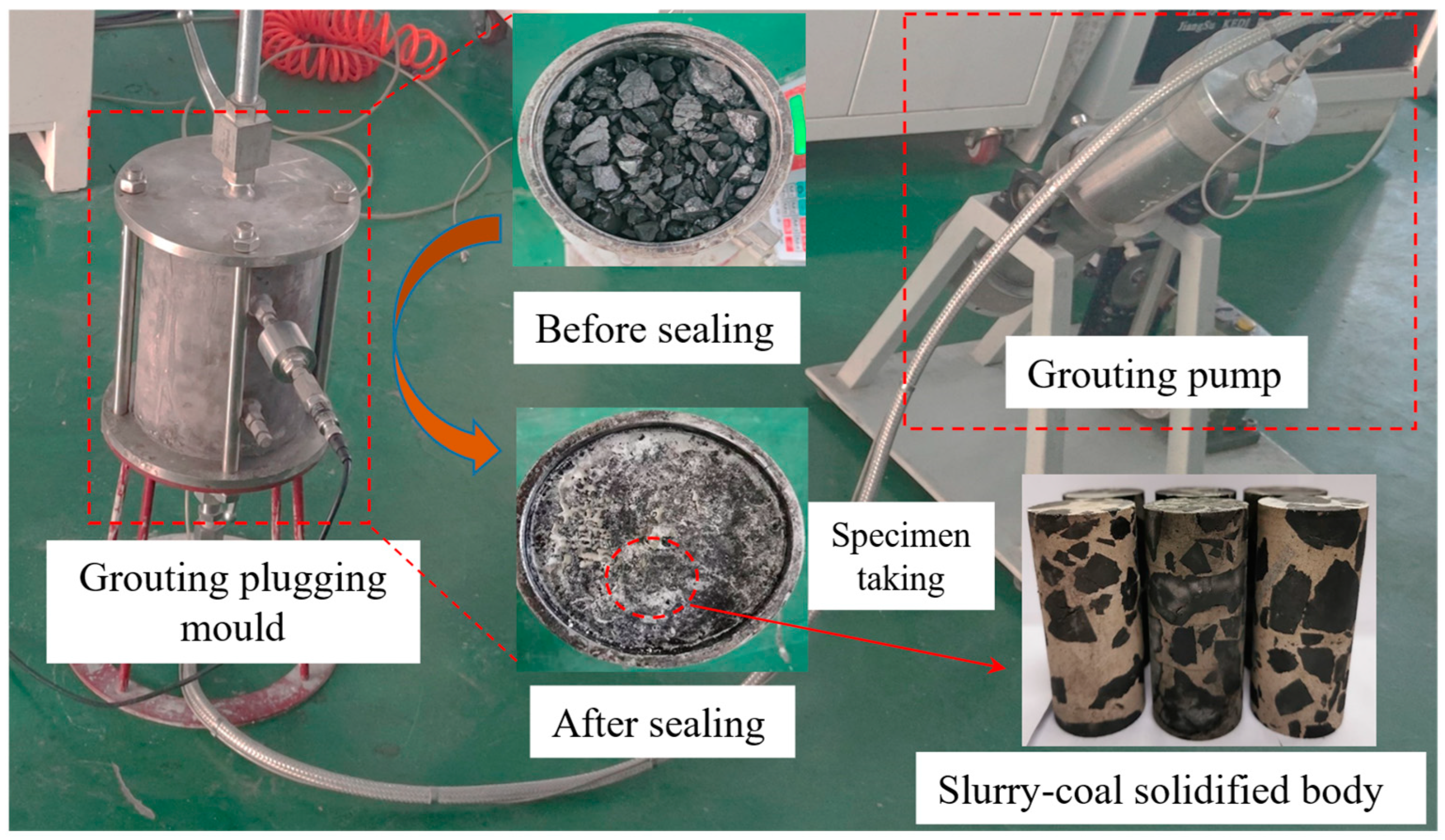
3.1. Sample Preparation
3.2. Test Program and Procedures
4. Analysis of Test Results
5. Model Validation
5.1. Model Basic Parameters
5.2. Validation of Experimental Versus Theoretical Values of Permeability of Coal-Slurry Consolidates
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Total stress | MPa | |
| Effective stress of the body | MPa | |
| Matrix average skeleton pressure | MPa | |
| Structural effective stress | MPa | |
| Pore pressure | MPa | |
| Porosity of coal-slurry cementation body | % | |
| Initial porosity | % | |
| Contact porosity | % | |
| Intrinsic Stress | % | |
| Initial strain | % | |
| Modulus of elasticity | MPa | |
| Initial permeability | mD | |
| Overall bulk modulus of the cemented body | MPa | |
| Bulk modulus of the matrix of the cemented body | MPa | |
| Gas seepage flow rate | cm3/s | |
| Gas dynamic viscosity coefficient | Pa·s | |
| Length of the specimen | mm | |
| Cross-sectional area of the specimen | cm2 |
References
- Li, S.; Gao, M.; Wu, B.; Xu, Y.; Li, Y.; Zeng, G. Dynamic compressive failure of coal at different burial depths. Geomech. Geophys. Geo 2023, 9, 53. [Google Scholar] [CrossRef]
- Shi, Z.; Li, B.; Li, L.; Wang, N.; Zhang, J. Study on the directional extension law of hydraulic fractures induced by pre-cast slot under true-triaxial. Theor. Appl. Fract. Mech. 2024, 133, 104546. [Google Scholar] [CrossRef]
- Li, B.; He, Y.; Shi, Z.; Jian, W.; Wang, N.; Zhang, Y. Mutual feedback and fracturing effect of hydraulic fractures in composite coal-rock reservoirs under different fracturing layer sequence conditions. Int. J. Rock. Mech. Min. Sci. 2024, 184, 105968. [Google Scholar] [CrossRef]
- Fan, C.; Li, S.; Luo, M.; Du, W.; Yang, Z. Coal and gas outburst dynamic system. Int. J. Min. Sci. Technol. 2017, 27, 49–55. [Google Scholar] [CrossRef]
- Chen, L.; Wang, E.; Ou, J.; Fu, J. Coal and gas outburst hazards and factors of the No. B-1 Coalbed, Henan, China. Geosci. J. 2018, 22, 171–182. [Google Scholar] [CrossRef]
- Li, A.; Ding, X.; Yu, Z.; Wang, M.; Mu, Q.; Dai, Z.; Li, H.; Zhang, B.; Han, T. Prediction model of fracture depth and water inrush risk zoning in deep mining coal seam floor. Environ. Earth Sci. 2022, 81, 315. [Google Scholar] [CrossRef]
- Celik, F. The observation of permeation grouting method as soil improvement technique with different grout flow models. Geomech. Eng. 2019, 17, 367–374. [Google Scholar] [CrossRef]
- Ren, Y.; Wei, J.; Zhang, L.; Zhang, J.; Zhang, L. A Fractal Permeability Model for Gas Transport in the Dual-Porosity Media of the Coalbed Methane Reservoir. Transp. Porous Med. 2021, 140, 511–534. [Google Scholar] [CrossRef]
- Zhu, X.; Zhang, Q.; Zhang, W.; Shao, J.; Wang, Z.; Wu, X. Experimental Study on the Basic Properties of a Green New Coal Mine Grouting Reinforcement Material. ACS Omega 2020, 5, 16722–16732. [Google Scholar] [CrossRef]
- Du, W.; Zhang, Y.; Meng, X.; Zhang, X.; Li, W. Deformation and seepage characteristics of gas-containing coal under true triaxial stress. Arab. J. Geosci. 2018, 11, 190. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, E.; Jiang, C.; Zhang, D.; Li, M.; Yu, B.; Zhao, D. True triaxial experimental study of anisotropic mechanical behavior and permeability evolution of initially fractured coal. Nat. Resour. Res. 2023, 32, 567–585. [Google Scholar] [CrossRef]
- Wang, K.; Du, F.; Zhang, X.; Wang, L.; Xin, C. Mechanical properties and permeability evolution in gas-bearing coal-rock combination body under triaxial conditions. Environ. Earth Sci. 2017, 76, 815. [Google Scholar] [CrossRef]
- Wang, K.; Du, F. Experimental investigation on mechanical behavior and permeability evolution in coal-rock combined body under unloading conditions. Arab. J. Geosci. 2019, 12, 422. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, P.; Wei, J.; Yu, C. The seepage properties and permeability enhancement mechanism in coal under temperature shocks during unloading confining pressures. J. Nat. Gas Sci. Eng. 2020, 77, 103242. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, D.; Wei, J.; Yao, B.; Zhang, H.; Fu, J.; Zeng, F. Damage constitutive model of gas-bearing coal using industrial CT scanning technology. J. Nat. Gas Sci. Eng. 2022, 101, 104543. [Google Scholar] [CrossRef]
- Shi, J.Q.; Durucan, S. Drawdown induced changes in permeability of coalbeds: A new interpretation of the reservoir response to primary recovery. Transp. Porous Med. 2004, 56, 1–16. [Google Scholar] [CrossRef]
- Xue, Y.; Dang, F.; Cao, Z.; Du, F.; Ren, J.; Chang, X.; Gao, F. Deformation, permeability and acoustic emission characteristics of coal masses under mining-induced stress paths. Energies 2018, 11, 2233. [Google Scholar] [CrossRef]
- Connell, L.D.; Lu, M.; Pan, Z. An analytical coal permeability model for tri-axial strain and stress conditions. Int. J. Coal Geol. 2010, 84, 103–114. [Google Scholar] [CrossRef]
- Bai, X.; Wang, Y.; He, G.; Zhou, Z.; Wang, D.; Zhang, D. Research on a permeability model of coal damaged under triaxial loading and unloading. Fuel 2023, 354, 129375. [Google Scholar] [CrossRef]
- Wang, G.; Liu, Z.; Wang, P.; Guo, Y.; Wang, W.; Huang, T.; Li, W. The effect of gas migration on the deformation and permeability of coal under the condition of true triaxial stress. Arab. J. Geosci. 2019, 12, 486. [Google Scholar] [CrossRef]
- Lu, J.; Yin, G.; Deng, B.; Zhang, W.; Li, M.; Chai, X.; Liu, C.; Liu, Y. Permeability characteristics of layered composite coal-rock under true triaxial stress conditions. J. Nat. Gas Sci. Eng. 2019, 66, 60–76. [Google Scholar] [CrossRef]
- Liu, B.; Zhu, L.; Liu, X.; Liu, Q.; Fan, Y.; Yao, W.; Deng, W. Energy evolution and damage deformation behavior of cemented broken coal specimen under triaxial compression condition. Energy 2024, 310, 133203. [Google Scholar] [CrossRef]
- Shen, R.; Wang, X.; Li, H.; Gu, Z.; Liu, W. Brittleness characteristics and damage evolution of coal under true triaxial loading based on the energy principle. Nat. Resour. Res. 2024, 33, 421–434. [Google Scholar] [CrossRef]
- Cao, A.; Wang, C.; Zhang, N.; Li, H.; Liu, Z.; Zhi, S. Experimental study on damage characteristics of coal samples under true triaxial loading and dynamic unloading. Lithosphere 2022, 2022, 5447973. [Google Scholar] [CrossRef]
- Liang, Y.; Ran, Q.; Zou, Q.; Zhang, B.; Hong, Y. Experimental study of mechanical behaviors and failure characteristics of coal under true triaxial cyclic loading and unloading and stress rotation. Nat. Resour. Res. 2022, 31, 971–991. [Google Scholar] [CrossRef]
- Duan, M.; Jiang, C.; Yin, W.; Yang, K.; Li, J.; Liu, Q. Experimental study on mechanical and damage characteristics of coal under true triaxial cyclic disturbance. Eng. Geol. 2021, 295, 106445. [Google Scholar] [CrossRef]
- Lu, S.; Li, M.; Ma, Y.; Wang, S.; Zhao, W. Permeability changes in mining-damaged coal: A review of mathematical models. J. Nat. Gas Sci. Eng. 2022, 106, 104739. [Google Scholar] [CrossRef]
- Liu, C.; Yu, B.; Zhao, H.; Hong, Z.; Tian, Z.; Zhang, D.; Liu, Y. Effective stress effect and slippage effect of gas migration in deep coal reservoirs. Int. J. Rock. Mech. Min. Sci. 2022, 155, 105142. [Google Scholar] [CrossRef]
- Guo, P.; Cheng, Y.; Jin, K.; Li, W.; Tu, Q.; Liu, H. Impact of effective stress and matrix deformation on the coal fracture permeability. Transp. Porous Med. 2014, 103, 99–115. [Google Scholar] [CrossRef]
- Kai, W.; Ang, L.; Zhou, A. Theoretical analysis of influencing factors on resistance in the process of gas migration in coal seams. Int. J. Min. Sci. Technol. 2017, 27, 315–319. [Google Scholar] [CrossRef]
- Jiang, C.; Wang, L.; Ding, K.; Wang, S.; Ren, B.; Guo, J. Experimental study on mechanical and damage evolution characteristics of coal during true triaxial cyclic loading and unloading. Materials 2023, 16, 2384. [Google Scholar] [CrossRef]


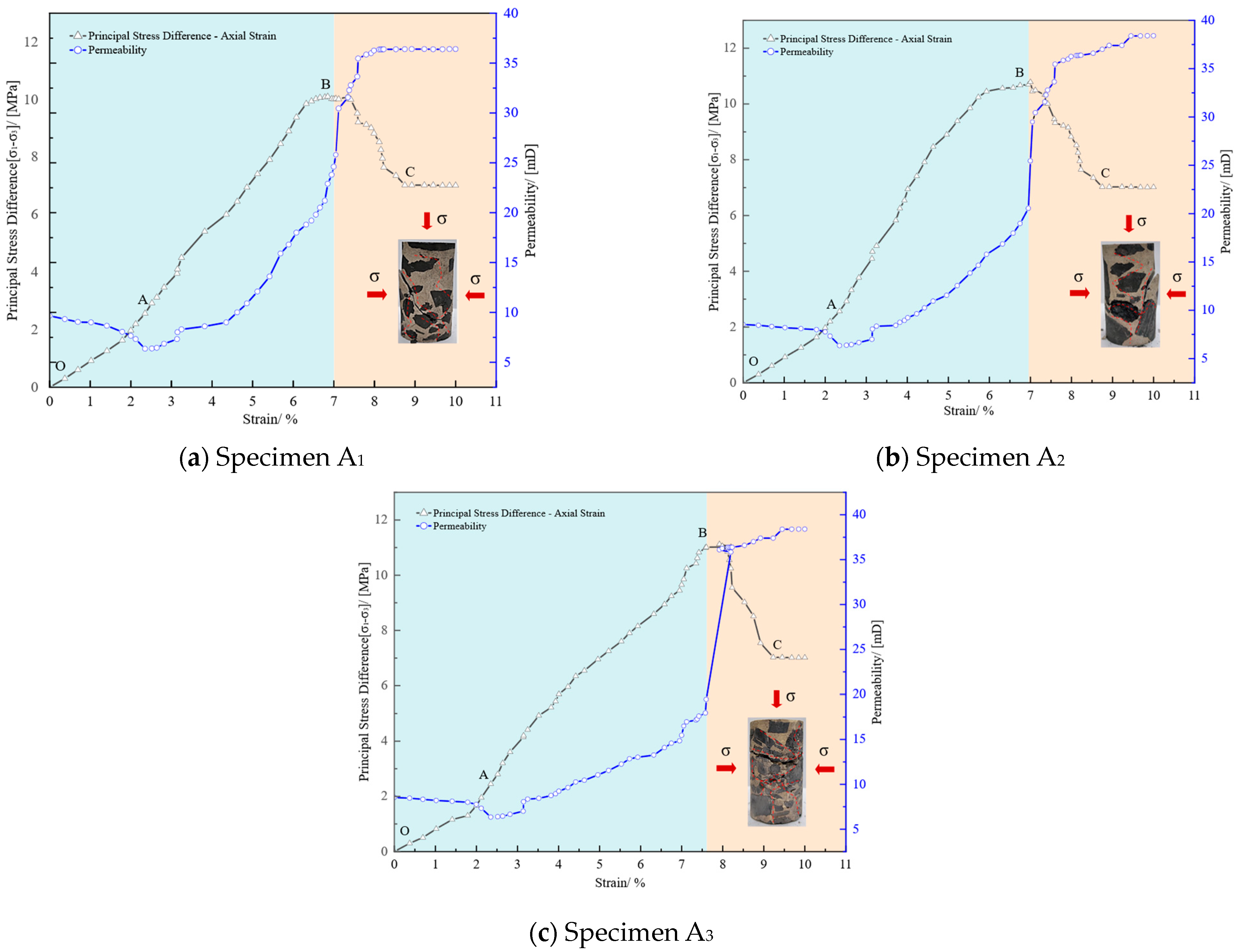
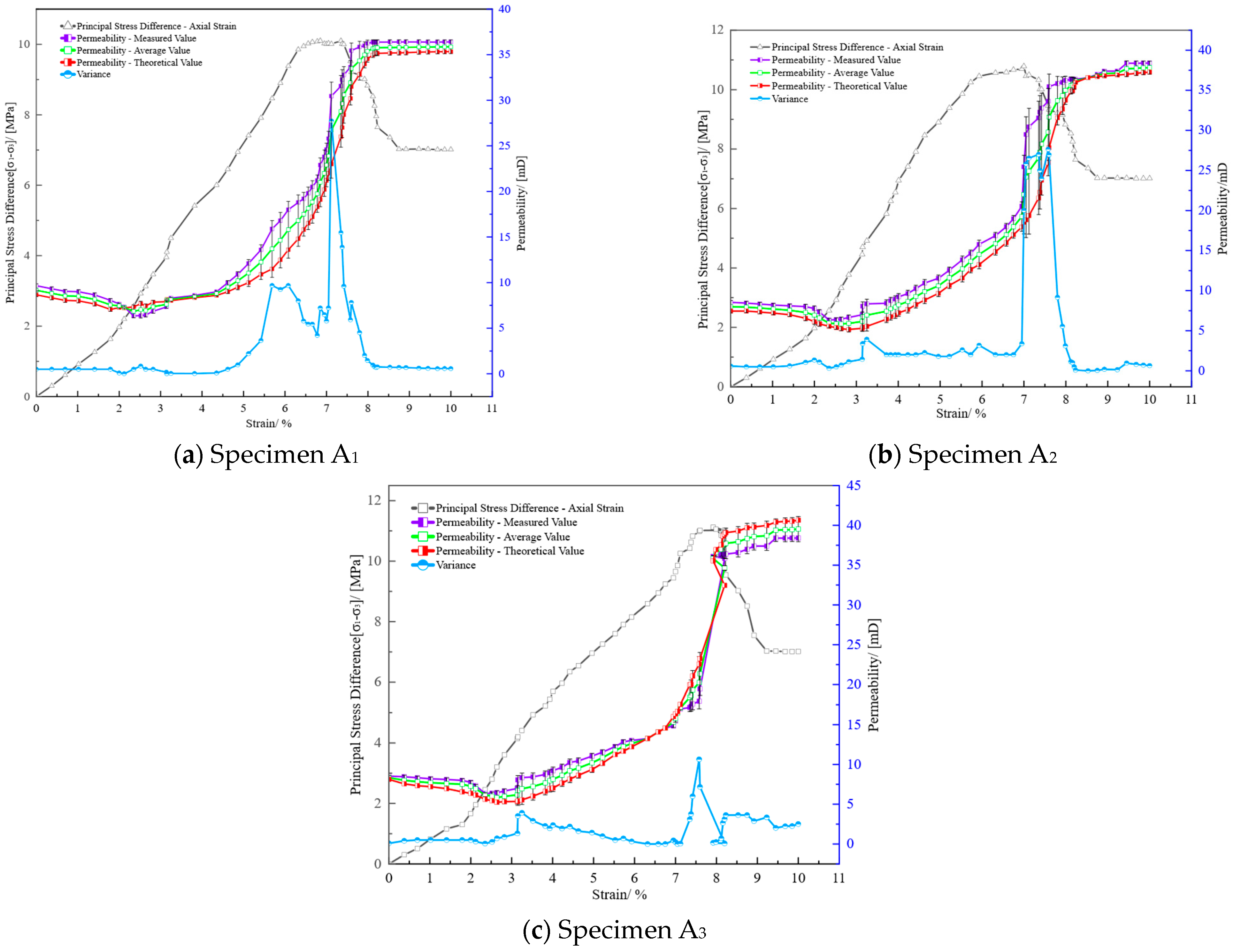
| Coal Sample | ρ/[g/cm3] | ρt/[g/cm3] | Mad/[%] | Vdaf/[%] | Aad/[%] | φ/[%] |
|---|---|---|---|---|---|---|
| anthracite coal | 1.56 | 1.49 | 4.12 | 11.18 | 9.72 | 4.49 |
| Performance Indicators | Compressive Strength/[MPa] | Mobility /[mm] | Initial Coagulation Time /[min] | Final Setting Time /[min] | Dilatation /[%] |
|---|---|---|---|---|---|
| Measured value | 9.5 | 350 | 45 | 245 | 1.13 |
| Specimen Number | Axial Pressure/[MPa] | Pressure on All Sides/[MPa] | Seepage Air Pressure/[MPa] |
|---|---|---|---|
| A1 | 0.5 | 1 | 1 |
| A2 | 0.5 | 2 | 1 |
| A3 | 1 | 2 | 1 |
| Specimen Number | /[MPa] | /[MPa] | /[MPa] | /[MPa] | /[%] | /[%] | /[mD] | |
|---|---|---|---|---|---|---|---|---|
| A1 | 2220 | 1002 | 960 | 875 | 0.19 | 0.18 | 0.09 | 9.66 |
| A2 | 2355 | 995 | 975 | 865 | 0.19 | 0.20 | 0.10 | 8.56 |
| A3 | 2100 | 1055 | 980 | 885 | 0.20 | 0.19 | 0.095 | 8.53 |
| Strain | Specimen A1 | Specimen A2 | Specimen A3 | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Measured Value | Theoretical Value | Average Value | Variance | Measured Value | Theoretical Value | Average Value | Variance | Measured Value | Theoretical Value | Average Value | Variance | |
| 0.37485 | 9.3254 | 8.3254 | 8.8254 | 0.5 | 8.4522 | 7.4522 | 7.9522 | 0.5 | 8.4522 | 7.5565 | 8.00435 | 0.40114 |
| 2.12 | 7.3255 | 7.2255 | 7.2755 | 0.005 | 7.3255 | 5.8544 | 6.58995 | 1.08207 | 7.3255 | 6.1424 | 6.93395 | 0.30662 |
| 3.15 | 8.02 | 8.15 | 8.085 | 0.00845 | 8.0552 | 5.4552 | 6.7552 | 3.38 | 8.0552 | 5.4044 | 6.7298 | 3.51337 |
| 5.12 | 12.1 | 10 | 11.05 | 2.205 | 9.6345 | 7.6345 | 8.6345 | 2 | 9.6345 | 7.6544 | 8.64445 | 1.9604 |
| 6.45 | 19.2 | 15.8 | 17.5 | 5.78 | 14.6454 | 12.6454 | 13.6454 | 2 | 12.8454 | 11.6544 | 12.2499 | 0.70924 |
| 7 | 24.6 | 21.2 | 22.9 | 5.78 | 25.45587 | 18.5254 | 21.99064 | 20.01571 | 15.45587 | 16.3558 | 15.80584 | 0.24495 |
| 7.58 | 33.64027 | 30.2 | 31.92014 | 5.91773 | 33.64027 | 25.9402 | 29.79024 | 27.64554 | 17.94027 | 22.5544 | 20.24734 | 10.6451 |
| 8.16 | 36.3624 | 35.12 | 35.7412 | 0.77178 | 36.3624 | 34.9455 | 35.65395 | 1.0038 | 36.3624 | 38.6624 | 37.5124 | 2.645 |
| 9.23 | 36.3965 | 35.28 | 35.83825 | 0.62329 | 37.3965 | 36.8945 | 37.1455 | 0.126 | 37.3965 | 39.9864 | 38.69145 | 3.35379 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, Y.; Lu, P.; Zhang, J.; Jian, W. Characterization of Damage and Infiltration Modeling of Coal-Slurry Consolidation Mechanics Under Loaded Conditions. Processes 2025, 13, 400. https://doi.org/10.3390/pr13020400
Tang Y, Lu P, Zhang J, Jian W. Characterization of Damage and Infiltration Modeling of Coal-Slurry Consolidation Mechanics Under Loaded Conditions. Processes. 2025; 13(2):400. https://doi.org/10.3390/pr13020400
Chicago/Turabian StyleTang, Yaocai, Peng Lu, Junxiang Zhang, and Wang Jian. 2025. "Characterization of Damage and Infiltration Modeling of Coal-Slurry Consolidation Mechanics Under Loaded Conditions" Processes 13, no. 2: 400. https://doi.org/10.3390/pr13020400
APA StyleTang, Y., Lu, P., Zhang, J., & Jian, W. (2025). Characterization of Damage and Infiltration Modeling of Coal-Slurry Consolidation Mechanics Under Loaded Conditions. Processes, 13(2), 400. https://doi.org/10.3390/pr13020400







