Numerical Simulation and Experimental Study of Gas–Solid Two-Phase Spraying of Dry Powder Fire-Extinguishing System Based on Fire-Extinguishing Inspection Robot

Abstract

In order to solve the problem where the traditional intelligent inspection robot only has a single inspection function, we studied the use of a dry powder (including an ultra-fine dry powder) as a fire-extinguishing medium for the first time. In fire-extinguishing robots, the spray pressure is difficult to control, and there are several other issues. For integrated inspection, an intelligent, nitrogen-driven fire-extinguishing robot using a dry powder in a pressure-controlled spray was developed. On this basis, in order to investigate nitrogen-driven dry powder particle spraying as a gas–solid two-phase mechanism, as well as the flow characteristics and the influence of relevant parameters on the spraying effect, a nitrogen-driven dry powder particle spraying system was established as part of a gas–solid two-phase computational fluid dynamics model. The flow field of the spraying system and the particle motion characteristics were analyzed to explore the micro-mechanisms of the influence of different driving pressures, pipe diameters, and nozzle configurations on the spraying of the dry powder. In order to investigate the macroscopic effect of dry powder spraying where the gas–solid two-phase micro-mechanisms could not be revealed, an experimental platform was set up, and the experiments verified the accuracy of the numerical simulation results. We also investigated the dry powder spraying effect under different driving pressures, pipe diameters, nozzle configurations, and loading ratios. Finally, an orthogonal test was designed based on the results of the single-factor experiments to find the best combination of parameters required to achieve the optimal spraying effect. The research results can provide a theoretical and technical reference for the design and development of nitrogen-driven dry powder spraying systems.

1. Introduction

The petroleum and petrochemical industry is a strategic pillar industry within the national economy. Petroleum and petrochemical facilities are often characterized by high temperatures, high pressures, flammability, and explosiveness. Once a sudden event such as a fire occurs, it may cause a series of secondary and derivative accidents, which can have catastrophic consequences and seriously threaten people’s lives and property, as well as national energy security. At present, the inspection and initial firefighting measures implemented to address fire risks on petroleum and petrochemical production sites rely on manual labor, and firefighting often relies on fixed firefighting facilities, resulting in delayed accident detection and difficulties in implementing fire prevention measures, which may lead to an increase in accidents. The use of robots to assist or replace personnel in high-risk environments to perform inspection operations and fire prevention measures effectively improves the safety management level of petroleum and petrochemical operations, allowing them to be executed in a streamlined, intelligent, and unmanned manner.

In response to the above issues, researchers at home and abroad have developed intelligent inspection robots for oil and gas leakage fires. The relevant technology has reached maturity and has been deployed and applied on petroleum and petrochemical sites; examples are Shell’s “Sensabot” robot and Anson Intelligence’s ACR inspection robot. However, through an analysis of the current status and implementation of oil and gas leakage fire inspection robots, the following problems can be determined. (1) After identifying the risks of accidents such as oil and gas leakage fires, the inspection robots need to notify the operators through alarm or command transmission, and then the operators implement early response measures. It is difficult to implement fire-extinguishing and emergency responses in a timely manner, and missing the ideal time window for fire extinguishing may lead to the occurrence of accidents. Currently, there are no relevant technical reports on robots that integrate inspection and fire-extinguishing functions. (2) In fire-extinguishing robots using a dry powder (including an ultra-fine dry powder) as the extinguishing medium, the spraying pressure cannot be controlled, making it difficult to achieve targeted treatment for large, medium, and small fires. It is difficult to implement efficient fire extinguishing with reasonable equipment for fires of different sizes, resulting in unnecessary resource waste.

Based on the above issues, an intelligent inspection and fire-extinguishing robot integrating inspection and dry powder fire-extinguishing functions has been developed.

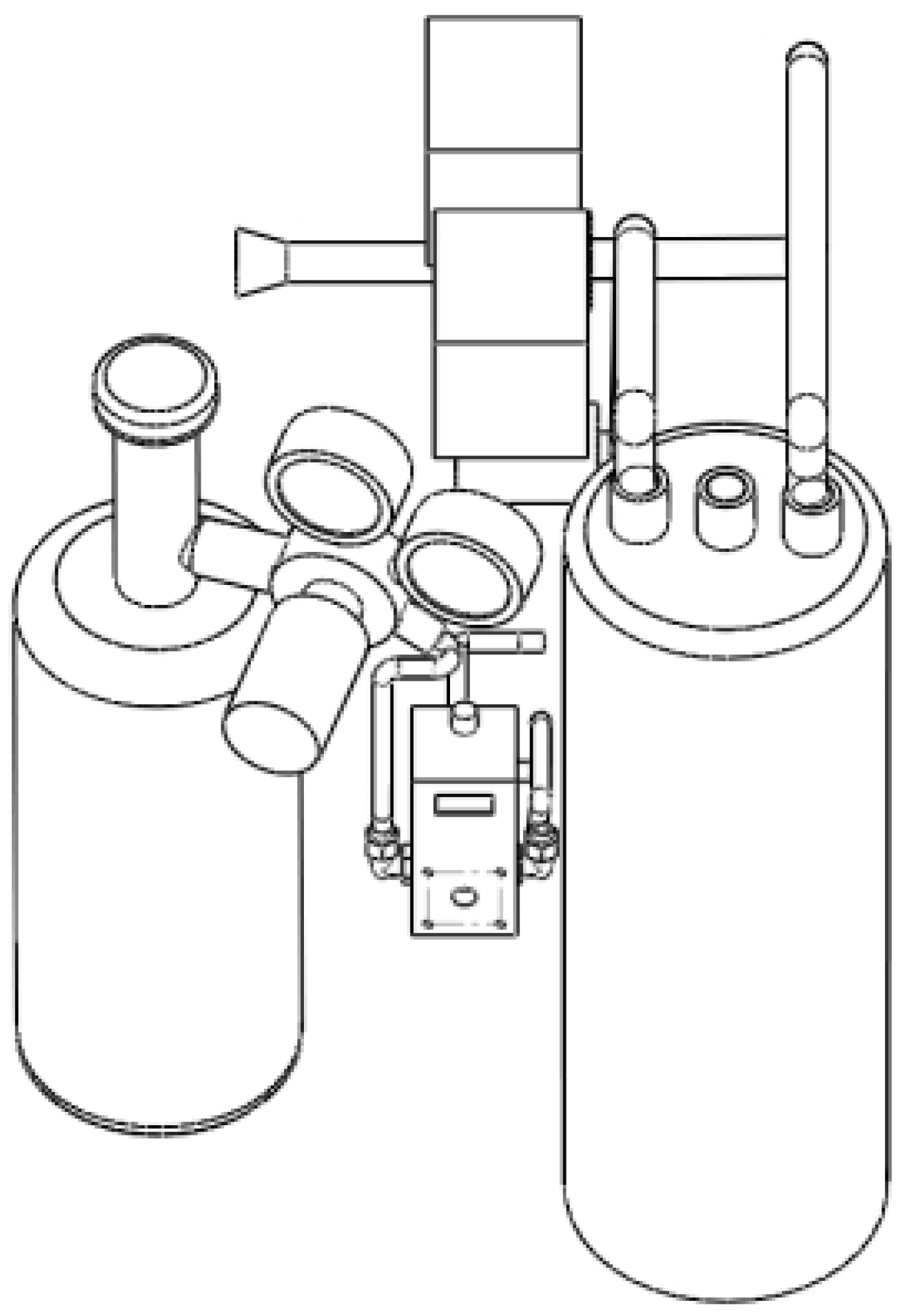
The robot consists of five modules, namely the main control module, power module, detection module, fire-extinguishing module, and wireless charging module, as well as a visual detection system and sensor detection system included in the detection module. It also contains four subsystems, including an automatic release system for the fire-extinguishing agent and a fire-extinguishing execution system included in the fire-extinguishing module. It has multiple excellent features such as intelligent autonomous inspection and control over the spray pressure of the dry powder fire-extinguishing agent (referred to as “pressure-controlled fire extinguishing”). During the autonomous inspection process, it can monitor the environment in real time through high-definition and infrared cameras, as well as sensors to detect toxic, harmful, and combustible gases and perform flame monitoring. If the system detects an abnormal temperature, fireworks, a combustible gas, etc., it can be reported to the backend operator in a timely manner. The backend operator can address the fire situation according to the size of the fire. They can choose different fire-extinguishing levels, including large, medium, and small, to implement fire-extinguishing measures through dry powder spraying. 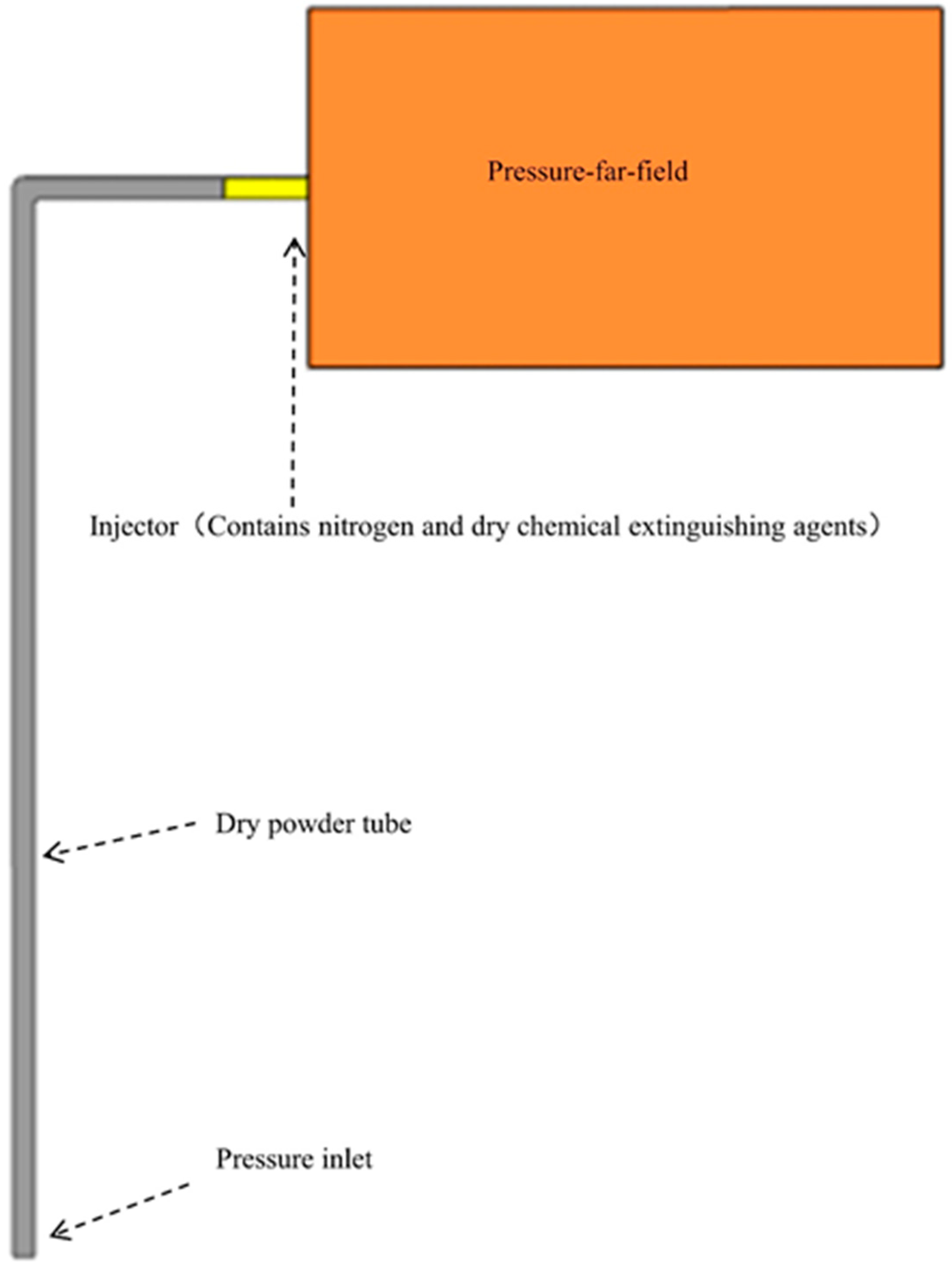A schematic diagram of the dry powder pressure control fire-extinguishing module is shown in Figure 1. It includes a nitrogen tank, a dry powder tank, and a pressure control drive valve group. The implementation method can be summarized as follows: the high-pressure nitrogen released by the nitrogen tank is reduced to the set pressure value through the relevant valve group, and the reduced nitrogen is regulated by the electromagnetic proportional valve to achieve a constant-pressure nitrogen input to the dry powder tank. When the pressure of the dry powder tank reaches the set value, the electric control ball valve at its outlet opens, driving the dry powder toward the nozzle for dry powder injection. Figure 2. Pressure control and fire suppression module system control diagram. The volume of the nitrogen tank is 3 L, and the initial pressure is 15 MPa; the volume of the dry powder tank is 8 L, and the inner diameter is 160 mm; the inner diameter of the valve is 10 mm.

Table 1. The robot motion parameters.

Sports Category	Parametric
50 m straight line travel speed	1.6 m/s
1.6 m/s braking distance when travelling	24 mm
smooth obstacle height	100 mm
minimum obstacle avoidance area	75,000 mm2
maximum smooth climbing gradient	15°

shows the robot motion parameters.

For the intelligent inspection and fire-extinguishing robot with a controlled-pressure dry powder fire-extinguishing system and for related nitrogen-driven dry powder injection fire-extinguishing devices, the injection pressure, injection pipe diameter, nozzle shape, and loading ratio all have an impact on the dry powder injection effect of the system. These are important parameters in the design and development of fire-extinguishing modules and are determined through robot injection pressure gear setting, pipe diameter selection, and nozzle design. However, the mechanism of the gas–solid two-phase interaction and the flow characteristics of nitrogen-driven dry powder particle injection, as well as the influence of the related parameters on the injection effect, are not yet clear. This is not conducive to the optimization and design of dry powder controlled-pressure injection fire extinguishing. Researchers have conducted relevant research on the mechanisms of powder particle spraying and the influence of the related parameters. Theodore Gabor et al. [1] studied the gas powder flow dynamics under different nozzle profiles and found that rectangular nozzles were not as effective in accelerating powder injection as circular nozzles. Woo Chang Sung et al. [2] analyzed the effect of the particle size distribution on the fluid dynamics of dilute-phase pneumatic conveying systems. The results showed that although the width in the particle size distribution became larger and the standard deviation increased, the pressure drop and jump velocity per unit length decreased with the increase in the fine powder fraction in the particle size distribution. Guanlong Ren et al. [3] used the Euler–Euler model to numerically simulate the effect of different intake positions on powder fluidization, and they found that moving the intake position backward could improve the stability of the powder output. Dmitry Portnikov et al. [4] proposed a simple method to predict particle wear during pneumatic conveying. The experimental results and parameter studies showed that the larger the bending radius ratio, the less damage to the conveyed material. Cao et al. [5] carried out an analytical study on the kinematic characteristics of the external flow field of a particle jet. The results showed that the jet width and boundary layer thickness had a certain effect on the velocity of the particles. With an increase in the distance from the nozzle exit position, the particle velocity showed an increase and then tended to stabilize, while the particle velocity distribution at the nozzle was not uniform and the difference was relatively large. Meyera et al. [6] investigated the relationship between the particle velocity and the particle loading ratio during the injection process and showed that the particle velocity decreased as the loading ratio increased, and the material and shape of the particles also had an effect on the particle velocity. Kovalev et al. [7] examined how different nozzles affected the kinematic properties of particles based on discrete particle trajectories, heat transfer models, and boundary conditions for the motion of partially slipping particles; to this aim, they used the third-order WENO finite-difference model proposed by Liu et al. [8] and mesh refinement in the region of irregularities and strong gradients. Bhattacharya et al. [9] compared the orders of magnitude of different forces on the particles in scaled micro-nozzles. The results of the study showed that the resistance was significantly greater than the other forces. Soliman et al. [10,11] investigated the effect of the particle size and pressure on the particle velocity in a jet using the finite volume method and second-order windward model, focusing on a two-phase flow in a vaccine-injected supersonic nozzle. Gilbert [12] derived the equations of motion for particles by implementing a linear relationship between the drag and velocity difference. The results showed that the particles had a small diameter and showed hysteresis effects in the reaction zone. Varaksin et al. [13] performed multiphase flow simulations with differently sized particles (polydisperse particles) and concluded that differently sized particles produce different velocities, affect the gas flow differently, and tend to collide with each other at lower concentrations. Yue et al. [14] used the CFD–DEM method to study the effect of the particle density on nozzle deflection under three conditions of constant fluidization velocity, amplified fluidization velocity, and particle density. Zhong et al. [15] used a CFD simulation to evaluate the impact of the fluid flow rate, particle size, sand volume ratio, and valve opening on the erosion and wear performance of a sleeve-type drain valve. Zhao et al. [16] simulated the influence of the injection pressure on the fire-extinguishing efficiency of fire-extinguishing agents. The results showed that the fire-extinguishing time decreased with the increase in the injection pressure. Xiao et al. [17] described the movement trajectories of ceramic slurry granules based on the CFD–DPM model and built a two-dimensional and three-dimensional axisymmetric mathematical model of the spray-drying process. Yang et al. [18] investigated the effect of a Laval nozzle structure on the flow characteristics of a supersonic gas–solid two-phase flow, and they concluded that various linear types of Laval nozzles exhibit excellent axial momentum for particle cores. Wu et al. [19] modeled CaO powders using the DPM method, investigated the effect of the carrier gas on mixing injection, and explored the gas–particle interaction. Fan et al. [20] developed a nozzle simulation model for the discrete particle method (DPM) to predict the air–powder flow under different diameters and divergence angles. Rahimi et al. [21,22] developed a mathematical model for the study of sulfur dioxide and carbon dioxide removal processes in a powder particle jet bed under non-isothermal operating conditions. They used a one-dimensional flow tube model and made many assumptions about the model. Li et al. [23] developed a numerical model to simulate a supersonic mixing jet in response to particle-induced shocks. They found that the powder significantly affected the oxygen jet by reducing the pressure wave sequence and showed that greater powder mass flow rates resulted in lower particle velocities. Chu et al. [24] numerically analyzed the effect of the solid loading rate on the gas–solid flow patterns and interaction forces (i.e., particle–gas, particle–particle, and particle–wall) in a cyclone separator by means of the CFD–DEM method. Lain et al. [25] numerically investigated the aerodynamic transport of spherical particles in a horizontal channel with a long rectangular cross-section. The calculations were carried out using the Eulerian–Lagrangian method with a gas-phase Reynolds stress turbulence model, taking into account the bi-directional coupling. The hydrodynamics and heat transfer of the gas–solid flow in a vertical pipe were numerically investigated by EI-Behery et al. [26]. The Euler–Lagrange model was used to simulate the gas–solid flow. Kabeel et al. [27] carried out an experimental and numerical computational study of solid particle injection into a turbulent crossflow under different operating conditions using the CFD–DEM method. The results showed that the inertia of the particles and the impact between the particles had a significant effect on the flow. a numerical simulation of a semi-dry desulfurization injection bed was performed by Wang et al. using the discrete element method (DEM) [28]. A numerical study of the effect of supersonic gas–solid jets on bypass spray dry powder extinguishing devices was performed by Zhang et al. [29].

In summary, the mechanism and flow characteristics of the gas–solid two-phase injection of nitrogen-driven dry powder particles, as well as the influence mechanisms of the driving pressure, dry powder pipe diameter, nozzle configuration, and loading ratio on dry powder injection, are not yet clear. Based on the above, this study establishes a computational fluid dynamics model for nitrogen-driven dry powder particle injection in a gas–solid two-phase flow. It explores the micro-effects of different driving pressures, pipe diameters, and nozzle configurations on dry powder injection. On this basis, a dry powder spraying experimental model is established to investigate the macroscopic spraying effect of dry powder fire extinguishing, which cannot be explored through the micro-mechanism of a gas–solid two-phase interaction. The dry powder spraying effect is investigated under different driving pressures, pipe diameters, nozzle configurations, and loading ratios. The simulation results are verified through experiments. Based on the single-factor experimental results, orthogonal experiments are designed to obtain the optimal parameter combination to achieve the best spraying effect. The research results can provide a theoretical and technical reference for the design and development of robotic controlled-pressure dry powder fire-extinguishing systems and related nitrogen-driven dry powder spraying fire-extinguishing devices.

2. Numerical Simulation of Nitrogen-Driven Gas–Solid Two-Phase Injection of Dry Powder Particles

2.1. Physical Model

Taking the dry powder pipe and nozzle of the fire-extinguishing device as the research object, we constructed a geometric model proportionally based on the actual size of the pressure-controlled fire-extinguishing device. The model consists of a pressure inlet, two dry powder pipes, a nozzle, and an external flow field. Among them, the vertical dry powder pipe is connected to the horizontal dry powder pipe through a right angle elbow, and the horizontal dry powder pipe is connected to the nozzle. The external flow field represents the calculation domain of the nozzle outlet. The geometric models of the dry powder pipe, nozzle, and external flow field are shown in Figure 3, and the specific dimensions of the geometric models are shown in

Table 2. Specific dimensions of geometry.

Parameter	Values
horizontal section dry powder pipe length	100 mm
vertical section dry powder pipe length	500 mm
dry powder tube diameter	10, 8, 6.5 mm
nozzle length	40 mm
external flow field length	300 mm
external flow field width	170 mm
nozzles	Straight, loudspeaker, three-hole

.

2.1.1. Control Equation

This study examines the flow characteristics of nitrogen-driven dry powder particle injection under supersonic conditions. The injection process is very short. For the two-dimensional high-speed viscous compressible ideal gas flow process, and according to the conservation relationship, the control equation is as follows.

Continuity equation:

$$\frac{\partial \rho }{\partial t}+div\left(\rho u\right)=0$$

(1)

Momentum conservation equation:

$$\frac{\partial \left(\rho u\right)}{\partial t}+div\left(\rho u\mathbf{u}\right)=div\left(\mu \nabla u\right)-\frac{\partial p}{\partial x}+S_u$$

(2)

$$\frac{\partial \left(\rho v\right)}{\partial t}+div\left(\rho v\mathbf{u}\right)=div\left(\mu \nabla v\right)-\frac{\partial p}{\partial y}+S_v$$

(3)

Energy conservation equation:

$$\frac{\partial \left(\rho T\right)}{\partial t}+div\left(\rho \mathbf{u}T\right)=div\left(\frac{k}{C_p}\nabla T\right)+S_T$$

(4)

Here, ρ is the gas density, kg/m³; u is the gas-phase velocity; u, v are the partial velocities of the gas-phase velocity in u in the x, y directions, m/s, respectively; μ denotes the dynamic viscosity, pa-s; k denotes the heat transfer coefficient of the fluid, W/(m²-K); Cp denotes the specific heat capacity, J/(kg-K); and S denotes the source term. Considering the compressibility of supersonic high-pressure nitrogen, the ideal gas state equation is

$$\mathbf{p}\mathbf{v}=\rho \mathrm{RT}$$

(5)

Here, R represents the gas constant, and T represents the temperature.

2.1.2. Interphase Interactions

In the dense gas–solid two-phase flow field accompanied by shock waves, particles are subjected to various forces. Due to the small ratio of the nitrogen density to the dry powder particle density, the size of the dry powder particles is 50 μm. However, the velocity of the dry powder particles is relatively high, so the effects of the Basset force, Magnus force, and Saffman force can be ignored, with a focus on the fluid resistance, pressure gradient force, virtual mass force, and particle collision force.

In the existing models, the fluid resistance (drag) experienced by particles can be expressed as

$$F_{qs}=\frac{\pi }{8}{d}_p^2{\rho }_f{C}_D\left(v_f-v_p\right)\left|v_f-v_p\right|$$

(6)

In the formula, dp is the particle diameter; ρf is the gas density; CD is the drag coefficient; vf is the velocity of fluid motion; and vp is the velocity of particle motion.

When there is a pressure gradient in the flow field, the particles will be subjected to a force caused by the pressure gradient, which is expressed as

$$F_{pg}=-V_p\cdot \frac{\partial p}{\partial x}$$

(7)

In the formula, Vp represents the volume of the particles.

As the particles accelerate in the flow field, they also drive the surrounding fluid to accelerate. The expression of the force that increases the kinetic energy of a fluid is

$$F_{am}=K_m{\rho }_f{V}_p\left(\frac{d{v}_f}{dt}-\frac{d{v}_p}{dt}\right)$$

(8)

In the formula, Km is the correction coefficient; for spherical particles, Km is taken as a constant of 0.5.

2.1.3. Turbulence Model

Due to the large Reynolds number in the computational flow field, turbulence effects need to be considered. The standard k-ω model is a turbulence model based on the model transport equations for turbulent kinetic energy (k) and the specific dissipation rate (ω). The k-ω model has been corrected several times to include a generating term in the k and ω equations, which improves the accuracy of the model in predicting a free shear flow. Its transport equation is

$$\frac{\partial }{\partial t}\left(\rho k\right)+\frac{\partial }{\partial x_i}\left(\rho k{u}_i\right)=\frac{\partial }{\partial x_j}\left({\mathrm{\Gamma }}_k\frac{\partial k}{\partial x_j}\right)+G_k-Y_k+S_k+G_b$$

(9)

$$\frac{\partial }{\partial t}\left(\rho \omega \right)+\frac{\partial }{\partial x_i}\left(\rho \omega u_i\right)=\frac{\partial }{\partial x_j}\left({\mathrm{\Gamma }}_{\omega }\frac{\partial \omega }{\partial x_j}\right)+G_{\omega }-Y_{\omega }+S_{\omega }+G_{\omega b}$$

(10)

where $G_k$ denotes the turbulent kinetic energy produced by the mean velocity gradient. $G_k$ represents the generation term for ω. ${\mathrm{\Gamma }}_{\omega }$ and ${\mathrm{\Gamma }}_{\omega }$ denote the effective diffusion coefficients for k and ω, respectively. $Y_k$ and $Y_{\omega }$ denotes the dissipation due to turbulence k and ω. $S_k$ and $S_{\omega }$ denote the customized source terms. $G_b$ and $G_{\omega b}$ denote the buoyancy terms as described in previous work on the consideration of buoyancy effects on turbulence in the k-ω model. On the basis of the standard k-ω model, the SST k-ω model introduces a mixing function inside the boundary layer, using the k-ω model near the wall and the k-ε model in the far field, making it more adaptable under high-Reynolds-number flow conditions. To ensure the computational efficiency and accuracy of the model, this study adopts the SST k-ω model, which is more accurate and suitable for simulation calculations.

2.1.4. Discrete-Phase DPM Model

In order to more intuitively analyze the dynamic characteristics of particles under different working conditions, this study takes the Euler–Lagrange model as the research framework, uses the Euler model to calculate the continuous-phase driving gas N₂, and uses the DPM model to carry out the numerical simulation of the discrete-phase dry powder particles. The equilibrium equation for the forces acting on the particles during their motion is as follows:

$$\frac{d{u}_p}{dt}=\frac{\left(\mathbf{u}-{\mathbf{u}}_p\right)}{{\tau }_p}+Fd$$

(11)

Here, up represents the velocity of the particle phase, u represents the velocity of the gas phase, and F represents the acceleration force of the particle and the non-uniform force of the flow field. τp represents the particle relaxation time, and its calculation formula is as follows:

$${\tau }_p=\frac{{\rho }_p{d}_p^2}{18\mu C_D{R}_e}$$

(12)

Here, dp represents the particle diameter, μ represents the gas-phase dynamic viscosity, CD represents the particle drag coefficient, and Re represents the particle-phase Reynolds number. The equation for the definition of the Reynolds number is

$$R_e=\frac{\rho d_p\left|\mathbf{u}-{\mathbf{u}}_p\right|}{\mu }$$

(13)

The trajectory calculation model for DPM particles is based on Newton’s second law equation as follows:

$$F=ma=m\frac{\Delta v}{\Delta t}$$

(14)

In the formula, F is the sum of the frictional and inertial forces between the fluid and particles. Due to the magnitude of gravity being much smaller than the inertial forces and resistance, the influence of gravity is ignored:

$$F=F_{drag}+F_{intertia}$$

(15)

The resistance between the fluid and particles is calculated using the Stokes formula, which is as follows:

$$F_{drag}=-\frac{d_p}{F_s}\left(\mathbf{V}-\mathbf{U}\right)$$

(16)

In the formula, dp represents the diameter of the particle, U represents the velocity of the fluid, and the shear stress Fs depends on the viscosity of the fluid and the Reynolds number of the particle. The calculation formula for Fs is

$$F_s=\frac{1}{\pi \mu 3C}$$

(17)

For high-Reynolds-number particles, $C=1+0.179R_{ep}^{0.5}+0.013R_{ep}$, where Rep is the particle’s Reynolds number.

2.1.5. Parameter Settings

Using N₂ as the driving gas, the particles are idealized as spherical, inert particles with a uniform particle size. The specific boundary conditions and material property parameters are shown in

Table 3. Boundary conditions.

Parameter	Values
Environmental pressure P/MPa	0.1
Nitrogen driving pressure P0/MPa	0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9
Ambient temperature T/K	300
Injection particle temperature Tp/K	300
Injection particle velocity Vp/(m·s−1)	10
Injecting particle mass flow rate mp/(kg·s−1)	0.26
Initial temperature of nitrogen/°C	300

and

Table 4. Material property parameters.

Material	Parameter Settings
Nitrogen	Density	Set as ideal gas
	Viscosity coefficient	Assuming compliance with Sutherland’s law
Dry powder fire-extinguishing agent	Particle size/μm	50

.

2.2. Analysis of Mechanism and Influence of Gas–Solid Two-Phase Flow in Dry Powder Injection under Different Driving Pressure Conditions

Numerical simulations were conducted using a 10-mm-diameter dry powder tube and a straight nozzle under different driving pressure conditions. The distribution of the Mach number contour lines for the gas–solid two-phase flow under a nitrogen drive at (0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9) MPa is shown in Figure 4, with all injected dry powder particles flowing through the entire computational flow field as the stopping point for the numerical simulation. As shown in Figure 4, there are obvious shock waves in the external flow field, and the velocity in the core area of the gas-phase jet is much higher than the velocity of the surrounding jet. As the injection distance increases, the Mach number gradually decreases, and the gas phase velocity gradually decreases. The maximum gas phase velocity is located at the central axis position of the dry powder tube. As the driving pressure increases, the influence of the Mach number distribution becomes greater. The core area of the jet increases, and the velocity of the core area also increases. The shock wave intensity continues to increase. When the driving pressure is 0.9 MPa, the maximum Mach number can reach 2.8. The difference between the Mach number contour lines of the pure gas phase and the driving pressure of 0.3 MPa is not significant.

Figure 5 shows the static pressure distribution cloud diagram of gas–solid two-phase flow driven by nitrogen at different pressures. From the figure, the following can be analyzed: at the nozzle mouth, the static pressure is relatively high; in the external flow field, it is in the obvious surge, and at the same time, there is the distribution of negative pressure, which is due to the excessive velocity of the gas phase, resulting in the generation of negative pressure, the generation of negative pressure, resulting in the gas compression, which produces a surge. Through the comparison of the static pressure distribution cloud diagram under different nitrogen pressures, it is found that the static pressure cloud diagram under the gas phase and 0.3 MPa is not much different. With the increase in nitrogen pressure, it can be found that the intensity of the shock wave is obviously strengthened, and the negative pressure area of the external flow field is also getting bigger. Meanwhile, the positive pressure area and the value of the pressure are also getting bigger, and the positive pressure and the negative pressure are appearing alternately; this is due to the increase in nitrogen pressure. The velocity of the gas phase of the two-phase flow is also increasing, which leads to the negative pressure being generated more easily and the area of negative pressure being produced more easily. This is due to the increase in nitrogen pressure and the increase in gas phase velocity of two-phase flow, which leads to negative pressure more easily; the area becomes larger, and the gas compression is more intense.

Figure 6 shows the velocity distribution cloud diagram of particles in gas–solid two-phase flow with different pressures, defining the particle velocity as V. From the figure, it can be seen that in the external flow field, the overall velocity of particles increases with the increase in driving pressure, and the particle velocity V can reach 430 m/s at 0.9 MPa, and the range of the core jet of dry powder particles in the external field decreases, and the range of the core jet in the external field is largest and most uniformly distributed in the dry powder particles at 0.3 MPa. is the largest and the velocity distribution is the most uniform. Under 0.9 MPa, the core jet range of dry powder particles in the outer flow field is the smallest, and the difference in the velocity of dry powder particles is relatively large. The velocity of dry powder particles in the central area can reach 430 m/s, and the velocity of the outermost particles is reduced to 300 m/s, and the maximum difference can be up to 130 m/s. For the dry powder fire extinguishing, the larger the extinguishing area of the dry powder jet is, the better. From the numerical simulation, under 0.3 MPa, the dry powder particles have the widest distribution in the outer flow field and the most uniform velocity distribution, which has a good spraying effect.

2.3. Analysis of Mechanism and Influence of Gas–Solid Two-Phase Flow in Dry Powder Injection under Different Pipe Diameter Conditions

Numerical simulations were conducted using a 0.3 MPa nitrogen-driven and straight nozzle under (6.5, 8, 10) mm pipe diameter conditions.

Figure 7 shows the Mach number contour lines of the gas–solid two-phase flow under different pipe diameter conditions. It can be seen from the figure that there is a shock wave phenomenon in the external flow field, and the velocity in the core area of the jet varies greatly. As the diameter of the dry powder pipe increases, the shock wave intensity increases, the core jet area becomes longer, and the Mach number in the core area also increases.

Figure 8 shows the static pressure distribution cloud map of the gas–solid two-phase flow under different pipe diameters. It can be seen from the figure that there are obvious shock waves and negative pressure in the external flow field. As the pipe diameter increases, the range of the shock waves’ influence in the external flow field becomes larger, and the negative pressure area also becomes larger, causing the shock wave position to move downward.

Figure 9 shows the particle velocity distribution cloud map of the gas–solid two-phase flow under dry powder pipes of different diameters. As shown in the figure, as the pipe diameter increases, the overall particle velocity increases. When the pipe diameter is 10 mm, the particle velocity in the central area of the external flow field is significantly higher than that in the outer layer, which may be due to the presence of obvious shock waves in the external flow field. When the diameter of the dry powder pipe is 8 mm, the particle velocity distribution in the external flow field is the most uniform and the dry powder distribution is the most concentrated, with a large diffusion range for the dry powder. When the diameter of the dry powder pipe is 6.5 mm, the particle distribution in the external flow field is relatively dispersed, which may be caused by the unstable radial static pressure and gas-phase velocity distribution.

2.4. Analysis of Mechanism and Influence of Gas–Solid Two-Phase Flow in Dry Powder Injection under Different Nozzle Configurations

Numerical simulations were conducted on a 10-mm-diameter dry powder tube driven by 0.3 MPa nitrogen gas under different nozzle configurations. The schematic diagram of the nozzle is shown in Figure 10. The distribution of the Mach number contour lines for the gas–solid two-phase flow under three types of nozzles is shown in Figure 11. It can be seen from the figure that when using a three-hole nozzle, the Mach number of the external flow field is the smallest and the shock wave is not obvious. When using a straight nozzle, the shock wave in the external flow field is obvious, the core area of the jet is the longest, and the gas velocity in the core area is the highest. When using a horn nozzle, the shock wave intensity is smaller, and the core area of the jet is shorter as compared to when a straight nozzle is used.

Figure 12 shows the static pressure distribution cloud map of the gas–solid two-phase flow under different nozzles. It can be seen from the figure that when the nozzle is of a straight mouth type, the shock wave is most obvious in the external flow field, and the negative pressure area is the widest. When the nozzle is horn-shaped, there is a shock wave distribution and negative pressure area at a certain distance in the external flow field. As the injection distance increases, the static pressure remains almost unchanged. When the nozzle is of a three-hole type, there are only shock waves and negative pressure areas at the nozzle outlet.

Figure 13 shows the distribution cloud map of the particle velocity in a two-phase flow under different nozzles. It can be seen from the figure that when the nozzle is of a three-hole type, the dry powder particle velocity is relatively small, at approximately 200 m/s; the particle distribution range is the smallest; and the outer layer particle distribution is relatively dispersed. When the nozzle is of a straight mouth type, the dry powder particle velocity is relatively high, at approximately 310 m/s, and there is a certain difference in the particle velocity between the core area and the outer layer. The distribution of the dry powder particles in the outer layer is relatively scattered, which may be due to the uneven distribution of the radial gas-phase velocity and static pressure at the nozzle outlet and the strong shock wave received. When the nozzle is of a horn type, the particle velocity is relatively uniform, at approximately 260 m/s, with a wide particle distribution that is more uniform for the outer dry powder particles. From the distribution cloud map of the particle velocity in the two-phase flow, it can be seen that when the nozzle is of a horn type, its dry powder spraying effect is the best and most conducive to fire extinguishing.

3. Experimental Study on the Macroscopic Effect of Dry Powder Spraying

Through the numerical simulation of the gas–solid two-phase flow in dry powder injection, the microscopic mechanism of the gas–solid two-phase flow was explored. However, the macroscopic effects of different technical parameters on nitrogen-driven dry powder injection, including the effective farthest distance, effective fire-extinguishing area, and average dry powder intensity, are still unclear, and experimental research is needed to determine their effectiveness.

3.1. Material and Methods

The apparatus used in this experiment mainly includes a dry powder collection box (L × W × H: 17 cm × 11 cm × 4 cm), funnel, high-speed camera (Figure 14), tape measure, and regulated DC power supply (Figure 15). The high-speed camera is used to record the dry powder spray, the tape measure is used to measure the distance, and the regulated DC power supply is used to provide power. The extinguishing agent used in the dry powder spraying experiments in this paper is ABC dry powder extinguishing agent, which comes from the extinguishing agent produced by Zhong’an Fire Protection, with a particle size of 50 μm and a bulk density of 0.5 g/mL.

3.2. Experimental Model Construction

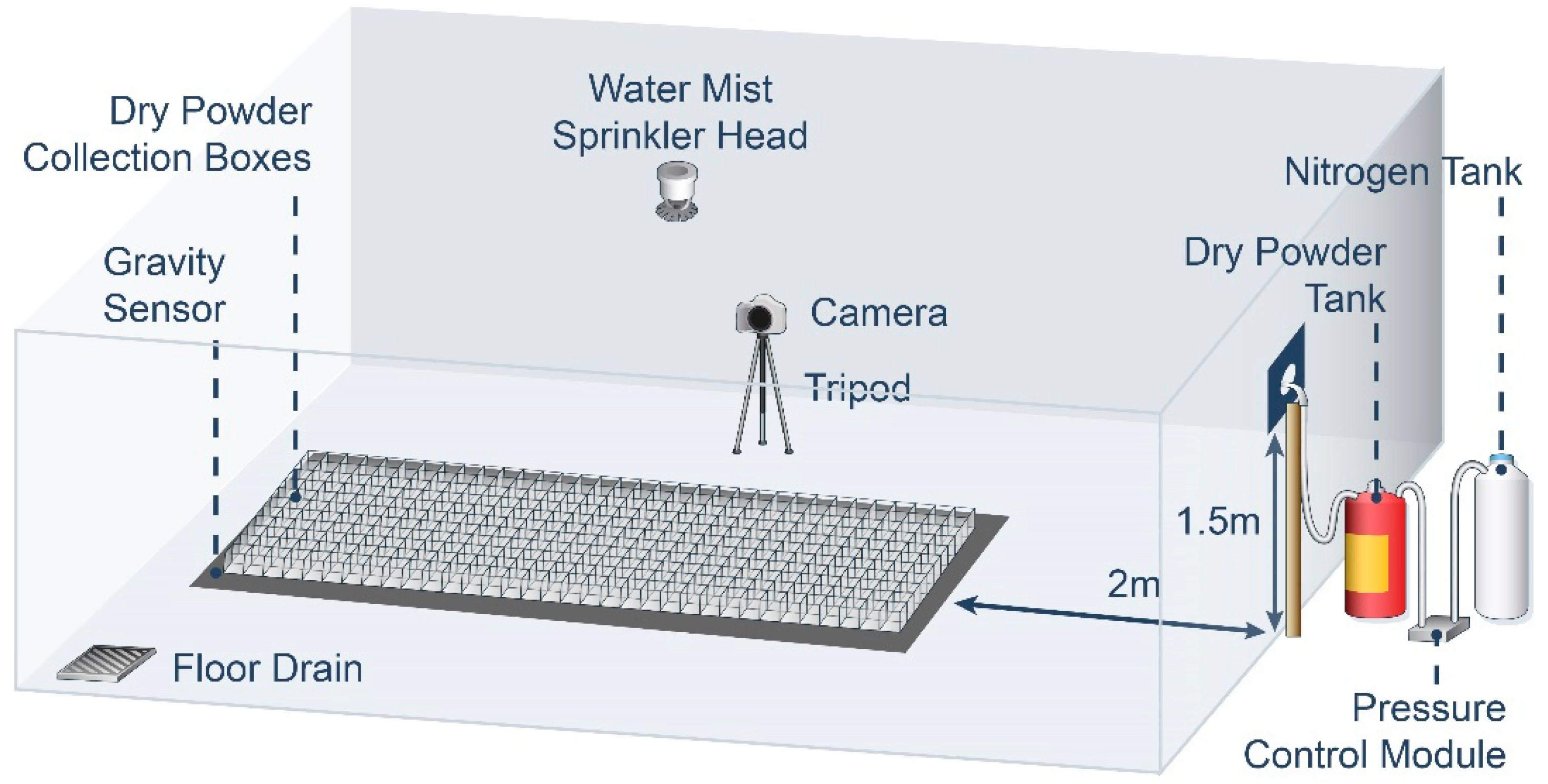
The dry powder spraying experimental device mainly consists of a dry powder tank, nitrogen tank, pressure-reducing valve, electromagnetic proportional valve, ball valve, dry powder collection box (L × W × H: 17 cm × 11 cm × 4 cm), gravity sensors, water spray nozzle, and other components. A diagram of the experimental device and the dry powder box setup is shown in Figure 16. The device nozzle is positioned 1.5 m from the ground; the nozzle is kept horizontal and is placed 2 m directly in front of the dry powder collection box consisting of 11 columns × 34 rows. The plane schematic diagram is shown in Figure 17. The nozzle outlet is defined as the origin, the horizontal direction of the center axis for the X-axis, and the vertical direction for the Y-axis. The sweeping device adopts a high-pressure fine mist water gun to carry out dust reduction and rinsing, and there is a filter screen at the floor drain to collect waste dry powder so as to reduce the amount of environmental pollution. The gravity sensor determines the quality of the sprayed dry powder in real time and feeds the result back to the computer.

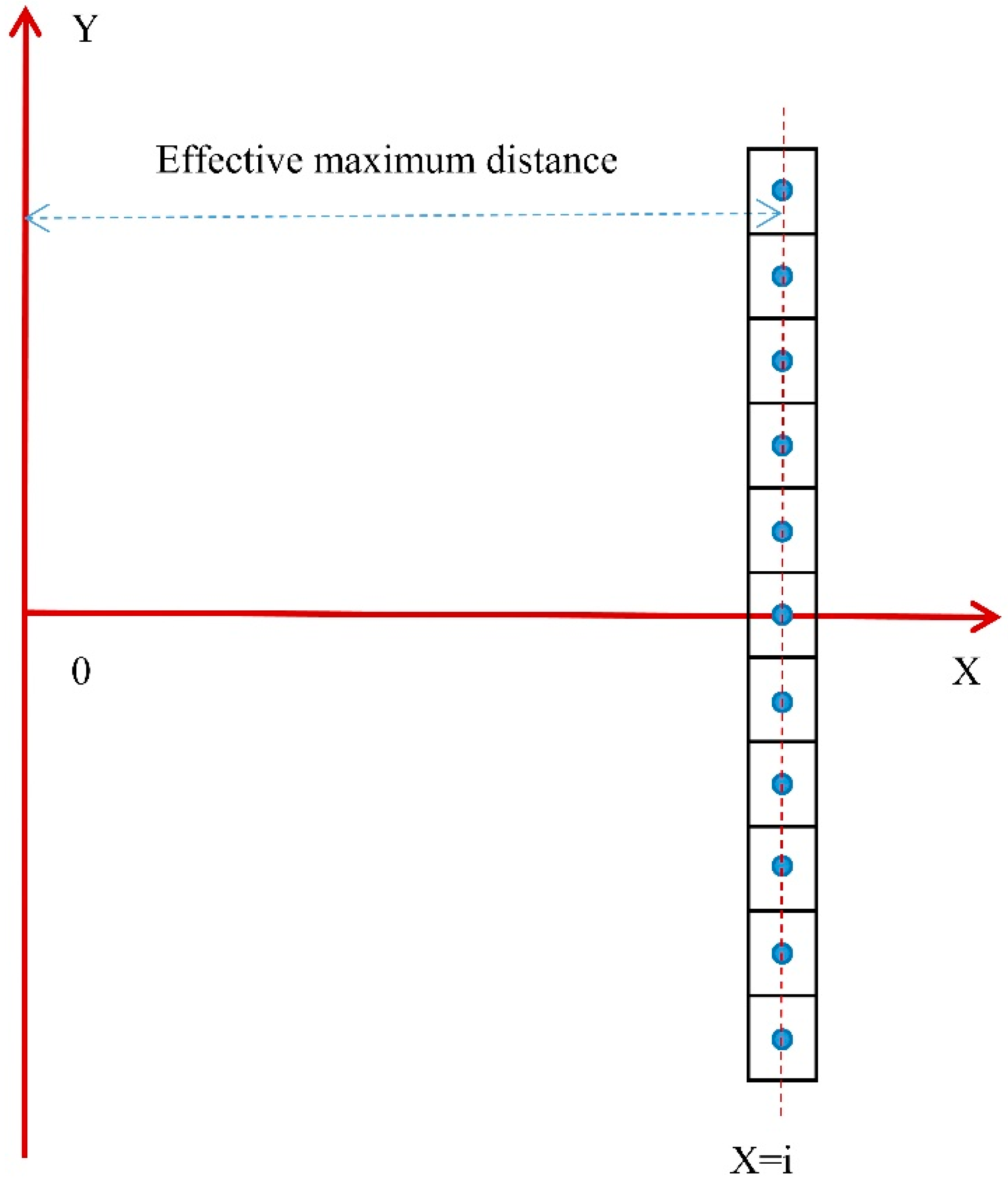
This study examines 374 dry powder collection boxes as 374 points (the schematic diagram of the plan was shown in Figure 18), and the dry powder quality at the center point of each dry powder collection box represents the dry powder quality inside the collection box. If the total dry powder mass at the point on the X = i axis is the largest, then the horizontal distance from the X = i axis to the origin 0 is the effective farthest distance of the fire-extinguishing device. The indicator diagram is shown in Figure 19.

By weighing each dry powder box, the average dry powder strength R in the area where each dry powder collection box is located can be calculated.

$$R=\frac{m}{St}$$

(18)

In the formula, R is the average strength of the dry powder, g/m²·s; M is the mass of the dry powder in the dry powder box, g; S is the bottom area of a dry powder box, 0.0187 m²; T is the time required for one dry powder injection, 10 s.

3.3. Experimental Study on Effect of Dry Powder Injection under Different Injection Pressure Conditions

Using a straight nozzle and a 10-mm-diameter dry powder pipe, we conducted dry powder spraying experiments under different driving pressures. The effective farthest distance results are shown in Table 4 and Figure 20.

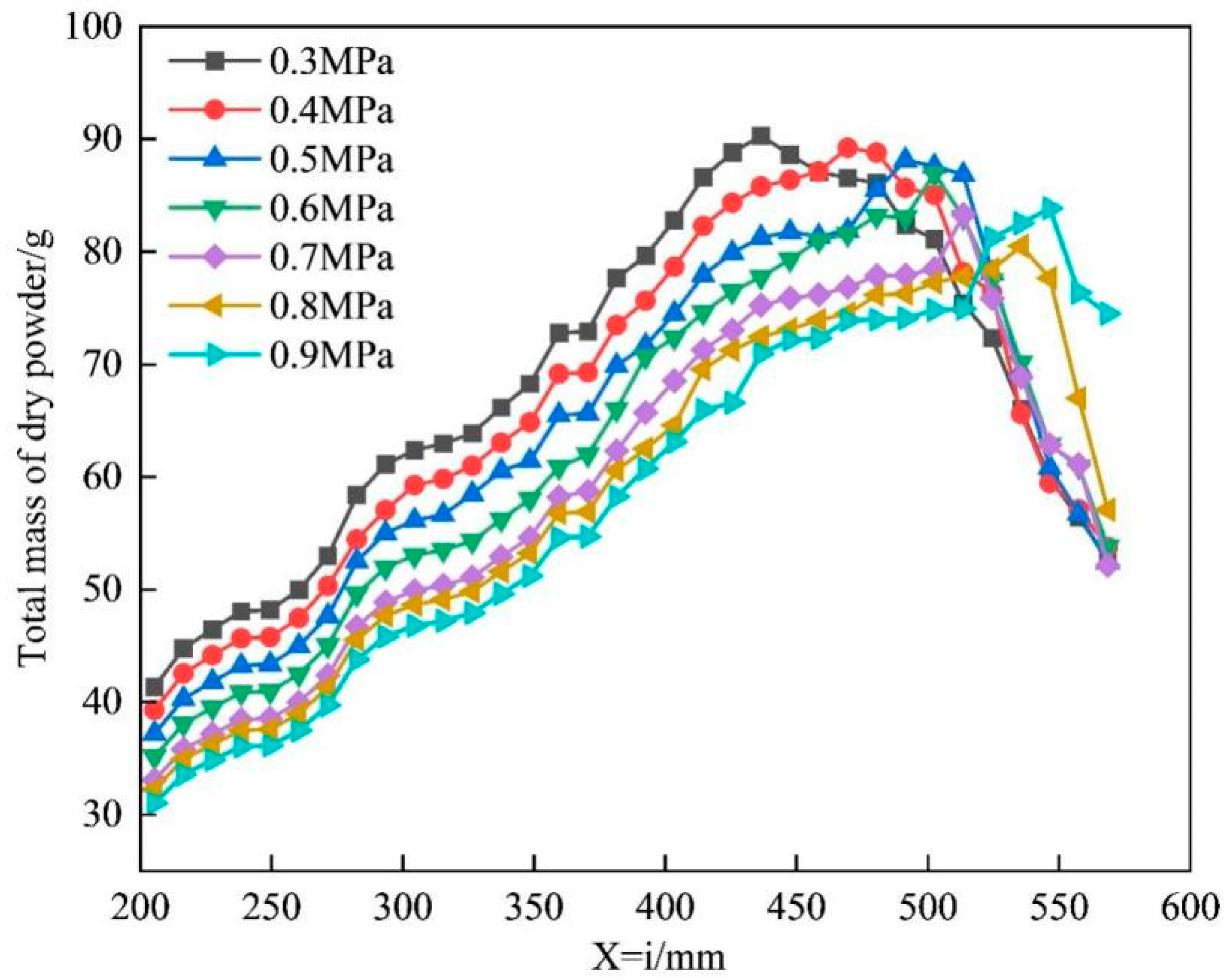
According to

Table 5. Comparison of the effective maximum distance under different pressures.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Farthest Effective Distance/mm
0.3	Straight mouth type	10	4365
0.4	Straight mouth type	10	4695
0.5	Straight mouth type	10	4915
0.6	Straight mouth type	10	5025
0.7	Straight mouth type	10	5135
0.8	Straight mouth type	10	5355
0.9	Straight mouth type	10	5465

, as the nitrogen driving pressure increases, the effective maximum distance of the fire-extinguishing device gradually increases.

Figure 20 shows the total mass curve of the dry powder on the X = i axis under different pressures. It can be seen from the graph that as the i value increases, the total mass of the dry powder on the axis first increases and then decreases. The peak value of the curve is the effective farthest fire-extinguishing distance. In the interval before reaching the peak, as the pressure increases, the total mass of the dry powder on the axis decreases.

The results for the effective fire-extinguishing area are shown in

Table 6. Comparison of effective fire-extinguishing areas under different pressures.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Effective Fire-Extinguishing Area/cm2
0.3	Straight mouth type	10	8415
0.4	Straight mouth type	10	8041
0.5	Straight mouth type	10	7854
0.6	Straight mouth type	10	7480
0.7	Straight mouth type	10	7293
0.8	Straight mouth type	10	7106
0.9	Straight mouth type	10	6732

and Figure 21.

Table 6 shows the comparison of the effective fire-extinguishing area under different pressures. It can be seen from the table that as the nitrogen driving pressure increases, the effective fire-extinguishing area gradually decreases. When the driving pressure increases from 0.3 MPa to 0.9 MPa, the effective fire-extinguishing area decreases by 1683 cm².

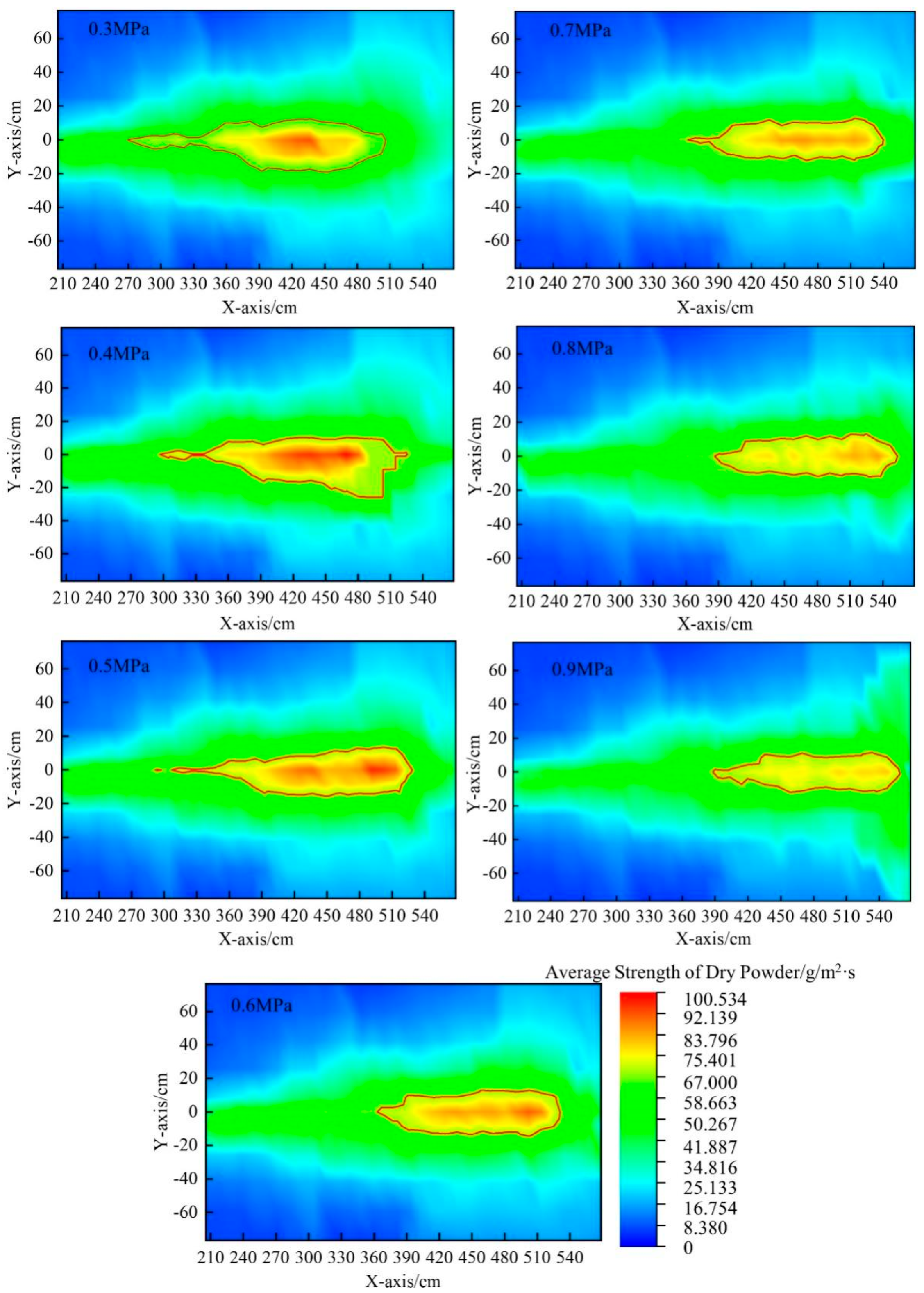
Figure 21 shows the cloud map of the average strength of the dry powder under different pressures. It is found that 67 g/m²·s is the critical average strength of the dry powder, and the red line in the cloud map is the contour line of 67 g/m²·s. The area within the red line is defined as the effective fire-extinguishing area. As shown in the figure, the dry powder diffuses in a “trumpet” shape, while the effective fire-extinguishing area forms a “V” shape. As the pressure increases, the average dry powder intensity of the effective fire-extinguishing area decreases, and the effective fire-extinguishing area moves backward, resulting in a reduction in the area.

In summary, the experimental and numerical simulation results are consistent. The shock wave intensity and influence range in the external flow field of the gas–solid two-phase flow increase, which affects the landing of the dry powder particles and leads to a decrease in the average dry powder intensity in the effective fire-extinguishing area. Based on the simulation and experiments, it is concluded that dry powder injection has the best effect at 0.3 MPa.

3.4. Experimental Study on Effect of Dry Powder Spraying under Different Pipe Diameter Conditions

Dry powder injection experiments were conducted on three different pipe diameters using a 0.3 MPa nitrogen-driven straight nozzle. The effective farthest distance results are shown in

Table 7. Most effective distances under different pipe diameters.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Farthest Effective Distance/mm
0.3	Straight mouth type	10	4365
0.3	Straight mouth type	8	4255
0.3	Straight mouth type	6.5	4035

and Figure 22.

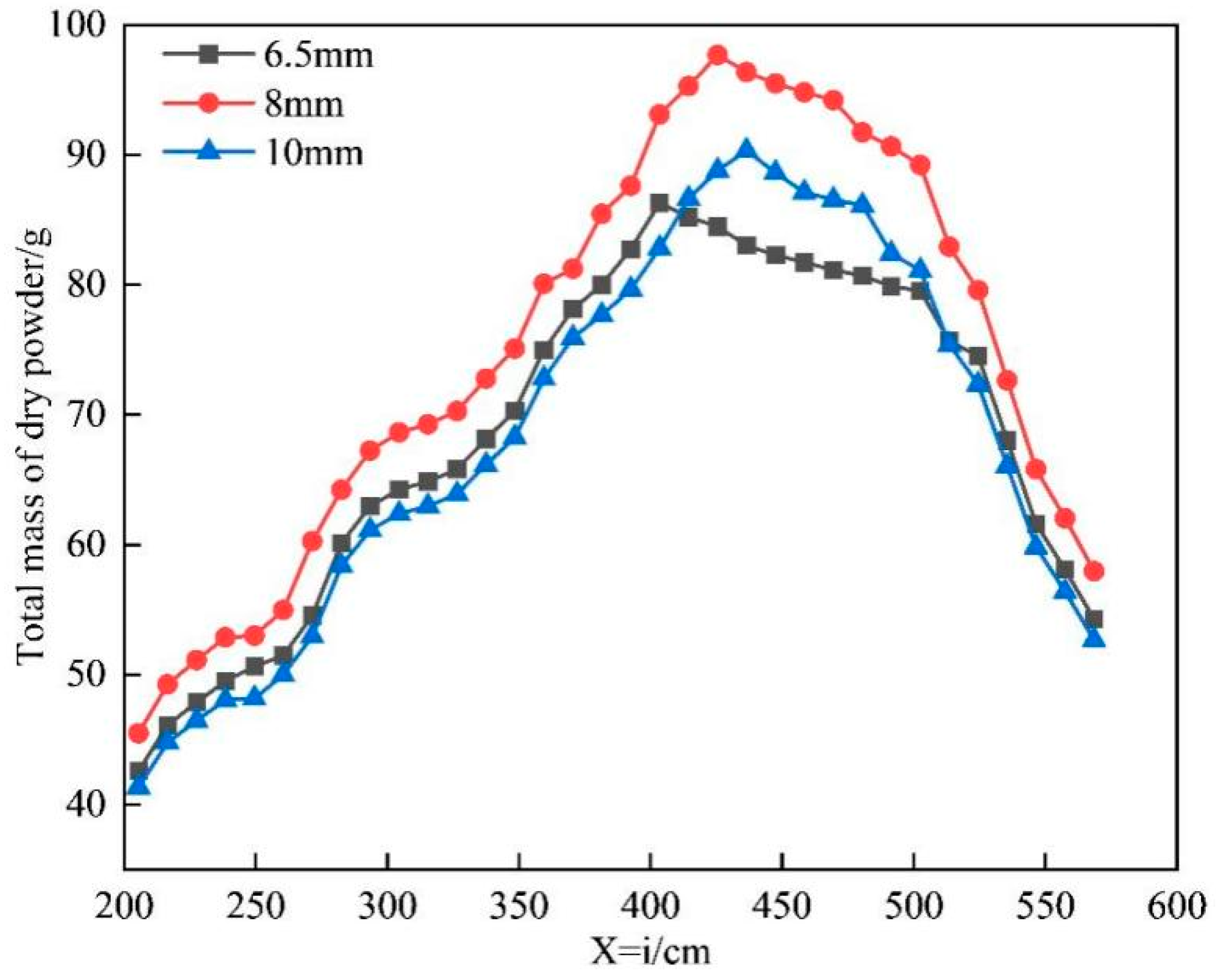
Table 7 shows the comparison of the maximum effective distances of the fire-extinguishing devices under different pipe diameters. It can be seen from the table that as the diameter of the dry powder pipe increases, the maximum effective distance of the fire-extinguishing device gradually increases.

Figure 22 shows the total mass curve of the dry powder on the X = i axis under dry powder pipes with different diameters. It can be seen from the figure that as the i value increases, the total mass of the dry powder on the line first increases and then decreases. Through the comparison, it can be found that, within the range of 200–400 cm, when the pipe diameter is 8 mm, the total mass of the dry powder on the axis is greater than that under other pipe diameters. When the pipe diameter is 10 mm, the total mass of the dry powder on the axis is the lowest.

The results for the effective fire-extinguishing area are shown in

Table 8. Comparison of effective fire-extinguishing areas under different pipe diameters.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Effective Fire-Extinguishing Area/cm2
0.3	Straight mouth type	10	8415
0.3	Straight mouth type	8	10,472
0.3	Straight mouth type	6.5	8976

and Figure 23.

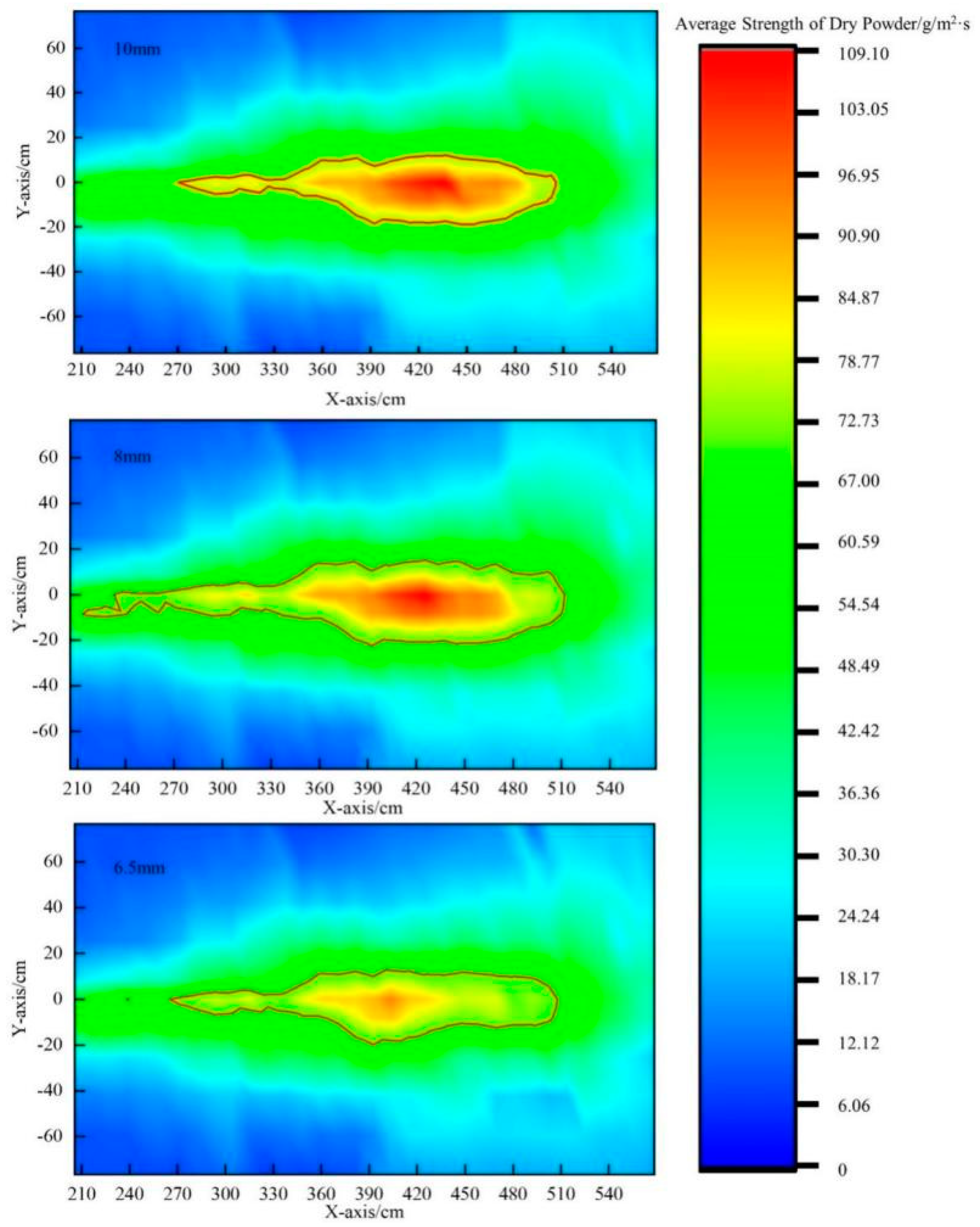
Table 8 shows the comparison of the effective fire-extinguishing area under dry powder pipes of different diameters. When the pipe diameter is 8 mm, the effective fire-extinguishing area is the largest, and when the pipe diameter is 10 mm, the effective fire-extinguishing area is the smallest, with a difference of 2057 cm² between the two.

Figure 23 shows the average strength cloud map of the dry powder under different pipe diameters. It can be seen from the figure that the dry powder diffuses in a “horn” shape, and the effective fire-extinguishing area has a “V” shape. When the diameter of the dry powder pipe is 8 mm, the average strength of the dry powder in the effective fire-extinguishing area is relatively high.

In summary, the experimental results are consistent with the numerical simulation results for the gas–solid two-phase flow in dry powder pipes with different diameters. Under a diameter of 8 mm, the shock wave intensity in the external flow field is smaller than that under a diameter of 10 mm. The gas velocity distribution is the most uniform at the nozzle outlet, which is more conducive to particle landing. When the diameter of the dry powder pipe is 8 mm, the best dry powder spraying effect is achieved.

3.5. Experimental Study on Effect of Dry Powder Injection under Different Nozzle Configurations

Dry powder injection experiments were conducted on different nozzle configurations using a 10 mm dry powder tube driven by 0.3 MPa nitrogen gas. The effective farthest distance results are shown in

Table 9. Effective maximum distance under different nozzles.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Farthest Effective Distance/mm
0.3	Straight mouth type	10	4365
0.3	Horn type	10	4255
0.3	Three-hole type	10	3045

and Figure 24.

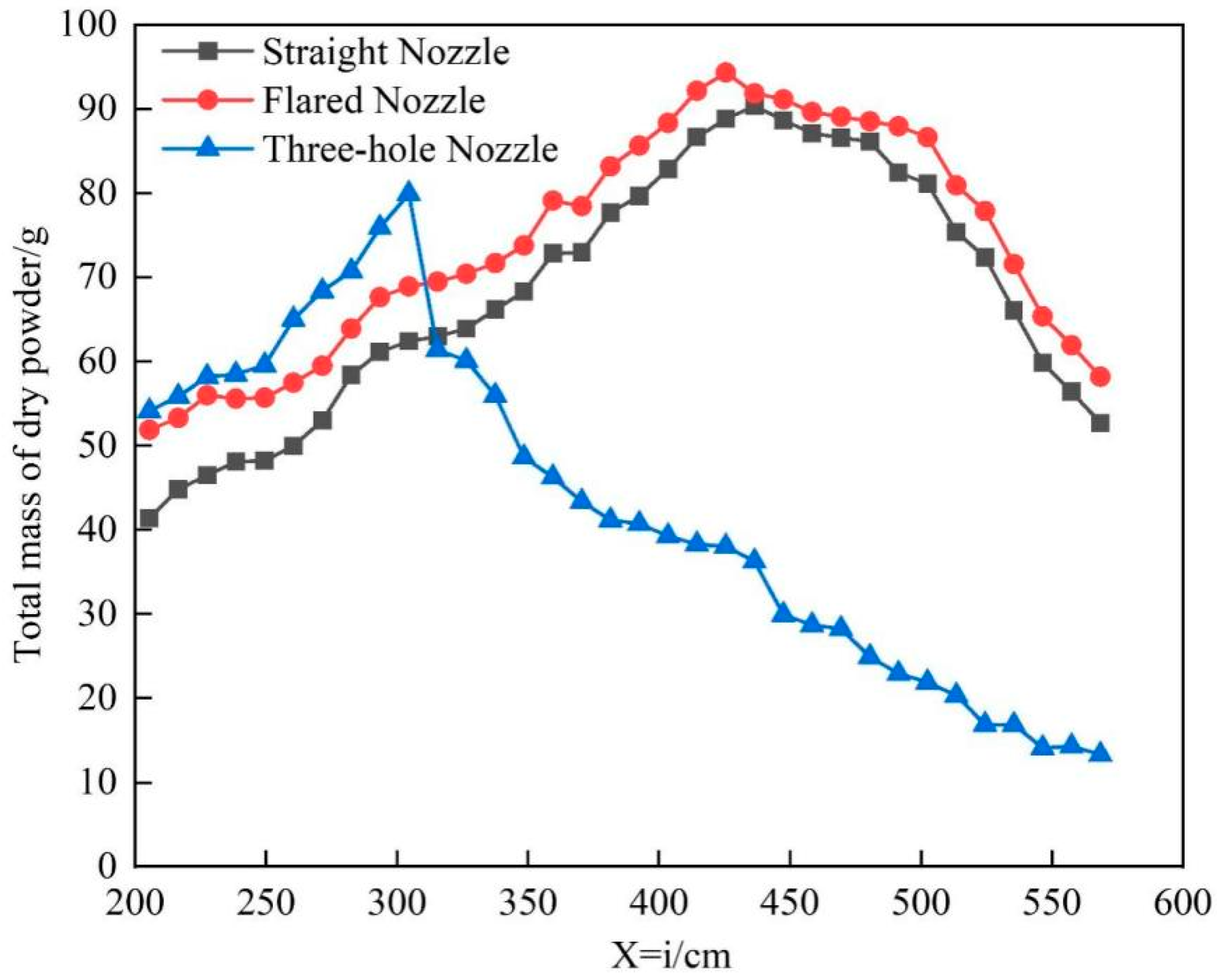
Table 9 shows a comparison of the maximum effective distance under different nozzles. When the nozzle is of a straight mouth type, the maximum effective distance is 436.5 cm, and under a three-hole-type nozzle, the maximum effective distance is 304.5 cm.

Figure 24 shows the total dry powder mass curve on the X = i axis under different nozzles. It can be seen from the figure that as the i value increases, the total dry powder mass on the axis first increases and then decreases. When the nozzle is of a three-hole type, the peak of the total dry powder mass curve on the axis appears the earliest. When the nozzle is of a horn type, the total dry powder mass on the axis is greater than that for the straight mouth type.

The results for the effective fire-extinguishing area are shown in

Table 10. Comparison of effective fire-extinguishing areas under different nozzles.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Pipe Diameter/mm	Effective Fire-Extinguishing Area/cm2
0.3	Straight mouth type	10	8415
0.3	Horn type	10	9163
0.3	Three-hole type	10	1683

and Figure 25.

Table 10 shows the comparison of the effective fire-extinguishing areas of the fire-extinguishing devices under different nozzles. When the nozzle is of a three-hole type, the effective fire-extinguishing area is the smallest. When the nozzle is of a horn type, the effective fire-extinguishing area is the largest, with a difference of 7480 cm² between the two.

Figure 25 shows the average intensity cloud map of the dry powder under different nozzles. When the nozzles are horn-shaped and straight-mouthed, the diffusion of the dry powder takes on a “horn” shape, and the effective fire-extinguishing area takes on a “V” shape. The effective fire-extinguishing area of the three-hole nozzle is the smallest, and the difference in the fire-extinguishing area between the straight-mouthed nozzle and the horn nozzle is not significant. Regarding the effective fire-extinguishing area of the horn type, the area with an average dry powder intensity greater than 90 g/m²s is greater than that with the straight-mouthed nozzle.

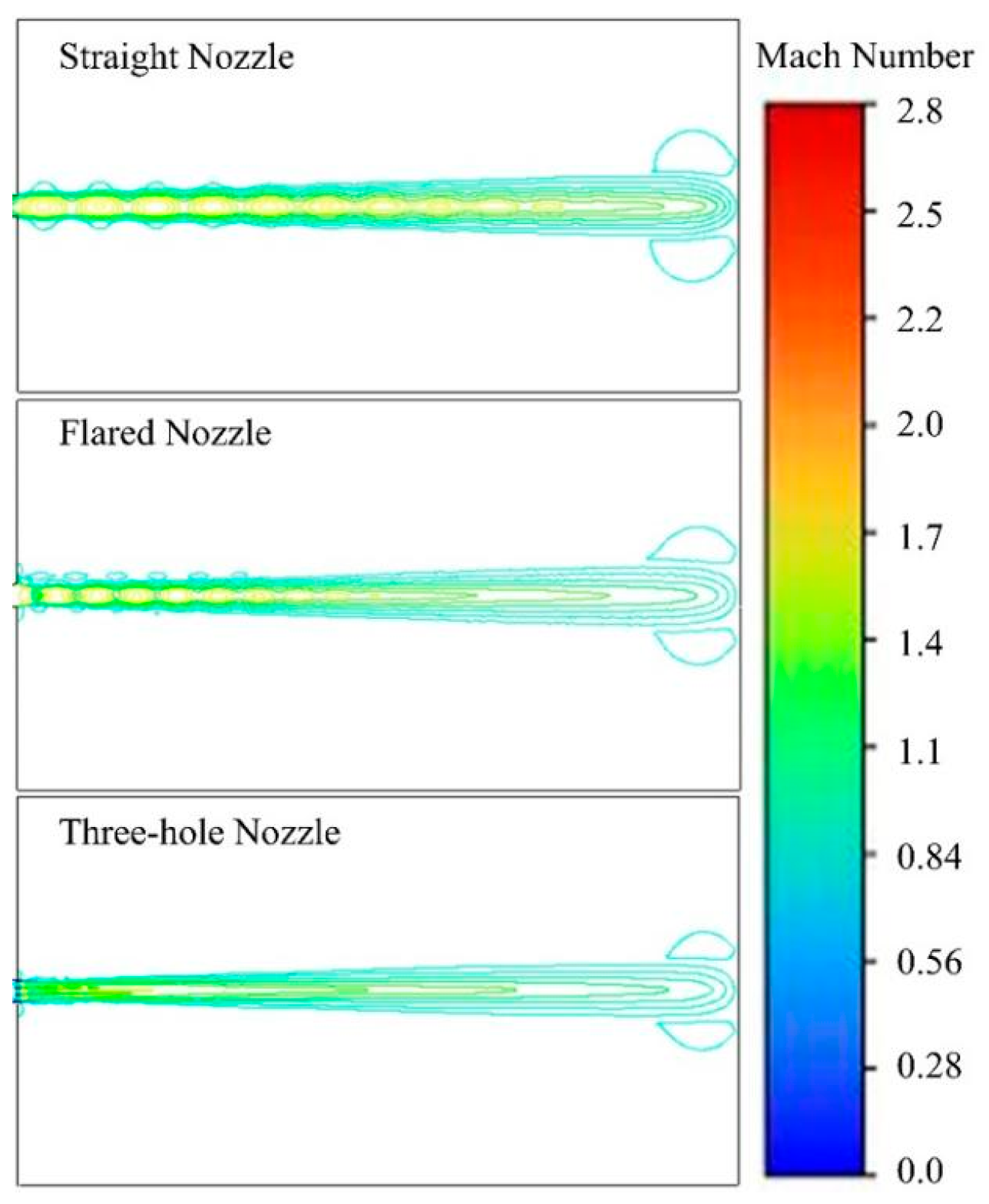
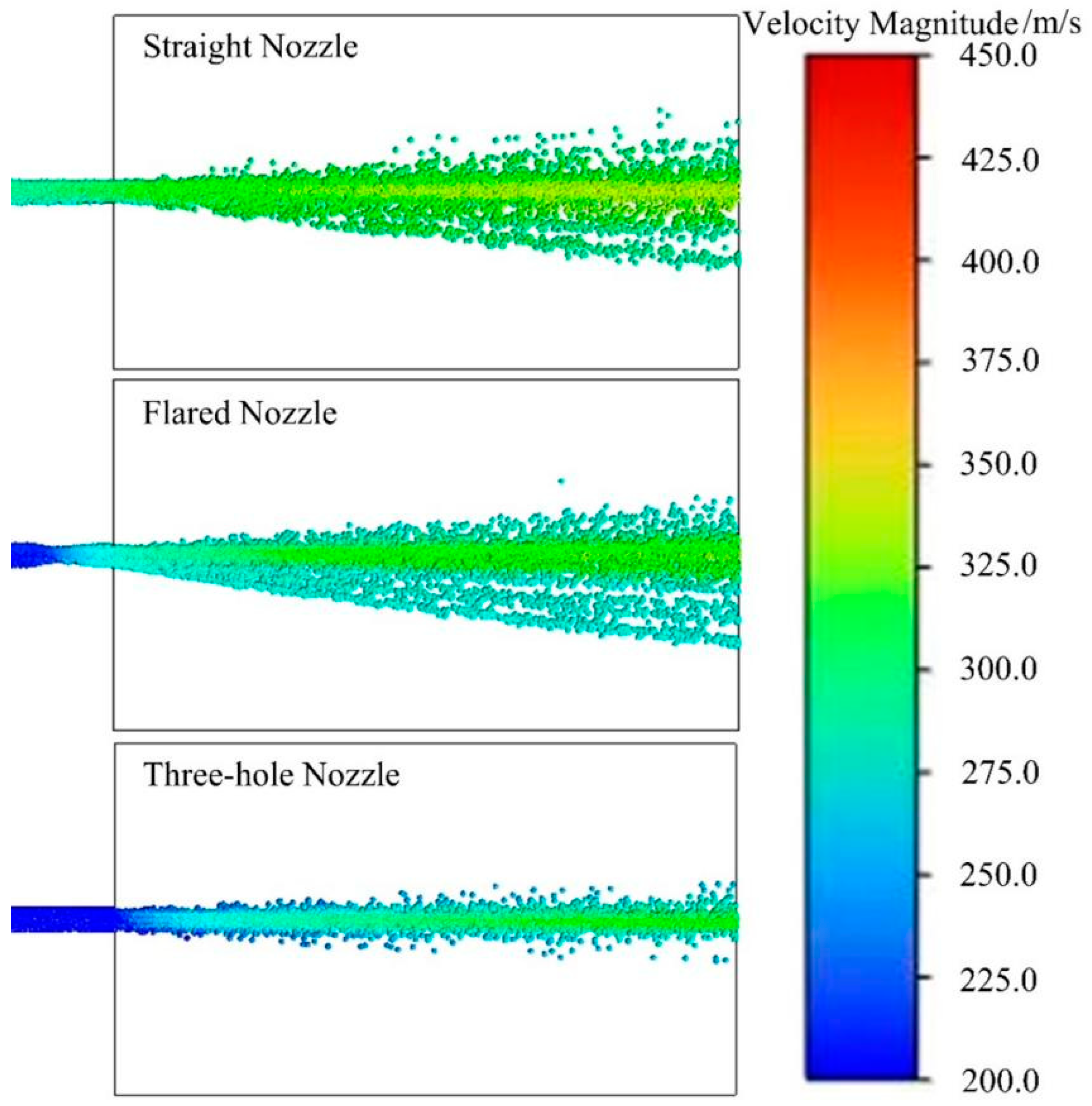
In summary, the experimental results are consistent with the simulation results for the gas–solid two-phase flow under different nozzles. When the nozzle is the three-hole type, the dry powder particle velocity is approximately 200 m/s in the external flow field, with a low particle velocity and a small particle diffusion range. The gas velocity distribution at the outlet of the horn-shaped nozzle is the most uniform, which is more conducive to dry powder injection. When the nozzle is of a straight mouth type, it has the largest fluctuation and the maximum range of gas-phase velocities, which is not conducive to the concentrated deposition of the dry powder. Therefore, when the nozzle is horn-shaped, the dry powder spraying effect is optimal.

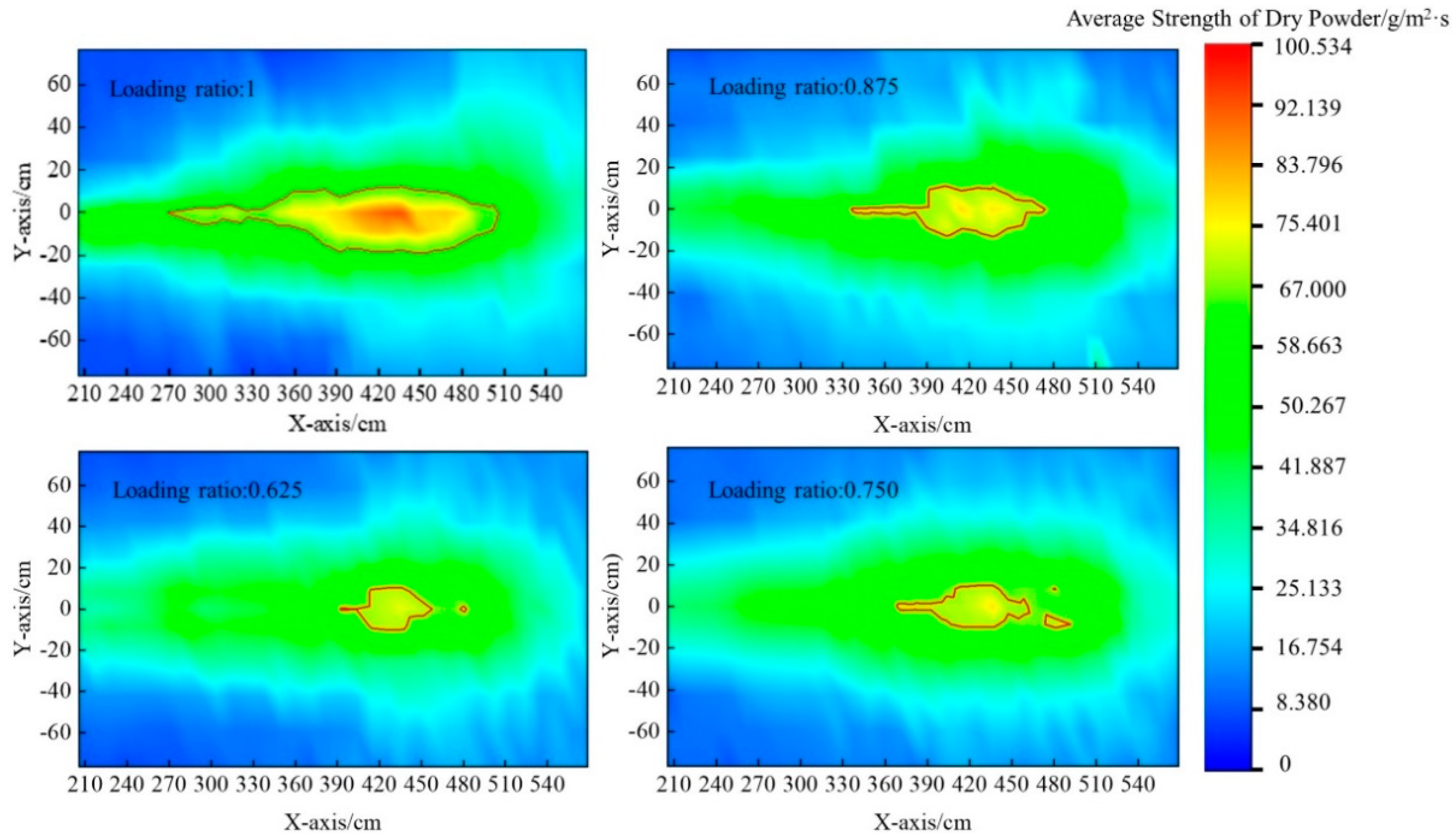
3.6. Experimental Study on the Effect of Dry Powder Spraying under Different Loading Ratios

Using a straight nozzle, driven by 0.3 MPa nitrogen gas, with a dry powder pipe diameter of 10 mm, dry powder injection experiments were conducted with different dry powder loading ratios. The formula for the calculation of the dry powder loading ratio K is as follows:

$$K=\frac{m}{v}$$

(19)

Here, K is the dry powder loading ratio, m is the mass of the filled dry powder fire-extinguishing agent in kg and is the volume of the dry powder tank in L. The volume of the dry powder tank designed in this study is 8 L.

The effective farthest distance results are shown in Table 10 and Figure 25.

Table 11. Most effective distances under different loading ratios.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Loading Ratio	Farthest Effective Distance/mm
0.3	Straight mouth type	1	4365
0.3	Straight mouth type	0.875	4365
0.3	Straight mouth type	0.75	4365
0.3	Straight mouth type	0.625	4365

shows the comparison of the effective longest distances of the fire-extinguishing devices under different dry powder loading ratios. The effective longest distance remains unchanged under the different dry powder loading ratios.

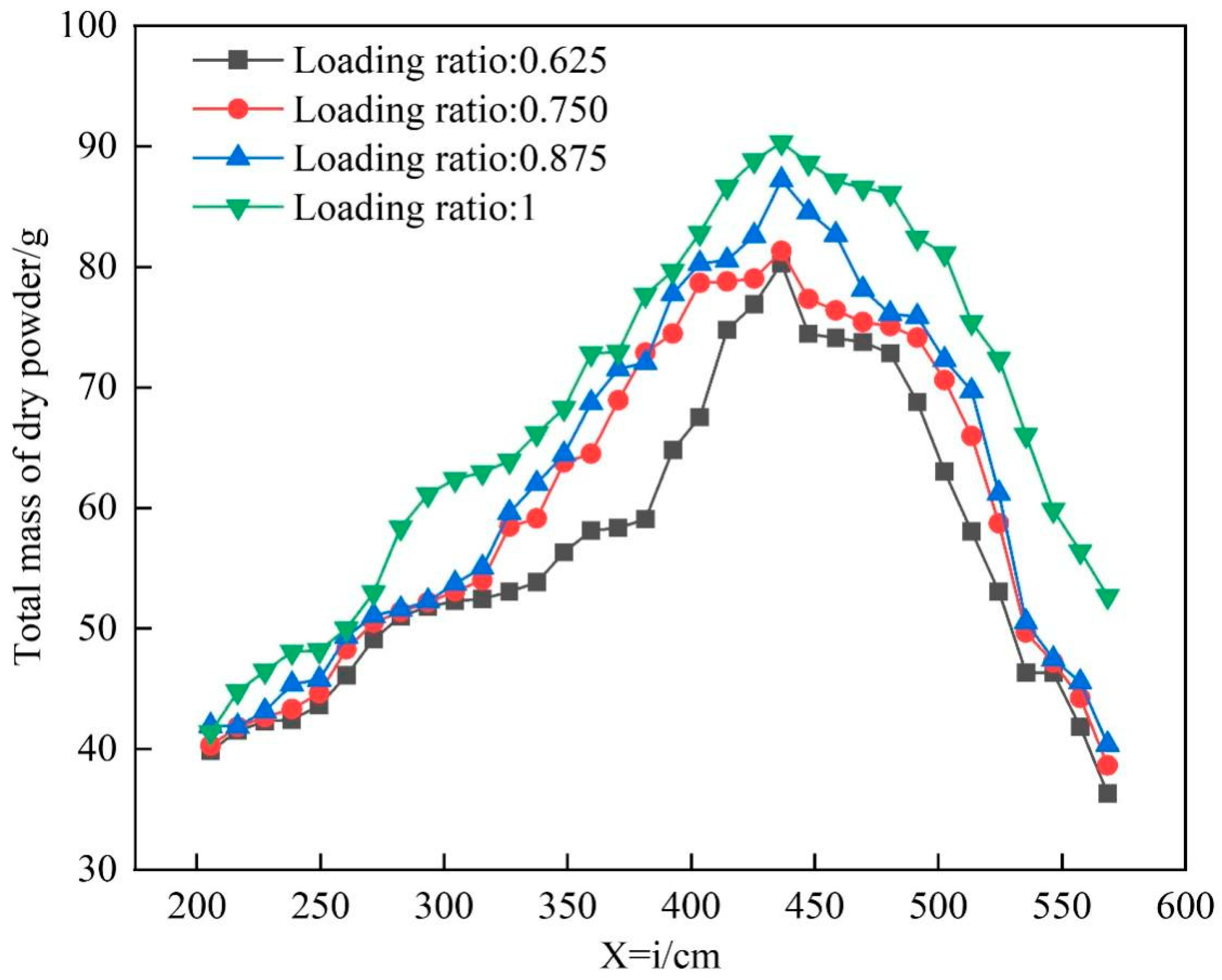
Figure 26 shows the total mass curve of the dry powder on the X = i axis under different dry powder loading ratios. It can be seen from the figure that the total mass of the dry powder on the axis first increases and then decreases. As the dry powder loading ratio increases, the total mass of the dry powder on the axis gradually increases. When the dry powder loading ratio is 1, the total mass of the dry powder on the axis is significantly higher than that at other loading ratios. This may be due to the increase in the dry powder concentration as the dry powder loading ratio increases.

The results for the effective fire-extinguishing area are shown in

Table 12. Effective extinguishing area under different loading ratios.

Nitrogen Pressure/MPa	Nozzle Type	Dry Powder Loading Ratio	Effective Fire-Extinguishing Area/cm2
0.3	Straight mouth type	1	8415
0.3	Straight mouth type	0.875	4488
0.3	Straight mouth type	0.75	2805
0.3	Straight mouth type	0.625	2244

and Figure 27.

Table 12 shows the effective fire-extinguishing area under different dry powder loading ratios. It can be seen from the table that when the dry powder loading ratio is 1, the effective area is the largest, with a difference of 6171 cm² compared to when the dry powder loading ratio is 0.625.

Figure 27 shows the average strength cloud map of the dry powder under different loading ratios. It can be seen from the figure that, under different loading ratios, the diffusion of the dry powder has a “horn” shape, and the effective fire-extinguishing area has a “V” shape. As the dry powder loading ratio increases, the effective fire-extinguishing area gradually increases, and the average dry powder strength in the effective fire-extinguishing area gradually increases. When the dry powder loading ratio is 1, the effective fire-extinguishing area is the largest, and the average dry powder strength in the effective fire-extinguishing area is also significantly higher than for other average dry powder strengths.

4. Research on Combination of Parameters for Optimal Spraying Effect in Dry Powder Controlled-Pressure Spraying Fire-Extinguishing System

The previous sections described numerical simulations and experiments on different pressures, pipe diameters, and nozzle configurations, as well as dry powder injection experiments using different dry powder loading ratios. However, the pressure-controlled dry powder fire-extinguishing device represents a system that is the result of the joint influence of each factor. Therefore, the four influencing factors were comprehensively studied to identify the optimal parameter ratio so as to achieve the maximum effective fire-extinguishing area, providing a reliable basis for the future design of pressure-controlled fire-extinguishing devices for intelligent fire-extinguishing robots.

This study uses the orthogonal experimental method to study the influence of a comprehensive set of factors on fire-extinguishing devices. After obtaining the results of each group of experiments through the orthogonal method, the optimal level for each factor is determined by calculating the average value of each factor at each level. By calculating the difference between the average values, i.e., the range, the most important factor affecting the experimental results is determined. The larger the range of the influencing factor, the greater its impact on the experimental results [30,31]. The formula is

$$k=\frac{K}{n}$$

(20)

$$R_j=k_{\mathbf{max}}-k_{\mathbf{min}}$$

(21)

Here, K is the sum of the experimental results for each factor at different levels, n is the number of levels, Rj is the range, kmax is the maximum value of the mean k, and kmin is the minimum value.

This study considers four influencing factors: A is the nitrogen driving pressure, B is the diameter of the dry powder pipe, C is the nozzle configuration, and D is the dry powder loading ratio (

Table 13. Factor level table.

Level	Factor
	A/MPa	B/mm	C	D
1	0.3	10	Horn type	1
2	0.4	8	Straight mouth type	0.875
3	0.5	6.5	Three-hole type	0.75

). Through the previous single-factor experiment, we select three optimal levels for each factor. According to the analysis of the dry powder spraying experiments described in the previous sections, with the exception of the three-hole nozzle, the effective farthest distance of the fire-extinguishing device can fulfill the standard under all conditions. Therefore, the effective fire-extinguishing area of the fire-extinguishing device is used as an experimental indicator to evaluate the spraying effect of the dry powder. Usually, the orthogonal experimental table L9 (34) can be used for four factors and three levels. Therefore, a total of nine sets of orthogonal experiments were designed in this study. The factor levels of the orthogonal experiments are shown in

Table 14. Orthogonal experiment results.

Experiment Number	A	B	C	D	Effective Fire-Extinguishing Area
1	1	1	1	1	49
2	1	2	2	2	30
3	1	3	3	3	13
4	2	1	2	3	12
5	2	2	3	1	19
6	2	3	1	2	21
7	3	1	3	2	10
8	3	2	1	3	23
9	3	3	2	1	22
K1	92	71	93	90
K2	52	72	64	61
K3	55	56	42	48
k1	30.667	23.667	31.000	30.000
k2	17.333	24.000	21.333	20.333
k3	18.333	18.667	14.000	16.000
R	13.334	5.333	17.000	14.000

.

For statistical convenience and an intuitive analysis, the effective fire-extinguishing area’s index value is simplified. The effective fire-extinguishing area is set as S, and the simplified effective fire-extinguishing area is s, where s = S/187, as 187 cm² is the size of the bottom area of the collection box.

This study adopts intuitive analysis, where k1 is the mean of the experimental results of this factor at all levels, i.e., k1, k2, k3, etc. By comparing the means of all levels under a certain factor, the level with the highest mean is obtained as the optimal level. From the analysis of the orthogonal experiments’ results, it can be seen that R3 > R4 > R1 > R2, and the four influencing factors, in order of significance, are the nozzle configuration, dry powder loading ratio, nitrogen driving pressure, and dry powder pipe diameter. The optimal level is determined based on the level with the highest mean. Therefore, the optimal combination to achieve the maximum effective fire-extinguishing area of the pressure-controlled fire-extinguishing device is A1B2C1D1, which means that the nitrogen driving pressure is 0.3 MPa, the dry powder pipe diameter is 8 mm, the nozzle is horn-shaped, and the dry powder loading ratio is 1. At these settings, the effective fire-extinguishing area of the pressure-controlled fire-extinguishing device is the largest, and the dry powder spraying effect is the best.

The optimal combination of the factors that affect the effective fire-extinguishing area of the fire-extinguishing device, as obtained from the orthogonal experiment, does not exist in the orthogonal experiment table. To verify the correctness of this conclusion, experimental verification is conducted. The experimental verification results are shown in

Table 15. Experimental verification results.

Experimental Condition				Result
Driving pressure	Pipe diameter	Loading ratio	Injector	Effective fire-extinguishing area	Effective farthest distance
0.3 MPa	8 mm	1	Horn type	11,033 cm2	414.5 cm

.

Through the experimental verification, it is found that when nitrogen is driven at 0.3 MPa, the diameter of the dry powder pipe is 8 mm, the nozzle is the horn type, and the dry powder loading ratio is 1, the effective farthest distance of the fire-extinguishing device is 414.5 cm, and the effective fire-extinguishing area of the fire-extinguishing device is 11,033 cm². This is much larger than the effective fire-extinguishing area measured in the previous single-factor experiment and orthogonal experiment. Therefore, the optimal parameter combination obtained from the orthogonal experiment is validated.

5. Conclusions

(1) An intelligent inspection and fire-extinguishing robot was developed, which included five modules: the main control module, power module, detection module, fire-extinguishing module, and wireless charging module. The robot also included a visual detection system and sensor detection system in the detection module, as well as four subsystems, including an automatic release system for the fire-extinguishing agent and a fire-extinguishing execution system in the fire-extinguishing module. It showed excellent performance regarding intelligent autonomous inspection and allowed control over the spray pressure of the dry powder fire-extinguishing agent (referred to as “pressure-controlled fire extinguishing”). Through the numerical simulation and experimental verification of different influencing factors, it was possible for the robot to achieve pressure control and extinguish the fire.

(2) A numerical simulation model for dry powder particle injection was established by considering the effects of the fluid resistance, pressure gradient force, virtual mass force, and particle collision force. The mechanism and influence of the dry powder injection gas–solid two-phase flow were studied based on different injection pressures, dry powder pipe diameters, and nozzle configurations. Under different driving pressures, the greater the pressure, the stronger the shock wave intensity is in the external flow field, and the larger the negative pressure area, the higher the overall particle velocity is and the smaller the diffusion range. The best dry powder particle injection effect is achieved under a driving pressure of 0.3 MPa. Under different pipe diameters, the larger the pipe diameter, the stronger the shock wave intensity is and the longer the core jet area. The particle velocity distribution is the most uniform, and the dry powder distribution is the most concentrated under an 8 mm pipe diameter. Under different nozzle configurations, the horn-shaped nozzle has a lower shock wave intensity, a more uniform particle velocity distribution, a wider range, and the best spraying effect.

(3) Considering the macroscopic effects of the driving pressure, dry powder pipe diameter, nozzle configuration, and loading ratio, a dry powder injection experimental platform was established, and dry powder injection experiments were conducted based on different influencing factors. When the driving pressure increased from 0.3 MPa to 0.9 MPa, the effective fire-extinguishing area decreased by 1683 cm². When the pipe diameter was 8 mm, the effective fire-extinguishing area was the largest. When the pipe diameter was 10 mm, the effective fire-extinguishing area was the smallest, with a difference of 2057 cm² between the two. When the nozzle was of a three-hole type, the effective fire-extinguishing area was the smallest. When the nozzle was of a horn type, the effective fire-extinguishing area was the largest, with a difference of 7480 cm² between the two. When the dry powder loading ratio was 1, the effective fire-extinguishing area was the largest, which differed by 6171 cm² from the case in which the dry powder loading ratio was 0.625.

(4) We obtained the optimal parameter combination by designing an orthogonal experiment table and conducting experimental verification on it. After verification, it was determined that the optimal nitrogen driving pressure was 0.3 MPa, the dry powder pipe diameter was 8 mm, the nozzle was a horn type, and the dry powder loading ratio was 1; this is the optimal parameter combination and provides a theoretical basis for subsequent robotic pressure-controlled fire-extinguishing work.