A Novel Approach to Predicting Critical Alternating Stall in a Centrifugal Pump Impeller with Even Blades Under Transient Conditions
Abstract
1. Introduction
2. Geometric Models and Numerical Methods
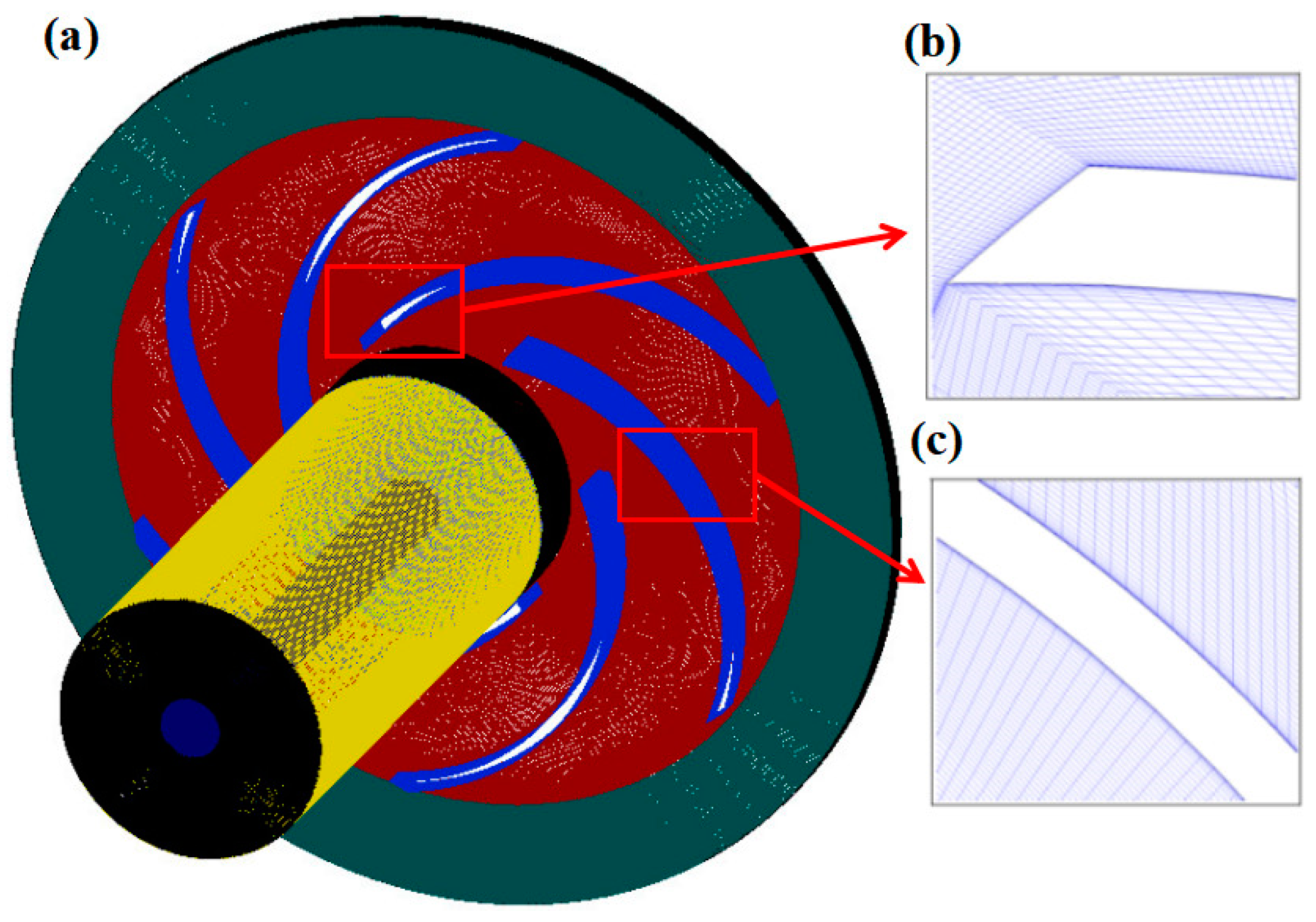
2.1. Physical Model
2.2. Governing Equations
- (1)
- Shear Stress Transport k-ω model
- (2)
- Dynamic Smagorinsky model
2.3. Numerical Methods and Boundary Conditions
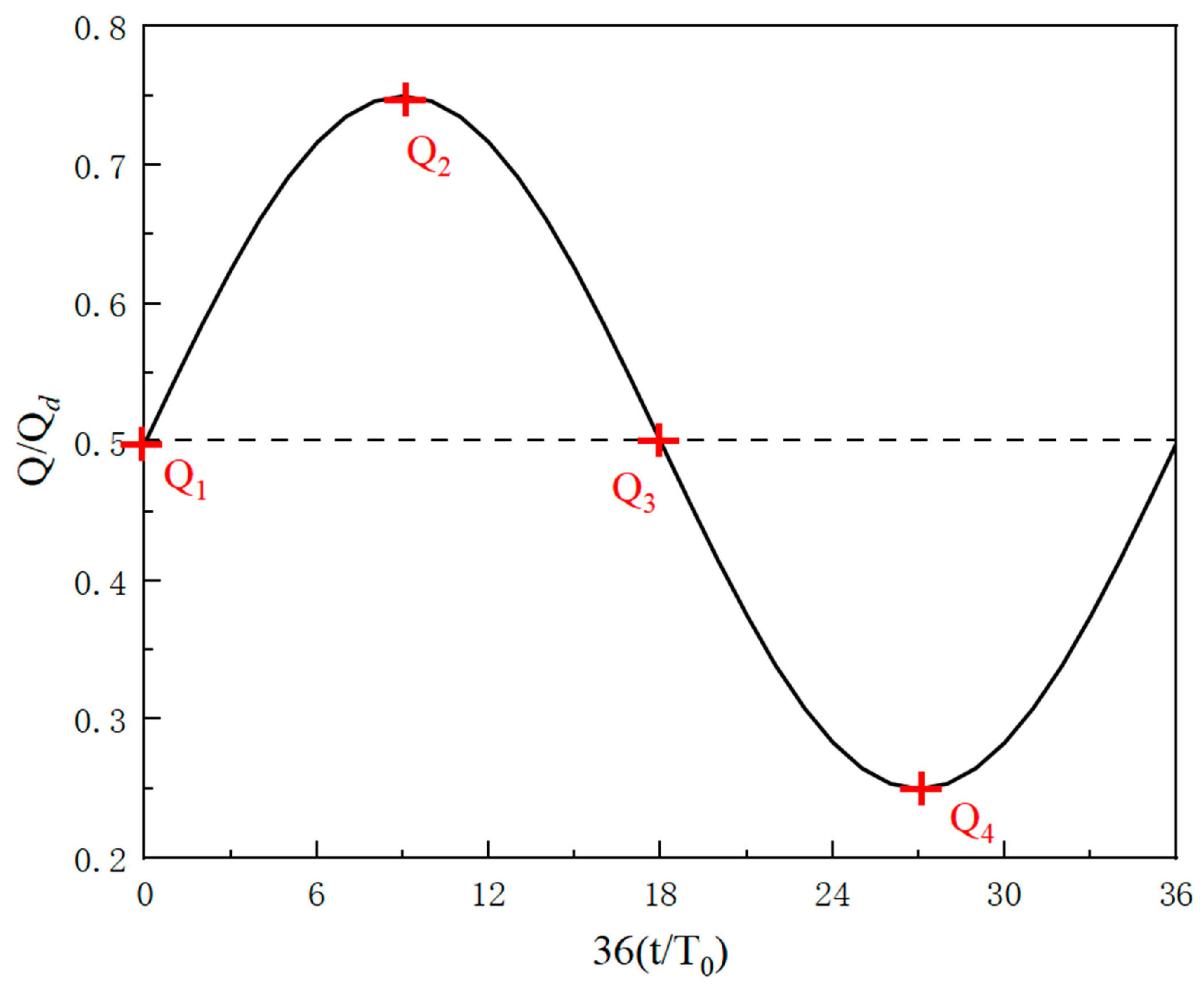
2.4. Flow Rate Conditions
3. Introduction and Validation of the Novel Approach
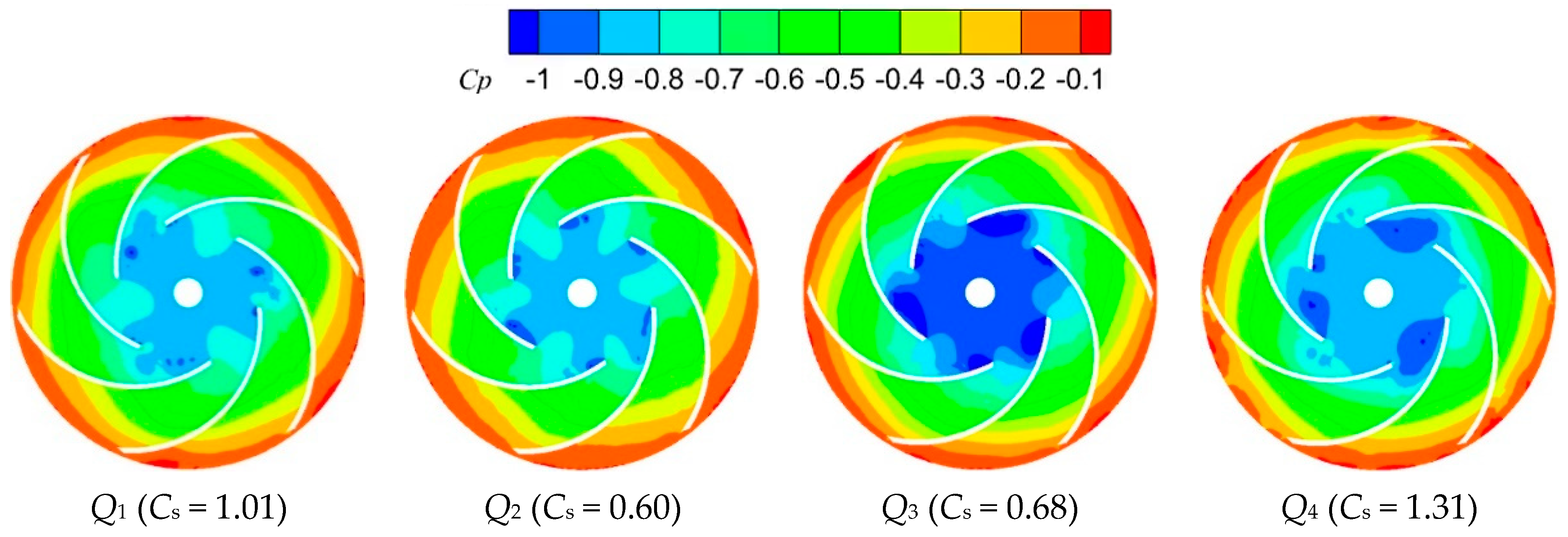
3.1. Detailed Approach Descriptions
3.2. Approach Validation Under Quasi-Steady Conditions
4. Application of the Novel Approach to Transient Conditions
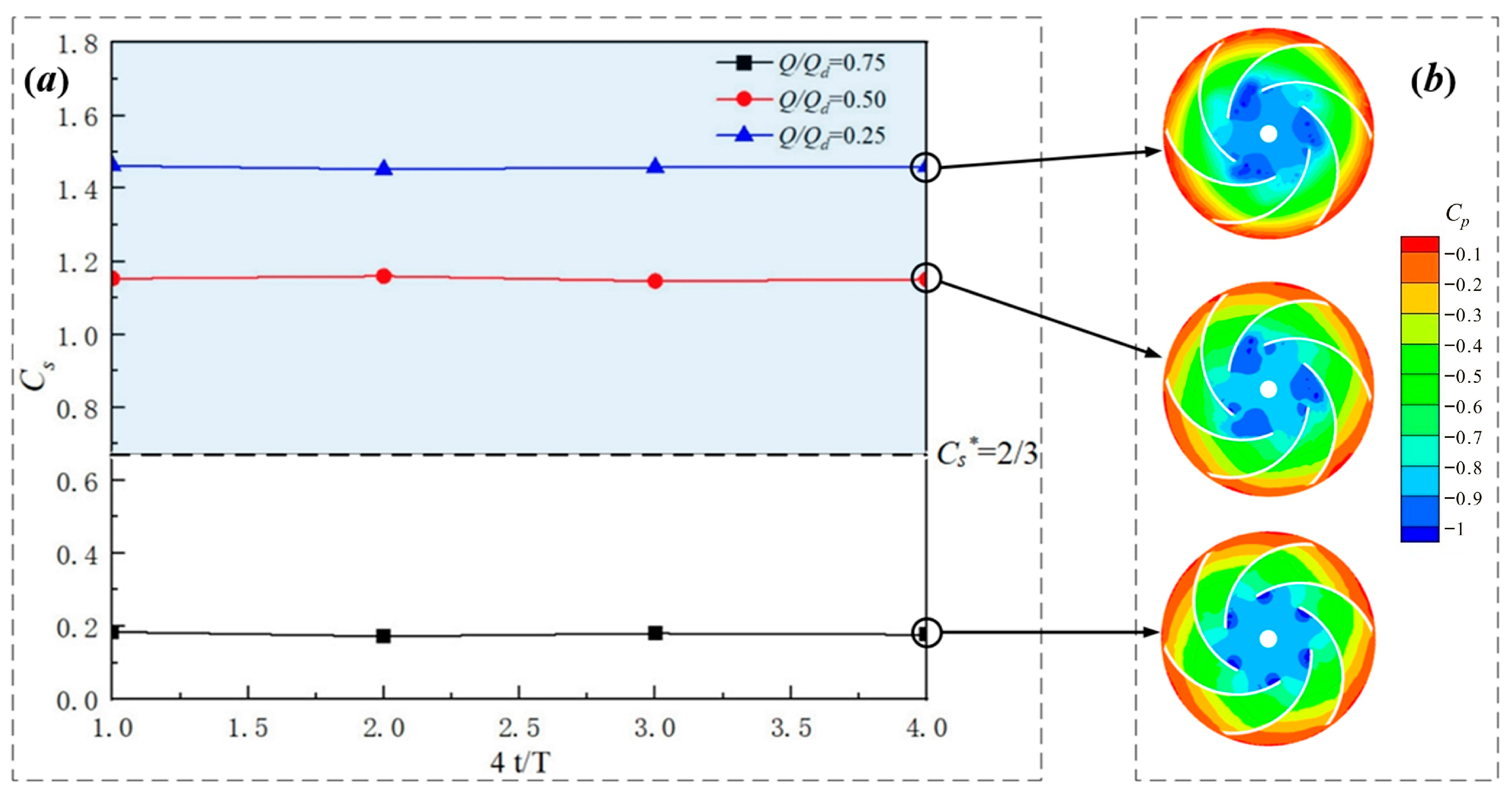
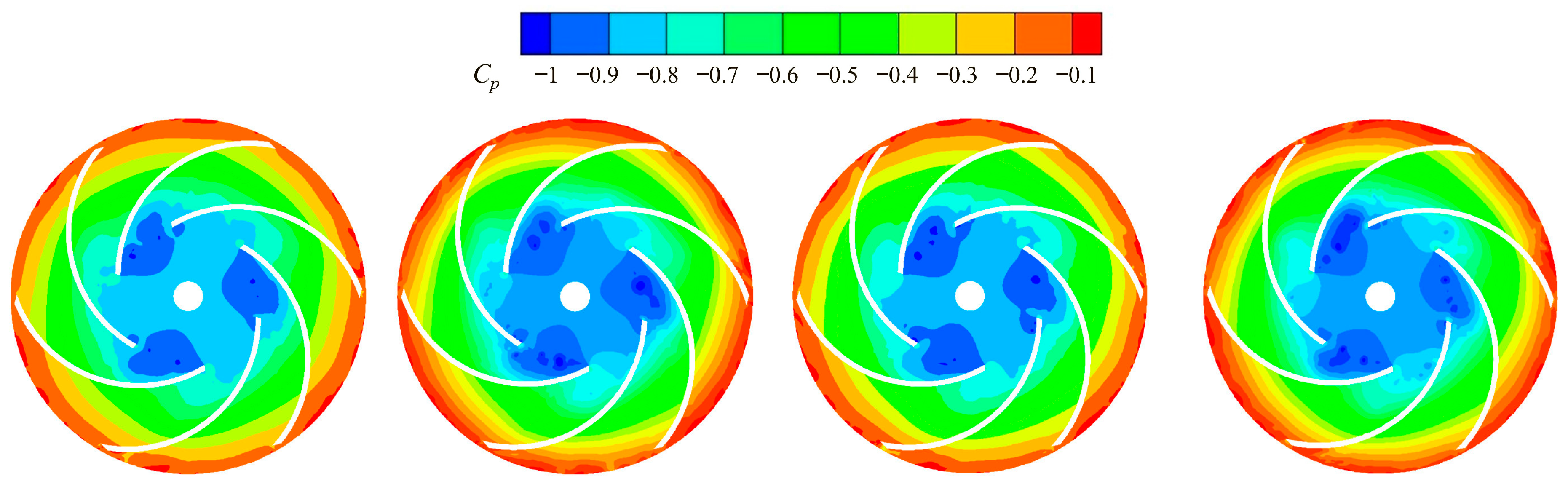
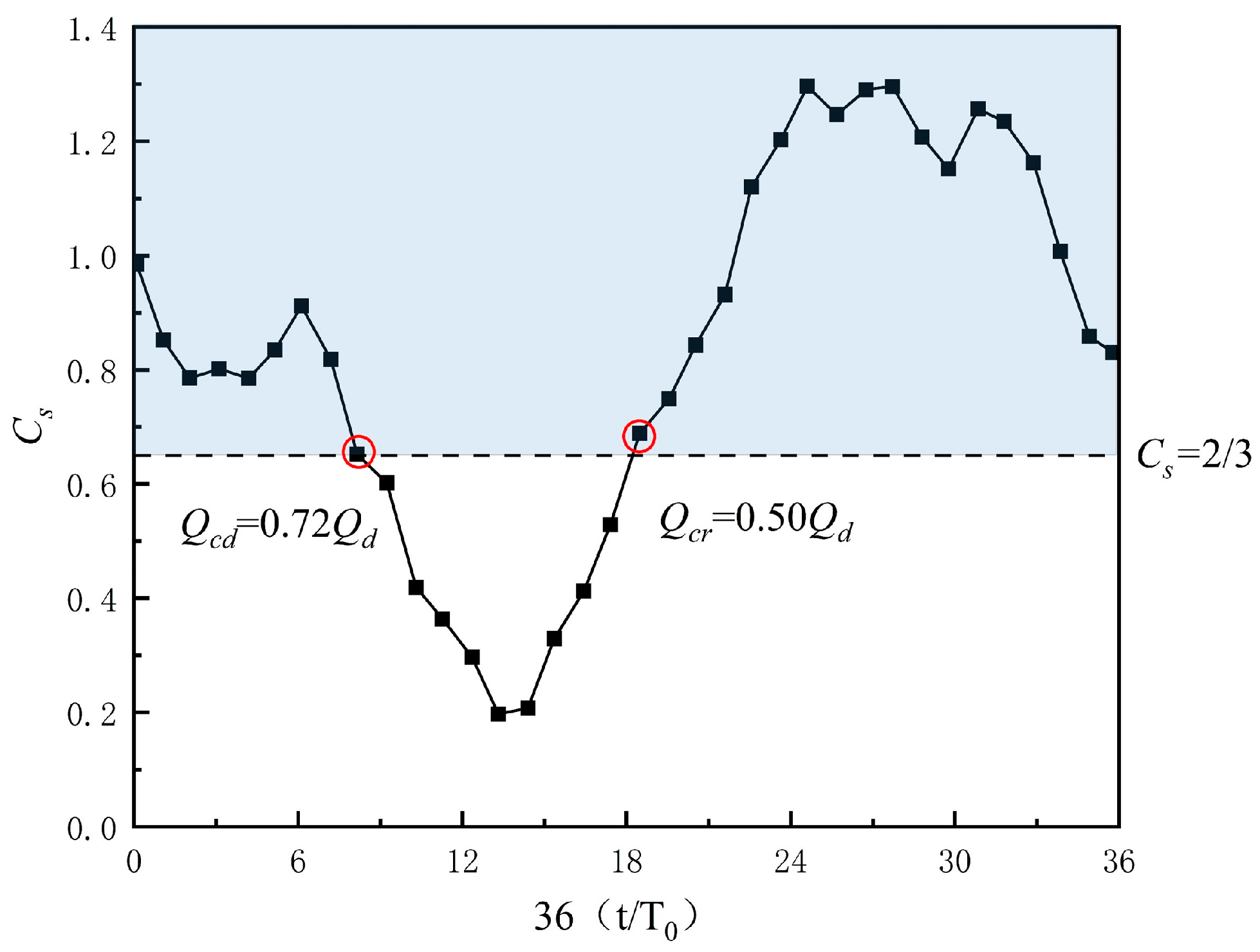
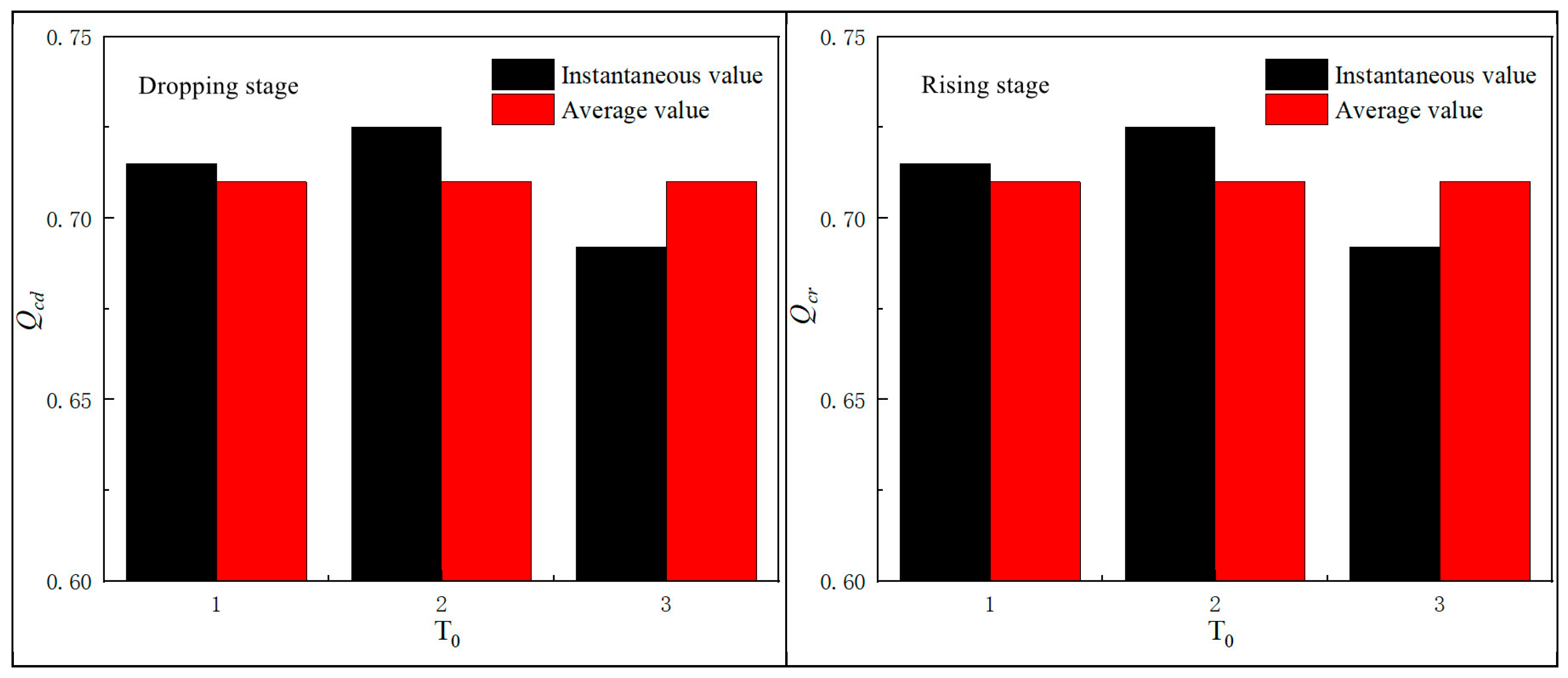
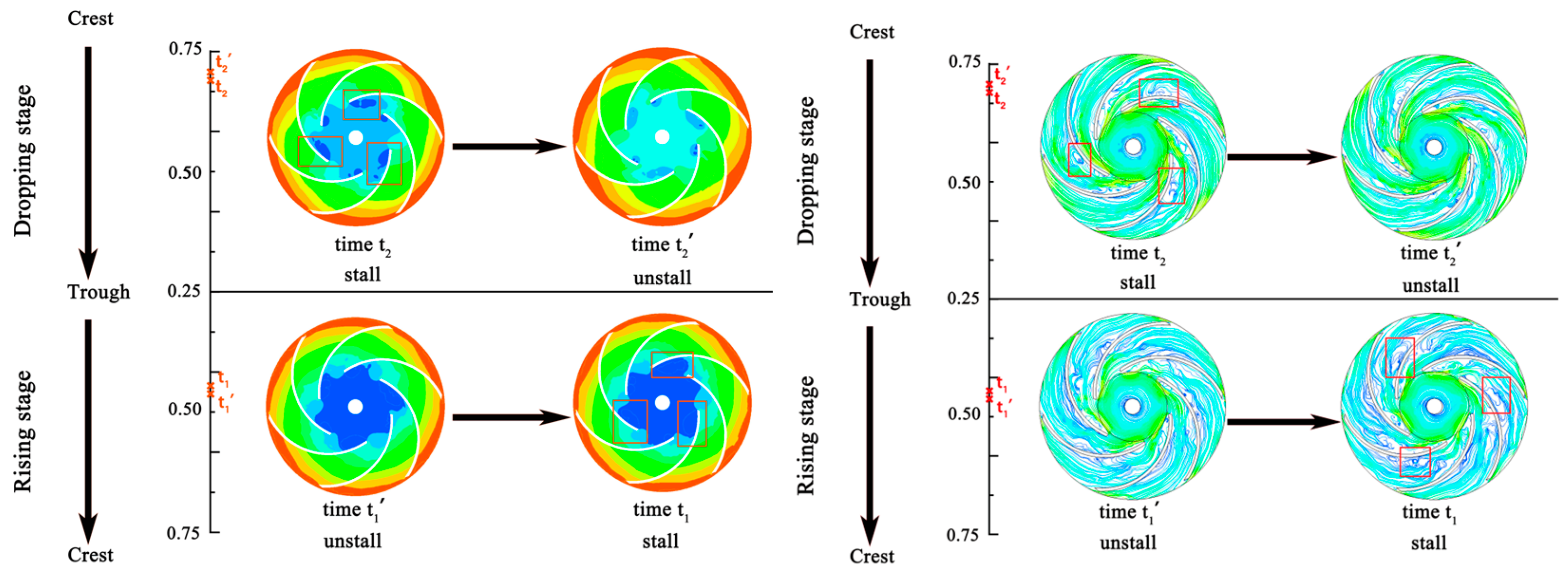
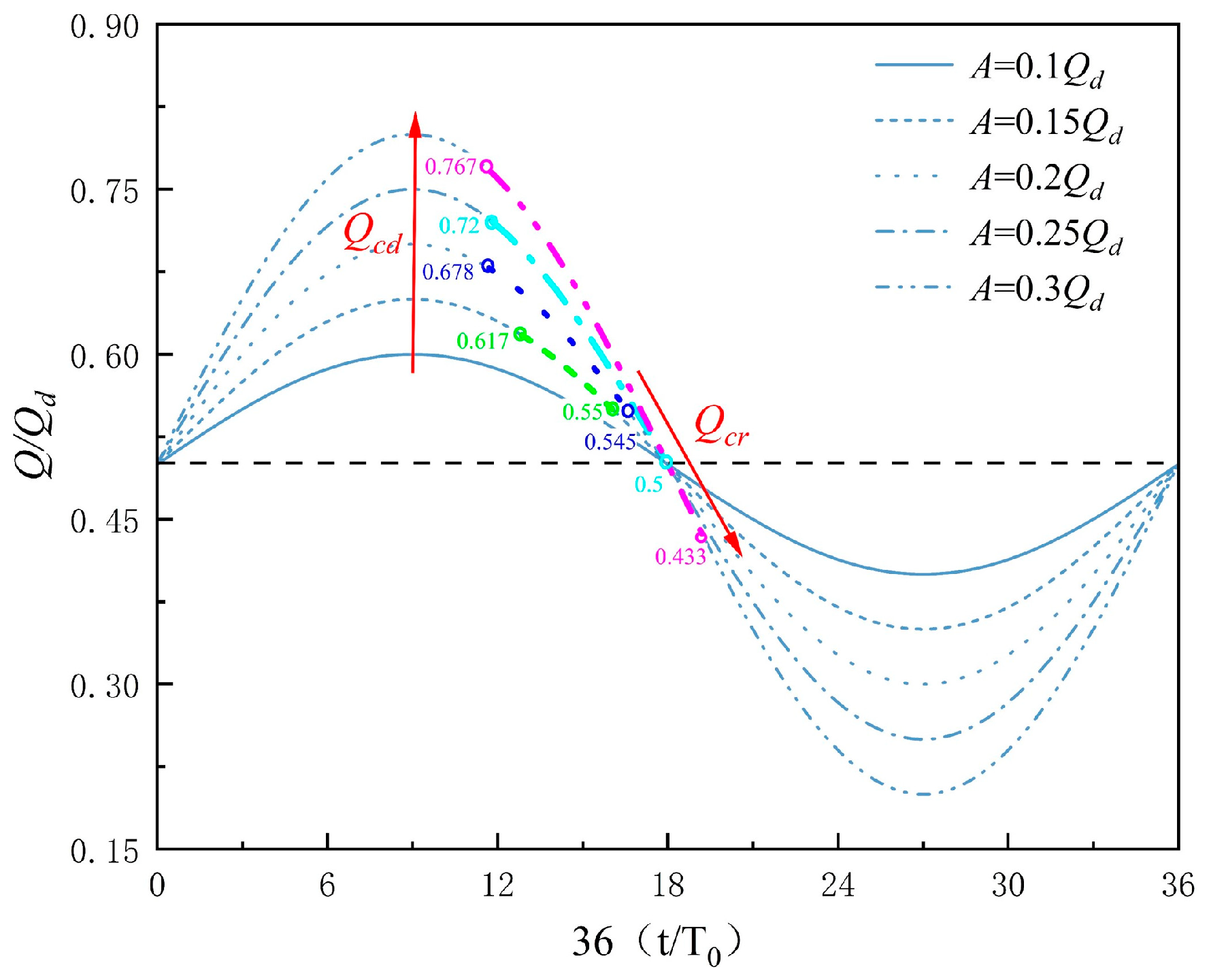
4.1. Determination of the Critical Alternating Stall
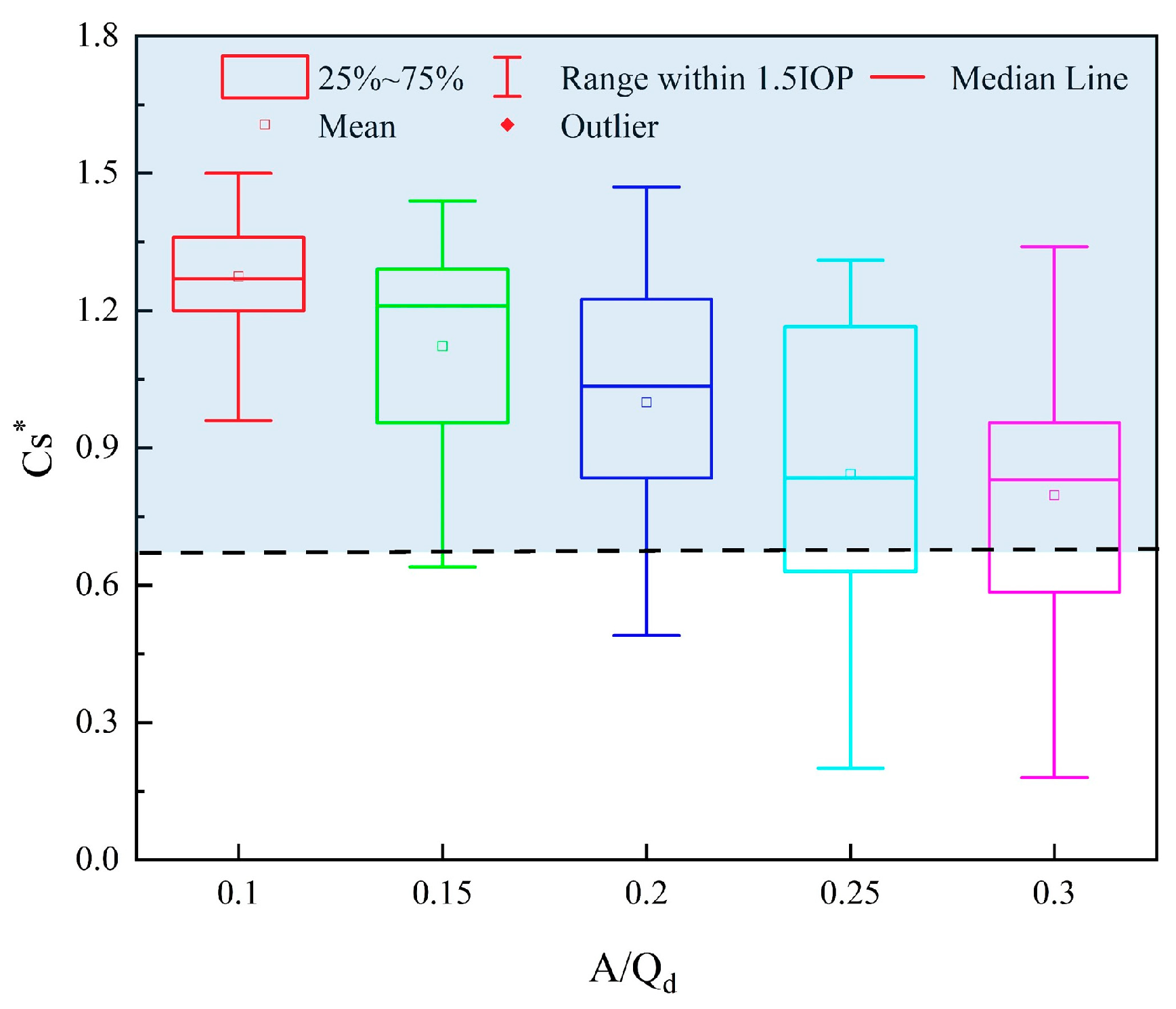
4.2. Oscillation Amplitude Effects
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhao, Z.Z.; Song, W.W.; Jin, Y.X.; He, L. Numerical study on flow stall and kinetic energy conversion of low-specific-speed centrifugal pump. Phys. Fluids 2023, 35, 044104. [Google Scholar] [CrossRef]
- Shi, L.; Liu, Z.Q.; Yang, W.; Li, Y.J.; Liu, X.D.; Tao, R. Prediction model of stall evolution stages in a centrifugal impeller based on characteristic analysis of the hydraulic torque of blades and support vector machine. J. Mech. Sci. Technol. 2023, 37, 5185–5198. [Google Scholar] [CrossRef]
- Liu, B.Q.; Yang, W.; Cheng, L.; Huang, X.B.; Jiao, W.X. Numerical study on jet-wake flow and its evolution in a centrifugal pump with alternating stall. Phys. Fluids 2023, 35, 115105. [Google Scholar] [CrossRef]
- Zhang, N.; Zheng, F.K.; Liu, X.K.; Gao, B.; Li, G.P. Unsteady flow fluctuations in a centrifugal pump measured by laser doppler anemometry and pressure pulsation. Phys. Fluids 2020, 32, 125108. [Google Scholar] [CrossRef]
- Mousmoulis, G.; Kassanos, I.; Aggidis, G.; Anagnostopoulos, I. Numerical simulation of the performance of a centrifugal pump with a semi-open impeller under normal and cavitating conditions. Appl. Math. Model. 2021, 89, 1814–1834. [Google Scholar] [CrossRef]
- Salehi, S.; Raisee, M.; Cervantes, M.J.; Nourbakhsh, A. On the flow field and performance of a centrifugal pump under operational and geometrical uncertainties. Appl. Math. Model. 2018, 61, 540–560. [Google Scholar] [CrossRef]
- Gülich, J.F. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Pedersen, N.; Larsen, P.S.; Jacobsen, C.B. Flow in a centrifugal pump impeller at design and off-design conditions-part I: Particle image velocimetry (PIV) and laser doppler velocimetry (LDV) measurements. J. Fluids Eng. 2003, 125, 61–72. [Google Scholar] [CrossRef]
- Byskov, R.K.; Jacobsen, C.B.; Pedersen, N. Flow in a centrifugal pump impeller at design and off-design conditions-Part II: Large eddy simulations. J. Fluids Eng. 2003, 125, 73–83. [Google Scholar] [CrossRef]
- Johnson, D.A.; Pedersen, N.; Jacobsen, C.B. Measurements of rotating stall inside a centrifugal pump impeller. In Proceedings of the ASME 2005 Fluids Engineering Division Summer Meeting, Houston, TX, USA, 19–23 June 2005. [Google Scholar]
- Tao, R.; Wang, Z. Comparative modeling and analysis of the flow asymmetricity in a centrifugal pump impeller at partial load. Proc. Inst. Mech. Eng. Part A J. Power Energy 2019, 234, 237–247. [Google Scholar] [CrossRef]
- Zhou, R.Z.; Liu, H.L.; Dong, L.; Ooi, T.K.; Dai, C.; Hua, R.N. Study on the stall vortex and vibration characteristics in multi-stage pump under natural flow condition. Phys. Fluids 2023, 35, 084117. [Google Scholar] [CrossRef]
- Li, W.; Ji, L.L.; Li, E.D.; Shi, W.D.; Agarwal, R.; Zhou, L. Numerical investigation of energy loss mechanism of mixed-flow pump under stall condition. Renew. Energy 2021, 167, 740–760. [Google Scholar] [CrossRef]
- Cheah, K.W.; Lee, T.S.; Winoto, S.H.; Zhao, Z.M. Numerical flow simulation in a centrifugal pump at design and off-design conditions. Int. J. Rotating Mach. 2007, 11, 83641–83648. [Google Scholar] [CrossRef]
- Zhou, P.J.; Dai, J.C.; Li, Y.F.; Chen, T.; Mou, J.G. Unsteady flow structures in centrifugal pump under two types of stall conditions. J. Hydrodyn. 2018, 30, 1038–1044. [Google Scholar] [CrossRef]
- Kline, S.J. On the nature of stall. J. Basic Eng. 1959, 81, 305–319. [Google Scholar] [CrossRef]
- Sano, T.; Nakamura, Y.; Yoshida, Y. Alternate blade stall and rotating stall in a vaned diffuser. JSME Int. J. Ser. B Fluids Therm. Eng. 2002, 45, 810–819. [Google Scholar] [CrossRef]
- Giannissis, G.L.; Mckenzie, A.B.; Elder, R.L. Experimental Investigation of Rotating Stall in a Mismatched Three-Stage Axial Flow Compressor. J. Turbomach. 1989, 111, 418–425. [Google Scholar] [CrossRef]
- Olivier, P.; Chisachi, K.; Guo, Y.; Yamade, Y.; Frangois, A. Large eddy simulation of the rotating stall in a pump-turbine operated in pumping Mode at a Part-Load Condition. J. Fluids Eng. 2016, 138, 111102. [Google Scholar] [CrossRef]
- Stosiak, M.; Karpenko, M.; Deptula, A.; Urbanowicz, K.; Skackauskas, P.; Deptula, A.M.; Danilevicius, A.; Sukevicius, S.; Lapka, M. Research of Vibration Effects on a Hydraulic Valve in the Pressure Pulsation Spectrum Analysis. J. Mar. Sci. Eng. 2023, 11, 301. [Google Scholar] [CrossRef]
- Zhang, B.W.; Li, C.; Jiao, W.X.; Zhang, D. Experimental and statistical analysis of the flap gate energy loss and pressure fluctuation spatiotemporal characteristics of a mixed-flow pump device. Energy 2023, 272, 127117. [Google Scholar] [CrossRef]
- Li, Z.F.; Wu, D.Z.; Wang, L.Q.; Huang, B. Numerical simulation of the transient flow in a centrifugal pump during starting period. J. Fluids Eng. 2010, 132, 081102. [Google Scholar] [CrossRef]
- Long, Y.; Lin, B.; Fang, J.; Zhu, R.S.; Fu, Q. Research on the transient hydraulic characteristics of multistage centrifugal pump during start-up process. Front. Energy Res. 2020, 8, 76. [Google Scholar] [CrossRef]
- Li, Z.F.; Wu, P.; Wu, D.Z.; Wang, L.Q. Experimental and numerical study of transient flow in a centrifugal pump during startup. J. Mech. Sci. Technol. 2011, 25, 749–757. [Google Scholar] [CrossRef]
- Tanaka, T.; Tsukamoto, H. Transient behaviour of a cavitating centrifugal pump at rapid change in operating conditions-part II;: Transient phenomena at pump start-up/sutdown. J. Fluids Eng. 1999, 121, 850–856. [Google Scholar] [CrossRef]
- Liu, J.; Li, Z.; Wang, L.; Jiao, L. Numerical simulation of the transient flow in a radial flow pump during stopping period. J. Fluids Eng. 2011, 133, 111101. [Google Scholar] [CrossRef]
- Tsukamoto, H.; Ohashi, H. Transient characteristics of a centrifugal pump during starting period. J. Fluids Eng. 1982, 104, 6–13. [Google Scholar] [CrossRef]
- Ohashi, H. Analytical and experimental study of dynamic characteristics of turbopumps: 2nd Report, Analytical Study of Dynamic Characteristics. Trans. Jpn. Soc. Jpn. Soc. Mech. Eng. 1967, 33, 1779–1788. [Google Scholar] [CrossRef]
- Tsukamoto, H.; Yoneda, H.; Sagara, K. The response of a centrifugal pump to fluctuating rotational speed. J. Fluids Eng. 1995, 117, 479–484. [Google Scholar] [CrossRef]
- Kuang, R.F.; Chen, X.P.; Zhang, Z.M.; Zhu, Z.C.; Li, Y. Large eddy simulation of periodic transient pressure fluctuation in a centrifugal pump impeller at low flow rate. Symmetry 2021, 13, 311. [Google Scholar] [CrossRef]
- Kuang, R.F.; Li, Y.; Chen, X.P. Effects of oscillation frequency on the flow characteristics in a centrifugal pump impeller with sinusoidal flow rate. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023, 237, 4246–4255. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, W.J.; Chen, X.P. Numerical investigation of flow characteristics in a centrifugal pump impeller with sinusoidal flow rates of different oscillation amplitudes. J. Appl. Fluid Mech. 2022, 15, 1869–1879. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- C.F.X. ANSYS, Inc. Theory Guide; ANSYS, Inc.: Canonsburg, PA, USA, 2016. [Google Scholar]
- Germano, M.; Piomelli, U.; Moin, P.; Cabot, W.H. A dynamic subgrid-scale eddy viscosity model. Phys. Fluids A 1991, 3, 1760–1765. [Google Scholar] [CrossRef]
- Lilly, D.K. A proposed modification of the Germano subgrid-scale closure method. Phys. Fluids 1992, 4, 633–635. [Google Scholar] [CrossRef]



| Parameter | Symbol | Numerical Value | Units |
|---|---|---|---|
| Specific speed | ns | 26.3 | rev/min |
| Blade radius of curvature | Rb | 70 | mm |
| Inlet placement angle | λ1 | 19.7 | deg |
| Outlet placement angle | λ2 | 18.4 | deg |
| Number of blades | Z | 6 | — |
| Blade thickness | d | 3 | mm |
| Inlet radius | R1 | 35.5 | mm |
| Outlet radius | R2 | 95 | mm |
| Inlet height | b1 | 13.8 | mm |
| Outlet height | b2 | 5.8 | mm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, X.; Chen, X.; Yu, D.; Li, Y.; Chen, X. A Novel Approach to Predicting Critical Alternating Stall in a Centrifugal Pump Impeller with Even Blades Under Transient Conditions. Processes 2024, 12, 2635. https://doi.org/10.3390/pr12122635
Zhou X, Chen X, Yu D, Li Y, Chen X. A Novel Approach to Predicting Critical Alternating Stall in a Centrifugal Pump Impeller with Even Blades Under Transient Conditions. Processes. 2024; 12(12):2635. https://doi.org/10.3390/pr12122635
Chicago/Turabian StyleZhou, Xiaojie, Xulai Chen, Di Yu, Yu Li, and Xiaoping Chen. 2024. "A Novel Approach to Predicting Critical Alternating Stall in a Centrifugal Pump Impeller with Even Blades Under Transient Conditions" Processes 12, no. 12: 2635. https://doi.org/10.3390/pr12122635
APA StyleZhou, X., Chen, X., Yu, D., Li, Y., & Chen, X. (2024). A Novel Approach to Predicting Critical Alternating Stall in a Centrifugal Pump Impeller with Even Blades Under Transient Conditions. Processes, 12(12), 2635. https://doi.org/10.3390/pr12122635






