Performance Evaluation of CO2 + SiCl4 Binary Mixture in Recompression Brayton Cycle for Warm Climates
Abstract
1. Introduction
2. System Arrangement: Modeling and Operating Parameters
3. Mathematical Model for the Quantitative Assessment of the Cycle
4. Findings and Analyses
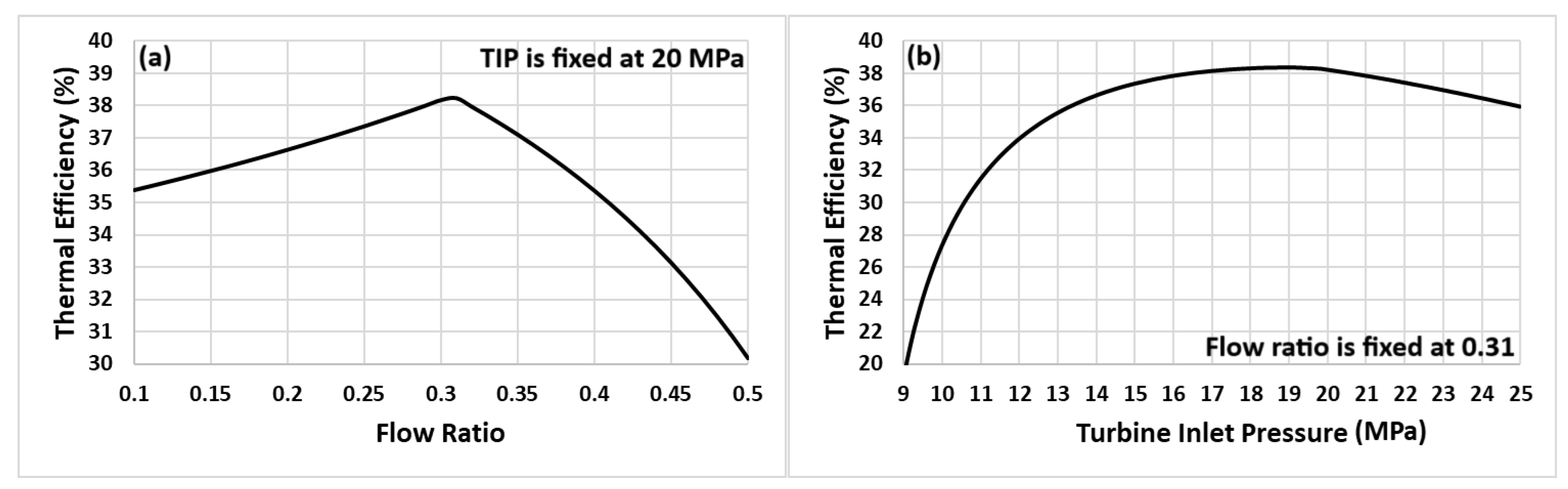
4.1. Factors Influencing Cycle Performance
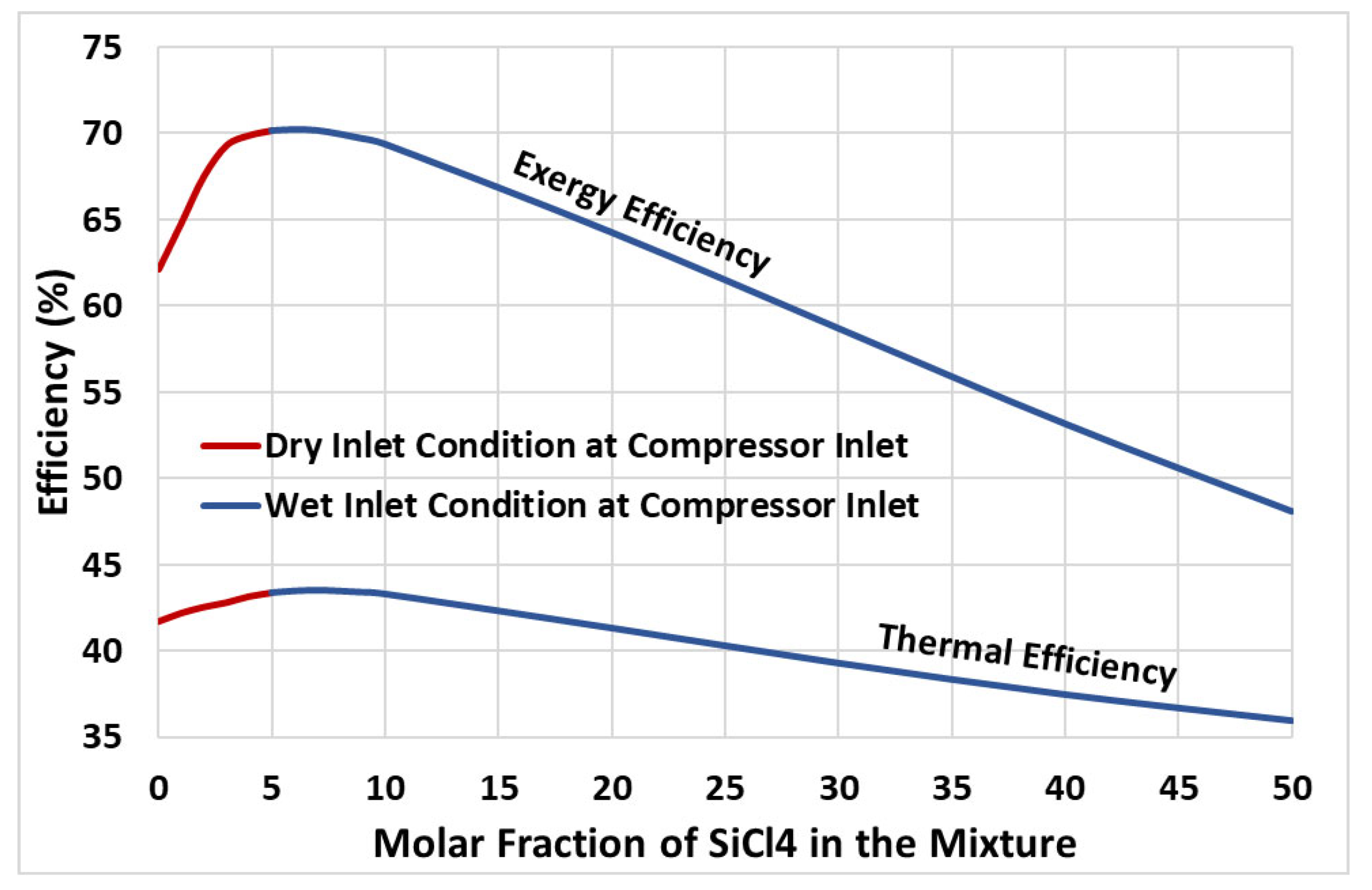
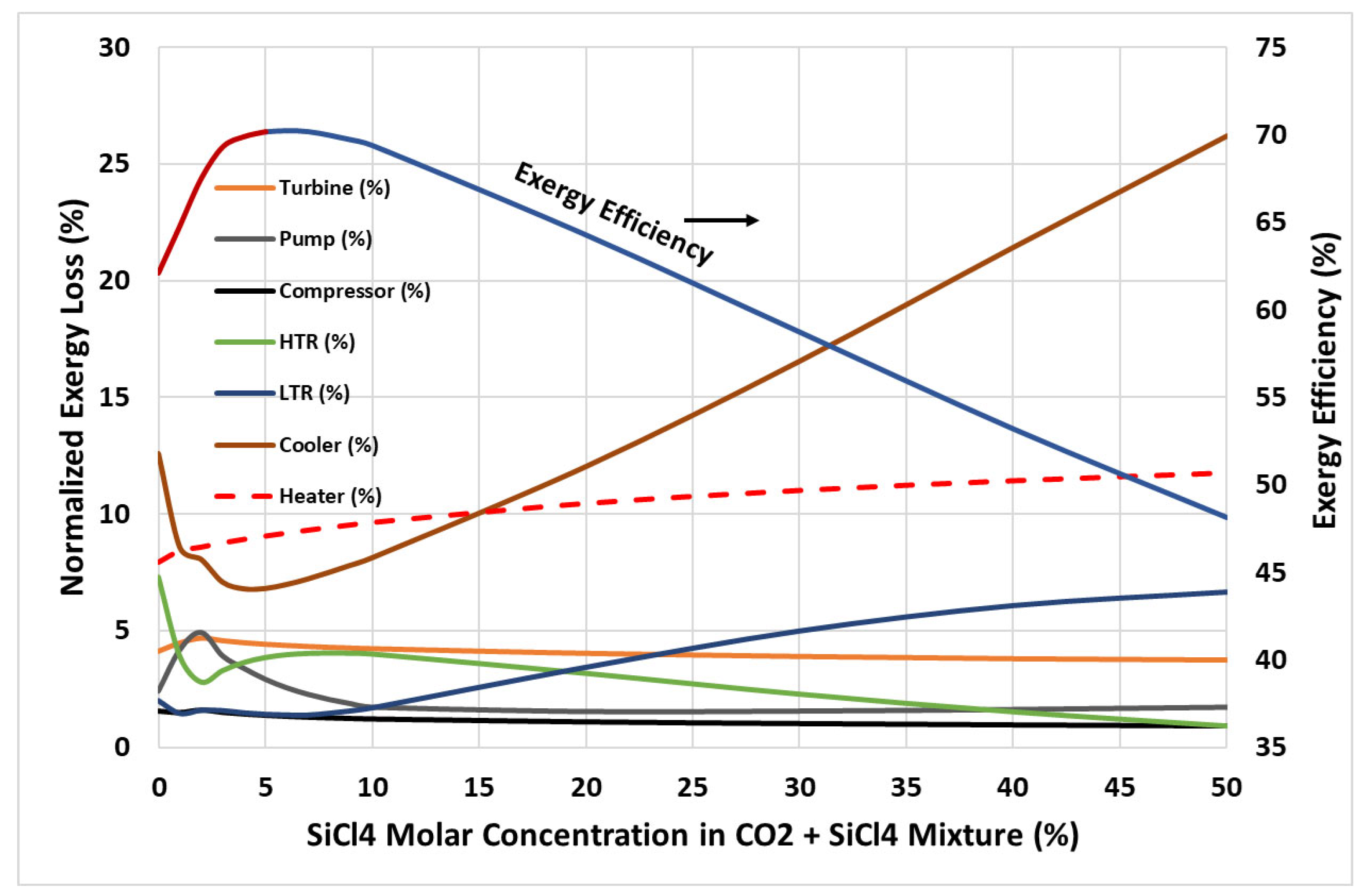
4.2. Energy and Exergy Performance
5. Conclusions
- The cycle thermodynamic performance improved significantly when using CO2 + SiCl4 binary mixture as a working fluid compared to pure CO2.
- At optimal mixture concentration, the thermal efficiency of the cycle improved by 1.6 percentage points, which corresponds to a nearly 4% improvement compared to pure CO2.
- A great improvement in the exergy performance of the cycle was observed for CO2 + SiCl4 binary mixture. At optimal operating conditions, the exergy efficiency increased by 8 percentage points, reflecting a nearly 13% improvement compared to pure CO2. This was mainly due to a significant decrease in irreversibility losses in the cooler and the HTR.
- With CO2 + SiCl4 as a working fluid, the energy and exergy performance parameters of the cycle improved with an increased molar concentration of SiCl4 in the mixture to a limit, and then a decline in the performance was observed.
- The increasing molar concentration of SiCl4 also resulted in a wet inlet condition to the compressor inlet, which resulted from the increasing trend of temperature glide with the increasing molar concentration of SiCl4 in the mixture. This was due to limited heat recovery from the LTR between hot and cold streams.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| CO2 | carbon dioxide |
| hi | specific enthalpy at i-th state point in the cycle |
| HTR | high-temperature recuperator |
| LTR | low-temperature recuperator |
| mass flow rate of the working fluid at i-th state point in the cycle | |
| ORC | organic Rankine cycle |
| PCHE | printed circuit heat exchanger |
| heat input to the cycle | |
| RBC | recompression Brayton cycle |
| s | specific entropy |
| SiCl4 | silicon tetrachloride |
| TIT | turbine inlet temperature |
| dead-state temperature | |
| VLE | vapor–liquid equilibrium |
| turbine power output | |
| pump power input | |
| compressor power input | |
| exergy efficiency of the cycle | |
| thermal efficiency of the cycle | |
| rate of exergy at any i-th state point in the cycle | |
| rate of exergy loss in turbine | |
| rate of exergy loss in pump | |
| rate of exergy loss in compressor | |
| rate of exergy loss in low-temperature recuperator | |
| rate of exergy loss in high-temperature recuperator | |
| rate of exergy loss in cooler | |
| rate of exergy loss in heater | |
| net rate of exergy loss in condenser | |
| rate of exergy input to the cycle |
References
- Chen, Y.; Lundqvist, P.; Johansson, A.; Platell, P. A Comparative Study of the Carbon Dioxide Transcritical Power Cycle Compared with an Organic Rankine Cycle with R123 as Working Fluid in Waste Heat Recovery. Appl. Therm. Eng. 2006, 26, 2142–2147. [Google Scholar] [CrossRef]
- Santini, L.; Accornero, C.; Cioncolini, A. On the Adoption of Carbon Dioxide Thermodynamic Cycles for Nuclear Power Conversion: A Case Study Applied to Mochovce 3 Nuclear Power Plant. Appl. Energy 2016, 181, 446–463. [Google Scholar] [CrossRef]
- Turchi, C.S.; Ma, Z.; Neises, T.W.; Wagner, M.J. Thermodynamic Study of Advanced Supercritical Carbon Dioxide Power Cycles for Concentrating Solar Power Systems. J. Sol. Energy Eng. 2013, 135, 041007. [Google Scholar] [CrossRef]
- Yang, M.-H.; Yeh, R.-H. Analyzing the Optimization of an Organic Rankine Cycle System for Recovering Waste Heat from a Large Marine Engine Containing a Cooling Water System. Energy Convers. Manag. 2014, 88, 999–1010. [Google Scholar] [CrossRef]
- Cheang, V.T.; Hedderwick, R.A.; McGregor, C. Benchmarking Supercritical Carbon Dioxide Cycles against Steam Rankine Cycles for Concentrated Solar Power. Solar Energy 2015, 113, 199–211. [Google Scholar] [CrossRef]
- Yang, Z.; Le Moullec, Y.; Zhang, J.; Zhang, Y. Dynamic Modeling of 5 MWe Supercritical CO2 Recompression Brayton Cycle. AIP Conf. Proc. 2018, 2033, 70003. [Google Scholar] [CrossRef]
- Khatoon, S.; Ishaque, S.; Kim, M.H. Modeling and Analysis of Air-Cooled Heat Exchanger Integrated with Supercritical Carbon Dioxide Recompression Brayton Cycle. Energy Convers. Manag. 2021, 232, 113895. [Google Scholar] [CrossRef]
- Dostal, V.; Hejzlar, P.; Driscoll, M.J. High-Performance Supercritical Carbon Dioxide Cycle for next-Generation Nuclear Reactors. Nucl. Technol. 2006, 154, 265–282. [Google Scholar] [CrossRef]
- Vesely, L.; Dostal, V.; Hajek, P. Design of Experimental Loop with Supercritical Carbon Dioxide. In Next Generation Reactors and Advanced Reactors: Nuclear Safety and Security, Proceedings of the 22nd International Conference on Nuclear Engineering, Prague, Czech Republic, 7 July 2014; American Society of Mechanical Engineers (ASME): New York, NY, USA, 2014; Volume 3. [Google Scholar]
- Crespi, F.; Sánchez, D.; Rodríguez, J.M.; Gavagnin, G. A Thermo-Economic Methodology to Select SCO2 Power Cycles for CSP Applications. Renew. Energy 2020, 147, 2905–2912. [Google Scholar] [CrossRef]
- Binotti, M.; Astolfi, M.; Campanari, S.; Manzolini, G.; Silva, P. Preliminary Assessment of SCO2 Cycles for Power Generation in CSP Solar Tower Plants. Appl. Energy 2017, 204, 1007–1017. [Google Scholar] [CrossRef]
- Gotelip, T.; Gampe, U.; Glos, S. Optimization Strategies of Different SCO2 Architectures for Gas Turbine Bottoming Cycle Applications. Energy 2022, 250, 123734. [Google Scholar] [CrossRef]
- Manente, G.; Fortuna, F.M. Supercritical CO2 Power Cycles for Waste Heat Recovery: A Systematic Comparison between Traditional and Novel Layouts with Dual Expansion. Energy Convers. Manag. 2019, 197, 111777. [Google Scholar] [CrossRef]
- Romei, A.; Gaetani, P.; Persico, G. Computational Fluid-Dynamic Investigation of a Centrifugal Compressor with Inlet Guide Vanes for Supercritical Carbon Dioxide Power Systems. Energy 2022, 255, 124469. [Google Scholar] [CrossRef]
- Meshram, A.; Jaiswal, A.K.; Khivsara, S.D.; Ortega, J.D.; Ho, C.; Bapat, R.; Dutta, P. Modeling and Analysis of a Printed Circuit Heat Exchanger for Supercritical CO2 Power Cycle Applications. Appl. Therm. Eng. 2016, 109, 861–870. [Google Scholar] [CrossRef]
- Nikitin, K.; Kato, Y.; Ngo, L. Printed Circuit Heat Exchanger Thermal—Hydraulic Performance in Supercritical CO 2 Experimental Loop “Circuit Imprime” Dans Une Boucle Echangeur de Chaleur a’ Rimentale Au CO2 Supracritique: Performance Expe Thermique et Hydraulique. Int. J. Refrig. 2006, 29, 807–814. [Google Scholar] [CrossRef]
- Hu, Z.C.; Wang, K. Effect of Channel Size on the Performance of a PCHE-Based Supercritical CO2 Thermoacoustic Engine. Appl. Therm. Eng. 2024, 236, 121457. [Google Scholar] [CrossRef]
- Arslan, F.; Güzel, B. Numerical and Experimental Thermal–Hydraulic Performance Analysis of a Supercritical CO2 Brayton Cycle PCHE Recuperator. Arab. J. Sci. Eng. 2021, 46, 7543–7556. [Google Scholar] [CrossRef]
- Wang, J.; Yan, X.; Lu, M.; Sun, Y.; Wang, J. Structural Assessment of Printed Circuit Heat Exchangers in Supercritical CO2 Waste Heat Recovery Systems for Ship Applications. J. Therm. Sci. 2022, 31, 689–700. [Google Scholar] [CrossRef]
- Liu, K.; Zhao, F.; Jin, Y.; Hu, C.; Ming, Y.; Liu, Y.; Tian, R.; Liu, S. S–CO2 Heat Transfer Characteristics Analysis in PCHE and Vertical Channel. Prog. Nucl. Energy 2022, 154, 104472. [Google Scholar] [CrossRef]
- Shahrooz, M.; Lundqvist, P.; Nekså, P. Performance of Binary Zeotropic Mixtures in Organic Rankine Cycles (ORCs). Energy Convers. Manag. 2022, 266, 115783. [Google Scholar] [CrossRef]
- Siddiqui, M.E.; Almatrafi, E.; Bamasag, A.; Saeed, U. Adoption of CO2-Based Binary Mixture to Operate Transcritical Rankine Cycle in Warm Regions. Renew Energy 2022, 199, 1372–1380. [Google Scholar] [CrossRef]
- Haroon, M.; Sheikh, N.A.; Ayub, A.; Tariq, R.; Sher, F.; Baheta, A.T.; Imran, M. Exergetic, Economic and Exergo-Environmental Analysis of Bottoming Power Cycles Operating with CO2-Based Binary Mixture. Energies 2020, 13, 5080. [Google Scholar] [CrossRef]
- Niu, X.; Ma, N.; Bu, Z.; Hong, W.; Li, H. Thermodynamic Analysis of Supercritical Brayton Cycles Using CO2-Based Binary Mixtures for Solar Power Tower System Application. Energy 2022, 254, 124286. [Google Scholar] [CrossRef]
- Shu, G.; Yu, Z.; Tian, H.; Liu, P.; Xu, Z. Potential of the Transcritical Rankine Cycle Using CO2-Based Binary Zeotropic Mixtures for Engine’s Waste Heat Recovery. Energy Convers. Manag. 2018, 174, 668–685. [Google Scholar] [CrossRef]
- Invernizzi, C.M.; Iora, P.; Manzolini, G.; Lasala, S. Thermal Stability of N-Pentane, Cyclo-Pentane and Toluene as Working Fluids in Organic Rankine Engines. Appl. Therm. Eng. 2017, 121, 172–179. [Google Scholar] [CrossRef]
- Calderazzi, L.; Colonna di Paliano, P. Thermal Stability of R-134a, R-141b, R-13I1, R-7146, R-125 Associated with Stainless Steel as a Containing Material. Int. J. Refrig. 1997, 20, 381–389. [Google Scholar] [CrossRef]
- Angelino, G.; Invernizzi, C. Experimental Investigation on the Thermal Stability of Some New Zero ODP Refrigerants. Int. J. Refrig. 2003, 26, 51–58. [Google Scholar] [CrossRef]
- Huo, E.; Liu, C.; Xin, L.; Li, X.; Xu, X.; Li, Q.; Wang, S.; Dang, C. Thermal Stability and Decomposition Mechanism of HFO-1336mzz(Z) as an Environmental Friendly Working Fluid: Experimental and Theoretical Study. Int. J. Energy Res. 2019, 43, 4630–4643. [Google Scholar] [CrossRef]
- Liu, J.; Liu, Y.; Liu, C.; Xin, L.; Yu, W. Experimental and Theoretical Study on Thermal Stability of Mixture R1234ze(E)/R32 in Organic Rankine Cycle. J. Therm. Sci. 2023, 32, 1595–1613. [Google Scholar] [CrossRef]
- Irriyanto, M.Z.; Lim, H.S.; Choi, B.S.; Myint, A.A.; Kim, J. Thermal Stability and Decomposition Behavior of HFO-1234ze(E) as a Working Fluid in the Supercritical Organic Rankine Cycle. J. Supercrit. Fluids 2019, 154, 104602. [Google Scholar] [CrossRef]
- Invernizzi, C.M.; Iora, P.; Preßinger, M.; Manzolini, G. HFOs as Substitute for R-134a as Working Fluids in ORC Power Plants: A Thermodynamic Assessment and Thermal Stability Analysis. Appl. Therm. Eng. 2016, 103, 790–797. [Google Scholar] [CrossRef]
- Invernizzi, C.; Binotti, M.; Bombarda, P.; Marcoberardino, G.D.; Iora, P.; Manzolini, G. Water Mixtures as Working Fluids in Organic Rankine Cycles. Energies 2019, 12, 2629. [Google Scholar] [CrossRef]
- Pasetti, M.; Invernizzi, C.M.; Iora, P. Thermal Stability of Working Fluids for Organic Rankine Cycles: An Improved Survey Method and Experimental Results for Cyclopentane, Isopentane and n-Butane. Appl. Therm. Eng. 2014, 73, 764–774. [Google Scholar] [CrossRef]
- Dai, X.; Shi, L.; An, Q.; Qian, W. Screening of Hydrocarbons as Supercritical ORCs Working Fluids by Thermal Stability. Energy Convers. Manag. 2016, 126, 632–637. [Google Scholar] [CrossRef]
- Lasala, S.; Invernizzi, C.; Iora, P.; Chiesa, P.; Macchi, E. Thermal Stability Analysis of Perfluorohexane. Energy Procedia 2015, 75, 1575–1582. [Google Scholar] [CrossRef]
- Doninelli, M.; Di Marcoberardino, G.; Iora, P.; Gelfi, M.; Invernizzi, C.M.; Manzolini, G. Silicon Tetrachloride as Innovative Working Fluid for High Temperature Rankine Cycles: Thermal Stability, Material Compatibility, and Energy Analysis. Appl. Therm. Eng. 2024, 249, 123239. [Google Scholar] [CrossRef]
- Doninelli, M.; Morosini, E.; Di Marcoberardino, G.; Invernizzi, C.M.; Iora, P.; Riva, M.; Stringari, P.; Manzolini, G. Experimental Investigation of the CO2 + SiCl4 Mixture as Innovative Working Fluid for Power Cycles: Bubble Points and Liquid Density Measurements. Energy 2024, 299, 131197. [Google Scholar] [CrossRef]
- Wan, Y.; Zhao, X.; Yan, D.; Yang, D.; Li, Y.; Guo, S. Research and Preparation of Ultra Purity Silicon Tetrachloride. AIP Conf. Proc. 2017, 1890, 40026. [Google Scholar] [CrossRef]
- Okamoto, Y.; Sumiya, M.; Nakamura, Y.; Suzuki, Y. Effective Silicon Production from SiCl4 Source Using Hydrogen Radicals Generated and Transported at Atmospheric Pressure. Sci. Technol. Adv. Mater. 2020, 21, 482–491. [Google Scholar] [CrossRef]
- Cheng, C.; Zhang, C.; Jiang, J.; Ma, E.; Bai, J.; Wang, J. Raman Spectroscopy Characterization of Dissolved Polysilicon Byproduct SiCl4 in Ionic Liquids. J. Spectrosc. 2018, 2018, 2329189. [Google Scholar] [CrossRef]
- Siddiqui, M.E.; Almitani, K.H. Proposal and Thermodynamic Assessment of S-CO2 Brayton Cycle Layout for Improved Heat Recovery. Entropy 2020, 22, 305. [Google Scholar] [CrossRef]
- Astolfi, M.; Alfani, D.; Lasala, S.; Macchi, E. Comparison between ORC and CO2 Power Systems for the Exploitation of Low-Medium Temperature Heat Sources. Energy 2018, 161, 1250–1261. [Google Scholar] [CrossRef]
- Alfani, D.; Astolfi, M.; Binotti, M.; Silva, P.; Macchi, E. Off-Design Performance of CSP Plant Based on Supercritical CO2 Cycles. AIP Conf. Proc. 2020, 2303, 130001. [Google Scholar] [CrossRef]
- Siddiqui, M.E.; Taimoor, A.A.; Almitani, K.H. Energy and Exergy Analysis of the S-CO2 Brayton Cycle Coupled with Bottoming Cycles. Processes 2018, 6, 153. [Google Scholar] [CrossRef]

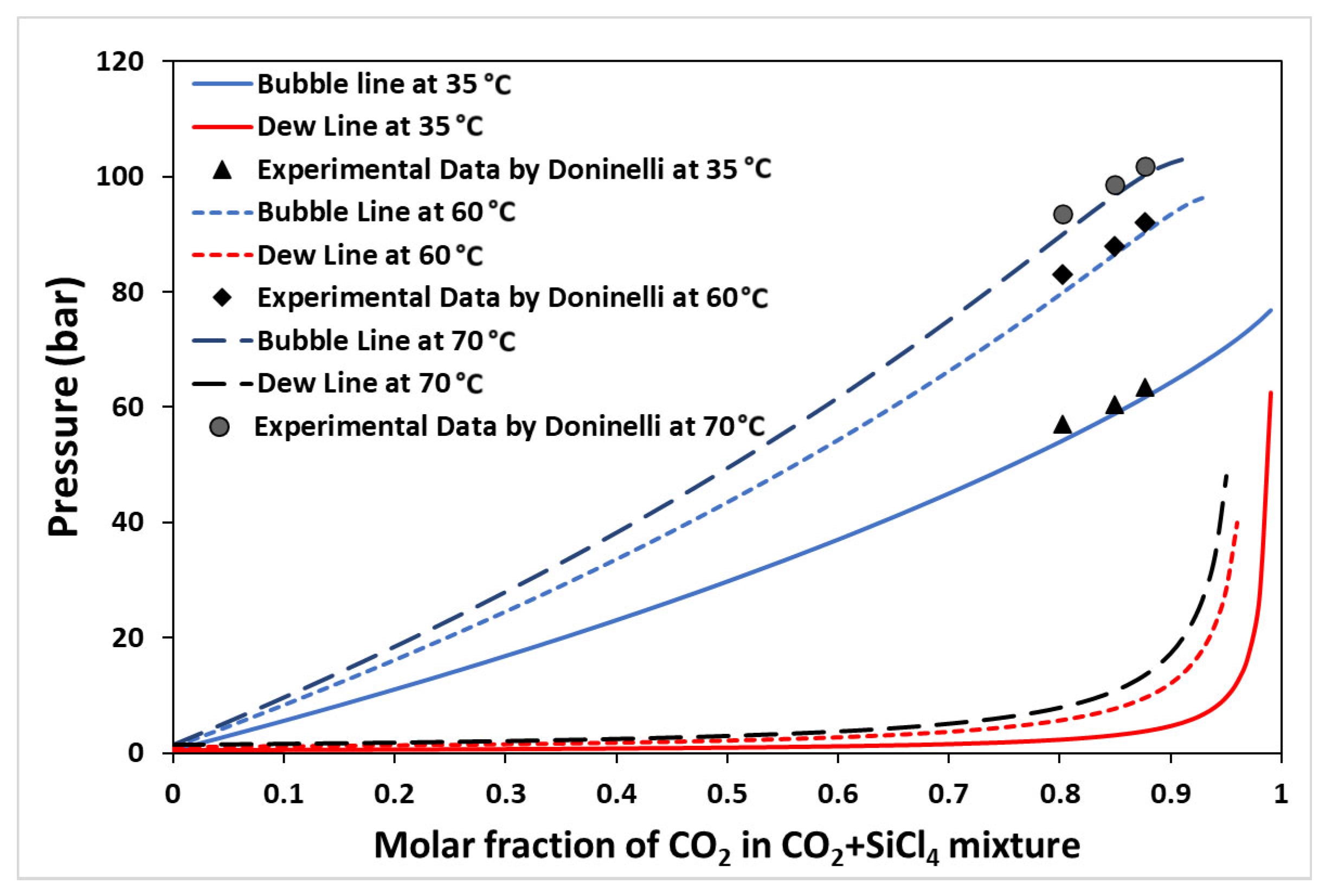

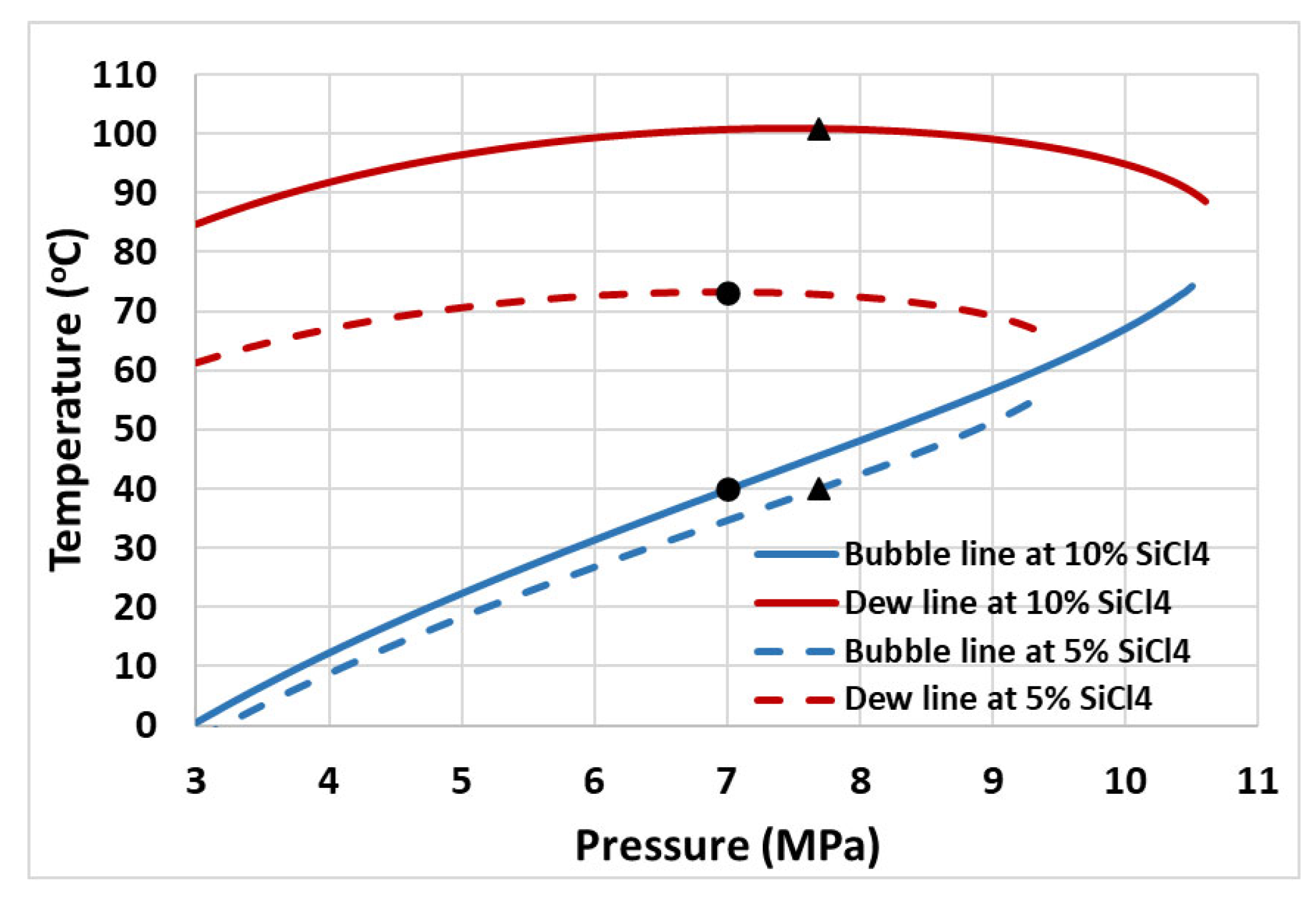
| Organic Fluids | Chemical Formula | Thermal Stability Cutoff (°C) | Reference |
|---|---|---|---|
| R-1311 | CF3I | 102 | [27] |
| R-141b | C2H3Cl2F | 90 | [27] |
| HFC-245fa | C3F5H3 | 300–320 | [28] |
| HFC-143a | C2H3F3 | 350 | [28] |
| HFC-236fa | C3H2F6 | 400 | [28] |
| HFC-23 | CHF3 | 400 | [28] |
| HFC-227ea | C3HF7 | 425 | [28] |
| HFC-134a | C2H2F4 | 368 | [27] |
| HFO-1336mzz(Z) | C4H2F6 | 250–270 | [29] |
| HFO-1336mzz(E) | C4H2F6 | 230–250 | [30] |
| HFO-1234ze(E) | C3H2F4 | 180 | [31] |
| HFO-1234yf | C3F4H2 | 170–200 | [32] |
| HFC-32 | CH2F2 | 250–270 | [30] |
| HFC-125 | CH2F5 | 396 | [27] |
| TFE | C2H3F3O | 250 | [33] |
| n-butanol | C4H10O | 220 | [33] |
| n-butane | C4H10 | 290 | [34] |
| Isopentane | C5H12 | 290 | [34] |
| Cyclopentane | C5H10 | 350 | [34] |
| n-pentane | C5H12 | 280–320 | [26,27] |
| Toluene | C7H8 | 400 | [26] |
| n-hexane | C6H14 | 260–280 | [35] |
| Perfluorohexane | C6F14 | 350–400 | [36] |
| Molar Fraction of CO2 in CO2 + SiCl4 Mixture | Mixture Temperature | ||
|---|---|---|---|
| 35 °C | 60 °C | 70 °C | |
| 0.80 | 5.5% | 4.2% | 4.2% |
| 0.85 | 2.6% | 1.5% | 1.8% |
| 0.88 | 2.0% | 1.4% | 1.2% |
| Cycle Components/Parameters | Operating Conditions |
|---|---|
| Source Temperature | 550 °C (Air) |
| Coolant Temperature | 35 °C (Water) |
| Turbine Inlet Temperature | 500 °C |
| Cycle Maximum Pressure (State 1) | 25 MPa |
| Cycle Minimum Temperature (State 5) | 40 °C |
| Cycle Minimum Pressure (State 5) | Saturation Pressure at 40 °C |
| Minimum Pinch Temperature in HTR, LTR, and Cooler | 5 °C [38] |
| Minimum Pinch Temperature in Heater | 10 °C |
| Turbine Isentropic Efficiency | 92% [38] |
| Pump/Compressor Isentropic Efficiency | 88% [38] |
| Pressure Loss in Heat Exchangers and in Pipelines | Neglected |
| Dead State | 101 kPa; 35 °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Siddiqui, M.E.; Almitani, K.H. Performance Evaluation of CO2 + SiCl4 Binary Mixture in Recompression Brayton Cycle for Warm Climates. Processes 2024, 12, 2155. https://doi.org/10.3390/pr12102155
Siddiqui ME, Almitani KH. Performance Evaluation of CO2 + SiCl4 Binary Mixture in Recompression Brayton Cycle for Warm Climates. Processes. 2024; 12(10):2155. https://doi.org/10.3390/pr12102155
Chicago/Turabian StyleSiddiqui, Muhammad Ehtisham, and Khalid H. Almitani. 2024. "Performance Evaluation of CO2 + SiCl4 Binary Mixture in Recompression Brayton Cycle for Warm Climates" Processes 12, no. 10: 2155. https://doi.org/10.3390/pr12102155
APA StyleSiddiqui, M. E., & Almitani, K. H. (2024). Performance Evaluation of CO2 + SiCl4 Binary Mixture in Recompression Brayton Cycle for Warm Climates. Processes, 12(10), 2155. https://doi.org/10.3390/pr12102155







