Numerical Prediction of Refrigerant Oil Two-Phase Flow from Scroll Compressor Discharge to the Suction Side via Back Pressure Chamber
Abstract
:1. Introduction
2. Modelling Approach
2.1. Geometry
2.2. Critical Flow
2.3. Subcritical Two-Phase Flow through Nozzles
2.4. Refrigerant R744 (CO2) Equation of State
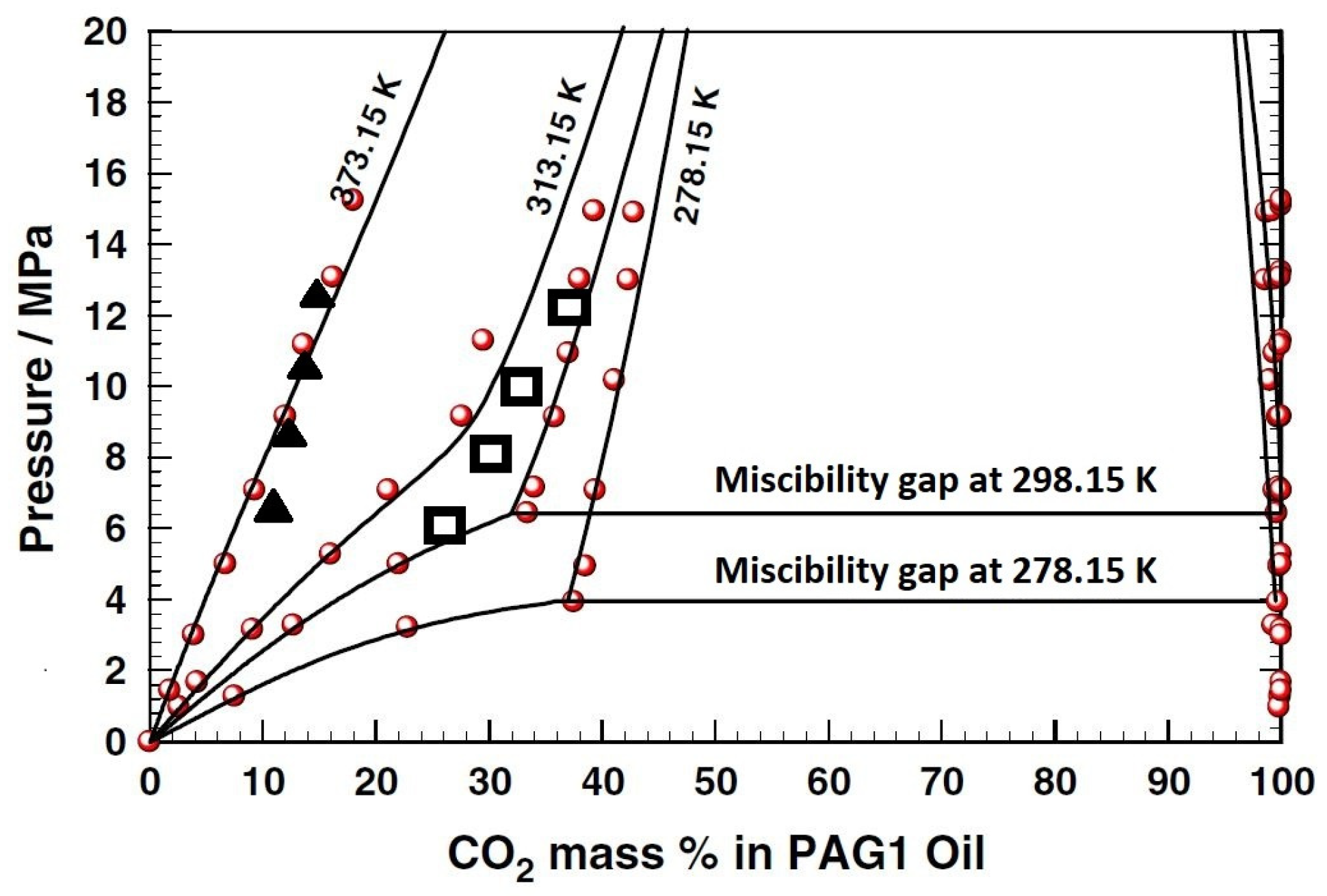
2.5. Solubility of CO2 and PAG Oil
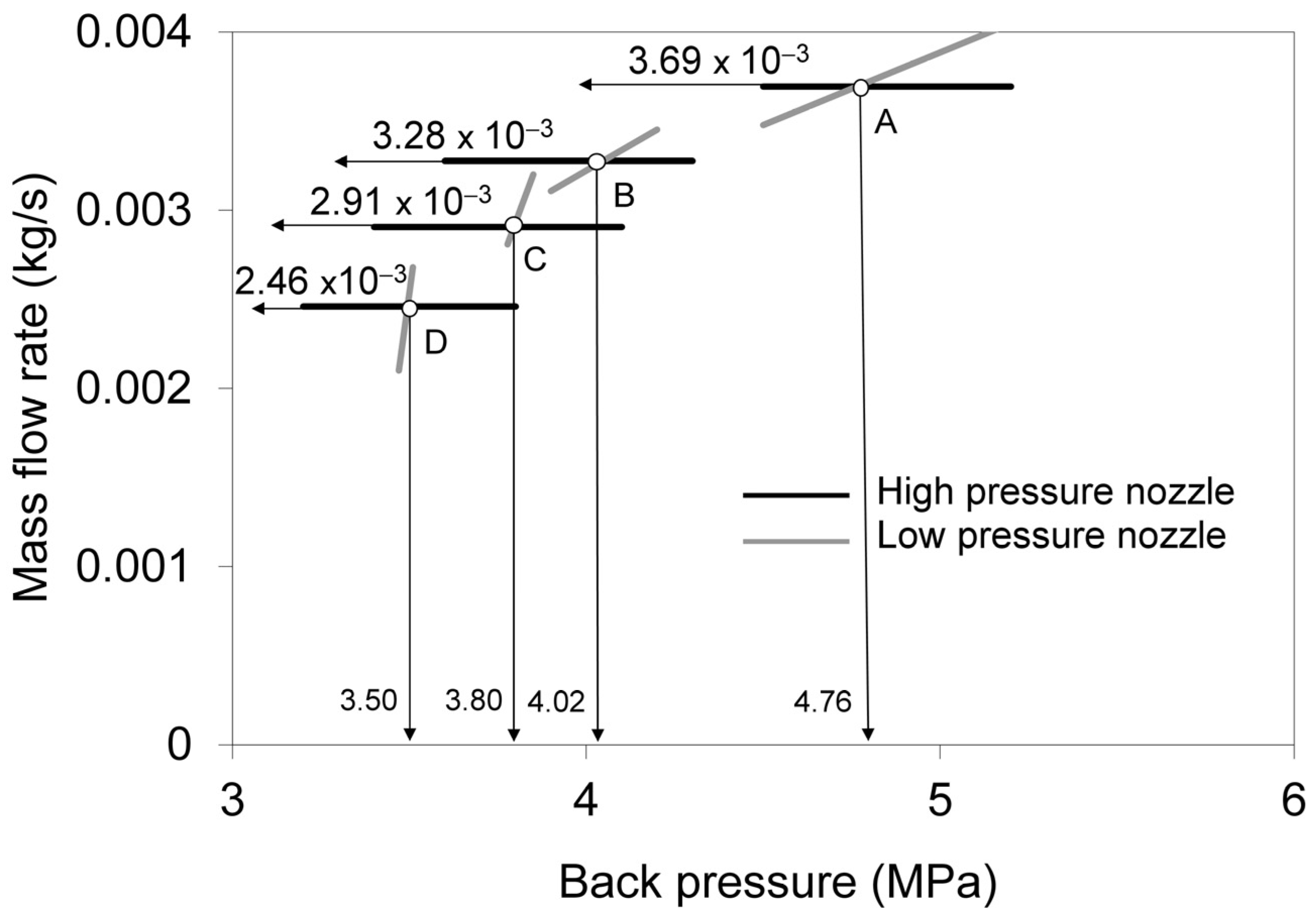
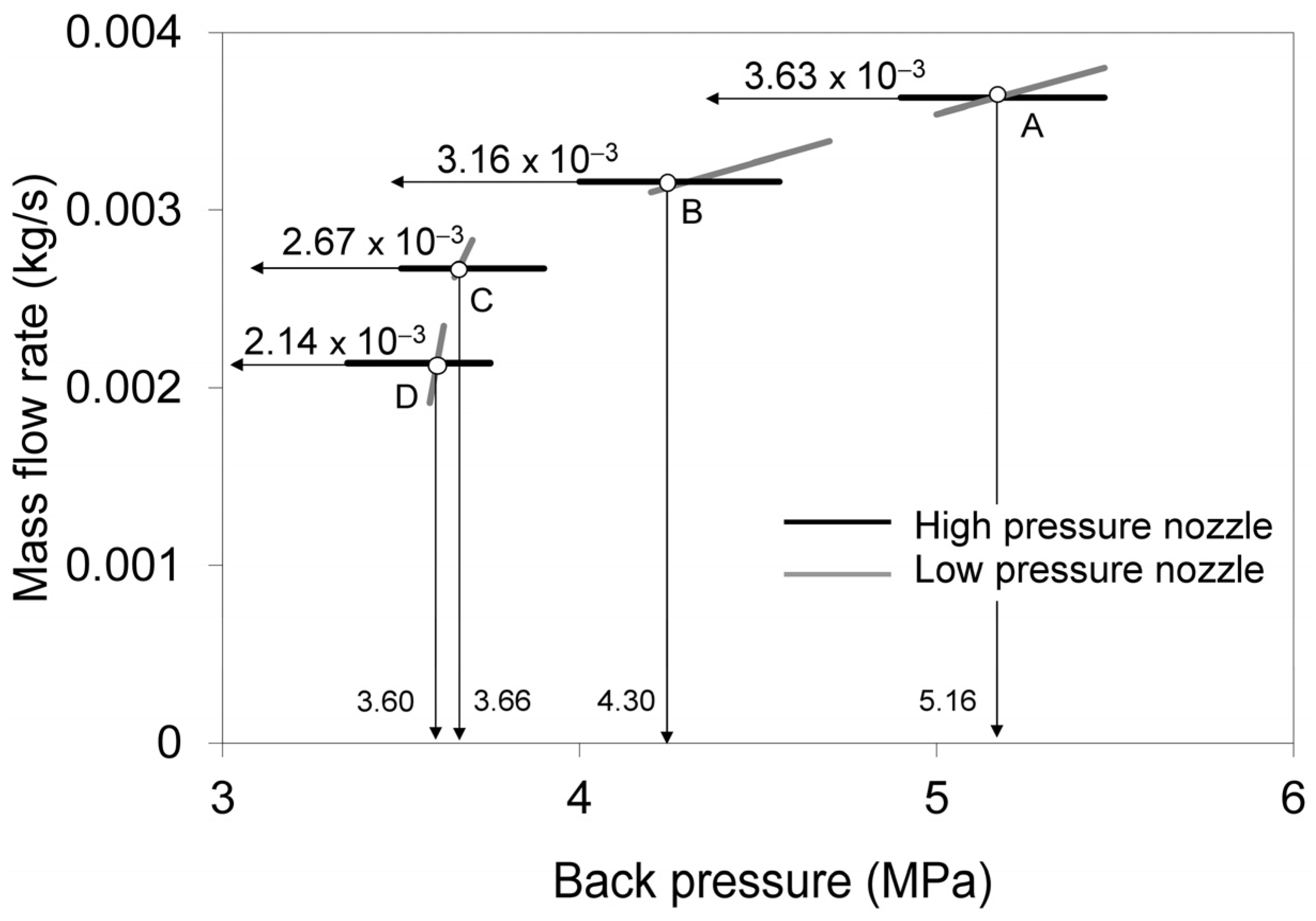
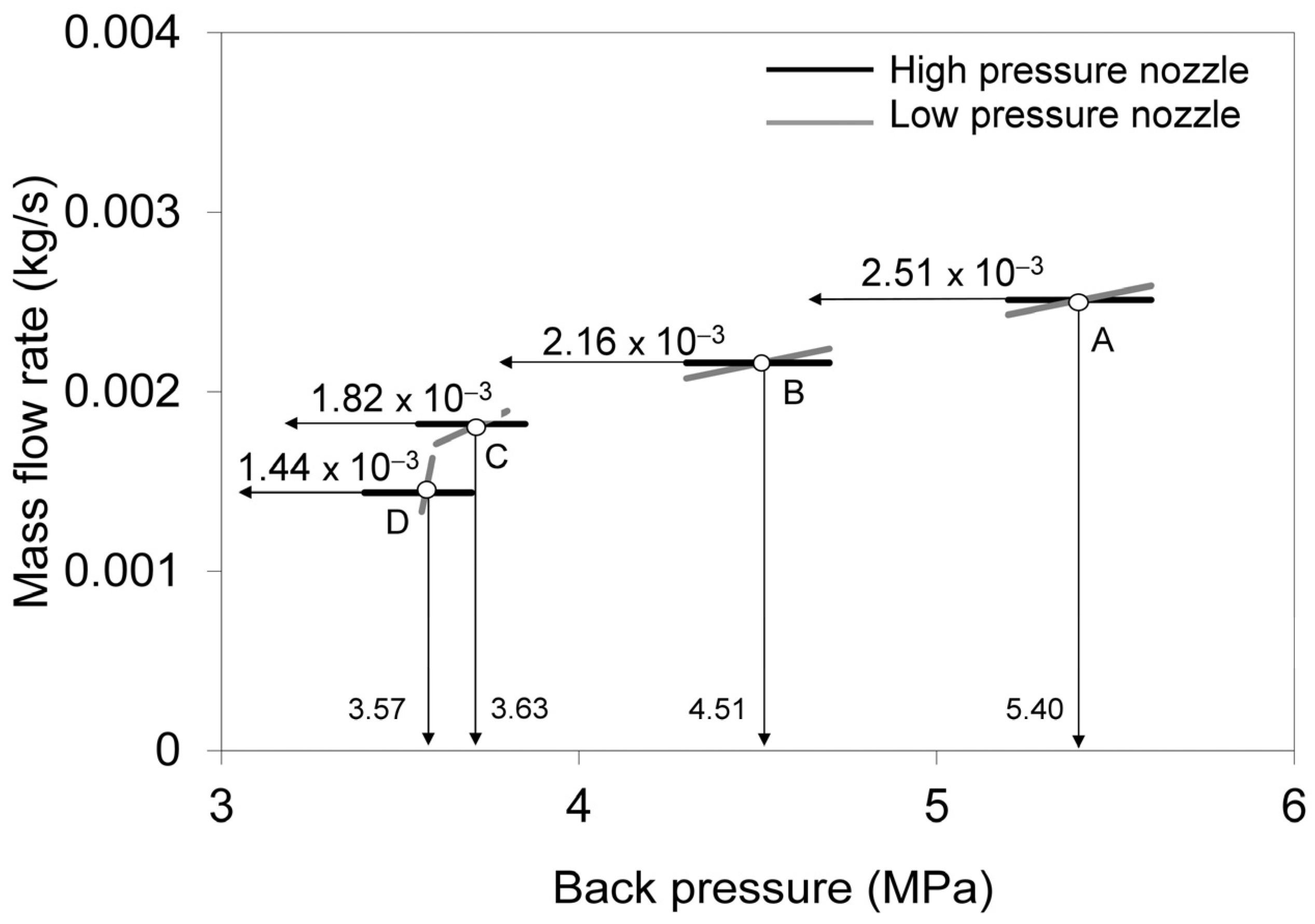
3. Results
4. Conclusions
- The refrigerant oil two-phase flow from the oil separator to the back pressure chamber is critical. The refrigerant oil two-phase flow from the back pressure chamber to the compressor suction side is generally critical, but it becomes subcritical for lower discharge pressures and greater oil mass fractions. In all cases with the discharge pressure equal to 6 MPa, the flow through the nozzle between the back pressure chamber and the suction side is subcritical, while the increase in the oil mass fraction in the oil separator to 80% leads also to a subcritical flow in cases with an 8 MPa pressure in the oil separator.
- The increase in the oil mass fraction in the oil separator was found to lead to an increase in the mass flow rate from the oil separator to the compressor suction side, but to a decrease in the pressure value in the back pressure chamber. The oil mass fraction increase from 10% to 80% increases the mass flow rate by more than two times.
- The refrigerant dissolvement in oil contributes to a slight increase in the refrigerant oil two-phase mass flow rate and to a decrease in the back pressure. This mass flow rate change by refrigerant dissolvement in oil is more pronounced for greater oil mass fractions and lower compressor discharge pressures. For instance, the greatest increase in the mass flow rate by 15% is obtained for 80% oil mass fraction and 6 MPa in the oil separator. The back pressure change by refrigerant dissolvement in oil is more pronounced for greater oil mass fractions and higher discharge pressures. For instance, the greatest back pressure decrease—8%—was obtained for an 80% oil mass fraction and 12 MPa in the oil separator. In case of low oil mass fraction, the refrigerant dissolvement in oil has a negligible influence on flow parameters.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area of flow cross-section, [m2] |
| C | concentration coefficient, [-] |
| constant in Equation (23), [-] | |
| c | sonic velocity, [m/s] |
| cp | specific isobaric heat capacity, [J/(kgK)] |
| Dh | hydraulic diameter, [m] |
| F | constant in in Equations (21), (24) and (25), [-] |
| g | mass fraction in the mixture with refrigerant, [-] |
| h | specific enthalpy, [J/kg] |
| M | molar mass, [kg/mol] |
| mass flow rate, [kg/s] | |
| P | perimeter, [m] |
| p | pressure, [Pa] |
| r | ratio of nozzle downstream and upstream pressure, [-] |
| R | universal gas constant, [J/(molK)] |
| Rg | gas constant, [J/(kgK)] |
| S | velocity slip, [-] |
| T | temperature, [K] |
| u | velocity, [m/s] |
| Vm | molar volume, [m3/mol] |
| Y | the reciprocal of the Lockhart–Martinelli parameter, [-] |
| Z | constant in in Equations (23) and (24), [-] |
| Greek letters | |
| α | gas-phase volume fraction in two-phase mixture, [-] |
| ζ | local flow resistance coefficient, [-] |
| k | isentropic coefficient, [-] |
| n | kinematic viscosity, [m2/s] |
| ρ | density, [kg/m3] |
| the two-phase multiplier, [-] | |
| χ | flow quality, [-] |
| ψ | acentric factor, [-] |
| ω | refrigerant mass fraction in the mixture with oil, [-] |
| Indices | |
| 1 | liquid phase |
| 2 | gas phase |
| BP | in the back pressure chamber |
| c | the location of critical flow |
| cr | critical state |
| h | isenthalpic process |
| in | in front of the high-pressure nozzle |
| o | at the nozzle, |
| Oil | |
| p | isobaric process |
| R | refrigerant |
| s | isentropic process |
| u | the upstream location |
| Abbreviations | |
| PAG | polyalkylene glycol oil |
Appendix A
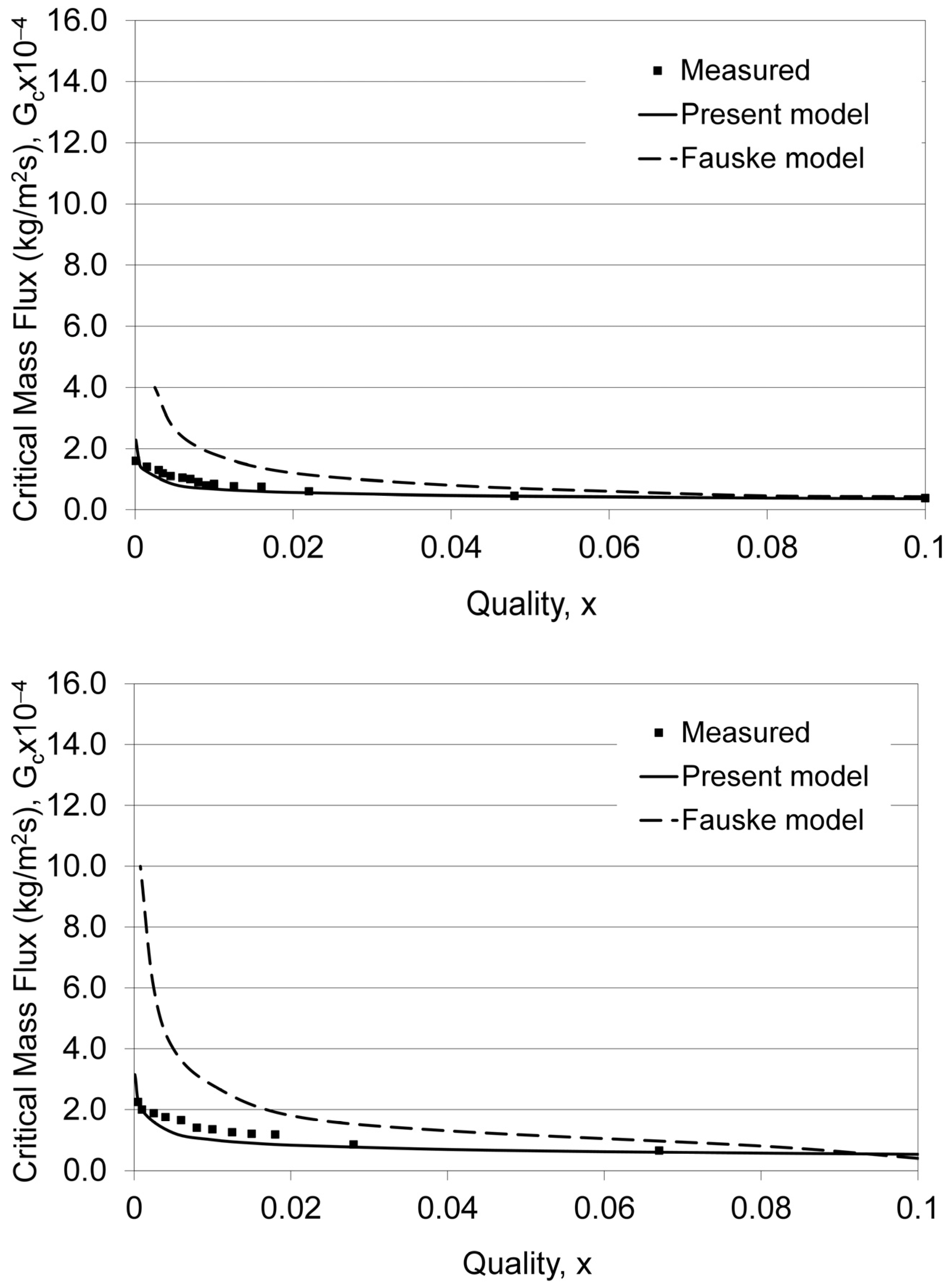
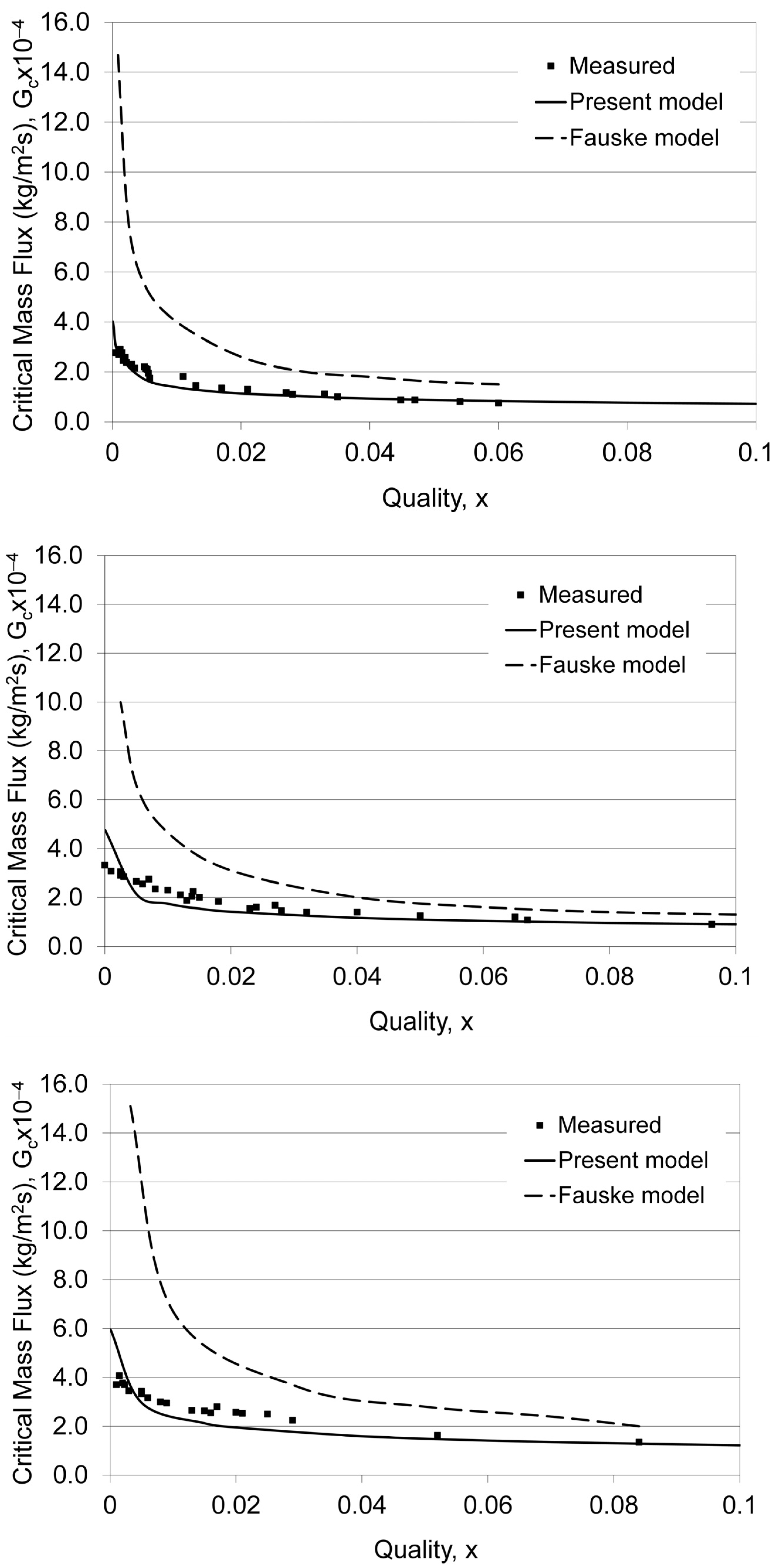
References
- Richardson, H.; Gatecliff, G. Comparison of the high side vs low side scroll compressor design. In Proceedings of the International Compressor Engineering at Purdue, West Lafayette, IN, USA, 14–17 July 1992; Available online: https://docs.lib.purdue.edu/icec/852 (accessed on 17 November 2023).
- Tang, Y.; Hung, C.; Chang, Y. Performance improvements in low side scroll compressor with extended operation speeds. Appl. Therm. Eng. 2011, 31, 3542–3551. [Google Scholar] [CrossRef]
- Qian, Z.; Zhang, Z. Back-pressure mechanism of scroll compressor. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1992; Available online: https://docs.lib.purdue.edu/icec/909 (accessed on 17 November 2023).
- Ghiaasiaan, S.M. Two-Phase Flow, Boiling and Condensation; Cambridge University Press: Cambridge, UK, 2008. [Google Scholar]
- Kim, Y.; O’Neal, D.L. The effect of oil on the two-phase critical flow of Refrigerant 134a through short tube orifices. Int. J. Heat Mass Transf. 1994, 37, 1377–1385. [Google Scholar]
- Zhang, Z.; Tian, L.; Tong, L.; Chen, Y. Choked Flow Characteristics of Subcritical Refrigerant Flowing Through Converging-Diverging Nozzles. Entropy 2014, 16, 5810–5821. [Google Scholar] [CrossRef]
- Kim, M.K.; Lee, H.; Jeong, J.H. Evaluation of correlations for mass flow rate of refrigerant through electronic expansion valve in air—Water heat pump system using R32. Int. J. Refrig. 2023, 151, 267–277. [Google Scholar] [CrossRef]
- Nikolov, A.; Brümmer, A. Two-phase mass flow rate through restrictions in liquid-flooded twin-screw compressors or expanders. Int. J. Refrig. 2023, 148, 152–167. [Google Scholar] [CrossRef]
- Tojo, K.; Hayase, I. Self-Adjusting Back Pressure Mechanism for Scroll Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 23–26 July 1996; Available online: https://docs.lib.purdue.edu/icec/1356/ (accessed on 17 November 2023).
- Tojo, K.; Hayase, I.; Tsuchiya, T. Improvement of Self-Adjusting Back Pressure Mechanism for Scroll Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 14–17 July 1998; Available online: https://docs.lib.purdue.edu/icec/1499/ (accessed on 17 November 2023).
- Beskok, A.; Karniadakis, G.; Trimmer, W. Rarefaction and Compressibility Effects in Gas Microflows. J. Fluids Eng. 1996, 118, 448–456. [Google Scholar] [CrossRef]
- Yoon, H.J.; Ishii, M.; Revankar, S.T. Choking flow modelling with mechanical non-equilibrium for two-phase two-component flow. Nucl. Eng. Des. 2006, 236, 1886–1901. [Google Scholar] [CrossRef]
- Grolmes, M.A.; Fauske, H.K. Comparison of the Propagation Characteristics of Compression and Rarefaction Pressure Pulses in Two-Phase, One-Component Bubble Flow. Trans. Am. Nucl. Soc. 1968, 11, 6833. [Google Scholar]
- Whalley, P.B. Two-Phase Flow and Heat Transfer; Oxford University Press: Oxford, UK, 1996. [Google Scholar]
- Chisholm, D. Two-Phase Flow in Pipelines and Heat Exchangers; George Godwin: London, UK, 1983; pp. 200–214. [Google Scholar]
- Idelchik, I.E. Handbook of Hydraulic Resistance; Mashinostroenie: Moscow, Russia, 1992; p. 175. (In Russian) [Google Scholar]
- Peng, D.Y.; Robinson, D.B. A new two-constant equation of state. Ind. Eng. Chem. Fundam. 1976, 15, 59–64. [Google Scholar] [CrossRef]
- Yokozeki, A. Solubility correlation and phase behaviours of carbon dioxide and lubricant oil mixtures. Appl. Energy 2007, 84, 159–175. [Google Scholar] [CrossRef]
- Dang, C.; Arai, S. Numerical simulation of two-phase flow pattern of supercritical carbon dioxide with PAG-type lubricating oil in gas cooler. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 16–19 July 2012; Available online: http://docs.lib.purdue.edu/iracc/1339 (accessed on 17 November 2023).
- Petrovic, M.M.; Stevanovic, V.D. Two-component two-phase critical flow. FME Trans. 2016, 44, 109–114. [Google Scholar] [CrossRef]
- Fauske, H.K. Contribution to the Theory of Two-Phase, One-Component Critical Flow; Argonne National Laboratory Report ANL-6633; Argonne National Lab.: Lemont, IL, USA, 1962. [Google Scholar]
- Henry, R.E.; Fauske, H.K. The two-phase critical flow of one composition mixtures in nozzle, orifices, and short tubes. J. Heat Transf. 1971, 93, 179–187. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stevanovic, V.D.; Petrovic, M.M.; Cucuz, S.; Milivojevic, S.; Ilic, M. Numerical Prediction of Refrigerant Oil Two-Phase Flow from Scroll Compressor Discharge to the Suction Side via Back Pressure Chamber. Processes 2024, 12, 6. https://doi.org/10.3390/pr12010006
Stevanovic VD, Petrovic MM, Cucuz S, Milivojevic S, Ilic M. Numerical Prediction of Refrigerant Oil Two-Phase Flow from Scroll Compressor Discharge to the Suction Side via Back Pressure Chamber. Processes. 2024; 12(1):6. https://doi.org/10.3390/pr12010006
Chicago/Turabian StyleStevanovic, Vladimir D., Milan M. Petrovic, Stojan Cucuz, Sanja Milivojevic, and Milica Ilic. 2024. "Numerical Prediction of Refrigerant Oil Two-Phase Flow from Scroll Compressor Discharge to the Suction Side via Back Pressure Chamber" Processes 12, no. 1: 6. https://doi.org/10.3390/pr12010006
APA StyleStevanovic, V. D., Petrovic, M. M., Cucuz, S., Milivojevic, S., & Ilic, M. (2024). Numerical Prediction of Refrigerant Oil Two-Phase Flow from Scroll Compressor Discharge to the Suction Side via Back Pressure Chamber. Processes, 12(1), 6. https://doi.org/10.3390/pr12010006







