Modified Model and Simulation Verification of Rock-Fatigue Damage Considering Repeated Discharge Impact
Abstract
1. Introduction
2. Existing Rock Damage Constitutive Model
2.1. TCK Model
2.2. Existing Problems
3. Model Correction
3.1. Model Improvement Formula
3.1.1. Rock Micro-Crack Cracking Degree
3.1.2. Tensile Strength Attenuation Formula
3.2. Fracture Pressure Weakening
3.2.1. Rock Samples
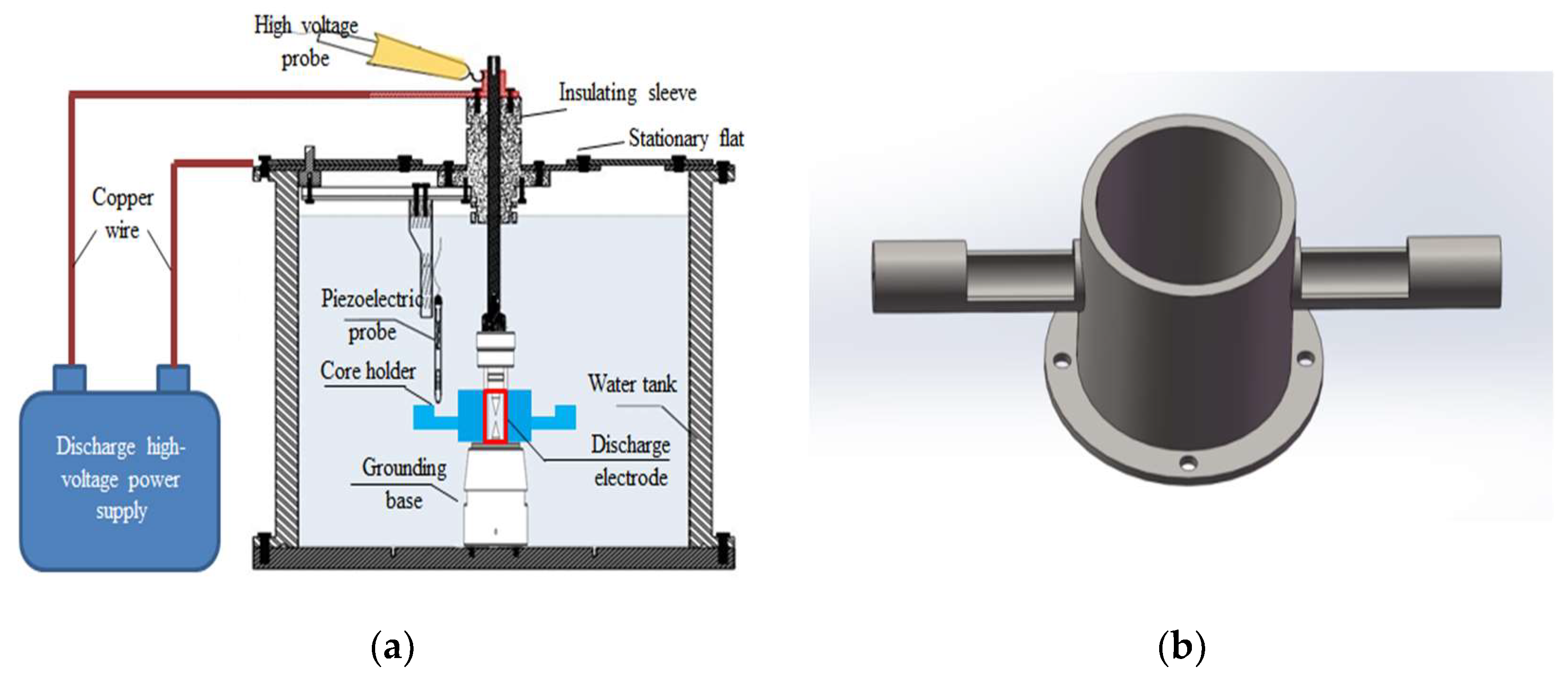
3.2.2. Discharge Test Platform
3.2.3. Test Scheme
3.2.4. Variation of Rock Mechanical Properties
3.2.5. Quantitative Characterization of Shock Wave Effect
3.3. Example Analysis
4. Simulation and Verification
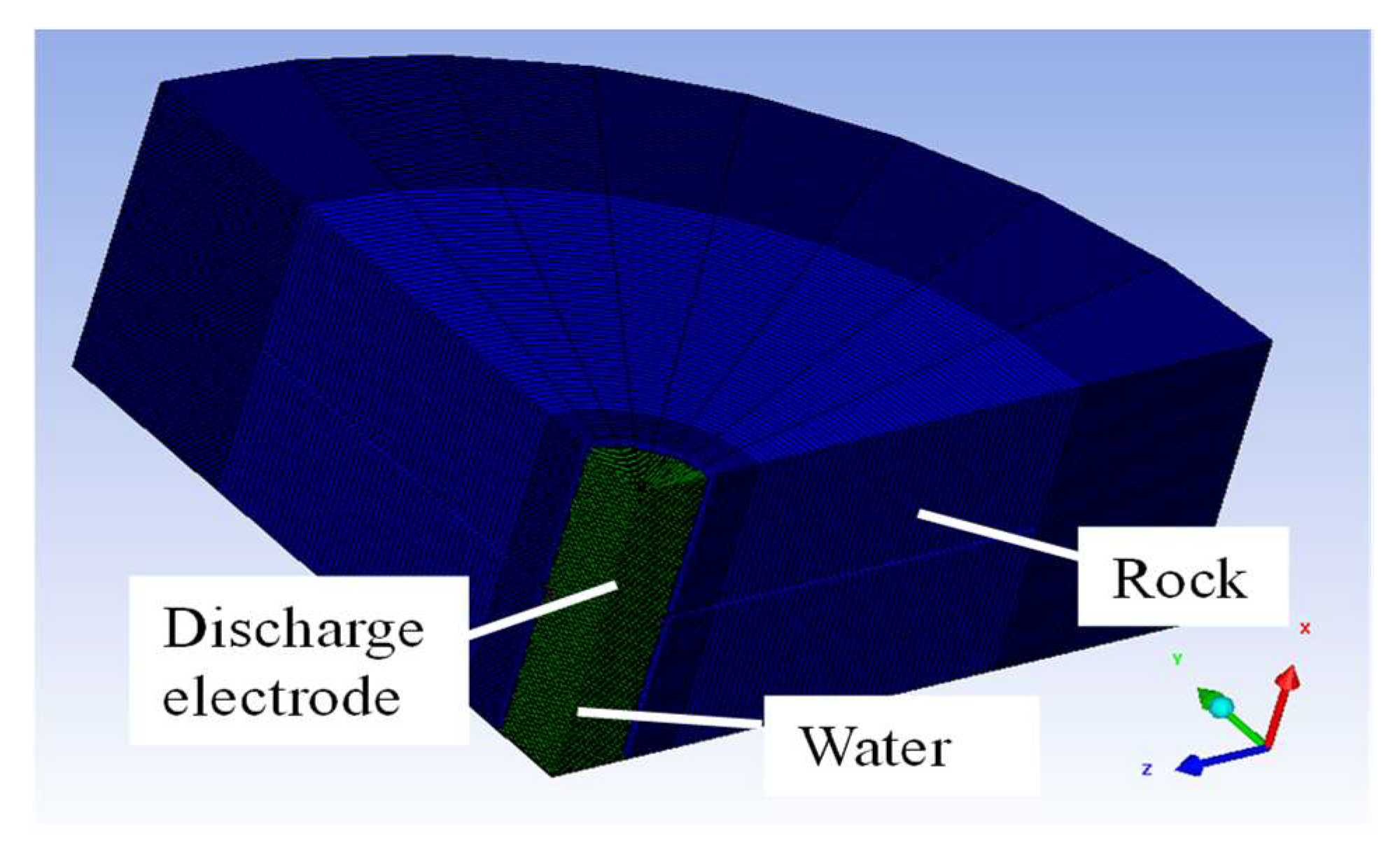
4.1. Simulation Model Establishment
4.1.1. Modelling
4.1.2. Discharge Shock Wave Energy Equivalent
4.2. Analysis of Simulation Results
- Under different discharge impact conditions, the crack initiation orientation of rock is basically the same, and the final parameters of crack are different due to the influence of discharge energy and discharge times.
- Under the same discharge energy condition, with the crease of discharge time, the crack length and width increase. However, when it increases to a certain extent, it will not continue to expand, but generate new cracks, indicating that the increase of discharge times is helpful to form new cracks.
- Under the same discharge times, with the crease of discharge energy, the crack initiation time in the model is earlier, the crack length is longer, and the width is wider, indicating that the higher the discharge energy, the more serious the rock damage and failure effect.
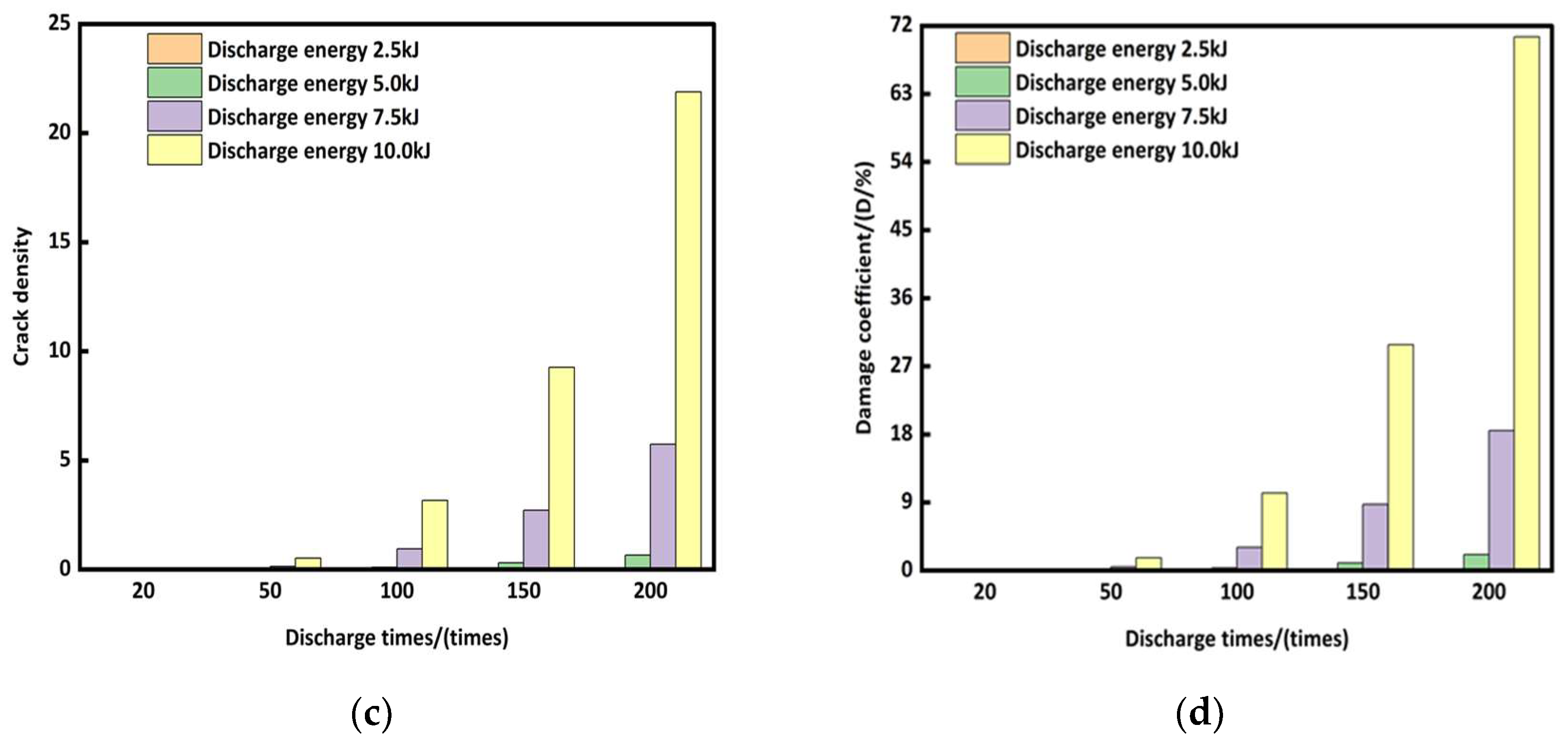
- When the discharge energy is less than or equal to 5.0 kJ, the increase of the number of discharges does not increase the number of cracks, but extends the length of the generated cracks. When the discharge energy is greater than or equal to 7.5 kJ, the initial crack length and width increase to a certain extent, and new cracks will be generated.
- The crack growth presents a staged pattern, with discharge times of 0–50 times in the crack initiation state, 50–150 times in the crack rapid extension state, and 150–200 times in the original crack reaching the extension limit, which is accompanied by the formation of some new cracks.
4.3. Comparison of Calculation Results
| Discharge Energy | 20 | 50 | 100 | 150 | 200 |
|---|---|---|---|---|---|
| 2.5 kJ | 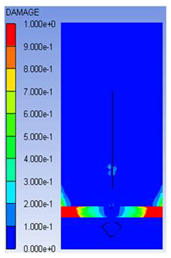 |  | 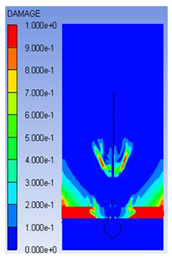 |  | 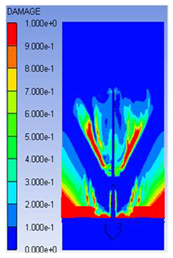 |
| 5.0 kJ |  | 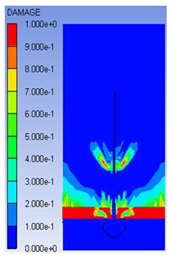 | 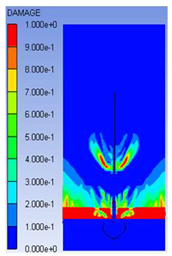 | 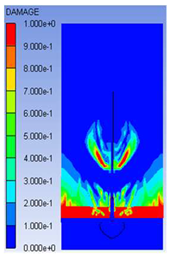 |  |
| 7.5 kJ | 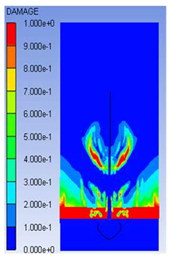 |  | 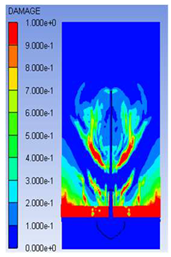 | 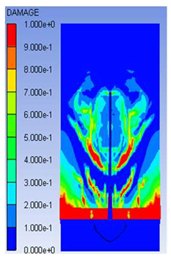 | 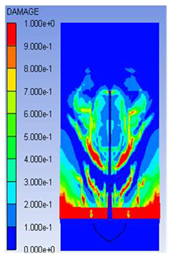 |
| 10.0 kJ | 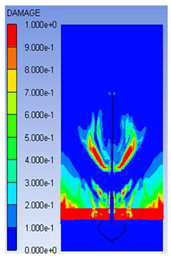 | 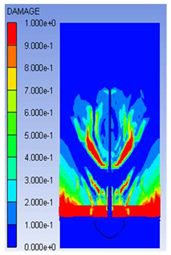 | 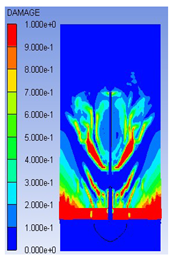 | 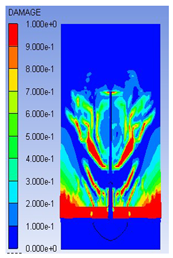 |  |
5. Conclusions
- Under the action of the discharge shock wave, the rock has micro-cracks. The higher the discharge energy and the more the number of times, the greater the tensile stress value generated at the crack tip, and the easier the crack is to crack. This shows that the method of repeated discharge impact fatigue damage to rock is effective and technically feasible, and the higher the discharge energy and the number of times, the better the damage effect.
- Considering rock failure damage and its fatigue effect, this paper points out the deficiency of dynamic damage calculation of TCK model criteria under repeated discharge, characterizes rock damage factor D in the way of the weakening degree of rock mechanical properties; and establishes a correction method that can truly and quantitatively reflect rock fatigue damage effect, micro-crack formation state, and the weakening degree of mechanical parameters under different discharge energy and discharge times.
- The results of the modified model are verified by establishing a simulation model that is consistent with the test materials and test conditions, and the results are basically consistent, confirming that the modified model is correct and feasible. At the same time, the simulation results show that the crack initiation time of high discharge energy is earlier, the number of cracks is more, the crack opening is longer, and the width is wider than that of low discharge energy under the same discharge times. Under the same discharge energy, with the crease of discharge times, new cracks will continue to form around the micro-cracks after they open to the limit value, until the rock is completely destroyed.
- The proposed model plays a significant role in advancing the application of discharge impact technology in the field of oil and gas reservoir development. It enables the rapid selection of discharge impact energy and frequency for different sandstone reservoir characteristics, leading to the most cost-effective and optimized reformation outcomes.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| σ | the stress in tensor form (MPa) |
| ε | the volume strain (dimensionless) |
| E | elastic modulus (MPa) |
| D | the damage variable (dimensionless) |
| n | the number of discharges (time) |
| K, K | the bulk modulus (MPa) |
| effective Poisson’s ratio (dimensionless) | |
| Cd | micro-crack density (quantity/cm2) |
| β | coefficient (dimensionless) |
| N | the number of cracks activated by rock impact (quantity) |
| α | the average radius of micro-cracks (mm) |
| k, m | rock parameters in damage model (dimensionless) |
| Ω | the damage tensor of jointed rock mass (MPa) |
| l | the average crack spacing (mm) |
| V | the sample volume (mm3) |
| αk | the surface area of the k-th crack in the sample (mm2) |
| nk | the unit normal vector on the surface of the k-th crack in the sample (dimensionless) |
| KIC | fracture toughness (MPa) |
| ρ | density (kg/m3) |
| CP | longitudinal wave velocity (m/s) |
| εmax | the maximum volume tensile strain rate (dimensionless) |
| unn, Δun | the normal displacement (mm) |
| G″ff | the tensile fracture energy (MPa) |
| Fn, Ff | tensile strength (MPa) |
| Fn(t1) | the interfacial tensile strength after the next impact |
| F(0) | the tensile strength of rock samples under hydrostatic pressure (MPa) |
| F(N), F(n) | the fatigue tensile strength of the sample when the number of shocks under shock wave loading is N/n (MPa) |
| σh | minimum horizontal principal stress (MPa) |
| σH | maximum horizontal principal stress (MPa) |
| σci | the uniaxial compressive strength of intact rock (MPa) |
| M | compressive strength (MPa) |
| Mn | the compressive strength of rock after n times of discharge (MPa) |
| Eb | discharge energy (kJ) |
| C | the capacitor capacitance in the discharge device (μF) |
| U0 | the initial discharge voltage (kV) |
| η0 | the efficiency of converting energy into action energy (%) |
| mb | the Hoke-Brown constant of rock (dimensionless) |
| s | constant (dimensionless) |
| r | a constant (dimensionless) |
| t | the attenuation coefficient of shock wave energy (dimensionless) |
| Pm | the reduction value of rock compressive strength after n discharge (MPa) |
References
- Wu, Q.; Xu, Y.; Zhang, L.; Wang, T.; Guan, B.; Wu, G.; Wang, X. Core theory and key to optimal design of unconventional reservoir volume transformation technology. J. Pet. 2014, 35, 706–714. [Google Scholar]
- Wu, Q.; Xu, Y.; Wang, T.; Wang, X. A major change in the concept of increasing production and Transformation—Introduction to volume transformation technology. Nat. Gas Ind. 2011, 31, 7–12, 16, 121–122. [Google Scholar]
- Chen, Z.; Xue, C.; Jiang, Y.; Qin, Y. Application suggestions of shale gas well volume fracturing technology in China. Nat. Gas Ind. 2010, 30, 30–32, 116–117. [Google Scholar]
- Peng, K.; Zhou, J.Q.; Zou, Q.L.; Song, X. Effect of loading frequency on the deformation behaviours of sandstones subjected to cyclic loads and its underlying mechanism. Int. J. Fatigue 2020, 131, 105349.1–105349.12. [Google Scholar]
- Peng, K.; Zhou, J.; Zou, Q.; Zhang, J.; Wu, F. Effects of stress lower limit during cyclic loading and unloading on deformation characteristics of sandstones. Constr. Build. Mater. 2019, 217, 202–215. [Google Scholar]
- Shi, Z.; Li, J.; Wang, J. Research on the fracture mode and damage evolution model of sandstone containing pre-existing crack under different stress paths. Eng. Fract. Mech. 2022, 264, 108299. [Google Scholar]
- Wang, J.; Li, J.; Shi, Z. Deformation damage and acoustic emission characteristics of red sandstone under fatigue-creep interaction. Theor. Appl. Fract. Mech. 2021, 117, 103192. [Google Scholar] [CrossRef]
- Liu, Y.; Dai, F. A review of experimental and theoretical research on the deformation and failure behavior of rocks subjected to cyclic loading. J. Rock Mech. Geotech. Eng. 2021, 13, 1203–1230. [Google Scholar] [CrossRef]
- Ge, X.R.; Jiang, Y.; Lu, Y.D.; Ren, J.X. Testing study on fatigue deformation law of rock under cyclic loading. J. Rock Mech. Eng. 2003, 22, 1581–1585. [Google Scholar]
- Zhou, Z.-L.; Wu, Z.-b.; Li, X.-b.; Li, X.; Ma, C.-d. Mechanical behavior of red sandstone under cyclic point loading. Trans. Nonferrous Met. Soc. China 2015, 25, 2708–2717. [Google Scholar]
- Song, Z.; Konietzky, H.; Herbst, M. Three-dimensional particle model based numerical simulation on multi-level compressive cyclic loading of concrete. Constr. Build. Mater. 2019, 225, 661–677. [Google Scholar]
- Song, Z.; Fruehwirt, T.; Konietzky, H. Inhomogeneous mechanical behaviour of concrete subjected to monotonic and cyclic loading. Int. J. Fatigue 2020, 132, 105383.1–105383.14. [Google Scholar]
- Zhou, B.; Wang, J.H. Three-dimensional sphericity, roundness and fractal dimension of sand particles. Geotechnique 2018, 68, 18–30. [Google Scholar] [CrossRef]
- Zhou, B.; Wei, D.; Quan, K.; Wang, J.; Zhang, A. Study on the effect of particle morphology on single particle breakage using a combined finite-discrete element method. Comput. Geotech. 2020, 122, 103532. [Google Scholar]
- Li, D.; Zhu, Q.; Zhou, Z.; Li, X.; Ranjith, P.G. Fracture analysis of marble specimens with a hole under uniaxial compression by digital image correlation. Eng. Fract. Mech. 2017, 183, 109–124. [Google Scholar]
- Wang, Y.; Tang, J.; Dai, Z.; Yi, T. Experimental study on mechanical properties and failure modes of low-strength rock samples containing different fissures under uniaxial compression. Eng. Fract. Mech. 2018, 197, 1–20. [Google Scholar]
- Yang, S.Q.; Huang, Y.H.; Ranjith, P.G. Failure mechanical and acoustic behavior of brine saturated-sandstone containing two pre-existing flaws under different confining pressures. Eng. Fract. Mech. 2018, 193, 108–121. [Google Scholar]
- Budiansky, B.; Connell, R.J. Elastic moduli of a cracked solid. Int. J. Solids Struct. 1976, 12, 81–97. [Google Scholar] [CrossRef]
- Grady, D.E.; Kipp, M.E. Continuum modelling of explosive fracture in oil shale. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1980, 17, 147–157. [Google Scholar] [CrossRef]
- Taylor, L.M.; Chen, E.P.; Kuszmaul, J.S. Microcrack-induced damage accumulation in brittle rock under dynamic loading. Comput. Methods Appl. Mech. Eng. 1986, 55, 301–320. [Google Scholar] [CrossRef]
- Zuo, Q.H.; Disilvestro, D.; Richter, J.D. A crack-mechanics based model for damage and plasticity of brittle materials under dynamic loading. Int. J. Solids Struct. 2010, 47, 2790–2798. [Google Scholar]
- Zlw, A.; Ycl, A.; Jgw, B. A damage-softening statistical constitutive model considering rock residual strength-sciencedirect. Comput. Geosci. 2007, 33, 1–9. [Google Scholar]
- Zhu, C.Y.; Yu, S.C. Study on the discriminance of rock mass damage induced by blasting. Eng. Blasting 2001, 7, 12–16. [Google Scholar]
- Kyoya, T.; Ohashi, T.; Kawamoto, T. Damage mechanics analysis of underground opening in jointed rock mass. J. Soc. Mater. Sci. Jpn. 1986, 35, 472–477. [Google Scholar] [CrossRef][Green Version]
- Kawamoto, T.; Ichikawa, Y.; Kyoya, T. Deformation and fracturing behavior of discontinuous rock mass and damage mechanics theory. Int. J. Numer. Anal. Method Geomech. 1988, 12, 1–30. [Google Scholar]
- Haeri, H. Experimental crack analyses of concrete-like cscbdspecimens using a higher order ddm. Comput. Concr. 2015, 16, 881–896. [Google Scholar]
- Li, H. Rock Fracture Mechanics; Chongqing University Press: Chongqing, China, 1988. [Google Scholar]
- Liu, Y.; Li, Z.; Li, X.; Zhou, G.; Li, H.; Zhang, Q. Energy transfer efficiency improvement of liquid pulsed current discharge by plasma channel length regulation method. IEEE Trans. Plasma Sci. 2017, 45, 3231–3239. [Google Scholar] [CrossRef]
- Yan, C.B.; Xu, G.Y.; Yang, F. Measurement of Sound Waves to Study Cumulative Damage Effect on Surrounding Rock Under Blasting Load. Chin. J. Geotech. Eng. 2007, 29, 88–93. [Google Scholar]
- Yan, C.; Wang, G.; Shi, S.; Xu, G.Y. Analysis of acoustic wave frequency spectrum characters of rock mass under blasting damage based on wavelet (packet) transformation. Chin. J. Rock Mech. Eng. 2010, 29, 1496–1502. [Google Scholar]
- Yang, R.L.; Rocque, P.; Katsababis, P. Measurement and analysis of near-field blast vibration and damage. Geotech. Geol. Eng. 1994, 12, 169–182. [Google Scholar] [CrossRef]
- Zhang, L.; Yue, Z.; Yang, Z.; Qi, J.; Liu, F. A displacement-based back-analysis method for rock mass modulus and horizontal in situ stress in tunneling—Illustrated with a case study. Tunn. Undergr. Space Technol. Inc. Trenchless Technol. Res. 2006, 21, 636–649. [Google Scholar] [CrossRef]
- Tan, C.; Wang, R.; Ye, S.; Lei, W.; Sun, W. Numerical modelling estimation of the ‘tectonic stress plane’ (tsp) beneath topography with quasi-u-shaped valleys. Int. J. Rock Mech. Min. Sci. 2004, 41, 303–310. [Google Scholar]
- Ishihara, K.; Towhata, I. Sand response to cyclic rotation of principal stress directions as induced by wave loads. Soils Found. 1983, 23, 11–26. [Google Scholar] [CrossRef] [PubMed]
- Sun, C.; Wei, Y.; Zhou, Z. The Application of Detonation Physics; National Defence Industry Press: Beijing, China, 2001. [Google Scholar]
- Dobratz, B.M. Properties of chemical explosives and explosive simulants. In LLNL Explosive Handbook; Lawrence Livermore National Lab. (LLNL): Livermore, CA, USA, 2018; Volume 51, pp. 339–340. [Google Scholar]
- Ma, G.W.; An, X.M. Numerical simulation of blasting-induced rock fractures. Int. J. Rock Mech. Min. Sci. 2008, 45, 966–975. [Google Scholar] [CrossRef]




| No. | Porosity before Discharge/(%) | Porosity after Discharge/(%) | Permeability before Discharge/(mD) | Permeability after Discharge/(mD) | Longitudinal Wave Velocity before Discharge/(m/s) | Longitudinal Wave Velocity after Discharge/(m/s) | Compressive Strength/(MPa) | Young’s Modulus/(MPa) | Poisson Ratio |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 16.48 | 18.2 | 61.18 | 66.3 | 3266.80 | 2642.15 | 68.1 | 8619.1 | 0.22 |
| 2 | 16.47 | 18.1 | 67.58 | 79.3 | 3213.14 | 2538.62 | 61.8 | 9614.5 | 0.23 |
| 3 | 16.1 | 18.5 | 68.31 | 77.7 | 3213.37 | 2276.51 | 51.6 | 7683.32 | 0.23 |
| 4 | 16.21 | 18.9 | 65.55 | 143 | 3186.18 | 2196.84 | 40.4 | 9244.57 | 0.23 |
| Discharge Energy/(kJ) | Discharge Times | Rock Compressive Strength/(MPa) | Uniaxial Test Results/(MPa) | Tension Strength of Rock/(MPa) |
|---|---|---|---|---|
| 2.5 | 20 | 69.68 | 68.1 | 23.5 |
| 50 | 69.20 | 23.1 | ||
| 100 | 69.33 | 22.6 | ||
| 150 | 68.41 | 22.3 | ||
| 200 | 68.89 | 21.7 | ||
| 5.0 | 20 | 66.79 | 64.8 | 19.3 |
| 50 | 65.01 | 18.7 | ||
| 100 | 63.90 | 18.4 | ||
| 150 | 62.17 | 18.2 | ||
| 200 | 64.03 | 17.9 | ||
| 7.5 | 20 | 66.07 | 51.6 | 16.2 |
| 50 | 63.26 | 15.5 | ||
| 100 | 58.78 | 15.2 | ||
| 150 | 54.61 | 14.4 | ||
| 200 | 50.74 | 13.7 | ||
| 10 | 20 | 64.74 | 40.4 | 12.7 |
| 50 | 60.08 | 11.3 | ||
| 100 | 52.88 | 10.6 | ||
| 150 | 46.54 | 10.1 | ||
| 200 | 40.96 | 9.6 |
| Parameter | σh/(MPa) | σH/(MPa) | M/(MPa) | E/(MPa) | β | m | υ |
|---|---|---|---|---|---|---|---|
| numerical value | 21 | 24 | 22 | 18 | 3 | 6 | 0.24 |
| Discharge Energy/(kJ) | Discharge Times | Roundness/(Round) | Micro-Crack Width/(μm) |
|---|---|---|---|
| 2.5 | 200 | 0.761 | 207.57 |
| 5.0 | 200 | 0.463 | 403.38 |
| 7.5 | 200 | 0.549 | 750.62 |
| 10.0 | 200 | 0.742 | 1055.89 |
| Discharge Energy (kJ) | Discharge Times/(n) | Corrected Model Calculation Crack Number N/(Crack) | Number of Cracks in Simulation Result N/(Crack) | Error/(%) | Corrected Model Calculation Crack Radius A/(mm) | Simulation Results Crack Radius A/(mm) | Error/(%) |
|---|---|---|---|---|---|---|---|
| 2.5 | 20 | 1.26 | 0 | / | 0.021 | 0 | / |
| 50 | 1.28 | 0.32 | 12.5 | 0.049 | 0 | / | |
| 100 | 1.31 | 0.5 | 2 | 0.097 | 0.113 | 14.2 | |
| 150 | 1.33 | 1.5 | 11.3 | 0.142 | 0.165 | 13.9 | |
| 200 | 1.37 | 2 | 11.5 | 0.185 | 0.213 | 13.1 | |
| 5 | 20 | 2.03 | 1 | 3 | 0.055 | 0 | / |
| 50 | 2.11 | 2 | 5.5 | 0.131 | 0.1575 | 16.8 | |
| 100 | 2.23 | 2 | 11.5 | 0.247 | 0.25 | 1.2 | |
| 150 | 2.38 | 2.1 | 13.3 | 0.349 | 0.388 | 10.1 | |
| 200 | 2.54 | 2.6 | 2.3 | 0.439 | 0.45 | 2.4 | |
| 7.5 | 20 | 3.88 | 4 | 3 | 0.097 | 0.115 | 15.7 |
| 50 | 4.14 | 4 | 3.5 | 0.227 | 0.257 | 11.7 | |
| 100 | 4.65 | 4 | 16.3 | 0.407 | 0.387 | 5.1 | |
| 150 | 5.31 | 6 | 11.5 | 0.554 | 0.636 | 12.9 | |
| 200 | 6.17 | 6 | 2.8 | 0.677 | 0.726 | 6.7 | |
| 10 | 20 | 3.98 | 4 | 0.5 | 0.151 | 0.163 | 7.4 |
| 50 | 4.43 | 4 | 10.8 | 0.338 | 0.374 | 9.6 | |
| 100 | 5.45 | 6 | 9.2 | 0.579 | 0.577 | 0.3 | |
| 150 | 7.07 | 8 | 11.6 | 0.759 | 0.7 | 8.4 | |
| 200 | 10.08 | 10 | 0.8 | 0.898 | 1.03 | 12.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Li, X.; Lu, C.; Zhu, X.; Huang, K.; Xu, S. Modified Model and Simulation Verification of Rock-Fatigue Damage Considering Repeated Discharge Impact. Processes 2023, 11, 2366. https://doi.org/10.3390/pr11082366
Guo J, Li X, Lu C, Zhu X, Huang K, Xu S. Modified Model and Simulation Verification of Rock-Fatigue Damage Considering Repeated Discharge Impact. Processes. 2023; 11(8):2366. https://doi.org/10.3390/pr11082366
Chicago/Turabian StyleGuo, Jianchun, Xinyang Li, Cong Lu, Xinlei Zhu, Kun Huang, and Shiqian Xu. 2023. "Modified Model and Simulation Verification of Rock-Fatigue Damage Considering Repeated Discharge Impact" Processes 11, no. 8: 2366. https://doi.org/10.3390/pr11082366
APA StyleGuo, J., Li, X., Lu, C., Zhu, X., Huang, K., & Xu, S. (2023). Modified Model and Simulation Verification of Rock-Fatigue Damage Considering Repeated Discharge Impact. Processes, 11(8), 2366. https://doi.org/10.3390/pr11082366







