A Study on the Stability of Reinforced Tunnel Face Using Horizontal Pre-Grouting
Abstract
1. Introduction
2. Establishment of the Limit Equilibrium Model
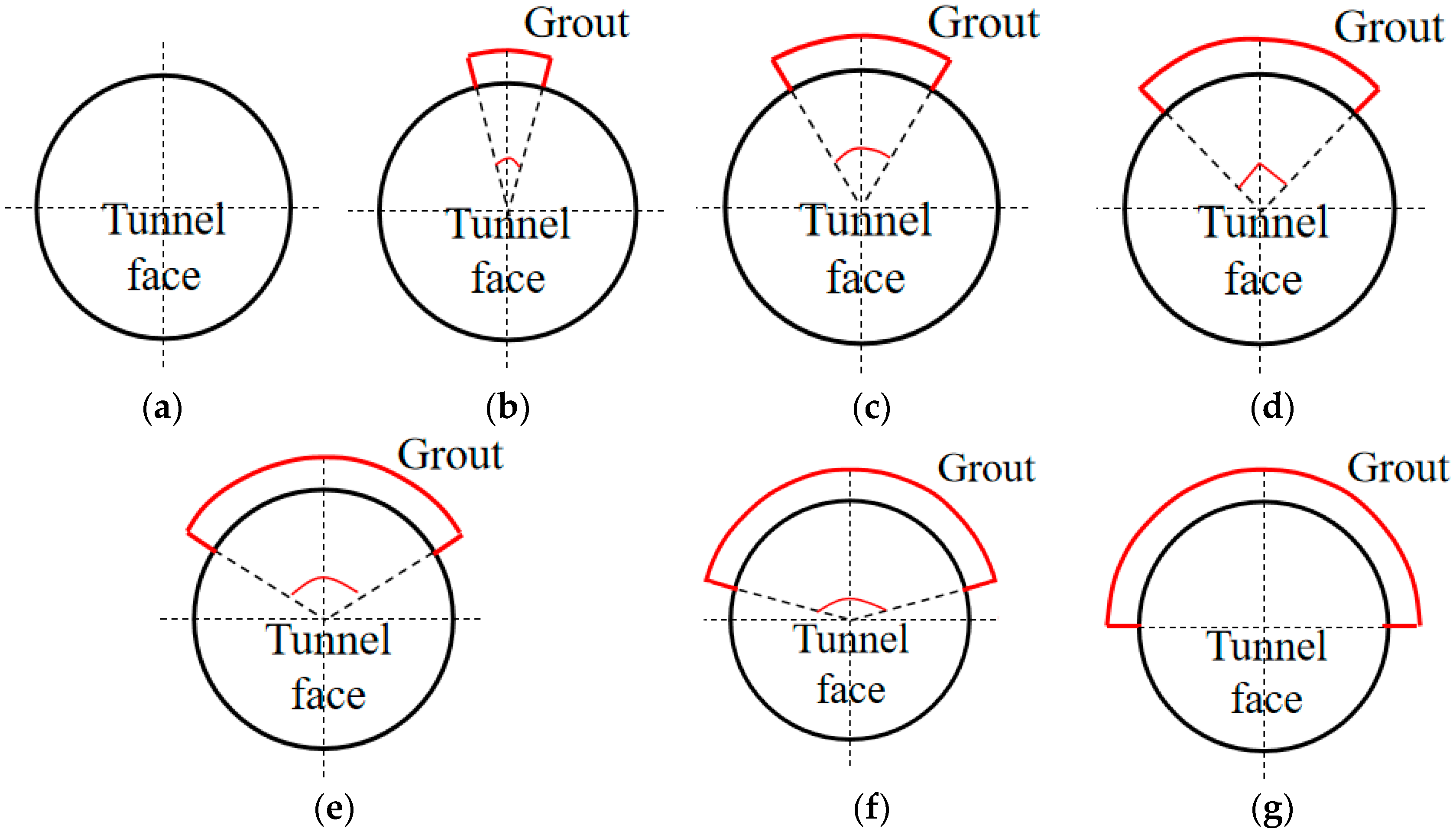
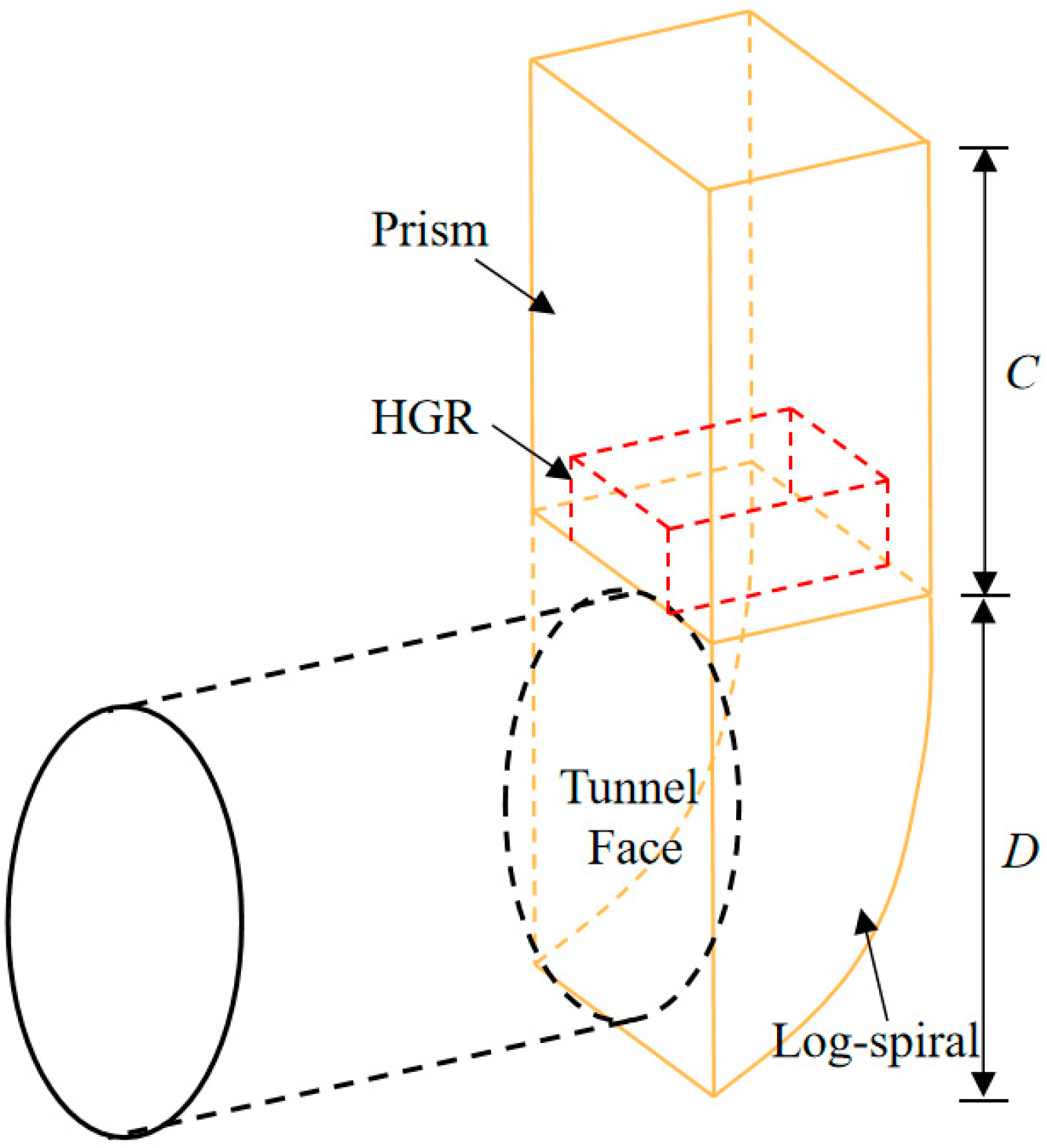
2.1. Overview
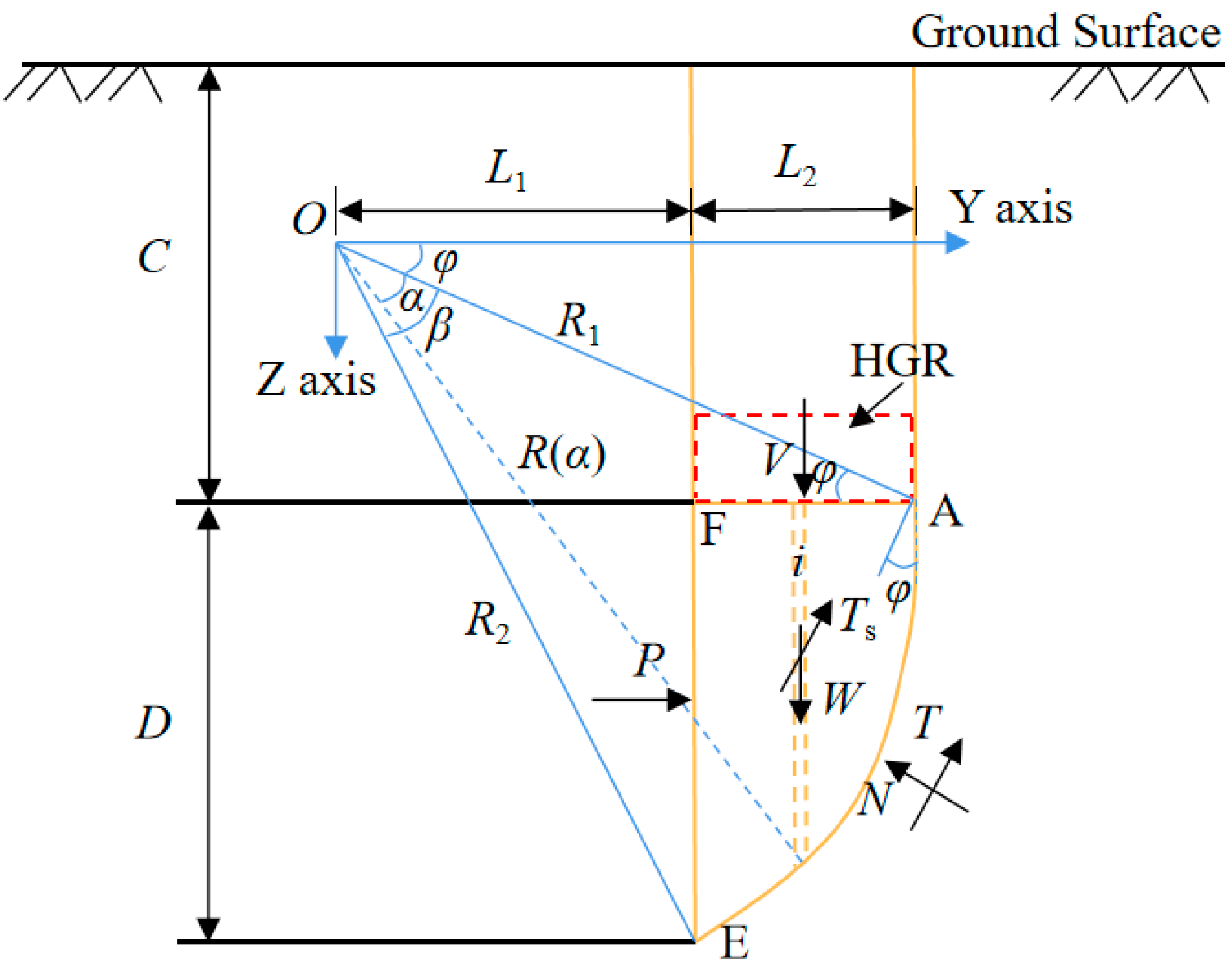
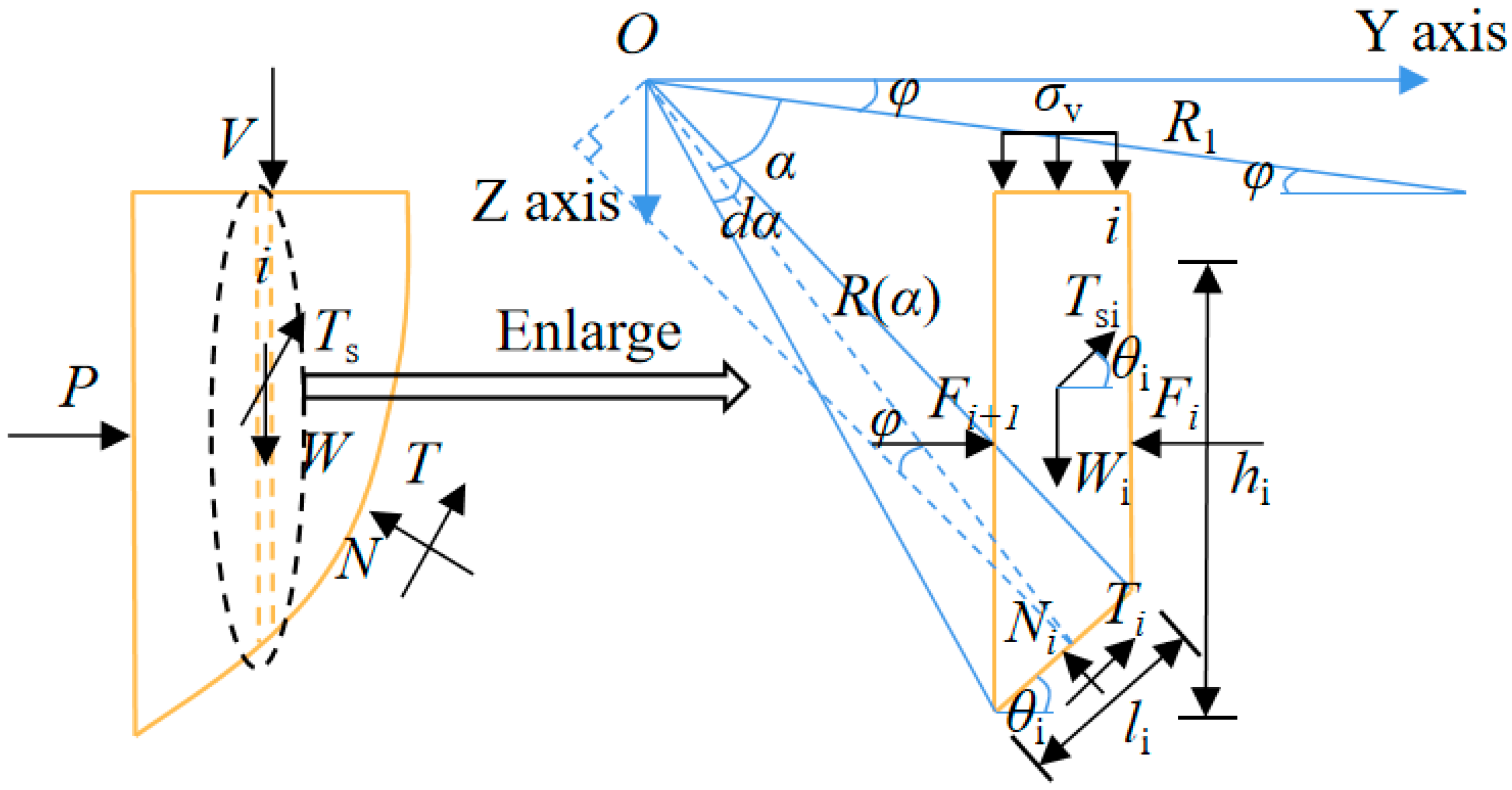
2.2. Solving for Limit Support Pressure
- (1)
- Calculation of MV
- (2)
- Calculation of MN,T, MTS, MW
- (3)
- Calculation of limit support pressure σp
3. Establishment of the Numerical Mode
3.1. Constitutive Model for Soil
3.2. Simulation Process
- (1)
- Establish corresponding numerical model, apply boundary conditions, and generate initial ground stress;
- (2)
- Replace the original soil with HGR, freeze the “soil unit” inside the tunnel, activate the “shell unit” of the lining and apply support pressure on the tunnel face;
- (3)
- Gradually decrease the support pressure until a small increment dramatically increases the horizontal displacement of the tunnel face, at which point the support pressure is defined as the limit support pressure.
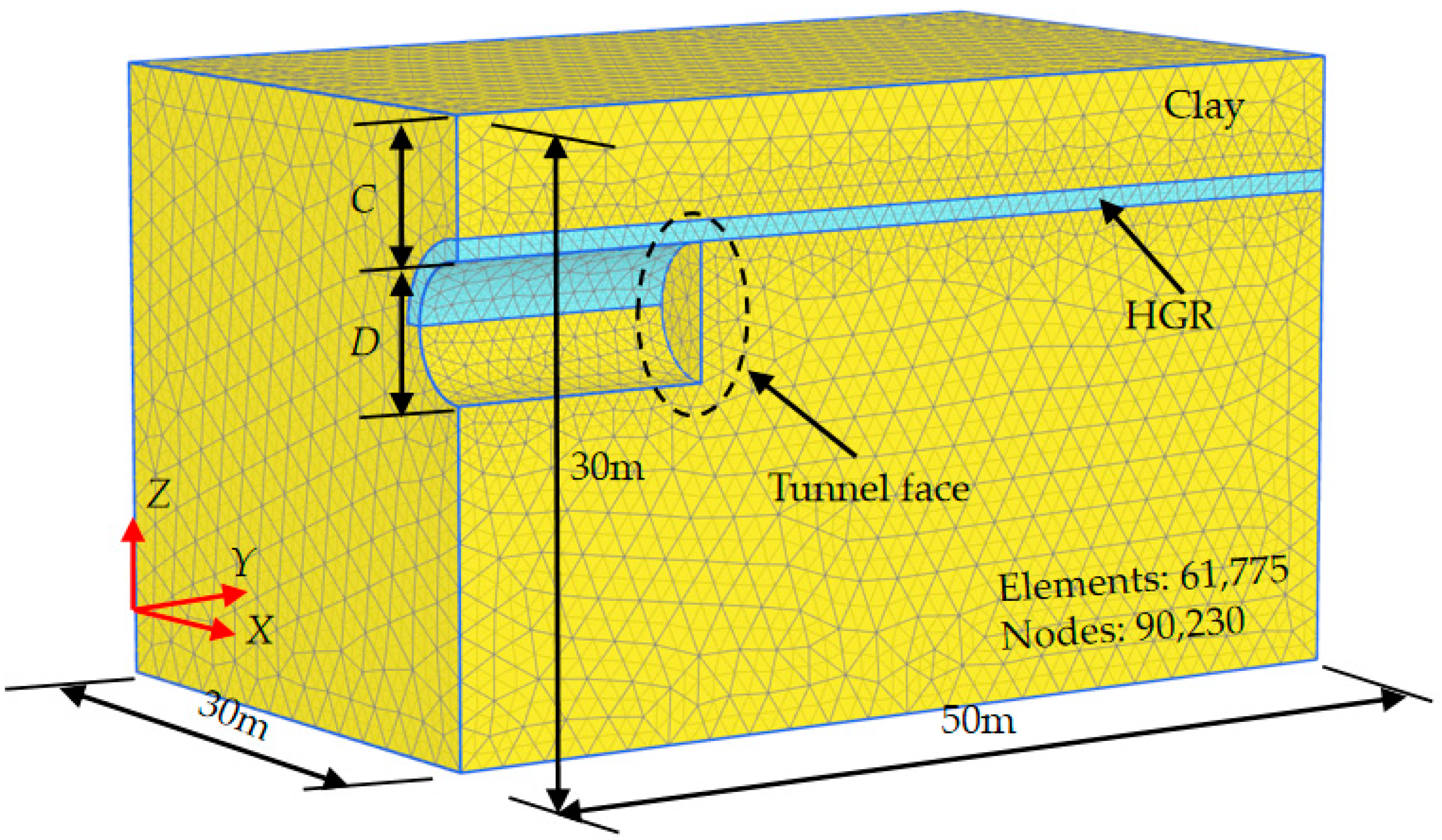
3.3. Numerical Model
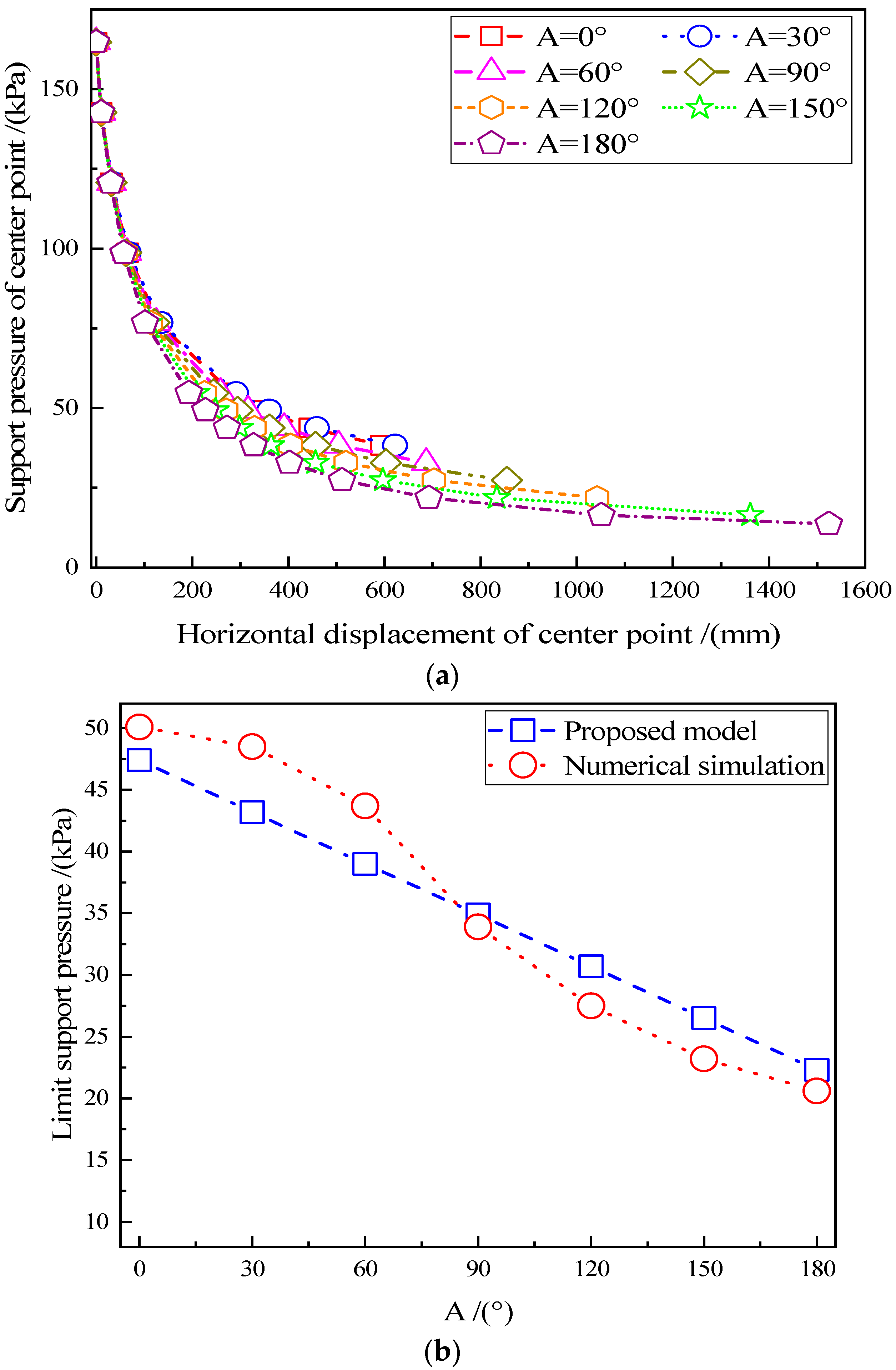
3.4. Limit Support Pressure
3.5. Parameter Analysis
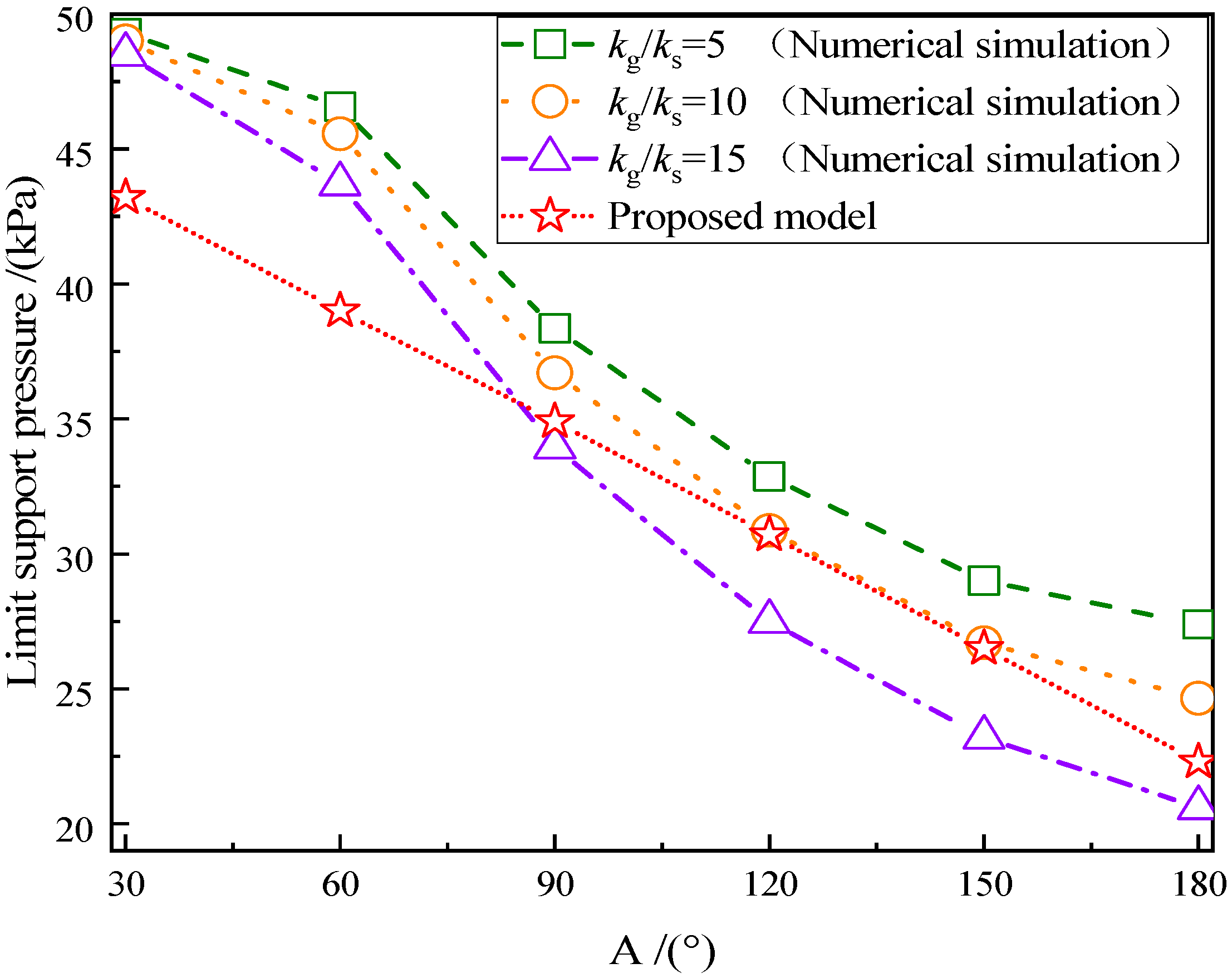
3.5.1. Effect of HGR Stiffness
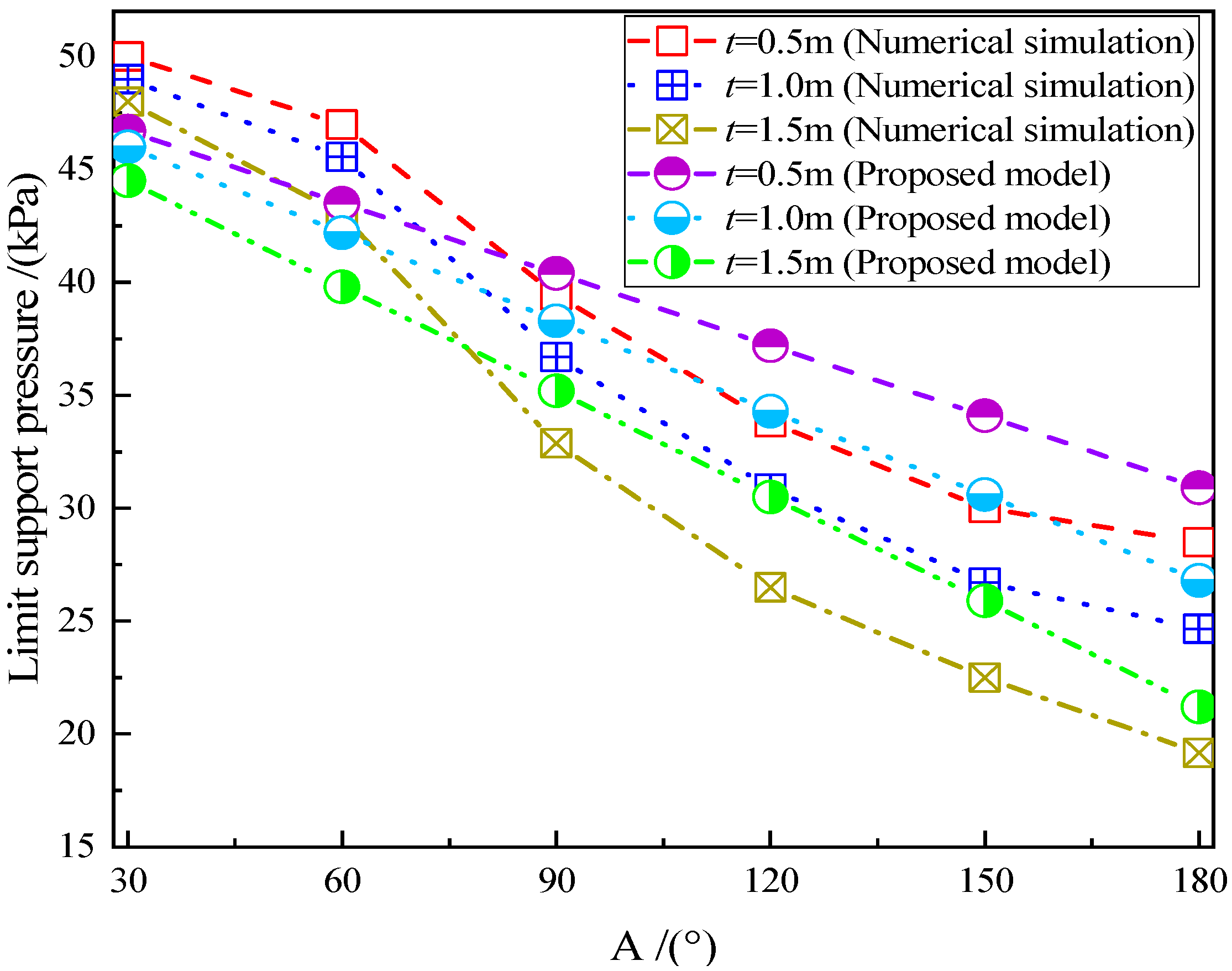
3.5.2. Effect of HGR Thickness
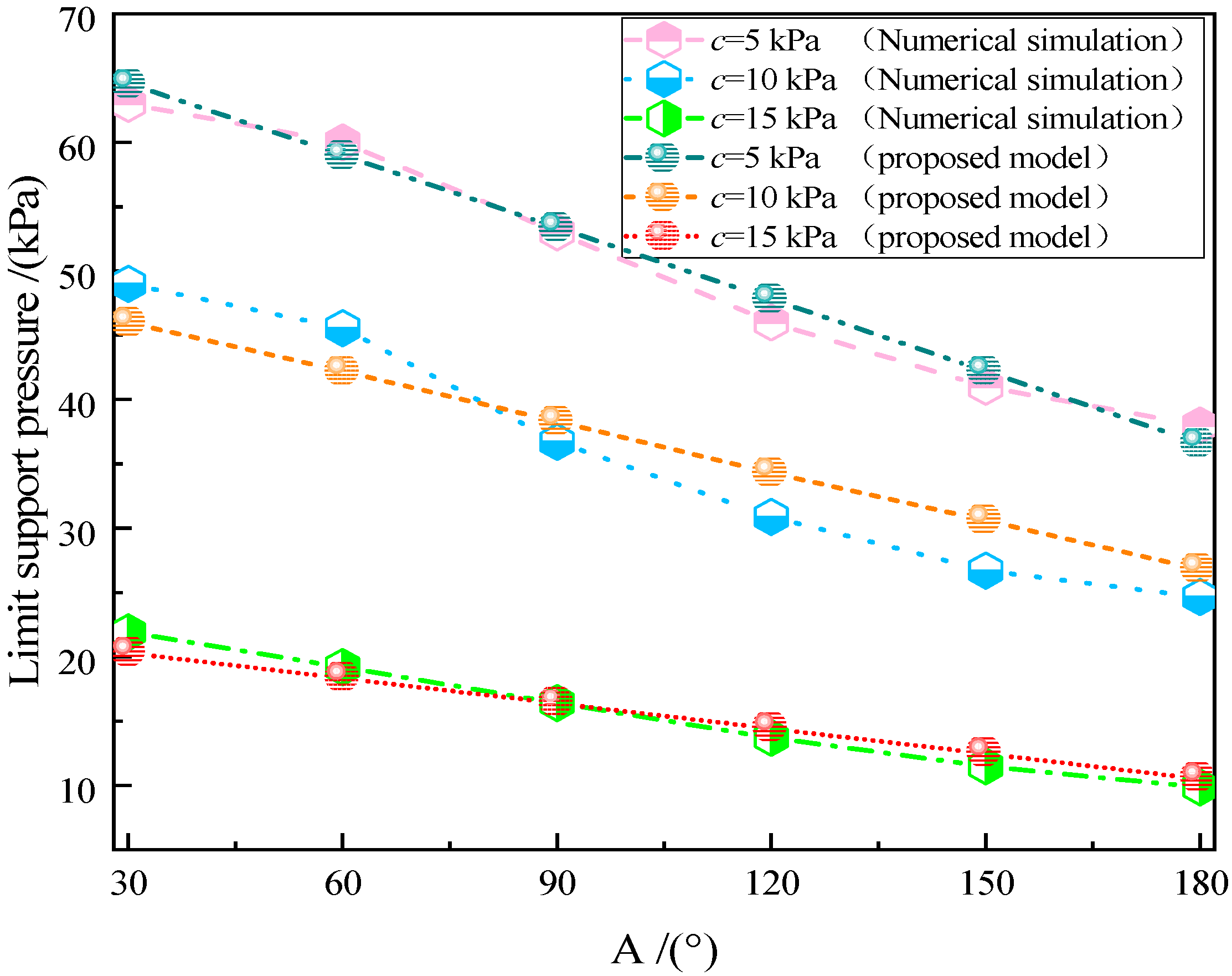
3.5.3. Effect of Effective Cohesion
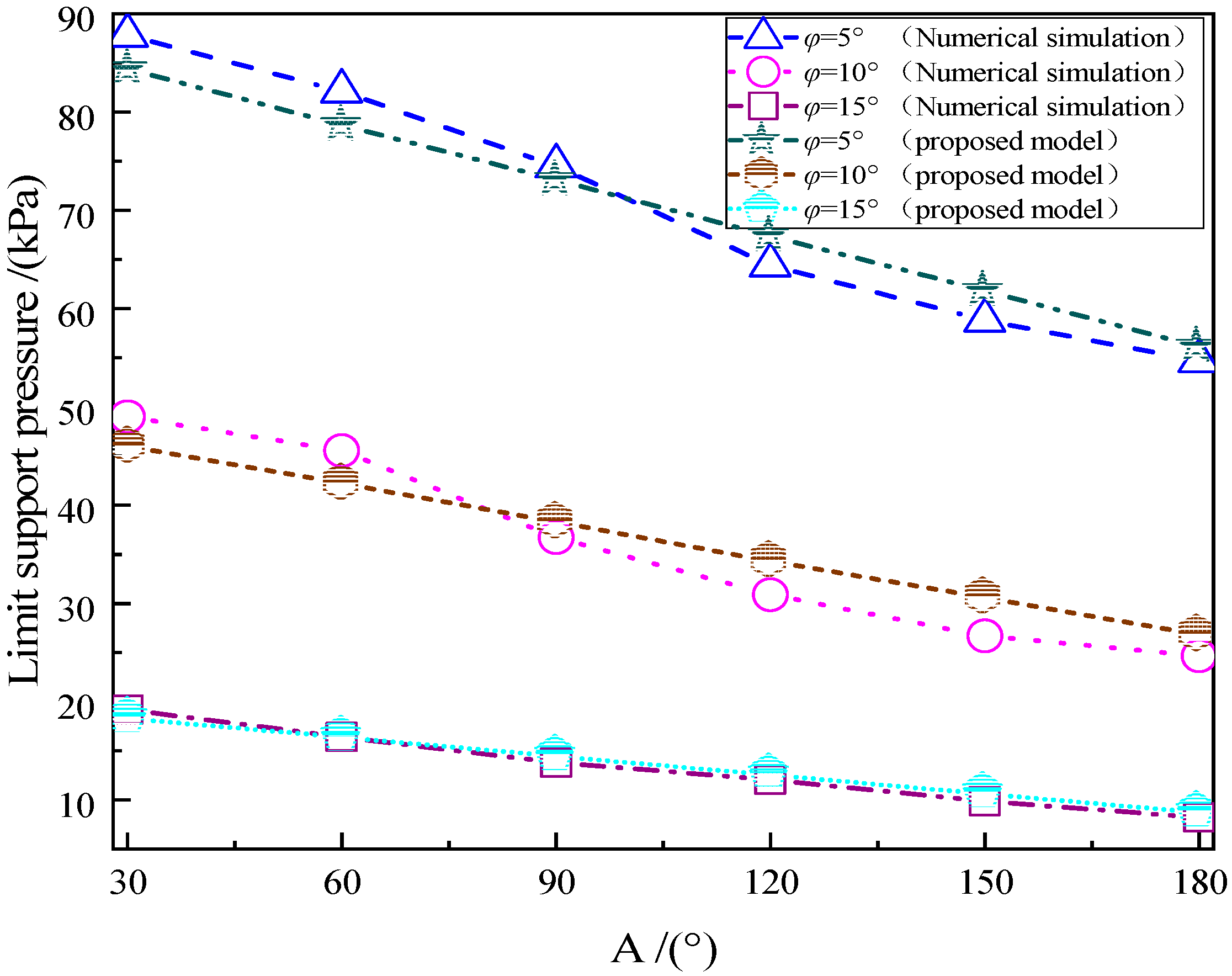
3.5.4. Effect of Effective Friction Angle
3.5.5. Parameter Comparison
4. Conclusions
- (1)
- The trend in the limit support pressure predicted by the numerical simulation and proposed model is similar. As A increases, the limit support pressure generally decreases. However, the differences are consistently approximately 10%.
- (2)
- The limit support pressure is linearly related to cohesion and friction angle. Moreover, changes in these two factors influence the limit support pressure more than changes in the stiffness ratio and thickness of HGR.
- (3)
- HGR is more effective in reducing the limit support pressure under low-cohesion (or friction angle) conditions than under high-cohesion (or friction angle) conditions. Increasing the friction angle and cohesion of soil layers significantly improves the ability of the tunnel face to maintain stability.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sterpi, D.; Cividini, A. A Physical and Numerical Investigation on the Stability of Shallow Tunnels in Strain Softening Media. Rock Mech. Rock Eng. 2004, 37, 277–298. [Google Scholar] [CrossRef]
- Kirsch, A. Experimental Investigation of the Face Stability of Shallow Tunnels in Sand. Acta Geotech. 2010, 5, 43–62. [Google Scholar] [CrossRef]
- Chen, R.; Li, J.; Kong, L.; Tang, L. Experimental Study on Face Instability of Shield Tunnel in Sand. Tunn. Undergr. Space Technol. 2013, 33, 12–21. [Google Scholar] [CrossRef]
- Lu, X.; Zhou, Y.; Huang, M.; Zeng, S. Experimental Study of the Face Stability of Shield Tunnel in Sands under Seepage Condition. Tunn. Undergr. Space Technol. 2018, 74, 195–205. [Google Scholar] [CrossRef]
- Chambon, P.; Corté, J. Shallow Tunnels in Cohesionless Soil: Stability of Tunnel Face. J. Geotech. Eng. 1994, 120, 1148–1165. [Google Scholar] [CrossRef]
- Idinger, G.; Aklik, P.; Wu, W.; Borja, R.I. Centrifuge Model Test on the Face Stability of Shallow Tunnel. Acta Geotech. 2011, 6, 105–117. [Google Scholar] [CrossRef]
- Chen, R.; Yin, X.; Tang, L.; Chen, Y. Centrifugal Model Tests on Face Failure of Earth Pressure Balance Shield Induced by Steady State Seepage in Saturated Sandy Silt Ground. Tunn. Undergr. Space Technol. 2018, 81, 315–325. [Google Scholar] [CrossRef]
- Wong, K.S.; Ng, C.W.W.; Chen, Y.M.; Bian, X.C. Centrifuge and Numerical Investigation of Passive Failure of Tunnel Face in Sand. Tunn. Undergr. Space Technol. 2012, 28, 297–303. [Google Scholar] [CrossRef]
- Paternesi, A.; Schweiger, H.F.; Scarpelli, G. Numerical Analyses of Stability and Deformation Behavior of Reinforced and Unreinforced Tunnel Faces. Comput. Geotech. 2017, 88, 256–266. [Google Scholar] [CrossRef]
- Han, K.; Wang, L.; Su, D.; Hong, C.; Chen, X.; Lin, X.-T. An Analytical Model for Face Stability of Tunnels Traversing the Fault Fracture Zone with High Hydraulic Pressure. Comput. Geotech. 2021, 140, 104467. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, M.; Lyu, C. Experimental and numerical study on tunnel faces reinforced by horizontal bolts in sandy ground. Tunn. Undergr. Space Technol. 2022, 123, 104412. [Google Scholar] [CrossRef]
- Man, J.; Huang, H.; Ai, Z.; Chen, J.; Wang, F. Stability of complex rock tunnel face under seepage flow conditions using a novel equivalent analytical model. Int. J. Rock Mech. Min. Sci. 2023, 170, 105427. [Google Scholar] [CrossRef]
- Wang, J.; Lin, G.; Xu, G.; Wei, Y.; Li, S.; Tang, X.; He, C. Face stability of EPB shield tunnels in multilayered ground with soft sand lying on hard rock considering dynamic excavation process: A DEM study. Tunn. Undergr. Space Technol. 2022, 120, 104268. [Google Scholar] [CrossRef]
- Fu, Y.; Zeng, D.; Xiong, H.; Li, X.; Chen, Y. Seepage effect on failure mechanisms of the underwater tunnel face via CFD-DEM coupling. Comput. Geotech. 2022, 146, 104591. [Google Scholar] [CrossRef]
- Yin, Z.-Y.; Wang, P.; Zhang, F. Effect of Particle Shape on the Progressive Failure of Shield Tunnel Face in Granular Soils by Coupled FDM-DEM Method. Tunn. Undergr. Space Technol. 2020, 100, 103394. [Google Scholar] [CrossRef]
- Horn, N. Horizontal earth pressure on the vertical surfaces of the tunnel tubes. In Proceedings of the National Conference of the Hungarian Civil Engineering Industry, Budapest, Hungary, 28 July 1961; pp. 7–16. [Google Scholar]
- Anagnostou, S. The Contribution of Horizontal Arching to Tunnel Face Stability. Geotechnik 2012, 35, 34–44. [Google Scholar] [CrossRef]
- Liu, X.; Wang, F.; Fang, H.; Yuan, D. Dual-Failure-Mechanism Model for Face Stability Analysis of Shield Tunneling in Sands. Tunn. Undergr. Space Technol. 2019, 85, 196–208. [Google Scholar] [CrossRef]
- Dormieux, L. Upper and Lower Bound Solutions for the Face Stability of Shallow Circular Tunnels in Frictional m. Géotechnique 1990, 40, 581–606. [Google Scholar]
- Mollon, G.; Dias, D.; Soubra, A.H. Probabilistic Analysis and Design of Circular Tunnels against Face Stability. Int. J. Geomech. 2009, 9, 237–249. [Google Scholar] [CrossRef]
- Mollon, G.; Dias, D.; Soubra, A. Face Stability Analysis of Circular Tunnels Driven by a Pressurized Shield. J. Geotech. Geoenviron. Eng. 2010, 136, 215–229. [Google Scholar] [CrossRef]
- Mollon, G.; Dias, D.; Soubra, A.H. Rotational Failure Mechanisms for the Face Stability Analysis of Tunnels Driven by a Pressurized Shield. Int. J. Numer. Anal. Methods Geomech. 2011, 35, 1363–1388. [Google Scholar] [CrossRef]
- Jancsecz, S.; Steiner, W. Face Support for a Large Mix-Shield in Heterogeneous Ground Conditions. In Proceedings of the Tunnelling’94, Chapman and Hall, London, UK, 5–7 July 1994; pp. 531–550. [Google Scholar]
- Krause, T. Schildvortrieb Mit Fluessigkeits-Und Erdgestuetzter Ortsbrust. Ph.D. Thesis, Technische Universitaet Braunschweig, Braunschweig, Germany, 1987. [Google Scholar]
- Chen, R.P.; Tang, L.J.; Yin, X.S.; Chen, Y.M.; Bian, X.C. An Improved 3D Wedge-Prism Model for the Face Stability Analysis of the Shield Tunnel in Cohesionless Soils. Acta Geotech. 2015, 10, 683–692. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, M.; Wang, Z.; Li, J.; Liu, D. A Limit Equilibrium Model for the Reinforced Face Stability Analysis of a Shallow Tunnel in Cohesive-Frictional Soils. Tunn. Undergr. Space Technol. 2020, 105, 103562. [Google Scholar] [CrossRef]
- Huang, M.; Li, Y.; Shi, Z.; Lu, X. Face Stability Analysis of Shallow Shield Tunneling in Layered Ground under Seepage Flow. Tunn. Undergr. Space Technol. 2022, 119, 104201. [Google Scholar] [CrossRef]
- Coulter, S.; Martin, C.D. Effect of Jet-Grouting on Surface Settlements above the Aeschertunnel, Switzerland. Tunn. Undergr. Space Technol. 2006, 21, 542–553. [Google Scholar] [CrossRef]
- Su, D.; Song, Q.; Lin, X.; Li, Q.; Chen, X. Limit Equilibrium Models for Active Failures of Large-Diameter Shield Tunnel Faces in Soft Clay Reinforced with Soil-Cement Walls. Comput. Geotech. 2023, 153, 105104. [Google Scholar] [CrossRef]
- Takano, D.; Otani, J.; Nagatani, H.; Mukunoki, T. Application of X-Ray CT on Boundary Value Problems in Geotechnical Engineering: Research on Tunnel Face Failure. In Proceedings of the ASCE, Clearwater Beach, FL, USA, 17–20 June 2012; pp. 1–6. [Google Scholar]
- Alagha, A.S.N.; Chapman, D.N. Numerical Modelling of Tunnel Face Stability in Homogeneous and Layered Soft Ground. Tunn. Undergr. Space Technol. 2019, 94, 103096. [Google Scholar] [CrossRef]
- Yu, L.; Zhang, D.; Fang, Q.; Cao, L.; Zhang, Y.; Xu, T. Face Stability of Shallow Tunnelling in Sandy Soil Considering Unsupported Length. Tunn. Undergr. Space Technol. 2020, 102, 103445. [Google Scholar] [CrossRef]
- Perazzelli, P.; Leone, T.; Anagnostou, G. Tunnel Face Stability under Seepage Flow Conditions. Tunn. Undergr. Space Technol. 2014, 43, 459–469. [Google Scholar] [CrossRef]
- Jallow, A.; Ou, C.-Y.; Lim, A. Three-Dimensional Numerical Study of Long-Term Settlement Induced in Shield Tunneling. Tunn. Undergr. Space Technol. 2019, 88, 221–236. [Google Scholar] [CrossRef]
- Zheng, G.; Yang, X.; Zhou, H.; Du, Y.; Sun, J.; Yu, X. A Simplified Prediction Method for Evaluating Tunnel Displacement Induced by Laterally Adjacent Excavations. Comput. Geotech. 2018, 95, 119–128. [Google Scholar] [CrossRef]
- Soubra, A.-H.; Dias, D.; Emeriault, F.; Kastner, R. Three-Dimensional Face Stability Analysis of Circular Tunnels by a Kinematical Approach. In Proceedings of the ASCE Geocongress, New Orleans, LA, USA, 9–12 March 2008; pp. 894–901. [Google Scholar]
- Mu, L.; Huang, M. Small Strain Based Method for Predicting Three-Dimensional Soil Displacements Induced by Braced Excavation. Tunn. Undergr. Space Technol. 2016, 52, 12–22. [Google Scholar] [CrossRef]
- Bolton, M.D. The Strength and Dilatancy of Sands. Geotechnique 1987, 36, 219–226. [Google Scholar] [CrossRef]
- Gu, X.; Hu, J.; Huang, M. K-0 of Granular Soils: A Particulate Approach. Granul. Matter 2015, 17, 703–715. [Google Scholar] [CrossRef]
- Yang, J.; Gu, X.Q. Shear Stiffness of Granular Material at Small Strains: Does It Depend on Grain Size? Geotechnique 2013, 63, 165–179. [Google Scholar] [CrossRef]
- Fu, Y.; He, S.; Zhang, S.; Yang, Y. Parameter Analysis on Hardening Soil Model of Soft Soil for Foundation Pits Based on Shear Rates in Shenzhen Bay, China. Adv. Mater. Sci. Eng. 2020, 2020, 7810918. [Google Scholar] [CrossRef]
- Zhang, C.; Han, K.; Zhang, D. Face Stability Analysis of Shallow Circular Tunnels in Cohesive-Frictional Soils. Tunn. Undergr. Space Technol. 2015, 50, 345–357. [Google Scholar] [CrossRef]

| Parameter | Method | Value | |
|---|---|---|---|
| Clay | HGR | ||
| Effective cohesion, c′ (kPa) | Triaxial consolidated and drained test | 10.1 | 250 |
| Effective friction angle, φ′ (°) | Triaxial consolidated and drained test | 10.3 | 35 |
| Dilation angle, Ψ (°) | Ψ = 0 when φ′ < 30° [38] | 0 | 0 |
| Lateral pressure coefficient, K0 | K0 = 1 − sin φ′ [39] | 0.83 | 0.43 |
| Failure ratio, Rf | Rf = 0.50 when e > 1.5, Rf =−0.9(e − 1.5) + 0.5 when 1.0 < e ≤ 1.5, [40] | 0.9 | 0.9 |
| Secant stiffness in triaxial test, E50ref (MPa) | E50ref = (0.7~1.0)Es1−2 [41] | 1.50 | 22.5 |
| Tangent stiffness, Eoedref (MPa) | Eoedref = 0.9Es1−2 [41] | 1.50 | 22.5 |
| Reference shear modulus, G0ref (MPa) | G0ref = (1.5~2.5)Eurref [40] | 13.0 | 195 |
| Reference stiffness stress, Pref (kPa) | Plaxis manual | 100 | 100 |
| Poisson’s ratio for unloading–reloading, νur | Plaxis manual | 0.2 | 0.2 |
| Unloading/reloading stiffness, Eurref (MPa) | Eurref = (3~8)Eoedref [41] | 6.0 | 90 |
| Power for dependency of stiffness, m | Plaxis manual | 0.5 | 0.1 |
| Shear strain corresponding to an initial shear modulus of 70%, γ0.7 | γ0.7 = (1~4) × 10−4 [40] | 1 × 10−4 | 4 × 10−4 |
| A (°) | 0 | 30 | 60 | 90 | 120 | 150 | 180 |
|---|---|---|---|---|---|---|---|
| Pa (kPa) | 47.4 | 43.2 | 39.0 | 34.9 | 30.7 | 26.5 | 22.3 |
| Pn (kPa) | 50.1 | 48.5 | 43.7 | 33.9 | 27.5 | 23.2 | 20.6 |
| Difference (%) | 5.5 | 11.4 | 11.4 | 2.9 | 11.0 | 13.3 | 7.9 |
| Parameters | Stiffness Ration (kg/ks) | Thickness (t) | Cohesion (c) | Friction Angle (φ) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 5 | 10 | 15 | 0.5 m | 1.0 m | 1.5 m | 5 kPa | 10 kPa | 15 kPa | 5° | 10° | 15° | |
| A = 30° (kPa) | 49.3 | 49.0 | 48.5 | 50.0 | 49.0 | 48.0 | 63.0 | 49.0 | 21.9 | 87.8 | 49.0 | 19.2 |
| A = 180° (kPa) | 27.4 | 24.7 | 20.6 | 28.48 | 24.65 | 19.17 | 38.0 | 24.7 | 9.9 | 54.7 | 24.7 | 8.2 |
| IR (%) | 14.6 | 16.2 | 18.6 | 14.3 | 16.2 | 19.2 | 16.7 | 16.2 | 8.0 | 22.1 | 16.2 | 7.3 |
| CR (%) | |18.6% − 14.6%| = 4 | |19.2% − 14.3%| = 4.9 | |8.0% − 16.7%| = 8.7 | |7.3% − 22.1%| = 14.8 | ||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Y.; Zhang, J.; Chen, D.; Sun, Y.; Kong, L. A Study on the Stability of Reinforced Tunnel Face Using Horizontal Pre-Grouting. Processes 2023, 11, 2044. https://doi.org/10.3390/pr11072044
Ren Y, Zhang J, Chen D, Sun Y, Kong L. A Study on the Stability of Reinforced Tunnel Face Using Horizontal Pre-Grouting. Processes. 2023; 11(7):2044. https://doi.org/10.3390/pr11072044
Chicago/Turabian StyleRen, Yuxin, Jingtao Zhang, Dongdong Chen, Yanding Sun, and Lingzhao Kong. 2023. "A Study on the Stability of Reinforced Tunnel Face Using Horizontal Pre-Grouting" Processes 11, no. 7: 2044. https://doi.org/10.3390/pr11072044
APA StyleRen, Y., Zhang, J., Chen, D., Sun, Y., & Kong, L. (2023). A Study on the Stability of Reinforced Tunnel Face Using Horizontal Pre-Grouting. Processes, 11(7), 2044. https://doi.org/10.3390/pr11072044








