1. Introduction
With consumers’ continuous demand for mobility, advanced features, and connectivity, there is a dynamic rise in the use of electronic components used in different applications, in order to improve or enhance the performance of existing products and solutions [
1,
2].
The increased performance of the electronic components is useful, but it also correlates with a higher dissipated power inside during functioning. Multiple electronic components or applications generate heat during functioning [
3,
4]. This results in a higher power density for the components. A higher power density on a component or a reduced surface also correlates to a higher component temperature for the same boundary conditions.
Temperature affects the efficiency of electronic components. For example, both electrical resistance and thermal conductivity are temperature dependent. A higher working temperature affects the electronic components long term and increases the risk of failure and accelerated ageing [
5,
6,
7,
8]. For more reliable electronic components, it is recommended to keep their temperature closer to the operating temperature with the best efficiency and remove the additional heat [
9,
10,
11,
12]. For a certain number of components, based on the size or amount of dissipated power, additional cooling is needed [
13].
The cooling methods can be divided into two main categories, passive and active. The active cooling methods can usually dissipate a higher power; therefore, they are more efficient. Sometimes this type of cooling method is mandatory. The disadvantages of these methods are the need for additional external energy for functioning, additional pumps for cooling fluid, fans, moving elements, or complicated infrastructure. In addition, maintenance is also required, and the risk of failure is higher than in the passive cooling solutions [
14,
15,
16].
If a component has a dissipated power small enough to be cooled by a passive cooling method, the use of active cooling is no longer necessary [
17]. The advantages of passive cooling methods are the smaller size, low risk of damage, and continuous heat transfer due to its passive nature [
18,
19,
20].
One of the most used passive cooling methods is the heat sink. The constructive and use advantages have generated the expansion of the use of this type of equipment in a multitude of domains, ranging from electronic equipment and photovoltaic systems to the aerospace industry. At the same time, off the shelf heat sinks can be found on the market, reducing the price of this cooling method even more thanks to the mass production [
8].
Heat sinks provide a passive cooling method used for electronic components [
21], where the cooling is made through convection and radiation into the surrounding fluid [
8,
20,
22,
23]. Systems where the cooling is achieved using passive cooling with heat sinks rely on the three basic heat transfer methods, the previously mentioned convection and radiation, and conduction [
24].
The fluid temperature next to the heat sink influences convection. As with other cooling fluid solid interfaces, a boundary layer forms next to the heat sink walls. A higher fluid speed will reduce the boundary layer, thereby improving the natural convection cooling [
24].
The thermal radiation coefficient dictates how much heat is dissipated by the heat sink through radiation. The aim is to have a radiation coefficient as close as possible to 1, the value of an ideal black body [
25,
26].
Conduction affects passively cooled systems mainly in two cases: One case is inside each component; therefore, the thermal conductivity of the material is important. A higher thermal conductivity of the heat sink can spread the heat more efficiently to the fins and release it into the ambient air using a higher surface.
The second case, where heat conduction is important, is at the boundary of two components in contact with each other. The best example found in every system is the contact between the heat source and the heat sink. An improper contact surface may lead to increased thermal resistance, thereby reducing the quantity of heat transferred between heat source and heat sink.
During the design phase, it is important to take into consideration the boundary conditions when designing or placing a passive cooling method. Hot air rises due to a lower density compared to the colder air. Therefore, the air next to the heat sink rises after the heat is transferred from heat sink to the surrounding air. The movement direction of the air is decided by gravity; therefore, gravity can have a role in improving or not improving the passive cooling process. This study investigates the effect gravity orientation relative to heat sink position and air moment has on cooling.
The finite element method is a method used to simulate the behavior of such a system with the same boundary conditions used in many applications and investigations [
1,
11,
14,
21]. The research conducted by other scholars shows that the finite element simulation software can provide similar results to the ones measured during experiments in the same conditions [
9,
27].
The second approach in this paper is to determine if the Siemens Simcenter Flotherm XT 2021.2 (Siemens, Munich, Germany) finite element analysis software is a reliable and fast method to investigate multiple designs of heatsinks. Due to a dynamic and fast product development, design engineers are searching for faster ways to choose the best design before reaching the testing stage. Finite element analysis is one of the best ways to reach the goal and choose the optimal design, but only if the results are similar to reality, especially when the changes to the designed part are incremental. In the second part of this paper, our own experimental results will be compared to the simulated results in order to evaluate the accuracy of the simulations [
28,
29].
Ataei et al. [
30] concluded that it is important to enhance the heat transfer inside the systems in order to improve the cooling. Liu X. et al. [
31] concluded that their simulation of thermal management systems using fins made with Flotherm simulation software provided similar results as the measurements. Liu X. et al. [
32] used Flotherm to optimize the thermal management system used for hybrid battery cooling. Chen D. et al. [
33] used Flotherm as a computation fluid dynamics software in their investigations and concluded that the design proposed after the numerical analysis shows similar results as the measurements, and that this method is a reliable one.
The results measured and simulated by Liu H et al. [
34] helped them to state that the numerical method using Flotherm is an effective method. Yang H. et al. [
35] found a maximum error between the measured and simulated values of 2.54%, concluding that Flotherm is considered to have a certain accuracy.
Krane P et al. [
36] used Flotherm to predict hot spots within electronic packages. This approach is used as a mechanism during design and testing, and also as a tool for active thermal management. Jiang R. et al. [
37] compares Flotherm with Abaqus in order to see the accuracy of both software since numerical simulation methods are frequently used in the design of the electronic products. Flotherm was also used for investigations by Chen P. et al. [
38], Seetharaman R. et al. [
39], Guggari S. [
40], Kim J. K. [
41], and Goswami A. et al. [
42] for its thermal and computational fluid dynamics capabilities.
2. Materials and Methods
In order to evaluate the cooling capability of a heat sink, a heat source is needed. For this experiment, we used a thick film heating element, Telpod HTS-16-230-300-3/6.3 [
43]. This thick film heating element is designed to be placed on flat surfaces and the heating elements are placed on a stainless-steel substrate. The shape of the heating element allows us to fix it on the heat sink using screws, creating a regular and known heat transfer area. Compared to the tubular heating elements, the heat transfer area in contact with the heat sink is easier to create in the virtual CAD model.
The supply voltage for this element is up to 230 V, and the generated power is up to 300 W. The maximum temperature of the element can reach 400 °C on the surface and 170 °C on the element temperature with soldered and wired connectors, which makes this type of heating element suitable for a wide range of applications. The current supply is made using two connectors of 6.3 mm width placed at a 90° angle on the heating element surface [
43].
The dimensions of the heating element are 40 mm width and 75.6 mm length, as shown in
Figure 1. With a thickness of only 1 mm, it is a very thin heating element, thereby reducing thermal resistance from the heating element to the heat sink. On the top, it has a reflective and transparent layer of lacquer or varnish, generating a heat transfer coefficient through radiation closer to 1.
The heating element was fixed using two M4 screws placed at 28 mm distance from each other. Using screws for the clamping of parts reduces the curvature or bending of the heating element, creating an improved contact area between the heating element and heat sink.
The heat sink used for cooling the heating element is the Relpol RH17A [
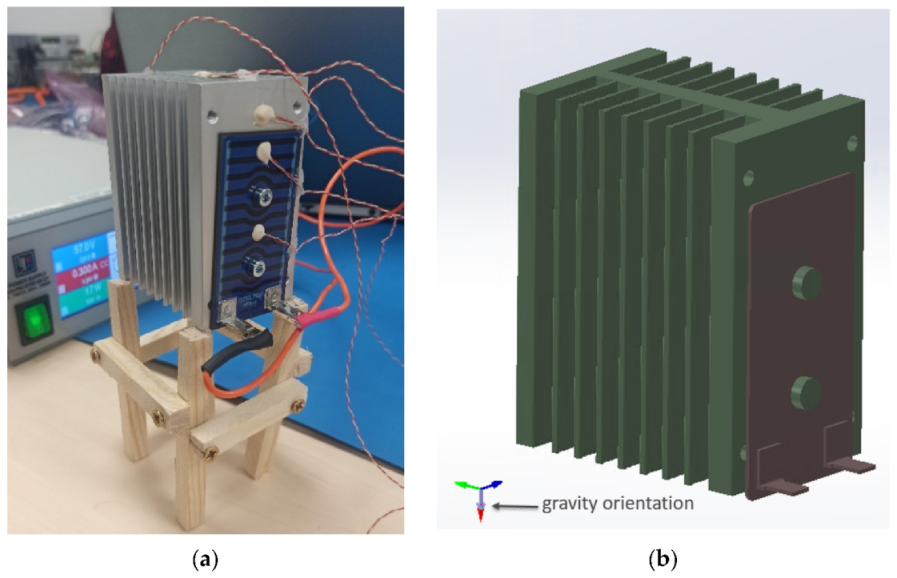
44]. It is an aluminum heat sink with a 350 g weight. It has an anodized grey color, which means it also has a higher radiation coefficient compared to shiny aluminum. An isometric view of the heat sink can be seen in
Figure 2.
It measures 90 mm in length and 50 mm in width, which is more than the surface of the heating element, and 69 mm in height. The fins have a wave-like surface geometry to increase the total surface area. It also has a central rib from the base of the heat sink to transfer the heat from the base to the top of the heat sink, then to each fin and to improve the cooling.
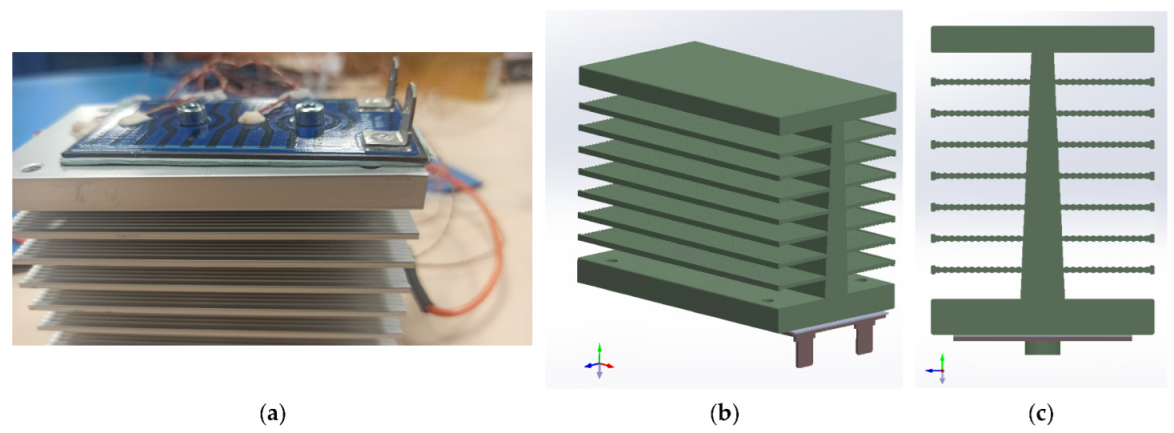
To measure the temperature, RS Pro Type K thermocouples are used. The thermocouples are attached to the thick film heater and heat sink using a ceramic glue. The effect of the ceramic glue on measuring was not evaluated; therefore, the error generated by this type of clamping is unknown. Seven thermocouples are used to measure the temperature as follows: two thermocouples on the thick film heater body (number 1 and 2), four thermocouples placed on the heat sink (number 3 to 6), and one thermocouple placed away from the heater to measure the ambient temperature. An overview of the thermocouple’s placement can be seen in
Table 1, and the exact position of the thermocouples on the heat source and heat sink can be seen in
Figure 3.
A power supply is used to supply current to the thick film element and generate heat. To gain the 17 W desired power, the power supply provided 56.7 V and 0.3 A. These values are fixed, and the same values were used for all the tested scenarios.
Figure 3a,b shows the measured assembly with thermocouples placed on it, and in
Figure 3c, the assembly has the gravity orientation parallel with the heating element length.
For the first measurement, the thick film heating element is placed under the heat sink. The corners of the heat sink are placed on the wood frame to allow the air natural movement around the heat sink and heat source. The placement of the assembly on the wood frame for Setup 1 measurements can be seen in
Figure 4a and the 3D CAD model used for the simulation with the gray arrow that shows gravity orientation perpendicular to the heating element can be seen in
Figure 4b.
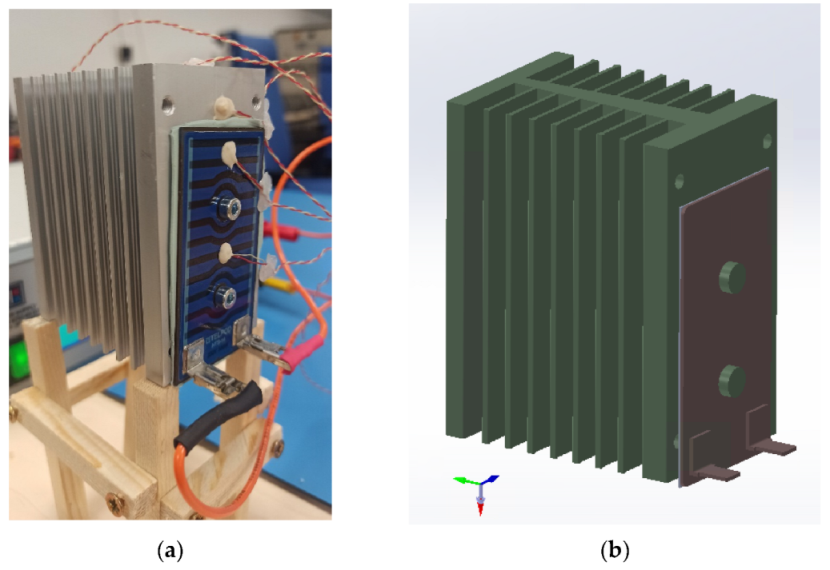
After the thick film heating element reached the steady state temperature, the heat sink was rotated 90° so that the fins are perpendicular to the working surface, as in
Figure 5. In this position, the hot air between the heat sink’s fins is expected to rise and start the natural cooling of the components. The assembly was kept in this position until there was no change in the temperatures measured; therefore, the temperature values reached a steady state.
The differences in measured values between the Setup 1 and Setup 2 are due to natural air movement and the improved passive cooling that comes with it. In both setups, the thick film heating element is fixed directly to the heat sink using screws, without any thermal interface material.
Setup 3 has the same orientation as the first one with the heating element parallel with the working surface. The only difference here is that a thermal interface material is used between the thick film heating element and heat sink, as can be seen in
Figure 6. The thermal interface material has the role of improving the thermal transfer between the heat source and heat sink; therefore, improving the cooling.
In the fourth setup used for the temperature measurement, the orientation of the assembly is same as in the second setup. The measured and simulated assembly can be seen in
Figure 7. The aim is to compare the measured temperatures from this setup with the ones from the third setup and evaluate the impact that changing the orientation has on this assembly.
The aim of the measurement is not to compare the setups with and without thermal interface material; therefore, the first and second setup will not be compared with the third and fourth.
To evaluate the influence of gravity using finite element analysis, a 3D CAD geometry was created to replicate the experimental assembly. The thick film heating element was simplified, using a stainless-steel substrate, and the heat dissipation component was placed on top of it. The heating element was fixed with two screws, also simplified, as shown in
Figure 8, since the thread of the screw requires too many computational resources to be simulated for a small thermal transfer influence.
An overview of the gravity vector orientation and use of thermal interface material can be seen in
Table 2.
The materials thermal conductivity used for the components are generic material from the Siemens Simcenter Flotherm XT 2021.2 library. The exact thermal conductivity and thermal radiation coefficient of the materials are unknown. The values used are the ones from
Table 3 and are assumptions, slightly modified in the calibration process to get similar results as in the real-life test, without significant modifications that may lead to an unrealistic setup.
The thermal radiation coefficient used for the heat sink is 0.8 since the aluminum has an anodized surface, significantly increasing the coefficient, compared to the shiny aluminum. The thick film has an even higher radiation coefficient because it is covered in a lacquer substrate that increases the value of the coefficient.
The model data information are presented in
Figure 9. The solution type is kept flowing. Additionally, the heat transfer analysis type is steady state, since the goal is to compare the temperature results after the system reaches thermal equilibrium, and no changes can be seen in the temperature values after that moment.
The turbulence type model is set to laminar and turbulent flow, which allows both types of fluid flows to be calculated in the simulation if needed. The default radiation surface emissivity is set to 0.4 for the components that do not have another thermal radiation coefficient applied particularly for that component.
The equations used by the software to calculate the solution are not visible, therefore, are not available to the user.
Flotherm XT 2021.2 uses a cell-centered finite volume method to obtain conservative approximations of the governing equations on the locally refined rectangular mesh. The governing equations are integrated over a control volume, which is a grid cell, then approximated with the cell centered values of the basic variables.
As in Setup 1 and Setup 2, the thick film is fixed directly on the heat sink without using any thermal interface material, a thermal resistance was added between the components, as shown in
Figure 10. The value set for the thermal insolence is 0.003 (Km
2)/W. As the virtual model the components are in perfect contact, which is ideal, the quantity of heat transferred between the heat source and heat sink was higher.
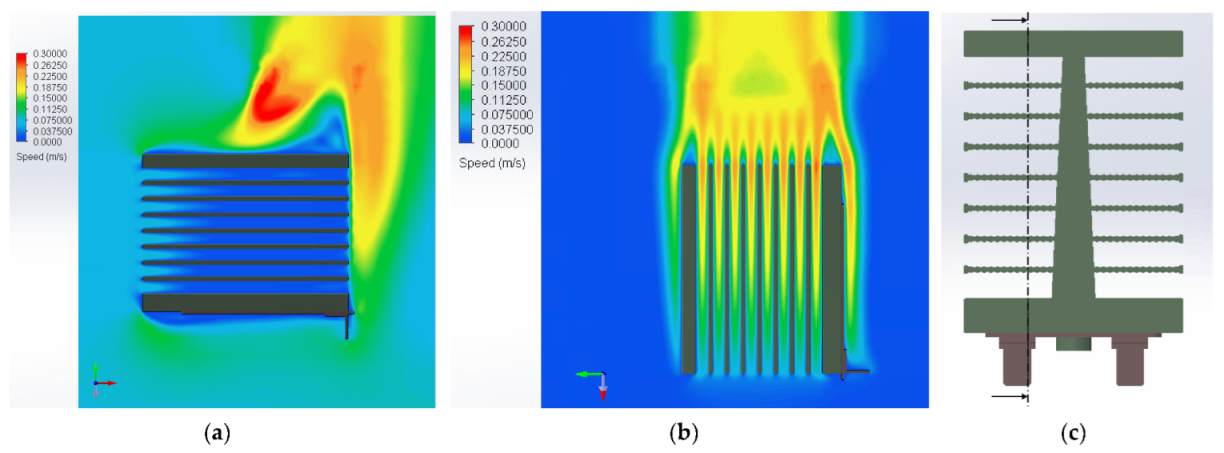
In Setup 1 and Setup 3, an external velocity value was set in the positive direction of the X axis, represented by the red arrow in
Figure 11. This is due to the natural air movement that occurs in the lab due to people moving or equipment fans functioning. The value of the velocity is 0.075 m/s and is an assumption, the actual value of the air speed in the laboratory was not measured.
In Setup 2 and Setup 4, where gravity increases the air movement on the X axis direction due to hot air moving, the external velocity was removed.
A mesh independent evaluation was completed at the beginning of the investigations. The goal was to reduce the error generated by the size of the mesh elements. An overview of the investigation results can be seen in
Table 4. The simulation temperature values between Try 6 and Try 7, measured in the same points, are less than 0.05 °C; therefore, the mesh from Try 7 is used for all four simulations. The total number of mesh elements exceeded 15,000,000.
In
Figure 12, the mesh resulted after Try 7 can be seen. A mesh region that includes all the components and surrounding areas is added, mesh refinement is applied on all the solid components, and a Surface Inflation Mesh was applied on the heat sink to reduce the element size in the proximity of the fins.
The first simulation, which replicates Setup 1, was calibrated. Therefore, certain parameters as emissivity, contact resistance and airflow were added until the simulation results were similar to the measured values.
For the rest of the simulation, the same parameters were kept, except the gravity orientation and thermal interface material for Setup 3 and Setup 4.
Flotherm XT 2021.2 uses Navier–Stokes Equations for laminar and turbulent fluid flows. The software also uses K-E model for the transport equations for the turbulent kinetic energy and its dissipation rate.
The conservation laws for mass, angular momentum and energy, in the conservation form, can be written as follows:
where:
is the fluid velocity;
is the fluid density;
is a mass-distributed external force per unit mass:
where:
is due to porous media resistance;
is due to buoyancy and ’; where is the gravitational acceleration component along the i-th coordinate direction;
is due to the coordinate system’s rotation;
is the thermal enthalpy;
is a heat source or sink per unit volume;
is the viscous shear stress tensor;
is the diffusive heat flux.
For Newtonian fluids, the viscous shear stress tensor is defined as:
Following Boussinesq assumption, the Reynolds-stress tensor has the following form:
is the Kronecker delta function (equal to one when I = j, otherwise zero);
is the dynamic viscosity coefficient;
is the turbulent eddy viscosity coefficient; and k is the turbulent kinetic energy.
Since Flotherm XT is also a thermal transfer simulation software, it is able to predict that the heat transfer is made through solids and fluids with energy exchanging between them. Heat transfer fluids are defined by Equation (3). Anisotropic heat conductivity in solids is described by the following equation:
is the specific internal energy: , where is specific heat;
is specific heat release (or absorption) per unit volume;
are the eigenvalues of the thermal conductivity tensor.
Heat dissipated through radiation by a surface or radiation source can be defined, for thermal radiation, as:
is the surface emissivity;
is the Stefan-Boltzmann constant;
is the temperature of the surface ( is the heat radiated by this surface in accordance with the Stefan-Boltzmann law);
is the radiative surface area;
is the incident thermal radiation arriving at this surface.