Numerical Analysis of the Free-Falling Process of a Water Droplet at Different Temperatures
Abstract
:1. Introduction
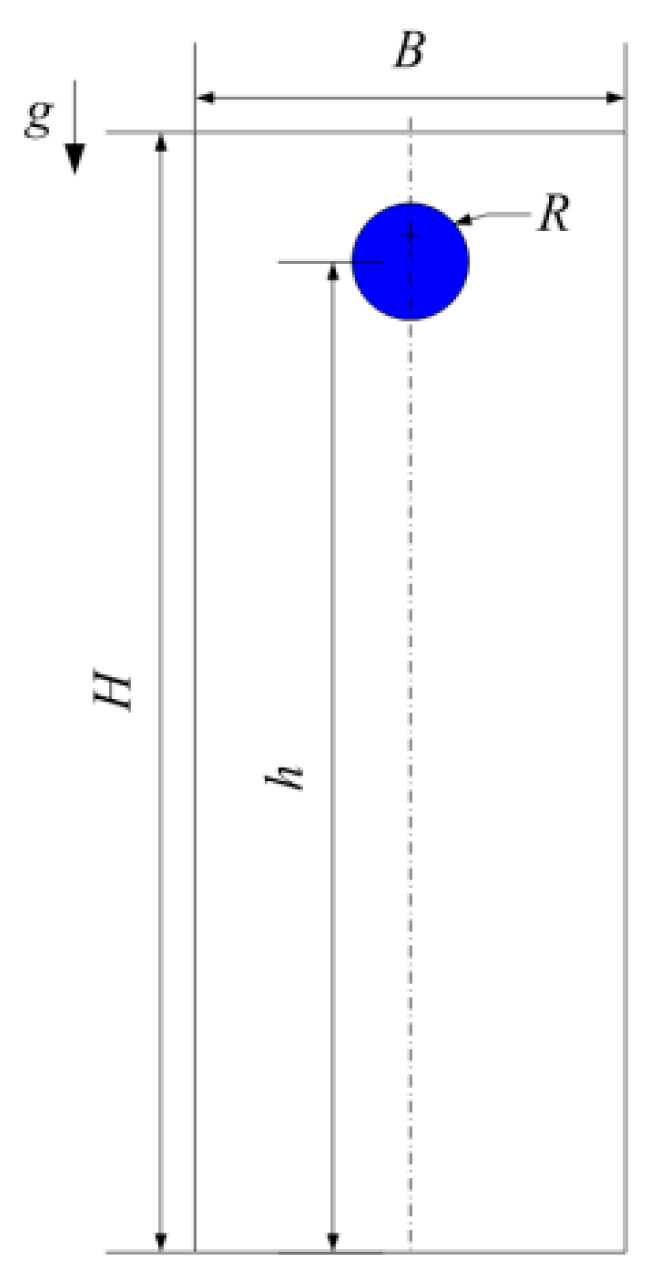
2. Force Description of a Falling Water Droplet
3. Two-Phase Flow Model of Water Droplet and Air
3.1. Numerical Model and Governing Equations
3.2. Grid Study and Validation
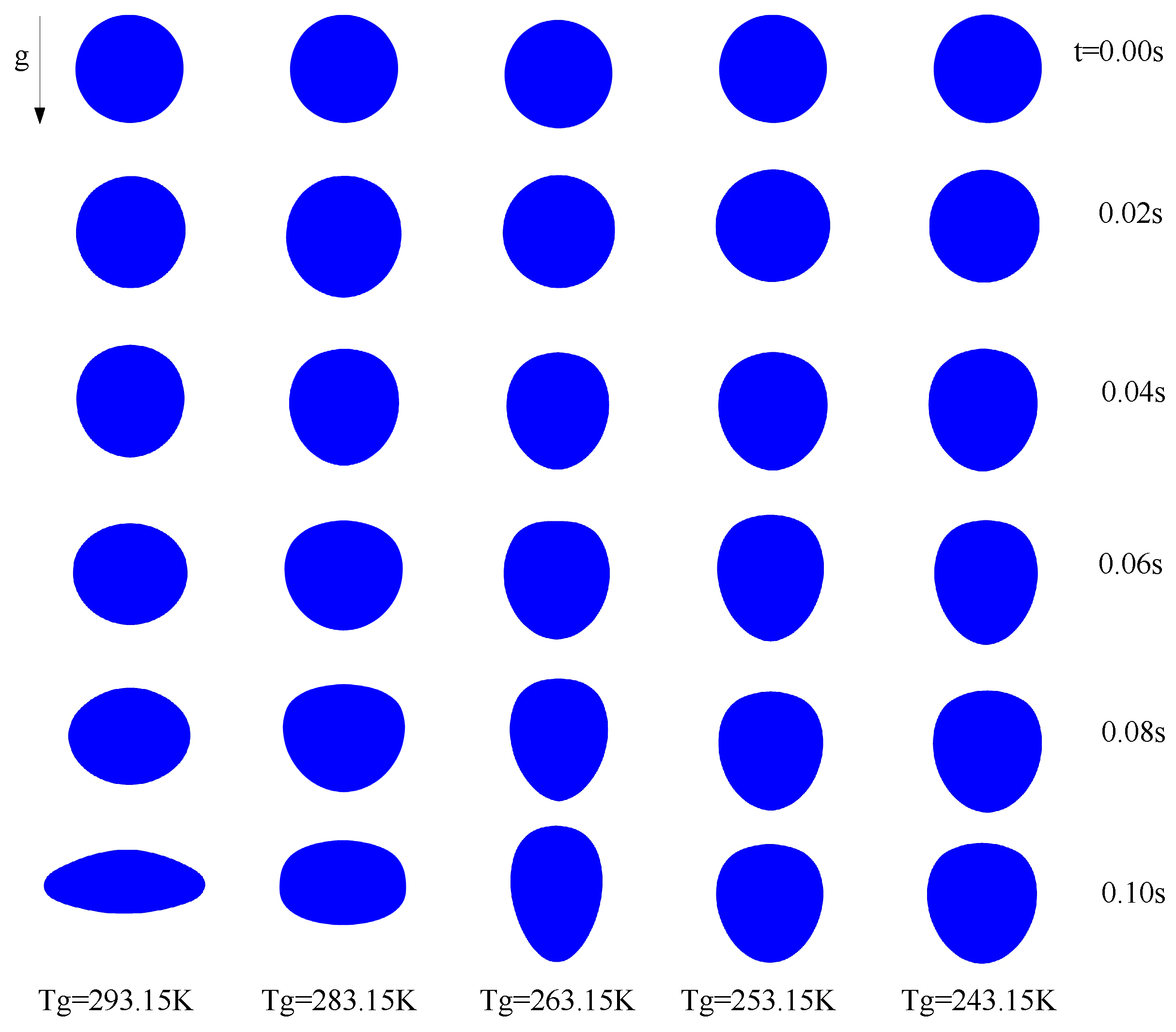
4. Numerical Results of the Free-Falling Process
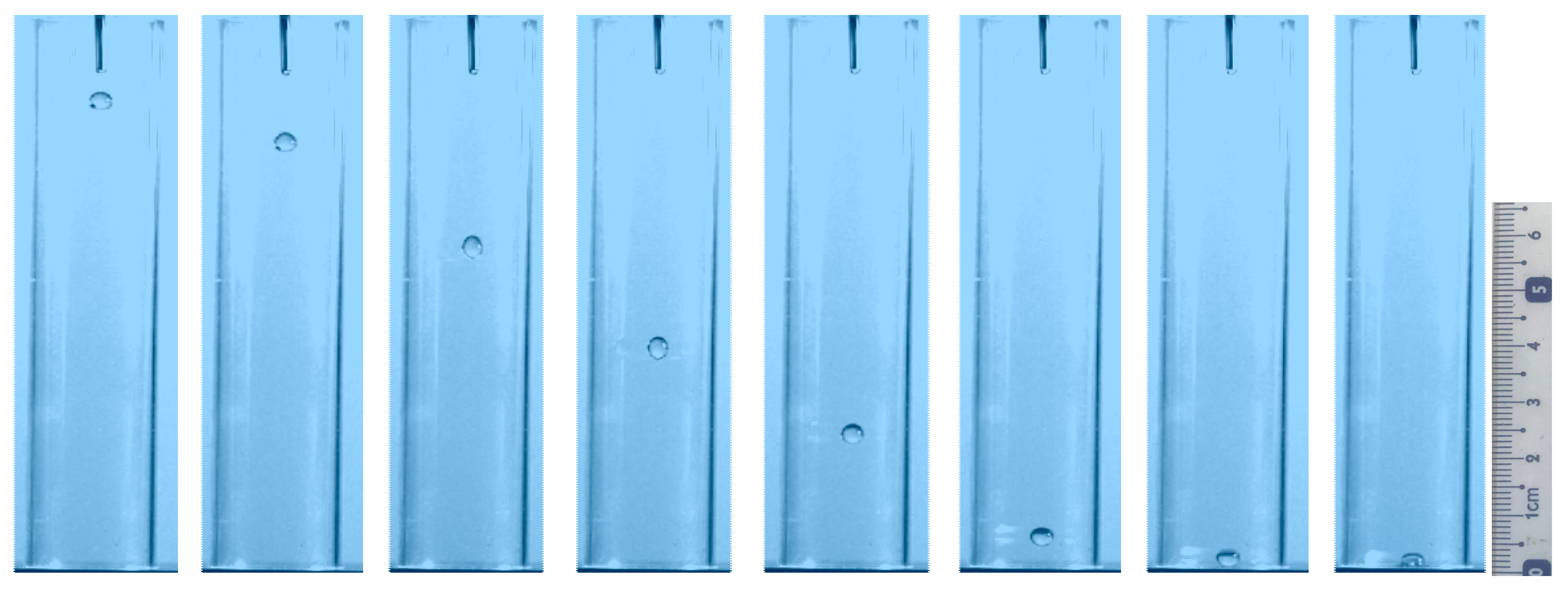
5. A Test of Free Falling
6. Discussion of Characteristic Parameters
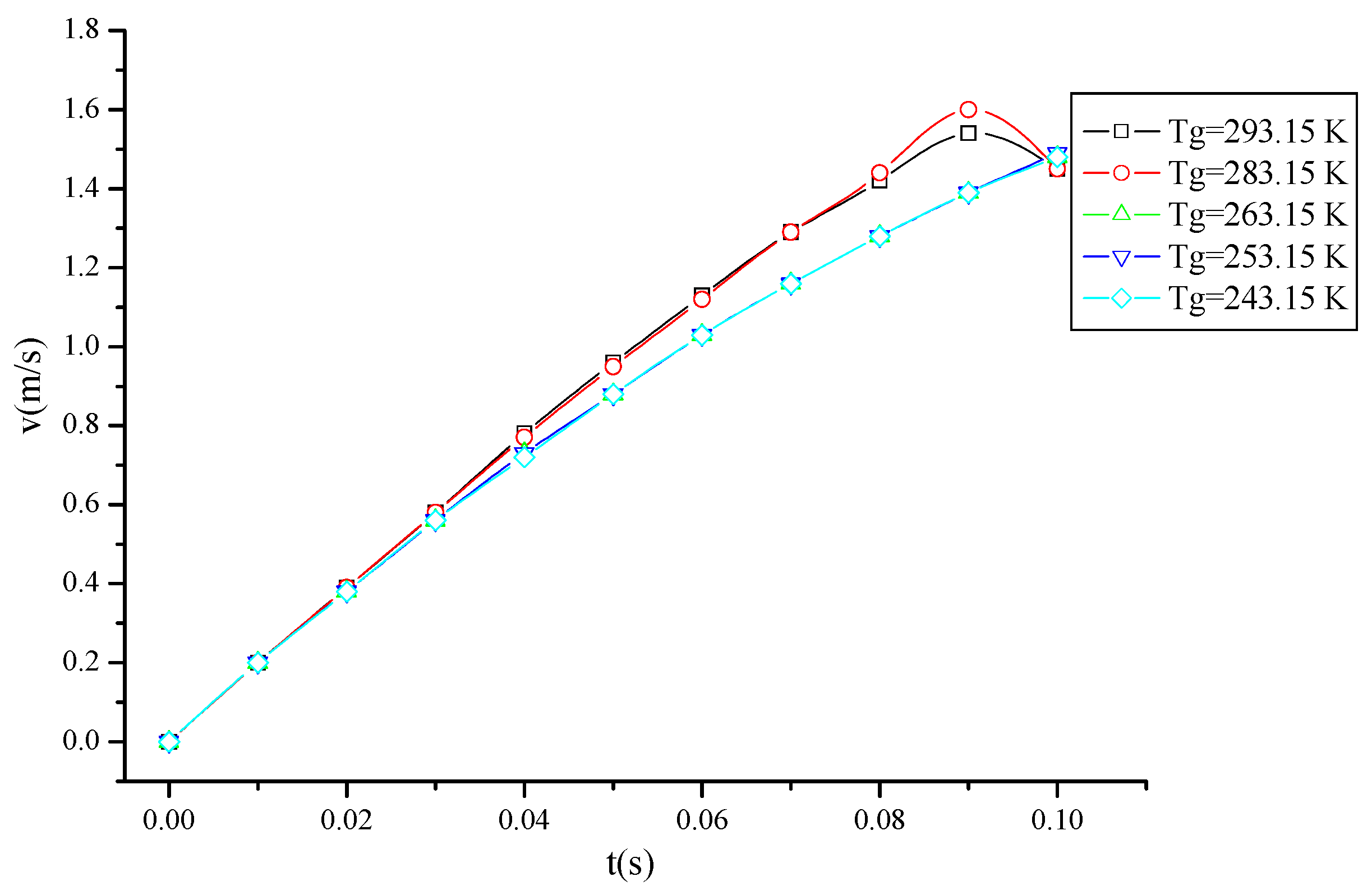
6.1. Velocity of Free-Falling Water Droplet
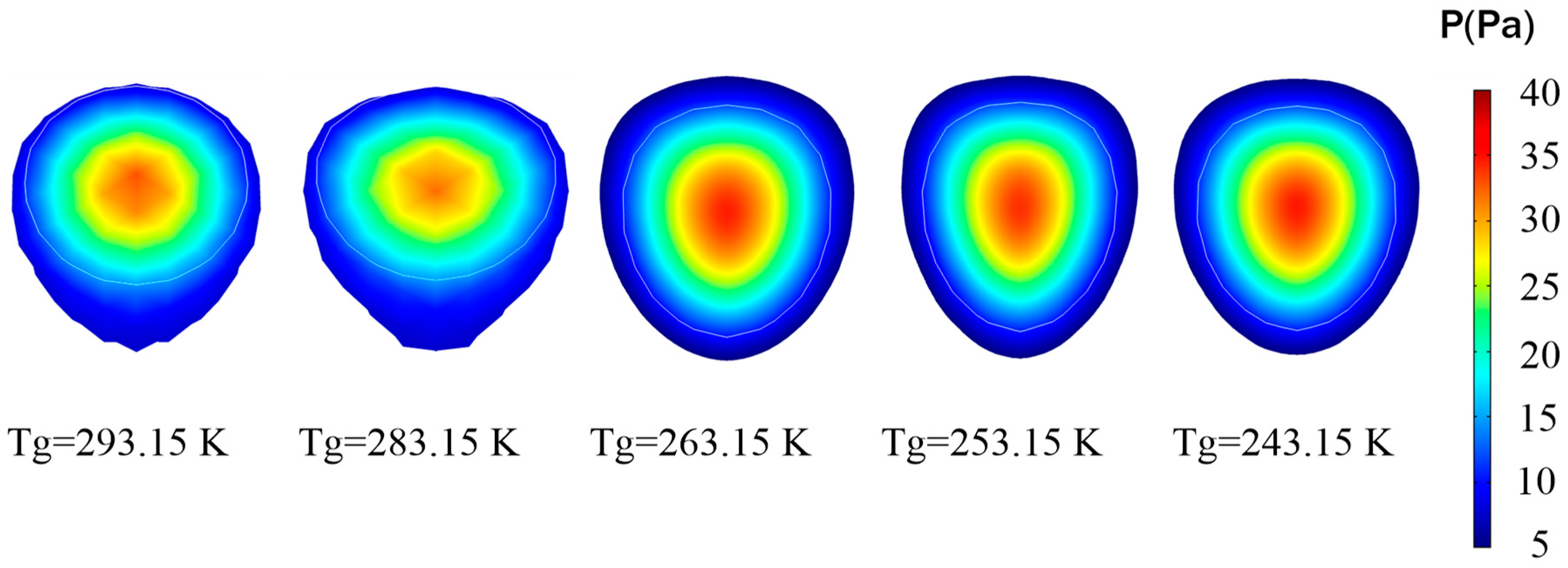
6.2. Pressure Distribution of Water Droplet
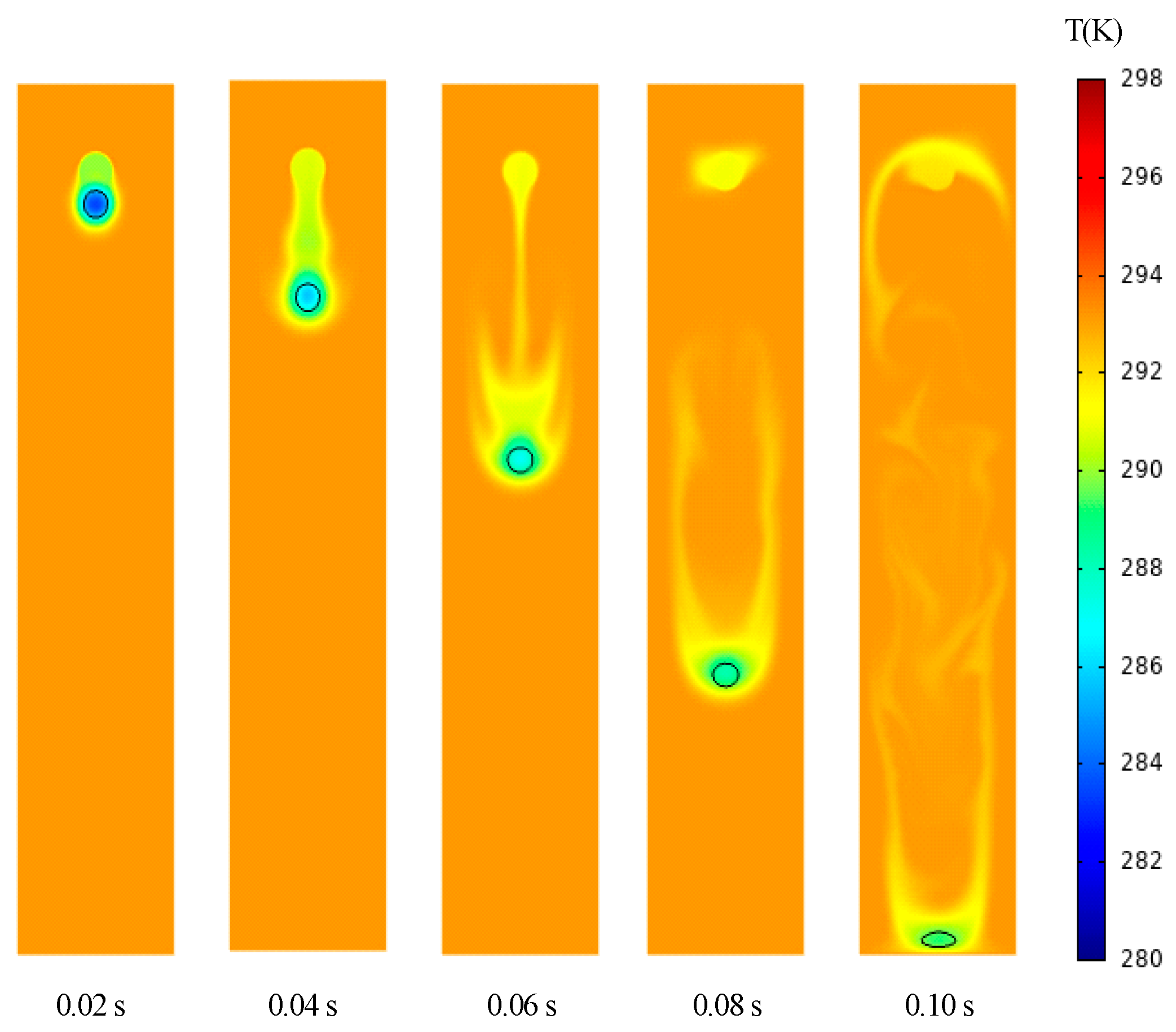
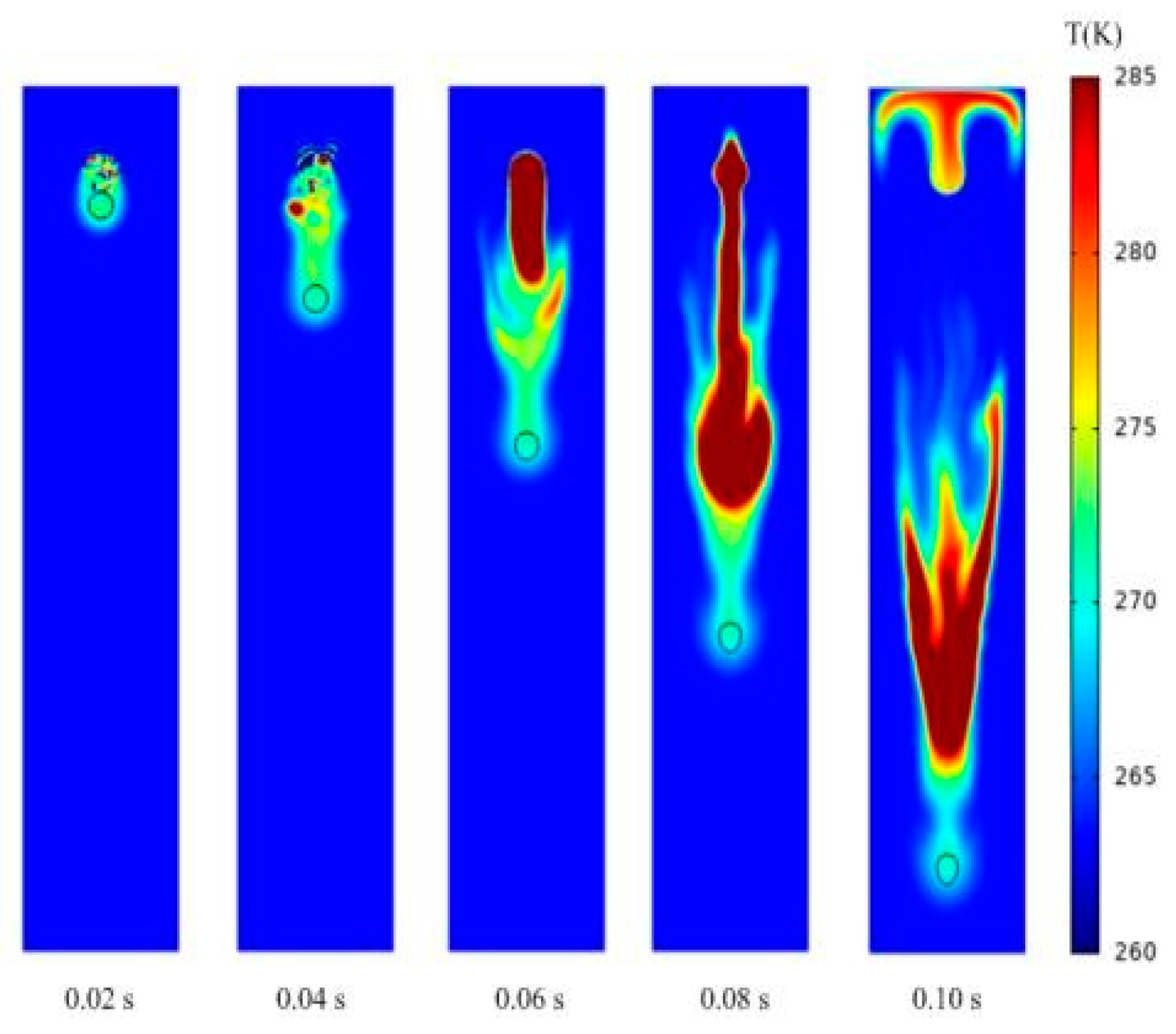
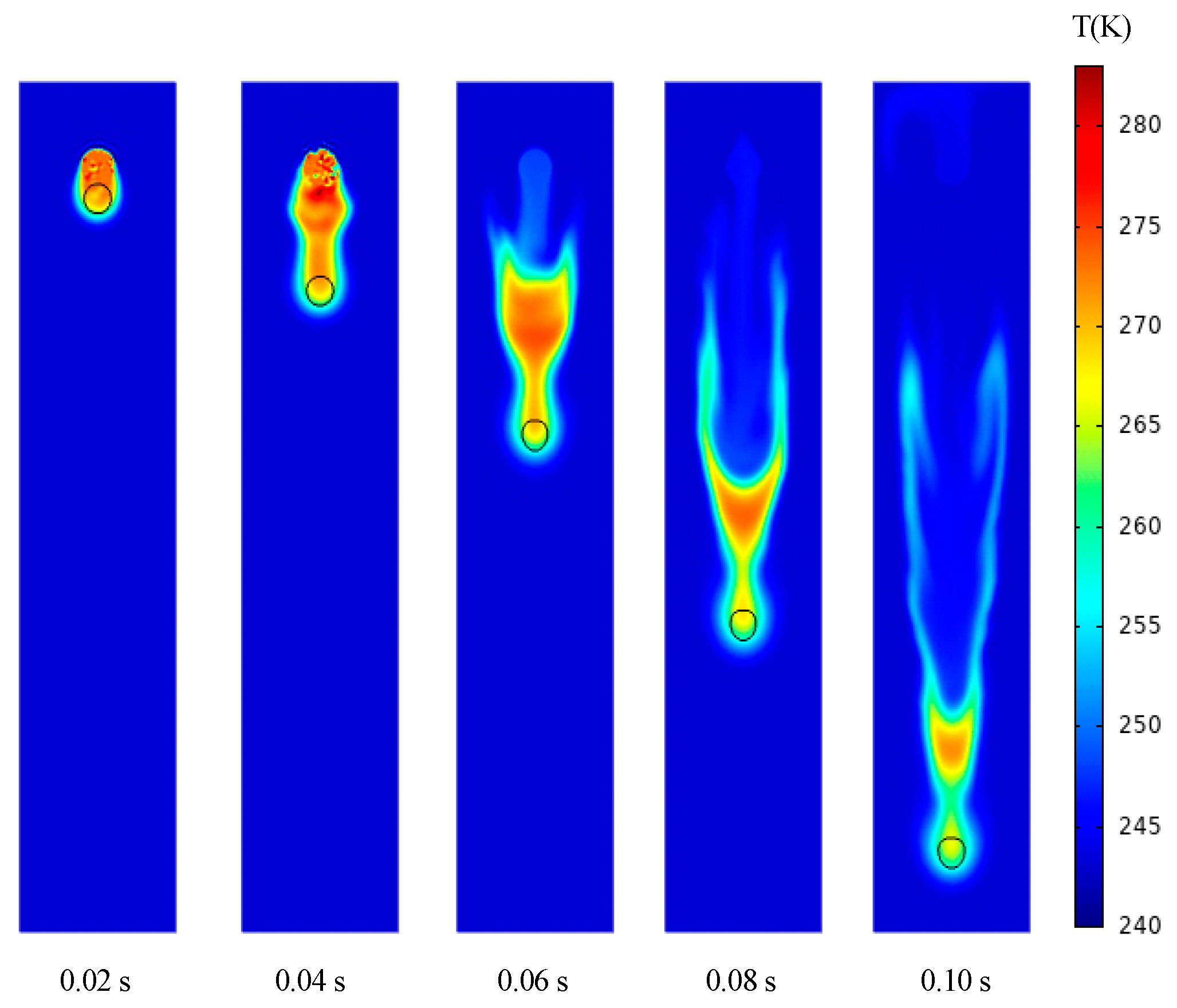
6.3. Temperature Field
6.4. Water Droplet Impacting on Bottom
6.5. Water Droplet Freezing on Bottom
7. Conclusions
- (1)
- In the process of free falling, the water droplet deforms due to its surrounding forces. Before impinging on the bottom, the water droplet is the shape of a flat ellipse at 293.15 K, a drum at 283.15 K, a melon seed at 263.15 K and a human face at 253.15 K and 243.15 K when the falling time is 0.10 s.
- (2)
- The falling velocity of the water droplet is affected by the temperature. In the process of falling in air at a sub-zero temperature, the water droplet experiences a continuous increase in its falling velocity. When the water droplet falls in air at a mild temperature, the falling velocity is slightly larger than that in air at a sub-zero temperature, and there is a velocity drop at 0.10 s due to the impinging on the bottom surface.
- (3)
- There are great differences in the distribution of inner pressure and temperature. When the water droplet falls in air at a sub-zero temperature, there is a temperature increase behind the water droplet due to the latent heat released by ice freezing.
- (4)
- In the air at a sub-zero temperature, the phase change of the water droplet first occurs at the bottom, and then propagates inwards from the top of the water droplet, and at last the phase change finishes at the inner of the water droplet with a line.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mader, G.; Thybo, C. A new method of defrosting evaporator coils. Appl. Therm. Eng. 2012, 39, 78–85. [Google Scholar] [CrossRef]
- Peng, H.; Wang, Q.; Wang, T.; Li, L.; Xia, Z.; Du, J.; Zheng, B.; Zhou, H.; Ye, L. Study on dynamics and freezing behaviors of water droplet on superhydrophobic aluminum surface. Appl. Phys. A 2020, 126, 811. [Google Scholar] [CrossRef]
- Rao, L.; Bao, Y.; Cai, Z.; Gao, Z. Effects of surfactants on the dynamic behavior of single buoyancy driven drops in Newtonian fluids. J. B. Univ. Chem. Technol. (Nat. Sci.) 2016, 43, 33–39. [Google Scholar]
- Yu, C.; Zhengzhi, W.; Chunling, Z. Numerical simulation of engine nacelle icing under supercooled large droplet conditions. Aeronaut. Comput. Tech. 2019, 49, 59–63. [Google Scholar]
- Quero, M.; Hammond, D.W.; Purvis, R.; Smith, F.T. Analysis of super-cooled water droplet impact on a thin water layer and ice growth. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006; p. 466. [Google Scholar]
- Weimin, S.; Yunze, J.; Tian, L. Numerical analysis of splashing for icing effects at supercooled large droplet impingement. J. Northwest. Polytech. Univ. 2016, 34, 739–746. [Google Scholar]
- Beacham, S.T.; Tilger, C.F. Oehlschlaeger, MA, Sound generation by water drop impact on surfaces. Exp. Therm Fluid Sci. 2020, 117, 110138. [Google Scholar] [CrossRef]
- Mengjiy, W.; Yilin, H.; Fanghsing, L.; Shiyow, L. Dynamic behaviors of droplet impact and spreading: A universal relationship study of dimensionless wetting diameter and droplet height. Exp. Therm Fluid Sci. 2009, 33, 1112–1118. [Google Scholar]
- Mengjiy, W.; Fanghsing, L.; Junyan, O.; Shiyow, L. Dynamic behaviors of droplet impact and spreading-Water on glass and paraffin. Colloids Surf. A 2009, 339, 224–231. [Google Scholar]
- Norouzi, M.; Sheykhian, M.K.; Shahmardan, M.M.; Shahbani-Zahiri, A. Experimental investigation of spreading and receding behaviors of Newtonian and viscoelastic droplet impacts on inclined dry surfaces. Meccanica 2021, 56, 125–145. [Google Scholar] [CrossRef]
- Chuankang, P.; Bingqian, W.; Hong, Y. Numerical investigation of large bubble entrapment mechanism for micron droplet impact on deep pool. Acta Phys. Sin. 2019, 68, 204703. [Google Scholar]
- Mingshun, J.; Zheyan, J.; Zhigang, Y. Summary of experimental study on droplet icing at different ambient temperatures. Machinery 2018, 56, 110–114. [Google Scholar]
- Gao, W.; Smith, D.W.; Sego, D.C. Freezing behavior of freely suspended industrial wastewater droplets. Cold Reg. Sci. Technol. 2000, 31, 13–26. [Google Scholar] [CrossRef]
- Zhang, H.F.; Zhao, Y.G.; Lv, R.; Yang, C. Freezing of sessile water droplet for various contact angles. Int. J. Therm. Sci. 2016, 101, 59–67. [Google Scholar] [CrossRef]
- Huang, L.Y.; Liu, Z.L.; Liu, Y.M.; Gou, Y.J.; Wang, L. Effect of contact angle on water droplet freezing process on a cold flat surface. Exp. Therm Fluid Sci. 2012, 40, 74–80. [Google Scholar] [CrossRef]
- Shang, Y.H.; Bai, B.F.; Hou, Y.; Zhong, X. Experimental research for freezing characteristics of droplets impacting on supercooled surface. J. XI’an Jiaotong Univ. 2021, 55, 144–149. [Google Scholar]
- Victor, V.; Mohammadreza, K.; Mohammad, A.M.; Mohamed, M.; Hameed, A.; Omar, K.M.; Christos, N.M. A combined experimental and computational study of phase-change dynamics and flow inside a sessile water droplet freezing due to interfacial heat transfer. Int. J. Heat Mass Transfer 2021, 180, 121803. [Google Scholar]
- Jin, Z.; Sui, D.; Yang, Z. The impact, freezing, and melting processes of a water droplet on an inclined cold surface. Int. J. Heat Mass Transfer 2015, 90, 439–453. [Google Scholar] [CrossRef]
- Chaudhary, G.; Li, R. Freezing of water droplets on solid surfaces: An experimental and numerical study. Exp. Therm Fluid Sci. 2014, 57, 86–93. [Google Scholar] [CrossRef]
- Attarzadeh, R.; Dolatabadi, A. Icephobic performance of superhydrophobic coatings: A numerical analysis. Int. J. Heat Mass Transfer 2019, 136, 1327–1337. [Google Scholar] [CrossRef]
- Meng, Z.; Zhang, P. Freezing dynamics of supercooled micro-sized water droplets. Int. J. Heat Mass Transfer 2022, 193, 122955. [Google Scholar] [CrossRef]
- Daichi, U.; Seia, F.; Yoshimichi, H. Numerical simulation on the freezing of deposited or impinged water droplets on a cold surface. J. Therm. Sci. Technol. 2021, 16, JTST0006. [Google Scholar]
- Wangt, L.; Tian, Z.; Jiang, G.; Luo, X.; Chen, C.; Hu, X.; Zhang, H.; Zhong, M. Spontaneous dewetting transitions of droplets during icing & melting cycle. Nat. Commun. 2022, 13, 378. [Google Scholar]
- Doston, S.; Dmitry, E.; Boris, V.B.; Svyatoslav, C.; Stein, T.J.; Iskander, A. Modeling Water Droplet Freezing and Collision with a Solid Surface. Energies 2021, 14, 1020. [Google Scholar]
- Sultana, K.; Pope, K.; Lam, L.; Muzychka, Y. Phase change and droplet dynamics for a free falling water droplet. Int. J. Heat Mass Transfer 2017, 115, 461–470. [Google Scholar] [CrossRef]
- Feng, D.; Jinsong, H.; Yan, H.; Huanming, L. Numerical analysis of dynamic and heat transfer characteristics of droplet sprayed in pressurizer. Nucl. Power Eng. 2013, 34 (Suppl. S1), 136–140. [Google Scholar]
- Farhan, N.M.; Tafreshi, H.V. Using magnetic field to measure detachment force between a nonmagnetic droplet and fibers. Langmuir 2019, 35, 8490–8499. [Google Scholar] [CrossRef]
- Zhihong, Z.; Xian, Y.; Yewei, G.; Jianmin, Q. Icing scaling law with the dynamic effects of water droplets. J. Exp. Fluid Mech. 2016, 30, 20–25. [Google Scholar]
- Xueqin, B.; Guiping, L. Prediction of collection efficiency and anti-icing surface temperature. J. Beijing Univ. Aeronaut. Astronaut. 2007, 33, 1182–1185. [Google Scholar]




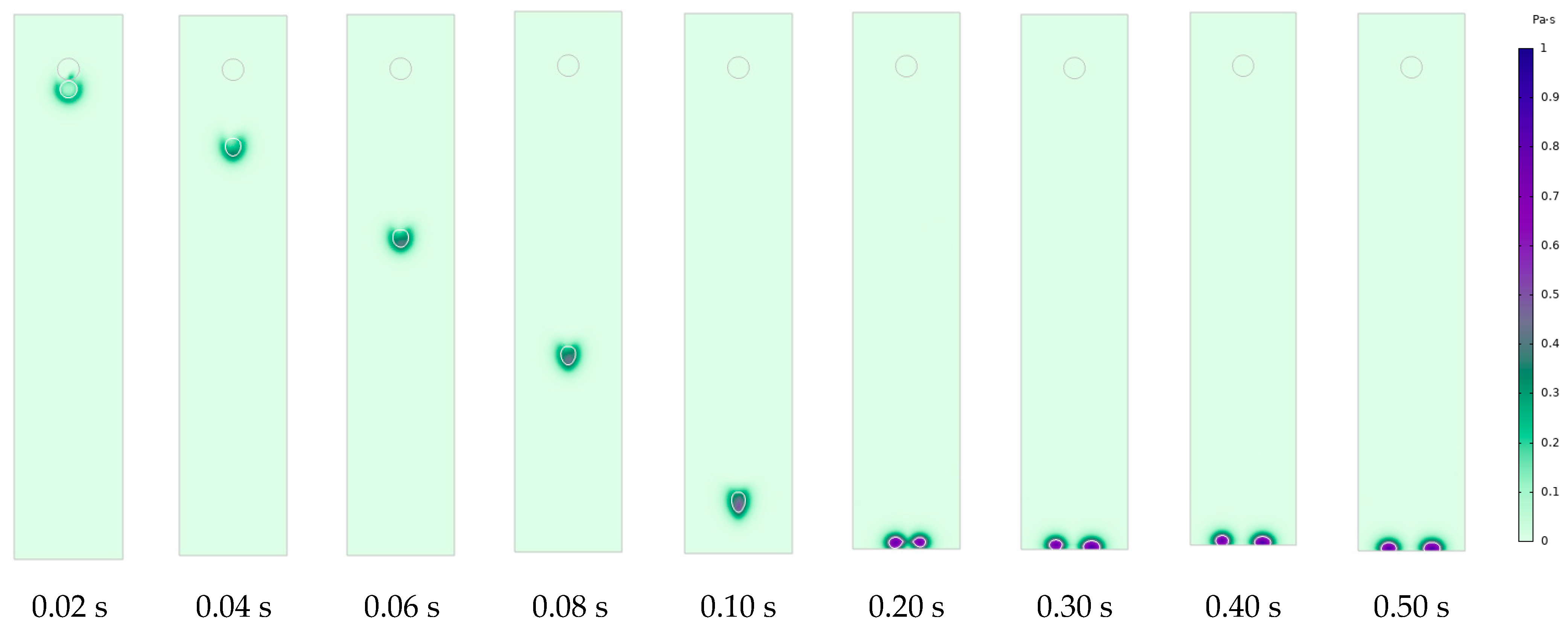
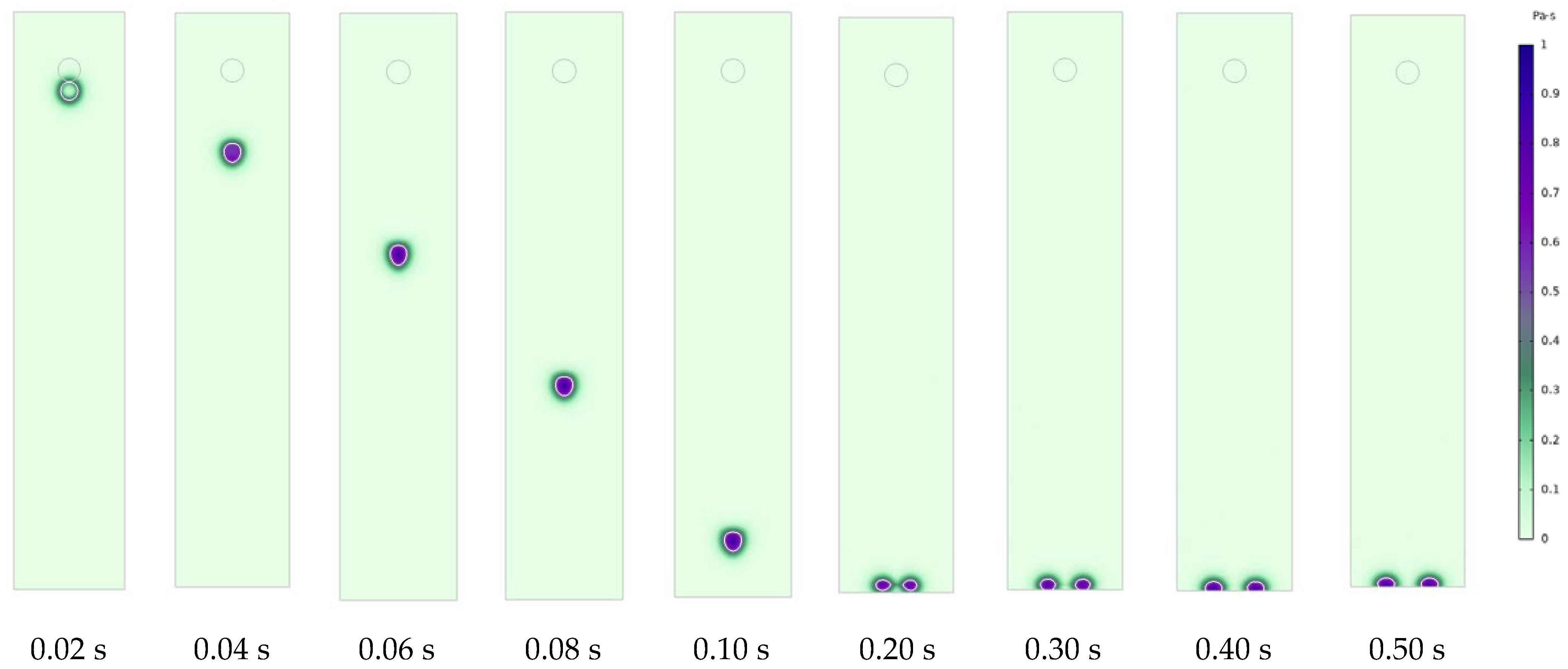




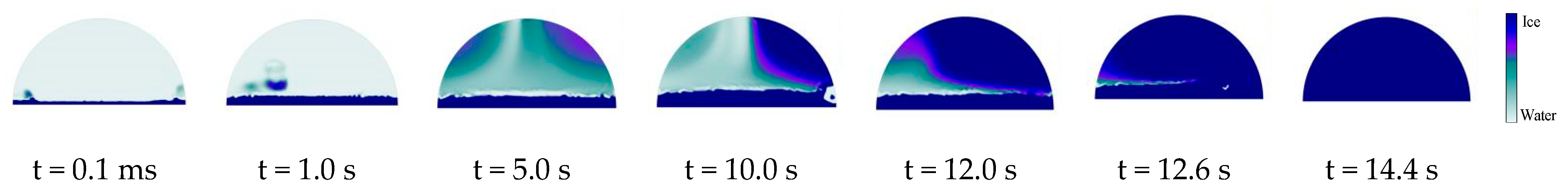
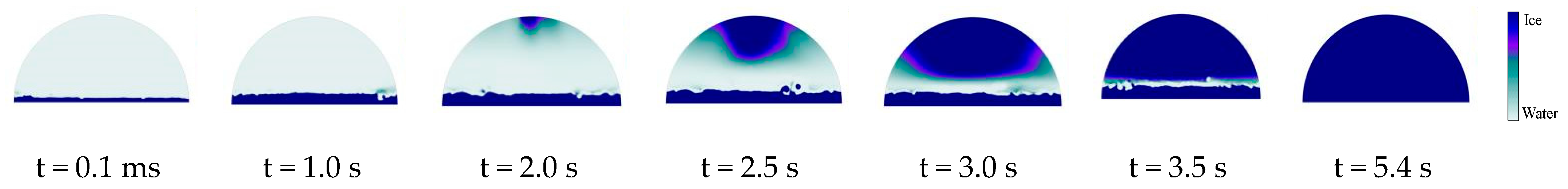
| Case | Mean Quality of Grid | The Lowest Quality of Grid |
|---|---|---|
| Case 1 | 0.8540 | 0.7316 |
| Case 2 | 0.8368 | 0.6929 |
| Case 3 | 0.8740 | 0.7633 |
| Case 4 | 0.8768 | 0.6960 |
| Tg (K) | t = 0.02 s | t = 0.04 s | t = 0.06 s | t = 0.08 s | t = 0.09 s | t = 0.10 s |
|---|---|---|---|---|---|---|
| 293.15 | 7.84 | 34.86 | 73.16 | 115.52 | 135.87 | 120.46 |
| 283.15 | 8.27 | 33.97 | 71.87 | 118.80 | 146.67 | 120.46 |
| 263.15 | 7.30 | 30.53 | 60.78 | 93.87 | 110.69 | 125.49 |
| 253.15 | 7.59 | 30.53 | 60.78 | 93.87 | 110.69 | 127.19 |
| 243.15 | 7.30 | 29.70 | 60.78 | 93.87 | 110.69 | 125.49 |
| Time | Tg (K) | V (m/s) | Dw (mm) | We(10−2) | Oh(10−4) | ||
|---|---|---|---|---|---|---|---|
| 0.04 s | 293.15 | 0.78 | 3.11 | 161.56 | 4.47 | 3.17 | 11.02 |
| 283.15 | 0.77 | 3.03 | 165.04 | 4.47 | 3.11 | 10.68 | |
| 263.15 | 0.73 | 3.03 | 177.60 | 4.24 | 3.01 | 9.76 | |
| 253.15 | 0.73 | 3.10 | 194.97 | 4.19 | 3.20 | 9.18 | |
| 243.15 | 0.72 | 3.03 | 202.13 | 4.16 | 3.17 | 8.81 | |
| 0.09 s | 293.15 | 1.54 | 3.27 | 335.21 | 8.60 | 12.98 | 10.75 |
| 283.15 | 1.60 | 3.00 | 340.68 | 9.32 | 13.32 | 10.71 | |
| 263.15 | 1.39 | 3.16 | 352.69 | 7.90 | 11.37 | 9.56 | |
| 253.15 | 1.39 | 2.96 | 354.36 | 8.16 | 11.08 | 9.39 | |
| 243.15 | 1.39 | 2.96 | 380.85 | 8.16 | 11.54 | 8.92 | |
| 0.10 s | 293.15 | 1.45 | 4.27 | 412.15 | 7.09 | 15.02 | 9.40 |
| 283.15 | 1.45 | 4.00 | 411.48 | 7.32 | 14.58 | 9.28 | |
| 263.15 | 1.48 | 4.16 | 494.46 | 7.33 | 16.97 | 8.33 | |
| 253.15 | 1.49 | 3.96 | 508.16 | 7.56 | 17.04 | 8.12 | |
| 243.15 | 1.48 | 3.96 | 542.48 | 7.51 | 17.51 | 7.71 |
| Grids | Normal | Refined 1 | Refined 2 |
|---|---|---|---|
| Numbers | 1548 | 2948 | 6292 |
| Mean mesh quality | 0.8183 | 0.8527 | 0.8337 |
| Time(s) | 7.8 | 5.4 | 5.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Zhang, Y.; Gao, H. Numerical Analysis of the Free-Falling Process of a Water Droplet at Different Temperatures. Processes 2023, 11, 258. https://doi.org/10.3390/pr11010258
Song Y, Zhang Y, Gao H. Numerical Analysis of the Free-Falling Process of a Water Droplet at Different Temperatures. Processes. 2023; 11(1):258. https://doi.org/10.3390/pr11010258
Chicago/Turabian StyleSong, Yuchao, Yafei Zhang, and Hongtao Gao. 2023. "Numerical Analysis of the Free-Falling Process of a Water Droplet at Different Temperatures" Processes 11, no. 1: 258. https://doi.org/10.3390/pr11010258
APA StyleSong, Y., Zhang, Y., & Gao, H. (2023). Numerical Analysis of the Free-Falling Process of a Water Droplet at Different Temperatures. Processes, 11(1), 258. https://doi.org/10.3390/pr11010258








