Simulation and Analysis of the Working Process of Soil Covering and Compacting of Precision Seeding Units Based on the Coupling Model of DEM with MBD
Abstract
:1. Introduction
2. Materials and Methods
2.1. Soil Bin Test
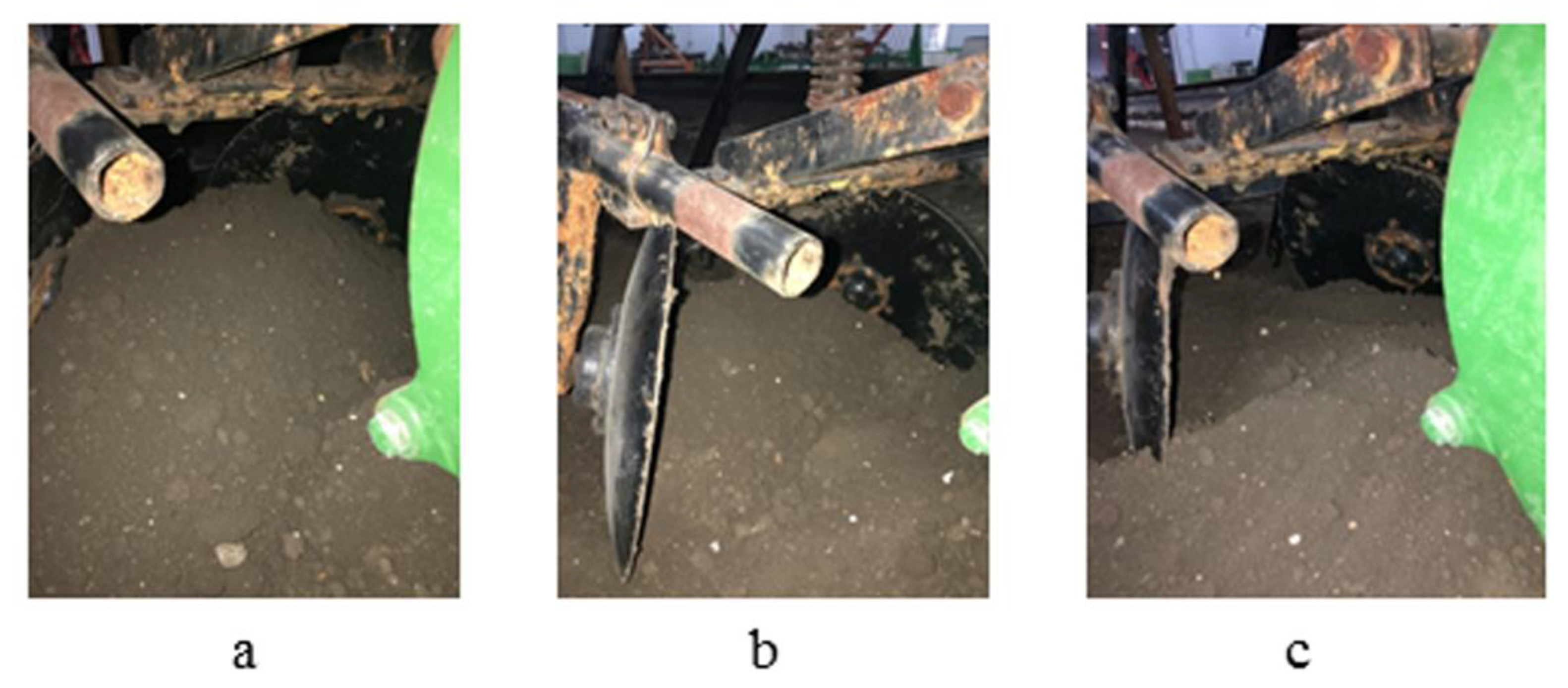
2.1.1. Soil Covering Test
2.1.2. Soil Compacting Test
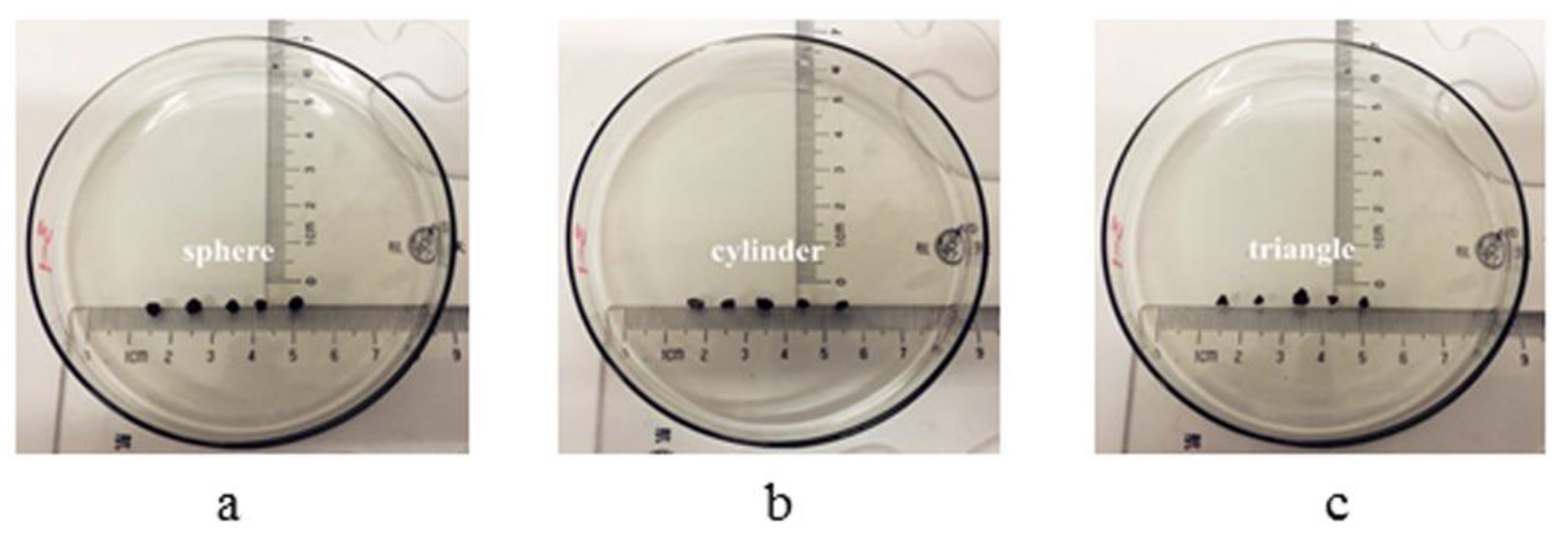
2.1.3. Experimental Results Analysis
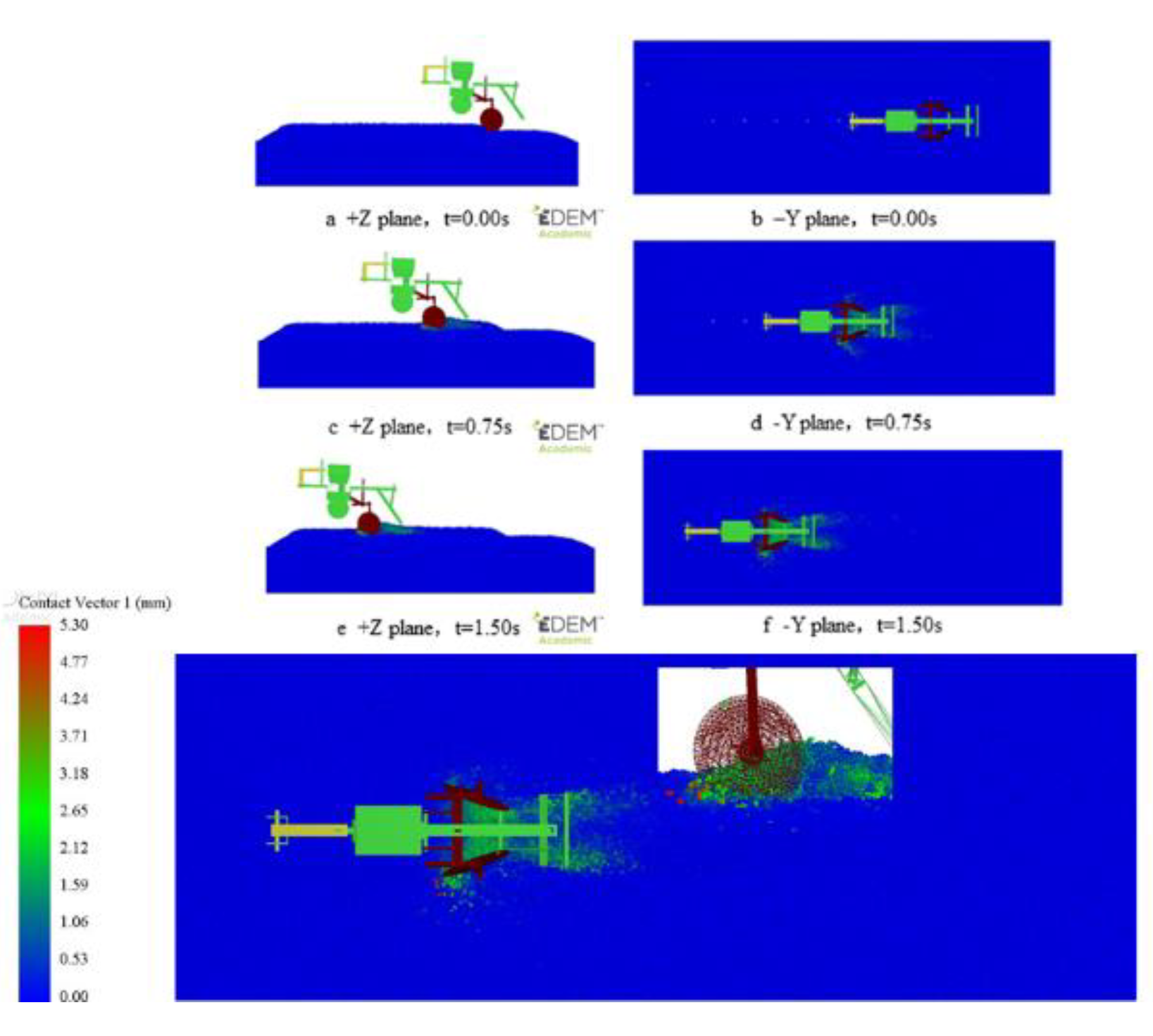
2.2. Simulation Study
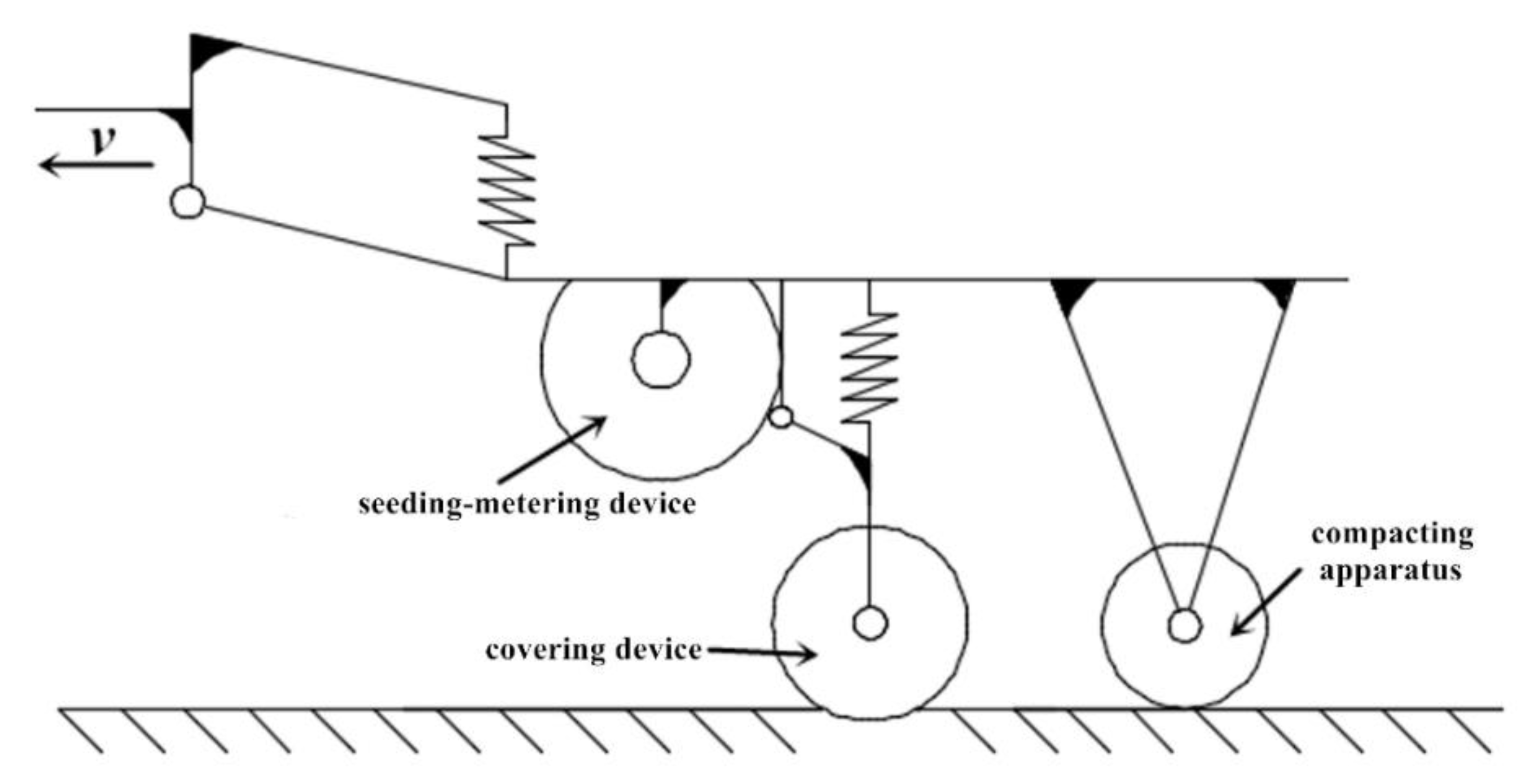
2.2.1. Precision Seeding Unit Modeling
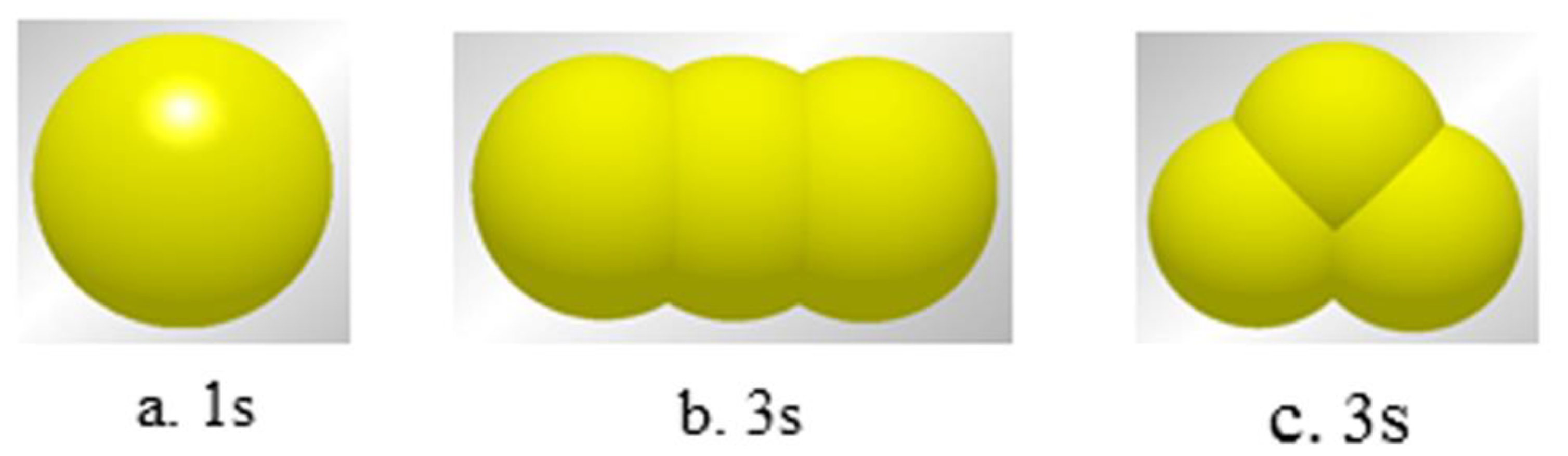
2.2.2. Particle Modeling
3. Discussion
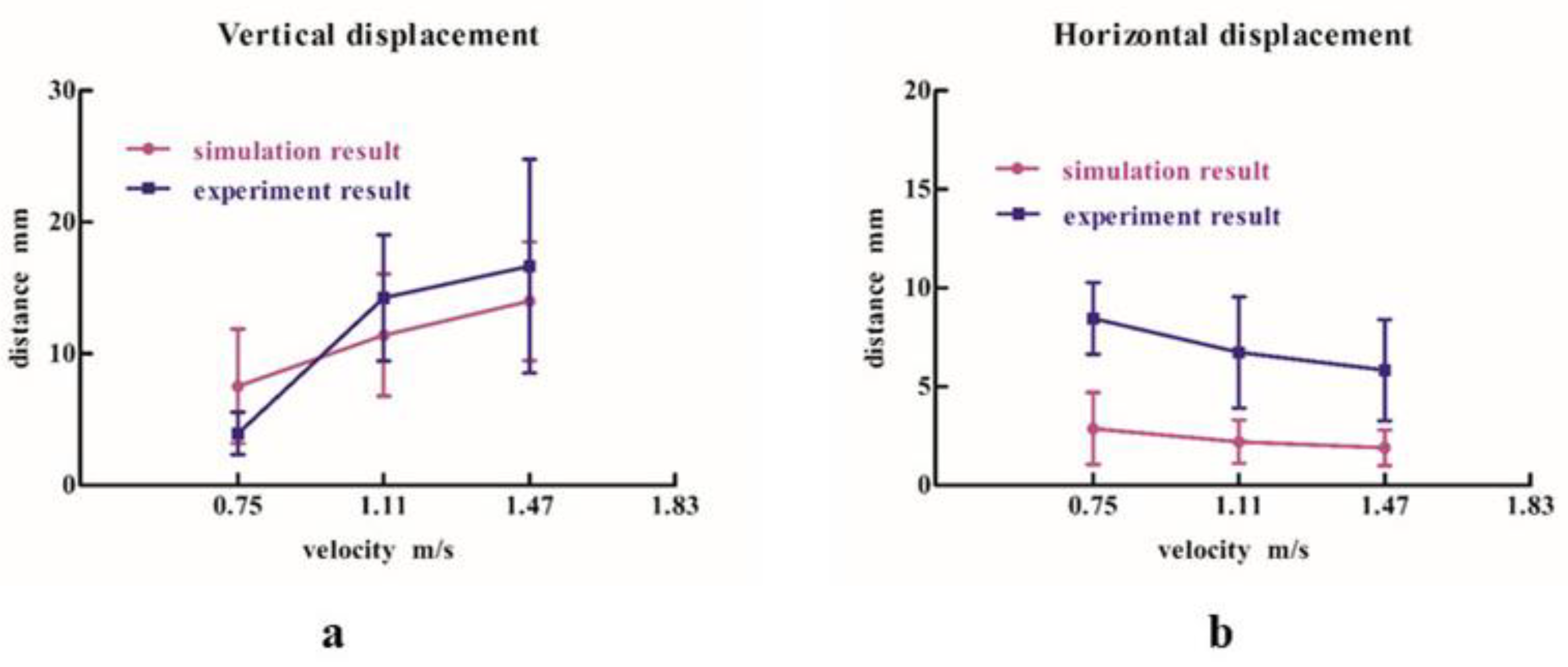
3.1. Comparison between the Simulated Results and the Experimental Results of the Soil Covering Test
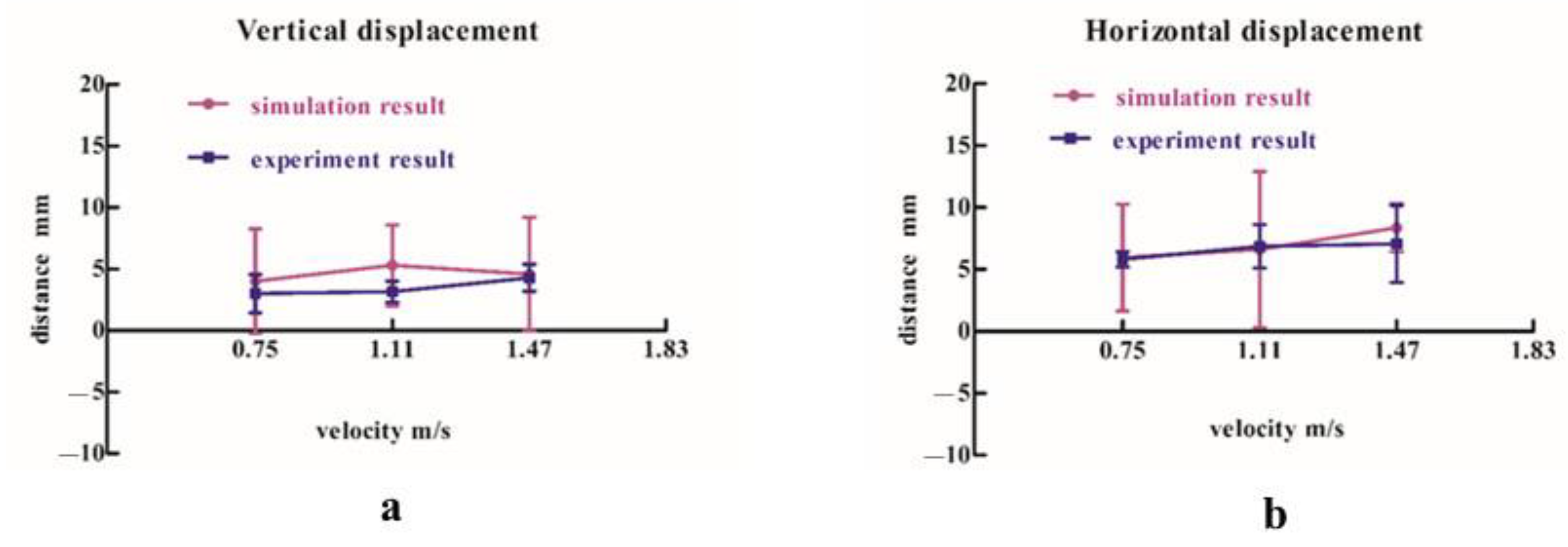
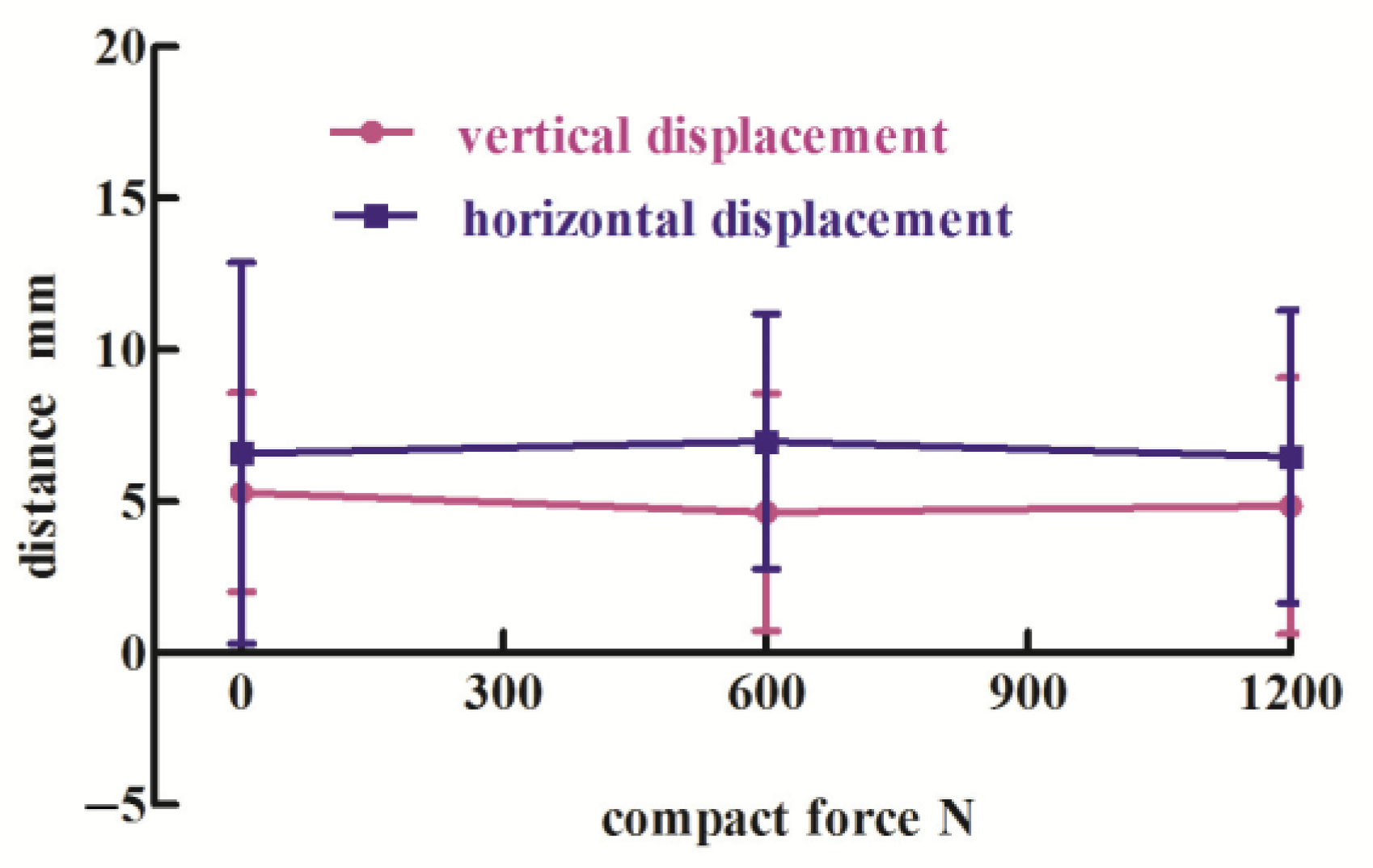
3.2. Comparison between the Simulated Results and the Experimental Results of Soil Compacting Test
4. Conclusions
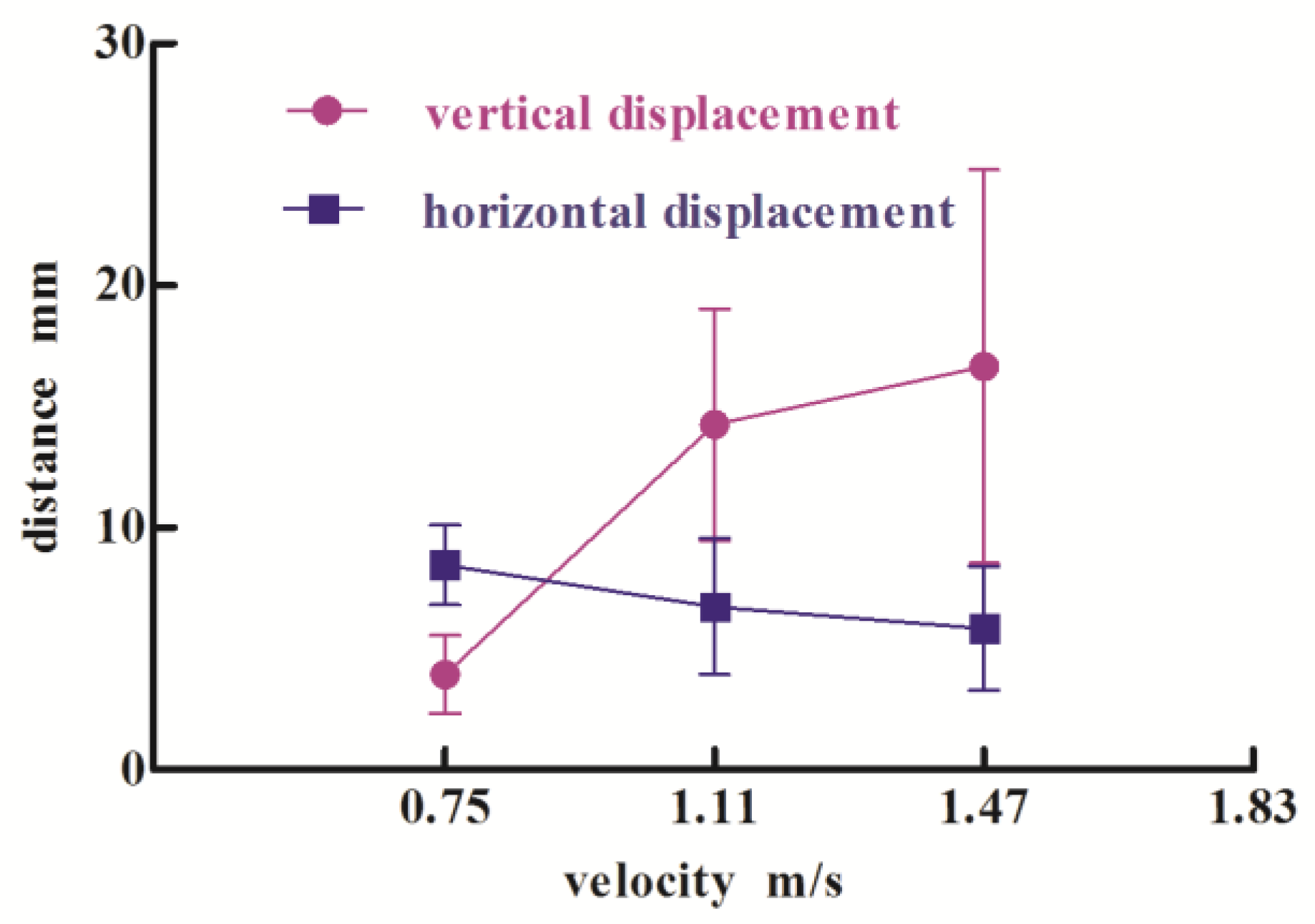
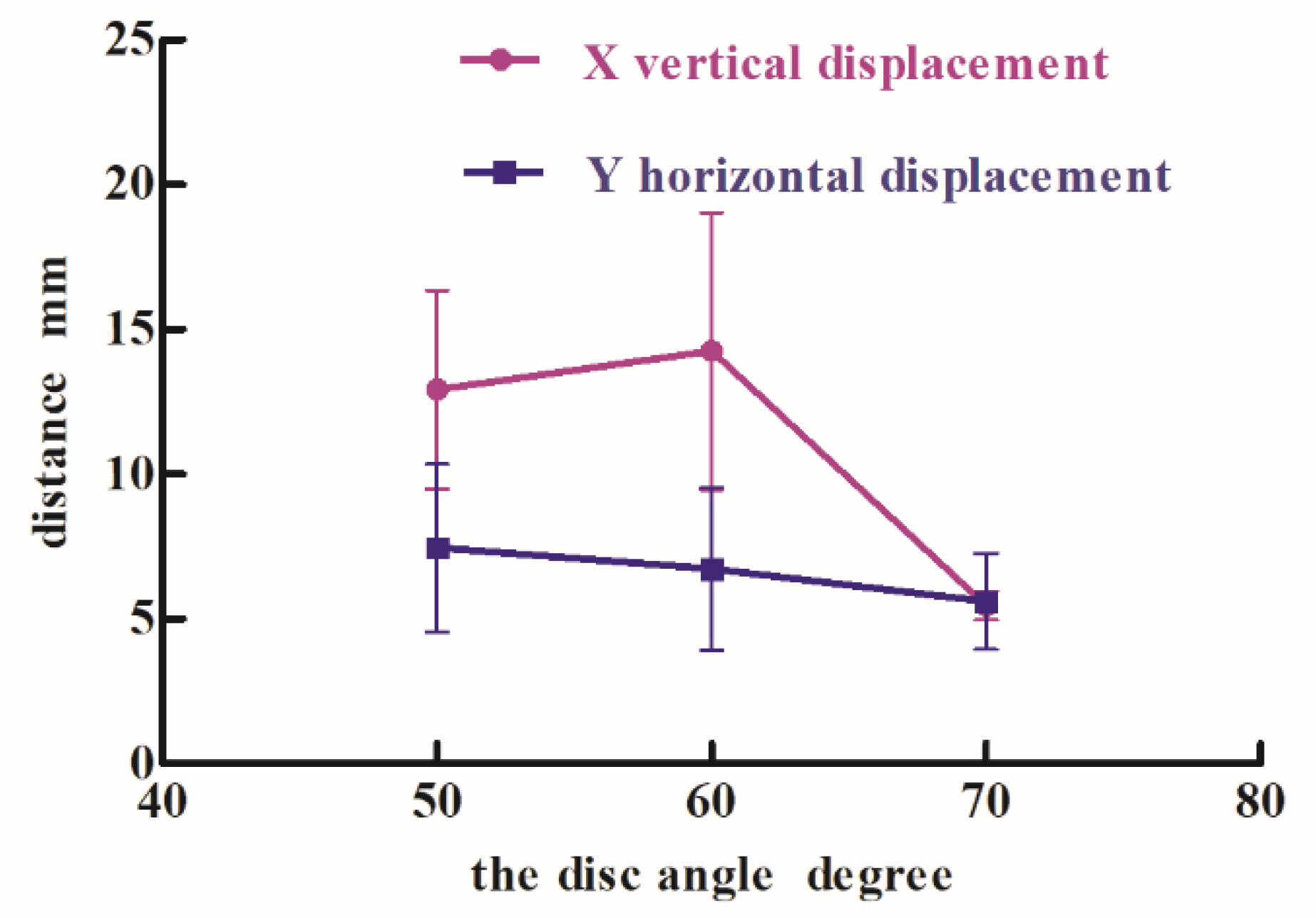
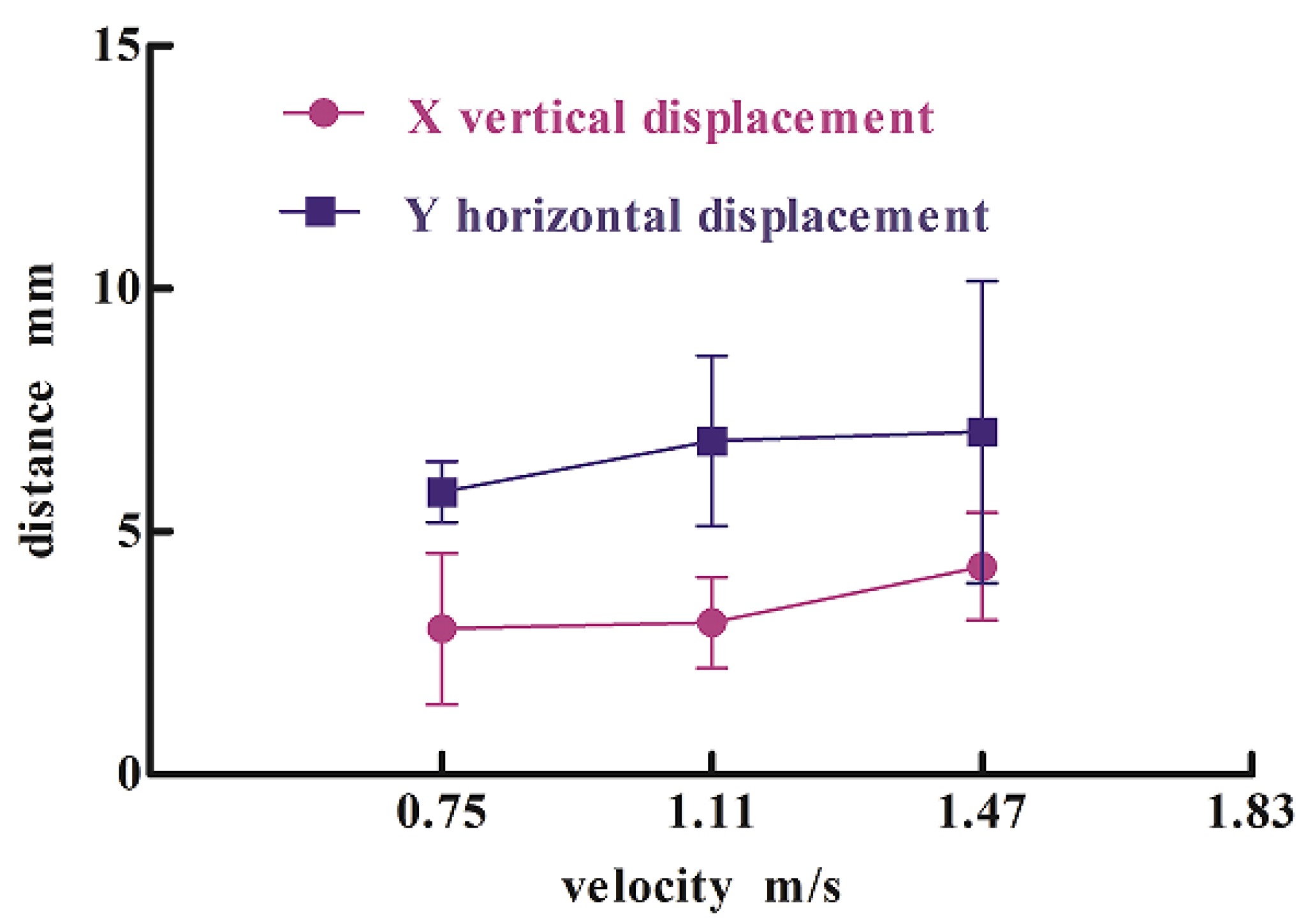
- With increasing forward velocity, the vertical displacement of seeds after soil covering increased, while the horizontal displacement decreased. The changes in the open angle of the soil covering discs had a significant effect on seed displacement. With increasing open angles of soil covering discs, the vertical and horizontal displacements of seeds decreased.
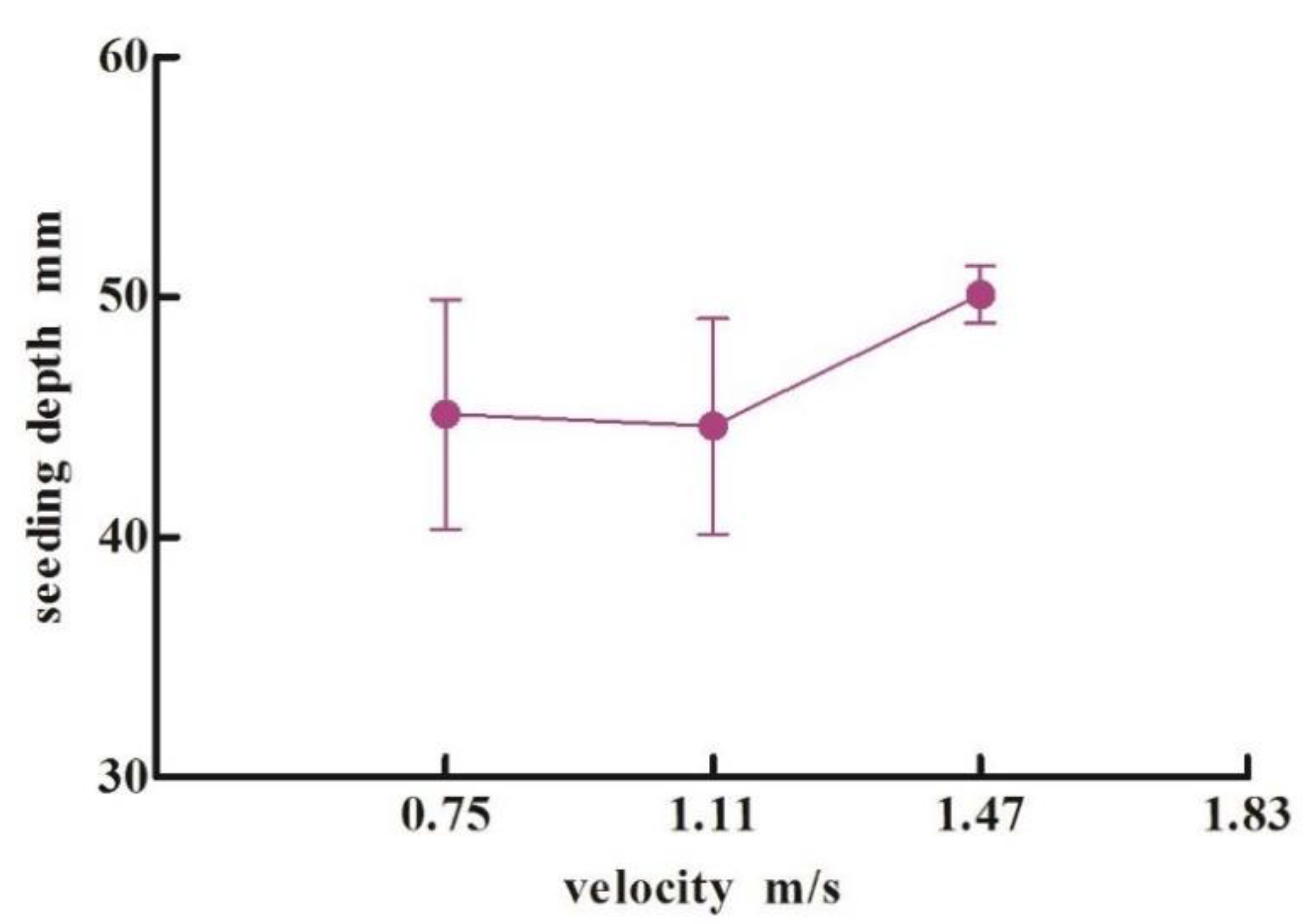
- With increasing forward velocity, the changes in the vertical and horizontal displacements of seeds after soil compacting appeared non-significant and similar to the seeding depths of the seeds. The seeding depths for the variety of forward velocities were always fluctuating in the range from 30 mm to 50 mm within the theoretical seeding depth.
- The soil covering and compacting working processing was simulated and analyzed by the coupling model of the DEM with MBDs. The comparison between the simulated results and the experimental results showed that the trend was similar and the two results were close. Thus, the feasibility and applicability of the coupling method were verified. It provided a new method for the design and optimization of covering and compacting components in precise seeding monomers.
- Further information about the paper is in the following: the other working apparatus (opener, seeding device, fertilizer drill, etc.) should be analyzed by experiments and simulations; a more complicated model of the precision seeding unit should be established by the coupling method of the DEM with MBDs. The organic matters, such as crop root residues, should be considered when soil modeling.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gao, X.; Zhou, Z.; Xu, Y.; Yu, Y.; Su, Y.; Cui, T. Numerical simulation of particle motion characteristics in quantitative seed feeding system. Powder Technol. 2020, 367, 643–658. [Google Scholar] [CrossRef]
- Yang, L.; Yan, B.; Zhang, D.; Zhang, T.; Zhang, Y.; Cui, T. Research progress on precision planting technology of maize. Trans. Chin. Soc. Agric. 2016, 11, 38–48. [Google Scholar]
- Sun, K.; Yu, J.; Liang, L.; Wang, Y.; Yan, D.; Zhou, L.; Yu, Y. A DEM-based general modelling method and experimental verification for wheat seeds. Powder Technol. 2022, 401, 117353. [Google Scholar] [CrossRef]
- Zhou, H.; Chen, Y.; Sadek, M.A. Modelling of soil–seed contact using the Discrete Element Method (DEM). Biosyst. Eng. 2014, 121, 56–66. [Google Scholar] [CrossRef]
- Bufton, L.P.; Richardson, P.; O’Dogherty, M.J. Seed displacement after impact on a soil surface. J. Agric. Eng. Res. 1974, 19, 327–338. [Google Scholar] [CrossRef]
- Abdolahzare, Z.; Mehdizadeh, S.A. Real time laboratory and field monitoring of the effect of the operational parameters on seed falling speed and trajectory of pneumatic planter. Comput. Electron. Agric. 2018, 145, 187–198. [Google Scholar] [CrossRef]
- Nielsen, S.K.; Munkholm, L.J.; Lamandé, M.; Nørremark, M.; Gareth, T.C.; Green, E.O. Seed drill depth control system for precision seeding. Comput. Electron. Agric. 2018, 144, 174–180. [Google Scholar] [CrossRef]
- Jia, H.; Wang, W.; Luo, X.; Zheng, J.; Guo, M.; Zhuang, J. Effects of profiling elastic press roller on seedbed properties and soybean emergence under double row ridge cultivation. Soil Tillage Res. 2016, 162, 34–40. [Google Scholar] [CrossRef]
- Gürsoy, S.; Türk, Z. Effects of land rolling on soil properties and plant growth in chickpea production. Soil Tillage Res. 2019, 195, 104425. [Google Scholar] [CrossRef]
- Tong, J.; Zhang, Q.; Guo, L.; Chang, Y.; Guo, Y.; Zhu, F.; Chen, D.; Liu, X. Compaction Performance of Biomimetic Press Roller to Soil. J. Bionic Eng. 2015, 12, 152–159. [Google Scholar] [CrossRef]
- Jingli, W. The Research of Displacement Control after Seed Contacting Soil in the Process of Soil Covering and Rolling with Precision Seeder; JinLin University: Changchun, China, 2012. [Google Scholar]
- Jin, T.; Qingzhu, Z.; Yuan, C.; Mo, L.; Leilei, Z.; Xin, L. Finite element analysis and experimental verification of bionic press roller in reducing adhesion and resistance. Trans. Chin. Soc. Agric. Mach. 2014, 7, 85–92. [Google Scholar]
- Hui, G. Study on Maize Sowing Quality Evaluation and Soil Covering-Compacting Device; JinLin University: Changchun, China, 2019. [Google Scholar]
- Sadeghi-Chahardeh, A.; Mollaabbasi, R.; Picard, D.; Taghavi, S.M.; Alamdari, H. Discrete Element Method Modeling for the Failure Analysis of Dry Mono-Size Coke Aggregates. Materials 2021, 14, 2174. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Ganwei, C.; Jian, Y. Dynamics simulation of direct shear test. Trans. Chin. Soc. Agric. Mach. 2011, 7, 96–101. [Google Scholar] [CrossRef]
- Xu, T.; Yu, J.; Yu, Y.; Wang, Y. A modelling and verification approach for soybean seed particles using the discrete element method. Adv. Powder Technol. 2018, 29, 3274–3290. [Google Scholar] [CrossRef]
- Horváth, D.; Poós, T.; Tamás, K. Modeling the movement of hulled millet in agitated drum dryer with discrete element method. Comput. Electron. Agric. 2019, 162, 254–268. [Google Scholar] [CrossRef]
- Chen, X.; Elliott, J.A. On the scaling law of JKR contact model for coarse-grained cohesive particles. Chem. Eng. Sci. 2020, 227, 115906. [Google Scholar] [CrossRef]
- Zhou, J.; Zhang, L.; Hu, C.; Li, Z.; Tang, J.; Mao, K.; Wang, X. Calibration of wet sand and gravel particles based on JKR contact model. Powder Technol. 2022, 397, 117005. [Google Scholar] [CrossRef]
- Feng, X.; Liu, T.; Wang, L.; Yu, Y.; Zhang, S.; Song, L. Investigation on JKR surface energy of high-humidity maize grains. Powder Technol. 2021, 382, 406–419. [Google Scholar] [CrossRef]
- Yaron, F.; Rubinstein, D.; Shmulevich, I. Determination of discrete element model parameters for soil-bulldozer blade interaction. In Proceedings of the 15th International Conference of the ISTVS, Hayama, Japan, 25–29 September 2005; pp. 25–29. [Google Scholar]
- Asaf, Z.; Shmulevich, I.; Rubinstein, D. Predicting soil-rigid wheel performance using distinct element methods. Trans. ASABE 2006, 49, 607–616. [Google Scholar] [CrossRef]
- Tamás, K.; Bernon, L. Role of particle shape and plant roots in the discrete element model of soil–sweep interaction. Biosyst. Eng. 2021, 211, 77–96. [Google Scholar] [CrossRef]
- Sadeghi-Chahardeh, A.; Mollaabbasi, R.; Picard, D.; Taghavi, S.M.; Alamdari, H. Effect of Particle Size Distributions and Shapes on the Failure Behavior of Dry Coke Aggregates. Material 2021, 14, 5558. [Google Scholar] [CrossRef] [PubMed]
- Ouadfel, H.; Rothenburg, L. An algorithm for detecting inter-ellipsoid contacts. Comput. Geotech. 1999, 24, 245–263. [Google Scholar] [CrossRef]
- Cleary, P.W.; Stokes, N. Efficient Collision Detection for Three Dimensional Super-ellipsoidal Particles. In Proceedings of the 8th International Computational Techniques and Applications Conference: CTAC97, Singapore, 29 September–1 October 1997. [Google Scholar]
- Cundall, P.A. Formulation of a three-dimensional distinct element model—Part I. A scheme to detect and represent contacts in a system composed of many polyhedral blocks. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1988, 25, 107–116. [Google Scholar] [CrossRef]
- Govender, N.; Wilke, D.N.; Kok, S.; Els, R. Development of a convex polyhedral discrete element simulation framework for NVIDIA Kepler based GPUs. J. Comput. Appl. Math. 2014, 270, 386–400. [Google Scholar] [CrossRef]
- Kruggel-Emden, H.; Rickelt, S.; Wirtz, S.; Scherer, V. A study on the validity of the multi-sphere discrete element method. Powder Technol. 2008, 188, 153–165. [Google Scholar] [CrossRef]






| Granular Diameter, mm | Sample 1, g | Sample 2, g | Sample 3, g | Mean, g | Proportion, % |
|---|---|---|---|---|---|
| 0.05–0.1 | 4.72 | 4.45 | 4.02 | 4.40 | 2.20 |
| 0.1–0.2 | 29.90 | 29.25 | 27.69 | 28.95 | 14.48 |
| 0.2–0.3 | 11.15 | 12.22 | 11.05 | 11.47 | 5.74 |
| 0.3–0.45 | 40.30 | 42.37 | 40.80 | 41.16 | 20.58 |
| 0.45–1 | 49.43 | 51.82 | 51.34 | 50.86 | 25.43 |
| 1–2 | 28.52 | 31.44 | 38.38 | 32.78 | 16.39 |
| ≥2 | 11.54 | 7.95 | 8.39 | 9.29 | 4.65 |
| No. | Connector Object | Type of Connectors |
|---|---|---|
| Joint_1 | Front beam–ground | Translation |
| Joint_2 | Front beam–beam | Revolute |
| Joint_3 | Beam–covering connecting part | Revolute |
| Joint_4 | Covering connecting part–çovering disc 1/covering disc 2 | Revolute |
| Joint_5 | Beam–roller | Revolute |
| Parameters | Symbol | Soil | Steel | Soybean Seed |
|---|---|---|---|---|
| Density (kg/m3) | ρ | 1950 | 7850 | 1211 |
| Poisson’s ratio | v | 0.25 | 0.3 | 0.4 |
| Shear modulus (Pa) | G | 2.73 × 106 | 7.92 × 1010 | 1.28 × 108 |
| Restitution coefficient | e | 0.3 | 0.5 | 0.57 |
| Static friction coefficient | μ | 0.5 | 0.1 | 0.2 |
| Rolling friction coefficient | μr | 0.03 | 0.02 | 0.01 |
| No. | x | y | z | No. | x | y | z |
|---|---|---|---|---|---|---|---|
| 1 | 1200.000 | −20.000 | 125.000 | 5 | 2400.000 | −20.000 | 125.000 |
| 2 | 1500.000 | −20.000 | 125.000 | 6 | 2700.000 | −20.000 | 125.000 |
| 3 | 1800.000 | −20.000 | 125.000 | 7 | 3000.000 | −20.000 | 125.000 |
| 4 | 2100.000 | −20.000 | 125.000 |
| No. | x | y | z | No. | x | y | z |
|---|---|---|---|---|---|---|---|
| 1 | 1174.600 | −58.115 | 129.000 | 5 | 2396.789 | −57.730 | 120.190 |
| 2 | 1491.774 | −67.713 | 138.900 | 6 | 2683.555 | −59.8447 | 117.000 |
| 3 | 1798.447 | −57.730 | 119.900 | 7 | 2988.300 | −73.200 | 123.050 |
| 4 | 2097.109 | −69.130 | 133.290 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, T.; Zhang, R.; Wang, Y.; Jiang, X.; Feng, W.; Wang, J. Simulation and Analysis of the Working Process of Soil Covering and Compacting of Precision Seeding Units Based on the Coupling Model of DEM with MBD. Processes 2022, 10, 1103. https://doi.org/10.3390/pr10061103
Xu T, Zhang R, Wang Y, Jiang X, Feng W, Wang J. Simulation and Analysis of the Working Process of Soil Covering and Compacting of Precision Seeding Units Based on the Coupling Model of DEM with MBD. Processes. 2022; 10(6):1103. https://doi.org/10.3390/pr10061103
Chicago/Turabian StyleXu, Tianyue, Ruxin Zhang, Yang Wang, Xinming Jiang, Weizhi Feng, and Jingli Wang. 2022. "Simulation and Analysis of the Working Process of Soil Covering and Compacting of Precision Seeding Units Based on the Coupling Model of DEM with MBD" Processes 10, no. 6: 1103. https://doi.org/10.3390/pr10061103
APA StyleXu, T., Zhang, R., Wang, Y., Jiang, X., Feng, W., & Wang, J. (2022). Simulation and Analysis of the Working Process of Soil Covering and Compacting of Precision Seeding Units Based on the Coupling Model of DEM with MBD. Processes, 10(6), 1103. https://doi.org/10.3390/pr10061103








