Dusty Nanoliquid Flow through a Stretching Cylinder in a Porous Medium with the Influence of the Melting Effect
Abstract
:1. Introduction
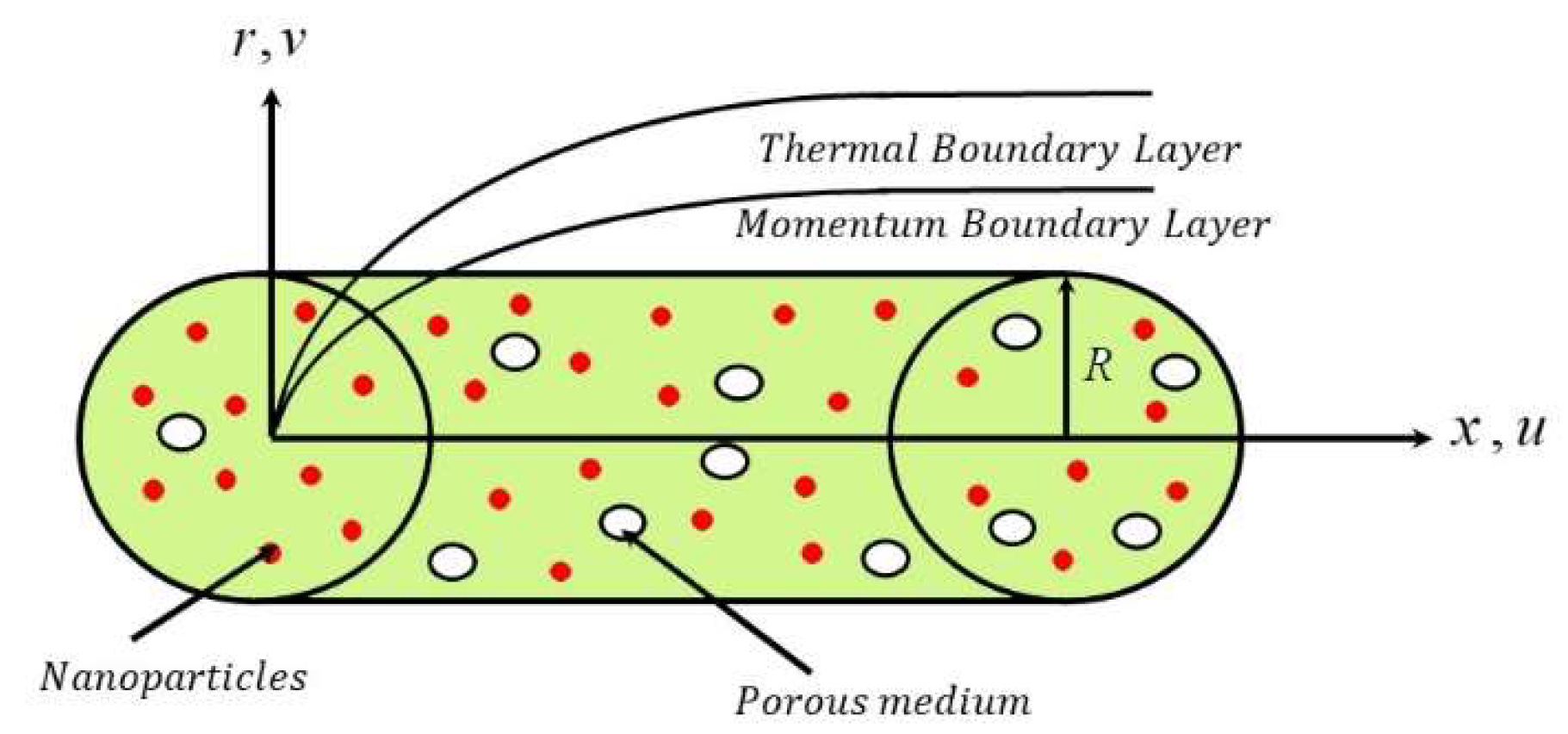
2. Mathematical Modeling
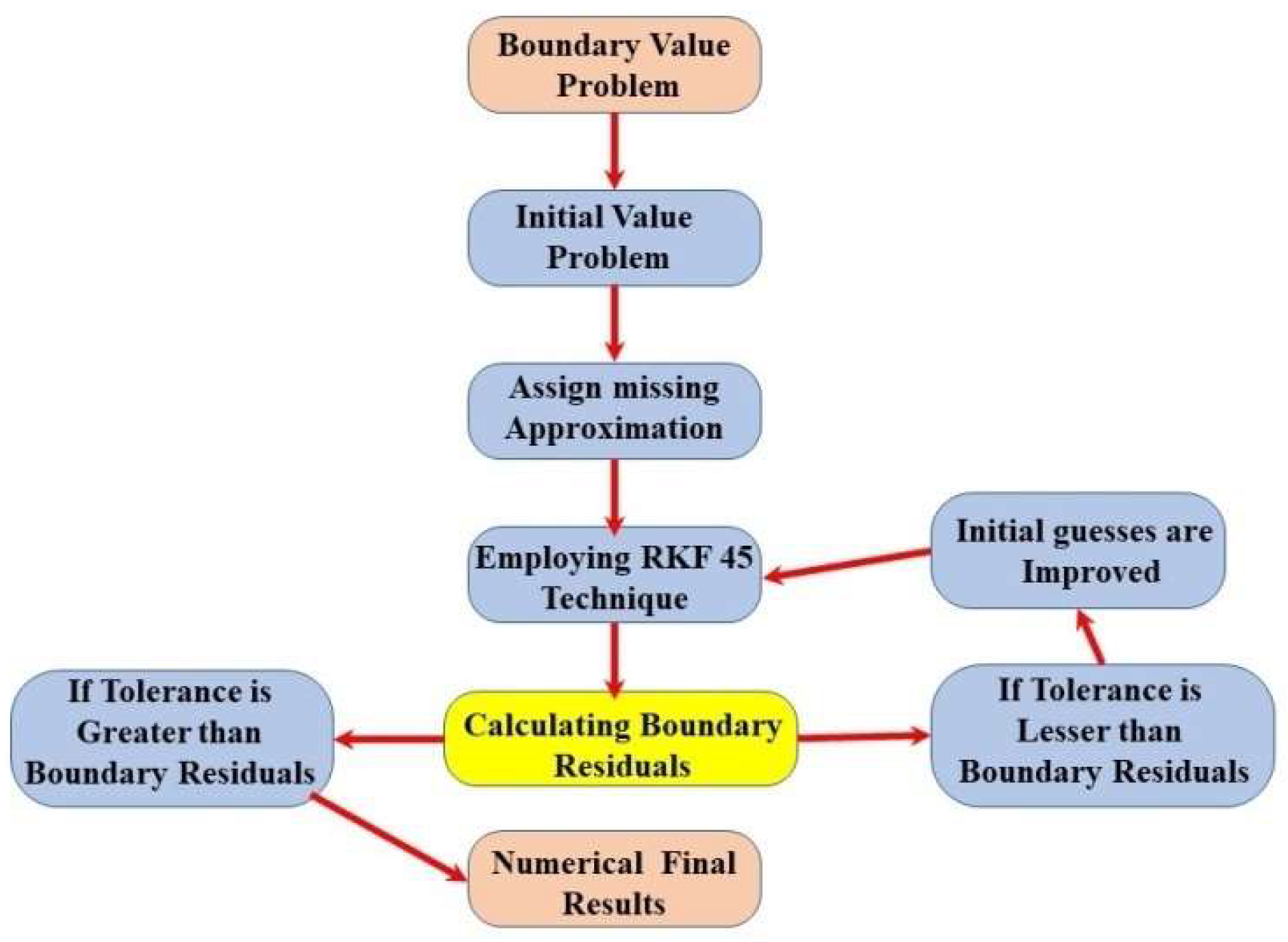
3. Numerical Procedure and Validation
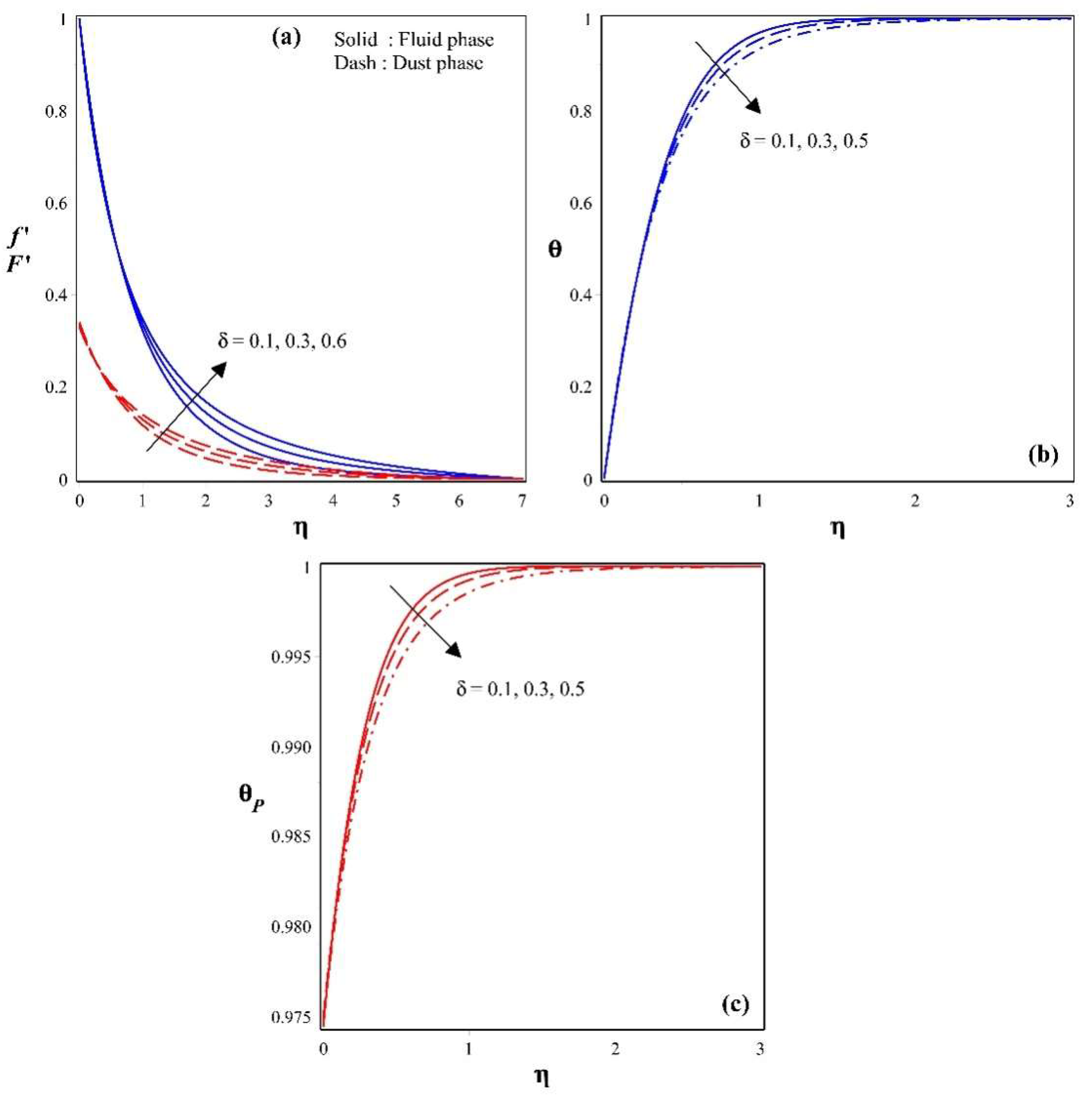
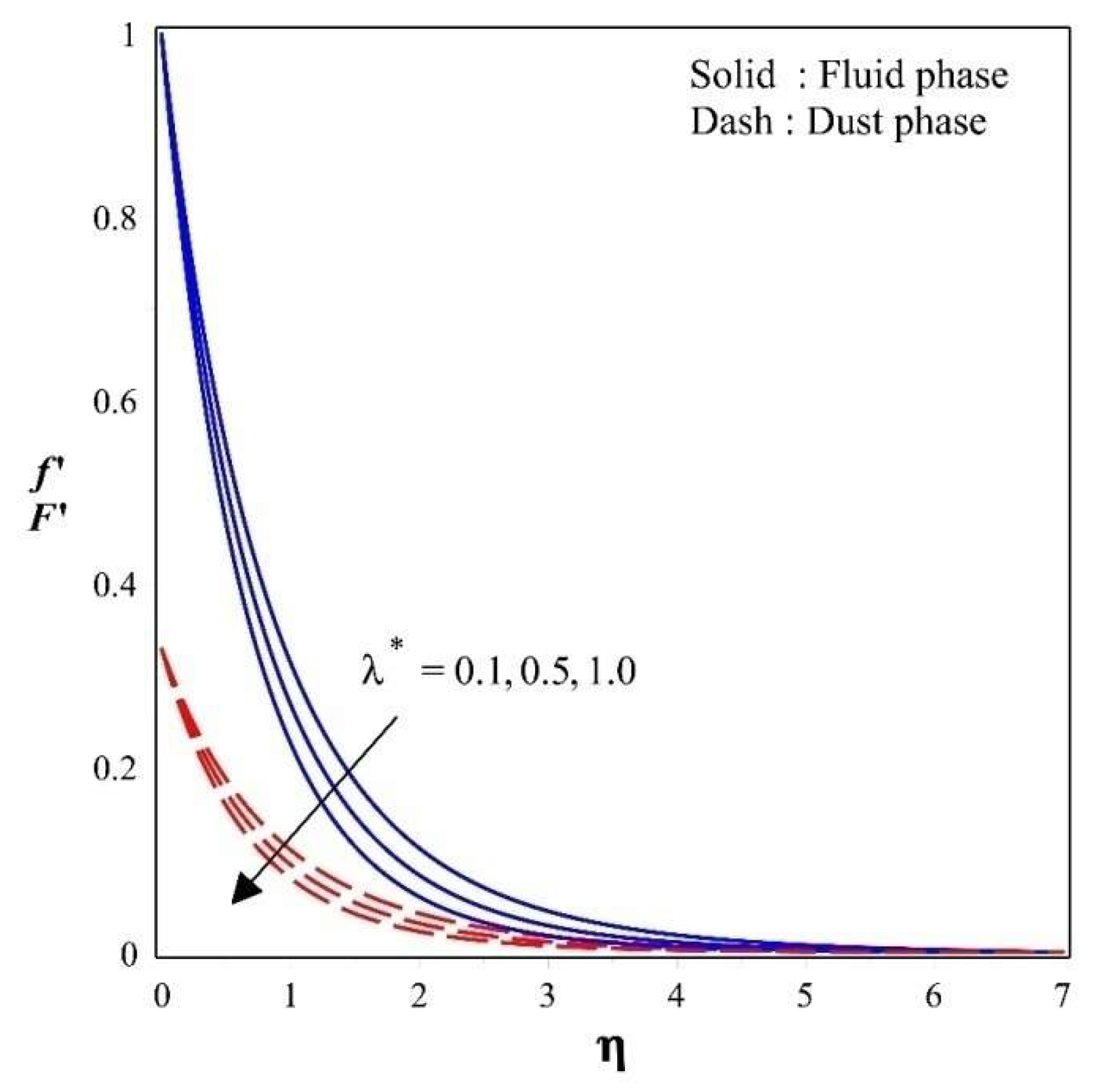
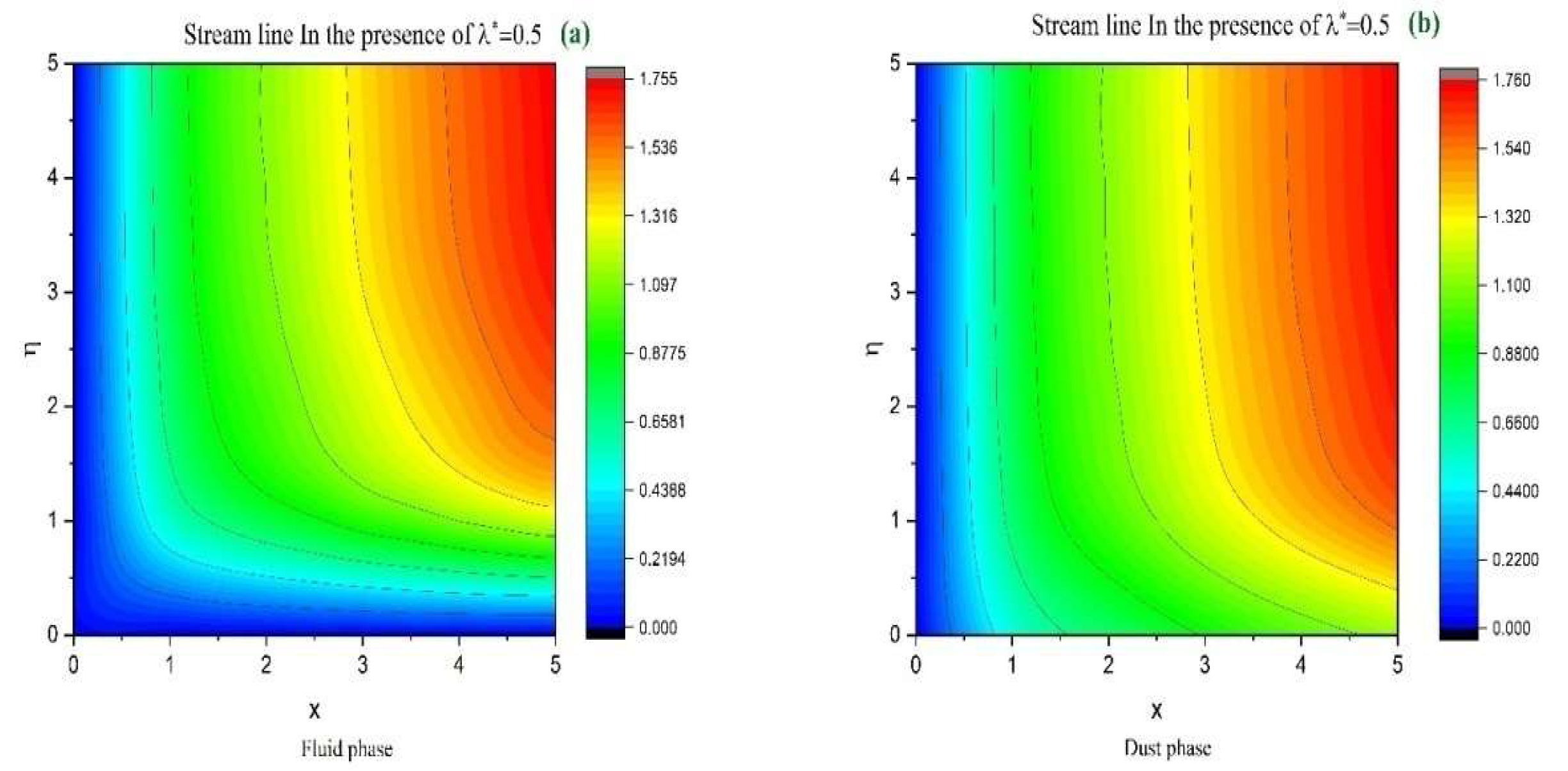
4. Results and Discussion
5. Final Remarks
- ❖ The fluid- and dust-phase velocities showed decreasing trends with an increase in the porosity parameter.
- ❖ The fluid- and dust-phase velocities improved with an increase in the curvature parameter. The same trend was observed in the fluid- and dust-phase temperature profiles.
- ❖ For lower values of the melting parameter, the temperature field for both the dust and liquid phases was enhanced significantly.
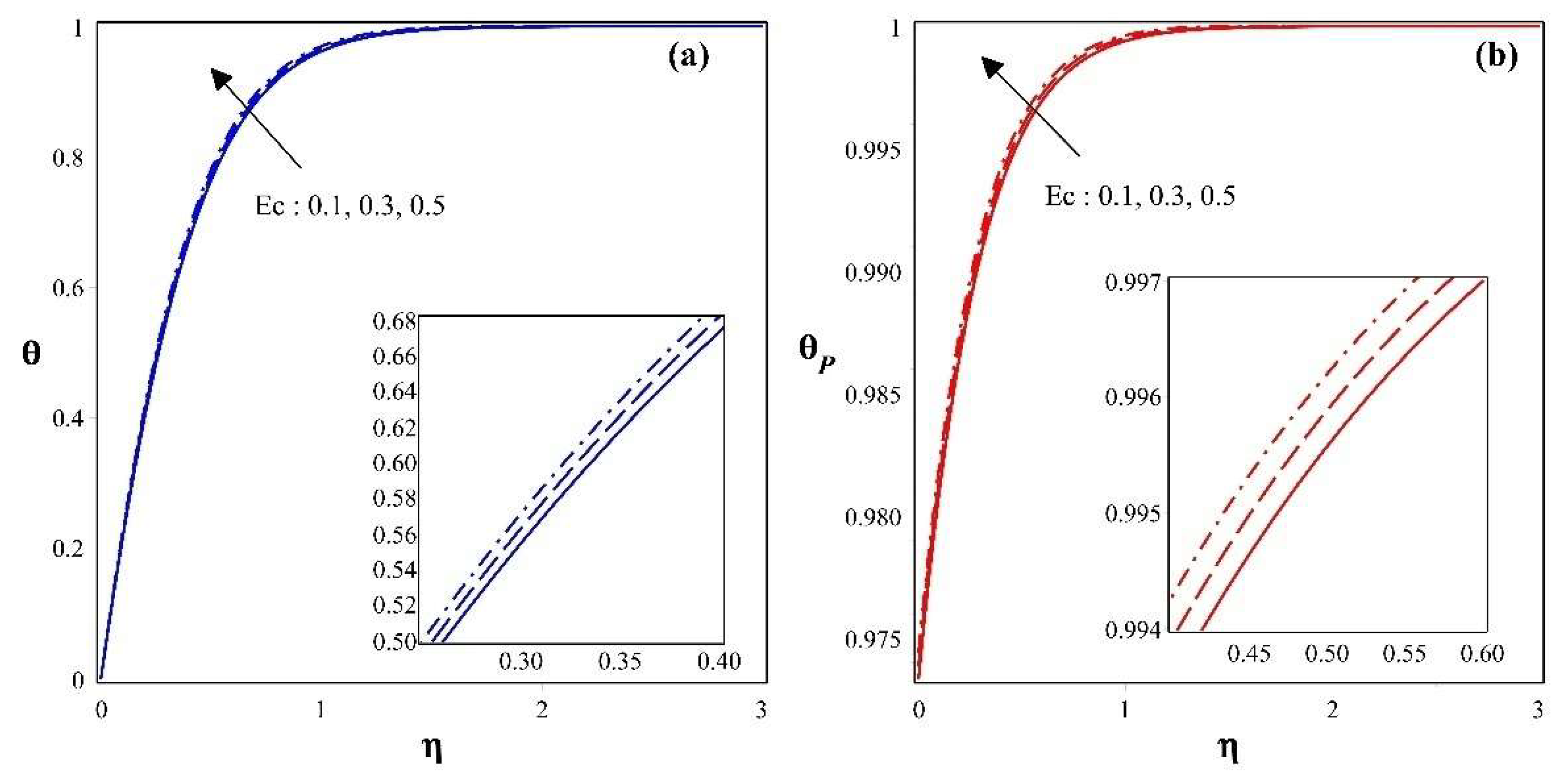
- ❖ The temperature profile for both the fluid and dust phases improves as the Eckert number increases.
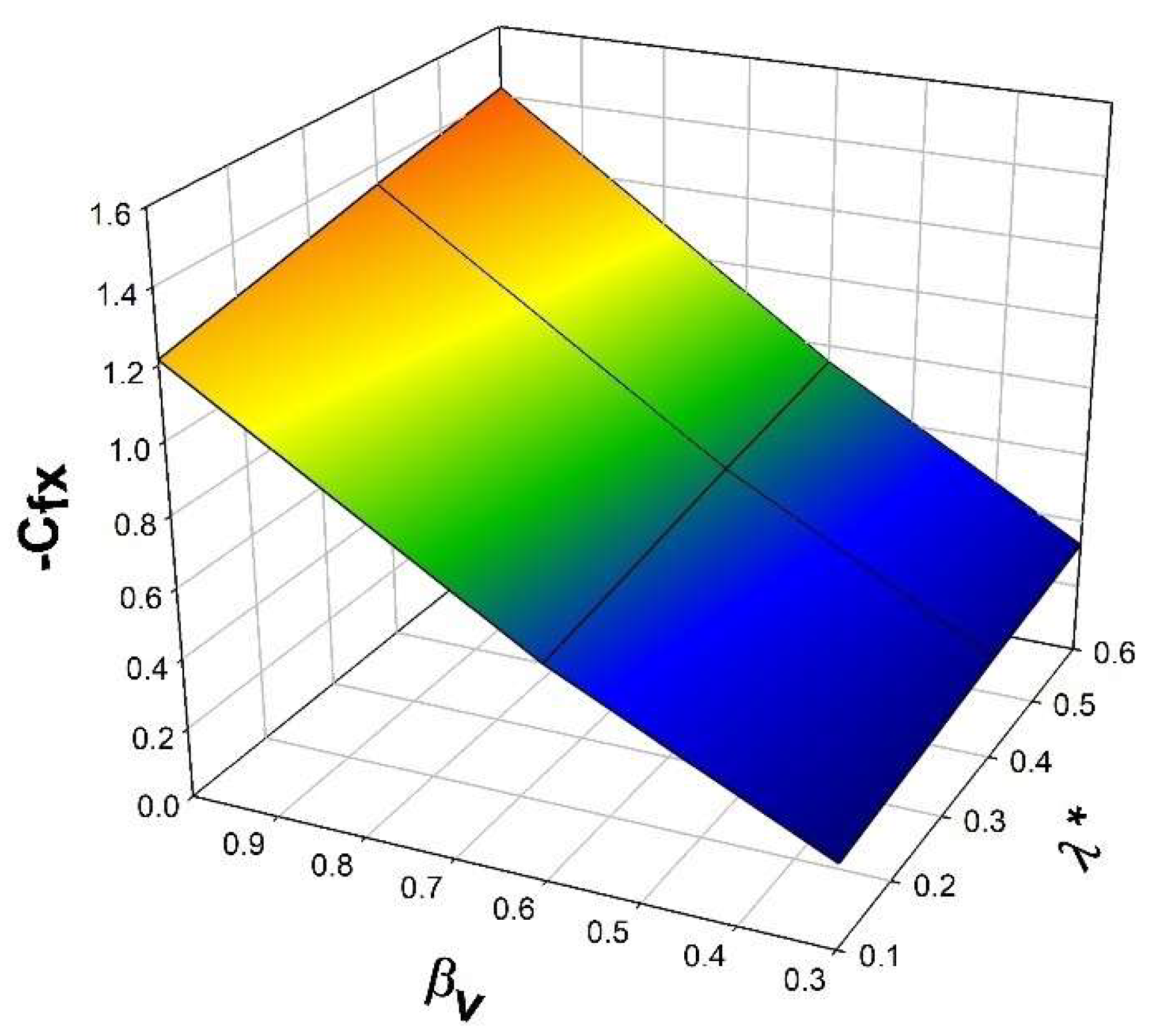
- ❖ The surface drag force reduces when the porosity and interaction parameters are increased.
- ❖ The rate of heat dispersal increases as the values of the interaction parameters increase.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Cartesian coordinate (m) | |
| Coordinate in the radial direction (m) | |
| Reference length (m) | |
| Radius of the cylinder (m) | |
| Uniform stretching velocity (ms−1) | |
| Stretching velocity (ms−1) | |
| & | Fluid-phase velocity components (ms−1) |
| & | Dust-phase velocity components (ms−1) |
| Temperature (K) | |
| Stokes resistance | |
| The number density of particles | |
| Kinematic viscosity (m2s−1) | |
| Porous medium permeability (m2) | |
| Thermal conductivity (kgms−3K−1) | |
| Density (kgm−3) | |
| The density of the dust particle (kgm−3) | |
| Specific heat capacity of fluid (m−2s−2K−1) | |
| Specific heat capacity of the solid surface (m−2s−2K−1) | |
| Latent heat of the fluid | |
| The temperature of the dust particle (K) | |
| Wall temperature (K) | |
| Melting temperature (K) | |
| Ambient temperature (K) | |
| Thermal equilibrium time | |
| The relaxation time of dust particles | |
| Mass of the dust particle (kg) | |
| Dynamic viscosity (kgm−1s−1) | |
| Solid volume fraction (-) | |
| Nanofluid | |
| Eckert number (-) | |
| Ratio of specific heat (-) | |
| Melting parameter (-) | |
| Pr | Prandtl number |
| Curvature parameter (-) | |
| Skin friction coefficient | |
| Nusselt number coefficient | |
| Local Reynolds number | |
| Dimensionless velocities of fluid phase | |
| Dimensionless velocities of dust phase | |
| Dimensionless temperature | |
| Dimensionless temperature for the dust particle | |
| Interaction parameter for velocity | |
| Interaction parameter for temperature |
References
- Abbas, S.Z.; Khan, M.I.; Kadry, S.; Khan, W.A.; Israr-Ur-Rehman, M.; Waqas, M. Fully developed entropy optimized second order velocity slip MHD nanofluid flow with activation energy. Comput. Methods Programs Biomed. 2020, 190, 105362. [Google Scholar] [CrossRef]
- Madhukesh, J.K.; Ramesh, G.K.; Aly, E.H.; Chamkha, A.J. Dynamics of water conveying SWCNT nanoparticles and swimming microorganisms over a Riga plate subject to heat source/sink. Alex. Eng. J. 2022, 61, 2418–2429. [Google Scholar] [CrossRef]
- Muhammad, T.; Waqas, H.; Manzoor, U.; Farooq, U.; Rizvi, Z.F. On doubly stratified bioconvective transport of Jeffrey nanofluid with gyrotactic motile microorganisms. Alex. Eng. J. 2021, 61, 1571–1583. [Google Scholar] [CrossRef]
- Ramesh, G.K.; Madhukesh, J.K.; Prasannakumara, B.C.; Shehzad, S.A.; Abbasi, F.M. Thermodynamics Examination of Fe3O4-CoFe2O4/Water + EG Nanofluid in a Heated Plate: Crosswise and Stream-wise Aspects. Arab. J. Sci. Eng. 2021, 1–10. [Google Scholar] [CrossRef]
- Xu, Y.-J.; Bilal, M.; Al-Mdallal, Q.; Khan, M.A.; Muhammad, T. Gyrotactic micro-organism flow of Maxwell nanofluid between two parallel plates. Sci. Rep. 2021, 11, 15142. [Google Scholar] [CrossRef]
- Hussain, S.A.; Ali, G.; Muhammad, S.; Shah, S.I.A.; Ishaq, M.; Khan, H. Dusty Casson Nanofluid Flow with Thermal Radiation Over a Permeable Exponentially Stretching Surface. J. Nanofluids 2019, 8, 714–724. [Google Scholar] [CrossRef]
- Rashed, Z.Z.; Ahmed, S.E. Unsteady three dimensional radiative-convective flow and heat transfer of dusty nanofluid within porous cubic enclosures. J. Dispers. Sci. Technol. 2021, 0, 1–15. [Google Scholar] [CrossRef]
- Ramzan, M.; Gul, H.; Baleanu, D.; Nisar, K.S.; Malik, M.Y. Role of Cattaneo–Christov heat flux in an MHD Micropolar dusty nanofluid flow with zero mass flux condition. Sci. Rep. 2021, 11, 19528. [Google Scholar] [CrossRef]
- Wahidunnisa, L.; Suneetha, S.; Reddy, S.; Reddy, P.B.A. Comparative study on electromagnetohydrodynamic single-wall carbon nanotube–water dusty nanofluid in the presence of radiation and Ohmic heating. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2021, 235, 950–958. [Google Scholar] [CrossRef]
- Sowmya, G.; Saleh, B.; Punith Gowda, R.J.; Naveen Kumar, R.; Varun Kumar, R.S.; Radhika, M. Analysis of radiative nonlinear heat transfer in a convective flow of dusty fluid by capitalizing a non-Fourier heat flux model. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2021, 09544089211041192. [Google Scholar] [CrossRef]
- Wahid, N.S.; Arifin, N.M.; Khashi’ie, N.S.; Pop, I. Marangoni hybrid nanofluid flow over a permeable infinite disk embedded in a porous medium. Int. Commun. Heat Mass Transf. 2021, 126, 105421. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F. Free convection and radiation effects in nanofluid (Silicon dioxide and Molybdenum disulfide) with second order velocity slip, entropy generation, Darcy-Forchheimer porous medium. Int. J. Hydrogen Energy 2021, 46, 1362–1369. [Google Scholar] [CrossRef]
- Ramesh, G.K.; Madhukesh, J.K.; Shehzad, S.A.; Rauf, A. Ternary nanofluid with heat source/sink and porous medium effects in stretchable convergent/divergent channel. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2022, 09544089221081344. [Google Scholar] [CrossRef]
- Ahmad, S.; Ali, K.; Rizwan, M.; Ashraf, M. Heat and mass transfer attributes of copper–aluminum oxide hybrid nanoparticles flow through a porous medium. Case Stud. Therm. Eng. 2021, 25, 100932. [Google Scholar] [CrossRef]
- Ali, K.; Ahmad, S.; Nisar, K.S.; Faridi, A.A.; Ashraf, M. Simulation analysis of MHD hybrid Cu-Al2O3/H2O nanofluid flow with heat generation through a porous media. Int. J. Energy Res. 2021, 45, 19165–19179. [Google Scholar] [CrossRef]
- Mabood, F.; Yusuf, T.A.; Khan, W.A. Cu–Al2O3–H2O hybrid nanofluid flow with melting heat transfer, irreversibility analysis and nonlinear thermal radiation. J. Therm. Anal. Calorim. 2021, 143, 973–984. [Google Scholar] [CrossRef]
- Waqas, H.; Muhammad, T.; Noreen, S.; Farooq, U.; Alghamdi, M. Cattaneo-Christov heat flux and entropy generation on hybrid nanofluid flow in a nozzle of rocket engine with melting heat transfer. Case Stud. Therm. Eng. 2021, 28, 101504. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, K.; Alsaedi, A. Numerical study of melting heat transfer in stagnation-point flow of hybrid nanomaterial (MWCNTs+Ag+kerosene oil). Int. J. Numer. Methods Heat Fluid Flow 2021, 31, 2580–2598. [Google Scholar] [CrossRef]
- Mallikarjuna, H.B.; Nirmala, T.; Punith Gowda, R.J.; Manghat, R.; Varun Kumar, R.S. Two-dimensional Darcy–Forchheimer flow of a dusty hybrid nanofluid over a stretching sheet with viscous dissipation. Heat Transf. 2021, 50, 3934–3947. [Google Scholar] [CrossRef]
- Khan, S.A.; Khan, M.I.; Alzahrani, F. Melting heat transportation in chemical reactive flow of third grade nanofluid with irreversibility analysis. Int. Commun. Heat Mass Transf. 2021, 129, 105696. [Google Scholar] [CrossRef]
- Abbas, N.; Nadeem, S.; Saleem, A.; Malik, M.Y.; Issakhov, A.; Alharbi, F.M. Models base study of inclined MHD of hybrid nanofluid flow over nonlinear stretching cylinder. Chin. J. Phys. 2021, 69, 109–117. [Google Scholar] [CrossRef]
- Waqas, H.; Manzoor, U.; Muhammad, T.; Hussain, S. Thermo-bioconvection transport of nanofluid over an inclined stretching cylinder with Cattaneo–Christov double-diffusion. Commun. Theor. Phys. 2021, 73, 075006. [Google Scholar] [CrossRef]
- Varun Kumar, R.S.; Punith Gowda, R.J.; Naveen Kumar, R.; Radhika, M.; Prasannakumara, B.C. Two-phase flow of dusty fluid with suspended hybrid nanoparticles over a stretching cylinder with modified Fourier heat flux. SN Appl. Sci. 2021, 3, 384. [Google Scholar] [CrossRef]
- Rashid, U.; Liang, H.; Ahmad, H.; Abbas, M.; Iqbal, A.; Hamed, Y.S. Study of (Ag and TiO2)/water nanoparticles shape effect on heat transfer and hybrid nanofluid flow toward stretching shrinking horizontal cylinder. Results Phys. 2021, 21, 103812. [Google Scholar] [CrossRef]
- Madhukesh, J.K.; Ramesh, G.K.; Roopa, G.S.; Prasannakumara, B.C.; Shah, N.A.; Yook, S.-J. 3D Flow of Hybrid Nanomaterial through a Circular Cylinder: Saddle and Nodal Point Aspects. Mathematics 2022, 10, 1185. [Google Scholar] [CrossRef]
- Manjunatha, P.T.; Gireesha, B.J.; Prasannakumara, B.C. Effect of Radiation on Flow and Heat Transfer of MHD Dusty Fluid Over a Stretching Cylinder Embedded in a Porous Medium in Presence of Heat Source. Int. J. Appl. Comput. Math. 2017, 3, 293–310. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Unsteady flow and heat transfer past a stretching/shrinking sheet in a hybrid nanofluid. Int. J. Heat Mass Transf. 2019, 136, 288–297. [Google Scholar] [CrossRef]
- Singh, K.; Pandey, A.K.; Kumar, M. Melting heat transfer assessment on magnetic nanofluid flow past a porous stretching cylinder. J. Egypt. Math. Soc. 2021, 29, 1. [Google Scholar] [CrossRef]
- Devi, S.P.A.; Devi, S.S.U. Numerical Investigation of Hydromagnetic Hybrid Cu – Al2O3/Water Nanofluid Flow over a Permeable Stretching Sheet with Suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Ramzan, M.; Shaheen, N.; Chung, J.D.; Kadry, S.; Chu, Y.-M.; Howari, F. Impact of Newtonian heating and Fourier and Fick’s laws on a magnetohydrodynamic dusty Casson nanofluid flow with variable heat source/sink over a stretching cylinder. Sci. Rep. 2021, 11, 2357. [Google Scholar] [CrossRef]
- Chu, Y.-M.; Nisar, K.S.; Khan, U.; Kasmaei, H.D.; Malaver, M.; Zaib, A.; Khan, I. Mixed Convection in MHD Water-Based Molybdenum Disulfide-Graphene Oxide Hybrid Nanofluid through an Upright Cylinder with Shape Factor. Water 2020, 12, 1723. [Google Scholar] [CrossRef]
- Gupta, P.S.; Gupta, A.S. Heat and mass transfer on a stretching sheet with suction or blowing. Can. J. Chem. Eng. 1977, 55, 744–746. [Google Scholar] [CrossRef]
- Ali, M.E. On thermal boundary layer on a power-law stretched surface with suction or injection. Int. J. Heat Fluid Flow 1995, 16, 280–290. [Google Scholar] [CrossRef]
- Grubka, L.J.; Bobba, K.M. Heat Transfer Characteristics of a Continuous, Stretching Surface with Variable Temperature. J. Heat Transf. 1985, 107, 248–250. [Google Scholar] [CrossRef]
- Abo-Eldahab, E.M.; El Aziz, M.A. Blowing/suction effect on hydromagnetic heat transfer by mixed convection from an inclined continuously stretching surface with internal heat generation/absorption. Int. J. Thermal Sci. 2004, 43, 709–719. [Google Scholar] [CrossRef]
- Abel, M.S.; Mahesha, N. Heat transfer in MHD viscoelastic fluid flow over a stretching sheet with variable thermal conductivity, non-uniform heat source and radiation. Appl. Math. Model. 2008, 32, 1965–1983. [Google Scholar] [CrossRef]



| Property | Pr | |||
|---|---|---|---|---|
| H2O | 997.1 | 4179 | 0.613 | 6.2 |
| GO | 1800 | 717 | 5000 | - |
| Pr | [32] | [33] | [34] | [35] | [36] | Present |
|---|---|---|---|---|---|---|
| 1.0 | 0.5820 | 0.59988 | 0.5820 | 0.58201 | 0.58197 | 0.582010 |
| 10 | - | 2.29589 | 2.3080 | 2.30801 | 2.30800 | 2.308001 |
| 0.1 | 0.5 | 0.5 | 0.5 | 1.168327 | 1.258053 | 2.115255 | 2.720979 |
| 0.2 | 1.211577 | 1.301255 | 2.107476 | 2.712020 | |||
| 0.3 | 1.253367 | 1.342980 | 2.100069 | 2.703531 | |||
| 0.1 | 1.071936 | 1.150952 | 2.067828 | 2.637433 | |||
| 0.3 | 1.129654 | 1.214091 | 2.105213 | 2.697497 | |||
| 0.5 | 1.168327 | 1.258053 | 2.115255 | 2.720979 | |||
| 0.1 | 1.187103 | 1.258053 | 1.663600 | 2.177235 | |||
| 0.3 | 1.177038 | 1.258053 | 1.905106 | 2.466492 | |||
| 0.5 | 1.168327 | 1.258053 | 2.115255 | 2.720979 | |||
| 0.5 | 1.168327 | 1.258053 | 2.115255 | 2.720979 | |||
| 1.0 | 1.163875 | 1.258053 | 2.223107 | 2.885525 | |||
| 1.5 | 1.159516 | 1.258053 | 2.328974 | 3.050072 | |||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Umeshaiah, M.; Madhukesh, J.; Khan, U.; Rana, S.; Zaib, A.; Raizah, Z.; Galal, A.M. Dusty Nanoliquid Flow through a Stretching Cylinder in a Porous Medium with the Influence of the Melting Effect. Processes 2022, 10, 1065. https://doi.org/10.3390/pr10061065
Umeshaiah M, Madhukesh J, Khan U, Rana S, Zaib A, Raizah Z, Galal AM. Dusty Nanoliquid Flow through a Stretching Cylinder in a Porous Medium with the Influence of the Melting Effect. Processes. 2022; 10(6):1065. https://doi.org/10.3390/pr10061065
Chicago/Turabian StyleUmeshaiah, Mahadevaiah, JavaliK Madhukesh, Umair Khan, Saurabh Rana, Aurang Zaib, Zehba Raizah, and Ahmed M. Galal. 2022. "Dusty Nanoliquid Flow through a Stretching Cylinder in a Porous Medium with the Influence of the Melting Effect" Processes 10, no. 6: 1065. https://doi.org/10.3390/pr10061065
APA StyleUmeshaiah, M., Madhukesh, J., Khan, U., Rana, S., Zaib, A., Raizah, Z., & Galal, A. M. (2022). Dusty Nanoliquid Flow through a Stretching Cylinder in a Porous Medium with the Influence of the Melting Effect. Processes, 10(6), 1065. https://doi.org/10.3390/pr10061065







