Log-Normal or Over-Dispersed Poisson?
Abstract
1. Introduction
2. Empirical Illustration of the Problem
3. Overview of the Rival Models
3.1. Data
3.2. Identification
3.3. Over-Dispersed Poisson Model
3.3.1. Assumptions
3.3.2. Estimation
3.3.3. Sampling Scheme
3.3.4. Asymptotic Theory
3.4. Generalized Log-Normal Model
3.4.1. Assumptions
3.4.2. Estimation
3.4.3. Sampling Scheme
3.4.4. Asymptotic Theory
4. Encompassing Tests
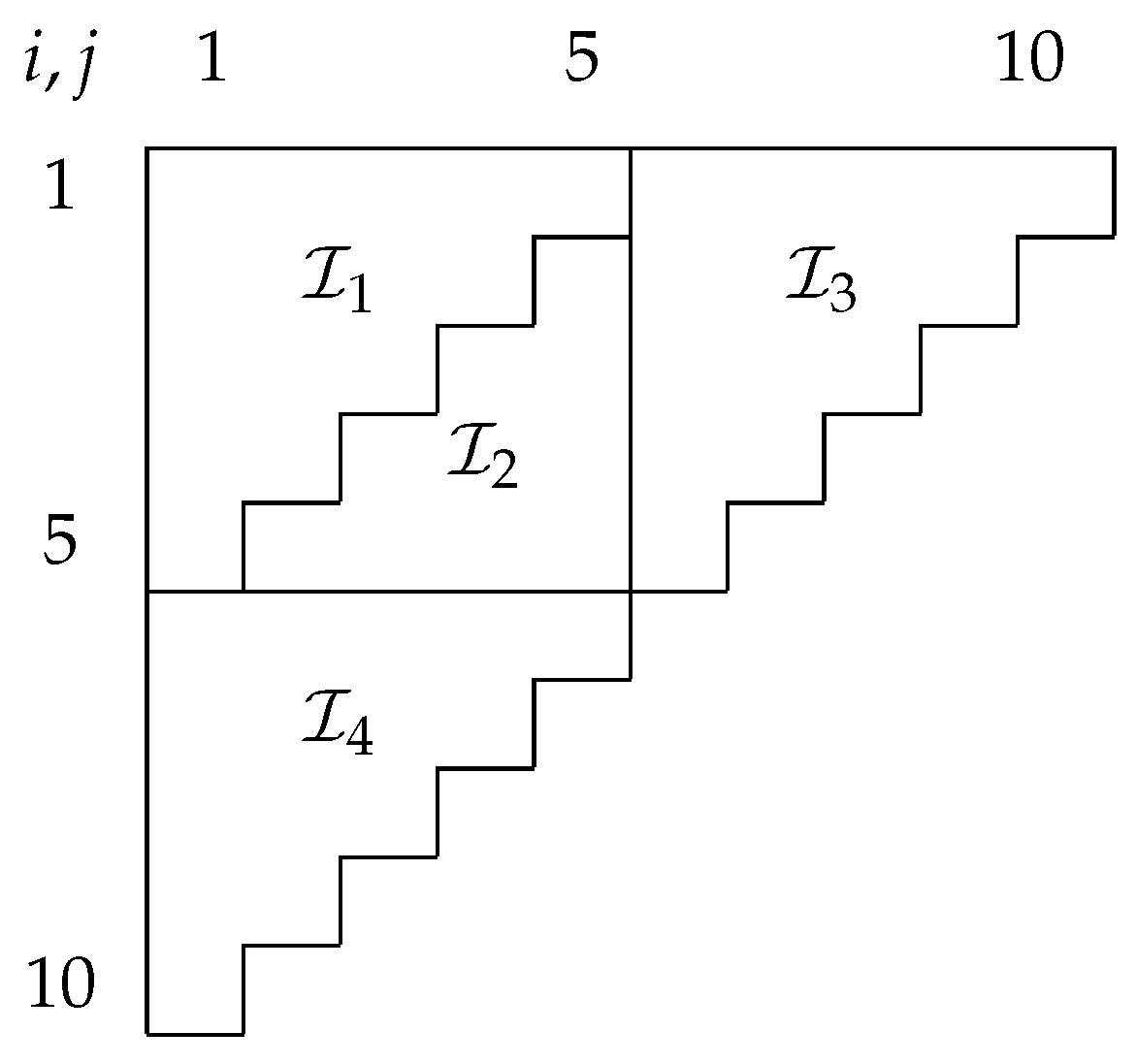
4.1. Identifiable Differences
4.2. Null Model: Over-Dispersed Poisson
4.3. Null Model: Generalized Log-Normal
4.4. Distribution of Ratios of Quadratic Forms
4.5. Power
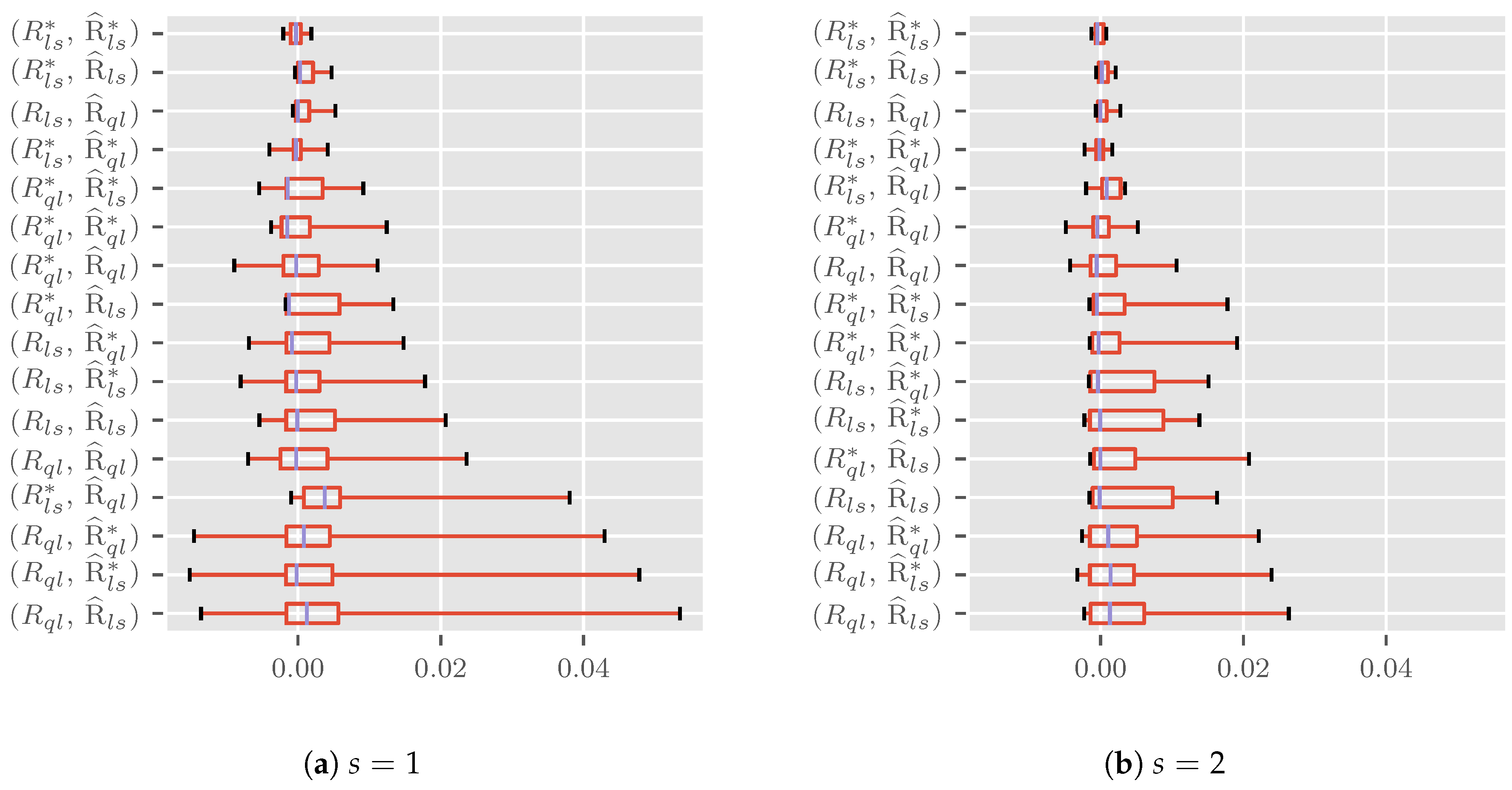
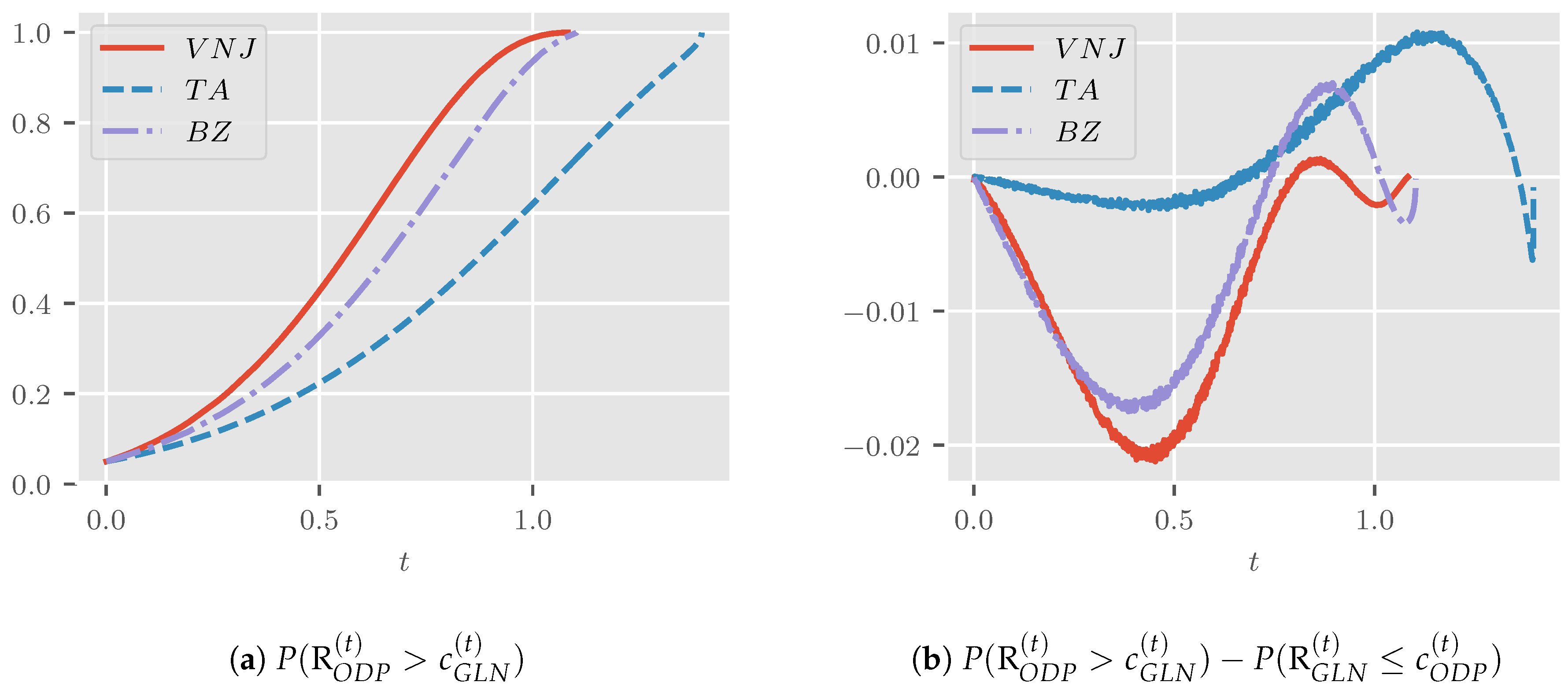
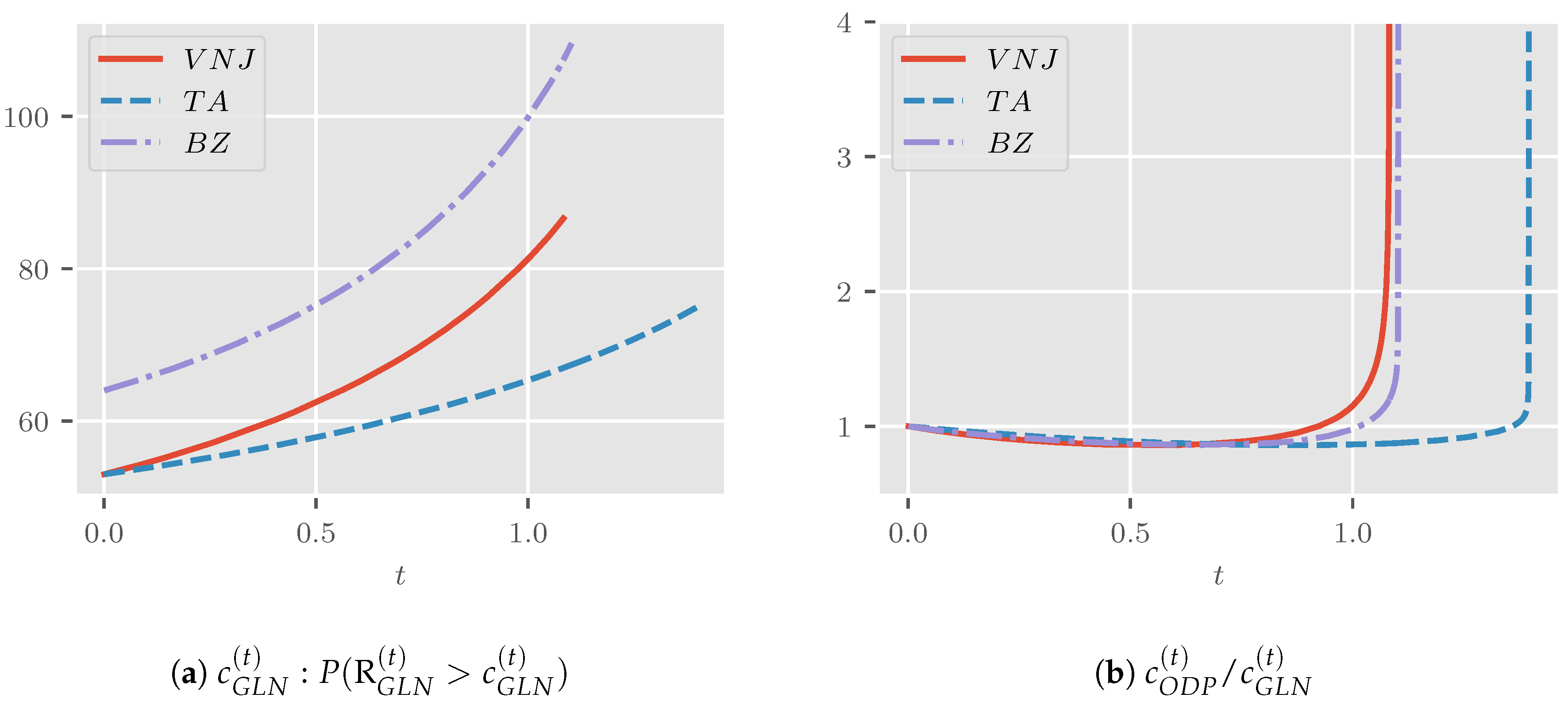
5. Simulations
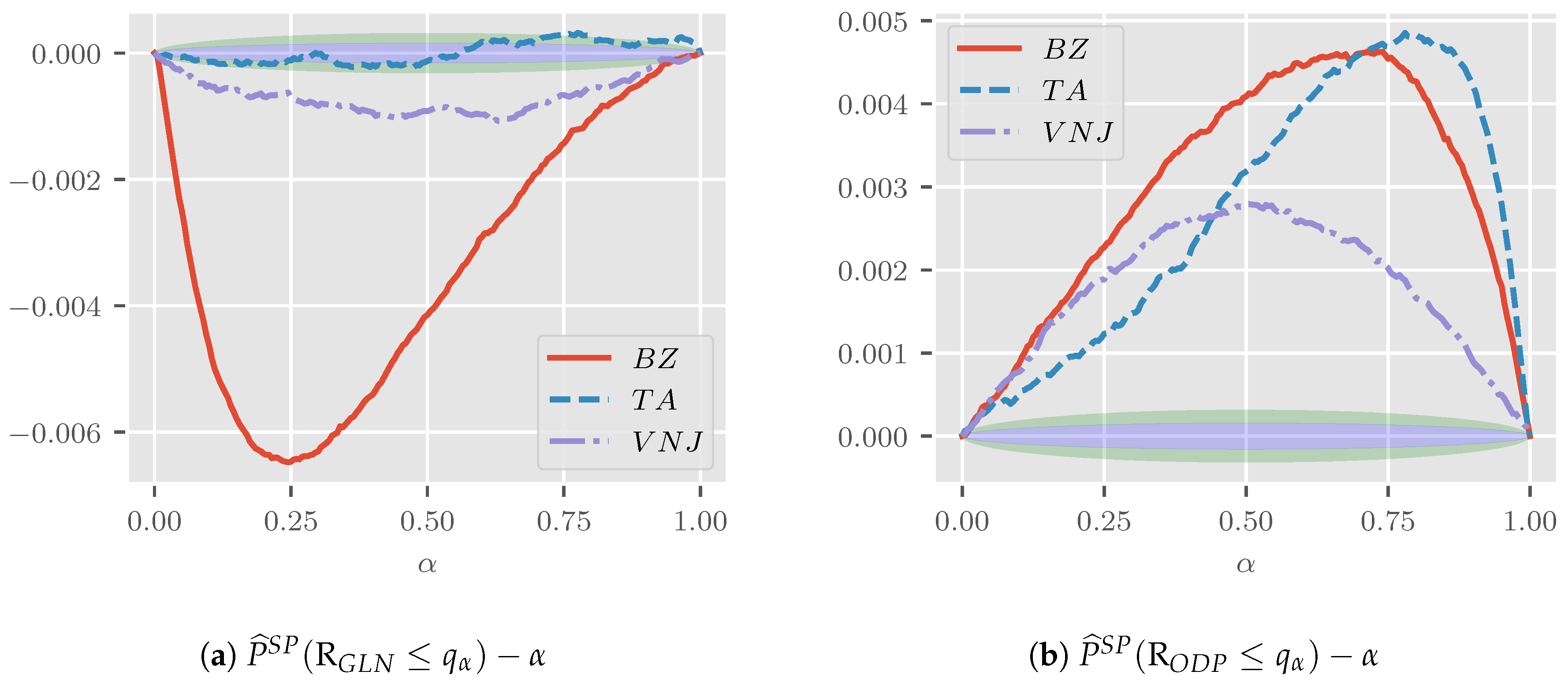
5.1. Quality of Saddle Point Approximations
5.2. Finite Sample Approximations under the Null
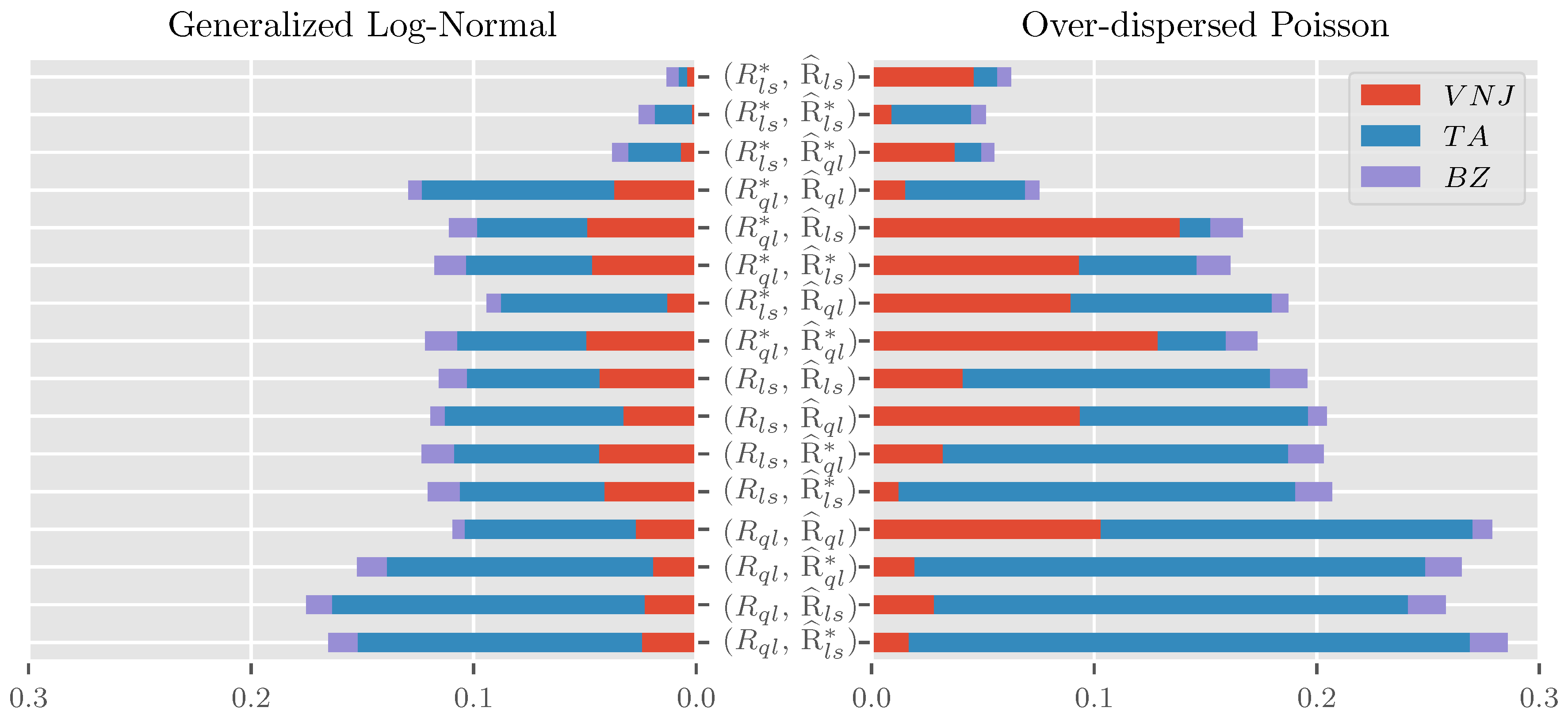
5.3. Power
5.3.1. Finite Sample Approximations Under the Alternative
5.3.2. Increasing Mean Dispersion in Limiting Distributions
6. Empirical Applications
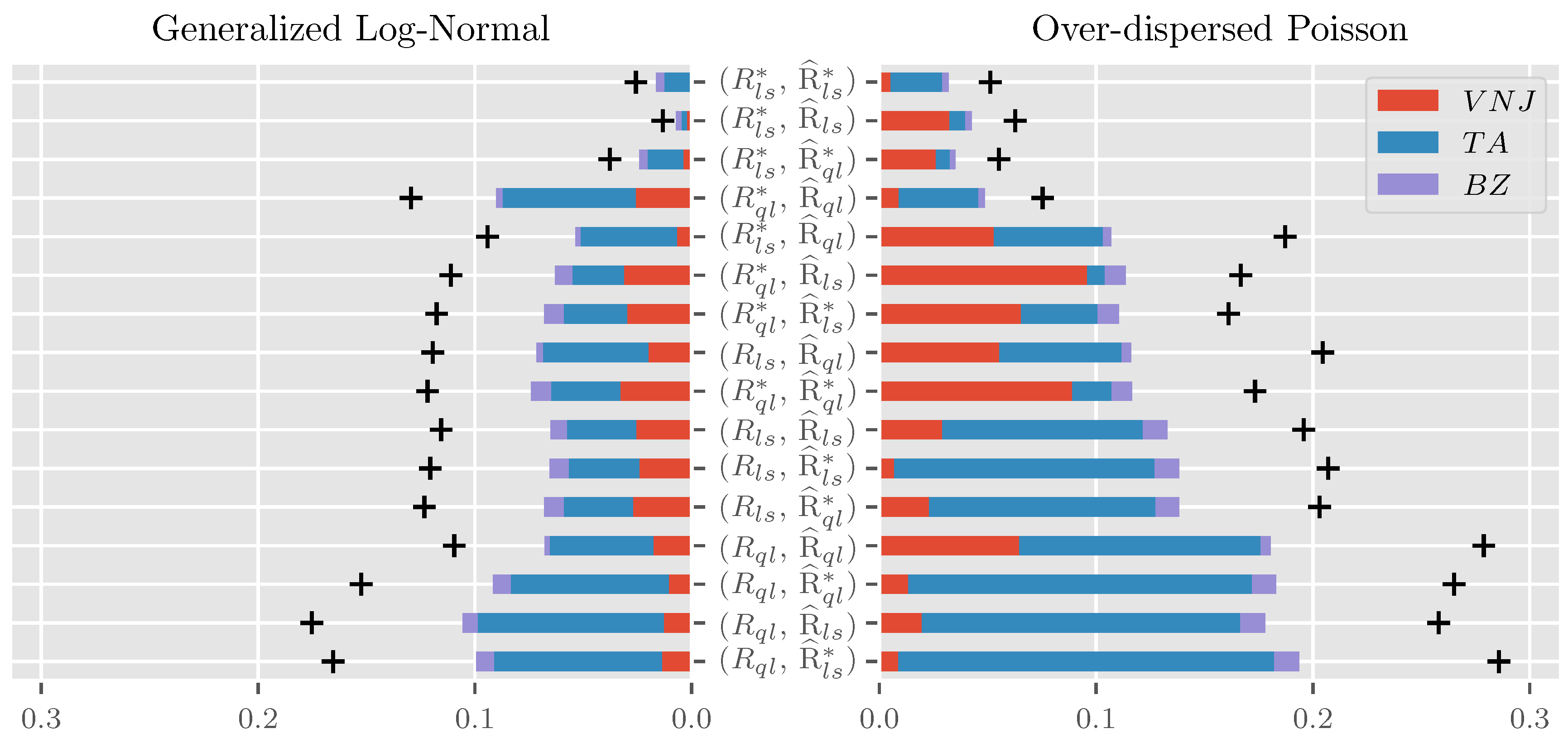
6.1. Empirical Illustration Revisited
6.2. Sensitivity to Invalid Model Reductions
6.3. A General to Specific Testing Procedure
7. Discussion
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Proof of Corollary 1
Appendix A.2. Proof of Lemma 1
Appendix A.3. Proof of Lemma 2
Appendix A.4. Proof of Lemma 3
Appendix A.5. Proof of Theorem 1
Appendix A.6. Proof of Lemma 4
Appendix A.7. Proof of Theorem 2
Appendix A.8. Proof of Lemma 5
Appendix A.9. Proof of Theorem 3
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 01 | 153,638 | 188,412 | 134,534 | 87,456 | 60,348 | 42,404 | 31,238 | 21,252 | 16,622 | 14,440 | 12,200 |
| 02 | 178,536 | 226,412 | 158,894 | 104,686 | 71,448 | 47,990 | 35,576 | 24,818 | 22,662 | 18,000 | - |
| 03 | 210,172 | 259,168 | 188,388 | 123,074 | 83,380 | 56,086 | 38,496 | 33,768 | 27,400 | - | - |
| 04 | 211,448 | 253,482 | 183,370 | 131,040 | 78,994 | 60,232 | 45,568 | 38,000 | - | - | - |
| 05 | 219,810 | 266,304 | 194,650 | 120,098 | 87,582 | 62,750 | 51,000 | - | - | - | - |
| 06 | 205,654 | 252,746 | 177,506 | 129,522 | 96,786 | 82,400 | - | - | - | - | - |
| 07 | 197,716 | 255,408 | 194,648 | 142,328 | 105,600 | - | - | - | - | - | - |
| 08 | 239,784 | 329,242 | 264,802 | 190,400 | - | - | - | - | - | - | - |
| 09 | 326,304 | 471,744 | 375,400 | - | - | - | - | - | - | - | - |
| 10 | 420,778 | 590,400 | - | - | - | - | - | - | - | - | - |
| 11 | 496,200 | - | - | - | - | - | - | - | - | - | - |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 01 | 357,848 | 766,940 | 610,542 | 482,940 | 527,326 | 574,398 | 146,342 | 139,950 | 227,229 | 67,948 |
| 02 | 352,118 | 884,021 | 933,894 | 1,183,289 | 445,745 | 320,996 | 527,804 | 266,172 | 425,046 | - |
| 03 | 290,507 | 1,001,799 | 926,219 | 1,016,654 | 750,816 | 146,923 | 495,992 | 280,405 | - | - |
| 04 | 310,608 | 1,108,250 | 776,189 | 1,562,400 | 272,482 | 352,053 | 206,286 | - | - | - |
| 05 | 443,160 | 693,190 | 991,983 | 769,488 | 504,851 | 470,639 | - | - | - | - |
| 06 | 396,132 | 937,085 | 847,498 | 805,037 | 705,960 | - | - | - | - | - |
| 07 | 440,832 | 847,631 | 1,131,398 | 1,063,269 | - | - | - | - | - | - |
| 08 | 359,480 | 1,061,648 | 1,443,370 | - | - | - | - | - | - | - |
| 09 | 376,686 | 986,608 | - | - | - | - | - | - | - | - |
| 10 | 344,014 | - | - | - | - | - | - | - | - | - |
References
- Barndorff-Nielsen, Ole E. 1978. Information and Exponential Families. Chichester: Wiley. [Google Scholar]
- Barnett, Glen, and Ben Zehnwirth. 2000. Best estimates for reserves. Proceedings of the Casualty Actuarial Society LXXXVII: 245–321. [Google Scholar]
- Bartlett, Maurice S. 1937. Properties of Sufficiency and Statistical Tests. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences 160: 268–82. [Google Scholar] [CrossRef]
- Beard, Robert E., Teivo Pentikäinen, and Erkki Pesonen. 1984. Risk Theory: The Stochastic Basis of Insurance, 3rd ed. London and New York: Chapman and Hall. [Google Scholar]
- Butler, Ronald W., and Marc S. Paolella. 2008. Uniform saddle point approximations for ratios of quadratic forms. Bernoulli 14: 140–54. [Google Scholar] [CrossRef]
- Casella, George, and Berger Roger L. 2002. Statistical Inference, 2nd ed. Pacific Grove: Duxbury/Thomson Learning. [Google Scholar]
- Casualty Actuarial Society. 2011. Loss Reserving Data Pulled From Naic Schedule P. Available online: http://www.casact.org/research/index.cfm?fa=loss_reserves_data (accessed on 8 July 2018).
- Chow, Gregory C. 1960. Tests of Equality Between Sets of Coefficients in Two Linear Regressions. Econometrica 28: 591–605. [Google Scholar] [CrossRef]
- Cox, David R. 1961. Tests of separate families of hypothesis. In Proceedings of the Fourth Berkeley Symposium on Mathematical Statistics and Probability. Berkeley: University of California Press, pp. 105–23. [Google Scholar]
- Cox, David R. 1962. Further Results on Tests of Separate Families of Hypotheses. Journal of the Royal Statistical Society, Series B 24: 406–24. [Google Scholar]
- Durbin, James, and Geoffrey S. Watson. 1950. Testing for serial correlation in least squares regression. I. Biometrika 37: 409–28. [Google Scholar] [PubMed]
- Durbin, James, and Geoffrey S. Watson. 1951. Testing for Serial Correlation in Least Squares Regression. II. Biometrika 38: 159–77. [Google Scholar] [CrossRef] [PubMed]
- England, Peter, and Richard Verrall. 1999. Analytic and bootstrap estimates of prediction errors in claims reserving. Insurance: Mathematics and Economics 25: 281–93. [Google Scholar] [CrossRef]
- England, Peter D., and Richard J. Verrall. 2002. Stochastic Claims Reserving in General Insurance. British Actuarial Journal 8: 443–518. [Google Scholar] [CrossRef]
- England, Peter. 2002. Addendum to “Analytic and bootstrap estimates of prediction errors in claims reserving”. Insurance: Mathematics and Economics 31: 461–66. [Google Scholar] [CrossRef]
- Ermini, Luigi, and David F. Hendry. 2008. Log income vs. linear income: An application of the encompassing principle. Oxford Bulletin of Economics and Statistics 70: 807–27. [Google Scholar] [CrossRef]
- Harnau, Jonas, and Bent Nielsen. 2017. Over-dispersed age-period-cohort models. Journal of the American Statistical Association. [Google Scholar] [CrossRef]
- Harnau, Jonas. 2017. apc. Available online: https://pypi.org/project/apc/ (accessed on 8 July 2018).
- Harnau, Jonas. 2018a. Misspecification Tests for Log-Normal and Over-Dispersed Poisson Chain-Ladder Models. Risks 6: 25. [Google Scholar] [CrossRef]
- Harnau, Jonas. 2018b. quad_form_ratio. Available online: https://pypi.org/project/quad-form-ratio/ (accessed on 8 July 2018).
- Hendry, David F., and Bent Nielsen. 2007. Econometric Modeling: A Likelihood Approach. Princeton: Princeton University Press. [Google Scholar]
- Hendry, David F., and Jean-Francois Richard. 1982. On the formulation of empirical models in dynamic econometrics. Journal of Econometrics 20: 3–33. [Google Scholar] [CrossRef]
- Johansen, Søren. 1979. Introduction to the Theory of Regular Exponential Families. Copenhagen: Institute of Mathematical Statistics, University of Copenhagen. [Google Scholar]
- Johnson, Norman Lloyd, Samuel Kotz, and N. Balakrishnan. 1995. Continuous Univariate Distributions Volume 1, 2nd ed. Chichester: Wiley. [Google Scholar]
- Kremer, Erhard. 1982. IBNR-claims and the two-way model of ANOVA. Scandinavian Actuarial Journal 1982: 47–55. [Google Scholar] [CrossRef]
- Kremer, Erhard. 1985. Einführung in die Versicherungsmathematik, 7th ed. Gottingen: Vandenhoeck & Ruprecht. [Google Scholar]
- Kuang, Di, and Bent Nielsen. 2018. Generalized Log-Normal Chain-Ladder. arXiv, arXiv:1806.05939. [Google Scholar]
- Kuang, Di, Bent Nielsen, and Jens P. Nielsen. 2008. Identification of the age-period-cohort model and the extended chain-ladder model. Biometrika 95: 979–86. [Google Scholar] [CrossRef]
- Kuang, Di, Bent Nielsen, and Jens Perch Nielsen. 2008. Forecasting with the age-period-cohort model and the extended chain-ladder model. Biometrika 95: 987–91. [Google Scholar] [CrossRef]
- Kuang, Di, Bent Nielsen, and Jens P. Nielsen. 2015. The geometric chain-ladder. Scandinavian Actuarial Journal 2015: 278–300. [Google Scholar] [CrossRef]
- Lancaster, Tony. 2000. The incidental parameter problem since 1948. Journal of Econometrics 95: 391–413. [Google Scholar] [CrossRef]
- Lee, Young K., Enno Mammen, Jens P. Nielsen, and Byeong U. Park. 2015. Asymptotics for in-sample density forecasting. Annals of Statistics 43: 620–51. [Google Scholar] [CrossRef]
- Lieberman, Offer. 1994. Saddle point approximation for the distribution of a ratio of quadratic forms in normal variables. Journal of the American Statistical Association 89: 924–28. [Google Scholar] [CrossRef]
- Lugannani, Robert, and Stephen Rice. 1980. Saddle point approximation for the distribution of the sum of independent random variables. Advances in Applied Probability 12: 475–90. [Google Scholar] [CrossRef]
- Mack, Thomas. 1993. Distribution free calculation of the standard error of chain ladder reserve estimates. ASTIN Bulletin 23: 213–25. [Google Scholar] [CrossRef]
- Martínez Miranda, María Dolores, Bent Nielsen, and Jens Perch Nielsen. 2015. Inference and forecasting in the age-period-cohort model with unknown exposure with an application to mesothelioma mortality. Journal of the Royal Statistical Society: Series A (Statistics in Society) 178: 29–55. [Google Scholar] [CrossRef]
- Mizon, Grayham E., and Jean-Francois Richard. 1986. The Encompassing Principle and its Application to Testing Non-Nested Hypotheses. Econometrica 54: 657–78. [Google Scholar] [CrossRef]
- Newcomb, Robert W. 1961. On the simultaneous diagonalization of two semi-definite matrices. Quarterly of Applied Mathematics 19: 144–46. [Google Scholar] [CrossRef]
- Neyman, Jerzy, and Elizabeth L. Scott. 1948. Consistent estimates based on partially consistent observations. Econometrica 16: 1–32. [Google Scholar] [CrossRef]
- Nielsen, Bent, and Jens P. Nielsen. 2014. Identification and forecasting in mortality models. The Scientific World Journal 2014: 347043. [Google Scholar] [CrossRef] [PubMed]
- Nielsen, Bent. 2015. apc: An R Package for Age-Period-Cohort Analysis. The R Journal 7: 52–64. [Google Scholar]
- R Core Team. 2017. R: A Language and Environment for Statistical Computing. Vienna: R Foundation for Statistical Computing. [Google Scholar]
- Taylor, Greg C., and Frank. R. Ashe. 1983. Second moments of estimates of outstanding claims. Journal of Econometrics 23: 37–61. [Google Scholar] [CrossRef]
- Thorin, Olof. 1977. On the infinite divisibility of the lognormal distribution. Scandinavian Actuarial Journal 1977: 121–48. [Google Scholar] [CrossRef]
- Verrall, Richard, Jens Perch Nielsen, and Anders Hedegaard Jessen. 2010. Prediction of RBNS and IBNR claims using claim amounts and claim counts. ASTIN Bulletin 40: 871–87. [Google Scholar]
- Verrall, Richard J. 1991. On the estimation of reserves from loglinear models. Insurance: Mathematics and Economics 10: 75–80. [Google Scholar] [CrossRef]
- Verrall, Richard J. 1994. Statistical methods for the chain ladder technique. Casualty Actuarial Society Forum 1: 393–446. [Google Scholar]
- Vuong, Quang H. 1989. Likelihood Ratio Tests for Model Selection and Non-Nested Hypotheses. Econometrica 57: 307–33. [Google Scholar] [CrossRef]
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | |
|---|---|---|---|---|---|---|---|---|---|---|
| 01 | 451,288 | 339,519 | 333,371 | 144,988 | 093,243 | 045,511 | 25,217 | 20,406 | 31,482 | 1729 |
| 02 | 448,627 | 512,882 | 168,467 | 130,674 | 056,044 | 033,397 | 56,071 | 26,522 | 14,346 | - |
| 03 | 693,574 | 497,737 | 202,272 | 120,753 | 125,046 | 037,154 | 27,608 | 17,864 | - | - |
| 04 | 652,043 | 546,406 | 244,474 | 200,896 | 106,802 | 106,753 | 63,688 | - | - | - |
| 05 | 566,082 | 503,970 | 217,838 | 145,181 | 165,519 | 091,313 | - | - | - | - |
| 06 | 606,606 | 562,543 | 227,374 | 153,551 | 132,743 | - | - | - | - | - |
| 07 | 536,976 | 472,525 | 154,205 | 150,564 | - | - | - | - | - | - |
| 08 | 554,833 | 590,880 | 300,964 | - | - | - | - | - | - | - |
| 09 | 537,238 | 701,111 | - | - | - | - | - | - | - | - |
| 10 | 684,944 | - | - | - | - | - | - | - | - | - |
| DGP | |||||||
| 99.02 | 00.25 | 00.14 | 0.18 | 00.22 | |||
| 94.61 | −0.94 | −0.96 | −0.97 | −0.94 | |||
| 65.39 | 04.18 | 03.41 | 5.28 | 03.15 | |||
| - | |||||||
| 99.23 | −0.30 | −0.25 | −0.34 | −0.20 | |||
| 94.67 | −1.14 | −1.15 | −1.12 | −1.12 | |||
| 64.73 | 00.81 | 00.15 | 04.68 | 02.49 | |||
| : Generalized Log-Normal | : Over-Dispersed Poisson | |||||||
|---|---|---|---|---|---|---|---|---|
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Harnau, J. Log-Normal or Over-Dispersed Poisson? Risks 2018, 6, 70. https://doi.org/10.3390/risks6030070
Harnau J. Log-Normal or Over-Dispersed Poisson? Risks. 2018; 6(3):70. https://doi.org/10.3390/risks6030070
Chicago/Turabian StyleHarnau, Jonas. 2018. "Log-Normal or Over-Dispersed Poisson?" Risks 6, no. 3: 70. https://doi.org/10.3390/risks6030070
APA StyleHarnau, J. (2018). Log-Normal or Over-Dispersed Poisson? Risks, 6(3), 70. https://doi.org/10.3390/risks6030070