On Central Branch/Reinsurance Risk Networks: Exact Results and Heuristics
Abstract
1. Introduction
2. General Background on Risk Networks
- The first time when (at least) one insurance company is ruined is given by
- The first time when all the insurance companies experience simultaneous ruin is denoted by
- The first time when the sum of the insurance companies is ruined is called
- A general class of insolvency sets was introduced in Hult and Lindskog (2006); Li et al. (2015) (inspired by the “bid-ask matrices” of Kabanov and Safarian (2009); Kabanov (1999)). The insolvency set is the set where it is impossible to cover the total negative position using fractions bounded by , out of the positive positions2Correspondingly, we introduceWhen we use the notation .
- The Gerber-Shiu/severity of ruin function induced by a ruin time :
- The combined optimal discounted dividends until ruin:induced by a ruin time , where denote the nonnegative cumulative dividends/consumption/ benefits processes of the i-th branch.This functional may be used, following the ideas of De Finetti (1957) and Miller and Modigliani (1961), to evaluate a network of collaborating companies, i.e., assign a numeric value to its performance, by using an approximation inspired by recent (yet unproved) results of Azcue et al. (2016)—see Section 6. Once this heuristic approximation for the value of the collaborating network is computed, one may compare it with the sums of the values of its components (defined analogously, using individual ruin times in the absence of interactions), and decide whether the existence of the collaboration is justified, as opposed to severing the connections between the subsidiaries.
- The Pollaczek-Khinchine type formula for provided in the foundational paper Chan et al. (2003).
- The asymptotic treatment of ruin probabilities for conic insolvency sets, assuming regularly varying tails—see Hult and Lindskog (2006).
- Optimizing “decoupled” objectives like total time “in the red”—see Loisel (2005) and asymptotic objectives like “orange time”—see Liu and Woo (2014).
3. A Bail-Out + Reinsurance Central Branch Risk Network Model
- 1.
- Several subsidiaries , with downward jumps, which must be kept in certain “solvability regions” by bail-outs from a central branch , or be liquidated otherwise. For example, they might need to be maintained above certain prescribed levels . For other possible solvability regions, see Section 1.We will denote by the j-th intervention time on the i-th subsidiary, to be referred from now on as bailout time.
- 2.
- The reserve of the CB is a process with downward jumps denoted by in the absence of subsidiaries, and by after subtracting the bailouts. The ruin timecauses the ruin of the whole network and leads to a severe penalty.
- 3.
- The CB must also cover a certain proportion of each claim of subsidiary i, leaving the subsidiary to pay only , where are called proportional reinsurance retention levels.
4. Laplace Transform for the “or”-Ruin Probability of a Proportional Reinsurer with a Dedicated Spectrally Negative Liability
4.1. Integro-Differential Equation
4.2. A Riemann-Hilbert Equation for the Laplace Transform
4.3. Explicit Formula for the Laplace Transform in the Presence of an Invariant Cone
5. A Two-Dimensional CB with Proportional Reinsurance and Crisis Bailouts
6. Valuation of Risk Networks by Optimal Dividends
6.1. Individual Valuation of Financial Companies
6.2. Evaluating a Conglomerate of Companies by Claims Line Dividend Policies
- Starting from the claims line, the optimal policy is to stay on this line by cashing the excess income of the subsidiary as dividends.
- Starting from points away from the claims line, in the cheap reinsurance case, the optimal policy is to reach the claims line by one lump sum payment4.
7. Conclusions
- Can the performance of a risk network be improved by using stop-loss reinsurance, or proportional reinsurance, or a combination of the two, or reinsurance strategies recently studied by Tan et al. (2018)?
- Our model considers intervention of the central branch at ruin times; however, other intervention times such as draw-down/regret times and Parisian ruin times may result in better network performance.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Asmussen, Søren, and Hansjörg Albrecher. 2010. Ruin Probabilities. Singapore: World Scientific, vol. 14. [Google Scholar]
- Albrecher, Hansjörg, Jevgenijs Ivanovs, and Xiaowen Zhou. 2016. Exit identities for Lévy processes observed at Poisson arrival times. Bernoulli 22: 1364–82. [Google Scholar] [CrossRef]
- Avram, Florin, and Andreea Minca. 2015. Steps towards a management toolkit for central branch risk networks, using rational approximations and matrix scale functions. In Modern Trends in Controlled Stochastic Processes: Theory and Applications. Edited by Alexey B. Piunovskyi. Luniver Press. [Google Scholar]
- Avram, Florin, and Andreea Minca. 2017. On the central management of risk networks. Advances in Applied Probability 49: 221–37. [Google Scholar] [CrossRef]
- Avram, Florin, and Xiaowen Zhou. 2016. On fluctuation theory for spectrally negative Lévy processes with Parisian reflection below, and applications. Theory of Probability and Mathematical Statistics 95: 14–36. [Google Scholar] [CrossRef]
- Avram, Florin, Martijn R. Pistorius, and Zbigniew Palmowski. 2006. A two-dimensional ruin problem on the positive quadrant, with exponential claims: Feynman-Kac formula, Laplace transform and its inversion. Paper presented at Ninth International Conference Zaragoza-Pau on Applied Mathematics and Statistics, Jaca, Spain, September 19–21. [Google Scholar]
- Avram, Florin, Zbigniew Palmowski, and Martijn R. Pistorius. 2007. On the optimal dividend problem for a spectrally negative Lévy process. The Annals of Applied Probability 17: 156–80. [Google Scholar] [CrossRef]
- Avram, Florin, Zbigniew Palmowski, and Martijn R. Pistorius. 2008a. Exit problem of a two-dimensional risk process from the quadrant: Exact and asymptotic results. The Annals of Applied Probability 18: 2421–49. [Google Scholar] [CrossRef]
- Avram, Florin, Zbigniew Palmowski, and Martijn R. Pistorius. 2008b. A two-dimensional ruin problem on the positive quadrant. Insurance: Mathematics and Economics 42: 227–34. [Google Scholar] [CrossRef]
- Avram, Florin, Andrei Badescu, Martijn R. Pistorius, and Landy Rabehasaina. 2016. On a class of dependent Sparre Andersen risk models and a bailout application. Insurance: Mathematics and Economics 71: 27–39. [Google Scholar] [CrossRef]
- Avram, Florin, Danijel Grahovac, and Ceren Vardar-Acar. 2017. The W,Z scale functions kit for first passage problems of spectrally negative Lévy processes, and applications to the optimization of dividends. arXiv, arXiv:1706.06841. [Google Scholar]
- Avram, Florin, José-Luis Pérez, and Kazutoshi Yamazaki. 2018. Spectrally negative Lévy processes with Parisian reflection below and classical reflection above. Stochastic Processes and Applications 128: 255–90. [Google Scholar] [CrossRef]
- Avram, Florin. 2017. Approximating central branch networks with one subsidiary via spectrally negative Markov additive processes. Work in Progress. Forthcoming. [Google Scholar]
- Azcue, Pablo, Nora Muler, and Zbigniew Palmowski. 2016. Optimal dividend payments for a two-dimensional insurance risk process. arXiv, arXiv:1603.07019. [Google Scholar]
- Badescu, Andrei L., Eric CK Cheung, and Landy Rabehasaina. 2011. A two-dimensional risk model with proportional reinsurance. Journal of Applied Probability 48: 749–65. [Google Scholar] [CrossRef]
- Badila, Serban E., Onno Boxma, Jacques Resing, and Erik Winands. 2014. Queues and risk models with simultaneous arrivals. Advances in Applied Probability 46: 812–31. [Google Scholar] [CrossRef]
- Bertoin, Jean. 1998. Lévy Processes. Cambridge: Cambridge University Press, vol. 121. [Google Scholar]
- Boonen, Tim J., Ken Seng Tan, and Sheng Chao Zhuang. 2016. The role of a representative reinsurer in optimal reinsurance. Insurance: Mathematics and Economics 70: 196–204. [Google Scholar] [CrossRef]
- Boonen, Tim J., Ken Seng Tan, and Sheng Chao Zhuang. 2018. Optimal Reinsurance with Multiple Reinsurers: Competitive Pricing and Coalition Stability. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3143224 (accessed on 11 April 2018).
- Boxma, Onno, and Jevgenijs Ivanovs. 2013. Two coupled Lévy queues with independent input. Stochastic Systems 3: 574–90. [Google Scholar] [CrossRef]
- Boxma, Onno, Esther Frostig, David Perry, and Rami Yosef. 2016. Partial coverage by a rich uncle until ruin: A reinsurance model. Research Report Eurandom 2016: 1. [Google Scholar]
- Boxma, Onno, Esther Frostig, and David Perry. 2017a. A reinsurance risk model with a threshold coverage policy: The Gerber–Shiu penalty function. Journal of Applied Probability 54: 267–85. [Google Scholar] [CrossRef]
- Boxma, Onno, Esther Frostig, David Perry, and Rami Yosef. 2017b. A state dependent reinsurance model. Insurance: Mathematics and Economics 74: 170–81. [Google Scholar] [CrossRef]
- Bäuerle, Nicole, and Anja Blatter. 2011. Optimal control and dependence modeling of insurance portfolios with Lévy dynamics. Insurance: Mathematics and Economics 48: 398–405. [Google Scholar] [CrossRef]
- Cai, Jun, and Haijun Li. 2005. Multivariate risk model of phase type. Insurance: Mathematics and Economics 36: 137–52. [Google Scholar] [CrossRef]
- Chan, Wai-Sum, Hailiang Yang, and Lianzeng Zhang. 2003. Some results on ruin probabilities in a two-dimensional risk model. Insurance: Mathematics and Economics 32: 345–58. [Google Scholar] [CrossRef]
- Chi, Yichun, and Hui Meng. 2014. Optimal reinsurance arrangements in the presence of two reinsurers. Scandinavian Actuarial Journal 2014: 424–38. [Google Scholar] [CrossRef]
- De Finetti, Bruno. 1957. Su un’impostazione alternativa della teoria collettiva del rischio. Paper presented at the Transactions of the XVth International Congress of Actuaries, New York, NY, USA, June 6; pp. 433–43. [Google Scholar]
- Frostig, Esther. 2008. On ruin probability for a risk process perturbed by a Lévy process with no negative jumps. Stochastic Models 24: 288–313. [Google Scholar] [CrossRef]
- Hu, Zechun, and Bin Jiang. 2013. On joint ruin probabilities of a two-dimensional risk model with constant interest rate. Journal of Applied Probability 50: 309–22. [Google Scholar] [CrossRef]
- Hult, Henrik, and Filip Lindskog. 2006. Heavy-Tailed Insurance Portfolios: Buffer Capital and Ruin Probabilities. Technical Report, 1441. Ithaca: School of ORIE Cornell University. [Google Scholar]
- Kabanov, Yuri, and Mher Safarian. 2009. Markets with Transaction Costs: Mathematical Theory. New York: Springer. [Google Scholar]
- Kabanov, Yuri. 1999. Hedging and liquidation under transaction costs in currency markets. Finance and Stochastics 3: 237–48. [Google Scholar] [CrossRef]
- Kriele, Marcus, and Jochen Wolf. 2014. Value-Oriented Risk Management of Insurance Companies. New York: Springer Science & Business Media. [Google Scholar]
- Kyprianou, Andreas E. 2014. Fluctuations of Lévy Processes with Applications: Introductory Lectures. New York: Springer Science & Business Media. [Google Scholar]
- Leobacher, Gunther, Michaela Szölgyenyi, and Stefan Thonhauser. 2014. Bayesian dividend optimization and finite time ruin probabilities. Stochastic Models 30: 216–49. [Google Scholar] [CrossRef][Green Version]
- Li, Junhai, Zaiming Liu, and Qihe Tang. 2007. On the ruin probabilities of a bidimensional perturbed risk model. Insurance: Mathematics and Economics 41: 185–95. [Google Scholar] [CrossRef]
- Li, Bo, Rong Wu, and Min Song. 2009. A renewal jump-diffusion process with threshold dividend strategy. Journal of Computational and Applied Mathematics 228: 41–55. [Google Scholar] [CrossRef]
- Li, Xiaohu, Jintang Wu, and Jinsen Zhuang. 2015. Asymptotic Multivariate Finite-time Ruin Probability with Statistically Dependent Heavy-tailed Claims. Methodology and Computing in Applied Probability 17: 463–477. [Google Scholar] [CrossRef]
- Liu, Jingchen, and Jae-Kyung Woo. 2014. Asymptotic analysis of risk quantities conditional on ruin for multidimensional heavy-tailed random walks. Insurance: Mathematics and Economics 55: 1–9. [Google Scholar] [CrossRef][Green Version]
- Loisel, Stephane. 2005. Differentiation of some functionals of risk processes, and optimal reserve allocation. Journal of Applied Probability 42: 379–92. [Google Scholar] [CrossRef]
- Miller, Merton H., and Franco Modigliani. 1961. Dividend policy, growth, and the valuation of shares. The Journal of Business 34: 411–433. [Google Scholar] [CrossRef]
- Rolski, Tomasz, Hanspeter Schmidli, Volker Schmidt, and Jozef L. Teugels. 2009. Stochastic Processes for Insurance and Finance. Hoboken: John Wiley & Sons, vol. 505. [Google Scholar]
- Salah, Zied Ben, and Jose Garrido. 2017. On Fair Reinsurance Premiums; Capital Injections in a Perturbed Risk Model. arXiv, arXiv:1710.11065. [Google Scholar]
- Suprun, V. 1976. Problem of destruction and resolvent of a terminating process with independent increments. Ukrainian Mathematical Journal 28: 39–51. [Google Scholar] [CrossRef]
- Tan, Ken Seng, Pengyu Wei, Wei Wei, and Sheng Chao Zhuang. 2018. Optimal Dynamic Reinsurance Policies Under Mean–CVaR–A Generalized Denneberg’s Absolute Deviation Principle. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=3138804 (accessed on 11 April 2018).
- Zhang, Zhimin, Hailiang Yang, and Hu Yang. 2013. On a Sparre Andersen risk model perturbed by a spectrally negative Lévy process. Scandinavian Actuarial Journal 2013: 213–39. [Google Scholar] [CrossRef]
| 1. | On a discrete lattice state space, the jumps may be bigger than one only downwards, and the diffusion is of course not allowed. |
| 2. | This concept does not involve actual transfers being carried out; must be viewed just as static limits of mutual solidarity. |
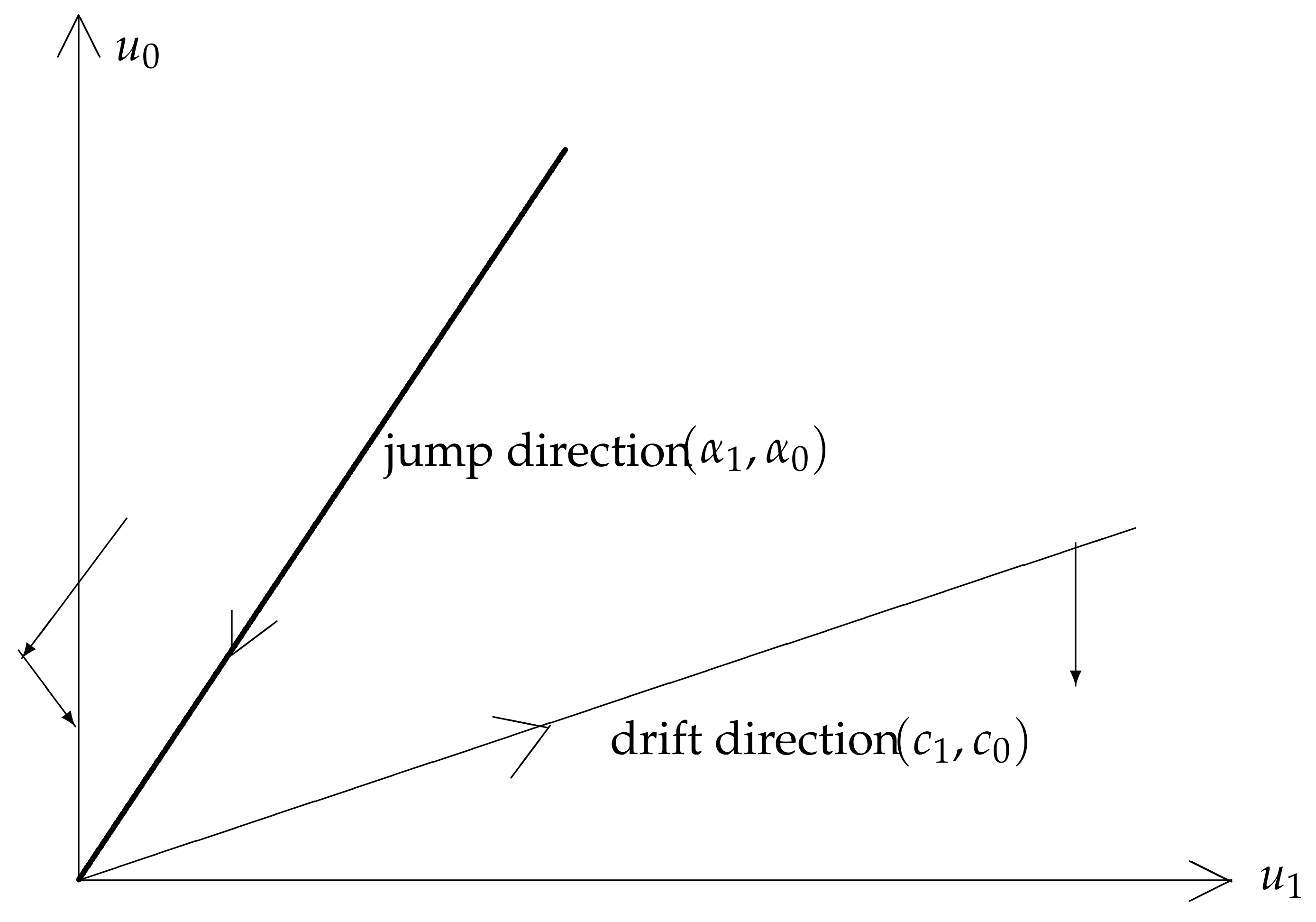
| 3. | Who computed an explicit value function maximizing an expected exponential utility at a fixed terminal time for multi-dimensional reinsurance model under the cheap reinsurance assumption that the drifts point along the line . |
| 4. | In the non-cheap reinsurance case, the optimal policy is more complicated, when starting in a certain egg-shaped subset of the non-invariant cone. There, parts of the premia must cashed, following a “shortest path”, in some sense. Later however, an error was discovered in this case and the paper Azcue et al. (2016) was withdrawn. |
| 5. | We may also describe the claims line policy informally as “follow the lead”, since the subsidiaries are always reducing their extra premiums and reserves to those of the CB. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Avram, F.; Loke, S.-H. On Central Branch/Reinsurance Risk Networks: Exact Results and Heuristics. Risks 2018, 6, 35. https://doi.org/10.3390/risks6020035
Avram F, Loke S-H. On Central Branch/Reinsurance Risk Networks: Exact Results and Heuristics. Risks. 2018; 6(2):35. https://doi.org/10.3390/risks6020035
Chicago/Turabian StyleAvram, Florin, and Sooie-Hoe Loke. 2018. "On Central Branch/Reinsurance Risk Networks: Exact Results and Heuristics" Risks 6, no. 2: 35. https://doi.org/10.3390/risks6020035
APA StyleAvram, F., & Loke, S.-H. (2018). On Central Branch/Reinsurance Risk Networks: Exact Results and Heuristics. Risks, 6(2), 35. https://doi.org/10.3390/risks6020035




