Optimal Reinsurance Under General Law-Invariant Convex Risk Measure and TVaR Premium Principle
Abstract
:1. Introduction
- (a)
- Generalizing the set of ceded loss functions. Cai, et al. [12] and Cheung [14] considered the set of all the increasing and convex functions as their feasible ceded loss function class. While, Lu, et al. [16] took the set of all the increasing and concave functions as their feasible ceded loss function class. Chi and Weng [15] extended their feasible ceded loss function class toChi and Tan [17] further extended their feasible ceded loss function class toOther more general feasible ceded loss function classcan be found in Cheung and Lo [18]. Different reinsurance contracts have been introduced in the reinsurance market among which there are quota-share, stop-loss, stop-loss after quota-share and quota-share after stop-loss, etc. It can be verified that all these reinsurance contracts belong to some or all of the above-mentioned feasible ceded loss function classes.
- (b)
- Generalizing the premium principles. To our knowledge, the most widely used premium principle in the existing works turns out to be the expected premium principle, see Cheung, et al. [19], Lu, et al. [16], Cai, et al. [5], Chi and Tan [17], etc.. Assa [20], Zheng and Cui [21], Cui, et al. [22] extended their premium principle to the distortion premium principle. Zhu, et al. [23] further extended their premium principle to very general one that satisfies three mild conditions: distribution invariance, risk loading and preserving the convex order, see also Chi and Tan [24].
- (c)
- Generalizing the risk measures. Using the VaR, CTE, AVaR, respectively, Hu, et al. [25], Cai and Tan [26], Cai, et al. [12], Cheung [14] and Chi and Tan [24] found the optimal reinsurance contract. In Asimit, et al. [27], a quantile-based risk measure was adopted in accordance with the insurer’s appetite. Assa [20], Zheng and Cui [21] and Cui, et al. [22] generalized their risk measures to the distortion risk measures. Cheung, et al. [19] further extended the problem by using a general law-invariant convex risk measure.
- (d)
- Constraints involved. Borch [1] (and also Arrow [4]) showed that, subject to a budget constraint, the stop-loss policy is an optimal reinsurance contract for the ceding company when the risk is measured by variance (or by a utility function). Reinsurance optimization problems involving premium constraint were also considered in Gajek and Zagrodny [10], Zhou, et al. [28], Zheng and Cui [21], Cui, et al. [22] and Cheung and Lo [18]. Cheung, et al. [29] introduced a reinsurer’s probabilistic benchmark constraint of his potential loss. In Tan and Weng [30], a profitability constraint was proposed.
2. The Mathematical Presentation of the Reinsurance Optimization Problem
3. Characterizing the Optimal Reinsurance Strategy
- (a)
- If , then for any . Hence (9) implies that
- (b)
- (c)
4. Sensitivity Analysis
- (i)
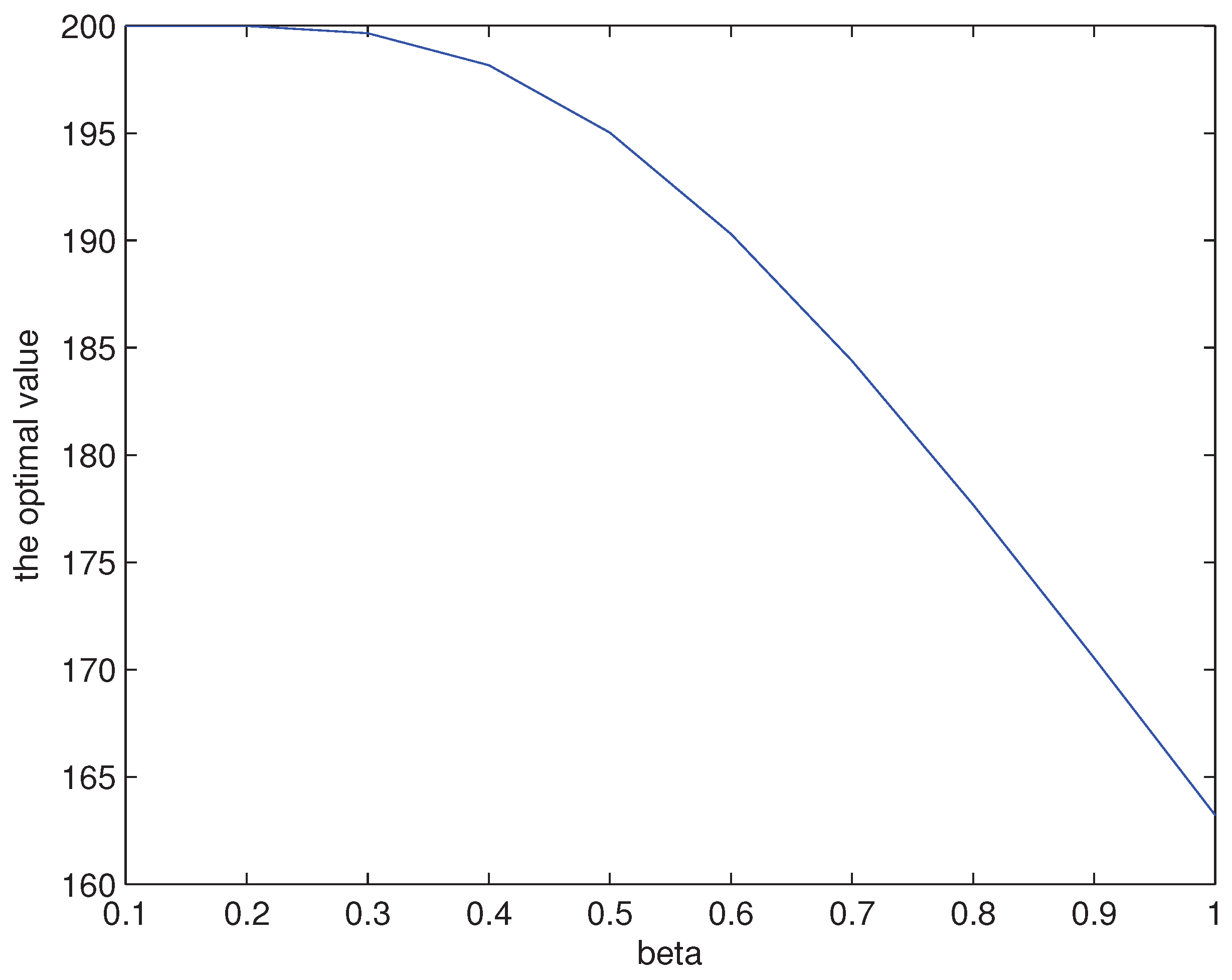
- According to Lemma 1, Problem (3) is equivalent toProblem (13) is reduced by Problem (2) by shrinking the probability measure set to the singleton with with fixed. Here,We take the infimum given by (13) as the bivariate function of , and numerically investigate how sensitively the value of will affect our optimal value. The corresponding numerical results are given by Figure 1 below. It seems that significant differences in the size of optimal value can be obtained depending on the value of . It also seems that the optimal value increases as α and β approaches 0.
- (ii)
5. Concluding Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
References
- K. Borch. “An attempt to determine the optimum amount of stop loss reinsurance.” In Proceedings of the Transactions of the 16th International Congress of Actuaries, Brussels, Belgium, 15–22 June 1960; pp. 597–610.
- M. Kaluszka. “Optimal reinsurance under mean-variance premium principles.” Insur. Math. Econ. 28 (2001): 61–67. [Google Scholar] [CrossRef]
- R. Kaas, M. Goovaerts, J. Dhaene, and M. Denuit. Modern Actuarial Risk Theory. Boston, MA, USA: Kluwer Academic Publishers, 2001. [Google Scholar]
- J. Cai, Y. Fang, Z. Li, and G. Willmot. “Optimal reciprocal reinsurance treaties under the joint survival probability and the joint profitable probability.” J. Risk Insur. 80 (2013): 145–168. [Google Scholar] [CrossRef]
- Y. Chi. “Reinsurance arrangements minimizing the risk-adjusted value of an insurer’s liability.” Insur. Math. Econ. 42 (2012): 529–557. [Google Scholar] [CrossRef]
- K.C. Cheung, W.F. Chong, and S.C.P. Yam. “The optimal insurance under disappointment theories.” Insur. Math. Econ. 64 (2015): 77–90. [Google Scholar] [CrossRef]
- M. Kaluszka. “An extension of Arrow’s result on optimality of a stop-loss contract.” Insur. Math. Econ. 35 (2004): 527–536. [Google Scholar] [CrossRef]
- M. Kaluszka. “Optimal reinsurance under convex principles of premium calculation.” Insur. Math. Econ. 36 (2005): 375–398. [Google Scholar] [CrossRef]
- L. Gajek, and D. Zagrodny. “Optimal reinsurance under general risk measures.” Insur. Math. Econ. 34 (2004): 227–240. [Google Scholar] [CrossRef]
- S.D. Promislow, and V.R. Young. “Unifying framework for optimal insurance.” Insur. Math. Econ. 36 (2005): 347–364. [Google Scholar] [CrossRef]
- J. Cai, K.S. Tan, C.G. Weng, and Y. Zhang. “Optimal reinsurance under VaR and CTE risk measures.” Insur. Math. Econ. 43 (2008): 185–196. [Google Scholar] [CrossRef]
- A. Balbás, B. Balbás, and A. Heras. “Optimal reinsurance with general risk measures.” Insur. Math. Econ. 44 (2009): 374–384. [Google Scholar] [CrossRef]
- K.C. Cheung. “Optimal reinsurance revisited-a geometric approach.” ASTIN Bull. 40 (2010): 221–239. [Google Scholar] [CrossRef]
- Y. Chi, and C. Weng. “Optimal reinsurance subject to Vajda condition.” Insur. Math. Econ. 53 (2013): 179–189. [Google Scholar] [CrossRef]
- Y. Chi, and K.S. Tan. “Optimal reinsurance under VaR and CVaR risk measures: A simplified approach.” ASTIN Bull. 41 (2011): 487–509. [Google Scholar]
- K.C. Cheung, and A. Lo. “Characterizations of optimal reinsurance treaties: A cost-benefit approach.” Scand. Actuar. J., 2015. [Google Scholar] [CrossRef]
- K.C. Cheung, K.C.J. Sung, S.C.P. Yam, and S.P. Yung. “Optimal reinsurance under general law-invariant risk measures.” Scand. Actuar. J. 2014 (2014): 72–91. [Google Scholar] [CrossRef]
- Z. Lu, L. Liu, Q. Shen, and L. Li. “Optimal reinsurance under VaR and CTE risk measures when ceded loss function is concave.” Commun. Stat. Theory Methods 43 (2014): 3223–3247. [Google Scholar] [CrossRef]
- H. Assa. “On optimal reinsurance policy with distortion risk measures and premiums.” Insur. Math. Econ. 61 (2015): 70–75. [Google Scholar] [CrossRef]
- Y.T. Zheng, and W. Cui. “Optimal reinsurance with premium constraint under distortion risk measures.” Insur. Math. Econ. 59 (2014): 109–120. [Google Scholar] [CrossRef]
- W. Cui, J.P. Yang, and L. Wu. “Optimal reinsurance minimizing the distortion risk measure under general reinsurance premium principles.” Insur. Math. Econ. 53 (2013): 74–85. [Google Scholar] [CrossRef]
- Y. Zhu, Y. Chi, and C. Weng. “Multivariate reinsurance designs for minimizing an insurer’s capital requirement.” Insur. Math. Econ. 59 (2014): 144–155. [Google Scholar] [CrossRef]
- Y. Chi, and K.S. Tan. “Optimal reinsurance with general premium principles.” Insur. Math. Econ. 52 (2013): 180–189. [Google Scholar] [CrossRef]
- X. Hu, H. Yang, and L. Zhang. “Optimal retention for a stop-loss reinsurance with incomplete information.” Insur. Math. Econ. 65 (2015): 15–21. [Google Scholar] [CrossRef]
- J. Cai, and K.S. Tan. “Optimal retention for a stop-loss reinsurance under the VaR and CTE risk measures.” ASTIN Bull. 37 (2007): 93–112. [Google Scholar] [CrossRef]
- A. Asimit, A. Badescu, and T. Verdonck. “Optimal risk transfer under quantile-based risk measurers.” Insur. Math. Econ. 53 (2013): 252–265. [Google Scholar] [CrossRef]
- K.J. Arrow. “Optimal insurance and generalized deductibles.” Scand. Actuar. J. 1 (1974): 1–42. [Google Scholar] [CrossRef]
- C.Y. Zhou, W.F. Wu, and C.F. Wu. “Optimal insurance in the presence of insurer’s loss limit.” Insur. Math. Econ. 46 (2010): 300–307. [Google Scholar] [CrossRef]
- K.C. Cheung, F. Liu, and S.C.P. Yam. “Average Value-at-Risk minimizing reinsurance under Wang’s premium principle with constraints.” ASTIN Bull. 42 (2012): 575–600. [Google Scholar]
- K. Tan, and C. Weng. “Enhancing insurer value using reinsurance and Value-at-Risk criterion.” Geneva Risk Insur. Rev. 37 (2012): 109–140. [Google Scholar] [CrossRef]
- V.R. Young. “Premium principles.” Encycl. Actuar. Sci. 3 (2004): 132–1331. [Google Scholar]
- H. Föllmer, and A. Schied. StochastiC Finance: An Introduction in Discrete Time, 4th ed. Berlin, Germany: Walter de Gruyter, 2016. [Google Scholar]
- J. Belles-Sampera, M. Guillén, and M. Santolino. “Beyond Value-at-Risk: GlueVaR Distortion Risk Measures.” Risk Anal. 34 (2014): 121–134. [Google Scholar] [CrossRef] [PubMed]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, M.; Wang, W.; Ming, R. Optimal Reinsurance Under General Law-Invariant Convex Risk Measure and TVaR Premium Principle. Risks 2016, 4, 50. https://doi.org/10.3390/risks4040050
Chen M, Wang W, Ming R. Optimal Reinsurance Under General Law-Invariant Convex Risk Measure and TVaR Premium Principle. Risks. 2016; 4(4):50. https://doi.org/10.3390/risks4040050
Chicago/Turabian StyleChen, Mi, Wenyuan Wang, and Ruixing Ming. 2016. "Optimal Reinsurance Under General Law-Invariant Convex Risk Measure and TVaR Premium Principle" Risks 4, no. 4: 50. https://doi.org/10.3390/risks4040050
APA StyleChen, M., Wang, W., & Ming, R. (2016). Optimal Reinsurance Under General Law-Invariant Convex Risk Measure and TVaR Premium Principle. Risks, 4(4), 50. https://doi.org/10.3390/risks4040050





