Substance Detection and Identification Using Frequency Doubling of the THz Broadband Pulse
Abstract
:1. Introduction
2. Physical Mechanism of the Absorption Frequency Doubling for the Pulse with a Broadband Spectrum
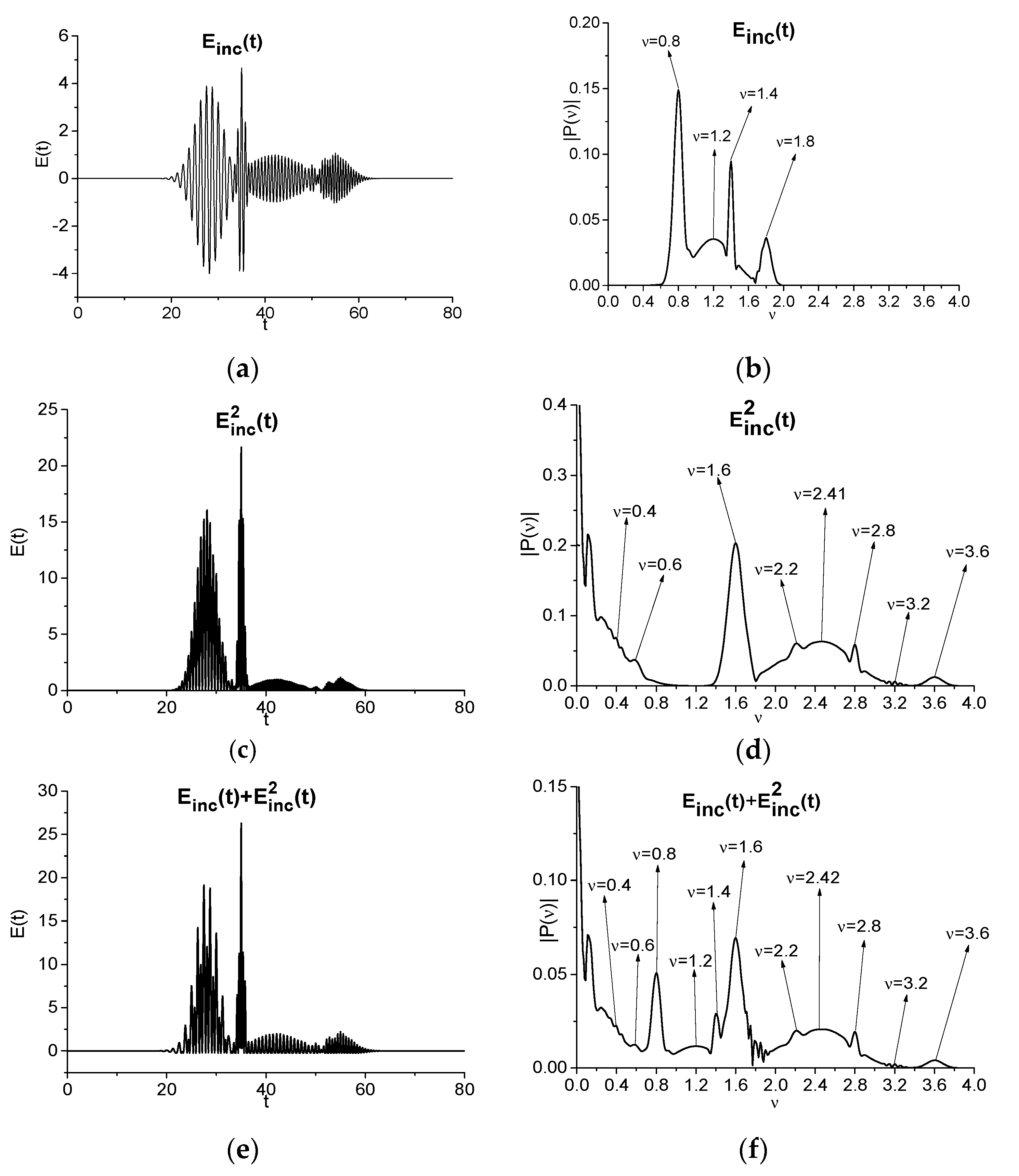
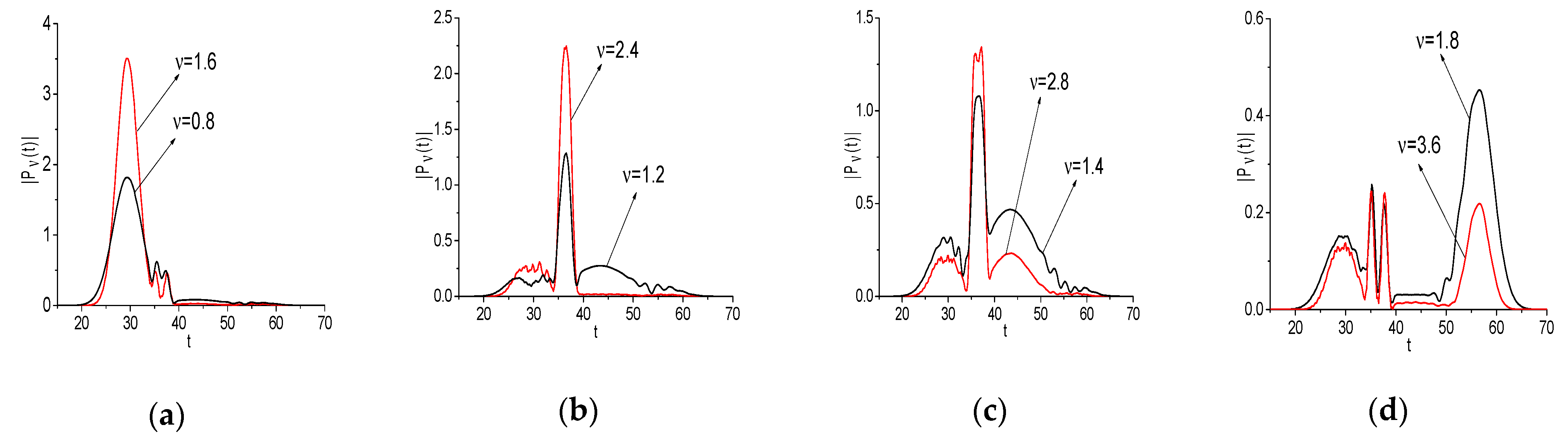
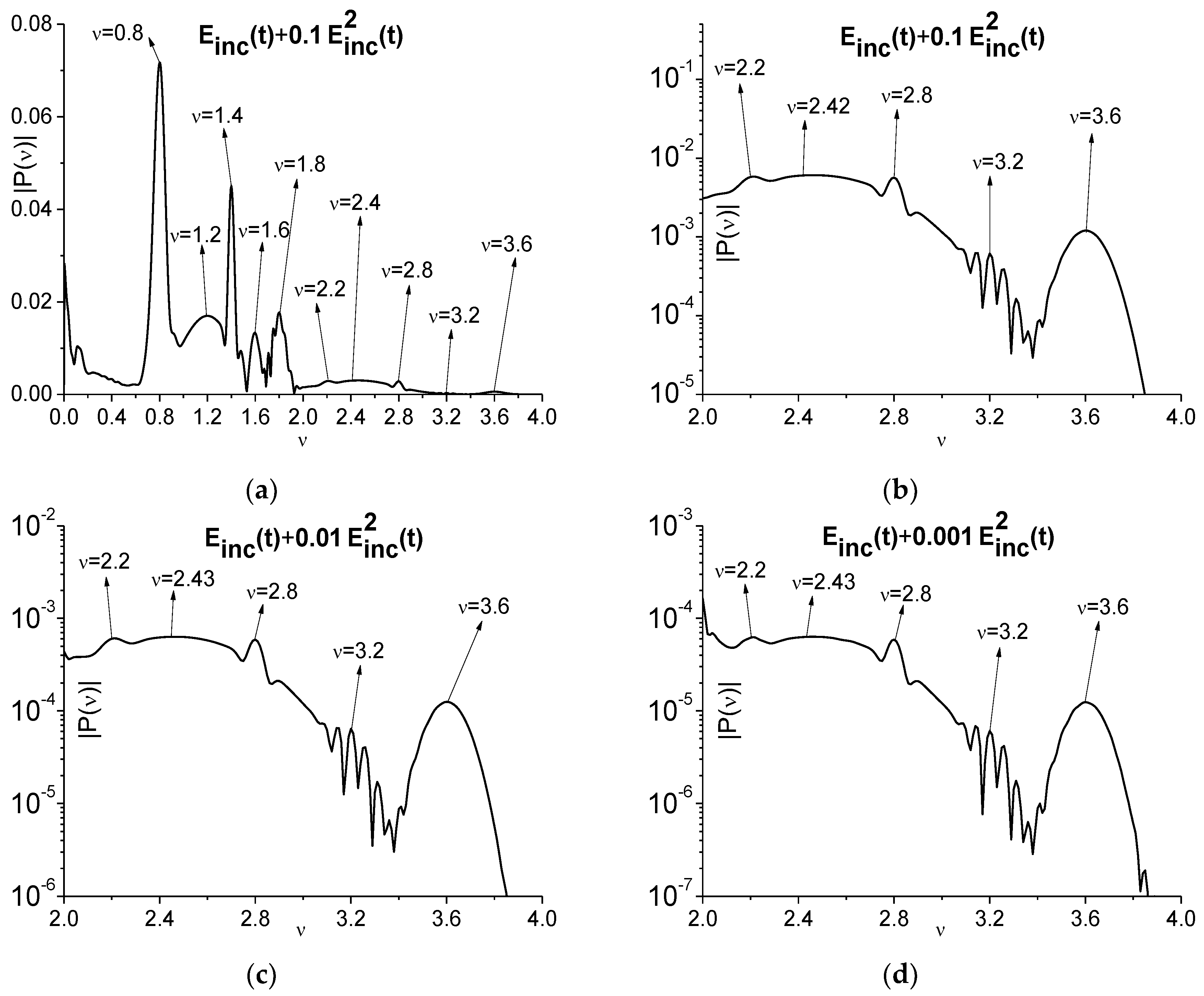
2.1. The Doubled Emission Frequencies
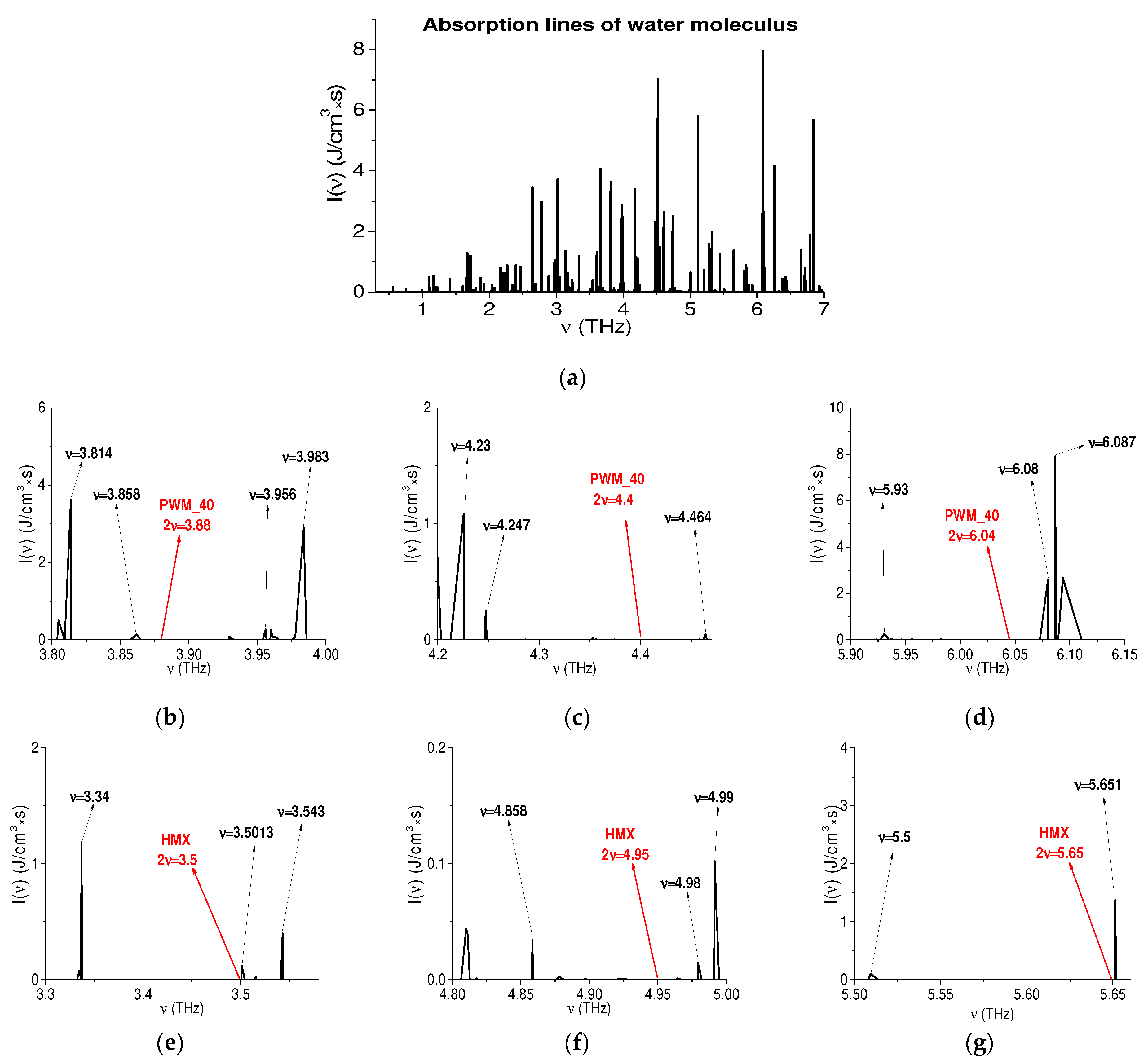
2.2. The Doubled Absorption Frequencies
3. SDA Method and Integral Correlation Criteria
4. Substance Detection and Identification in the Reflection Mode Based on Using Doubled Absorption Frequencies
4.1. THz Signal Reflected from the Tablet with a Rough Surface
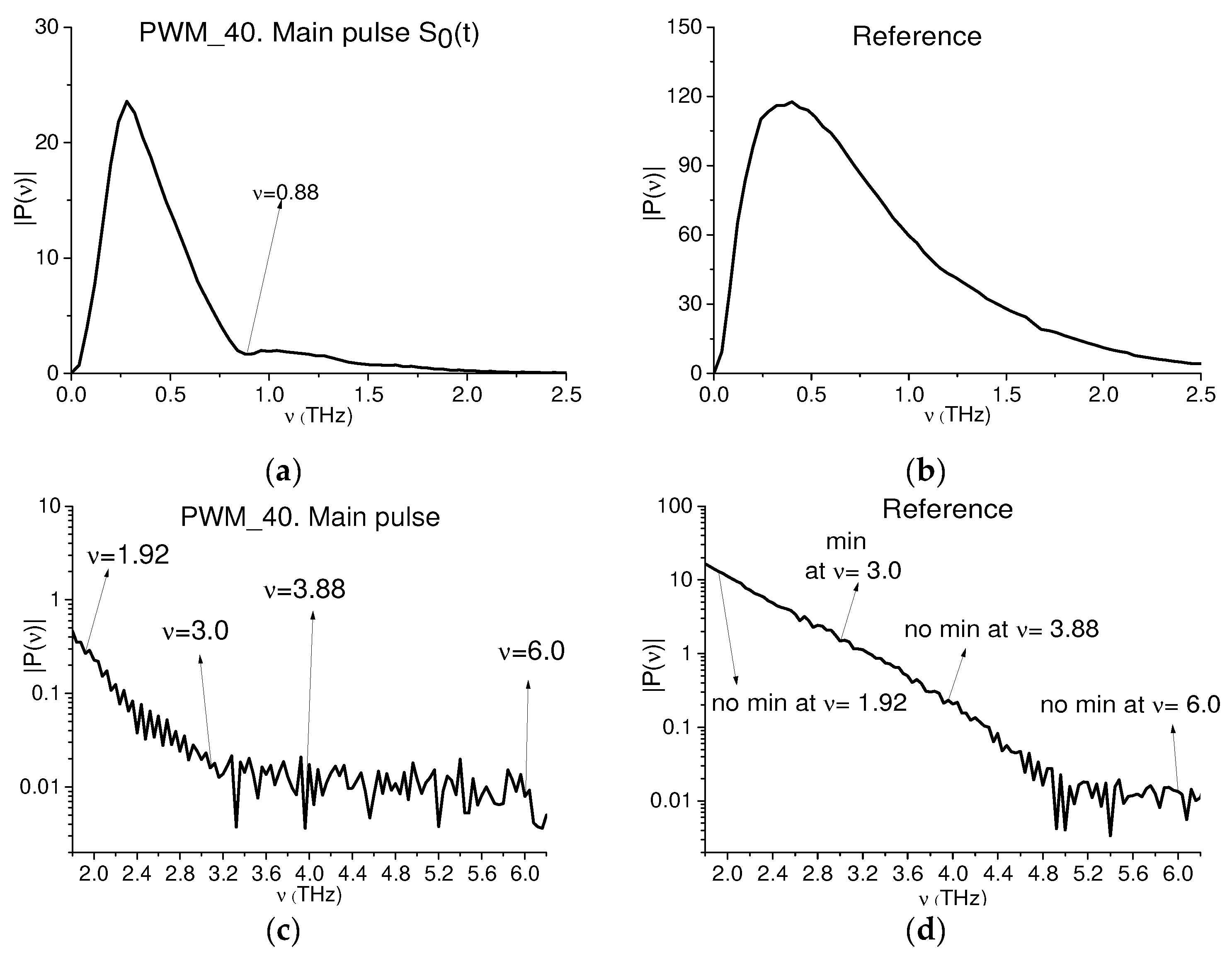
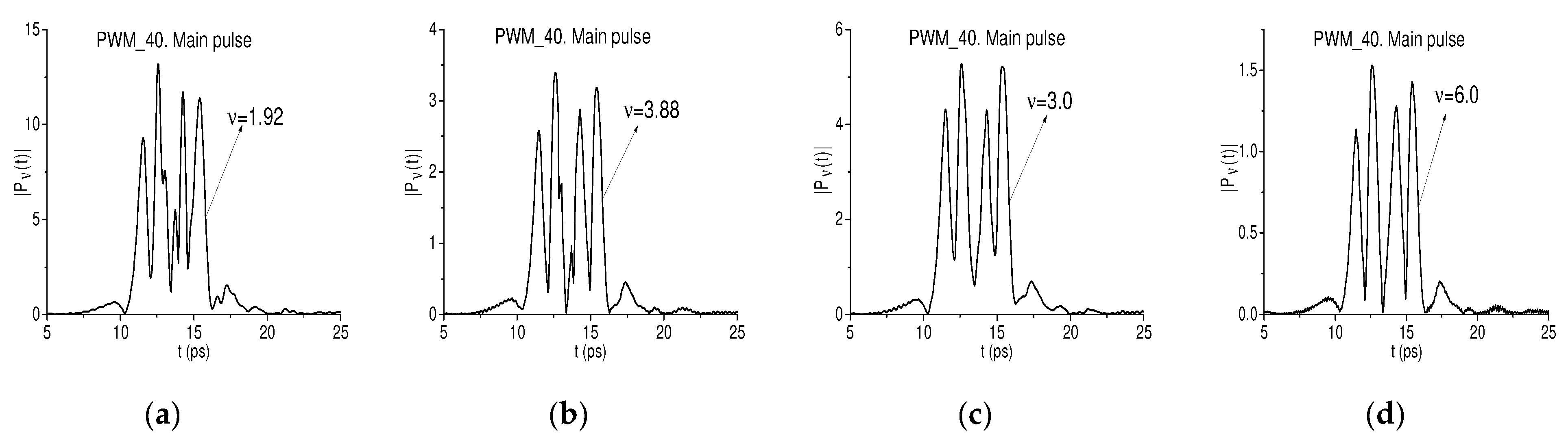
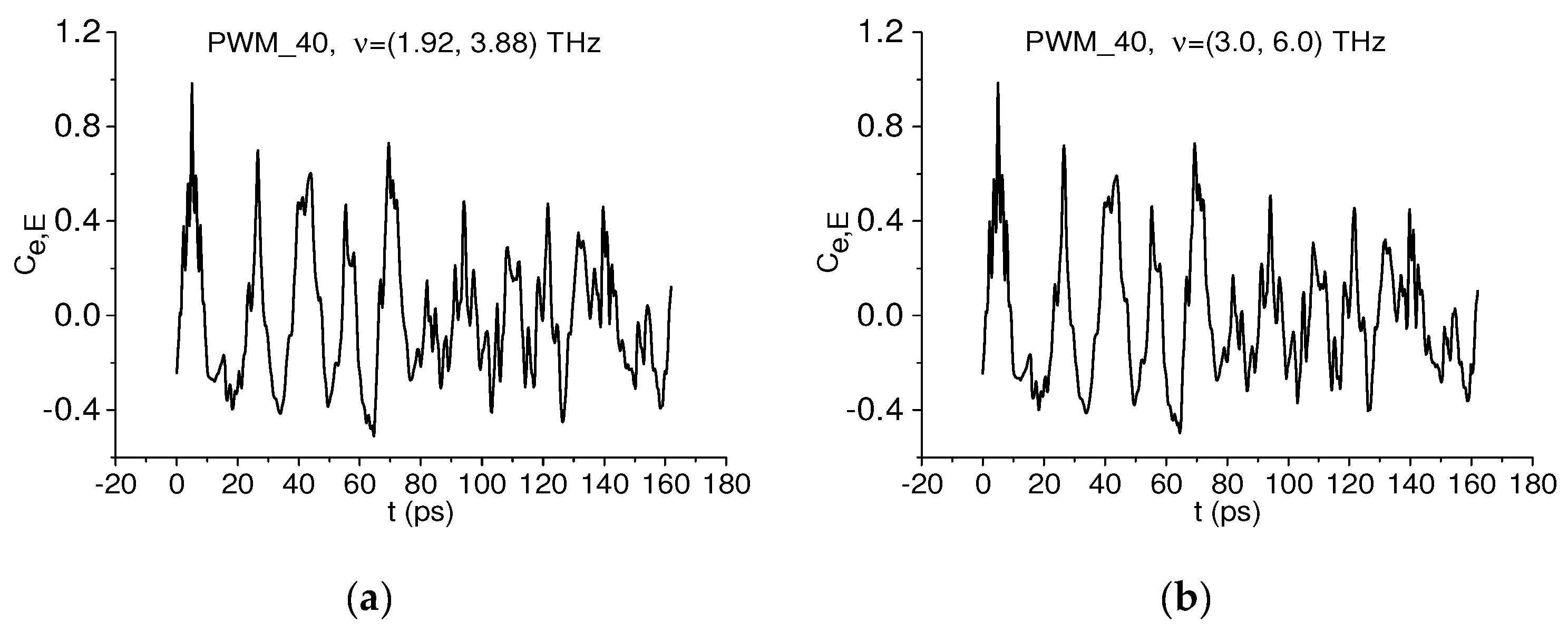
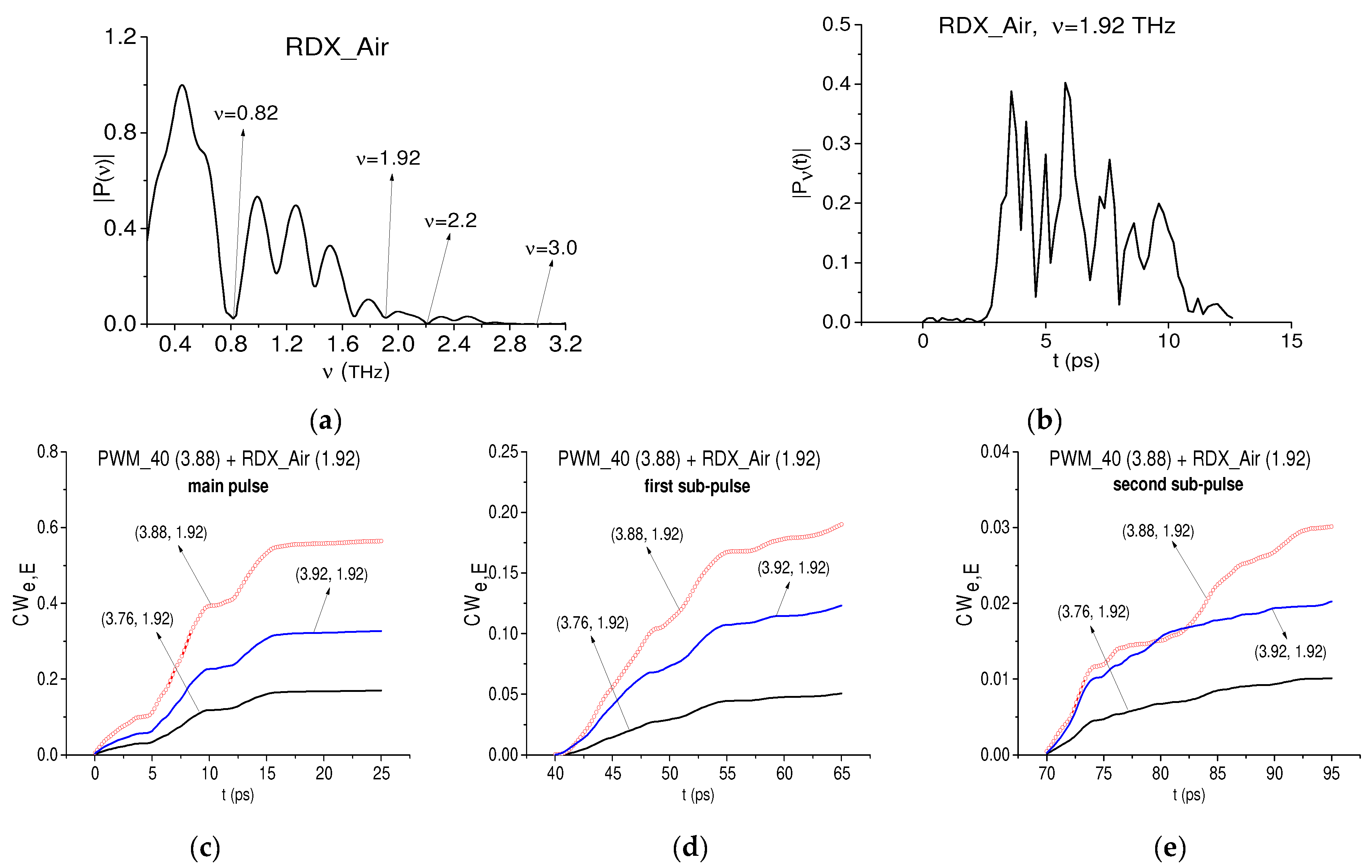
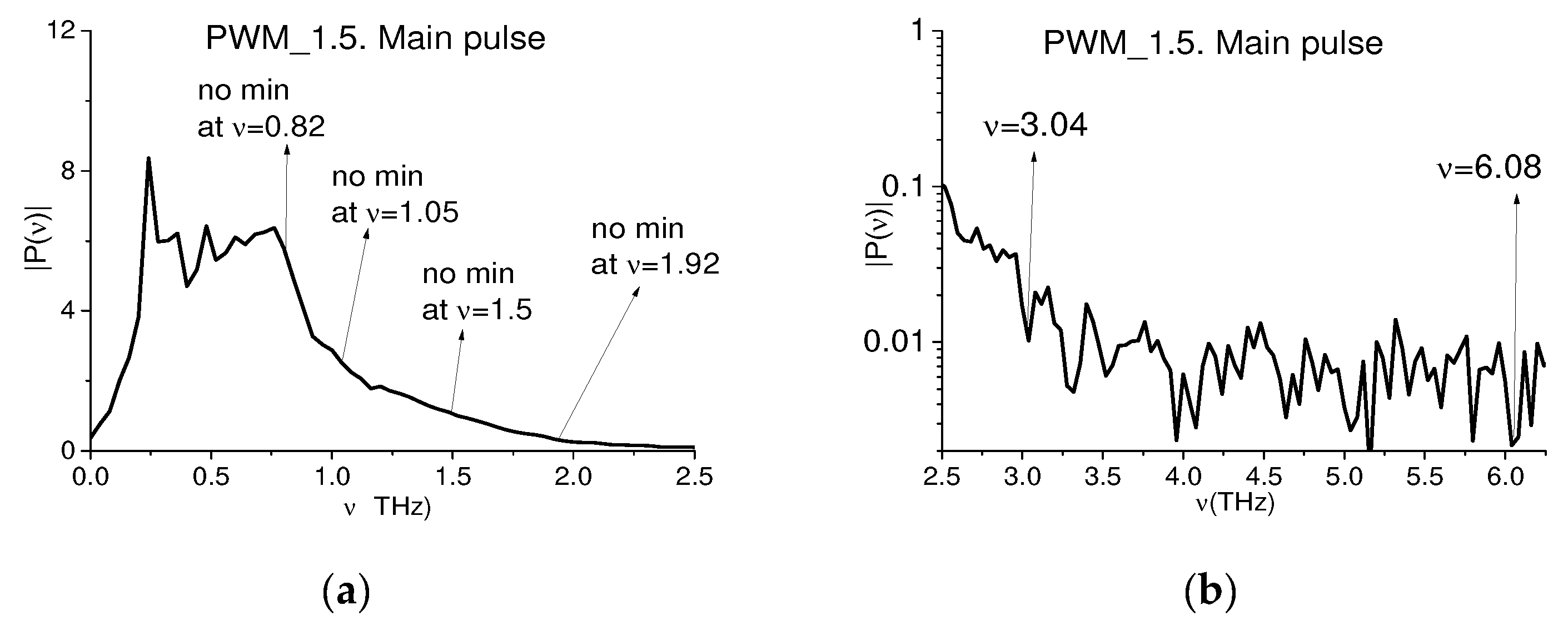
4.1.1. Observing Doubled Absorption Frequency Belonging to the Main Pulse, t = [0, 25] ps
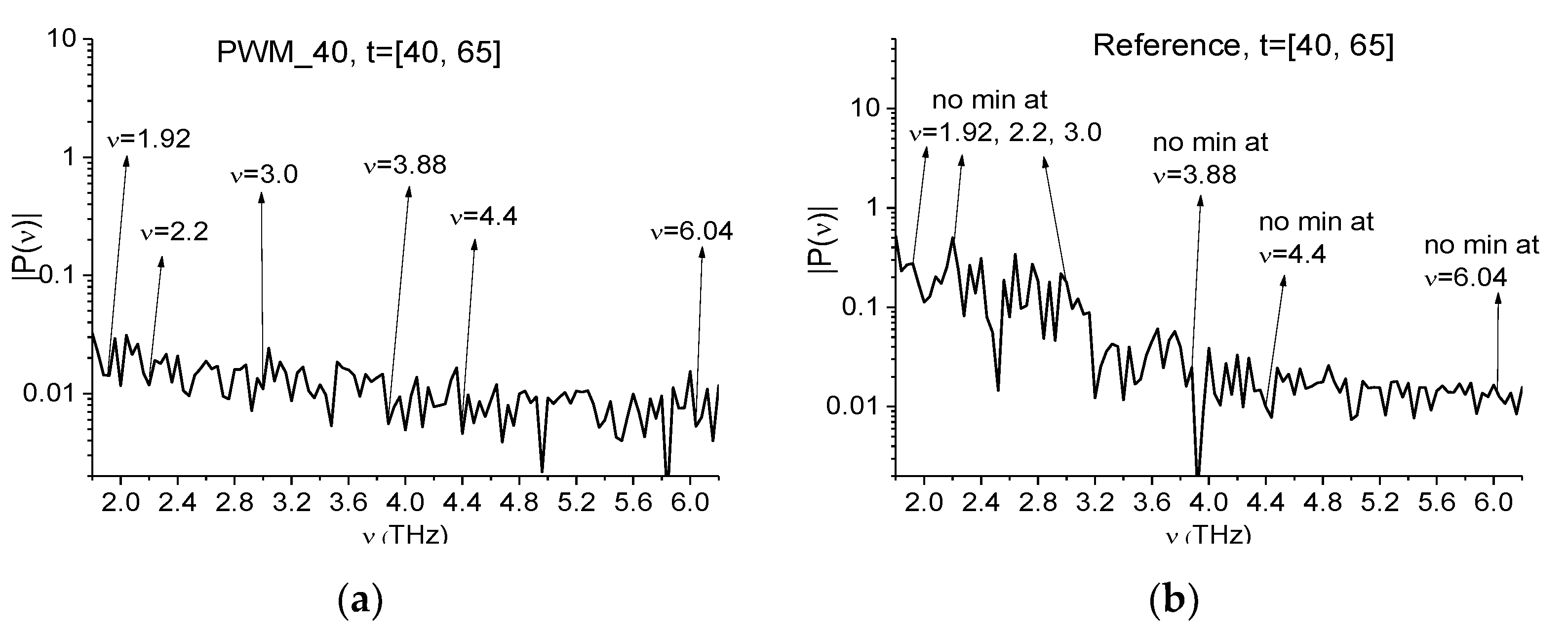
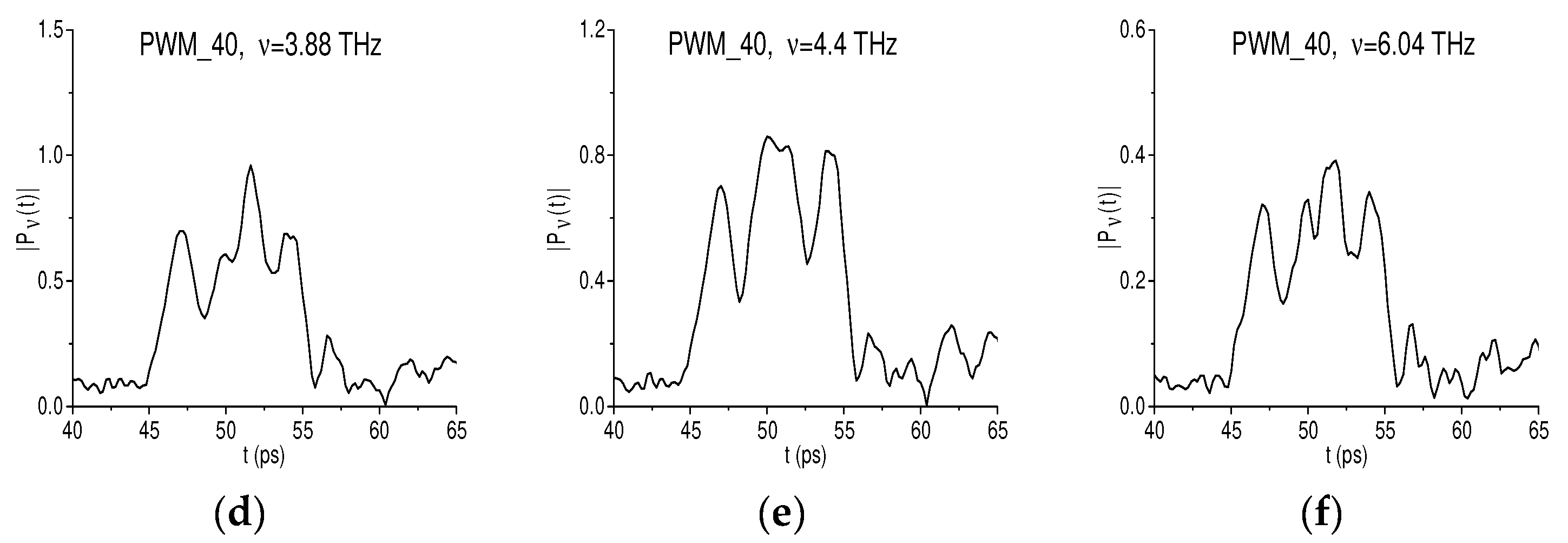
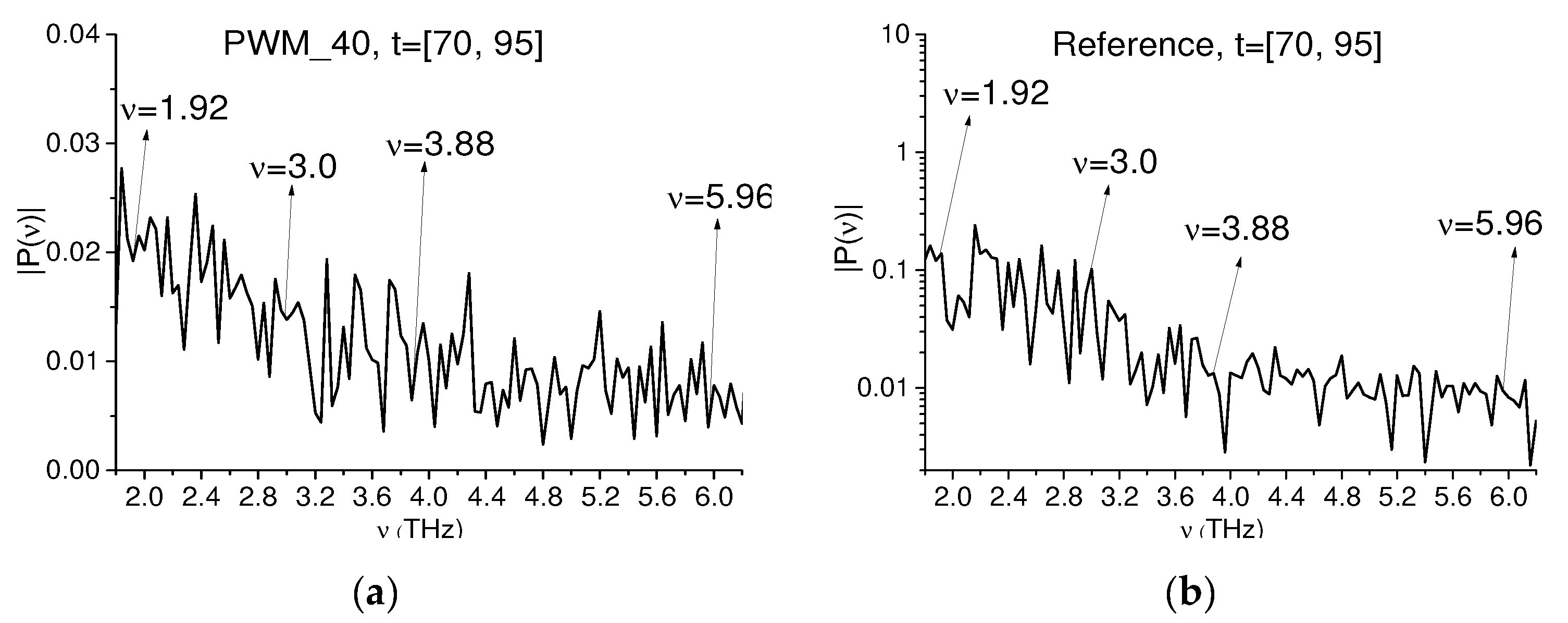
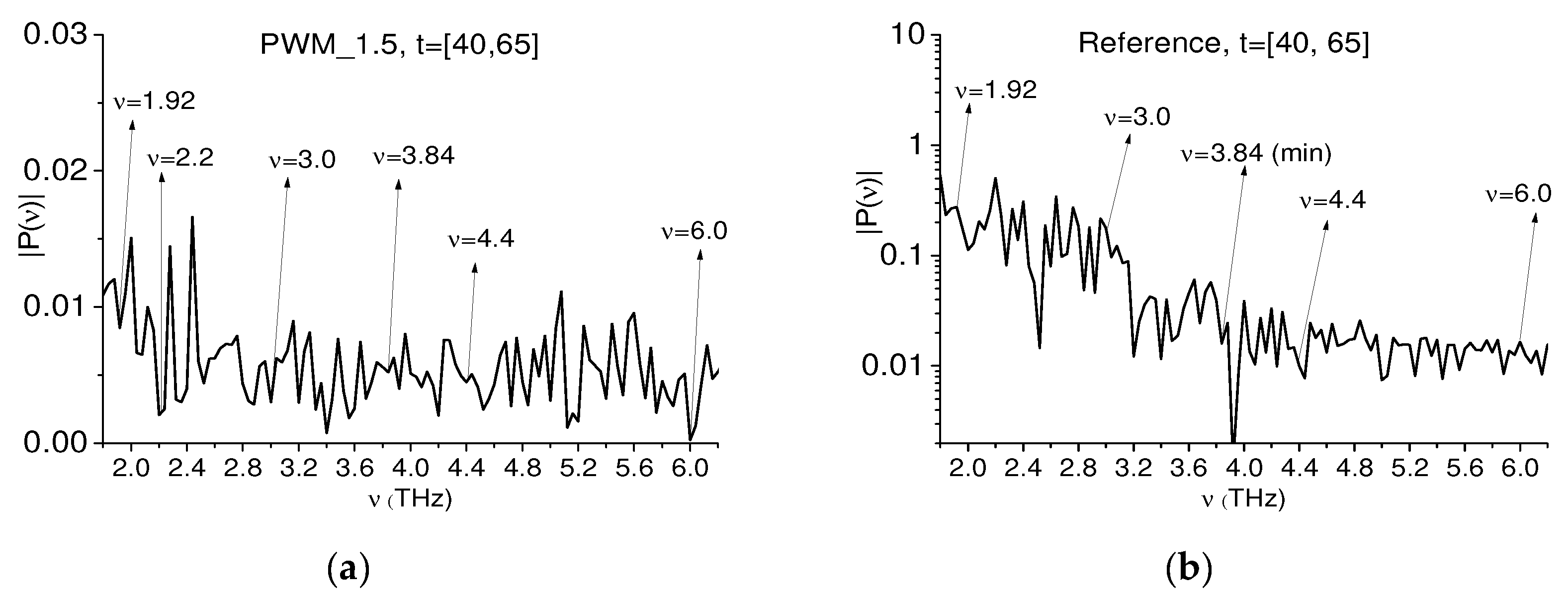
4.1.2. Finding Doubled Absorption Frequency Belonging to the First Sub-Pulse, t = [40, 65] ps
4.1.3. Observing Doubled Absorption Frequency in the Time Intervals after the First Sub-Pulse
4.1.4. Detection of the PWM C4 Substance with a Rough Surface Using a Doubled Absorption Frequency in Different Time Intervals
4.2. THz Signal Reflected from the Sample with Concave Surface
4.2.1. Observing the Doubled Absorption Frequency in the Main Pulse (Concave Surface)
4.2.2. Observing Doubled Absorption Frequency in the First Sub-Pulse (Concave Surface)
4.2.3. Observing Doubled Absorption Frequency in the Time Intervals after the First Sub-Pulse (Concave Surface)
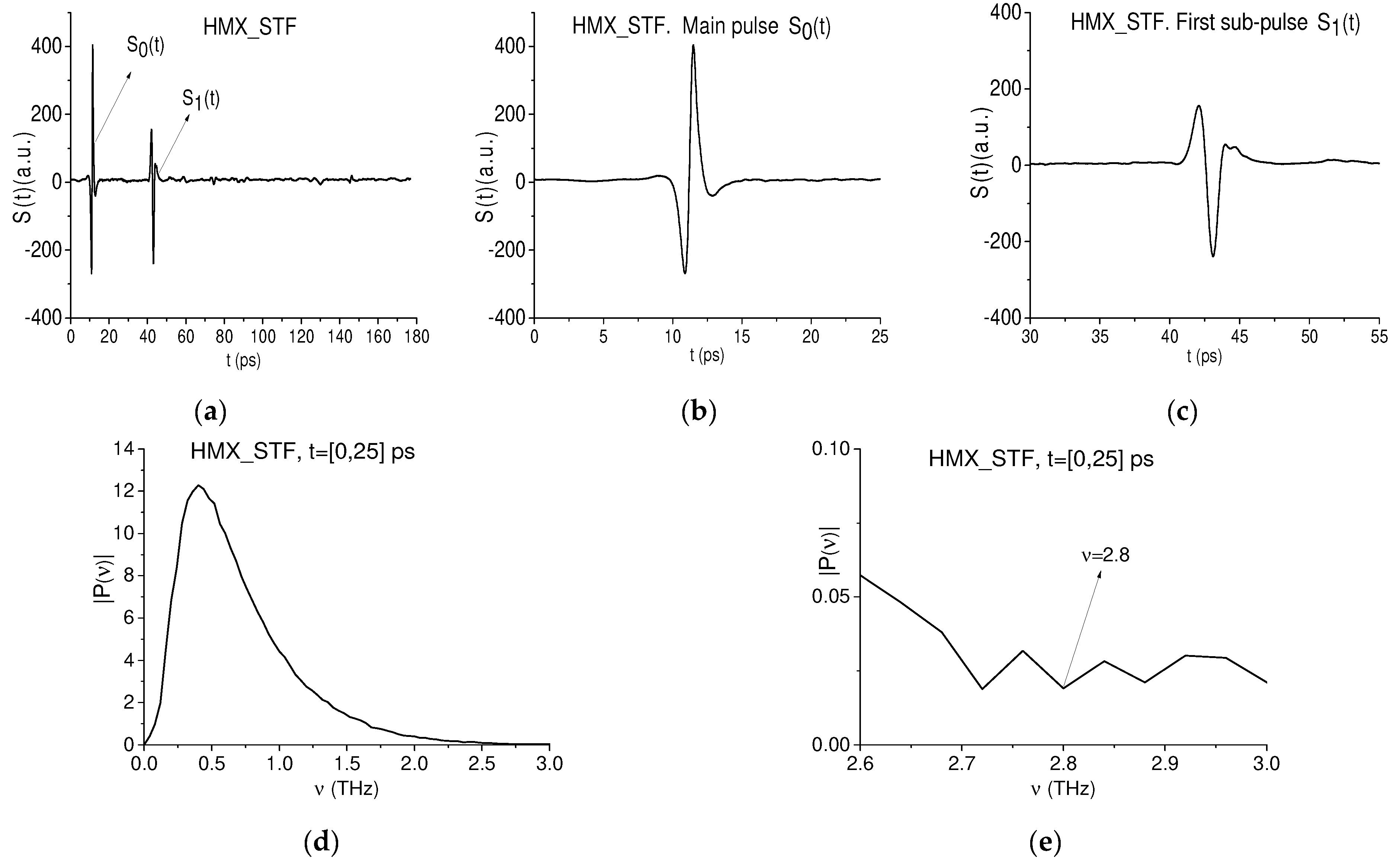
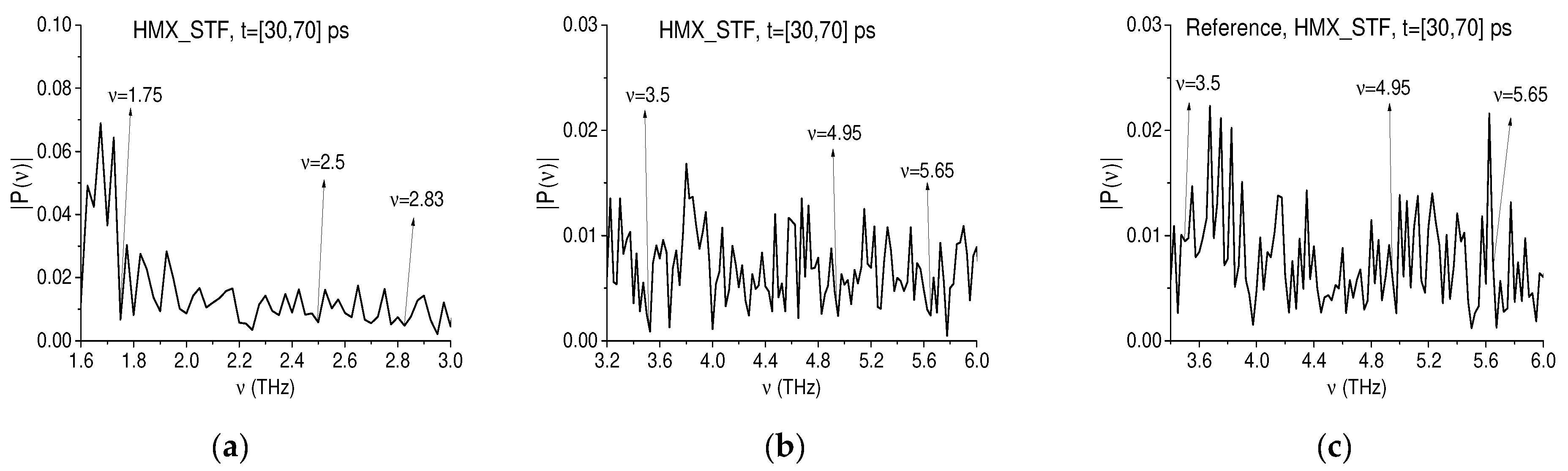
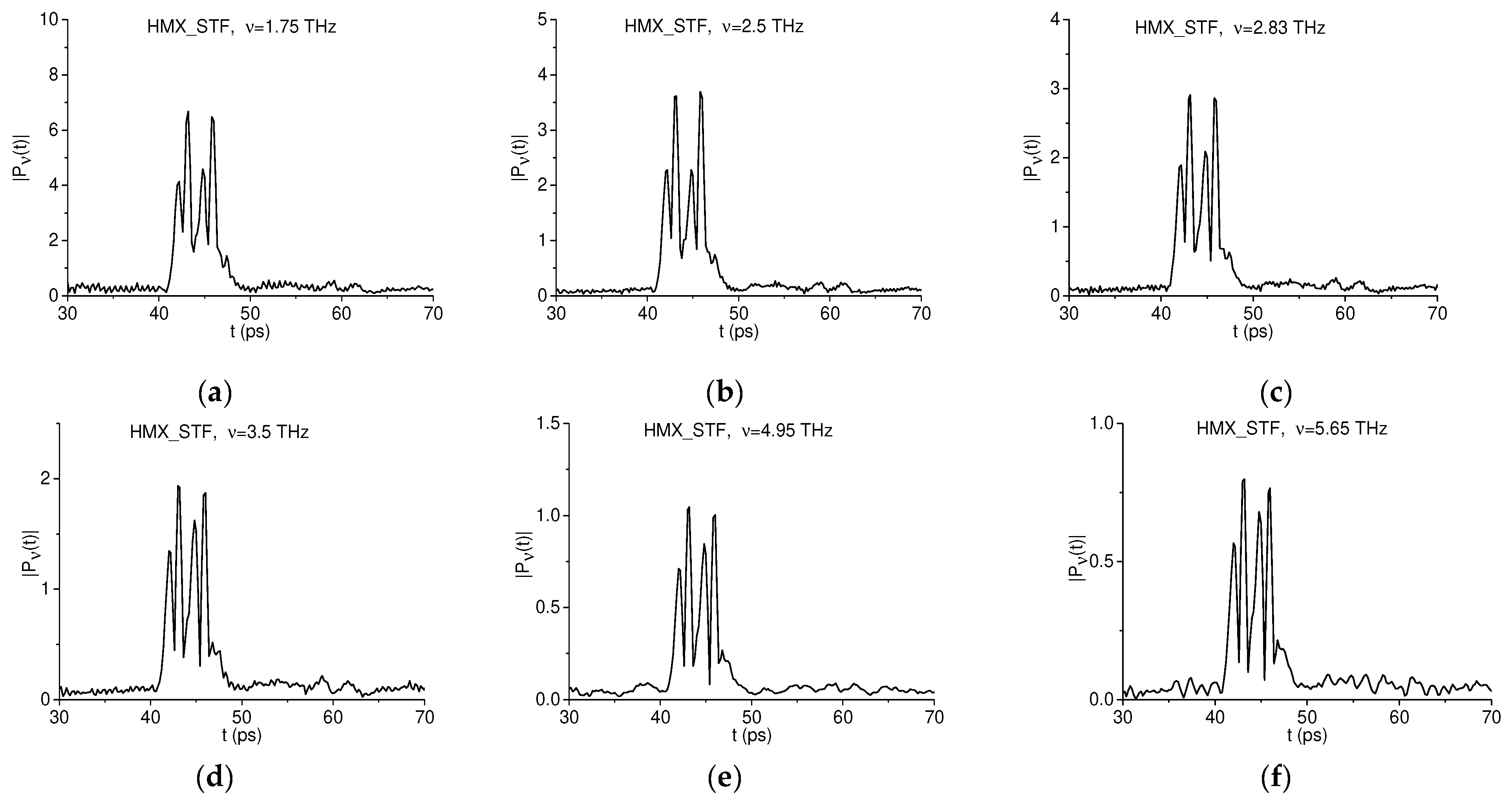
4.3. Observing Doubled Absorption Frequencies in the THz Pulse Reflected from the Substance HMX
4.4. Influence of Water Vapor on Observing Doubled Absorption Frequencies under Real Conditions
5. Discussion of the Characteristics of the Proposed Method for the Detection of a Substance
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Leahy-Hoppa, M.R.; Fitch, M.J.; Zheng, X.; Hayden, L.M.; Osiander, R. Wideband terahertz spectroscopy of explosives. Chem. Phys. Lett. 2007, 434, 227–230. [Google Scholar] [CrossRef]
- Chen, J.; Chen, Y.; Zhao, H.; Bastiaans, G.J.; Zhang, X.-C. Absorption coefficients of selected explosives and related compounds in the range of 0.1–2.8 THz. Opt. Express 2007, 15, 12060. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.B.; Zhong, H.; Karpowicz, N.; Chen, Y.; Zhang, X.C. Terahertz spectroscopy and imaging for defense and security applications. Proc. IEEE 2007, 95, 1514–1527. [Google Scholar] [CrossRef]
- Michalopoulou, Z.H.; Mukherjee, S.; Hor, Y.L.; Su, K.; Liu, Z.; Barat, R.B.; Gary, D.E.; Federici, J.F. RDX detection with THz spectroscopy. J. Infrared Millim. Terahertz Waves 2010, 31, 1171–1181. [Google Scholar] [CrossRef]
- Choi, K.; Hong, T.; Sim, K.I.; Ha, T.; Park, B.C.; Chung, J.H.; Cho, S.G.; Kim, J.H. Reflection terahertz time-domain spectroscopy of RDX and HMX explosives. J. Appl. Phys. 2014, 115, 023105. [Google Scholar] [CrossRef]
- Palka, N.; Szala, M. Transmission and reflection terahertz spectroscopy of insensitive melt-cast high-explosive materials. J. Infrared Millim. Terahertz Waves 2016, 37, 977–992. [Google Scholar] [CrossRef] [Green Version]
- He, T.; Shen, J.L. Applications of terahertz spectroscopy in illicit drugs detection. Spectrosc. Spect. Anal. 2013, 33, 2348–2353. [Google Scholar]
- Wang, C.H.; Terracciano, A.C.; Masunov, A.E.; Xu, M.; Vasu, S.S. Accurate prediction of terahertz spectra of molecular crystals of fentanyl and its analogs. Sci. Rep. 2021, 11, 4062. [Google Scholar] [CrossRef]
- McIntosh, A.I.; Yang, B.; Goldup, S.M.; Watkinson, M.; Donnan, R.S. Terahertz spectroscopy: A powerful new tool for the chemical sciences? Chem. Soc. Rev. 2012, 41, 2072–2082. [Google Scholar] [CrossRef]
- Nickel, D.V.; Ruggiero, M.T.; Korter, T.M.; Mittleman, D.M. Terahertz disorder-localized rotational modes and lattice vibrational modes in the orientationally-disordered and ordered phases of camphor. Phys. Chem. Chem. Phys. 2015, 17, 6671–7078. [Google Scholar] [CrossRef]
- Damari, R.; Weinberg, O.; Krotkov, D.; Demina, N.; Akulov, K.; Golombek, A.; Schwartz, T.; Fleischer, S. Strong coupling of collective intermolecular vibrations in organic materials at terahertz frequencies. Nat. Commun. 2019, 10, 3248. [Google Scholar] [CrossRef] [PubMed]
- D’Arco, A.; Di Fabrizio, M.; Dolci, V.; Petrarca, M.; Lupi, S. THz pulsed imaging in biomedical applications. Condens. Matter 2020, 5, 25. [Google Scholar] [CrossRef] [Green Version]
- Shen, Y.C. Terahertz pulsed spectroscopy and imaging for pharmaceutical applications: A review. Int. J. Pharm. 2011, 417, 48–60. [Google Scholar] [CrossRef] [PubMed]
- Kawase, K.; Shibuya, T.; Hayashi, S.I.; Suizu, K. THz imaging techniques for nondestructive inspections. Comptes Rendus Phys. 2010, 11, 510–518. [Google Scholar] [CrossRef]
- Ahi, K.; Anwar, M. Advanced terahertz techniques for quality control and counterfeit detection. Proc. SPIE 2016, 9856, 98560G. [Google Scholar]
- Ahi, K.; Shahbazmohamadi, S.; Asadizanjani, N. Quality control and authentication of packaged integrated circuits using enhanced-spatial-resolution terahertz time-domain spectroscopy and imaging. Opt. Lasers Eng. 2018, 104, 274–284. [Google Scholar] [CrossRef]
- Wang, K.; Sun, D.W.; Pu, H. Emerging non-destructive terahertz spectroscopic imaging technique: Principle and applications in the agri-food industry. Trends Food Sci. Technol. 2017, 67, 93–105. [Google Scholar] [CrossRef]
- Karaliūnas, M.; Nasser, K.E.; Urbanowicz, A.; Kašalynas, I.; Bražinskienė, D.; Asadauskas, S.; Valušis, G. Non-destructive inspection of food and technical oils by terahertz spectroscopy. Sci. Rep. 2018, 8, 18025. [Google Scholar] [CrossRef]
- Wang, C.; Zhou, R.; Huang, Y.; Xie, L.; Ying, Y. Terahertz spectroscopic imaging with discriminant analysis for detecting foreign materials among sausages. Food Control 2019, 97, 100–104. [Google Scholar] [CrossRef]
- Kemp, M.C. Explosives detection by terahertz spectroscopy—A bridge too far? IEEE Trans. Terahertz Sci. Technol. 2011, 1, 282–292. [Google Scholar] [CrossRef]
- Kato, M.; Tripathi, S.R.; Murate, K.; Imayama, K.; Kawase, K. Non-destructive drug inspection in covering materials using a terahertz spectral imaging system with injection-seeded terahertz parametric generation and detection. Opt. Express 2016, 24, 6425–6432. [Google Scholar] [CrossRef]
- Federici, J.F. Review of moisture and liquid detection and mapping using terahertz imaging. J. Infrared Millim. Terahertz Waves 2012, 33, 97–126. [Google Scholar] [CrossRef]
- Huang, Y.; Sun, P.; Zhang, Z.; Jin, C. Numerical method based on transfer function for eliminating water vapor noise from terahertz spectra. Appl. Opt. 2017, 56, 5698–5704. [Google Scholar] [CrossRef] [PubMed]
- Trofimov, V.A.; Varentsova, S.A. An effective method for substance detection using the broad spectrum THz signal: A “Terahertz nose”. Sensors 2015, 15, 12103–12132. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Trofimov, V.A.; Varentsova, S.A. Essential limitations of the standard THz TDS method for substance detection and identification and a way of overcoming them. Sensors 2016, 16, 502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Taday, P.F. Applications of terahertz spectroscopy to pharmaceutical sciences. Philos. Trans. A Math. Phys. Eng. Sci. 2004, 362, 351–364. [Google Scholar] [CrossRef] [PubMed]
- Chen, T.; Li, Z.; Hu, F.R.; Mo, W. Quantitative analysis of mixtures using terahertz time-domain spectroscopy and different PLS algorithms. Adv. Mater. Res. 2013, 804, 23–28. [Google Scholar] [CrossRef]
- Jiang, D.; Zhao, S.; Shen, J. Quantitative analysis of the mixtures of illicit drugs using terahertz time-domain spectroscopy. Proc. SPIE 2008, 6840, 68400U. [Google Scholar]
- Sleiman, J.B.; Perraud, J.B.; Bousquet, B.; Guillet, J.P.; Palka, N.; Mounaix, P. Discrimination and identification of RDX/PETN explosives by chemometrics applied to terahertz time-domain spectral imaging. Proc. SPIE 2015, 9651, 965109. [Google Scholar]
- Shen, Y.C.; Taday, P.F.; Pepper, M. Elimination of scattering effects in spectral measurement of granulated materials using terahertz pulsed spectroscopy. Appl. Phys. Lett. 2008, 92, 051103. [Google Scholar] [CrossRef] [Green Version]
- Henry, S.; Kniffin, G.; Schecklman, S.; Zurk, L.; Chen, A. Measurement and modeling of rough surface effects on terahertz spectroscopy and imaging. Proc. SPIE 2010, 7601, 760108. [Google Scholar]
- Malevich, V.L.; Sinitsyn, G.V.; Sochilin, G.B.; Rosanov, N.N. Manifestations of radiation scattering in the method of pulsed terahertz spectroscopy. Opt. Spectrosc. 2018, 124, 889–894. [Google Scholar] [CrossRef]
- Sheikh, F.; Gao, Y.; Kaiser, T. A study of diffuse scattering in massive MIMO channels at terahertz frequencies. IEEE Trans. Antennas Propag. 2019, 68, 997–1008. [Google Scholar] [CrossRef]
- Trofimov, V.A.; Zakharova, I.G.; Zagursky, D.Y.; Varentsova, S.A. New approach for detection and identification of substances using THz TDS. Proc. SPIE 2017, 10441, 1044107. [Google Scholar]
- Trofimov, V.A.; Zakharova, I.G.; Varentsova, S.A. New method of substance detection and identification using the substance emission frequency up-conversion in the THz frequency range. Proc. SPIE 2018, 10639, 106392C. [Google Scholar]
- Trofimov, V.A.; Wang, N.N.; Qiu, J.H.; Varentsova, S.A. Spurious absorption frequency appearance due to frequency conversion processes in pulsed THz TDS problems. Sensors 2020, 20, 1859. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Trofimov, V.A.; Varentsova, S.A.; Szustakowski, M.; Palka, N. Influence of surface of explosive on its detection and identification using the SDA method for analysis of the reflected THz signal. Proc. SPIE 2013, 8734, 87340Q. [Google Scholar]
- Trofimov, V.A.; Varentsova, S.A. High effective time-dependent THz spectroscopy method for the detection and identification of substances with inhomogeneous surface. PLoS ONE 2018, 13, e0201297. [Google Scholar] [CrossRef] [Green Version]
- Barsumian, B.R.; Jones, T.H. Pulse Transmitting Non-Linear Junction Detector. U.S. Patent 6,163,259, 19 December 2000. [Google Scholar]
- Holmes, S.J.; Stephen, A.B. Non-Linear Junction Detector. U.S. Patent 6,897,777, 24 May 2005. [Google Scholar]
- Mazzaro, G.J.; Martone, A.F.; McNamara, D.M. Detection of RF electronics by multitone harmonic radar. IEEE Trans. Aerosp. Electron. Syst. 2014, 50, 477–490. [Google Scholar] [CrossRef]
- Aniktar, H.; Baran, D.; Karav, E.; Akkaya, E.; Birecik, Y.S.; Sezgin, M. Getting the bugs out: A portable harmonic radar system for electronic countersurveillance applications. IEEE Microw. Mag. 2015, 16, 40–52. [Google Scholar] [CrossRef]
- Aniktar, H.; Baran, D. An efficient implementation of intermodulation radar with an integrated EMI sensor. IEEE Sens. J. 2021, 21, 23492–23497. [Google Scholar] [CrossRef]
- Information Security Associates. Boomerang Series Non-Linear Junction Detector Data Sheet. Available online: http://isa-technology.com/products/items/boomarang4.html (accessed on 8 April 2022).
- Trofimov, V.A.; Varentsova, S.A.; Zakharova, I.G.; Zagursky, D.Y. Conservative finite-difference scheme for the problem of THz pulse interaction with multilevel layer covered by disordered structure based on the density matrix formalism and 1D Maxwell equation. PLoS ONE 2018, 13, e0201572. [Google Scholar] [CrossRef] [PubMed]
- Boyd, R.W. Nonlinear Optics, 3rd ed.; Academic Press: Cambridge, MA, USA, 2008; p. 611. [Google Scholar]
- Zhang, X.C.; Xu, J. Introduction to THz Wave Photonics; Springer: Berlin/Heidelberg, Germany, 2010; p. 330. [Google Scholar]
- Loata, G.C.; Thomson, M.D.; Loffler, T.; Roskos, H.G. Radiation field screening in photoconductive antennae studied via pulsed terahertz emission spectroscopy. Appl. Phys. Lett. 2007, 91, 232506. [Google Scholar] [CrossRef]
- Siegman, A.E. Laser; University Science Books: Mill Valley, CA, USA, 1986. [Google Scholar]
- Agrawal, G.P. Optical pulse propagation in doped fiber amplifiers. Phys. Rev. A 1991, 44, 7493–7501. [Google Scholar] [CrossRef] [PubMed]
- Trofimov, V.A.; Zakharova, I.G.; Zagursky, D.Y.; Varentsova, S.A. Detection and identification of substances using noisy THz signal. Proc. SPIE 2017, 10194, 101942O. [Google Scholar]
- Hofmann, M.C.; Brant, N.C.; Hwang, H.Y.; Yeh, K.-L.; Nelson, K.A. Terahertz Kerr effect. Appl. Phys. Lett. 2009, 95, 231105. [Google Scholar] [CrossRef] [Green Version]
- Lengyel, K.; Péter, Á.; Kovács, L.; Corradi, G.; Pálfalvi, L.; Hebling, J.; Unferdorben, M.; Dravecz, G.; Hajdara, I.; Szaller, Z.; et al. Growth, defect structure, and THz application of stoichiometric lithium niobite. Appl. Phys. Rev. 2015, 2, 040601. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.C.; Shkurinov, A.; Zhang, Y. Extreme terahertz science. Nat. Photonics 2017, 11, 16–18. [Google Scholar] [CrossRef]
- Landau, L.D.; Akhiezer, A.I.; Lifshitz, E.M. General Physics: Mechanics and Molecular Physics; Pergamon Press: Oxford, UK; New York, NY, USA, 1967; p. 372. [Google Scholar]
- TeraView Corporation. Available online: www.teraview.com (accessed on 9 May 2022).
- Trofimov, V.A.; Varentsova, S.A. Detection and identification of drugs under real conditions by using noisy terahertz broadband pulse. Appl. Opt. 2016, 55, 9605–9618. [Google Scholar] [CrossRef]
- HITRAN Database. Available online: www.hitran.org (accessed on 8 April 2022).
- Leary, P.E.; Kammrath, B.W.; Lattman, K.J.; Beals, G.L. Deploying portable gas chromatography—Mass spectrometry (GC-MS) to military users for the identification of toxic chemical agents in theater. Appl. Spectrosc. 2019, 73, 841–858. [Google Scholar] [CrossRef]
- Chen, K.; Liu, S.; Zhang, B.; Gong, Z.; Chen, Y.; Zhang, M.; Deng, H.; Guo, M.; Ma, F.; Zhu, F.; et al. Highly sensitive photoacoustic multi-gas analyzer combined with mid-infrared broadband source and near-infrared laser. Opt. Lasers Eng. 2020, 124, 105844. [Google Scholar] [CrossRef]
- Trofimov, V.A.; Varentsova, S.A. About efficiency of identification of materials using spectrum dynamics of medium response under the action of THz radiation. Proc. SPIE 2009, 7311, 73110U. [Google Scholar]

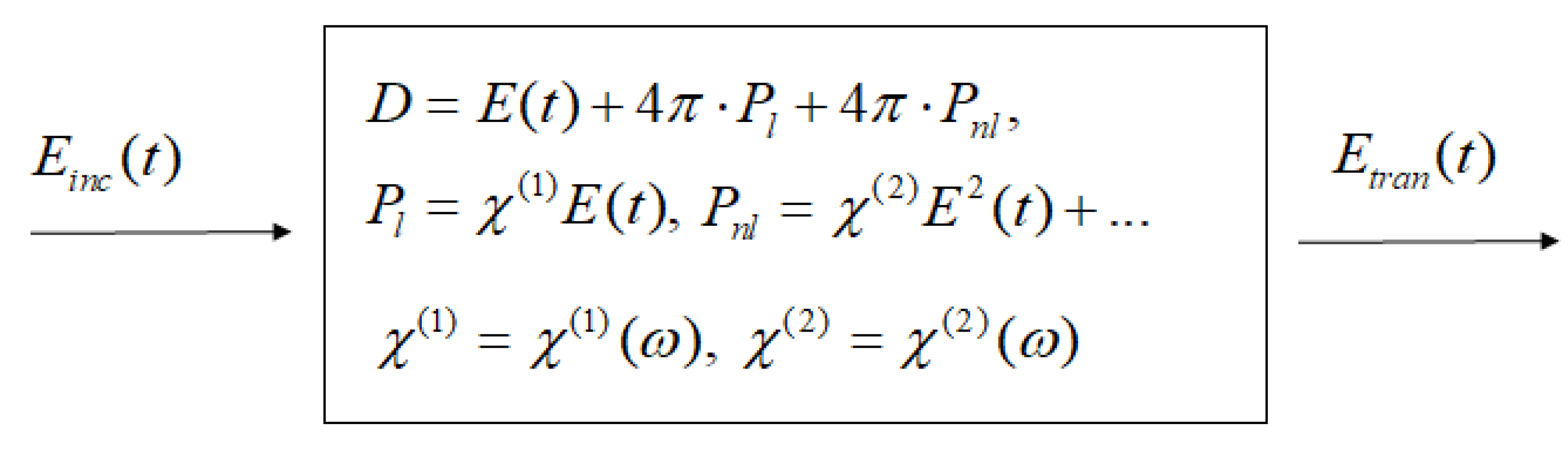
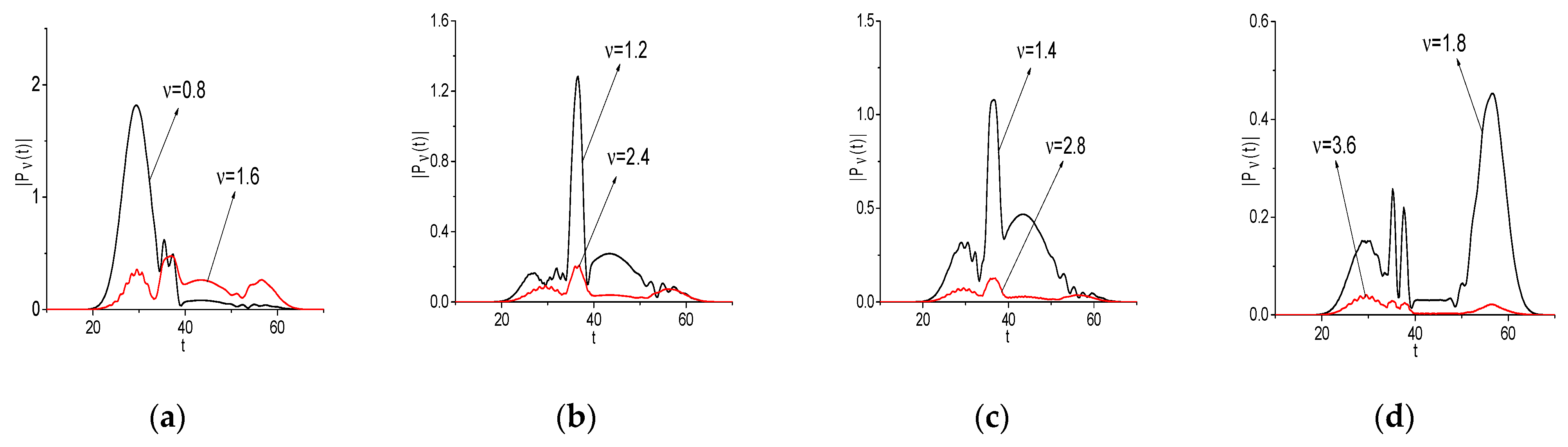
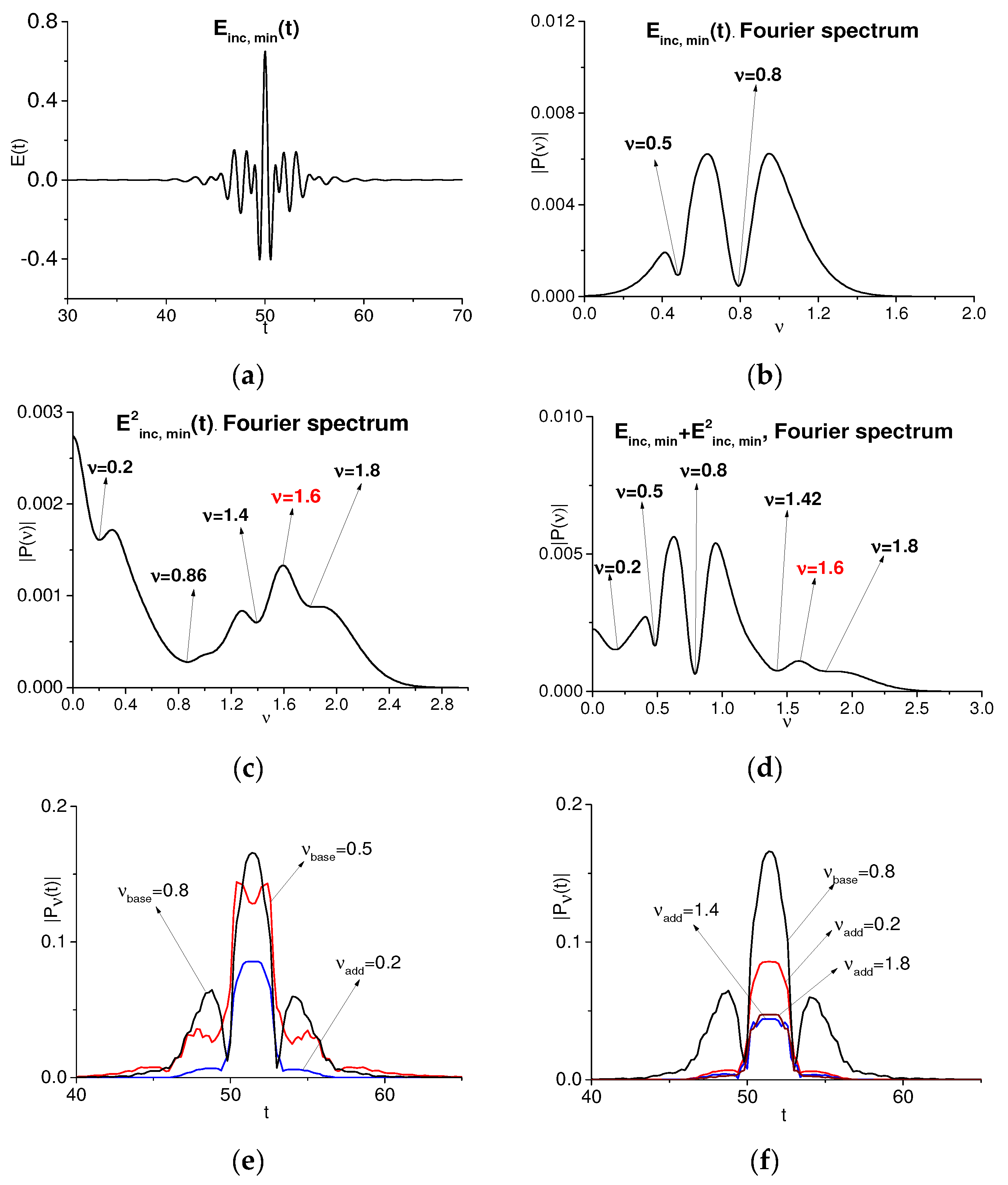
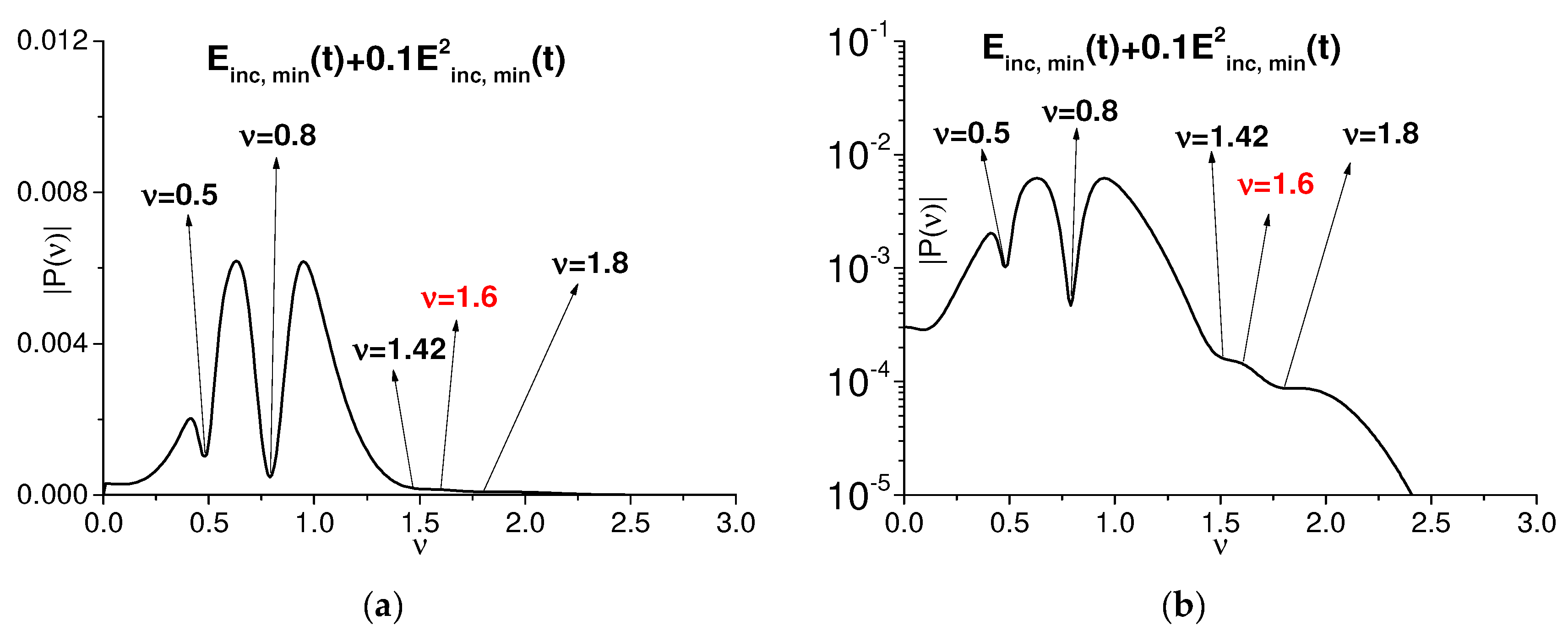
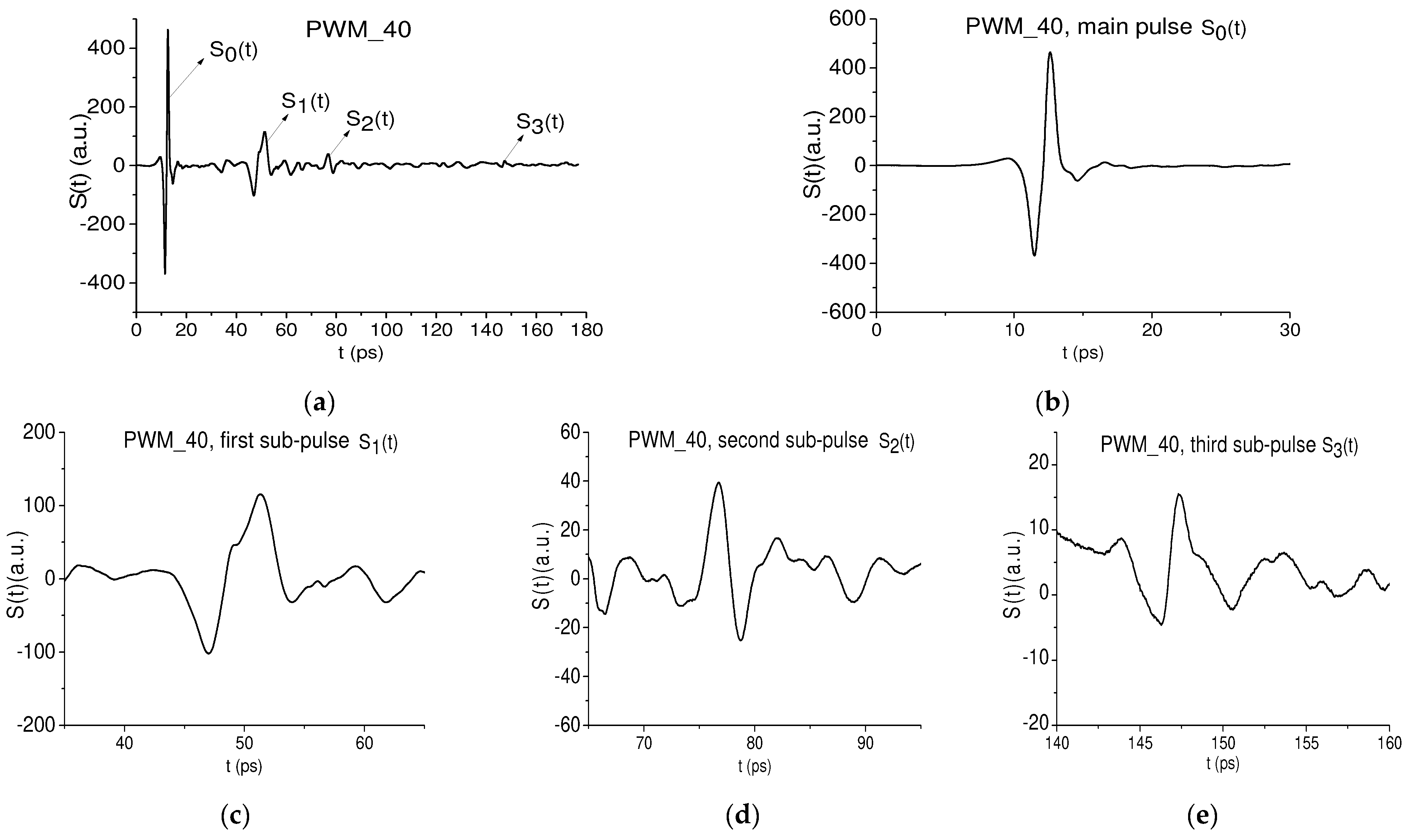




| A1 | A2 | A3 | A4 | t1 | t2 | t3 | t4 | τ1 | τ2 | τ3 | τ4 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 4.0 | 4.0 | 1.0 | 1.0 | 28.0 | 35.0 | 42.0 | 55.0 | 8.0 | 2.0 | 16.0 | 8.0 |
| 0.5 | 0.8 | 0.8 | 0.8 | 0.8 | |
| 0.2 | 0.2 | 1.4 | 1.8 | 1.6 | |
| 0.925 | 0.925 | 0.923 | 0.916 | 0.922 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trofimov, V.A.; Varentsova, S.A.; Yang, Y.; Cai, Z. Substance Detection and Identification Using Frequency Doubling of the THz Broadband Pulse. Chemosensors 2022, 10, 275. https://doi.org/10.3390/chemosensors10070275
Trofimov VA, Varentsova SA, Yang Y, Cai Z. Substance Detection and Identification Using Frequency Doubling of the THz Broadband Pulse. Chemosensors. 2022; 10(7):275. https://doi.org/10.3390/chemosensors10070275
Chicago/Turabian StyleTrofimov, Vyacheslav A., Svetlana A. Varentsova, Yongqiang Yang, and Zihao Cai. 2022. "Substance Detection and Identification Using Frequency Doubling of the THz Broadband Pulse" Chemosensors 10, no. 7: 275. https://doi.org/10.3390/chemosensors10070275
APA StyleTrofimov, V. A., Varentsova, S. A., Yang, Y., & Cai, Z. (2022). Substance Detection and Identification Using Frequency Doubling of the THz Broadband Pulse. Chemosensors, 10(7), 275. https://doi.org/10.3390/chemosensors10070275







