Calculation of Two Types of Quaternion Step Derivatives of Elementary Functions
Abstract
1. Introduction
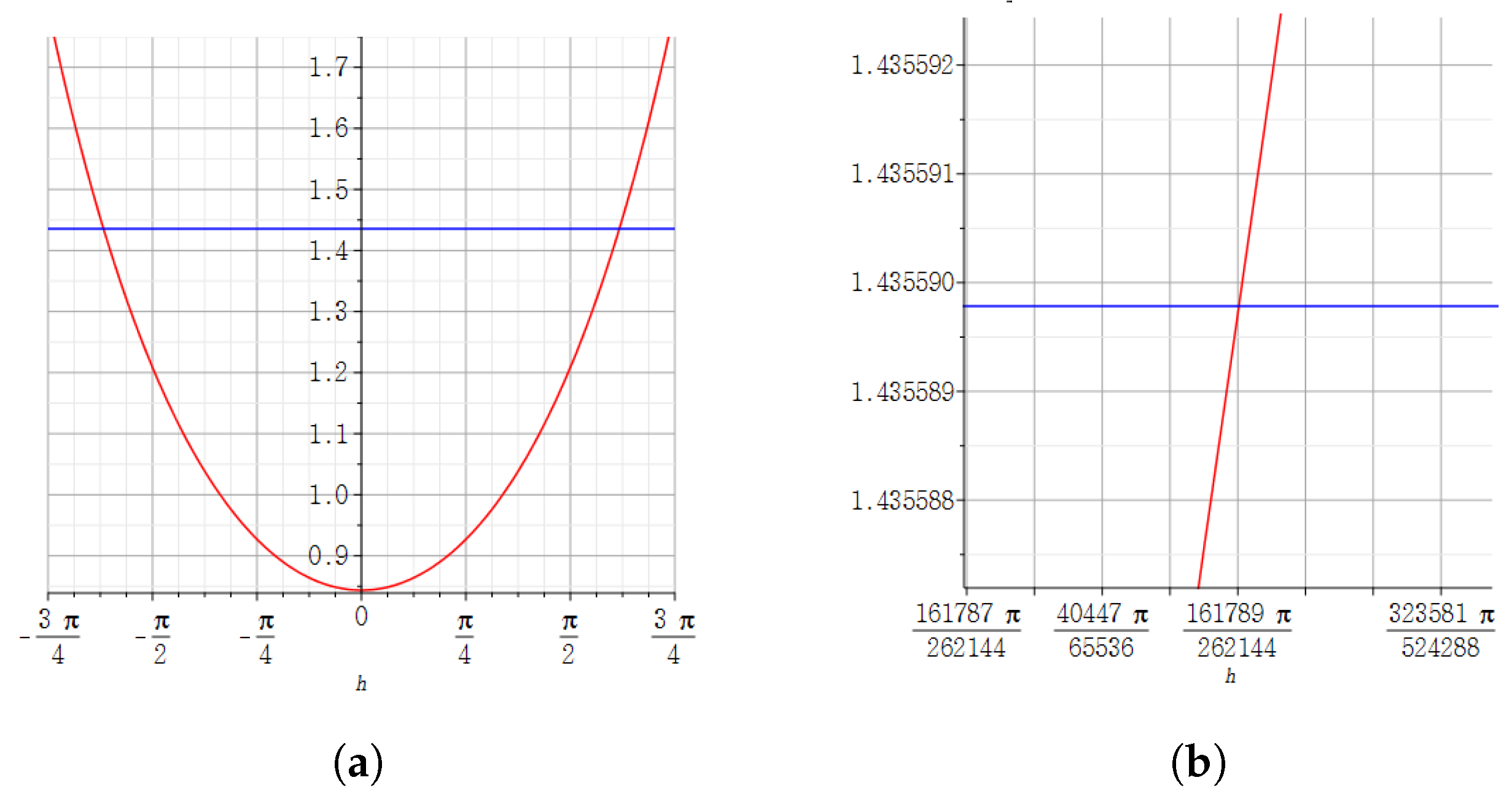
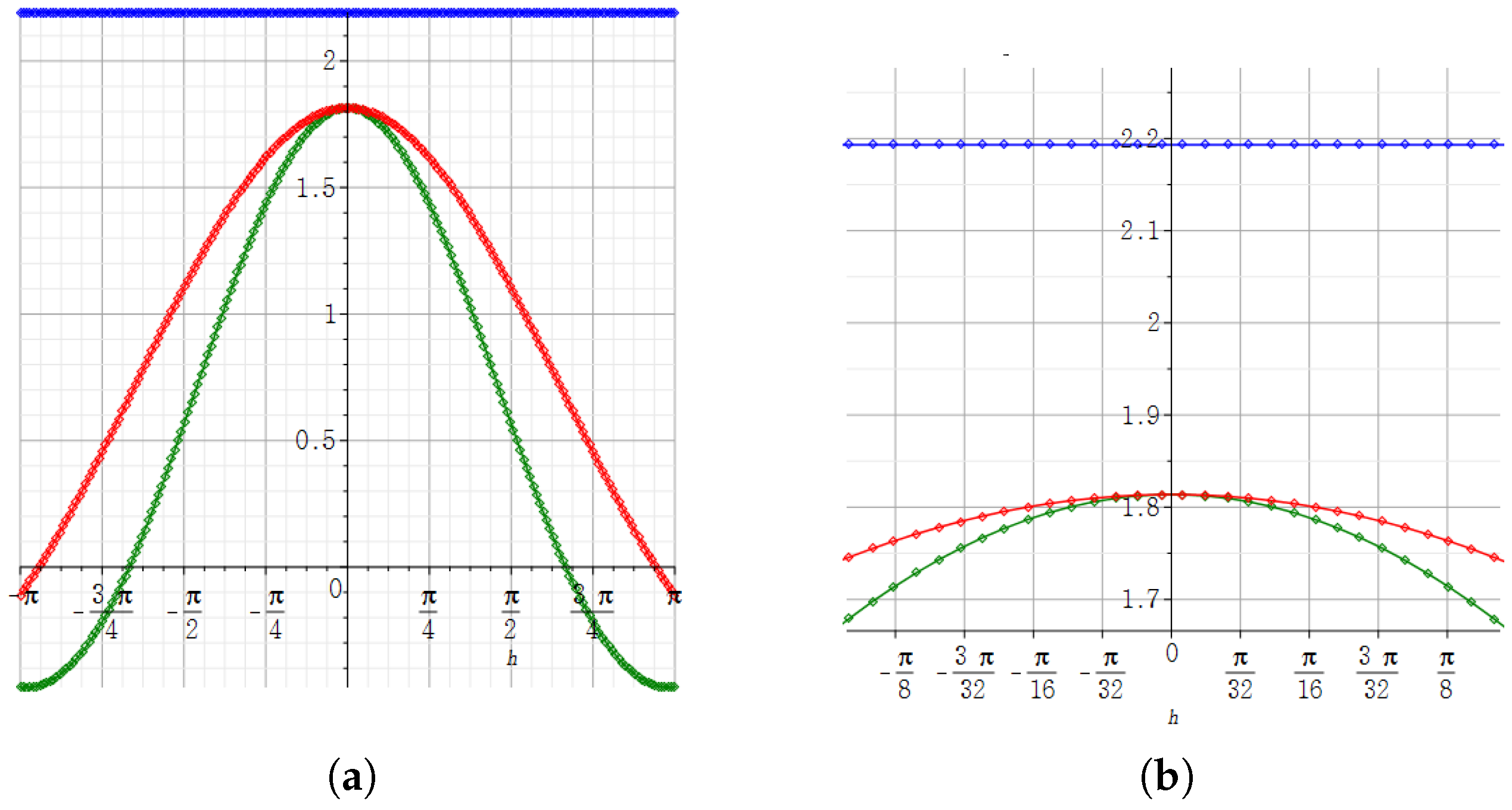
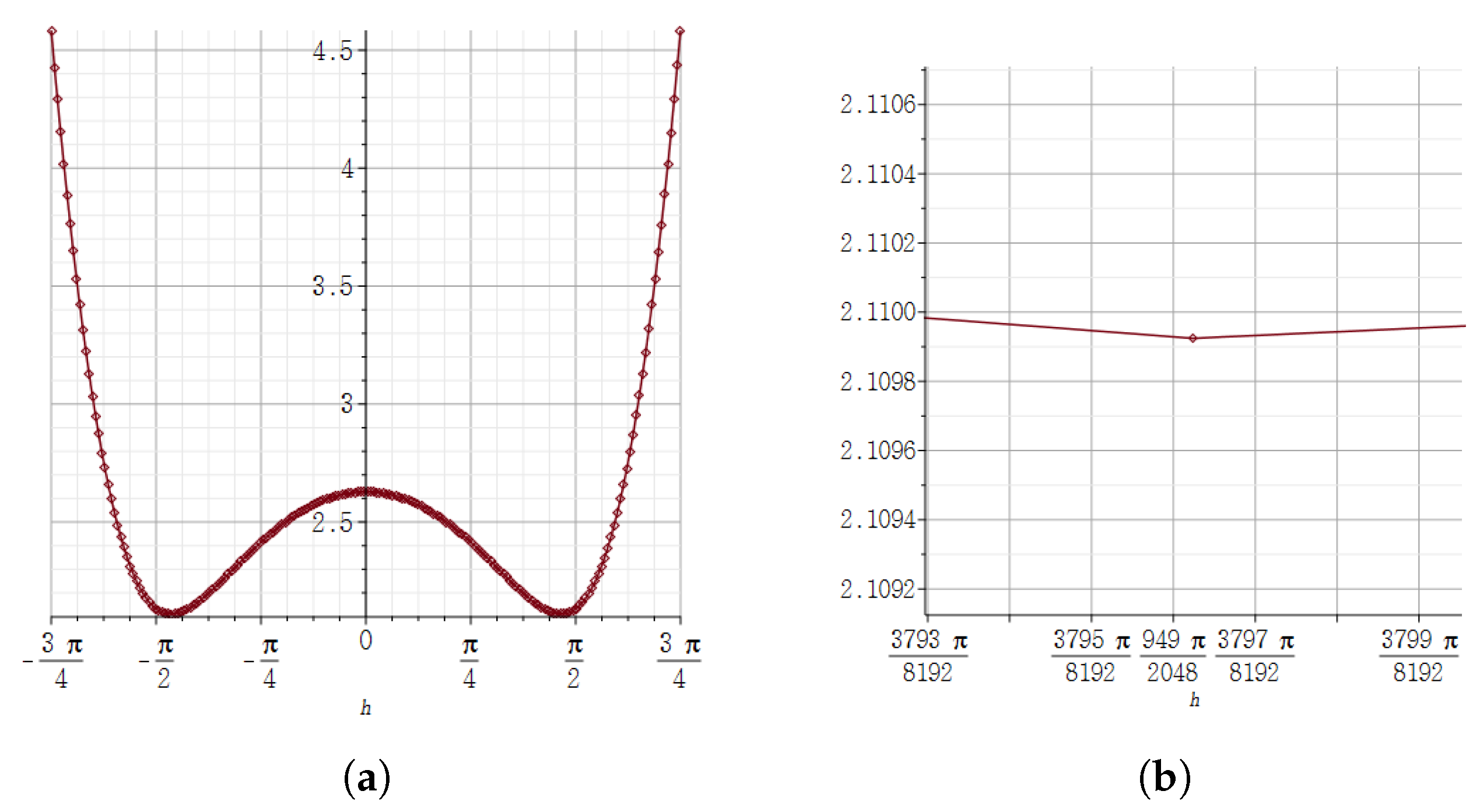
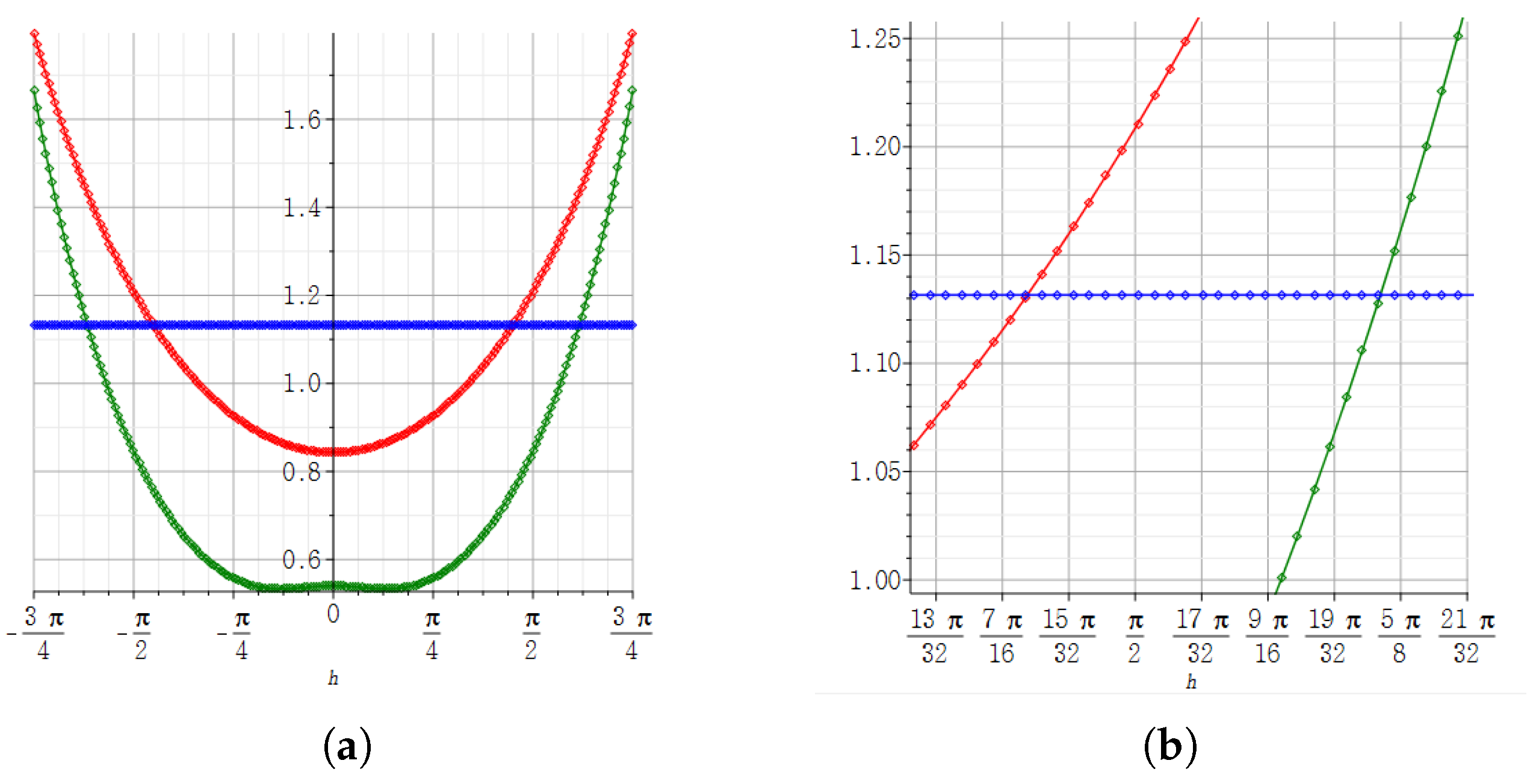
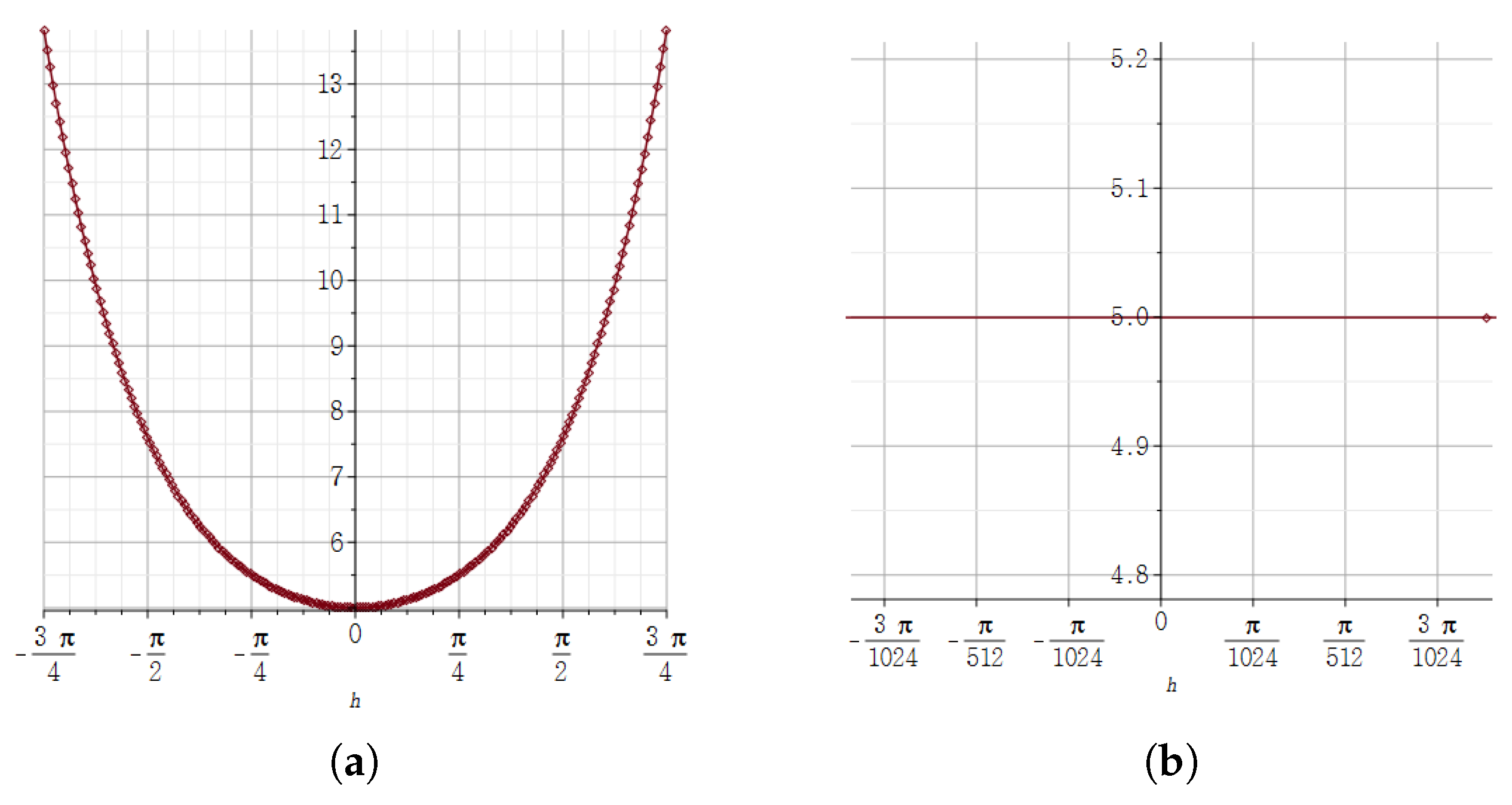
2. Quaternion -Step Derivative
3. Quaternion -Step Derivative
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Martins, J.R.; Sturdza, P.; Alonso, J.J. The connection between the complex-step derivative approximation and algorithmic differentiation. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 8–11 January 2001. [Google Scholar]
- Martins, J.R.; Sturdza, P.; Alonso, J.J. The complex-step derivative approximation. ACM Trans. Math. Softw. 2003, 29, 142–149. [Google Scholar] [CrossRef]
- Agarwal, R.P.; Bohner, M. Basic calculus on time scales and some of its applications. Res. Math. 1999, 35, 3–22. [Google Scholar] [CrossRef]
- Agarwal, R.; Belmekki, M.; Benchohra, M. A survey on semilinear differential equations and inclusions involving Riemann-Liouville fractional derivative. Adv. Diff. Equ. 2009, 2009, 1–47. [Google Scholar] [CrossRef]
- Lyness, J.N.; Moler, C.B. Numerical differentiation of analytic functions. SIAM J. Numer. Anal. 1967, 4, 202–210. [Google Scholar] [CrossRef]
- Lyness, J.N. Numerical algorithms based on the theory of complex variable. In Proceedings of the ACM National Meeting (Washington, D.C.); ACM: New York, NY, USA, 1967; pp. 125–133. [Google Scholar]
- Squire, W.; Trapp, G. Using complex variables to estimate derivatives of real functions. SIAM Rev. 1998, 40, 110–112. [Google Scholar] [CrossRef]
- Newman, J.C.; Anderson, W.K.; Whitfield, L.D. Multidisciplinary Sensitivity Derivatives Using Complex Variables; Tech. Rep. MSSU-COE-ERC-98-08; Computational Fluid Dynamics Laboratory: Seoul, Korea, 1998. [Google Scholar]
- Newman, J.C., III; WhitZ̆field, D.L.; Anderson, W.K. Step-size independent approach for multidisciplinary sensitivity analysis. J. Aircr. 2003, 40, 566–573. [Google Scholar] [CrossRef]
- Lai, K.L.; Crassidis, J. Generalizations of the complex-step derivative approximation. In Proceedings of the AIAA Guidance, Navigation, and Control Conference and Exhibit, Keystone, CO, USA, 21–24 August 2006. [Google Scholar]
- Lai, K.L.; Crassidis, J.L. Extensions of the first and second complex-step derivative approximations. J. Comput. Appl. Math. 2008, 219, 142–149. [Google Scholar] [CrossRef]
- Cossette, C.C.; Walsh, A.; Forbes, J.R. The Complex-Step Derivative Approximation on Matrix Lie Groups. IEEE Robot. Autom. Lett. 2020, 5, 906–913. [Google Scholar] [CrossRef]
- Kumar, K. Expansion of a function of noncommuting operators. J. Math. Phys. 1965, 1965 6, 1923–1927. [Google Scholar] [CrossRef]
- Lantoine, G.; Russell, R.P.; Dargent, T. Using multicomplex variables for automatic computation of high-order derivatives. ACM Trans. Math. Softw. (TOMS) 2012, 38, 1–21. [Google Scholar] [CrossRef]
- Turner, J. Quaternion-based partial derivative and state transition matrix calculations for design optimization. In Proceedings of the 40th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 14–17 January 2002. [Google Scholar]
- Kim, J.E.; Lim, S.J.; Shon, K.H. Regularity of functions on the reduced quaternion field in Clifford analysis. Abstr. Appl. Anal. 2014, 2014. [Google Scholar] [CrossRef]
- Kim, J.E.; Lim, S.J.; Shon, K.H. Taylor series of functions with values in dual quaternion. Pure Appl. Math. 2013, 20, 251–258. [Google Scholar] [CrossRef][Green Version]
- Kim, J.E. The corresponding inverse of functions of multidual complex variables in Clifford analysis. J. Nonlinear Sci. Appl. 2016, 9, 4520–4528. [Google Scholar] [CrossRef][Green Version]
- Roelfs, M.; Dudal, D.; Huybrechs, D. Quaternionic Step Derivative: Automatic Differentiation of Holomorphic Functions using Complex Quaternions. arXiv 2020, arXiv:2010.09543. [Google Scholar]
- Brown, J.W.; Churchill, R.V. Complex Variables and Applications, 8th ed.; McGraw-Hill Book Company: New York, NY, USA, 2009; Chapter 3. [Google Scholar]
- Georgiev, S.; Morais, J.; Spröss, W. New aspects on elementary functions in the context of quaternionic analysis. Cubo (Temuco) 2012, 14, 93–110. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.E. Calculation of Two Types of Quaternion Step Derivatives of Elementary Functions. Mathematics 2021, 9, 668. https://doi.org/10.3390/math9060668
Kim JE. Calculation of Two Types of Quaternion Step Derivatives of Elementary Functions. Mathematics. 2021; 9(6):668. https://doi.org/10.3390/math9060668
Chicago/Turabian StyleKim, Ji Eun. 2021. "Calculation of Two Types of Quaternion Step Derivatives of Elementary Functions" Mathematics 9, no. 6: 668. https://doi.org/10.3390/math9060668
APA StyleKim, J. E. (2021). Calculation of Two Types of Quaternion Step Derivatives of Elementary Functions. Mathematics, 9(6), 668. https://doi.org/10.3390/math9060668




