Designing Tasks for a Dynamic Online Environment: Applying Research into Students’ Difficulties with Linear Equations

Abstract

Despite almost half a century of research into students’ difficulties with solving linear equations, these difficulties persist in everyday mathematics classes around the world. Furthermore, the difficulties reported decades ago are the same ones that persist today. With the immense number of dynamic online environments for mathematics teaching and learning that are emerging today, we are presented with a perhaps unique opportunity to do something about this. This study sets out to apply the research on lower secondary school students’ difficulties with equation solving, in order to eventually inform students’ personalised learning through a specific task design in a particular dynamic online environment (matematikfessor.dk). In doing so, task design theory is applied, particularly variation theory. The final design we present consists of eleven general equation types—ten types of arithmetical equations and one type of algebraic equation—and a broad range of variations of these, embedded in a potential learning-trajectory-tree structure. Besides establishing this tree structure, the main theoretical contribution of the study and the task design we present is the detailed treatment of the category of arithmetical equations, which also involves a new distinction between simplified and non-simplified arithmetical equations.

1. Introduction

Dynamic online environments for teaching and learning mathematics are emerging all over the world. These online environments feature everything from video lectures to adaptive, gamified quizzes, and vary from simple training tasks to complex modelling tasks. A dynamic online environment is an online platform (or portal) where students may study mathematics in various ways. Initially, environments such as these consisted of just video lectures on various subjects or were gateways that provided access to a large number of training tasks to supplement the physical or electronic books used in the classroom. Recently, however, platforms have been developed where teachers and students interact on homework assignments, do differentiated group tasks, and so forth [1].

Dynamic online environments have the advantage of both offering access and having the capacity to process data provided in the form solutions to tasks. Such data may be, and is often advertised as, providing students with a highly streamlined experience in the environment, and an opportunity for a guided experience that leads to improved greater learning outcomes. Providing the user with an individualised learning path may be referred to as personalised learning. Personalised learning is an emerging concept that builds on the idea that no one, fixed learning path is suited to all learners [2]. However, according to Chen [2], one problem is that personalised e-learning systems tend to not consider whether a learner’s ability and the difficulty level of the recommended courseware match each other. Chen [2] emphasises that learner ability should be considered when attempting to develop personalised learning. With regard to mathematics, learner ability may be interpreted in terms of the level of understanding of certain mathematical concepts related to a given situation.

To develop personalised learning paths in the field of linear equations, one must attempt to measure the student’s ability or understanding of the concepts in this field. In this paper, we take a look at how dynamic online environments may benefit from the many years of mathematics education research through task design and collected data concerning students’ work with linear equations, in order to promote personalised learning by determining learner ability when solving linear equations.

The task design principles presented in this paper yielded a collection of linear equations that target lower secondary school students. This collection of equations is intended to cover the intersection of the set of equations that may or ought to appear in the lower secondary school curriculum, and the set of equations highlighted in the literature as presenting difficulty to lower secondary school students. When addressing these design principles, we apply an adapted definition of the two types of difficulty that Jankvist and Niss associate with the concept of the linear equation [3]:

‘The first kind of difficulty... is to do with goal-oriented transformation of equations (and, more fundamentally, algebraic expressions) into equivalent ones by way of permissible operations. [...] The second kind of difficulty, which appears to be of a more fundamental nature, is to do with what an equation actually is, and with what is meant by a solution to it.’

[3] (p. 276)

By gaining a strong understanding of the prevalence of the concrete difficulties associated with seemingly common training tasks involving equations, one may find new possibilities related to both personalised learning and formative assessment. Feedback is a crucial part of task design in general [4]. The long term aim of the present design under discussion is to not only provide feedback on individual tasks, but also to offer the teacher additional refined feedback and auto-generated suggestions for how a student might improve their set of equation-solving strategies and knowledge related to the various mathematical concepts involving linear equations.

1.1. Matematikfessor.dk—A Dynamic Online Learning Environment

In Denmark, the dynamic online environment, matematikfessor.dk (Danish slang for ‘mathematics professor’) has existed for 10 years, and has approximately 500,000 student users who pay for access. To contextualise this, Denmark has a total of 700,000 students in primary school and lower secondary school, which means that over 70% of Danish school students have access to, and use the content on matematikfessor.dk. On an average day, 45,000 unique Danish students use the variety of tasks offered by the site, and collectively provide solutions to 1,500,000 tasks. This means that on an average day, almost 10% of the user base accesses this online environment. Every day, 45,000 of the 500,000 student users complete 33 tasks, on average. During the average Danish school year, approximately 300,000,000 tasks are completed on matematikfessor.dk. To illustrate how widespread the use of this environment is, when Denmark was locked down on 11 March 2020, owing to COVID-19, the number of tasks completed daily rose to approximately 6,000,000. The number of unique daily visitors on a standard weekday was approximately 45,000–50,000. The number of unique daily logins rose to 130,000.

The dynamic aspect of the platform consists in the platform’s ability to learn certain things about the students. When a student provides a solution to a task, the system (an algorithm) may present the student the next task based on this solution (as well as previous solutions). As an example, the equations presented in this study could be assigned to a student in a dynamic manner. This is done by the platform (system) learning what types of equations and which variations the student is able to solve. On the one hand, the system can present the student with tasks that are challenging in relation to level and performance. On the other hand, the system can gather information about the student’s ability as an equation solver, and thereby potentially inform the student’s teacher in relation to future learning trajectories. This dynamism is what leads to the enabling of personalised learning.

Dynamic online environments are subject to several obvious characteristics. The platform’s content may dynamically change; new tasks may be added to the environment and old tasks may be removed or updated. This provides the editors of online environments with the added flexibility of dynamically adapting it to the needs of its user base. These parameters give online environments a clear advantage over printed teaching material materials such as textbooks. Another characteristic of online learning environments is that teachers may assign tasks from the online environment to the students individually, including deadlines, monitoring the solutions and providing feedback. Many online environments also provide the students with auto-generated feedback and suggestions for how they could have completed a given task, for example, if a wrong solution is submitted. This feedback may vary, and may provide the student with the correct solution to a detailed explanation of how the task should (could) be correctly completed.

Although some of the disadvantages of using dynamic online environments may be obvious, others are more hidden. A teacher may have certain beliefs regarding how a subject and its concepts should be explained or taught. When presenting video lectures in an online environment, one may fear that students are merely presented with algorithms that solve a textbook problem, and not with a general strategy for solving a whole class of problems. Other—perhaps less obvious—disadvantages are the consequences of particular task designs. As the online environment is expected to provide instant feedback, the user is allowed only certain input types. Some problems would require a student to justify an assumption or in some way provide a string of text, or even spoken words. Most of the tasks presented in matematikfessor.dk are what many would refer to as training tasks. Therefore, in most cases the two types of input that students may provide are either responses to multiple-choice questions, or numbers. In task design, this becomes a significant disadvantage, particularly when working with algebraic expressions. It means that it is not possible to prompt students for an algebraic expression without presenting the student with multiple choices. One might argue that this is acceptable in many cases. Nevertheless, it forces upon the task designer an additional objective, namely, to create distractors. Preferably, such distractors should be chosen or designed based on scientific or experiential results that qualify the answer as a good distractor. One could argue that for a student, a poorly designed wrong solution would make a task easier or ‘bad’—and in most cases, more problematic—in terms of conducting research.

1.2. Research Question

The preceding discussion led us to the following research question:

How may research on lower secondary school students’ difficulties with linear equations inform task design in a dynamic online environment with the possibility to promote/support students’ personalised learning?

2. Methodology Part 1: Finding Key Publications

With regard to conducting a review of the literature concerning the difficulties lower secondary school students encounter when working with linear equations, the reader should be aware of the sheer volume of the body of mathematics education literature concerning students’ difficulties with algebra. Although this review mainly seeks to uncover the difficulties that arise when students work with equations and attempt to solve them, many of these difficulties have a natural relationship to the subject of algebra. Hence, it became clear that a strategy focused solely on a database search would be neither sufficient nor possible to accomplish. Instead, the literature review relies on parts of the hermeneutic approach [5]. This means that the strategy was not to apply a systematic approach that covers all the literature on this subject, but to use initial database search iterations, and handbooks and existing literature reviews, to pave the way for a suitable citation-tracking—snowballing—approach to identify key publications that address the difficulties encountered when working with equations [5]. Several major literature reviews on children’s difficulties with algebra have been conducted over the years. Snowballing based on database searches made it possible to identify a number of extensive literature reviews on difficulties with algebra. Similarly, it was possible to identify key publications that described students’ difficulties with linear equations in chapters in the leading handbooks on mathematics education. Finally, the advice and knowledge of experts in the field of mathematics education has been valuable.

The database searches were carried out using the MathEduc (note that MathEduc is no longer operational: https://www.zentralblatt-math.org/matheduc/, autumn 2018) and ERIC (https://eric.ed.gov/) databases, with various search strings containing keywords such as ‘equation’, ‘arithmetic’, ‘algebra’, ‘misconception’, ‘difficulties’ and ‘error’, which, after culling out duplicates, yielded 232 items. After studying their titles and abstracts, 68 items were found to be relevant to our study. These studies are listed in Appendix A. Citation tracking was applied to the remaining references and to a search for books and comprehensive works on difficulties with algebra. For example, Rhine, Harrington and Starr’s book [6] includes over 900 works of reference covering the last five decades of research on learning algebra. Their work and others’—such as Booth et al. [7], Jupri, Drijvers and van den Heuvel-Panhuizen [8], Kieran [9,10] and Booth [11]—paved the way for practical citation tracking (snowballing) [5].

In the following, we present the findings of our study of the literature. As mentioned, these findings are restricted to information that describes difficulty in understanding and solving equations (particularly among lower secondary school students), which may inform task design related to linear equations in dynamic online environments. As part of this, we also present and analyse existing didactic categorisations of linear equations and key difficulties that students encounter when working with linear equations.

3. Key References from Five Decades of Research

We have organised our findings (or students’ difficulties) into the following categories and subcategories: (1) existing categorisations of linear equations; (2) difficulties related to the concept of ‘number’; (3) difficulties related to interpretations and the role of the equals sign; (4) strategic and transformational difficulties; (5a) operations and conventions; and (5b) letters and expressions.

3.1. Existing Didactic Categorisations of Linear Equations

Filloy and Rojano [12] argue for the presence of a ‘didactic cut’, which appears when the unknown in an equation is present on both sides of the equals sign, in which case the students must work with the unknown. Due to this didactic cut, Filloy and Rojano [12] separate linear equations into the following two categories:

• Arithmetical equations: $ax+b=c$
• Non-arithmetical equations: $ax+b=cx+d$

Although quite useful as a preliminary rough approximation, Filloy and Rojano’s distinction is a little too simple (e.g., [13]), when it comes to all the different types of linear equations that one can possibly imagine. The didactic cut argument is that students with no prior instruction in solving equations can solve those of the general ax + b = c type, but are unable to solve equations of the ax + b = cx + d type. Still, Filloy and Rojano’s [12] distinction deserves attention.

Finding additional categorisations of linear equations in the literature proved to be a challenge. However, Vlassis [14] does present an extension of Filloy and Rojano’s distinction. As others have, Vlassis [14] challenges this distinction. She argues that both arithmetical- and non-arithmetical equations should be further divided into two categories each, where $a,b,c$ and $d$ are positive integers:

• Arithmetical equations

  ○

  Concrete arithmetical equations:   $ax+b=c$

  ○

  Abstract arithmetical equations:   $ax + b-cx = d$, $-x=c$

• Non-arithmetical equations

  ○

  Pre-algebraic equations:       $ax+b=cx+d$

  ○

  Algebraic equations:         $-ax=cx-d$,

The justification for this separation is based on the argument that not all arithmetical equations may be solved with an arithmetical approach to the expression. Vlassis argues that equations such as −x = 7, or even 6x + 5 − 8x = 27, fall into the arithmetical equation category, but cannot be solved entirely with arithmetic alone. Hence, the need for the abstract arithmetical equation category. In the case of the non-arithmetical equations, Vlassis argues that the presence of negative numbers (in coefficients and terms) causes the main detachment from a use of a model, e.g., the balance model, to solve or interpret an equation [14].

3.2. Difficulties Related to the Concept of the Number

Working with negative numbers is a major theme when addressing students’ difficulties in working with linear equations [14,15,16,17,18]. In arithmetic, the negative sign indicates only the operation of subtraction. However, in algebra the negative sign has new predicative purposes, namely unary, binary and symmetrical [18]. The binary role is one that lower secondary school students may know from carrying out arithmetic calculations, whereas in algebra, they are presented with additive inverses (symmetrical role) and the minus sign as a predicate to a number (unary role). It is argued that beginning algebra students find the negative sign’s new role counterintuitive. Thus, the difficulties the students encounter include both negative numbers and the separation of the sign from the number or letter [13,17]. Gallardo [16] presents four levels of interpretation of negative numbers:

• Subtrahend, where the notion of number is subordinated to the magnitude (for example, in a − b, a is always greater than b, where a and b are natural numbers).
• Relative or directed number, where the idea of opposite quantities in relation to a quality arises in the discrete domain and the idea of symmetry appears in the continuous domain.
• Isolated number, that of the result of an operation or as the solution to a problem or equation.
• Formal negative number, a mathematical notion of negative number, within an enlarged concept of number embracing both positive and negative numbers (today’s integers). (p. 179)

Kieran [19] argues that two overarching difficulties emerge when solving equations with negative numbers, namely:

• Inversing a subtraction with a subtraction or failure to do so when necessary, e.g., solving 16x − 215 = 265 by subtracting 215 from 265 or solving 37 − b = 18 by adding 37 and 18. (p. 143)
• Leaving the unknown with a negative sign in front of it, e.g., −x = −17. (p. 144)

This leads to the observation that negative numbers, the predicative and operation minus, are of paramount importance when we consider students’ difficulties with various types of linear equations. Negative numbers are not only difficult to work with, as is mentioned later in the section on transformational activity, but they are also difficult to work with when they are the solution to a problem [6,16,18,19]. Christou et al. [20] emphasise that the unknown value may stand for either a positive or a negative number, independently of the sign that is attached to it.

Negative numbers are not the only ones that present difficulties for students. Fractions, as coefficients and as solutions to equations, also do. Christou and his colleagues [20] point out that for the students, fractions may be ‘unexpected’ solutions to linear equations. This may partly be understood with reference to the didactic contract [21], in particular when none of the numbers in a given equation are rational (fractions, decimal numbers, etc.) (e.g., [3,22]); it may also be the result of the common misconception that multiplication always makes a number ‘bigger’ [20], or of common misinterpretations of letters that we will touch on later.

Research suggests that students have difficulties when working with, or encountering, the number 0. In a literature review, Rhine et al. [6] present a table of various understandings of 0 in mathematics:

• Nil zero: Zero has no value and students act as if it was not there.
• Place value zero: Zero is used as a placeholder in a large number when there is none of that place value (over half of students up to the eighth grade could not write a number such as ‘two hundred thousand forty three’).
• Implicit zero: The zero does not appear in writing, but is used in solving a task. A student might solve a problem by thinking about obtaining a zero in the process. For example, 5 − 17 = 5 − 5 − 12 = 0 − 12 = −12 might be the thought process while 5 − 17 = −12 is the only thing written.
• Total zero: The combination of number opposites. For example, 34 + (−34) = 0.
• Arithmetic zero: The result of an arithmetic operation.
• Algebraic zero: The result of an algebraic operation or the solution of an equation. (p. 145)

The foregoing understandings are also related to the concept of the equation, especially when it comes to equation-solving or solutions to equations that are 0. Rhine et al. [6] also emphasise that students often are confused by the appearance of the number 0 in equations, and mistakenly believe that the solution to the equation is 0 (e.g., 0x = 5).

3.3. Difficulties Related to the Equals Sign

In the cohort of publications we selected for our study, several research studies report findings that support the observation that students’ understanding of the equals sign has a tremendous impact on their success with algebra. Studies also report that this is true of success with equation-solving, mainly in relation to the ability to carry out the same operation on either side of an equation [13,23,24,25,26,27]. One of the central difficulties that students encounter in the cognitive transition from an arithmetic approach—making calculations—to an algebraic approach—simplifying expressions and working with equations—is that they continue to view the equals sign as a ‘“do something” signal’ [25,28], or they maintain an urge to ‘calculate’, out of habit [29,30].

Behr, Erlwanger and Nichols [31], and Kieran [28] reported on students who viewed expressions such as ⎕ = 2 + 3 as being ‘backwards’. Such findings suggest that some students look for the opportunity to ‘calculate’ or to get ‘the solution, and that decomposing a number is an acquired way of thought that they bring from arithmetic. This links to another phenomenon, namely, accepting a lack of closure, which effectively means not accepting an expression as ‘the solution or an expression on the right side of the equals sign [32,33,34]. For example, Linsell [35] presents data that shows that the equation, 26 = 10 + 4n, is much more challenging for students than the seemingly similar equation, 4n + 9 = 37.

Beginning algebra students tend to believe that only a single entity should be present on the right side of the equals sign, which, in Falkner, Levi and Carpenter’s [23] famous type of equation, 8 + 4 = ⎕ + 5, means that students often believe that 12 must go in the box, as they simply calculate from left to right. For this same equation, some students believe that a 5 should be added to the 12, so 17 goes in the box, as they desperately attempt to keep the ‘answer’ free of operators. In their study, only 2% of 5th and 6th grade students gave the correct answer, that is, 7 [36]. This example demonstrates that students may be willing to ignore the meaning of the operator’s position in their efforts to give the proposition meaning [37]. This also demonstrates some students’ willingness to disregard the last term, to keep the equation in an ‘operation equals answer’ form [28].

In another example, students may believe that in the equation, 4 + 5 = 3 + 6, the term 3 + 6 should be combined into the ‘solution, owing to its position to the right of the equals sign. This is also the case with a similar problem involving an algebraic expression that includes letters as ‘the answer’ [11,38]. The way such a view is linked to the concept of the equation may prove rather problematic. If students’ perception of the equals sign is associated with the button on a calculator that indicates that a calculation is made, or must take place, they are clearly still in an arithmetic mindset, and will experience difficulties when working with certain types of arithmetical and non-arithmetical equations.

Sfard and Linchevski [39] address students’ incorrect use of strings of equations. For instance, when handling a word problem such as, ‘How many marbles do you have after you win 4 marbles 3 times and 2 marbles 5 times?’, the child will often write: ‘3 ⋅ 4 = 12 + 5 ⋅ 2 = 12 + 10 = 22′ (p. 209). They emphasise that such a use of the equals sign clearly demonstrates an arithmetic or process-oriented mindset. These arguments suggest that the position of the equals sign and the structure of the right side of the equation play major roles in a didactic categorisation of various linear equations, according to students’ difficulties with such.

Matthews et al. [27] suggest the following levels of understanding of the equals sign (see

Table 1. ‘Construct map for knowledge of the equals sign as an indicator of mathematical equality.’ (adapted from [27] (p. 224)).

Level 4: Comparative Relational	Successfully solve and evaluate equations by comparing the expressions on the two sides of the equal sign, including using compensatory strategies and recognising transformations maintain equality. Consistently generate a relational interpretation of the equal sign.	Equations that can be most efficiently solved by applying simplifying transformations: For example, without adding 67 + 86, can you tell if the number sentence ‘67 + 86 = 68 + 85′ is true or false?
Level 3: Basic Relational	Successfully solve, evaluate, and encode equation structures with operations on both sides of the equal sign. Recognise relational definition of the equal sign as correct.	Operations on both sides: $a + b = c + d$ $a + b - c = d + e$
Level 2: Flexible Operational	Successfully solve, evaluate, and encode atypical equation structures that remain compatible with an operational view of the equal sign.	Operations on right: $c = a + b$ No operations: $a = a$
Level 1: Rigid Operational	Only successful with equations with an operations-equals answer structure, including solving, evaluating, and encoding equations with this structure. Define the equal sign operationally.	Operations on left: $a + b = c$ (including when blank is before the equal sign)

).

These levels suggest that a student should at least have a ‘flexible operations’ conception of the equals sign, to be able to solve, manipulate or possibly even understand (decode [40]) algebraic equations [14].

3.4. Difficulties Related to Strategies and Transformations

Jankvist and Niss [3] mention two major concerns that are at stake with regard to the difficulty related to goal-oriented transformations of equations into equivalent expressions. First, there is the difficulty of choosing a sequence of efficient operations that leads to transformations of the equation in question, and gives the student an opportunity to find an equation from which the solution may be inferred. Second, there is the difficulty of making valid transformations of the elements in the equation. Several studies show that for students, the difficulty of an equation increases with the number of steps required to solve it (with the formal approach) (e.g., [41,42,43]). For example, Rhine et al. state [6]:

One- and two-step equations represent classes of algebraic relations that are often the easiest for students to solve. These equations often take the form of ax + b = c where a, b and c are constants. Students may visualise what has been ‘done to’ and ‘undoing what has been done’ as an informal way of approaching the task that is often successful.

(p. 100)

According to Araya et al. [44], when solving arithmetical equations, students must solve equations with the unknown on the right-hand side of the equals sign instead of the left. This is consistent with Behr et al.’s findings [31] that students may view certain equations as ‘backwards’, and with Linsell’s [35] mentioning that 4n + 9 = 37 is less challenging for students than 26 = 10 + 4n. Furthermore, Araya et al. [44] mention that changing the letter used to represent the unknown, such as ‘x’, for a less commonly used letter, such as ‘s’, makes an equation more difficult for some students, since they do not have a strategy for ‘s’. Additionally, constructing equations with an infinite number of solutions and equations with no solutions are further possibilities for distinction. In a study of 621 lower secondary school students, Linsell [35] measures their ability in terms of the equation-solving strategies they apply, and in this way establishes a hierarchy of equation-solving strategies. Linsell mentions that the strategies applied varied from equation to equation, indicating that some strategies are only applied in certain situations involving certain properties of the equations. For example, Linsell [35] mentions that the equation, 5n − 2 = 3n + 6, was solved using only a guess and check approach, or formal transformations.

Most of the strategies listed in

Table 2. Classification of strategies used for coding [35] (p. 333), drawing on [10].

1.	Unable to answer question
2.	Known basic facts
3.	Counting techniques
4.	Inverse operation
5.	Guess and check
6.	Cover up
7.	Working backwards then guess and check
8.	Working backwards then known fact
9.	Working backwards
10.	Transformations/equation as object

are more or less self-explanatory, except perhaps for the ‘cover-up’ strategy. This strategy or technique is based on the idea of working backwards, and substitution. The student covers the term (or expression, in some cases) containing the unknown, and solves the equation using the ‘cover’ as a new unknown. Next, the student solves for the initial unknown by setting the solution found for the cover ‘equal to’ the cover. For example, one could cover the term 6x in the equation, 6x + 5 = 23, to get ⎕ + 5 = 23, realising that 6x = 18, before solving for x in this new equation.

Strategy no. 10 (Table 2) is also referred to as the ‘formal strategy’. Kieran elaborates that students bring the two techniques of ‘using a known basic fact’ and ‘the counting technique’ with them from arithmetic, to solve ‘missing addend sentences’. Even though we cannot necessarily list these strategies in a hierarchy of sophistication for all linear equations, Linsell’s findings suggest that the various types of linear equation do have some hierarchy [35]. The meaning a student assigns to the equals sign or to the equation as a concept is a key to choosing a sequence of transformations that will lead to the solution to an equation. It should be noted here that an algorithmic approach to equation-solving could lead to the correct solution without the student having a proper understanding of the concepts and theorems involved. The second aspect of the difficulty presented by transformational activity [3] involves the valid manipulations of the elements in an equation, both the actions that should maintain the equality of the expressions and the manipulations that take place in the expressions on either side of the equals sign. This aspect of the transformational difficulties may bear a closer relationship to difficulties with arithmetic and algebra in general. Kieran [19] describes a range of common errors children make in their attempts to solve a linear equation. Some errors are made by beginners, some by intermediates, and some errors are made by both groups. Errors common to both groups were:

• Giving up when attempting to solve using the substitution procedure.
• Inversing subtraction with subtraction and addition with addition
• Computing a coefficient with a non-coefficient
• Forgetting that concatenation means multiplication (p. 143)

When students learn to solve equations, the ‘change side—change sign’ strategy may be a powerful tool for quickly progressing and solving equations rapidly. However, this strategy may lead the students to commit errors 2 and 3 [6]. Among beginning algebra students, observed difficulties included [19]:

• Not using the order of operations convention.
• Not knowing how to start solving a given equation-type.
• Inversing a multi-operation equation before collecting the multiplicative terms.
• Not using the convention that two occurrences of the same unknown are the same number.
• Giving precedence to an addition when it is preceded by a subtraction.
• Inversing a two-operation equation only once and then using the result of that operation as the solution. (p. 144)

Observed errors committed by intermediate algebra students included [19]:

• Leaving the unknown with a negative sign in front of it, e.g., −x = −17.
• Changing an addition to a subtraction when transposing, but then commuting the subtraction, e.g., 30 = x + 7 → 7 − 30 = x.
• Transposing only the literal part of the term and leaving the coefficient behind, e.g., solving 7 × c = c + 8 by writing 7 − 8 = c ÷ c.
• Dividing larger by smaller rather than respecting the order for inversing, e.g., 11x = 9 → x = 11/9.
• Computational error involving positive and negative numbers.
• Inversing a one-operation addition equation twice by inversing the addition and then dividing the unknown by the result of the subtraction, e.g., solving e.g., solving n + 6 = 18 by subtracting 6 from 18 and then attempting to divide n by 12. (p. 144)

Prediger [34] argues that “If, in the calculation aspect, the variables are considered to be meaningless symbols, terms are also meaningless expressions and equivalent terms are those which can be transformed into each other according to the transformation rules.” [34] (p. 7).

3.5. Difficulties with Letters in Expressions of Linear Equations

When examining the literature related to students’ difficulties with interpreting and working with letters in mathematical expressions, many researchers report findings related to algebra in general [6,9,10]. The majority of these studies are not specifically about equations or equation-solving. Usiskin [45] suggests that the concept of algebraic variables may be seen in four different ways: generalising arithmetic; solving equations, including making sense of solutions; exercising algebraic rules while studying relationships among quantities; and building algebraic structures and learning from these. Usiskin [45] raises the point that:

Under the conception of algebra as a generalizer of patterns, we do not have unknowns. We generalize known relationships among numbers, and so we do not have even the feeling of unknowns.

(p. 12)

Usiskin elaborates that working with variables as unknowns is different from working with variables when generalising arithmetic and when working with variables in formulas.

When working towards a solution to an equation, students assign different meanings to the letters in it. During the CSMS project [46], Küchemann [38,47] developed six categories of views that students apply to letters in their attempts to make sense of algebraic expressions. These categories are mentioned extensively in the literature on children’s thinking about algebra, yet, they should not be interpreted as hierarchical levels (e.g., [6]). In Küchemann’s first three categories, we find student difficulties that relate to working with unknowns in equations. We address these categories one by one.

In the ‘letter evaluated’ category, students will avoid operating on the letter as an unknown by assigning a value to the letter. An example would be assigning an ‘alphabetical value’ to the letter. This value corresponds to the numerical value based on the letters’ positions in the alphabet. Similarly, students may assign the letter the value 1, because there is one ‘thing’ present. Lastly, students may assign the letter a contextual value drawn from the given situation [38]. In the best-case scenario, adopting this view of the unknown letter when solving a linear equation may be successful, as long as no operations on the unknown are required.

In the ‘letter not used’ category, students ignore the letter, or at best, acknowledge its existence without ascribing a meaning to it. This category is exemplified by solving this equation: if a + b = 43, a + b + 2 =____. In this example, the students do not need to assign values to the letters a and b to reach a solution. However, consider the following example: if e + f = 8, e + f + g =____. Although the same view may be applied here, acceptance of the lack of closure is required. In the so-called CSMS study, this led students to evaluate the letter g in order to give answers such as 4 + 4 + 4 = 12 or 15, because g is the 7th letter of the alphabet. Such alphabetical evaluation has also been reported by MacGregor and Stacey [48]. Both Kieran [19], Küchemann [38], and Booth [11] report on students who compute coefficients with non-coefficients (also known as conjoining). In an equation, this could result in 3x + 7 being substituted with 10x.

In the third, ‘letter used as an object’, category, students regard the letter as shorthand for an object, or as an object in its own right. This view of letters used in algebra may prove beneficial in some cases, but disastrous in others. If this view is applied in order to collect like terms, treating the letters as objects works well. For example, when simplifying 2x—3 + x to 3x − 3, the x’s may be thought of as bananas, chocolate bars or just x’s. However, some students tend to treat the letter as an abbreviated word, such as ‘height’ or ‘blue’, instead of merely being a placeholder for a number. This view of the letters corresponds with the idea of the equation fitting a model, for example, the balance model [14].

After presenting the three, above-noted original categories, Küchemann [38] presents three additional ones, namely, ‘letter as a specific unknown’, ‘letter generalised number’ and ‘letter used as variable’. In each of these three additional categories, students may operate on the unknown number in such a way that it facilitates the solution of an equation. Hodgen et al. [49] argue that students’ understandings of letters in mathematics have not changed over the years, that is, not since Küchemann first described these.

4. Methodology Part 2: Task Design

In this section, we present the task design principles and the reasons behind them. When designing tasks for any environment, one should be aware of the main purpose of developing these tasks, since they may serve several purposes. Burkhardt and Swan [50] present four general categories of task design: specifying a curriculum; high-stakes assessment; classroom assessment; and teaching and learning. Burkhardt and Swan [50] mention that high-stakes assessment tasks may play three roles, namely: measuring performance; exemplifying performance goals; and driving classroom learning activities. Notably, the task design in this project covers a range—or a sequence—of tasks that are not meant to be evaluated or assessed individually. To some extent, our sequence of tasks resembles high-stakes assessment, in that it may be seen as measuring performance. However, as mentioned in the introduction, the overarching purpose of this project is to offer a formative assessment of what may initially resemble regular, repetitive training tasks.

Applying variation theory [51] to a concept such as the linear equation makes it possible to carefully—and meaningfully—design a sequence of tasks that may be seen as a whole [52]. By varying the construction of each task in a sequence to address the difficulties presented in the preceding section, it becomes possible to develop meaningful tasks that may be evaluated as a set. In variation theory you work with the intended object of learning, which is what the teacher or the task intends to demonstrate to the student; and the lived object of learning, which is what actually becomes the student’s focus, when he/she executes a task. The assumption is that if the tasks are carefully constructed, the intended object will become obvious under an assessment. The goal is to make the intended object and the lived object coincide.

When specifying a sequence of tasks nearly similar to each other for online use, Bokhove [53] suggests the element of crisis as an important factor. Such a crisis occurs when the student completing a range of tasks—or parts of the task range—encounters a task that is impossible, or nearly impossible, to solve. This element of crisis resembles a cognitive conflict (e.g., [54]) or an inadequate conceptual field [55]. Bokhove and Drijvers [4] use the element of crisis in their variations, when designing a sequence of nearly similar tasks to show that students attempting to solve a crisis-provoking task similarly to pre-crisis tasks may result in incompleteness, because the earlier strategy is inadequate.

Burkhardt and Swan [50] suggest that the question of task difficulty must not be ignored, and is an important part of task classification and design. They propose four considerations related to task difficulty:

• Complexity—the number of variables, the variety and amount of data, and the number of modes in which information is presented, are some of the aspects of task complexity that affect the difficulty it presents.
• Unfamiliarity—non-routine tasks (those which are not just like the tasks one has practised solving) are more difficult than routine exercises.
• Technical demand—tasks that require more sophisticated mathematics for their solution are more difficult than those that can be solved with more elementary mathematics.
• Student autonomy—guidance from an expert (usually the teacher), or from the task itself (e.g., by structuring or ‘scaffolding’ it into successive parts) makes a task easier than if it is presented without such guidance. [50] (p. 433)

Watson and Mason [52] propose the controlled variation of tasks, which offers the following elements:

• Analysis of concepts in the conventional canon that one hopes learners will encounter.
• Identification of regularities in conventional examples of that concept (and its related techniques, images, language, contexts) that might help learners (re)construct generalities associated with the concept. Even an algorithm can be seen as a generality.
• Identification of variation(s) that would exemplify these generalities; decide which dimensions to vary and how to vary them;
• Construct exercises that offer micro-modelling opportunities, by presenting controlled variation, so that learners might observe regularities and differences, develop expectations, make comparisons, have surprises, test, adapt and confirm their conjectures within the exercise;
• Provide sequences of micro-modelling opportunities, based on sequences of hypothetical responses to variation, that nurture shifts between focusing on changes, relationships, properties, and relationships between properties. [52] (pp. 26–27)

An Example Task

As an example, let us take a look at the general arithmetical equation, ax + b = c [12], where a, b and c are all positive integers. In many cases, $c$ would be a larger number than b, to keep the value of $x$ (the solution) positive. However, bearing in mind that students often have difficulty with negative numbers, a variation might look like this:

$$4x+8=4$$

The values of a, b and c are chosen so that $a$ is a factor in c + b, c − b and b − c. The solution to an equation such as the example above takes the form of x = (c − b)/a = (4 − 8)/4 = −1. If we recall that lower secondary school students may not be comfortable with negative numbers, and therefore unwilling to proceed by rearranging the numbers when subtracting the 4 − 8, they may end up with 4 instead of −4, and thus reach the incorrect solution of x = 1. To address another common difficulty, inverting addition by addition, some students may add b and c, and find x = 3. Finding the wrong answer to an equation may be due to unfamiliarity with this sort of equation, so a negative number is found for the solution [50]. This may also lead to reversing the order of the subtraction, resulting in b − c, to make the task make sense, and the same student might have subtracted b from c if c − b yielded a positive number. Some may describe this as a result of breaking the didactic contract [21] between the teacher (issuing the task) and the student. The goal of the design is to increase complexity, unfamiliarity and technical demands for the range of tasks, while using variation theory to present subtypes that vary to address the range of difficulties reported in the literature.

When working towards the foregoing general design goals, some minor detours will be made, to address specific difficulties (explained in the next section). The intentional crisis, combined with the knowledge of major difficulties that students encounter when solving linear equations and their knowledge of useful strategies, becomes the strategy used to design a broad range of increasingly complex, unfamiliar, technically demanding linear equations, in order to assess online students’ abilities to solve linear equations.

5. Establishing Categories of Linear Equations

In this section, we explain our task design of the various types of linear equations, based on the results of research into students’ difficulties with linear equations, and the task-design principles guided by variation theory. More precisely, the task design takes its point of departure in the categorisation established by Filloy and Rojano [12], and Vlassis’s later expansion [14], bearing in mind that Vlassis’ categorisation [14] is based on the problems and difficulties encountered when linear equations are made abstract by a negative sign/number. When separating linear equations into arithmetical and non-arithmetical (algebraic) equations, the reader should also bear in mind that the term simplified arithmetical equation indicates arithmetical equations with a basic arithmetic form, that is, ax + b = c [12]. Similarly, the term simplified algebraic equation indicates algebraic equations with the form, ax + b = cx + d.

Our design included a set of boundaries. First, we wanted to make sure that we had a clear framework for separating the equations, or types of equations, which were included in the design. When we say ‘separating’, we mean a separation in terms of the difficulty and complexity of solving an equation, supported by our findings in the literature [50]. Second, to the extent possible, we set out to design a comprehensive arsenal of tasks for beginning or early intermediate equation solvers (e.g., Danish lower secondary school students in their 7th year). Bear in mind that, on the one hand, these tasks should present a learning path that also serves as a tool for detecting students with difficulties, or in the best-case scenario, a tool for showcasing students’ concrete (categories of) difficulties with linear equations. Each type of equation in the design includes several variations of equations derived from the general type. In the next section, we further specify the design requirements.

5.1. Overarching Design Requirements and Goals

We remind the reader of the overall variations due to students’ difficulties identified through the literature, task design theory, and in particular, the restrictions that exist when designing tasks for dynamic online environments. The intent of our design is to vary across:

• Negative numbers and the minus sign

  ○

  as solutions

  ○

  as terms

  ○

  as operations

• Rational numbers

  ○

  as solutions

  ○

  as present numbers

• Interpretation of the equals sign

  ○

  Situations that invoke an element of crises from an arithmetic point of view

• Strategic, conventional and transformational questions

  ○

  Increasingly complex and strategically demanding

  ○

  Conventions concerning brackets

  ○

  Conventions concerning missing multiplication

As mentioned previously, student responses to tasks in the dynamic online environment that underpins our design are restricted to multiple-choice and input fields. For our design, the ‘input field’ was chosen as the only option. This was done to avoid influencing the students’ answers, as may occur when presenting multiple choices. This, and the concept of the element of crisis [4], establishes an outset for applying variation theory [51], when designing and developing the tasks for the online environment.

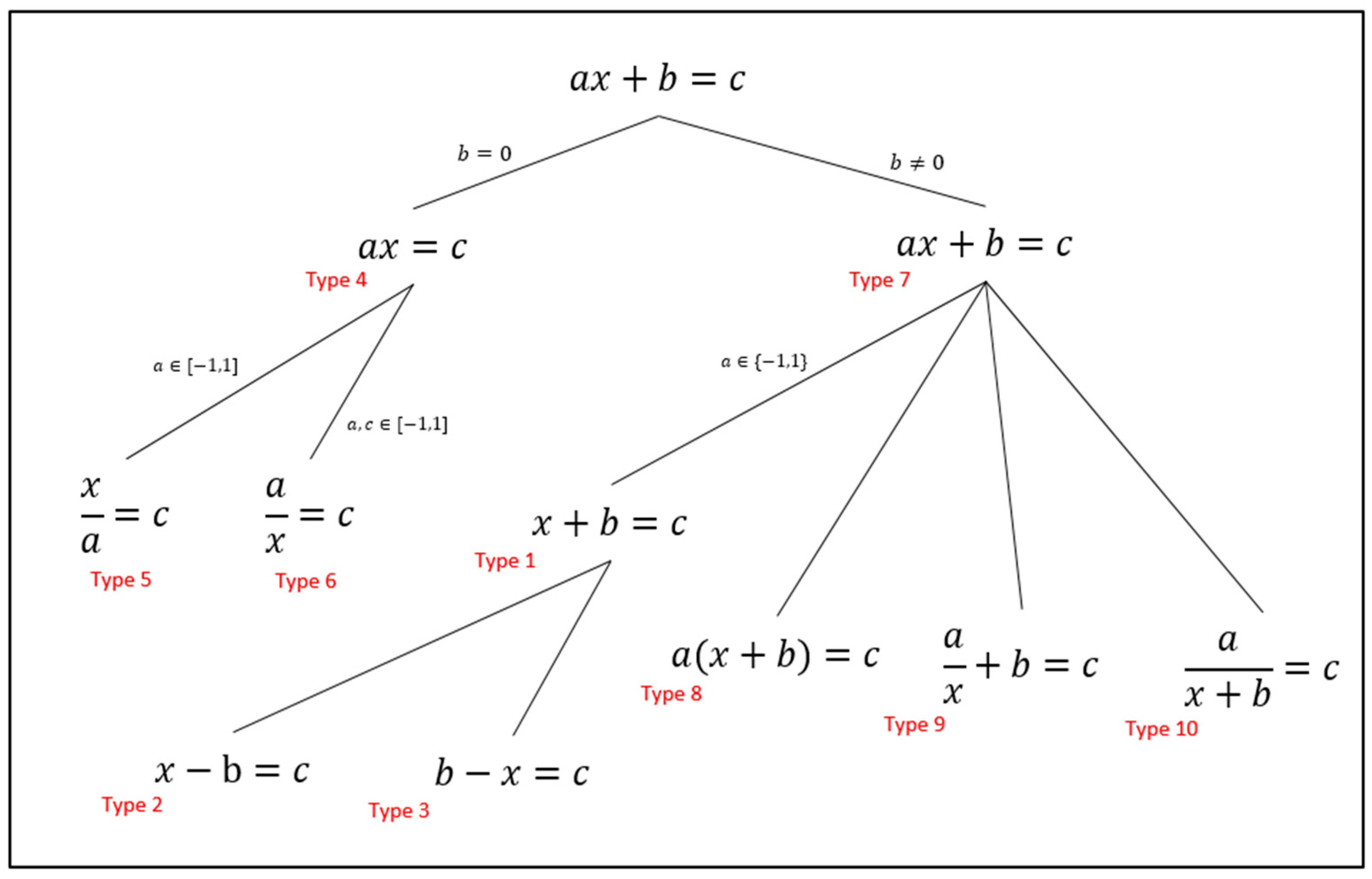
5.2. Arithmetical Equations

Even though arithmetical equations [12] may appear simple, the smallest variations may be deal-breakers for some students. Small variations in, and considerations of task design, lead to tasks better suited to addressing the difficulties mentioned in the literature. This should not be seen as an attempt to trick the students into giving incorrect solutions, but as exposing a tendency to apply inadequate schemes when completing the task. Every type of simplified arithmetical equation (see Figure 1) is categorised into subtypes (or variations), using variation theory related to students’ difficulties mentioned in the literature.

The reader should note that in several of the variations, constant values (a, b and c) are simply switched, or in some cases one value is simply inverted, to establish a connection between the tasks, thereby adding another layer of possible comparison.

We now address and discuss each of the ten different types of arithmetical equations.

5.2.1. Types 1 and 2: $x+b=c$ and $x-b=c$

In many cases, these types of equations are what many teachers and students consider the simplest setup possible, because addition is arguably the simplest and most familiar operation to many students. All these equations are designed around the x + b = c template, with various b and c values. Technically speaking, type 2 is simply a variation on type 1. For the sake of clarity, these are separated into two types. Although this form does not necessarily yield the simplest equations, many would consider several forms of the equations in this category easy or beginner level.

The two types of variations of these equations address the negative (for the values b, c and x) and the order of operations in the expression. To introduce a rational solution to these equations, one would have to let either b and/or c be a rational number. This was not deemed a necessary variation at this stage. The first subtype consists of simple addition and simple subtraction expressions, with small natural numbers for the b and c values. These are meant to be simple and easy for most upper secondary school students to solve. A solution typically requires a ‘guess and check’, a counting technique or the ‘knowing’ strategy, or a formal transformation, subtracting b from c, and isolating x. These equations add to the overarching task design by serving as a reference point.

Table 3. Examples of type 1 and type 2 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$4+x=7$	$7=x+4$	$7+x=4$	$4+x=-7$	$7+x=-4$	$x+4=7$
$x-3=5$	$5=x-3$	$x-5=3$	$x-3=-5$	$x-5=-3$	$-5+x=3$

below presents examples from this set of tasks. Note that the b and c values are exchanged whenever possible, to facilitate further a potential comparison of students’ task performances.

Variation 1 consists of simply flipping the equation so the addition is on the right hand side of the equals sign. This variation was chosen because of students’ perceptions of the equals sign described in the literature. Variation 2 has a negative $x$ value (solution), because the b and c values are exchanged. Variation 3 also has a negative $x$ value, since the value of c is an additive inverse. The reason for including variation 3 is to try to determine whether the presence of a negative c value has any influence on the task performance, in comparison to variation 2. In variation 4, the b and c values are again exchanged, but the minus sign remains on the right side. This has no major influence on the tasks, where $x$ is added to b. However, it changes the value of x from negative to positive in the tasks where b is subtracted from x. In variation 5, the order of operations on the left side is exchanged, and the value of x remains positive.

5.2.2. Type 3: $b-x=c$

This type of equation differs from types 1 and 2 in technically being a two-step equation, if solved formally or through formal transformations. Still, in many cases these equations are presumably solved by using ‘guess-and-check’, ‘knowing’, or counting strategies. For many students, an example equation would read as ‘6 less some amount makes 3′. If such equations are solved formally, the students are faced with an equation that looks like –x = p, where p = c − b. This type of equation is mentioned by Vlassis [14] as being one of the certain indicators of and reasons for finding that difficulty is not determined merely by whether an equation is arithmetical or non-arithmetical, but also by the level of abstraction it involves.

The two variations that appear in these equations are the use of the negative (for the values b, c and x), and the changes in the order of terms in the expression. Examples are given in

Table 4. Examples of type 3 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$6-x=3$	$3-x=6$	$-6-x=3$	$6-x=-3$	$-6-x=-3$	$-x+3=6$

.

The first variation exchanges the b and c values. This results in negative x values, when compared to the original, which has a positive x value. In variations 2, 3 and 4, $b$ and $c$ values are exchanged for their negative counterparts. In variation 5, the equations actually become a special case of type 7 (see Figure 1) equations. This variation is intentionally presented here.

5.2.3. Type 4: $ax=c$

This type of equation is the first, and technically simplest, version of an arithmetical equation that involves multiplication, with b = 0. Presumably, as with the previous types, many students will be able to apply a ‘guess-and-check’ or ‘knowing’ strategy, or a ‘cover up’ strategy when working with these equations. Using small positive integers ensures that the solution is also a natural number. In the standard or original variation, the equation may remind students of multiplication tables. Examples are provided in

Table 5. Examples of type 4 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$3x=15$ $8x=8$	$3x=-15$	$-3x=15$	$-3x=-15$	$15=3x$	$28x=14$ $2x=9$

.

Variation 1 introduces a negative c value, which results in a negative x value (solution). The same idea is present in variation 2, where the a value is negative. In variation 3, the x value is again positive, but both a and c values are negative. Variation 4 introduces what was also introduced in type 1 (see Figure 1), where the expressions flip sides. In variation 5, rational x values are introduced. These are chosen to only contain one decimal place in order not to overcomplicate the task. The numbers are chosen so that students who are uncomfortable to end up with a rational solution, where 0 < x < 1, are incentivised to carry out inverse operations, resulting in the student answering x = 2, instead of x = 0.5. In other cases, a student may multiply instead of divide, to make the equation make sense.

5.2.4. Type 5: $x/b=c$

This type of equation follows a similar pattern to type 4 (see Figure 1). Technically, the number $b$ could be considered the fraction $\frac{1}{b}$ in type 4, in which case we would end up with type 5. However, one of the main criteria that restricts our design was the decision to use integers only, and in some cases, rather simple decimal numbers.

Table 6. Examples of type 5 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$\frac{x}{3}=9$ $\frac{x}{2}=2$	$\frac{x}{9}=3$	$\frac{x}{2}=-9$	$\frac{x}{-3}=9$	$\frac{x}{-3}=-9$	$\frac{x}{4}=3.4$ $\frac{x}{-4}=3.4$

exemplifies this.

The original variation also uses number pairs (a, c), where a is a factor of c. From the literature we know that some students may be tempted to divide c by a instead of multiplying. Variation 1 features equations whose number pairs are exchanged. This may be expected to result in a ‘crisis’ [53] for those students who divide instead of multiply. Variations 2, 3 and 4 use combinations of negative numbers, as do the preceding types. Variation 5 uses rational numbers for the c value, and integers for the a value. The value of a remains an integer, since multiplication with rational numbers is beyond the scope of this study (and design).

5.2.5. Type 6: $a/x=c$

This form of equation is the third and final version of the single operation variant that centres on multiplication (see Figure 1). What is different about this variation is that technically, these must be considered two-step equations. In these equations, the unknown is positioned in the denominator, which makes a tremendous difference in complexity, compared to the previous type (type 5). That said, some straightforward strategies may be easily applied to solving these problems. The ‘guess-and-check’ or a knowing strategy may be very efficient here, if the students are able to read and understand what the problem requires of them. To solve these formally, one would have to multiply each side by x before dividing each side by c, which is known to be a demanding procedure for some students.

Table 7. Examples of type 6 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4
$\frac{9}{x}=3$ $\frac{2}{x}=2$	$\frac{9}{x}=-3$	$-\frac{9}{x}=3$	$-\frac{9}{x}=-3$	$\frac{6.6}{x}=2$ $\frac{4.5}{x}=9$ $-\frac{6.6}{x}=2$

provides examples.

The original variation actually uses the numbers in pairs, as they were used in the previous type (type 5). This makes future comparisons possible. The intent is to observe the extent to which type 5 and type 6 equations may generate the same, or similar, wrong answers. Variation 1 is not simply a switching of a and c (as seen in type 5), since this would result in a solution that exceeds scope of this study. Hence, variations 1, 2 and 3 have negative numbers for a and/or c, which gives negative solutions for variations 1 and 2, and a positive solution for variation 3. Variation 4 has rational numbers for the a values, and therefore has rational solutions. The c value is kept as a natural number in order to determine whether this suggests to students that the solution is natural.

5.2.6. Type 7: $ax+b=c$ and $ax-b=c$

This type of equation is the first to include two operations on the left hand side of the equals sign, and which introduces a third constant to the task design. To many teachers and researchers, these equations may resemble classic arithmetical equations [12]. However, some level of distinct abstraction is possible. First, with these equations we must move further away from the application of the ‘guess-and-check’ strategy, and toward more refined solution strategies, which, according to the literature, are likely to be required. These equations must also be considered two-step equations, with a ∉ {0,1}. To some extent, equation type 3 (b − x = c, cf. Figure 1) does require two steps for its solution, but does not require two operations on the left side, and is arguably easier to solve. The reason for using the minus sign between the terms $ax$ and $b$ for the categorisation is the immediate jump in the level of abstraction involved (e.g., [14]). The design involves manipulating the values of a and c accordingly, to identify the subtypes. See

Table 8. Examples of type 7 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$2x+7=21$ $2x-7=21$	$8x+16=8$	$8x+10=30$ $8x+30=10$	$-7x-9=33$ $-2x-5=-3$	$4x+12=-10$ $-6x+2=-13$	$10=2x+6$

for examples.

The original variation is the most straightforward. The a, b and c are natural numbers with values less than 30, which is intended to ensure positive natural number solutions (c > b). In contrast, we have an identical task design idea, but with the b value inverted. The point here is to develop a task where a is a true divisor in c − b, b − c, c + b and b + c. This form ensures that the equations are interesting from a data-analysis perspective, and may encapsulate the students’ difficulties with this setup or these types of equations.

Variation 1 is similar to the first subtype with respect to the a, b and c values. However, now b > c, to generate negative integer solutions. Again, a is kept as a true divisor in c − b, b − c, c + b and b + c. Variation 2 has a positive rational number solution. The reason for restricting the design to generate positive solutions is to enable future analyses of students’ difficulties. If the tasks have both positive and negative solutions, this complicates the distinction between difficulties related to negatives and difficulties related to rational numbers. The solutions are limited to p/2, where p is a natural number. The reason for restricting the design in this way is to avoid over complication. The tasks are intentionally designed to take advantage of students’ willingness to alter operations (for the sake of sense-making). In these cases, some students may be tempted to add 30 to 10 or 10 to 30, to reach an integer solution. Variations 3 and 4 use negative $a$ and $c$ integers. Variation 3 keeps the integer solutions, whereas variation 4 advances to rational number solutions (p/2, where p ∈ Z). Variation 5 introduces a reversed version of the equation, similar to those in types 1 and 4.

5.2.7. Type 8: $a\left(x+b\right)=c$

This type of equation is the first of three versions of the classic arithmetical equation ax + b = c (type 7). In many cases, this variation of ax + b = c may be regarded as easy to solve. However, some students may be challenged by the necessity of working with calculations with brackets. Technically, the brackets are easily managed in this type of task, since the equations may be rewritten by dividing by a on each side. Predictably, many students will begin by expanding the brackets, and many may have difficulty with this expansion, and end up with an equation that is not equivalent to the initial equation [6]. Presumably, some students may observe the factorisation of c into a and (b + x). With this train of thought, a ‘working backwards’ or ‘undoing’ strategy would be suitable for these equations. This type of equation provides a direct contrast to the type 7 (ax + b = c) equation. Arguably, for this variation it may be difficult to apply a ‘guess-and-check’ strategy, when compared to type 7. For examples, see

Table 9. Examples of type 8 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$2\left(x+4\right)=12$	$2\left(x+12\right)=20$	$3\left(x-5\right)=6$	$2\left(x+4\right)=8$	$2\left(x+4\right)=13$	$-2\left(x+3\right)=-10$$-6\left(x+8\right)=-12$

.

The original variation consists of ‘pretty’ versions of these equations, with natural values for a, b and c. The equations have natural number solutions because a is chosen as a factor of c. Variation 1 also uses natural numbers for a, b and c, and a as a factor of c. However, in these instances, a times b is greater than c, which results in negative solutions. Variation 2 includes subtraction in the brackets: in other words, a negative b value and positive solutions. Variation 3 has 0 as a solution, because a times b equals c. Variation 4 has rational numbers as solutions. The solutions are limited to p/2, where p is a natural number. Variation 5 uses a negative a and c, with both positive and negative integer solutions.

5.2.8. Type 9: $a/x+b=c$

This type of equation is the second variation of type 7 (ax + b = c), but also has many technical similarities to type 6 (b/x = c). Formally, solving these equations requires three steps. The reader may agree that the likelihood of successfully applying a ‘guess-and-check’ or a ‘knowing’ strategy at this stage is unlikely. To some extent a ‘cover up’ or a ‘working backwards’ strategy may work, and the task is rather easily transformed into a type 6 equation.

Table 10. Examples of type 9 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4
$\frac{3}{x}+2=5$ $\frac{6}{x}+3=5$	$\frac{6}{x}+5=3$	$\frac{-4}{x}+4=2$ $\frac{-4}{x}+2=4$	$\frac{9}{x}-2=1$ $\frac{9}{x}-5=-8$	$\frac{7.5}{x}+5.5=8.5$

provides examples.

The original equation uses natural numbers and natural number solutions, with b < c. In variation 1, c < b, to yield negative integer solutions. In variation 2, a has a negative integer value, with the added variation of c < b and b < c (b and c are exchanged). Both positive and negative solutions may result, because of the relationship between c and b. Variation 3 includes a subtraction or a negative b value. Both positive and negative solutions may result, owing to positive and negative c values. Variation 4 has rational a, b and c values. Again, the values are limited to p/2, where p is a natural number.

5.2.9. Type 10: $a/\left(x+b\right)=c$

This final variation of type 7 equations (ax + b = c) may be considered the most difficult or complex of the arithmetical equations. However, to develop a more advanced three/four-step equation, we designed this version of type 7, where the denominator includes an operation. In these tasks, too, the students must work with brackets to find a formal solution (cf. type 8). A ‘guess-and-check’ strategy may not be out of the question, but a ‘cover up’ strategy may be more fruitful in this situation. A ‘formal strategy’ would involve multiplying with the denominator on each side, yielding a type 7 (ax + b = c) equation. Presumably, this would be the strategy of choice for students who are able to solve these equations. See

Table 11. Examples of type 10 equations and variations.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$\frac{6}{x+1}=3$ $\frac{15}{x+3}=3$	$\frac{15}{x-2}=3$	$\frac{5}{x+3}=5$ $\frac{12}{x+8}=3$	$\frac{12}{x-7}=-4$	$\frac{-15}{x-6}=15$ $\frac{-15}{x-1}=3$	$\frac{19}{x+7}=2$ $\frac{19}{x+12}=2$

for examples.

The original variation of this equation uses natural numbers and natural number solutions. Variation 1 involves subtraction from the unknown in the denominator, but still with natural number solutions. Variation 2 consists of addition in the denominator (as in the original variation), but with negative integer solutions. Variation 3 involves subtraction in the denominator, but now with negative integer solutions, because of a negative c value. Variation 4 uses a negative value of a, with both positive and negative solutions. Variation 5 has rational number solutions limited to p/2, where $p$ is a natural number.

5.3. Algebraic Equations

This subsection discusses algebraic equations [12]. These equations have the unknown in two terms, on either side of the equals sign. In the literature, it is evident that difficulties similar to those described for arithmetical equations may be observed for the algebraic. However, algebraic equations present the added difficulty of requiring operations with an unknown [12,14]. ‘Simplified algebraic equation’ is as mentioned the term we use for equations that have the form, ax + b = cx + d, where a, b, c and d are known, and $x$ is the unknown for which we solve. Simplified algebraic equations involve other aspects related to students’ difficulties, which separates them from the simplified arithmetical equations. Algebraic equations have multiple terms to the right of the equals sign. This addresses the acceptance of the problem of a lack of closure [32]. With arithmetical equations, a student may apply an arithmetic understanding of the equals sign, and to a great extent, view the equation as a simple calculation. A student cannot apply this view to algebraic equations.

Type 11: $ax+b=cx+d$

Before presenting the variations of algebraic equations, we must establish a perhaps not-so-obvious—or underappreciated—distinction. When accustomed to solving arithmetical equations, many beginning equation-solvers predictably wish to isolate the unknown to the left hand of the equals sign. The solution to an equation is usually presented or written as x = p, where x is the unknown. Bearing this in mind, there emerges a clear distinction between algebraic equations in which c is larger than a, and equations where $a$ is larger than $c$. Of course, this is considered from a strategic perspective. Nonetheless, the variations begin with this distinction. However, we will limit the scope of the task design to simplified algebraic equations, which comprise algebraic equations of the form, ax + b = cx + d, where $a, b, c$ and $d$ are constants, and x represents the unknown. It is important to remember that if students are not applying a ‘guessing’ or a ‘knowing’ strategy to these equations, they must operate on the unknown. This observation makes it important to design tasks to address the role of the coefficients of the unknowns present. Conventions dictate that we do not have a present coefficient when it is equal to 1. Errors such as conjoinment (e.g., [11,19]) are an additional factor in the design. We consider the two general cases of variation in the simplified algebraic equations, where a > c and a < c. Examples are given in

Table 12. Examples of type 11 equations and variations with a > c.

Original	Variation 1	Variation 2	Variation 3	Variation 4
$2x+1=x+8$	$2x+8=x+1$	$5x+13=-5x+33$	$2x+5=1x+5$	$3x+8=x+3$

and

Table 13. Examples of type 11 equations and variations with a < c.

Original	Variation 1	Variation 2	Variation 3	Variation 4
$x+8=2x+1$	$x+1=2x+8$	$-5x+33=5x+13$	$1x+5=2x+5$	$x+3=3x+8$

.

The original variation has a natural number solution, and has only natural number entries. This variation also uses c values between 1 and 20 (bear in mind that this type use a values greater than c values). In variation 1, the b and d values are exchanged to obtain a negative solution. Variation 2 uses c as an additive inverse of a. This addresses the question of inverting subtraction with subtraction, or inverting addition with addition. If this is the case, some students may find that the unknown has ‘disappeared’, thus creating a scenario in which any number may be a solution. The literature indicates that if this happens, some students may become confused, and conclude that x = 0. Variation 3 uses identical b and d values, which gives 0 as the solution. Variation 4 uses rational numbers as solutions.

These variations are simply reversed variations of the equations in Table 12. These are presented to investigate the extent to which equations where it is not the ideal strategy to collect the unknowns on the left side of the equality sign are more difficult than those in which it is. Ultimately, these equations are identical to the equations in Table 12.

5.4. Different Branches of Variations of Linear Equations

In this subsection, we touch on some of the slight variations in the concept of the linear equation. In all the above-mentioned varieties of linear equation we used the letter x to represent the unknown. However, the literature [44] mentions that some students encounter difficulties when the name or the label of the unknown is an unfamiliar letter. In Denmark, the letter most commonly used to represent an unknown in a linear equation is x. Therefore, we have chosen to present variations on some of the above-mentioned types of equations with the letter x exchanged for a different one. For this we chose, types 1, 2, 4 and 7. We chose these to capture a broad variety of tasks in the section with arithmetical equations. We chose to not include an algebraic version, since we already made a comparison of arithmetic and algebraic equations, and we saw no need to present another, similar, comparison.

Table 14. Examples of type 1 and type 2 equation variations with n as the unknown.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$4+n=7$	$7=n+4$	$7+n=4$	$4+n=-7$	$7+n=-4$	$n+4=7$
$n-3=5$	$5=n-3$	$n-5=3$	$n-3=-5$	$n-5=-3$	$-5+n=3$

,

Table 15. Examples of type 4 equation variations with n as the unknown.

Original	Variation 1	Variation 2	Variation 3	Variation 4	Variation 5
$3n=15$ $8n=8$	$3n=-15$	−$3n=15$	$-3n=-15$	$15=3n$	$28n=14$ $2n=9$

and

Table 16. Examples of type 7 equation variations with n as the unknown.

Original	Variation 1	Variation 2	Variation 3	Variation 4
$2n+7=21$ $2n-7=21$	$8n+16=8$	$8n+10=30$ $8n+30=10$	$-7n-9=33$ $-2n-5=-3$	$4n+12=-10$ $-6n+2=-13$

present the specific equations we use in the dynamic online environment.

We now briefly address the question raised by Vlassis [14] regarding Filloy and Rojano’s [12] categorisation of linear equations. Vlassis [14] pointed out that the original categorisation was based on the ‘didactic cut’ [12], which some consider problematic. This has to do with abstract arithmetical equations [14]. Although many of these abstract arithmetical equations are covered by types 1 through 10, we intentionally excluded what we call non-simplified arithmetical equations (subset of pre-algebraic equations [14]). By non-simplified we mean that the unknown is represented in multiple terms, but on only one side of the equals sign. To complete our design, we included variations of the following equation template, ax + b + cx = d, as shown in

Table 17. Examples of non-simplified equations and variations based on $ax+b+cx=d$ [14].

Original	Variation 1	Variation 2	Variation 3	Variation 4
$x+6+2x=15$	$4x-3+2x=15$	$4x+3-2x=13$$4x-3-2x=11$	$2x+3-4x=13$	$-2x+3-4x=21$

.

The original variation uses positive a and c values, and a natural number for d − b. This results in natural number solutions. The idea is to measure how these non-simplified arithmetical equations perform when compared to simplified arithmetical equations. The number of variations is restricted for this particular reason. The intension is not to present every variation of a simplified arithmetical equation (types 1–7) transformed into an equivalent non-simplified version. Variation 2 uses a negative integer value for b, still with natural number solutions. Variation 3 reverses the a and c values. This results in a + c being a negative integer, and in negative integer solutions. Variation 4 uses negative integer values for a and for c. This still yields negative integer solutions.

5.5. Limitations and Opt-Outs

Some initial limitations, which we discovered in the early phase of the design process, were those set by the format of the dynamic online environment. As mentioned, we are limited to input fields handling only rational numbers (as decimal numbers) and multiple-choice. The intention, regarding the future data analysis, was thus to create a large amount of distinguishable linear equations in order to make a successful attempt of categorizing students as equation-solvers. Other approaches to diagnostics require in-depth analysis of students’ mathematical behaviour through conversations, interviews, and structured situations [3].

Due to the limitations of the input methods in the dynamic environment, we have also opted out designing linear equations with infinitely many or no solutions. If we were to implement such equations in the online environment, we would have to use the multiple choice input method. As previously explained, this was deemed undesirable.

6. Discussion

The question we initially set out to answer was, ‘How may research on lower secondary school students’ difficulties with linear equations inform task design in a dynamic online environment with the possibility to promote/support students’ personalised learning?’ As explained, we approached this question by applying a two-tier methodology: the first tier involved reviewing the existing literature on students’ difficulties with solving linear equations; the second tier involved applying theoretical constructs from task design, in particular variation theory, to developing tasks that promote students’ personalised learning. As is evident from the final design presented above, although these two tiers initially ran along separate tracks, they eventually became rather entangled. Our discussion below examines this.

First, we would like to discuss why we chose to address the aspects of the literature that are presented in Section 3. When we carried out the snowballing portion of the hermeneutic review process, a picture of the general categories of the literature began to take shape, including the questions that this research literature addresses has dealt with. This prompted a deeper dive into the general categories of the literature, while we kept in mind the future implementation linear equation tasks in a dynamic online environment. As we mentioned, we knew that we would end up with a design that consisted of a number of easily accessible tasks, not being one combined diagnostic task [52]. We wished to determine whether quite ordinary training tasks may be designed and implemented to address the difficulties that lower secondary school students encounter when solving equations. It was also important to us to keep our focus on the relevant age group at all times, while snowballing the literature.

As lower secondary school students are our focus, the difficulties mentioned in the literature gave rise to the design of assignments for this particular group of students. In Danish lower secondary schools, algebraic equations are not a dominant topic. Many of the difficulties that the literature describes as surrounding the concept of the equation may be addressed by using arithmetical equations. We have thus chosen to put a greater emphasis on designing such equations. This was done mainly because of the target group. In lower secondary school, many students struggle with the concept of equations [6]. We also know from the literature that students’ difficulties arise when operations have to be done on the unknown or the unknown is present on both sides of the equal sign [6,12,13,14]. However, several of the remaining difficulties (specifically issues regarding the presence of negative numbers) that students experience during equations solving can arise when solving arithmetical equations. For this reason, we have chosen to focus the task design more towards arithmetical equations and negative numbers. However, it is clear that the difficulties that may arise because of the presence of the unknown in several terms of an equation may be addressed only via a non-simplified arithmetical equation or an algebraic equation. This conclusion underlies the significant difference in the number of types of equations presented in the general division into arithmetical equations and algebraic equations (cf. Figure 1). This conclusion, together with the difficulties described in the literature, also underlies the way the various types of equations are designed. This is expressed in the learning tree of arithmetical equations (Figure 1) and the single type of algebraic equations (type 11). Thus, the difficulties described in the literature influenced the design process in two ways. First, the general categories of documented difficulties of the target group gave rise to the types of equation that are deemed relevant. Second, the theory of variation used on the more nuanced documented difficulties within each category gave rise to the varieties that are considered relevant to each type of equation.

Next, we discuss the mathematical elements present in the general equation types and their varieties. First of all, the opt-outs of mathematical content related to students’ documented difficulties, were made during the development of the tasks. Fractional coefficients have not been prioritised here, since they are not regarded as having a direct impact on students’ understanding of the concept of the equation. Surely, the concept of the fraction is difficult. However, we say that the concept of the fraction is difficult for lower secondary school students, regardless of whether or not a fraction appears in an equation. That said, there are a few fractions in equation types 5, 6, 9 and 10. However, these fractions can be replaced with another syntax indicating division, meaning the fractions in the task design are purely operational. When examining the literature (e.g., [6]), we find that fractions do in fact present a source of great difficulty for younger students, who are doing algebra and arithmetic. We have not included fractions (as coefficients, terms or solutions) for two reasons. The first being that the online environment does not support fractions as an input in the input field, which rules fractions as solutions out. Fractions as coefficients and terms have not been a part of the design due to the second reason, which can be stated as a question: Fractions do arguably make algebra and arithmetic more difficult, but do fractions make equations more difficult? Knowing that a student cannot solve equations with fractions for a,b,c or x, does not mean that the student does not know how to solve equations, rather that it means that the student has difficulties working with fractions. However, we wanted to make it possible to evaluate as many varied situations as possible in which the unknown must be treated differently. In type 5, (x/b = c), we decided that a student should be able to handle the fraction as a process, i.e., the process it may be seen as, rather than using other mathematical symbols to indicate division. On the other hand, this also clarifies the scope of the findings that address difficulties with the concept of the equation, and solving equations. For us, the extent of the difficulties surrounding negative numbers, or the concept of numbers in general, is now much clearer; this also applies to working with the concept of the equation. It may be difficult to make a concrete comparison of the difficulties. Nevertheless, difficulties surrounding negative numbers—as solutions, coefficients and constant terms—may be observed at every step of a learning path. However, we have strongly emphasised negative numbers as solutions, as constant terms and as coefficients. With regard to the literature on difficulties with the concept of the equation, it is striking how much emphasis is actually placed on negative numbers. Lower secondary school students form our target group, and most of these students may be considered inexperienced equation solvers. Several research studies place great emphasis on the fact that negative numbers and the use of the negative generally becomes a focal point when teaching the concept of the equation [6,14,18]. Therefore, negative numbers became a pervasive element of our designs. To generate a negative solution to a standard arithmetical equation (ax + b = c), either b must be greater than c, or a minus sign must be present in some way. If we factor this into our design, we may see a difference in the answers to tasks, where negative solutions emerge with or without the presence of the minus sign.

The second category of difficulty in solving equations concerns the equals sign. Much of what is emphasised in the literature is not necessarily easy to transfer to a design that must consist of simple equation-solving tasks. Many might argue that if it is to be possible to determine whether a student has a good understanding of, or has difficulty in interpreting the equals sign in a given situation. In this case, some completely different tasks may be needed. Much of what is described regarding the equals sign has to do with relation versus process. Based on our task design, it would be inaccurate to claim that we may determine whether or not a student has a strong grasp of the equals sign. However, what it has been possible to apply to the design is the question of the ‘acceptance of lack of closure’ [32]. Here, we have been able to develop variations of individual equation types, in order to investigate whether or not everyone who can solve the equation ax = b can also solve the equation b = ax. We have done this with equation types 1, 4 and 7. If a student has difficulty with ‘the acceptance of lack of closure’, algebraic equations may easily present an insurmountable challenge. A rigid arithmetic interpretation of the equals sign may lead to omission of the d term in algebraic equations such as ax + b = cx + d [23,27].

Another important question related to the task design concerns the deliberate omission of the multiplication sign. In Denmark, it is customary to indicate the multiplication of two numbers as a ⋅ b. This is not the case in all countries: for example an ‘×’ is used in some English-speaking countries. In general, in Danish lower secondary schools, arithmetical equations are presented in the form ax + b = c, with the multiplication sign omitted. We have chosen to design and present our tasks in the same way, with the multiplication sign omitted. This is also partly influenced by the format of the dynamic online environment in question, matematikfessor.dk.

As previously mentioned, we wanted to make sure that we were likely to have a clear way of separating the linear equations, or types of linear equations included in our task design. We have also mentioned that we designed a reasonably comprehensive arsenal of tasks for beginning or early intermediate equation solvers (e.g., Danish lower secondary school students in the 7th year). The task design must form a learning path that serves as a tool for detecting students with difficulties related to linear equations. We suggest that the tree (Figure 1) that describes the relationship of the types of arithmetical equations may give rise to a learning path by traversing its branches. When a student has to work with arithmetical equations, the underlying structure of this tree may clarify the connections between difficulties and progress. This is not to say that every branch of the tree represents difficulties that cannot be found in other branches of the tree. The tree gives teachers and students the opportunity to look at the difficulties that arise while students progress along the mathematics learning path, and therefore they should try to take a step back to identify the cause of their difficulties. It may be said that students may have deeper mathematical difficulties that are not directly related to equation-solving, but related to embedded elements such as the concept of the number, the equals sign, and so forth, and their errors when solving equations are to be considered symptoms of something more deeply rooted [3,22].

With regard to the goal of personalised learning, the development of comparable tasks became an integral part of the task design. At best, a large data set of students’ answers to these exercises should reveal patterns of students as equation solvers’. When we say ‘comparable’, we mean that many of the task variations have been constructed in such a way that e.g., simply swapping two values within an equation creates a completely different solution. As mentioned, the possibility of substantial data extraction is one of the obvious benefits of including the tasks of the 11 types of linear equations in a dynamic online environment. Hence, selecting the parts of the literature that described students’ difficulties with the concept of linear equation, and which enabled us to form comparable tasks, became our mission. It must be said that when the literature on students’ difficulties with equation-solving is studied as far back as five decades, it may be difficult to determine how time has left its mark on the implementation of the curriculum and the expectations of today’s student, in terms of equation-solving. From previous studies [22], we know that in Denmark, upper secondary school students have significant difficulties with the concept of the equation and with equation-solving. Therefore, this initiative may provide teachers with an opportunity to become more familiar with, and thereby focus more on, the concept of the equation in lower secondary schools.

The guidelines presented by Watson and Mason [52] had a major impact on our overarching, initial design requirements. It helped us make clear which elements and sub-concepts of the concept of the equation we wanted address. Additionally, we made it possible for mathematical concepts that may cause students difficulties to come into play in the equations. From the perspective of the individual task, variation theory [51] and the element of the crisis [53] played major roles. If the equations of types 1 through 11 are seen in the context of problem difficulty [50], then an increase in difficulty may be noted, not only in the progression of types, but also in the variations of each type. The complexity increases through this progression, as the number of terms, operations on the unknown and the contexts in which the unknown is included, increase. Unrecognizability is addressed through the many variations, where apparently similar equations may require very different solutions (negative and rational numbers). The technical demands are also increased through the progression of the types. The unknown is part of the more advanced constellations of the ten arithmetical equation types (1–10), and also in the leap to algebraic equations (type 11), where the unknown occurs on both sides of the equals sign. It was not possible to address the question of the student’s autonomy [52] when solving problems. This is solely because the tasks need to be implemented in a dynamic online environment. Many of the tasks in the design also seek to address some of the extreme examples of equations that may easily be overlooked or omitted during ordinary classroom teaching. Such extreme cases may lead to crises [4] that ensure that as many as possible of the categories and subcategories of difficulty identified have the opportunity to come into play.

It should be noted that though we have focused on equation-solving at Danish upper secondary schools, the research shows that students’ mathematical difficulties, including difficulties with equation-solving, are not determined by national borders [3]; if not universal, they are at least international in nature. A remark may also be made with respect to the task design being affected by a specific dynamic online environment, which is matematikfessor.dk. Given that the mathematics difficulties we have addressed are universal, the ways in which they need to be addressed are bound to be similar on various online platforms.

7. Conclusions

We set out to address the question of how five decades of research into students’ difficulties with equation-solving may inform the design of the now-widespread, dynamic online environments to which many lower secondary school students turn for everyday mathematics instruction, and how the designs of these potentially may promote and support students’ personalised learning. Our approach to this research question has been to present a concrete design of eleven types of equations, including carefully selected variants of these types, to illustrate possible personalised learning paths as the branches of a tree construct that comprises these equation types. By making students and teachers aware of existing difficulties, and the positions of these in the tree structure, teachers may have an additionally unique opportunity to help students to overcome these difficulties as part of their teaching. The future role of the dynamic online environment will be to not only identify students, who have difficulty solving linear equations, and to diagnose the nature of their difficulties, but eventually to also intervene, although preferably in some sort of collaboration with the student’s mathematics teacher. Once a student’s difficulties are diagnosed, the dynamic online environment may have the student explore all the aspects of these, by carefully traversing the variations in the tree structure. However, for this to happen, one must begin with the concrete design of types of equations. We have attempted to present such a design by drawing on previous research on task design, both with and without digital technology, and particularly by applying the accumulated research on students’ difficulties with linear equation-solving where it belongs: to the students.