A New Approach for Approximate Solution of ADE: Physical-Based Modeling of Carriers in Doping Region
Abstract
:1. Introduction
- Facility of implementation in circuit simulators through analog electrical components with the system of equations developed as a solution of the ADE.
- The mathematical procedure to solve the spatio-temporal second-order differential ADE equation is not complex in comparison to the numerical solutions proposed in the previous reported references.
- The calculation variables used in each expression are a function of time and space, ensuring an adequate approximation with experimental values.
- The developed model calculates the low doping zone width during the space charge accumulation, allowing the excess of the carriers’ injected calculation on the N-region.
- A three-dimensional variable analysis of the carriers’ behavior is presented.
- The results show that the proposed solution is robust for its integration in commercial electrical circuit simulators.
- The obtained solution ensures a balance between mathematical calculations and accuracy results that are appropriate for its use by electronic designers.
- A graphical and numerical estimated error is presented to validate the accuracy of the obtained results.
2. Ambipolar Diffusion Equation (ADE)
2.1. Carrier Transport
2.1.1. Drift Mechanisms
2.1.2. Diffusion
2.2. Carrier Control Model
3. The Empirical Solution of the ADE
3.1. Static Phase
3.2. Conduction Phase
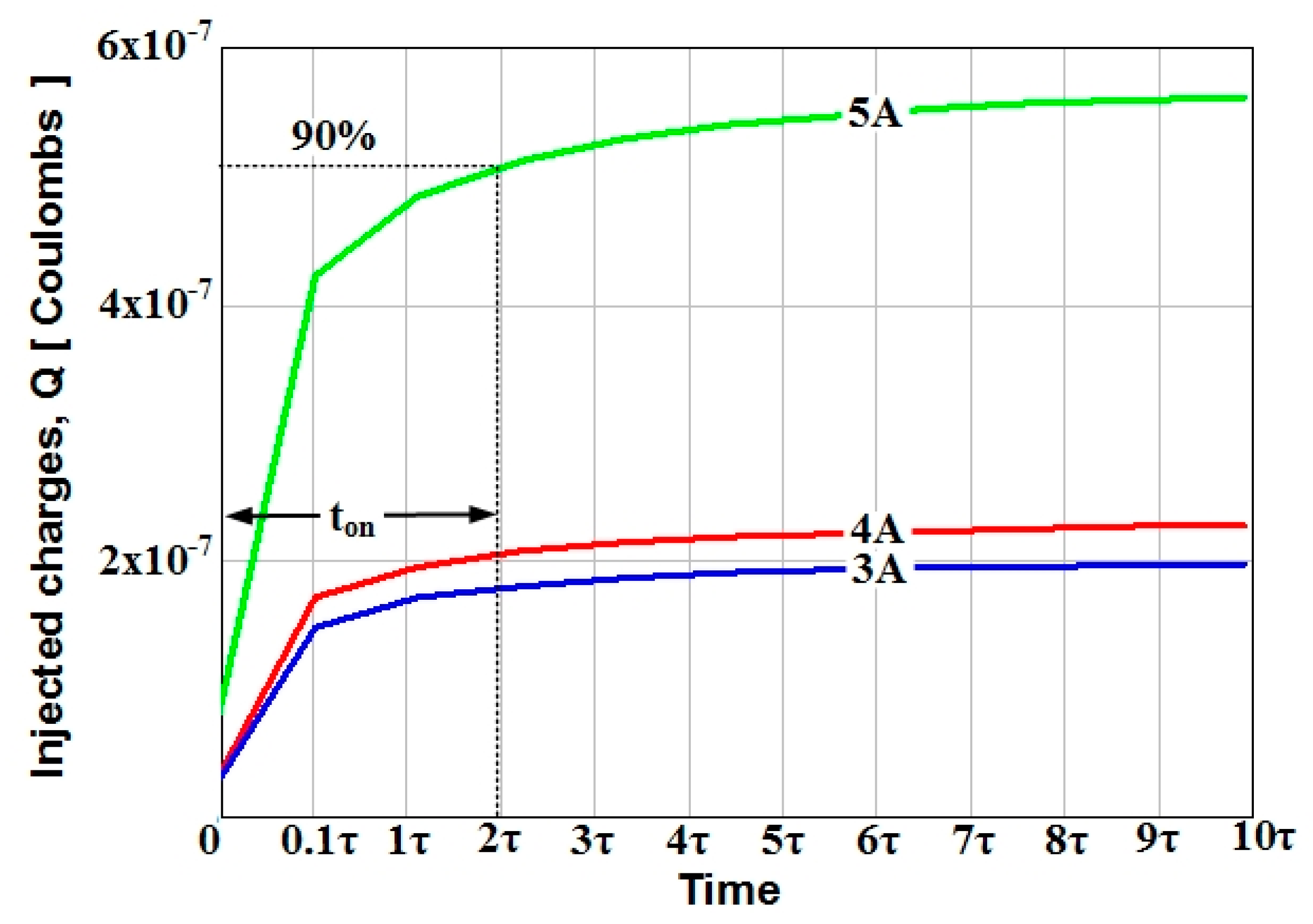
3.2.1. Activation
3.2.2. Blocking
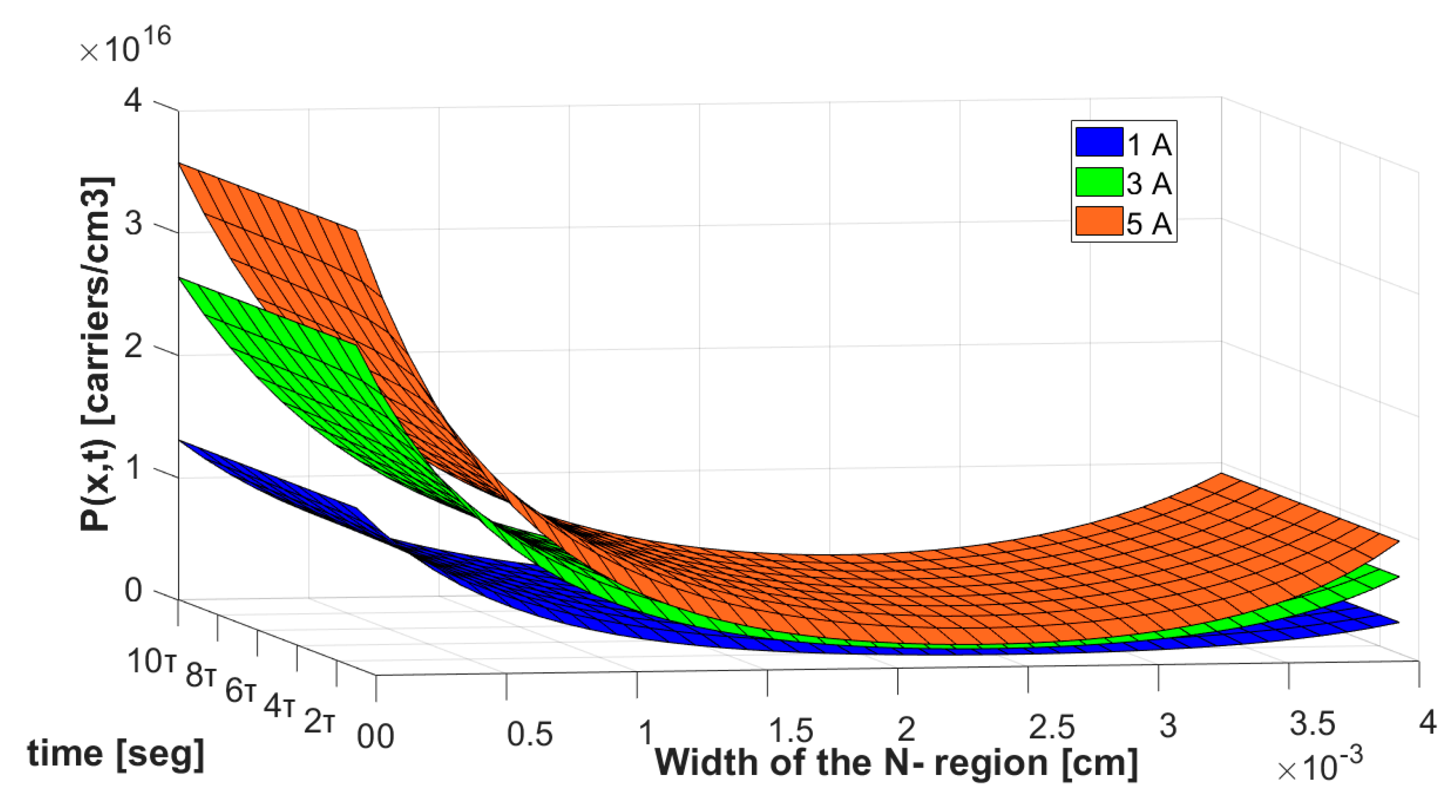
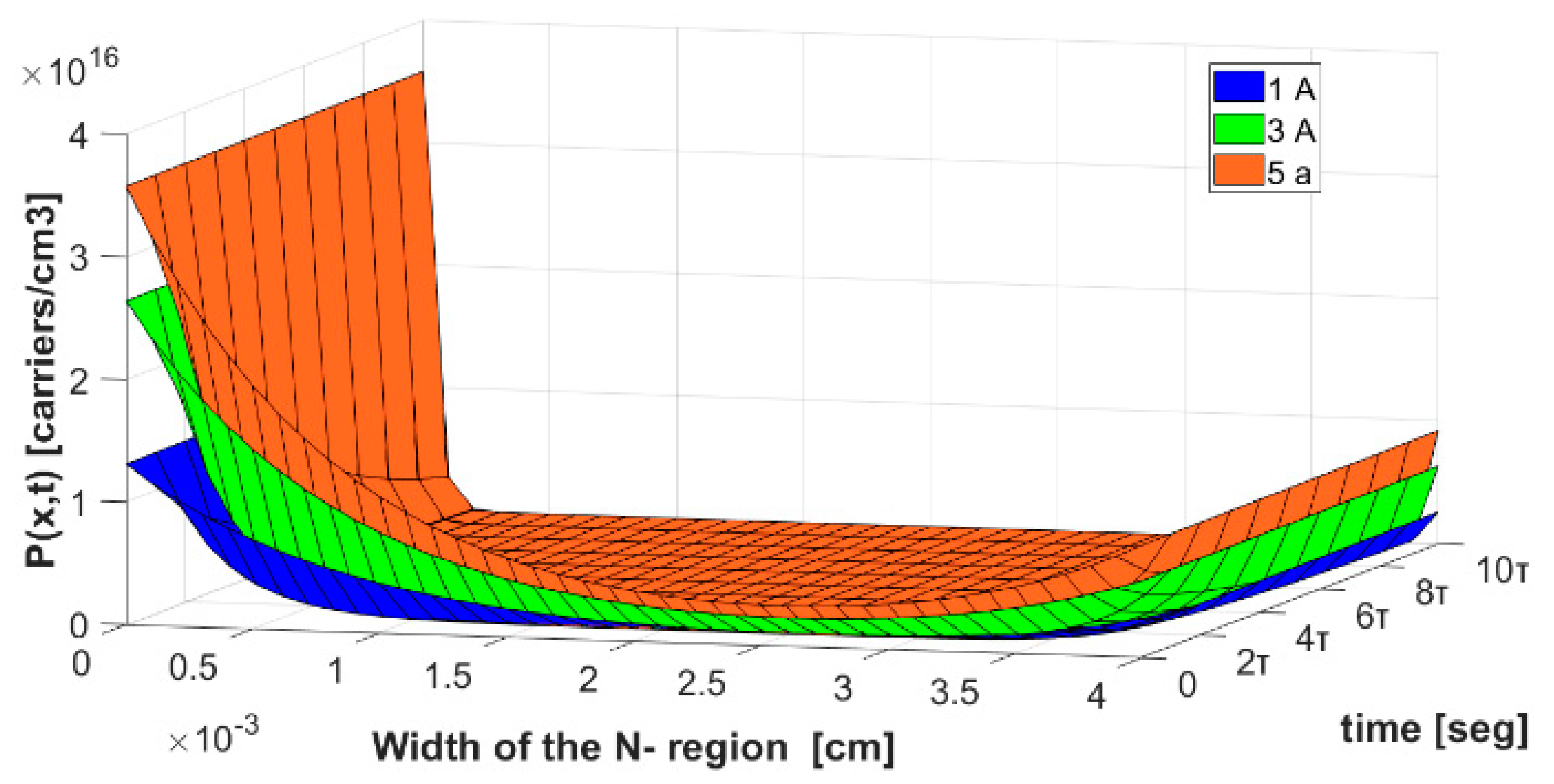
4. Simulation Results
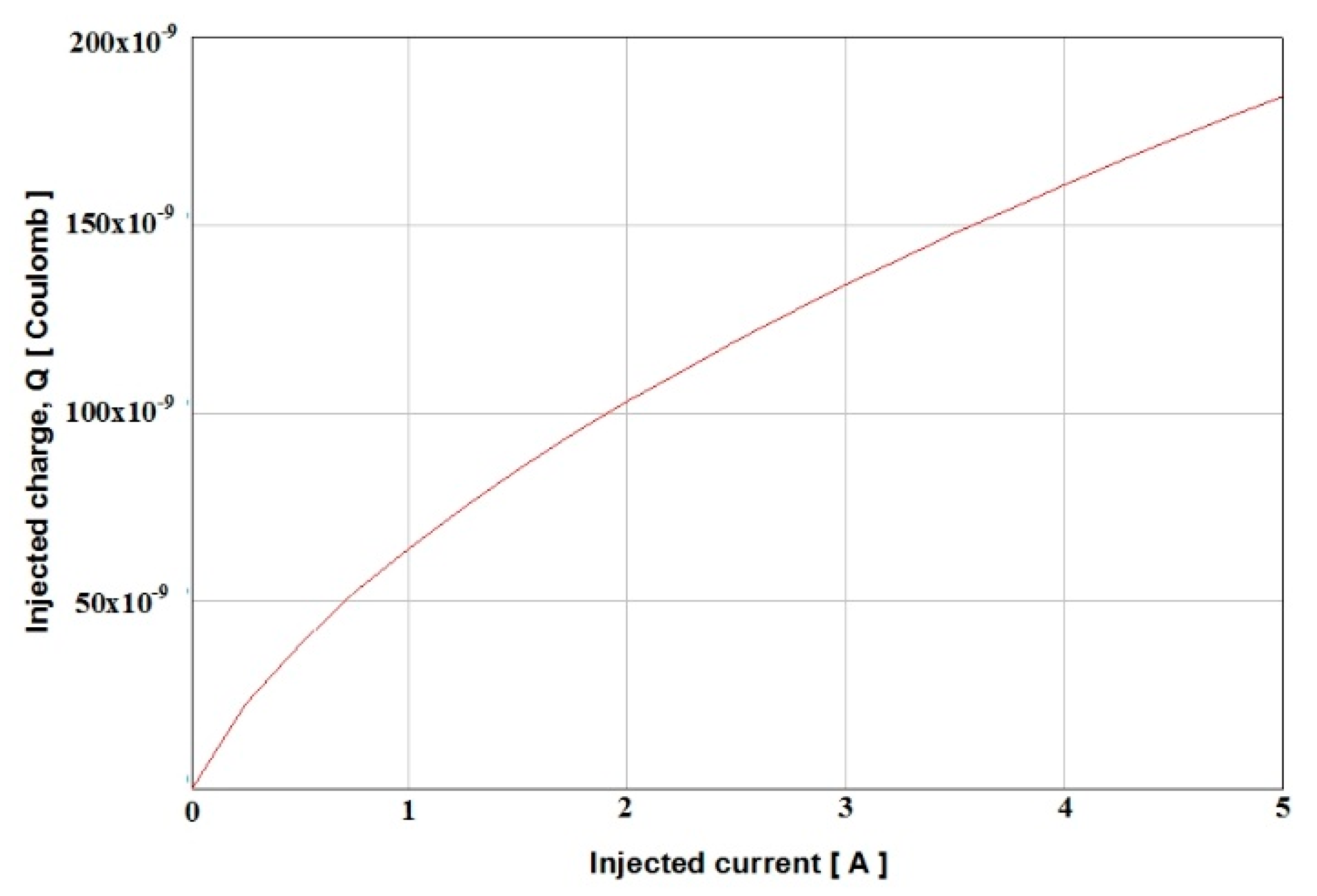
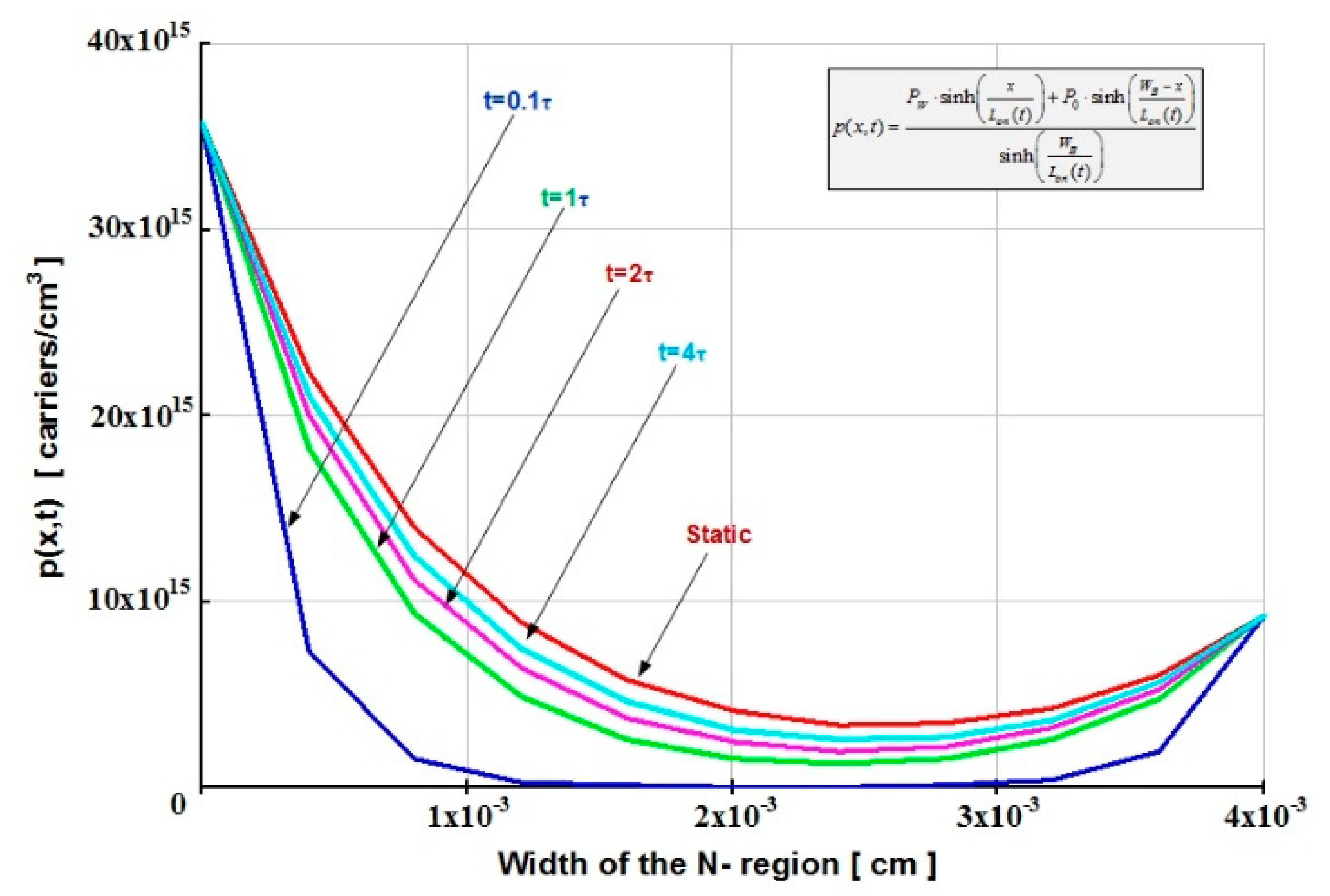
4.1. Static Phase
4.2. Conduction Phase
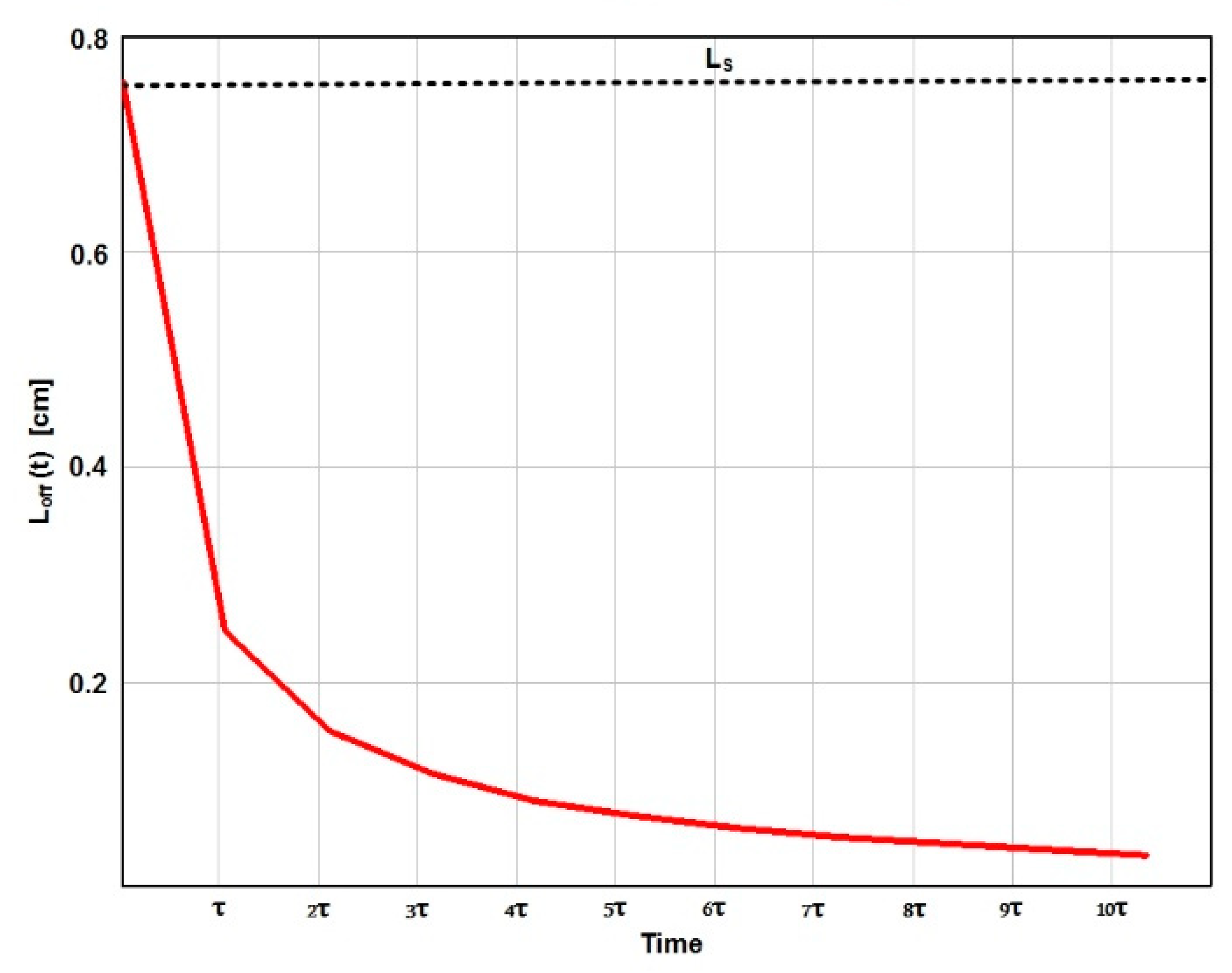
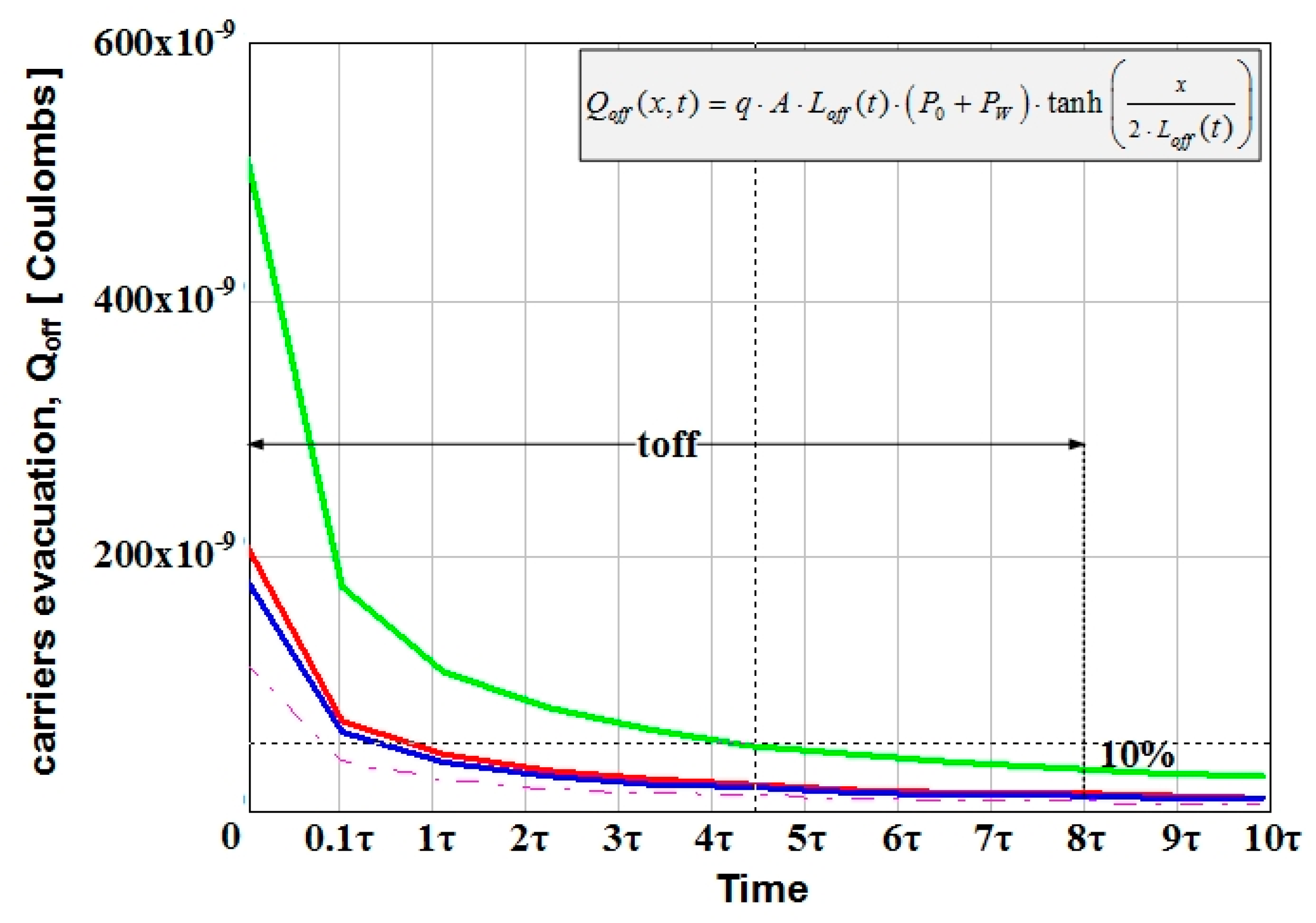
4.3. Reverse Blocking Phase
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Active area |
| ADE | Ambipolar diffusion equation |
| B = µn/µp | Mobility relation |
| BJT | Bipolar Junction Transistor |
| D | Ambipolar diffusion constant. |
| Dn | Ambipolar diffusion constant for electrons |
| Dp | Ambipolar diffusion constant for holes |
| E | Electric field |
| Eg | Bandgap |
| error(n) | Quadratic error |
| Exp(n) | Sampled Experimental data |
| Gn,p | Generation rate of electrons and holes |
| IGBT | Insulated-Gate Bipolar Transistor |
| In(0,t) | Electron current injected in the union P+N-. |
| In_drift | Electron drift current |
| In(WB,t) | Electron current injected in the union N-N+. |
| In_difusion | Electron diffusion current |
| Ip(0,t) | Hole current injected in the union P+N- |
| Ip_drif | Hole drift current |
| Ip(WB,t) | Hole current injected in union N-N+ |
| Ip_difusion | Hole diffusion current |
| ITD(x,t) | Total injected current in the diode |
| IT_drift | Total drift current in the diode |
| Lon | Ambipolar diffusion lengthen in turn-on phase |
| Loff | Ambipolar diffusion length in turn-off phase |
| LS | Ambipolar diffusion length in static phase |
| MSE | Mean Square Error |
| ni | Intrinsic concentration. |
| nP+ | Electron concentration in the region P+ |
| n(x,t) | Electron concentration in the region N- in function of space and time |
| Px=0 | Initial concentration close to the union P+N- |
| Px=WB | Initial concentration close to the union N-N+ |
| p(x,t) | Hole concentration in the region N- in function of space and time |
| Sim(n) | Simulated sampled data |
| WB | Low doping region width |
| Vn | Electron drift velocity |
| Vp | Hole drift velocity |
References
- Sze, S.M.; Kwok, K. Ng. p-n Juntion Diode. In Physics of Semiconductor Devices, 2nd ed.; Laboratories, B., Ed.; Wiley: New York, NY, USA, 1981; pp. 64–84. [Google Scholar]
- Neamen, D.A. The pn Juntion. In Semiconductor Physics and Devices, 3rd ed.; McGraw Hill: New York, NY USA, 2003; pp. 238–268. [Google Scholar]
- Liang, Y.; Gosbell, V.J. Diode Forward and Reverse Recovery Model for Power Electronic SPICE Simulations. IEEE Trans. Power Electron. 1990, 5, 346–356. [Google Scholar] [CrossRef]
- Chibante, R.; Araujo, A.; Carvalho, A. A new approach for physical-based modelling of bipolar power semiconductor devices. Solid-State Electron. 2008, 52, 1766–1772. [Google Scholar] [CrossRef]
- Sttrollo, A.G.M.; Napoli, E. Improved PIN diode circuit model with automatic parameter extraction technique. IEE Proc. Circuit Devices Syst. 1997, 144, 329–334. [Google Scholar] [CrossRef]
- Maxim, A.; Andreu, D.; Boucher, J. A new SPICE behavioral macromodeling method of magnetic components including the self-heating process. In Proceedings of the 30th Annual IEEE Power Electronics Specialists Conference, Charleston, SC, USA, 27 June–1 July 1999; pp. 735–740. [Google Scholar]
- Maxim, A.; Maxim, G. A novel power PIN diode behavioral SPICE macromodel including the forward and reverse recoveries and the self-heating process. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition, New Orleans, LA, USA, 6–10 August 2002; pp. 1088–1094. [Google Scholar]
- Zhang, H.; Pappas, J.A. A Moving Boundary Diffusion Model for PIN Diodes. IEEE Trans. Magn. 2001, 37, 406–410. [Google Scholar] [CrossRef]
- Petzoldt, J.; Reimann, T.; Lorentz, L.; Zverev, I. Influence of device and circuit parameters on the switching losses of an ultra fast CoolMOS/SiC-diode device-set: Simulation and measurement. In Proceedings of the 13th International Symposium on Power Semiconductor Devices & ICs, Osaka, Japan, 4–7 June 2001; pp. 187–190. [Google Scholar]
- McNutt, T.R.; Hefner, A.R.; Mantooth, H.A.; Duliere, J.L.; Berning, D.W. Parameter Extraction Sequence for Silicon Carbide Schottky, Merged Pin Schottky, and Pin Power Diode Models. In Proceedings of the IEEE Power Electronics Specialists Conference, Cairns, QLD, Australia, 23–27 June 2002; pp. 1269–1276. [Google Scholar]
- McNutt, T.; Hefner, A.; Mantooth, A.; Berning, D.; Singh, R. Compact models for silicon carbide power devices. Solid-State Electron. 2004, 48, 1757–1762. [Google Scholar] [CrossRef]
- Levinshtein, M.E.; Mnatsakanov, T.T.; Ivanov, P.A.; Singh, R.; Palmour, J.W.; Yurkov, S.N. Steady-state and transient characteristics of 10 kV 4H-SiC diodes. Solid-State Electron. 2004, 48, 807–811. [Google Scholar] [CrossRef]
- Giesselmann, M.; Edwards, R.; Bayne, S.; Kaplan, S.; Shaffer, E. Forward and Reverse Recovery Spice Model of a JBS Silicon Carbide Diode. In Proceedings of the IEEE Twenty-Sixth International Power Modulator Symposium, San Francisco, CA, USA, 23–26 May 2004; pp. 364–367. [Google Scholar]
- Loulo, M.; Abdelkrim, M.; Gharbi, R.; Fathallah, M.; Pirri, C.F.; Tresso, E.; Tartaglia, A. Modelling and analysis of a-SiC:H p–i–n photodetectors: Effect of hydrogen dilution on dynamic model. Solid-State Electron. 2007, 51, 1067–1072. [Google Scholar] [CrossRef]
- Kolessar, R.; Nee, H.-P. A New Physics-Based Circuit Model for 4H-SIC Power Diodes Implemented in SABER. In Proceedings of the IEEE Applied Power Electronics Conference and Exposition, Anaheim, CA, USA, 4–8 March 2001; pp. 989–994. [Google Scholar]
- Hatakeyama, T.; Nishio, J.; Ota, C.; Shinohe, T. Physical Modeling and Scaling Properties of 4H-SiC Power Devices. In Proceedings of the IEEE International Conference on Simulation of Semiconductor Processes and Devices, Tokyo, Japan, 8–11 September 2005; pp. 171–174. [Google Scholar]
- Vogler, T.; Schroder, D. A New Accuracy Circuit-Modelling Approach for the Power Diode. In Proceedings of the IEEE Power Electronics Specialists Conference, Toledo, Spain, 29 June–3 July 1992; pp. 870–876. [Google Scholar]
- Metzner, D.; Vogler, T.; Schroder, D. A modular Concept for the Circuit Simulation of Bipolar Power Semiconductor. Trans. Power Electron. 1994, 9, 506–513. [Google Scholar] [CrossRef]
- Ma, C.L.; Lauritzen, P.O. A simple power diode model with forward and reverse recovery. In Proceedings of the IEEE Power Electronics Specialists Conference, Cambridge, MA, USA, 24–27 June 1991; pp. 411–415. [Google Scholar]
- Kraus, R.; Hoffmann, K.; Mattausch, H.J. A precise Model for the Transient Characteristics of Power Diodes. In Proceedings of the IEEE Power Electronics Specialists Conference, Toledo, Spain, 29 June–3 July 1992; pp. 863–869. [Google Scholar]
- Igic, P.M.; Mawby, P.A.; Towers, M.S.; Batcup, S. New physically-based PiN diode compact model for circuit applications. IEEE Proc. Circuit Devices Syst. 2002, 149, 257–263. [Google Scholar] [CrossRef]
- Carvalho, A.; Araujo, A.; Martins de Carvalho, J.L. A New Method for Solving the Ambipolar Diffusion Equation (ADE) Based on a Finite Element Formulation. In Proceedings of the Portuguese Conference on Automatic Control, Porto, Portugal, 15–17 September 1996. [Google Scholar]
- Manhong, Z. A modified finite difference model to the reverse recovery of silicon PIN diodes. Solid-State Electron. 2020, 171, 107839. [Google Scholar]
- Bryant, A.T.; Palmer, P.R.; Santi, E.; Hudgins, J.L. A Compact Diode Model for the Simulation of Fast Power Diodes including the Effects of Avalanche and Carrier Lifetime Zoning. In Proceedings of the IEEE Power Electronics Specialists Conference, Recife, Brazil, 12–16 June 2005; pp. 2042–2048. [Google Scholar]
- Liqing, L.; Angus, B.; Palmer, P.R.; Santi, E.; Hudgins, J.L. Physical Modeling of Fast p-i-n Diodes with Carrier Lifetime Zoning, Part I Device Model. IEEE Trans. Power Electron. 2008, 23, 189–197. [Google Scholar]
- Hernandez, L.; Claudio-Sanches, A.; Rodriguez, M.A.; Ponce-Silva, M. Physical Modeling of SiC Power Diodes with Empirical Approximation. J. Power Electron 2011, 11, 381–388. [Google Scholar] [CrossRef] [Green Version]
- Dyakonova, N.V.; Ivanov, P.A.; Kozlov, V.A.; Levinshtein, M.E.; Palmour, J.W.; Rumyantsev, S.L.; Singh, R. Steady-State and Transient Forward Current-Voltage Characteristics of 4H-Silicon Carbide 5.5. kV Diodes at High and Superhigh Current Densities. Trans. Electron. Devices 1999, 46, 2188–2193. [Google Scholar] [CrossRef]



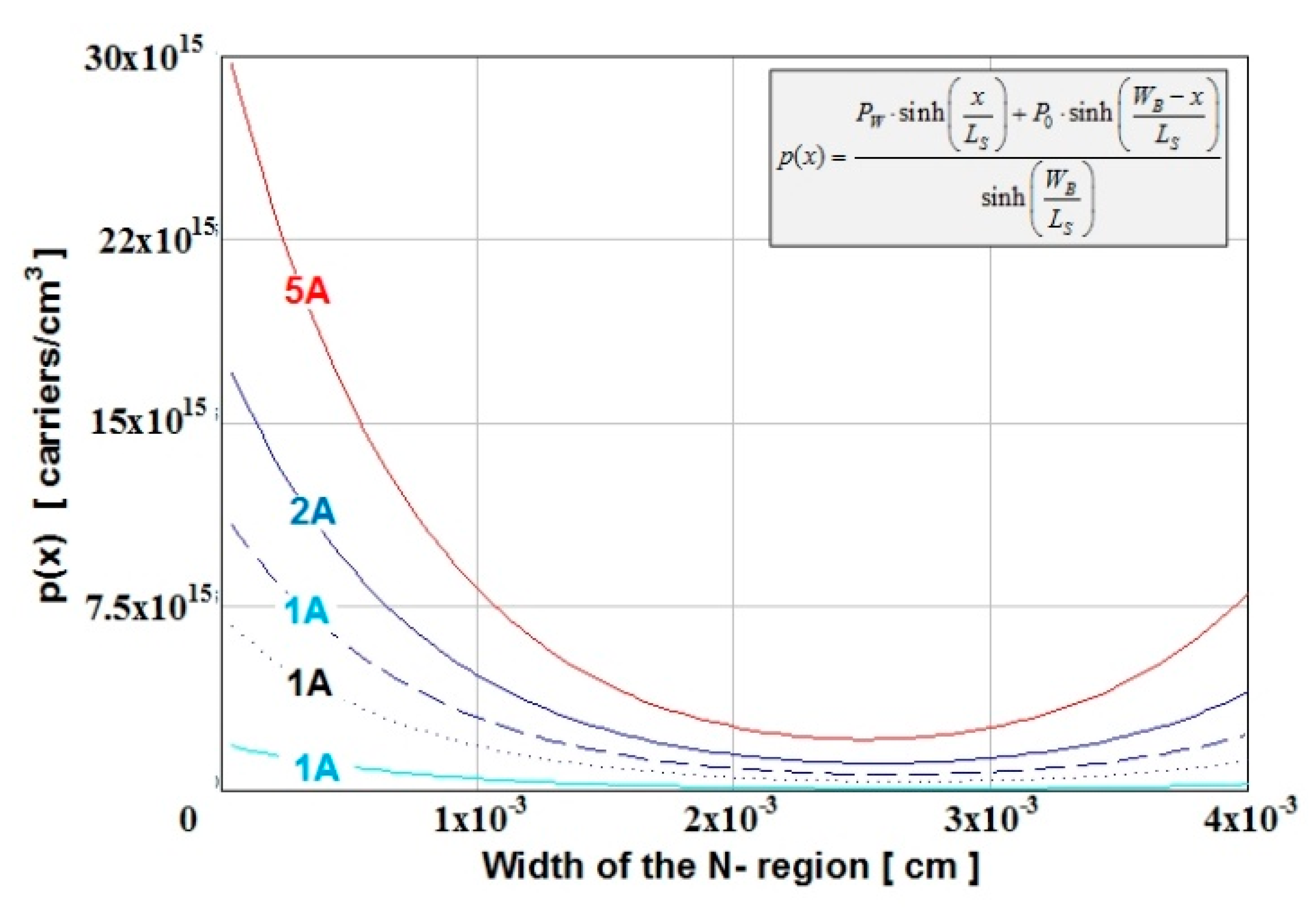


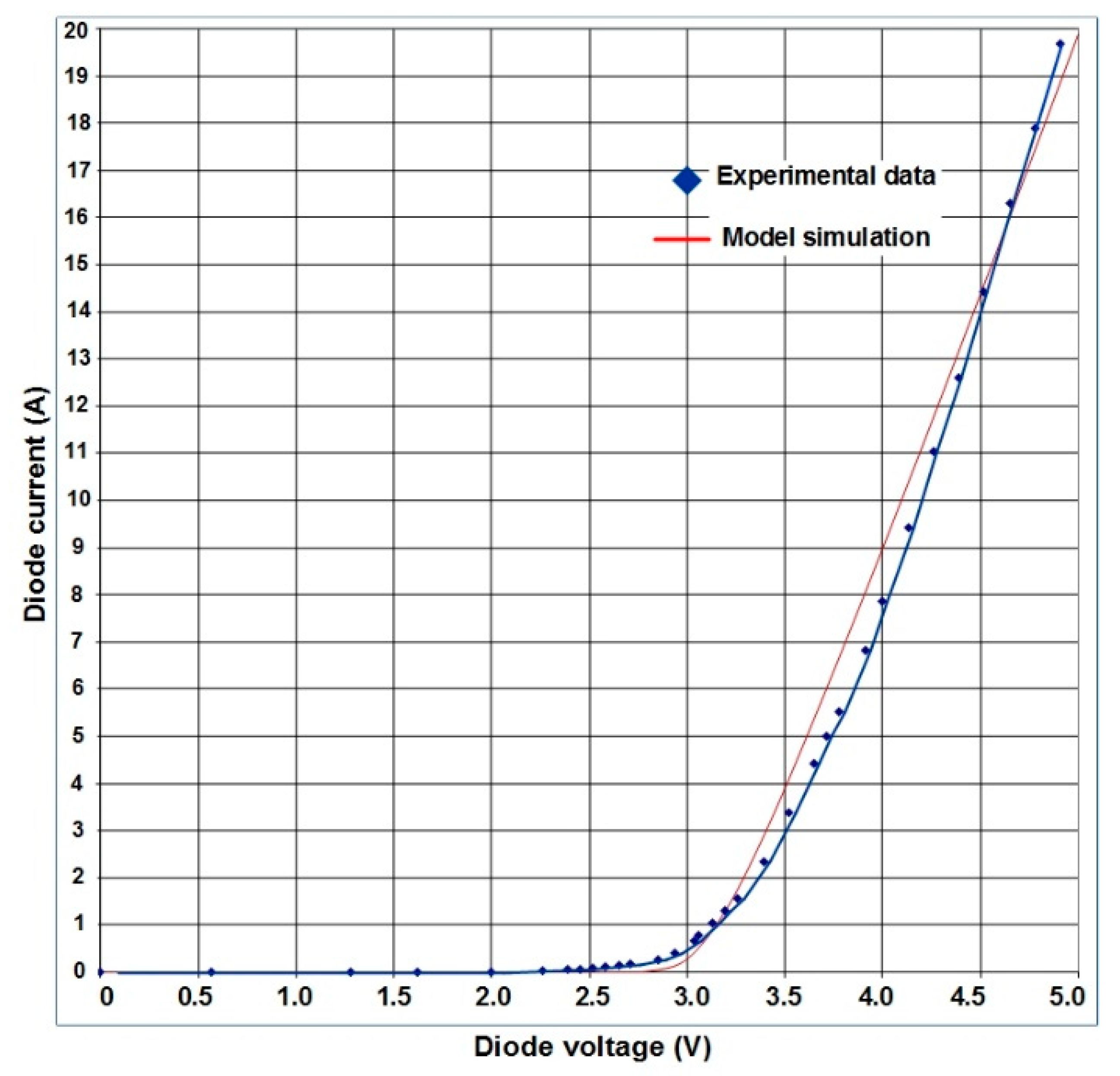
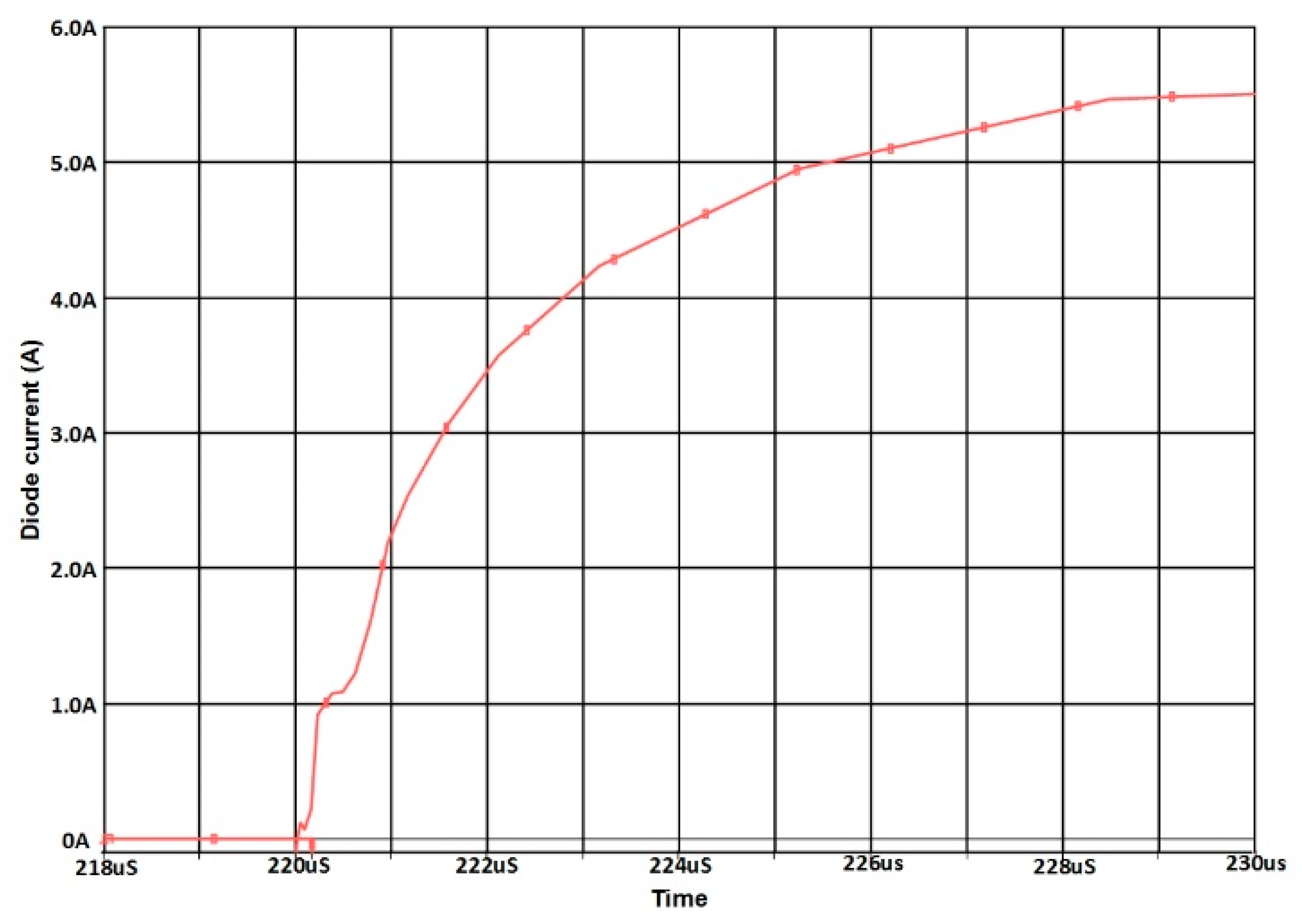

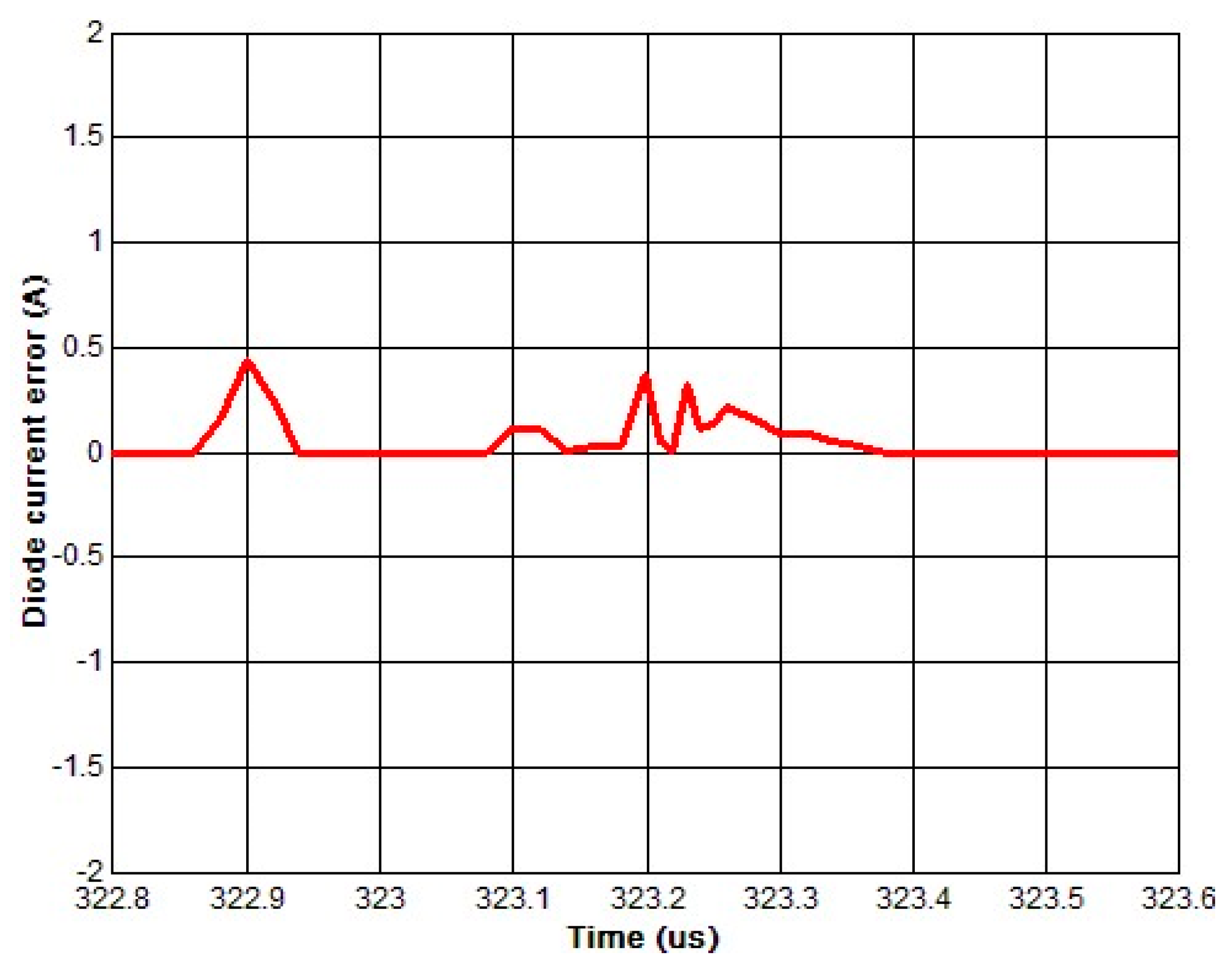
| Current [A] | P0 [Charges/cm3] | PW [Charges/cm3] |
|---|---|---|
| 0.5 | 8.043 × 1015 | 1.384 × 1015 |
| 1 | 1.310 × 1016 | 2.571 × 1015 |
| 1.5 | 1.712 × 1016 | 3.627 × 1015 |
| 2 | 2.056 × 1016 | 4.588 × 1015 |
| 2.5 | 2.362 × 1016 | 5.476 × 1015 |
| 3 | 2.640 × 1016 | 6.305 × 1015 |
| 3.5 | 2.897 × 1016 | 7.085 × 1015 |
| 4 | 3.137 × 1016 | 7.824 × 1015 |
| 4.5 | 3.362 × 1016 | 8.529 × 1015 |
| 5 | 3.576 × 1016 | 9.203 × 1015 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hernandez-Gonzalez, L.; Ramirez-Hernandez, J.; Ulises Juarez-Sandoval, O.; Olivares-Robles, M.A.; Sanchez, R.B.; Gibert Delgado, R.d.P. A New Approach for Approximate Solution of ADE: Physical-Based Modeling of Carriers in Doping Region. Mathematics 2021, 9, 458. https://doi.org/10.3390/math9050458
Hernandez-Gonzalez L, Ramirez-Hernandez J, Ulises Juarez-Sandoval O, Olivares-Robles MA, Sanchez RB, Gibert Delgado RdP. A New Approach for Approximate Solution of ADE: Physical-Based Modeling of Carriers in Doping Region. Mathematics. 2021; 9(5):458. https://doi.org/10.3390/math9050458
Chicago/Turabian StyleHernandez-Gonzalez, Leobardo, Jazmin Ramirez-Hernandez, Oswaldo Ulises Juarez-Sandoval, Miguel Angel Olivares-Robles, Ramon Blanco Sanchez, and Rosario del Pilar Gibert Delgado. 2021. "A New Approach for Approximate Solution of ADE: Physical-Based Modeling of Carriers in Doping Region" Mathematics 9, no. 5: 458. https://doi.org/10.3390/math9050458
APA StyleHernandez-Gonzalez, L., Ramirez-Hernandez, J., Ulises Juarez-Sandoval, O., Olivares-Robles, M. A., Sanchez, R. B., & Gibert Delgado, R. d. P. (2021). A New Approach for Approximate Solution of ADE: Physical-Based Modeling of Carriers in Doping Region. Mathematics, 9(5), 458. https://doi.org/10.3390/math9050458








