The Influence of an Integration Time Step on Dynamic Calculation of a Vehicle-Track-Bridge under High-Speed Railway
Abstract
:1. Introduction
2. Mechanical Model
2.1. High-Speed Train Model
2.2. Dynamic Model of Ballastless Track on Bridge
2.3. Wheel-Rail Interaction Relationship
2.4. Track Irregularity Model
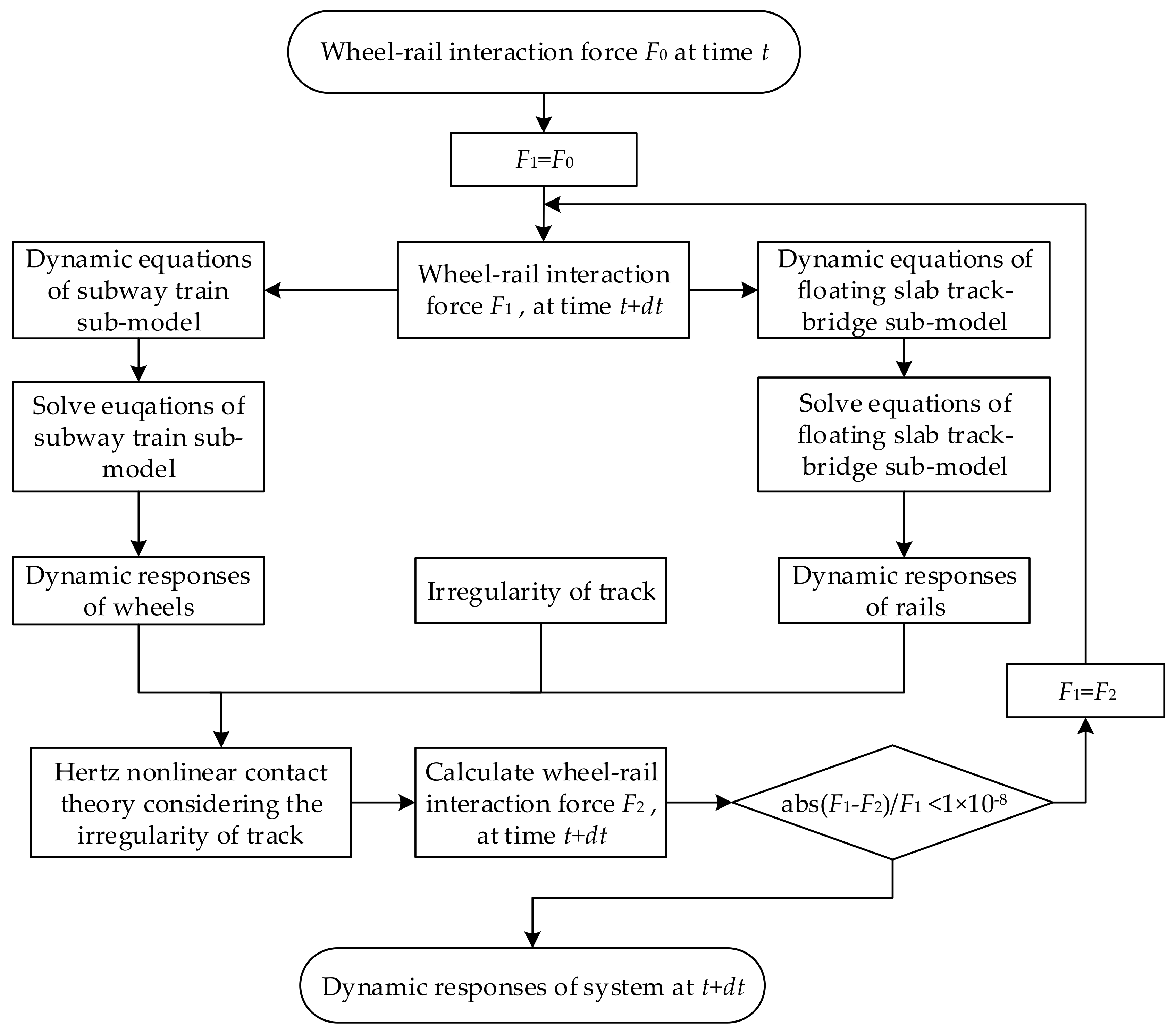
3. Calculation Process
3.1. System Equation
3.1.1. Equation of the Vehicle System
3.1.2. Equation of the Rail
3.1.3. Equation of the Track Slab
3.1.4. Equation of the Bridge
3.2. Calculation Method
3.3. System Parameters
3.4. Evaluation Index
3.4.1. Calculation Accuracy
3.4.2. Calculation Stability
4. Model Validation
5. Results and Discussion
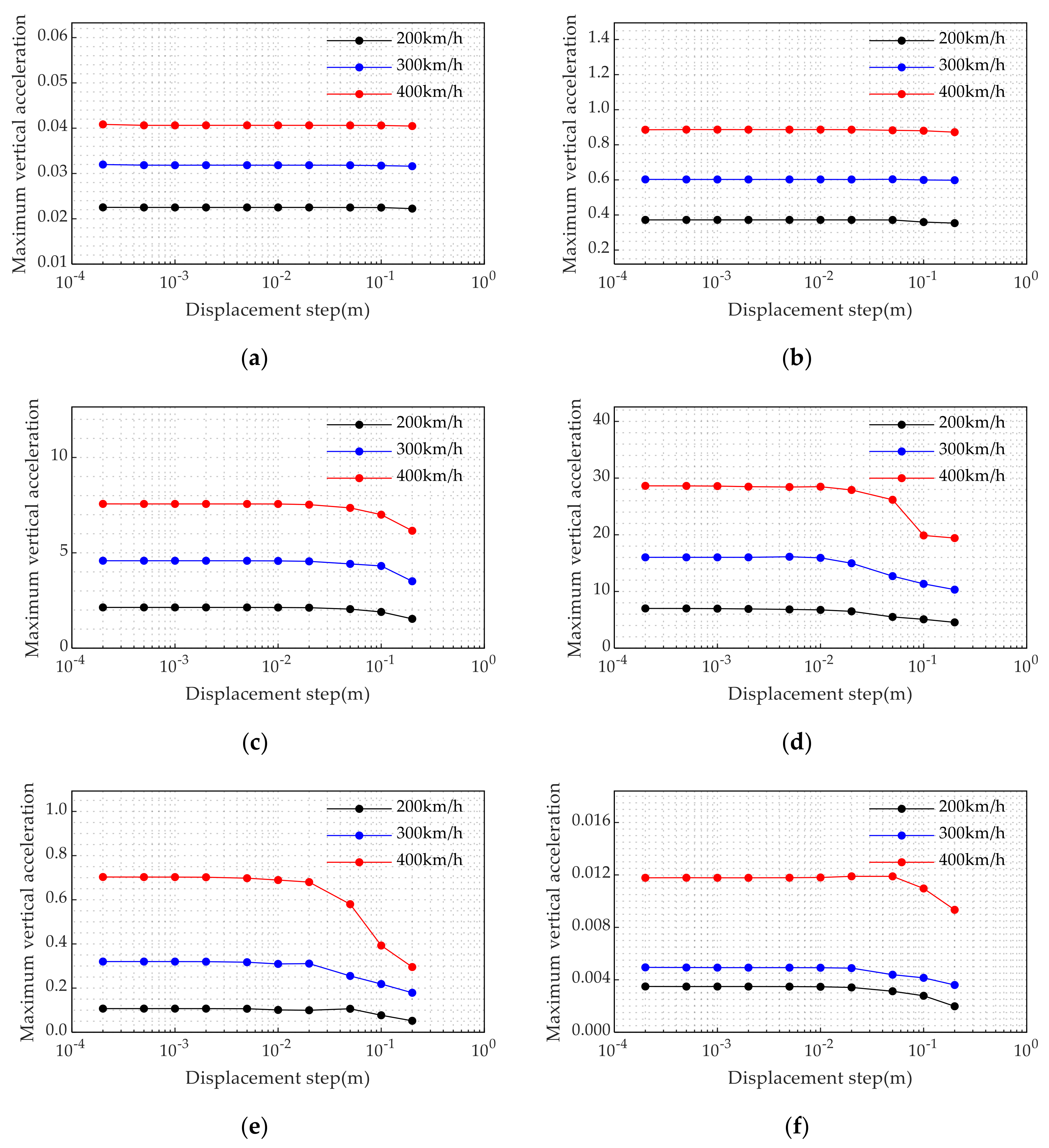
5.1. Effect under Different Speeds
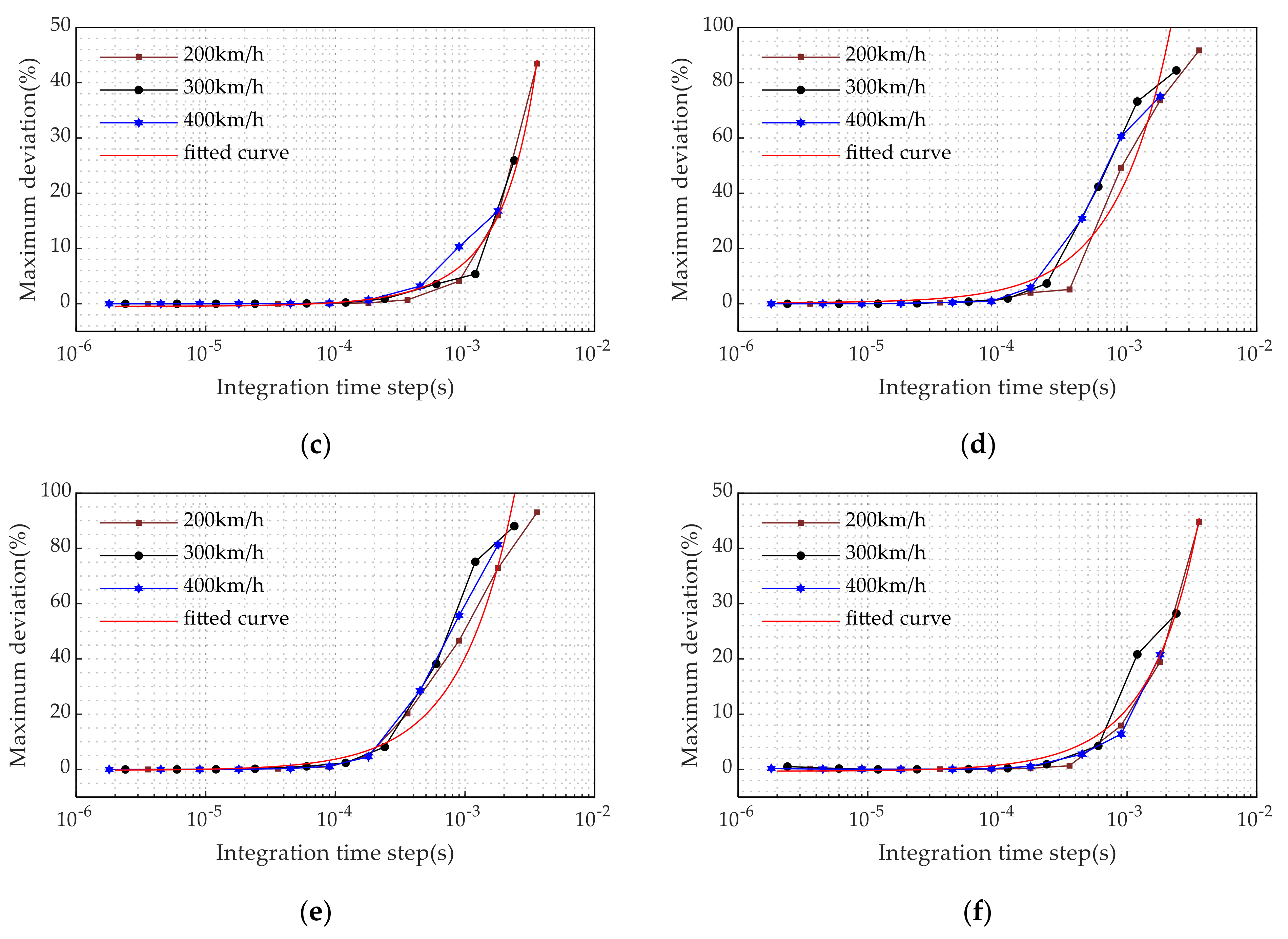
5.1.1. The Effect of Integration Time Step on Calculation Accuracy at Different Speeds
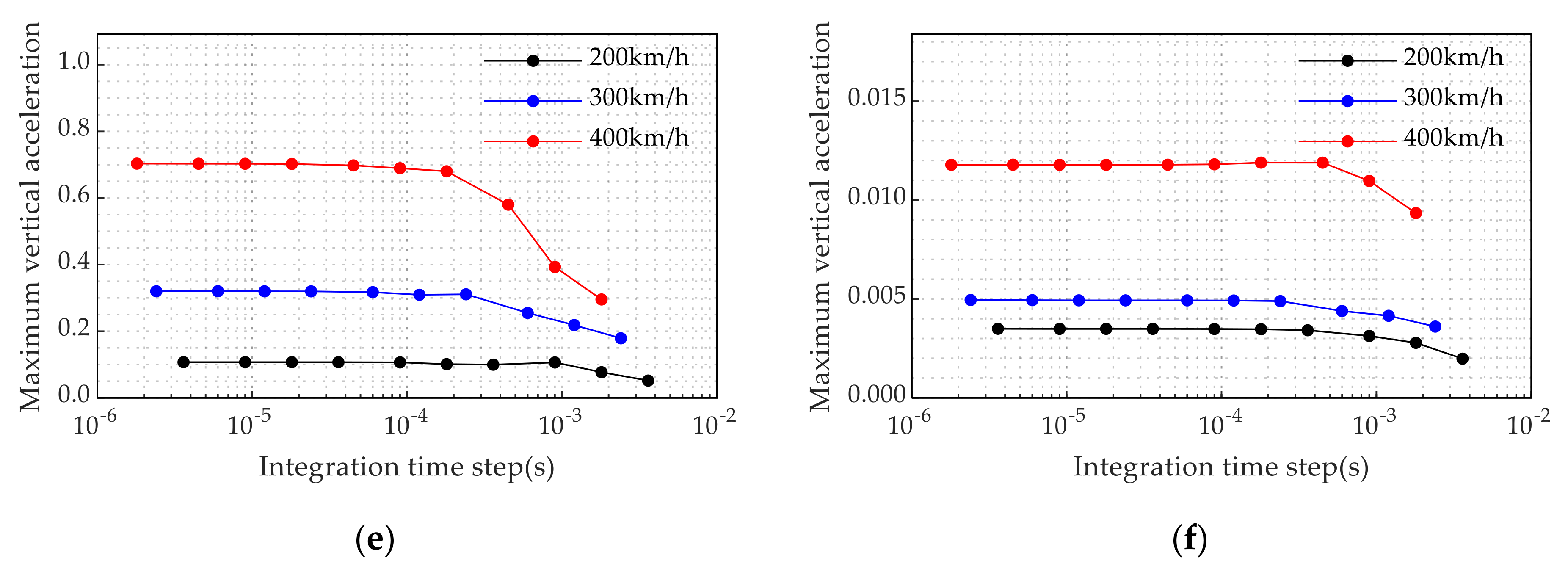
5.1.2. The Effect of the Integration Time Step on Calculation Stability at Different Speeds
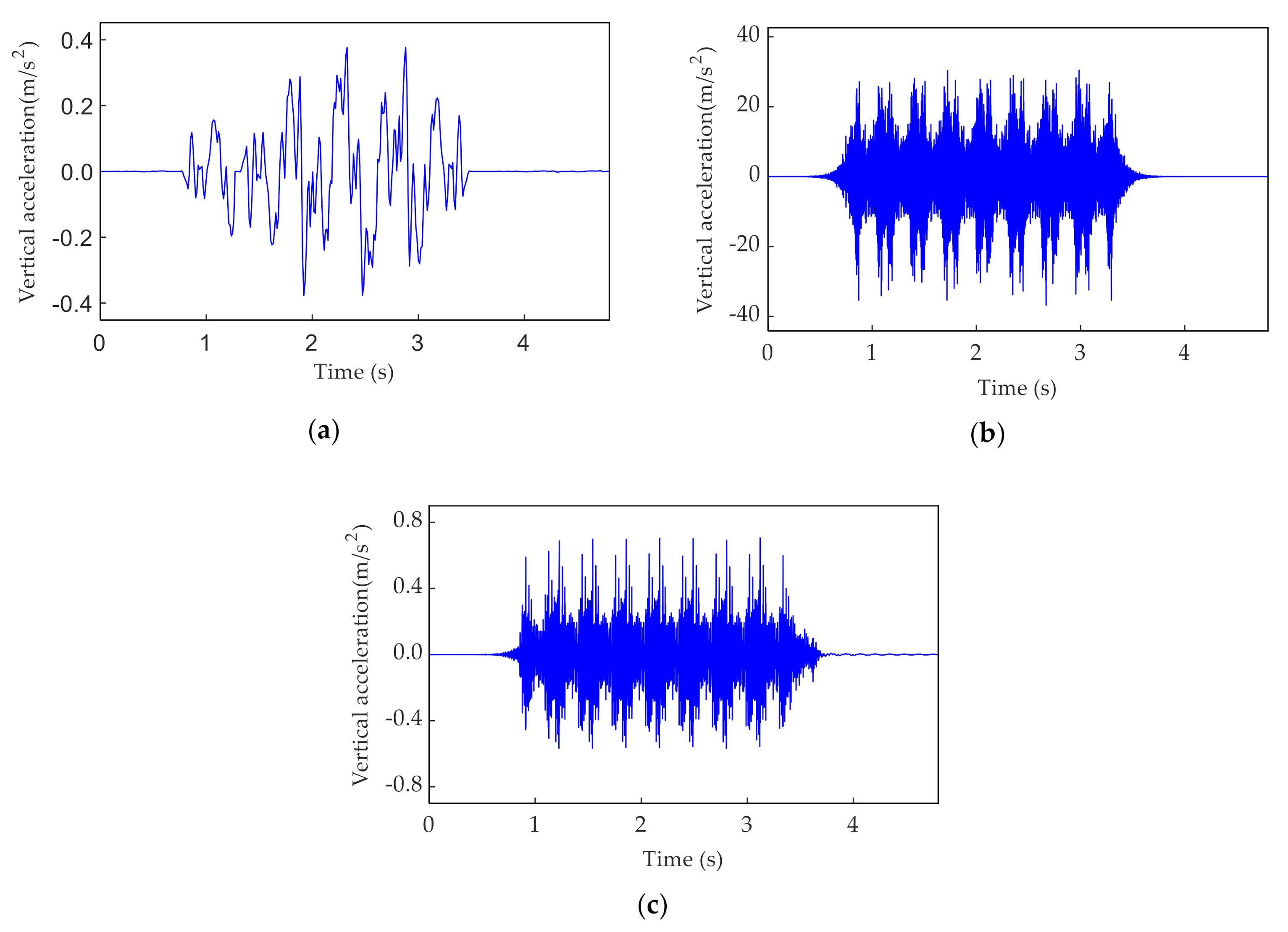
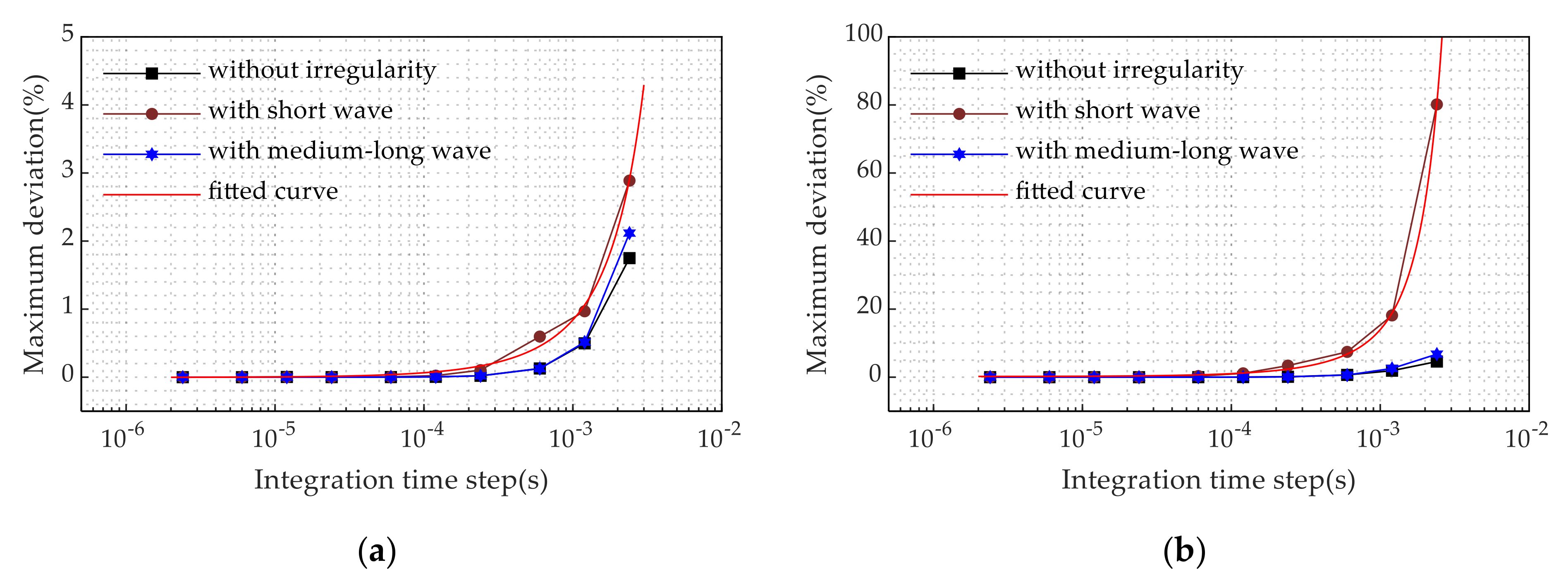
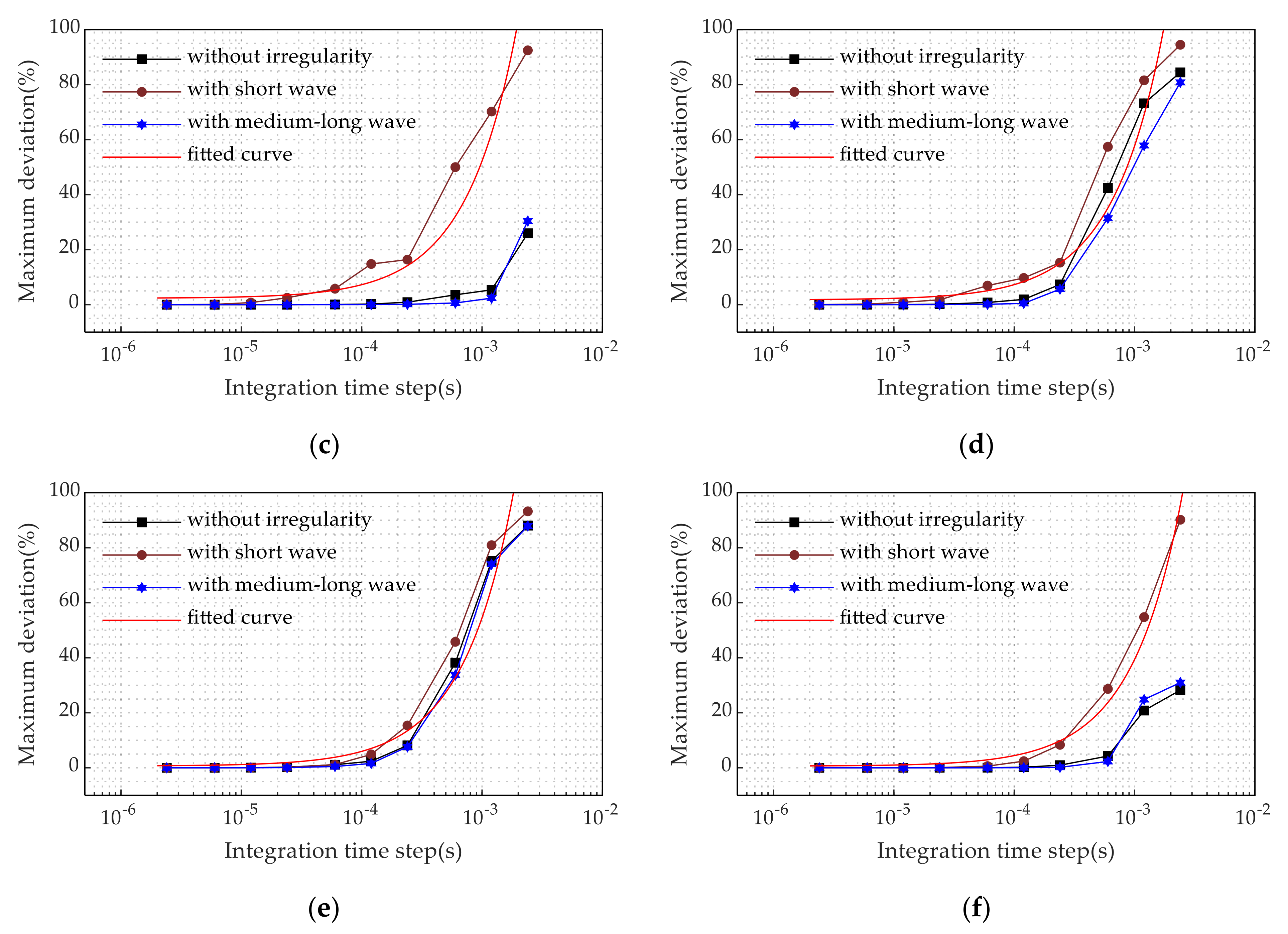
5.2. Effect under Different Track Irregularity States
5.2.1. The Effect of the Integration Time Step on Calculation Accuracy under Different Track Irregularity States
5.2.2. The Effect of the Integration Time Step on Calculation Stability under Different Track Irregularity States
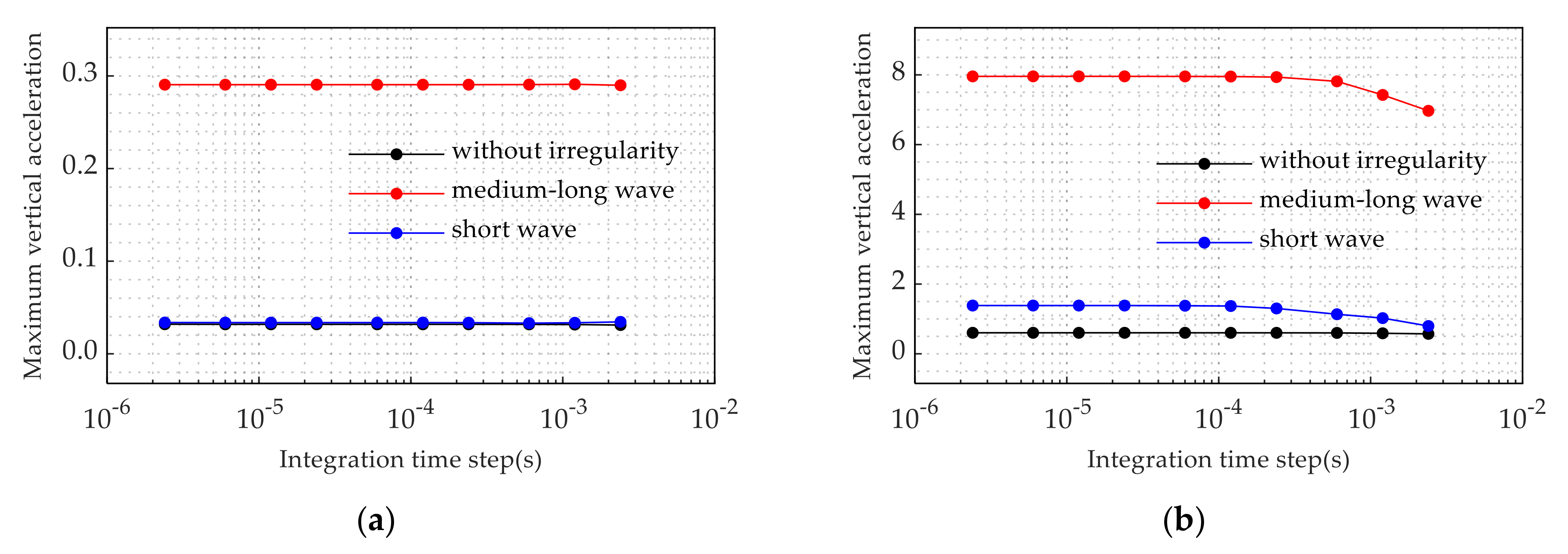
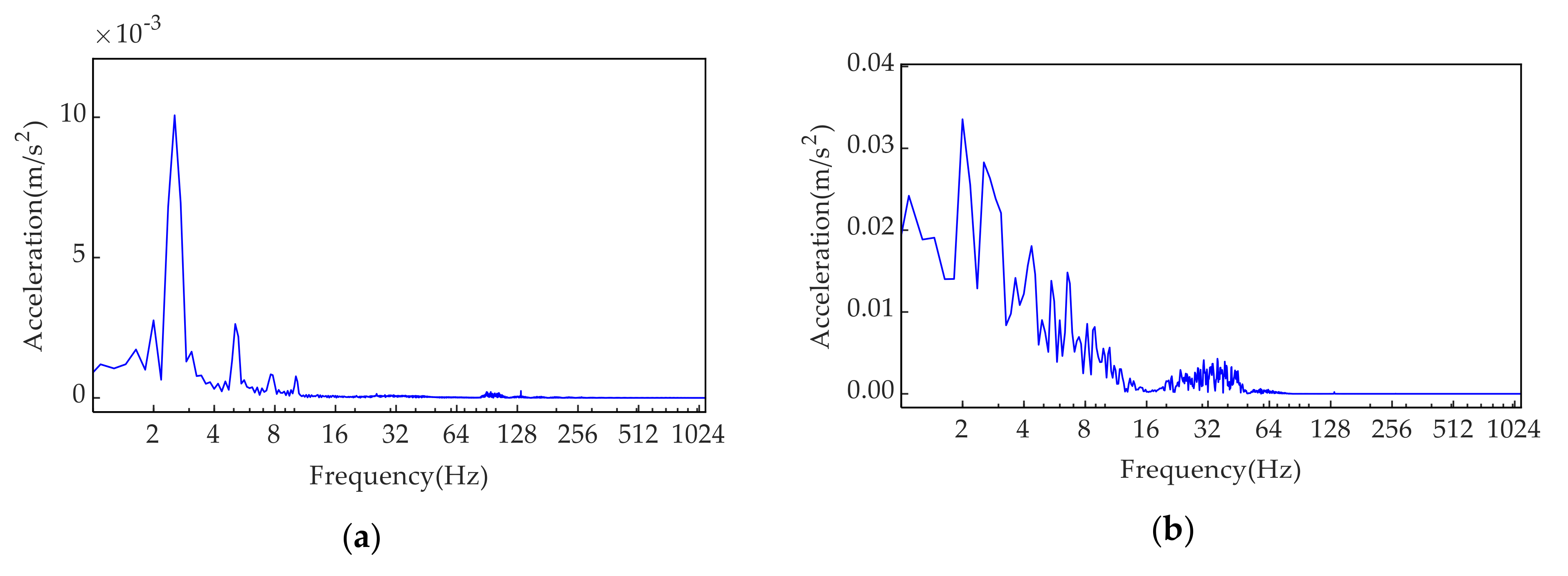
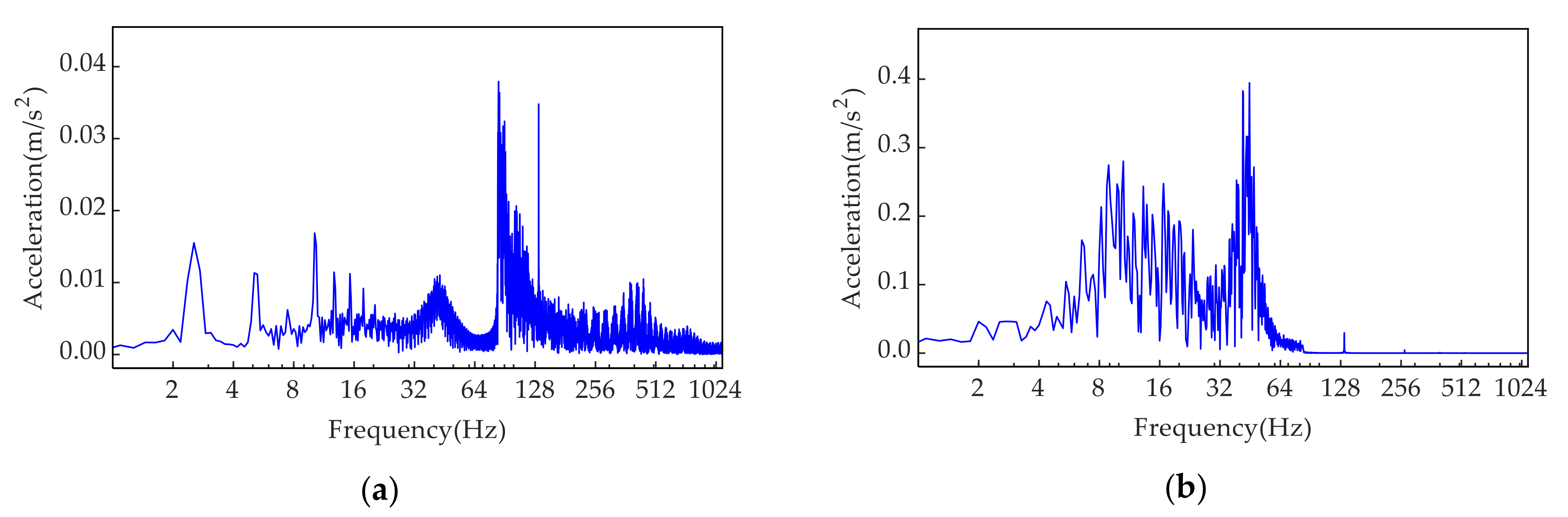
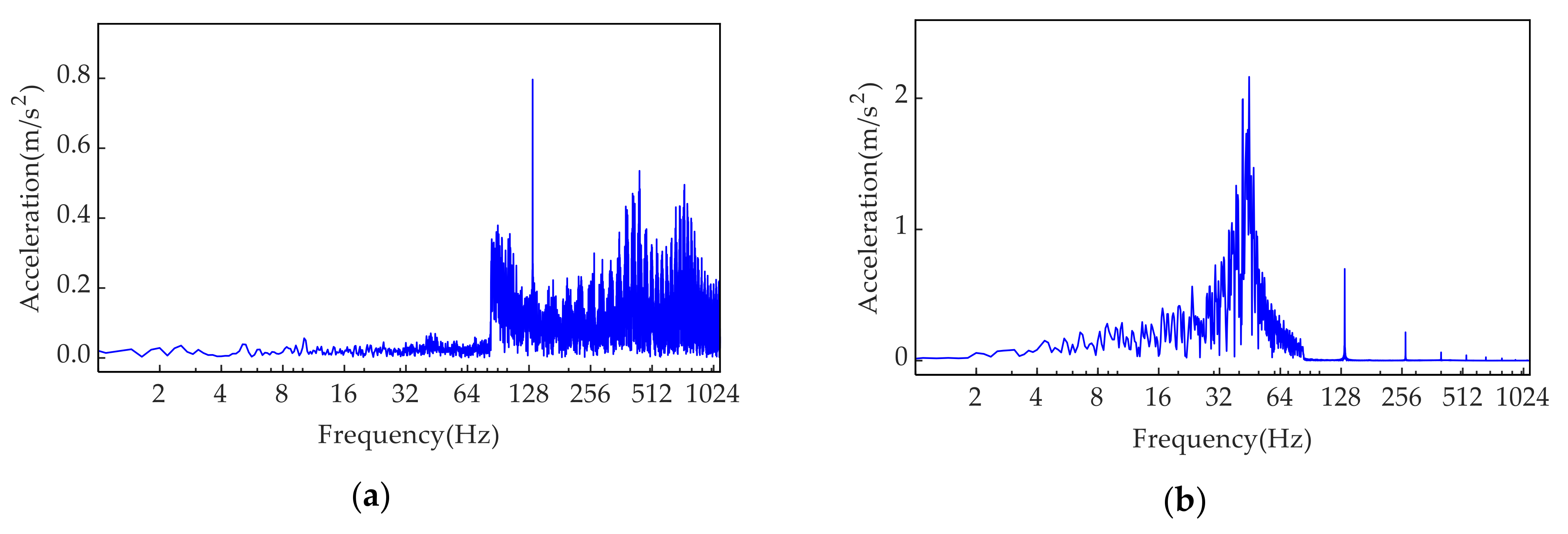
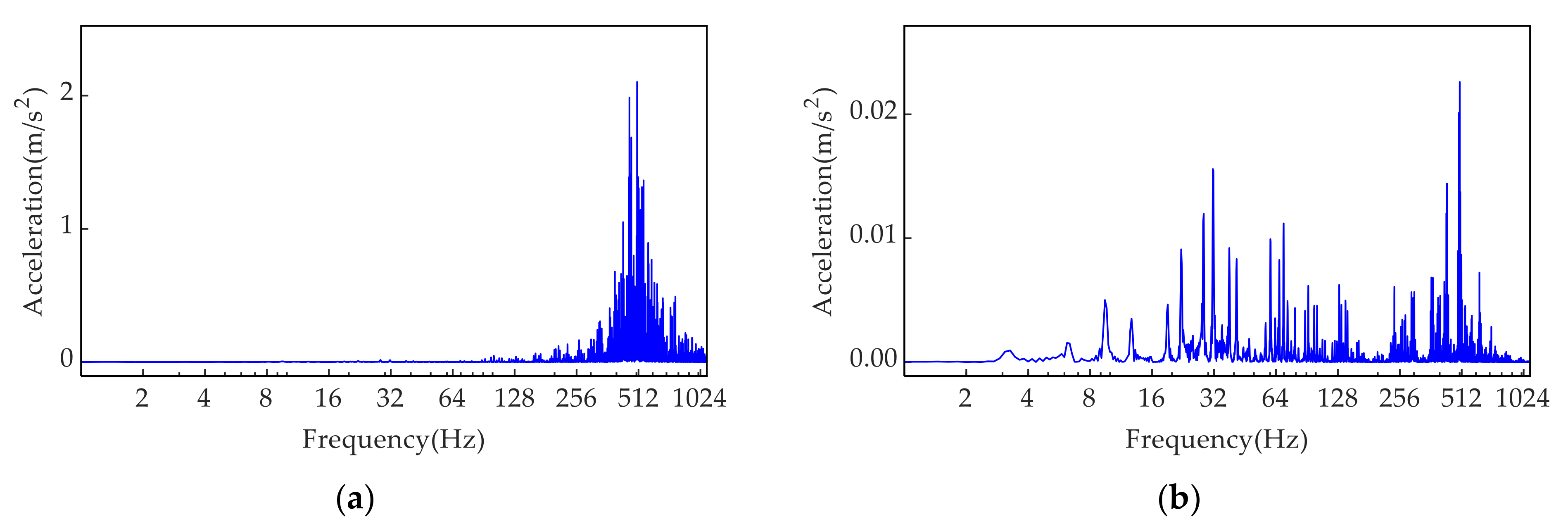
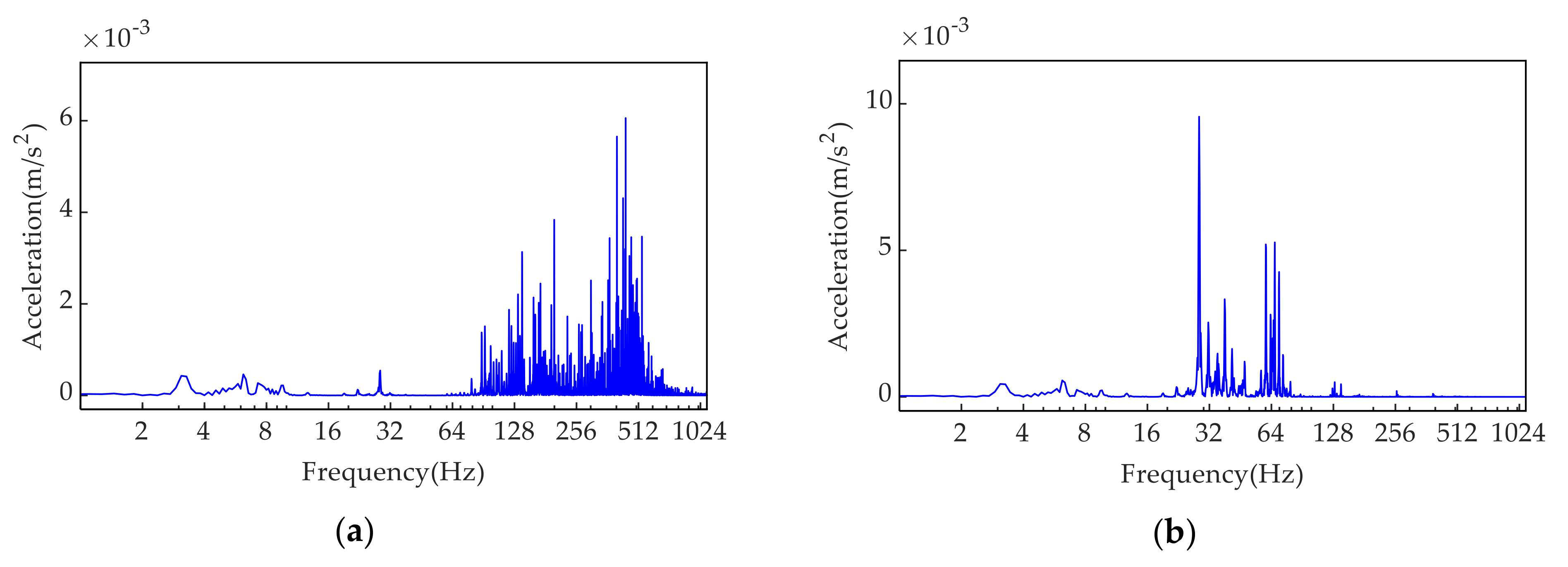
5.2.3. Spectrum Characteristic of Components under Different Track Irregularity States
6. Conclusions
- (1)
- The integration time step has a great influence on the calculation accuracy of each component, but the disparity among the effect of the integration time step on the calculation accuracy at different speeds is very small. The integration time step has a greater influence on the calculation deviation of the maximum vertical acceleration of the rail and track slab. The range of the integration time step of the car body, bogie, wheel-sets, rail, track slab and bridge should be less than 5.2 × 10−3, 4 × 10−3, 2 × 10−3, 1 × 10−3, 3 × 10−4 and 4 × 10−4 s, respectively.
- (2)
- The effect of the integration time step on the calculation stability of the maximum vertical acceleration of each component at different speeds is somewhat different, but the effect of the displacement step on the maximum vertical acceleration of each component at three speeds is more consistent. Higher speed requires a smaller integration time step to keep the calculation results stable. The mechanism of the effect of the integration time step on the calculation stability of the vehicle-track-bridge coupled system is that the corresponding displacement at the integration time step is different. The range of the displacement step of the bogie, wheel-sets, rail, track slab and bridge should be less than 0.2, 0.1, 0.02, 0.02 and 0.02 m, respectively.
- (3)
- The calculation deviation of the maximum vertical acceleration of the car body, bogie, wheel-sets, rail, track slab and bridge under track medium-long wave irregularity is more consistent with that under without track irregularity; however, the calculation deviation of the maximum vertical acceleration of the car body, wheel-sets and bridge under track short wave irregularity are greatly increased compared with that under without track irregularity. The range of integration time step of the car body, bogie, wheel-sets, rail, track slab and bridge under the track short wave irregularity state should be less than 5.9 × 10−3, 1.5 × 10−3, 2.4 × 10−4, 2.4 × 10−4, 2.4 × 10−4 and 4 × 10−4 s, respectively.
- (4)
- The effect of the integration time step on the calculation stability of the maximum vertical acceleration of each component under the track medium-long wave irregularity state is little, however, while the maximum vertical acceleration of the wheel-sets, rail, track slab and bridge under track short wave irregularity all show a significant declining trend. The range of the integration time step of the bogie, wheel-sets, rail, track slab and bridge under the track medium-long wave irregularity state should be less than 2 × 10−3, 2 × 10−3, 1.5 × 10−3, 3 × 10−4 and 1 × 10−3 s, respectively, and the range under the track short wave irregularity state should be less than 3 × 10−4, 6 × 10−5, 3 × 10−5, 2.4 × 10−4 and 1.5 × 10−4 s, respectively.
- (5)
- Short wave irregularity mainly excites high-frequency vibration, and medium-long wave irregularity mainly excites low-frequency vibration. The larger the vibration frequency is, the smaller the range of the integration time step is for dynamic calculation to maintain calculation stability.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, J.; Wang, P.; An, B.; Ma, X.; Chen, R. Damage detection of ballastless railway tracks by the impact-echo method. Proc. Inst. Civ. Eng. Transp. 2018, 171, 106–114. [Google Scholar] [CrossRef]
- Zhu, S.; Fu, Q.; Cai, C.; Spanos, P.D. Damage evolution and dynamic response of cement asphalt mortar layer of slab track under vehicle dynamic load. Sci. China Ser. E Technol. Sci. 2014, 57, 1883–1894. [Google Scholar] [CrossRef]
- Han, J.; Zhao, G.; Xiao, X.; Jin, X. Effect of cement asphalt mortar damage location on dynamic behavior of high-speed track. Adv. Mech. Eng. 2018, 10. [Google Scholar] [CrossRef] [Green Version]
- Liang, H.; Liu, P.; Wang, T.; Wang, H.; Zhang, K.; Cao, Y.; An, D. Influence of wheel polygonal wear on wheel-rail dynamic contact in a heavy-haul locomotive under traction conditions. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2020. [Google Scholar] [CrossRef]
- Shan, Y.; Zhou, S.; Zhou, H.; Wang, B.; Zhao, Z.; Shu, Y.; Yu, Z. Iterative Method for Predicting Uneven Settlement Caused by High-Speed Train Loads in Transition-Zone Subgrade. Transp. Res. Rec. J. Transp. Res. Board 2017, 2607, 7–14. [Google Scholar] [CrossRef]
- Liu, J.; He, Y.; Zhang, J.; Hong, J.; Wen, X.; Xiao, J.; Wang, F.; Zheng, X. Polymer Injection Rehabilitation Technology for Lifting Differential Settlement of Turnout Ballastless Track. In Proceedings of the MATEC Web of Conferences, Cape Town, South Africa, 19–21 November 2018. [Google Scholar]
- Zhai, W. The vertical model of vehicle-track system and its coupling dynamics. J. China Railw. Soc. 1992, 3, 10–21. [Google Scholar]
- Xin, T.; Wang, P.; Ding, Y. Effect of Long-Wavelength Track Irregularities on Vehicle Dynamic Responses. Shock. Vib. 2019, 2019, 1–11. [Google Scholar] [CrossRef]
- Gao, J.; Zhai, W.; Guo, Y. Wheel–rail dynamic interaction due to rail weld irregularity in high-speed railways. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2018, 232, 249–261. [Google Scholar] [CrossRef]
- Palomo, M.L.; Barceló, F.R.; Llario, F.R.; Herráiz, J.R. Effect of vehicle speed on the dynamics of track transitions. J. Vib. Control 2017. [Google Scholar] [CrossRef]
- Li, T.; Su, Q.; Kaewunruen, S. Influences of dynamic material properties of slab track components on the train-track vibration interactions. Eng. Fail. Anal. 2020, 115, 104633. [Google Scholar] [CrossRef]
- Yang, H.; Chen, Z.; Zhang, H.; Fan, J. Dynamic Analysis of Train-Rail-Bridge Interaction considering Concrete Creep of a Multi-Span Simply Supported Bridge. Adv. Struct. Eng. 2014, 17, 709–720. [Google Scholar] [CrossRef]
- Ling, L.; Dhanasekar, M.; Thambiratnam, D.P. Dynamic response of the train–track–bridge system subjected to derailment impacts. Veh. Syst. Dyn. 2017, 56, 638–657. [Google Scholar] [CrossRef]
- Zhai, W.M.; Wang, Q.C.; Lu, Z.W.; Wu, X.S. Dynamic effects of vehicles on tracks in the case of raising train speeds. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2001, 215, 125–135. [Google Scholar] [CrossRef]
- Zhai, W.; Xia, H.; Cai, C.; Gao, M.; Li, X.; Guo, X.; Zhang, N.; Wang, K. High-speed train–track–bridge dynamic interactions—Part I: Theoretical model and numerical simulation. Int. J. Rail Transp. 2013, 1, 3–24. [Google Scholar] [CrossRef]
- Chen, Z.; Zhai, W.; Yin, Q. Analysis of structural stresses of tracks and vehicle dynamic responses in train–track–bridge system with pier settlement. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2018, 232, 421–434. [Google Scholar] [CrossRef]
- Chen, Z.; Zhai, W.; Cai, C.; Sun, Y. Safety threshold of high-speed railway pier settlement based on train-track-bridge dynamic interaction. Sci. China Ser. E Technol. Sci. 2014, 58, 202–210. [Google Scholar] [CrossRef]
- Xiao, X.; Yan, Y.; Chen, B. Stochastic dynamic analysis for vehicle-track-bridge system based on probability density evolution method. Eng. Struct. 2019, 188, 745–761. [Google Scholar] [CrossRef]
- A Zakeri, J.; Feizi, M.M.; Shadfar, M.; Naeimi, M. Sensitivity analysis on dynamic response of railway vehicle and ride index over curved bridges. Proc. Inst. Mech. Eng. Part K J. Multi-body Dyn. 2016, 231, 266–277. [Google Scholar] [CrossRef]
- Xia, H.; Guo, W.; Zhang, N.; Sun, G. Dynamic analysis of a train–bridge system under wind action. Comput. Struct. 2008, 86, 1845–1855. [Google Scholar] [CrossRef]
- Olmos, J.M.; Astiz, M.A. Analysis of the lateral dynamic response of high pier viaducts under high-speed train travel. Eng. Struct. 2013, 56, 1384–1401. [Google Scholar] [CrossRef]
- Xia, H.; Han, Y.; Zhang, N.; Guo, W. Dynamic analysis of train–bridge system subjected to non-uniform seismic excitations. Earthq. Eng. Struct. Dyn. 2006, 35, 1563–1579. [Google Scholar] [CrossRef]
- Zhai, W.; Wang, K.; Cai, C. Fundamentals of vehicle–track coupled dynamics. Veh. Syst. Dyn. 2009, 47, 1349–1376. [Google Scholar] [CrossRef]
- Zhu, Z.; Gong, W.; Wang, L.; Li, Q.; Bai, Y.; Yu, Z.; Harik, I.E. An efficient multi-time-step method for train-track-bridge interaction. Comput. Struct. 2018, 196, 36–48. [Google Scholar] [CrossRef]
- Jin, Z.; Hu, C.; Pei, S.; Liu, H. An integrated explicit–implicit algorithm for vehicle–rail–bridge dynamic simulations. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2018, 232, 1895–1913. [Google Scholar] [CrossRef]
- Zhai, W. Vehicle-Track Coupled Dynamics, 4th ed.; Science Press: Beijing, China, 2015; p. 41. [Google Scholar]
- Xu, Q.; Chen, X.; Yan, B.; Guo, W. Study on vibration reduction slab track and adjacent transition section in high-speed railway tunnel. J. Vibroeng. 2015, 17, 905–916. [Google Scholar]
- Sato, Y. Study on High-Frequency Vibrations in Track Operated with High-Speed Trains; Railway Technical Research Institute, Quarterly Reports; National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 1977; p. 18. [Google Scholar]
- Zeng, Q. The principle of total potential energy with stationary value in elastic system dynamics. J. Hua Zhong Univ. Sci. Technol. 2000, 1–3, 113163909. [Google Scholar]
- Lou, P.; Zeng, Q.-Y. Formulation of Equations of Motion for a Simply Supported Bridge under a Moving Railway Freight Vehicle. Shock. Vib. 2007, 14, 429–446. [Google Scholar] [CrossRef]
- Lou, P. Finite element analysis for train–track–bridge interaction system. Arch. Appl. Mech. 2007, 77, 707–728. [Google Scholar] [CrossRef]
- Wu, Y.-S.; Yang, Y.-B. Steady-state response and riding comfort of trains moving over a series of simply supported bridges. Eng. Struct. 2003, 25, 251–265. [Google Scholar] [CrossRef]
- Newmark, N.M. A method of computation for stuctural dynamics. J. Eng. Mech. Div. 1959, 85, 67–94. [Google Scholar] [CrossRef]
- Lou, P.; Zeng, Q. Formulation of equations of vertical motion for vehicle-track-bridge system. J. China Railw. Soc. 2004, 5, 71–80. [Google Scholar]
- Xu, J. Study on Random Vibration Analysis of High Speed Vehicle track Coupling System and Evaluation Method of Track Irregularity. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2016. [Google Scholar]
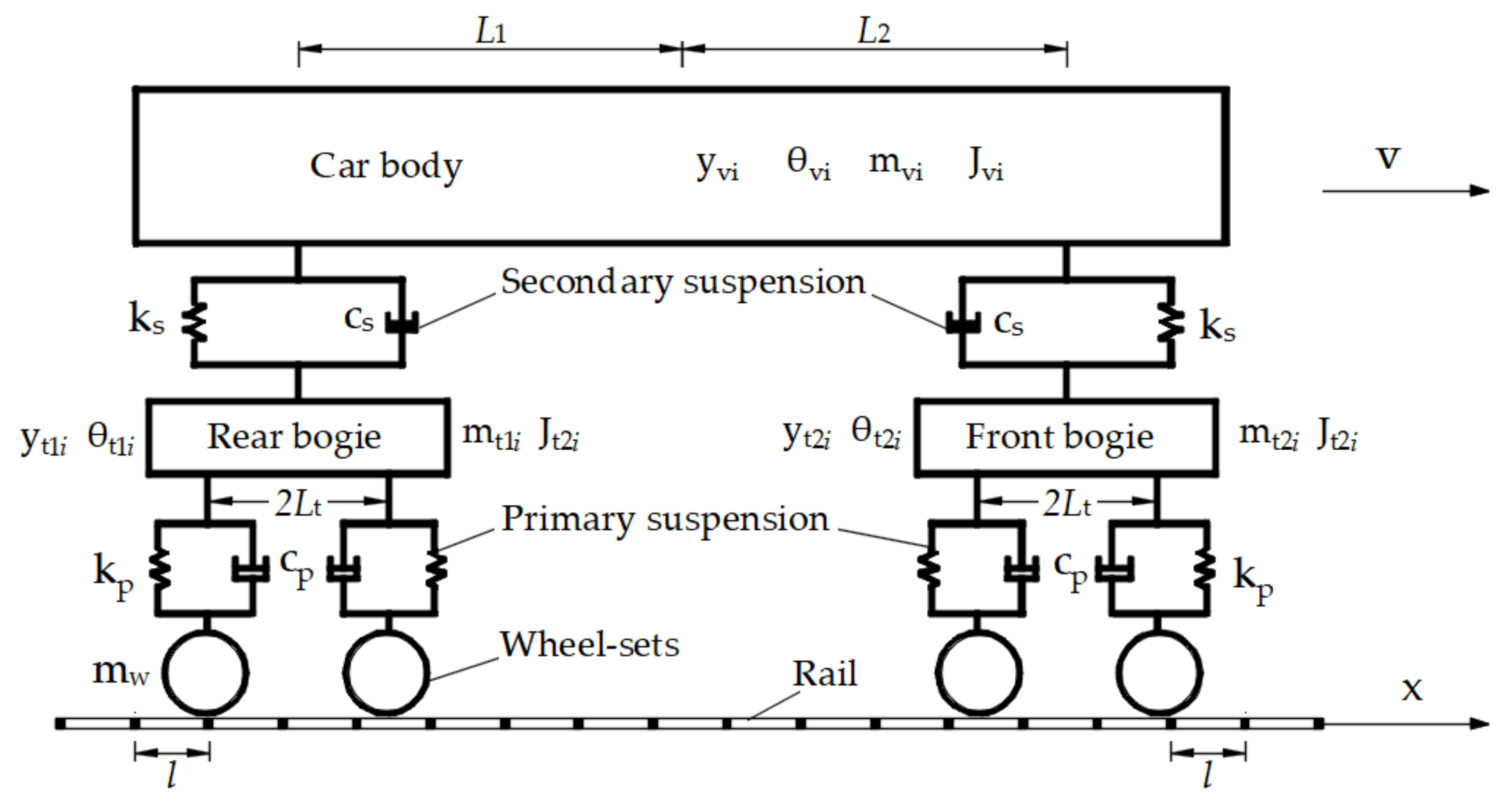
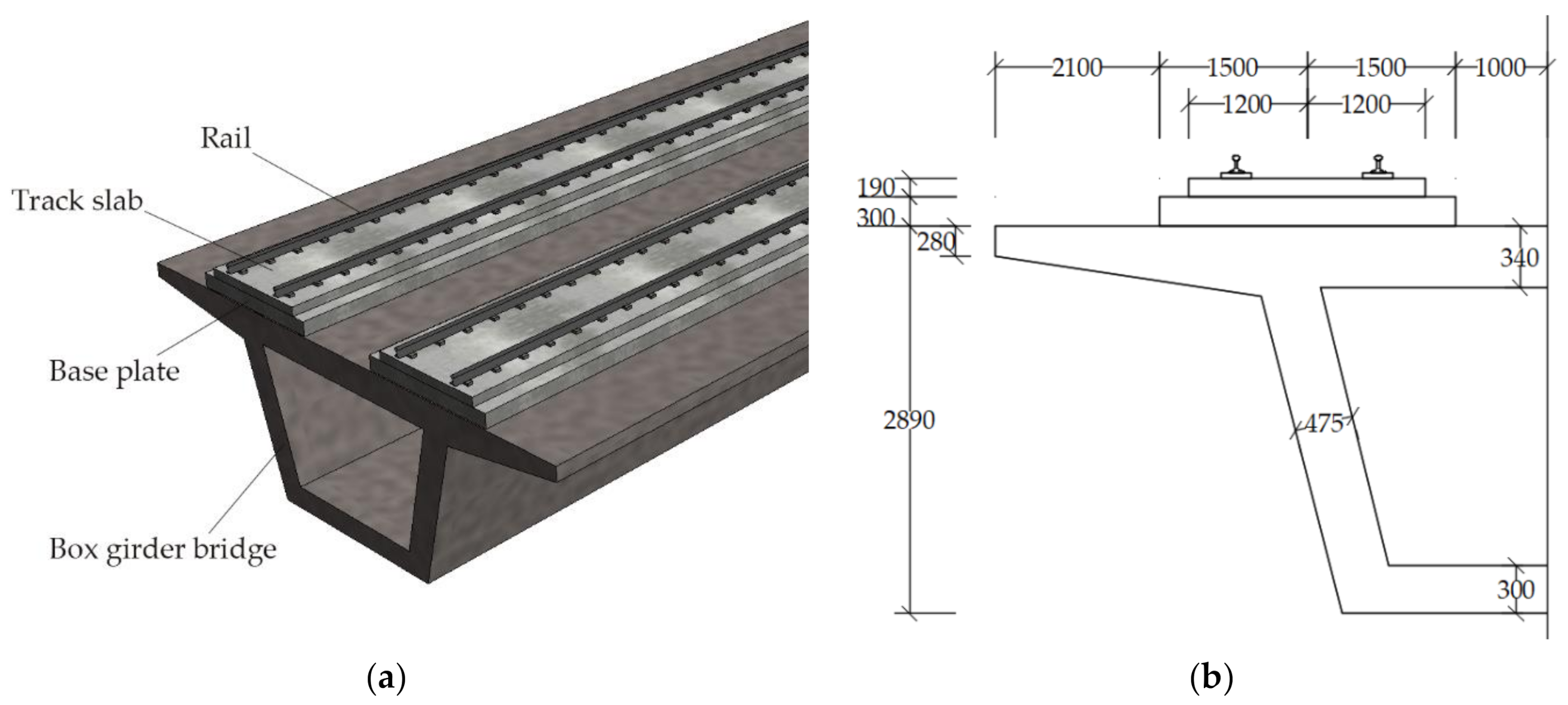

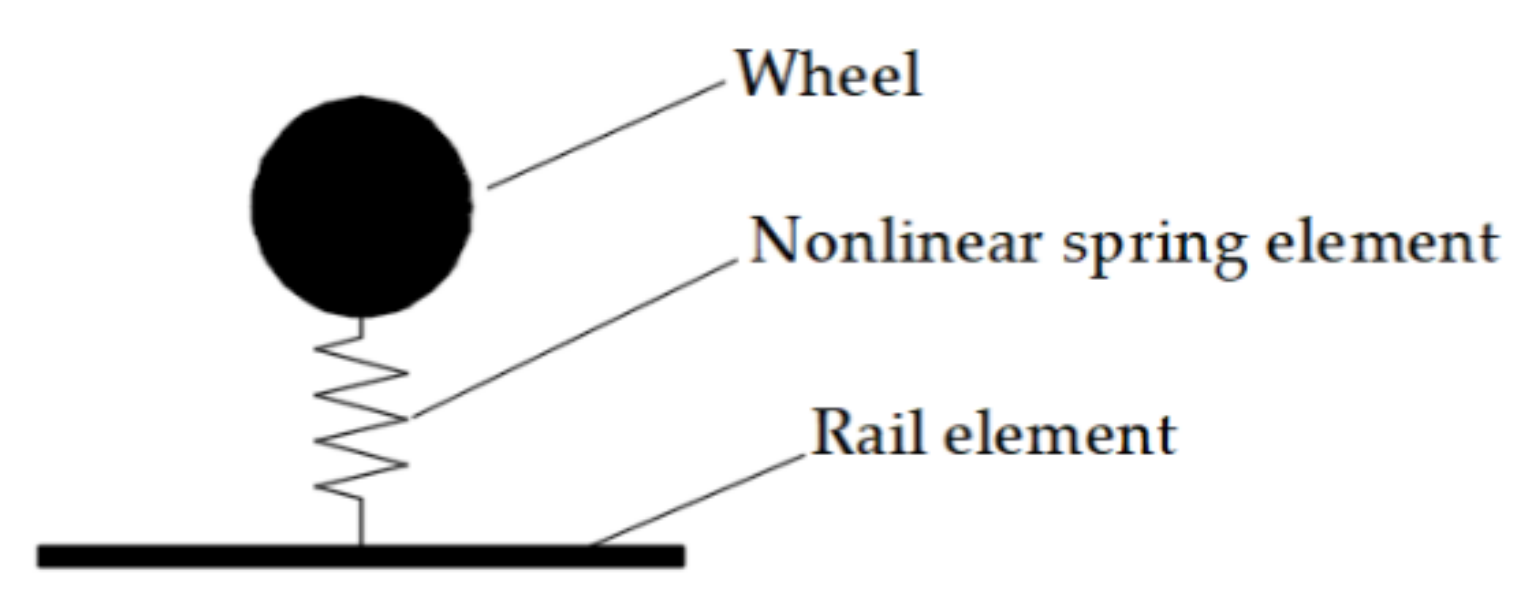
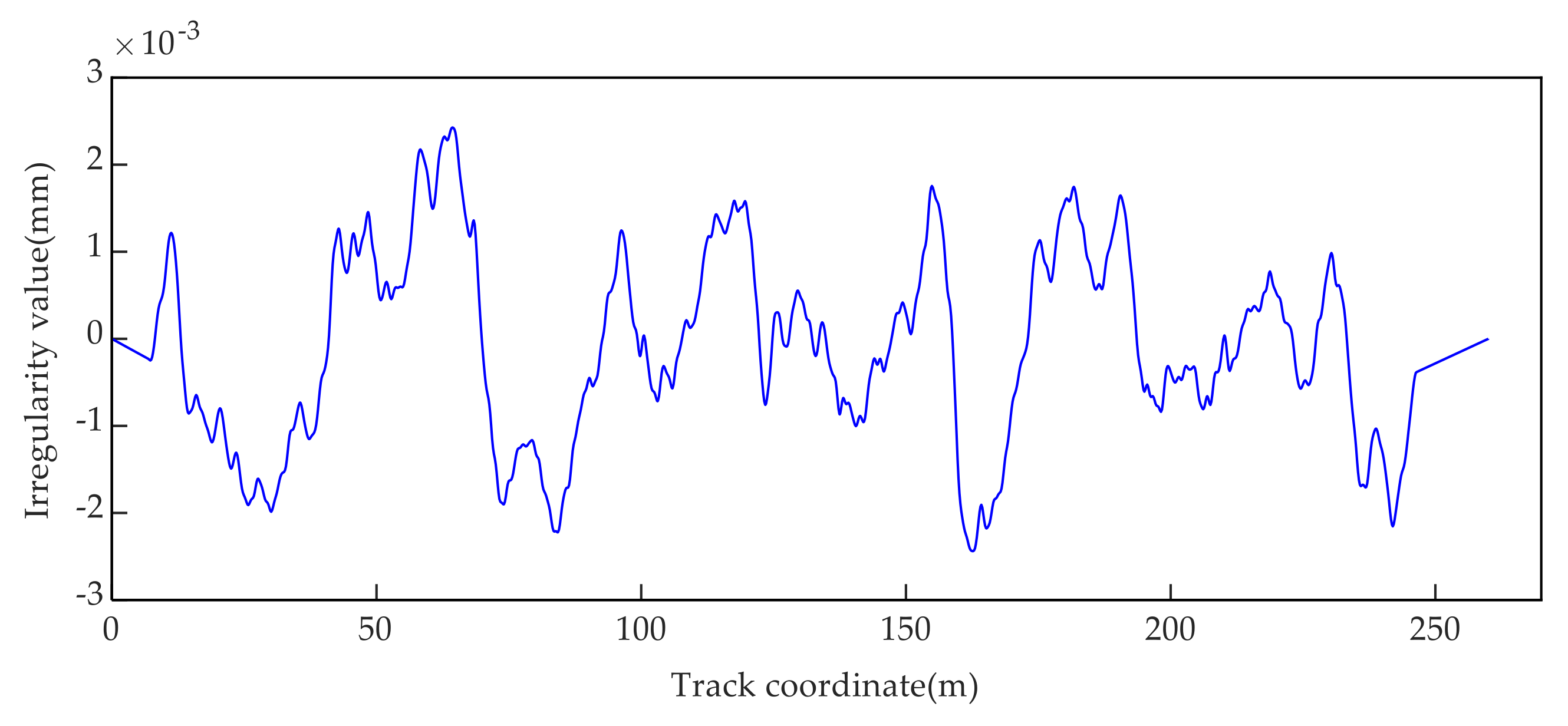





| Parameter | Notation | Value |
|---|---|---|
| Mass of the car body | 19,800 kg | |
| Mass of the wheel-sets | 1000 kg | |
| Rotational inertia of the car body | 970,200 kg·m2 | |
| Mass of the rear bogie | 1600 kg | |
| Mass of the front bogie | 1600 kg | |
| Rotational inertia of the rear bogie | 876 kg·m2 | |
| Rotational inertia of the front bogie | 876 kg·m2 | |
| Vertical damping of the primary suspension | 19,600 N·s·m−1 | |
| Vertical stiffness of the primary suspension | 1,176,000 N·m−1 | |
| Vertical damping of the secondary suspension | 19,600 N·s·m−1 | |
| Vertical stiffness of the secondary suspension | 441,000 N·m−1 | |
| Half of the bogie axle base | 0.625 m | |
| Mass of the wheel-sets | 1000 kg | |
| Distance between the center of mass of the Car body and rear wheel-sets | 8.75 m | |
| Distance between the center of mass of the Car body and front wheel-sets | 8.75 m | |
| Speed of the train | 200, 300 and 400 km/h |
| Parameter | Notation | Value |
|---|---|---|
| Elastic modulus of the rail | 210 GPa | |
| Inertia moment of the rail | 3217 cm4 | |
| Density of the rail | 7800 kg·m−3 | |
| Spacing of the fastener | 0.625 m | |
| Stiffness coefficient between the rail and track slab | 30 kN·mm−1 | |
| Damping coefficient between the rail and track slab | 20 kN·s·m−1 | |
| Stiffness coefficient between the track slab and bridge | 30 kN·mm−1 | |
| Damping coefficient between the track slab and bridge | 20 kN·s·m−1 | |
| Elastic modulus of the track slab | 46.8 GPa | |
| Inertia moment of the track slab | 337,500 cm4 | |
| Density of the bridge | 2500 kg·m−3 | |
| Inertia moment of the bridge | 5.49 × 108 cm4 | |
| Elastic modulus of the bridge | 44.85 GPa | |
| Density of the bridge | 2500 kg·m−3 |
| Displacement Step(m) | Integration Time Step(s) | ||
|---|---|---|---|
| 200 km/h | 300 km/h | 400 km/h | |
| 1/5 | 3.6 × 10−3 | 2.4 × 10−3 | 1.8 × 10−3 |
| 1/10 | 1.8 × 10−3 | 1.2 × 10−3 | 9.0 × 10−4 |
| 1/20 | 9.0 × 10−4 | 6.0 × 10−4 | 4.5 × 10−4 |
| 1/50 | 3.6 × 10−4 | 2.4 × 10−4 | 1.8 × 10−4 |
| 1/100 | 1.8 × 10−4 | 1.2 × 10−4 | 9.0 × 10−5 |
| 1/200 | 9.0 × 10−5 | 6.0 × 10−5 | 4.5 × 10−5 |
| 1/500 | 3.6 × 10−5 | 2.4 × 10−5 | 1.8 × 10−5 |
| 1/1000 | 1.8 × 10−5 | 1.2 × 10−5 | 9.0 × 10−6 |
| 1/2000 | 9.0 × 10−6 | 6.0 × 10−6 | 4.5 × 10−6 |
| 1/5000 | 3.6 × 10−6 | 2.4 × 10−6 | 1.8 × 10−6 |
| Parameter | a | b | c |
|---|---|---|---|
| Car body | 28.13 | 15.43 | −28.22 |
| Bogie | 10.88 | 217.19 | −10.96 |
| Wheel-sets | 22.85 | 300.59 | −23.32 |
| Rail | 1864.13 | 23.98 | −1863.80 |
| Track slab | 1858.71 | 21.73 | −1859.03 |
| Bridge | 119.93 | 89.86 | −120.24 |
| Component | 200 km/h | 300 km/h | 400 km/h | |||
|---|---|---|---|---|---|---|
| Limit of Accuracy | Limit of Stability | Limit of Accuracy | Limit of Stability | Limit of Accuracy | Limit of Stability | |
| Car body | 5.2 × 10−3 | - | 5.2 × 10−3 | - | 5.2 × 10−3 | - |
| Bogie | 4.0 × 10−3 | 3.6 × 10−3 | 4.0 × 10−3 | 2.4 × 10−3 | 4.0 × 10−3 | 1.8 × 10−3 |
| Wheel-sets | 2.0 × 10−3 | 1.8 × 10−3 | 2.0 × 10−3 | 1.2 × 10−3 | 2.0 × 10−3 | 9.0 × 10−4 |
| Rail | 1.0 × 10−3 | 3.6 × 10−4 | 1.0 × 10−3 | 2.4 × 10−4 | 1.0 × 10−3 | 1.8 × 10−4 |
| Track slab | 3.0 × 10−4 | 3.6 × 10−4 | 3.0 × 10−4 | 2.4 × 10−4 | 3.0 × 10−4 | 1.8 × 10−4 |
| Bridge | 4.0 × 10−4 | 3.6 × 10−4 | 4.0 × 10−4 | 2.4 × 10−4 | 4.0 × 10−4 | 1.8 × 10−4 |
| Parameter | a | b | c |
|---|---|---|---|
| Car body | 1.45 | 459.56 | −1.45 |
| Bogie | 8.04 | 998.63 | −7.85 |
| Wheel-sets | 1908.13 | 25.82 | −1905.80 |
| Rail | 2128.93 | 25.94 | −2127.17 |
| Track slab | 2050.82 | 25.94 | −2050.17 |
| Bridge | 1318.25 | 28.99 | −1317.64 |
| Component | Without Track Irregularity | Short Wave Irregularity | Medium-Long Wave Irregularity | |||
|---|---|---|---|---|---|---|
| Limit of Accuracy | Limit of Stability | Limit of Accuracy | Limit of Stability | Limit of Accuracy | Limit of Stability | |
| Car body | 5.2 × 10−3 | - | 5.9 × 10−3 | - | 5.2 × 10−3 | - |
| Bogie | 4.0 × 10−3 | 2.4 × 10−3 | 1.5 × 10−3 | 3.0 × 10−4 | 4.0 × 10−3 | 2.0 × 10−3 |
| Wheel-sets | 2.0 × 10−3 | 1.2 × 10−3 | 2.4 × 10−4 | 6.0 × 10−5 | 2.0 × 10−3 | 2.0 × 10−3 |
| Rail | 1.0 × 10−3 | 2.4 × 10−4 | 2.4 × 10−4 | 3.0 × 10−5 | 1.0 × 10−3 | 1.5 × 10−3 |
| Track slab | 3.0 × 10−4 | 2.4 × 10−4 | 2.4 × 10−4 | 2.4 × 10−4 | 3.0 × 10−4 | 3.0 × 10−4 |
| Bridge | 4.0 × 10−4 | 2.4 × 10−4 | 4.0 × 10−4 | 1.5 × 10−4 | 4.0 × 10−4 | 1.0 × 10−3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ye, J.; Sun, H. The Influence of an Integration Time Step on Dynamic Calculation of a Vehicle-Track-Bridge under High-Speed Railway. Mathematics 2021, 9, 431. https://doi.org/10.3390/math9040431
Ye J, Sun H. The Influence of an Integration Time Step on Dynamic Calculation of a Vehicle-Track-Bridge under High-Speed Railway. Mathematics. 2021; 9(4):431. https://doi.org/10.3390/math9040431
Chicago/Turabian StyleYe, Junjie, and Hao Sun. 2021. "The Influence of an Integration Time Step on Dynamic Calculation of a Vehicle-Track-Bridge under High-Speed Railway" Mathematics 9, no. 4: 431. https://doi.org/10.3390/math9040431
APA StyleYe, J., & Sun, H. (2021). The Influence of an Integration Time Step on Dynamic Calculation of a Vehicle-Track-Bridge under High-Speed Railway. Mathematics, 9(4), 431. https://doi.org/10.3390/math9040431






