Investigation of the Stochastic Modeling of COVID-19 with Environmental Noise from the Analytical and Numerical Point of View
Abstract
:1. Introduction and Backgrounds
2. Existence of the Solution
- Set ;
- Assume a complete probability space .
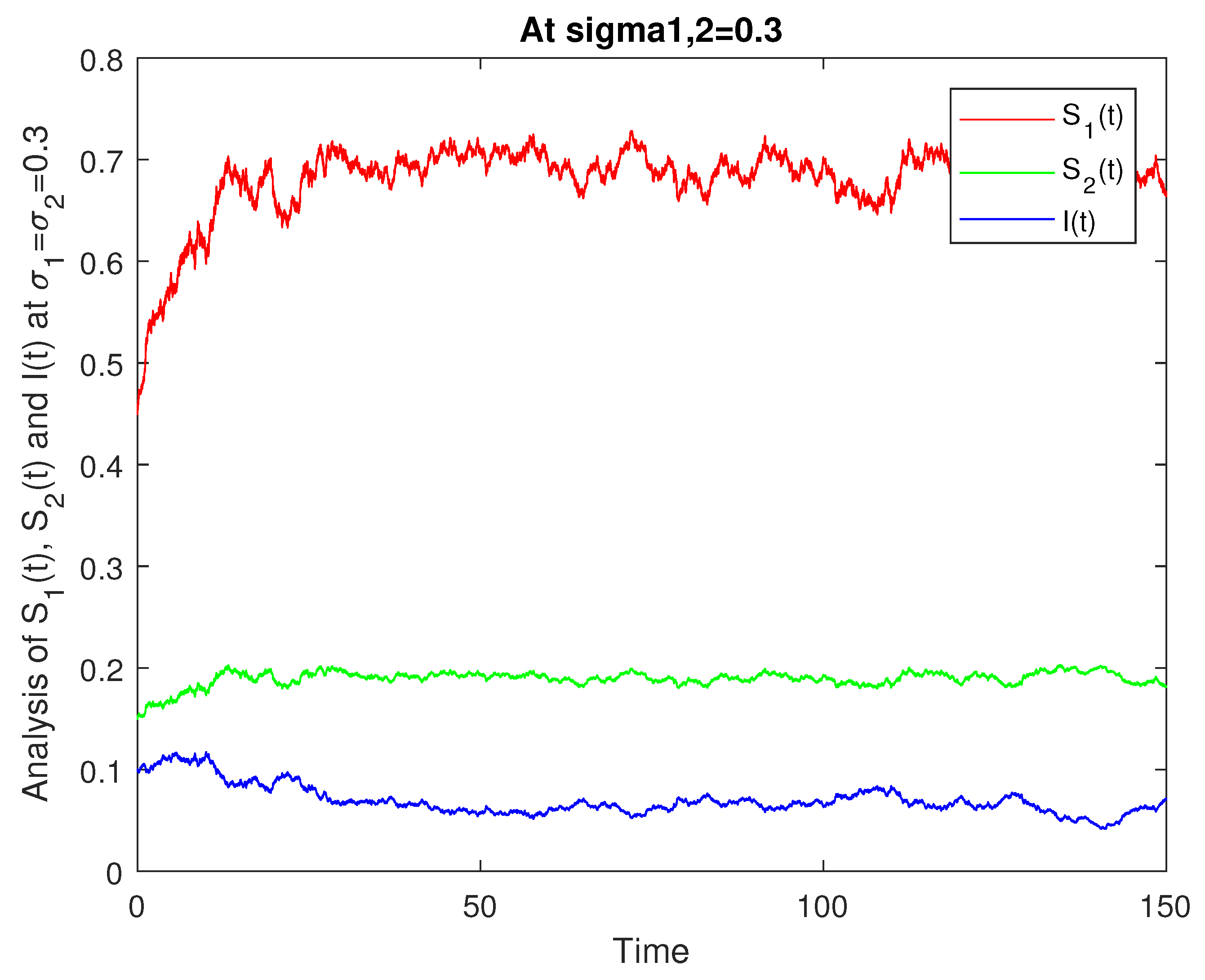
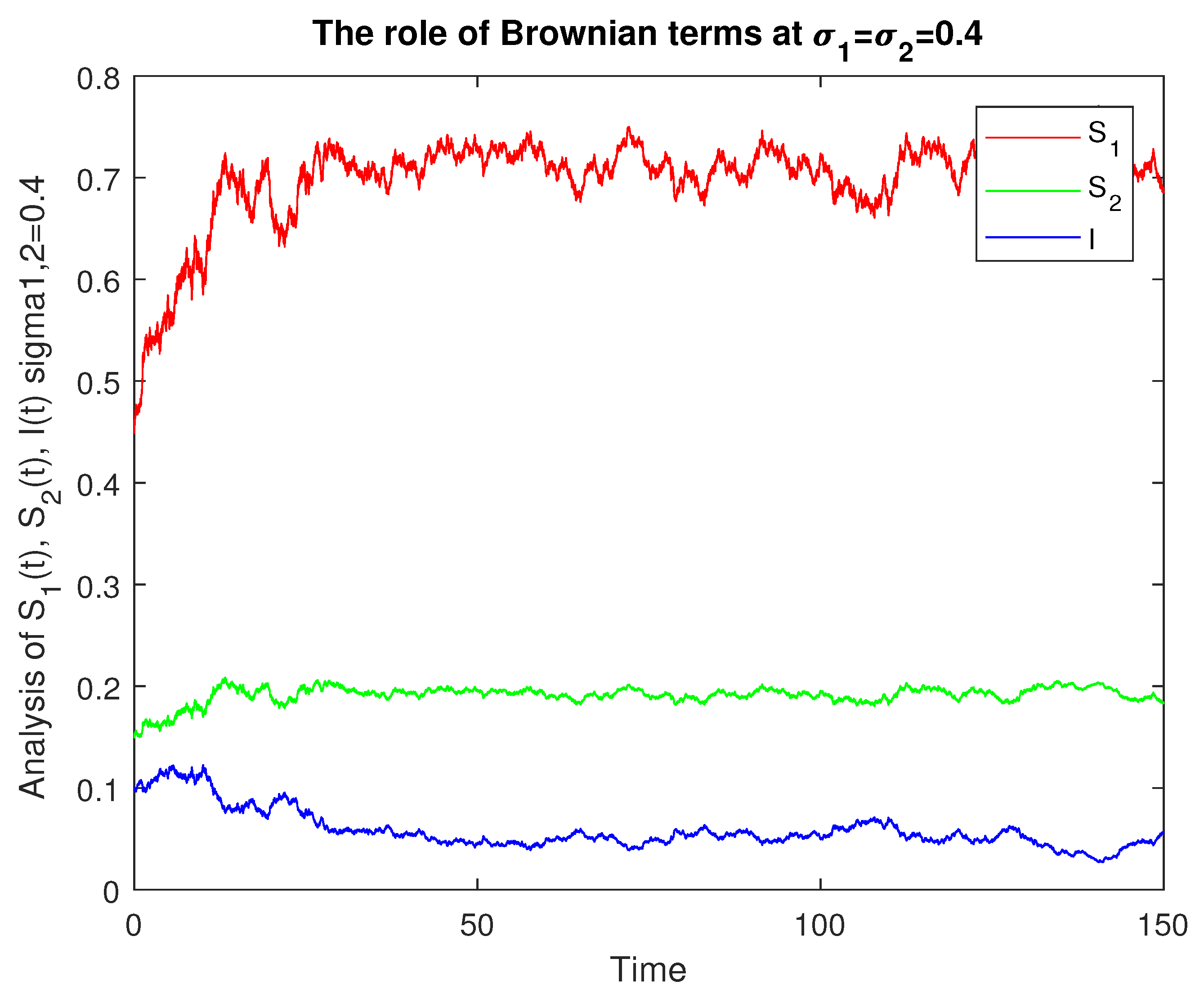
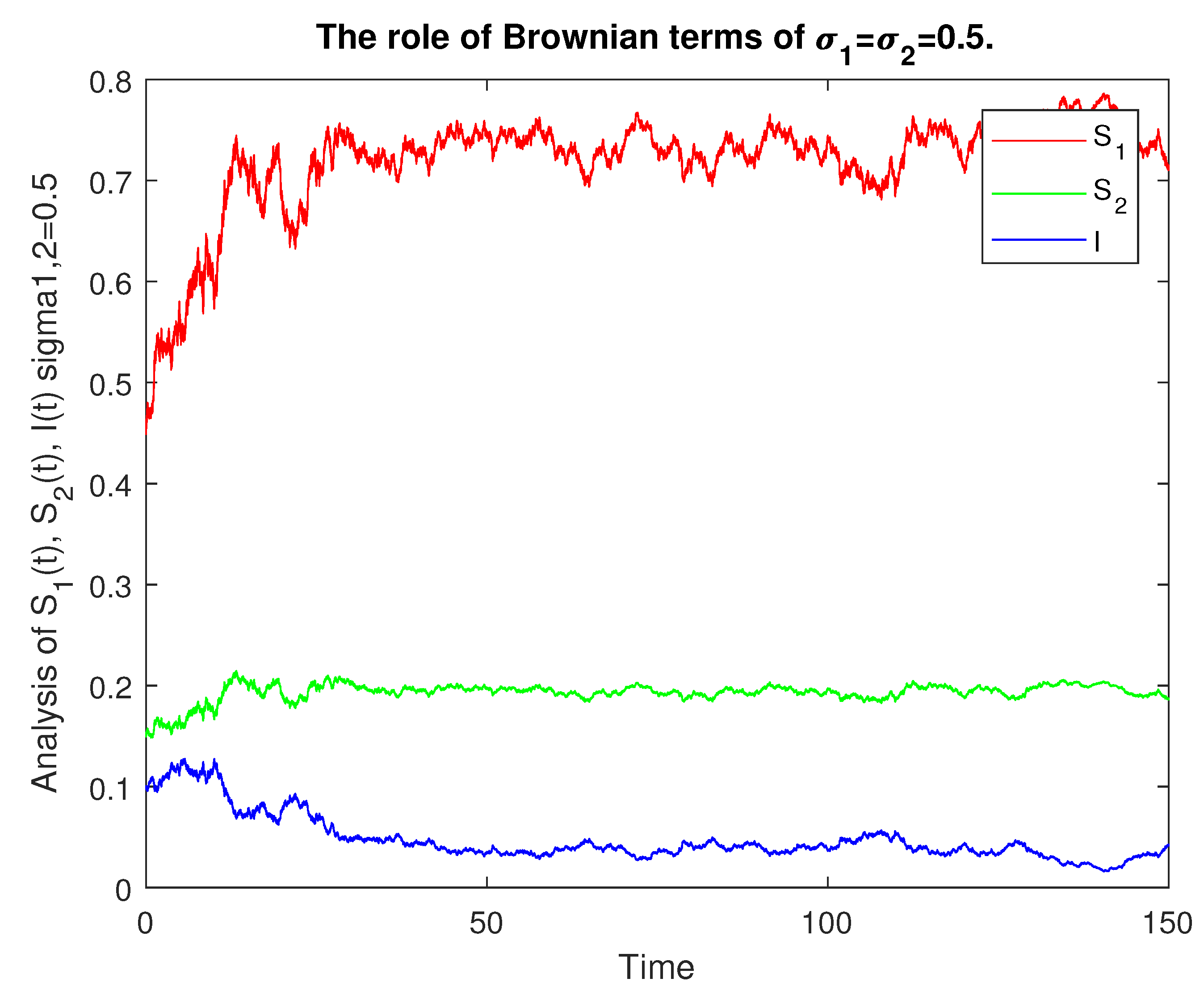
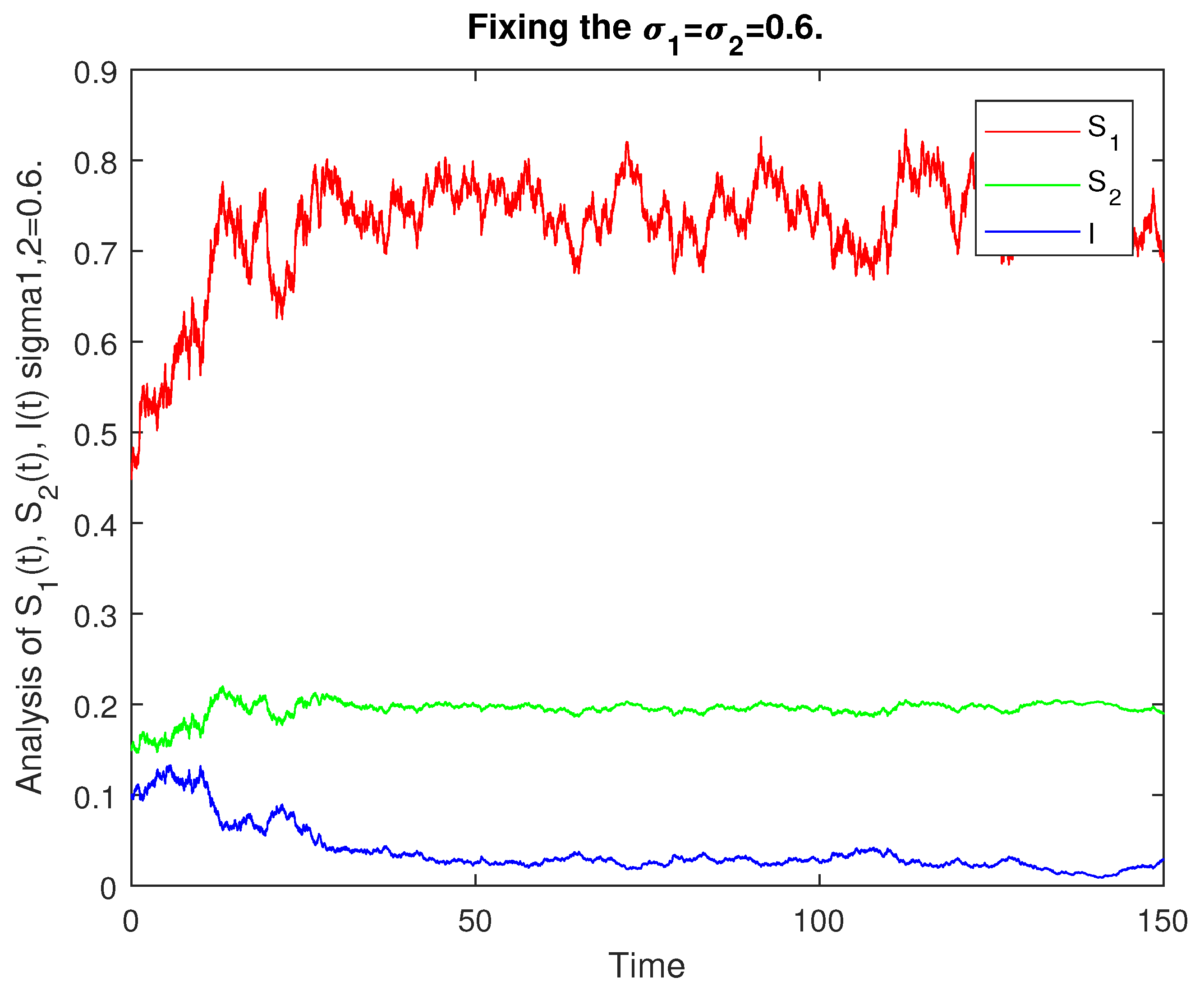
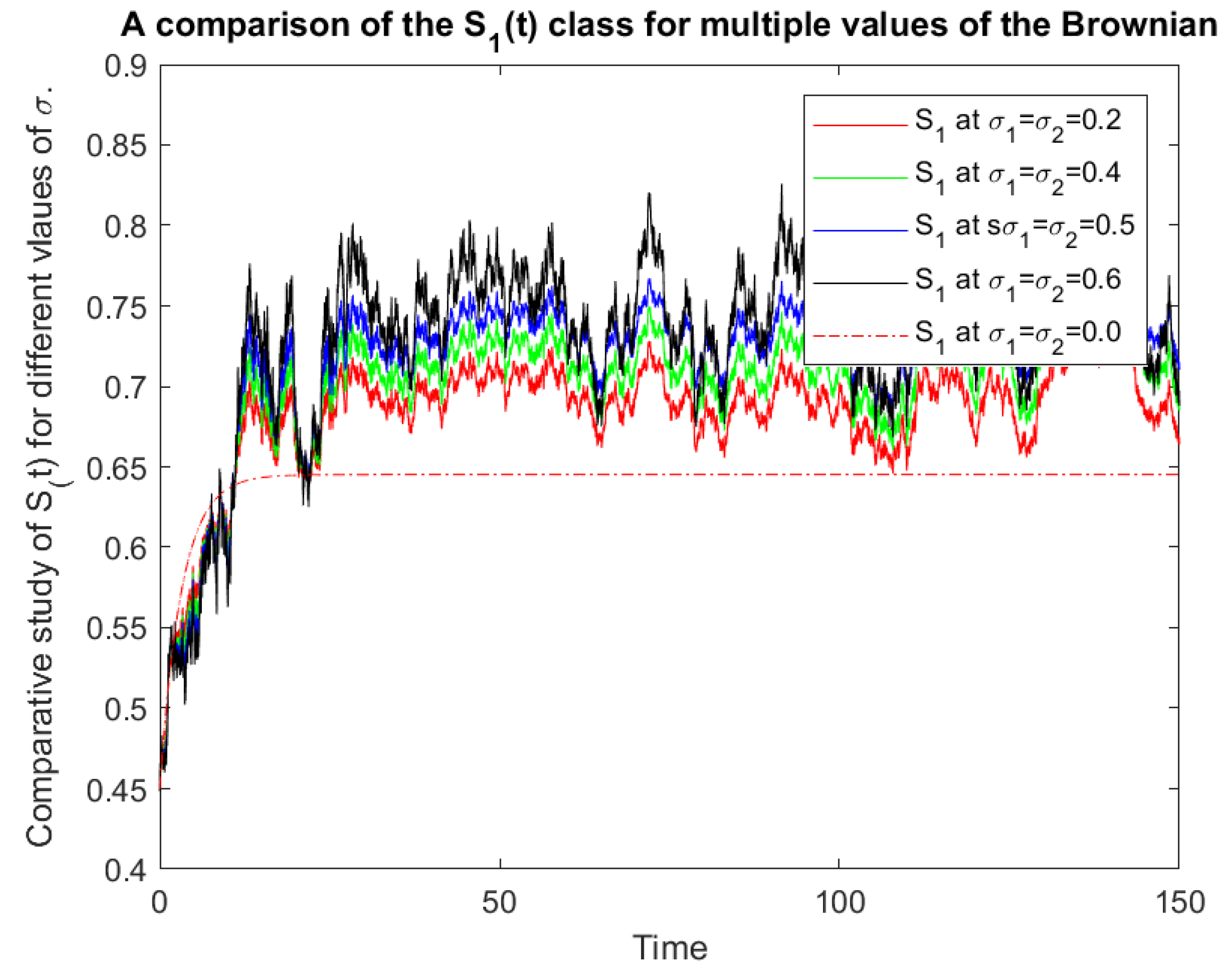
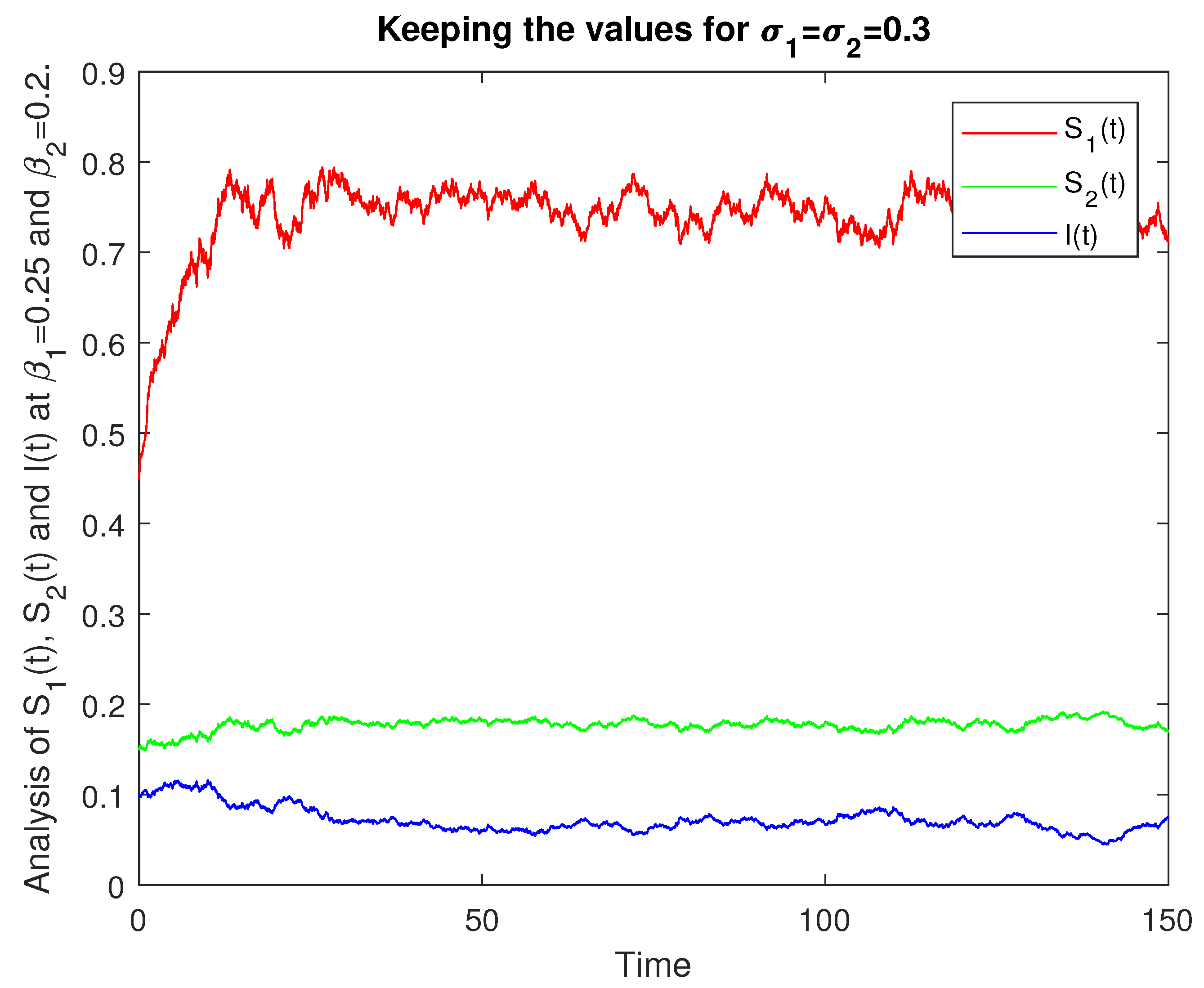
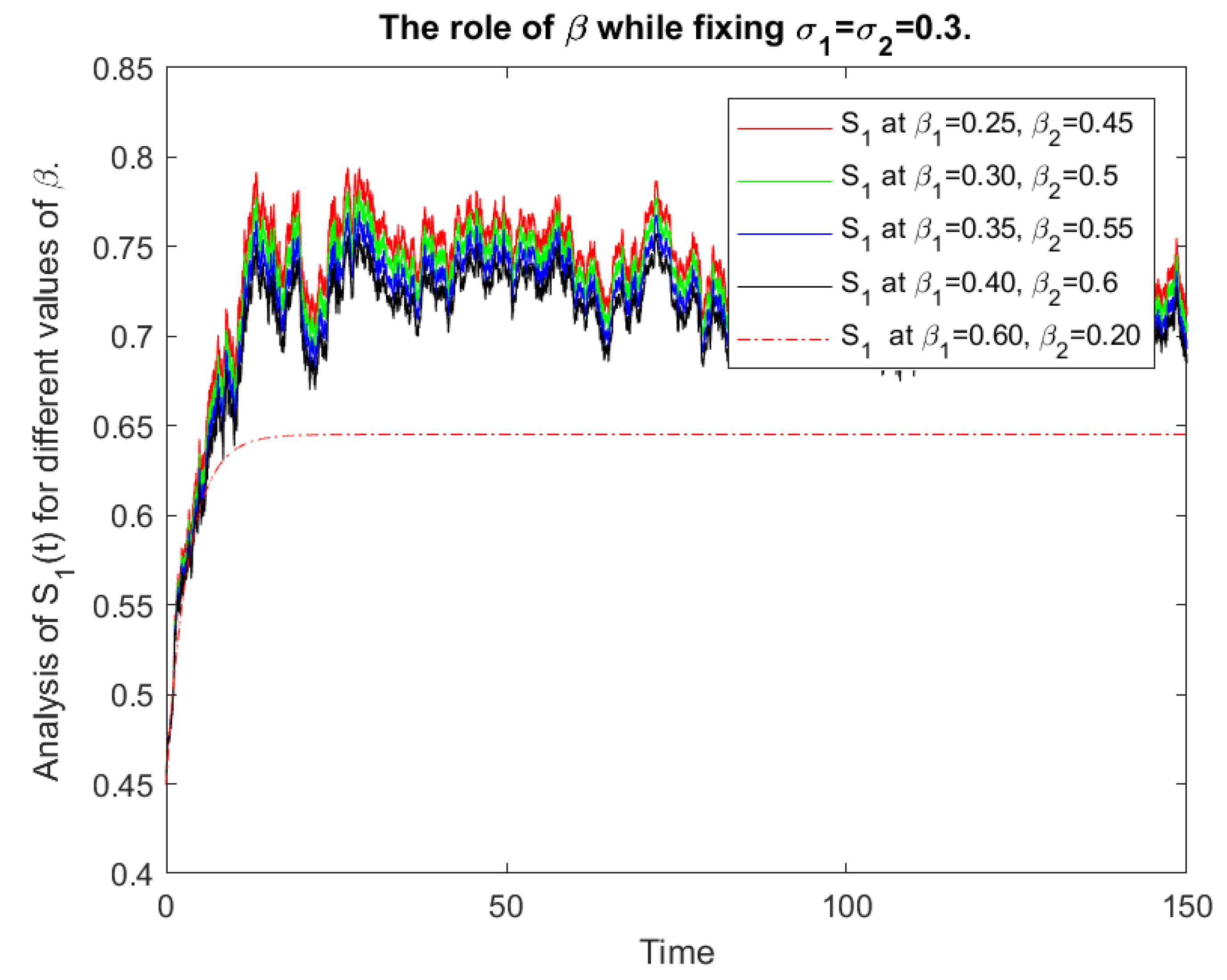
3. Numerical Scheme and Simulations
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gorbalenya, A.E.; Baker, S.C.; Baric, R.S.; de Groot, R.J.; Drosten, C.; Gulyaeva, A.A.; Haagmans, B.L.; Lauber, C.; Leontovich, A.M.; Neuman, B.W. Coronaviridae study group of the international committee on taxonomy of viruses. The species severe acute respiratory syndrome-related coronavirus: Classifying 2019-nCoV and naming it SARS-CoV-2. Nat. Microbiol. 2020, 5, 536–544. [Google Scholar]
- Morrison, S.A.; Jurak, G.; Starc, G. Responding to a global pandemic: Republic of Slovenia on maintaining physical activity during self-isolation. Scand. J. Med. Sci. Sports 2020, 30, 1546–1548. [Google Scholar] [CrossRef] [PubMed]
- Kokudo, N.; Sugiyama, H. Call for international cooperation and collaboration to effectively tackle the COVID-19 pandemic. Global Health Med. 2020, 2, 60–62. [Google Scholar] [CrossRef]
- Omer, S.; Ali, S.; ud Din Babar, Z. Preventive measures and management of COVID-19 in pregnancy. Drugs Ther. Perspect. 2020, 36, 246–249. [Google Scholar] [CrossRef]
- Owusu, K.F.; Goufo, E.F.D.; Mugisha, S. Modelling intracellular delay and therapy interruptions within Ghanaian HIV population. Adv. Differ. Equ. 2020, 401, 2020. [Google Scholar] [CrossRef]
- Anderson, M.; Mckee, M.; Mossialos, E. Developing a sustainable exit strategy for COVID-19: Health, economic and public policy implications. J. Roy. Soc. Med. 2020, 113, 176–178. [Google Scholar] [CrossRef]
- Goufo, E.F.D.; Maritz, R. A note on Ebola’s outbreak and human migration dynamic. J. Hum. Ecol. 2015, 51, 257–263. [Google Scholar] [CrossRef]
- Djomegni, P.M.T.; Tekle, A.; Dawed, M.Y. Pre-exposure prophylaxis HIV/AIDS mathematical model with non classical isolation. Jpn. J. Ind. Appl. Math. 2020, 37, 781–801. [Google Scholar] [CrossRef]
- Khan, Z.A.; Jarad, F.; Khan, A.; Khan, H. Nonlinear discrete fractional sum inequalities related to the theory of discrete fractional calculus with applications. Adv. Differ. Equ. 2021, 100, 2021. [Google Scholar] [CrossRef]
- Khan, A.; Alshehri, H.M.; Abdeljawad, T.; Al-Mdallal, Q.M.; Khan, H. Stability analysis of fractional nabla difference COVID-10 model. Res. Phys. 2021, 22, 103888. [Google Scholar]
- Shah, A.; Khan, R.A.; Khan, A.; Khan, H.; Gomez-Aguilar, J.F. Investigation of a system of nonlinear fractional order hybrid differential equations under usual boundary conditions for existence of solution. Math. Methods Appl. Sci. 2021, 44, 1628–1638. [Google Scholar] [CrossRef]
- Shah, K.; Alqudah, M.A.; Jarad, F.; Abdeljawad, T. Semi-analytical study of pine wilt disease model with convex rate under Caputo-Febrizio fractional order derivative. Chaos Solitons Fractals 2020, 135, 109754. [Google Scholar] [CrossRef]
- Boutiara, A.; Etemad, S.; Hussain, A.; Rezapour, S. The generalized U-H and U-H stability and existence analysis of a coupled hybrid system of integro-differential IVPs involving φ-Caputo fractional operators. Adv. Differ. Equ. 2021, 95, 2021. [Google Scholar] [CrossRef]
- Amara, A.; Etemad, S.; Rezapour, S. Topological degree theory and Caputo-Hadamard fractional boundary value problems. Adv. Differ. Equ. 2020, 369, 2020. [Google Scholar] [CrossRef]
- Sulaiman, I.M.; Mamat, M.; Umar, A.O.; kamfa, K.; Madi, E.N. Some three-term conjugate gradient algorithms with descent condition for unconstrained optimization models. J. Adv. Res. Dyn. Control Syst. 2020, 12, 2494–2501. [Google Scholar]
- Madi, E.N.; Naim, S.; Yaafar, A.; Yaakob, A.M.; Yusoff, B. Agreement matrix based on fuzzy decision-making to rank ship Berthing criteria. Int. J. Eng. Trends Tech. 2020, 68, 31–36. [Google Scholar] [CrossRef]
- Etemad, S.; Rezapour, S.; Samei, M.E. α-ψ-contractions and solutions of a q-fractional differential inclusion with three-point boundary value conditions via computational results. Adv. Differ. Equ. 2020, 218, 2020. [Google Scholar] [CrossRef]
- Zhu, L.; Hu, H. A stochastic SIR epidemic model with density dependent birth rate. Adv. Differ. Equ. 2015, 330, 2015. [Google Scholar] [CrossRef] [Green Version]
- Mohammadi, H.; Kumar, S.; Rezapour, S.; Etemad, S. A theoretical study of the Caputo-Fabrizio fractional modeling for hearing loss due to Mumps virus with optimal control. Chaos Solitons Fractals 2021, 144, 110668. [Google Scholar] [CrossRef]
- Rezapour, S.; Ntouyas, S.K.; Iqbal, M.Q.; Hussain, A.; Etemad, S.; Tariboon, J. An analytical survey on the solutions of the generalized double-order ϕ-integrodifferential equation. J. Funct. Spaces 2021, 6667757, 2021. [Google Scholar]
- Rezapour, S.; Etemad, S.; Mohammadi, H. A mathematical analysis of a system of Caputo-Fabrizio fractional differential equations for the anthrax disease model in animals. Adv. Differ. Equ. 2020, 481, 2020. [Google Scholar] [CrossRef]
- Kongson, J.; Thaiprayoon, C.; Sudsutad, W. Analysis of a fractional model for HIV CD4+ T-cell with treatment under generalized Caputo fractional derivative. AIMS Math. 2021, 6, 7285–7304. [Google Scholar] [CrossRef]
- Matar, M.M.; Abbas, M.I.; Alzabut, J.; Kaabar, M.K.A.; Etemad, S.; Rezapour, S. Investigation of the p-Laplacian nonperiodic nonlinear boundary value problem via generalized Caputo fractional derivatives. Adv. Differ. Equ. 2021, 68, 2021. [Google Scholar] [CrossRef]
- Baleanu, D.; Etemad, S.; Rezapour, S. On a fractional hybrid integro-differential equation with mixed hybrid integral boundary value conditions by using three operators. Alex. Eng. J. 2020, 59, 3019–3027. [Google Scholar] [CrossRef]
- Brown, R. XXVII. A brief account of microscopical observations made in the months of June, July and August 1827, on the particles contained in the pollen of plants; and on the general existence of active molecules in organic and inorganic bodies. Philos. Mag. 1828, 4, 161–173. [Google Scholar] [CrossRef] [Green Version]
- Doob, J.L. Stochastic Processes; Wiley: New York, NY, USA, 1991; 664p. [Google Scholar]
- Williams, D. Probability with Martingales; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Rogers, L.C.G.; Williams, D. Diffusions, Markov Processes and Martingales: Volume 1, Foundations, 2nd ed.; John Wiley and Sons, Ltd.: Chichester, UK, 1994. [Google Scholar]
- Burdzy, K. Brownian Motion and its Applications to Mathematical Analysis; Springer: Cham, Switzerland, 2014. [Google Scholar]
- Ross, R. An application of the theory of probabilities to the study of a priori pathometry, Part I. Proc. R. Soc. Lond. Ser. A 1916, 92, 204–230. [Google Scholar]
- Ross, R.; Hudson, H.P. An application of the theory of probabilities to the study of a priori pathometry, Part II. Proc. R. Soc. Lond. Ser. A 1917, 93, 212–225. [Google Scholar]
- Ross, R.; Hudson, H.P. An application of the theory of probabilities to the study of a priori pathometry, Part III. Proc. R. Soc. Lond. Ser. A 1917, 93, 225–240. [Google Scholar]
- Kermack, W.O.; McKendrick, A.G. A contribution to the mathematical theory of epidemics. Proc. R. Soc. Lond. Ser. A 1927, 115, 700–721. [Google Scholar]
- Harko, T.; Lobo, F.S.N.; Mak, M.K. Exact analytical solutions of the susceptible-infected-recovered (SIR) epidemic model and of the SIR model with equal death and birth rates. Appl. Math. Comput. 2014, 236, 184–194. [Google Scholar] [CrossRef] [Green Version]
- Kroger, M.; Schlickeiser, R. Analytical solution of the SIR-model for the temporal evolution of epidemics. Part A: Time-independent reproduction factor. J. Phys. A Math. Theor. 2020, 53, 505601. [Google Scholar] [CrossRef]
- Schlickeiser, R.; Kroger, M. Analytical solution of the SIR-model for the temporal evolution of epidemics: Part B. Semi-time case. J. Phys. A Math. Theor. 2021, 54, 175601. [Google Scholar] [CrossRef]
- Yang, W.; Zhang, D.; Peng, L.; Zhuge, C.; Hong, L. Rational evaluation of various epidemic models based on the COVID-19 data of China. Epidemics 2021, 37, 100501. [Google Scholar] [CrossRef] [PubMed]
- Ajbar, A.; Alqahtani, R.T.; Boumaza, M. Dynamics of an SIR-based COVID-19 model with linear incidence rate, nonlinear removal rate, and public awareness. Front. Phys. 2021, 9, 13. [Google Scholar] [CrossRef]
- Alanazi, S.A.; Kamruzzaman, M.M.; Alruwaili, M.; Alshammari, N.; Alqahtani, S.A.; Karime, A. Measuring and preventing COVID-19 using the SIR model and machine learning in smart health care. J. Healthc. Eng. 2020, 2020, 8857346. [Google Scholar] [CrossRef] [PubMed]
- Moein, S.; Nickaeen, N.; Roointan, A.; Borhani, N.; Heidary, Z.; Javanmard, S.H.; Ghaisari, J.; Gheisari, Y. Inefficiency of SIR models in forecasting COVID-19 epidemic: A case study of Isfahan. Sci. Rep. 2021, 11, 4725. [Google Scholar] [CrossRef]
- Sweilam, N.H.; Al-Mekhlafi, S.M.; Baleanu, D. A hybrid stochastic fractional order Coronavirus (2019-nCov) mathematical model. Chaos Solitons Fractals 2021, 145, 110762. [Google Scholar] [CrossRef]
- Babaei, A.; Jafari, H.; Banihashemi, S.; Ahmadi, M. Mathematical analysis of a stochastic model for spread of Coronavirus. Chaos Solitons Fractals 2021, 145, 110788. [Google Scholar] [CrossRef]
- Danane, J.; Allali, K.; Hammouch, Z.; Nisar, K.S. Mathematical analysis and simulation of a stochastic COVID-19 Levy jump model with isolation strategy. Res. Phys. 2021, 23, 103994. [Google Scholar] [CrossRef]
- Moualkia, S.; Xu, Y. On the existence and uniqueness of solutions for multidimensional fractional stochastic differential equations with variable order. Mathematics 2021, 9, 2106. [Google Scholar] [CrossRef]
- Sakthivel, R.; Revathi, P.; Ren, Y. Existence of solutions for nonlinear fractional stochastic differential equations. Nonlilear Anal. 2013, 81, 70–86. [Google Scholar] [CrossRef]
- Rafiq, M.; Ali, J.; Riaz, M.B.; Awrejcewicz, J. Numerical analysis of a bi-modal COVID-19 sitr model. Alex. Eng. J. 2022, 61, 227–235. [Google Scholar] [CrossRef]
- Mao, X. Stochastic Differential Equations and Applications, 2nd ed.; Woodhead Publishing: Chichester, UK, 2008. [Google Scholar]
- Sanchez, Y.G.; Sabir, Z.; Guirao, J.L.G. Design of a nonlinear SITR fractal model based on the dynamics of a novel Coronavirus (COVID-19). Fractals 2020, 28, 2040026. [Google Scholar] [CrossRef]
- Umar, M.; Sabir, Z.; Raja, M.A.Z.; Shoaib, M.; Gupta, M.; Sanchez, Y.G. A stochastic intelligent computing with neuro-evolution heuristics for nonlinear SITR system of novel COVID-19 dynamics. Symmetry 2020, 12, 1628. [Google Scholar] [CrossRef]
- Higham, D.J. An algorithmic introduction to numerical simulation of stochastic differential equations. SIAM Rev. 2001, 43, 525–546. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, W.; Yuan, S. Survival and stationary distribution of a SIR epidemic model with stochastic perturbations. Appl. Math. Comput. 2014, 244, 118–131. [Google Scholar] [CrossRef]
- Kabir, K.M.A.; Risa, T.; Tanimoto, J. Prosocial behavior of wearing a mask during an epidemic: An evolutionary explanation. Sci. Rep. 2021, 11, 12621. [Google Scholar] [CrossRef]
- Chowdhury, A.; Kabir, K.M.A.; Tanimoto, J. How quarantine and social distancing policy can suppress the outbreak of novel coronavirus in developing or under poverty level countries: A mathematical and statistical analysis. Biom. Biostat. Int. J. 2021, 10, 145–152. [Google Scholar]
- Tanimoto, J. Sociophysics Approach to Epidemics; Springer: Singapore, 2021; Volume 23. [Google Scholar]
- Madi, E.N.; Yusoff, B. Modelling perceptive-based information (words) for decision support system. Int. J. Recent Technol. Eng. 2019, 7, 665–671. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hussain, S.; Madi, E.N.; Khan, H.; Etemad, S.; Rezapour, S.; Sitthiwirattham, T.; Patanarapeelert, N. Investigation of the Stochastic Modeling of COVID-19 with Environmental Noise from the Analytical and Numerical Point of View. Mathematics 2021, 9, 3122. https://doi.org/10.3390/math9233122
Hussain S, Madi EN, Khan H, Etemad S, Rezapour S, Sitthiwirattham T, Patanarapeelert N. Investigation of the Stochastic Modeling of COVID-19 with Environmental Noise from the Analytical and Numerical Point of View. Mathematics. 2021; 9(23):3122. https://doi.org/10.3390/math9233122
Chicago/Turabian StyleHussain, Shah, Elissa Nadia Madi, Hasib Khan, Sina Etemad, Shahram Rezapour, Thanin Sitthiwirattham, and Nichaphat Patanarapeelert. 2021. "Investigation of the Stochastic Modeling of COVID-19 with Environmental Noise from the Analytical and Numerical Point of View" Mathematics 9, no. 23: 3122. https://doi.org/10.3390/math9233122
APA StyleHussain, S., Madi, E. N., Khan, H., Etemad, S., Rezapour, S., Sitthiwirattham, T., & Patanarapeelert, N. (2021). Investigation of the Stochastic Modeling of COVID-19 with Environmental Noise from the Analytical and Numerical Point of View. Mathematics, 9(23), 3122. https://doi.org/10.3390/math9233122







