Hybrid Carbon Nanotube Flow near the Stagnation Region over a Permeable Vertical Plate with Heat Generation/Absorption
Abstract
:1. Introduction
2. Mathematical Analysis
2.1. Flow Formulation
2.2. Physical Quantities
2.3. Stability Analysis
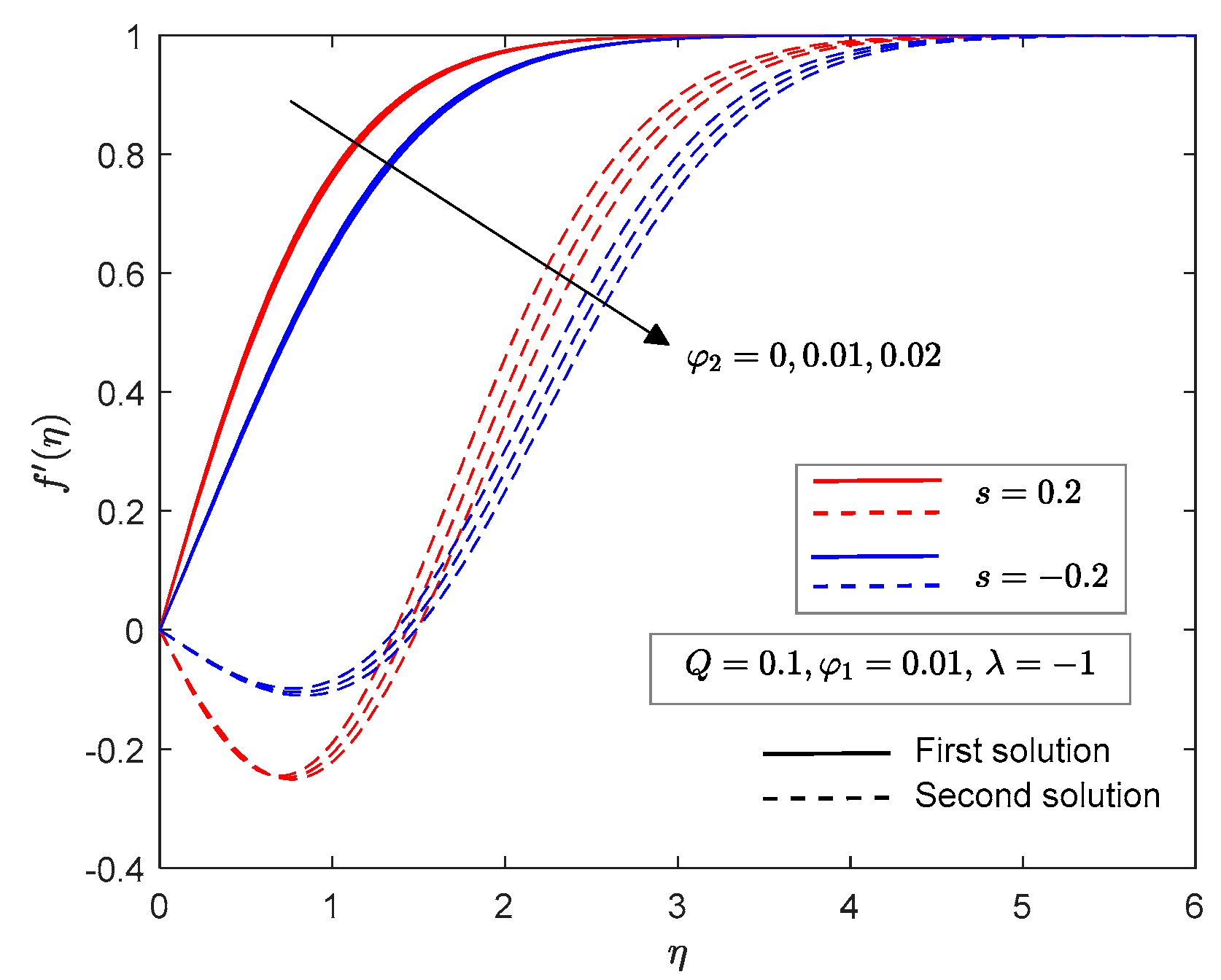
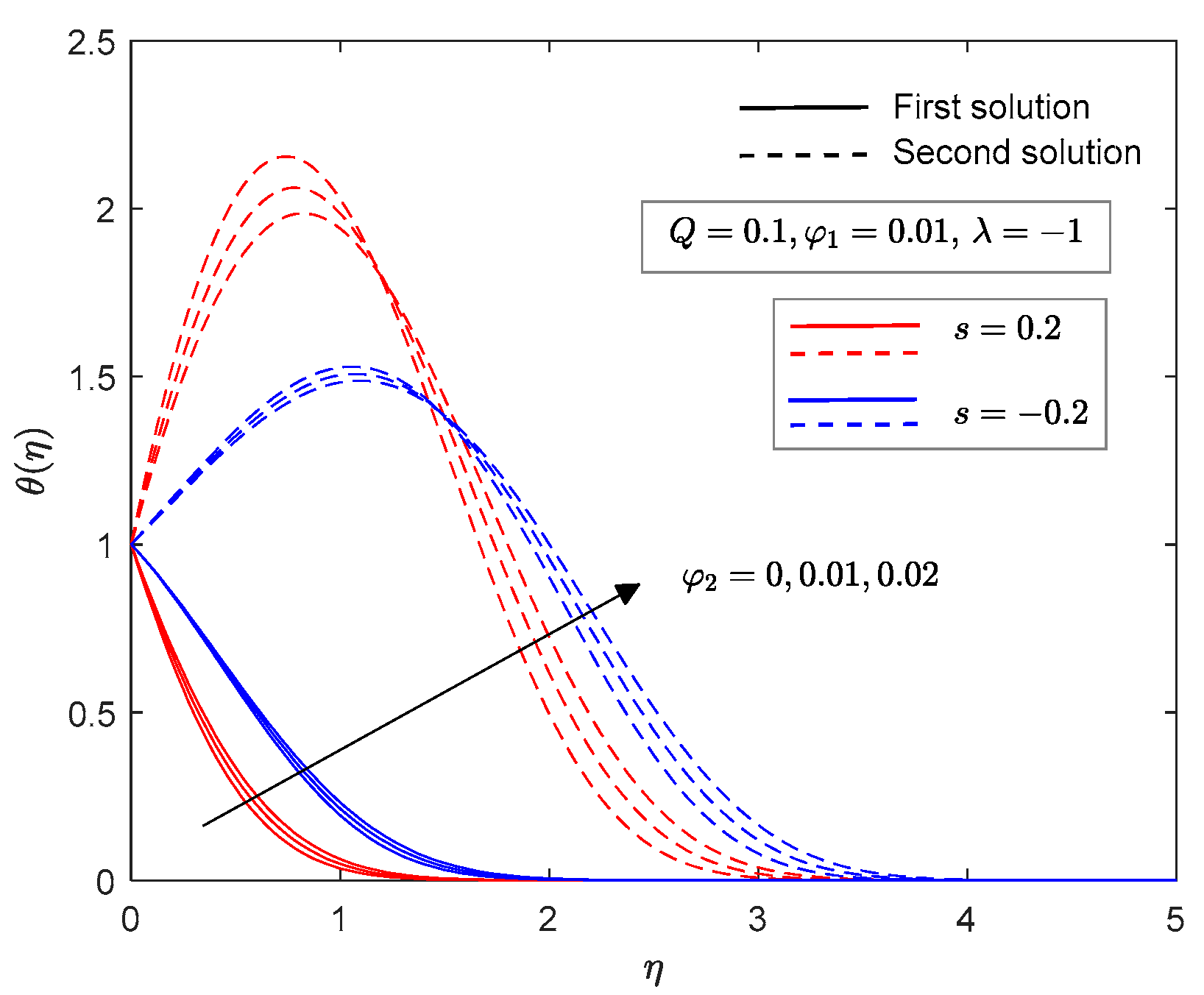
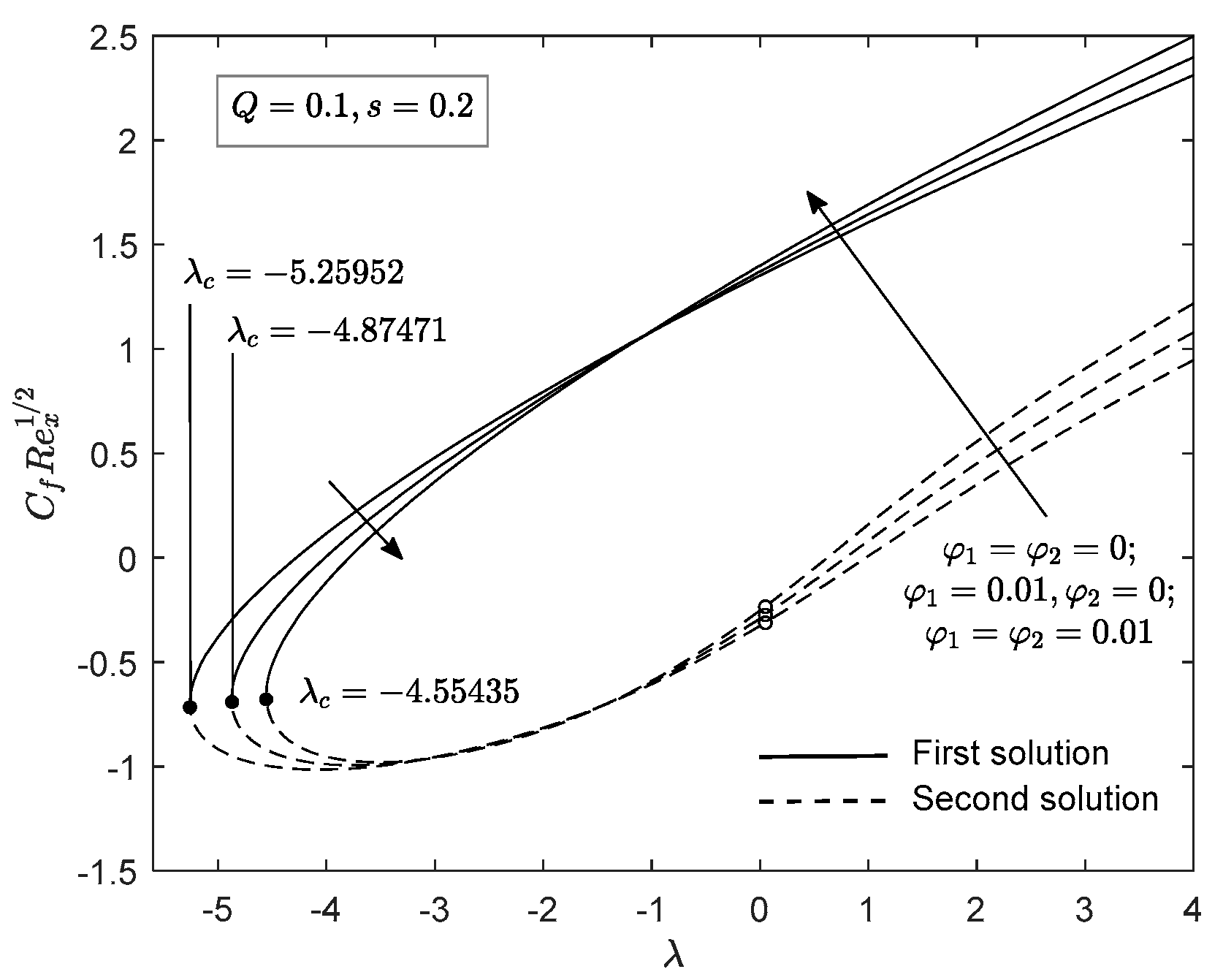
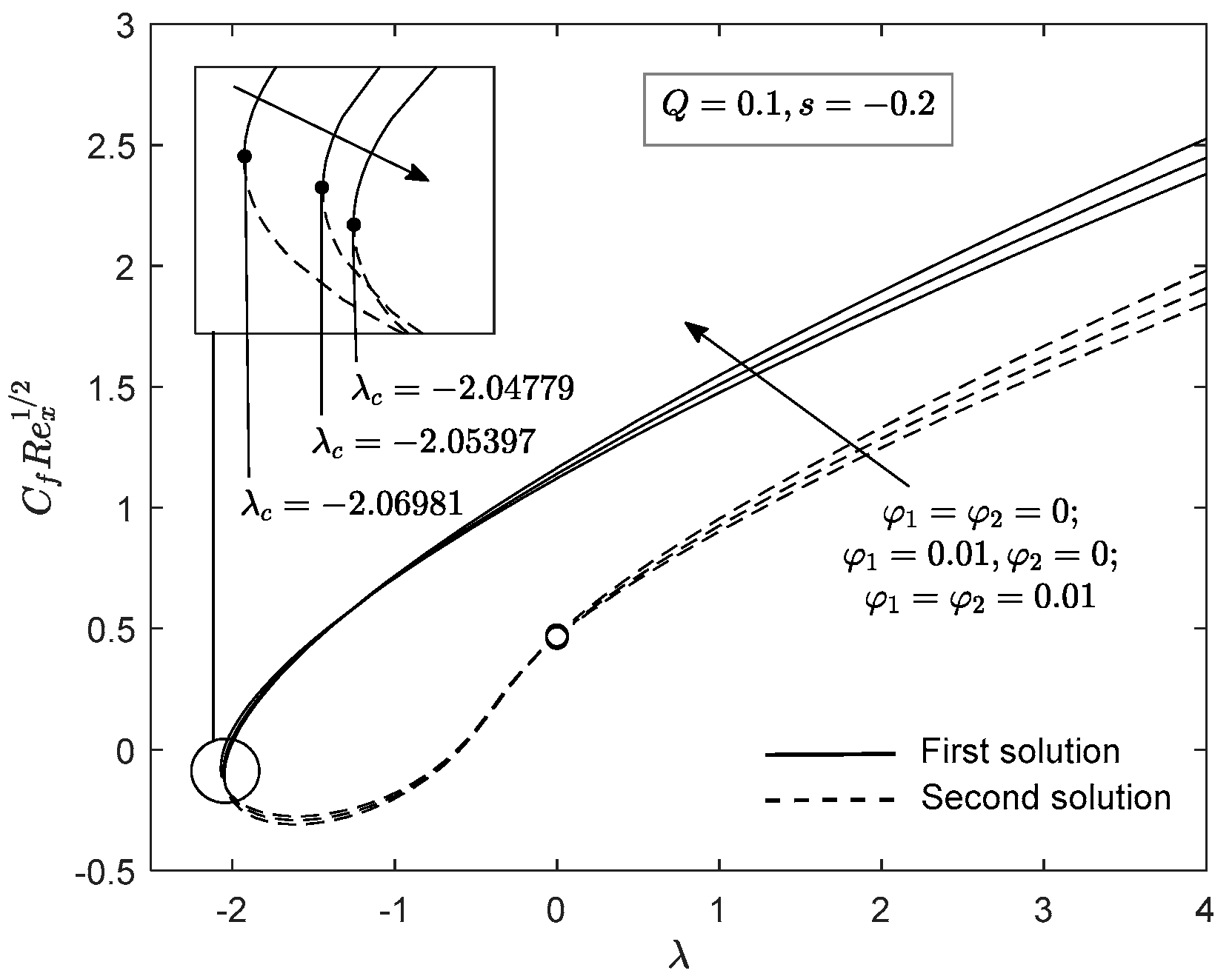
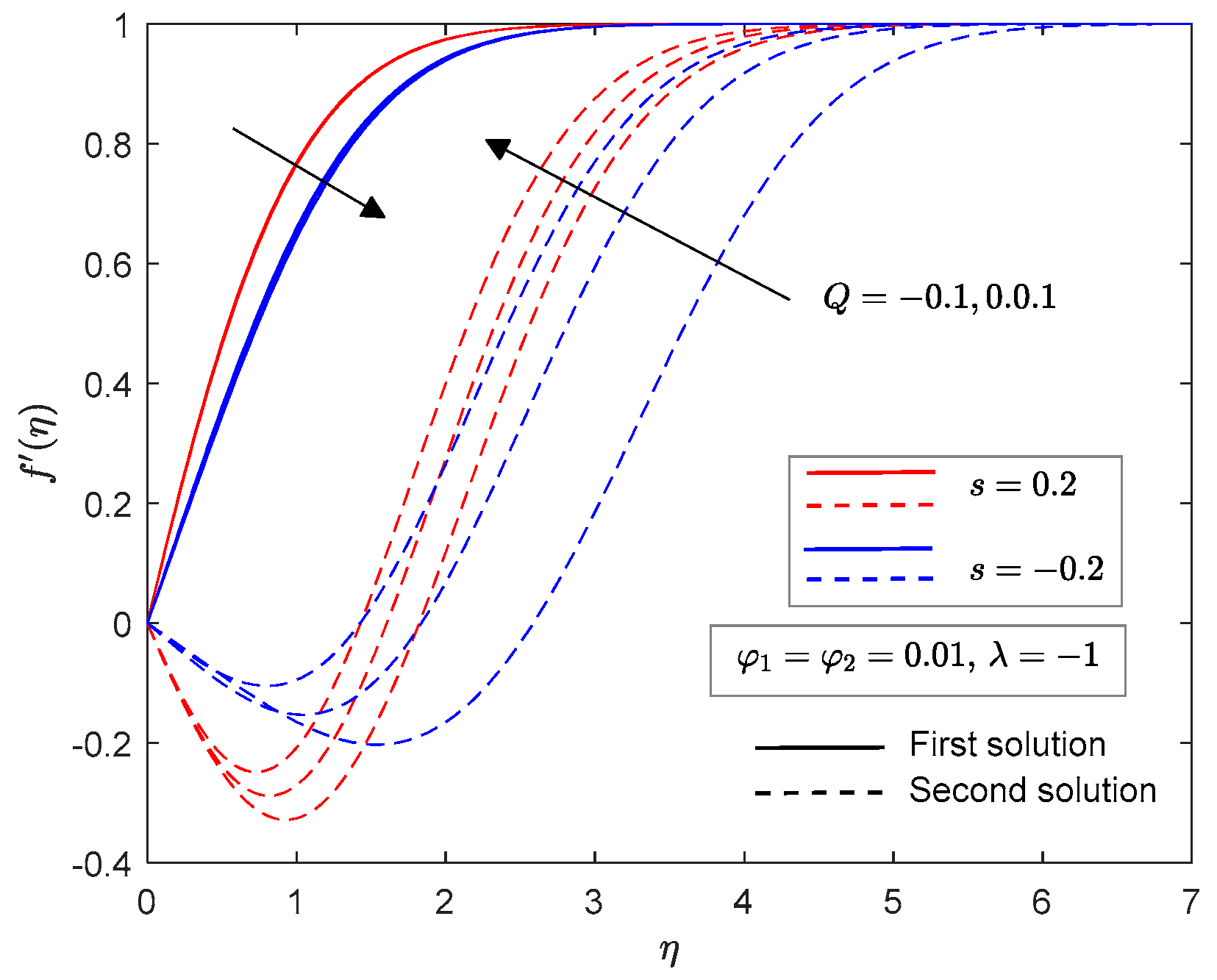
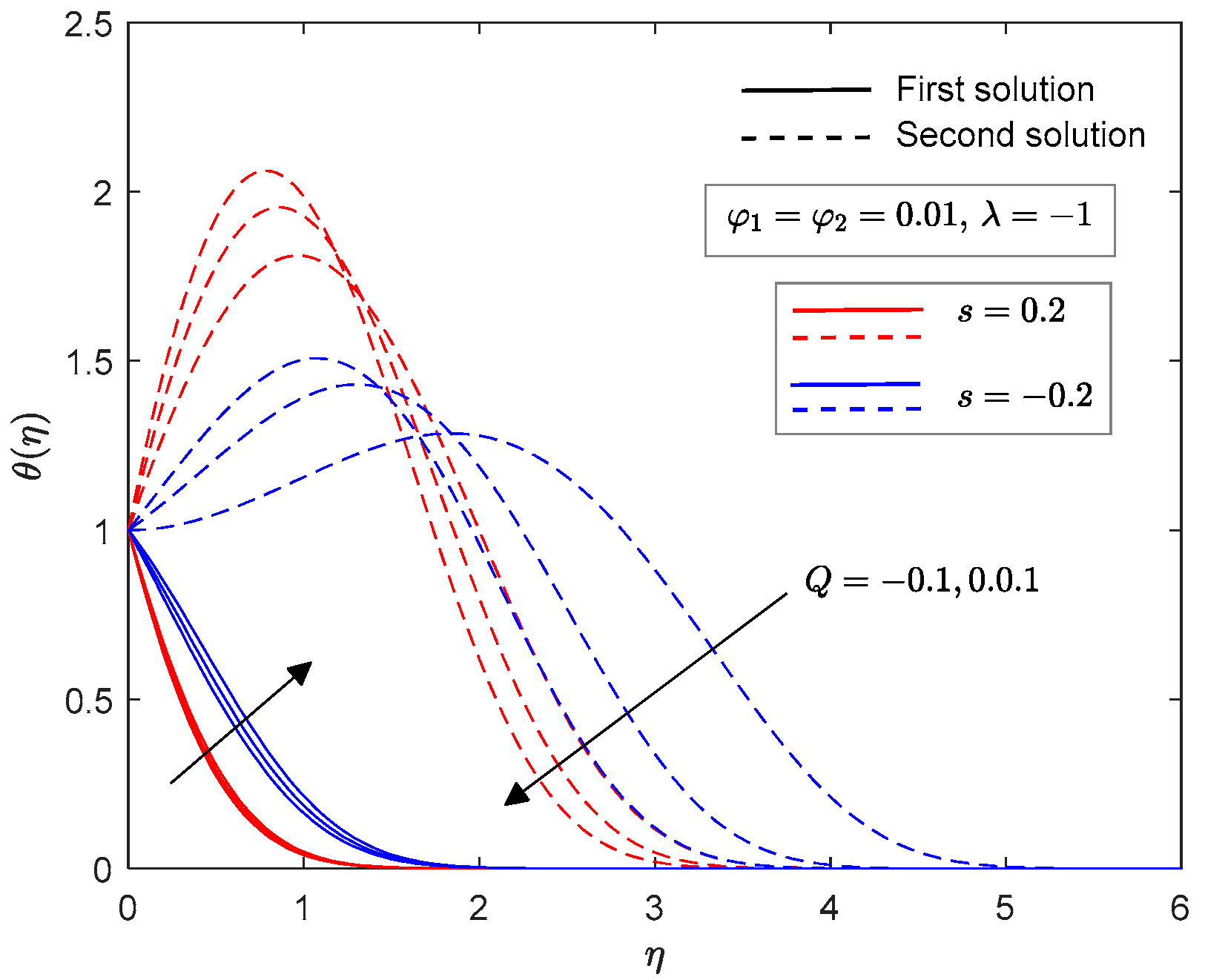
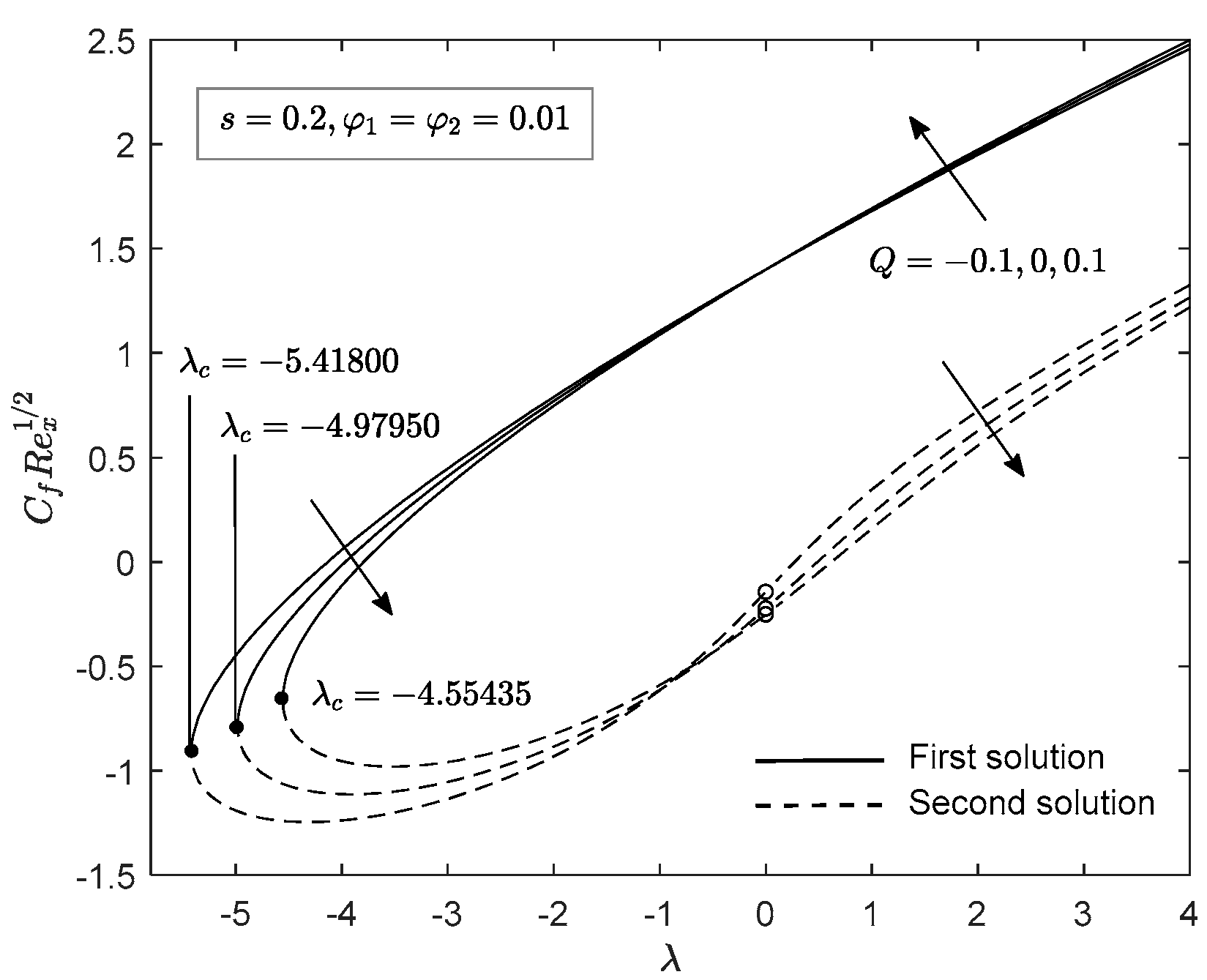
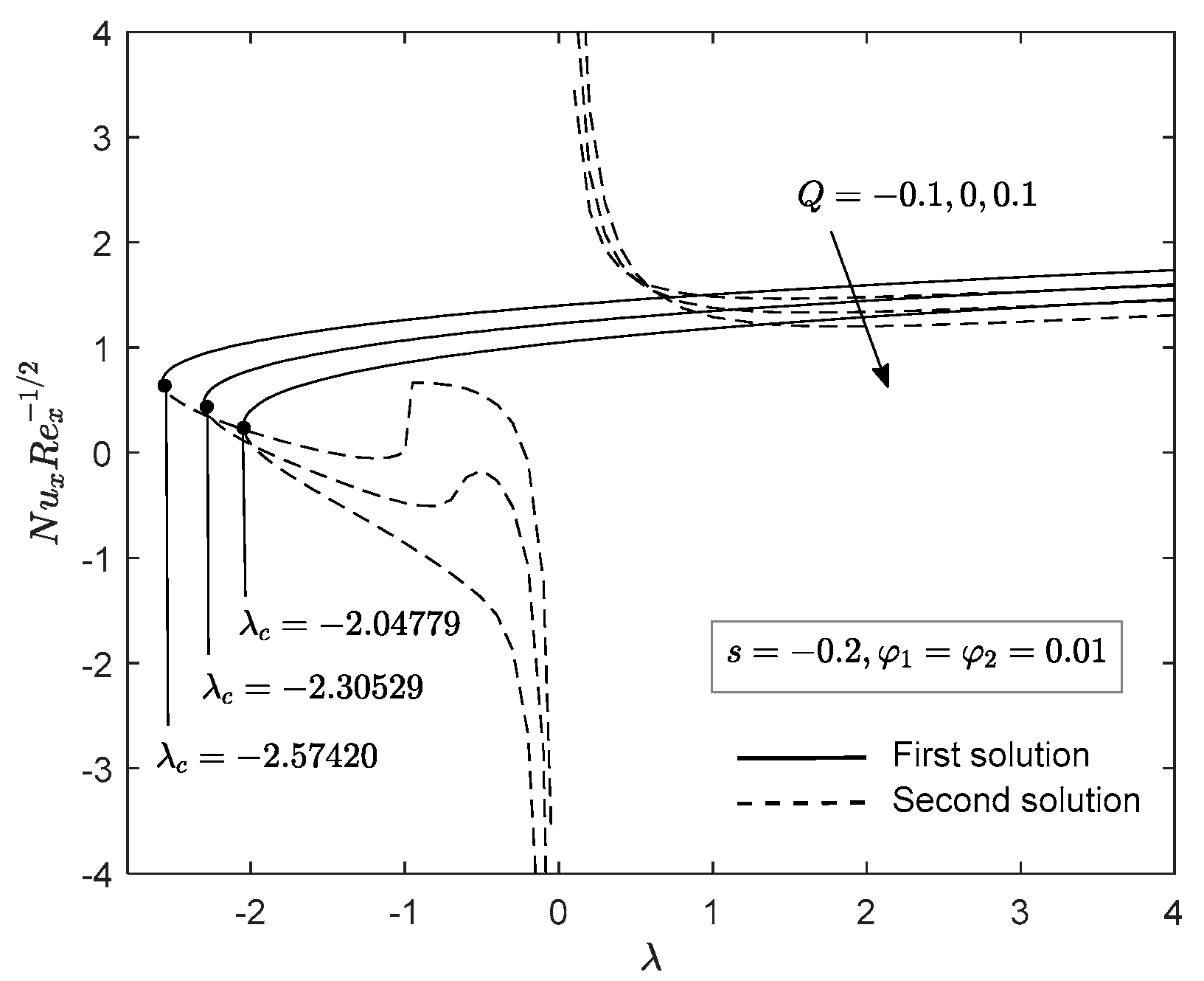
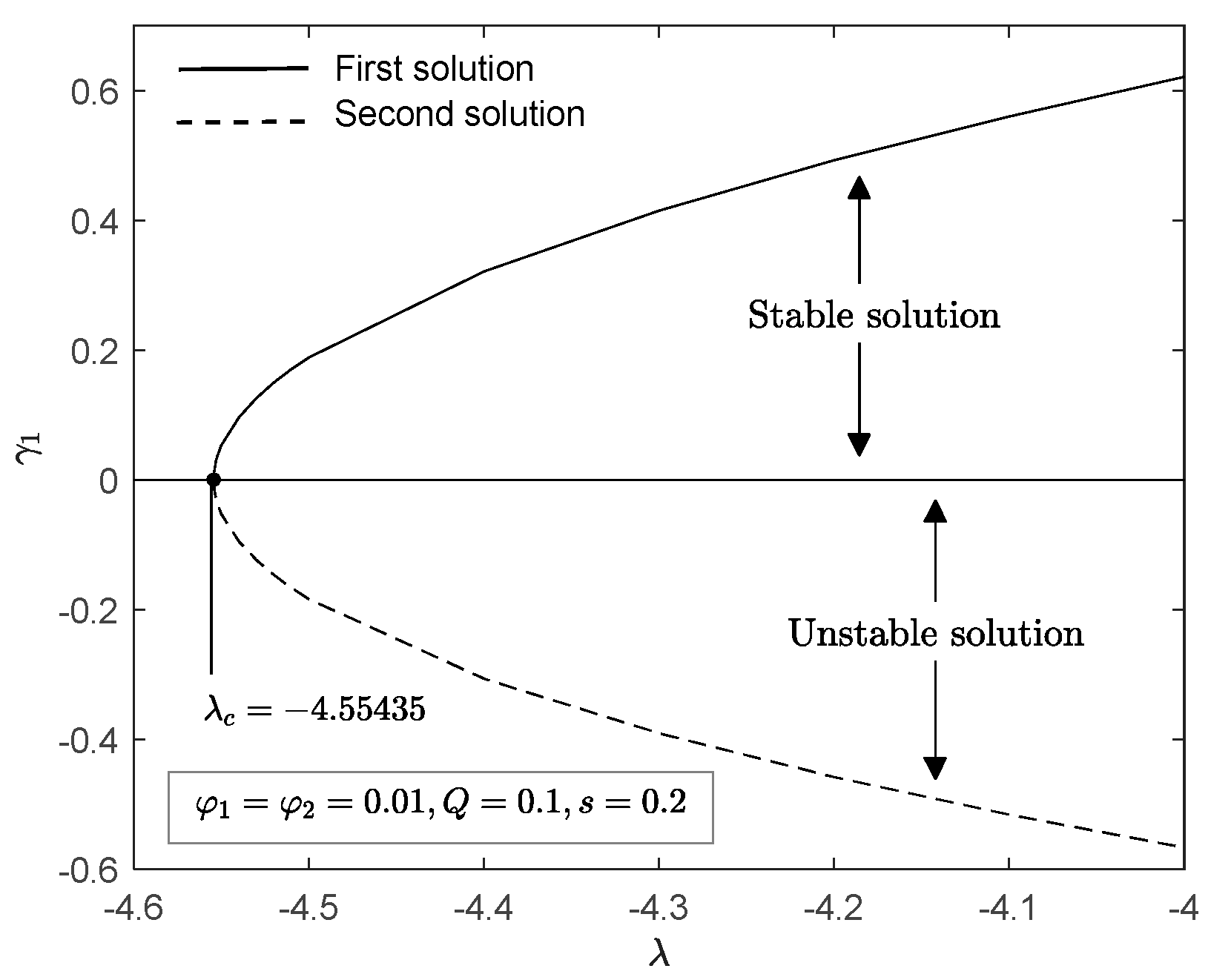
3. Analysis of Results
4. Concluding Remarks
- Two numerical solutions are discoverable for assisting buoyancy flow parameter and opposing buoyancy flow parameter .
- The stability analysis demonstrates that the first solution is stable whilst the second solution is not.
- The domains of the similarity solutions decrease with the use of SWCNT–MWCNT/water hybrid nanofluid, injection effect, and heat generation. Therefore, it fastens the boundary layer separation. However, suction effect and heat absorption parameter delay the boundary layer separation.
- The energy transport rate and skin friction coefficient of SWCNT–MWCNT/water hybrid nanofluid is more advanced than MWCNT/water nanofluid and ordinary fluid.
- Heat absorption enhances the rate of heat transfer while heat generation decreases it.
- The magnitude of local Nusselt number and skin friction coefficient are higher for assisting buoyancy flow than the opposing buoyancy flow case.
- The heat-transfer rate is elevated in the presence of suction effect compared to the presence of injection effect.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Argonne National Lab.: Lemont, IL, USA, 1995. [Google Scholar]
- Bovand, M.; Rashidi, S.; Ahmadi, G.; Esfahani, J.A. Effects of trap and reflect particle boundary conditions on particle transport and convective heat transfer for duct flow-A two-way coupling of Eulerian-Lagrangian model. Appl. Therm. Eng. 2016, 108, 368–377. [Google Scholar] [CrossRef]
- Guthrie, D.G.; Torabi, M.; Karimi, N. Combined heat and mass transfer analyses in catalytic microreactors partially filled with porous material-The influences of nanofluid and different porous-fluid interface models. Int. J. Therm. Sci. 2019, 140, 96–113. [Google Scholar] [CrossRef] [Green Version]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3–Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surf. A Physicochem. Eng. Asp. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Takabi, B.; Salehi, S. Augmentation of the heat transfer performance of a sinusoidal corrugated enclosure by employing hybrid nanofluid. Adv. Mech. Eng. 2014, 6, 147059. [Google Scholar] [CrossRef]
- Devi, S.A.; Devi, S.S.U. Numerical investigation of hydromagnetic hybrid Cu–Al2O3/water nanofluid flow over a permeable stretching sheet with suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Ghadikolaei, S.S.; Hosseinzadeh, K.; Hatami, M.; Ganji, D.D. MHD boundary layer analysis for micropolar dusty fluid containing Hybrid nanoparticles (Cu-Al2O3) over a porous medium. J. Mol. Liq. 2018, 268, 813–823. [Google Scholar] [CrossRef]
- Anuar, N.S.; Bachok, N.; Pop, I. Influence of buoyancy force on Ag-MgO/water hybrid nanofluid flow in an inclined permeable stretching/shrinking sheet. Int. Commun. Heat Mass Transf. 2021, 123, 105236. [Google Scholar] [CrossRef]
- Ghadikolaei, S.S.; Hosseinzadeh, K.; Ganji, D.D.; Hatami, M. Fe3O4–(CH2OH)2 nanofluid analysis in a porous medium under MHD radiative boundary layer and dusty fluid. J. Mol. Liq. 2018, 258, 172–185. [Google Scholar] [CrossRef]
- Kumar, K.G.; Hani, E.H.B.; Assad, M.E.H.; Rahimi-Gorji, M.; Nadeem, S. A novel approach for investigation of heat transfer enhancement with ferromagnetic hybrid nanofluid by considering solar radiation. Microsyst. Technol. 2021, 27, 97–104. [Google Scholar] [CrossRef]
- Abbas, N.; Nadeem, S.; Saleem, A.; Malik, M.Y.; Issakhov, A.; Alharbi, F.M. Models base study of inclined MHD of hybrid nanofluid flow over nonlinear stretching cylinder. Chin. J. Phys. 2021, 69, 109–117. [Google Scholar] [CrossRef]
- Riasat, S.; Ramzan, M.; Sun, Y.L.; Malik, M.Y.; Chinram, R. Comparative analysis of Yamada-Ota and Xue models for hybrid nanofluid flow amid two concentric spinning disks with variable thermophysical characteristics. Case Stud. Therm. Eng. 2021, 26, 101039. [Google Scholar] [CrossRef]
- Iijima, S. Helical microtubules of graphitic carbon. Nature 1991, 354, 56–58. [Google Scholar] [CrossRef]
- Harrison, B.S.; Atala, A. Carbon nanotube applications for tissue engineering. Biomaterials 2007, 28, 344–353. [Google Scholar] [CrossRef]
- Raphey, V.R.; Henna, T.K.; Nivitha, K.P.; Mufeedha, P.; Sabu, C.; Pramod, K. Advanced biomedical applications of carbon nanotube. Mater. Sci. Eng. C 2019, 100, 616–630. [Google Scholar] [CrossRef] [PubMed]
- Jame, S.A.; Zhou, Z. Electrochemical carbon nanotube filters for water and wastewater treatment. Nanotechnol. Rev. 2016, 5, 41–50. [Google Scholar] [CrossRef]
- Yin, Z.; Cui, C.; Chen, H.; Yu, X.; Qian, W. The Application of Carbon Nanotube/Graphene-Based Nanomaterials in Wastewater Treatment. Small 2020, 16, 1902301. [Google Scholar] [CrossRef] [PubMed]
- Peng, L.M.; Zhang, Z.; Wang, S. Carbon nanotube electronics: Recent advances. Mater. Today 2014, 17, 433–442. [Google Scholar] [CrossRef]
- Zhao, J.; Shen, L.; Liu, F.; Zhao, P.; Huang, Q.; Han, H.; Peng, L.; Liang, X. Quality metrology of carbon nanotube thin films and its application for carbon nanotube-based electronics. Nano Res. 2020, 13, 1749–1755. [Google Scholar] [CrossRef]
- Nadeem, S.; Saleem, S. Unsteady mixed convection flow of nanofluid on a rotating cone with magnetic field. Appl. Nanosci. 2014, 4, 405–414. [Google Scholar] [CrossRef] [Green Version]
- Ishak, A.; Nazar, R.; Pop, I. Dual solutions in mixed convection boundary-layer flow with suction or injection. IMA J. Appl. Math. 2007, 72, 451–463. [Google Scholar] [CrossRef]
- Ishak, A.; Nazar, R.; Bachok, N.; Pop, I. MHD mixed convection flow near the stagnation-point on a vertical permeable surface. Phys. A Stat. Mech. Appl. 2010, 389, 40–46. [Google Scholar] [CrossRef]
- Tamim, H.; Dinarvand, S.; Hosseini, R.; Pop, I. MHD mixed convection stagnation-point flow of a nanofluid over a vertical permeable surface: A comprehensive report of dual solutions. Heat Mass Transf. 2014, 50, 639–650. [Google Scholar] [CrossRef]
- Rostami, M.N.; Dinarvand, S.; Pop, I. Dual solutions for mixed convective stagnation-point flow of an aqueous silica–alumina hybrid nanofluid. Chin. J. Phys. 2018, 56, 2465–2478. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Roşca, N.C.; Roşca, A.V.; Pop, I. Mixed convection and stability analysis of stagnation-point boundary layer flow and heat transfer of hybrid nanofluids over a vertical plate. Int. J. Numer. Methods Heat Fluid Flow 2019, 30, 3737–3754. [Google Scholar] [CrossRef]
- Gireesha, B.J.; Mahanthesh, B.; Shivakumara, I.S.; Eshwarappa, K.M. Melting heat transfer in boundary layer stagnation-point flow of nanofluid toward a stretching sheet with induced magnetic field. Eng. Sci. Technol. Int. J. 2016, 19, 313–321. [Google Scholar] [CrossRef] [Green Version]
- Kumar, D.; Singh, A.K.; Kumar, D. Influence of heat source/sink on MHD flow between vertical alternate conducting walls with Hall effect. Phys. A Stat. Mech. Appl. 2020, 544, 123562. [Google Scholar] [CrossRef]
- Bhattacharyya, K. Effects of radiation and heat source/sink on unsteady MHD boundary layer flow and heat transfer over a shrinking sheet with suction/injection. Front. Chem. Sci. Eng. 2011, 5, 376–384. [Google Scholar] [CrossRef]
- Ahmed, S.E.; Hussein, A.K.; Mohammed, H.A.; Sivasankaran, S. Boundary layer flow and heat transfer due to permeable stretching tube in the presence of heat source/sink utilizing nanofluids. Appl. Math. Comput. 2014, 238, 149–162. [Google Scholar] [CrossRef]
- Makinde, O.D.; Mabood, F.; Ibrahim, M.S. Chemically reacting on MHD boundary-layer flow of nanofluids over a non-linear stretching sheet with heat source/sink and thermal radiation. Therm. Sci. 2018, 22, 495–506. [Google Scholar] [CrossRef]
- Agrawal, P.; Dadheech, P.K.; Jat, R.N.; Bohra, M.; Nisar, K.S.; Khan, I. Lie similarity analysis of MHD flow past a stretching surface embedded in porous medium along with imposed heat source/sink and variable viscosity. J. Mater. Res. Technol. 2020, 9, 10045–10053. [Google Scholar] [CrossRef]
- Anwar, T.; Kumam, P.; Shah, Z.; Watthayu, W.; Thounthong, P. Unsteady radiative natural convective MHD nanofluid flow past a porous moving vertical plate with heat source/sink. Molecules 2020, 25, 854. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Reddy, N.N.; Rao, V.S.; Reddy, B.R. Chemical reaction impact on MHD natural convection flow through porous medium past an exponentially stretching sheet in presence of heat source/sink and viscous dissipation. Case Stud. Therm. Eng. 2021, 25, 100879. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Anuar, N.S.; Bachok, N. Double solutions and stability analysis of micropolar hybrid nanofluid with thermal radiation impact on unsteady stagnation point flow. Mathematics 2021, 9, 276. [Google Scholar] [CrossRef]
- Arani, A.A.A.; Aberoumand, H. Stagnation-point flow of Ag-CuO/water hybrid nanofluids over a permeable stretching/shrinking sheet with temporal stability analysis. Powder Technol. 2021, 380, 152–163. [Google Scholar] [CrossRef]
- Wahid, N.S.; Arifin, N.M.; Khashi’ie, N.S.; Pop, I. Hybrid Nanofluid Slip Flow over an Exponentially Stretching/Shrinking Permeable Sheet with Heat Generation. Mathematics 2021, 9, 30. [Google Scholar] [CrossRef]
- Khan, U.; Waini, I.; Ishak, A.; Pop, I. Unsteady hybrid nanofluid flow over a radially permeable shrinking/stretching surface. J. Mol. Liq. 2021, 331, 115752. [Google Scholar] [CrossRef]
- Aman, S.; Khan, I.; Ismail, Z.; Salleh, M.Z.; Al-Mdallal, Q.M. Heat transfer enhancement in free convection flow of CNTs Maxwell nanofluids with four different types of molecular liquids. Sci. Rep. 2017, 7, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Ramachandran, N.; Chen, T.S.; Armaly, B.F. Mixed convection in stagnation flows adjacent to vertical surfaces. J. Heat Transf. 1988, 110, 373–377. [Google Scholar] [CrossRef]





| Properties | Hybrid Nanofluid |
|---|---|
| Dynamic viscosity | |
| Heat capacity | |
| Thermal expansion | |
| Density | |
| Thermal conductivity |
| Physical Properties | ||||
|---|---|---|---|---|
| Base fluid: | ||||
| water | 0.613 | 4179 | 997.1 | 21 |
| Carbon nanotube: | ||||
| SWCNT | 6600 | 425 | 2600 | 27 |
| MWCNT | 3000 | 796 | 1600 | 44 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anuar, N.S.; Bachok, N.; Pop, I. Hybrid Carbon Nanotube Flow near the Stagnation Region over a Permeable Vertical Plate with Heat Generation/Absorption. Mathematics 2021, 9, 2925. https://doi.org/10.3390/math9222925
Anuar NS, Bachok N, Pop I. Hybrid Carbon Nanotube Flow near the Stagnation Region over a Permeable Vertical Plate with Heat Generation/Absorption. Mathematics. 2021; 9(22):2925. https://doi.org/10.3390/math9222925
Chicago/Turabian StyleAnuar, Nur Syazana, Norfifah Bachok, and Ioan Pop. 2021. "Hybrid Carbon Nanotube Flow near the Stagnation Region over a Permeable Vertical Plate with Heat Generation/Absorption" Mathematics 9, no. 22: 2925. https://doi.org/10.3390/math9222925
APA StyleAnuar, N. S., Bachok, N., & Pop, I. (2021). Hybrid Carbon Nanotube Flow near the Stagnation Region over a Permeable Vertical Plate with Heat Generation/Absorption. Mathematics, 9(22), 2925. https://doi.org/10.3390/math9222925






