Two Different Views for Generalized Rough Sets with Applications

Abstract

Rough set philosophy is a significant methodology in the knowledge discovery of databases. In the present paper, we suggest new sorts of rough set approximations using a multi-knowledge base; that is, a family of the finite number of general binary relations via different methods. The proposed methods depend basically on a new neighborhood (called basic-neighborhood). Generalized rough approximations (so-called, basic-approximations) represent a generalization to Pawlak’s rough sets and some of their extensions as confirming in the present paper. We prove that the accuracy of the suggested approximations is the best. Many comparisons between these approaches and the previous methods are introduced. The main goal of the suggested techniques was to study the multi-information systems in order to extend the application field of rough set models. Thus, two important real-life applications are discussed to illustrate the importance of these methods. We applied the introduced approximations in a set-valued ordered information system in order to be accurate tools for decision-making. To illustrate our methods, we applied them to find the key foods that are healthy in nutrition modeling, as well as in the medical field to make a good decision regarding the heart attacks problem.

1. Introduction

Rough set theory, proposed by Pawlak [1,2], has been conceived as a tool to conceptualize and analyze various kinds of data. It can be used in attribute value representation models to describe the dependencies among attributes, evaluate the significance of attributes and derive decision rules. The theory presents important applications to intelligent decision-making and cognitive sciences, as a tool for dealing with vagueness and uncertainty of information [3,4,5,6,7,8,9,10,11]. Originally, the rough set theory was based on an assumption that every object in the universe of discourse is associated with some information. Objects which are characterized by the same information are indiscernible. The indiscernibility relation generated in this way shapes the mathematical basis for the theory of rough sets. The set of all indiscernible objects is called an elementary set or equivalent class. Any set of objects, being a union of some elementary sets, is referred to as a crisp set; otherwise it is called a rough set. A rough set can be described by a pair of crisp sets, called the lower and upper approximations. However, this relation represents the basic block in this methodology which is an equivalence relation. But the constraints of this relation restrict the application fields.

By relaxing indiscernibility in relation to more general binary relation, the classical rough set can be extended to a more general model. Slowinski and Vanderpooten [12], Abo Khadra and El-Bably [13], Abo-Tabl [14], and Dai et al. [15] have discussed rough approximation based on the similarity relation. Based on pre-order relation, Kin et al. [16] proposed generalized approximations. Kondo [17] used a reflexive binary relation to suggest generalized definition of rough approximations and compared it with other definitions. Yao [18], Abo Khadra et al. [19], Allam et al. [20], and Ali et al. [21] have studied the approximation operators defined by general binary relation. Rough set models were generalized using topological approaches [3,4,22,23,24,25,26,27] and coverings [28,29,30]. On the other hand, there are some proposals to generalize and extend the application fields of this theory such as [31,32,33,34,35,36]. In the recent paper, we introduced the opposite definition of the new neighborhood (initial-neighborhood), which was presented by El-Sayed et al. [8]. In addition, we studied and examined the relationships among them and provided the common relationship between them and the concept of (core-neighborhood [36]).

The basic motivations of the present research were:

(1)

To initiate a novel neighborhood (so-called basic-neighborhood) construct from any general binary relation and study its properties;

(2)

To propose new generalized rough approximations and investigate their properties based on this neighborhood;

(3)

To solve some problems in set-valued ordered information systems concerning finding the key foods suitable in order to be healthy.

Consequently, the main goals of our approaches were to extend the application fields of rough sets by applying the proposed techniques in decision-making problems such as finding the key foods that are healthy in nutrition modeling, and also in the medical diagnosis of the heart attacks problem. Besides, introducing and studying a novel approach to generalize rough sets of multi-information systems based on a finite number of binary relations. In other words, the main objectives of the present work are to use an arbitrary binary relation to generalize the equivalence relation in the element-based definition from a neighborhood’s point of view. Neighborhood systems are a pivotal technique to reduce the boundary region and to improve the accuracy measure. Thus, we suggested the new notion “basic-neighborhood” which is induced via a general binary relation to investigate this aim. On the other hand, the concept of a neighborhood generated by relations represents a vital bridge between the rough set theory and the other important models such as graph theory, which has many different applications in real-life problems. In simple directed graphs, the rough set theory is used to study nano-topology. Adjacent vertices in digraphs are only used to define their neighborhoods. Therefore, we can use the basic-neighborhoods to introduce new types of vertices neighborhood systems that are dependent on both adjacent vertices and associated edges. Furthermore, the generalization of some concepts presented by Pawlak and Lellis Thivagar, as well as some of their properties, are investigated. Lellis Thivagar has introduced the concept of “nano-topology” based on Pawlak’s rough sets which depend basically on an equivalence relation. Accordingly, their methods restrict the application fields of real-life problems. Thus, we can extend the application fields of graph theory such as “present a new model of the human heart’s blood circulation system based on blood paths and introduce a modern model to smart city which makes a restructuring for the factors which build smart cities in terms of a connected graph”.

The remainder of the paper is organized as follows: The next section provides the basic concepts and results that are used in the paper. Section 3 is devoted to defining the new neighborhood (basic-neighborhood) and to study its properties. Further, we examined the relationships of this neighborhood with other neighborhoods. The main goal of Section 4 was to suggest two different methods to generalize Pawlak rough sets. The first method depends basically on the basic-neighborhood that is induced from any general binary relation and hence we proved that the proposed approximations are generalizations to the Pawlak approach and their generalizations included [12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34]. In the second method, we presented an improvement to Marei’s method [31]. We introduced a novel method for generalized rough approximations based on a finite number of binary relations. This method solved the problems in multi-information systems which depend on many attributes and hence, this method extends the application fields of rough set theory. Comparisons between the proposed methods and the other techniques mentioned in the introduction section were investigated. Finally, in the last section, we introduced two real-life applications, the first of which was in nutrition modeling for our methods. This application shows the significance of our approaches in decision-making. The second was in medical applications; we illustrated the importance of the suggested methods in decision making regarding the heart attacks problem.

2. Pawlak Rough Sets and Some of Its Generalizations

This section provides the basic concepts and results Pawlak’s rough sets, as well as some of their generalizations (namely, Yao [18], Allam et al. [19], Dai et al. [15], and Marei [31]) that are used in the paper.

2.1. Pawlak Rough Set Theory

Definition 1 

([37]). A binary relation $ℛ$ between the two sets $ℳ$ and $𝒩$ (or from $ℳ$ to $𝒩$ ) is a subset of the Cartesian product $ℳ\times 𝒩$, which is a set of ordered pairs $\left(𝓂,𝓃\right)\in ℛ$ where $𝓂\in ℳ$ and $𝓃\in 𝒩$.

Note that: If $ℛ$ is a binary relation from a set $ℳ$ to itself, then it is said to be a binary relation on $ℳ$. Moreover, if $\left(𝓂,𝓃\right)\in ℛ$, then we write $𝓂ℛ𝓃$ to express that $𝓂$ is $ℛ$-related to $𝓃$. On the other hand, the class $𝓂ℛ=\left\{𝓍\in ℳ:𝓂ℛ𝓍\right\}$ (resp. $ℛ𝓂=\left\{𝓍\in ℳ:𝓍ℛ𝓂\right\}$) is called the after set (resp. fore set) of $𝓂\in ℳ$. In the other words, $𝓂ℛ$ (resp. $ℛ𝓂$) is interpreted as the right-neighborhood (resp. left-neighborhood) [18].

Definition 2 

([1]). Suppose that ${ℛ}_{eq}$ represents an equivalence relation on the finite non-empty set $𝒮$, called the universe; thus, the pair ${\rm P}=\left(𝒮,{ℛ}_{eq}\right)$ is said to be the Pawlak approximation space. In addition, the Pawlak-lower (resp. Pawlak-upper) approximation of a subset $ℳ\subseteq 𝒮$ is:

${{\rm P}}_{*}(ℳ)=\left\{𝓍\in 𝒮:{\left[𝓍\right]}_{{ℛ}_{eq}}\subseteq ℳ\right\}$(resp.${{\rm P}}^{*}(ℳ)=\left\{𝓍\in 𝒮:{\left[𝓍\right]}_{{ℛ}_{eq}}\cap ℳ\ne \Phi \right\}$), where${\left[𝓍\right]}_{{ℛ}_{eq}}$represents an equivalence class of$𝓍\in 𝒮$. The boundary and accuracy of Pawlak approximations are defined, respectively, by:

$ℬn{d}_{{ℛ}_{eq}}(ℳ)={{\rm P}}^{*}(ℳ)-{{\rm P}}_{*}(ℳ)$and${\Theta }_{{ℛ}_{eq}}(ℳ)=\frac{\left|{{\rm P}}_{*}(ℳ)\right|}{\left|{{\rm P}}^{*}(ℳ)\right|}$, where${{\rm P}}^{*}(ℳ)\ne \Phi$.

Remark 1. 

Pawlak in [1], stated that:

(i) 

The subset$ℳ$is called an exact set if${{\rm P}}_{*}(ℳ)={{\rm P}}^{*}(ℳ)$and we refer to the pair (${{\rm P}}_{*}(ℳ),{{\rm P}}^{*}(ℳ))$by rough sets with respect to${ℛ}_{eq}$. Otherwise,$ℳ$is called a rough set;

(ii) 

If$ℳ$is exact, then$ℬn{d}_{{ℛ}_{eq}}(ℳ)=\Phi$and${\Theta }_{{ℛ}_{eq}}(ℳ)=1$. On the other hand, if$ℳ$is rough, then$ℬn{d}_{{ℛ}_{eq}}(ℳ)\ne \Phi$and${\Theta }_{{ℛ}_{eq}}(ℳ)\ne 1$.

For more details about Pawlak’s rough sets, see [1,2].

2.2. Yao’s Rough Sets

Definition 3 

([18]). If $ℛ$ is a binary relation on the universe $𝒮$, then for each $𝓍\in 𝒮$, we propose its “right neighborhood” by ${𝓃}_{𝓇}\left(𝓍\right)=\left\{𝓎\in 𝒮:𝓍ℛ𝓎\right\}$.

Definition 4 

([18]). If $ℛ$ is a binary relation on the universe $𝒮$, then the right-lower and right-upper approximations of $ℳ\subseteq 𝒮$ are proposed, respectively, by:

$${\underset{\_}{ℒ}}_{𝓇}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{𝓇}\left(𝓍\right)\subseteq ℳ\right\}\mathrm{and}{\overline{𝒰}}_{𝓇}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{𝓇}\left(𝓍\right)\cap ℳ\ne \Phi \right\}.$$

The right-boundary and the right-accuracy of approximations of a subset$ℳ\subseteq 𝒮$are defined, respectively, by:

$$ℬn{d}_{𝓇}(ℳ)={\overline{𝒰}}_{𝓇}(ℳ)-{\underset{\_}{ℒ}}_{𝓇}(ℳ)\mathrm{and}{\Theta }_{𝓇}(ℳ)=\frac{\left|{\underset{\_}{ℒ}}_{𝓇}(ℳ)\right|}{\left|{\overline{𝒰}}_{𝓇}(ℳ)\right|}$$

where${\overline{𝒰}}_{𝓇}(ℳ)\ne \Phi$.

Note that: The above approximations satisfied some properties of Pawlak’s basic properties in a general case and the remainders of the properties are achieved only if the relation is a preorder relation.

2.3. Allam et al.’s Rough Sets

Definition 5 

([19]). Consider that $ℛ$ represents an arbitrary binary relation on the universe $𝒮$. The minimal neighborhood (briefly, $⋏$-neighborhood) of $𝓍\in 𝒮$, is given by:

$${𝓃}_{⋏}\left(𝓍\right)=\{\begin{array}{c}\underset{𝓍\in 𝓎ℛ}{{\displaystyle \cap }}𝓎ℛ\mathrm{if}\exists 𝓎\in 𝒱s.t.𝓍\in 𝓎ℛ,\\ \Phi \mathrm{Otherwise}.\end{array}$$

Definition 6 

([19]). Consider that $ℛ$ represents an arbitrary binary relation on the universe $𝒮$. Then the minimal-lower (resp. minimal-upper) approximations are:

$${\underset{\_}{ℒ}}_{⋏}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{⋏}\left(𝓍\right)\subseteq ℳ\right\}\mathrm{and}{\overline{𝒰}}_{⋏}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{⋏}\left(𝓍\right)\cap ℳ\ne \Phi \right\}.$$

The boundary and the accuracy of minimal-approximations of a subset$ℳ\subseteq 𝒮$are given, respectively, by:

$$ℬn{d}_{⋏}(ℳ)={\overline{𝒰}}_{⋏}(ℳ)-{\underset{\_}{ℒ}}_{⋏}(ℳ)and{\Theta }_{⋏}(ℳ)=\frac{\left|{\underset{\_}{ℒ}}_{⋏}(ℳ)\right|}{\left|{\overline{𝒰}}_{⋏}(ℳ)\right|},\mathrm{where}{\overline{𝒰}}_{⋏}(ℳ)\ne \Phi .$$

Note that: The above approximations satisfied some properties of Pawlak’s basic properties in a general case and the remainders of the properties are achieved only if the relation is a reflexive relation.

2.4. Dai et al.’s Rough Sets

Definition 7 

([15]). Consider that $ℛ$ represents an arbitrary binary relation on the universe $𝒮$. The maximal neighborhood (briefly, $⋎$-neighborhood) of $𝓍\in 𝒮$, is given by:

$${𝓃}_{⋎}\left(𝓍\right)=\{\begin{array}{c}\underset{𝓍\in 𝓎ℛ}{{\displaystyle \cup }}𝓎ℛ\mathrm{if}\exists 𝓎\in 𝒱s.t.𝓍\in 𝓎ℛ,\\ \Phi \mathrm{Otherwise}.\end{array}$$

Definition 8 

([15]). Consider that $ℛ$ represents an arbitrary binary relation on the universe $𝒮$. Then, the maximal-lower (resp. maximal-upper) approximation of $ℳ\subseteq 𝒱$ is given by:

$${\underset{\_}{ℒ}}_{⋎}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{⋎}\left(𝓍\right)\subseteq ℳ\right\}(\mathrm{resp}.{\overline{𝒰}}_{⋎}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{⋎}\left(𝓍\right)\cap ℳ\ne \Phi \right\}).$$

The boundary and the accuracy of maximal-approximations of a subset$ℳ\subseteq 𝒱$are given, respectively, by:

$$ℬn{d}_{⋎}(ℳ)={\overline{𝒰}}_{⋎}(ℳ)-{\underset{\_}{ℒ}}_{⋎}(ℳ)\mathrm{and}{\Theta }_{⋎}(ℳ)=\frac{\left|{\underset{\_}{ℒ}}_{⋎}(ℳ)\right|}{\left|{\overline{𝒰}}_{⋎}(ℳ)\right|},\mathrm{where}{\overline{𝒰}}_{⋎}(ℳ)\ne \Phi .$$

Note that: The above approximations satisfied some properties of Pawlak’s basic properties in a general case and the remainders of the properties are achieved only if the relation is a similarity relation.

2.5. Marei’s Rough Sets

Marei (2007), in [31], proposed a framework for generalizing Pawlak’s rough sets based on a finite number of reflexive relations as shown in the following definitions.

Definition 9

([31]). Consider $\left\{{ℛ}_i:𝒾=1,2,3,\dots ,n\right\}$ to be a family of binary relations on $𝒮$. Then:

(i) 

The first kind of$n$-lower (resp.$n$-upper) approximation of$ℳ\subseteq 𝒮$ is given by:

$${}_1{}^n\underset{\_}{ℝ}(ℳ)=\left\{𝓍\in 𝒮:\left({{\displaystyle \cap }}_{i=1}^n𝓍{ℛ}_{𝒾}\right)\subseteq ℳ\right\}(\mathrm{resp}.{}_1{}^n\overline{ℝ}(ℳ)=\left\{𝓍\in 𝒮:\left({{\displaystyle \cap }}_{i=1}^n𝓍{ℛ}_{𝒾}\right){\displaystyle \cap }ℳ\ne \Phi \right\});$$

(ii) 

The first kind of$n$-lower (resp.$n$-upper) approximation of$ℳ\subseteq 𝒮$ is given by:

$${}_2{}^n\underset{\_}{ℝ}(ℳ)={{\displaystyle \cup }}_{i=1}^n{\underset{\_}{ℛ}}_i(ℳ)(\mathrm{resp}.{}_2{}^n\overline{ℝ}(ℳ)={{\displaystyle \cap }}_{i=1}^n{\overline{ℛ}}_i(ℳ))$$

where${\underset{\_}{ℛ}}_i(ℳ)=\left\{𝓍\in 𝒮:𝓍{ℛ}_i\subseteq ℳ\right\}$and${\overline{ℛ}}_i(ℳ)=\left\{𝓍\in 𝒮:𝓍{ℛ}_i{\displaystyle \cap }ℳ\ne \Phi \right\}$.

The boundary region and the accuracy of$n$-approximations of$ℳ\subseteq 𝒮$are given, respectively, by:${}_k{}^nℬnd(ℳ)={}_k{}^n\overline{ℝ}(ℳ)-{}_k{}^n\underset{\_}{ℝ}(ℳ)\mathrm{and}{}_k{}^n\Theta (ℳ)=\frac{\left|ℳ{\displaystyle \cap }{}_k{}^n\underset{\_}{ℝ}(ℳ)\right|}{\left|ℳ{\displaystyle \cup }{}_k{}^n\overline{ℝ}(ℳ)\right|}.$

• where${}_k{}^n\overline{ℝ}(ℳ)\ne \Phi$and$k\in \{1,2\}$.

Note that: The above approximations satisfied some properties of Pawlak’s basic properties in a general case and the remainders of the properties are achieved only if the relation is a reflexive relation.

3. New Types of Generalized Neighborhoods

This section introduces and studies a novel kind of neighborhood (called basic-neighborhood) which is induced from a binary relation. In fact, this neighborhood represents the reverse definition for the neighborhood “initial-neighborhood” [8]. Moreover, these two neighborhoods represent an extension of the concept of “core-neighborhood” [37]. Comparisons between the suggested neighborhood and the other types are superimposed.

First, let us remember the definition of “initial-neighborhood” and “core-neighborhood”:

Definition 10 

([8]). Consider that $ℛ$ is an arbitrary binary relation on the universe $𝒮$. The initial-neighborhood of $𝓍\in 𝒮$ is:

${𝓃}_{𝒾}\left(𝓍\right)=\left\{𝓎\in 𝒮:𝓍ℛ\subseteq 𝓎ℛ\right\}=\left\{𝓎\in 𝒮:{𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓎\right)\right\}$.

Definition 11 

([37]). Consider that $ℛ$ is an arbitrary binary relation on the universe $𝒮$. The core-neighborhood of $𝓍\in 𝒮$ is given by:

$${𝓃}_c\left(𝓍\right)=\left\{𝓎\in 𝒮:𝓍ℛ=𝓎ℛ\right\}=\left\{𝓎\in 𝒮:{𝓃}_{𝓇}\left(𝓍\right)={𝓃}_{𝓇}\left(𝓎\right)\right\}.$$

The following definition introduces the new notion of a neighborhood of an element induced by a binary relation which represents the opposite concept of the “initial-neighborhood”.

Definition 12.

Consider that$ℛ$is an arbitrary binary relation on the universe$𝒮$. The basic-neighborhood of$𝓍$(in briefly,$b$-neighborhood) of $𝓍\in 𝒮$, is given by:

$${𝓃}_b\left(𝓎\right)=\left\{𝓎\in 𝒮:𝓎ℛ\subseteq 𝓍ℛ\right\}=\left\{𝓎\in 𝒮:{𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)\right\}.$$

The main goal of the following results was to introduce the basic properties of $\mathfrak{b}$-neighborhoods. Further, we illustrate the relationships among different types of neighborhoods.

Lemma 1.

If$ℛ$is an arbitrary binary relation on$𝒮$, then:

(a) 

For each$𝓍\in 𝒮$,$𝓍\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$;

(b) 

For each$𝓍,𝓎\in 𝒮$,$𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$if and only if${𝓃}_{\mathfrak{b}}\left(𝓎\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$;

Proof. 

(a)

By using Definition 10, the proof is obvious;

(b)

By using Definition 10, if $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. Then ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ … (1)

Now, let $𝓏\in {𝓃}_{\mathfrak{b}}\left(𝓎\right)$. Then ${𝓃}_{𝓇}\left(𝓏\right)\subseteq {𝓃}_{𝓇}\left(𝓎\right)$. Therefore, by (1), ${𝓃}_{𝓇}\left(𝓏\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$, and hence $𝓏\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. Accordingly, ${𝓃}_{\mathfrak{b}}\left(𝓎\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$;

Conversely, let ${𝓃}_{\mathfrak{b}}\left(𝓎\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. However, by (a), $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓎\right)$. Hence, $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. □

Remark 2.

The following example illustrates that:

(a) 

The inclusion sign in (a) of Lemma 1 need not be equal, in general;

(b) 

The “basic-neighborhood” and “initial-neighborhood” are independent (non-comparable);

(c) 

The neighborhoods${𝓃}_{𝓇}\left(𝓍\right)$,${𝓃}_{⋏}\left(𝓍\right)$,${𝓃}_{⋎}\left(𝓍\right)$, and${𝓃}_{\mathfrak{b}}\left(𝓍\right)$, for each$𝓍\in 𝒮$, are independent (i.e., non-comparable) in general.

Example 1.

Consider that$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$and$ℛ=\left\{\left(ℊ,ℊ\right),\left(𝓀,ℊ\right),\left(ℊ,𝓂\right),\left(\ell ,𝓀\right)\right\}$is a binary relation on$𝒮$. Then, we attained the following:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{ℊ,𝓂\right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\{ℊ\}\\ {𝓃}_{𝓇}(\ell )=\left\{𝓀\right\}\\ {𝓃}_{𝓇}\left(𝓂\right)=\Phi \end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋏}(ℊ)=\{ℊ\}\\ {𝓃}_{⋏}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{⋏}(\ell )=\Phi \\ {𝓃}_{⋏}\left(𝓂\right)=\left\{ℊ,𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,𝓂\right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{⋎}(\ell )=\Phi \\ {𝓃}_{⋎}\left(𝓂\right)=\left\{ℊ,𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{𝒾}(ℊ)=\{ℊ\}\\ {𝓃}_{𝒾}\left(𝓀\right)=\left\{ℊ,𝓀\right\}\\ {𝓃}_{𝒾}(\ell )=\{\ell \}\\ {𝓃}_{𝒾}\left(𝓂\right)=𝒮\end{array}and\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}(\ell )=\left\{\ell ,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}\end{array}$$

The following result proves that these two neighborhoods are generalization to “core-neighborhood”.

Lemma 2. 

If$ℛ$is a binary relation on$𝒮$. Then, for each$𝓍\in 𝒮$:

(a) 

${𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }{𝓃}_{𝒾}\left(𝓍\right)={𝓃}_c\left(𝓍\right)$.

(b) 

${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {𝓃}_c\left(𝓍\right)$and${𝓃}_{𝒾}\left(𝓍\right)\subseteq {𝓃}_c\left(𝓍\right)$.

Proof. 

We proved (a) and (b) in a similar way.

$𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }{𝓃}_{𝒾}\left(𝓍\right)$ if and only if $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$ and $𝓎\in {𝓃}_{𝒾}\left(𝓍\right)$ if and only if ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ and ${𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓎\right)$. Therefore, $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }{𝓃}_{𝒾}\left(𝓍\right)$ if and only if ${𝓃}_{𝓇}\left(𝓍\right)={𝓃}_{𝓇}\left(𝓎\right)$ if and only if $𝓎\in {𝓃}_c\left(𝓍\right)$. □

Lemma 3. 

If$ℛ$is a reflexive relation on$𝒮$, then, for each$𝓍\in 𝒮$:

(a) 

${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{⋎}\left(𝓍\right)$;

(b) 

$and{𝓃}_{⋏}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{⋎}\left(𝓍\right)$.

Proof. 

We proved (a) and in a similar way it is easy to demonstrate (b).

Let $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$, then ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ … (1)

Since $ℛ$ is a reflexive relation on $𝒮$, then $𝓎\in 𝓎ℛ$ and $𝓎\in {𝓃}_{𝓇}\left(𝓎\right),\mathrm{and}$ thus, by (1), $𝓎\in {𝓃}_{𝓇}\left(𝓍\right)$ and hence ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$. Similarly, we can prove ${𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{⋎}\left(𝓍\right)$. □

Remark 3. 

The next example shows that:

(a) 

The opposite of Lemma 3 is not true in general;

(b) 

The neighborhoods${𝓃}_{\mathfrak{b}}\left(𝓍\right)$and${𝓃}_{⋏}\left(𝓍\right)$are independent in the case of a reflexive relation.

Example 2. 

Consider$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$and$ℛ=\left\{\left(ℊ,ℊ\right),\left(ℊ,𝓀\right),\left(ℊ,\ell \right),\left(𝓀,𝓀\right),\left(𝓀,\ell \right),\left(𝓀,𝓂\right),\left(\ell ,\ell \right),\left(𝓂,𝓂\right)\right\}$is a reflexive relation on$𝒮$. Then, we attain the following:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{ℊ,𝓀,\ell \right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\left\{𝓀,\ell ,𝓂\right\}\\ {𝓃}_{𝓇}(\ell )=\{\ell \}\\ {𝓃}_{𝓇}\left(𝓂\right)=\left\{𝓂\right\}\end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋏}(ℊ)=\left\{ℊ,𝓀,\ell \right\}\\ {𝓃}_{⋏}\left(𝓀\right)=\left\{𝓀,\ell \right\}\\ {𝓃}_{⋏}(\ell )=\{\ell \}\\ {𝓃}_{⋏}\left(𝓂\right)=\left\{𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,𝓀,\ell \right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{𝒮\right\}\\ {𝓃}_{⋎}(\ell )=\left\{𝒮\right\}\\ {𝓃}_{⋎}\left(𝓂\right)=\{𝓀,\ell ,𝓂\}\end{array}\mathrm{and}\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\left\{ℊ,\ell \right\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,\ell ,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}(\ell )=\{\ell \}.\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}.\end{array}$$

Remark 4. 

Let$𝒮$be a finite set and$ℛ$be a symmetric relation to$𝒮$. Then, for each$𝓍\in 𝒮$, neighborhoods${𝓃}_{𝓇}\left(𝓍\right)$,${𝓃}_{⋏}\left(𝓍\right)$,${𝓃}_{⋎}\left(𝓍\right)$, and${𝓃}_{\mathfrak{b}}\left(𝓍\right)$, for each$𝓍\in 𝒮$, are independent (i.e., non-comparable) as demonstrated in the subsequent example.

Example 3. 

Consider$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$and a relation$ℛ=\left\{\left(ℊ,𝓀\right),\left(𝓀,ℊ\right),\left(𝓀,\ell \right),\left(\ell ,𝓀\right),\left(\ell ,𝓂\right),\left(𝓂,\ell \right)\right\}$is a symmetric relation on$𝒮$. Hence, we attain the following:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{𝓀\right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\left\{ℊ,\ell \right\}\\ {𝓃}_{𝓇}(\ell )=\left\{𝓀,𝓂\right\}\\ {𝓃}_{𝓇}\left(𝓂\right)=\{\ell \}\end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋏}(ℊ)=\left\{ℊ,\ell \right\}\\ {𝓃}_{⋏}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{⋏}(\ell )=\{\ell \}\\ {𝓃}_{⋏}\left(𝓂\right)=\left\{𝓀,𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,\ell \right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{𝓀,𝓂\right\}\\ {𝓃}_{⋎}(\ell )=\left\{ℊ,\ell \right\}\\ {𝓃}_{⋎}\left(𝓂\right)=\left\{𝓀,𝓂\right\}\end{array}\mathrm{and}\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\{ℊ\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}(\ell )=\left\{ℊ,\ell \right\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}.\end{array}$$

Corollary 1. 

If a similarity relation on$𝒮$. Then, for each$𝓍\in 𝒮$:

(a) 

${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{⋎}\left(𝓍\right)$;

(b) 

$and{𝓃}_{⋏}\left(𝓍\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{⋎}\left(𝓍\right)$.

Note that: The inverse of the above result is not correct generally.

Lemma 4. 

If$ℛ$is a transitive relation on$𝒮$, then${𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$, for each$𝓍\in 𝒮$.

Proof. 

Firstly let $𝓎\in {𝓃}_{𝓇}\left(𝓍\right)$, then $𝓍ℛ𝓎$ … (1)

Now, we need to prove that ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ as follows:

Let $𝓏\in {𝓃}_{𝓇}\left(𝓎\right)$, then $𝓎ℛ𝓏$. However, $ℛ$ is a transitive relation and by using (1), we obtain $𝓍ℛ𝓏$ and $𝓏\in {𝓃}_{𝓇}\left(𝓍\right)$. Therefore, ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ and then $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. Hence, ${𝓃}_{𝓇}\left(𝓍\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. □

Remark 5. 

The next example shows that:

(a) 

The converse of Lemma 4 is not true in general;

(b) 

The neighborhoods${𝓃}_{\mathfrak{b}}\left(𝓍\right)$and${𝓃}_{⋏}\left(𝓍\right)$are independent in the case of a transitive relation;

(c) 

The neighborhoods${𝓃}_{\mathfrak{b}}\left(𝓍\right)$and${𝓃}_{⋎}\left(𝓍\right)$are independent in the case of a transitive relation.

Example 4. 

Consider$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$and$ℛ=\left\{\left(ℊ,ℊ\right),\left(ℊ,𝓀\right),\left(𝓀,𝓂\right),\left(\ell ,\ell \right),\left(ℊ,𝓂\right)\right\}$is a transitive relation on$𝒮$. Then we attain the following:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\left\{𝓂\right\}\\ {𝓃}_{𝓇}(\ell )=\{\ell \}\\ {𝓃}_{𝓇}\left(𝓂\right)=\Phi \end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋏}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋏}\left(𝓀\right)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋏}(\ell )=\{\ell \}\\ {𝓃}_{⋏}\left(𝓂\right)=\left\{𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋎}(\ell )=\{\ell \}\\ {𝓃}_{⋎}\left(𝓂\right)=\left\{ℊ,𝓀,𝓂\right\}\end{array}\mathrm{and}\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}\left(c\right)=\left\{\ell ,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}.\end{array}$$

Lemma 5. 

If$ℛ$is a preorder relation on$𝒮$, then$\forall 𝓍\in 𝒮$,${𝓃}_{𝓇}\left(𝓍\right)={𝓃}_{⋏}\left(𝓍\right)={𝓃}_{\mathfrak{b}}\left(𝓍\right)$.

Proof. 

Firstly, by using Lemmas 3 and 4, we obtain ${𝓃}_{𝓇}\left(𝓍\right)={𝓃}_{\mathfrak{b}}\left(𝓍\right)$.

Now, let $𝓎\in {𝓃}_{⋏}\left(𝓍\right)$. Then $𝓎$ belongs to every after set that contains $𝓍$. Therefore, $𝓎\in 𝓍ℛ$ (since, $𝓍\in 𝓍ℛ$, by the reflexivity of $ℛ$) and then $𝓍ℛ𝓎$ … (1)

Therefore, we need to prove ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ as follows:

Let $𝓏\in {𝓃}_{𝓇}\left(𝓎\right)$, then $𝓎ℛ𝓏$ and by (1), we obtain $𝓍ℛ𝓏$ (since $ℛ$ is transitive). Therefore, $𝓏\in {𝓃}_{𝓇}\left(𝓍\right)$ and this implies ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$. Accordingly, $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$ and then ${𝓃}_{⋏}\left(𝓍\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$.

Conversely, let $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$. Then ${𝓃}_{𝓇}\left(𝓎\right)\subseteq {𝓃}_{𝓇}\left(𝓍\right)$ … (2)

Thus, we need to prove that $𝓎$ belongs to every after set that contains $𝓍$ as follows:

Let $\exists 𝓏\in 𝒮$ such that $𝓍\in 𝓏ℛ$ and $𝓎\notin 𝓏ℛ$. Then, $𝓏ℛ𝓍$ and by (2), $𝓎\in 𝓍ℛ$ (by reflexivity of $ℛ$) which tends to $𝓏ℛ𝓍$ and $𝓍ℛ𝓎$ such that $𝓎\notin 𝓏ℛ$ which is a contradiction to $ℛ$ is a transitive relation. Thus, $𝓎\in 𝓏ℛ$ and this implies $𝓎$ must be belonging to every after set containing $𝓍$. Therefore, $𝓎\in {𝓃}_{⋏}\left(𝓍\right)$ and hence ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {𝓃}_{⋏}\left(𝓍\right)$. □

Corollary 2. 

If$ℛ$is an equivalence relation on$𝒮$, then${𝓃}_{\mathfrak{b}}\left(𝓍\right)={𝓃}_{⋏}\left(𝓍\right)={𝓃}_{⋎}\left(𝓍\right)={\left[𝓍\right]}_{ℛ}$,$\forall 𝓍\in 𝒮$.

Remark 6. 

If$ℛ$is a preorder relation on$𝒮$, then$\forall 𝓍\in 𝒮$,${𝓃}_{⋎}\left(𝓍\right)\ne {𝓃}_{\mathfrak{b}}\left(𝓍\right)$as demonstrated by the next example.

Example 5. 

Consider$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$and$ℛ=\left\{\left(ℊ,ℊ\right),\left(𝓀,𝓀\right),\left(\ell ,\ell \right),\left(𝓂,𝓂\right),\left(ℊ,𝓀\right),\left(𝓀,𝓂\right),\left(ℊ,𝓂\right)\right\}$is a preorder relation on$𝒮$. Then we attain the following:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\left\{𝓀,𝓂\right\}\\ {𝓃}_{𝓇}(\ell )=\{\ell \}\\ {𝓃}_{𝓇}\left(𝓂\right)=\left\{𝓂\right\}\end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{⋎}(\ell )=\{\ell \}\\ {𝓃}_{⋎}\left(𝓂\right)=\left\{ℊ,𝓀,𝓂\right\}\end{array},\mathrm{and}\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,𝓂\right\}.\\ {𝓃}_{\mathfrak{b}}\left(c\right)=\{\ell \}.\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}.\end{array}$$

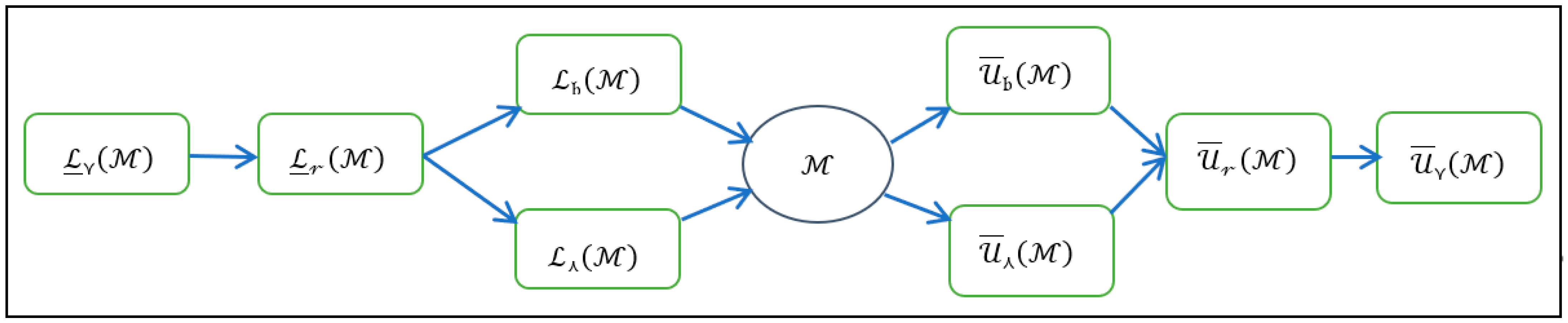
4. Two Different Views for Generalized Rough Sets via Basic Neighborhoods

In the present section, we suggest and study two different methods to generalize and improve Pawlak’s rough set models. The second method is very important for multi-information systems and hence, it can be useful in multi attributes decision making. Many comparisons between the proposed approaches and the previous methods (namely, Yao [18], Allam et al. [19], Dai et al. [15], and Marei [31]) are investigated.

4.1. The First Method to Generalization Based on a One Binary Relation

Based on the neighborhood (basic-neighborhood), new rough approximations are presented and their properties are studied. We proved that these approaches are stronger than the other methods.

Definition 13. 

Consider$ℛ$is any arbitrary binary relation on$𝒮$. The basic-lower (resp. basic-upper) approximation of$ℳ\subseteq 𝒮$ is given by:

$${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq ℳ\right\}(\mathrm{resp}.{\overline{𝒰}}_{\mathfrak{b}}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }ℳ\ne \Phi \right\}).$$

The boundary and the accuracy of maximal-approximations of a subset$ℳ\subseteq 𝒮$are given, respectively, by:

$$ℬn{d}_{\mathfrak{b}}(ℳ)={\overline{𝒰}}_{\mathfrak{b}}(ℳ)-{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\mathrm{and}{\Theta }_{\mathfrak{b}}(ℳ)=\frac{\left|{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right|}{\left|{\overline{𝒰}}_{\mathfrak{b}}(ℳ)\right|},\mathrm{where}{\overline{𝒰}}_{\mathfrak{b}}(ℳ)\ne \Phi .$$

Obviously,$0\le {\Theta }_{\mathfrak{b}}(ℳ)\le 1$and if${\Theta }_{\mathfrak{b}}(ℳ)=1$, then $ℳ$is a basic-exact set (briefly,$\mathfrak{b}$-exact). Otherwise, it is a basic-rough set (briefly,$\mathfrak{b}$-rough).

The following proposition provides the main properties of the “basic-approximations”.

Theorem 1. 

If$ℛ$is an arbitrary binary relation on$𝒮$, and$ℳ,𝒩\subseteq 𝒮$. Then, the following axioms are held:

$$\begin{gathered} \left(\mathrm{L}1\right){\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\subseteq ℳ \\ \left(\mathrm{L}2\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left(\Phi \right)=\Phi \\ \left(\mathrm{L}3\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒮\right)=𝒮 \\ \left(\mathrm{L}4\right)\mathrm{If}ℳ\subseteq 𝒩,\mathrm{then}{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right) \\ \left(\mathrm{L}5\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)={\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right) \\ \left(\mathrm{L}6\right){\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cup }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cup }𝒩\right) \\ \left(\mathrm{L}7\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left({ℳ}^c\right)={({\overline{𝒰}}_{\mathfrak{b}}(ℳ))}^c \\ \left(\mathrm{L}8\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right)={\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ) \\ \left(\mathrm{LU}\right){\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\subseteq {\overline{𝒰}}_{\mathfrak{b}}(ℳ) \end{gathered}$$

$$\begin{gathered} \left(\mathrm{U}1\right)ℳ\subseteq {\overline{𝒰}}_{\mathfrak{b}}(ℳ) \\ \left(\mathrm{U}2\right){\overline{𝒰}}_{\mathfrak{b}}\left(\Phi \right)=\Phi \\ \left(\mathrm{U}3\right){\overline{𝒰}}_{\mathfrak{b}}\left(𝒮\right)=𝒮 \\ \left(\mathrm{U}4\right)\mathrm{If}ℳ\subseteq 𝒩,\mathrm{then}{\overline{𝒰}}_{\mathfrak{b}}(ℳ)\subseteq {\overline{𝒰}}_{\mathfrak{b}}\left(𝒩\right) \\ \left(\mathrm{U}5\right){\overline{𝒰}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cup }𝒩\right)={\overline{𝒰}}_{\mathfrak{b}}(ℳ){\displaystyle \cup }{\overline{𝒰}}_{\mathfrak{b}}\left(𝒩\right) \\ \left(\mathrm{U}6\right){\overline{𝒰}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\overline{𝒰}}_{\mathfrak{b}}\left(𝒩\right)\supseteq {\overline{𝒰}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right) \\ \left(\mathrm{U}7\right){\overline{𝒰}}_{\mathfrak{b}}\left({ℳ}^c\right)={({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ))}^c \\ \left(\mathrm{U}8\right){\overline{𝒰}}_{\mathfrak{b}}\left({\overline{𝒰}}_{\mathfrak{b}}(ℳ)\right)={\overline{𝒰}}_{\mathfrak{b}}(ℳ) \end{gathered}$$

Proof. 

The properties (L1–L3, LU) and (U1–U3) are straightforward.

Thus, we prove the remaining properties as follows:

(L4) Let $ℳ\subseteq 𝒩,$ then ${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq ℳ\right\}\subseteq \left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq 𝒩\right\}={\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)$;

(L5) First, by (L4), we have ${\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)$ (since $ℳ{\displaystyle \cap }𝒩\subseteq ℳ$ and $ℳ{\displaystyle \cap }𝒩\subseteq 𝒩$);

Now, let $𝓍\in \left[{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)\right]$. Then $𝓍\in {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$ and $𝓍\in {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)$ which implies ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq ℳ$ and ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq 𝒩$. Thus, ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq \left(ℳ{\displaystyle \cap }𝒩\right)$ and $𝓍\in {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)$. Therefore, ${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)$ and then ${\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)={\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)$;

(L6) Since $ℳ\subseteq \left(ℳ{\displaystyle \cup }𝒩\right)$ and $𝒩\subseteq \left(ℳ{\displaystyle \cup }𝒩\right)$. Then, by (L4), ${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cup }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cup }𝒩\right)$;

(L7) $$\begin{gathered} {({\overline{𝒰}}_{\mathfrak{b}}(ℳ))}^c={\left(\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }ℳ\ne \Phi \right\}\right)}^c=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right){\displaystyle \cap }ℳ=\Phi \right\} \\ =\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {ℳ}^c\right\}={\underset{\_}{ℒ}}_{\mathfrak{b}}\left({ℳ}^c\right); \end{gathered}$$

(L8) First, ${\underset{\_}{ℒ}}_{\mathfrak{b}}\left({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$, by using (L1);

Now, let $𝓍\in {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$, then ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq ℳ$. We need to prove that ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$ as follows:

Let $𝓎\in {𝓃}_{\mathfrak{b}}\left(𝓍\right)$, then ${𝓃}_{\mathfrak{b}}\left(𝓎\right)\subseteq {𝓃}_{\mathfrak{b}}\left(𝓍\right)$ and thus ${𝓃}_{\mathfrak{b}}\left(𝓎\right)\subseteq ℳ$. Accordingly, $𝓎\in {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$ and this implies ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$ and $𝓍\in {\underset{\_}{ℒ}}_{\mathfrak{b}}\left({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right)$. Therefore, ${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}\left({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right)$.

In similar way, we can prove the properties (U4) and (U8). □

Remark 7. 

The reverse relations in the properties (L6) and (U6) of Theorem 1 do not hold in general as Example 6 illustrates.

Example 6. 

(Continued toExample 3), let$ℳ=\left\{ℊ,𝓀\right\}$and$𝒩=\left\{𝓂\right\}$. Then we attain:

$ℳ{\displaystyle \cap }𝒩=\Phi$and$ℳ{\displaystyle \cup }𝒩=\left\{ℊ,𝓀,𝓂\right\}$. Thus, their basic-approximations are${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)=\{ℊ\}$,${\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)=\left\{𝓂\right\}$,and${\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cup }𝒩\right)=\left\{ℊ,𝓀,𝓂\right\}$. In addition,${\overline{𝒰}}_{\mathfrak{b}}(ℳ)=\left\{ℊ,𝓀,\ell \right\}$,${\overline{𝒰}}_{\mathfrak{b}}\left(𝒩\right)=\left\{𝓀,𝓂\right\}$and${\overline{𝒰}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)=\Phi$.

Obviously,${\underset{\_}{ℒ}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cup }𝒩\right)\ne {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ){\displaystyle \cup }{\underset{\_}{ℒ}}_{\mathfrak{b}}\left(𝒩\right)$ and${\overline{𝒰}}_{\mathfrak{b}}\left(ℳ{\displaystyle \cap }𝒩\right)\ne {\overline{𝒰}}_{\mathfrak{b}}(ℳ){\displaystyle \cap }{\overline{𝒰}}_{\mathfrak{b}}\left(𝒩\right)$.

By using Corollary 2, we can easily prove the following result. Thus, the proof was omitted.

Theorem 2. 

If$ℛ$is an equivalence relation on$𝒮$, and$ℳ\subseteq 𝒮$, then the following is held:

$$\left(\mathrm{L}9\right){\underset{\_}{ℒ}}_{\mathfrak{b}}\left({\overline{𝒰}}_{\mathfrak{b}}(ℳ)\right)={\overline{𝒰}}_{\mathfrak{b}}(ℳ).\left(\mathrm{U}9\right){\overline{𝒰}}_{\mathfrak{b}}\left({\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\right)={\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ).$$

Remark 8. 

The above results (Theorems 1 and 2) proved that the suggested approximations (basic-approximations) represent the natural generalization to Pawlak’s rough set methodology and its generalizations. Since our methods satisfy all properties of Pawlak’s models without any constraints, and thus these extend the application field of this interesting theory in many real-life problems and sciences.

4.2. The Second Method to Generalization Based on a Finite Number of Binary Relations

This subsection is devoted to suggesting generalized rough sets based on a finite number of binary relations. In fact, we extend Marei’s [31] definition to any binary relation and provide some modifications and improvements for his approaches. Moreover, our approaches solve some problems in Marei’s definition.

Definition 14. 

Consider$\left\{{ℛ}_i:𝒾=1,2,3,\dots ,n\right\}$to be a family of binary relations on$𝒮$. Then:

(i) 

The first kind of$n$-basic lower (resp.$n$-basic upper) approximation of$ℳ\subseteq 𝒮$ is given by:

$$\begin{gathered} {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)=\left\{𝓍\in 𝒮:\left({{\displaystyle \cap }}_{i=1}^n{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)\right)\subseteq ℳ\right\}(\mathrm{resp}.{}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)=\left\{𝓍\in 𝒮:\left({{\displaystyle \cap }}_{i=1}^n{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)\right){\displaystyle \cap }ℳ\ne \Phi \right\}),\mathrm{where} \\ {𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)=\left\{𝓎\in 𝒮:𝓎{ℛ}_{𝒾}\subseteq 𝓍{ℛ}_{𝒾}\right\}; \end{gathered}$$

(ii) 

The second kind of$n$-basic lower (resp.$n$-basic upper) approximation of$ℳ\subseteq 𝒮$ is given by:

$${}_2{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)={{\displaystyle \cup }}_{i=1}^n{\underset{\_}{ℒ}}_{{\mathfrak{b}}_i}(ℳ)(\mathrm{resp}.{}_2{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)={{\displaystyle \cap }}_{i=1}^n{\overline{𝒰}}_{{\mathfrak{b}}_i}(ℳ))$$

where${\underset{\_}{ℒ}}_{{\mathfrak{b}}_i}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}{}_i\left(𝓍\right)\subseteq ℳ\right\}$and${\overline{𝒰}}_{{\mathfrak{b}}_i}(ℳ)=\left\{𝓍\in 𝒮:{𝓃}_{\mathfrak{b}}{}_i\left(𝓍\right){\displaystyle \cap }ℳ\ne \Phi \right\}$.

The boundary and the accuracy of $n$-basic approximations of a subset $ℳ\subseteq 𝒮$ are given, respectively, by:

$${}_k{}^nℬn{d}_{\mathfrak{b}}(ℳ)={}_k{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)-{}_k{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\mathrm{and}{}_k{}^n\Theta {}_{\mathfrak{b}}(ℳ)=\frac{\left|{}_k{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\right|}{\left|{}_k{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\right|},\mathrm{where}{}_k{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\ne \Phi \mathrm{and}k\in \left\{1,2\right\}.$$

If ${}_k{}^n\Theta {}_{\mathfrak{b}}(ℳ)=1$, then ${}_k{}^nℬn{d}_{\mathfrak{b}}(ℳ)=\Phi$ and $ℳ$ is called $n$-basic exact set. Otherwise, it is $n$-basic rough.

It is easy to prove the next results using Theorem 1, so we omitted the proof.

Theorem 3. 

Let$\left\{{ℛ}_i:𝒾=1,2,3,\dots ,n\right\}$be a family of binary relations on$𝒮$. Then, the$n$-basic approximations satisfy the properties of Pawlak’s rough sets (L1–L8) and (U1–U8) in the general case without any conditions on the relations ${ℛ}_{𝒾}$.

Note that: The previous result (Theorem 3) represents the first differences between our approaches and Marei’s approaches. Moreover, it demonstrates that the suggested approximations in Definition 14 represent a generalization to Pawlak’s rough sets and some of its generalizations.

Theorem 4. 

Let$\left\{{ℛ}_{𝒾}:𝒾=1,2,3,\dots ,n\right\}$be a family of equivalence relations on$𝒮$. Then, the$n$-basic approximations satisfy the properties of Pawlak’s rough sets (L1–L10) and (U1–U10).

The elementary objective of the following result is to explain the relationship between the first and second kinds of $n$-basic approximations.

Theorem 5. 

Let$\left\{{ℛ}_{𝒾}:𝒾=1,2,3,\dots ,n\right\}$be a family of binary relations on$𝒮$. Then, the following axioms are true:

(i) 

${}_2{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$and${}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_2{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)$;

(ii) 

${}_1{}^nℬn{d}_{\mathfrak{b}}(ℳ)\subseteq {}_2{}^nℬn{d}_{\mathfrak{b}}(ℳ)$and${}_2{}^n\Theta {}_{\mathfrak{b}}(ℳ)\le {}_1{}^n\Theta {}_{\mathfrak{b}}(ℳ)$;

(iii) 

If$ℳ$is of second kind$n$-basic exact, then it is of first kind$n$-basic exact.

Proof. 

We shall prove (i) only, and the other statements directly by (i).

Let $𝓍\in {}_2{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$, then $𝓍\in {{\displaystyle \cup }}_{i=1}^n{\underset{\_}{ℒ}}_{{\mathfrak{b}}_{𝒾}}(ℳ)$ and this implies $\exists i_0\in I$ such that $𝓍\in {\underset{\_}{ℒ}}_{{\mathfrak{b}}_{i_0}}(ℳ)$. Therefore, ${𝓃}_{\mathfrak{b}}{}_{i_0}\left(𝓍\right)\subseteq ℳ$, but $\left({{\displaystyle \cap }}_{i=1}^n{𝓃}_{\mathfrak{b}}{}_i\left(𝓍\right)\right)\subseteq {𝓃}_{\mathfrak{b}}{}_{i_0}\left(𝓍\right)$ and hence, $\left({{\displaystyle \cap }}_{i=1}^n{𝓃}_{\mathfrak{b}}{}_i\left(𝓍\right)\right)\subseteq ℳ$. Thus, $𝓍\in {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$. Accordingly, ${}_2{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$.

Similarly, we can prove that ${}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_2{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)$. □

Note that: The opposite statements in the above result need not be true, in general, as illustrated in the following example.

Example 7. 

Suppose that${ℛ}_1=\{\left(ℊ,ℊ\right),\left(ℊ,𝓀\right),\left(𝓀,𝓀\right),$ $\left(𝓀,\ell \right),\left(\ell ,𝓀\right),\left(𝓂,ℊ\right),\left(𝓂,𝓀\right)\}$and${ℛ}_2=\{(\left(ℊ,ℊ\right),$ $\left(𝓀,𝓀\right),$ $\left(\ell ,ℊ\right),\left(\ell ,𝓀\right),\left(𝓂,ℊ\right)\}$are two binary relations on$𝒮=\left\{ℊ,𝓀,\ell ,𝓂\right\}$. Hence, we attain the following:

$$\begin{array}{c}{𝓃}_{{\mathfrak{b}}_1}(ℊ)=\{ℊ,\ell ,𝓂\}\\ {𝓃}_{{\mathfrak{b}}_1}\left(𝓀\right)=\{𝓀,\ell \}\\ {𝓃}_{{\mathfrak{b}}_1}(\ell )=\{\ell \}\\ {𝓃}_{{\mathfrak{b}}_1}\left(𝓂\right)=\{ℊ,\ell ,𝓂\}\\ {𝓃}_{{\mathfrak{b}}_2}(ℊ)=\{ℊ,𝓂\}\\ {𝓃}_{{\mathfrak{b}}_2}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{{\mathfrak{b}}_2}(\ell )=\{ℊ,𝓀,\ell \}\\ {𝓃}_{{\mathfrak{b}}_2}\left(𝓂\right)=\{ℊ,𝓂\}\end{array}\}\Rightarrow \{\begin{array}{c}\underset{i=1}{\overset{2}{\cap }}{𝓃}_{{\mathfrak{b}}_i}(ℊ)=\{ℊ,𝓂\}.\\ \underset{i=1}{\overset{2}{\cap }}{𝓃}_{{\mathfrak{b}}_i}\left(𝓀\right)=\left\{𝓀\right\}.\\ \underset{i=1}{\overset{2}{\cap }}{𝓃}_{{\mathfrak{b}}_i}(\ell )=\{\ell \}.\\ \underset{i=1}{\overset{2}{\cap }}{𝓃}_{{\mathfrak{b}}_i}\left(𝓂\right)=\left\{𝓂\right\}.\end{array}$$

Now, let$ℳ=\left\{𝓀,\ell ,𝓂\right\}$. Then,${\underset{\_}{ℒ}}_{{\mathfrak{b}}_1}(ℳ)=\left\{𝓀,\ell \right\}$and${\underset{\_}{ℒ}}_{{\mathfrak{b}}_2}(ℳ)=\left\{𝓀\right\}$. Therefore,${}_2{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)=\left\{𝓀,\ell \right\}$, but${}_1{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)=\left\{𝓀,\ell ,𝓂\right\}$. Clearly,${}_2{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)⊊{}_1{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$. Moreover,${}_2{}^2\overline{ℝ}{}_{\mathfrak{b}}(ℳ)=𝒮$and${}_1{}^2\overline{ℝ}{}_{\mathfrak{b}}(ℳ)=\left\{𝓀,\ell ,𝓂\right\}$. Thus,${}_1{}^2\overline{ℝ}{}_{\mathfrak{b}}(ℳ)⊊{}_2{}^2\overline{ℝ}{}_{\mathfrak{b}}(ℳ)$. Accordingly,${}_1{}^2ℬn{d}_{\mathfrak{b}}(ℳ)=\Phi$and${}_1{}^2\Theta {}_{\mathfrak{b}}(ℳ)=1$, but${}_2{}^2ℬ𝓃d_{\mathfrak{b}}(ℳ)=\left\{ℊ,𝓂\right\}$and${}_2{}^2\Theta {}_{\mathfrak{b}}(ℳ)=\frac{1}{2}$. Thus,$ℳ$is first kind$2$-basic exact although it is asecond kind$2$-basic rough set.

4.3. Comparisons between the Suggested Approaches and the Other Methods

The following results demonstrate the relationships among the suggested approximations (b-approximations) and some of the other approaches (existing methods in the literature).

Case (1): General binary relation.

Firstly, the different approximations (Yao [18], Allam [19], Dai [15], and current approaches) are independent. Further, the previous methods failed in satisfying the basic properties of Pawlak’s methodology. On the other hand, our approaches satisfy all Pawlak’s exact criteria for rough sets in general case without any restrictions. The following example shows these facts.

Example 8. 

(Continued with Example 3), we attained:

$$\begin{array}{c}{𝓃}_{𝓇}(ℊ)=\left\{ℊ,𝓂\right\}\\ {𝓃}_{𝓇}\left(𝓀\right)=\{ℊ\}\\ {𝓃}_{𝓇}(\ell )=\left\{𝓀\right\}\\ {𝓃}_{𝓇}\left(𝓂\right)=\Phi \end{array}\}\Rightarrow \{\begin{array}{c}{𝓃}_{⋏}(ℊ)=\{ℊ\}\\ {𝓃}_{⋏}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{⋏}(\ell )=\Phi \\ {𝓃}_{⋏}\left(𝓂\right)=\left\{ℊ,𝓂\right\}\end{array},\{\begin{array}{c}{𝓃}_{⋎}(ℊ)=\left\{ℊ,𝓂\right\}\\ {𝓃}_{⋎}\left(𝓀\right)=\left\{𝓀\right\}\\ {𝓃}_{⋎}(\ell )=\Phi \\ {𝓃}_{⋎}\left(𝓂\right)=\left\{ℊ,𝓂\right\}\end{array},\mathrm{and}\{\begin{array}{c}{𝓃}_{\mathfrak{b}}(ℊ)=\left\{ℊ,𝓀,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}\left(𝓀\right)=\left\{𝓀,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}(\ell )=\left\{\ell ,𝓂\right\}\\ {𝓃}_{\mathfrak{b}}\left(𝓂\right)=\left\{𝓂\right\}\end{array}$$

Thus, we shall compute the approximations for all subsets of $𝒮$ using the suggested method (in Definition 13) and Allam et al.’s method (in Definition 4) as shown in

Table 1. Comparison between Yao [18], Allam [19], Dai [15] approaches and the suggested method in the general case.

$ℳ$	Yao’s Method		Allam’s Method		Dai’s Method		Current Method
	${\underset{\_}{\mathbf{ℒ}}}_{\mathit{r}}(\mathbf{ℳ})$	${\overline{\mathit{𝒰}}}_{\mathit{r}}(\mathbf{ℳ})$	${\underset{\_}{\mathbf{ℒ}}}_{⋏}(\mathbf{ℳ})$	${\overline{\mathit{𝒰}}}_{⋏}(\mathbf{ℳ})$	${\underset{\_}{\mathbf{ℒ}}}_{⋎}(\mathbf{ℳ})$	${\overline{\mathit{𝒰}}}_{⋎}(\mathbf{ℳ})$	${\underset{\_}{\mathbf{ℒ}}}_{\mathfrak{b}}(\mathbf{ℳ})$	${\overline{\mathit{𝒰}}}_{\mathfrak{b}}(\mathbf{ℳ})$
$\{\mathbf{ℊ}\}$	$\left\{𝓀,𝓂\right\}$	$\left\{ℊ,𝓀\right\}$	$\left\{ℊ,\ell \right\}$	$\left\{ℊ,𝓂\right\}$	$\{\ell \}$	$\left\{ℊ,𝓂\right\}$	$\Phi$	$\{ℊ\}$
$\left\{\mathit{𝓀}\right\}$	$\left\{\ell ,𝓂\right\}$	$\{\ell \}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀\right\}$	$\Phi$	$\left\{ℊ,𝓀\right\}$
$\{\mathbf{\ell }\}$	$\left\{𝓂\right\}$	$\Phi$	$\{\ell \}$	$\Phi$	$\{\ell \}$	$\Phi$	$\Phi$	$\{\ell \}$
$\left\{𝓂\right\}$	$\left\{𝓂\right\}$	$\{ℊ\}$	$\{\ell \}$	$\left\{𝓂\right\}$	$\{\ell \}$	$\left\{ℊ,𝓂\right\}$	$\left\{𝓂\right\}$	$𝒮$
$\left\{ℊ,𝓀\right\}$	$\left\{𝓀,\ell ,𝓂\right\}$	$\left\{ℊ,𝓀,\ell \right\}$	$\left\{ℊ,𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\Phi$	$\left\{ℊ,𝓀\right\}$
$\left\{ℊ,\ell \right\}$	$\left\{𝓀,𝓂\right\}$	$\left\{ℊ,𝓀\right\}$	$\left\{ℊ,\ell \right\}$	$\left\{ℊ,𝓂\right\}$	$\{\ell \}$	$\left\{ℊ,𝓂\right\}$	$\Phi$	$\left\{ℊ,\ell \right\}$
$\left\{ℊ,𝓂\right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{ℊ,𝓀\right\}$	$\left\{ℊ,\ell ,𝓂\right\}$	$\left\{ℊ,𝓂\right\}$	$\left\{ℊ,\ell ,𝓂\right\}$	$\left\{ℊ,𝓂\right\}$	$\left\{𝓂\right\}$	$𝒮$
$\left\{𝓀,\ell \right\}$	$\left\{\ell ,𝓂\right\}$	$\{\ell \}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀\right\}$	$\Phi$	$\left\{ℊ,𝓀,\ell \right\}$
$\left\{𝓀,𝓂\right\}$	$\left\{\ell ,𝓂\right\}$	$\left\{ℊ,\ell \right\}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀,𝓂\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{𝓀,𝓂\right\}$	$𝒮$
$\left\{\ell ,𝓂\right\}$	$\left\{𝓂\right\}$	$\{ℊ\}$	$\{\ell \}$	$\left\{𝓂\right\}$	$\{\ell \}$	$\left\{ℊ,𝓂\right\}$	$\left\{\ell ,𝓂\right\}$	$𝒮$
$\left\{ℊ,𝓀,\ell \right\}$	$\left\{𝓀,\ell ,𝓂\right\}$	$\left\{ℊ,𝓀,\ell \right\}$	$\left\{ℊ,𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\Phi$	$\left\{ℊ,𝓀,\ell \right\}$
$\left\{ℊ,𝓀,𝓂\right\}$	$𝒮$	$\left\{ℊ,𝓀,\ell \right\}$	$𝒮$	$\left\{ℊ,𝓀,𝓂\right\}$	$𝒮$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$𝒮$
$\left\{ℊ,\ell ,𝓂\right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{ℊ,𝓀\right\}$	$\left\{ℊ,\ell ,𝓂\right\}$	$\left\{ℊ,𝓂\right\}$	$\left\{ℊ,\ell ,𝓂\right\}$	$\left\{ℊ,𝓂\right\}$	$\left\{\ell ,𝓂\right\}$	$𝒮$
$\left\{𝓀,\ell ,𝓂\right\}$	$\left\{\ell ,𝓂\right\}$	$\left\{ℊ,\ell \right\}$	$\left\{𝓀,\ell \right\}$	$\left\{𝓀,𝓂\right\}$	$\left\{𝓀,\ell \right\}$	$\left\{ℊ,𝓀,𝓂\right\}$	$\left\{𝓀,\ell ,𝓂\right\}$	$𝒮$
$𝒮$	$𝒮$	$\left\{ℊ,𝓀,\ell \right\}$	$𝒮$	$\left\{ℊ,𝓀,𝓂\right\}$	$𝒮$	$\left\{ℊ,𝓀,𝓂\right\}$	$𝒮$	$𝒮$
$\Phi$	$\left\{𝓂\right\}$	$\Phi$	$\{\ell \}$	$\Phi$	$\{\ell \}$	$\Phi$	$\Phi$	$\Phi$

.

Remark 9. 

From Table 1, we can notice the following:

(i) 

The Yao, Allam, and Dai methods are not suitable to approximate the rough sets in the general case, since they could not be applied for any relation (since the main properties of the approximations did not hold), and thus these methods restrict the applications of rough set theory, for instance:

a.

${\underset{\_}{ℒ}}_k(ℳ)⊈ℳ⊈{\overline{𝒰}}_k(ℳ)$,$\forall ℳ\subseteq 𝒮$,$k\in \left\{𝓇,⋏,⋎\right\}$.

b.

${\overline{𝒰}}_k\left(𝒮\right)\ne 𝒮$and${\underset{\_}{ℒ}}_k\left(\Phi \right)\ne \Phi$.

For example, see the highlighted cells in Table 1. Accordingly, these methods make some contradictions to the rough set theory. Further, all subsets were rough according to these methods and this represents vagueness for data;

(ii) 

On the other hand, our methods in the present paper were the best methods for approximating the sets in the general case, since these approximations satisfied all properties of Pawlak’s rough sets without any conditions or restrictions. Therefore, the suggested method can help in discovering the vagueness in the data.

Case (2): Special cases of a binary relation.

Now, the different approximations (Yao [18], Allam [19], Dai [15], and current approaches) are independent. The following theorem demonstrates this fact.

Theorem 6. 

If$ℛ$is a reflexive relation on$𝒮$, then for each$ℳ\subseteq 𝒮$:

(a) 

${\underset{\_}{ℒ}}_{⋎}(ℳ)\subseteq {\underset{\_}{ℒ}}_{𝓇}(ℳ)\subseteq {\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)\subseteq ℳ\subseteq {\overline{𝒰}}_{\mathfrak{b}}(ℳ)\subseteq {\overline{𝒰}}_{𝓇}(ℳ)\subseteq {\overline{𝒰}}_{⋎}(ℳ)$;

(b) 

${\underset{\_}{ℒ}}_{⋎}(ℳ)\subseteq {\underset{\_}{ℒ}}_{𝓇}(ℳ)\subseteq {\underset{\_}{ℒ}}_{⋏}(ℳ)\subseteq ℳ\subseteq {\overline{𝒰}}_{⋏}(ℳ)\subseteq {\overline{𝒰}}_{𝓇}(ℳ)\subseteq {\overline{𝒰}}_{⋎}(ℳ)$.

Proof. 

According to Lemma 2, the proof is obvious. □

Corollary 3. 

If$ℛ$is a reflexive relation on$𝒮$, then for each$ℳ\subseteq 𝒮$:

(a) 

$ℬn{d}_{\mathfrak{b}}(ℳ)\subseteq ℬn{d}_{𝓇}(ℳ)\subseteq ℬn{d}_{⋎}(ℳ)$;

(b) 

$ℬn{d}_{⋏}(ℳ)\subseteq ℬn{d}_{𝓇}(ℳ)\subseteq ℬn{d}_{⋎}(ℳ)$;

(c) 

${\Theta }_{⋎}(ℳ)\le {\Theta }_{𝓇}(ℳ)\le {\Theta }_{\mathfrak{b}}(ℳ)$;

(d) 

${\Theta }_{⋎}(ℳ)\le {\Theta }_{𝓇}(ℳ)\le {\Theta }_{⋏}(ℳ)$;

(e) 

If$ℳ$is$⋎$-exact$\Rightarrow ℳ$is$𝓇$-exact$\Rightarrow ℳ$is$\mathfrak{b}$-exact.

If$ℳ$is$⋎$-exact$\Rightarrow ℳ$is$𝓇$-exact$\Rightarrow ℳ$is$⋏$-exact.

Note that: The opposite of the previous results is not true in general as shown in the next example.

Example 9. 

Consider Example 5 if$ℳ=\left\{𝓂\right\}$. Then,${\underset{\_}{ℒ}}_{⋎}(ℳ)=\Phi$and${\overline{𝒰}}_{⋎}(ℳ)=\left\{𝓀,\ell ,𝓂\right\}$which implies$ℬn{d}_{⋎}(ℳ)=\left\{𝓀,\ell ,𝓂\right\}$and${\Theta }_{⋎}(ℳ)=0$. However,${\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)={\overline{𝒰}}_{\mathfrak{b}}(ℳ)=ℳ$which tends to$ℬn{d}_{\mathfrak{b}}(ℳ)=\Phi$and${\Theta }_{\mathfrak{b}}(ℳ)$. Therefore,${\underset{\_}{ℒ}}_{⋎}(ℳ)⊊{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$,${\overline{𝒰}}_{\mathfrak{b}}(ℳ)⊊{\overline{𝒰}}_{⋎}(ℳ)$,$ℬn{d}_{\mathfrak{b}}(ℳ)⊊ℬ𝓃d_{⋎}(ℳ)$, and${\Theta }_{⋎}(ℳ)<{\Theta }_{\mathfrak{b}}(ℳ)$. In addition,$ℳ$is$\mathfrak{b}$-exact although it is a$⋎$-rough set.

According to Lemma 4, we can easily prove the following theorem. Thus, the proof was omitted.

Theorem 7. 

If$ℛ$is a preorder relation on$𝒮$, then for each$ℳ\subseteq 𝒮$:

(a) 

${\underset{\_}{ℒ}}_{⋎}(ℳ)\subseteq {\underset{\_}{ℒ}}_{𝓇}(ℳ)\mathrm{=}{\underset{\_}{ℒ}}_{⋏}(ℳ)\mathrm{=}{\underset{\_}{ℒ}}_{\mathfrak{b}}(ℳ)$and

${\overline{𝒰}}_{𝓇}(ℳ)={\overline{𝒰}}_{⋏}(ℳ)={\overline{𝒰}}_{\mathfrak{b}}(ℳ)\subseteq {\overline{𝒰}}_{⋎}(ℳ)$;

(b) 

$ℬn{d}_{𝓇}(ℳ)=ℬn{d}_{⋏}(ℳ)=ℬn{d}_{\mathfrak{b}}(ℳ)\subseteq ℬn{d}_{⋎}(ℳ)$and

${\Theta }_{⋎}(ℳ)\le {\Theta }_{𝓇}(ℳ)={\Theta }_{⋏}(ℳ)={\Theta }_{\mathfrak{b}}(ℳ)$.

Figure 1 summarizes the relationships between the suggested approach and the other methods (namely, Yao, Dai, and Allam) in the case of the reflexivity of the relation (where each arrow represents $\subseteq$).

The core objective of the next result is to prove that our methods represent a generalization to Marei’s approaches.

Theorem 8. 

If$\left\{{ℛ}_{𝒾}:𝒾=1,2,3,\dots ,n\right\}$is a family of reflexive relations on$𝒮$, then, for each$ℳ\subseteq 𝒮$:

(i) 

${}_k{}^n\underset{\_}{ℝ}(ℳ)\subseteq {}_k{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq ℳ\subseteq {}_k{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_k{}^n\overline{ℝ}(ℳ)$, for each$k\in \{1,2\}$;

(ii) 

${}_k{}^nℬn{d}_{\mathfrak{b}}(ℳ)\subseteq {}_k{}^nℬnd(ℳ)$and${}_k{}^n\Theta (ℳ)\le {}_k{}^n\Theta {}_{\mathfrak{b}}(ℳ)$, for each$k\in \{1,2\}$;

(iii) 

If$ℳ$is$n$-exact, then it is$𝓃$-basic exact.

Proof. 

We shall verify (i) only in the case of $k=1$, and the others similarly.

Let $𝓍\in {}_1{}^n\underset{\_}{ℝ}(ℳ)$, then $\left({{\displaystyle \cap }}_{i=1}^n𝓍{ℛ}_i\right)\subseteq ℳ$. However, by using Lemma 2, ${𝓃}_{\mathfrak{b}}\left(𝓍\right)\subseteq 𝓍ℛ$, for each $𝓍\in 𝒮$. Hence, $\left({{\displaystyle \cap }}_{i=1}^n{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)\right)\subseteq \left({{\displaystyle \cap }}_{i=1}^n𝓍{ℛ}_i\right)\subseteq ℳ$ and then $𝓍\in {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$. Therefore, ${}_1{}^n\underset{\_}{ℝ}(ℳ)\subseteq {}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$.

Similarly, we can prove that: ${}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq {}_1{}^n\overline{ℝ}(ℳ)$. □

Note that: The next example demonstrated that the inverse of Theorem 8 was not correct generally.

Example 10. 

Suppose that${ℛ}_1=\left\{\left(𝓍,𝓍\right),\left(𝓎,𝓎\right),\left(𝓎,𝓏\right),\left(𝓏,𝓍\right),\left(𝓏,𝓏\right)\right\}$and${ℛ}_2=\{\left(\mathrm{x;},𝓍\right),\left(𝓍,𝓎\right),\left(𝓎,𝓎\right),$ $\left(𝓎,𝓏\right),\left(𝓏,𝓎\right),\left(𝓏,𝓏\right)\}$are two reflexive relations on$𝒮=\{𝓍,𝓎,𝓏\}$. Hence, we attain the following:

$$\begin{array}{c}\underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓍{ℛ}_{𝒾}=\left\{𝓍\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓎{ℛ}_{𝒾}=\left\{𝓎,𝓏\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓏{ℛ}_{𝒾}=\left\{𝓏\right\}.\\ \end{array}\}and\{\begin{array}{c}\underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)=\left\{𝓍\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓎\right)=\left\{𝓎\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓏\right)=\left\{𝓏\right\}.\\ \end{array}$$

Now, let$ℳ=\left\{𝓍,𝓎\right\}$. Thus, we obtain:

According to Marei’s approach,${}_1{}^2\underset{\_}{ℝ}(ℳ)=\left\{𝓍\right\}$and${}_1{}^2\overline{ℝ}(ℳ)=\left\{𝓍,𝓎\right\}$, i.e.,$ℳ$is rough.

According to our approach (Definition 14 (i)),${}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)={}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)=ℳ$, i.e.,$ℳ$is an exact set.

Furthermore, it is clear that${}_1{}^n\underset{\_}{ℝ}(ℳ)⊊{}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)$and${}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)⊊{}_1{}^n\overline{ℝ}(ℳ)$.

Note that: We present the following example to show that the relationship between Marei’s techniques and our approaches in the general case. Besides, this example illustrates that the suggested methods improve and solve the errors in the last methods (Marei’s methods).

Example 11. 

Suppose that${ℛ}_1=\left\{\left(𝓍,𝓎\right),\left(𝓎,𝓏\right)\right\}$and${ℛ}_2=\{\left(𝓍,𝓏\right),$ $\left(𝓎,𝓏\right)\}$are two binary relations on$𝒮=\{𝓍,𝓎,𝓏\}$. Hence, we attain the following:

$$\begin{array}{c}\underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓍{ℛ}_{𝒾}=\Phi .\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓎{ℛ}_{𝒾}=\left\{𝓏\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}𝓏{ℛ}_{𝒾}=\Phi .\\ \end{array}\}and\{\begin{array}{c}\underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓍\right)=\left\{𝓍,𝓏\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓎\right)=\left\{𝓎,𝓏\right\}.\\ \underset{𝒾=1}{\overset{2}{{\displaystyle \cap }}}{𝓃}_{\mathfrak{b}}{}_{𝒾}\left(𝓏\right)=\left\{𝓏\right\}.\\ \end{array}$$

Now, let$ℳ=\left\{𝓏\right\}$. Thus, we obtain:

According to Marei’s approach,${}_1{}^2\underset{\_}{ℝ}(ℳ)=\left\{𝓍,𝓎,𝓏\right\}=𝒮⊈ℳ$and${}_1{}^2\overline{ℝ}(ℳ)=\left\{𝓎\right\}⊉ℳ$; i.e.,$ℳ$is rough. Moreover,${}_1{}^2\underset{\_}{ℝ}\left(\Phi \right)=\left\{𝓍,𝓏\right\}\ne \Phi$and${}_1{}^2\overline{ℝ}\left(𝒮\right)=\left\{𝓎\right\}\ne 𝒮$which is a contradiction to the main properties of Pawlak’s rough methodology.

According to our approach (Definition 14 (i)),${}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)={}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ)=ℳ$, i.e.,$ℳ$is an exact set. Moreover,${}_1{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}\left(\Phi \right)={}_1{}^2\overline{ℝ}{}_{\mathfrak{b}}\left(\Phi \right)=\Phi$and

${}_1{}^2\underset{\_}{ℝ}{}_{\mathfrak{b}}\left(𝒮\right)={}_1{}^2\overline{ℝ}{}_{\mathfrak{b}}\left(𝒮\right)=𝒮$. Besides, for each$ℳ\subseteq 𝒮$,

$${}_1{}^n\underset{\_}{ℝ}{}_{\mathfrak{b}}(ℳ)\subseteq ℳ\subseteq {}_1{}^n\overline{ℝ}{}_{\mathfrak{b}}(ℳ).$$

5. Applications

Here, we present two real-life applications to illustrate the importance of the suggested techniques.

5.1. Set-Valued Information Systems

Set-valued information systems represent generalized models of single-valued information systems. Firstly, in this subsection, we provide some fundamental concepts of set-valued information systems.

Definition 15 

[10]. Suppose that $𝒮$ represents a finite set of objects, called the universe of discourse, $𝒜𝓉$ be a finite set of attributes such that, for each attribute $𝒶\in 𝒜𝓉$, associated with a set of its values ${𝒱}_{𝒶}$, and the map $ℱ:𝒮\times 𝒜𝓉\to 𝒱$ is a total function such that $ℱ\left(𝓍,𝒶\right)\subseteq {𝒱}_{𝒶}$ for every $𝒶\in 𝒜𝓉$, $𝓍\in 𝒮$, and $𝒱={{\displaystyle \cup }}_{𝒶\in 𝒜𝓉}{𝒱}_{𝒶}$ called an information function. The quadruple $\left(𝒮,𝒜𝓉,𝒱,ℱ\right)$ is called a single-valued information system if each attribute has a unique attribute value; if a system is not a single-valued information system, it is called a multi-valued information system (or set-valued information system).

Note that: If the set of attributes $𝒜𝓉$ is divided into condition $𝒞$ and decision $𝒹$ attributes, then this information system is called a set-valued decision information system and denoted by $\mathbb{D}\mathbb{I}\mathbb{S}=\left(𝒮,𝒞{\displaystyle \cup }\left\{𝒹\right\},𝒱,ℱ\right)$, where $𝒜𝓉=𝒞{\displaystyle \cup }\left\{𝒹\right\}$, $𝒞$ is a finite set of condition attributes, and $𝒹$ is a decision attribute with $𝒞{\displaystyle \cap }𝒹=\Phi$. If the domain (scale) of a condition attribute is ordered according to a decreasing or increasing preference, then the attribute is a criterion.

Definition 16 

[10]. In any set-valued information system $\left(𝒮,𝒜𝓉,𝒱,ℱ\right)$, if the domain of a condition attribute is ordered according to a decreasing or increasing preference, then the attribute is a criterion. If every condition attribute is a criterion, then it is said to be a set-valued ordered information system.

Definition 17 

[10]. If the values of some objects in $𝒮$ under a condition attribute can be ordered according to an inclusion increasing or decreasing preference, then the attribute is an inclusion criterion.

5.2. Decision-Making of Multi-Information System of Nutrition Modeling via B-Rough Approximations

Each nutrient performs one or more of the general functions listed below. Heat, energy, and power are provided by carbohydrates and fats. Proteins, minerals, and vitamins help to build and promote growth, as well as renew and regulate body tissues and processes. For practical purposes, the recommended daily dietary allowances are divided into the following basic food groups, which are represented by a table.

Consider

Table 2. Multi-information system of eight adolescents’ eating habits.

Students	$\mathbf{Group}\mathbf{I}\left({\mathbf{A}}_1\right)$	Group II (A2)	Group III (A3)	Group IV (A4)	Group V (A5)	Decision (D)
${ℊ}_1$	$\left\{𝒱,ℳ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒫\right\}$	$\left\{𝒞,ℳ\right\}$	$\left\{𝒫,ℱ\right\}$	Unhealthy
${ℊ}_2$	$\left\{𝒞,𝒱,ℳ\right\}$	$\left\{𝒞,𝒫\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒞,𝒫,ℳ\right\}$	$\left\{𝒫,ℱ\right\}$	Healthy
${ℊ}_3$	$\left\{𝒞,ℳ\right\}$	$\left\{𝒞,𝒫,ℱ\right\}$	$\left\{ℱ\right\}$	$\left\{𝒞,𝒫,ℳ\right\}$	$\{ℱ\}$	Healthy
${ℊ}_4$	$\left\{𝒞,𝒱,ℳ\right\}$	$\left\{𝒞,ℱ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒫,ℳ\right\}$	$\left\{𝒫,ℱ\right\}$	Unhealthy
${ℊ}_5$	$\left\{𝒞,𝒱\right\}$	$\left\{𝒞,𝒫,ℱ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒞,ℳ\right\}$	$\left\{𝒫,ℱ\right\}$	Healthy
${ℊ}_6$	$\left\{𝒱,ℳ\right\}$	$\left\{𝒞,𝒫,ℱ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒞,𝒫,ℳ\right\}$	$\left\{ℱ\right\}$	Healthy
${ℊ}_7$	$\left\{𝒱,ℳ\right\}$	$\left\{𝒞,ℱ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒞,𝒫\right\}$	$\left\{𝒫\right\}$	Unhealthy
${ℊ}_8$	$\left\{𝒱,ℳ\right\}$	$\left\{𝒞,𝒫,ℱ\right\}$	$\left\{𝒫,ℱ\right\}$	$\left\{𝒞,𝒫,ℳ\right\}$	$\left\{𝒫,ℳ\right\}$	Healthy

, which contains information about eight adolescents’ eating habits.

Accordingly, Table 2 represents a set valued ordered information system, where $𝒮=\left\{{ℊ}_1,{ℊ}_2,{ℊ}_3,\dots ,{ℊ}_8\right\}$ is a finite set of students and $𝒜𝓉=\left\{{\mathrm{A}}_1,{\mathrm{A}}_2,{\mathrm{A}}_3,{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$ is a finite set of attributes of the basic food groups and D is the decision attribute. The set of attribute values is given by ${𝒱}_{𝒜𝓉}=\{𝒞,𝒫,ℱ,𝒱,ℳ\}$, where $𝒞,𝒫,ℱ,𝒱$ and $ℳ$ interpret, respectively, as carbohydrate, protein, fat, vitamins, and minerals.

From Table 2: $f\left({ℊ}_1,{\mathrm{A}}_1\right)=\left\{𝒱,ℳ\right\}$ and $f\left({ℊ}_2,{\mathrm{A}}_1\right)=\left\{𝒞,𝒱,ℳ\right\}$. Therefore, we attain $f\left({ℊ}_1,{\mathrm{A}}_1\right)⊊f\left({ℊ}_2,{\mathrm{A}}_1\right)$ and hence, the intake of fruits and vegetables by ${ℊ}_2$ is much better than that by ${ℊ}_1$.

Thus, Table 2 represents a multi-valued information system with general binary relations:

$${ℊ}_{𝒾}{ℛ}_{{\mathrm{A}}_k}{ℊ}_{𝒿}\iff f\left({ℊ}_{𝒾},{\mathrm{A}}_k\right)⊊f\left({ℊ}_{𝒾},{\mathrm{A}}_{𝓀}\right),\mathrm{where}𝒾,𝒿\in \left\{1,2,3,\dots ,8\right\}\&k=1,2,3,4,5.$$

The above relations are transitive. Thus, Pawlak’s approach and some of its generalizations (such as Allam, Yao, Dai, and Marei methods) did not apply here and hence they could not deal with this problem, as illustrated in the following application.

Therefore, we use the approximations in Definition 14 to make an accurate decision for the nutrition system in Table 2.

Accordingly,

Table 3. Right neighborhoods of Table 2.

Students	${\mathit{g}}_{\mathit{i}}{\mathbf{ℛ}}_{{\mathbf{A}}_1}$	${\mathit{g}}_{\mathit{i}}{\mathbf{ℛ}}_{{\mathbf{A}}_2}$	${\mathit{g}}_{\mathit{i}}{\mathbf{ℛ}}_{{\mathbf{A}}_3}$	${\mathit{g}}_{\mathit{i}}{\mathbf{ℛ}}_{{\mathbf{A}}_4}$	${\mathit{g}}_{\mathit{i}}{\mathbf{ℛ}}_{{\mathbf{A}}_5}$
${ℊ}_1$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\left\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$	$\left\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\right\}$	$\left\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$
${ℊ}_2$	$\Phi$	$\left\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$	$\Phi$	$\Phi$
${ℊ}_3$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\Phi$	$\left\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\right\}$	$\Phi$	$\left\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\right\}$
${ℊ}_4$	$\Phi$	$\left\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$	$\left\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$
${ℊ}_5$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\Phi$	$\Phi$	$\left\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$
${ℊ}_6$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\Phi$	$\Phi$	$\Phi$	$\left\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\right\}$
${ℊ}_7$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\left\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$	$\Phi$	$\left\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\right\}$	$\left\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\right\}$
${ℊ}_8$	$\left\{{ℊ}_2,{ℊ}_4\right\}$	$\Phi$	$\Phi$	$\Phi$	$\Phi$

and

Table 4. $\mathfrak{b}$-neighborhoods of Table 2.

Students	$${\mathit{n}}_{\mathfrak{b}}{}_{{\mathbf{A}}_1}\left({\mathbf{ℊ}}_{\mathit{i}}\right)$$	$${\mathit{n}}_{\mathfrak{b}}{}_{{\mathbf{A}}_2}\left({\mathbf{ℊ}}_{\mathit{i}}\right)$$	$${\mathit{n}}_{\mathfrak{b}}{}_{{\mathbf{A}}_3}\left({\mathbf{ℊ}}_{\mathit{i}}\right)$$	$${\mathit{n}}_{\mathfrak{b}}{}_{{\mathbf{A}}_4}\left({\mathbf{ℊ}}_{\mathit{i}}\right)$$	$${\mathit{n}}_{\mathfrak{b}}{}_{{\mathbf{A}}_5}\left({\mathbf{ℊ}}_{\mathit{i}}\right)$$
${ℊ}_1$	$𝒮$	$𝒮$	$𝒮$	$𝒮$	$\left\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\right\}$
${ℊ}_2$	$\{{ℊ}_2,{ℊ}_4\}$	$𝒮$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\}$	$\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\}$
${ℊ}_3$	$𝒮$	$\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\}$	$𝒮$	$\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\}$	$𝒮$
${ℊ}_4$	$\{{ℊ}_2,{ℊ}_4\}$	$𝒮$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$𝒮$	$\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\}$
${ℊ}_5$	$𝒮$	$\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\}$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$𝒮$	$\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\}$
${ℊ}_6$	$𝒮$	$\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\}$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$𝒮$	$𝒮$
${ℊ}_7$	$𝒮$	$𝒮$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$𝒮$	$𝒮$
${ℊ}_8$	$𝒮$	$\{{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\}$	$\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}$	$\{{ℊ}_2,{ℊ}_3,{ℊ}_6,{ℊ}_8\}$	$\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\}$

provide the right and $\mathfrak{b}$-neighborhoods of Table 2. Thus, by using Definition 14 we attain:

$$\begin{array}{c}\underset{k=1}{\overset{5}{\cap }}{ℊ}_1{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_2{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_3{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_4{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_5{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_6{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_7{ℛ}_{{\mathrm{A}}_k}=\Phi .\\ \underset{k=1}{\overset{5}{\cap }}{ℊ}_8{ℛ}_{{\mathrm{A}}_k}=\Phi .\end{array}\}𝒶𝓃d\begin{array}{c}\underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_1\right)=\{{ℊ}_1,{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_8\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_2\right)=\left\{{ℊ}_2\right\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_3\right)=\{{ℊ}_3,{ℊ}_6,{ℊ}_8\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_4\right)=\{{ℊ}_2,{ℊ}_4\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_5\right)=\{{ℊ}_5,{ℊ}_8\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_6\right)=\{{ℊ}_5,{ℊ}_6,{ℊ}_8\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_7\right)=\{{ℊ}_2,{ℊ}_4,{ℊ}_5,{ℊ}_6,{ℊ}_7,{ℊ}_8\}.\\ \underset{k=1}{\overset{5}{\cap }}{𝓃}_{{\mathfrak{b}}_k}\left({ℊ}_8\right)=\left\{{ℊ}_8\right\}.\end{array}$$

We computed the approximations, the boundary region, and the accuracy of the approximations of only two decision sets (healthy set and unhealthy set) using the proposed method in Definition 14 and the other previous methods. Thus, we explain the importance of the suggested techniques in approximating the sets for helping in decision-making. From Table 2: The set of healthy food was $\mathscr{H}=\left\{{ℊ}_2,{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$.

According to Marei’s approach:

${}_1{}^5\underset{\_}{ℝ}(\mathscr{H})=𝒮$ and ${}_1{}^5\overline{ℝ}(\mathscr{H})=\Phi$. This means that $\mathscr{H}$ is totally rough set according to Marei’s technique. Therefore, we were unable to decide whether the healthy or unhealthy food. On the other hand, there were some contradictions to Pawlak’s axioms [1], namely ${}_1{}^5\underset{\_}{ℝ}(\mathscr{H})⊈\mathscr{H}⊈{}_1{}^5\overline{ℝ}(\mathscr{H})$.

According to our approach (Definition 14 (i)):

${}_1{}^5\underset{\_}{ℝ}{}_{\mathfrak{b}}(\mathscr{H})=\mathscr{H}$ and ${}_1{}^5\overline{ℝ}{}_{\mathfrak{b}}(\mathscr{H})=𝒮$. This means that the set $\left\{{ℊ}_2,{ℊ}_3,{ℊ}_5,{ℊ}_6,{ℊ}_8\right\}$ only represented the healthy food, which coincided with the decision system Table 2. Moreover, ${}_1{}^5\underset{\_}{ℝ}{}_{\mathfrak{b}}(\mathscr{H})\subseteq \mathscr{H}\subseteq {}_1{}^5\overline{ℝ}{}_{\mathfrak{b}}(\mathscr{H})$.

Observation: From the above comparisons, we noticed the following:

(1)

We could not use Pawlak’s rough set model in the previous application because of its transitivity as we applied Pawlak’s rough set model only in the case of equivalence relation. Moreover, these methods were applied easily without restrictions, so this may expand the field of the application of Pawlak’s rough sets;

(2)

Using Marei’s methods was not suitable for obtaining an accurate decision, since they produced some contradictions and vagueness. Consequently, we were unable to decide the suitable healthy food;

(3)

On the other hand, by using the suggested approximations, we confirmed between the experimental data and its mathematical analysis. The mathematical study depends on the classification of data by using the $\mathfrak{b}$-neighborhoods. Hence, we minimized the vagueness in the data and also obtained a higher accuracy measure. Accordingly, we can say that the suggested approximations were more accurate than the previous methods for extracting the information and helping to eliminate the ambiguity of the data in the real-life problems, especially in the medical diagnosis which needed accurate decisions.

5.3. A Medical Application in Decision-Making of the Heart Attacks Problem

Since medical diagnosis always needs accurate tools to make decisions, we then applied the proposed methods in decision making to heart attacks. The data set in

Table 5. The information’s decisions data set [38].

$\mathfrak{U}$	${\mathbf{A}}_1$	${\mathbf{A}}_2$	${\mathbf{A}}_3$	${\mathbf{A}}_4$	${\mathbf{A}}_5$	$\mathbf{D}$
${𝓅}_1$	yes	yes	yes	yes	no	yes
${𝓅}_2$	no	no	no	yes	yes	no
${𝓅}_3$	yes	yes	yes	yes	yes	yes
${𝓅}_4$	no	no	no	yes	no	no
${𝓅}_5$	yes	no	no	yes	yes	no
${𝓅}_6$	no	no	no	yes	no	no
${𝓅}_7$	yes	yes	yes	yes	yes	yes
${𝓅}_8$	yes	yes	no	yes	yes	yes
${𝓅}_9$	yes	no	yes	yes	no	yes
${𝓅}_{10}$	no	no	no	yes	yes	no
${𝓅}_{11}$	yes	no	yes	yes	no	yes
${𝓅}_{12}$	yes	no	no	yes	yes	no

Here, “yes” interpreted the patient had symptoms and “no” interpreted the patient had no symptoms.

shows the outcomes of five symptoms for twelve patients. The research was carried out at Al-Azhar University’s cardiology department [38] (Hospital of Sayed Glal University-Cairo, Egypt). The study included twelve patients who presented to this hospital with different symptoms, as well as a detailed history, physical examination, full labs, a resting ECG, and a conventional echo assessment. In the end, the diagnosis of heart attacks was confirmed or ruled out. In other words, the columns represent the symptoms. Thus, the set of attributes was $𝒜𝓉=\left\{{\mathrm{A}}_1,{\mathrm{A}}_2,{\mathrm{A}}_3,{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$, where ${\mathrm{A}}_1$ represents the breathlessness, ${\mathrm{A}}_2$ represents the orthopnea, ${\mathrm{A}}_3$ represents the paroxysmal nocturnal dyspnea, ${\mathrm{A}}_4$ represents reduced exercise tolerance, and ${\mathrm{A}}_5$ represents ankle swelling. Attribute $\mathrm{D}$ is the decision of heart attacks. On the other hand, the rows in Table 5 represent the patients, where $\mathfrak{U}=\left\{{𝓅}_1,{𝓅}_2,\cdots ,{𝓅}_{12}\right\}$. In the present application, we used a general binary relation to illustrate the significance of the suggested technique in decision-making. Therefore, the other methods (such as Pawlak [1,2], Yao [18], Allam [19], and Dai [15]) could not be applied here and hence we could say that our technique extended the application field of rough sets. Accordingly, we demonstrated that the suggested tools were more accurate than the other methods.

Note that: The patients ${𝓅}_3$ and ${𝓅}_7$ had the same values, the patients ${𝓅}_9$ and ${𝓅}_{11}$ also had the same values, and the patients ${𝓅}_5$ and ${𝓅}_{12}$ had the same values. Thus, we omitted the patients ${𝓅}_7$, ${𝓅}_{11}$, and ${𝓅}_{12}$ and hence $\mathfrak{U}=\left\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_4,{𝓅}_5,{𝓅}_6,{𝓅}_8,{𝓅}_9,{𝓅}_{10}\right\}$.

To determine the symptoms of every patient, we defined a map $V:\mathfrak{U}\to 𝒫\left(\mathfrak{U}\right)$ such that a symptom belonged to $V\left({𝓅}_{𝒾}\right)$, $\forall 𝒾=1,2,\dots ,12$ if the patient ${𝓅}_{𝒾}$ had this symptom.

Therefore, from Table 5, we obtained the symptoms of every patient as follows: $V\left({𝓅}_1\right)=\left\{{\mathrm{A}}_1,{\mathrm{A}}_2,{\mathrm{A}}_3,{\mathrm{A}}_4\right\}$, $V\left({𝓅}_2\right)=V\left({𝓅}_{10}\right)=\left\{{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$, $V\left({𝓅}_3\right)=\left\{{\mathrm{A}}_1,{\mathrm{A}}_2,{\mathrm{A}}_3,{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$, $V\left({𝓅}_4\right)=V\left({𝓅}_6\right)=\left\{{\mathrm{A}}_4\right\}$, $V\left({𝓅}_5\right)=\left\{{\mathrm{A}}_1,{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$, $V\left({𝓅}_8\right)=\left\{{\mathrm{A}}_1,{\mathrm{A}}_2,{\mathrm{A}}_4,{\mathrm{A}}_5\right\}$, and $V\left({𝓅}_9\right)=\left\{{\mathrm{A}}_1,{\mathrm{A}}_3,{\mathrm{A}}_4\right\}$.

Thus, we could generate a binary relation between the patients depending on the map $V$ as follows: ${𝓅}_{𝒾}ℛ{𝓅}_{𝒿}\iff V\left({𝓅}_{𝒾}\right)⊊V\left({𝓅}_{𝒿}\right)$, for each $𝒾,𝒿\in \left\{1,2,\dots 12\right\}$.

Note that: The relation was identified according to the viewpoint of the system’s experts.

Thus, we attained the following:

• $ℛ=\{\left({𝓅}_1,{𝓅}_3\right),\left({𝓅}_2,{𝓅}_3\right),\left({𝓅}_2,{𝓅}_5\right),\left({𝓅}_2,{𝓅}_8\right),\left({𝓅}_4,{𝓅}_1\right),\left({𝓅}_4,{𝓅}_2\right),\left({𝓅}_4,{𝓅}_3\right),\left({𝓅}_4,{𝓅}_5\right),\left({𝓅}_4,{𝓅}_8\right),$
• $\left({𝓅}_4,{𝓅}_9\right),\left({𝓅}_4,{𝓅}_9\right),\left({𝓅}_4,{𝓅}_{10}\right),\left({𝓅}_5,{𝓅}_3\right),\left({𝓅}_5,{𝓅}_8\right),\left({𝓅}_6,{𝓅}_1\right),\left({𝓅}_6,{𝓅}_2\right),\left({𝓅}_6,{𝓅}_3\right),\left({𝓅}_6,{𝓅}_5\right),$
• $\left({𝓅}_6,{𝓅}_8\right),\left({𝓅}_6,{𝓅}_9\right),\left({𝓅}_6,{𝓅}_{10}\right),\left({𝓅}_8,{𝓅}_3\right),\left({𝓅}_9,{𝓅}_1\right),\left({𝓅}_9,{𝓅}_3\right),\left({𝓅}_{10},{𝓅}_3\right),\left({𝓅}_{10},{𝓅}_5\right),\left({𝓅}_{10},{𝓅}_8\right),\}$

Thus, the right neighborhoods of each element in $\mathfrak{U}$ of this relation were:

• ${𝓅}_1ℛ={𝓅}_8ℛ=\left\{{𝓅}_3\right\}$, ${𝓅}_2ℛ={𝓅}_{10}ℛ=\left\{{𝓅}_3,{𝓅}_5,{𝓅}_8\right\}$, ${𝓅}_3ℛ=\Phi$, ${𝓅}_4ℛ={𝓅}_6ℛ=\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_5,$ ${𝓅}_8,{𝓅}_9,{𝓅}_{10}\}$, ${𝓅}_5ℛ=\left\{{𝓅}_3,{𝓅}_8\right\}$, and ${𝓅}_9ℛ=\left\{{𝓅}_1,{𝓅}_3\right\}$.

Accordingly, the maximal-neighborhoods were:

• ${𝓃}_{⋎}\left({𝓅}_1\right)={𝓃}_{⋎}\left({𝓅}_2\right)={𝓃}_{⋎}\left({𝓅}_3\right)={𝓃}_{⋎}\left({𝓅}_5\right)={𝓃}_{⋎}\left({𝓅}_8\right)={𝓃}_{⋎}\left({𝓅}_9\right)={𝓃}_{⋎}\left({𝓅}_{10}\right)=\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_5,$
• ${𝓅}_8,{𝓅}_9,{𝓅}_{10}\}$, and ${𝓃}_{⋎}\left({𝓅}_4\right)={𝓃}_{⋎}\left({𝓅}_6\right)=\Phi$.

In addition, the $\mathfrak{b}$-neighborhoods were:

• ${𝓃}_{\mathfrak{b}}\left({𝓅}_1\right)={𝓃}_{\mathfrak{b}}\left({𝓅}_8\right)=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_8\right\}$, ${𝓃}_{\mathfrak{b}}\left({𝓅}_2\right)={𝓃}_{\mathfrak{b}}\left({𝓅}_{10}\right)=\left\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_5,{𝓅}_8,{𝓅}_{10}\right\}$, ${𝓃}_{\mathfrak{b}}\left({𝓅}_3\right)=\left\{{𝓅}_3\right\}$, ${𝓃}_{\mathfrak{b}}\left({𝓅}_4\right)={𝓃}_{\mathfrak{b}}\left({𝓅}_6\right)=\mathfrak{U}$, ${𝓃}_{\mathfrak{b}}\left({𝓅}_5\right)=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_5,{𝓅}_8\right\}$, and ${𝓃}_{\mathfrak{b}}\left({𝓅}_9\right)=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_5,{𝓅}_8,{𝓅}_9\right\}$.

Thus, Table 5 represents a decision system and thus the patients with confirmed heart attacks were surely $\mathscr{H}=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_8,{𝓅}_9\right\}$. Thus, we computed the approximations, the boundary, and the accuracy measure of $\mathscr{H}$ using the suggested method and the previous approach [15] to explain the significance of the suggested techniques in decision-making.

Dai et al.’s approach [15]:

By calculating, we obtained ${\underset{\_}{ℒ}}_{⋎}(\mathscr{H})=\left\{{𝓅}_4,{𝓅}_6\right\}\ne \mathscr{H}$, and ${\overline{𝒰}}_{⋎}(\mathscr{H})=\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_5,{𝓅}_8,$ ${𝓅}_9,{𝓅}_{10}\}$. This meant that the boundary region was $ℬn{d}_{⋎}(\mathscr{H})=\{{𝓅}_1,{𝓅}_2,{𝓅}_3,{𝓅}_5,{𝓅}_8,{𝓅}_9,{𝓅}_{10}\}$, and the accuracy measure was ${\Theta }_{⋎}(\mathscr{H})=2/7$, which meant that $\mathscr{H}$ was a rough set according to the Dai technique. Further, the patients ${𝓅}_4$ and ${𝓅}_6$ experienced heart attacks, which contradicted the decision system in Table 5. Therefore, we were unable to decide whether the patient was experiencing heart attacks.

Current approach:

By calculating, we obtained ${\underset{\_}{ℒ}}_{\mathfrak{b}}(\mathscr{H})=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_8\right\}$, and ${\overline{𝒰}}_{\mathfrak{b}}(\mathscr{H})=\mathfrak{U}$. This meant that the boundary region was $ℬn{d}_{\mathfrak{b}}(\mathscr{H})=\left\{{𝓅}_2,{𝓅}_4,{𝓅}_5,{𝓅}_6,{𝓅}_9,{𝓅}_{10}\right\}$ and the accuracy measure was ${\Theta }_{\mathfrak{b}}(\mathscr{H})=4/9=44.4\%$, which meant that the patients $\{{𝓅}_1,{𝓅}_3,{𝓅}_8\}$ surely experienced heart attacks according to the proposed technique. On the other hand, the patient ${𝓅}_9$ may may or may not have experienced heart attacks.

Concluding remark: From the above comparison, we noticed the following:

(1)

Pawlak’s rough set model cannot be used in the above application because the used relation was transitive. Pawlak’s rough set model was applied only when the relation was an equivalence relation. On the other hand, the suggested 664 methods were applied and hence, this extended the application fields of Pawlak’s rough sets;

(2)

Using Dai et al.’s methods was not suitable for obtaining an accurate decision, since the boundary region was $ℬn{d}_{⋎}(\mathscr{H})=\left\{{𝓅}_2,{𝓅}_4,{𝓅}_5,{𝓅}_6,{𝓅}_9,{𝓅}_{10}\right\}$, and the accuracy measure was ${\Theta }_{⋎}(\mathscr{H})=2/7$, which meant that all patients in $\mathfrak{U}$ may have been infected, although the infected patients were surely specified with the set $\mathscr{H}=\left\{{𝓅}_1,{𝓅}_3,{𝓅}_8,{𝓅}_9\right\}$. Consequently, we were unable to decide whether the patient was infected with heart attacks, and this produced vagueness in decision making regarding the medical diagnosis;

(3)

On the other hand, using the suggested approximations, we attained the boundary $ℬn{d}_{\mathfrak{b}}(\mathscr{H})=\left\{{𝓅}_2,{𝓅}_4,{𝓅}_5,{𝓅}_6,{𝓅}_9,{𝓅}_{10}\right\}$, and the accuracy measure was ${\Theta }_{\mathfrak{b}}(\mathscr{H})=\frac{4}{9}$. Hence, we minimized the vagueness in the data and also obtained a higher accuracy measure. Accordingly, we can say that the suggested approximations were more accurate than the previous methods for extracting the information and helping to eliminate the ambiguity of the data in real-life problems, especially in the medical diagnosis which needed accurate decisions.

6. Conclusions

The philosophy of rough sets is characteristically considered a creation based on the idea of an approximation space and the constructed lower and upper approximations of subsets of a universe. In the original rough set theory, the constraint of the equivalence relation has excessively restricted those energies against the application’s fields of this theory. Thus, in the present paper, we introduced two different methods to generalize this theory. These methods were based on a novel neighborhood that was induced from a general binary relation. The first method depended on one binary relation to define the approximations which were compared with the previous approaches. Theorems 1, 2, 6 and 7 and Corollary 3 proved that the suggested methods represented a generalization to Pawlak’s models and their generalizations. Furthermore, the generalization of some concepts which were presented by Pawlak and Lellis Thivagar, as well as some of their properties, can be investigated using the basic-rough sets. Since Lellis Thivagar introduced the concept of “nano-topology” based on Pawlak’s rough sets, which are basically dependent on an equivalence relation, their methods restricted the application fields of real-life problems. Thus, we extended the application fields of graph theory. Moreover, these results illustrate that our approaches were more accurate and stronger than the other methods, such as Yao [18], Allam [19], Dai [15], and Marei [31]. In the second method, we succeeded in presenting improvements to Marei’s methods. Consequently, the second method was to propose new methods for generalizing rough sets by a finite number of binary relations which were induced in multi-information systems, and hence we extended the application field of rough set theory in order to solve many problems of multi-attribute decision-making (MADM).

Finally, to illustrate the significance of the suggested methods, two different applications were investigated. First, we applied the proposed method in multi-information systems of nutrition modeling, and hence we succeeded in identifying the best feeding systems that were healthy. In medicine, the medical diagnosis always needs accurate tools to make the decision, so we applied the proposed methods in decision-making to the issue of heart attacks. We used a data set of five symptoms for twelve patients. The research was carried out at Al-Azhar University’s cardiology department [38] and hence, we proved that the suggested methods were more accurate than the other methods (namely, Yao [18], Allam [19], Dai [15], and Marei [31]). In fact, we set an establishment between the experimental data and its mathematical analysis.

Overall, this work supplies a readable framework to the respective areas with interesting applications such as COVID-19, MADM, and graph theory. Generalized rough sets based solely on binary relations may not support MADM, so in our future work, we will study the suggested approaches in MADM as a starting point for future research. Further, we will use the proposed methods in extending the theory of fuzzy soft sets.