Planar Typical Bézier Curves with a Single Curvature Extremum
Abstract
:1. Introduction
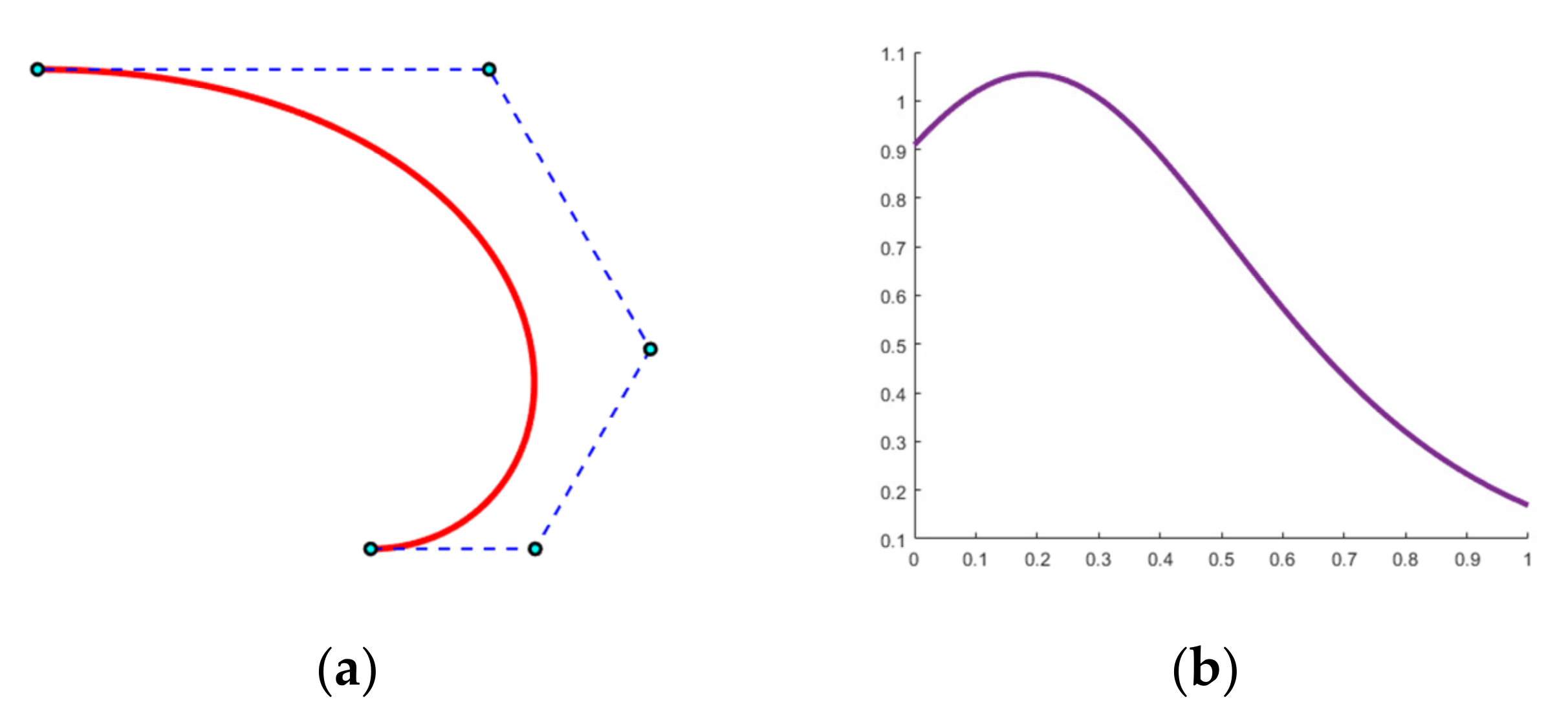
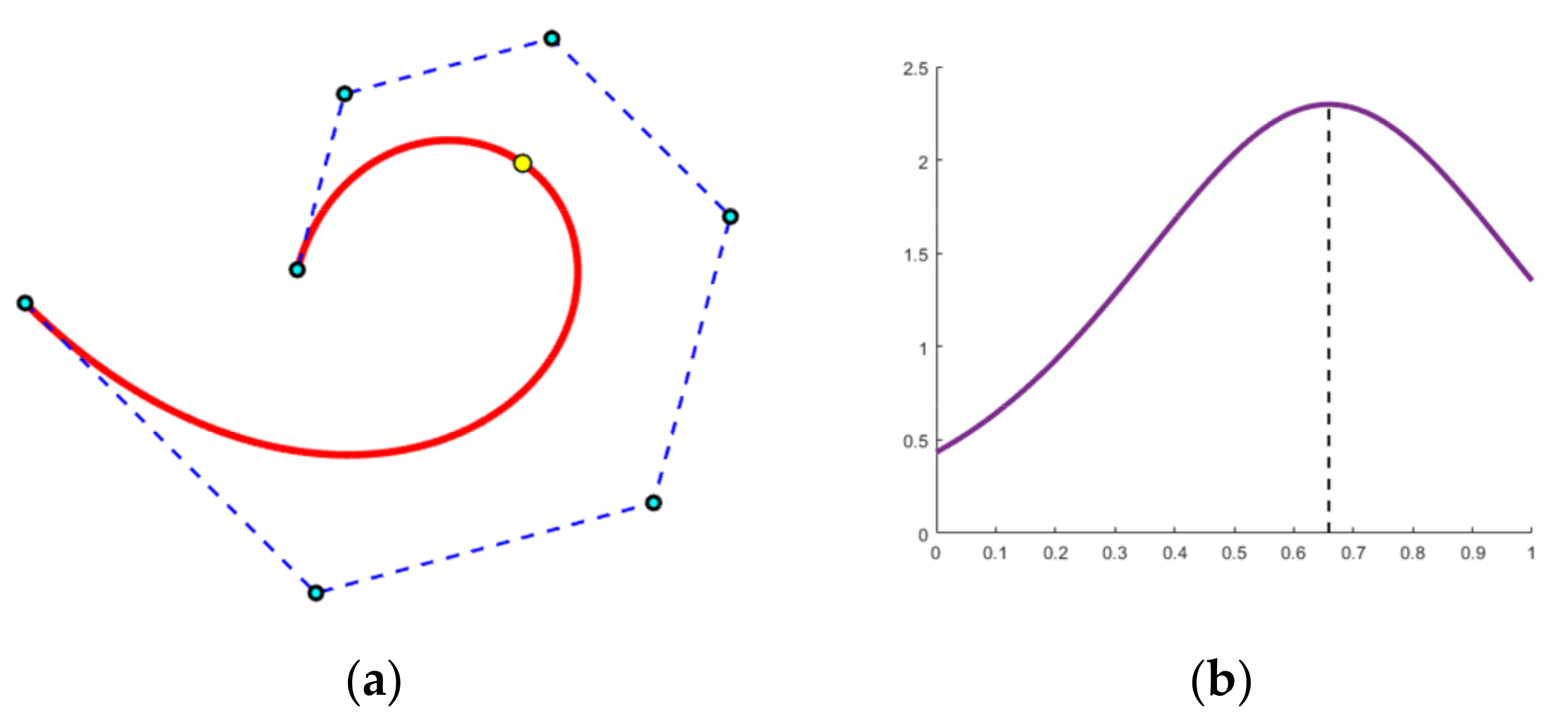
2. Typical Bézier Curves with One Curvature Extremum
- (I).
- increases in then decreases in .
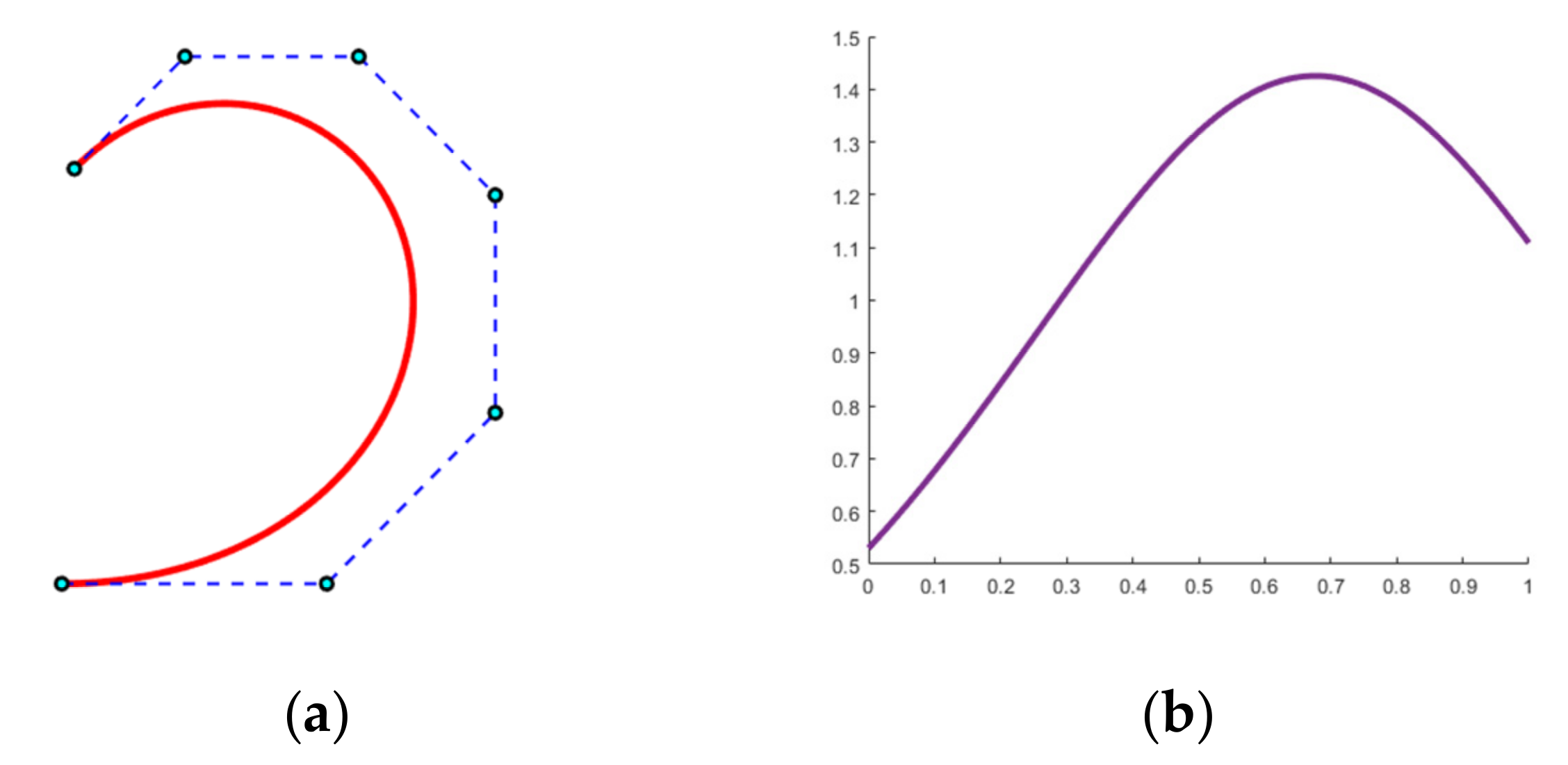
- (II).
- increases in then decreases in .
- (III).
- increases in and then decreases in .
3. Parameter Formula and Subdivision at Curvature Extremum
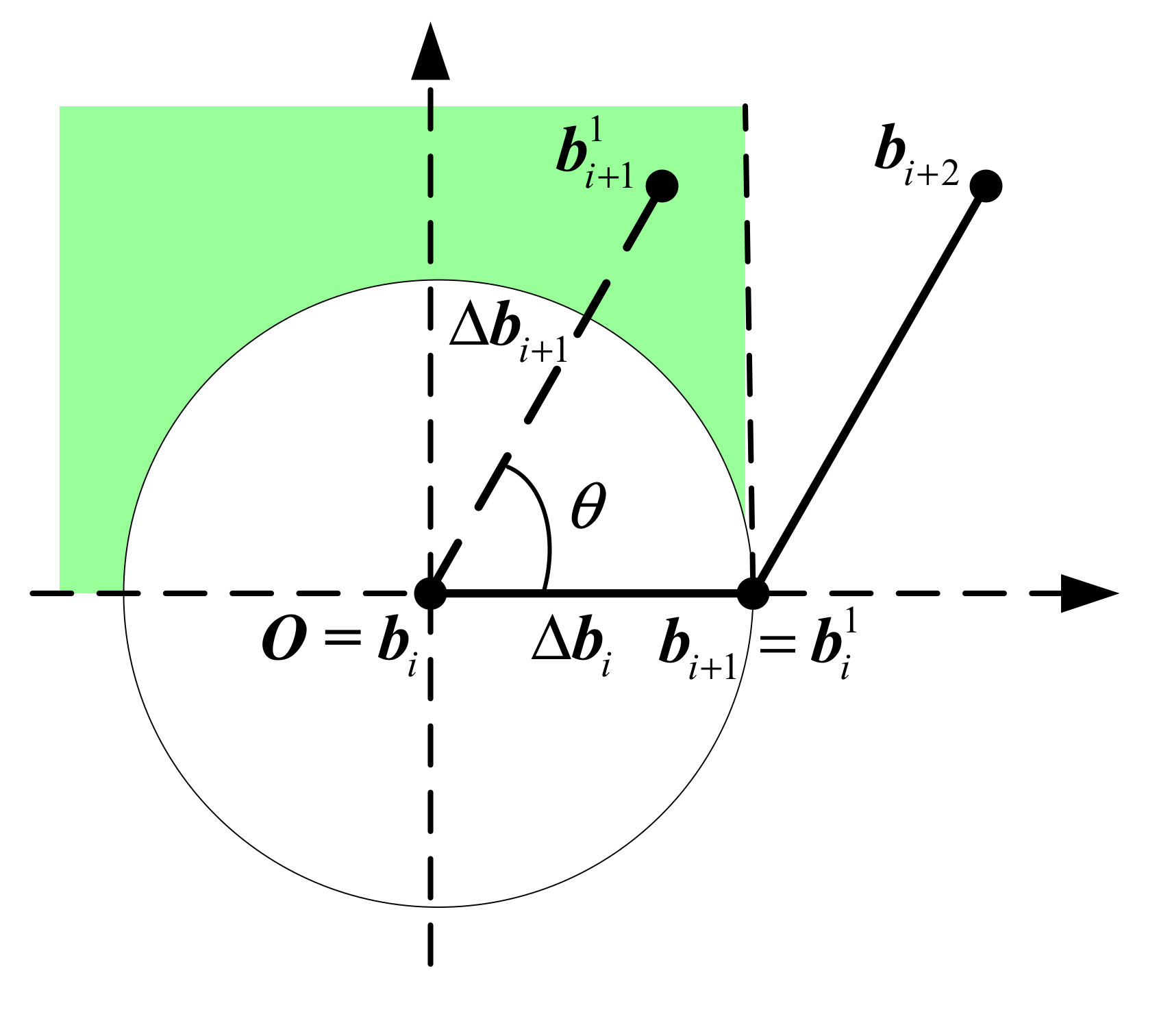
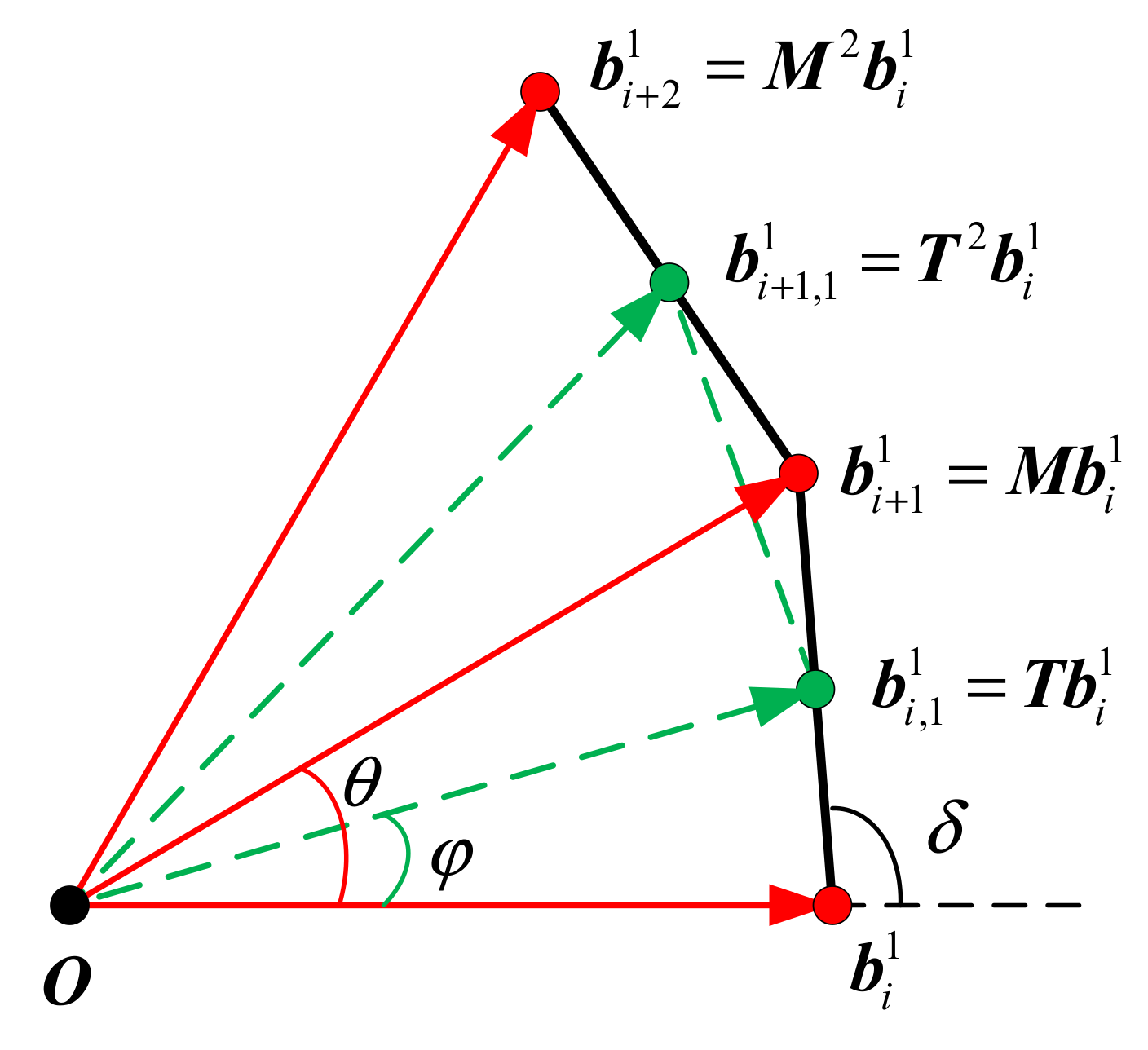
3.1. Fast Parameter Formula
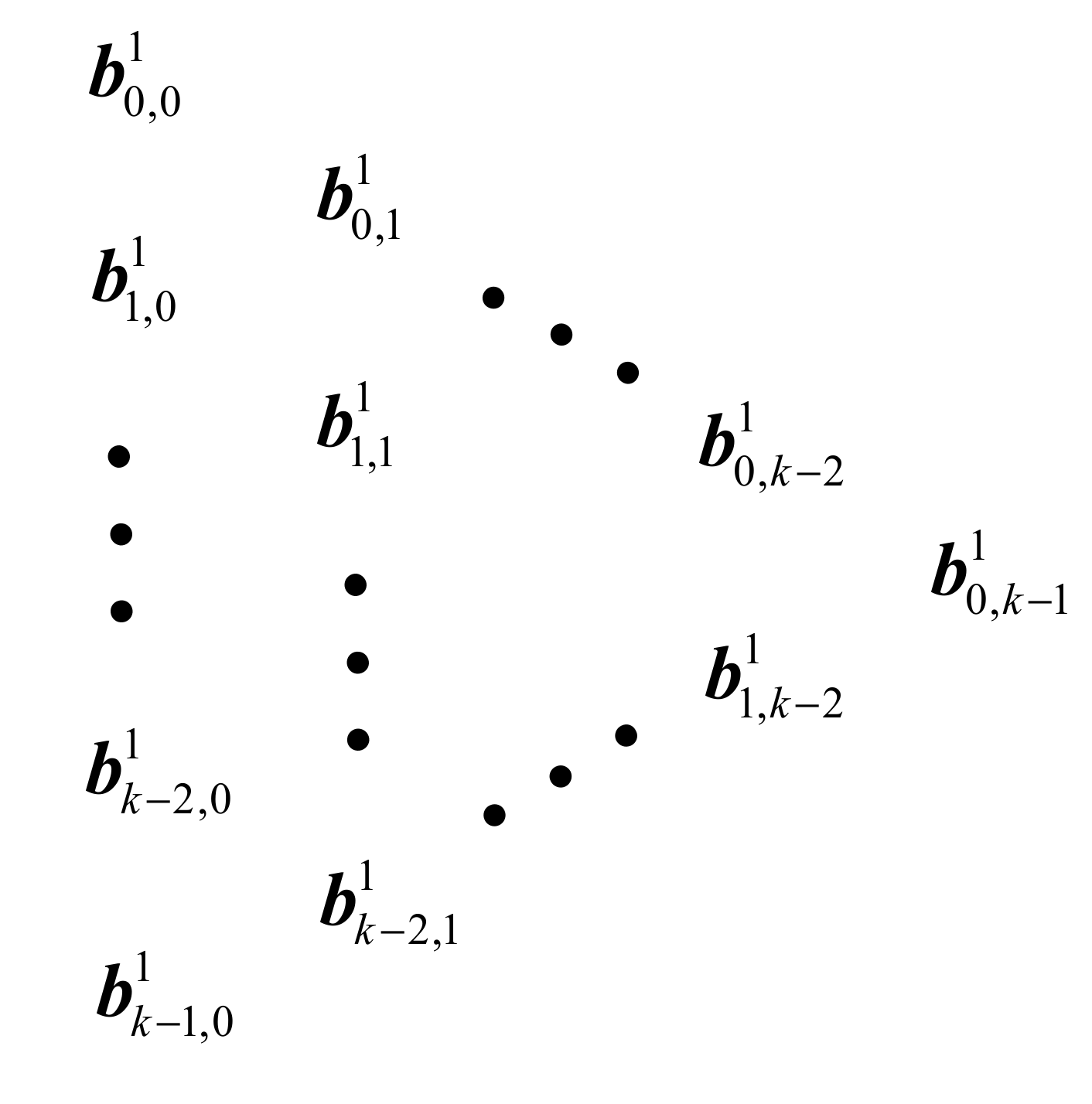
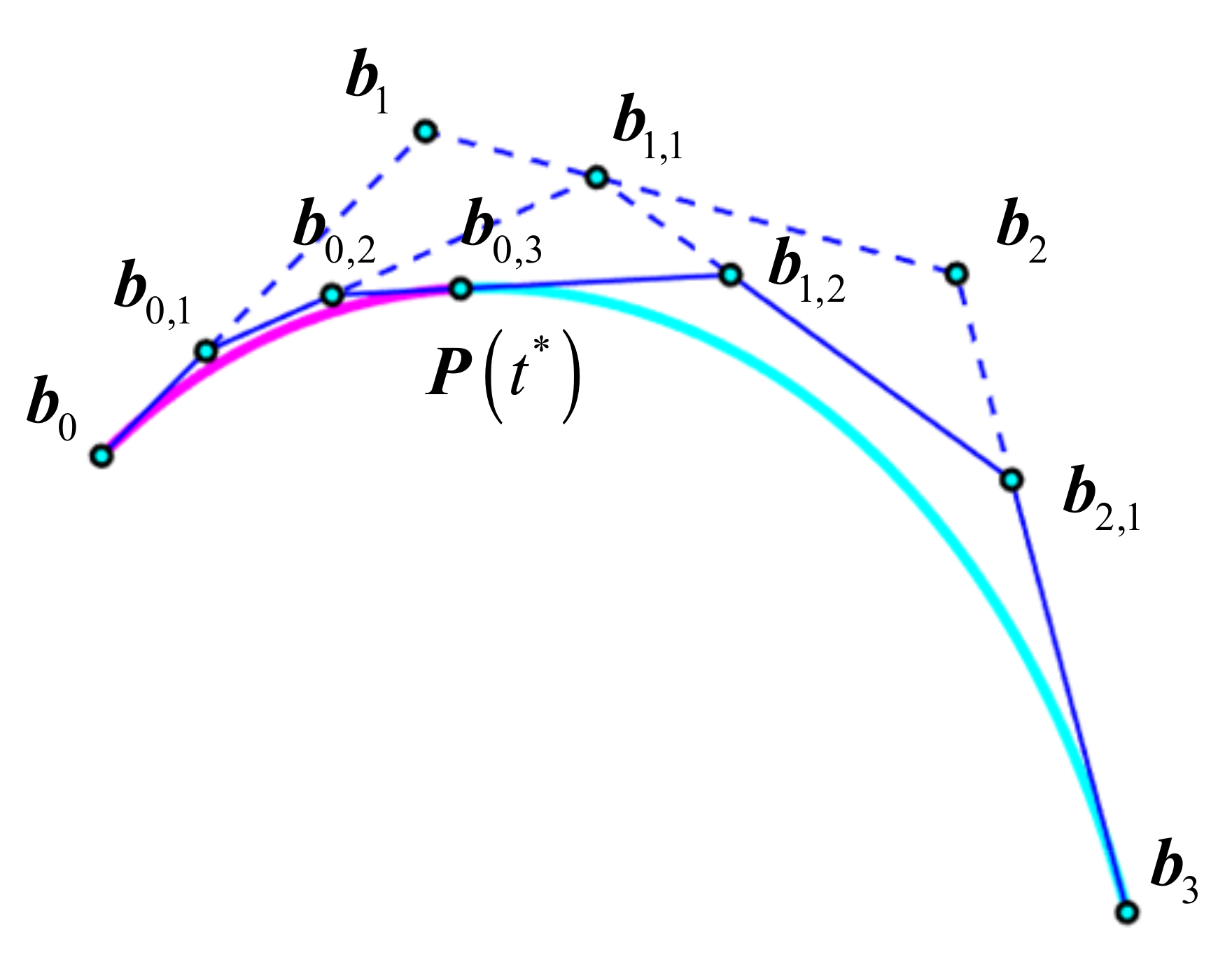
3.2. Subdivision at Curvature Extremum
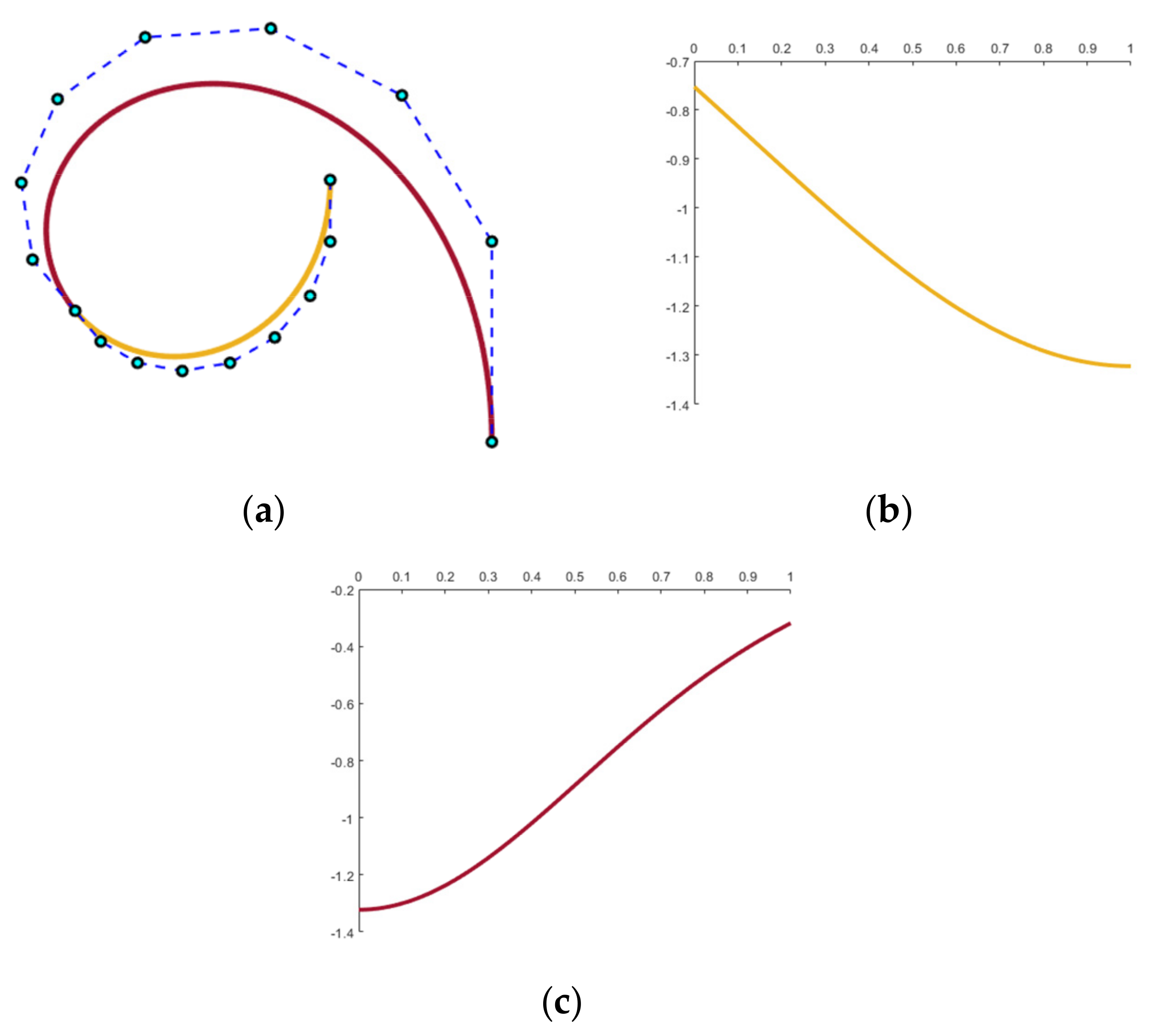
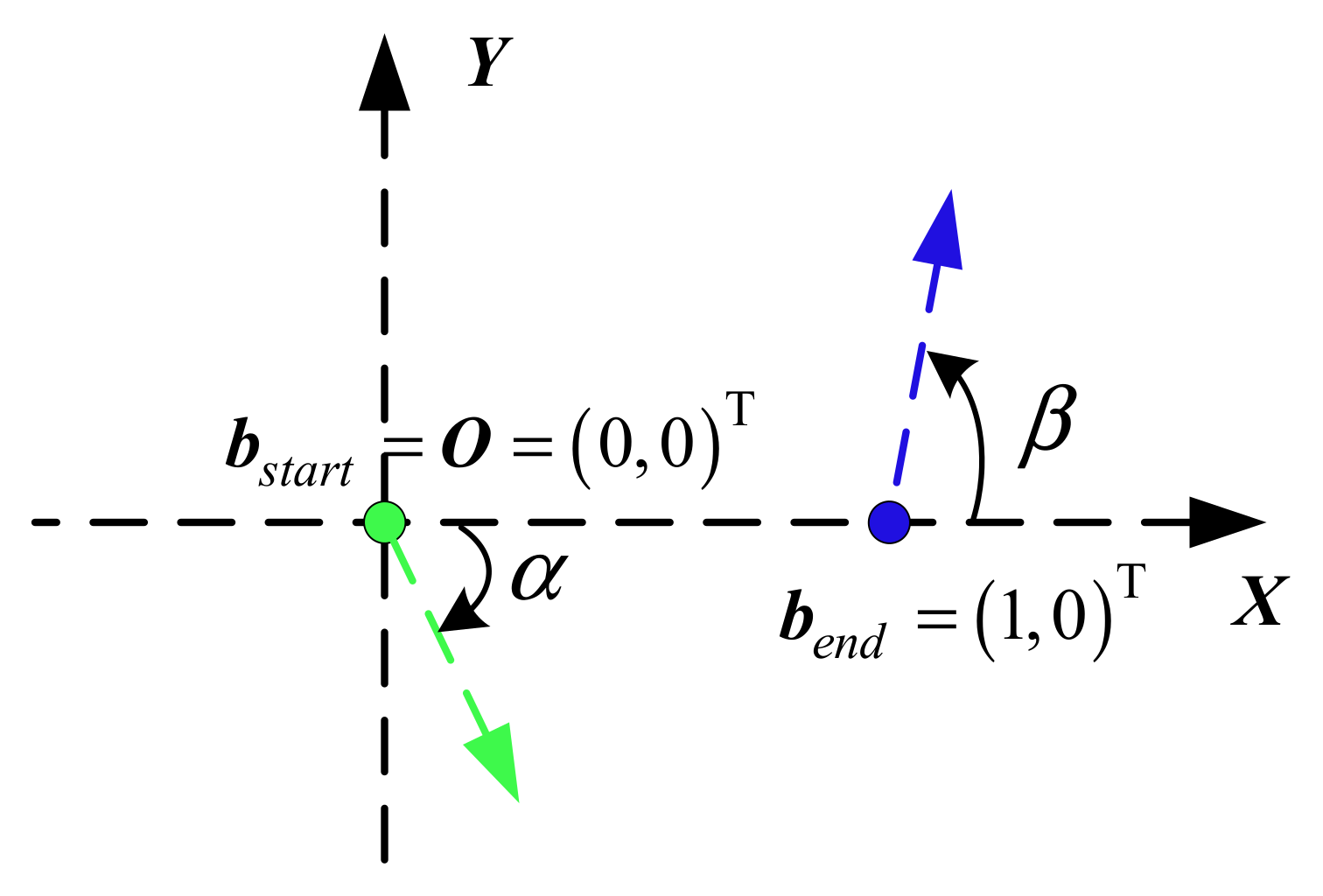
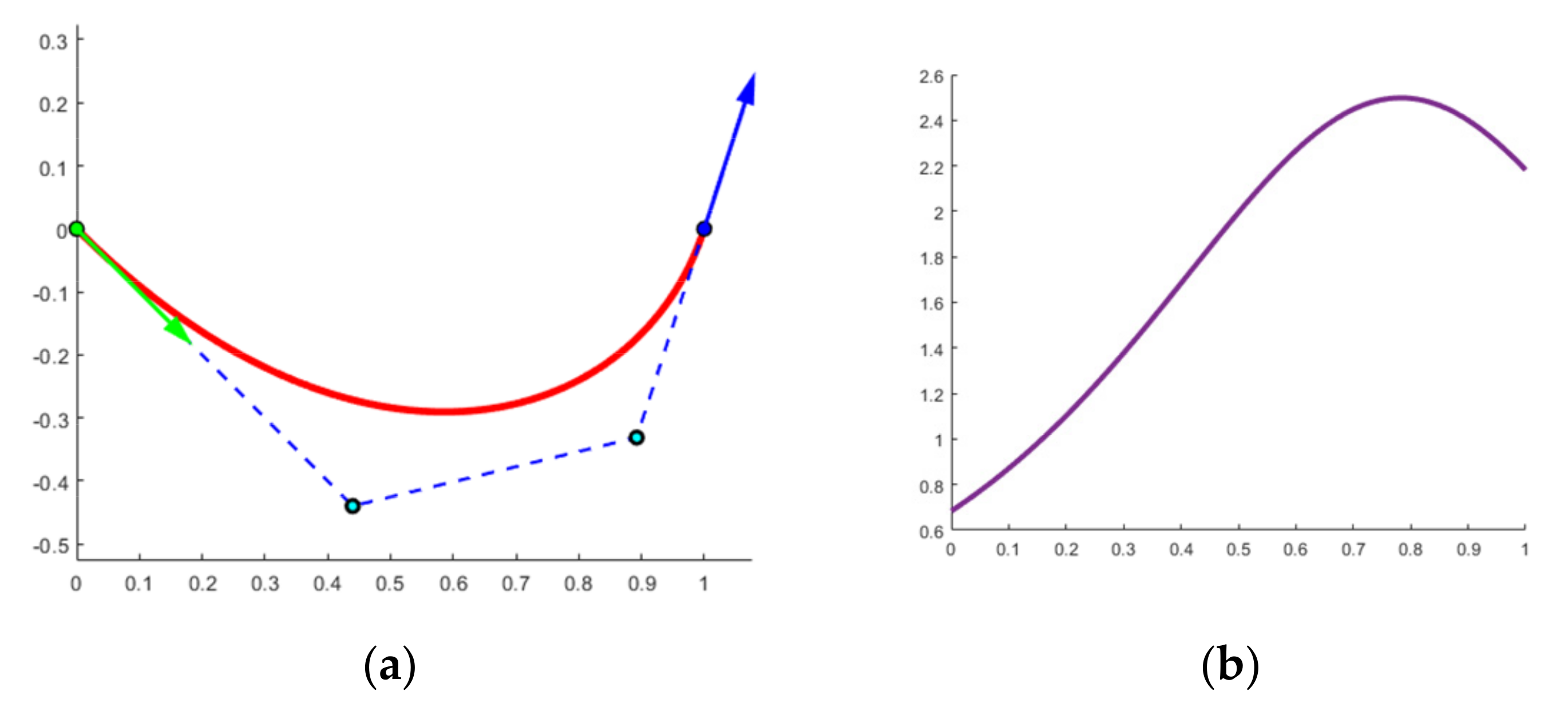
4. Solving G1 Interpolation with a Single Typical Bézier Curve
4.1. A Normalized form for G1 Interpolation
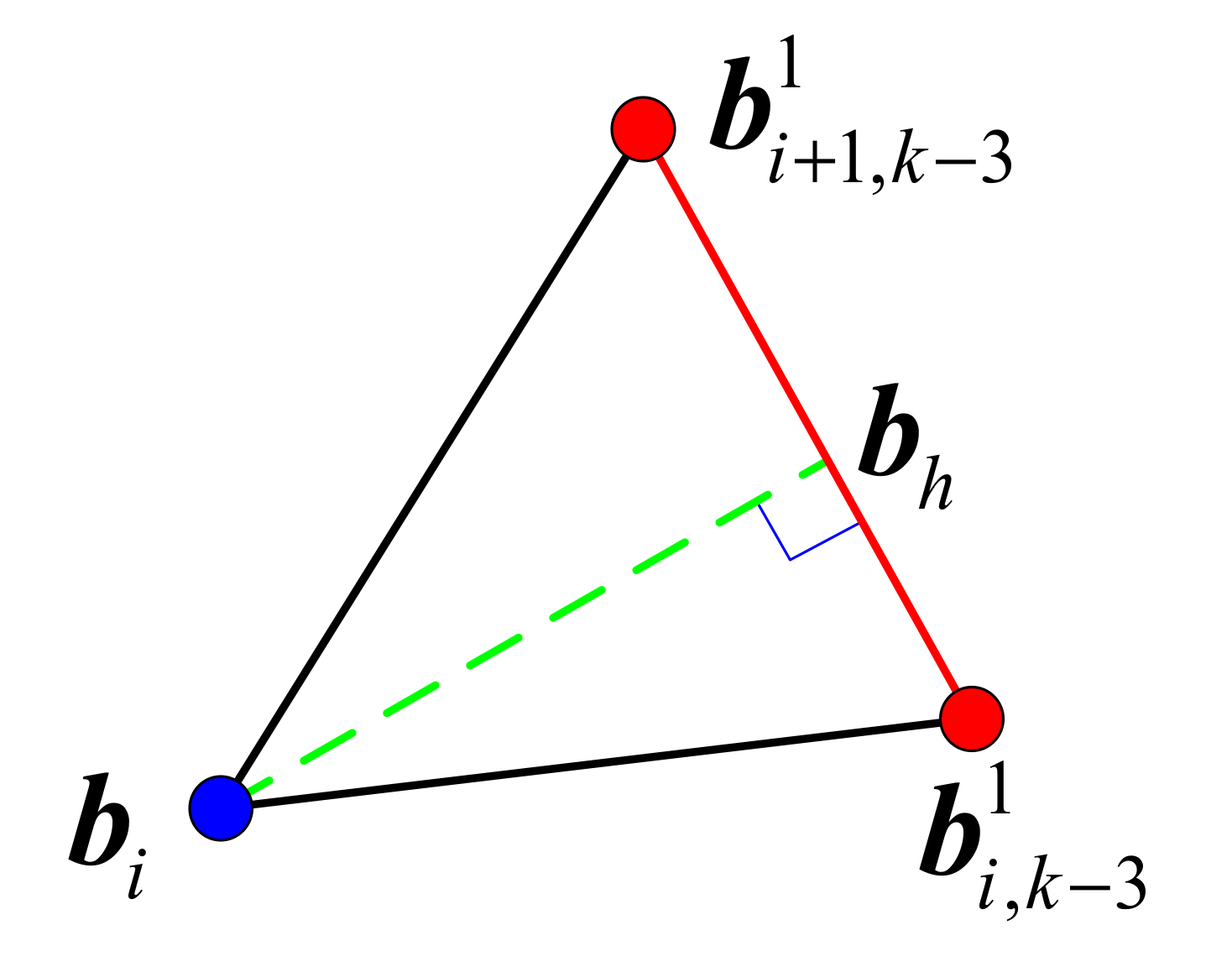
4.2. Sufficient Condition for G1 Interpolation with Typical Bézier Curve Solution
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Farin, G.; Sapidis, N. Curvature and the fairness of curves and surfaces. IEEE Comput. Graph. Appl. 1989, 9, 52–57. [Google Scholar] [CrossRef]
- Farin, G. Curves and Surfaces for CAGD: A Practical Guide, 5th ed.; Morgan Kaufmann: Burlington, MA, USA, 2002. [Google Scholar]
- Beeker, E. Smoothing of shapes designed with free-form surfaces. Comput.-Aided Des. 1986, 18, 224–232. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, B.; Zhang, L.; Xu, J.; Wang, K.; Wang, S. Designing fair curves using monotone curvature pieces. Comput. Aided Geom. Des. 2004, 21, 515–527. [Google Scholar] [CrossRef]
- Sapidis, N.S.; Frey, W.H. Controlling the curvature of a quadratic Bézier curve. Comput. Aided Geom. Des. 1992, 9, 85–91. [Google Scholar] [CrossRef]
- Mineur, Y.; Lichah, T.; Castelain, J.M.; Giaume, H. A shape controled fitting method for Bézier curves. Comput. Aided Geom. Des. 1998, 15, 879–891. [Google Scholar] [CrossRef]
- Farin, G. Class A Bézier curves. Comput. Aided Geom. Des. 2006, 23, 573–581. [Google Scholar] [CrossRef]
- Cao, J.; Wang, G. A note on Class a Bézier curves. Comput. Aided Geom. Des. 2008, 25, 523–528. [Google Scholar] [CrossRef]
- Wang, A.; Zhao, G. Counter examples of “Class A Bézier curves”. Comput. Aided Geom. Des. 2018, 61, 6–8. [Google Scholar] [CrossRef]
- Cantón, A.; Fernández-Jambrina, L.; Vázquez-Gallo, M.J. Curvature of planar aesthetic curves. J. Comput. Appl. Math. 2021, 381, 113042. [Google Scholar] [CrossRef]
- Tong, W.; Chen, M. A sufficient condition for 3D typical curves. Comput. Aided Geom. Des. 2021, 87, 101991. [Google Scholar] [CrossRef]
- Wang, A.; Zhao, G.; Hou, F. Constructing Bézier curves with monotone curvature. J. Comput. Appl. Math. 2019, 355, 1–10. [Google Scholar] [CrossRef]
- Wang, A.; He, C.; Hou, F.; Cai, Z.; Zhao, G. Designing planar cubic B-spline curves with monotonic curvature for curve interpolation. Comput. Vis. Media 2020, 6, 349–354. [Google Scholar] [CrossRef]
- Levien, R.; Séquin, C.H. Interpolating splines: Which is the fairest of them all? Comput.-Aided Des. Appl. 2009, 6, 91–102. [Google Scholar] [CrossRef] [Green Version]
- Yan, Z.; Schiller, S.; Wilensky, G.; Carr, N.; Schaefer, S. K-curves: Interpolation at local maximum curvature. ACM Trans. Graph. (TOG) 2017, 36, 1–7. [Google Scholar] [CrossRef]
- Yan, Z.; Schiller, S.; Schaefer, S. Circle reproduction with interpolatory curves at local maximal curvature points. Comput. Aided Geom. Des. 2019, 72, 98–110. [Google Scholar] [CrossRef]
- Miura, K.T.; Gobithaasan, R.U.; Salvi, P.; Wang, D.; Sekine, T.; Usuki, S.; Inoguchi, J.; Kajiwara, K. ϵκ-Curves: Controlled local curvature extremum. Vis. Comput. 2021, 1–16. [Google Scholar] [CrossRef]
- Yuksel, C. A Class of C 2 Interpolating Splines. ACM Trans. Graph. (TOG) 2020, 39, 1–14. [Google Scholar] [CrossRef]
- Kimia, B.B.; Frankel, I.; Popescu, A.M. Euler spiral for shape completion. Int. J. Comput. Vis. 2003, 54, 159–182. [Google Scholar] [CrossRef]
- Henrici, P. Applied and Computational Complex Analysis; Wiley: New York, NY, USA, 1988; Volume 1. [Google Scholar]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, C.; Zhao, G.; Wang, A.; Li, S.; Cai, Z. Planar Typical Bézier Curves with a Single Curvature Extremum. Mathematics 2021, 9, 2148. https://doi.org/10.3390/math9172148
He C, Zhao G, Wang A, Li S, Cai Z. Planar Typical Bézier Curves with a Single Curvature Extremum. Mathematics. 2021; 9(17):2148. https://doi.org/10.3390/math9172148
Chicago/Turabian StyleHe, Chuan, Gang Zhao, Aizeng Wang, Shaolin Li, and Zhanchuan Cai. 2021. "Planar Typical Bézier Curves with a Single Curvature Extremum" Mathematics 9, no. 17: 2148. https://doi.org/10.3390/math9172148
APA StyleHe, C., Zhao, G., Wang, A., Li, S., & Cai, Z. (2021). Planar Typical Bézier Curves with a Single Curvature Extremum. Mathematics, 9(17), 2148. https://doi.org/10.3390/math9172148






