A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices
Abstract
:1. Introduction
2. Auxiliary Results
- (a)
- (b)
- (c)
- (d)
- (e)
- is convergent,
- (f)
- The inequality is satisfied for finite positive integers and and for each
3. Statistical Convergence via Four Dimensional Matrices
- (i)
- (ii)
- (iii)
- (iv)
- (v)
4. Korovkin Theorem for the Operators via Power Series Method
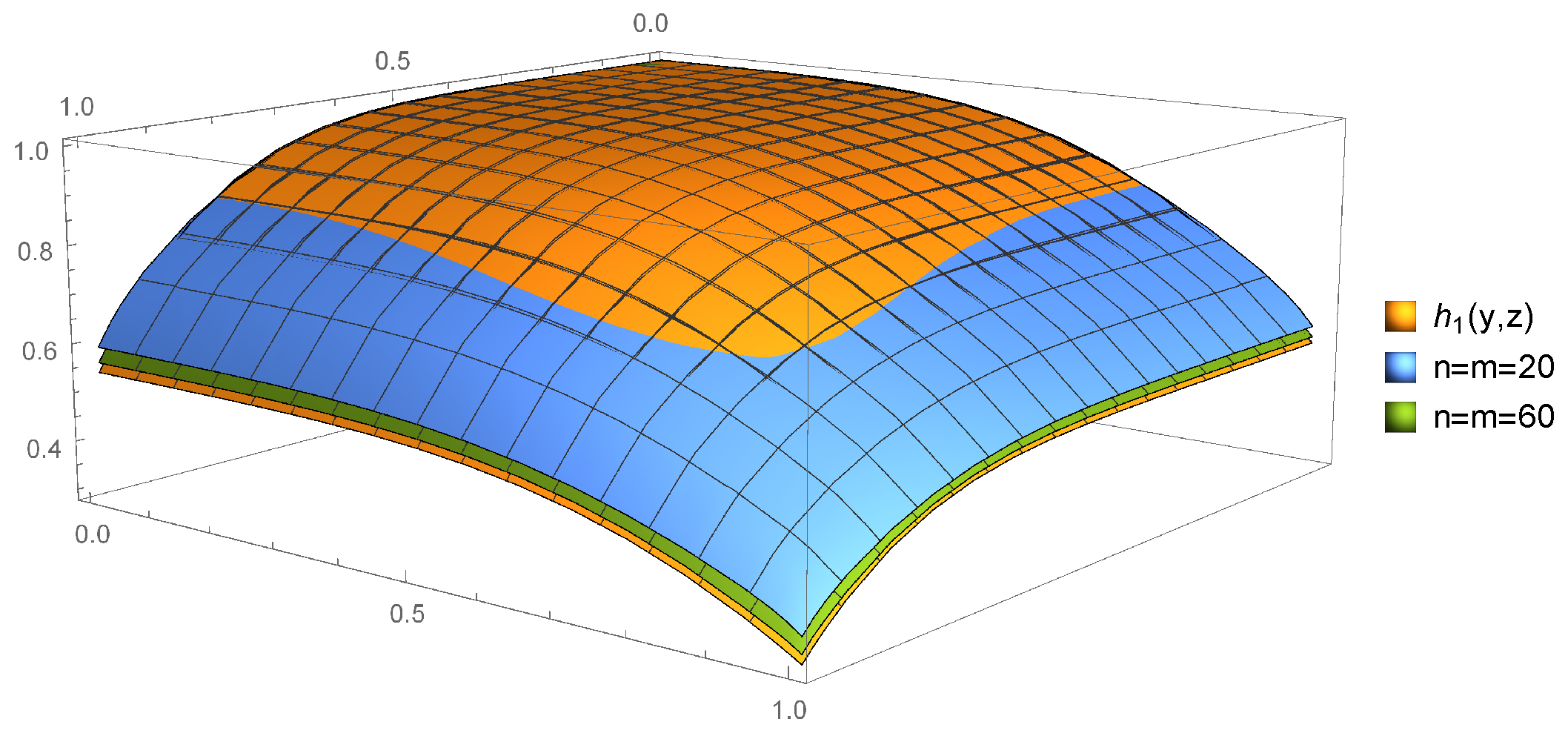
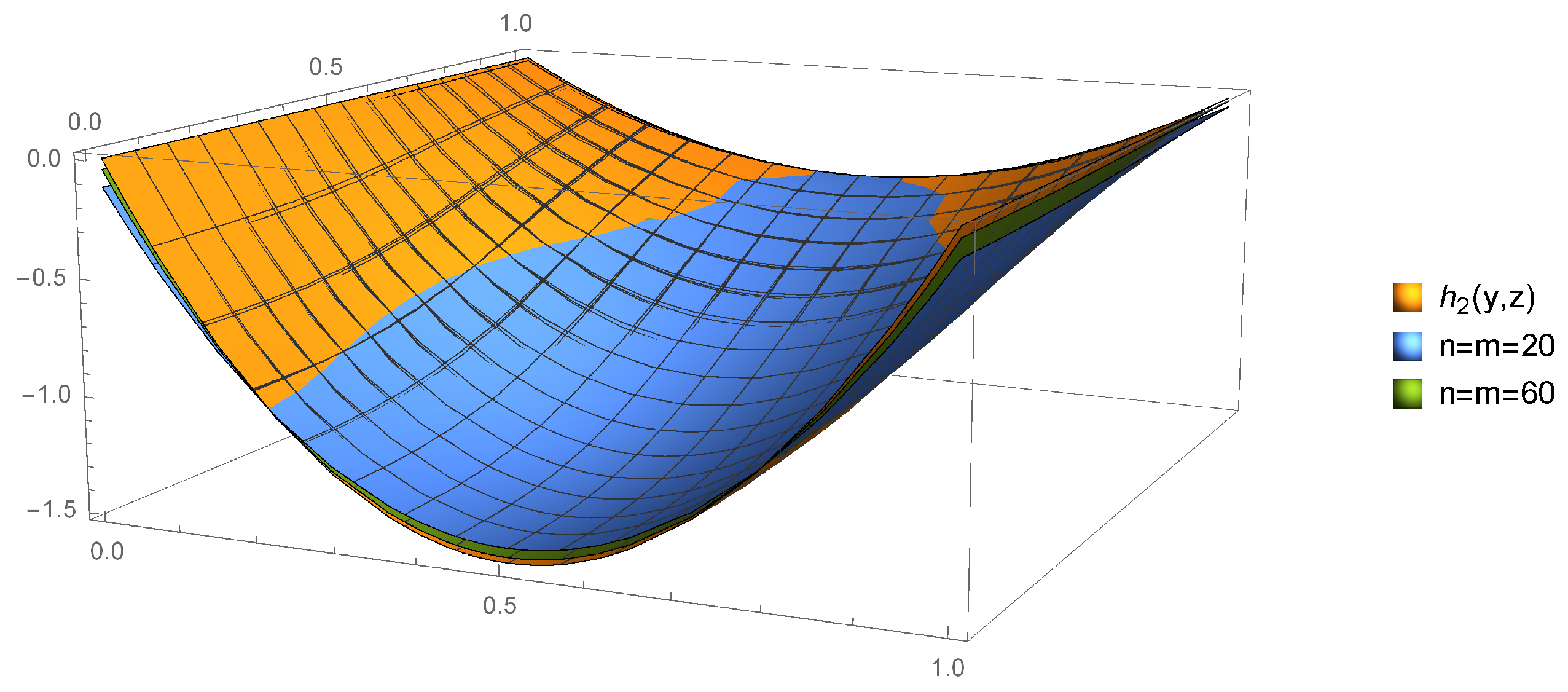
5. The Convergence Rate of Operators
6. Numerical Results
7. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Weierstrass, V.K. Ueber Die Analytische Darstellbarkeit Sogennanter Willkürlicher Functionen Einer Reellen Veranderliches. Sitzungsberichte der Königlich Preußischen Akademie der Wissenschaften zu Berlin 1885, 2, 633–639. [Google Scholar]
- Bernstein, S.N. Démonstration du théorème de Weierstrass fondée sur le calcul des probabilités. Comm. Soc. Math. Kharkow 1912, 13, 1–2. [Google Scholar]
- Bernstein, S.N. Sur les recherches récentes relatives à la meilleure approximation des fonctions continues par les polynomes. In Proceedings of the fifth International Congress of Mathematicians, Cambridge, UK, 22–28 August 1912; Volume 1, pp. 256–266. [Google Scholar]
- Kantorovich, L. Sur Certains Developpements Suivant les Polynomes de la Forme de S. Bernstein I II Dokal Akad Nauk SSSR 1930, 563, 568. [Google Scholar]
- Cai, Q.B.; Lian, B.-Y.; Zhou, G. Approximation properties of λ-Bernstein operators. J. Ineq. App. 2018, 2018, 61. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Özger, F. Approximation of functions by Stancu variant of Bernstein-Kantorovich operators based on shape parameter α. RACSAM 2020, 114, 70. [Google Scholar] [CrossRef]
- Mursaleen, M.; Ansari, K.J.; Khan, A. Some approximation results by Bernstein-Kantorovich operators based on (p,q)-calculus. U.P.B. Sci.-Bull.-Ser. A 2016, 78, 129–142. [Google Scholar]
- Mursaleen, M.; Ansari, K.J.; Khan, A. On (p,q)-analogue of Bernstein operators. Appl. Math. Comput. 2016, 266, 874–882, Erratum in Appl. Math. Comput. 2016, 278, 70–71. [Google Scholar] [CrossRef]
- Özger, F. Weighted statistical approximation properties of univariate and bivariate λ-Kantorovich operators. Filomat 2019, 33, 3473–3486. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Özger, F.; Mohiuddine, S.A. Construction of Stancu-type Bernstein operators based on Bézier bases with shape parameter λ. Symmetry 2019, 11, 316. [Google Scholar] [CrossRef] [Green Version]
- Cai, Q.B. The Bézier variant of Kantorovich type λ-Bernstein operators. J. Inequal. Appl. 2018, 90. [Google Scholar] [CrossRef]
- Acu, A.M.; Manav, N.; Sofonea, D.F. Approximation properties of λ-Kantorovich operators. J. Inequal. Appl. 2018, 202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Malkowsky, E.; Velickovic, V. Topologies of some new sequence spaces, their duals, and the graphical representation of neighbourhoods. Topol. Appl. 2011, 158, 1369–1380. [Google Scholar] [CrossRef] [Green Version]
- Maioa, G.D.; Kocinac, L.D.R. Statistical convergence in topology. Topol. Appl. 2008, 156, 28–45. [Google Scholar] [CrossRef] [Green Version]
- Pehlivan, S.; Mamedov, M.A. Statistical cluster points and turnpike. Optimization 2000, 48, 93–106. [Google Scholar] [CrossRef]
- Miller, H.I. A measure theoretical subsequence characterization of statistical convergence. Trans. Amer. Math. Soc. 1995, 347, 1811–1819. [Google Scholar] [CrossRef]
- Erdös, P.; Tenenbaur, G. Sur Les Densités de Certaines Suites D’Entiers. Proc. London Math. Soc. 1989, 59, 417–438. [Google Scholar] [CrossRef]
- Zygmund, A. Trigonometric Series, 2nd ed.; Cambridge University Press: Cambridge, UK, 1979. [Google Scholar]
- Edelya, O.H.H.; Mursaleen, M.; Khan, A. Approximation for periodic functions via weighted statistical convergence. Appl. Math. Comput. 2013, 219, 8231–8236. [Google Scholar] [CrossRef]
- Gadjiev, A.D.; Orhan, C. Some approximation theorems via statistical convergence. Rocky Mt. J. Math. 2002, 32, 129–138. [Google Scholar] [CrossRef]
- Özger, F.; Srivastava, H.M.; Mohiuddine, S.A. Approximation of functions by a new class of generalized Bernstein-Schurer operators. Rev. R. Acad. Cienc. Exactas Fís. Nat. Ser. A Math. RACSAM 2020, 114, 173. [Google Scholar] [CrossRef]
- Özger, F. Applications of generalized weighted statistical convergence to approximation theorems for functions of one and two variables. Numer. Funct. Anal. Optim. 2020, 41, 1990–2006. [Google Scholar] [CrossRef]
- Özger, F. On new Bézier bases with Schurer polynomials and corresponding results in approximation theory. Commun. Fac. Sci. Univ. Ank. Ser. A1 Math. Stat. 2019, 69, 376–393. [Google Scholar] [CrossRef]
- Mohiuddine, S.A.; Ahmad, N.; Özger, F.; Alotaibi, A.; Hazarika, B. Approximation by the Parametric Generalization of Baskakov-Kantorovich Operators Linking with Stancu Operators. Iran. J. Sci. Technol. Trans. Sci. 2021. [Google Scholar] [CrossRef]
- Alotaibi, A.; Özger, F.; Mohiuddine, S.A.; Alghamdi, M.A. Approximation of functions by a class of Durrmeyer–Stancu type operators which includes Euler’s beta function. Adv. Differ. Equ. 2021, 13. [Google Scholar] [CrossRef]
- Fast, H. Sur la convergence statistique. Colloq. Math. 1951, 2, 241–244. [Google Scholar] [CrossRef]
- Moricz, F. Statistical convergence of multiple sequences. Arch. Math. 2003, 81, 82–89. [Google Scholar] [CrossRef]
- Steinhaus, H. Sur la convergence ordinaire et la convergence asymptotique. Colloq. Math. 1951, 2, 73–74. [Google Scholar]
- Bardaro, C.; Boccuto, A.; Demirci, K.; Mantellini, I.; Orhan, S. Triangular A-statistical approximation by double sequences of positive linear operators. Results Math. 2015, 68, 271–291. [Google Scholar] [CrossRef]
- Demirci, K.; Orhan, S.; Kolay, B. Statistical relative A-summation process for double sequences on modular spaces. Rev. Real Acad. Cienc. Exactas Físicas Nat. Ser. A Matemáticas 2018, 112, 1249–1264. [Google Scholar] [CrossRef]
- Dirik, F.; Demirci, K. Korovkin type approximation theorem for functions of two variables in statistical sense. Turk. J. Math. 2010, 34, 73–83. [Google Scholar]
- Braha, N.L. Some properties of new modified Szász-Mirakyan operators in polynomial weight spaces via power summability methods. Bull. Math Anal. Appl. 2018, 10, 53–65. [Google Scholar]
- Braha, N.L. Some properties of Baskakov-Schurer-Szasz operators via power summability methods. Quaest. Math. 2019, 42, 1411–1426. [Google Scholar] [CrossRef]
- Braha, N.L.; Kadak, U. Approximation properties of the generalized Szasz operators by multiple Appell polynomials via power summability method. Math. Methods Appl. Sci. 2020, 43, 2337–2356. [Google Scholar] [CrossRef]
- Braha, N.L.; Mansour, T.; Srivastava, H.M. A parametric generalization of the Baskakov-Schurer-Szász-Stancu approximation operators. Symmetry 2021, 13, 980. [Google Scholar] [CrossRef]
- Söylemez, D.; Ünver, M. Korovkin type theorems for Cheney–Sharma Operators via summability methods. Results Math. 2017, 72, 1601–1612. [Google Scholar] [CrossRef]
- Pringsheir, A. Zur theorie der zweifach unendlichen zahlenfolges. Math. Ann. 1900, 53, 289–321. [Google Scholar] [CrossRef]
- Robison, G.M. Divergent double sequences and series. Amer. Math. Soc. Transl. 1926, 28, 50–73. [Google Scholar] [CrossRef]
- Miller, H.I. A-statistical convergence of subsequence of double sequences. Boll. U.M.I. 2007, 8, 727–739. [Google Scholar]
- Baron, S.; Stadtmüller, U. Tauberian theorems for power series methods applied to double sequences. J. Math. Anal. Appl. 1997, 211, 574–589. [Google Scholar] [CrossRef] [Green Version]
- Özger, F.; Demirci, K.; Yıldız, S. Approximation by Kantorovich Variant of λ-Schurer Operators and Related Numerical Results, Topics in Contemporary Mathematical Analysis and Applications; CRC Press: Boca Raton, FL, USA, 2020; pp. 77–94. [Google Scholar]
- Schurer, F. Linear Positive Operators in Approximation Theory. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1962. [Google Scholar]
- Ye, Z.; Long, X.; Zeng, X.-M. Adjustment algorithms for Bezier curve and surface. In Proceedings of the International Conference on Computer Science and Education, Hefei, China, 24–27 August 2010; pp. 1712–1716. [Google Scholar]
- Demirci, K.; Yıldız, S.; Dirik, F. Approximation via power series method in two-dimensional weighted spaces. Bull. Malays. Math. Sci. Soc. 2020, 43, 3871–3883. [Google Scholar] [CrossRef]
- Dirik, F.; Yıldız, S.; Demirci, K. Abstract Korovkin theory for double sequences via power series method in modular spaces. Oper. Matrices 2019, 13, 1023–1034. [Google Scholar] [CrossRef] [Green Version]
- Ünver, M. Abel transforms of positive linear operators on weighted spaces. Bull. Belg. Math. Soc. Simon Stevin 2014, 21, 813–822. [Google Scholar] [CrossRef]
- Yavuz, E.; Talo, Ö. Approximation of continuous periodic functions of two variables via power series methods of summability. Bull. Malays. Math. Sci. Soc. 2019, 42, 1709–1717. [Google Scholar] [CrossRef] [Green Version]
- Natanson, I.P. Constructive Function Theory Volume I Uniform Approximation; Frederick Ungar Publising Company: New York, NY, USA, 1964. [Google Scholar]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Srivastava, H.M.; Ansari, K.J.; Özger, F.; Ödemiş Özger, Z. A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices. Mathematics 2021, 9, 1895. https://doi.org/10.3390/math9161895
Srivastava HM, Ansari KJ, Özger F, Ödemiş Özger Z. A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices. Mathematics. 2021; 9(16):1895. https://doi.org/10.3390/math9161895
Chicago/Turabian StyleSrivastava, Hari M., Khursheed J. Ansari, Faruk Özger, and Zeynep Ödemiş Özger. 2021. "A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices" Mathematics 9, no. 16: 1895. https://doi.org/10.3390/math9161895
APA StyleSrivastava, H. M., Ansari, K. J., Özger, F., & Ödemiş Özger, Z. (2021). A Link between Approximation Theory and Summability Methods via Four-Dimensional Infinite Matrices. Mathematics, 9(16), 1895. https://doi.org/10.3390/math9161895








