From Teacher of Nations to Teacher of Mathematics
Abstract
1. Introduction
2. Historical Background
2.1. Comenius as the Founder of Czech Educational Traditions
2.2. Beginnings and Development of Basic Research and Establishment of the Didactics of Mathematics in Czechoslovakia
2.3. Support for Mathematics Education from “Pure” Mathematics
2.4. Teachers and Reconstruction of Mathematics Education
3. Data and Methods
4. Theoretical Background
4.1. Knowledge of Content and Pedagogical Content Knowledge
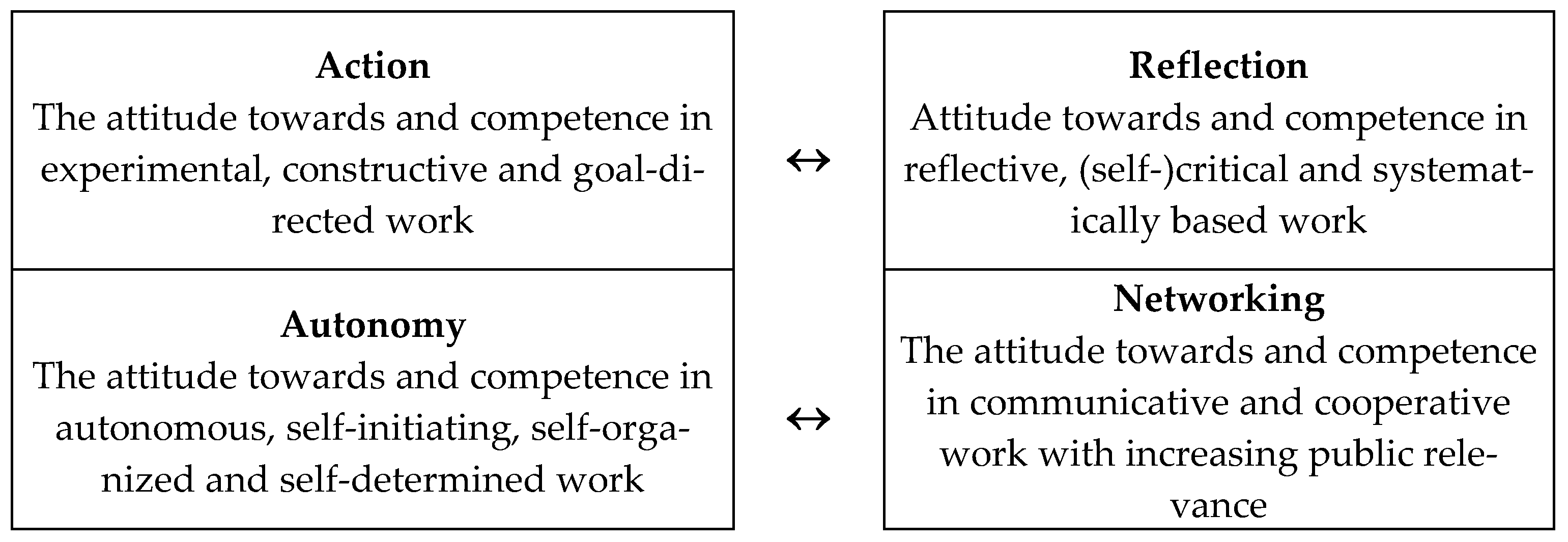
4.2. Teachers’ Competences
- (a)
- Competence related to pupils (namely creating conditions for the development of pupils’ abilities and skills by designing procedures for an effective pedagogical intervention, etc.).
- (b)
- Subject-didactic competence encompassing mastery of the scientific basis of teaching a subject and didactic creativity (i.e., the ability to keep the subject matter up to date in its cognitive, motivational and social significance).
- (c)
- Pedagogical-organizational competence aimed at creating an effective educational environment, together with a supportive and stimulating climate.
- (d)
- Competence in qualified pedagogical (self-)reflection with an emphasis on the analysis of the teacher’s own thinking and dealing with pupils in a way that is suited to their ability (abbreviated text from [2], pp. 38–39, our translation).
5. Six Strands of Research on Teacher Education in the Czech Republic
5.1. Developing Teachers’ Pedagogical Content Knowledge via Lesson Study
5.1.1. Data and Methods
5.1.2. Selected Results
5.2. Joint Reflection in Primary School Teachers’ Professional Development
5.2.1. Data and Methods
5.2.2. Selected Results
- -
- Self-confidence concerning the content and methods of mathematics teaching at the beginning of their research program;
- -
- Uncertainty about their competences, which originated after several discussions;
- -
- Ambition to change their practice, to improve subject-didactic competences and to better understand pupils’ cognition of mathematics.
5.3. Professional Vision of Pre-Service Teachers
5.3.1. Measuring Noticing Skills
5.3.2. Developing Noticing and Reasoning Skills via Video-Interventions
5.4. Culture of Problem Solving and Teachers’ Growth
5.4.1. Data and Methods
5.4.2. Selected Results
5.5. Supporting Subject-Didactic Competence Through Problem Posing
5.5.1. Data and Methods
- (a)
- (b)
- Solvable by a given calculation (namely, “Pose a task (several tasks) that can be solved by calculation: 1/4 × 2/3”) [25].
5.5.2. Selected Results
5.6. Teachers’ Competences for Content and Language Integrated Learning (CLIL) and for Culturally Responsive Teaching
5.6.1. Pre-Service CLIL Teachers’ Skills with a Focus on Lesson Planning Skills
5.6.2. Mathematics Teacher Education for Teaching in Culturally and Linguistically Heterogeneous Classes
6. Discussion and Conclusions
6.1. Professional Vision and Related Concepts
6.2. Professional Knowledge and Professional Action (Problem Solving and Problem Posing)
6.3. Methodological Issues
6.4. Implications and Follow-Up Research Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Helus, Z. Four theses on school reform. Pedagogika 2001, 51, 25–41. (In Czech) [Google Scholar]
- Comenius, J.A. The Great Didactic [Didactica Magna]; Keatinge, M.W., Ed.; Russell & Russell: New York, NY, USA, 1967; Available online: https://web.archive.org/web/20140202105252/ (accessed on 27 February 2021).
- Komenský, J.A. Analytical Didactics; Brno: Tvořivá škola, Czech Republic, 2004. (In Czech) [Google Scholar]
- Pikkarainen, E. Signs of Reality: The Idea of general Bildung by J. A. Comenius. In Theories of Bildung and Growth: Connections and Controversies between Continental Educational Thinking and American Pragmatism; Siljander, P., Kivelä, A., Sutinen, A., Sense, B., Eds.; Springer Science and Business Media LLC: Berlin/Heidelberg, Germany, 2012; pp. 19–29. [Google Scholar] [CrossRef]
- Shulman, L.S. Those who understand: Knowledge growth in teaching. Educ. Res. 1986, 15, 4–14. [Google Scholar] [CrossRef]
- Vyšín, J. About basic research and the work of the Section for the Modernization of Mathematics Teaching. Mat. a Fyzika ve Škole 1979, 10, 104–112. (In Czech) [Google Scholar]
- Vyšín, J. Genetic method in teaching mathematics. Mat. a Fyzika ve Škole 1976, 6, 582–593. (In Czech) [Google Scholar]
- Vyšín, J. Research work in the theory of mathematics education. PMFA 1973, 18, 32–38. (In Czech) [Google Scholar]
- Kuřina, F. Mathematics as a Pedagogical Problem: My Didactical Creed; Hradec Králové: Gaudeamus, Czech Republic, 2016. (In Czech) [Google Scholar]
- Novotná, J.; Tichá, M.; Vondrová, N. Czech and Slovak research in didactics of mathematics: Tradition and a glance at present state. In European Traditions in Didactics of Mathematics; Blum, W., Artigue, M., Mariotti, M.A., Strässer, R., van den, M.-P., Eds.; Springer Open: Berlin/Heidelberg, Germany, 2019; pp. 187–212. [Google Scholar] [CrossRef]
- Vondrová, N. Lesson Study, enhanced with video-based tasks, in the education of mathematics teachers: Lesson Study in the middle. In Supporting teachers: Improving instruction. Examples of Research-Based Teacher Education; Janík, T., Delehefte, I.M., Zehetmeier, S.M., Eds.; Waxmann Publishing House: New York, NY, USA, 2019; pp. 129–151. [Google Scholar]
- Vondrová, N.; Cachová, J.; Coufalová, J.; Krátká, M. “Lesson study” in the Czech context: How teachers perceived their participation and what influence it had on their noticing mathematics education phenomena. Pedagogika 2016, 66, 427–442. (In Czech) [Google Scholar] [CrossRef][Green Version]
- Vondrová, N.; Novotná, M.; Pavlasová, L.; Robová, J.; Stará, J.; Uličná, K. Video-Interventions: Bridges between Theory and Practice in Pre-Service Teachers’ Development; Karolinum: Praha, Czech Republic, 2020. [Google Scholar]
- Simpson, A.; Vondrová, N. Developing pre-service teachers’ professional vision with video interventions: A divergent replication. J. Educ. Teach. 2019, 45, 567–584. [Google Scholar] [CrossRef]
- Stehlíková, N. Interpretation of some mathematics education phenomena by pre-service and in-service teachers. Pedagogika 2010, 60, 303–313. (In Czech) [Google Scholar]
- Simpson, A.; Vondrová, N.; Žalská, J. Sources of shifts in pre-service teachers’ patterns of attention: The roles of teaching experience and of observational experience. J. Math. Teach. Educ. 2018, 21, 607–630. [Google Scholar] [CrossRef]
- Tichá, M.; Hošpesová, A. Qualified pedagogical reflection as a way to improve mathematics education. J. Math. Teach. Educ. 2006, 9, 129–156. [Google Scholar] [CrossRef]
- Vondrová, N.; Žalská, J. Do pre-service teachers attend to mathematics specific phenomena when observing mathematics teaching on video? Orb. Sch. 2012, 6, 85–101. [Google Scholar] [CrossRef]
- Pavlasová, L.; Stará, J.; Vondrová, N.; Novotná, M.; Robová, J.; Uličná, K. Selective attention of pre-service teachers and the nature of their interpretations. Pedagogika 2018, 68, 5–24. (In Czech) [Google Scholar] [CrossRef][Green Version]
- Vondrová, N.; Žalská, J. Ability to notice mathematics specific phenomena: What exactly do pre-service teachers attend to? Orb. Sch. 2015, 9, 77–101. [Google Scholar] [CrossRef]
- Vondrová, N. The effect of a video-based intervention on the knowledge-based reasoning of future mathematics teachers. In Invited Lectures from the 13th International Congress on Mathematical Education; Kaiser, G., Ed.; Springer: Berlin/Heidelberg, Germany, 2018; pp. 699–717. [Google Scholar]
- Eisenmann, P.; Novotná, J.; Přibyl, J.; Břehovský, J. The development of a culture of problem solving with secondary students through heuristic strategies. Math. Educ. Res. J. 2015, 27, 535–562. [Google Scholar] [CrossRef]
- Tichá, M.; Hošpesová, A. Developing teachers’ subject didactic competence through problem posing. Educ. Stud. Math. 2013, 83, 133–143. [Google Scholar] [CrossRef]
- Hošpesová, A.M.; Tichá, M. Problem posing in primary school teacher training. In Mathematical Problem Posing: From Research to Effective Practice; Singer, F.M., Ellerton, N., Cai, J., Eds.; Springer: Berlin/Heidelberg, Germany, 2015; pp. 433–447. [Google Scholar] [CrossRef]
- Tichá, M.; Hošpesová, A. Problem Posing and Development of Pedagogical Content Knowledge in Pre-Service Teacher Training. In Proceedings of CERME 6; Lyon, I.N.R.P., Ed.; Institut National de Recherche Pédagogiqu: Lyon, France, 2010; pp. 1941–1950. Available online: www.inrp.fr/editions/cerme6 (accessed on 1 March 2021).
- Novotná, J.; Hadj-Moussová, Z.; Hofmannová, M. Teacher training for CLIL—Competences of a CLIL teacher. In International Symposium Elementary Maths Teaching SEMT ’01. Proceedings; Novotná, J., Hejný, M., Eds.; Charles University, Faculty of Education: Prague, Czech Republic, 2001; pp. 121–126. [Google Scholar]
- Moraová, H.; Novotná, J. How do pre-service teachers of mathematics plan their CLIL mathematics lessons? In Proceedings of the 14th International Conference Efficiency and Responsibility in Education 2017; Houška, M., Ed.; Czech University of Life Sciences Prague: Prague, Czech Republic, 2017; pp. 262–269. [Google Scholar]
- Moraová, H.; Novotná, J. Mathematics lessons in culturally and linguistically heterogeneous classrooms. In Proceedings of the 15th International Conference Efficiency and Responsibility in Education 2018; Fejfar, J., Fejfarová, M., Flégl, M., Houška, M., Husák, J., Krejčí, I., Urbancová, H., Eds.; Czech University of Life Sciences Prague: Prague, Czech Republic, 2018; pp. 221–228. [Google Scholar]
- Ulovec, A.; Moraová, H.; Favilli, F.; Grevholm, B.; Novotná, J.; Piccione, M. Multiculturalism in theory and teachers’ practice. In International Symposium Elementary Maths Teaching SEMT ’13. Proceedings; Novotná, J., Moraová, H., Eds.; Univerzita Karlova, Pedagogická Fakulta: Praha, Czech Republic, 2013; pp. 322–330. [Google Scholar]
- Moraová, H.; Novotná, J.; Favilli, F. Ornaments and tessellations—encouraging creativity in mathematics classroom. In Mathematical Creativity and Mathematical Giftedness: Enhancing Creative Capacities in Mathematically Promising Students; Singer, M., Ed.; Springer: Cham, Switzerland, 2018; pp. 253–284. [Google Scholar] [CrossRef]
- Hošpesová, A.; Tichá, M.; Macháčková, J. Differences and similarities in (qualified) pedagogical reflection. In Proceedings of CERME5; Pitta-Pantazi, D., Philippou, G., Eds.; University of Cyprus: Nicosia, Cyprus, 2005; pp. 1906–1915. [Google Scholar]
- Scheerens, J.; Hendriks, M.; Luyten, H.; Sleegers, P.; Steen, R. Teachers’ Professional Development: Europe in International Comparison. An Analysis of Teachers’ Professional Development Based on the OECD’s Teaching and Learning International Survey (TALIS); European Commission Publication: Luxembourg, Belgium, 2010. [Google Scholar] [CrossRef]
- Brousseau, G. Theory of Didactical Situations in Mathematics; Dordrecht: Kluwer, Switzerland, 1997. [Google Scholar]
- Fennema, E.; Franke, M.L. Teachers’ knowledge and its impact. In Handbook of Research on Mathematics Teaching and Learning; Grows, D.A., Ed.; MacMillan: New York, NY, USA, 1992; pp. 147–165. [Google Scholar]
- Ball, D.L.; Thames, M.H.; Phelps, G. Content knowledge for teaching: What makes it special? J. Teach. Educ. 2008, 59, 389–407. [Google Scholar] [CrossRef]
- Krainer, K. Some considerations on problems and perspectives of in service mathematics teacher education. In 8th International Congress on Mathematics Education: Selected Lectures; Alsina, C., Ed.; SAEM Thales: Seville, Spain, 1996; pp. 303–321. [Google Scholar]
- Švec, V. Student’s self-reflection during the undergraduate didactic education. Pedagogika 1996, 46, 266–276. (In Czech) [Google Scholar]
- Scherer, P.; Steinbring, H. The Professionalisation of Mathematics Teachers’ Knowledge—Teachers Commonly Reflect Feedbacks to Their Own Instruction Activity. 2003. Available online: http://www.dm.unipi.it/~didattica/CERME3/WG11/papers_pdf/TG11_Scherer.pdf (accessed on 10 March 2021).
- Climent, N.; Carrillo, J. Developing and researching professional knowledge with primary teachers. In CERME 2. European Research in Mathematics Education II, Part 1; Novotná, J., Ed.; Charles University, Faculty of Education: Prague, Czech Republic, 2001; pp. 269–280. [Google Scholar]
- Jaworski, B. Research practice into/influencing mathematics teaching and learning development: Towards a theoretical framework based on co-learning partnerships. Educ. Stud. Math. 2003, 54, 249–282. [Google Scholar] [CrossRef]
- Mason, J. Noticing: Roots and branches. In Mathematics Teacher Noticing: Seeing through Teachers’ Eyes; Sherin, M.G., Jacobs, V.R., Philipp, R.A., Eds.; Taylor & Francis: New York, NY, USA; London, UK, 2011; pp. 35–50. [Google Scholar]
- van Es, E.A.; Sherin, M.G. Mathematics teachers’ ‘learning to notice’ in the context of a video club. Teach. Teach. Educ. 2008, 24, 244–276. [Google Scholar] [CrossRef]
- Sherin, M.G.; Russ, R.S.; Colestock, A.A. Assessing mathematics teachers’ in-the-moment noticing. In Mathematics Teacher Noticing: Seeing through Teachers’ Eyes; Sherin, M.G., Jacobs, V.R., Philipp, R.A., Eds.; Taylor & Francis: London, UK, 2011; pp. 79–94. [Google Scholar]
- Fujii, T. Designing and adapting tasks in lesson planning: A critical process of Lesson Study. ZDM 2016, 48, 411–423. [Google Scholar] [CrossRef]
- Lawrence, C.A.; Chong, W.H. Teacher collaborative learning through the lesson study: Identifying pathways for instructional success in a Singapore high school. Asia Pac. Educ. Rev. 2010, 11, 565–572. [Google Scholar] [CrossRef]
- Lewis, J.M.; Fischman, D.; Riggs, I.; Wasserman, K. Teacher learning in Lesson Study. Mont. Math. Enthus. 2013, 10, 583–620. [Google Scholar]
- Pang, M.F. The use of learning study to enhance teacher professional learning in Hong Kong. Teach. Educ. 2006, 17, 27–42. [Google Scholar] [CrossRef]
- White, A.L.; Lim, C.S. Lesson study in Asia Pacific classrooms: Local responses to a global movement. ZDM 2008, 40, 915–925. [Google Scholar] [CrossRef]
- Beisiegel, M.; Mitchell, R.; Hill, H.C. The design of video-based professional development: An exploratory experiment intended to identify effective features. J. Teach. Educ. 2018, 69, 69–89. [Google Scholar] [CrossRef]
- Borko, H.; Jacobs, J.; Eiteljorg, E.; Pittman, M.E. Video as a tool for fostering productive discussions in mathematics professional development. Teach. Teach. Educ. 2008, 24, 417–436. [Google Scholar] [CrossRef]
- Jacobs, V.R.; Lamb, L.L.C.; Philipp, R.A. Professional noticing of children’s mathematical thinking. J. Res. Math. Educ. 2010, 41, 169–202. [Google Scholar] [CrossRef]
- Sherin, M.G.; van Es, E.A. Using video to support teachers’ ability to notice classroom interactions. J. Technol. Teach. Educ. 2005, 13, 475–491. [Google Scholar]
- Putnam, R.T.; Borko, H. What do new views of knowledge and thinking have to say about research on teacher learning? Educ. Res. 2000, 29, 4–15. [Google Scholar] [CrossRef]
- Little, J.W. Locating learning in teachers’ communities of practice: Opening up problems of analysis in records of everyday work. Teach. Teach. Educ. 2002, 18, 917–946. [Google Scholar] [CrossRef]
- Star, J.; Lynch, K.; Perova, N. Using video to improve preservice mathematics teachers’ abilities to attend to classroom features: A replication study. In Mathematics Teacher Noticing: Seeing through Teachers’ Eyes; Sherin, M.G., Jacobs, V.R., Philipp, A.R., Eds.; Taylor & Francis: New York, NY, USA, 2011; pp. 117–133. [Google Scholar]
- OECD. Teacher enthusiasm. In PISA 2018 Results (Volume III): What School LIFE means for Students’ Lives; OECD Publishing: Paris, France, 2020; pp. 87–95. [Google Scholar] [CrossRef]
- Eisenmann, P.; Novotná, J.; Přibyl, J. Culture of solving problems—One approach to assessing students’ culture of mathematics problem solving. In Aplimat 2014—13th Conference on Applied Mathematics; Ve-lichová, D., Ed.; Publishing House of STU: Bratislava, Slovak, 2014; pp. 115–122. [Google Scholar]
- Brousseau, G.; Novotná, J. La culture scolaire des problèmes de mathé-matiques. In Les Didactiques et Leurs Rapports à l‘ Enseignement et à la Formation. Quel Statut Epistémologique de Leurs Modèles et de Leurs Résultats? Sarrazy, B., Ed.; AFIRSE: Bordeaux, France, 2008; Available online: http://people.fjfi.cvut.cz/novotant/jarmila.novotna/2008Brousseau_Novotna.pdf (accessed on 15 March 2021).
- Polya, G. How to Solve It: A New Aspect of Mathematical Method; Princeton University Press: Princeton, NJ, USA, 2004. [Google Scholar]
- Schoenfeld, A.H. Mathematical Problem Solving; Academic Press Inc: London, UK, 1985. [Google Scholar]
- Kilpatrick, J. Problem formulating: Where do good problems come from? In Cognitive Science and Mathematics Education; Schoenfeld, A.H., Ed.; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1987; pp. 123–147. [Google Scholar]
- Silver, E.A.; Cai, J. An analysis of arithmetic problem posing by middle school students. J. Res. Math. Educ. 1996, 27, 521–539. [Google Scholar] [CrossRef]
- English, L.D. The development of fifth-grade children’s problem-posing abilities. Educ. Stud. Math. 1997, 34, 183–217. [Google Scholar] [CrossRef]
- Pittalis, M.; Christou, C.; Mousolides, N.; Pitta-Pantazi, D. A structural model for problem posing. In Proceedings of 28th PME; Hoines, M.J., Bishop, A.J., Eds.; Bergen University College: Bergen, Norway, 2004; pp. 49–56. [Google Scholar]
- Singer, F.M.; Ellerton, N.; Cai, J. Mathematical Problem Posing: From Research to EFFECTIVE practice; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar] [CrossRef]
- Tichá, M. Auf den Spuren der Entdeckung der Mathematik in der Alltagssituationen. In Beiträge zum Mathematikunterricht. Vorträge auf der 35. Tagung für Didaktik der Mathematik; Haiser, G., Ed.; Franzbecker: Hildesheim/Berlin, Germany, 2001; pp. 616–619. [Google Scholar]
- Koman, M.; Tichá, M. On travelling together and sharing expenses. Teach. Math. Appl. 1998, 17, 117–122. [Google Scholar]
- Tichá, M.; Koman, M. Towards developing teachers’ ability for grasping situations. In Proceedings of the International Conference “Teachers and their University Education at the Turn of the Millennium”; Kohnová, J., Ed.; Charles University: Praha, Czech Republic, 2000; pp. 300–306. [Google Scholar]
- Coyle, D. Theory and planning for effective classrooms: Supporting students in content and language integrated learning contexts. In Learning through a Foreign Language: Models, Methods and Outcomes; Masih, J., Ed.; CILT: London, UK, 1999; pp. 46–62. [Google Scholar]
- Moraová, H.; Novotná, J.; Favilli, F. Teaching mathematics in culturally heterogeneous classrooms: What do teachers really need? E-pedagogium 2015, 1, 34–44. (In Czech) [Google Scholar] [CrossRef]
- Wittmann, E.C. Mathematics education as a “Design Science”. Educ. Stud. Math. 1995, 29, 355–374. [Google Scholar] [CrossRef]
- Minaříková, E.; Janík, T. Teachers’ professional vision: Exploring and clarifying the concept. Pedagog. Orientace 2012, 22, 181–204. [Google Scholar] [CrossRef]

| Ref. | Author(s) | Research Aim (Strands of Research in Bold) | Research Design (Qualitative, Quantitative, Mixed; Sample) |
|---|---|---|---|
| [11] | Vondrová | Creating a learning community influencing the participating ISTs via lesson study and video-based tasks | Quali (13 ISTs) |
| [12] | Vondrová, Cachová, Coufalová, and Krátká | ISTs’ perception of their participation in a lesson study cycle and changes in their noticing mathematics-specific phenomena in a lesson and their interpretation | Mixed (13 ISTs) |
| [13] | Vondrová, Novotná, M., Pavlasová, Robová, Stará, and Uličná | The effect of the lesson study cycle and video-based course on PSTs’ professional vision | Mixed (60 PSTs, not only of mathematics) |
| [14] | Simpson and Vondrová | A difference in PSTs’ professional vision when viewing their own videos or public videos and differences between disciplines | Quanti (51 PSTs, not only of mathematics) |
| [15] | Stehlíková (Vondrová) | PSTs’ and ISTs ability to notice in videoed teaching events and differences in their interpretations | Mixed (119 PSTs and ISTs) |
| [16] | Simpson, Vondrová, and Žalská | Development of PSTs’ professional vision due to their teaching experience or a video-club | Quanti (82, resp. 32 PSTs) |
| [17] | Tichá and Hošpesová | The development of ISTs’ competence to reflect | Quali (7 ISTs) |
| [18] | Vondrová and Žalská | PSTs’ ability to notice mathematics-specific phenomena and to interpret them | Mixed (30 PSTs) |
| [19] | Pavlasová, Stará, Vondrová, Novotná, Robová, and Uličná | The level of professional vision of PSTs at the beginning of their two years master’s studies | Quanti (211 PSTs, not only of mathematics) |
| [20] | Vondrová and Žalská | PSTs’ ability to notice mathematics-specific phenomena in a mathematics lesson on video and the differences between PSTs at the beginning and end of their master’s study | Mixed (169 PSTs) |
| [21] | Vondrová | The effect of a video-based intervention on PSTs’ knowledge-based reasoning as part of professional vision, the influence of the lesson observed | Mixed (32 PSTs) |
| [22] | Eisenmann, Novotná, Přibyl, and Břehovský | ISTs’ problem solving and problem posing ability to serve the needs of a multi-cultural class | Mixed (62 pupils, 3 ISTs) |
| [23] | Tichá and Hošpesová | PSTs’ problem posing as a diagnostic tool in the area of fraction | Quali (30 PSTs) |
| [24] | Hošpesová and Tichá | PSTs’ ability to use their content knowledge in problem posing and their perception of the problems posed | Quali (30 PSTs) |
| [25] | Tichá and Hošpesová | Problem posing as a possible method leading to the development of PSTs’ pedagogical content knowledge | Quali (35 PSTs) |
| [26] | Novotná, Hadj-Moussová, and Hofmannová | PSTs’ professional competences to teach in CLIL (content and language integrated learning) | Quali (PSTs) |
| [27] | Moraová and Novotná | PSTs’ subject-didactic competence to plan a lesson for a L2 (additional language) class | Mixed (16 PSTs) |
| [28] | Moraová and Novotná | ISTs’ subject-didactic competence in adapting to teaching in culturally and linguistically heterogeneous classrooms | Quali (ISTs) |
| [29] | Ulovec, Moraová, Favilli, Grevholm, Novotná, and Piccione | ISTs’ subject-didactic competence needed to teach in culturally and linguistically heterogeneous classrooms | Mixed (124 respondents) |
| [30] | Moraová, Novotná, and Favilli | ISTs’ subject-didactic competence in designing teaching units to support immigrant pupils and pupils from different sociocultural backgrounds | Quali (ISTs) |
| [31] | Hošpesová, Tichá, and Macháčková | The changes in reflections related to individual interests of the participants | Quali (2 researchers, 1 IST) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hošpesová, A.; Novotná, J.; Vondrová, N.; Moraová, H.; Tichá, M. From Teacher of Nations to Teacher of Mathematics. Mathematics 2021, 9, 1583. https://doi.org/10.3390/math9141583
Hošpesová A, Novotná J, Vondrová N, Moraová H, Tichá M. From Teacher of Nations to Teacher of Mathematics. Mathematics. 2021; 9(14):1583. https://doi.org/10.3390/math9141583
Chicago/Turabian StyleHošpesová, Alena, Jarmila Novotná, Naďa Vondrová, Hana Moraová, and Marie Tichá. 2021. "From Teacher of Nations to Teacher of Mathematics" Mathematics 9, no. 14: 1583. https://doi.org/10.3390/math9141583
APA StyleHošpesová, A., Novotná, J., Vondrová, N., Moraová, H., & Tichá, M. (2021). From Teacher of Nations to Teacher of Mathematics. Mathematics, 9(14), 1583. https://doi.org/10.3390/math9141583








