Coupling the Cell Method with the Boundary Element Method in Static and Quasi–Static Electromagnetic Problems
Abstract
:1. Introduction
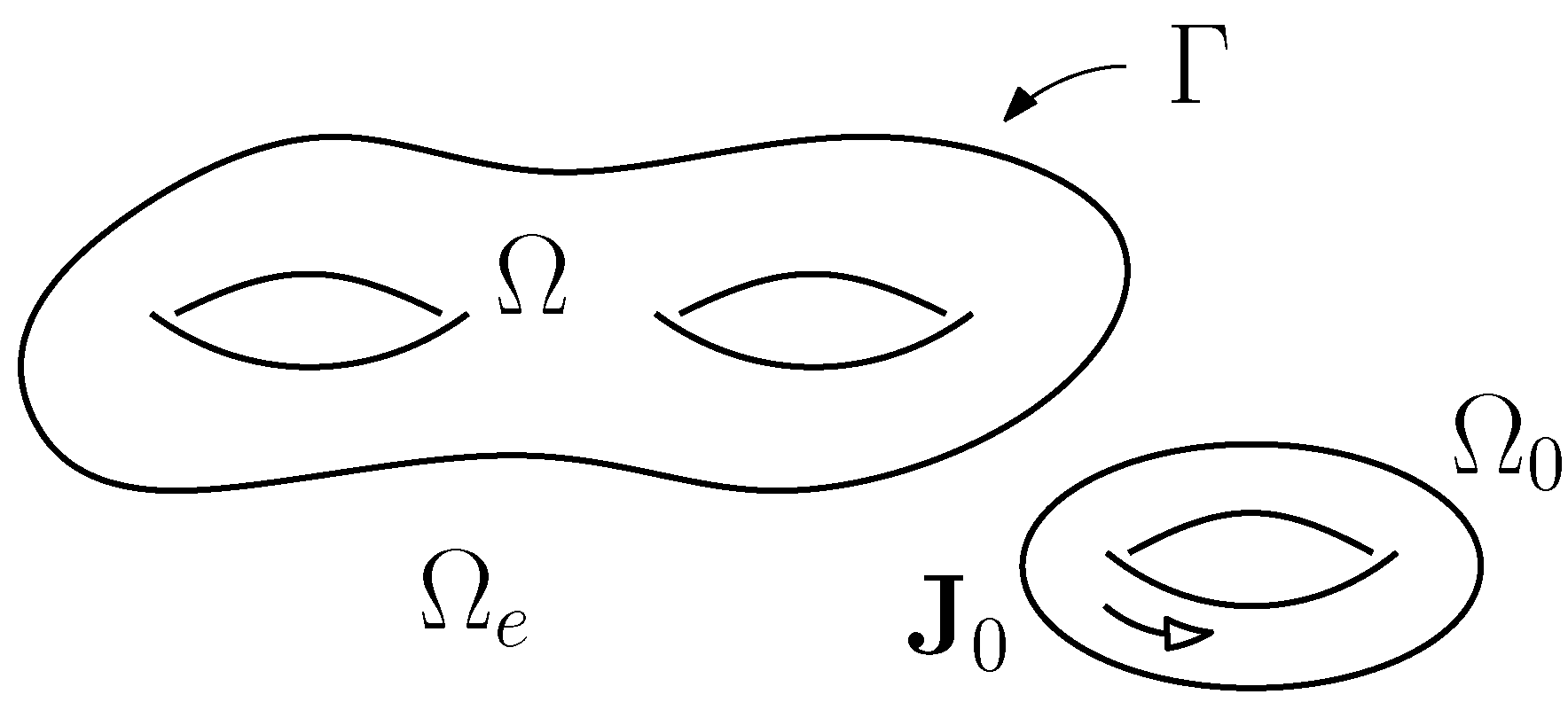
2. Electromagnetic Field Problems
2.1. Interior Problem
2.2. Interface Conditions
2.3. Exterior Problem
2.4. Direct Approach
2.5. Indirect Approach
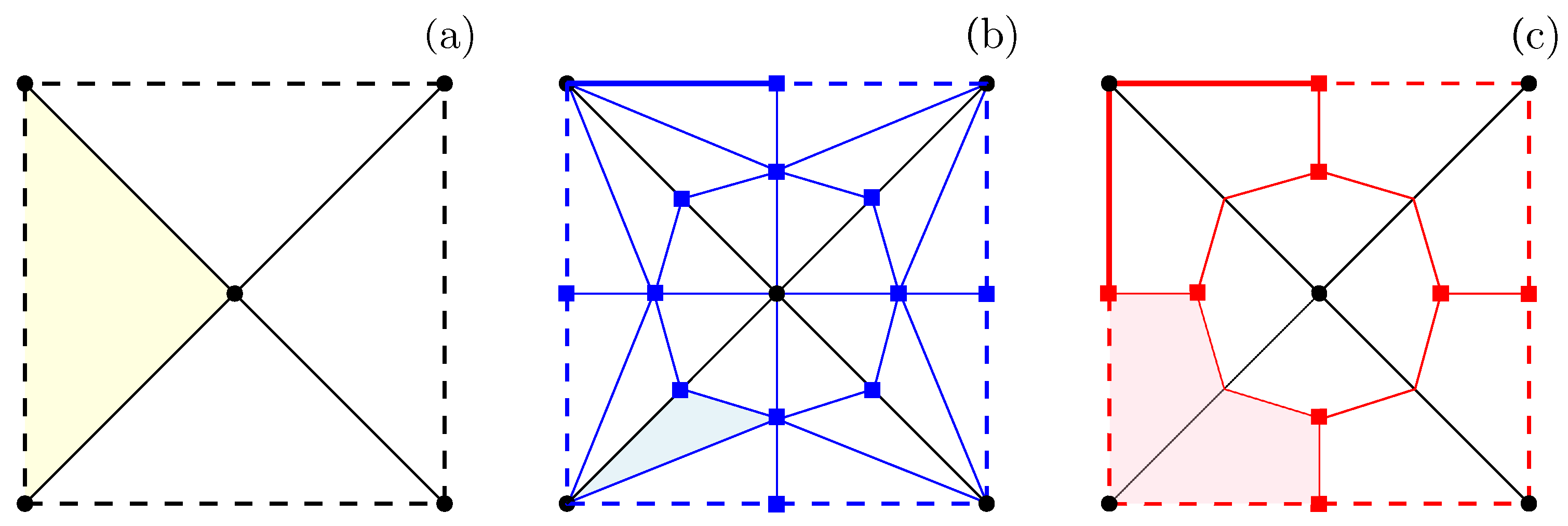
3. Cell Method with Augmented Dual Grid
3.1. Discrete Field Variables
3.2. Topological Operators
3.3. Discrete Constitutive Relations
4. Boundary Element Method
4.1. Direct Approach
4.2. Indirect Approach
5. Hydrid Formulations
5.1. Unsymmetric Formulation
5.2. Symmetric Formulation
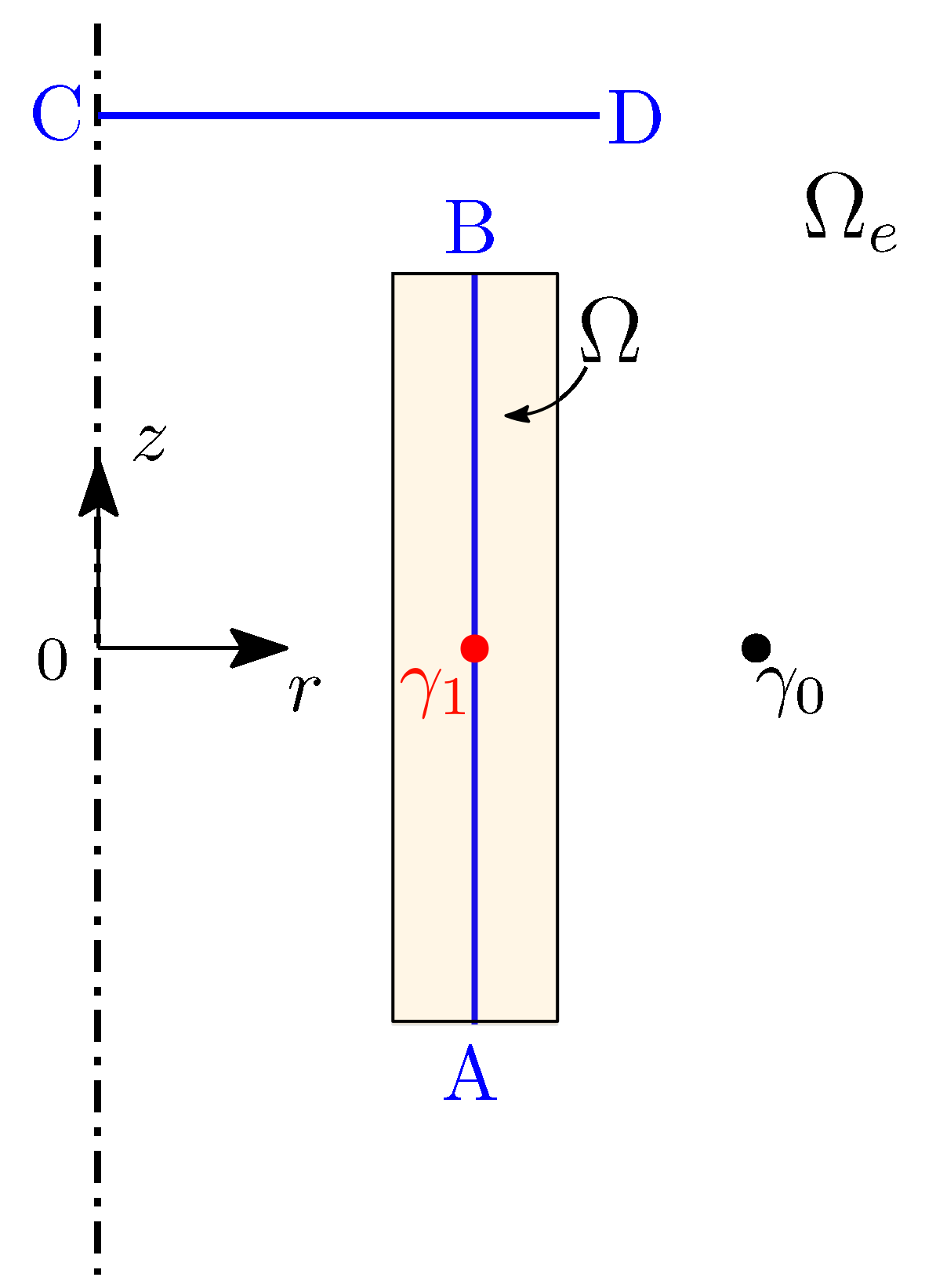
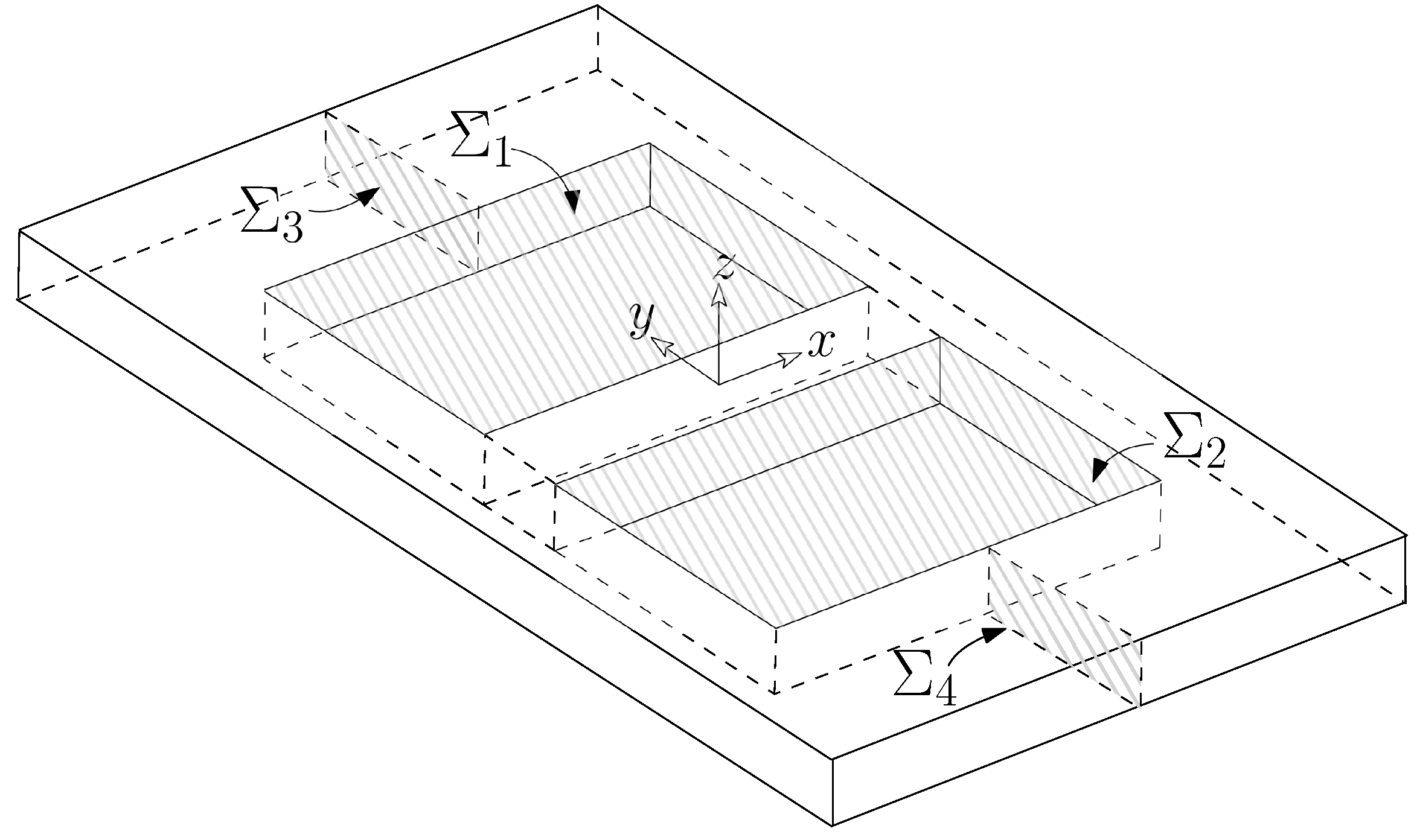
5.3. Multiply-Connected Domains
6. Numerical Results
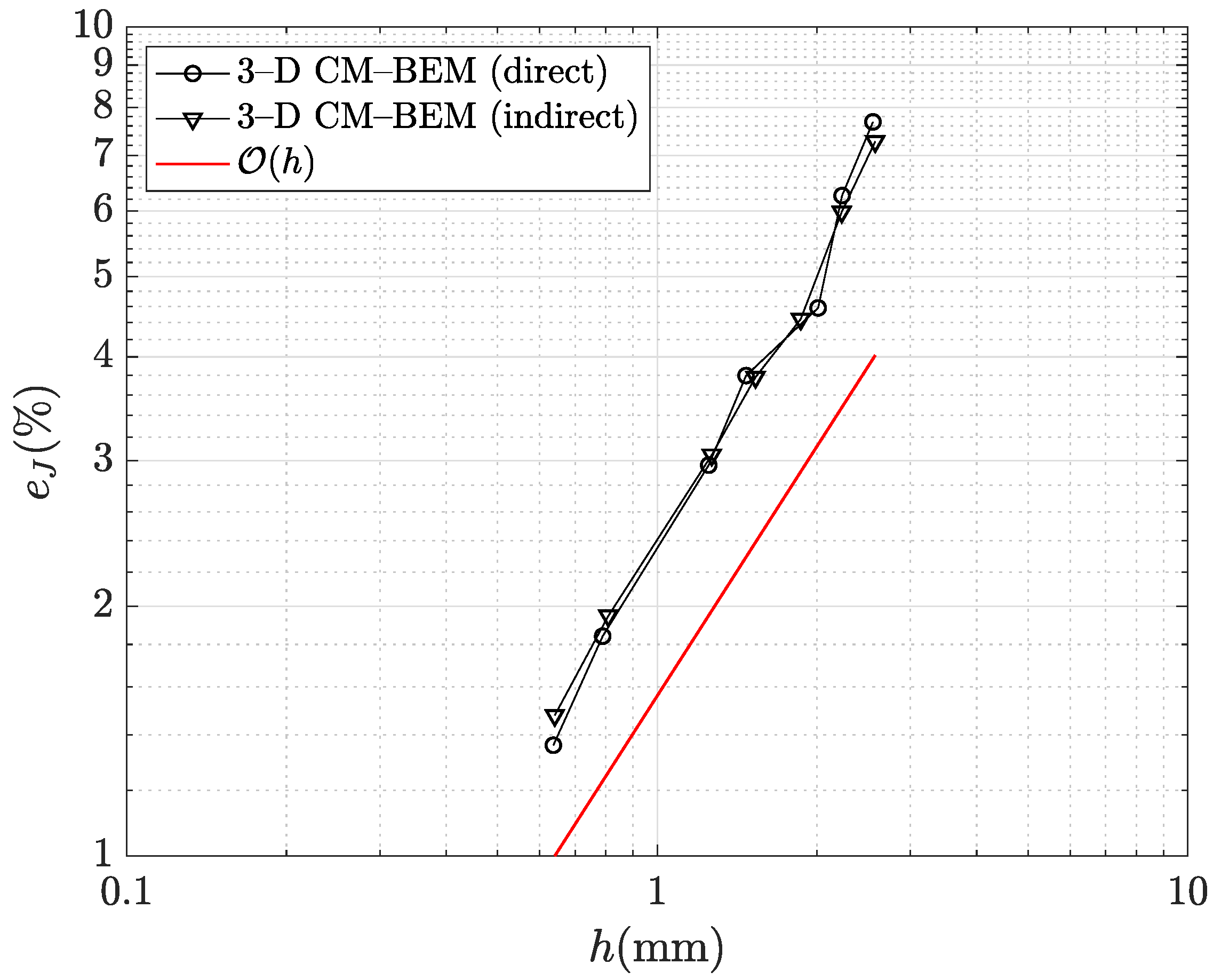
6.1. Axisymmetric Inductor
6.2. Bath Plate
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chen, Q.; Konrad, A. A review of finite element open boundary techniques for static and quasi-static electromagnetic field problems. IEEE Trans. Magn. 1997, 33, 663–676. [Google Scholar] [CrossRef]
- Bossavit, A. Computational Electromagnetism: Variational Formulations, Complementarity, Edge Elements; Academic Press: San Diego, CA, USA, 1998. [Google Scholar] [CrossRef]
- Tonti, E. A Direct Discrete Formulation of Field Laws: The Cell Method. Comput. Model. Eng. Sci. 2001, 2, 237–258. [Google Scholar]
- Tonti, E. Why starting from differential equations for computational physics? J. Comput. Phys. 2014, 257, 1260–1290. [Google Scholar]
- Codecasa, L.; Minerva, V.; Politi, M. Use of barycentric dual grids for the solution of frequency domain problems by FIT. IEEE Trans. Magn. 2004, 40, 1414–1419. [Google Scholar]
- Codecasa, L.; Politi, M. Explicit, Consistent, and Conditionally Stable Extension of FD-TD to Tetrahedral Grids by FIT. IEEE Trans. Magn. 2008, 44, 1258–1261. [Google Scholar]
- Codecasa, L.; Trevisan, F. Constitutive equations for discrete electromagnetic problems over polyhedral grids. J. Comput. Phys. 2007, 225, 1894–1918. [Google Scholar]
- Giuffrida, C.; Gruosso, G.; Repetto, M. Finite formulation of nonlinear magnetostatics with Integral boundary conditions. IEEE Trans. Magn. 2006, 42, 1503–1511. [Google Scholar]
- Gruosso, G.; Repetto, M. Magnetostatic solution by hybrid technique and fast multipole method. Phys. B Condens. Matter 2008, 403, 368–371. [Google Scholar]
- Alotto, P.; Gruosso, G.; Moro, F.; Repetto, M. A Boundary Integral Formulation for Eddy Current Problems Based on the Cell Method. IEEE Trans. Magn. 2008, 44, 770–773. [Google Scholar]
- Codecasa, L. Refoundation of the Cell Method Using Augmented Dual Grids. IEEE Trans. Magn. 2014, 50, 497–500. [Google Scholar]
- Moro, F.; Codecasa, L. Indirect Coupling of the Cell Method and BEM for Solving 3D Unbounded Magnetostatic Problems. IEEE Trans. Magn. 2016, 52. [Google Scholar]
- Moro, F.; Codecasa, L. A 3D Hybrid Cell Method for Induction Heating Problems. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar]
- Moro, F.; Codecasa, L. A 3D Hybrid Cell Boundary Element Method for Time-Harmonic Eddy Current Problems on Multiply Connected Domains. IEEE Trans. Magn. 2019, 55. [Google Scholar]
- Hiptmair, R.; Ostrowski, J. Coupled boundary-element scheme for eddy-current computation. J. Eng. Math. 2005, 51, 231–250. [Google Scholar]
- Rodríguez, A.A.; Bertolazzi, E.; Ghiloni, R.; Valli, A. Construction of a Finite Element Basis of the First de Rham Cohomology Group and Numerical Solution of 3D Magnetostatic Problems. SIAM J. Numer. Anal. 2013, 51, 2380–2402. [Google Scholar]
- Hiptmair, R.; Sterz, O. Current and voltage excitations for the eddy–current model. Int. J. Numer. Model. Electron. Netw. Devices Fields 2005, 18, 1–21. [Google Scholar]
- Folland, G. Introduction to Partial Differential Equations; Princeton University Press: Princeton, NJ, USA, 1995. [Google Scholar]
- Brebbia, C.; Butterfield, R. Formal equivalence of direct and indirect boundary element methods. Appl. Math. Model. 1978, 2, 132–134. [Google Scholar] [CrossRef]
- Rucker, W.M.; Richter, K.R. Three-dimensional magnetostatic field calculation using boundary element method. IEEE Trans. Magn. 1988, 24, 23–26. [Google Scholar] [CrossRef]
- Ren, Z.; Bouillault, F.; Razek, A.; Vérité, J. Comparison of different boundary integral formulations when coupled with finite elements in three dimensions. IEE Proc. (Phys. Sci. Meas. Instrum. Manag. Educ. Rev.) 1988, 135, 501–507. [Google Scholar] [CrossRef]
- Graciani, E.; Mantič, V.; París, F.; Cañas, J. A critical study of hypersingular and strongly singular boundary integral representations of potential gradient. Comput. Mech. 2000, 25, 542–559. [Google Scholar] [CrossRef]
- Costabel, M. Principles of boundary element methods. Comput. Phys. Rep. 1987, 6, 243–274. [Google Scholar] [CrossRef]
- Andjelic, Z.; Of, G.; Steinbach, O.; Urthaler, P. Boundary element methods for magnetostatic field problems: A critical view. Comput. Vis. Sci. 2011, 14, 117–130. [Google Scholar] [CrossRef]
- Moro, F.; Smajic, J.; Codecasa, L. A Novel h–φ Approach for Solving Eddy–Current Problems in Multiply Connected Regions. IEEE Access 2020, 8, 170659–170671. [Google Scholar] [CrossRef]
- Moro, F.; Codecasa, L. Domain Decomposition With Non-Conforming Polyhedral Grids. IEEE Access 2021, 9, 1465–1481. [Google Scholar] [CrossRef]
- Zhou, P.B. Numerical Analysis of Electromagnetic Fields; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Bossavit, A.; Vérité, J. The “TRIFOU” Code: Solving the 3D eddy-currents problem by using H as state variable. IEEE Trans. Magn. 1983, 19, 2465–2470. [Google Scholar] [CrossRef]
- Rodger, D. Benchmark problem 3 (the Bath Plate). COMPEL 1988, 7, 47–63. [Google Scholar]
Short Biography of Authors
 | Federico Moro received the Laurea degree in Electrical Engineering (2003), the Ph.D. degree in Bioelectromagnetic and Electromagnetic Compatibility (2007), and the B.S. degree in Mathematics (2012) from the University of Padova, Italy. He has been a Visiting Student at the Department of Physics, Swansea University, Wales, UK (2005) and a Visiting Professor at the G2ELab, Grenoble, France (2020). He was awarded the best oral presentation at UPEC 2006 and the best paper at ASME IDETC/CIE 2017 and Electrimacs 2019 conferences. He obtained the National Scientific Qualification as Full Professor (09/E1-Elettrotecnica) in 2021. From 2007 to 2010 he was a Research Associate at the Department of Electrical Engineering, University of Padova. From 2010 to 2020 he was an Assistant Professor of Electrical Engineering at the Department of Industrial Engineering of the same university. Since 2020 he has been working as an Associate Professor of Electrical Engineering at the same department. His research interests include numerical methods for computing electromagnetic problems and the numerical modeling of multiphysics and multiscale problems. He is author of more than 100 articles in peer-reviewed international journals and conference proceedings. |
 | Lorenzo Codecasa received the Ph.D. degree in Electronic Engineering from Politecnico di Milano in 2001. From 2002 to 2010 he worked as an Assistant Professor of Electrical Engineering at the Department of Electronics, Information, and Bioengineering of Politecnico di Milano. Since 2010 he has worked as an Associate Professor of Electrical Engineering at the same department. His main research contributions are in the theoretical analysis and in the computational investigation of electric circuits and electromagnetic fields. In his research on heat transfer and thermal management of electronic components, he has introduced original industrial-strength approaches to the extraction of compact thermal models, currently available in market leading commercial software. For these activities, in 2016 he received the Harvey Rosten Award for Excellence. He has been serving as an Associate Editor for the IEEE Transactions of Components, Packaging and Manufacturing Technology. He has also been serving as a Chair of the conference THERMal INvestigation of Integrated Circuits (THERMINIC). In his research areas he has authored or coauthored over 200 papers in refereed international journals and conference proceedings. |


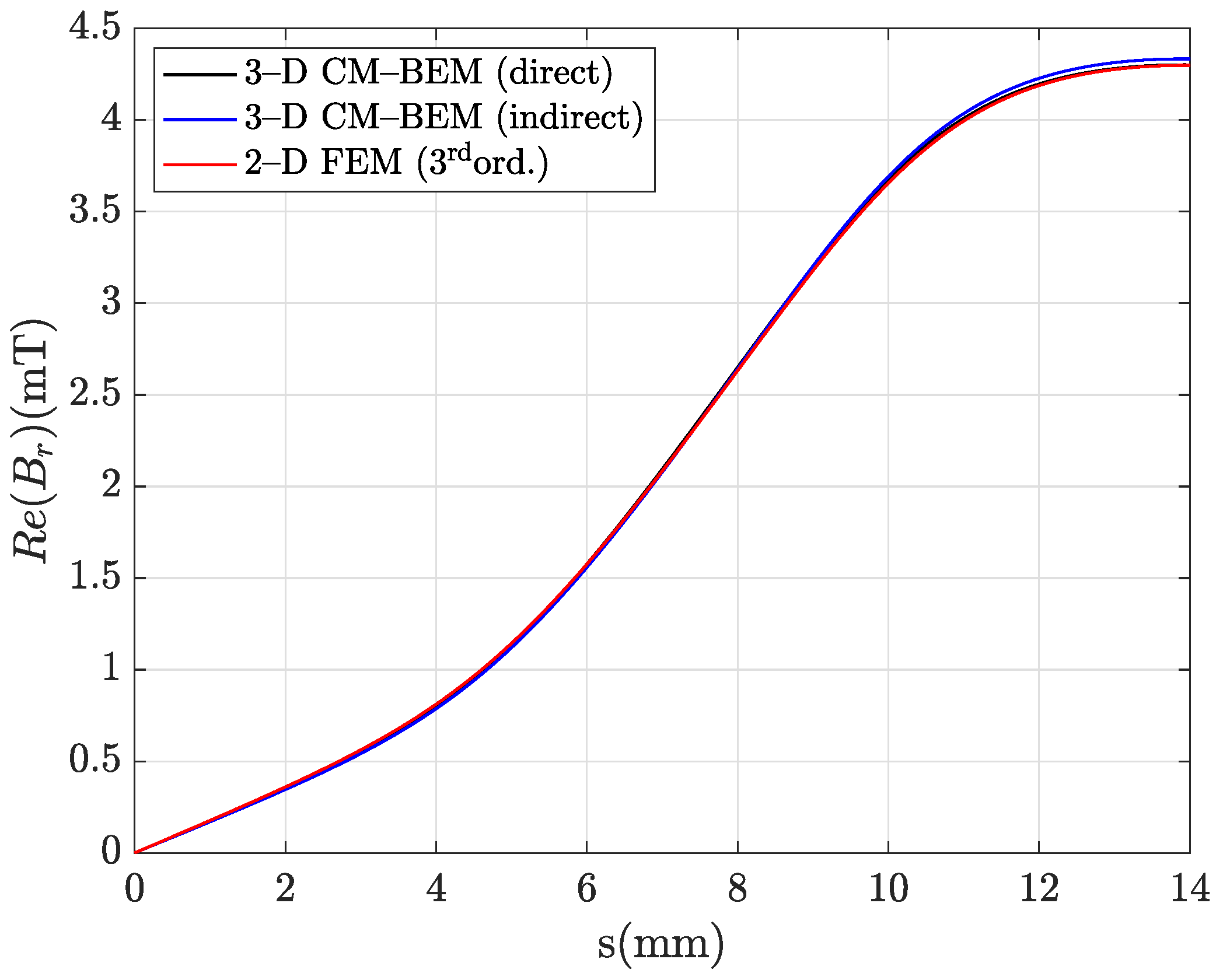
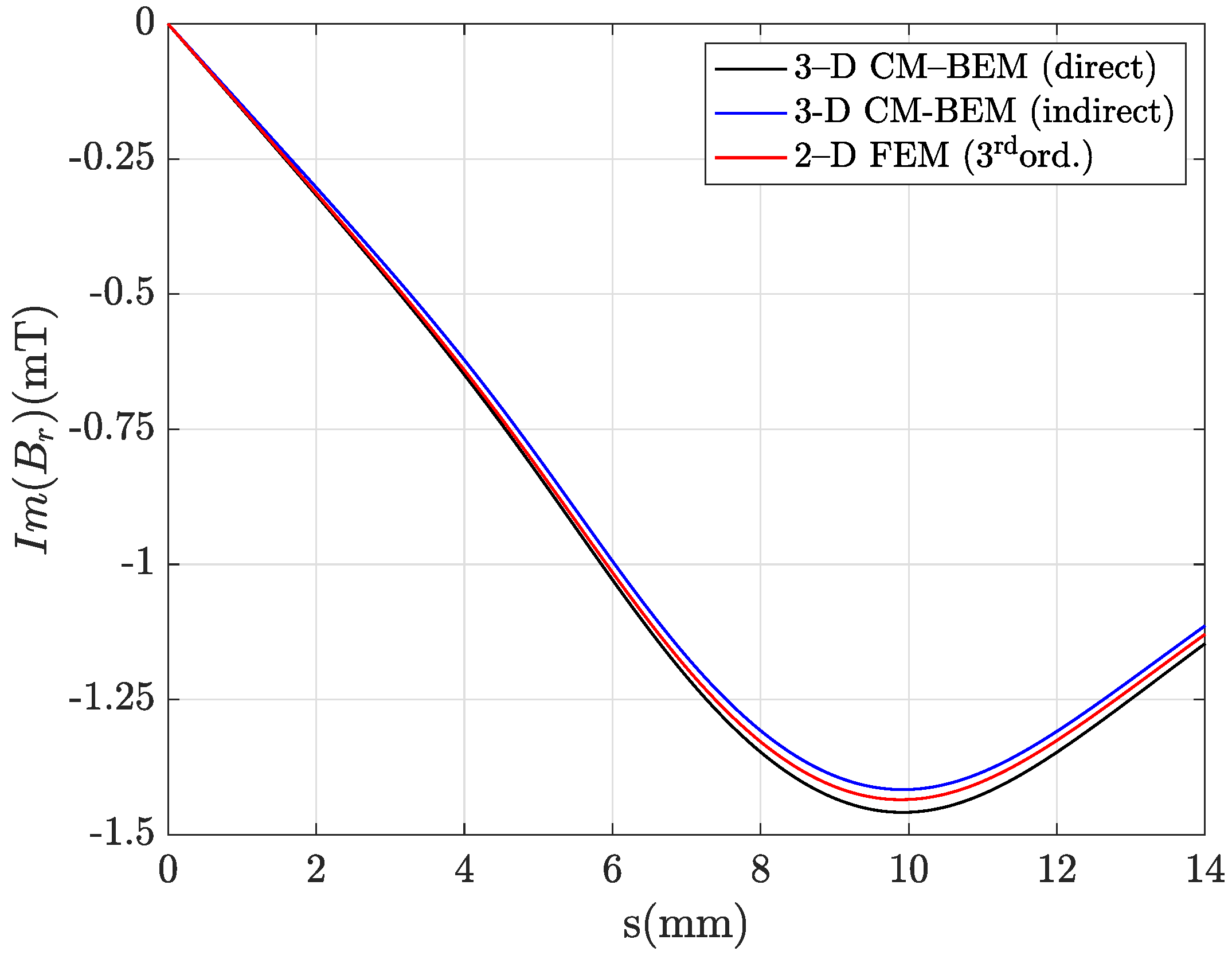




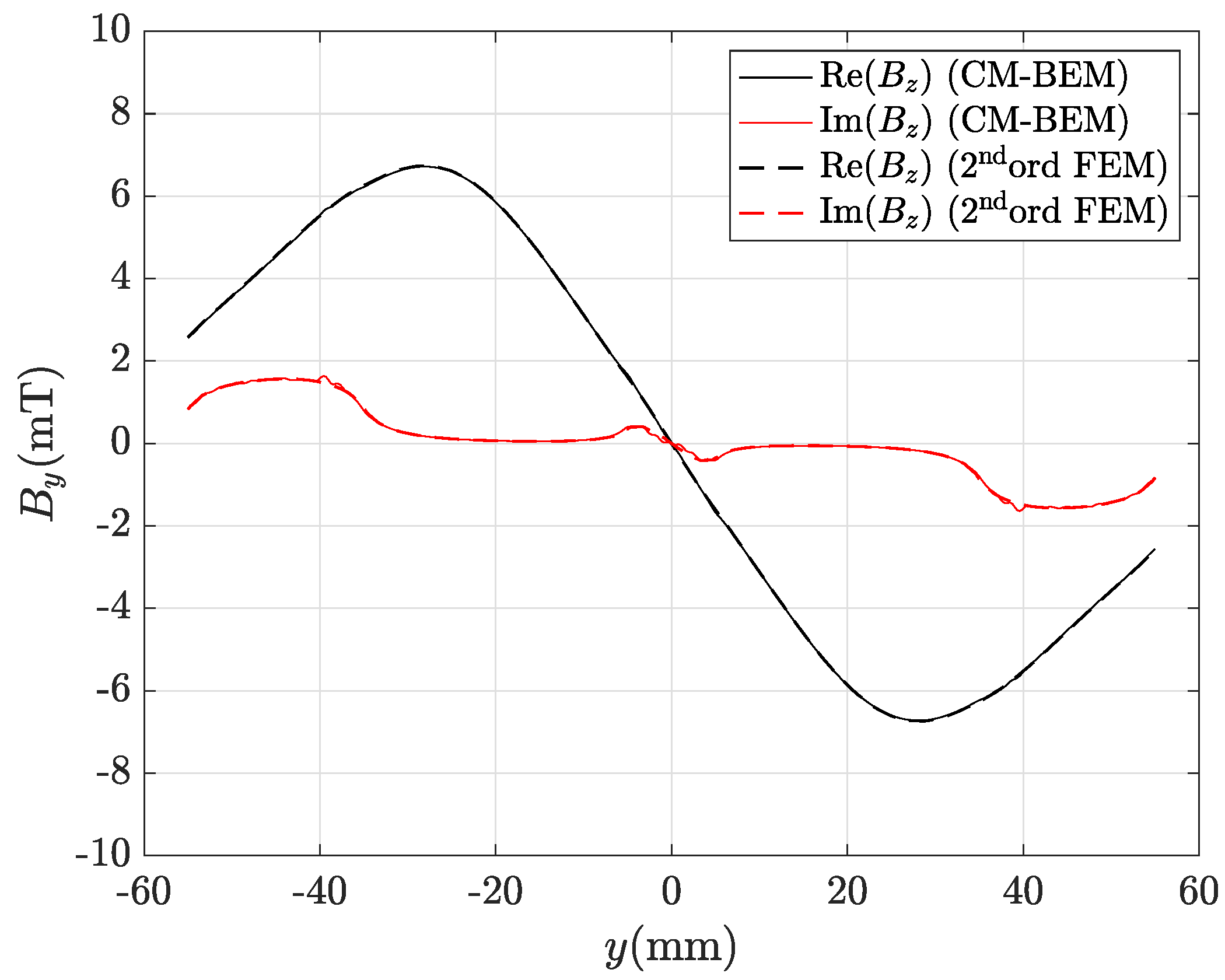
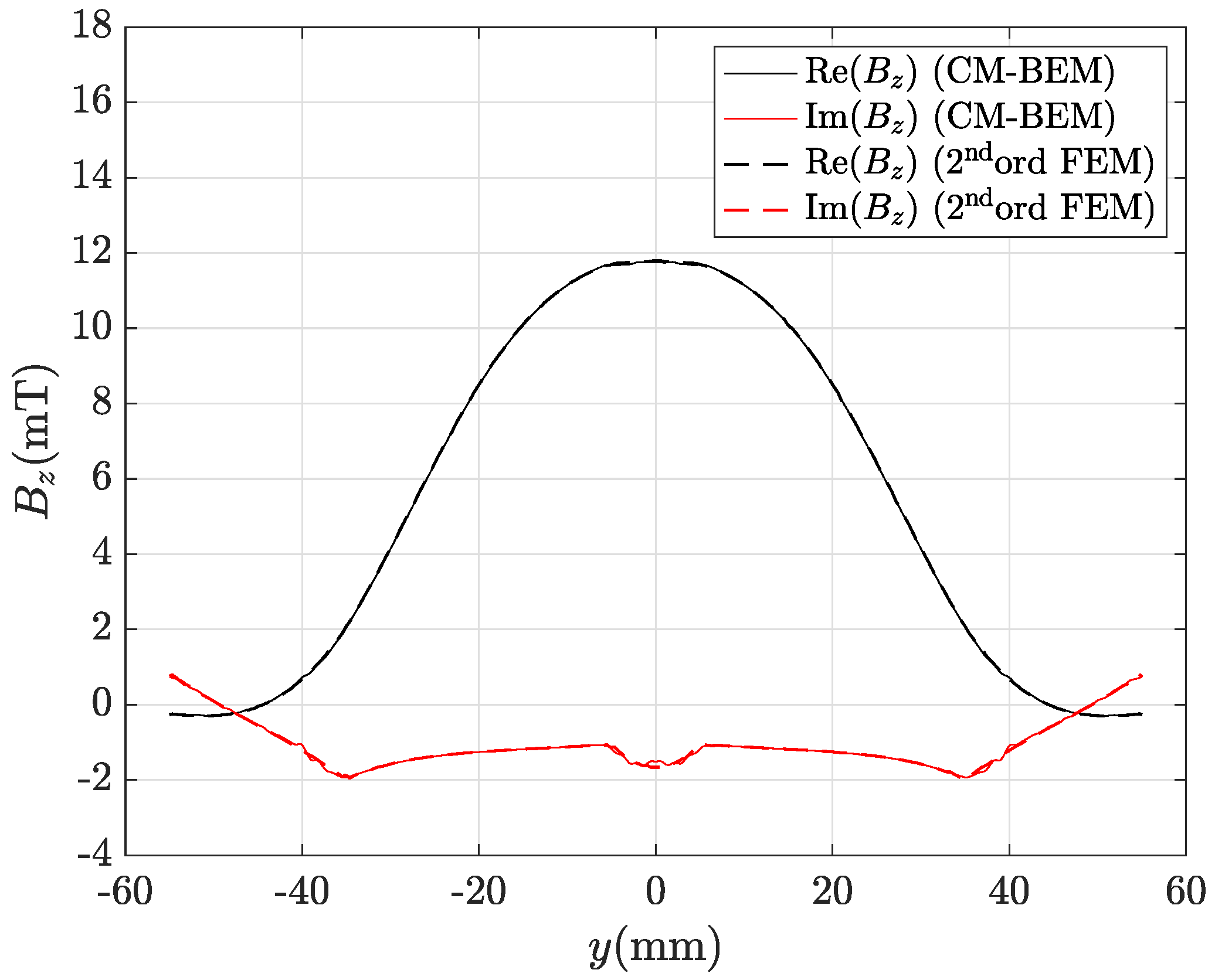

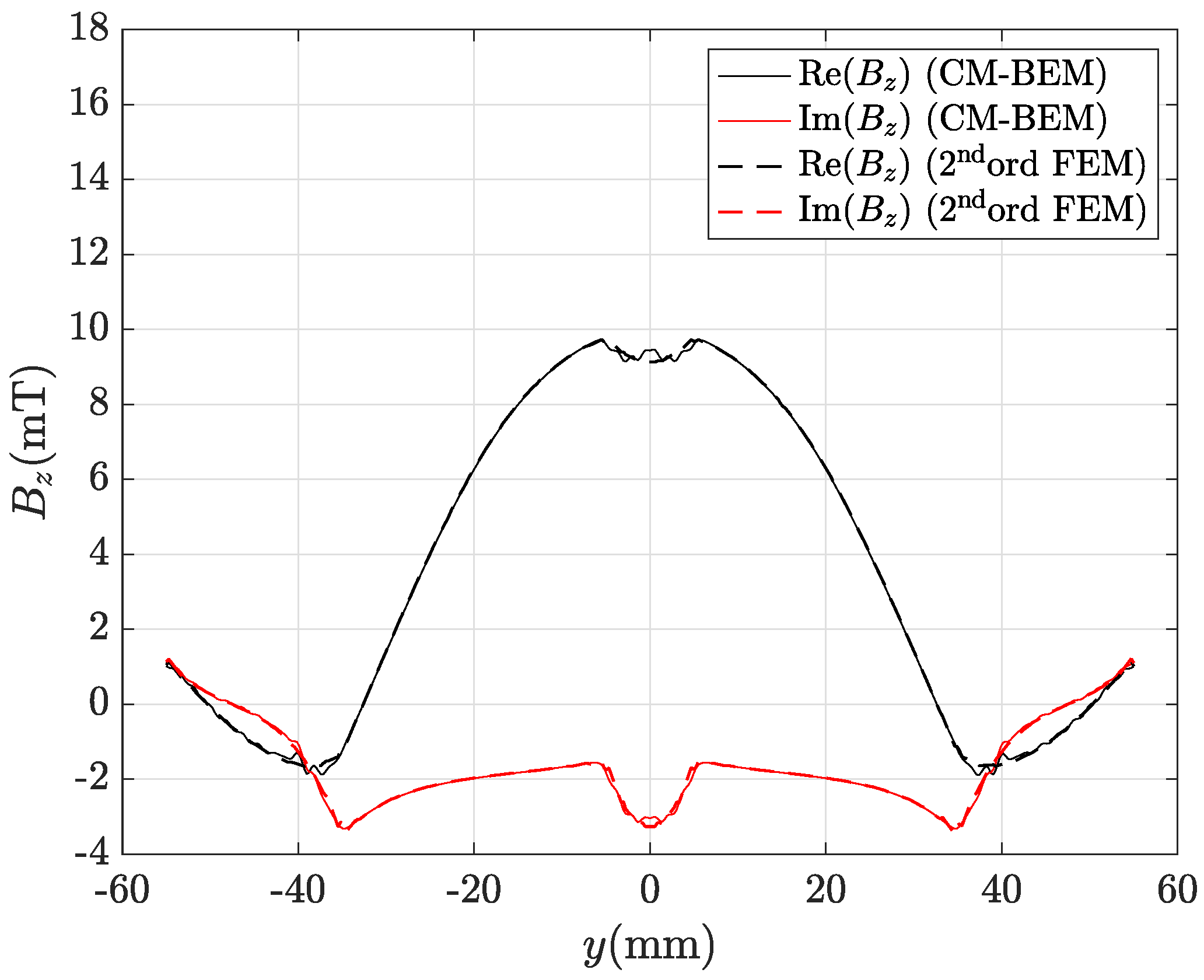
| 50 Hz | 3D CM–BEM | ||||
| FEM (2nd ord.) | |||||
| 200 Hz | 3D CM–BEM | ||||
| FEM (2nd ord.) | |||||
| 50 Hz | 3D CM–BEM | ||||
| FEM (2nd ord.) | |||||
| 200 Hz | 3D CM–BEM | ||||
| FEM (2nd ord.) | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moro, F.; Codecasa, L. Coupling the Cell Method with the Boundary Element Method in Static and Quasi–Static Electromagnetic Problems. Mathematics 2021, 9, 1426. https://doi.org/10.3390/math9121426
Moro F, Codecasa L. Coupling the Cell Method with the Boundary Element Method in Static and Quasi–Static Electromagnetic Problems. Mathematics. 2021; 9(12):1426. https://doi.org/10.3390/math9121426
Chicago/Turabian StyleMoro, Federico, and Lorenzo Codecasa. 2021. "Coupling the Cell Method with the Boundary Element Method in Static and Quasi–Static Electromagnetic Problems" Mathematics 9, no. 12: 1426. https://doi.org/10.3390/math9121426
APA StyleMoro, F., & Codecasa, L. (2021). Coupling the Cell Method with the Boundary Element Method in Static and Quasi–Static Electromagnetic Problems. Mathematics, 9(12), 1426. https://doi.org/10.3390/math9121426







