1. Introduction
The first order system of the piecewise difference equation of the form
for
with a given initial condition
has been considered by several researchers. System (
1) is actually motivated by the Lozi map [
1,
2] which is the system given by
and
, where
and a system
and
given in [
3,
4] or, equivalently, the Devaney’s Gingerbread man map
studied in [
5]. It is known that if the sequences
and
satisfy (
1) and the given initial conditions for all
, then
is called a solution of (
1). Let
be the solutions of (
1) with a given initial condition
. If there exist real numbers
and
and an integer
N such that
for all
, then we say that the solution
eventually becomes the equilibrium point
of (
1). On the other hand, if
p is the smallest positive integer such that
for all
, then we say that the solution
eventually becomes the solution of prime period
p of (
1). For more details about the system of difference equations and their solutions, one may see [
6,
7]. Actually, to establish the stability of the system of difference equations involves derivatives of a function. However, the system (
1) contains absolute value which is not differentiable. Thus, to study the behavior of the solution of (
1), one needs to consider several regions of initial conditions and gather the information to obtain the results.
In 2010, Tikjha et al. [
8] considered (
1) when
and
. They proved that, for a given initial condition
, the solution of (
1) either eventually becomes the solution of prime period 5 or the equilibrium point of (
1). For
,
and
, Grove et al. [
9] showed that for a given initial condition
, the solution of (
1) is either (from the beginning) the equilibrium point or eventually becomes the solution of prime period 3 of (
1). In the doctoral dissertation written by Lapierre [
10], he studied some properties of solutions for 81 possible forms of (
1) where
. Kongtan and Tikjha [
11] let
and
,
and
and proved that the solution of (
1) eventually becomes the solution of prime period 4 of (
1). With
,
,
and
, Tikjha et al. [
12] showed that the solution of (
1) either eventually becomes the solution of prime period 3 or the equilibrium point of (
1) for all initial conditions
. In 2017, Tikjha et al. [
13] considered the case that
,
b and
d in
and proved that the solution of (
1) eventually becomes the equilibrium point of (
1) for all initial conditions
within 6 iterations. In the same year, Tikjha [
14] wrote a manuscript in Thai where, if
,
,
and
, then the solution of (
1) eventually becomes the solution of prime period 4 of (
1). Recently, Tikjha and Piasu [
15] considered
and
with initial condition
being in a specific region in the first quadrant and showed that the solution of (
1) either eventually becomes the equilibrium point or the solution of prime period 4. Tikjha and Lapaierre [
16] also studied (
1) with
and
and the initial condition
is an element in the closed second or fourth quadrant. They proved that the solution of (
1) either eventually becomes the solution of prime period 3 or 4. In addition, Tikjha et al. [
17] proved that, if
,
and
, then under some conditions on
the solution of (
1) eventually becomes the solution of prime period 4.
In this paper, we let
and
. That is, we consider the system
Let us first establish the lemma about the equilibrium of (
2).
Lemma 1. Let .
- (i)
The equilibrium point of (2) is . - (ii)
Let be the solution of (2). Assume that there exists a positive integer N such that and . Then, for all .
Proof. (i) By considering four cases of and , we can solve the system of equations and and the only case that gives the solution is when x and y are negative, which is .
(ii) Assume that there exists a positive integer
N such that
and
. Then,
Since
,
. Thus,
Therefore, by mathematical induction, we have for all . □
In
Section 2 of this article, a global behavior for the case
is proved. We can conclude that all solutions eventually become the equilibrium point
. Local behavior for
with large values of
and
is studied in
Section 3. It is revealed that, locally, all solutions of Equation (
2) for
eventually become the equilibrium point. It can be seen that for
, some solutions have a chance to becomes periodic. Finally, a conclusion and discussion about our work and our conjecture are provided in the last section.
2. Global Behavior for
In this section, we investigate the global behavior where . The first four lemmas deal with the case when or .
Lemma 2. If and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. Let and .
Case 1 . We have
By Lemma 1, we have for all .
Case 2 . We have the same
as in Case 1, while,
and
By Lemma 1, we have for all .
Case 3 . We have the same
as in Case 2, while
By Lemma 1, we have for all .
Case 4 . Then,
. We have the same
as in Case 1, while
Direct computation gives and . By Lemma 1, we have for all .
Case 5 . We have
Similar to Case 4, we can conclude that for all .
Case 6 . Then, . We have the same as in Case 5, while and . By direct computation, we can conclude that for all . □
Lemma 3. If and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. By separate cases as in Lemma 2, we can conclude the behavior of the solution in
Table 1.
□
Lemma 4. If and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. By separate cases as in Lemma 2, we can conclude the behavior of the solution in
Table 2.
□
Lemma 5. If and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. By separate cases as in Lemma 2, we can conclude the behavior of the solution in
Table 3.
□
The next four lemmas consider in each quadrant. The only complicated cases are the second and the forth quadrants. For the first and the third quadrants we just show the regions considered without the detail of the proof.
Lemma 6. If , and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. If , then for all , where:
; and .
; and .
and .
and .
; ; and .
; ; and .
□
Lemma 7. If , and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. If , then for all , where:
and .
and .
; and .
and .
; and .
and .
and .
; and .
□
Lemma 8. If , and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. Let
and
. Then,
Case 1 . We have
and
and
By using the result of Lemma 7, we obtain that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all .
Case 2 . We have the same
as in Case 1, while
By using the result of Lemma 7, we obtain that
By Lemma 1, we have for all .
Case 3 . We have the same
and
as in Case 2, while
and
By using the result of Lemma 7, we obtain that
By Lemma 1, we have for all .
Case 4 . We have and . By using the result of Lemma 7, we obtain that
if , then , and . By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
By Lemma 1, we have for all ;
By Lemma 1, we have for all ;
If
, then
By Lemma 1, we have for all ;
If
, then
By Lemma 1, we have for all ;
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all .
Case 5 . We have
and
. Then,
By using the result of Lemma 7, we obtain that
By Lemma 1, we have for all .
Case 6 . We have the same
as in Case 5, while
and
By using the result of Lemma 7, we obtain that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
By Lemma 1, we have for all .
Case 7 . We have the same
and
as in Case 6, while
and
By using the result of Lemma 7, we obtain that
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
By Lemma 1, we have for all .
Case 8 . We have the same and as in Case 6, while and . By using the results of Lemmas 2, 3 and 7, we obtain that
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all .
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all .
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
Similar to the proof of Case 6 of Lemma 2, we can conclude that for all .
Case 9 .
. Then,
. We have the same
and
as in Case 6, while
,
and
By using the result of Lemma 7, we obtain that
By Lemma 1, we have for all .
Case 10 . We have the same
-
as in Case 9, while
and
By using the result of Lemma 7, we obtain that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all .
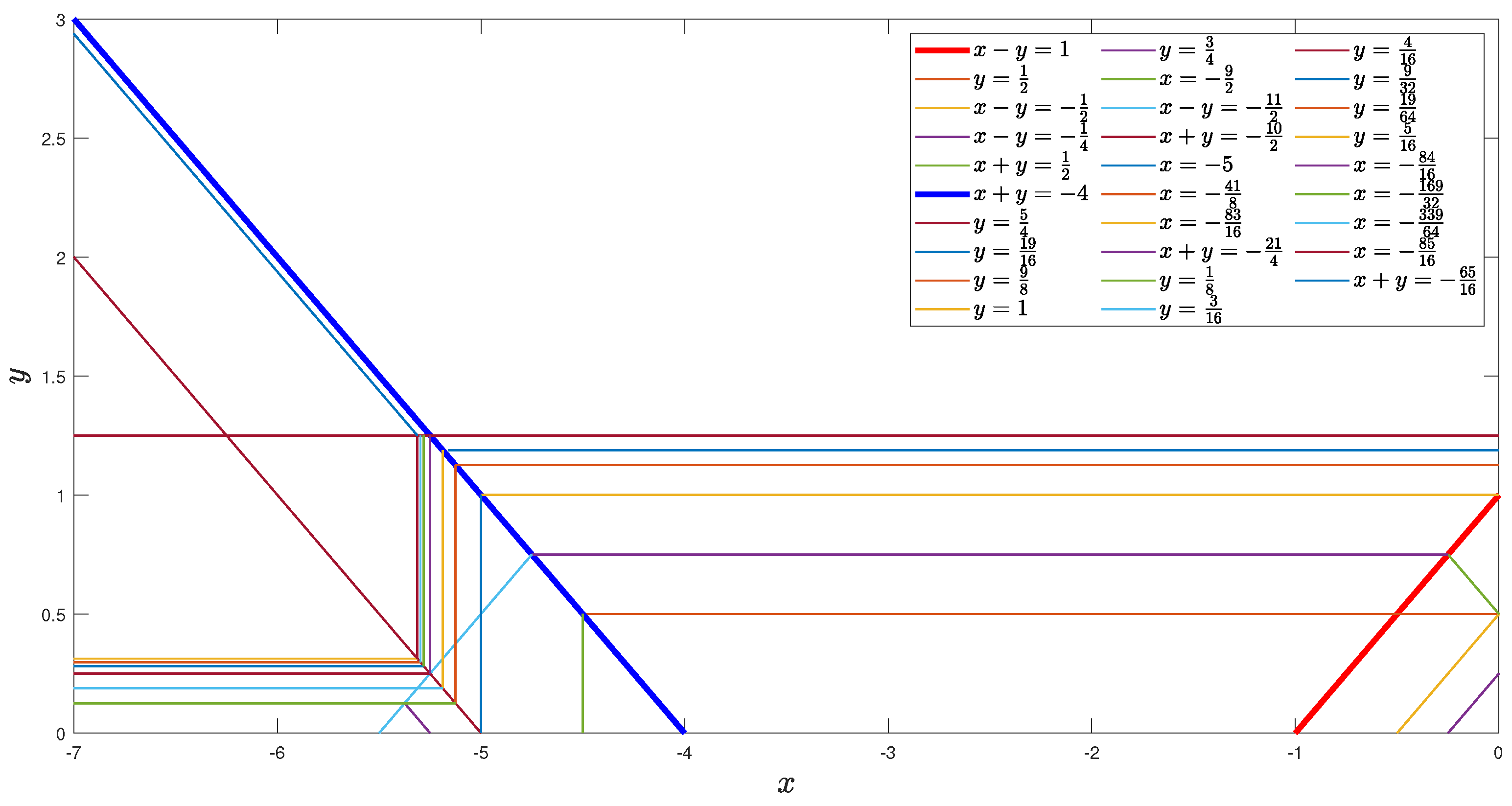
Case 11 . We have the same - as in Case 9. Since , and . Then, and . By using the result of Lemma 6, we obtain that
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
if
, then
By using the result of Lemma 5, we have for all .
Figure 1 shows region of each case presented in the proof of this lemma.
□
Lemma 9. If , and , then the solution of (2) eventually becomes the equilibrium point of (2). Proof. Let
and
. Then,
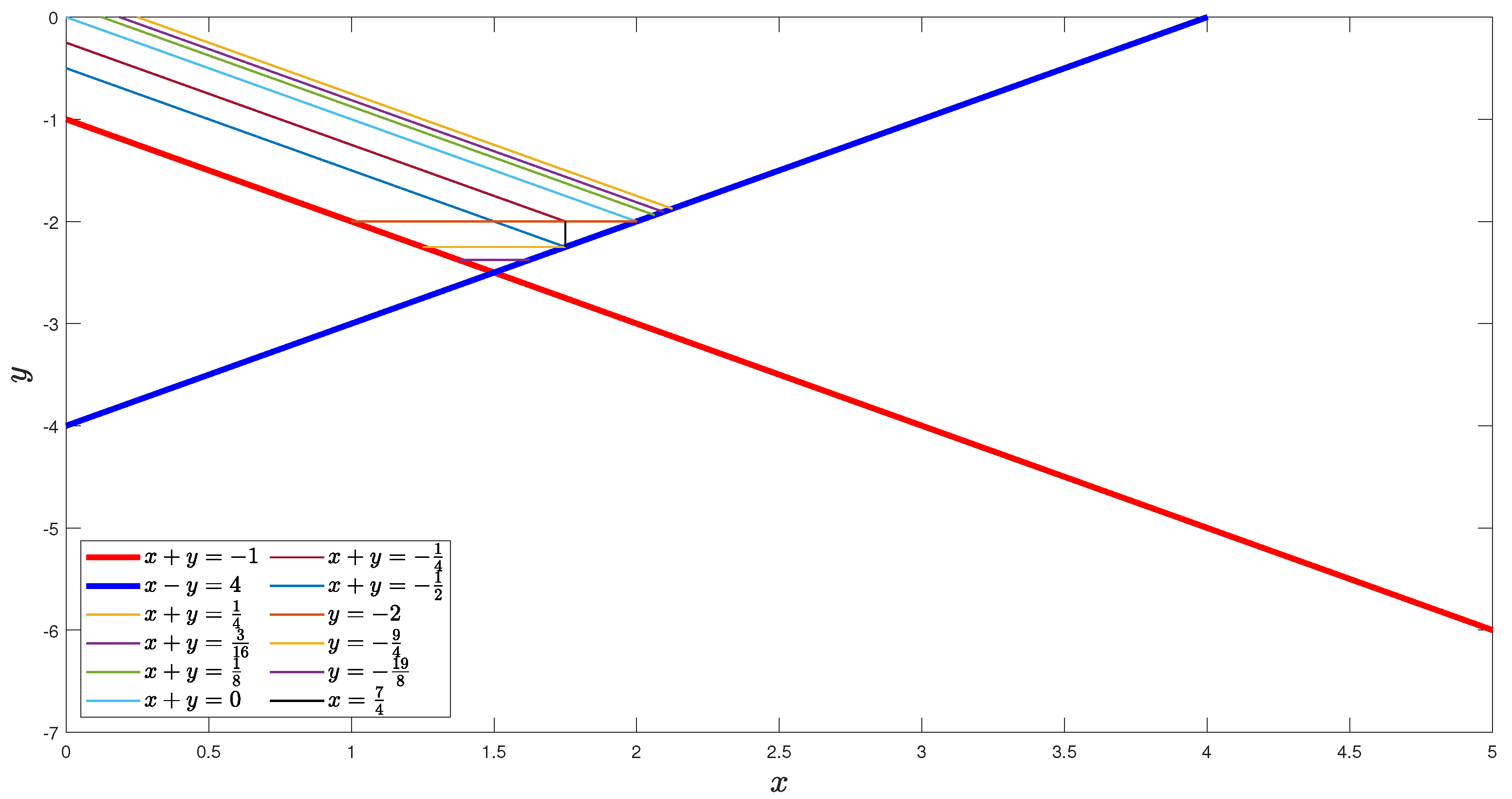
Case 1 . We have and . By using the results of Lemmas 5 and 8, we have that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Since and , . By Lemma 1, we have for all ;
if
, then
Since and , . Then, . By Lemma 1, we have for all .
Figure 2 shows regions considered in the proof of Case 1 of this lemma.
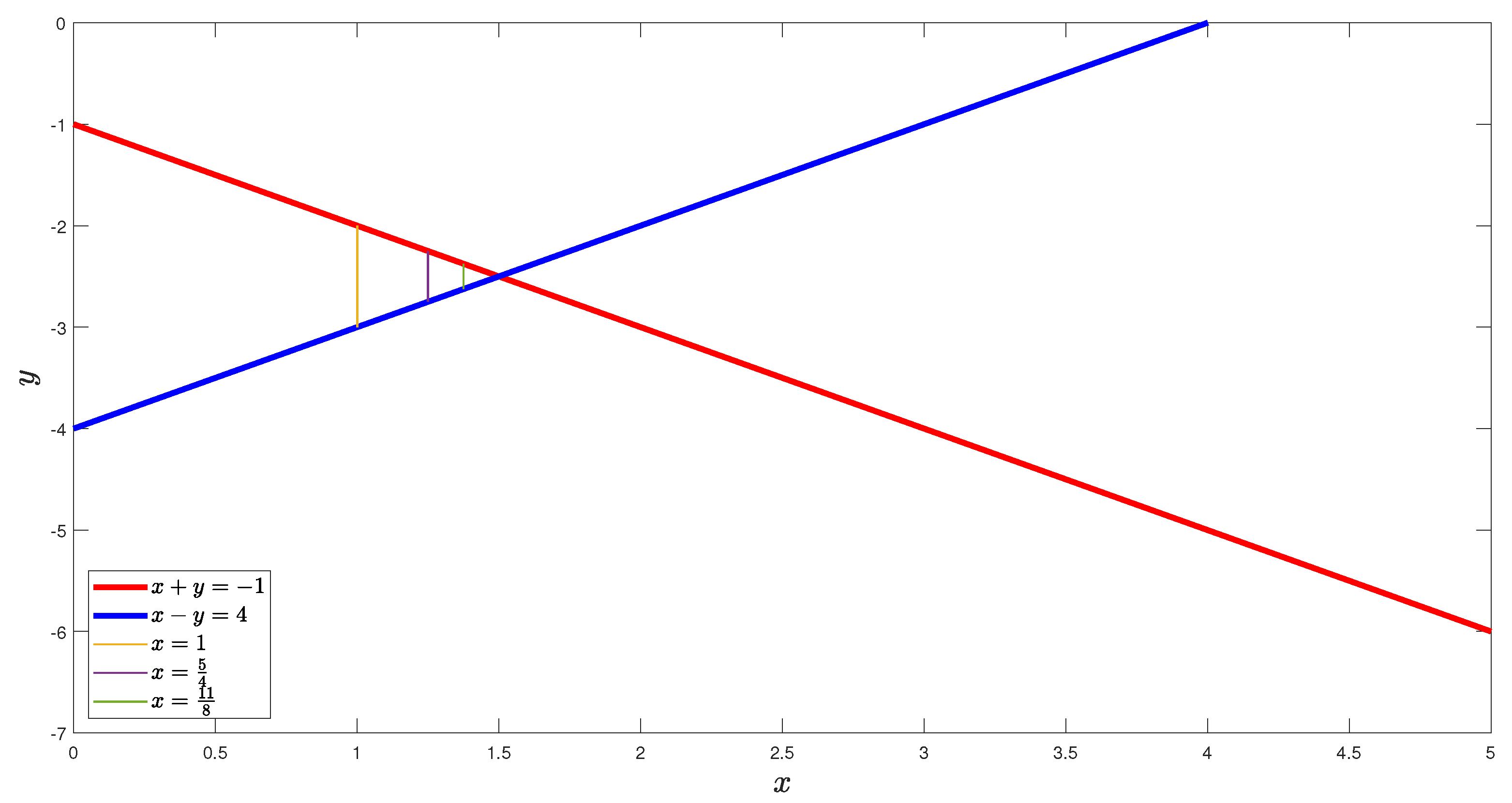
Case 2 . We have and . By using the result of Lemma 7, we have that
By Lemma 1, we have for all ;
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
By Lemma 1, we have for all .
Figure 3 shows regions considered in the proof of Case 2 of this lemma.
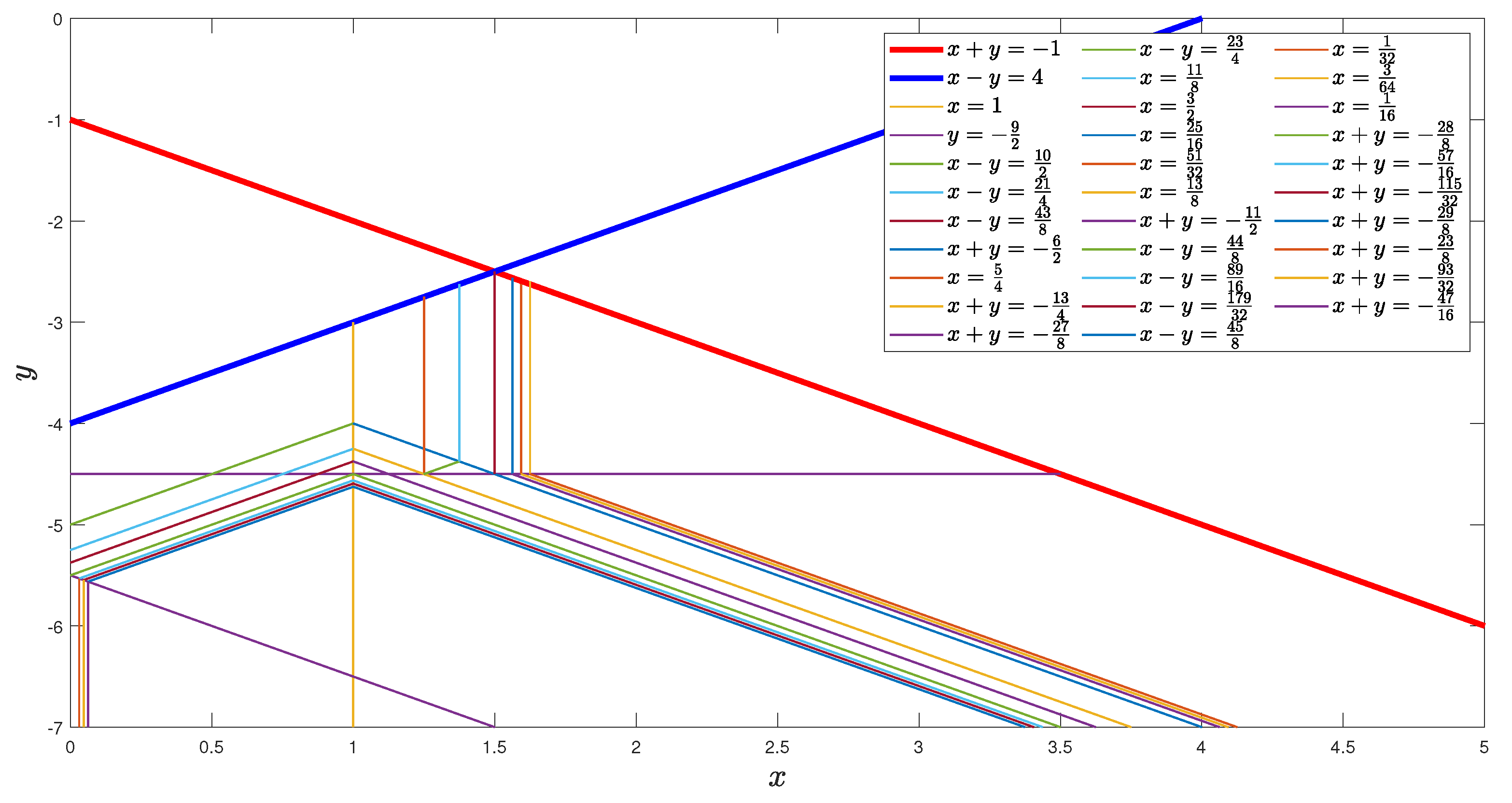
Case 3 . We have and .
Case 3.1 . Then,
By using the result of Lemma 7, we have that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all .
Case 3.2 . We have the same as in Case 3.1, while and . By using the results of Lemmas 5 and 8, we have that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all .
Case 3.3 . We have the same
as in Case 3.2, while
,
and
By using the result of Lemma 7, we have that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
and
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all .
Case 3.4 . We have the same as in Case 3.2, while and . By using the results of Lemmas 2, 3 and 6, we have that
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
if
, then
Similar to the proof of Case 6 of Lemma 2, we can conclude that for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all .
Figure 4 shows regions considered in the proof of Case 3 of this lemma.
Case 4 . We have and . By using the results of Lemmas 2, 3 and 6, we have that
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
By Lemma 1, we have for all ;
if
, then
Similar to the proof of Case 4 of Lemma 2, we can conclude that for all ;
Similar to the proof of Case 6 of Lemma 2, we can conclude that for all .
Figure 5 shows regions considered in the proof of Case 4 of this lemma.
□
Therefore, as we combine Lemmas 2–9, we can have the following theorem. It can be seen that the solutions do not show any periodic behavior. This is not the same as the other values of
b considered in [
11,
14,
15,
17].
Theorem 1. If , , then the solution of (2) eventually becomes the equilibrium point of (2) within 14 iterations. 4. Conclusions and Discussion
It is shown completely that for
, all solutions of (
2) eventually becomes the equilibrium point
. It is also suspected from Lemmas 10–13 and Lemmas 18 (i)–19 (i) that for
, all solutions of (
2) eventually becomes the equilibrium point
, while for
, concerning the solutions of (
2) with a chance to possess the periodic behavior of prime period 5 and for some small values of
and
, it may also becomes the equilibrium point.
As we mentioned before that since the absolute value function is not differentiable, one needs to find an alternative method to analyze the behavior of (
2). Thus, we choose to use a fundamental method to complete our full analysis. However, this give some insight to those who want to do further investigation concerning this type of problem. For example, (i) one can roughly see how many cases need to be considered for each value of
b; (ii) one can see, for a big region, that the behavior of the solutions remains the same; (iii) one can estimate the maximum iteration until the behavior of the solutions become either equilibrium or periodic.
Finally, these complete results for
and partial results for
, where all solutions asymptotically become equilibrium, are in contrast with the existing results concerning Equation (
1) which usually involving periodic behavior. Thus, one may try to consider these cases of
b and prove our conjecture that only for
and 5 do all solutions eventually become the equilibrium point, while for
all solutions eventually become either equilibrium or periodic of prime period 5.