On the Connection Problem for Painlevé Differential Equation in View of Geometric Function Theory
Abstract
1. Introduction
2. Methodology
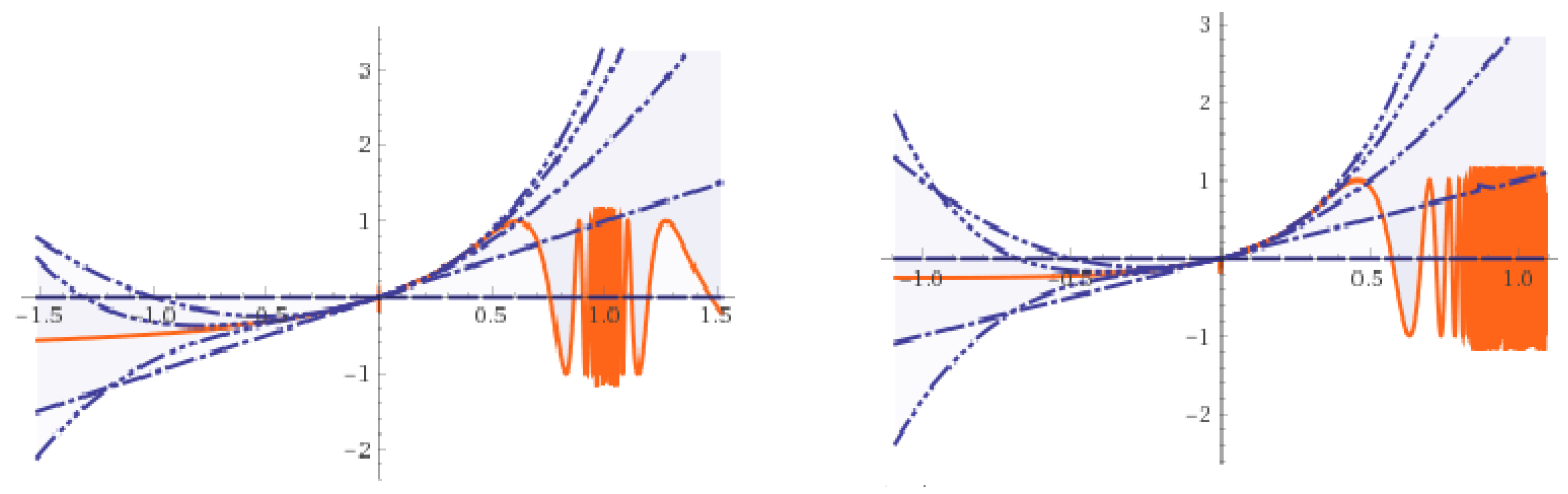
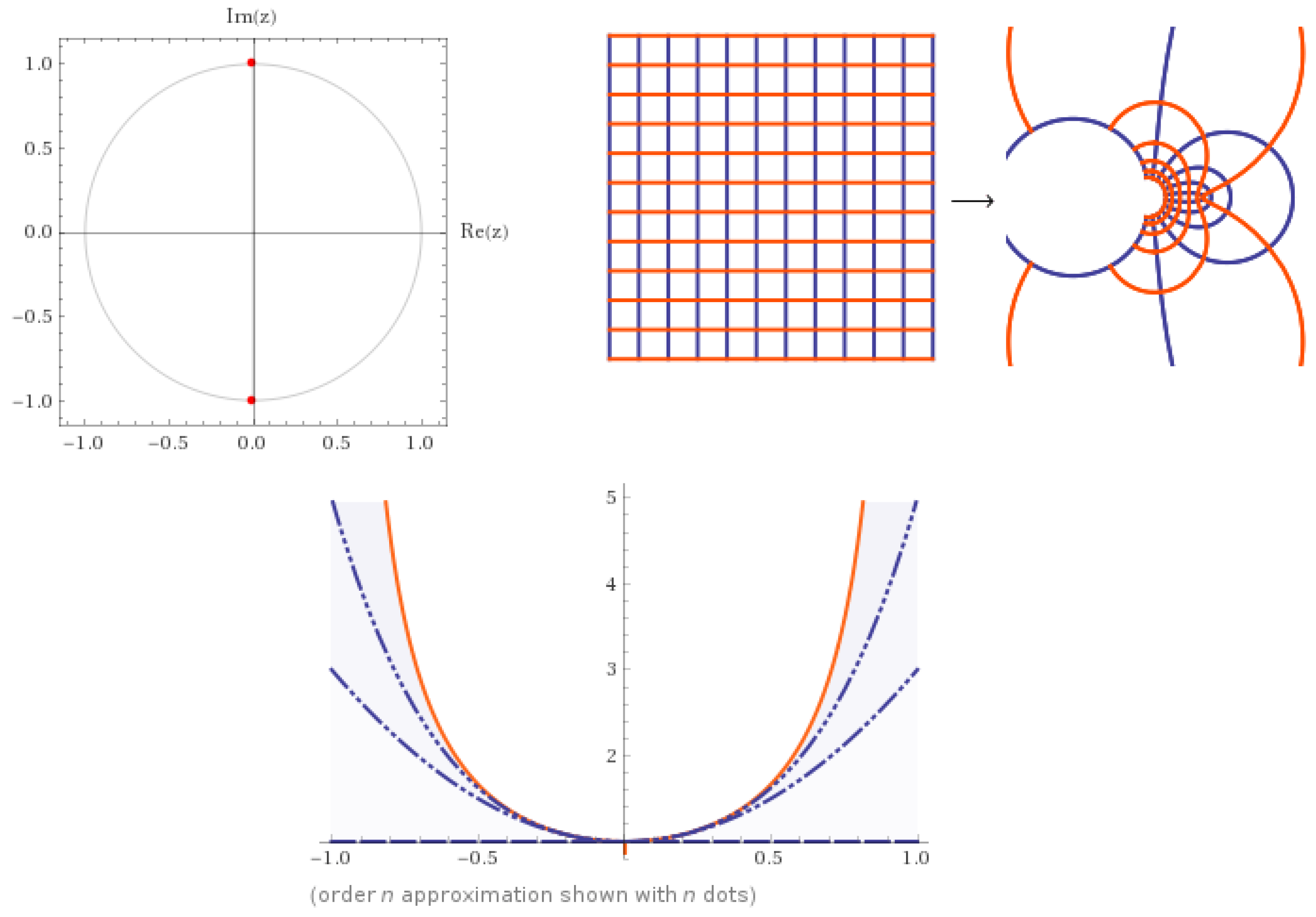
3. Connection Bounds
- Letthen
- Letthen
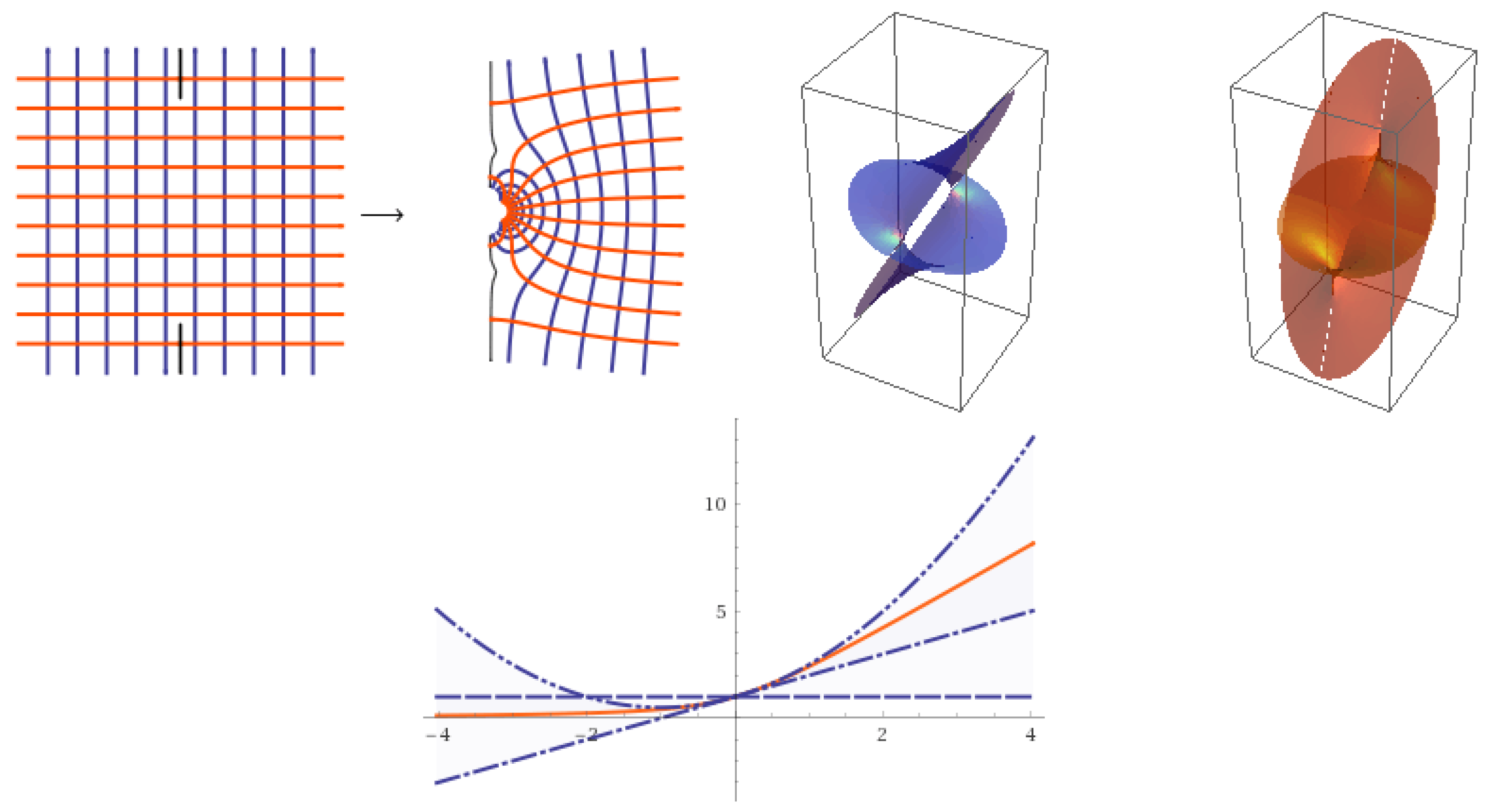
4. Geometric Behaviors
- Let, we have
- Letthen we get
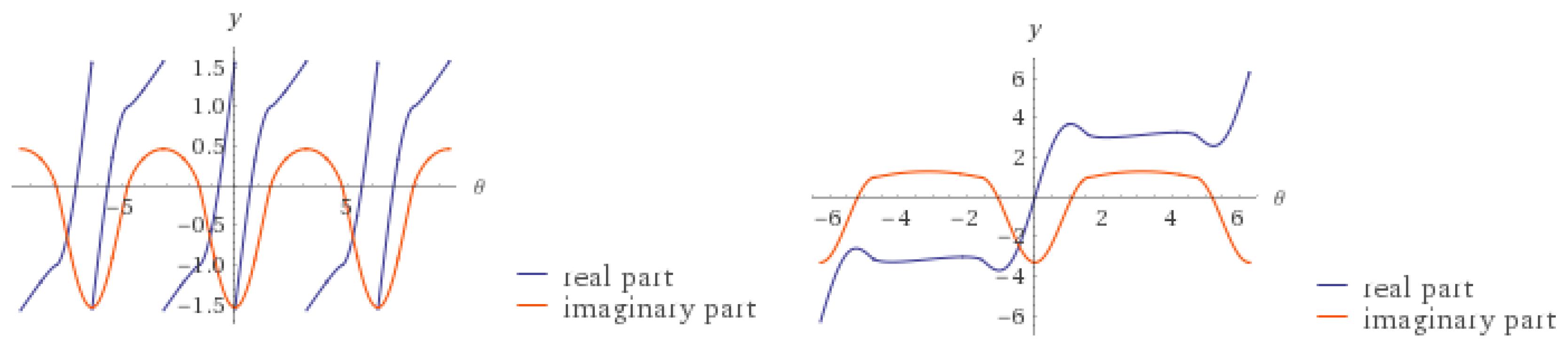
5. Symmetric Solution
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dormieux, L.; Ulm, F.J. (Eds.) Applied Micromechanics of Porous Materials; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007; Volume 480. [Google Scholar]
- Shimomura, S. A family of solutions of a nonlinear ordinary differential equation and its application to Painlevé equations (III), (V) and (VI). J. Math. Soc. Jpn. 1987, 39, 649–662. [Google Scholar] [CrossRef]
- Kajiwara, K.; Tetsu, M. On the Umemura polynomials for the Painlevé III equation. Phys. Lett. A 1999, 260, 462–467. [Google Scholar] [CrossRef]
- Kajiwara, K.; Kinji, K. On a q-Difference Painlevé III Equation: I. Derivation, Symmetry and Riccati Type Solutions. J. Nonlinear Math. Phys. 2003, 10, 86–102. [Google Scholar] [CrossRef]
- Gu, Y.; Aminakbari, N.; Yuan, W.; Wu, Y.H. Meromorphic solutions of a class of algebraic differential equations related to Painlevé equation III. Houst. J. Math. 2017, 43, 1045–1055. [Google Scholar]
- Bothner, T.; Miller, P.D.; Sheng, Y. Rational solutions of the Painlevé-III equation. Stud. Appl. Math. 2018, 141, 626–679. [Google Scholar] [CrossRef]
- Fasondini, M.; Fornberg, B.; Weideman, J.A.C. A computational exploration of the McCoy-Tracy-Wu solutions of the third Painlevé equation. Phys. D Nonlinear Phenom. 2018, 363, 18–43. [Google Scholar] [CrossRef]
- Bonelli, G.; Grassi, A.; Tanzini, A. Quantum curves and q-deformed Painlevé equations. Lett. Math. Phys. 2019, 109, 1961–2001. [Google Scholar] [CrossRef]
- Amster, P.; Rogers, C. On a Neumann boundary value problem for Ermakov-Painlevé III. Electron. J. Qual. Theory Differ. Equ. 2019, 2019, 1–10. [Google Scholar] [CrossRef]
- Xu, H.Y.; Tu, J. Existence of rational solutions for q-difference Painlevé equations. Electron. J. Differ. Equ. 2020, 2020, 1–14. [Google Scholar]
- Bilman, D.; Ling, L.; Miller, P.D. Extreme superposition: Rogue waves of infinite order and the Painlevé-III hierarchy. Duke Math. J. 2020, 169, 671–760. [Google Scholar] [CrossRef]
- Zeng, Z.Y.; Hu, L. On the connection problem for nonlinear differential equation. Bound. Value Probl. 2019, 2019, 73. [Google Scholar] [CrossRef]
- Kitaev, A.V. Parametric Painlevé equations. J. Math. Sci. 2013, 192, 81–90. [Google Scholar] [CrossRef]
- Miller, S.S.; Mocanu, P.T. Differential Subordinations: Theory and Applications; CRC Press: Boca Raton, FL, USA, 2000. [Google Scholar]
- Ma, W.; Minda, D. A unified treatment of some special classes of univalent functions. In Proceedings of the Conference on Complex Analysis, Tianjin, China, 19–23 June 1992; pp. 157–169. [Google Scholar]
- Ali, R.M.; Lee, S.K.; Ravichandran, V.; Supramaniam, S. Coefficient estimates for bi-univalent Ma-Minda starlike and convex functions. Appl. Math. Lett. 2012, 25, 344–351. [Google Scholar] [CrossRef]
- Raina, R.K.; Sokol, J. Some properties related to a certain class of starlike functions. Comptes Rendus Math. 2015, 353, 973–978. [Google Scholar] [CrossRef]
- Littlewood, J.E. On inequalities in the theory of functions. Proc. Lond. Math. Soc. 1925, 23, 481–519. [Google Scholar] [CrossRef]
- Ruscheweyh, S. Convolutions in geometric function Theory; Gaetan Morin Editeur Ltee: Montreal, QC, CA, 1982. [Google Scholar]
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ibrahim, R.W.; Elobaid, R.M.; Obaiys, S.J. On the Connection Problem for Painlevé Differential Equation in View of Geometric Function Theory. Mathematics 2020, 8, 1198. https://doi.org/10.3390/math8071198
Ibrahim RW, Elobaid RM, Obaiys SJ. On the Connection Problem for Painlevé Differential Equation in View of Geometric Function Theory. Mathematics. 2020; 8(7):1198. https://doi.org/10.3390/math8071198
Chicago/Turabian StyleIbrahim, Rabha W., Rafida M. Elobaid, and Suzan J. Obaiys. 2020. "On the Connection Problem for Painlevé Differential Equation in View of Geometric Function Theory" Mathematics 8, no. 7: 1198. https://doi.org/10.3390/math8071198
APA StyleIbrahim, R. W., Elobaid, R. M., & Obaiys, S. J. (2020). On the Connection Problem for Painlevé Differential Equation in View of Geometric Function Theory. Mathematics, 8(7), 1198. https://doi.org/10.3390/math8071198






