Squeezed Hybrid Nanofluid Flow Over a Permeable Sensor Surface
Abstract
1. Introduction
2. Mathematical Formulation
3. Stability Analysis
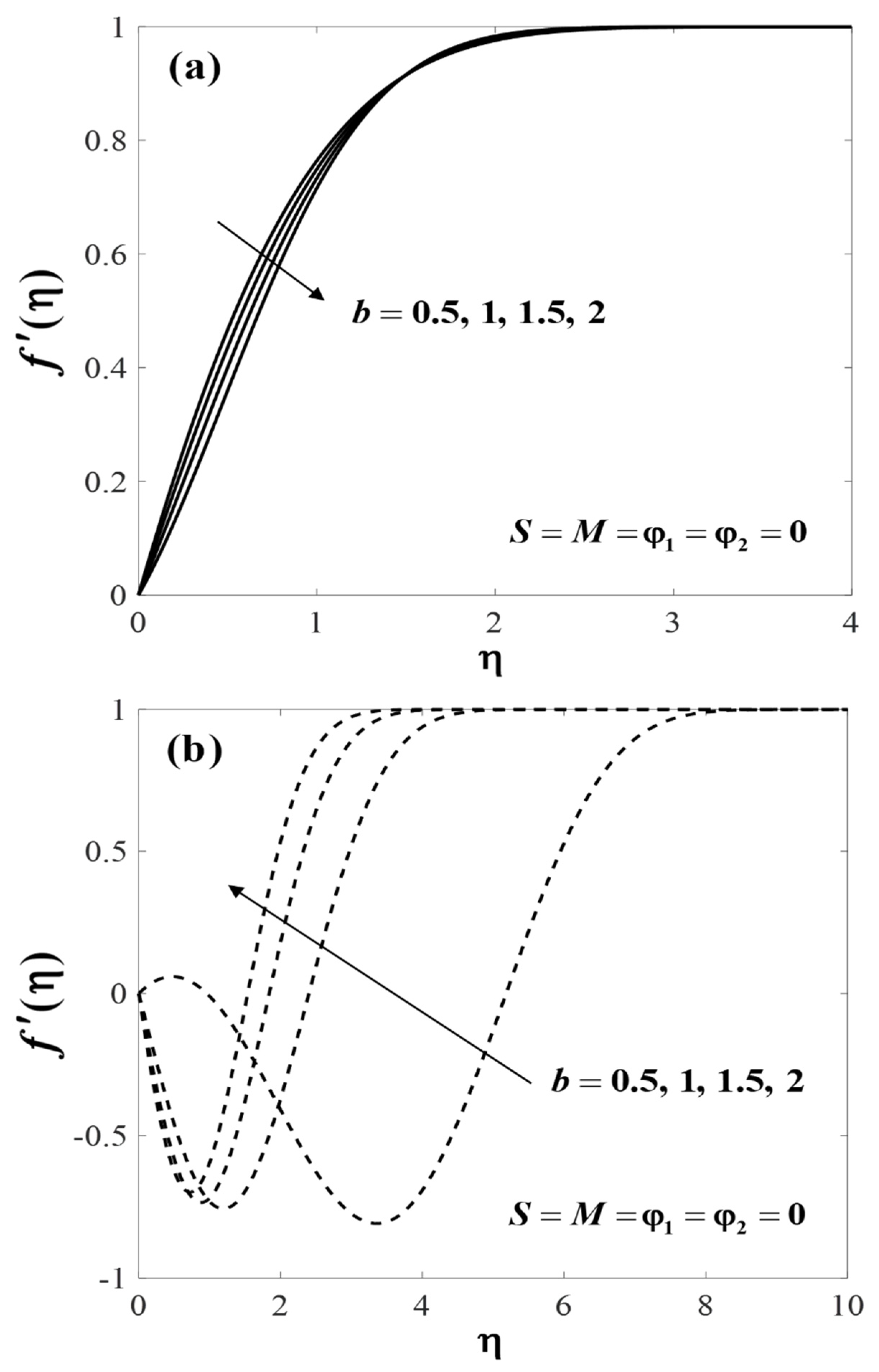
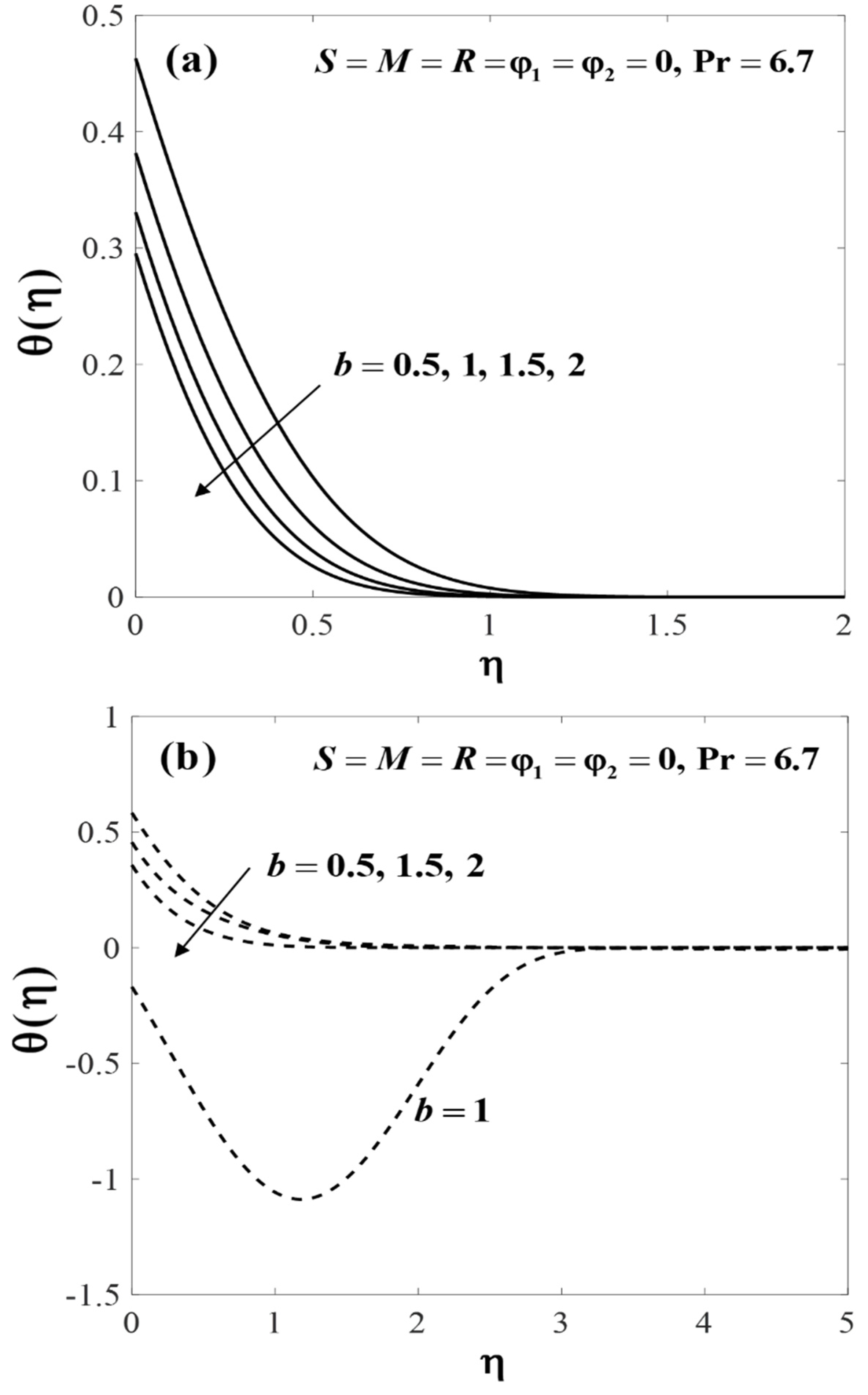
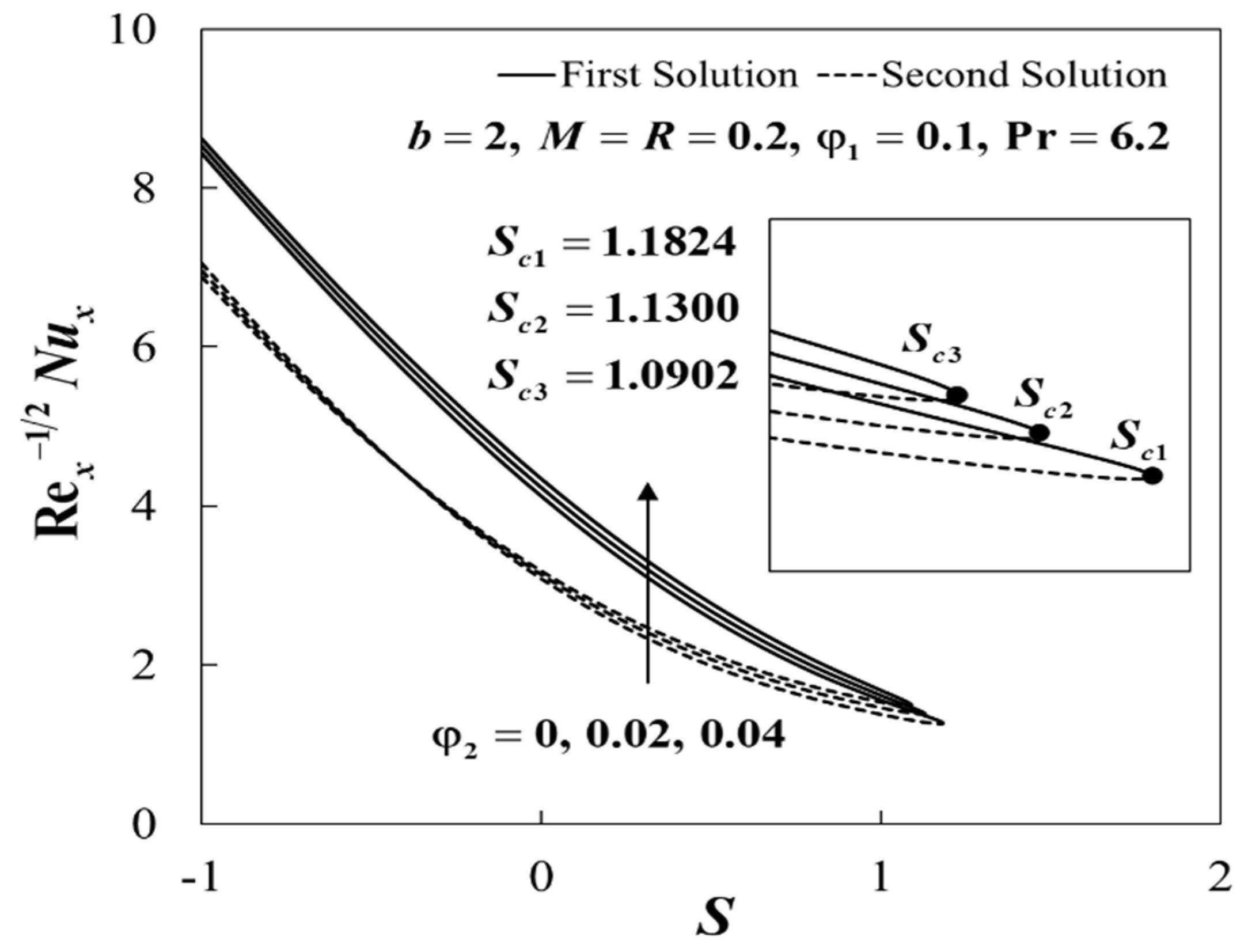
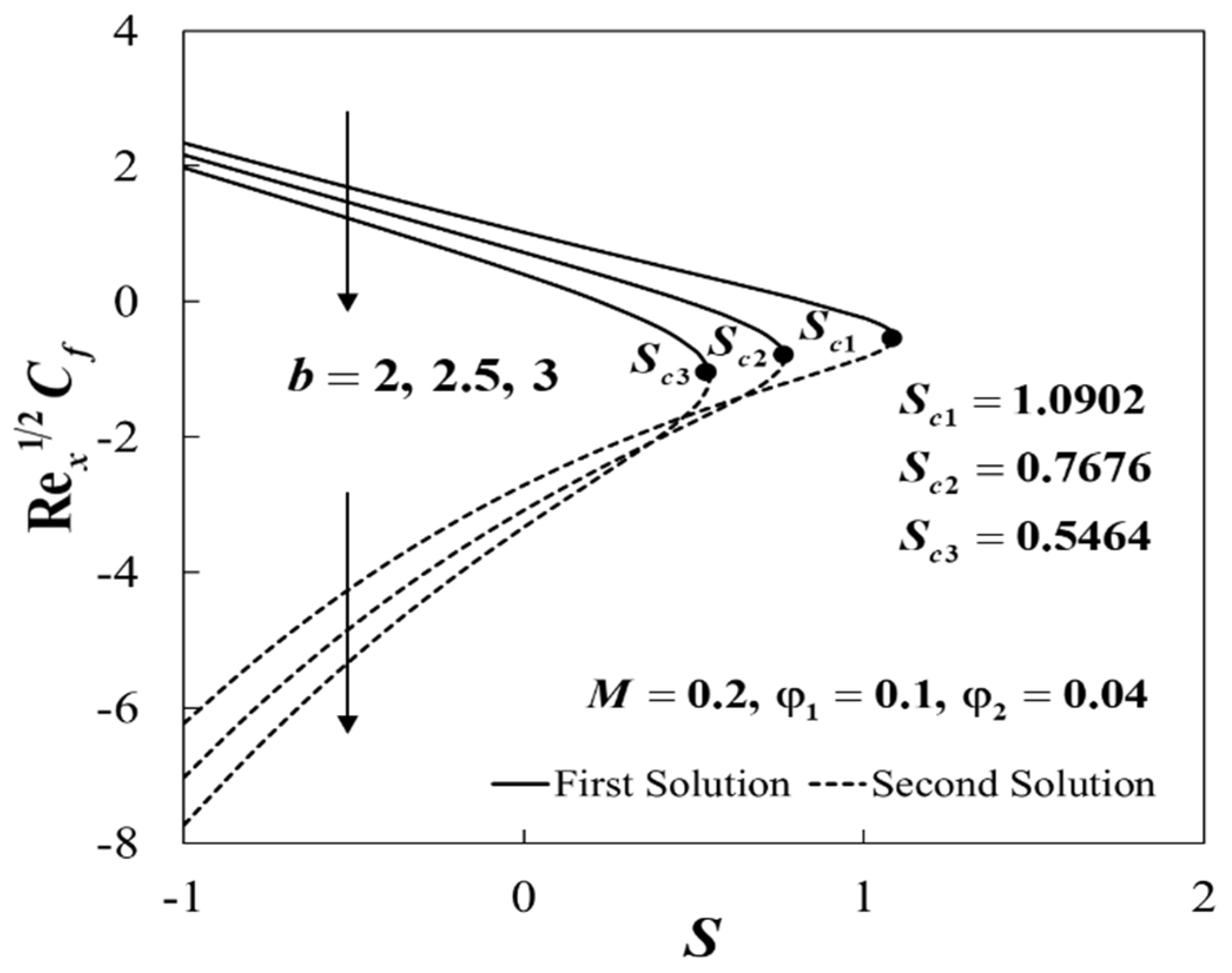
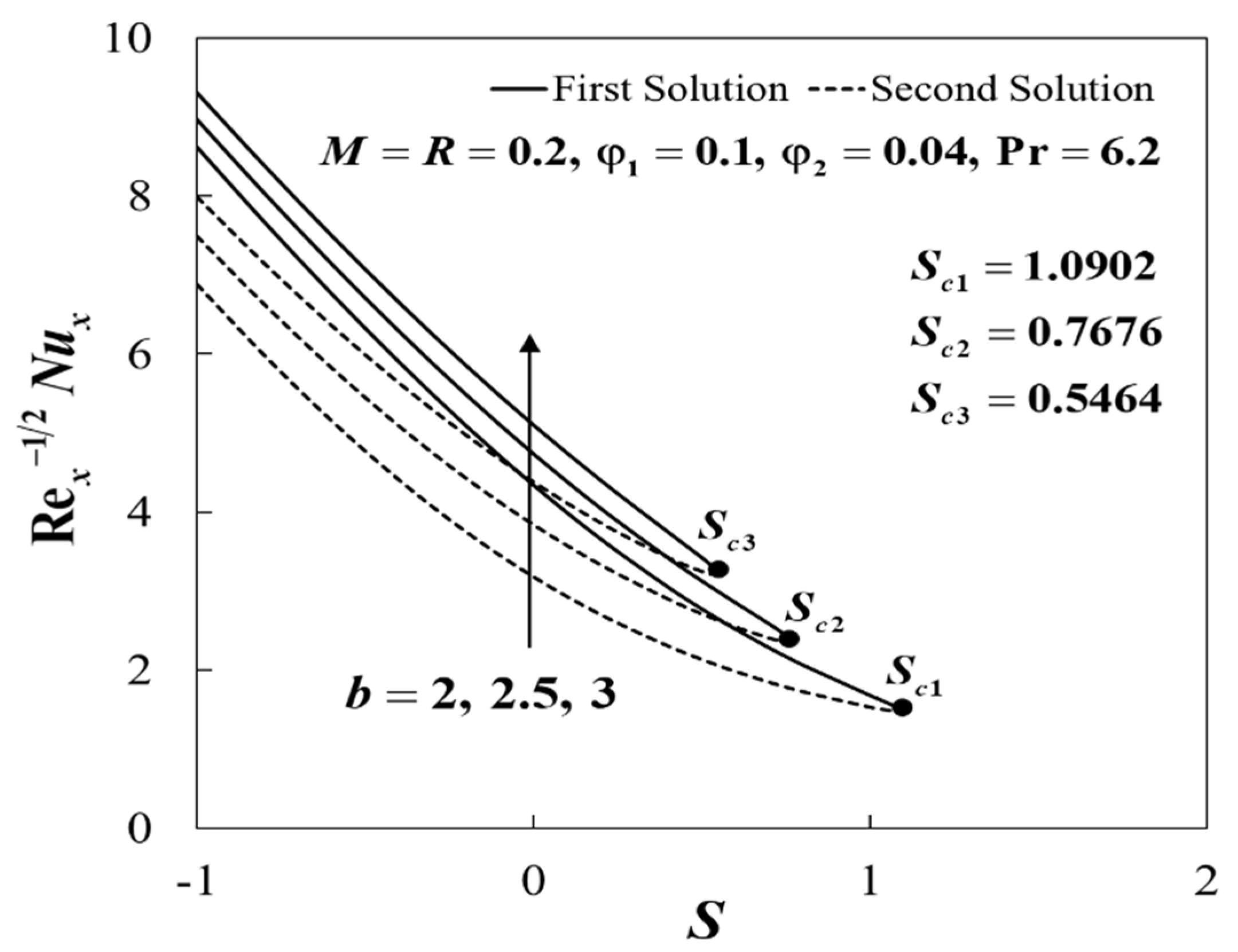
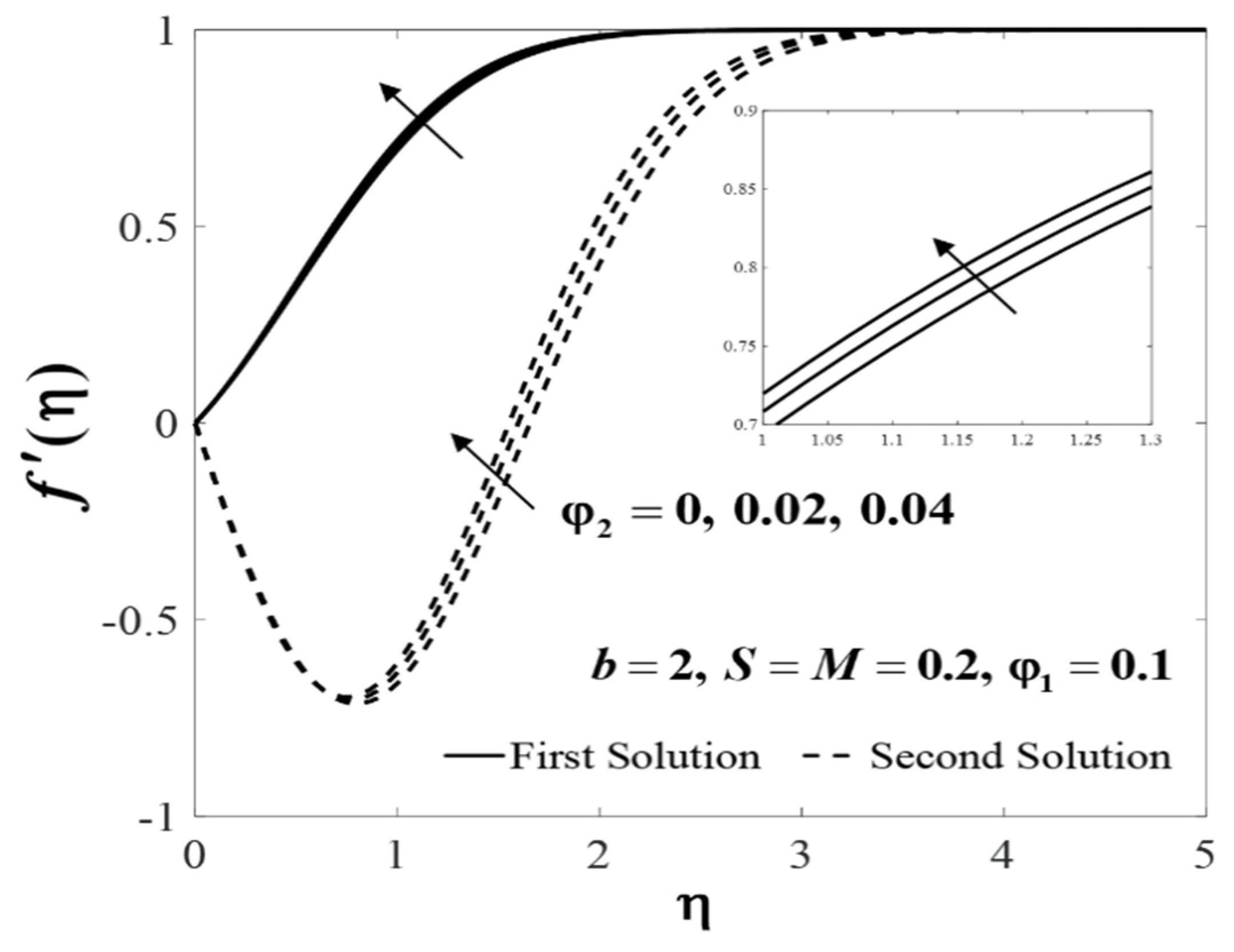
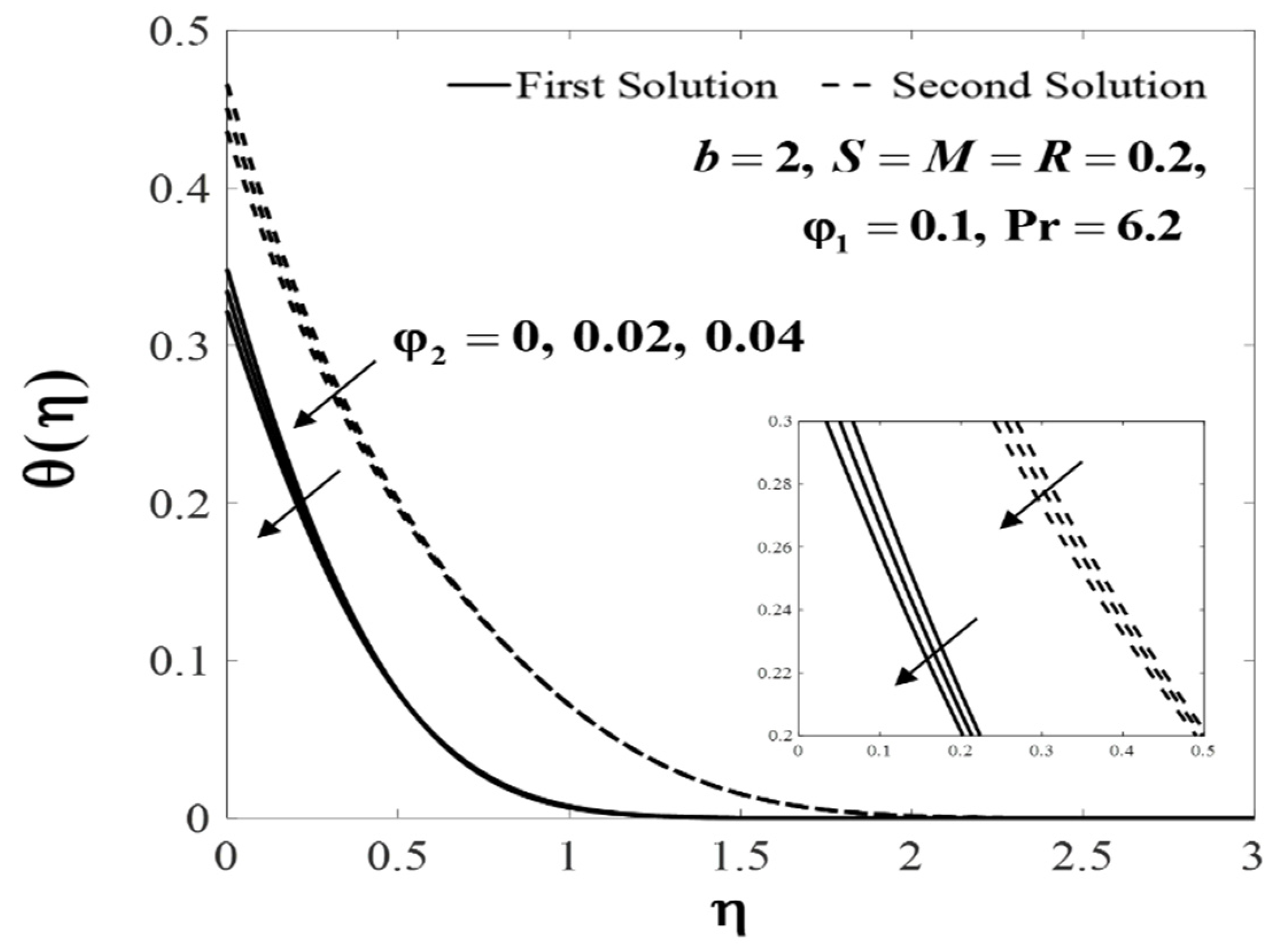
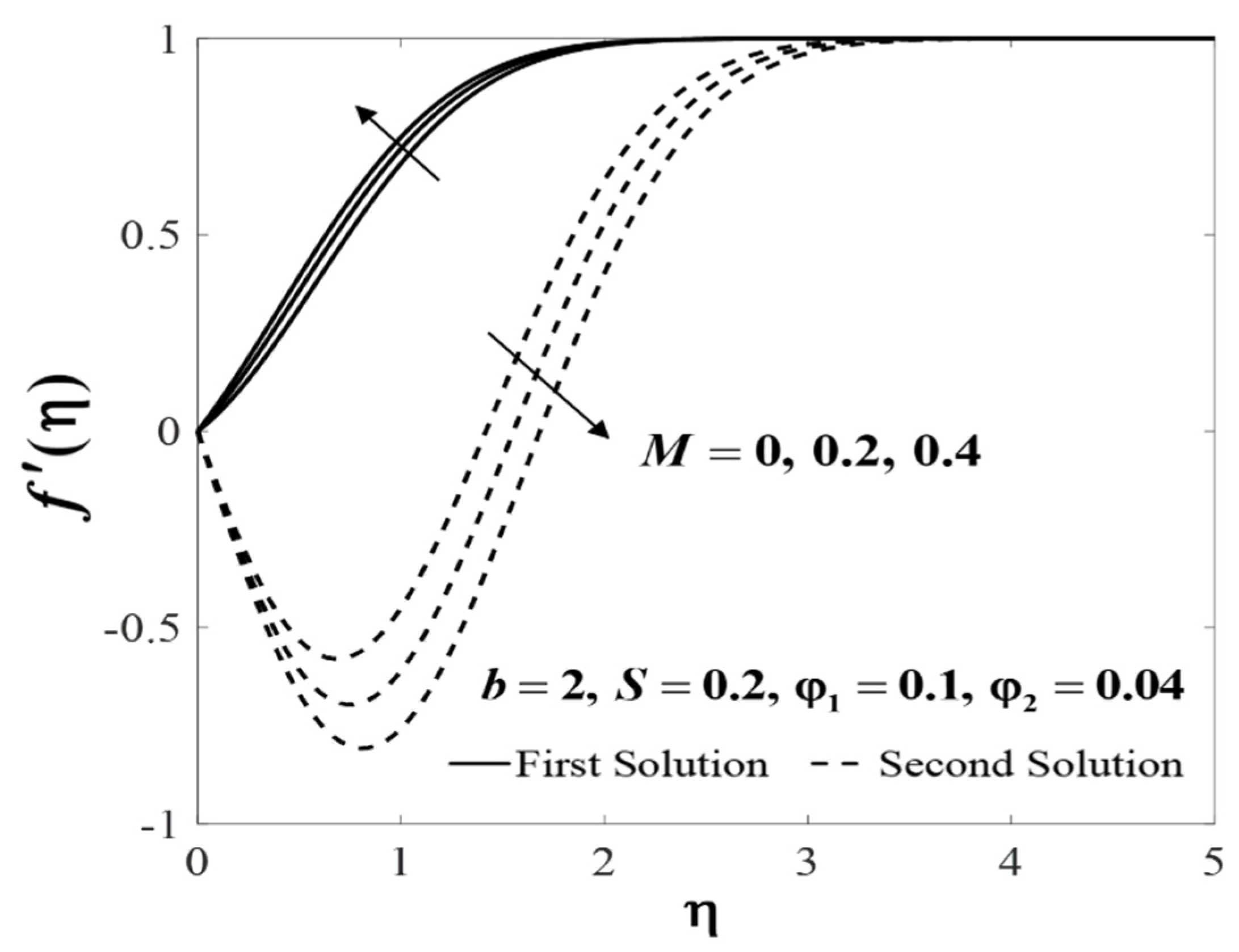
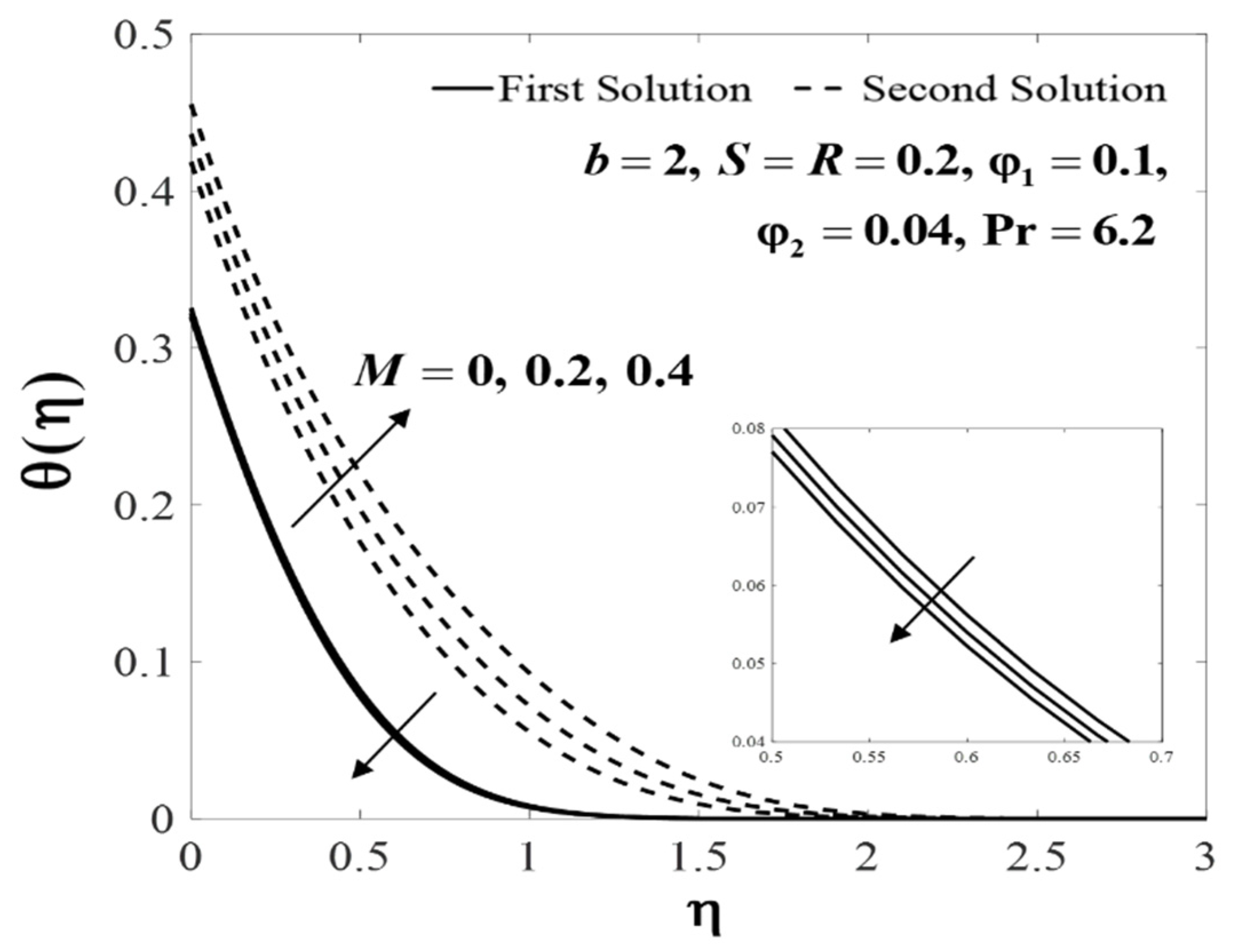
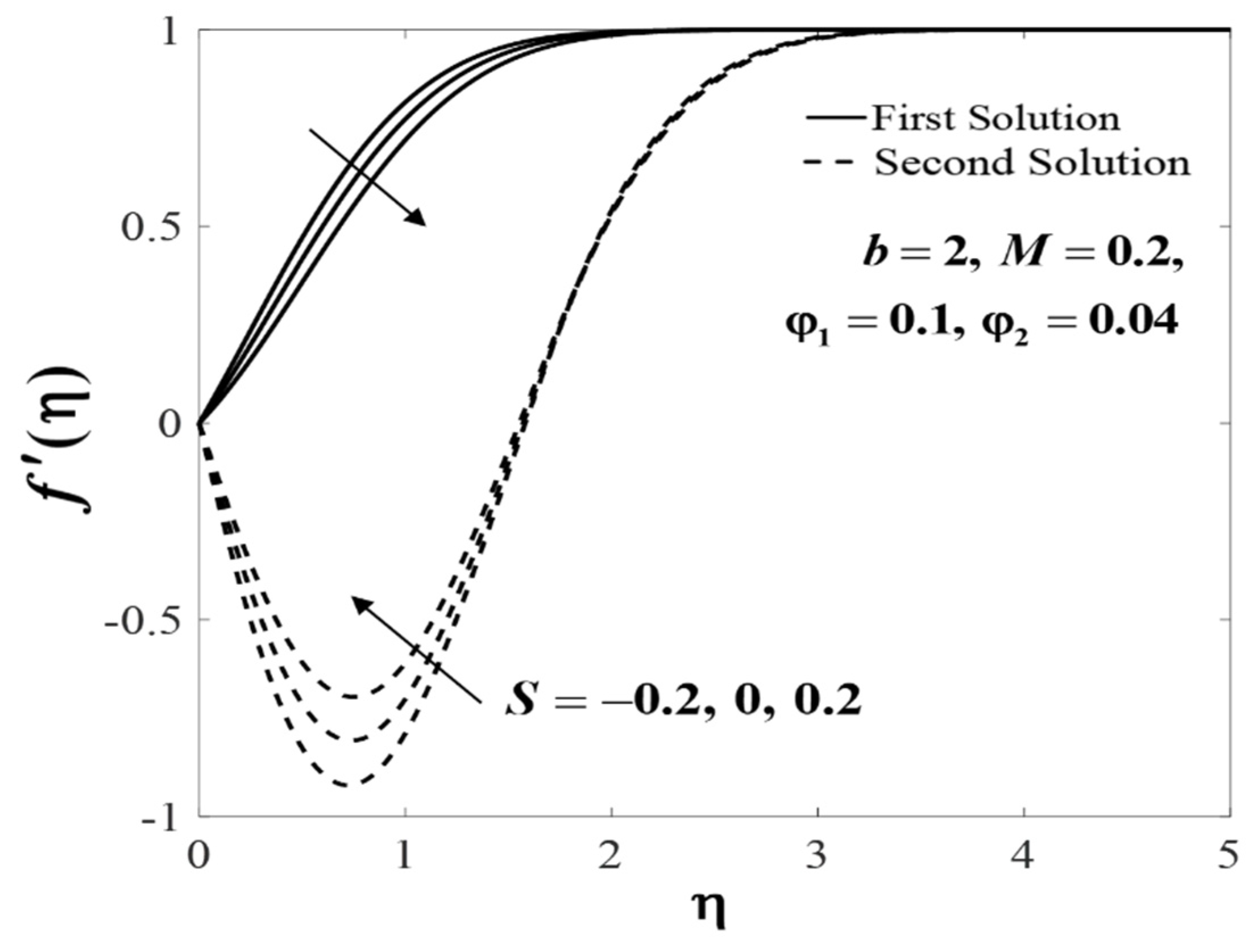
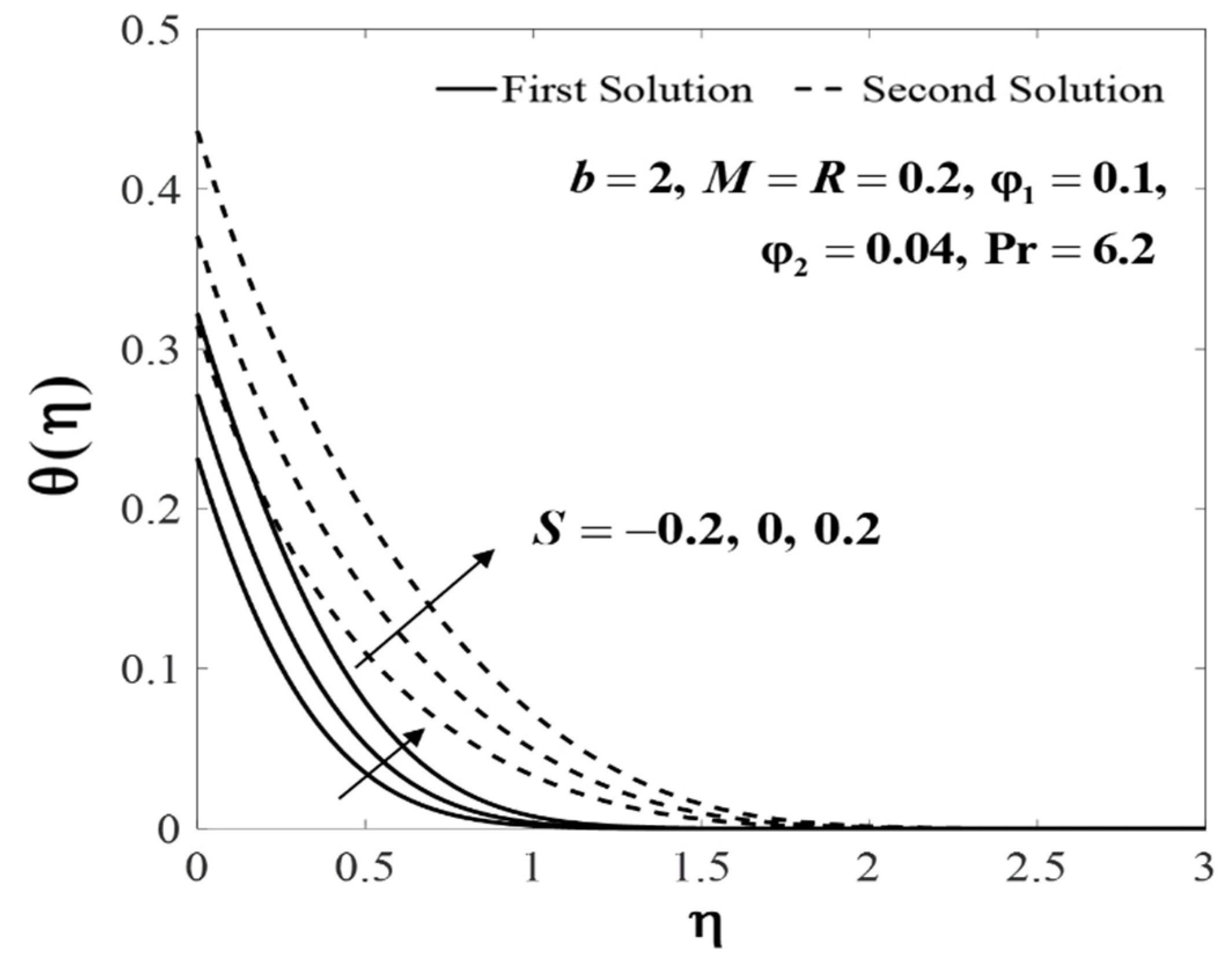
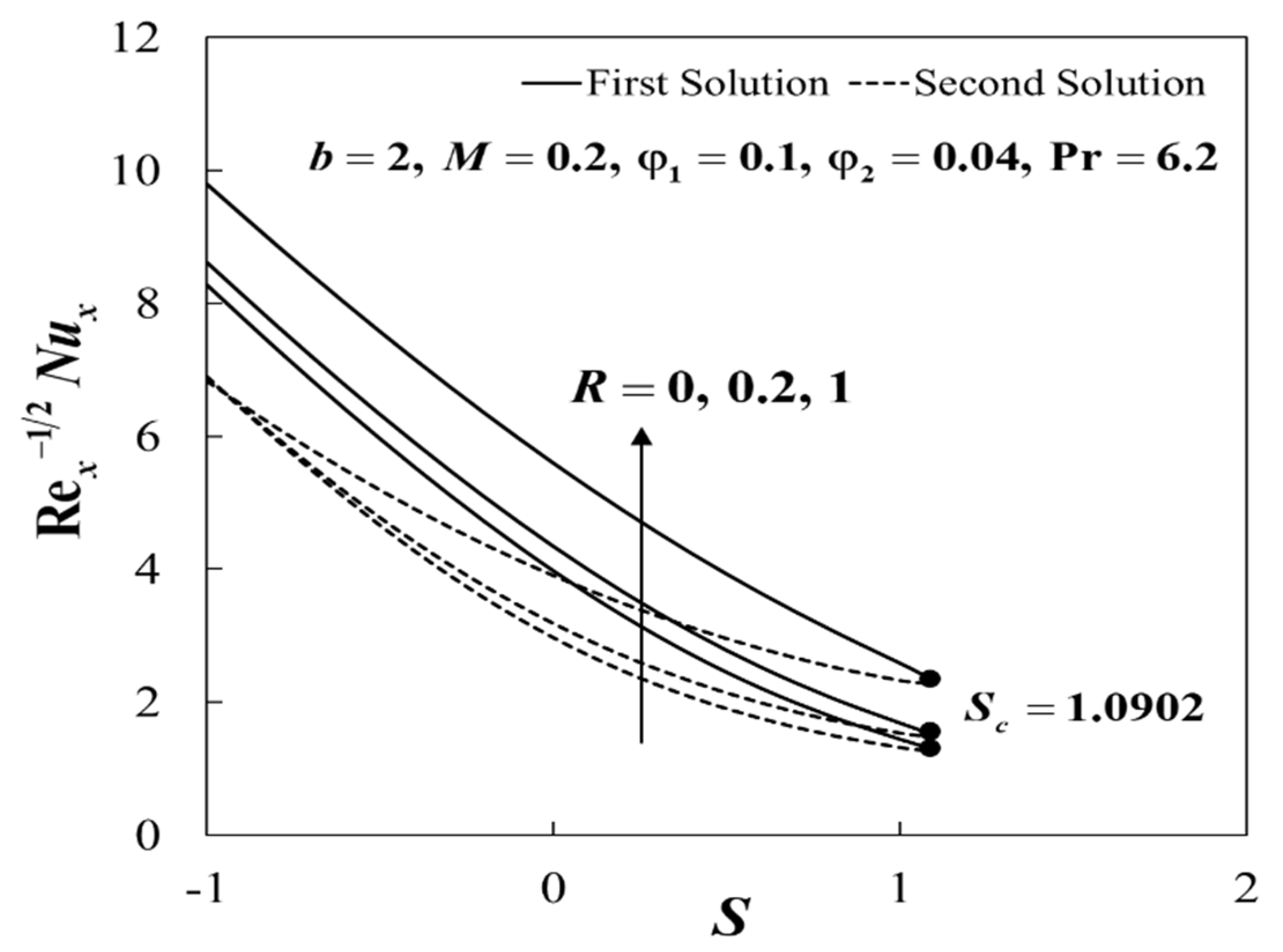
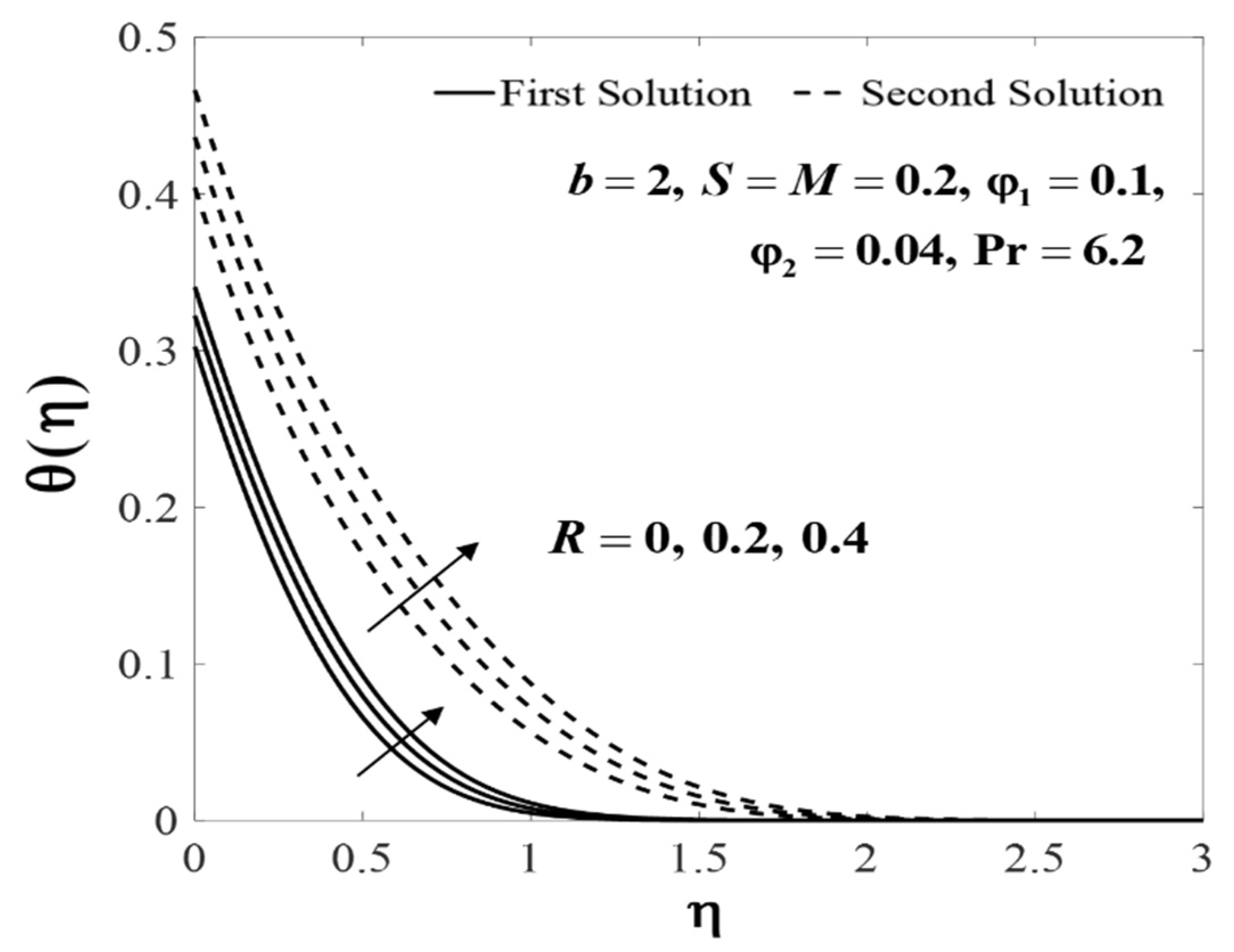
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| constant | |
| squeeze flow strength | |
| squeeze flow index | |
| magnetic field | |
| reference magnetic field strength | |
| skin friction coefficient | |
| specific heat at constant pressure | |
| heat capacitance of the fluid | |
| dimensionless stream function | |
| height of the channel | |
| thermal conductivity of the fluid | |
| Rosseland mean absorption coefficient | |
| sensor surface length | |
| local Nusselt number | |
| magnetic parameter | |
| Prandtl number | |
| pressure | |
| reference heat flux | |
| radiative heat flux in y direction | |
| surface heat flux | |
| radiation parameter | |
| local Reynolds number | |
| permeable parameter | |
| time | |
| fluid temperature | |
| surface temperature | |
| ambient temperature | |
| velocity component in the x- and y- directions | |
| free stream velocity | |
| reference surface permeable velocity | |
| surface permeable velocity | |
| Cartesian coordinates | |
| Greek symbols | |
| eigenvalue | |
| similarity variable | |
| dimensionless temperature | |
| dynamic viscosity of the fluid | |
| kinematic viscosity of the fluid | |
| density of the fluid | |
| electrical conductivity of the fluid | |
| Stefan–Boltzmann constant | |
| dimensionless time variable | |
| wall shear stress | |
| nanoparticle volume fractions for Al2O3 (alumina) | |
| nanoparticle volume fractions for Cu (copper) | |
| stream function | |
| Subscripts | |
| base fluid | |
| nanofluid | |
| hybrid nanofluid | |
| solid component for Al2O3 (alumina) | |
| solid component for Cu (copper) | |
| Superscript | |
| ′ | differentiation with respect to |
References
- Choi, S.U.S.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 International Mechanical Engineering Congress and Exhibition, San Francisco, CA, USA, 12–17 November 1995. [Google Scholar]
- Turcu, R.; Darabont, A.; Nan, A.; Aldea, N.; Macovei, D.; Bica, D.; Vekas, L.; Pana, O.; Soran, M.L.; Koos, A.A.; et al. New polypyrrole-multiwall carbon nanotubes hybrid materials. J. Optoelectron. Adv. Mater. 2006, 8, 643–647. [Google Scholar]
- Jana, S.; Salehi-Khojin, A.; Zhong, W.H. Enhancement of fluid thermal conductivity by the addition of single and hybrid nano-additives. Thermochim. Acta 2007, 462, 45–55. [Google Scholar] [CrossRef]
- Suresh, S.; Venkitaraj, K.P.; Selvakumar, P.; Chandrasekar, M. Synthesis of Al2O3-Cu/water hybrid nanofluids using two step method and its thermo physical properties. Colloids Surfaces A Physicochem. Eng. Asp. 2011, 388, 41–48. [Google Scholar] [CrossRef]
- Devi, S.P.A.; Devi, S.S.U. Numerical investigation of hydromagnetic hybrid Cu- Al2O3/water nanofluid flow over a permeable stretching sheet with suction. Int. J. Nonlinear Sci. Numer. Simul. 2016, 17, 249–257. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Unsteady flow and heat transfer past a stretching/shrinking sheet in a hybrid nanofluid. Int. J. Heat Mass Transf. 2019, 136, 288–297. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Flow and heat transfer along a permeable stretching/shrinking curved surface in a hybrid nanofluid. Phys. Scr. 2019, 94, 105219. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow and heat transfer over a nonlinear permeable stretching/shrinking surface. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 3110–3127. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow and heat transfer past a vertical thin needle with prescribed surface heat flux. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 4875–4894. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Transpiration effects on hybrid nanofluid flow and heat transfer over a stretching/shrinking sheet with uniform shear flow. Alexandria Eng. J. 2020, 59, 91–99. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. MHD flow and heat transfer of a hybrid nanofluid past a permeable stretching/shrinking wedge. Appl. Math. Mech. 2020, 41, 507–520. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Groşan, T.; Pop, I. Mixed convection of a hybrid nanofluid flow along a vertical surface embedded in a porous medium. Int. Commun. Heat Mass Transf. 2020, 114, 104565. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Hybrid nanofluid flow past a permeable moving thin needle. Mathematics 2020, 8, 612. [Google Scholar] [CrossRef]
- Hayat, T.; Nadeem, S.; Khan, A.U. Rotating flow of Ag-CuO/H2O hybrid nanofluid with radiation and partial slip boundary effects. Eur. Phys. J. E 2018, 41, 75. [Google Scholar] [CrossRef] [PubMed]
- Yousefi, M.; Dinarvand, S.; Eftekhari Yazdi, M.; Pop, I. Stagnation-point flow of an aqueous titania-copper hybrid nanofluid toward a wavy cylinder. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 1716–1735. [Google Scholar] [CrossRef]
- Rostami, M.N.; Dinarvand, S.; Pop, I. Dual solutions for mixed convective stagnation-point flow of an aqueous silica–alumina hybrid nanofluid. Chinese J. Phys. 2018, 56, 2465–2478. [Google Scholar] [CrossRef]
- Subhani, M.; Nadeem, S. Numerical analysis of micropolar hybrid nanofluid. Appl. Nanosci. 2019, 9, 447–459. [Google Scholar] [CrossRef]
- Aly, E.H.; Pop, I. MHD flow and heat transfer over a permeable stretching/shrinking sheet in a hybrid nanofluid with a convective boundary condition. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 3012–3038. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N.; Pop, I. Magnetohydrodynamics (MHD) axisymmetric flow and heat transfer of a hybrid nanofluid past a radially permeable stretching/shrinking sheet with Joule heating. Chinese J. Phys. 2020, 64, 251–263. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Raza, J.; Khan, I. Magnetohydrodynamic flow of Cu–Fe3O4/H2O hybrid nanofluid with effect of viscous dissipation: Dual similarity solutions. J. Therm. Anal. Calorim. 2020. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D. Nanofluid convective heat transfer using semi analytical and numerical approaches: A review. J. Taiwan Inst. Chem. Eng. 2016, 65, 43–77. [Google Scholar] [CrossRef]
- Myers, T.G.; Ribera, H.; Cregan, V. Does mathematics contribute to the nanofluid debate? Int. J. Heat Mass Transf. 2017, 111, 279–288. [Google Scholar] [CrossRef]
- Khanafer, K.; Vafai, K. Applications of nanofluids in porous medium A critical review. J. Therm. Anal. Calorim. 2019, 135, 1479–1492. [Google Scholar] [CrossRef]
- Sarkar, J.; Ghosh, P.; Adil, A. A review on hybrid nanofluids: Recent research, development and applications. Renew. Sustain. Energy Rev. 2015, 43, 164–177. [Google Scholar] [CrossRef]
- Sidik, N.A.C.; Adamu, I.M.; Jamil, M.M.; Kefayati, G.H.R.; Mamat, R.; Najafi, G. Recent progress on hybrid nanofluids in heat transfer applications: A comprehensive review. Int. Commun. Heat Mass Transf. 2016, 78, 68–79. [Google Scholar] [CrossRef]
- Sundar, L.S.; Sharma, K.V.; Singh, M.K.; Sousa, A.C.M. Hybrid nanofluids preparation, thermal properties, heat transfer and friction factor–A review. Renew. Sustain. Energy Rev. 2017, 68, 185–198. [Google Scholar] [CrossRef]
- Babu, J.A.R.; Kumar, K.K.; Rao, S.S. State-of-art review on hybrid nanofluids. Renew. Sustain. Energy Rev. 2017, 77, 551–565. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. Hybrid nanofluids for heat transfer applications–A state-of-the-art review. Int. J. Heat Mass Transf. 2018, 125, 82–103. [Google Scholar] [CrossRef]
- Ahmadi, M.H.; Mirlohi, A.; Alhuyi Nazari, M.; Ghasempour, R. A review of thermal conductivity of various nanofluids. J. Mol. Liq. 2018, 265, 181–188. [Google Scholar] [CrossRef]
- Datskos, P.G.; Oden, P.I.; Thundat, T.; Wachter, E.A.; Warmack, R.J.; Hunter, S.R. Remote infrared radiation detection using piezoresistive microcantilevers. Appl. Phys. Lett. 1996, 69, 2986–2988. [Google Scholar] [CrossRef]
- Hussain, A.; Zetoon, R.; Ali, S.; Nadeem, S. Magnetically driven flow of pseudoplastic fluid across a sensor surface. J. Brazilian Soc. Mech. Sci. Eng. 2019, 41, 185. [Google Scholar] [CrossRef]
- Khaled, A.R.A.; Vafai, K. Hydromagnetic squeezed flow and heat transfer over a sensor surface. Int. J. Eng. Sci. 2004, 42, 509–519. [Google Scholar] [CrossRef]
- Mahmood, M.; Asghar, S.; Hossain, M.A. Squeezed flow and heat transfer over a porous surface for viscous fluid. Heat Mass Transf. 2007, 44, 165–173. [Google Scholar] [CrossRef]
- Hayat, T.; Hussain, M.; Nadeem, S.; Alsaedi, A.; Obaidat, S. Squeezed flow and heat transfer in a second grade fluid over a sensor surface. Therm. Sci. 2014, 18, 357–364. [Google Scholar] [CrossRef]
- Khan, M.; Malik, M.Y.; Salahuddin, T.; Khan, I. Heat transfer squeezed flow of Carreau fluid over a sensor surface with variable thermal conductivity: A numerical study. Results Phys. 2016, 6, 940–945. [Google Scholar] [CrossRef]
- Salahuddin, T.; Malik, M.Y.; Hussain, A.; Bilal, S.; Awais, M.; Khan, I. MHD squeezed flow of Carreau-Yasuda fluid over a sensor surface. Alexandria Eng. J. 2017, 56, 27–34. [Google Scholar] [CrossRef]
- Ganesh Kumar, K.; Gireesha, B.J.; Krishanamurthy, M.R.; Rudraswamy, N.G. An unsteady squeezed flow of a tangent hyperbolic fluid over a sensor surface in the presence of variable thermal conductivity. Results Phys. 2017, 7, 3031–3036. [Google Scholar] [CrossRef]
- Ul Haq, R.; Nadeem, S.; Khan, Z.H.; Noor, N.F.M. MHD squeezed flow of water functionalized metallic nanoparticles over a sensor surface. Phys. E 2015, 73, 45–53. [Google Scholar] [CrossRef]
- Hamzah, N.S.A.; Kandasamy, R.; Muhammad, R. Thermal radiation energy on squeezed MHD flow of Cu, Al2O3 and CNTs-nanofluid over a sensor surface. Alexandria Eng. J. 2016, 55, 2405–2421. [Google Scholar] [CrossRef]
- Akbar, N.S.; Khan, Z.H. Variable fluid properties analysis with water based CNT nanofluid over a sensor sheet: Numerical solution. J. Mol. Liq. 2017, 232, 471–477. [Google Scholar] [CrossRef]
- Kandasamy, R.; Mohammad, R.; Zailani, N.A.B.M.; Jaafar, N.F.B. Nanoparticle shapes on squeezed MHD nanofluid flow over a porous sensor surface. J. Mol. Liq. 2017, 233, 156–165. [Google Scholar] [CrossRef]
- Nayak, M.K.; Bhatti, M.M.; Makinde, O.D.; Akbar, N.S. Transient magneto-squeezing flow of NaCl-CNP nanofluid over a sensor surface inspired by temperature dependent viscosity. Defect Diffus. Forum 2018, 387, 600–614. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Rosseland, S. Astrophysik und Atom-Theoretische Grundlagen; Springer: Berlin, Germany, 1931; ISBN 9783662245330. [Google Scholar]
- Cortell, R. Heat and fluid flow due to non-linearly stretching surfaces. Appl. Math. Comput. 2011, 217, 7564–7572. [Google Scholar] [CrossRef]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Raza, J.; Rohni, A.; Omar, Z. Numerical investigation of copper-water (Cu-water) nanofluid with different shapes of nanoparticles in a channel with stretching wall: Slip effects. Math. Comput. Appl. 2016, 21, 43. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Shampine, L.F.; Gladwell, I.; Thompson, S. Solving ODEs with MATLAB; Cambridge University Press: Cambridge, UK, 2003; ISBN 9780521824040. [Google Scholar]
- Soid, S.K.; Ishak, A.; Pop, I. MHD stagnation-point flow over a stretching/shrinking sheet in a micropolar fluid with a slip boundary. Sains Malays 2018, 47, 2907–2916. [Google Scholar] [CrossRef]
- Kamal, F.; Zaimi, K.; Ishak, A.; Pop, I. Stability analysis of MHD Stagnation-point flow towards a permeable stretching/shrinking sheet in a nanofluid with chemical reactions effect. Sains Malays 2019, 48, 243–250. [Google Scholar] [CrossRef]
- Jusoh, R.; Nazar, R.; Pop, I. Magnetohydrodynamic boundary layer flow and heat transfer of nanofluids past a bidirectional exponential permeable stretching/shrinking sheet with viscous dissipation effect. J. Heat Transfer 2019, 141, 012406. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. On the stability of the flow and heat transfer over a moving thin needle with prescribed surface heat flux. Chinese J. Phys. 2019, 60, 651–658. [Google Scholar] [CrossRef]
- Khashi’ie, N.S.; Arifin, N.M.; Nazar, R.; Hafidzuddin, E.H.; Wahi, N.; Pop, I. A stability analysis for magnetohydrodynamics stagnation point flow with zero nanoparticles flux condition and anisotropic slip. Energies 2019, 12, 1268. [Google Scholar] [CrossRef]
- Sparrow, E.M.; Eckert, E.R.G.; Minkowycz, W.J. Transpiration cooling in a magneto-hydrodynamic stagnation-point flow. Appl. Sci. Res. 1963, 11, 125–147. [Google Scholar] [CrossRef]
- Yih, K.A. The effect of uniform suction/blowing on heat transfer of magnetohydrodynamic Hiemenz flow through porous media. Acta Mech. 1998, 130, 147–158. [Google Scholar] [CrossRef]
- Ridha, A. Aiding flows non-unique similarity solutions of mixed-convection boundary-layer equations. Zeitschrift fur Angew. Math. Phys. 1996, 47, 341–352. [Google Scholar] [CrossRef]
- Ishak, A.; Merkin, J.H.; Nazar, R.; Pop, I. Mixed convection boundary layer flow over a permeable vertical surface with prescribed wall heat flux. Zeitschrift fur Angew. Math. Phys. 2008, 59, 100–123. [Google Scholar] [CrossRef]





| Thermophysical Properties | Nanofluid | Hybrid Nanofluid |
|---|---|---|
| Density | ||
| Heat capacity | ||
| Dynamic viscosity | ||
| Thermal conductivity | where | |
| Electrical conductivity | where |
| Thermophysical Properties | Al2O3 | Cu | Water |
|---|---|---|---|
| 3970 | 8933 | 997.1 | |
| 765 | 385 | 4179 | |
| 40 | 400 | 0.613 | |
| 3.69 × 107 | 5.96 × 107 | 0.05 | |
| Prandtl number, | 6.2 |
| Sparrow et al. [57] | Yih [58] | Present Results | ||
|---|---|---|---|---|
| 0 | 1 | 0.7605 | 0.756575 | 0.756575 |
| 0 | 1.231 | 1.232588 | 1.232588 | |
| −1 | 1.889314 | 1.889314 | ||
| 1 | 1 | 1.124 | 1.116421 | 1.116421 |
| 0 | 1.584 | 1.585331 | 1.585331 | |
| −1 | 2.202940 | 2.202940 |
| Ul Haq et al. [38] | Present Results | ||||
|---|---|---|---|---|---|
| 0 | −0.5 | 1.718541 | 3.840316 | ||
| 0.5 | 1.162236 | 0.46815 | 1.162236 | 0.468150 | |
| 1 | −0.5 | 1.481134 | 4.696999 | 1.481134 | 4.696999 |
| 0.5 | 0.866523 | 1.208932 | 0.866523 | 1.208932 | |
| 2 | −0.5 | 1.222208 | 5.380508 | ||
| 0.5 | 0.506833 | 1.839583 | |||
| 0 | 0 | 0 | 0 | 0 | 1.232588 | 1.573433 | 1.602057 | 1.860326 |
| 0.02 | 1.361008 | 1.655977 | 1.737286 | 1.947250 | ||||
| 0.04 | 1.489346 | 1.736260 | 1.874379 | 2.033031 | ||||
| 0.04 | 0.5 | 1.310038 | 2.261957 | 1.648715 | 2.611603 | |||
| 1 | 1.115515 | 2.712764 | 1.403904 | 3.110646 | ||||
| 2 | 0.668444 | 3.469936 | 0.841253 | 3.952873 | ||||
| 2 | 0.2 | 0.801908 | 3.489410 | 1.024317 | 3.978345 | |||
| 0.4 | 0.922159 | 3.506375 | 1.187917 | 4.000253 | ||||
| 0.6 | 1.032259 | 3.521447 | 1.336850 | 4.019529 | ||||
| 0.2 | 0.2 | 0.801908 | 3.903484 | 1.024317 | 4.342731 | |||
| 0.4 | 0.801908 | 4.282869 | 1.024317 | 4.682781 | ||||
| 0.6 | 0.801908 | 4.635574 | 1.024317 | 5.003124 | ||||
| 0.2 | −0.2 | 1.012031 | 4.672733 | 1.278661 | 5.091581 | |||
| 0 | 0.801908 | 3.903484 | 1.024317 | 4.342731 | ||||
| 0.2 | 0.596024 | 3.214263 | 0.775292 | 3.661256 | ||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Waini, I.; Ishak, A.; Pop, I. Squeezed Hybrid Nanofluid Flow Over a Permeable Sensor Surface. Mathematics 2020, 8, 898. https://doi.org/10.3390/math8060898
Waini I, Ishak A, Pop I. Squeezed Hybrid Nanofluid Flow Over a Permeable Sensor Surface. Mathematics. 2020; 8(6):898. https://doi.org/10.3390/math8060898
Chicago/Turabian StyleWaini, Iskandar, Anuar Ishak, and Ioan Pop. 2020. "Squeezed Hybrid Nanofluid Flow Over a Permeable Sensor Surface" Mathematics 8, no. 6: 898. https://doi.org/10.3390/math8060898
APA StyleWaini, I., Ishak, A., & Pop, I. (2020). Squeezed Hybrid Nanofluid Flow Over a Permeable Sensor Surface. Mathematics, 8(6), 898. https://doi.org/10.3390/math8060898







