On the Efficient Monitoring of Multivariate Processes with Unknown Parameters
Abstract
1. Introduction
2. Preliminaries and Existing Multivariate Control Charts
2.1. Existing Multivariate Charts
2.1.1. and Hotelling’s Charts
2.1.2. Chart
2.1.3. Chart
2.1.4. Chart
2.1.5. Chart
3. The Proposed Control Chart for Subgroups
Estimation of Unknown Parameters for MHWMAP Chart for Subgroups
4. Performance Evaluations and Comparisons
4.1. Monte Carlo Simulation Procedure
- Generate the random samples from multivariable normal distribution and compute the values of the charting statistics Hotelling’s,, , , , and .
- Establish the control limits for all charting statistics.
- Calculate the values of ARL and SDRL for each charting statistics at changing shift values (0.50, 1.0, 1.5, 2.0, 2.5, and 3) for fixed.
- Multiply ARL with and inverse of range of values as .
- Get by integrating the result (obtained in step i) over range using the Simpson or Trapezoidal integration technique.
- Take the ratio of the s of a specific to optimum/standard charts and multiply with the inverse of entire range of .
- Calculate by integrating the output over range.
- Get PCI by taking the ratio of of a particular chart to the optimum/standard chart.
4.2. Performance Analysis and Comparisons
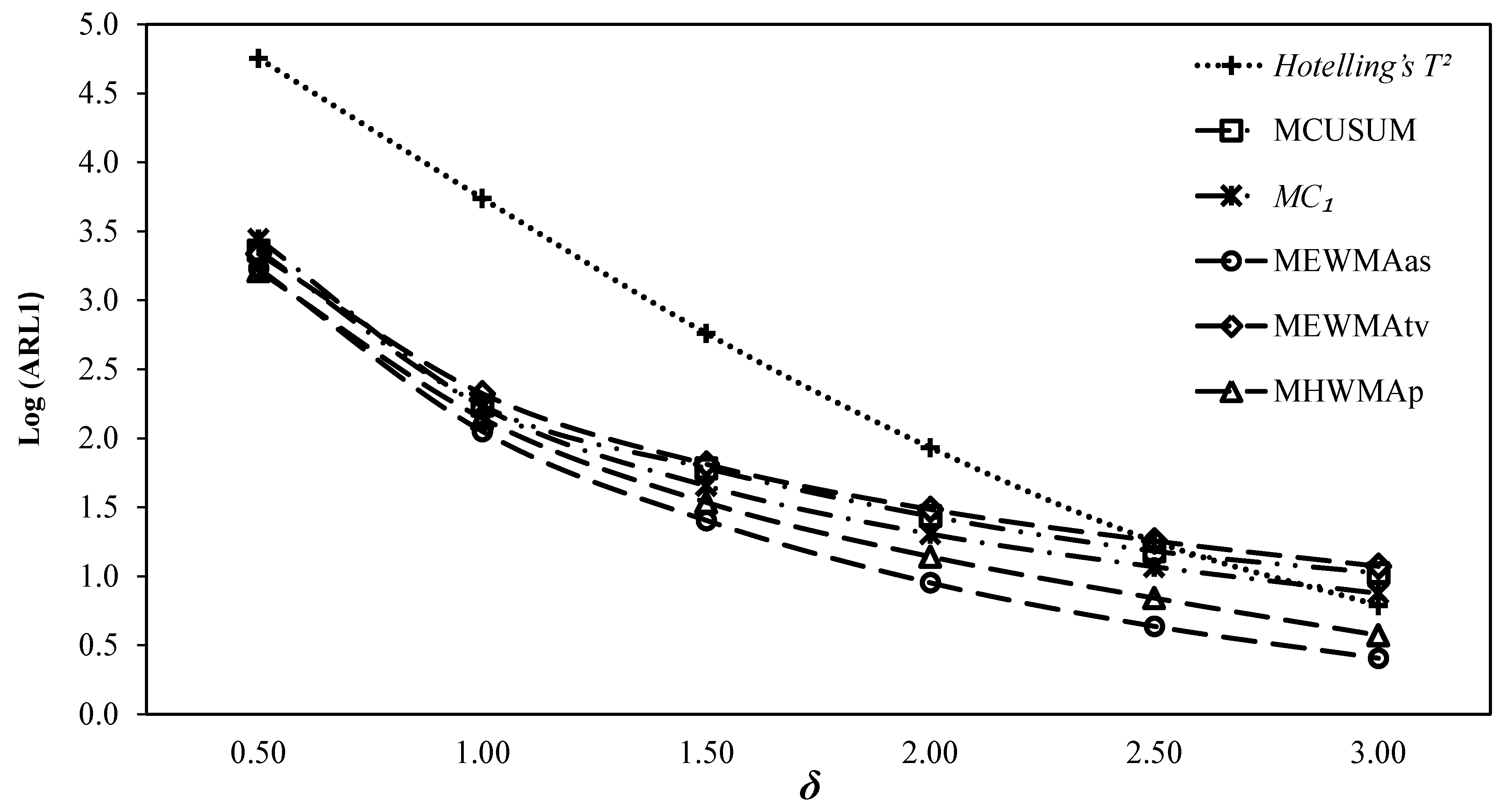
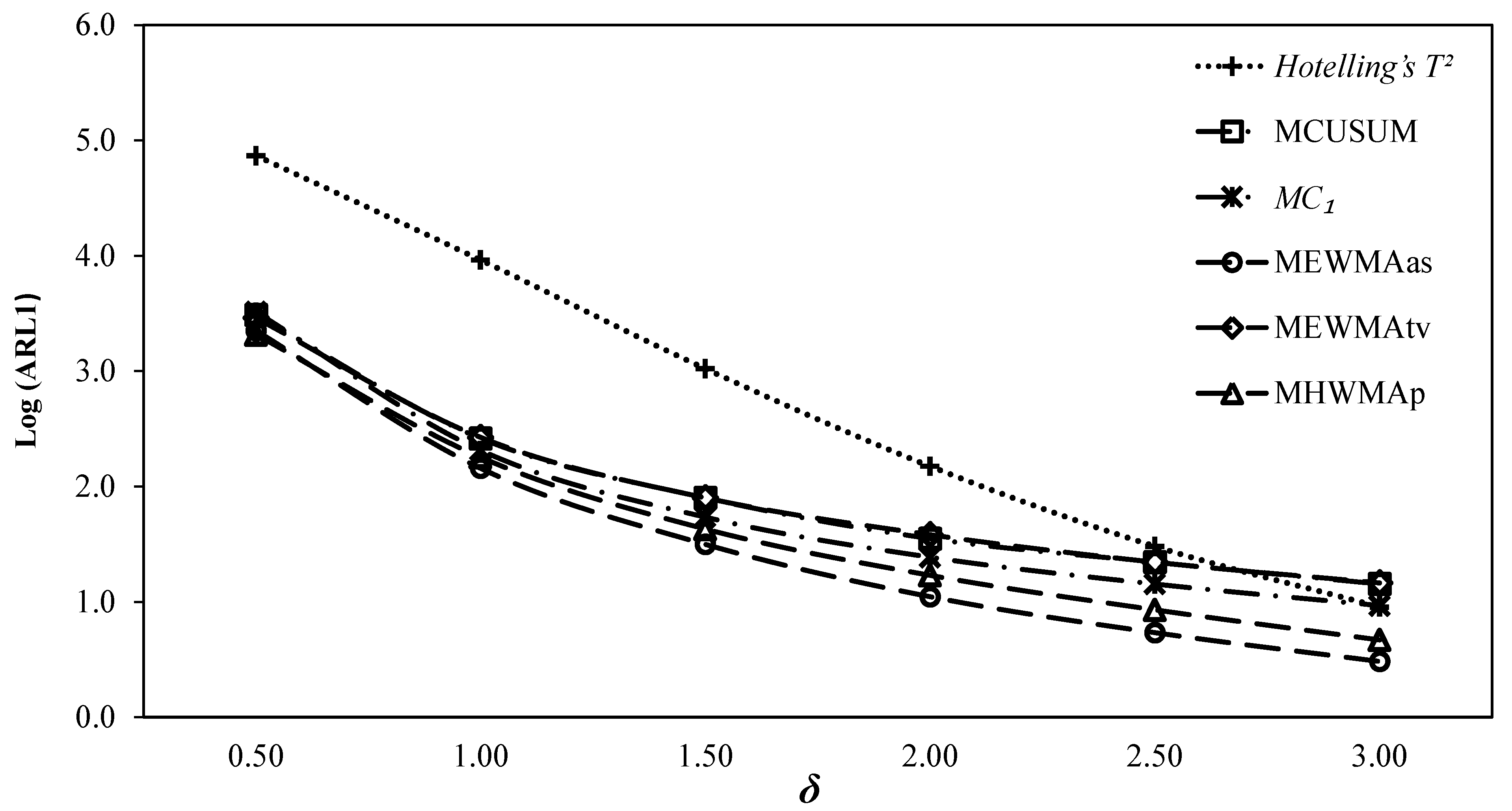
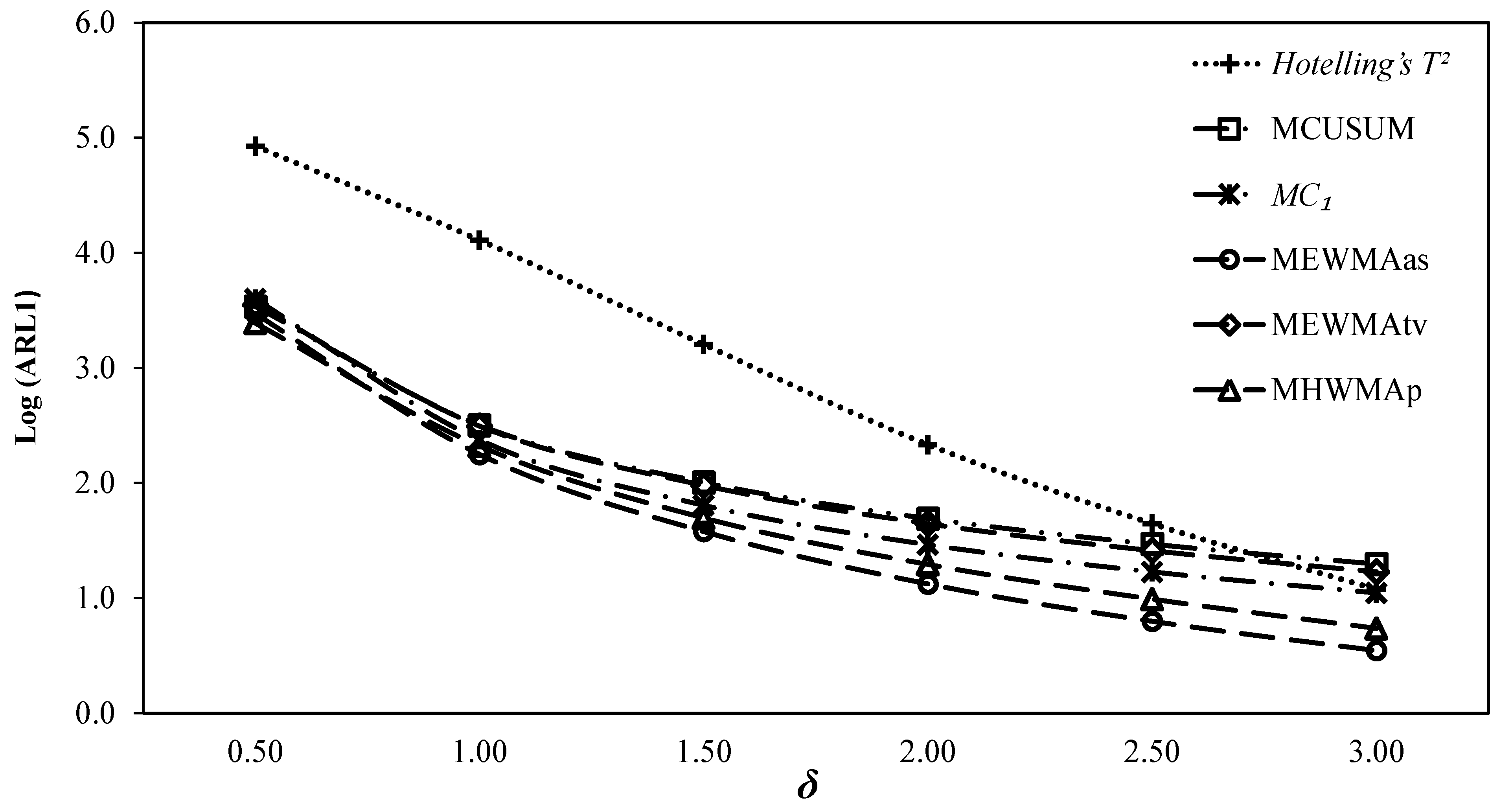
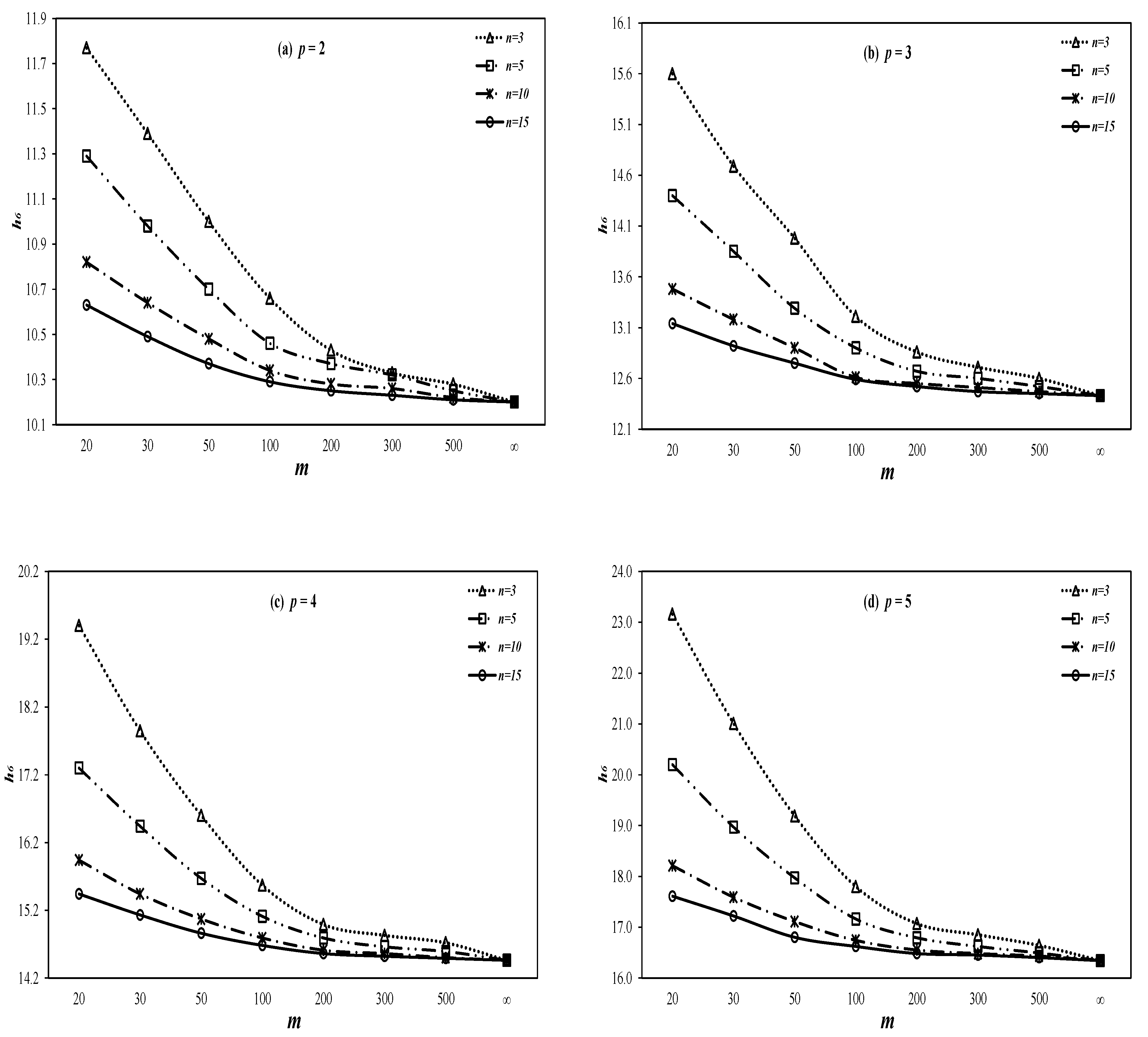
- The values of the proposed and other competing charts decrease as the value of decreases, and vice versa. For example, at and 2, 3, 4, 5, 10, the values of chart are 24.80, 27.55, 29.61, 31.56, and 37.87, respectively. This reveals that the detection ability of charts improves as the number of variables increases in multivariate monitoring. A similar type of behavior can be observed for other charts (see Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5).
- The chart exhibits the best performance at small followed by. For example, at, the values of chart are 3.44, 3.70, 3.95, and 4.73, while those of the chart are 3.56, 4.56, 4.29, and 5.54 for 2, 3, 4, and 5, respectively. At, the values of chart are 5.10, 5.72, 6.38, and 6.84, while those of the chart are 5.23, 5.82, 6.25, and 6.65 for 2, 3, 4, and 5, respectively (see Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5).
- The Hotelling’s chart exhibits the most inferior performance among all charts. For example, for and , the for Hotelling’s chart, while 28.8, 31.3, 25.3, 28.1, and 24.8 for , , , , and charts, respectively. Similarly, for and , the for Hotelling’s chart, while 43.2, 43.9, 44.3, 44.1, and 37.87 for , , , , and charts, respectively. The inferiority of Hotelling’s chart can be observed for other choices of (see Figure 1, Figure 2, Figure 3, Figure 4 and Figure 5).
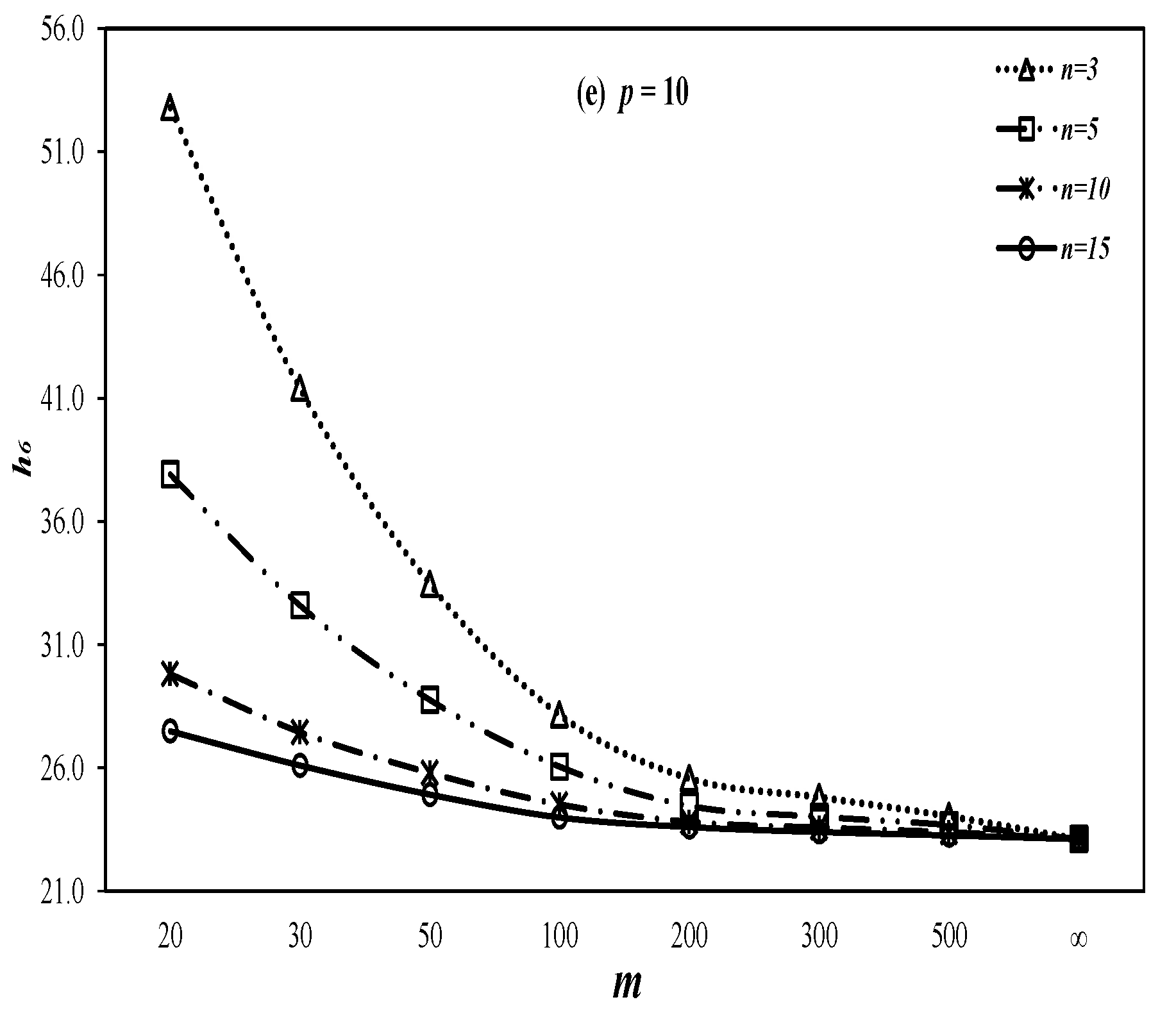
- The values are inversely related with the value of in the case of estimated parameters. This means that, as the number of variables increases in the multivariate set up, the values decrease. For example, for, , and , the 91.12, 65.51, 48.25, 36.36, and 8.87 at 2, 3, 4, 5, and 10, respectively (see Table 5). We may also oberserv behavior of the 115.34, 83.32, 64.08, 51.48, and 19.13 at 2, 3, 4, 5, and 10, respectively (see Table 6).
- The values based on estimated parameters decrease as the value of smoothing parameter increases. This means that the in-control moved downward as . For example, at the = 132.80, 147.64, 160.42, 167.85, 173.39, and 178.77 at respectively (see Table 5). Similarly, we may observe the results at , where the = 131.47, 163.29, 198.09, 212.99, 222.16, and 229.72 at respectively (see Table 6).
- As the sample size and/or sub-group size increase, the false alarms produced by chart decrease, which means that the estimated version of converges to as. For instance, for, as increases from 3 to 15, the increases from 110.26 to 169.61.72 at ; as increases from 30 to 500, the increases from 110.26 to 187.72 at (see Table 5). Similar behavior of the results can be observed as , because, if increases from 3 to 15, the increases from 122.86 to 145.34 at ; if increases from 30 to 500, the increases from 122.86 to 155.36 at (see Table 6).
5. An Application
6. Summary, Conclusions, and Future Recommendations
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| X | Vector of multivariate variables |
| Mean vector | |
| Variance–covariance matrix | |
| Number of variables | |
| Multivariate normal distribution | |
| As the () observation of the () quality Charectersitic on the () sample | |
| sample mean vector | |
| sample variance–covariance matrix | |
| Known mean vector | |
| Known variance–covariance matrix | |
| Estimator of | |
| Estimator of | |
| Chi-qquare probability distribution with t degrees of freedom | |
| Control limit of Hotelling’s charts when and are used | |
| Plotting statistics of Hotelling’s chart when and are estimated | |
| Control limit Hotelling’s chart when and are used | |
| Distribution with degree of freedom and | |
| Charting statistic of chart | |
| Charting statistic of chart | |
| Constant of chart | |
| Plotting statistic of chart | |
| Vector forchart | |
| Number of subgroups | |
| Plotting statistic ofchart | |
| Control limit of chart | |
| Scalar observation for chart | |
| Plotting statistic of chart | |
| Control limit of chart | |
| Observations vector for the chart | |
| Vector of constant | |
| Identity matrix | |
| Plotting statistic of Chart | |
| Asymptotic variance-covariance matrix of Chart | |
| Control limit of Chart | |
| Observations vector for control chart | |
| Plotting statistic of control chart | |
| Inverse variance covariance matric for control chart | |
| Control limit of control chart | |
| Represents the variance of statistic when = 1 | |
| Observations vector for chart | |
| Plotting statistic of chart | |
| Inverse variance covariance matric for the chart | |
| Control limit of the chart | |
| Estimator of | |
| Estimator of | |
| Multivariate normal distribution of | |
| shift | |
| Out-of-control mean vector | |
| Maximum value of | |
| Minimum value of |
Appendix A
| 1 | |
| 2 | |
- For an in-control processNow,By substituting from (A1), becomesFor an in-control process and withAs and are vectors consisting of independent random sample values, therefore,, soNow,As and are independent sample means vector, therefore, soBy substituting from (A3), becomesAs the variance of a constant is zero, hence
- For an in-control processAs are the sample means vector of first sample, respectively, and all these means vector are independent of the next, that is, means vector. This indicates that, hence
- For in-control process
- For in-control processAs the estimators of and are and, hence (A6) and (A7) become the following, respectively:and
References
- Hotelling, H. Multivariate Quality Control-Illustrated by the Air Testing of Sample Bombsights; Mcgraw-Hill: New York, NY, USA, 1947. [Google Scholar]
- Montgomery, D.C. Introduction to Statistical Quality Control, 8th ed.; Wiley: Hoboken, NJ, USA, 2019. [Google Scholar]
- Lowry, C.A.; Montgomery, D.C. A review of multivariate control charts. IIE Trans. 1995, 27, 800–810. [Google Scholar] [CrossRef]
- Mahmoud, M.A.; Maravelakis, P.E. The Performance of the MEWMA Control Chart when Parameters are Estimated. Commun. Stat. Simul. Comput. 2010, 39, 1803–1817. [Google Scholar] [CrossRef]
- Crosier, R.B. Multivariate Generalizations of Cumulative Sum Quality-Control Schemes. Technometrics 1988, 30, 291–303. [Google Scholar] [CrossRef]
- Pignatiello, J.J.; Runger, J.C. Comparisons of Multivariate CUSUM Charts. J. Qual. Technol. 1990, 22, 173–186. [Google Scholar] [CrossRef]
- Santos-Fernandez, E. Multivariate Statistical Quality Control Using R; Springer: New York, NY, USA, 2013; ISBN 978-1-4614-5452-6. [Google Scholar]
- Tracy, N.D.; Young, J.C.; Mason, R.L. Multivariate Control Charts for Individual Observations. J. Qual. Technol. 1992, 24, 88–95. [Google Scholar] [CrossRef]
- Woodall, W.H.; Ncube, M.M. Multivariate CUSUM Quality-Control Procedures. Technometrics 1985, 27, 285–292. [Google Scholar] [CrossRef]
- Page, E.S. Continuous Inspection Schemes. Biometrika 1954, 41, 100–115. [Google Scholar] [CrossRef]
- Lowry, C.A.; Woodall, W.H.; Champ, C.W.; Rigdon, S.E. A Multivariate Exponentially Weighted Moving Average Control Chart. Technometrics 1992, 34, 46–53. [Google Scholar] [CrossRef]
- Roberts, S.W. Control Chart Tests Based on Geometric Moving Averages. Technometrics 1959, 1, 239–250. [Google Scholar] [CrossRef]
- Mahmoud, M.A.; Zahran, A.R. A Multivariate Adaptive Exponentially Weighted Moving Average Control Chart. Commun. Stat. Theory Methods 2010, 39, 606–625. [Google Scholar] [CrossRef]
- Waterhouse, M.; Smith, I.; Assareh, H.; Mengersen, K. Implementation of multivariate control charts in a clinical setting. Int. J. Qual. Heal. Care 2010, 22, 408–414. [Google Scholar] [CrossRef] [PubMed]
- Jiang, W.; Wang, K.; Tsung, F. A Variable-Selection-Based Multivariate EWMA Chart for Process Monitoring and Diagnosis. J. Qual. Technol. 2012, 44, 209–230. [Google Scholar] [CrossRef]
- Lee, M.H. Variable Sampling Rate Multivariate Exponentially Weighted Moving Average Control Chart with Double Warning Lines. Qual. Technol. Quant. Manag. 2013, 10, 353–368. [Google Scholar] [CrossRef]
- Shen, X.; Tsung, F.; Zou, C. A new multivariate EWMA scheme for monitoring covariance matrices. Int. J. Prod. Res. 2014, 52, 2834–2850. [Google Scholar] [CrossRef]
- Park, J.; Jun, C.-H. A new multivariate EWMA control chart via multiple testing. J. Process Control 2015, 26, 51–55. [Google Scholar] [CrossRef]
- Tsung, F.; Wang, K. Adaptive Charting Techniques: Literature Review and Extensions BT—Frontiers in Statistical Quality Control 9; Lenz, H.-J., Wilrich, P.-T., Schmid, W., Eds.; Physica-Verlag HD: Heidelberg, Germany, 2010; pp. 19–35. ISBN 978-3-7908-2380-6. [Google Scholar]
- Psarakis, S. Adaptive Control Charts: Recent Developments and Extensions. Qual. Reliab. Eng. Int. 2015, 31, 1265–1280. [Google Scholar] [CrossRef]
- Kang, J.H.; Yu, J.; Kim, S.B. Adaptive nonparametric control chart for time-varying and multimodal processes. J. Process Control 2016, 37, 34–45. [Google Scholar] [CrossRef]
- Aslam, M.; AL-Marshadi, H.A.; Khan, N. A New X-Bar Control Chart for Using Neutrosophic Exponentially Weighted Moving Average. Mathematics 2019, 7, 957. [Google Scholar] [CrossRef]
- Ajadi, J.O.; Riaz, M. Mixed multivariate EWMA-CUSUM control charts for an improved process monitoring. Commun. Stat. Theory Methods 2017, 46, 6980–6993. [Google Scholar] [CrossRef]
- Adegoke, N.A.; Abbasi, S.A.; Smith, A.N.H.; Anderson, M.J.; Pawley, M.D.M. A Multivariate Homogeneously Weighted Moving Average Control Chart. IEEE Access 2019, 7, 9586–9597. [Google Scholar] [CrossRef]
- Abbas, N. Homogeneously weighted moving average control chart with an application in substrate manufacturing process. Comput. Ind. Eng. 2018, 120, 460–470. [Google Scholar] [CrossRef]
- Bersimis, S.; Panaretos, J.; Psarakis, S. Multivariate Statistical Process Control Charts and the Problem of Interpretation: A Short Overview and Some Applications in Industry. In Proceedings of the 7th Hellenic European Conference on Computer Mathematics and Its Applications, Athens, Greece, 22–24 September 2005. [Google Scholar]
- Anderson, T.W. An Introduction to Multivariate Statistical Analysis, 3rd ed.; Wiley: Hoboken, NJ, USA, 2003; ISBN 978-0-471-36091-9. [Google Scholar]
- Wu, Z.; Jiao, J.; Yang, M.; Liu, Y.; Wang, Z. An enhanced adaptive CUSUM control chart. IIE Trans. 2009, 41, 642–653. [Google Scholar] [CrossRef]
- Riaz, M.; Does, R.J.M.M. An Alternative to the Bivariate Control Chart for Process Dispersion. Qual. Eng. 2008, 21, 63–71. [Google Scholar] [CrossRef]
- Abbasi, S.A.; Miller, A. MDEWMA chart: An efficient and robust alternative to monitor process dispersion. J. Stat. Comput. Simul. 2013, 83, 247–268. [Google Scholar] [CrossRef]
- Abid, M.; Nazir, H.Z.; Riaz, M.; Lin, Z. An Efficient Nonparametric EWMA Wilcoxon Signed-Rank Chart for Monitoring Location. Qual. Reliab. Eng. Int. 2017, 33, 669–685. [Google Scholar] [CrossRef]
- Ahmad, S.; Riaz, M.; Abbasi, S.A.; Lin, Z. On efficient median control charting. J. Chin. Inst. Eng. 2014, 37, 358–375. [Google Scholar] [CrossRef]
- Mahmood, T.; Riaz, M.; Omar, H.M.; Xie, M. Alternative methods for the simultaneous monitoring of simple linear profile parameters. Int. J. Adv. Manuf. Technol. 2018, 97, 2851–2871. [Google Scholar] [CrossRef]
- Haridy, S.; Maged, A.; Kaytbay, S.; Araby, S. Effect of sample size on the performance of Shewhart control charts. Int. J. Adv. Manuf. Technol. 2017, 90, 1177–1185. [Google Scholar] [CrossRef]
- Reynolds, M.R.; Stoumbos, Z.G. Control Charts and the Efficient Allocation of Sampling Resources. Technometrics 2004, 46, 200–214. [Google Scholar] [CrossRef]
- Ou, Y.; Wen, D.; Wu, Z.; Khoo, M.B. A comparison study on effectiveness and robustness of control charts for monitoring process mean and variance. Qual. Reliab. Eng. Int. 2012, 28, 3–17. [Google Scholar] [CrossRef]
- Ou, Y.; Wu, Z.; Tsung, F. A comparison study of effectiveness and robustness of control charts for monitoring process mean. Int. J. Prod. Econ. 2012, 135, 479–490. [Google Scholar] [CrossRef]
- Robert, C.P.; Casella, G. Monte Carlo Statistical Methods; Springer: New York, NY, USA, 2004; ISBN 978-1-4419-1939-7. [Google Scholar]
- Robert, C.P.; Casella, G. Introducing Monte Carlo Methods with R; Springer: New York, NY, USA, 2010; ISBN 978-1-4419-1575-7. [Google Scholar]
- Mundform, D.J.; Schaffer, J.R.; Kim, M.-J.; Shaw, D.; Thongteeraparp, A.; Supawan, P. Number of Replications Required in Monte Carlo Simulation Studies: A Synthesis of Four Studies. J. Mod. Appl. Stat. Methods 2011, 10, 4. [Google Scholar] [CrossRef]
- Jones, O.; Maillardet, R.; Robinson, A. Introduction to Scientific Programming and Simulation Using R, 2nd ed.; Chapman and Hall/CRC: London, UK, 2014; ISBN 9781466569997. [Google Scholar]
- Kim, M.-J. Number of Replications Required in Control Chart Monte Carlo Simulation Studies. Ph.D. Thesis, University of Northern Colorado, Greeley, CO, USA, 2005. [Google Scholar]
- Schaffer, J.R.; Kim, M.-J. Number of Replications Required in Control Chart Monte Carlo Simulation Studies. Commun. Stat. Simul. Comput. 2007, 36, 1075–1087. [Google Scholar] [CrossRef]
- Khaliq, Q.-U.-A.; Riaz, M.; Ahmad, S. On designing a new Tukey-EWMA control chart for process monitoring. Int. J. Adv. Manuf. Technol. 2016, 82, 1–23. [Google Scholar] [CrossRef]
- Riaz, M.; Mahmood, T.; Abbasi, S.A.; Abbas, N.; Ahmad, S. Linear profile monitoring using EWMA structure under ranked set schemes. Int. J. Adv. Manuf. Technol. 2017, 91, 2751–2775. [Google Scholar] [CrossRef]
- Albers, W.; Kallenberg, W.C.M. Estimation in Shewhart control charts: Effects and corrections. Metrika 2004, 59, 207–234. [Google Scholar] [CrossRef]
- Riaz, M.; Mehmood, R.; Ahmad, S.; Abbasi, S.A. On the Performance of Auxiliary-based Control Charting under Normality and Nonnormality with Estimation Effects. Qual. Reliab. Eng. Int. 2013, 29, 1165–1179. [Google Scholar] [CrossRef]
- Flores, M.; Naya, S.; Fernández-Casal, R.; Zaragoza, S.; Raña, P.; Tarrío-Saavedra, J. Constructing a Control Chart Using Functional Data. Mathematics 2020, 8, 58. [Google Scholar] [CrossRef]





| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 14.50 | 3.60 | 3.91 | 3.16 | 3.51 | 3.10 | 16.25 | 4.09 | 4.19 | 3.56 | 3.98 | 3.44 |
| 1 | 25.00 | 5.94 | 6.23 | 5.10 | 6.06 | 5.23 | 29.40 | 6.89 | 6.71 | 5.72 | 6.80 | 5.82 |
| 1.5 | 29.59 | 7.74 | 7.66 | 6.22 | 8.04 | 6.65 | 36.05 | 8.97 | 8.28 | 6.93 | 8.93 | 7.38 |
| 2 | 30.09 | 9.58 | 9.06 | 7.11 | 9.95 | 7.86 | 37.21 | 10.96 | 9.80 | 7.88 | 11.01 | 8.68 |
| 2.5 | 29.02 | 11.38 | 10.55 | 7.90 | 11.92 | 8.99 | 36.04 | 13.04 | 11.42 | 8.74 | 13.14 | 9.90 |
| 3 | 27.65 | 13.27 | 12.10 | 8.69 | 13.95 | 10.03 | 34.27 | 15.24 | 13.14 | 9.58 | 15.35 | 11.03 |
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 17.25 | 4.28 | 4.56 | 4.01 | 4.34 | 3.70 | 18.13 | 4.65 | 5.49 | 4.29 | 4.71 | 3.95 |
| 1 | 32.48 | 7.33 | 7.25 | 6.38 | 7.36 | 6.25 | 35.15 | 8.15 | 8.64 | 6.84 | 7.94 | 6.65 |
| 1.5 | 41.03 | 9.70 | 8.90 | 7.64 | 9.64 | 7.90 | 45.47 | 10.86 | 10.73 | 8.20 | 10.30 | 8.40 |
| 2 | 42.84 | 12.07 | 10.55 | 8.63 | 11.85 | 9.28 | 48.32 | 13.57 | 13.03 | 9.25 | 12.17 | 9.85 |
| 2.5 | 41.63 | 14.54 | 12.30 | 9.52 | 14.11 | 10.56 | 47.36 | 16.42 | 15.53 | 10.17 | 14.27 | 11.19 |
| 3 | 39.60 | 17.11 | 14.15 | 10.38 | 16.46 | 11.77 | 45.07 | 19.29 | 18.19 | 11.05 | 16.83 | 12.46 |
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | ||||||
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | ||||||
| 0 | NA | NA | NA | NA | NA | NA | ||||||
| 0.5 | 20.25 | 5.40 | 5.49 | 5.54 | 6.01 | 4.73 | ||||||
| 1 | 43.45 | 10.05 | 8.64 | 8.66 | 9.99 | 8.03 | ||||||
| 1.5 | 61.20 | 14.23 | 10.73 | 10.21 | 12.74 | 10.16 | ||||||
| 2 | 68.77 | 18.38 | 13.03 | 11.41 | 15.41 | 11.83 | ||||||
| 2.5 | 69.44 | 22.62 | 15.53 | 12.45 | 18.17 | 13.36 | ||||||
| 3 | 66.93 | 26.90 | 18.19 | 13.44 | 21.03 | 14.84 | ||||||
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 2.84 | 1.08 | 1.14 | 1.01 | 1.07 | 1.00 | 2.86 | 1.09 | 1.11 | 1.02 | 1.08 | 1.00 |
| 1 | 3.90 | 1.12 | 1.17 | 1.00 | 1.13 | 1.01 | 4.05 | 1.15 | 1.13 | 1.00 | 1.13 | 1.01 |
| 1.5 | 4.15 | 1.19 | 1.19 | 1.00 | 1.23 | 1.05 | 4.48 | 1.23 | 1.16 | 1.00 | 1.22 | 1.05 |
| 2 | 3.93 | 1.28 | 1.23 | 1.00 | 1.32 | 1.08 | 4.32 | 1.32 | 1.20 | 1.00 | 1.32 | 1.08 |
| 2.5 | 3.59 | 1.36 | 1.28 | 1.00 | 1.41 | 1.11 | 3.98 | 1.40 | 1.26 | 1.00 | 1.41 | 1.10 |
| 3 | 3.27 | 1.43 | 1.33 | 1.00 | 1.49 | 1.12 | 3.62 | 1.49 | 1.31 | 1.00 | 1.49 | 1.12 |
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 2.83 | 1.08 | 1.12 | 1.05 | 1.09 | 1.00 | 2.80 | 1.09 | 1.20 | 1.04 | 1.10 | 1.00 |
| 1 | 4.08 | 1.13 | 1.13 | 1.03 | 1.13 | 1.00 | 4.12 | 1.16 | 1.24 | 1.03 | 1.15 | 1.00 |
| 1.5 | 4.59 | 1.21 | 1.13 | 1.00 | 1.20 | 1.02 | 4.72 | 1.25 | 1.25 | 1.00 | 1.20 | 1.01 |
| 2 | 4.50 | 1.32 | 1.18 | 1.00 | 1.30 | 1.05 | 4.70 | 1.37 | 1.34 | 1.00 | 1.26 | 1.05 |
| 2.5 | 4.17 | 1.43 | 1.24 | 1.00 | 1.39 | 1.08 | 4.39 | 1.50 | 1.44 | 1.00 | 1.33 | 1.07 |
| 3 | 3.81 | 1.53 | 1.30 | 1.00 | 1.48 | 1.10 | 4.03 | 1.61 | 1.54 | 1.00 | 1.43 | 1.10 |
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | ||||||
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | ||||||
| 0 | NA | NA | NA | NA | NA | NA | ||||||
| 0.5 | 2.64 | 1.07 | 1.08 | 1.09 | 1.13 | 1.00 | ||||||
| 1 | 4.15 | 1.17 | 1.07 | 1.07 | 1.19 | 1.00 | ||||||
| 1.5 | 5.01 | 1.30 | 1.06 | 1.02 | 1.21 | 1.00 | ||||||
| 2 | 5.32 | 1.49 | 1.11 | 1.00 | 1.29 | 1.02 | ||||||
| 2.5 | 5.13 | 1.66 | 1.19 | 1.00 | 1.38 | 1.05 | ||||||
| 3 | 4.78 | 1.82 | 1.28 | 1.00 | 1.47 | 1.08 | ||||||
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 4.68 | 1.16 | 1.26 | 1.02 | 1.13 | 1.00 | 4.72 | 1.19 | 1.22 | 1.03 | 1.15 | 1.00 |
| 1 | 4.90 | 1.16 | 1.22 | 1.00 | 1.19 | 1.03 | 5.14 | 1.20 | 1.17 | 1.00 | 1.19 | 1.02 |
| 1.5 | 4.76 | 1.24 | 1.23 | 1.00 | 1.29 | 1.07 | 5.20 | 1.29 | 1.19 | 1.00 | 1.29 | 1.07 |
| 2 | 4.23 | 1.35 | 1.28 | 1.00 | 1.40 | 1.11 | 4.72 | 1.39 | 1.24 | 1.00 | 1.40 | 1.10 |
| 2.5 | 3.67 | 1.44 | 1.33 | 1.00 | 1.51 | 1.14 | 4.12 | 1.49 | 1.31 | 1.00 | 1.50 | 1.13 |
| 3 | 3.18 | 1.53 | 1.39 | 1.00 | 1.60 | 1.15 | 3.58 | 1.59 | 1.37 | 1.00 | 1.60 | 1.15 |
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp |
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 |
| 0 | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA | NA |
| 0.5 | 4.66 | 1.16 | 1.23 | 1.08 | 1.17 | 1.00 | 4.59 | 1.18 | 1.39 | 1.09 | 1.19 | 1.00 |
| 1 | 5.20 | 1.17 | 1.16 | 1.02 | 1.18 | 1.00 | 5.28 | 1.23 | 1.30 | 1.03 | 1.19 | 1.00 |
| 1.5 | 5.37 | 1.27 | 1.16 | 1.00 | 1.26 | 1.03 | 5.54 | 1.32 | 1.31 | 1.00 | 1.26 | 1.02 |
| 2 | 4.96 | 1.40 | 1.22 | 1.00 | 1.37 | 1.08 | 5.23 | 1.47 | 1.41 | 1.00 | 1.32 | 1.06 |
| 2.5 | 4.37 | 1.53 | 1.29 | 1.00 | 1.48 | 1.11 | 4.66 | 1.61 | 1.53 | 1.00 | 1.40 | 1.10 |
| 3 | 3.82 | 1.65 | 1.36 | 1.00 | 1.59 | 1.13 | 4.08 | 1.75 | 1.65 | 1.00 | 1.52 | 1.13 |
| p | ||||||||||||
| Chart | χ2 | MCUSUMC | MC1 | MEWMALas | MEWMALtv | MHWMAp | ||||||
| δ | - | = 0.50 | = 0.50 | λ = 0.10 | λ = 0.10 | λ = 0.10 | ||||||
| 0 | NA | NA | NA | NA | NA | NA | ||||||
| 0.5 | 4.28 | 1.14 | 1.16 | 1.17 | 1.27 | 1.00 | ||||||
| 1 | 5.41 | 1.25 | 1.08 | 1.08 | 1.24 | 1.00 | ||||||
| 1.5 | 6.03 | 1.40 | 1.06 | 1.01 | 1.25 | 1.00 | ||||||
| 2 | 6.03 | 1.61 | 1.14 | 1.00 | 1.35 | 1.04 | ||||||
| 2.5 | 5.58 | 1.82 | 1.25 | 1.00 | 1.46 | 1.07 | ||||||
| 3 | 4.98 | 2.00 | 1.35 | 1.00 | 1.57 | 1.10 | ||||||
| δ | 1 | 2 | 3 | 4 | 5 | 6 | |
|---|---|---|---|---|---|---|---|
| 2 | 0.5 | ||||||
| 1.0 | |||||||
| 1.5 | |||||||
| 2.0 | |||||||
| 2.5 | |||||||
| 3.0 | |||||||
| 3 | 0.5 | ||||||
| 1.0 | |||||||
| 1.5 | |||||||
| 2.0 | |||||||
| 2.5 | |||||||
| 3.0 | |||||||
| 4 | 0.5 | ||||||
| 1.0 | |||||||
| 1.5 | |||||||
| 2.0 | |||||||
| 2.5 | |||||||
| 3.0 | |||||||
| 5 | 0.5 | ||||||
| 1.0 | |||||||
| 1.5 | |||||||
| 2.0 | |||||||
| 2.5 | |||||||
| 3.0 | |||||||
| 10 | 0.5 | ||||||
| 1.0 | |||||||
| 1.5 | |||||||
| 2.0 | |||||||
| 2.5 | |||||||
| 3.0 |
| 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | ||
| 2 | 3 | 91.1 | 110.3 | 132.8 | 156.8 | 175.2 | 182.2 | 187.7 | 199.9 | 113.4 | 129.0 | 147.6 | 167.7 | 181.4 | 187.0 | 192.4 | 200.3 |
| 5 | 116.4 | 133.1 | 152.6 | 171.1 | 184.0 | 188.6 | 191.8 | 199.9 | 133.8 | 149.0 | 163.5 | 178.6 | 188.7 | 191.8 | 195.8 | 200.3 | |
| 10 | 144.7 | 158.1 | 171.6 | 183.8 | 191.0 | 194.1 | 196.8 | 199.9 | 157.6 | 169.2 | 180.3 | 189.3 | 195.1 | 196.9 | 198.6 | 200.3 | |
| 15 | 158.3 | 169.6 | 179.4 | 188.2 | 192.9 | 195.5 | 197.2 | 199.9 | 168.5 | 177.6 | 186.0 | 192.1 | 195.5 | 196.4 | 199.7 | 200.3 | |
| 3 | 3 | 65.5 | 86.8 | 113.2 | 144.4 | 166.6 | 176.8 | 185.2 | 200.4 | 79.5 | 99.5 | 123.4 | 150.9 | 171.7 | 179.2 | 186.7 | 199.2 |
| 5 | 94.6 | 114.6 | 138.2 | 162.9 | 179.8 | 187.1 | 192.1 | 200.4 | 107.8 | 126.4 | 146.8 | 169.0 | 182.3 | 187.7 | 192.3 | 199.2 | |
| 10 | 128.9 | 146.2 | 163.3 | 179.9 | 189.9 | 193.8 | 197.8 | 200.4 | 139.5 | 154.9 | 169.5 | 181.8 | 190.4 | 193.1 | 195.2 | 199.2 | |
| 15 | 146.4 | 160.4 | 173.8 | 186.9 | 193.6 | 196.5 | 199.0 | 200.4 | 156.2 | 166.2 | 178.1 | 187.5 | 193.3 | 194.6 | 196.1 | 199.2 | |
| 4 | 3 | 48.2 | 69.4 | 96.5 | 131.2 | 158.4 | 170.3 | 181.2 | 199.5 | 60.2 | 80.4 | 107.3 | 139.0 | 164.2 | 175.3 | 183.7 | 199.8 |
| 5 | 77.8 | 100.2 | 125.0 | 153.2 | 172.4 | 181.7 | 187.3 | 199.5 | 90.4 | 110.5 | 134.6 | 160.8 | 178.1 | 184.6 | 191.0 | 199.8 | |
| 10 | 116.0 | 134.4 | 154.2 | 174.2 | 186.1 | 190.8 | 194.4 | 199.5 | 127.3 | 143.9 | 162.0 | 177.7 | 189.0 | 191.6 | 195.4 | 199.8 | |
| 15 | 134.7 | 150.5 | 167.0 | 181.2 | 191.0 | 192.4 | 195.6 | 199.5 | 144.2 | 159.2 | 172.8 | 185.4 | 193.0 | 194.9 | 196.9 | 199.8 | |
| 5 | 3 | 36.3 | 57.0 | 85.2 | 121.7 | 151.5 | 164.1 | 176.3 | 200.7 | 47.2 | 66.4 | 93.0 | 128.9 | 156.6 | 169.0 | 179.6 | 200.1 |
| 5 | 65.8 | 88.5 | 115.3 | 145.8 | 168.6 | 178.4 | 186.4 | 200.7 | 77.1 | 97.7 | 123.8 | 153.6 | 173.8 | 181.7 | 188.9 | 200.1 | |
| 10 | 106.2 | 125.6 | 147.8 | 168.8 | 183.7 | 189.2 | 191.7 | 200.7 | 115.8 | 134.0 | 155.2 | 174.8 | 186.0 | 191.7 | 194.6 | 200.1 | |
| 15 | 126.4 | 143.4 | 162.1 | 178.2 | 187.8 | 192.0 | 194.9 | 200.7 | 135.7 | 151.7 | 168.3 | 182.4 | 190.8 | 193.4 | 195.5 | 200.1 | |
| 10 | 3 | 8.9 | 22.3 | 47.6 | 88.0 | 125.6 | 144.3 | 162.6 | 200.7 | 16.9 | 30.4 | 52.6 | 90.8 | 128.2 | 146.8 | 164.9 | 200.5 |
| 5 | 30.4 | 52.4 | 81.3 | 120.0 | 151.4 | 164.4 | 177.3 | 200.7 | 39.6 | 58.9 | 86.3 | 124.5 | 155.3 | 168.3 | 180.0 | 200.5 | |
| 10 | 71.4 | 95.2 | 122.1 | 153.0 | 172.5 | 180.8 | 189.0 | 200.7 | 77.8 | 100.9 | 127.1 | 157.4 | 176.4 | 183.3 | 190.5 | 200.5 | |
| 15 | 95.6 | 117.6 | 141.5 | 165.9 | 180.7 | 187.5 | 192.6 | 200.7 | 102.2 | 123.3 | 147.0 | 170.2 | 183.8 | 190.2 | 193.4 | 200.5 | |
| 2 | 3 | 128.5 | 143.3 | 160.4 | 177.0 | 187.3 | 191.7 | 194.3 | 200.7 | 140.3 | 155.6 | 167.9 | 181.8 | 190.8 | 194.3 | 196.4 | 200.6 |
| 5 | 146.6 | 159.8 | 173.5 | 184.2 | 193.1 | 195.9 | 198.0 | 200.7 | 156.8 | 169.2 | 180.1 | 189.0 | 195.6 | 196.9 | 199.4 | 200.6 | |
| 10 | 167.2 | 176.1 | 184.6 | 192.7 | 196.2 | 198.1 | 199.4 | 200.7 | 174.8 | 182.0 | 190.1 | 194.7 | 198.3 | 197.5 | 199.1 | 200.6 | |
| 15 | 177.2 | 184.1 | 190.3 | 195.9 | 198.4 | 198.9 | 200.2 | 200.7 | 183.1 | 187.7 | 192.3 | 196.9 | 199.0 | 199.2 | 199.8 | 200.6 | |
| 3 | 3 | 87.1 | 108.2 | 131.7 | 158.9 | 175.9 | 183.6 | 189.7 | 199.9 | 95.1 | 115.6 | 140.8 | 165.0 | 181.5 | 187.3 | 193.0 | 200.4 |
| 5 | 116.2 | 135.3 | 155.6 | 173.6 | 186.9 | 191.2 | 194.7 | 199.9 | 126.7 | 144.2 | 162.9 | 179.8 | 188.9 | 193.1 | 194.9 | 200.4 | |
| 10 | 149.0 | 162.2 | 175.0 | 186.9 | 192.8 | 195.4 | 197.9 | 199.9 | 157.2 | 169.0 | 180.8 | 191.1 | 195.4 | 197.4 | 198.3 | 200.4 | |
| 15 | 162.7 | 172.9 | 183.0 | 190.6 | 195.4 | 196.2 | 198.1 | 199.9 | 170.1 | 179.8 | 186.9 | 193.3 | 197.8 | 198.2 | 200.5 | 200.4 | |
| 4 | 3 | 64.3 | 85.9 | 112.9 | 146.4 | 170.0 | 179.2 | 187.3 | 200.8 | 67.8 | 90.8 | 119.0 | 150.6 | 172.6 | 181.2 | 187.5 | 199.8 |
| 5 | 97.6 | 118.4 | 142.5 | 167.5 | 183.3 | 188.1 | 192.5 | 200.8 | 103.6 | 124.5 | 148.0 | 171.3 | 184.2 | 190.4 | 195.1 | 199.8 | |
| 10 | 135.4 | 152.0 | 168.9 | 183.3 | 191.9 | 194.9 | 196.9 | 200.8 | 142.1 | 158.8 | 172.9 | 184.7 | 191.9 | 195.5 | 196.3 | 199.8 | |
| 15 | 152.1 | 165.5 | 179.4 | 189.9 | 195.9 | 197.1 | 198.6 | 200.8 | 158.6 | 169.9 | 182.2 | 190.7 | 194.1 | 196.7 | 197.4 | 199.8 | |
| 5 | 3 | 48.3 | 68.9 | 98.1 | 134.0 | 160.6 | 173.2 | 182.4 | 199.8 | 50.7 | 72.2 | 102.4 | 139.6 | 164.8 | 175.5 | 184.7 | 200.6 |
| 5 | 81.1 | 104.0 | 130.2 | 158.4 | 177.8 | 185.2 | 191.0 | 199.8 | 86.5 | 110.1 | 136.0 | 163.3 | 179.4 | 186.4 | 190.7 | 200.6 | |
| 10 | 122.4 | 142.0 | 161.4 | 178.3 | 189.0 | 193.2 | 195.1 | 199.8 | 129.4 | 148.1 | 164.3 | 180.5 | 190.5 | 193.7 | 195.8 | 200.6 | |
| 15 | 142.2 | 157.3 | 172.2 | 184.6 | 191.7 | 194.8 | 196.1 | 199.8 | 148.5 | 162.9 | 176.6 | 187.3 | 193.6 | 194.8 | 197.0 | 200.6 | |
| 10 | 3 | 16.0 | 28.4 | 51.9 | 91.8 | 131.8 | 150.4 | 167.6 | 200.2 | 15.1 | 27.9 | 52.0 | 94.2 | 133.6 | 152.2 | 168.6 | 199.9 |
| 5 | 38.7 | 59.1 | 88.9 | 128.8 | 159.1 | 171.0 | 182.0 | 200.2 | 39.1 | 60.4 | 91.8 | 131.8 | 162.2 | 172.9 | 182.5 | 199.9 | |
| 10 | 80.4 | 104.8 | 132.5 | 161.2 | 179.1 | 186.0 | 191.8 | 200.2 | 84.0 | 109.4 | 137.0 | 164.8 | 180.7 | 185.9 | 191.1 | 199.9 | |
| 15 | 106.9 | 128.6 | 151.9 | 173.5 | 186.7 | 191.5 | 194.1 | 200.2 | 110.1 | 132.3 | 154.6 | 175.0 | 186.9 | 191.5 | 193.9 | 199.9 | |
| 2 | 3 | 151.2 | 162.6 | 173.4 | 185.2 | 191.6 | 194.1 | 196.1 | 200.4 | 158.0 | 169.4 | 178.8 | 187.5 | 192.7 | 196.1 | 197.1 | 200.1 |
| 5 | 164.6 | 175.0 | 182.5 | 190.7 | 195.1 | 196.7 | 198.1 | 200.4 | 171.6 | 179.5 | 186.7 | 192.6 | 198.1 | 197.4 | 198.1 | 200.1 | |
| 10 | 180.1 | 185.8 | 192.1 | 194.7 | 198.1 | 200.2 | 197.7 | 200.4 | 184.3 | 189.3 | 192.6 | 196.2 | 199.3 | 198.9 | 199.3 | 200.1 | |
| 15 | 185.6 | 190.5 | 193.5 | 196.8 | 197.6 | 199.4 | 199.3 | 200.4 | 188.8 | 194.3 | 195.9 | 198.0 | 198.0 | 199.2 | 200.2 | 200.1 | |
| 3 | 3 | 101.7 | 121.3 | 144.8 | 168.8 | 183.1 | 188.4 | 192.5 | 200.2 | 106.4 | 127.0 | 148.6 | 170.9 | 185.1 | 189.8 | 193.3 | 199.9 |
| 5 | 132.7 | 148.7 | 166.2 | 182.3 | 190.2 | 192.4 | 195.7 | 200.2 | 137.2 | 154.3 | 169.1 | 184.3 | 191.7 | 195.5 | 196.6 | 199.9 | |
| 10 | 162.1 | 172.7 | 183.7 | 191.5 | 194.8 | 197.0 | 198.9 | 200.2 | 166.2 | 175.8 | 184.6 | 193.6 | 197.1 | 197.4 | 198.9 | 199.9 | |
| 15 | 172.2 | 180.3 | 187.2 | 194.8 | 196.6 | 196.5 | 199.1 | 200.2 | 176.0 | 184.4 | 190.8 | 195.1 | 197.4 | 199.0 | 198.8 | 199.9 | |
| 4 | 3 | 72.3 | 95.3 | 123.8 | 155.2 | 174.5 | 184.2 | 189.2 | 200.1 | 75.0 | 99.2 | 127.9 | 156.4 | 177.4 | 184.3 | 189.5 | 199.5 |
| 5 | 108.6 | 130.9 | 153.1 | 174.3 | 185.3 | 191.6 | 194.5 | 200.1 | 113.2 | 134.2 | 155.2 | 175.6 | 186.1 | 191.0 | 195.2 | 199.5 | |
| 10 | 146.5 | 161.1 | 176.6 | 186.6 | 193.0 | 194.6 | 198.0 | 200.1 | 150.7 | 164.5 | 177.2 | 189.0 | 194.2 | 196.1 | 197.2 | 199.5 | |
| 15 | 162.7 | 173.3 | 182.6 | 191.1 | 194.3 | 197.3 | 197.3 | 200.1 | 164.9 | 175.6 | 184.5 | 190.8 | 195.8 | 197.5 | 197.1 | 199.5 | |
| 5 | 3 | 52.6 | 75.7 | 106.1 | 141.5 | 167.5 | 176.9 | 186.0 | 199.2 | 55.2 | 78.5 | 109.0 | 144.4 | 168.4 | 177.8 | 185.9 | 199.1 |
| 5 | 90.3 | 114.4 | 140.3 | 166.0 | 182.1 | 186.9 | 191.9 | 199.2 | 93.8 | 117.3 | 143.0 | 167.5 | 182.2 | 188.3 | 191.5 | 199.1 | |
| 10 | 133.3 | 151.2 | 167.6 | 182.4 | 190.2 | 193.3 | 196.0 | 199.2 | 136.1 | 154.9 | 169.5 | 183.3 | 192.0 | 193.1 | 196.3 | 199.1 | |
| 15 | 153.1 | 165.5 | 177.3 | 188.1 | 192.2 | 195.0 | 196.2 | 199.2 | 154.5 | 168.3 | 179.2 | 189.0 | 192.7 | 195.4 | 197.5 | 199.1 | |
| 10 | 3 | 14.6 | 27.9 | 52.9 | 96.2 | 135.6 | 154.1 | 171.1 | 199.7 | 14.6 | 28.0 | 54.3 | 98.3 | 138.0 | 155.2 | 171.5 | 199.3 |
| 5 | 40.1 | 62.2 | 95.2 | 134.7 | 163.6 | 174.2 | 183.3 | 199.7 | 40.8 | 63.8 | 96.7 | 136.8 | 164.0 | 175.8 | 183.4 | 199.3 | |
| 10 | 87.3 | 111.8 | 139.9 | 165.9 | 181.9 | 186.7 | 192.2 | 199.7 | 88.6 | 114.4 | 141.0 | 167.6 | 182.3 | 189.2 | 192.8 | 199.3 | |
| 15 | 113.9 | 135.8 | 158.2 | 176.0 | 187.4 | 191.3 | 194.5 | 199.7 | 116.2 | 138.3 | 158.8 | 179.0 | 189.2 | 190.7 | 195.2 | 199.3 | |
| p | n m | ||||||||||||||||
| 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | ||
| 2 | 3 | 115.3 | 122.9 | 131.5 | 139.6 | 148.2 | 151.7 | 155.4 | 161.0 | 164.9 | 161.9 | 163.3 | 167.9 | 173.8 | 174.6 | 177.9 | 181.2 |
| 5 | 123.8 | 129.1 | 138.2 | 145.8 | 152.2 | 154.3 | 156.3 | 161.0 | 155.9 | 161.4 | 166.6 | 170.2 | 174.8 | 177.0 | 178.4 | 181.2 | |
| 10 | 133.5 | 138.9 | 145.2 | 151.6 | 155.9 | 157.6 | 159.1 | 161.0 | 159.4 | 166.0 | 171.1 | 175.5 | 178.2 | 179.7 | 180.5 | 181.2 | |
| 15 | 138.7 | 145.3 | 149.4 | 154.7 | 156.7 | 157.9 | 159.4 | 161.0 | 164.5 | 169.5 | 172.2 | 177.0 | 178.0 | 178.9 | 181.1 | 181.2 | |
| 3 | 3 | 83.3 | 96.6 | 111.7 | 128.2 | 141.5 | 148.3 | 153.4 | 163.1 | 101.7 | 117.1 | 130.7 | 149.0 | 161.3 | 167.4 | 173.0 | 181.4 |
| 5 | 100.7 | 111.8 | 124.9 | 139.2 | 150.1 | 154.4 | 158.0 | 163.1 | 120.7 | 131.2 | 144.7 | 160.7 | 169.2 | 172.9 | 176.6 | 181.4 | |
| 10 | 119.4 | 129.0 | 139.2 | 149.0 | 155.9 | 159.2 | 161.6 | 163.1 | 138.8 | 150.3 | 160.1 | 169.0 | 174.7 | 176.5 | 177.8 | 181.4 | |
| 15 | 128.9 | 137.6 | 145.6 | 154.2 | 158.5 | 160.9 | 162.6 | 163.1 | 150.7 | 157.0 | 165.6 | 172.6 | 176.2 | 177.0 | 178.2 | 181.4 | |
| 4 | 3 | 64.1 | 78.0 | 94.9 | 116.7 | 135.2 | 143.0 | 150.3 | 163.9 | 71.0 | 88.5 | 111.1 | 134.8 | 153.8 | 163.1 | 169.7 | 183.5 |
| 5 | 84.8 | 97.8 | 112.8 | 130.9 | 143.9 | 151.1 | 154.1 | 163.9 | 95.6 | 111.3 | 130.5 | 152.0 | 165.6 | 170.8 | 175.3 | 183.5 | |
| 10 | 107.3 | 118.8 | 131.0 | 144.9 | 153.9 | 158.0 | 160.2 | 163.9 | 125.5 | 138.1 | 152.0 | 164.1 | 173.7 | 175.3 | 178.8 | 183.5 | |
| 15 | 118.8 | 129.1 | 140.3 | 150.1 | 157.7 | 159.1 | 160.8 | 163.9 | 137.8 | 149.5 | 159.8 | 169.9 | 176.5 | 177.7 | 180.1 | 183.5 | |
| 5 | 3 | 51.5 | 67.2 | 84.8 | 108.5 | 129.5 | 137.1 | 147.1 | 165.5 | 51.9 | 68.8 | 92.6 | 123.3 | 146.3 | 157.1 | 165.9 | 184.3 |
| 5 | 72.5 | 87.1 | 103.9 | 124.4 | 140.9 | 148.2 | 155.2 | 165.5 | 78.9 | 96.8 | 118.2 | 143.3 | 159.8 | 167.6 | 173.6 | 184.3 | |
| 10 | 98.5 | 110.9 | 126.2 | 141.0 | 152.2 | 156.5 | 158.5 | 165.5 | 111.8 | 126.0 | 145.3 | 161.1 | 171.3 | 176.3 | 179.0 | 184.3 | |
| 15 | 111.6 | 123.5 | 135.9 | 148.3 | 156.1 | 158.6 | 161.4 | 165.5 | 128.6 | 140.8 | 156.1 | 168.3 | 174.9 | 177.9 | 178.3 | 184.3 | |
| 10 | 3 | 19.1 | 33.4 | 52.6 | 80.0 | 106.2 | 121.1 | 136.2 | 168.3 | 18.3 | 29.6 | 48.6 | 83.8 | 117.9 | 134.8 | 152.3 | 184.8 |
| 5 | 40.9 | 56.3 | 75.0 | 102.5 | 126.9 | 137.7 | 147.4 | 168.3 | 37.6 | 53.8 | 79.2 | 114.0 | 142.5 | 154.5 | 164.8 | 184.8 | |
| 10 | 68.9 | 84.7 | 103.8 | 127.5 | 144.0 | 151.1 | 157.7 | 168.3 | 71.2 | 92.6 | 116.3 | 145.0 | 162.7 | 168.5 | 174.7 | 184.8 | |
| 15 | 85.1 | 101.1 | 119.1 | 138.5 | 151.0 | 155.2 | 161.0 | 168.3 | 93.6 | 111.9 | 134.8 | 156.7 | 168.8 | 175.5 | 178.8 | 184.8 | |
| 2 | 3 | 223.0 | 202.2 | 198.1 | 193.4 | 190.0 | 191.8 | 191.6 | 192.7 | 245.9 | 233.5 | 213.0 | 204.2 | 201.1 | 199.1 | 197.8 | 196.6 |
| 5 | 191.3 | 189.7 | 188.6 | 189.5 | 190.3 | 191.2 | 192.8 | 192.7 | 211.1 | 206.7 | 201.5 | 198.1 | 197.3 | 198.0 | 198.5 | 196.6 | |
| 10 | 183.5 | 185.3 | 187.2 | 191.2 | 191.6 | 191.2 | 193.2 | 192.7 | 197.9 | 195.9 | 197.3 | 197.4 | 197.5 | 195.4 | 197.2 | 196.6 | |
| 15 | 186.0 | 187.2 | 188.8 | 190.5 | 192.1 | 192.5 | 193.4 | 192.7 | 197.0 | 195.2 | 195.7 | 197.0 | 197.0 | 197.1 | 197.5 | 196.6 | |
| 3 | 3 | 128.8 | 141.7 | 154.1 | 169.0 | 178.3 | 182.3 | 186.1 | 193.3 | 147.9 | 157.2 | 170.9 | 179.8 | 186.8 | 191.0 | 194.3 | 196.2 |
| 5 | 140.2 | 153.1 | 166.2 | 174.0 | 184.7 | 187.7 | 189.8 | 193.3 | 161.7 | 167.3 | 178.5 | 187.2 | 190.4 | 193.2 | 192.6 | 196.2 | |
| 10 | 160.2 | 168.2 | 175.7 | 182.8 | 189.1 | 190.2 | 190.3 | 193.3 | 175.7 | 179.9 | 186.8 | 193.3 | 194.5 | 195.2 | 196.1 | 196.2 | |
| 15 | 168.4 | 175.3 | 181.5 | 186.3 | 189.3 | 189.3 | 189.9 | 193.3 | 180.3 | 186.1 | 189.6 | 192.9 | 196.6 | 196.0 | 197.5 | 196.2 | |
| 4 | 3 | 84.8 | 104.7 | 126.8 | 153.4 | 171.4 | 177.0 | 183.7 | 194.5 | 97.8 | 116.8 | 141.3 | 161.7 | 177.5 | 183.0 | 188.5 | 196.6 |
| 5 | 113.7 | 129.6 | 149.2 | 168.3 | 180.8 | 184.3 | 187.9 | 194.5 | 125.1 | 143.0 | 159.2 | 176.8 | 185.5 | 191.1 | 193.9 | 196.6 | |
| 10 | 143.0 | 155.8 | 168.6 | 179.8 | 185.2 | 189.0 | 191.7 | 194.5 | 154.8 | 169.0 | 178.1 | 186.1 | 190.0 | 195.1 | 194.1 | 196.6 | |
| 15 | 154.6 | 166.7 | 177.7 | 184.9 | 190.1 | 191.3 | 192.7 | 194.5 | 166.1 | 175.0 | 184.2 | 190.1 | 191.2 | 195.3 | 194.6 | 196.6 | |
| 5 | 3 | 59.0 | 79.6 | 106.8 | 137.9 | 160.6 | 171.0 | 179.0 | 192.4 | 68.0 | 88.4 | 118.2 | 148.7 | 170.3 | 177.9 | 184.1 | 197.5 |
| 5 | 91.1 | 111.5 | 134.3 | 158.3 | 175.2 | 180.6 | 186.8 | 192.4 | 102.5 | 123.6 | 145.2 | 167.3 | 179.7 | 186.4 | 189.6 | 197.5 | |
| 10 | 127.9 | 145.2 | 161.0 | 175.6 | 184.7 | 187.9 | 188.9 | 192.4 | 139.6 | 155.0 | 167.2 | 182.5 | 188.4 | 191.9 | 193.3 | 197.5 | |
| 15 | 144.2 | 156.4 | 169.8 | 179.3 | 186.3 | 188.4 | 190.2 | 192.4 | 155.0 | 167.2 | 178.7 | 186.5 | 191.2 | 191.8 | 195.1 | 197.5 | |
| 10 | 3 | 16.4 | 28.5 | 51.9 | 91.0 | 129.9 | 146.6 | 164.2 | 194.4 | 16.1 | 29.5 | 54.7 | 97.6 | 134.9 | 154.3 | 169.2 | 197.8 |
| 5 | 38.9 | 59.2 | 88.1 | 127.0 | 155.0 | 166.6 | 176.1 | 194.4 | 41.5 | 63.7 | 94.7 | 133.7 | 162.6 | 172.0 | 181.6 | 197.8 | |
| 10 | 79.5 | 103.5 | 129.9 | 156.9 | 174.0 | 180.4 | 186.1 | 194.4 | 87.6 | 111.8 | 139.3 | 163.9 | 179.4 | 184.1 | 188.5 | 197.8 | |
| 15 | 105.7 | 126.4 | 148.3 | 168.1 | 180.7 | 186.4 | 189.0 | 194.4 | 112.7 | 134.2 | 154.0 | 175.0 | 185.9 | 189.1 | 191.7 | 197.8 | |
| 2 | 3 | 273.9 | 248.4 | 222.2 | 209.2 | 203.4 | 201.3 | 199.1 | 197.6 | 285.3 | 256.6 | 229.7 | 213.2 | 205.8 | 205.8 | 201.7 | 198.5 |
| 5 | 224.5 | 217.0 | 206.5 | 201.5 | 198.7 | 200.1 | 198.4 | 197.6 | 238.1 | 225.9 | 212.8 | 205.9 | 203.3 | 201.7 | 199.1 | 198.5 | |
| 10 | 204.4 | 203.8 | 201.1 | 198.2 | 199.1 | 200.2 | 197.8 | 197.6 | 213.9 | 208.4 | 205.0 | 201.1 | 201.2 | 200.3 | 199.5 | 198.5 | |
| 15 | 203.7 | 200.9 | 199.6 | 199.4 | 197.8 | 198.2 | 199.0 | 197.6 | 207.3 | 205.9 | 202.9 | 200.6 | 199.1 | 199.3 | 199.5 | 198.5 | |
| 3 | 3 | 166.2 | 170.9 | 178.4 | 186.3 | 191.6 | 194.3 | 194.9 | 199.0 | 178.0 | 182.0 | 183.8 | 191.3 | 195.7 | 195.9 | 195.6 | 199.3 |
| 5 | 171.7 | 177.2 | 183.9 | 191.1 | 194.2 | 194.0 | 196.4 | 199.0 | 179.1 | 184.5 | 189.7 | 194.9 | 196.5 | 197.5 | 198.3 | 199.3 | |
| 10 | 182.7 | 186.4 | 192.6 | 194.7 | 195.8 | 197.1 | 198.6 | 199.0 | 187.5 | 191.6 | 193.1 | 199.2 | 199.7 | 198.0 | 197.8 | 199.3 | |
| 15 | 185.7 | 189.5 | 192.0 | 197.6 | 196.6 | 195.7 | 197.0 | 199.0 | 189.7 | 194.5 | 197.0 | 197.4 | 199.0 | 198.9 | 199.5 | 199.3 | |
| 4 | 3 | 108.1 | 125.5 | 150.1 | 170.4 | 181.9 | 189.7 | 191.9 | 198.9 | 112.2 | 134.8 | 155.0 | 172.0 | 186.3 | 190.9 | 192.8 | 199.0 |
| 5 | 136.2 | 152.6 | 168.1 | 183.1 | 188.7 | 193.3 | 196.1 | 198.9 | 143.1 | 157.1 | 171.6 | 183.5 | 190.4 | 193.7 | 196.7 | 199.0 | |
| 10 | 162.3 | 172.1 | 184.5 | 190.0 | 194.4 | 193.6 | 197.3 | 198.9 | 169.5 | 177.0 | 184.0 | 192.5 | 196.0 | 197.8 | 198.2 | 199.0 | |
| 15 | 173.7 | 179.7 | 185.8 | 192.7 | 195.2 | 197.3 | 196.9 | 198.9 | 175.2 | 183.4 | 190.7 | 193.4 | 196.2 | 197.8 | 196.4 | 199.0 | |
| 5 | 3 | 74.9 | 96.8 | 125.5 | 152.0 | 174.6 | 181.9 | 188.3 | 197.5 | 80.0 | 102.3 | 128.3 | 158.5 | 175.7 | 182.2 | 188.2 | 198.3 |
| 5 | 111.0 | 131.0 | 152.6 | 171.3 | 185.1 | 187.9 | 192.4 | 197.5 | 115.6 | 135.5 | 155.6 | 175.2 | 185.4 | 189.2 | 191.5 | 198.3 | |
| 10 | 146.2 | 160.6 | 173.4 | 185.4 | 190.9 | 193.4 | 196.4 | 197.5 | 149.4 | 165.0 | 176.1 | 186.0 | 193.5 | 193.0 | 197.0 | 198.3 | |
| 15 | 162.8 | 171.0 | 179.9 | 189.3 | 192.2 | 194.3 | 194.5 | 197.5 | 163.7 | 174.9 | 182.8 | 190.2 | 193.4 | 195.1 | 197.5 | 198.3 | |
| 10 | 3 | 16.3 | 31.3 | 58.4 | 101.3 | 137.9 | 155.7 | 172.1 | 199.3 | 16.8 | 31.8 | 60.6 | 103.9 | 141.9 | 158.7 | 173.9 | 199.3 |
| 5 | 44.3 | 66.9 | 101.2 | 138.9 | 165.2 | 176.1 | 183.7 | 199.3 | 45.8 | 70.0 | 102.7 | 140.7 | 166.3 | 177.2 | 184.9 | 199.3 | |
| 10 | 92.6 | 115.7 | 143.0 | 166.4 | 182.8 | 186.8 | 191.0 | 199.3 | 94.4 | 120.1 | 145.3 | 169.4 | 183.9 | 189.5 | 191.7 | 199.3 | |
| 15 | 118.0 | 138.8 | 159.1 | 176.8 | 187.2 | 190.9 | 192.8 | 199.3 | 120.9 | 142.2 | 161.6 | 180.4 | 189.6 | 190.8 | 195.0 | 199.3 | |
| 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | 20 | 30 | 50 | 100 | 200 | 300 | 500 | ∞ | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 3 | 115.3 | 122.9 | 131.5 | 139.6 | 148.2 | 151.7 | 155.4 | 161.0 | 164.9 | 161.9 | 163.3 | 167.9 | 173.8 | 174.6 | 177.9 | 181.2 |
| 5 | 123.8 | 129.1 | 138.2 | 145.8 | 152.2 | 154.3 | 156.3 | 161.0 | 155.9 | 161.4 | 166.6 | 170.2 | 174.8 | 177.0 | 178.4 | 181.2 | |
| 10 | 133.5 | 138.9 | 145.2 | 151.6 | 155.9 | 157.6 | 159.1 | 161.0 | 159.4 | 166.0 | 171.1 | 175.5 | 178.2 | 179.7 | 180.5 | 181.2 | |
| 15 | 138.7 | 145.3 | 149.4 | 154.7 | 156.7 | 157.9 | 159.4 | 161.0 | 164.5 | 169.5 | 172.2 | 177.0 | 178.0 | 178.9 | 181.1 | 181.2 | |
| 3 | 3 | 83.3 | 96.6 | 111.7 | 128.2 | 141.5 | 148.3 | 153.4 | 163.1 | 101.7 | 117.1 | 130.7 | 149.0 | 161.3 | 167.4 | 173.0 | 181.4 |
| 5 | 100.7 | 111.8 | 124.9 | 139.2 | 150.1 | 154.4 | 158.0 | 163.1 | 120.7 | 131.2 | 144.7 | 160.7 | 169.2 | 172.9 | 176.6 | 181.4 | |
| 10 | 119.4 | 129.0 | 139.2 | 149.0 | 155.9 | 159.2 | 161.6 | 163.1 | 138.8 | 150.3 | 160.1 | 169.0 | 174.7 | 176.5 | 177.8 | 181.4 | |
| 15 | 128.9 | 137.6 | 145.6 | 154.2 | 158.5 | 160.9 | 162.6 | 163.1 | 150.7 | 157.0 | 165.6 | 172.6 | 176.2 | 177.0 | 178.2 | 181.4 | |
| 4 | 3 | 64.1 | 78.0 | 94.9 | 116.7 | 135.2 | 143.0 | 150.3 | 163.9 | 71.0 | 88.5 | 111.1 | 134.8 | 153.8 | 163.1 | 169.7 | 183.5 |
| 5 | 84.8 | 97.8 | 112.8 | 130.9 | 143.9 | 151.1 | 154.1 | 163.9 | 95.6 | 111.3 | 130.5 | 152.0 | 165.6 | 170.8 | 175.3 | 183.5 | |
| 10 | 107.3 | 118.8 | 131.0 | 144.9 | 153.9 | 158.0 | 160.2 | 163.9 | 125.5 | 138.1 | 152.0 | 164.1 | 173.7 | 175.3 | 178.8 | 183.5 | |
| 15 | 118.8 | 129.1 | 140.3 | 150.1 | 157.7 | 159.1 | 160.8 | 163.9 | 137.8 | 149.5 | 159.8 | 169.9 | 176.5 | 177.7 | 180.1 | 183.5 | |
| 5 | 3 | 51.5 | 67.2 | 84.8 | 108.5 | 129.5 | 137.1 | 147.1 | 165.5 | 51.9 | 68.8 | 92.6 | 123.3 | 146.3 | 157.1 | 165.9 | 184.3 |
| 5 | 72.5 | 87.1 | 103.9 | 124.4 | 140.9 | 148.2 | 155.2 | 165.5 | 78.9 | 96.8 | 118.2 | 143.3 | 159.8 | 167.6 | 173.6 | 184.3 | |
| 10 | 98.5 | 110.9 | 126.2 | 141.0 | 152.2 | 156.5 | 158.5 | 165.5 | 111.8 | 126.0 | 145.3 | 161.1 | 171.3 | 176.3 | 179.0 | 184.3 | |
| 15 | 111.6 | 123.5 | 135.9 | 148.3 | 156.1 | 158.6 | 161.4 | 165.5 | 128.6 | 140.8 | 156.1 | 168.3 | 174.9 | 177.9 | 178.3 | 184.3 | |
| 10 | 3 | 19.1 | 33.4 | 52.6 | 80.0 | 106.2 | 121.1 | 136.2 | 168.3 | 18.3 | 29.6 | 48.6 | 83.8 | 117.9 | 134.8 | 152.3 | 184.8 |
| 5 | 40.9 | 56.3 | 75.0 | 102.5 | 126.9 | 137.7 | 147.4 | 168.3 | 37.6 | 53.8 | 79.2 | 114.0 | 142.5 | 154.5 | 164.8 | 184.8 | |
| 10 | 68.9 | 84.7 | 103.8 | 127.5 | 144.0 | 151.1 | 157.7 | 168.3 | 71.2 | 92.6 | 116.3 | 145.0 | 162.7 | 168.5 | 174.7 | 184.8 | |
| 15 | 85.1 | 101.1 | 119.1 | 138.5 | 151.0 | 155.2 | 161.0 | 168.3 | 93.6 | 111.9 | 134.8 | 156.7 | 168.8 | 175.5 | 178.8 | 184.8 | |
| ∞ | 5000 | 1000 | 500 | 100 | 50 | 20 | 10 | 5 | 4 | 3 | 2 | 1 | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 0.1 | 199.89 | 199.43 | 197.51 | 193.35 | 176.68 | 158.15 | 117.46 | 79.07 | 42.39 | 32.55 | 22.41 | 12.6 | 4.92 |
| 0.2 | 200.3 | 199.63 | 196.87 | 192.41 | 165.48 | 140.12 | 93.04 | 57.25 | 29.59 | 23.32 | 16.62 | 10.22 | 4.63 | |
| 0.3 | 200.73 | 199.7 | 195.68 | 192.06 | 160.02 | 131.26 | 81.79 | 47.5 | 23.71 | 18.67 | 13.55 | 8.65 | 4.22 | |
| 0.4 | 200.64 | 199.99 | 194.8 | 190.95 | 157.54 | 127.04 | 76.56 | 42.22 | 20.78 | 16.27 | 11.92 | 7.7 | 3.95 | |
| 0.5 | 200.36 | 198.7 | 194.69 | 190.18 | 154.39 | 124.89 | 73.36 | 39.86 | 19.15 | 14.9 | 10.83 | 7.07 | 3.69 | |
| 0.6 | 200.13 | 198.57 | 195.15 | 190.69 | 154.52 | 122.98 | 71.67 | 38.52 | 18.24 | 14.08 | 10.29 | 6.7 | 3.56 | |
| 3 | 0.1 | 200.44 | 200.06 | 199.3 | 196.08 | 174.24 | 153.42 | 108.93 | 69.87 | 35.21 | 26.84 | 17.98 | 10.06 | 4.18 |
| 0.2 | 199.19 | 198.25 | 194.61 | 189.93 | 157.81 | 128.95 | 80.85 | 47.88 | 23.98 | 18.83 | 13.48 | 8.31 | 3.97 | |
| 0.3 | 199.9 | 198.92 | 193.96 | 188.38 | 151.06 | 118.84 | 69.15 | 38.39 | 18.93 | 14.88 | 10.93 | 7.09 | 3.67 | |
| 0.4 | 200.4 | 198.62 | 194.48 | 188.51 | 148.31 | 113.97 | 63.8 | 33.87 | 16.42 | 12.93 | 9.55 | 6.29 | 3.39 | |
| 0.5 | 200.23 | 198.15 | 193.47 | 186.7 | 146.04 | 112.35 | 60.97 | 31.64 | 14.93 | 11.74 | 8.66 | 5.81 | 3.21 | |
| 0.6 | 199.92 | 198.64 | 193 | 186.51 | 145.67 | 111.16 | 59.5 | 30.25 | 14.07 | 11 | 8.09 | 5.47 | 3.08 | |
| 4 | 0.1 | 199.49 | 198.86 | 197.87 | 194.06 | 168.85 | 146.21 | 100.81 | 62.12 | 30.44 | 22.92 | 15.38 | 8.63 | 3.76 |
| 0.2 | 199.83 | 199.78 | 194.85 | 188.48 | 152.47 | 121.23 | 72.95 | 41.7 | 20.78 | 16.22 | 11.58 | 7.26 | 3.62 | |
| 0.3 | 200.81 | 199.71 | 192.86 | 187.14 | 143.99 | 110.93 | 61.56 | 33.18 | 16.23 | 12.81 | 9.45 | 6.21 | 3.33 | |
| 0.4 | 199.84 | 198.59 | 193.88 | 186.42 | 140.98 | 105.57 | 55.55 | 28.88 | 13.95 | 11.02 | 8.21 | 5.53 | 3.11 | |
| 0.5 | 200.07 | 198.87 | 192.59 | 184.21 | 138.15 | 102.51 | 52.46 | 26.4 | 12.57 | 9.91 | 7.43 | 5.06 | 2.95 | |
| 0.6 | 199.49 | 198.25 | 192.16 | 183.7 | 137.65 | 100.5 | 50.87 | 25.05 | 11.78 | 9.25 | 6.92 | 4.79 | 2.82 | |
| 5 | 0.1 | 200.68 | 199.94 | 196.38 | 191.28 | 165.48 | 140.99 | 94.31 | 56.76 | 27.04 | 20.17 | 13.53 | 7.59 | 3.49 |
| 0.2 | 200.06 | 198.86 | 192.79 | 187.55 | 146.82 | 114.83 | 66.32 | 37.53 | 18.46 | 14.35 | 10.34 | 6.54 | 3.37 | |
| 0.3 | 199.8 | 197.01 | 190.67 | 184.02 | 138.07 | 102.94 | 55.22 | 29.06 | 14.41 | 11.38 | 8.42 | 5.66 | 3.12 | |
| 0.4 | 200.61 | 197.44 | 191.48 | 182.25 | 133.8 | 96.89 | 49.14 | 25.15 | 12.27 | 9.73 | 7.37 | 5.01 | 2.93 | |
| 0.5 | 199.16 | 196.97 | 190.67 | 181.13 | 130.2 | 93.9 | 46.29 | 22.84 | 11.01 | 8.72 | 6.63 | 4.6 | 2.76 | |
| 0.6 | 199.11 | 196.89 | 189.76 | 182.08 | 129.63 | 92.41 | 44.17 | 21.68 | 10.24 | 8.16 | 6.18 | 4.35 | 2.66 | |
| 10 | 0.1 | 200.68 | 199.95 | 195.09 | 189.11 | 153.14 | 123.52 | 75.04 | 41.61 | 18.26 | 13.53 | 9.08 | 5.48 | 2.91 |
| 0.2 | 200.51 | 197.82 | 190.02 | 181.02 | 127.12 | 92.08 | 48.82 | 26.06 | 12.67 | 9.99 | 7.37 | 4.86 | 2.79 | |
| 0.3 | 200.24 | 198.84 | 188.31 | 177.04 | 115.77 | 78.76 | 38 | 19.65 | 9.84 | 7.96 | 6.08 | 4.27 | 2.63 | |
| 0.4 | 199.87 | 196.52 | 184.71 | 171.38 | 105.8 | 66.8 | 28.1 | 13.56 | 6.84 | 5.61 | 4.48 | 3.36 | 2.26 | |
| 0.5 | 199.69 | 197.41 | 186.16 | 173.29 | 107.8 | 68.81 | 29.9 | 14.57 | 7.4 | 6.07 | 4.81 | 3.55 | 2.34 | |
| 0.6 | 199.32 | 196.56 | 186.13 | 172.82 | 105.69 | 66.64 | 28.08 | 13.43 | 6.87 | 5.6 | 4.46 | 3.34 | 2.26 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbas, N.; Riaz, M.; Ahmad, S.; Abid, M.; Zaman, B. On the Efficient Monitoring of Multivariate Processes with Unknown Parameters. Mathematics 2020, 8, 823. https://doi.org/10.3390/math8050823
Abbas N, Riaz M, Ahmad S, Abid M, Zaman B. On the Efficient Monitoring of Multivariate Processes with Unknown Parameters. Mathematics. 2020; 8(5):823. https://doi.org/10.3390/math8050823
Chicago/Turabian StyleAbbas, Nasir, Muhammad Riaz, Shabbir Ahmad, Muhammad Abid, and Babar Zaman. 2020. "On the Efficient Monitoring of Multivariate Processes with Unknown Parameters" Mathematics 8, no. 5: 823. https://doi.org/10.3390/math8050823
APA StyleAbbas, N., Riaz, M., Ahmad, S., Abid, M., & Zaman, B. (2020). On the Efficient Monitoring of Multivariate Processes with Unknown Parameters. Mathematics, 8(5), 823. https://doi.org/10.3390/math8050823





