Effective Method for Solving Different Types of Nonlinear Fractional Burgers’ Equations
Abstract
:1. Introduction
2. Preliminaries and Fractional Derivative Order
2.1. The Gamma Function
2.2. Fractional Derivative
- The fractional derivative of an analytical function is analytic.
- Backward compatibility: when the order is positive, the integer fractional derivative gives the same result as the ordinary derivative.
- Identity: the zero order derivative of a function returns the function itself.
- Linearity: the operator must be linear.
- The index law of fractional integrals holds, that is,
3. Fractional Reduced Differential Transform Method
4. Numerical Experiments
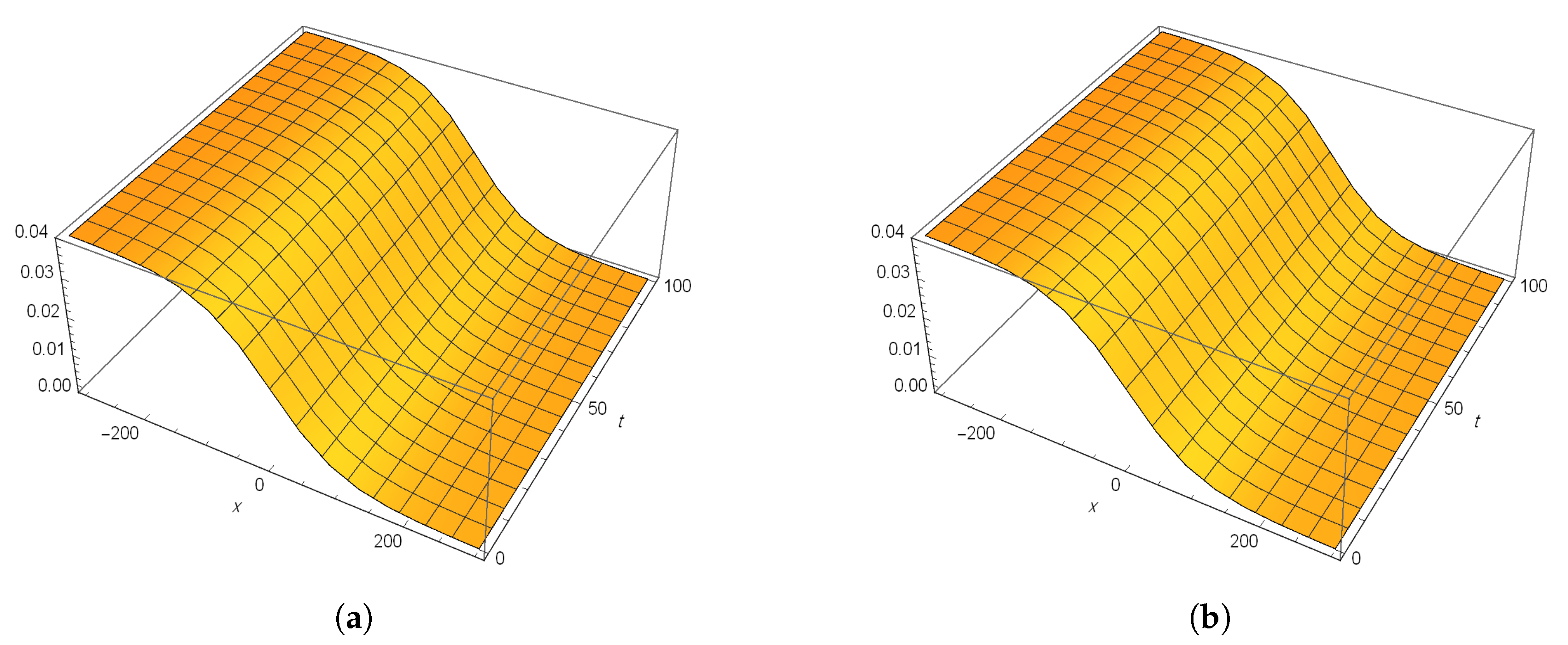
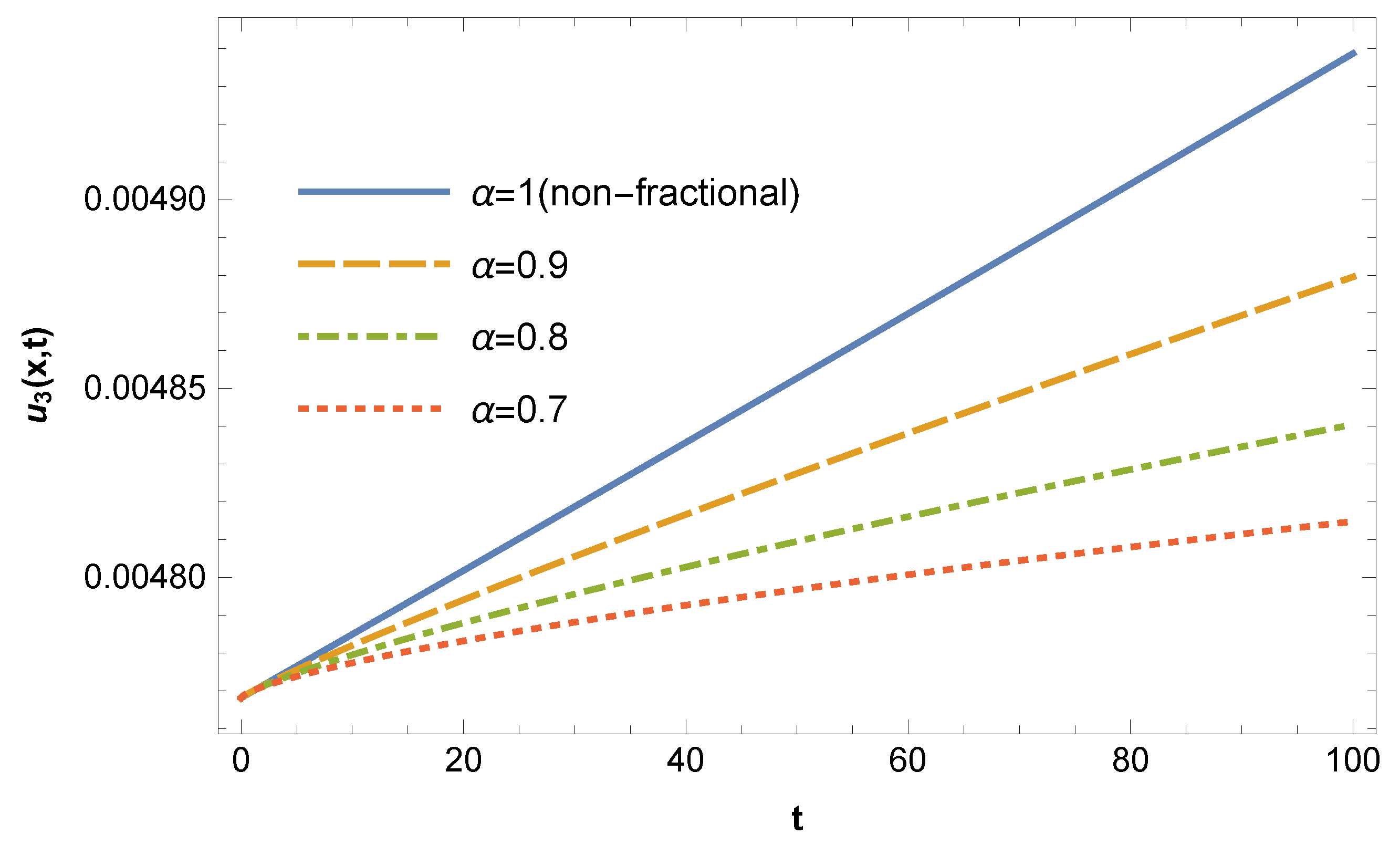
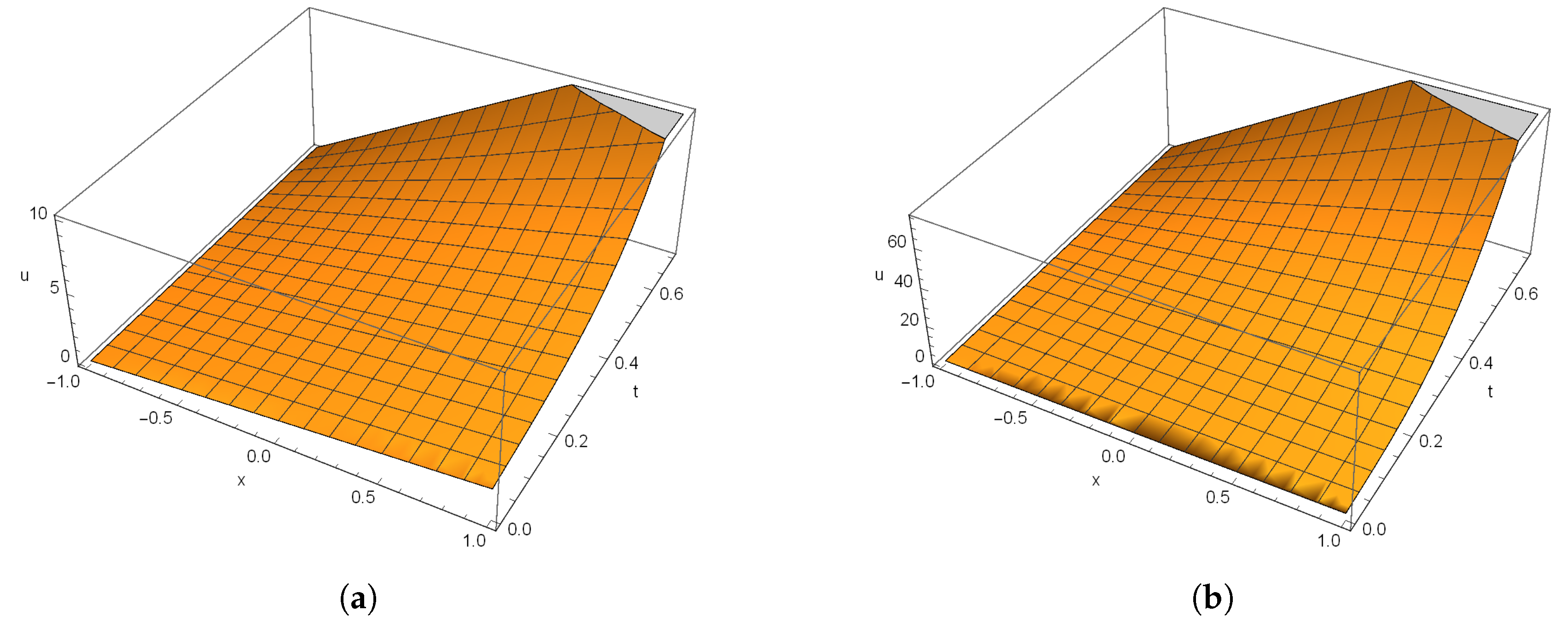
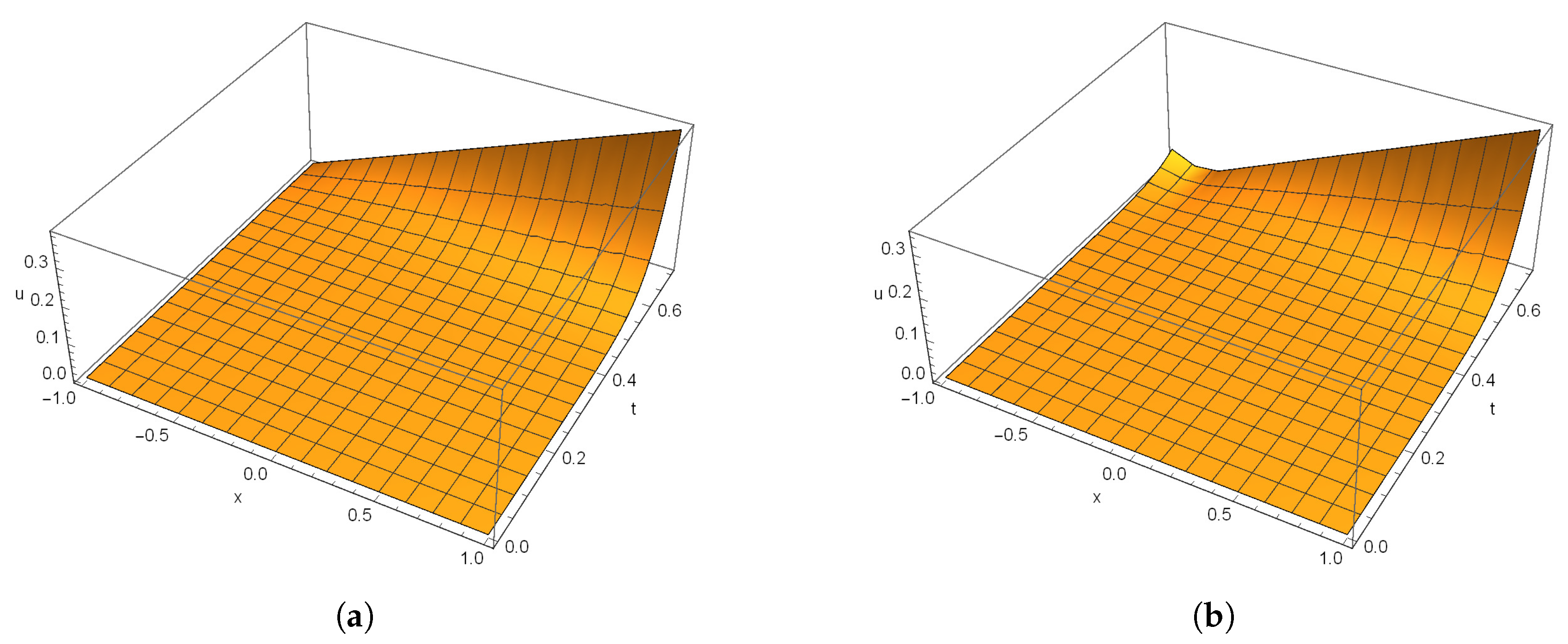
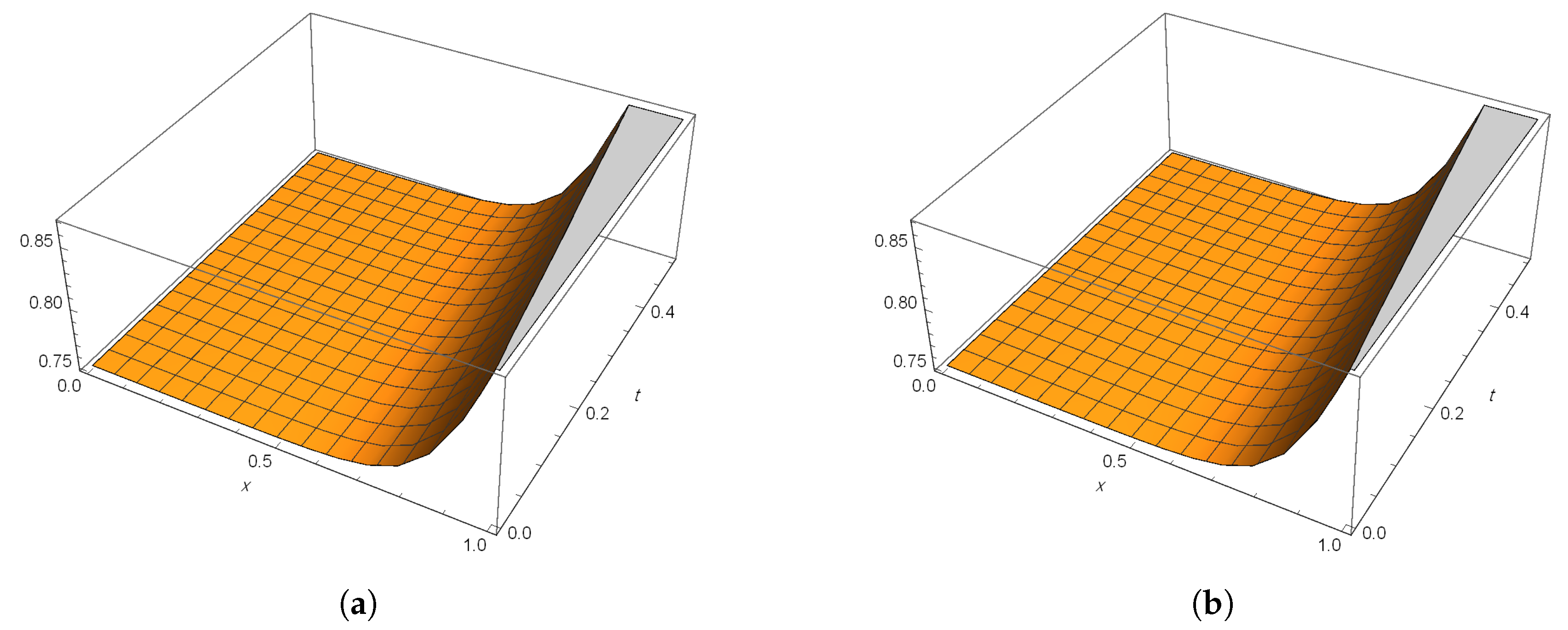
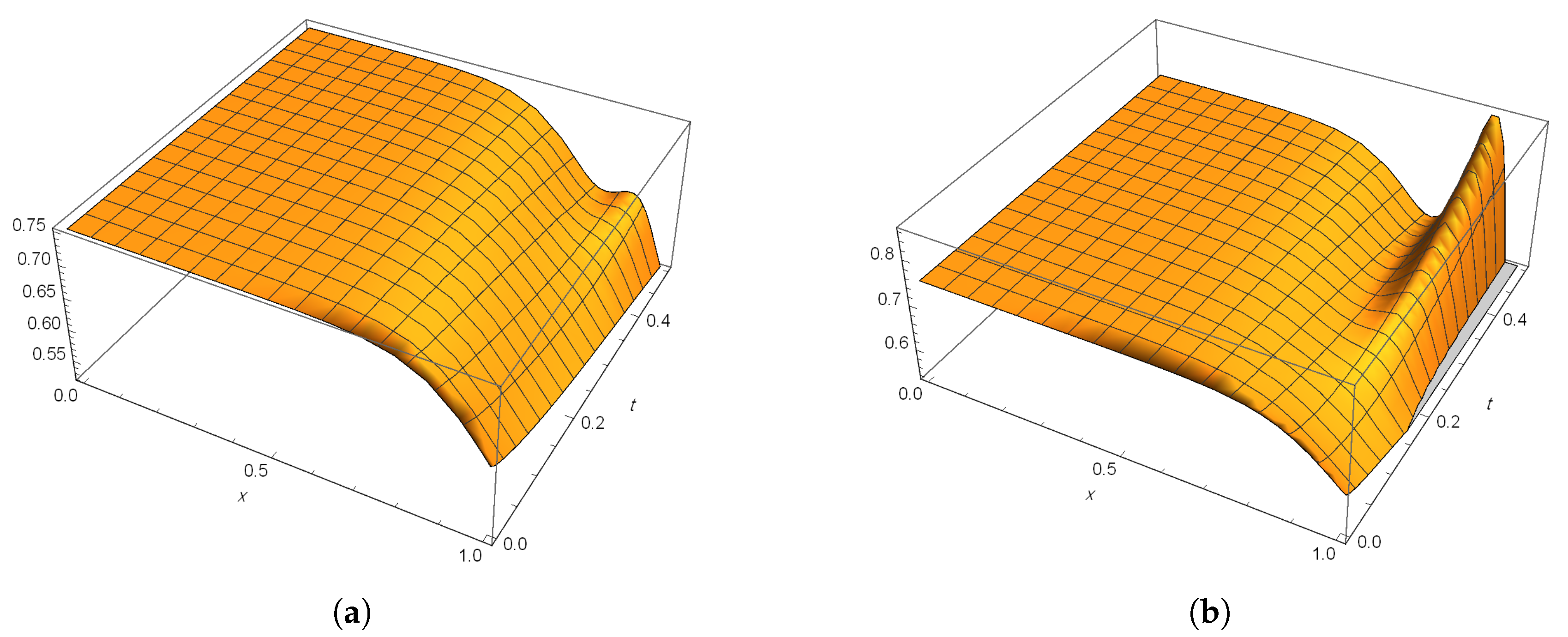
4.1. Example 1
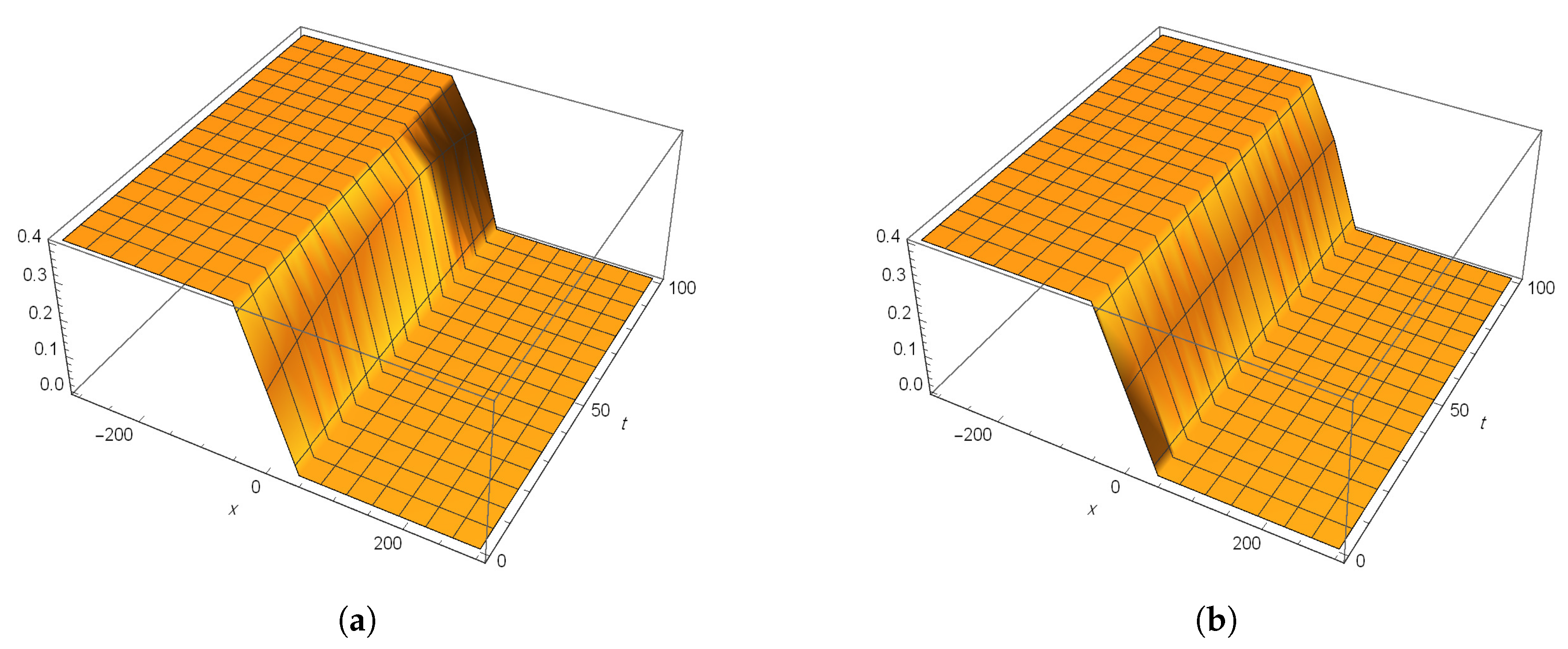
4.2. Example 2
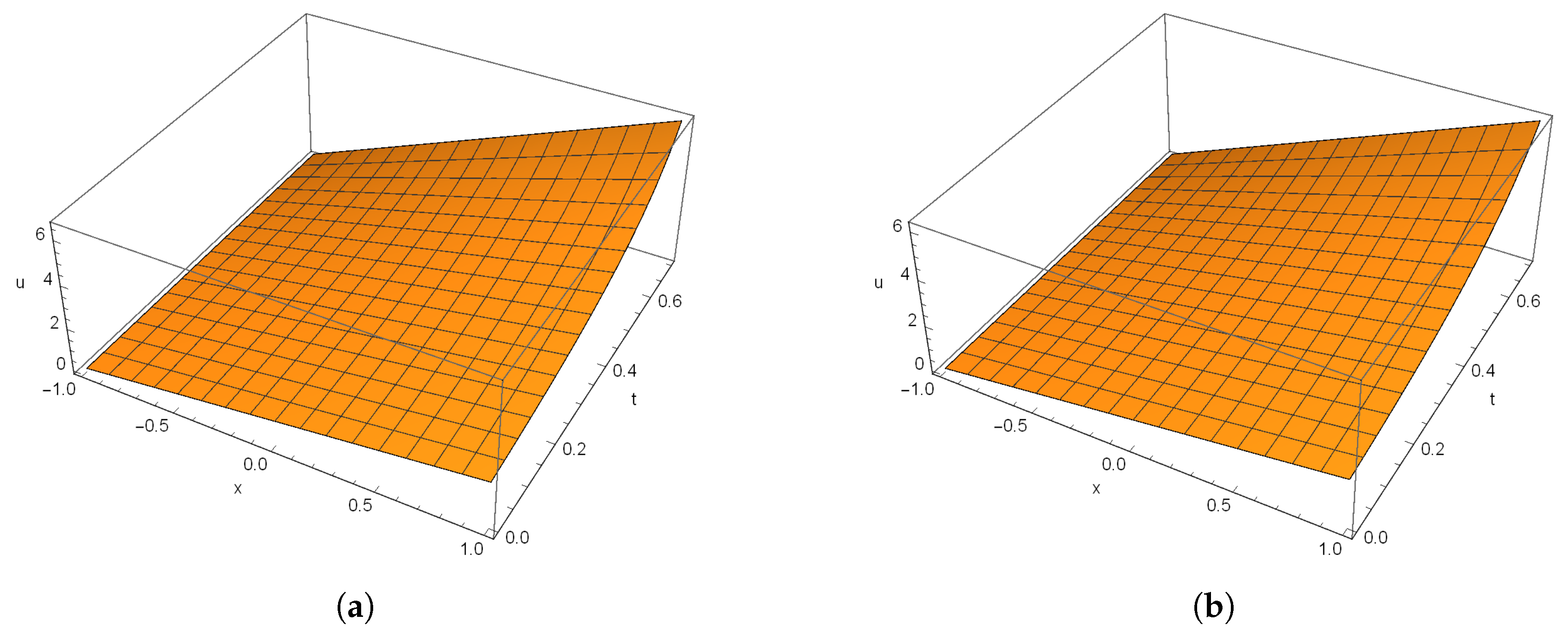
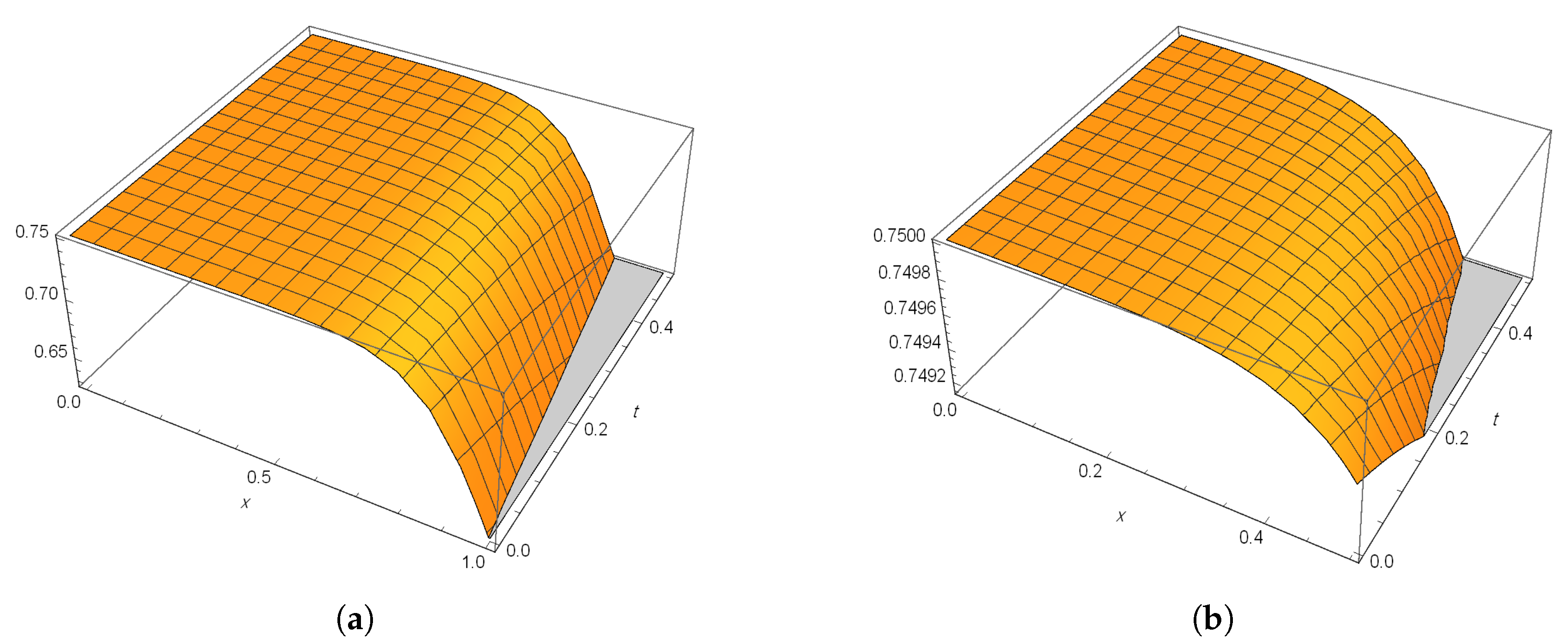
4.3. Example 3
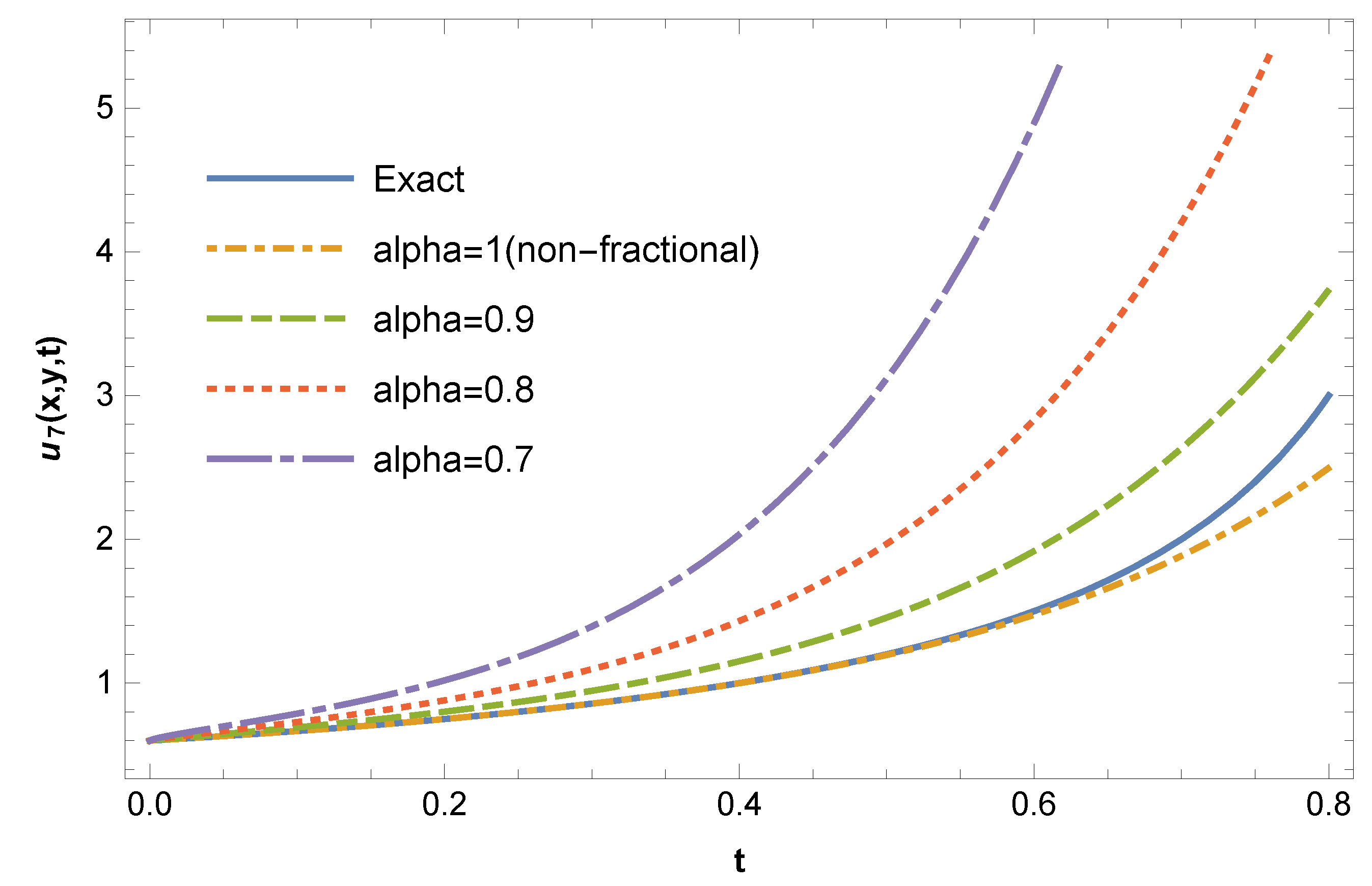
5. Results and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North Holland Mathematics Studies; Elsevier: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley-Interscience: New York, NY, USA, 1993. [Google Scholar]
- Oldham, K.; Spanier, J. The Fractional Calculus Theory and Applications of Differentiation and Integration to Arbitrary Order; Academic Press: New York, NY, USA, 1974; Volume 111. [Google Scholar]
- Podlubny, I. Fractional Differential Equations, Mathematics in Science and Engineering; Academic Press: San Diego, CA, USA, 1999; Volume 198. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach Science Publishers: Yverdon, Switzerland, 1993. [Google Scholar]
- Abuasad, S.; Yildirim, A.; Hashim, I.; Abdul Karim, S.A.; Gómez-Aguilar, J. Fractional Multi-Step Differential Transformed Method for Approximating a Fractional Stochastic SIS Epidemic Model with Imperfect Vaccination. Int. J. Environ. Res. Public Health 2019, 16, 973. [Google Scholar] [CrossRef] [Green Version]
- Ross, B. A Brief History and Exposition of the Fundamental Theory of Fractional Calculus. In Fractional Calculus and Its Applications. Lecture Notes in Mathematics; Springer: Berlin/Heidelberg, Germany, 1975; Volume 457, pp. 1–36. [Google Scholar]
- Abuasad, S.; Hashim, I. Homotopy decomposition method for solving one-dimensional time-fractional diffusion equation. In AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2018; Volume 1940, p. 020126. [Google Scholar]
- Abuasad, S.; Hashim, I. Homotopy Decomposition Method for Solving Higher-Order Time- Fractional Diffusion Equation via Modified Beta Derivative. Sains Malays. 2018, 47, 2899–2905. [Google Scholar] [CrossRef]
- Zhou, J. Differential Transformation and Its Applications for Electrical Circuits; Huazhong University Press: Wuhan, China, 1986. [Google Scholar]
- Ayaz, F. Solutions of the system of differential equations by differential transform method. Appl. Math. Comput. 2004, 147, 547–567. [Google Scholar] [CrossRef]
- Ayaz, F. Applications of differential transform method to differential-algebraic equations. Appl. Math. Comput. 2004, 152, 649–657. [Google Scholar] [CrossRef]
- Ayaz, F. On the two-dimensional differential transform method. Appl. Math. Comput. 2003, 143, 361–374. [Google Scholar] [CrossRef]
- Hassan, I.A.H. Application to differential transformation method for solving systems of differential equations. Appl. Math. Model. 2008, 32, 2552–2559. [Google Scholar] [CrossRef]
- Arikoglu, A.; Ozkol, I. Solution of boundary value problems for integro-differential equations by using differential transform method. Appl. Math. Comput. 2005, 168, 1145–1158. [Google Scholar] [CrossRef]
- Keskin, Y.; Oturanc, G. Reduced differential transform method for partial differential equations. Int. J. Nonlinear Sci. Numer. Simul. 2009, 10, 741–749. [Google Scholar] [CrossRef]
- Srivastava, V.K.; Mishra, N.; Kumar, S.; Singh, B.K.; Awasthi, M.K. Reduced differential transform method for solving (1 + n)–Dimensional Burgers’ equation. Egypt. J. Basic Appl. Sci. 2014, 1, 115–119. [Google Scholar] [CrossRef] [Green Version]
- Keskin, Y.; Oturanc, G. The Reduced Differential Transform Method: A New Approach to Fractional Partial Differential Equations. Nonlinear Sci. Lett. A 2010, 1, 207–217. [Google Scholar]
- Abuasad, S.; Hashim, I.; Abdul Karim, S.A. Modified Fractional Reduced Differential Transform Method for the Solution of Multiterm Time-Fractional Diffusion Equations. Adv. Math. Phys. 2019, 2019, 5703916. [Google Scholar] [CrossRef]
- Gupta, P.K. Approximate analytical solutions of fractional Benney–Lin equation by reduced differential transform method and the homotopy perturbation method. Comput. Math. Appl. 2011, 61, 2829–2842. [Google Scholar] [CrossRef] [Green Version]
- Rawashdeh, M.S. An efficient approach for time-fractional damped Burger and time-sharma-tasso-Olver equations using the FRDTM. Appl. Math. Inf. Sci. 2015, 9, 1239–1246. [Google Scholar]
- Srivastava, V.K.; Kumar, S.; Awasthi, M.K.; Singh, B.K. Two-dimensional time fractional-order biological population model and its analytical solution. Egypt. J. Basic Appl. Sci. 2014, 1, 71–76. [Google Scholar] [CrossRef] [Green Version]
- Rawashdeh, M. A new approach to solve the fractional Harry Dym equation using the FRDTM. Int. J. Pure Appl. Math. 2014, 95, 553–566. [Google Scholar] [CrossRef] [Green Version]
- Singh, B.K.; Srivastava, V.K. Approximate series solution of multi-dimensional, time fractional-order (heat-like) diffusion equations using FRDTM. R. Soc. Open Sci. 2015, 2, 140511–140524. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Singh, B.K. Fractional reduced differential transform method for numerical computation of a system of linear and nonlinear fractional partial differential equations. Int. J. Open Probl. Comput. Math. 2016, 9, 20–38. [Google Scholar] [CrossRef]
- Rawashdeh, M. A reliable method for the space–time fractional Burgers and time-fractional Cahn-Allen equations via the FRDTM. Adv. Differ. Equ. 2017, 2017, 99. [Google Scholar] [CrossRef]
- Arshad, M.; Lu, D.; Wang, J. (N + 1)-dimensional fractional reduced differential transform method for fractional order partial differential equations. Commun. Nonlinear Sci. Numer. Simul. 2017, 48, 509–519. [Google Scholar] [CrossRef]
- Abuasad, S.; Moaddy, K.; Hashim, I. Analytical treatment of two-dimensional fractional Helmholtz equations. J. King Saud Univ. Sci. 2019, 31, 659–666. [Google Scholar] [CrossRef]
- Burgers, J.M. A mathematical model illustrating the theory of turbulence. Adv. Appl. Mech. 1948, 1, 171–199. [Google Scholar]
- Hopf, E. The partial differential equation ut + uux = μxx. Comm. Pure Appl. Math. 1950, 3, 201–230. [Google Scholar] [CrossRef]
- Gurbatov, S.; Simdyankin, S.; Aurell, E.; Frisch, U.; Toth, G. On the decay of Burgers turbulence. J. Fluid Mech. 1997, 344, 339–374. [Google Scholar] [CrossRef] [Green Version]
- Musha, T.; Higuchi, H. Traffic current fluctuation and the Burgers equation. Jpn. J. Appl. Phys. 1978, 17, 811–816. [Google Scholar] [CrossRef]
- Blackstock, D.T. Generalized Burgers equation for plane waves. J. Acoustical. Soc. Am. 1985, 77, 2050–2053. [Google Scholar] [CrossRef]
- Esen, A.; Tasbozan, O. Numerical solution of time fractional Burgers equation by cubic B-spline finite elements. Mediterr. J. Math. 2016, 13, 1325–1337. [Google Scholar] [CrossRef]
- Li, D.; Zhang, C.; Ran, M. A linear finite difference scheme for generalized time fractional Burgers equation. Appl. Math. Model. 2016, 40, 6069–6081. [Google Scholar] [CrossRef]
- Miškinis, P. Some properties of fractional Burgers equation. Math. Model. Anal. 2002, 7, 151–158. [Google Scholar] [CrossRef] [Green Version]
- Momani, S. Non-perturbative analytical solutions of the space-and time-fractional Burgers equations. Chaos Solitons Fractals 2006, 28, 930–937. [Google Scholar] [CrossRef]
- Safari, M.; Ganji, D.; Moslemi, M. Application of He’s variational iteration method and Adomian’s decomposition method to the fractional KdV–Burgers–Kuramoto equation. Comput. Math. Appl. 2009, 58, 2091–2097. [Google Scholar] [CrossRef] [Green Version]
- Hilfer, R.; Luchko, Y. Desiderata for Fractional Derivatives and Integrals. Mathematics 2019, 7, 149. [Google Scholar] [CrossRef] [Green Version]
- Baleanu, D.; Fernandez, A. On Fractional Operators and Their Classifications. Mathematics 2019, 7, 830. [Google Scholar] [CrossRef] [Green Version]
- Saad, K.; Al-Sharif, E.H. Analytical study for time and time-space fractional Burgers’ equation. Adv. Differ. Equ. 2017, 2017, 300. [Google Scholar] [CrossRef] [Green Version]
- Ray, S.S. A New Coupled Fractional Reduced Differential Transform Method for the Numerical Solutions of (2 + 1)–Dimensional Time Fractional Coupled Burger Equations. Model. Simul. Eng. 2014, 960241. [Google Scholar] [CrossRef] [Green Version]



| Original Function | Transformed Function |
|---|---|
| , | |
| , | |
| , |
| Non-Fractional Order | Fractional Order | ||||||
|---|---|---|---|---|---|---|---|
| x | t | ||||||
| −300 | 0 | 0.0399011 | 0.0399011 | 0 | 0 | 0.0399011 | 0.0399011 |
| 20 | 0.0399019 | 0.0399019 | 1.6237 × 10−14 | 8.36083 × 10−12 | 0.0399017 | 0.0399016 | |
| 40 | 0.0399027 | 0.0399027 | 2.59348 × 10−13 | 6.67572 × 10−11 | 0.0399022 | 0.0399019 | |
| 60 | 0.0399034 | 0.0399034 | 1.31092 × 10−12 | 2.2487 × 10−10 | 0.0399027 | 0.0399022 | |
| 80 | 0.0399042 | 0.0399042 | 4.13682 × 10−12 | 5.31996 × 10−10 | 0.0399032 | 0.0399025 | |
| 100 | 0.039905 | 0.039905 | 1.00842 × 10−11 | 1.03705 × 10−9 | 0.0399036 | 0.0399028 | |
| −100 | 0 | 0.0352319 | 0.0352319 | 0 | 0 | 0.0352319 | 0.0352319 |
| 20 | 0.0352654 | 0.0352654 | 1.40942 × 10−13 | 2.47381 × 10−10 | 0.0352577 | 0.0352517 | |
| 40 | 0.0352987 | 0.0352987 | 2.23993 × 10−12 | 1.98016 × 10−9 | 0.03528 | 0.0352663 | |
| 60 | 0.0353318 | 0.0353318 | 1.12634 × 10−11 | 6.68676 × 10−9 | 0.035301 | 0.0352794 | |
| 80 | 0.0353646 | 0.0353646 | 3.5358 × 10−11 | 1.58587 × 10−8 | 0.0353212 | 0.0352916 | |
| 100 | 0.0353973 | 0.0353973 | 8.57394 × 10−11 | 3.09908 × 10−8 | 0.0353409 | 0.0353031 | |
| 100 | 0 | 0.00476812 | 0.00476812 | 0 | 0 | 0.00476812 | 0.00476812 |
| 20 | 0.00480182 | 0.00480182 | 1.42837 × 10−13 | 1.78436 × 10−11 | 0.00479407 | 0.00478797 | |
| 40 | 0.00483572 | 0.00483572 | 2.30062 × 10−12 | 1.41591 × 10−10 | 0.00481666 | 0.00480275 | |
| 60 | 0.00486984 | 0.00486984 | 1.17243 × 10−11 | 4.73909 × 10−10 | 0.00483819 | 0.00481609 | |
| 80 | 0.00490415 | 0.00490415 | 3.73003 × 10−11 | 1.11383 × 10−9 | 0.00485909 | 0.00482858 | |
| 100 | 0.00493868 | 0.00493868 | 9.16666 × 10−11 | 2.15664 × 10−9 | 0.00487955 | 0.00484049 | |
| 300 | 0 | 0.0000989049 | 0.0000989049 | 0 | 4.20128 × 10−19 | 0.0000989049 | 0.0000989049 |
| 20 | 0.0000996974 | 0.0000996974 | 1.62847 × 10−14 | 8.22805 × 10−22 | 0.0000995152 | 0.0000993717 | |
| 40 | 0.000100496 | 0.000100496 | 2.60942 × 10−13 | 6.59551 × 10−11 | 0.000100047 | 0.0000997194 | |
| 60 | 0.000101301 | 0.000101301 | 1.32305 × 10−12 | 2.2304 × 10−10 | 0.000100555 | 0.000100034 | |
| 80 | 0.000102113 | 0.000102113 | 4.18795 × 10−12 | 5.29741 × 10−10 | 0.000101048 | 0.000100328 | |
| 100 | 0.000102931 | 0.000102931 | 1.02402 × 10−11 | 1.03671 × 10−9 | 0.000101532 | 0.000100609 |
| Non-Fractional Order | Fractional Order | ||||||
|---|---|---|---|---|---|---|---|
| x | t | Absolute error | |||||
| −0.8 | 0 | 0.2 | 0.2 | 0 | 0.2 | 0.2 | 0.2 |
| 0.2 | 0.249999 | 0.25 | 6.4 × 10−7 | 0.267012 | 0.339529 | 0.705107 | |
| 0.4 | 0.333115 | 0.333333 | 0.000218453 | 0.383913 | 0.677598 | 2.51926 | |
| 0.6 | 0.491602 | 0.5 | 0.00839808 | 0.639169 | 1.6304 | 7.11893 | |
| −0.5 | 0 | 0.5 | 0.5 | 0 | 0.5 | 0.5 | 0.5 |
| 0.2 | 0.624998 | 0.625 | 1.6 × 10−6 | 0.66753 | 0.848823 | 1.76277 | |
| 0.4 | 0.832787 | 0.833333 | 0.000546133 | 0.959782 | 1.694 | 6.29816 | |
| 0.6 | 1.229 | 1.25 | 0.0209952 | 1.59792 | 4.07599 | 17.7973 | |
| 0 | 0 | 1 | 1 | 0 | 1 | 1 | 1 |
| 0.2 | 1.25 | 1.25 | 3.2 × 10−6 | 1.33506 | 1.69765 | 3.52553 | |
| 0.4 | 1.66557 | 1.66667 | 0.00109227 | 1.91956 | 3.38799 | 12.5963 | |
| 0.6 | 2.45801 | 2.5 | 0.0419904 | 3.19585 | 8.15198 | 35.5946 | |
| 0.5 | 0 | 1.5 | 1.5 | 0 | 1.5 | 1.5 | 1.5 |
| 0.2 | 1.875 | 1.875 | 4.8 × 10−6 | 2.00259 | 2.54647 | 5.2883 | |
| 0.4 | 2.49836 | 2.5 | 0.0016384 | 2.87935 | 5.08199 | 18.8945 | |
| 0.6 | 3.68701 | 3.75 | 0.0629856 | 4.79377 | 12.228 | 53.3919 | |
| 1 | 0 | 2 | 2 | 0 | 2 | 2 | 2 |
| 0.2 | 2.49999 | 2.5 | 6.4 × 10−6 | 2.67012 | 3.39529 | 7.05107 | |
| 0.4 | 3.33115 | 3.33333 | 0.00218453 | 3.83913 | 6.77598 | 25.1926 | |
| 0.6 | 4.91602 | 5. | 0.0839808 | 6.39169 | 16.304 | 71.1893 |
| Non-Fractional Order | Fractional Order | ||||||
|---|---|---|---|---|---|---|---|
| x | t | Absolute error | |||||
| 0.1 | 0 | 0.749997 | 0.749997 | 0 | 0.749997 | 0.749997 | 0.749997 |
| 0.1 | 0.749951 | 0.749996 | 0.0000441493 | 0.749951 | 0.749951 | 0.749951 | |
| 0.2 | 0.749911 | 0.749994 | 0.0000827147 | 0.749911 | 0.749911 | 0.749911 | |
| 0.3 | 0.74987 | 0.749992 | 0.00012135 | 0.74987 | 0.74987 | 0.74987 | |
| 0.4 | 0.749829 | 0.749989 | 0.000159937 | 0.749829 | 0.749829 | 0.749829 | |
| 0.5 | 0.749786 | 0.749984 | 0.000198136 | 0.749786 | 0.749786 | 0.749786 | |
| 0.2 | 0 | 0.749989 | 0.749989 | 0 | 0.749989 | 0.749989 | 0.749989 |
| 0.1 | 0.74983 | 0.749984 | 0.000154016 | 0.74983 | 0.74983 | 0.74983 | |
| 0.2 | 0.74969 | 0.749979 | 0.000288529 | 0.74969 | 0.74969 | 0.74969 | |
| 0.3 | 0.749548 | 0.749971 | 0.00042328 | 0.749548 | 0.749548 | 0.749548 | |
| 0.4 | 0.749403 | 0.74996 | 0.000557856 | 0.749403 | 0.749403 | 0.749403 | |
| 0.5 | 0.749255 | 0.749946 | 0.000691078 | 0.749255 | 0.749255 | 0.749255 | |
| 0.3 | 0 | 0.74996 | 0.74996 | 0 | 0.74996 | 0.74996 | 0.74996 |
| 0.1 | 0.749409 | 0.749946 | 0.000536592 | 0.749409 | 0.749409 | 0.749409 | |
| 0.2 | 0.748921 | 0.749926 | 0.00100496 | 0.748921 | 0.748921 | 0.748921 | |
| 0.3 | 0.748425 | 0.749899 | 0.00147406 | 0.748425 | 0.748425 | 0.748425 | |
| 0.4 | 0.747919 | 0.749862 | 0.0019425 | 0.747919 | 0.747919 | 0.747919 | |
| 0.5 | 0.747405 | 0.749811 | 0.00240619 | 0.747405 | 0.747405 | 0.747405 | |
| 0.4 | 0 | 0.749862 | 0.749862 | 0 | 0.749862 | 0.749862 | 0.749862 |
| 0.1 | 0.74795 | 0.749811 | 0.00186104 | 0.74795 | 0.74795 | 0.74795 | |
| 0.2 | 0.74626 | 0.749742 | 0.00348202 | 0.74626 | 0.74626 | 0.74626 | |
| 0.3 | 0.744543 | 0.749647 | 0.00510455 | 0.744543 | 0.744543 | 0.744543 | |
| 0.4 | 0.742794 | 0.749518 | 0.00672411 | 0.742794 | 0.742794 | 0.742794 | |
| 0.5 | 0.741015 | 0.749342 | 0.00832666 | 0.741015 | 0.741015 | 0.741015 | |
| 0.4 | 0 | 0.749518 | 0.749518 | 0 | 0.749518 | 0.749518 | 0.749518 |
| 0.1 | 0.742989 | 0.749342 | 0.0063529 | 0.742989 | 0.742989 | 0.742989 | |
| 0.2 | 0.737257 | 0.749102 | 0.011845 | 0.737257 | 0.737257 | 0.737257 | |
| 0.3 | 0.731444 | 0.748774 | 0.0173301 | 0.731444 | 0.731444 | 0.731444 | |
| 0.4 | 0.72553 | 0.748327 | 0.0227968 | 0.72553 | 0.72553 | 0.72553 | |
| 0.5 | 0.719519 | 0.747719 | 0.0281996 | 0.719519 | 0.719519 | 0.719519 |
| Non-Fractional Order | Fractional Order | ||||||
|---|---|---|---|---|---|---|---|
| x | t | Absolute error | |||||
| 0.1 | 0 | 0.750003 | 0.749997 | 6.50356e-6 | 0.750003 | 0.750003 | 0.750003 |
| 0.1 | 0.750049 | 0.749996 | 0.0000530386 | 0.750049 | 0.750049 | 0.750049 | |
| 0.2 | 0.750089 | 0.749994 | 0.0000948648 | 0.750089 | 0.750089 | 0.750089 | |
| 0.3 | 0.75013 | 0.749992 | 0.000137957 | 0.75013 | 0.75013 | 0.75013 | |
| 0.4 | 0.750171 | 0.749989 | 0.000182636 | 0.750171 | 0.750171 | 0.750171 | |
| 0.5 | 0.750214 | 0.749984 | 0.000229162 | 0.750214 | 0.750214 | 0.750214 | |
| 0.2 | 0 | 0.750011 | 0.749989 | 0.0000226989 | 0.750011 | 0.750011 | 0.750011 |
| 0.1 | 0.75017 | 0.749984 | 0.000185041 | 0.75017 | 0.75017 | 0.75017 | |
| 0.2 | 0.75031 | 0.749979 | 0.000330935 | 0.75031 | 0.75031 | 0.75031 | |
| 0.3 | 0.750452 | 0.749971 | 0.000481239 | 0.750452 | 0.750452 | 0.750452 | |
| 0.4 | 0.750597 | 0.74996 | 0.000637074 | 0.750597 | 0.750597 | 0.750597 | |
| 0.5 | 0.750745 | 0.749946 | 0.00079935 | 0.750745 | 0.750745 | 0.750745 | |
| 0.3 | 0 | 0.75004 | 0.74996 | 0.0000792181 | 0.75004 | 0.75004 | 0.75004 |
| 0.1 | 0.750591 | 0.749946 | 0.000644864 | 0.750591 | 0.750591 | 0.750591 | |
| 0.2 | 0.751079 | 0.749926 | 0.00115293 | 0.751079 | 0.751079 | 0.751079 | |
| 0.3 | 0.751575 | 0.749899 | 0.0016763 | 0.751575 | 0.751575 | 0.751575 | |
| 0.4 | 0.752081 | 0.749862 | 0.00221889 | 0.752081 | 0.752081 | 0.752081 | |
| 0.5 | 0.752595 | 0.749811 | 0.00278389 | 0.752595 | 0.752595 | 0.752595 | |
| 0.4 | 0 | 0.750138 | 0.749862 | 0.000276389 | 0.750138 | 0.750138 | 0.750138 |
| 0.1 | 0.75205 | 0.749811 | 0.00223874 | 0.75205 | 0.75205 | 0.75205 | |
| 0.2 | 0.75374 | 0.749742 | 0.00399813 | 0.75374 | 0.75374 | 0.75374 | |
| 0.3 | 0.755457 | 0.749647 | 0.00580973 | 0.755457 | 0.755457 | 0.755457 | |
| 0.4 | 0.757206 | 0.749518 | 0.00768747 | 0.757206 | 0.757206 | 0.757206 | |
| 0.5 | 0.758985 | 0.749342 | 0.0096425 | 0.758985 | 0.758985 | 0.758985 | |
| 0.5 | 0 | 0.750482 | 0.749518 | 0.000963367 | 0.750482 | 0.750482 | 0.750482 |
| 0.1 | 0.757011 | 0.749342 | 0.00766873 | 0.757011 | 0.757011 | 0.757011 | |
| 0.2 | 0.762743 | 0.749102 | 0.0136418 | 0.762743 | 0.762743 | 0.762743 | |
| 0.3 | 0.768556 | 0.748774 | 0.0197828 | 0.768556 | 0.768556 | 0.768556 | |
| 0.4 | 0.77447 | 0.748327 | 0.0261433 | 0.77447 | 0.77447 | 0.77447 | |
| 0.5 | 0.780481 | 0.747719 | 0.0327624 | 0.780481 | 0.780481 | 0.780481 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mukhtar, S.; Abuasad, S.; Hashim, I.; Abdul Karim, S.A. Effective Method for Solving Different Types of Nonlinear Fractional Burgers’ Equations. Mathematics 2020, 8, 729. https://doi.org/10.3390/math8050729
Mukhtar S, Abuasad S, Hashim I, Abdul Karim SA. Effective Method for Solving Different Types of Nonlinear Fractional Burgers’ Equations. Mathematics. 2020; 8(5):729. https://doi.org/10.3390/math8050729
Chicago/Turabian StyleMukhtar, Safyan, Salah Abuasad, Ishak Hashim, and Samsul Ariffin Abdul Karim. 2020. "Effective Method for Solving Different Types of Nonlinear Fractional Burgers’ Equations" Mathematics 8, no. 5: 729. https://doi.org/10.3390/math8050729
APA StyleMukhtar, S., Abuasad, S., Hashim, I., & Abdul Karim, S. A. (2020). Effective Method for Solving Different Types of Nonlinear Fractional Burgers’ Equations. Mathematics, 8(5), 729. https://doi.org/10.3390/math8050729






