A Closed-Form Solution of Prestressed Annular Membrane Internally-Connected with Rigid Circular Plate and Transversely-Loaded by Central Shaft
Abstract
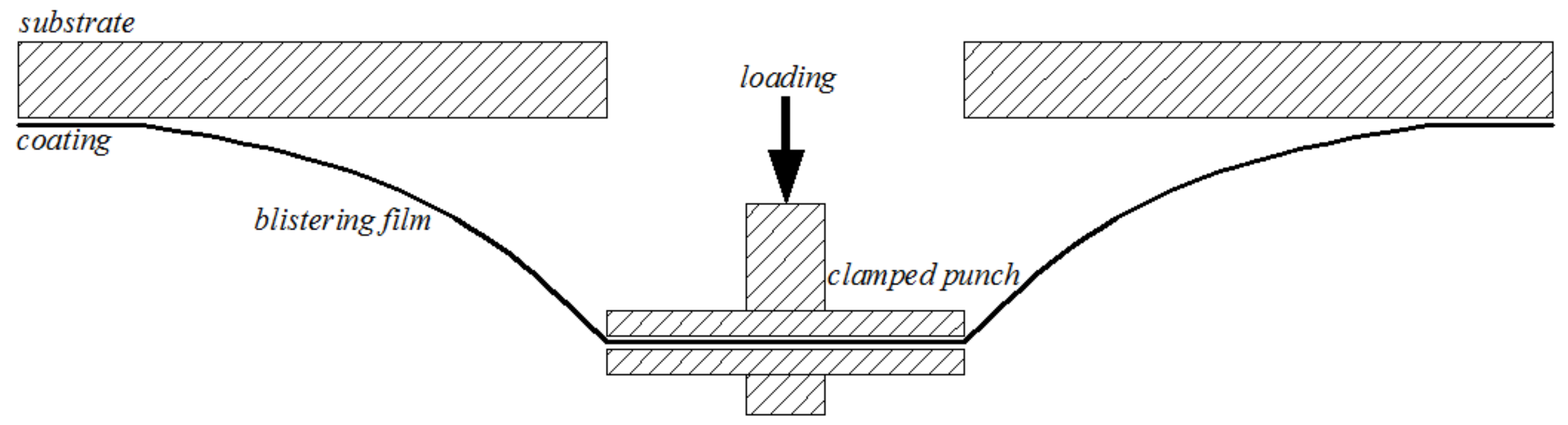
:1. Introduction
2. Membrane Equation and Its Solution
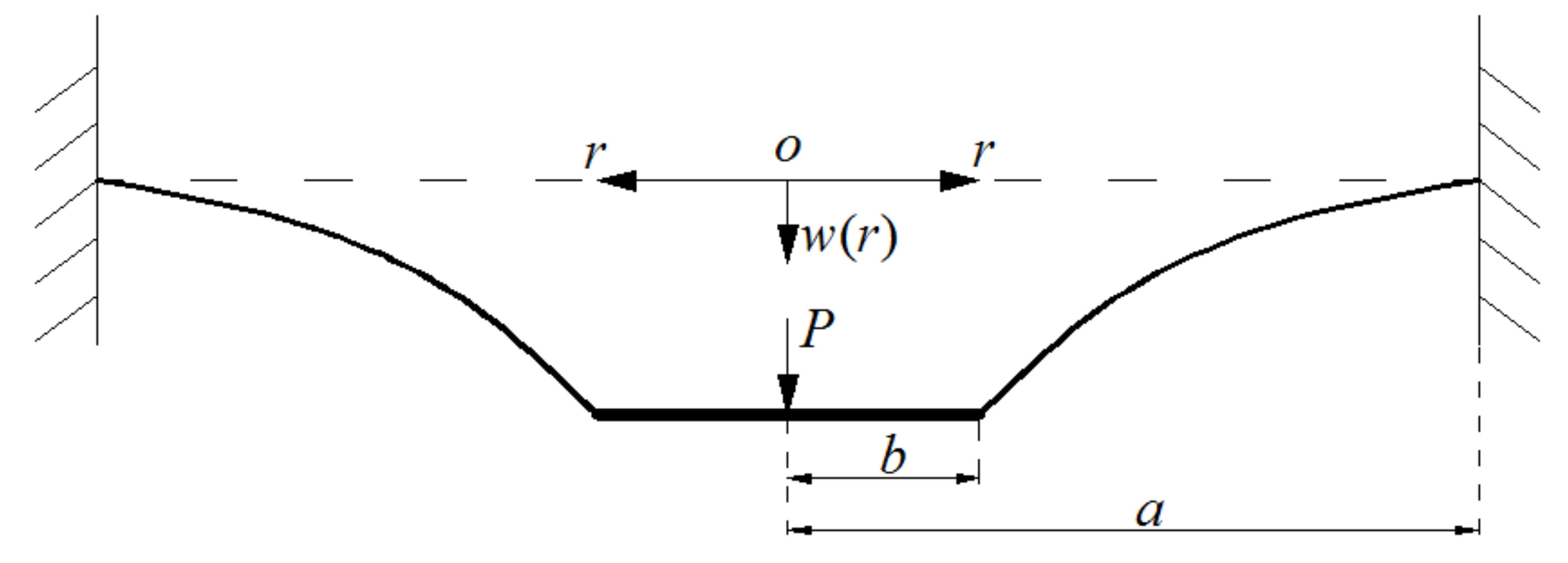
2.1. Establishment of Membrane Equations
2.2. Establishment of Boundary Conditions Considering Initial Stress
2.3. Nondimensionalization
2.4. Analytical Solution to Equation (31)
3. Results and Discussions
3.1. Comparison with Existing Work
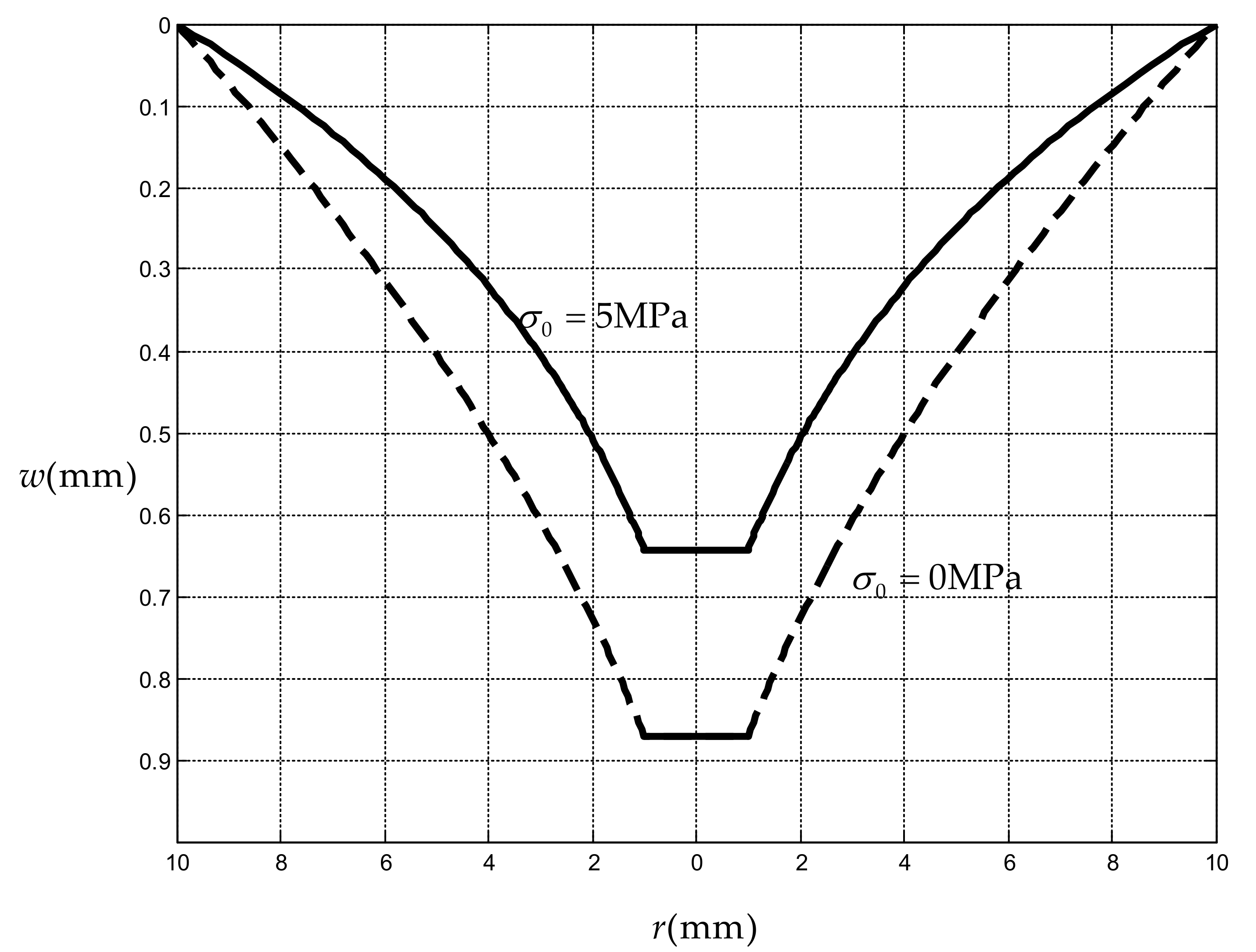
3.2. Numerical Example
4. Concluding Remarks
Author Contributions
Funding
Conflicts of Interest
References
- Küçük, I. Optimality conditions for the control of a double-membrane complex system by a modal decomposition technique. Appl. Math. Model. 2008, 32, 562–574. [Google Scholar] [CrossRef]
- Zhao, M.; Zheng, W.; Fan, C. Mechanics of shaft-loaded blister test for thin film suspended on compliant substrate. Int. J. Solids Struct. 2010, 47, 2525–2532. [Google Scholar] [CrossRef] [Green Version]
- Sun, J.-Y.; Hu, J.-L.; Zheng, Z.-L.; He, X.-T.; Geng, H.-H. A practical method for simultaneous determination of Poisson’s ratio and Young’s modulus of elasticity of thin films. J. Mech. Sci. Technol. 2011, 25, 3165–3171. [Google Scholar] [CrossRef]
- Xu, D.; Liechti, K. Analytical and experimental study of a circular membrane in adhesive contact with a rigid substrate. Int. J. Solids Struct. 2011, 48, 2965–2976. [Google Scholar] [CrossRef]
- Sun, J.-Y.; Qian, S.-H.; Li, Y.-M.; He, X.-T.; Zheng, Z.-L. Theoretical study of adhesion energy measurement for film/substrate interface using pressurized blister test: Energy release rate. Meas. 2013, 46, 2278–2287. [Google Scholar] [CrossRef]
- Di Barba, P.; Fattorusso, L.; Versaci, M. A 2D Non-Linear Second-Order Differential Model for Electrostatic Circular Membrane MEMS Devices: A Result of Existence and Uniqueness. Math. 2019, 7, 1193. [Google Scholar] [CrossRef] [Green Version]
- Arthurs, A.M.; Clegg, J. On the Solution of a Boundary Value Problem for the Nonlinear Föppl-Hencky Equation. ZAMM 1994, 74, 281–284. [Google Scholar] [CrossRef]
- Kublanov, L.; Bottega, W. On pressing of a buckled film. Appl. Math. Model. 1995, 19, 499–507. [Google Scholar] [CrossRef]
- Plaut, R. Linearly elastic annular and circular membranes under radial, transverse, and torsional loading. Part I: Large unwrinkled axisymmetric deformations. Acta Mech. 2008, 202, 79–99. [Google Scholar] [CrossRef]
- Soares, R.M.; Gonçalves, P.B. Nonlinear vibrations and instabilities of a stretched hyperelastic annular membrane. Int. J. Solids Struct. 2012, 49, 514–526. [Google Scholar] [CrossRef] [Green Version]
- Long, R.; Hui, C.-Y. Axisymmetric membrane in adhesive contact with rigid substrates: Analytical solutions under large deformation. Int. J. Solids Struct. 2012, 49, 672–683. [Google Scholar] [CrossRef] [Green Version]
- Atai, A.; Steigmann, D. Numerical analysis of wrinkled, anisotropic, nonlinearly elastic membranes. Mech. Res. Commun. 2014, 57, 1–5. [Google Scholar] [CrossRef]
- Sun, J.-Y.; Rong, Y.; He, X.-T.; Gao, X.-W.; Zheng, Z.-L. Power series solution of circular membrane under uniformly distributed loads: Investigation into Hencky transformation. Struct. Eng. Mech. 2013, 45, 631–641. [Google Scholar] [CrossRef]
- Zhumanazarova, A.; Cho, Y.I. Asymptotic Convergence of the Solution of a Singularly Perturbed Integro-Differential Boundary Value Problem. Mathematics 2020, 8, 213. [Google Scholar] [CrossRef] [Green Version]
- Ahmad, B.; Alruwaily, Y.; Alsaedi, A.; Ntouyas, S.K. Existence and Stability Results for a Fractional Order Differential Equation with Non-Conjugate Riemann-Stieltjes Integro-Multipoint Boundary Conditions. Mathematics 2019, 7, 249. [Google Scholar] [CrossRef] [Green Version]
- Mahmudov, N.I.; Emin, S.; Bawanah, S. On the Parametrization of Caputo-Type Fractional Differential Equations with Two-Point Nonlinear Boundary Conditions. Mathematics 2019, 7, 707. [Google Scholar] [CrossRef] [Green Version]
- Versaci, M.; Angiulli, G.; Fattorusso, L.; Jannelli, A. On the uniqueness of the solution for a semi-linear elliptic boundary value problem of the membrane MEMS device for reconstructing the membrane profile in absence of ghost solutions. Int. J. Non-linear Mech. 2019, 109, 24–31. [Google Scholar] [CrossRef]
- Hencky, H. Über den Spannungszustand in kreisrunden Platten mit verschwindender Biegungssteifigkeit. Z. Math. Physik 1915, 63, 311–317. [Google Scholar]
- Chien, W.Z. Asymptotic behavior of a thin clamped circular plate under uniform normal pressure at very large deflection. Sci. Rep. Natl. Tsinghua Univ. 1948, 5, 193–208. [Google Scholar]
- Alekseev, S.A. Elastic circular membranes under the uniformly distributed loads. Eng. Corpus 1953, 14, 196–198. [Google Scholar]
- Alekseev, S.A. Elastic annular membranes with a stiff centre under the concentrated force. Eng. Corpus 1951, 10, 71–80. [Google Scholar]
- Sun, J.-Y.; Hu, J.-L.; He, X.-T.; Zheng, Z.-L. A theoretical study of a clamped punch-loaded blister configuration: The quantitative relation of load and deflection. Int. J. Mech. Sci. 2010, 52, 928–936. [Google Scholar] [CrossRef]
- Chien, W.Z.; Wang, Z.Z.; Xu, Y.G.; Chen, S.L. The symmetrical deformation of circular membrane under the action of uniformly distributed loads in its portion. Appl. Math. Mech. 1981, 2, 653–668. [Google Scholar]
- Sun, J.-Y.; Hu, J.-L.; He, X.-T.; Zheng, Z.-L.; Geng, H.-H. A Theoretical Study of Thin Film Delamination Using Clamped Punch-Loaded Blister Test: Energy Release Rate and Closed-Form Solution. J. Adhes. Sci. Technol. 2011, 25, 2063–2080. [Google Scholar] [CrossRef]
- Sun, J.-Y.; Lian, Y.S.; Li, Z.; He, X.-T.; Zheng, Z. Theoretical study on shaft-loaded blister test technique: Synchronous characterization of surface and interfacial mechanical properties. Int. J. Adhes. Adhes. 2014, 51, 128–139. [Google Scholar] [CrossRef]
- Lian, Y.S.; Sun, J.-Y.; Yang, Z.-X.; He, X.-T.; Zheng, Z.-L. Closed-form solution of well-known Hencky problem without small-rotation-angle assumption. ZAMM 2016, 96, 1434–1441. [Google Scholar] [CrossRef]
- Yang, Z.X.; Sun, J.-Y.; Ran, G.M.; He, X.-T. A New Solution to Főppl-Hencky Membrane Equation. J. Mech. 2017, 33, N7–N11. [Google Scholar] [CrossRef]
- Angiulli, G.; Jannelli, A.; Morabito, F.C.; Versaci, M. Reconstructing the membrane detection of a 1D electrostatic-driven MEMS device by the shooting method: Convergence analysis and ghost solutions identification. Comput. Appl. Math. 2018, 37, 4484–4498. [Google Scholar] [CrossRef]
- Versaci, M.; Morabito, F.C. Membrane Micro Electro-Mechanical Systems for Industrial Applications. Implement. Comput. Intell. Tech. Secur. Syst. Des. 2020, 139–175. [Google Scholar]
- Crăciun, E.M.; Baesu, E.; Soós, E. “General solution in terms of complex potentials in antiplane states in initial stressed and prepolarized piezoelectric crystals: Application to Mode III fracture propagation. J. Appl. Math. 2005, 70, 39–52. [Google Scholar]

| Symbol | Description |
|---|---|
| , , | Young’s modulus, Poisson’s ratio and slope angle of the annular membrane |
| , , | Thickness, outer radius and inner radius of annular membrane |
| , | Radial coordinate with dimensions and without dimensions |
| , , | Radial stress, circumferential stress and initial stress |
| , | Radial stress and circumferential stress without dimensions |
| , | Transversal displacement and radial displacement |
| , | Radial strain and circumferential strain |
| , | Transverse load and proportional coefficient |
| , | Transversal displacement and transverse load without dimensions |
| , , | Integration constants |
| , | at and at |
| 1.078759576642000 | 0.27020250784724 | |
| 1.460302799082030 | 1.06847431744777 | |
| −0.165260708778378 | −4.05406145381641 | |
| −0.000452908553254 | −0.00117630695707 | |
| 0.513183613662808 | 1.99562059107809 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.-X.; Sun, J.-Y.; Zhao, Z.-H.; Li, S.-Z.; He, X.-T. A Closed-Form Solution of Prestressed Annular Membrane Internally-Connected with Rigid Circular Plate and Transversely-Loaded by Central Shaft. Mathematics 2020, 8, 521. https://doi.org/10.3390/math8040521
Yang Z-X, Sun J-Y, Zhao Z-H, Li S-Z, He X-T. A Closed-Form Solution of Prestressed Annular Membrane Internally-Connected with Rigid Circular Plate and Transversely-Loaded by Central Shaft. Mathematics. 2020; 8(4):521. https://doi.org/10.3390/math8040521
Chicago/Turabian StyleYang, Zhi-Xin, Jun-Yi Sun, Zhi-Hang Zhao, Shou-Zhen Li, and Xiao-Ting He. 2020. "A Closed-Form Solution of Prestressed Annular Membrane Internally-Connected with Rigid Circular Plate and Transversely-Loaded by Central Shaft" Mathematics 8, no. 4: 521. https://doi.org/10.3390/math8040521
APA StyleYang, Z.-X., Sun, J.-Y., Zhao, Z.-H., Li, S.-Z., & He, X.-T. (2020). A Closed-Form Solution of Prestressed Annular Membrane Internally-Connected with Rigid Circular Plate and Transversely-Loaded by Central Shaft. Mathematics, 8(4), 521. https://doi.org/10.3390/math8040521





