Fractional Order Complexity Model of the Diffusion Signal Decay in MRI
Abstract
1. Introduction
“One of the principal objects of theoretical research in my department of knowledge is to find the point of view from which the subject appears in the greatest simplicity”. J. Willard Gibbs, Letter in Proc. Amer. Acad. Arts & Sci. (1881), pp. 420–421.
2. Definitions and Properties
2.1. Caputo Fractional Derivative
2.2. Mittag-Leffer Function
2.3. Exponential Function
2.4. One Parameter Mittag-Leffer Function
2.5. Two Parameter Mittag-Leffer Function
2.6. Three Parameter Mittag-Leffer Function
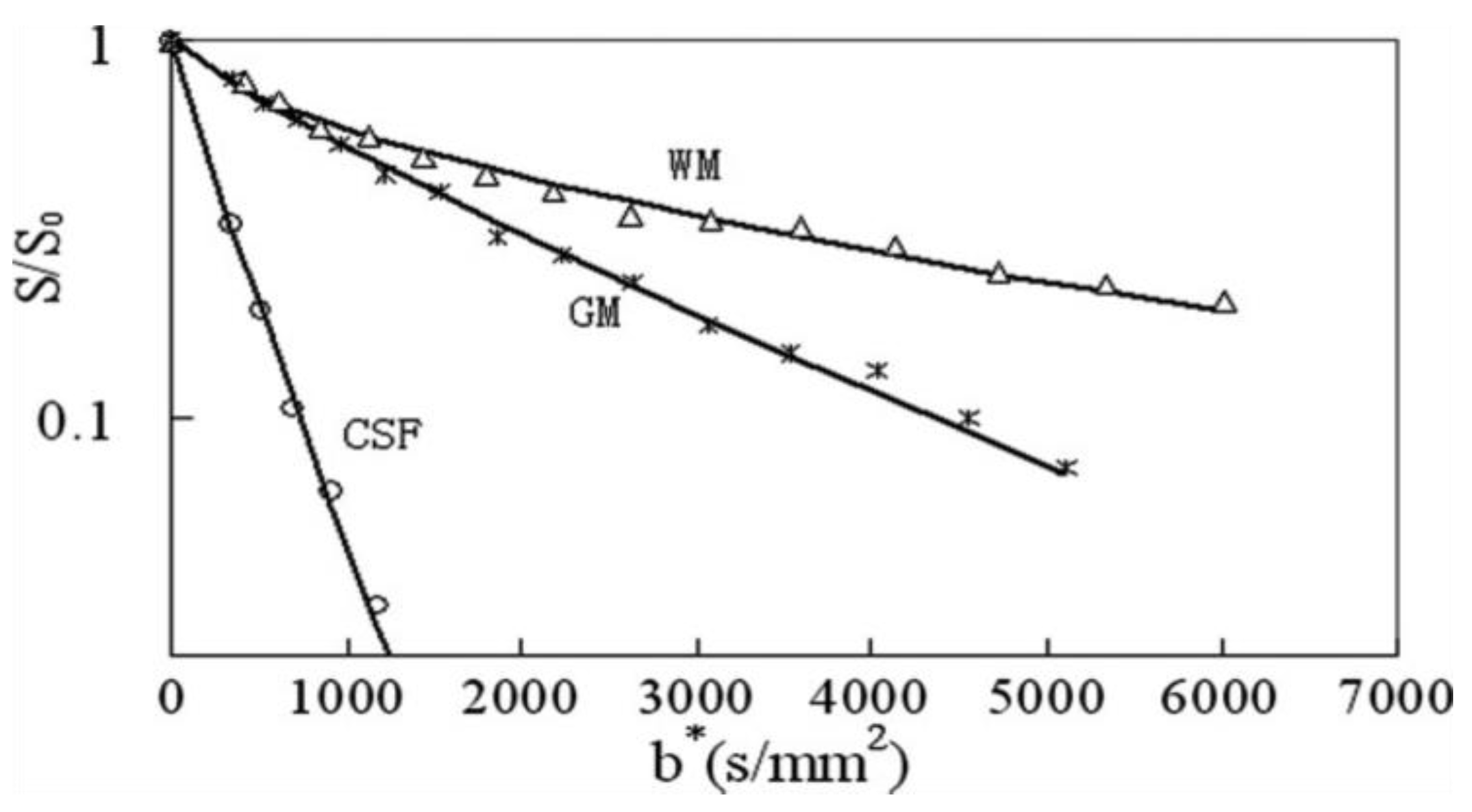
3. Results
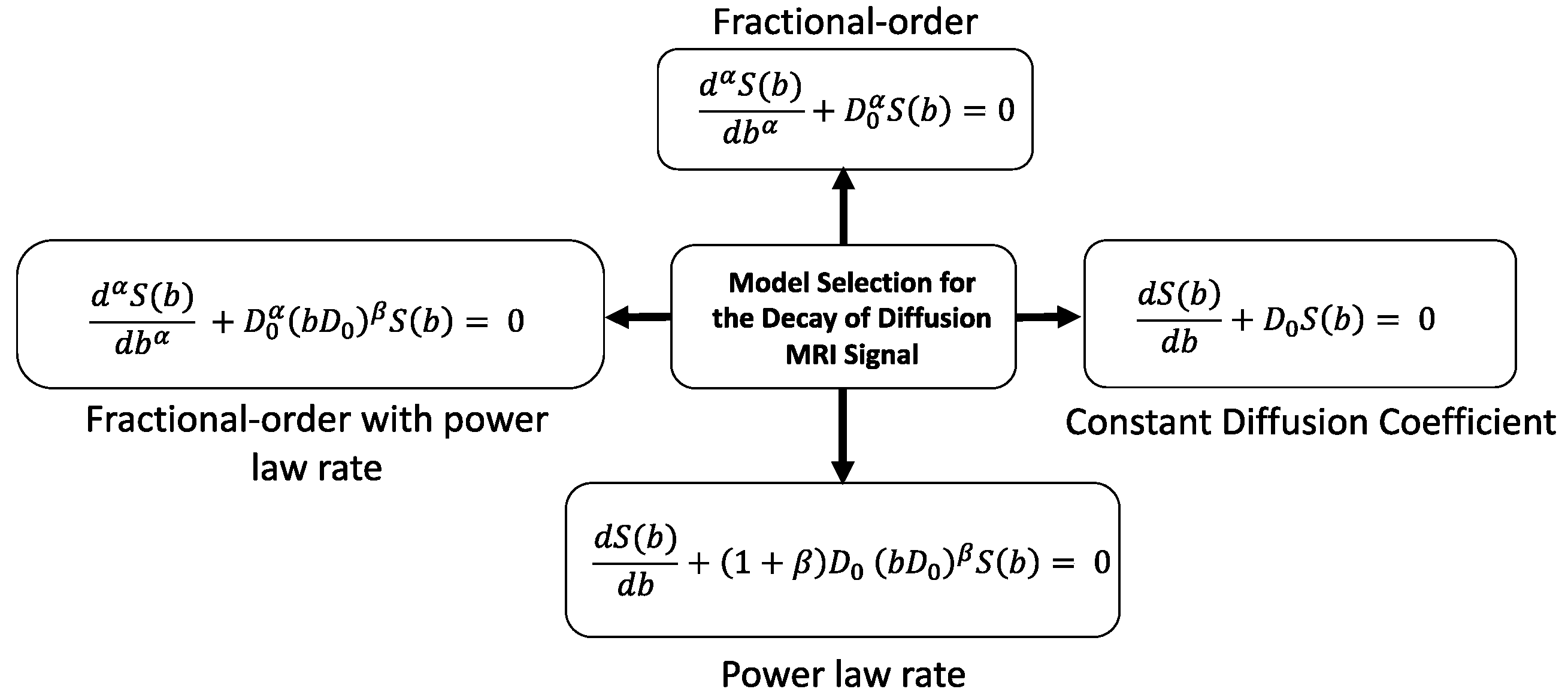
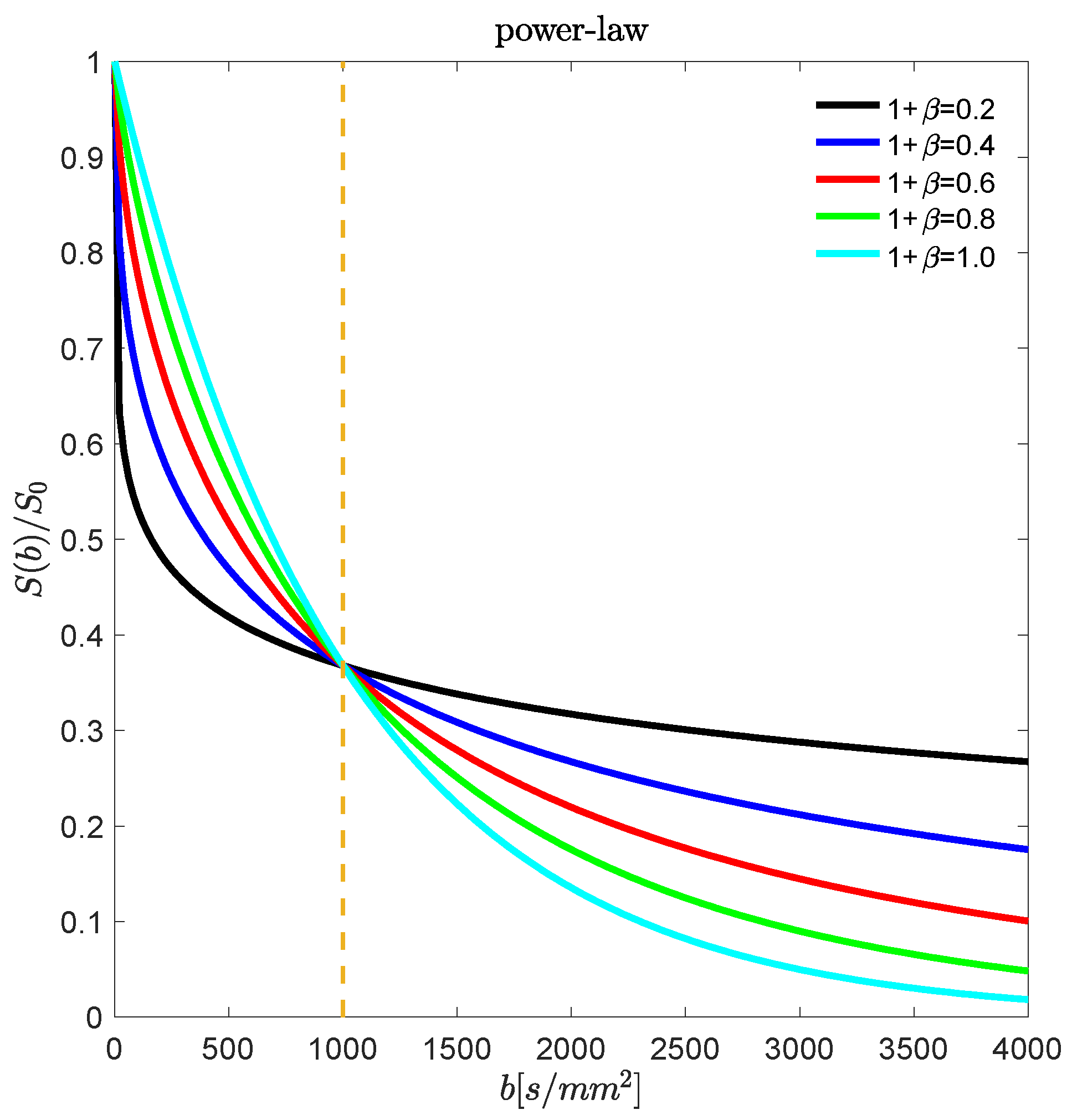
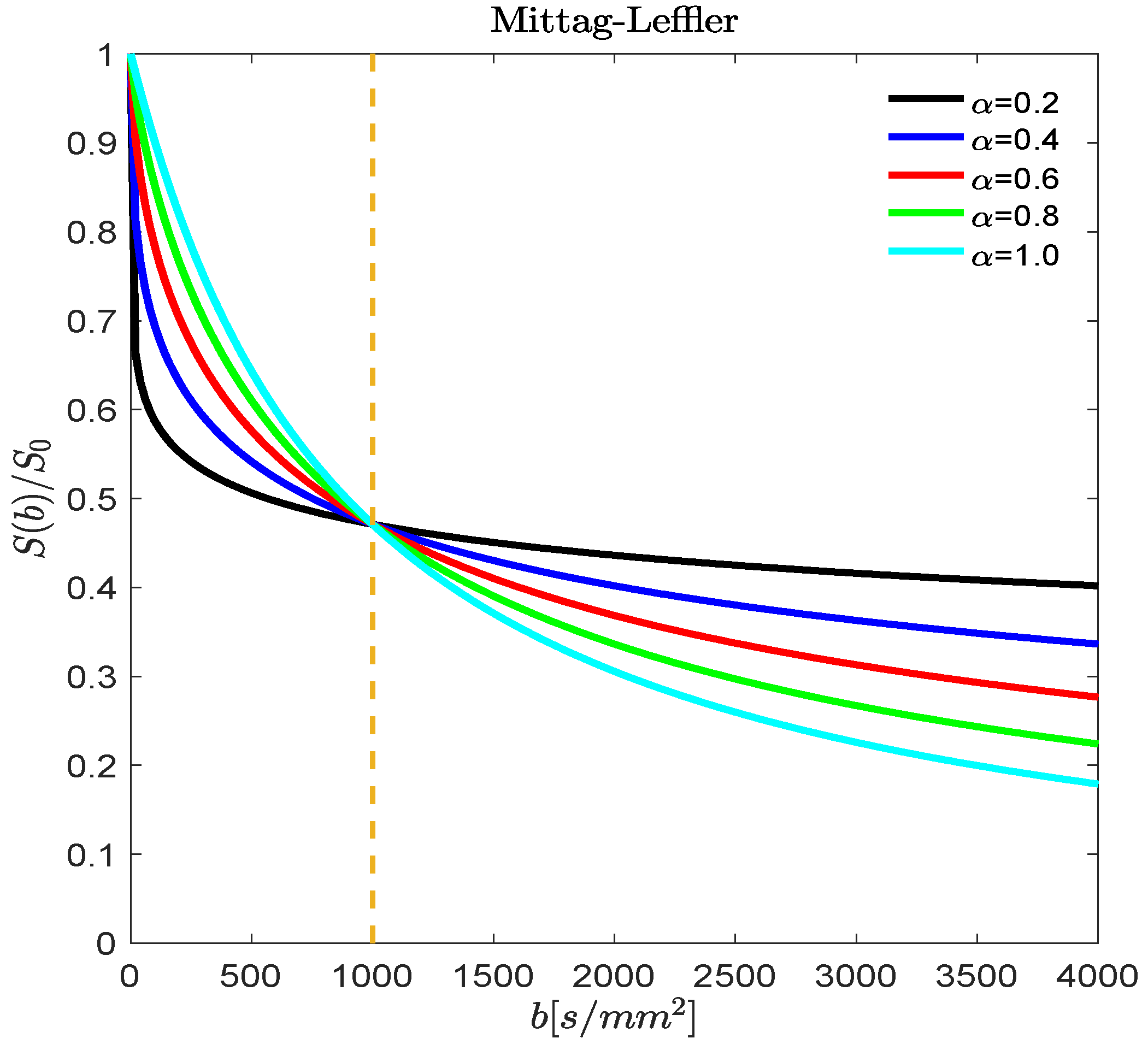
3.1. Fractional Order D(b) Models
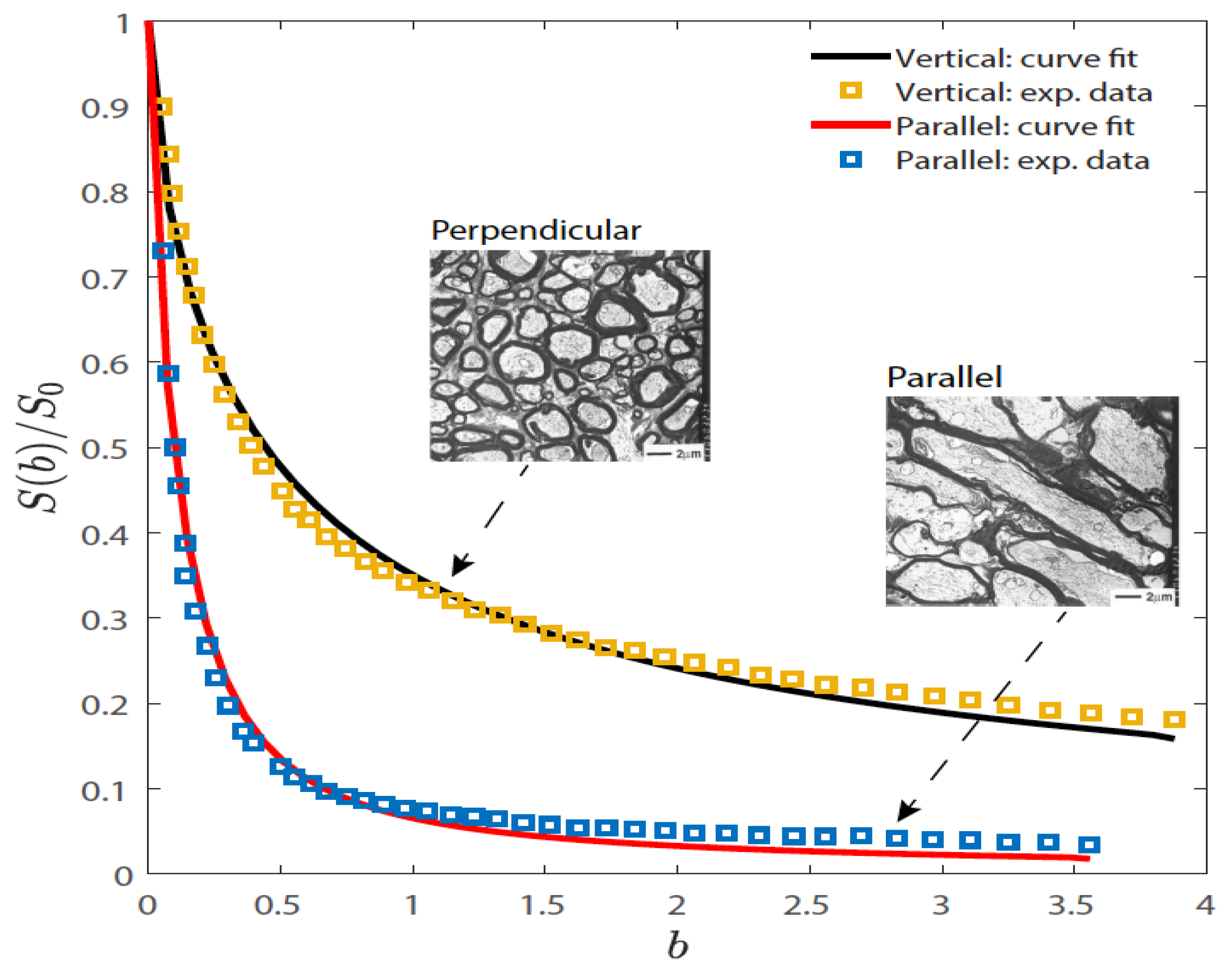
3.2. Fitting Fractional Order D(b) Models to DW-MRI Data
4. Discussion
“One cannot collect all the beautiful shells on the beach” Anne Morrow Lindbergh, Gift from the Sea, 1955.
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gershenfeld, N. The Nature of Mathematical Modeling; Cambridge University Press: Cambridge, UK, 1999; 344p. [Google Scholar]
- Haacke, E.M.; Brown, R.W.; Thompson, M.R.; Venkatesan, R. Magnetic Resonance Imaging: Physical Principles and Sequence Design; Wiley-Blackwell: New York, NY, USA, 1999; 914p. [Google Scholar]
- Jones, D.K. Diffusion MRI: Theory, Methods, and Applications; Oxford University Press: Oxford, UK, 2011; 748p. [Google Scholar]
- Callaghan, P.T. Translational Dynamics and Magnetic Resonance: Principles of Pulsed Gradient Spin Echo NMR; Oxford University Press: Oxford, UK, 2011; 568p. [Google Scholar]
- Stejskal, E.O.; Tanner, J.E. Spin diffusion measurements: Spin echoes in the presence of a time-dependent field gradient. J. Chem. Phys. 1965, 42, 288–292. [Google Scholar] [CrossRef]
- Liang, Z.-P.; Lauterbur, P.C. Principles of Magnetic Resonance Imaging: A Signal Processing Approach; Wiley—IEEE Press: Piscataway, NJ, USA, 1999; 416p. [Google Scholar]
- Kimmich, R. NMR: Tomography, Diffusometry, Relaxometry; Springer: New York, NY, USA, 1997; 526p. [Google Scholar]
- Bouchaud, J.-P.; Georges, A. Anomalous diffusion in disordered media: Statistical mechanisms, models and physical applications. Phys. Rep. 1990, 195, 127–293. [Google Scholar] [CrossRef]
- Höfling, F.; Franosch, T. Anomalous transport in the crowded world of biological cells. Rep. Prog. Phys. 2013, 76, 046602. [Google Scholar] [CrossRef] [PubMed]
- Mori, S.; Tournier, J.D. Introduction to Diffusion Tensor Imaging: And Higher Order Models; Academic Press: New York, NY, USA, 2013; 126p. [Google Scholar]
- Price, W.S. NMR Studies of Translational Motion: Principles and Applications; Cambridge University Press: Cambridge, UK, 2009; 393p. [Google Scholar]
- Magin, R.L.; Ingo, C.; Colon-Perez, L.; Triplett, W.; Mareci, T.H. Characterization of anomalous diffusion in porous biological tissues using fractional order derivatives and entropy. Microporous Mesoporous Mater. 2013, 178, 39–43. [Google Scholar] [CrossRef]
- Magin, R.L.; Abdullah, O.; Baleanu, D.; Zhou, X.J. Anomalous diffusion expressed through fractional order differential operators in the Bloch-Torrey equation. J. Magn. Reson. 2008, 190, 255–270. [Google Scholar] [CrossRef] [PubMed]
- Magin, R.L. Models of diffusion signal decay in magnetic resonance imaging: Capturing complexity. Concepts Magn. Reson. 2016, 45A, 21401. [Google Scholar] [CrossRef]
- Alexander, D.C.; Hubbard, P.L.; Hall, M.G.; Moore, E.A.; Ptito, M.; Parker, G.J.M.; Dyrby, T.B. Orientationally invariant indices of axon diameter and density from diffusion MRI. Neuroimage 2010, 52, 1374–1389. [Google Scholar] [CrossRef] [PubMed]
- Jensen, J.H.; Helpern, J.A.; Ramani, A.; Lu, H.; Kaczynski, K. Diffusional kurtosis imaging: The quantification of non-Gaussian water diffusion by means of magnetic resonance imaging. Magn. Reson. Med. 2005, 53, 1432–1440. [Google Scholar] [CrossRef]
- Bennett, K.M.; Schmaidna, K.M.; Bennett, R.; Rowe, D.B.; Lu, H.; Hyde, J.S. Characterization of continuously distributed cortical water diffusion rates with a stretched-exponential model. Magn. Reson. Med. 2003, 50, 727–734. [Google Scholar] [CrossRef]
- Jelescu, I.O.; Veraart, J.; Fieremans, E.; Novikov, D.S. Degeneracy in model parameter estimation for multi-compartmental diffusion in neuronal tissue. NMR Biomed. 2016, 29, 33–47. [Google Scholar] [CrossRef] [PubMed]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999; 368p. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F.; Rogosin, S.V. Mittag-Leffler Functions, Related Topics and Applications; Springer-Verlag: Berlin, Germany, 2014; 443p. [Google Scholar]
- Kilbas, A.A.; Saigo, M.; Saxena, R.K. Generalized Mittag-Leffler function and generalized fractional calculus operators. Integr. Trans. Spec. Funct. 2004, 15, 31–49. [Google Scholar] [CrossRef]
- de Oliveira, E.C.; Mainardi, F.; Vaz, J. Fractional models of anomalous relaxation based on the Kilbas and Saigo function. Meccanica 2014, 49, 2049–2060. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006; 523p. [Google Scholar]
- Hall, M.G.; Barrick, T.R. From diffusion-weighted MRI to anomalous diffusion imaging. Magn. Reson. Med. 2008, 59, 447–455. [Google Scholar] [CrossRef]
- Berberan-Santos, M.N. A luminescence decay function encompassing the stretched exponential and the compressed hyperbola. Chem. Phys. Lett. 2008, 460, 146–150. [Google Scholar] [CrossRef][Green Version]
- Ingo, C.; Magin, R.L.; Colon-Perez, L.; Triplett, W.; Mareci, T.H. On random walks and entropy in diffusion weighted magnetic resonance imaging studies of neural tissue. Magn. Reson. Med. 2014, 71, 617–627. [Google Scholar] [CrossRef]
- R.L.M. Kilbas and Saigo Function. Available online: https://www.mathworks.com/matlabcentral/fileexchange/70999-kilbas-and-saigo-function (accessed on 12 March 2018).
- Berberan-Santos, M.N.; Bodunov, E.N.; Valeur, B. Mathematical functions for the analysis of luminescence decays with underlying distributions: 1. Kohlrausch decay function (stretched exponential). Chem. Phys. 2005, 315, 171–182. [Google Scholar] [CrossRef]
- Berberan-Santos, M.N.; Valeur, B. Luminescence decays with underlying distributions: General properties and analysis with mathematical functions. J. Lumin. 2007, 126, 263–272. [Google Scholar] [CrossRef]
- Spanier, J.; Oldham, K.B. An Atlas of Functions; Hemisphere Publishing Corp: New York, NY, USA, 1987; pp. 253–262. [Google Scholar]
- Carpinteri, A.; Mainardi, F. Fractals and Fractional Calculus in Continuum Mechanics CISM Courses and Lectures No. 378; Springer-Wien: New York, NY, USA, 1997; 348p. [Google Scholar]
- Hilfer, R. (Ed.) Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2007; 472p. [Google Scholar]
- Berberan-Santos, M.N. Relation between the inverse Laplace transforms of I(tb) and I(t): Application to the Mittag-Leffler and asymptotic inverse power law relaxation functions. J. Math. Chem. 2005, 38, 265–270. [Google Scholar] [CrossRef]
- Hanyga, A.; Seredynska, M. Anisotropy in high-resolution diffusion-weighted MRI and anomalous diffusion. J. Magn. Reson. 2012, 220, 85–93. [Google Scholar] [CrossRef]
- Stanisz, G.J.; Szafer, A.; Wright, G.A.; Henkelman, R.M. An analytical model of restricted diffusion in bovine optic nerve. Magn. Reson. Med. 1997, 37, 103–111. [Google Scholar] [CrossRef]
- Zhou, X.J.; Gao, Q.; Abdullah, O.; Magin, R.L. Studies of anomalous diffusion in the human brain using fractional order calculus. Magn. Reson. Med. 2010, 63, 562–569. [Google Scholar] [CrossRef]
- Sui, Y.; He, W.; Damen, F.W.; Wanamaker, C.; Li, Y.; Zhou, X.J. Differentiation of low- and high-grade pediatric brain tumors with high b-value diffusion weighted MR imaging and a fractional order calculus model. Radiology 2015, 277, 489–496. [Google Scholar] [CrossRef]
- Karaman, M.M.; Sui, Y.; Wang, H.; Magin, R.L.; Li, Y.; Zhou, X.J. Differentiating low- and high-grade pediatric brain tumors using a continuous-time random-walk diffusion model at high b-values. Magn. Reson. Med. 2016, 76, 1149–1157. [Google Scholar] [CrossRef]
- Magin, R.L.; Karaman, M.M.; Hall, M.G.; Zhu, W.; Zhou, X.J. Capturing complexity of the diffusion-weighted MR signal decay. Magn. Reson. Imaging 2019, 56, 110–118. [Google Scholar] [CrossRef]
- Lin, G. Analysis of PFG anomalous diffusion via real-space and phase-space approaches. Mathematics 2018, 6, 17. [Google Scholar] [CrossRef]
- Lin, G. Describe NMR relaxation by anomalous rotational or translational diffusion. Commun. Nonlinear Sci. Numer. Simul. 2019, 72, 232–239. [Google Scholar] [CrossRef]
- Novikov, D.S.; Kiselev, V.G.; Jespersen, S.N. On modeling. Magn. Reson. Med. 2018, 79, 317–3193. [Google Scholar] [CrossRef]
- Grebenkov, D.S. From the microstructure to diffusion NMR, and back. In Diffusion NMR of Confined Systems: Fluid Transport in Porous Solids and Heterogeneous Materials; Valiullin, R., Ed.; The Royal Society of Chemistry: Cambridge, UK, 2017; pp. 52–110. [Google Scholar]
- Grinberg, F.; Farrher, F.; Shah, N.J. Diffusion magnetic resonance imaging in brain tissue. In Diffusion NMR of Confined Systems: Fluid Transport in Porous Solids and Heterogeneous Materials; Valiullin, R., Ed.; The Royal Society of Chemistry: Cambridge, UK, 2017; pp. 497–528. [Google Scholar]
- Topgaard, D. Multidimensional diffusion MRI. J. Magn. Reson. 2019, 275, 98–113. [Google Scholar] [CrossRef]
- Klages, R.; Radons, G.; Sokolov, I.M. (Eds.) Anomalous Transport: Foundations and Applications; Wiley-VCH: Weinheim, Germany, 2008; 583p. [Google Scholar]
- Evangelista, L.R.; Lenzi, E.K. Fractional Diffusion Equations and Anomalous Diffusion; Cambridge Univ. Press: Cambridge, UK, 2018; 345p. [Google Scholar]
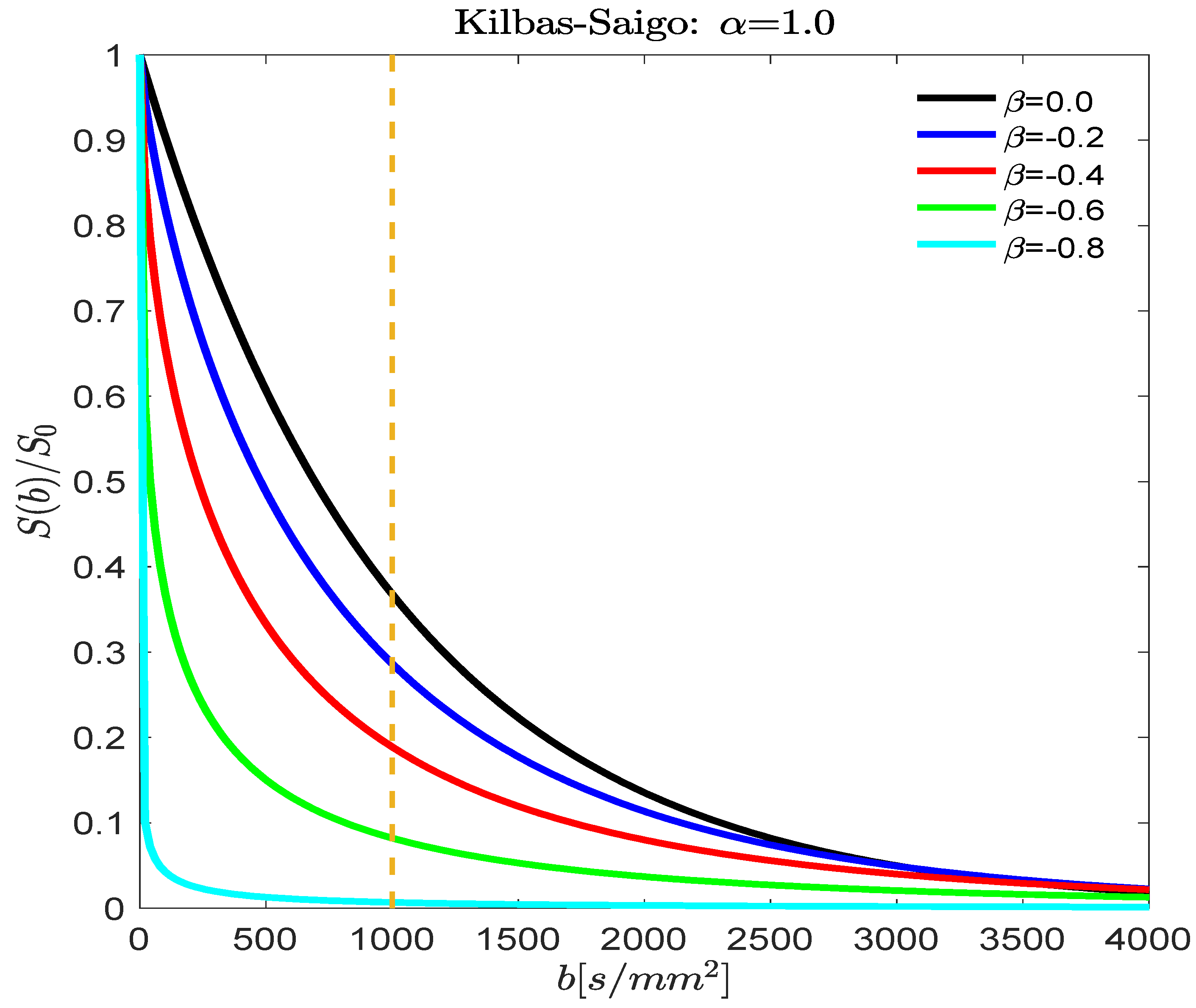
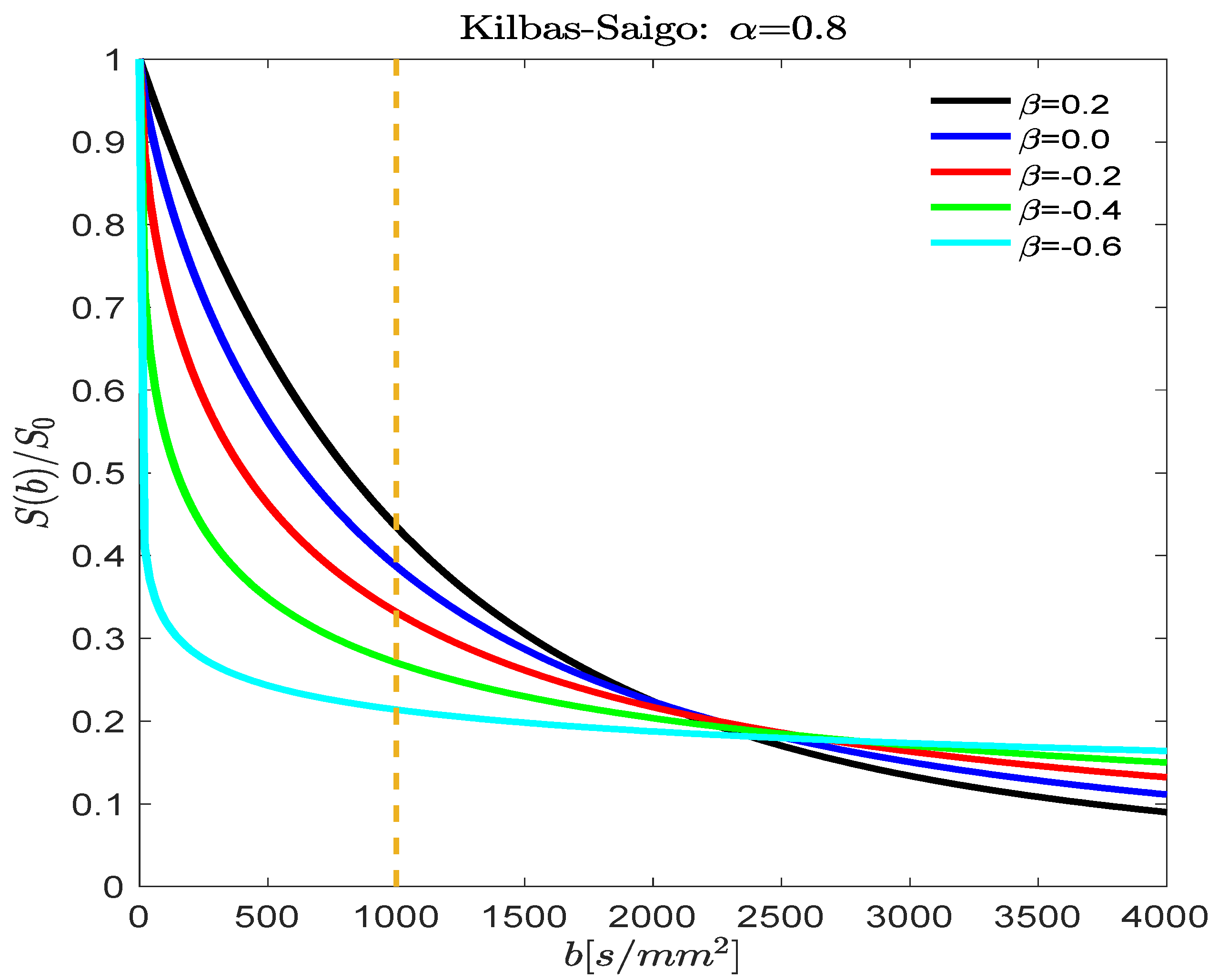
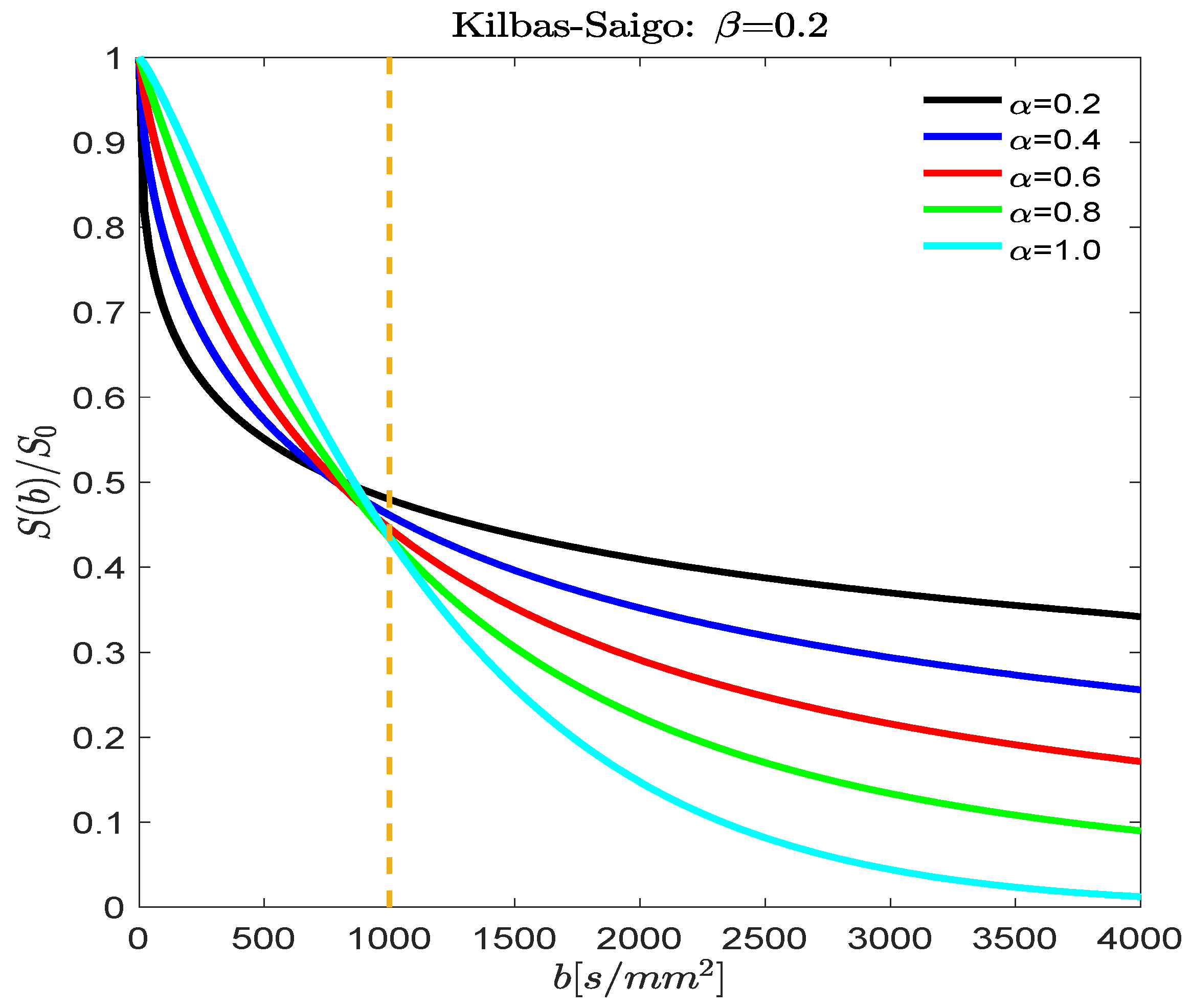
| Constant | Power Law |
|---|---|
| Mittag-Leffler | Kilbas-Saigo |
| Δ = 30 ms | Δ = 20 ms | Δ = 10 ms | Δ = 8 ms | ||
|---|---|---|---|---|---|
| Parallel | D × 104 mm2/s | 7.19 | 7.14 | 8.86 | 8.74 |
| Perpendicular | 1.95 | 2.05 | 2.72 | 2.75 | |
| Parallel | α | 0.76 | 0.75 | 0.64 | 0.74 |
| Perpendicular | 0.38 | 0.42 | 0.38 | 0.62 | |
| Parallel | β | 0.06 | 0.14 | 0.33 | 0.26 |
| Perpendicular | 0.31 | 0.27 | 0.46 | 0.20 | |
| Parallel | Mean-squared error | 5.84 × 10−4 | 5.09 × 10−4 | 3.47 × 10−4 | 1.64 × 10−4 |
| Perpendicular | 4.38 × 10−4 | 2.77 × 10−4 | 2.53 × 10−4 | 4.61 × 10−4 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Magin, R.L.; Karani, H.; Wang, S.; Liang, Y. Fractional Order Complexity Model of the Diffusion Signal Decay in MRI. Mathematics 2019, 7, 348. https://doi.org/10.3390/math7040348
Magin RL, Karani H, Wang S, Liang Y. Fractional Order Complexity Model of the Diffusion Signal Decay in MRI. Mathematics. 2019; 7(4):348. https://doi.org/10.3390/math7040348
Chicago/Turabian StyleMagin, Richard L., Hamid Karani, Shuhong Wang, and Yingjie Liang. 2019. "Fractional Order Complexity Model of the Diffusion Signal Decay in MRI" Mathematics 7, no. 4: 348. https://doi.org/10.3390/math7040348
APA StyleMagin, R. L., Karani, H., Wang, S., & Liang, Y. (2019). Fractional Order Complexity Model of the Diffusion Signal Decay in MRI. Mathematics, 7(4), 348. https://doi.org/10.3390/math7040348







