Analysis of General Humoral Immunity HIV Dynamics Model with HAART and Distributed Delays
Abstract
1. Introduction
2. Mathematical Model
2.1. Properties of Solutions
2.2. Equilibria
3. Global Stability
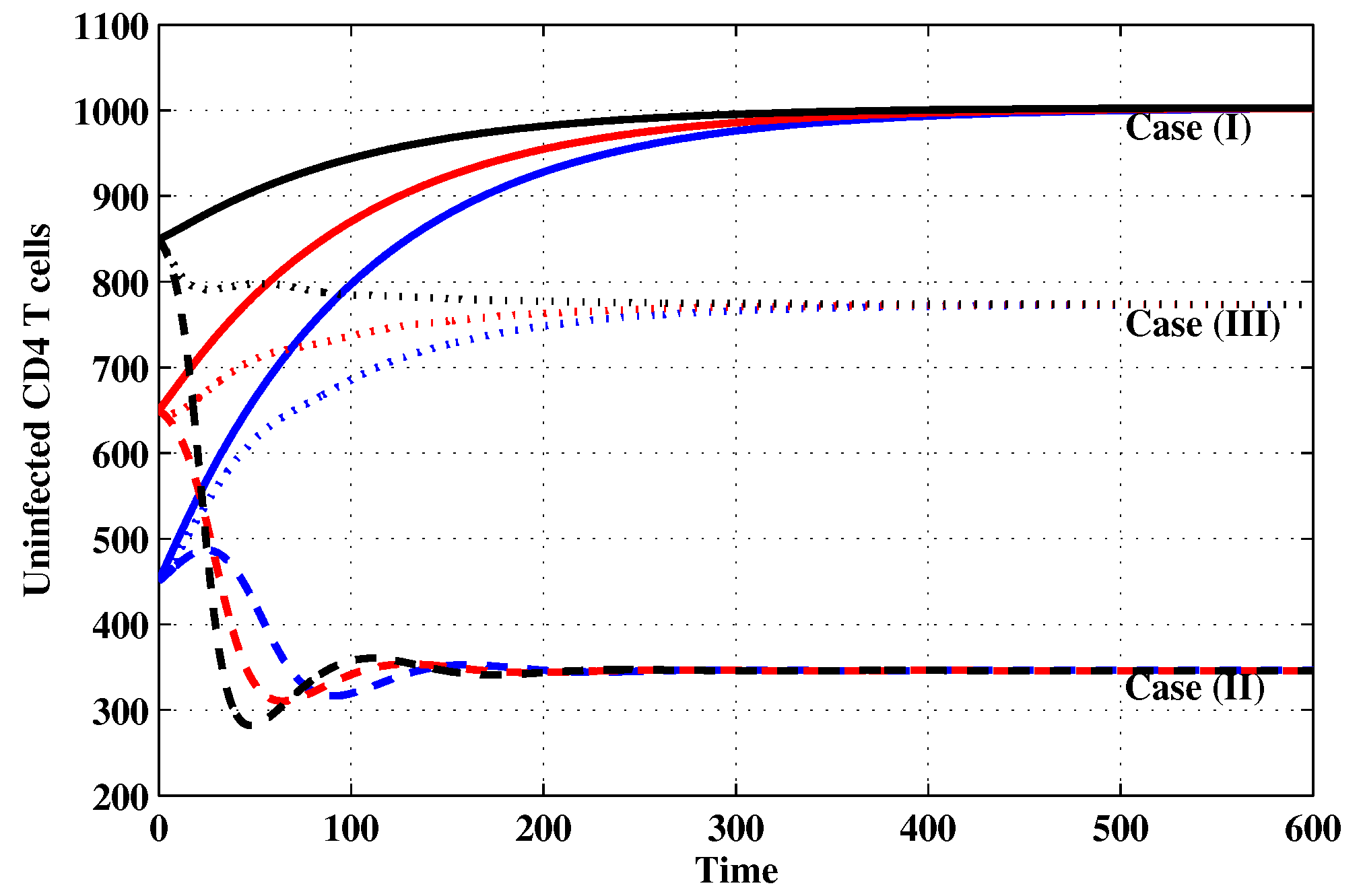
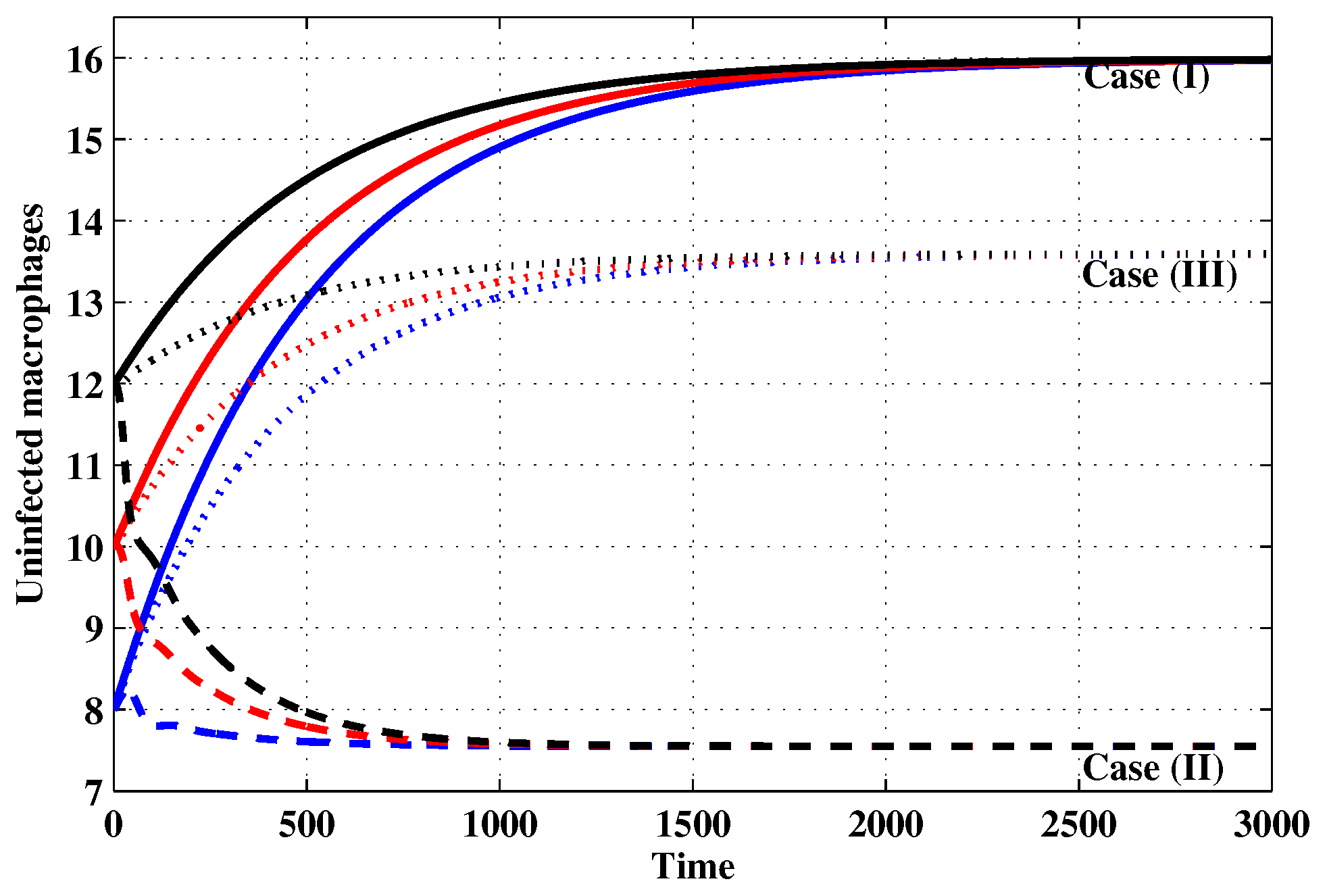
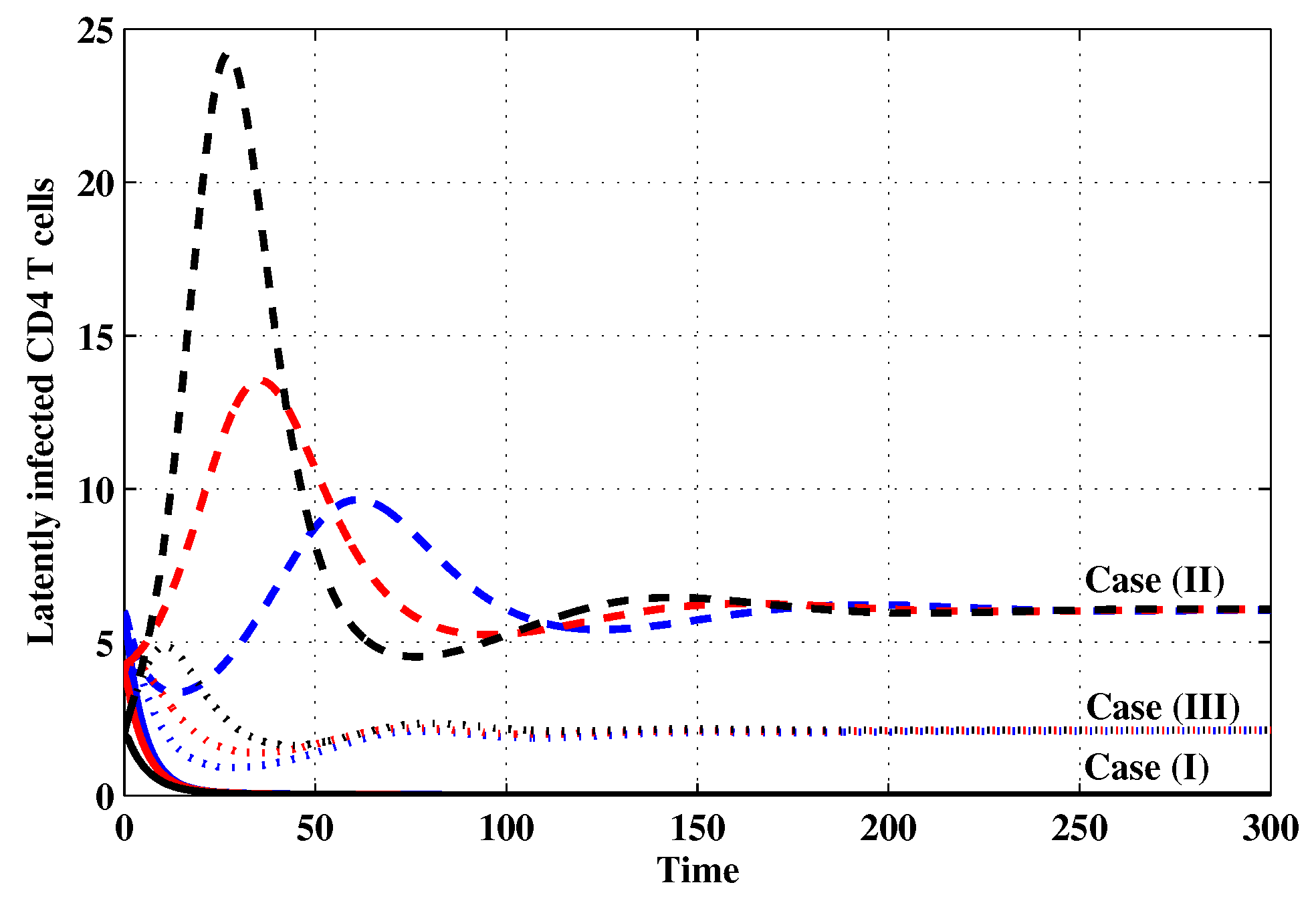
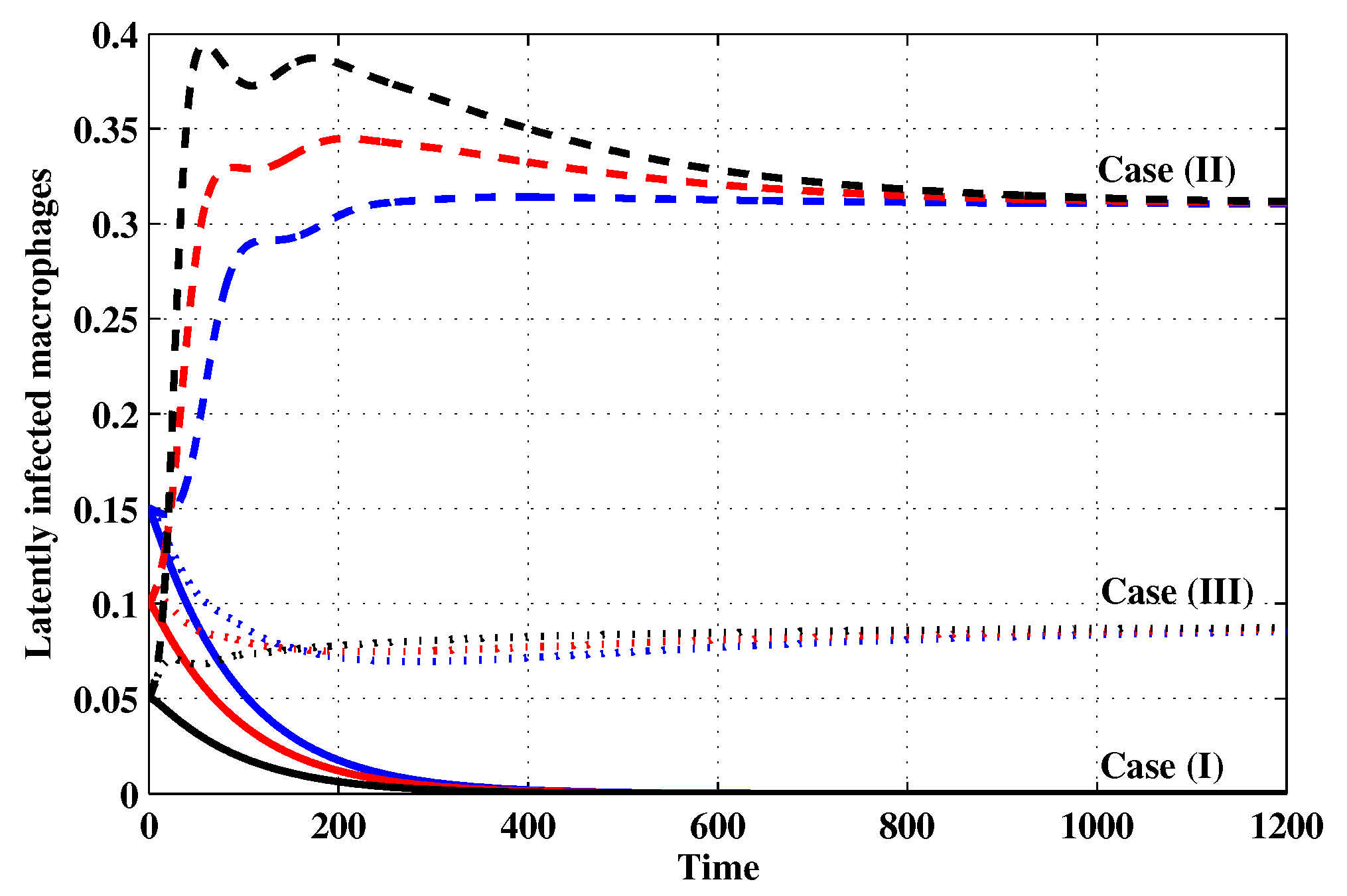
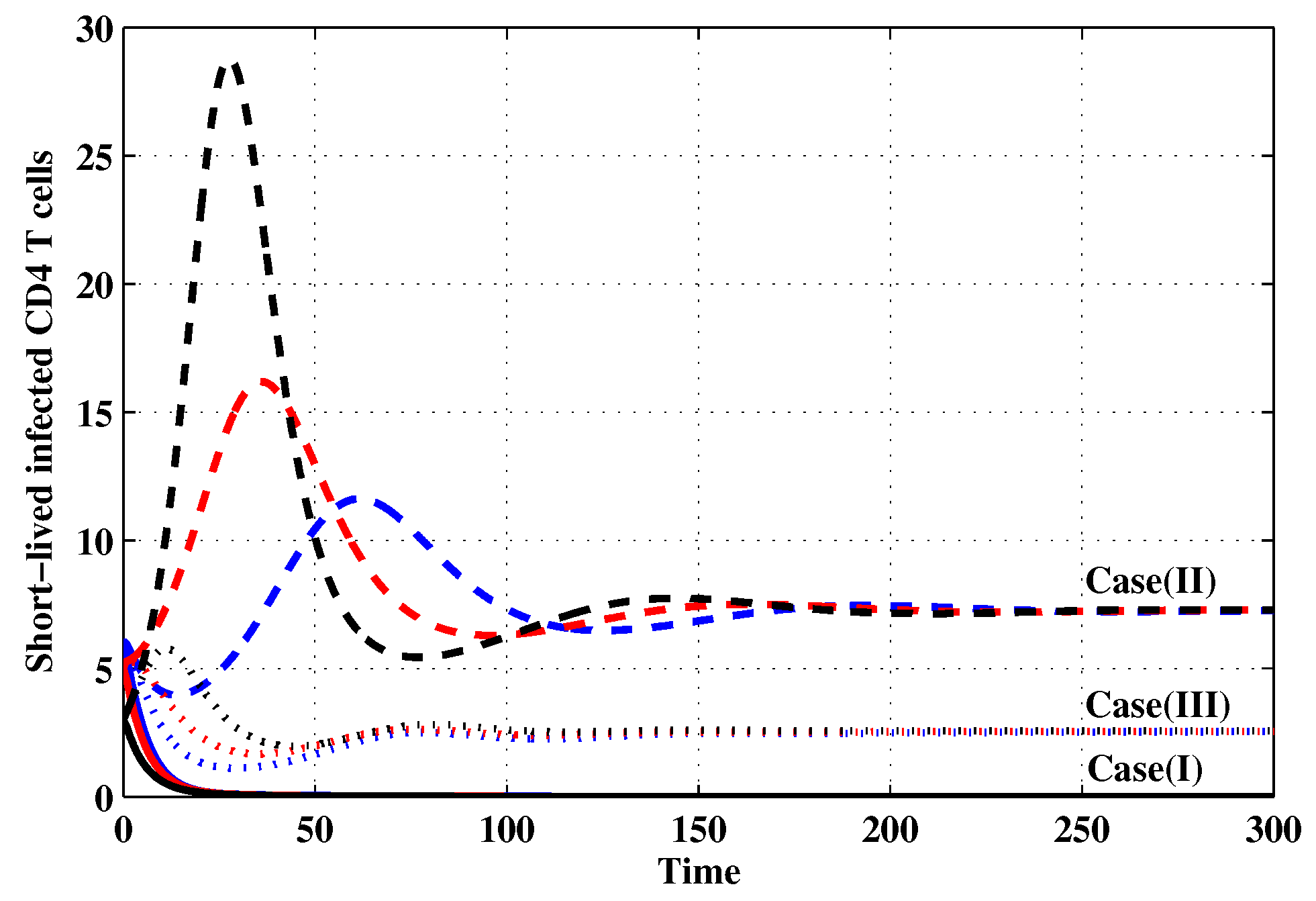
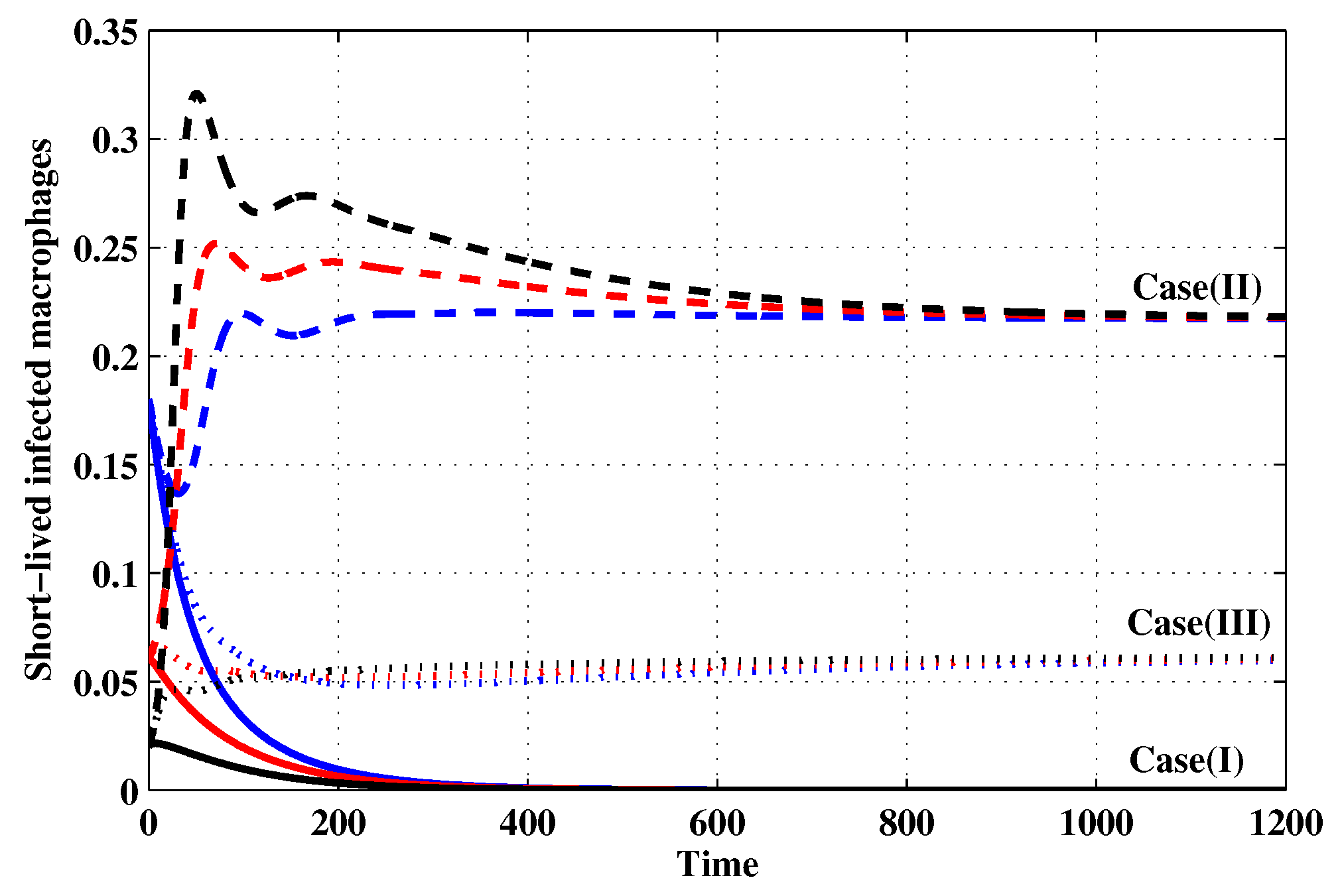
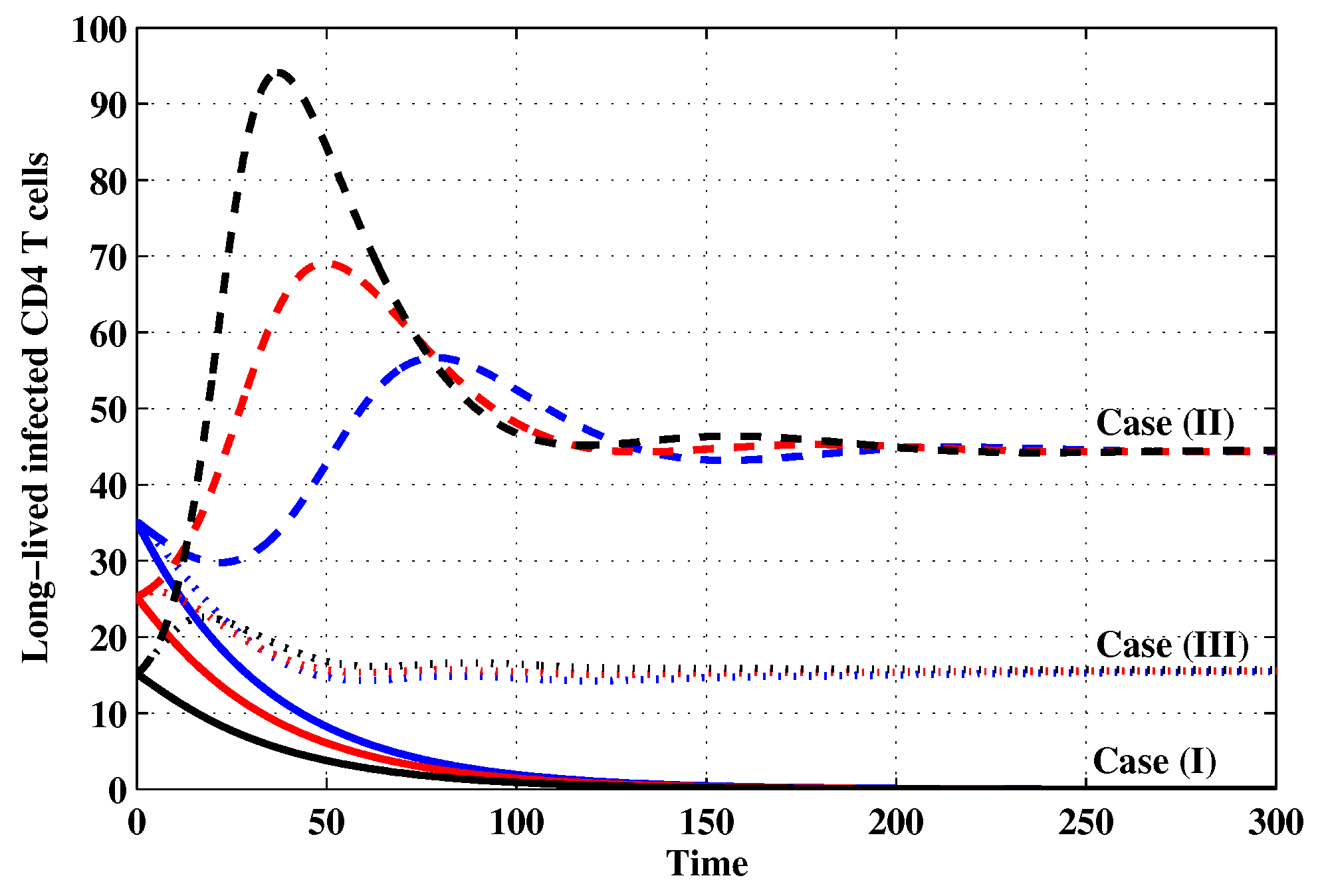
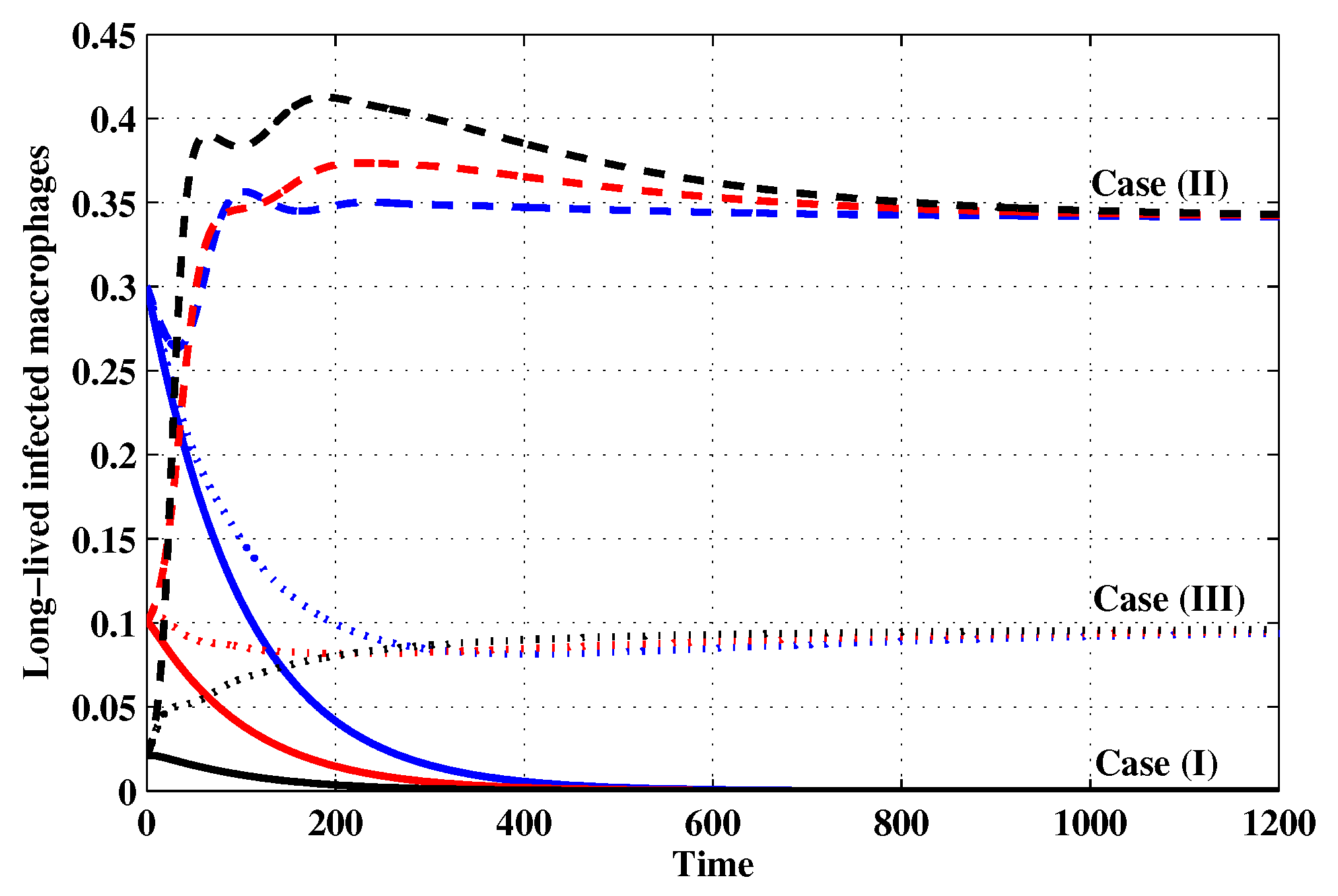
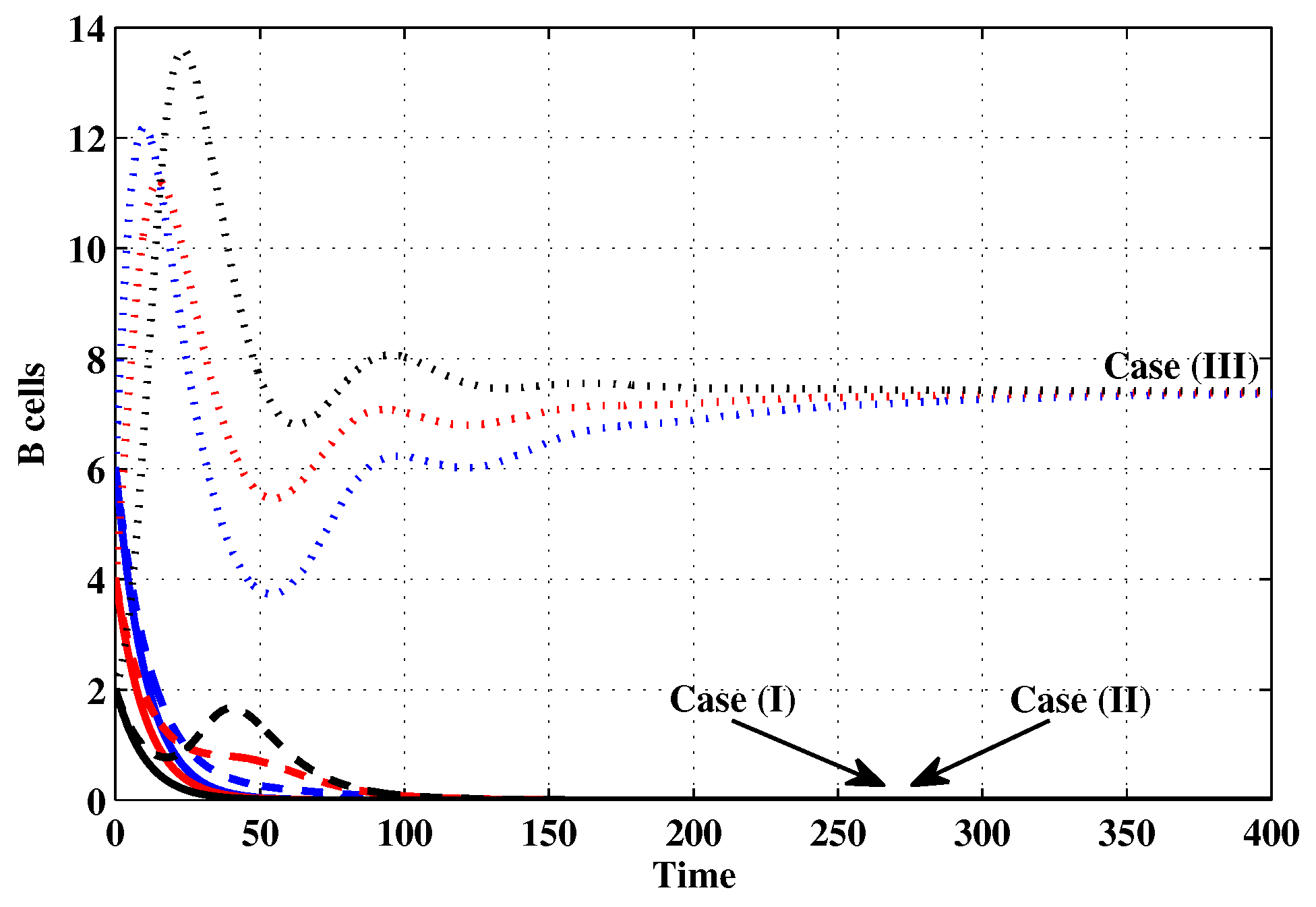
4. Numerical Simulations
4.1. Stability of the Equilibria of the System
- IC1:
- IC2:
- IC3:
Effect of the Drug Efficacy on the Stability of the System
- (i)
- if , then , exists and it is globally asymptotically stable,
- (ii)
- if , then , exists and it is globally asymptotically stable and
- (iii)
- if , then and is globally asymptotically stable.
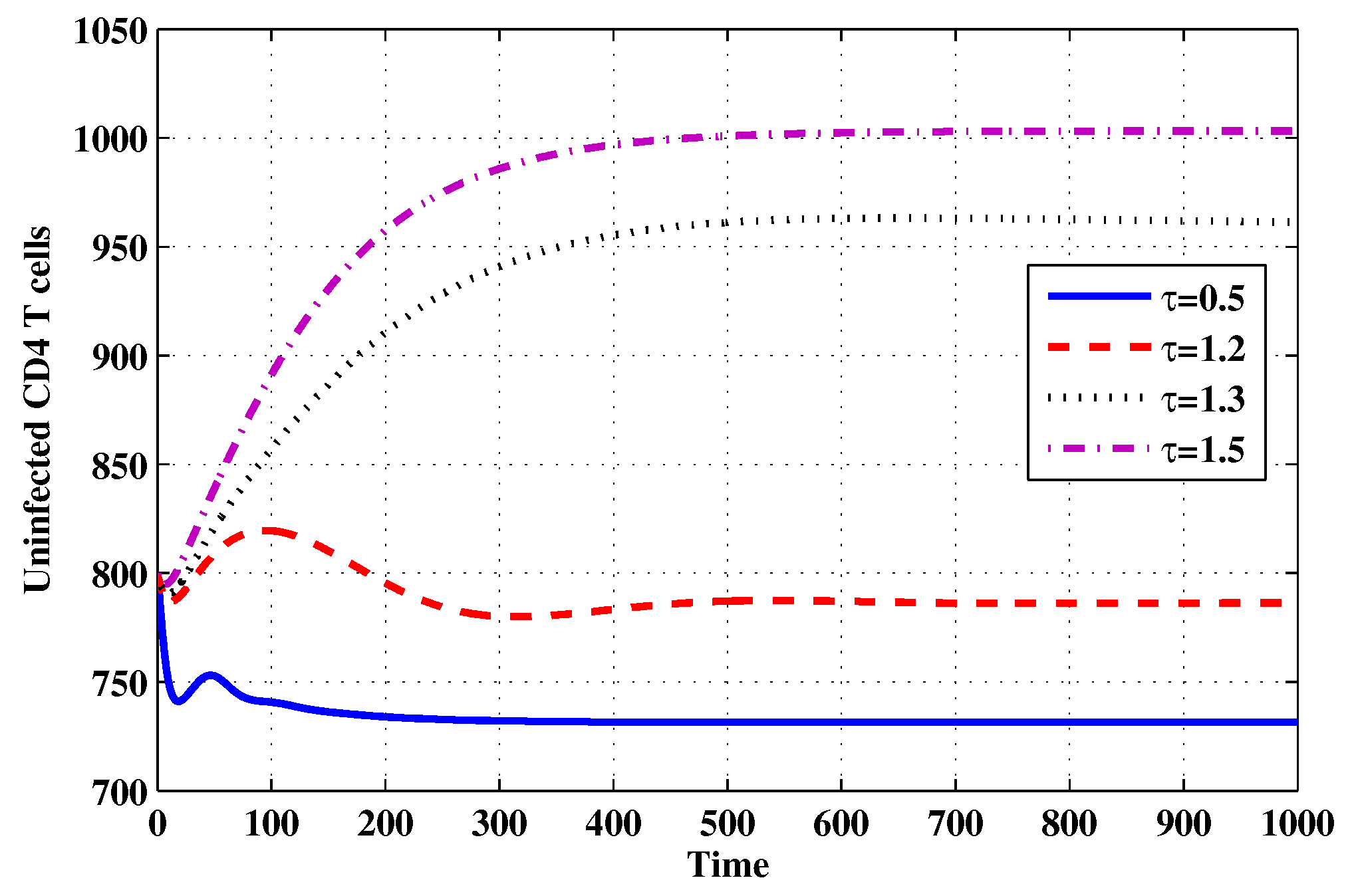
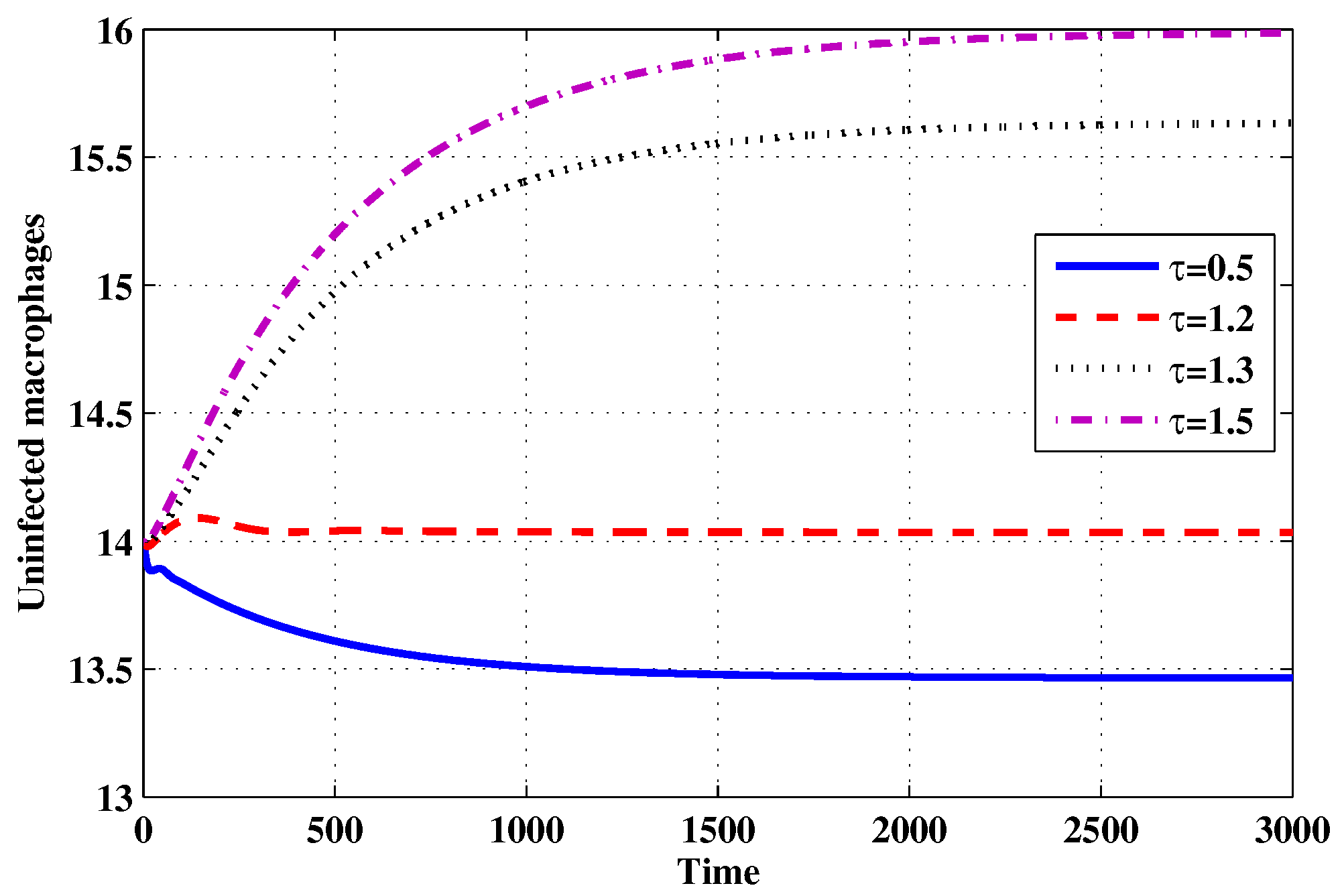
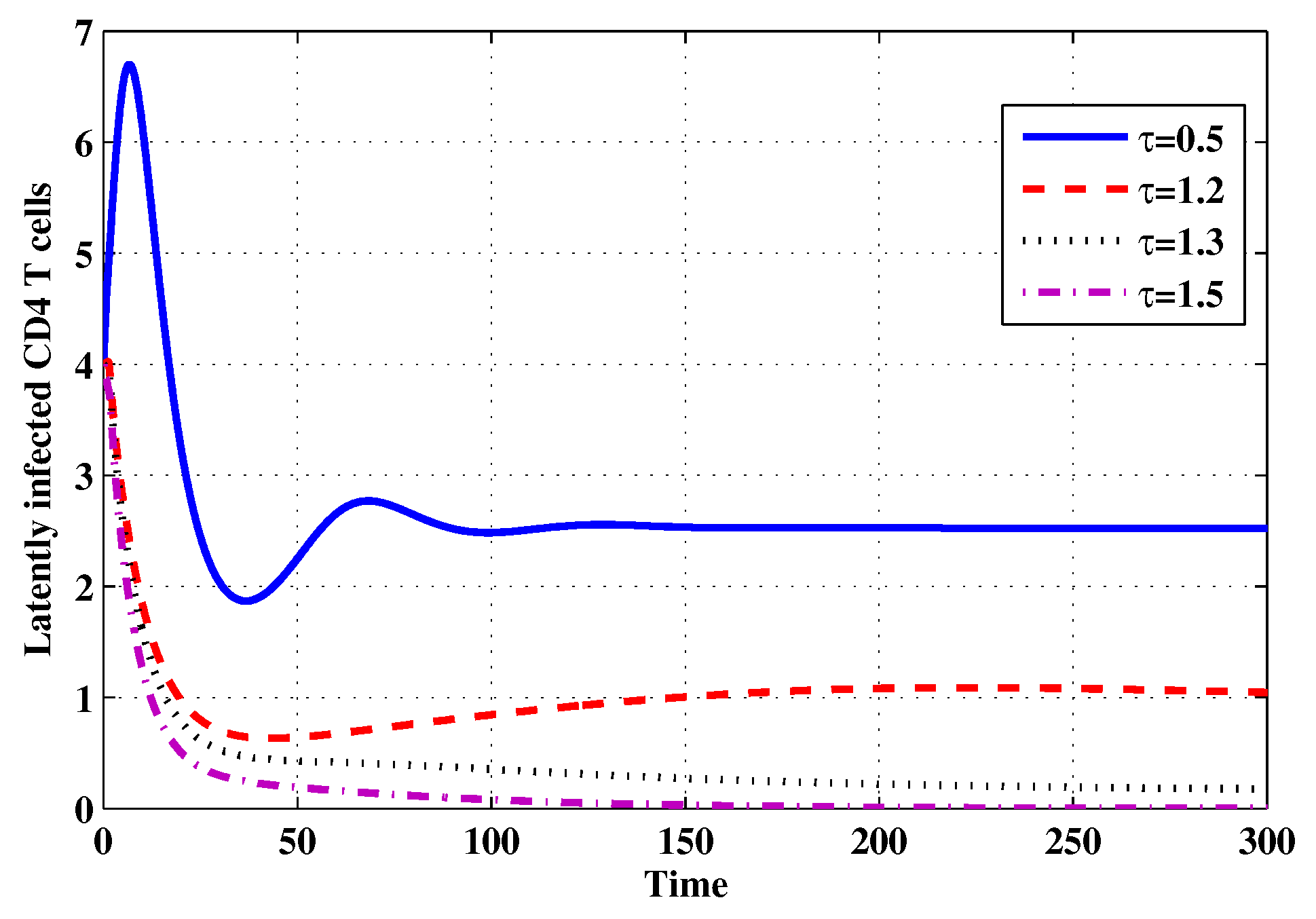
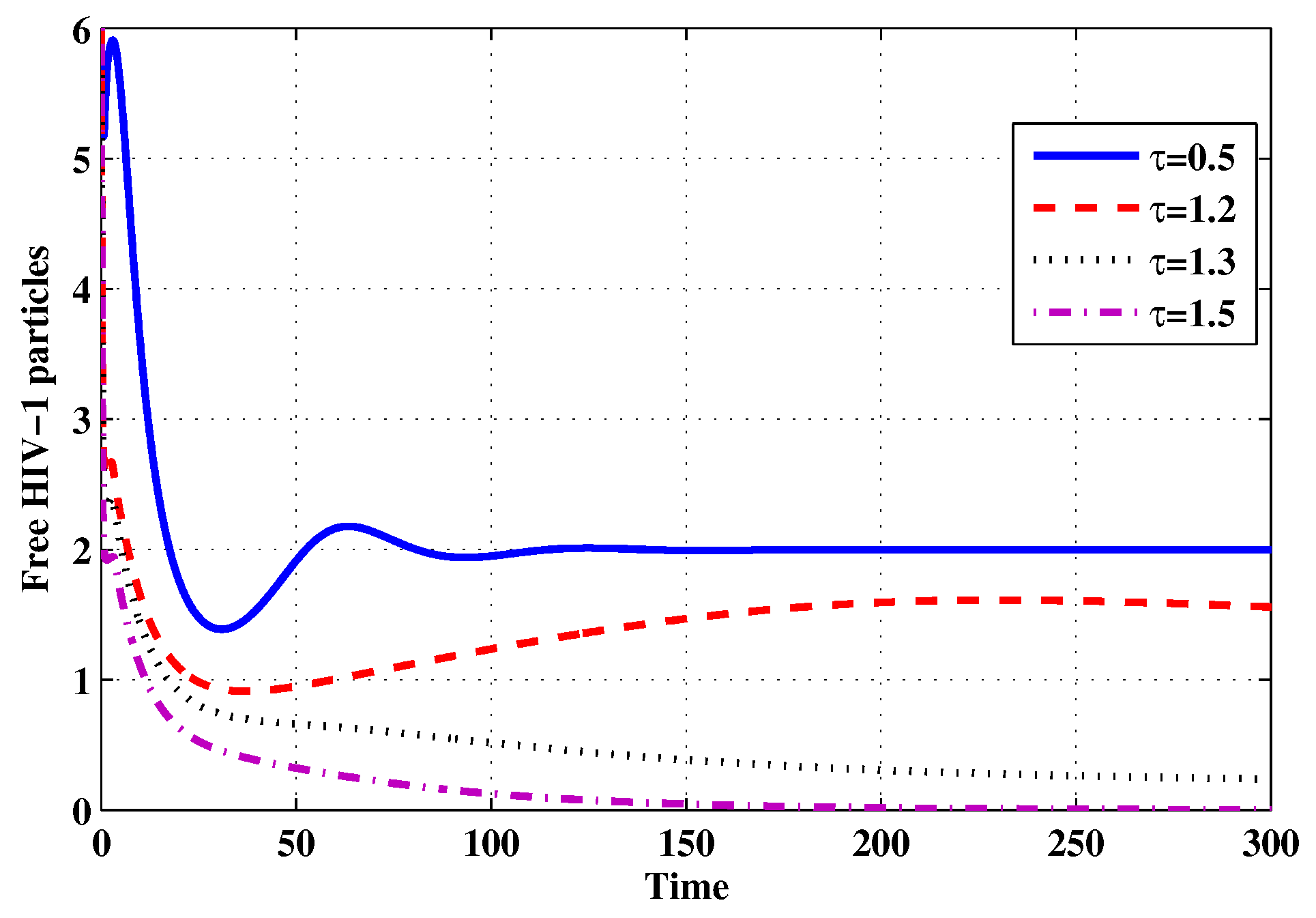
4.2. Effect of the Time Delay on the Stability of the System
5. Discussion
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Nowak, M.A.; Bangham, C.R.M. Population dynamics of immune responses to persistent viruses. Science 1996, 272, 74–79. [Google Scholar] [CrossRef] [PubMed]
- Culshaw, R.V.; Ruan, S. A delay-differential equation model of HIV infection of CD4 + T-cells. Math. Biosci. 2000, 165, 27–39. [Google Scholar] [CrossRef]
- Nelson, P.W.; Murray, J.D.; Perelson, A.S. A model of HIV-1 pathogenesis that includes an intracellular delay. Math. Biosci. 2000, 163, 201–215. [Google Scholar] [CrossRef]
- Culshaw, R.V.; Ruan, S.; Webb, G. A mathematical model of cell-to-cell spread of HIV-1 that includes a time delay. J. Math. Biol. 2003, 46, 425–444. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Li, M.Y. Mathematical analysis of the global dynamics of a model for HIV infection of CD4 + T cells. Math. Biosci. 2006, 200, 44–57. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.; Dimitrov, D.T.; Liu, H.; Kuang, Y. Mathematical insights in evaluating state dependent effectiveness of HIV prevention interventions. Bull. Math. Biol. 2013, 75, 649–675. [Google Scholar] [CrossRef]
- Huang, G.; Takeuchi, Y.; Ma, W. Lyapunov functionals for delay differential equations model of viral infections. SIAM J. Appl. Math. 2010, 70, 2693–2708. [Google Scholar] [CrossRef]
- Elaiw, A.M.; AlShamrani, N.H. Global properties of nonlinear humoral immunity viral infection models. Int. J. Biomath. 2015, 8, 1550058. [Google Scholar] [CrossRef]
- Elaiw, A.M.; AlShamrani, N.H.; Hattaf, K. Dynamical behaviors of a general humoral immunity viral infection model with distributed invasion and production. Int. J. Biomath. 2017, 10, 1750035. [Google Scholar] [CrossRef]
- Elaiw, A.M.; AlShamrani, N.H. Stability of a general delay-distributed virus dynamics model with multi-staged infected progression and immune response. Math. Methods Appl. Sci. 2017, 40, 699–719. [Google Scholar] [CrossRef]
- Hattaf, K.; Yousfi, N. A generalized virus dynamics model with cell-to-cell transmission and cure rate. Adv. Differ. Equat. 2016, 2016, 174. [Google Scholar] [CrossRef][Green Version]
- Wang, J.; Teng, Z.; Miao, H. Global dynamics for discrete-time analog of viral infection model with nonlinear incidence and CTL immune response. Adv. Differ. Equat. 2016, 2016, 143. [Google Scholar] [CrossRef]
- Blankson, J.N.; Persaud, D.; Siliciano, R.F. The challenge of viral reservoirs in HIV-1 infection. Ann. Rev. Med. 2002, 53, 557–593. [Google Scholar] [CrossRef] [PubMed]
- Elaiw, A.M.; Raezah, A.A. Stability of general virus dynamics models with both cellular and viral infections and delays. Math. Methods Appl. Sci. 2017, 40, 5863–5880. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Raezah, A.A.; Hattaf, K. Stability of HIV-1 infection with saturated virus-target and infected-target incidences and CTL immune response. Int. J. Biomath. 2017, 10, 1750070. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Raezah, A.A.; Alofi, B.S. Dynamics of delayed pathogen infection models with pathogenic and cellular infections and immune impairment. AIP Adv. 2018, 8, 025323. [Google Scholar] [CrossRef]
- Dutta, A.; Gupta, P.K. A mathematical model for transmission dynamics of HIV/AIDS with effect of weak CD4 + T cells. Chin. J. Phys. accepted. [CrossRef]
- Elaiw, A.M.; Almatrafi, A.A.; Hobiny, A.D. Effect of antibodies on pathogen dynamics with delays and two routes of infection. AIP Adv. 2018, 8, 065104. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Almatrafi, A.A.; Hobiny, A.D.; Abbas, I.A. Stability of latent pathogen infection model with CTL immune response and saturated cellular infection. AIP Adv. 2018, 8, 125021. [Google Scholar] [CrossRef]
- Li, M.Y.; Wang, L. Backward bifurcation in a mathematical model for HIV infection in vivo with anti-retroviral treatment. Nonlinear Anal. Real World Appl. 2014, 17, 147–160. [Google Scholar] [CrossRef]
- Li, B.; Chen, Y.; Lu, X.; Liu, S. A delayed HIV-1 model with virus waning term. Math. Biosci. Eng. 2016, 13, 135–157. [Google Scholar] [CrossRef] [PubMed]
- Pinto, C.M.A.; Carvalho, A.R.M. A latency fractional order model for HIV dynamics. J. Comput. Appl. Math. 2017, 312, 240–256. [Google Scholar] [CrossRef]
- Buonomo, B.; Vargas-De-Leon, C. Global stability for an HIV-1 infection model including an eclipse stage of infected cells. J. Math. Anal. Appl. 2012, 385, 709–720. [Google Scholar] [CrossRef]
- Elaiw, A.M.; AlShamrani, N.H. Global stability of humoral immunity virus dynamics models with nonlinear infection rate and removal. Nonlinear Anal. Real World Appl. 2015, 26, 161–190. [Google Scholar] [CrossRef]
- Elaiw, A.M.; AlShamrani, N.H. Stability of an adaptive immunity pathogen dynamics model with latency and multiple delays. Math. Methods Appl. Sci. 2018, 36, 125–142. [Google Scholar] [CrossRef]
- Korobeinikov, A. Global properties of basic virus dynamics models. Bull. Math. Biol. 2004, 66, 879–883. [Google Scholar] [CrossRef] [PubMed]
- Elaiw, A.M.; Elnahary, E.K.; Raezah, A.A. Effect of cellular reservoirs and delays on the global dynamics of HIV. Adv. Differ. Equat. 2018, 2018, 85. [Google Scholar] [CrossRef]
- Perelson, A.S.; Essunger, P.; Cao, Y.; Vesanen, M.; Hurley, A.; Saksela, K.; Markowitz, M.; Ho, D.D. Decay characteristics of HIV-1-infected compartments during combination therapy. Nature 1997, 387, 188–191. [Google Scholar] [CrossRef]
- Callaway, D.S.; Perelson, A.S. HIV-1 infection and low steady state viral loads. Bull. Math. Biol. 2002, 64, 29–64. [Google Scholar] [CrossRef]
- Elaiw, A.M. Global properties of a class of HIV models. Nonlinear Anal. Real World Appl. 2010, 11, 2253–2263. [Google Scholar] [CrossRef]
- Elaiw, A.M. Global properties of a class of virus infection models with multitarget cells. Nonlinear Dyn. 2012, 69, 423–435. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Almuallem, N.A. Global dynamics of delay-distributed HIV infection models with differential drug efficacy in cocirculating target cells. Math. Methods Appl. Sci. 2016, 39, 4–31. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, X. Global properties and bifurcation analysis of an HIV-1 infection model with two target cells. Comput. Appl. Math. 2018, 37, 3455–3472. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Hassanien, I.A.; Azoz, S.A. Global stability of HIV infection models with intracellular delays. J. Korean Math. Soc. 2012, 49, 779–794. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Azoz, S.A. Global properties of a class of HIV infection models with Beddington-DeAngelis functional response. Math. Methods Appl. Sci. 2013, 36, 383–394. [Google Scholar] [CrossRef]
- Elaiw, A.M. Global dynamics of an HIV infection model with two classes of target cells and distributed delays. Discret. Dyn. Nat. Soc. 2012, 2012, 253703. [Google Scholar] [CrossRef]
- Elaiw, A.M.; Raezah, A.A.; Azoz, S.A. Stability of delayed HIV dynamics models with two latent reservoirs and immune impairment. Adv. Differ. Equat. 2018, 2018, 414. [Google Scholar] [CrossRef]
- Hale, J.K.; Verduyn Lunel, S. Introduction to Functional Differential Equations; Springer: New York, NY, USA, 1993. [Google Scholar]
- Huang, Y.; Rosenkranz, S.L.; Wu, H. Modeling HIV dynamic and antiviral response with consideration of time-varying drug exposures, adherence and phenotypic sensitivity. Math. Biosci. 2003, 184, 165–186. [Google Scholar] [CrossRef]
- Yang, X.; Chen, S.L.; Chen, J.F. Permanence and positive periodic solution for the single-species nonautonomous delay diffusive models. Comput. Math. Appl. 1996, 32, 109–116. [Google Scholar] [CrossRef]


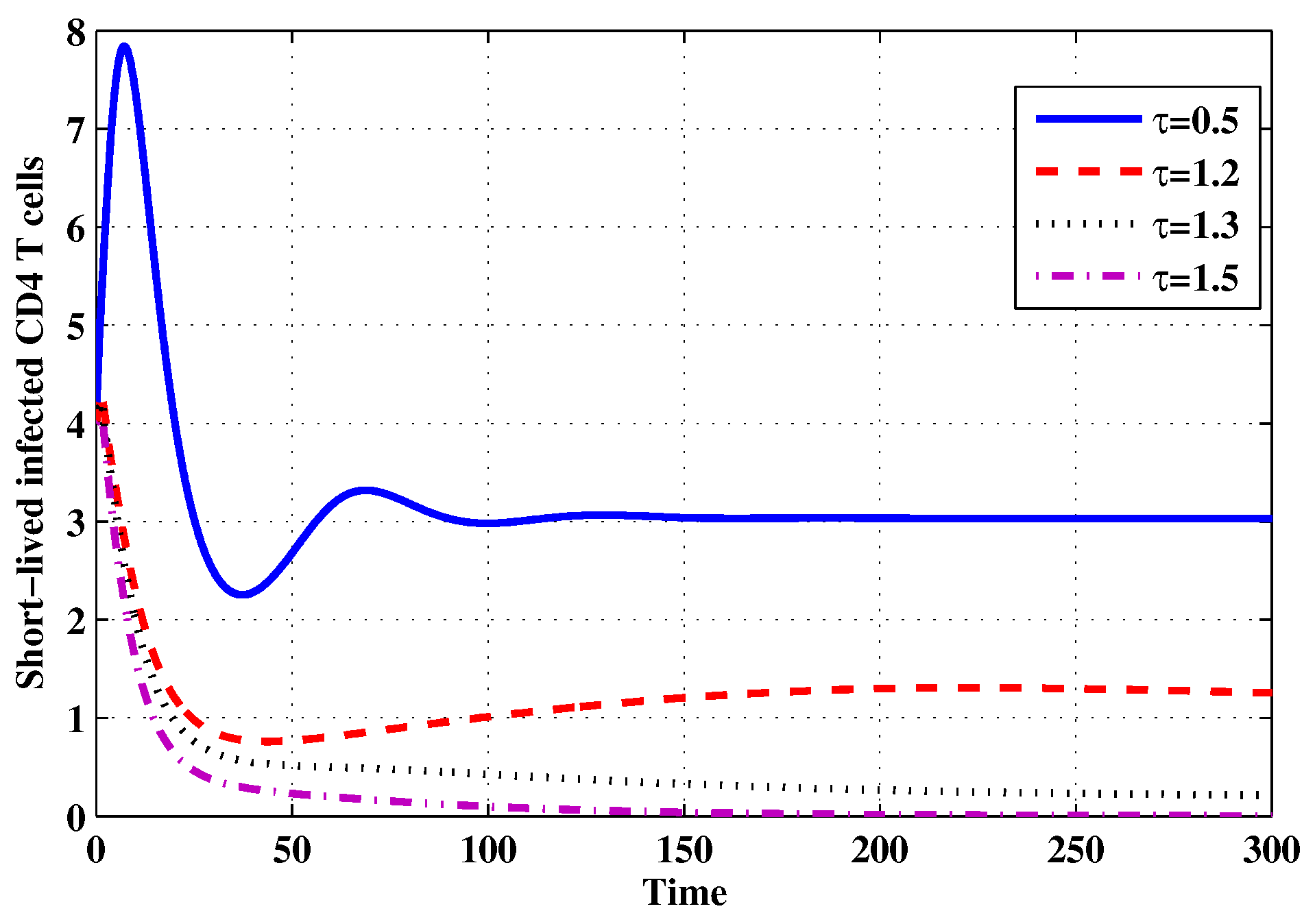
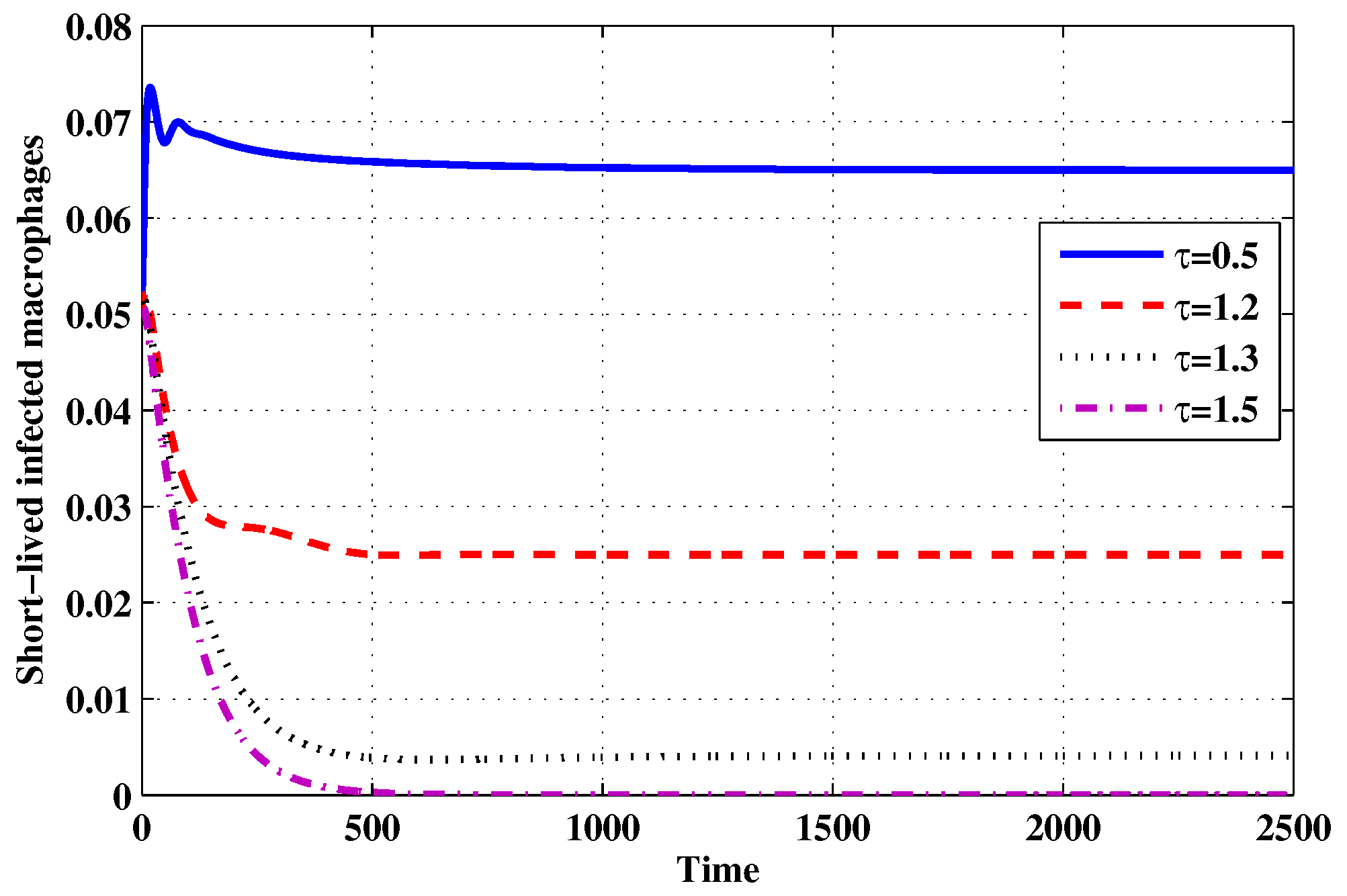
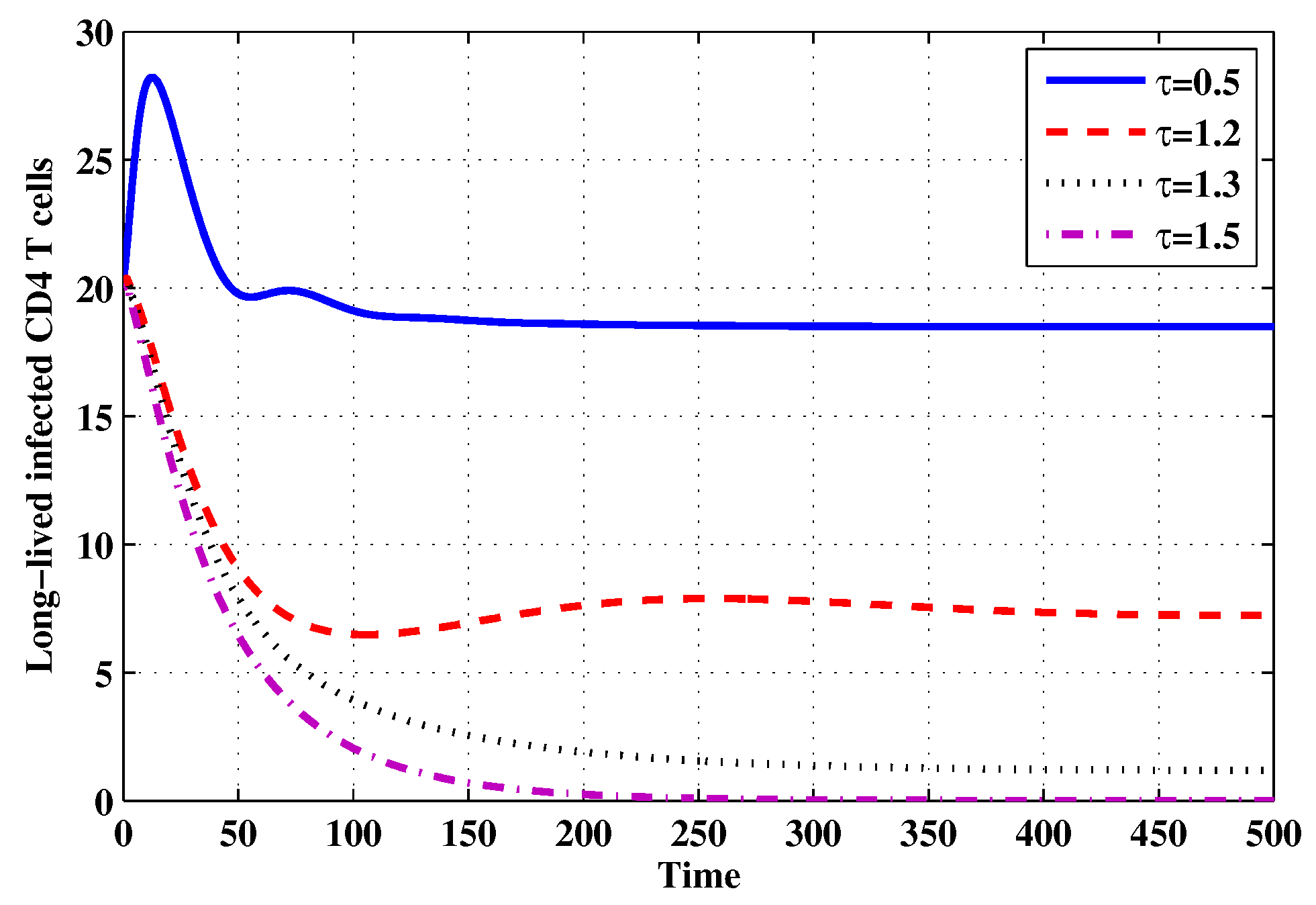
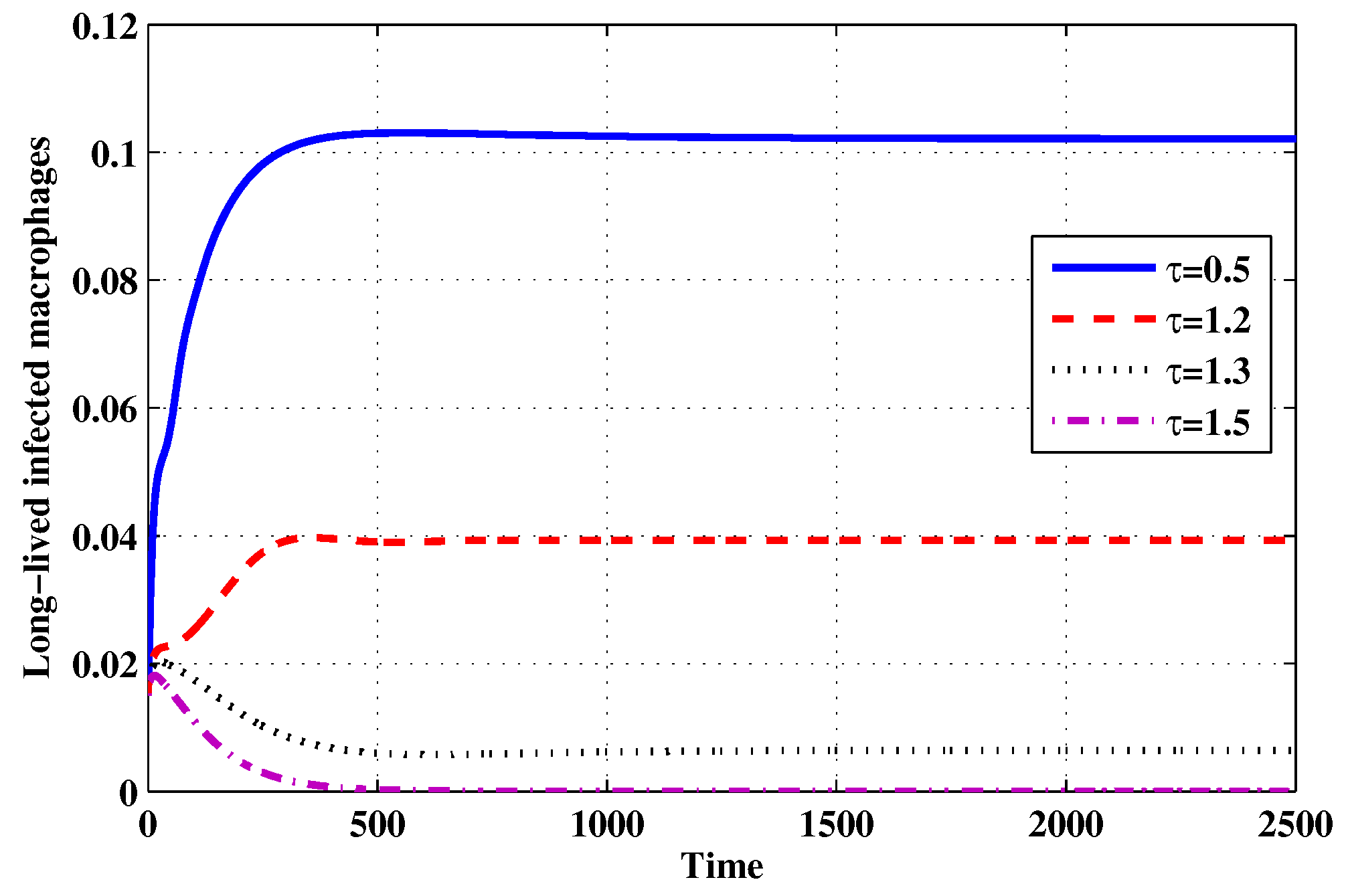

| Parameter | Value | Parameter | Value | Parameter | Value | Parameter | Value |
|---|---|---|---|---|---|---|---|
| 10 | |||||||
| B | 1200 | g | 3 | ||||
| 1 | |||||||
| f | h | ||||||
| 30 | 5 | 10 | 2 | ||||
| varied | varied | r | varied | varied | |||
| - | - | - | - |
| Equilibria | |||
|---|---|---|---|
| 0 | |||
| 1 | |||
| 1 | |||
| Equilibria | |||
|---|---|---|---|
| 2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elaiw, A.M.; Elnahary, E.K. Analysis of General Humoral Immunity HIV Dynamics Model with HAART and Distributed Delays. Mathematics 2019, 7, 157. https://doi.org/10.3390/math7020157
Elaiw AM, Elnahary EK. Analysis of General Humoral Immunity HIV Dynamics Model with HAART and Distributed Delays. Mathematics. 2019; 7(2):157. https://doi.org/10.3390/math7020157
Chicago/Turabian StyleElaiw, A. M., and E. Kh. Elnahary. 2019. "Analysis of General Humoral Immunity HIV Dynamics Model with HAART and Distributed Delays" Mathematics 7, no. 2: 157. https://doi.org/10.3390/math7020157
APA StyleElaiw, A. M., & Elnahary, E. K. (2019). Analysis of General Humoral Immunity HIV Dynamics Model with HAART and Distributed Delays. Mathematics, 7(2), 157. https://doi.org/10.3390/math7020157




