Numerical Analysis of Thermal Non-Equilibrium in Porous Medium Subjected to Internal Heating
Abstract
1. Introduction
2. Analysis
- Fluid does not change the phase.
- Domain properties are isotropic and homogeneous.
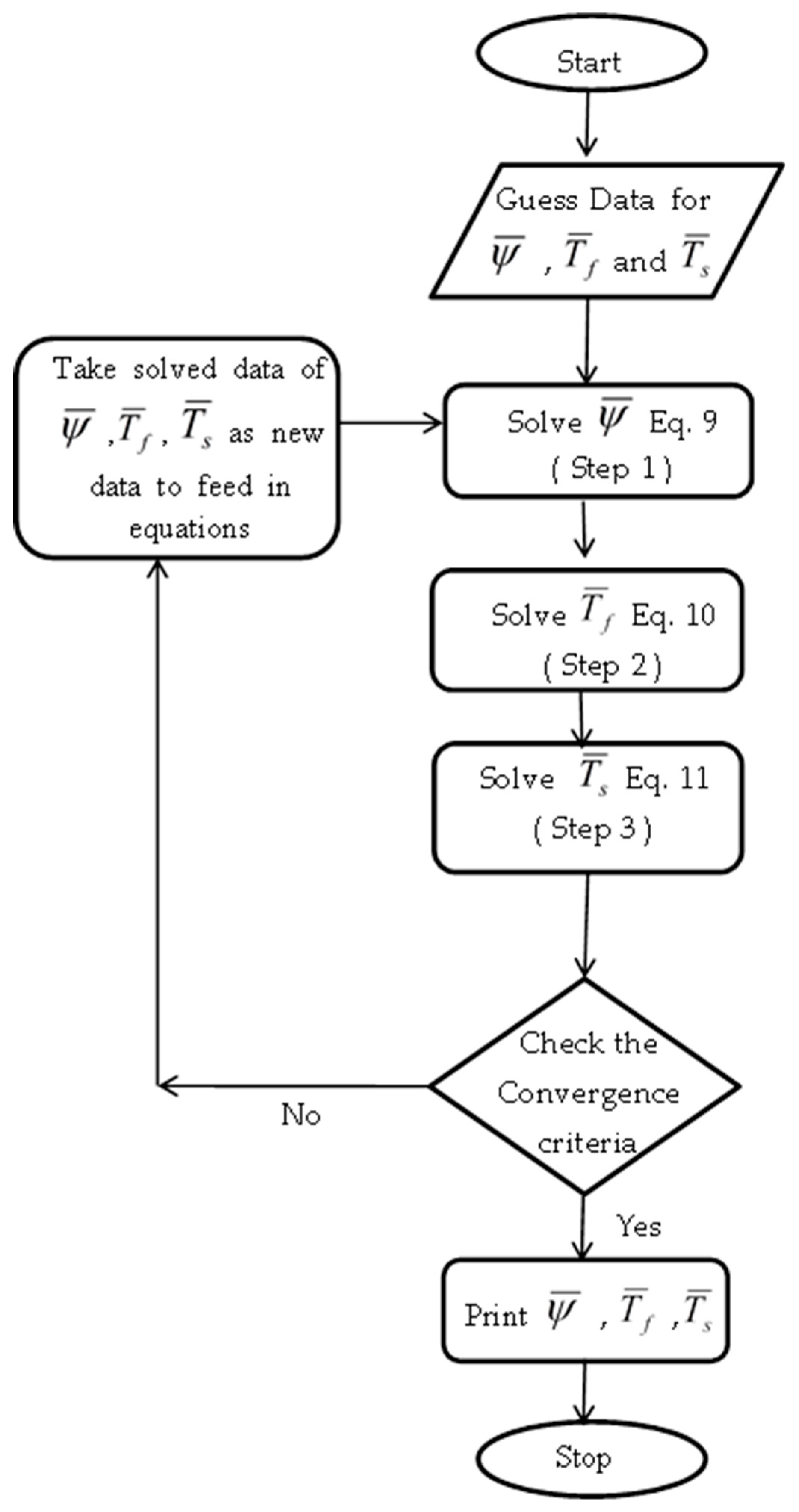
Solution Strategy
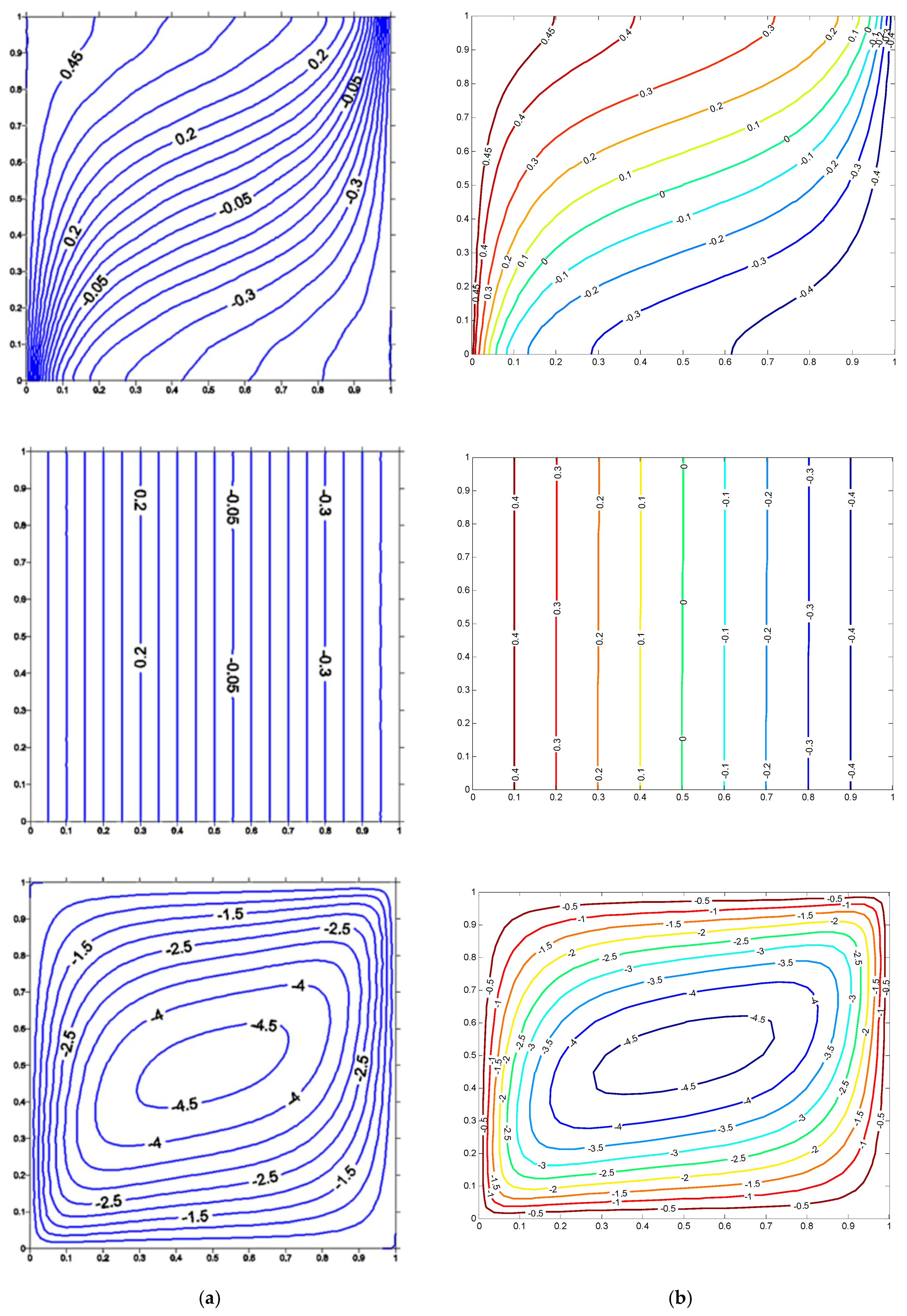
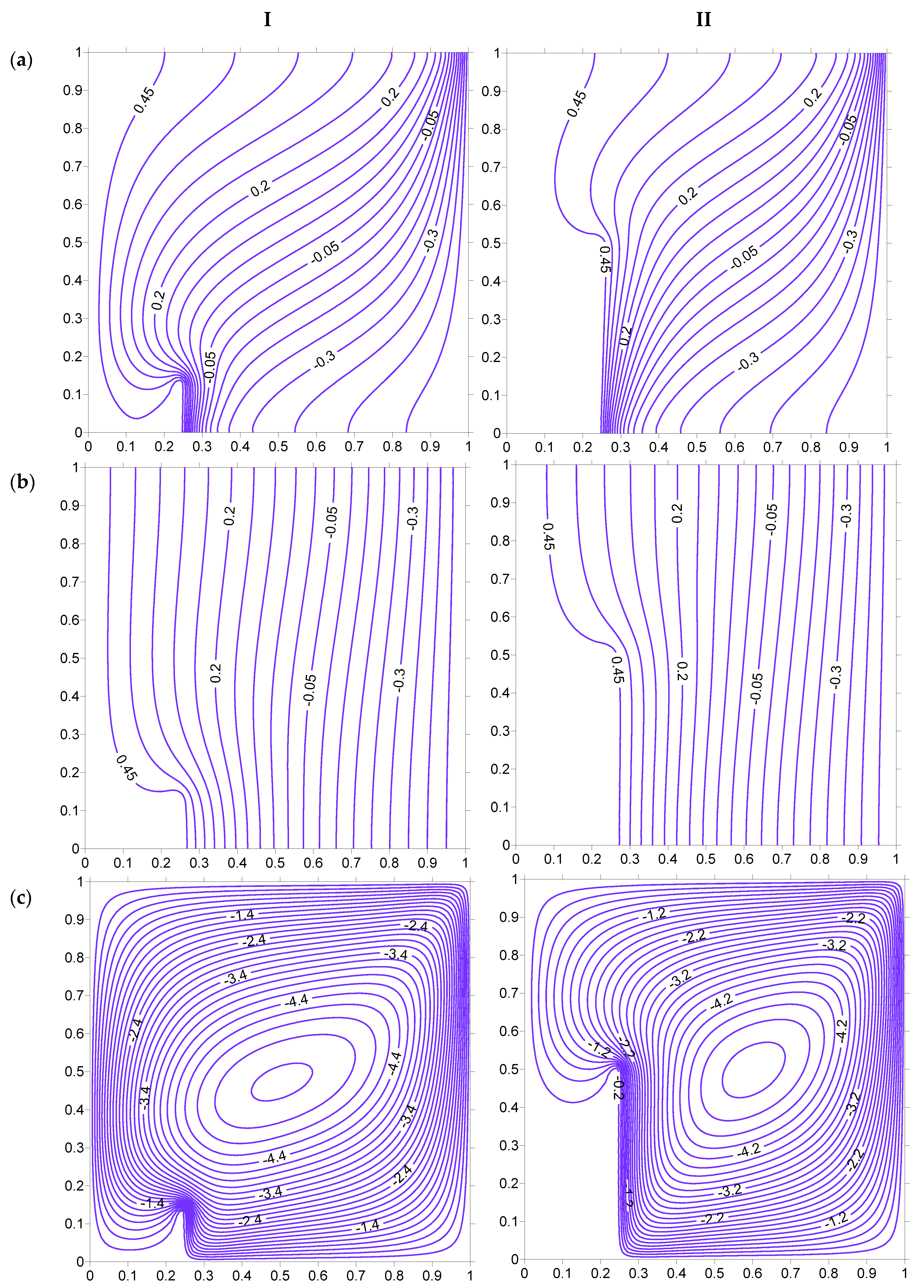
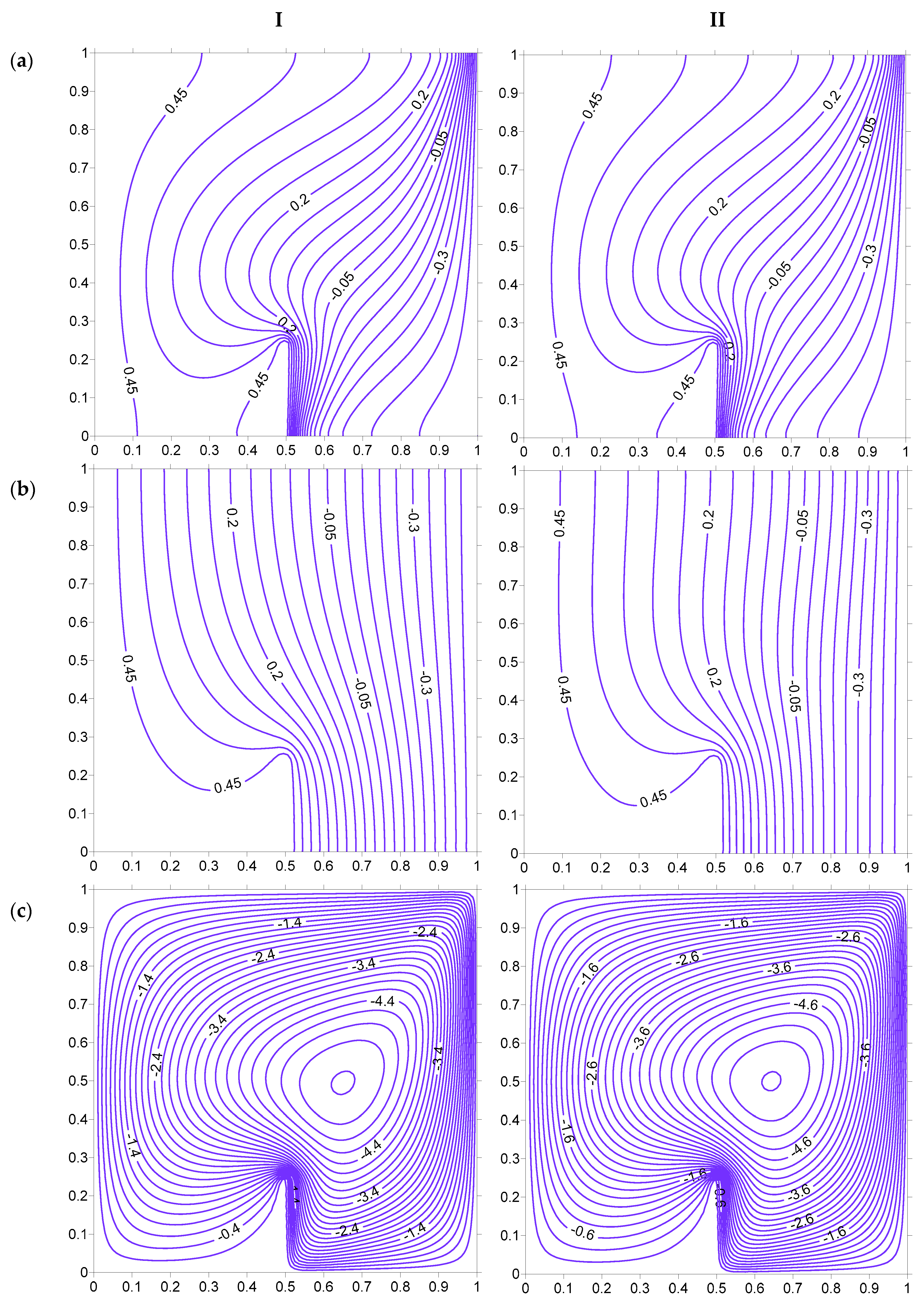
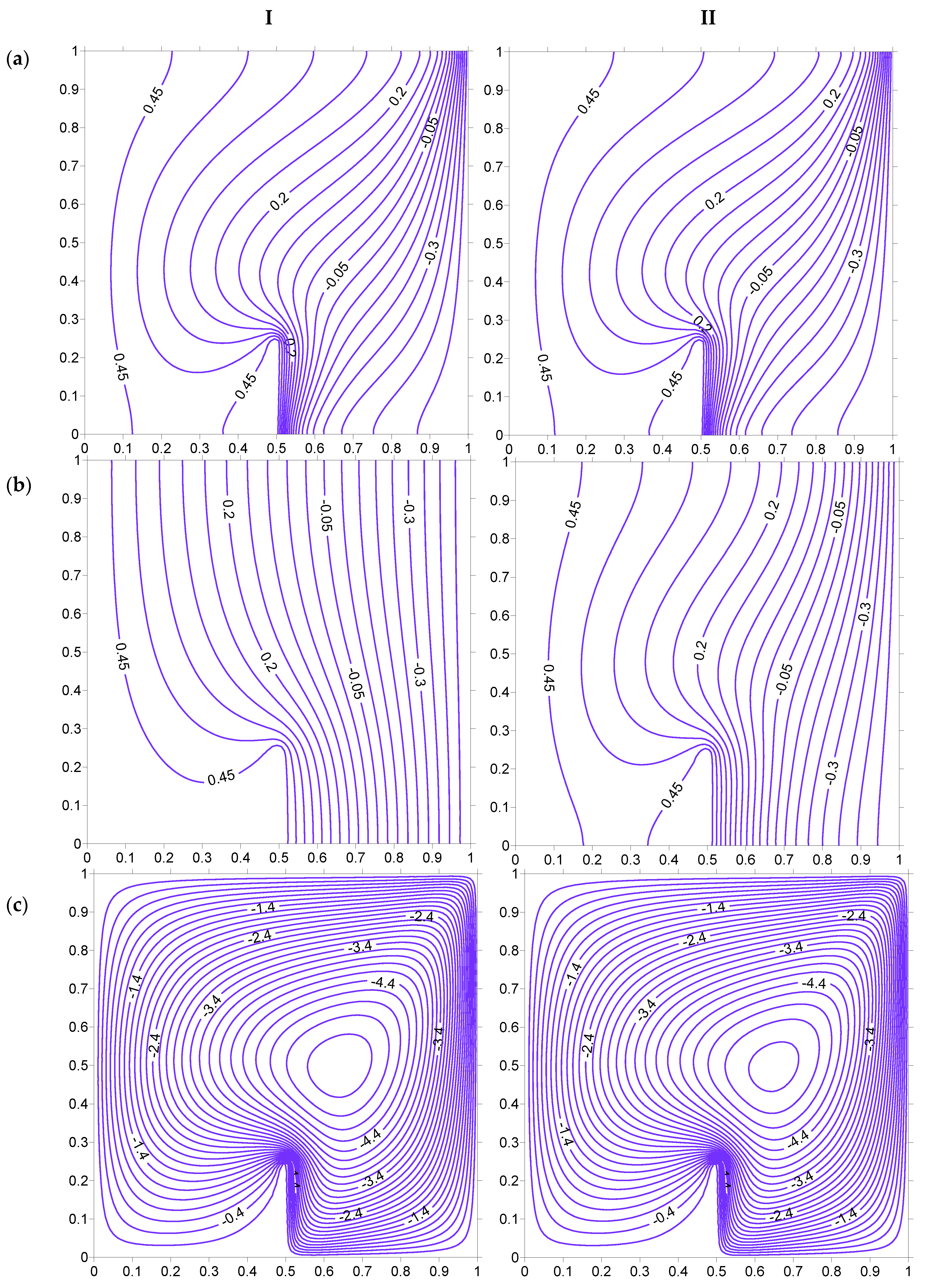
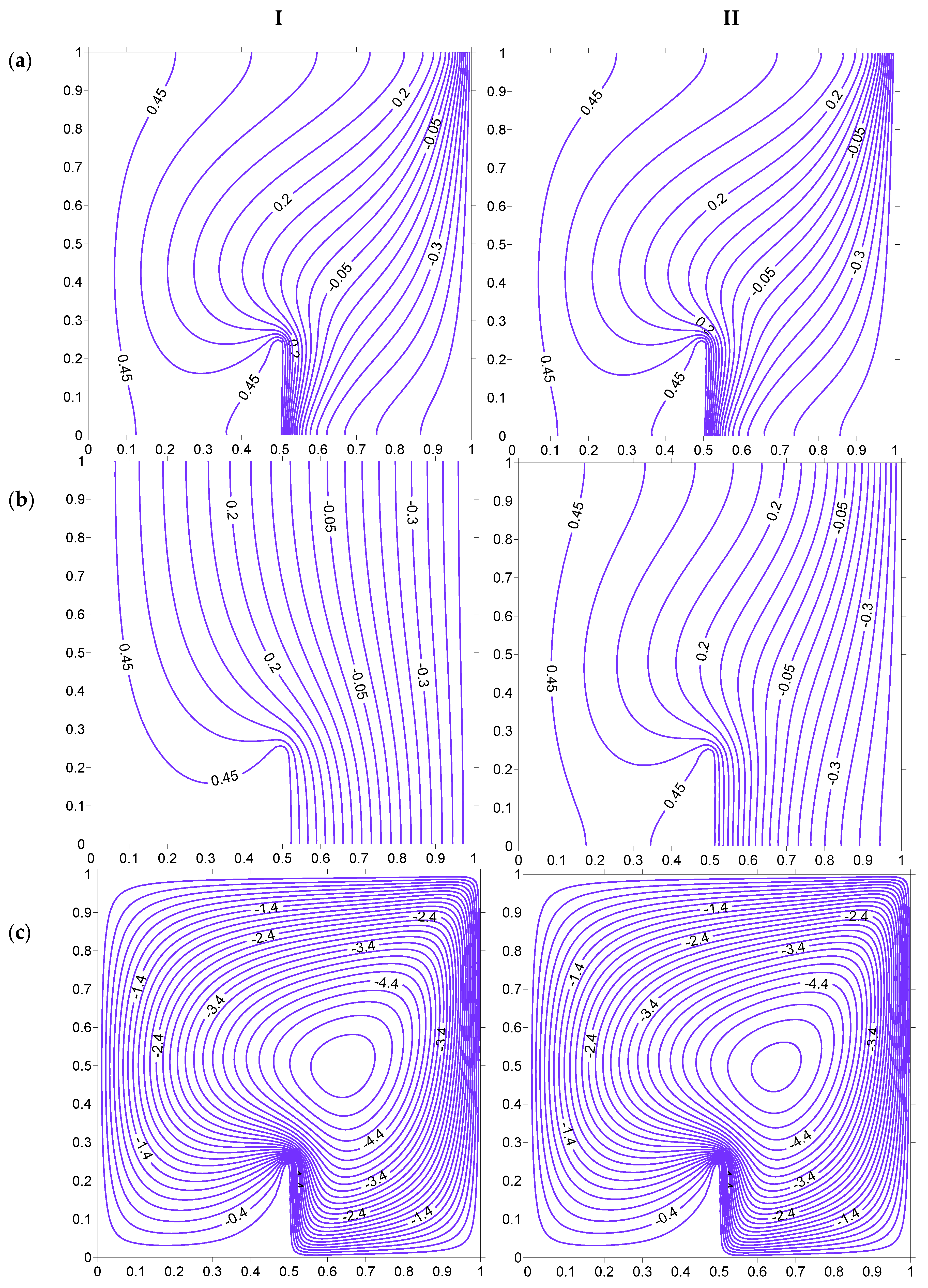
3. Results and Discussion
3.1. Thermal Non-Equilibrium Analysis of Porous Cavity Due to the Heated Strip
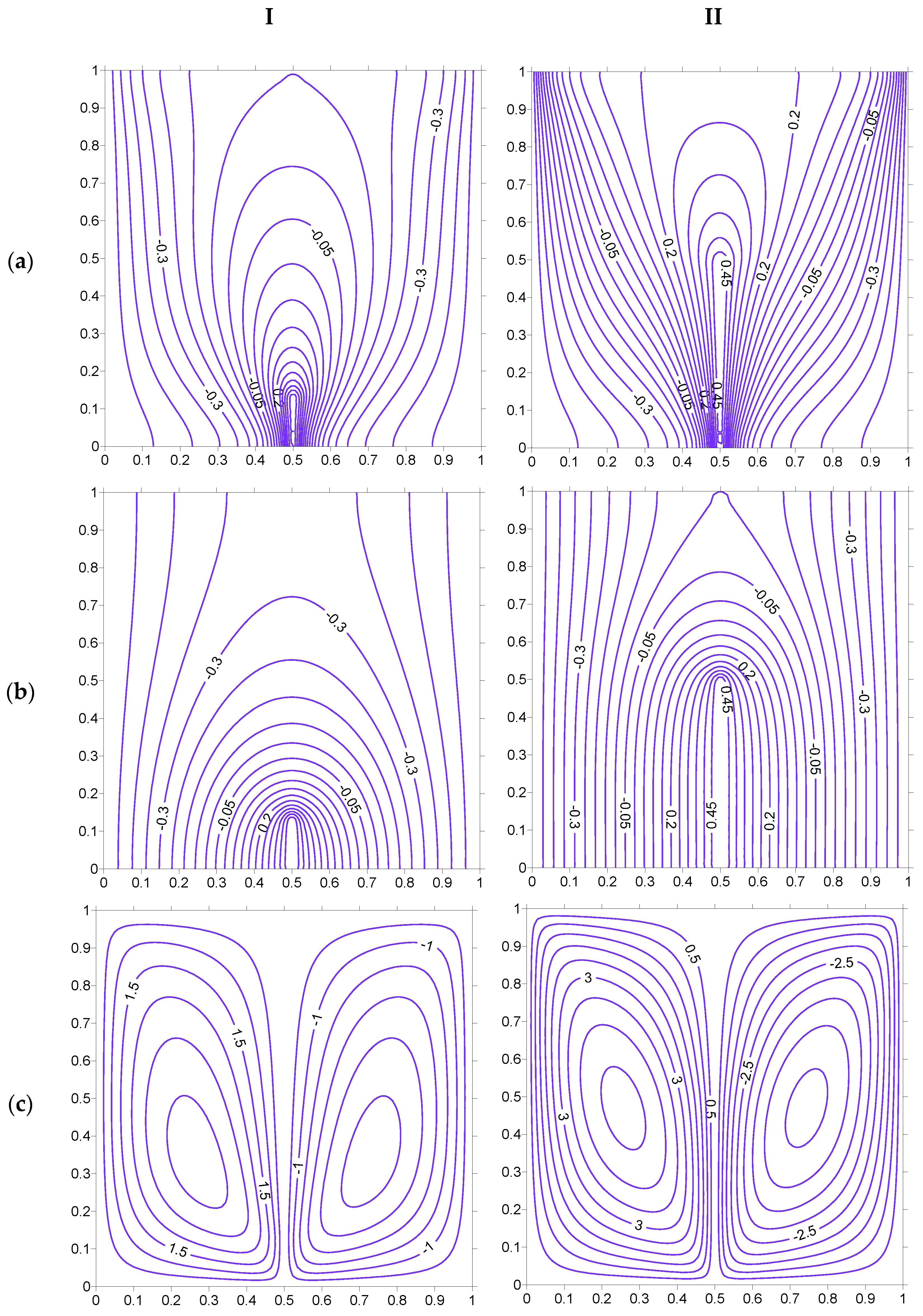
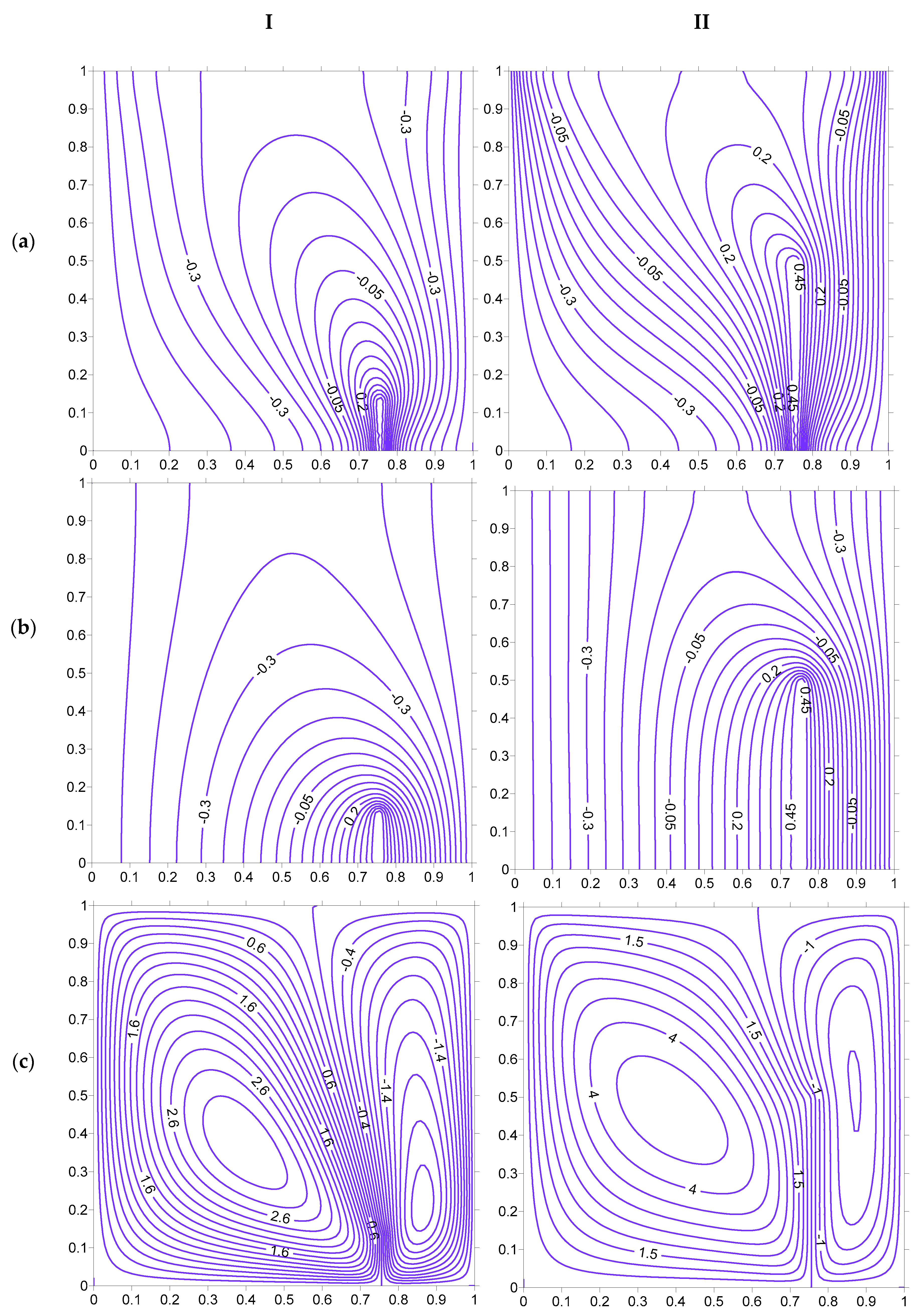
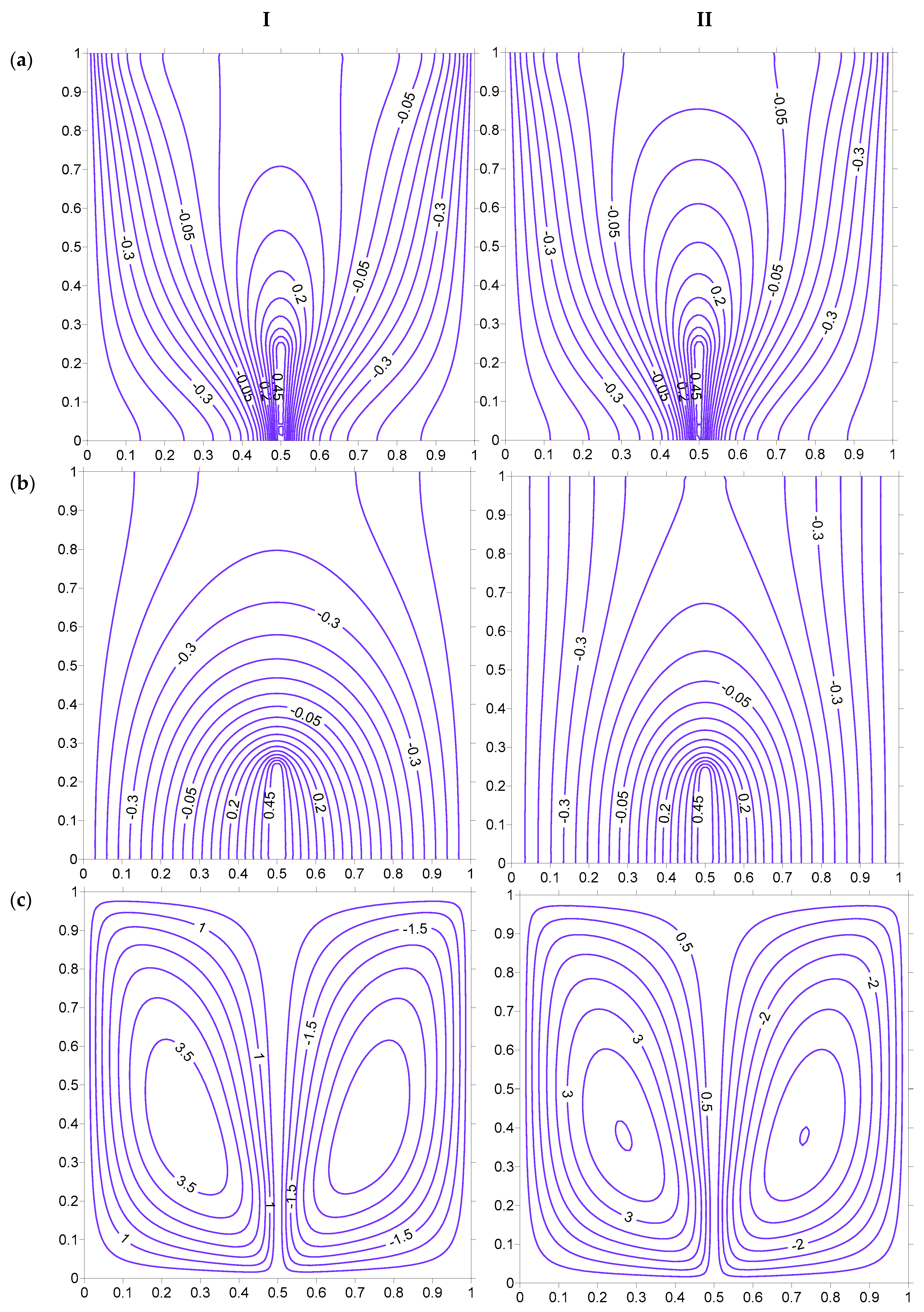
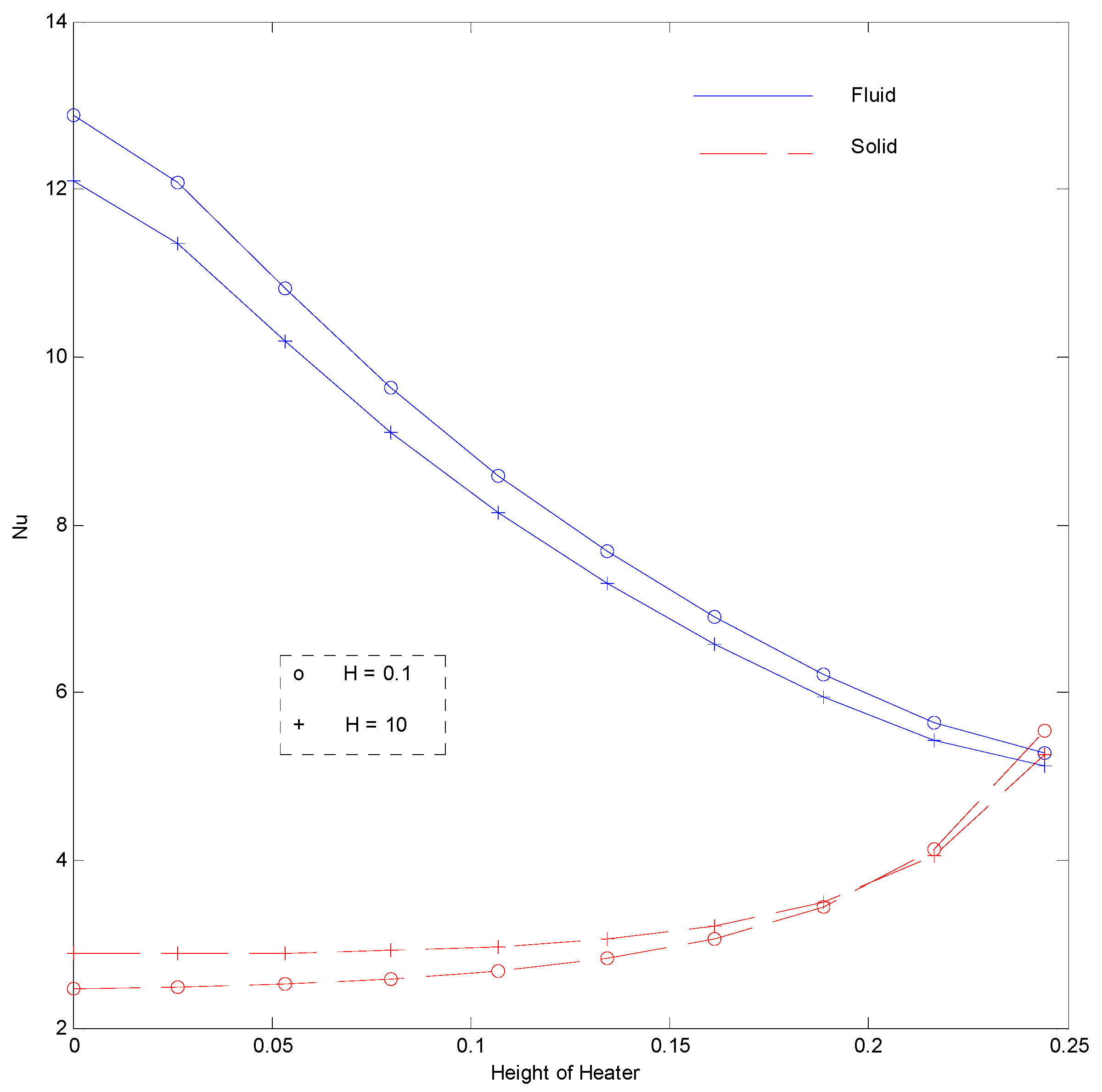
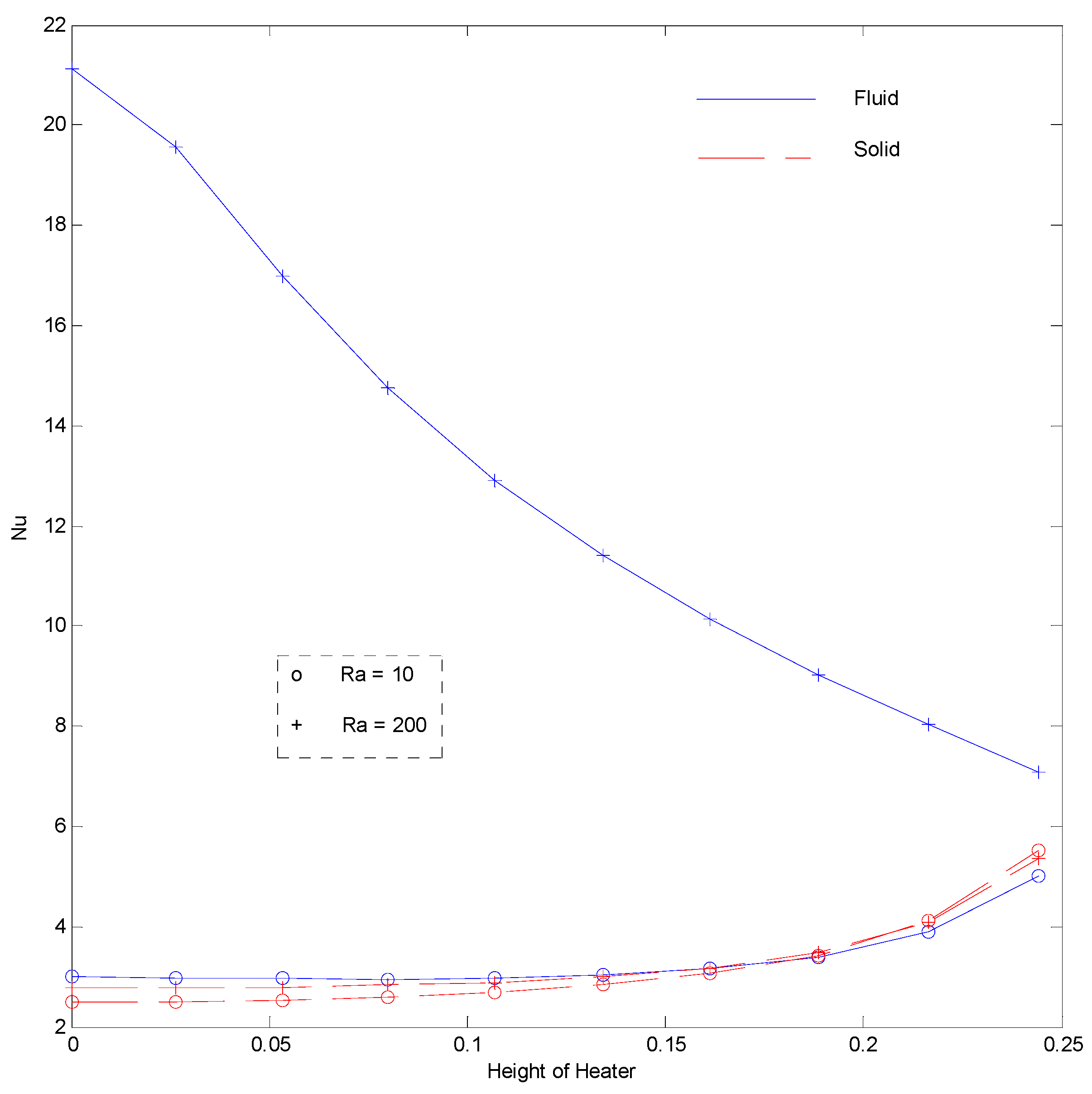
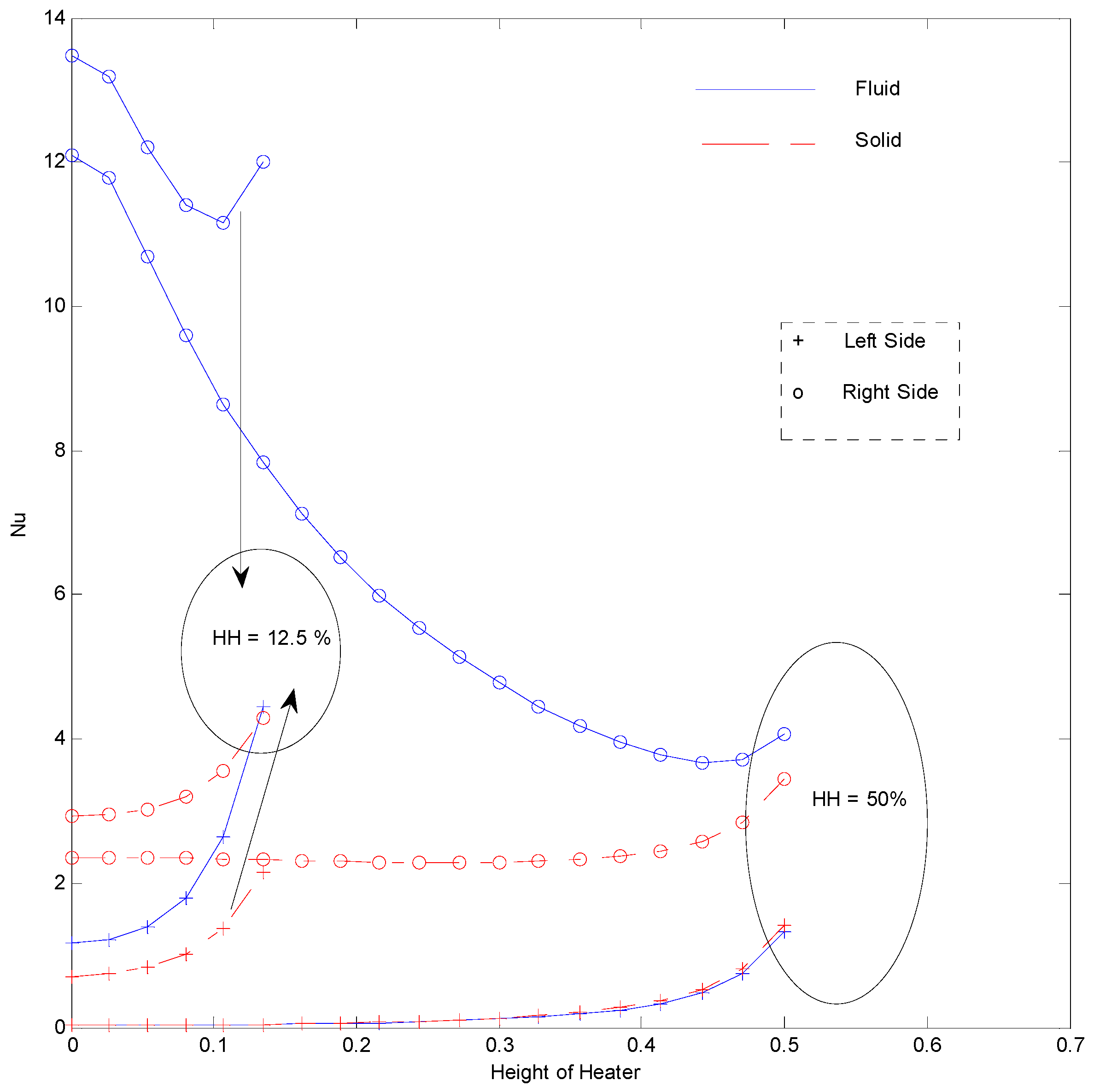
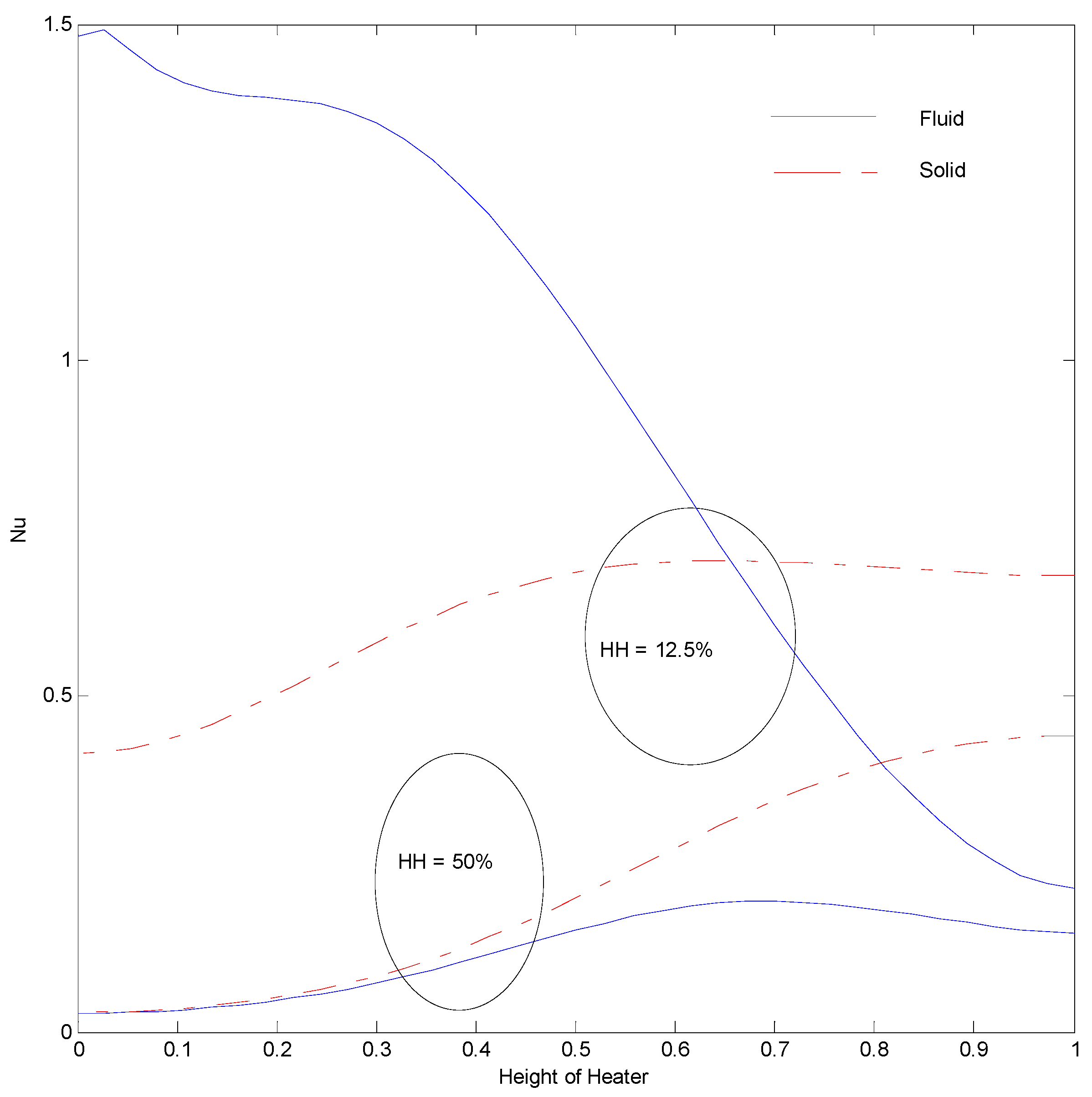
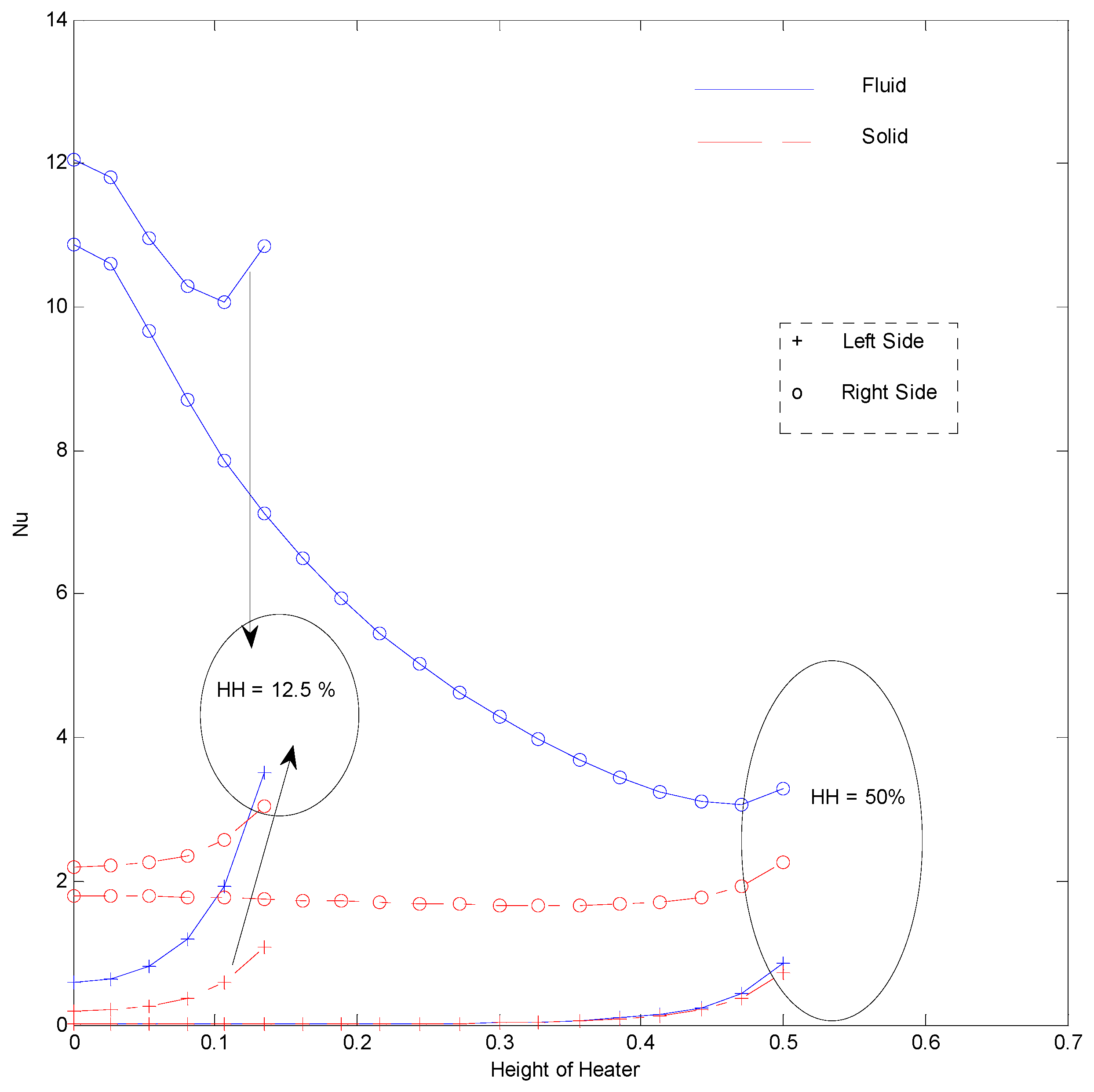
3.2. Case I
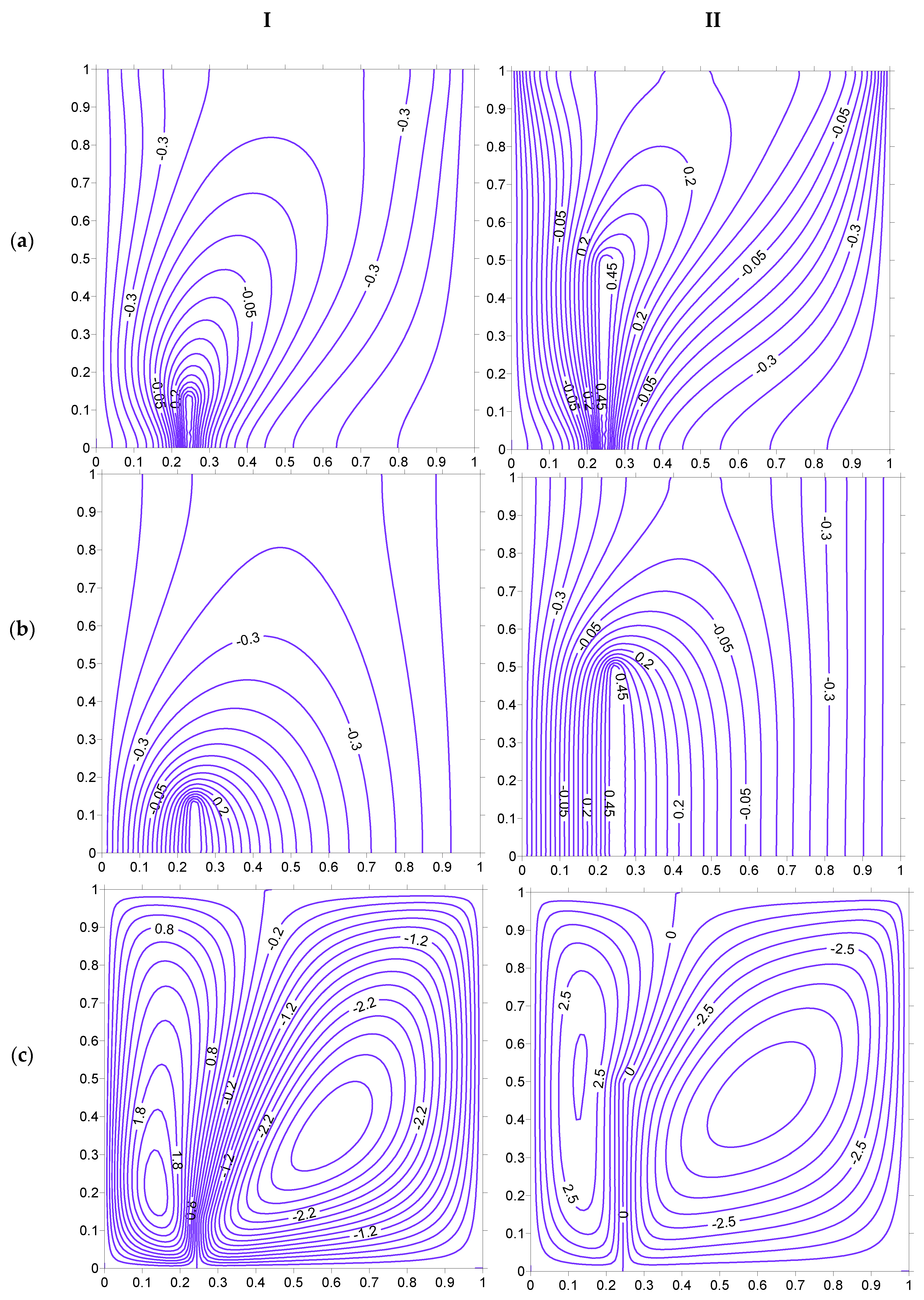
3.3. Case II
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Element area |
| g | Acceleration due to gravity (m/s2) |
| h | Heat transfer coefficient (W/m2·C) |
| k | Thermal conductivity (W/m·°C) |
| kp, ks | Porous and solid thermal conductivity respectively (W/m·°C) |
| HH | Strip height |
| K | Permeability of porous medium (m2) |
| Kr = ks/kp | Conductivity ratio |
| L | Height and length of cavity (m) |
| Radiation parameter | |
| Modified Raleigh number | |
| Temperature | |
| u,v | Velocity component sin x and y direction respectively (m/s) |
| Cartesian coordinates | |
| Non-dimensional coordinates | |
| Greek Symbols | |
| α | Thermal diffusivity (m2/s) |
| β | Coefficient of thermal expansion (1/°C) |
| ρ | Density (kg/m3) |
| v | Coefficient of kinematic viscosity (m2/s) |
| Stephan Boltzmann constant (W/m2·K4) | |
| Absorption coefficient (1/m) | |
| Stream function | |
| Non-dimensional stream function | |
| Subscripts | |
| h | Hot |
| c | Cold |
| s | Solid |
| f | Fluid |
References
- Segerland, L.J. Applied Finite Element Analysis; John Wiley and Sons: New York, NY, USA, 1982. [Google Scholar]
- Lewis, R.W.; Morgan, K.; Thomas, H.R.; Seetharamu, K.N. The Finite Element Method in Heat Transfer Analysis; John Wiley & Sons: Chichester, UK, 1996. [Google Scholar]
- Ahmed, N.S.; Badruddin, I.A.; Kanesan, J.; Zainal, Z.A.; Ahamed, K.N. Study of mixed convection in an annular vertical cylinder filled with saturated porous medium, using thermal non-equilibrium model. Int. J. Heat Mass Transf. 2011, 54, 3822–3825. [Google Scholar] [CrossRef]
- Badruddin, I.A.; Al-Rashed, A.A.; Ahmed, N.S.; Kamangar, S.; Jeevan, K. Natural convection in a square porous annulus. Int. J. Heat Mass Transf. 2012, 55, 7175–7187. [Google Scholar] [CrossRef]
- Amiri, A.; Vafai, K. Transient analysis of incompressible flow through a packed bed. Int. J. Heat Mass Transf. 1998, 41, 4259–4279. [Google Scholar] [CrossRef]
- Jiang, P.X.; Ren, Z.P. Numerical investigation of forced convection heat transfer in porous media using a thermal non-equilibrium model. Int. J. Fluid Flow 2001, 22, 102–110. [Google Scholar] [CrossRef]
- Wong, S.W.; Rees, D.A.S.; Pop, I. Forced convection past a heated cylinder in a porous medium using a thermal non-equilibrium model: Finite Peclet number effects. Int. J. Therm. Sci. 2004, 43, 213–220. [Google Scholar] [CrossRef]
- Rajamani, R.; Srinivasa, C.; Nitiarasu, P.; Seetharamu, K.N. Convective heat transfer in axisymmetric porous bodies. Int. J. Numer. Methods Heat Fluid Flow 1995, 5, 829–837. [Google Scholar] [CrossRef]
- Balla, C.S.; Naikoti, K. Soret and Dufour effects on free convective heat and solute transfer in fluid saturated inclined porous cavity. Eng. Sci. Technol. Int. J. 2015, 18, 543–554. [Google Scholar] [CrossRef]
- Badruddin, I.A.; Zainal, Z.A.; Aswatha Narayana, P.A.; Seetharamu, K.N. Thermal non-equilibrium modeling of heat transfer through vertical annulus embedded with porous medium. Int. J. Heat Mass Transf. 2006, 49, 4955–4965. [Google Scholar] [CrossRef]
- Mahmoudi, Y. Effect of thermal radiation on temperature differential in a porous medium under local thermal non-equilibrium condition. Int. J. Heat Mass Transf. 2014, 76, 105–121. [Google Scholar] [CrossRef]
- Bera, P.; Pippal, S.; Sharma, A.K. A thermal non-equilibrium approach on double-diffusive natural convection in a square porous-medium cavity. Int. J. Heat Mass Transf. 2014, 78, 1080–1094. [Google Scholar] [CrossRef]
- Baytas, A.C.; Pop, I. Free convection in a square porous cavity using a thermal nonequalibruim model. Int. J. Therm. Sci. 2002, 41, 861–870. [Google Scholar] [CrossRef]
- Sheremet, M.; Pop, I.; Baytas, A. Non-equilibrium natural convection in a differentially-heated nanofluid cavity partially filled with a porous medium. Int. J. Numer. Methods Heat Fluid Flow 2019, 29, 2524–2544. [Google Scholar] [CrossRef]
- Abdollahzadeh, J.M.; Oveisi, M. Local thermal non-equilibrium forced convection of a third grade fluid between parallel stretching permeable plates embedded in a porous medium. Therm. Sci. 2019, 23, 475–484. [Google Scholar] [CrossRef]
- Rajarathinam, M.; Nithyadevi, N. Magneto convection heat transfer in a porous square cavity using local thermal non-equilibrium approach. Spec. Top. Rev. Porous Media Int. J. 2019, 10, 129–141. [Google Scholar] [CrossRef]
- Alizadeh, R.; Karimi, N.; Nourbakhsh, A. Effects of radiation and magnetic field on mixed convection stagnation-point flow over a cylinder in a porous medium under local thermal non-equilibrium. J. Therm. Anal. Calorim. 2019. [Google Scholar] [CrossRef]
- Saeid, N.H. Analysis of free convection about a horizontal cylinder in a porous media using a thermal non-equilibrium model. Int. Commun. Heat Mass Transf. 2006, 33, 158–165. [Google Scholar] [CrossRef]
- Saeid, N.H. Analysis of mixed convection in a vertical porous layer using non-equilibrium model. Int. J. Heat Mass Transf. 2004, 47, 5619–5627. [Google Scholar] [CrossRef]
- Espinosa-Paredes, G.; Vázquez-Rodríguez, A.; Espinosa-Martínez, E.G.; Cazarez-Candia, O.; Viera, M.D.; Moctezuma-Berthier, A. A numerical analysis of non-equilibrium thermodynamic effects in an oil field: Two-equation model. Pet. Sci. Technol. 2013, 31, 192–203. [Google Scholar] [CrossRef]
- Vadasz, P. Explicit conditions for local thermal equilibrium in porous media heat conduction. Transp. Porous Media 2005, 59, 341–355. [Google Scholar] [CrossRef]
- Omara, A.; Bourouis, A.; Abboudi, S. Numerical Approach of Thermal non Equilibrium Natural Convection in a Square Porous Cavity with Partially Thermally Active Side Walls. J. Appl. Fluid Mech. 2016, 9, 223–233. [Google Scholar]
- Rees, D.A.S. Microscopic modelling of the two-temperature model for conduction in heterogeneous media. J. Porous Media 2010, 13, 125–143. [Google Scholar] [CrossRef]
- Rees, D.A.S. The effect of local thermal non-equilibrium on the stability of convection in a vertical porous channel. Transp. Porous Media 2011, 87, 459–464. [Google Scholar] [CrossRef]
- Nicolas, L.S.; Straughan, B. A Nonlinear Stability Analysis of Convection in a Porous Vertical Channel Including Local Thermal Nonequilibrium. J. Math. Fluid Mech. 2013, 15, 171–178. [Google Scholar]
- Straughan, B. Global nonlinear stability in porous convection with a thermal nonequilibrium model. Proc. R. Soc. A 2006, 462, 409–418. [Google Scholar] [CrossRef]
- Badruddin, I.A.; Zainal, Z.A.; Aswatha Narayana, P.A.; Seetharamu, K.N. Numerical analysis of convection conduction and radiation using a non-equilibrium model in a square porous cavity. Int. J. Therm. Sci. 2007, 46, 20–29. [Google Scholar] [CrossRef]
- Manole, D.M.; Lage, J.L. Numerical benchmark results for natural convection in a porous medium cavity. In Proceedings of the Heat and Mass Transfer in Porous Media, ASME Conference, HTD 216, Anaheim, CA, USA, 8–13 November 1992; pp. 55–60. [Google Scholar]
- Bekermann, C.; Viskanta, R.; Ramadhyani, S. A numerical study of non-Darcian natural convection in a vertical enclosure filled with a porous medium. Numer. Heat Transf. Part A 1986, 10, 557–570. [Google Scholar]
- Walker, K.L.; Homsy, G.M. Convection in a porous cavity. J. Fluid Mech. 1978, 87, 449–474. [Google Scholar] [CrossRef]
- Bejan, A. On the boundary layer regime in a vertical enclosure filled with a porous medium. Lett. Heat Mass Transf. 1979, 6, 93–102. [Google Scholar] [CrossRef]
- Gross, R.J.; Bear, M.R.; Hickox, C.E. The application of flux-corrected transport (FCT) to high Rayleigh number natural convection in a porous medium. In Proceedings of the 8th International Heat Transfer Conference, San Francisco, CA, USA, 17–22 August 1986. [Google Scholar]
- Moya, S.L.; Ramos, E.; Sen, M. Numerical study of natural convection in a tilted rectangular porous material. Int. J. Heat Mass Transf. 1987, 30, 741–756. [Google Scholar] [CrossRef]
- Baytas, A.C.; Pop, I. Free convection in oblique enclosures filled with a porous medium. Int. J. Heat Mass Transf. 1999, 42, 1047–1057. [Google Scholar] [CrossRef]
- Misirlioglu, A.; Baytas, A.C.; Pop, I. Free convection in a wavy cavity filled with a porous medium. Int. J. Heat Mass Transf. 2005, 48, 1840–1850. [Google Scholar] [CrossRef]















| H | Ref [27] | Present | ||||
|---|---|---|---|---|---|---|
| 0.1 | 3.6438 | 1.8081 | 3.6088 | 3.6014 | 1.8079 | 3.5663 |
| 1 | 3.5833 | 2.6024 | 3.6033 | 3.5805 | 2.5995 | 3.5612 |
| 10 | 3.1050 | 4.2474 | 3.6080 | 3.0833 | 4.2263 | 3.5665 |
| 100 | 1.6877 | 5.4715 | 3.6110 | 1.6335 | 5.4130 | 3.5704 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Badruddin, I.A. Numerical Analysis of Thermal Non-Equilibrium in Porous Medium Subjected to Internal Heating. Mathematics 2019, 7, 1085. https://doi.org/10.3390/math7111085
Badruddin IA. Numerical Analysis of Thermal Non-Equilibrium in Porous Medium Subjected to Internal Heating. Mathematics. 2019; 7(11):1085. https://doi.org/10.3390/math7111085
Chicago/Turabian StyleBadruddin, Irfan Anjum. 2019. "Numerical Analysis of Thermal Non-Equilibrium in Porous Medium Subjected to Internal Heating" Mathematics 7, no. 11: 1085. https://doi.org/10.3390/math7111085
APA StyleBadruddin, I. A. (2019). Numerical Analysis of Thermal Non-Equilibrium in Porous Medium Subjected to Internal Heating. Mathematics, 7(11), 1085. https://doi.org/10.3390/math7111085





