Exact Solution for the Heat Transfer of Two Immiscible PTT Fluids Flowing in Concentric Layers through a Pipe
Abstract
:1. Introduction
2. Basic Equations
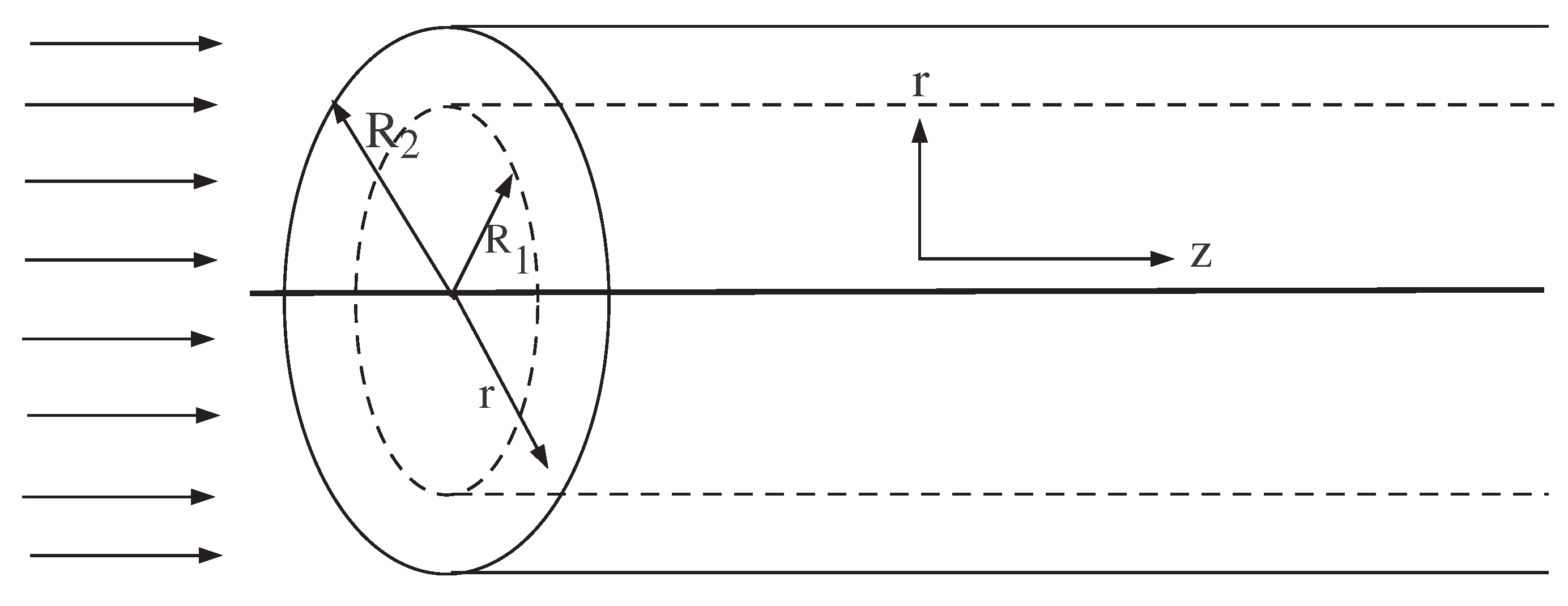
3. Problem Formulation
3.1. Boundary Conditions
- Shear Stresses
- Velocities
- Temperatures
3.2. Dimensionless Parameter
4. Solution of the Problem
4.1. Stress Distributions
4.2. Velocity Profile
4.3. Maximum Velocity
4.4. Total Volume Flow Rate through a Cross Section of the Pipe
4.5. Temperature Distributions
5. Graphical Results and Discussion
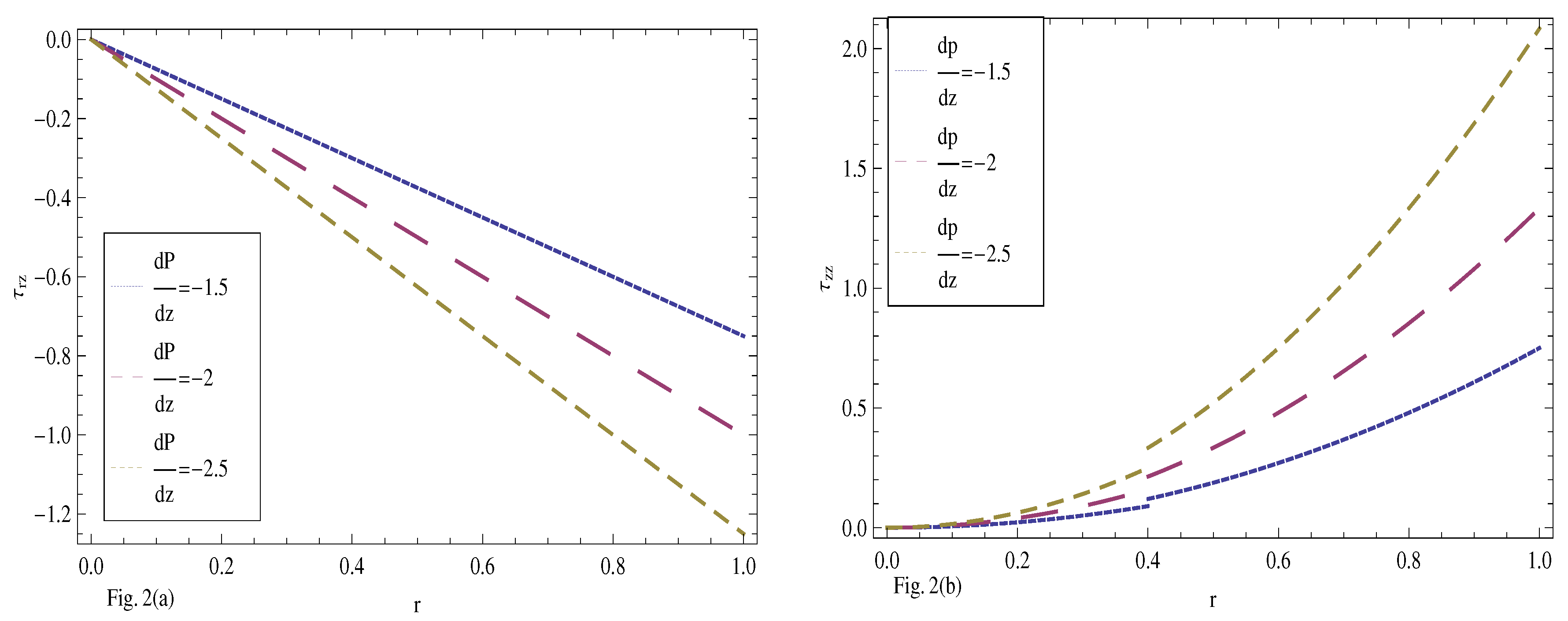
5.1. Stresses
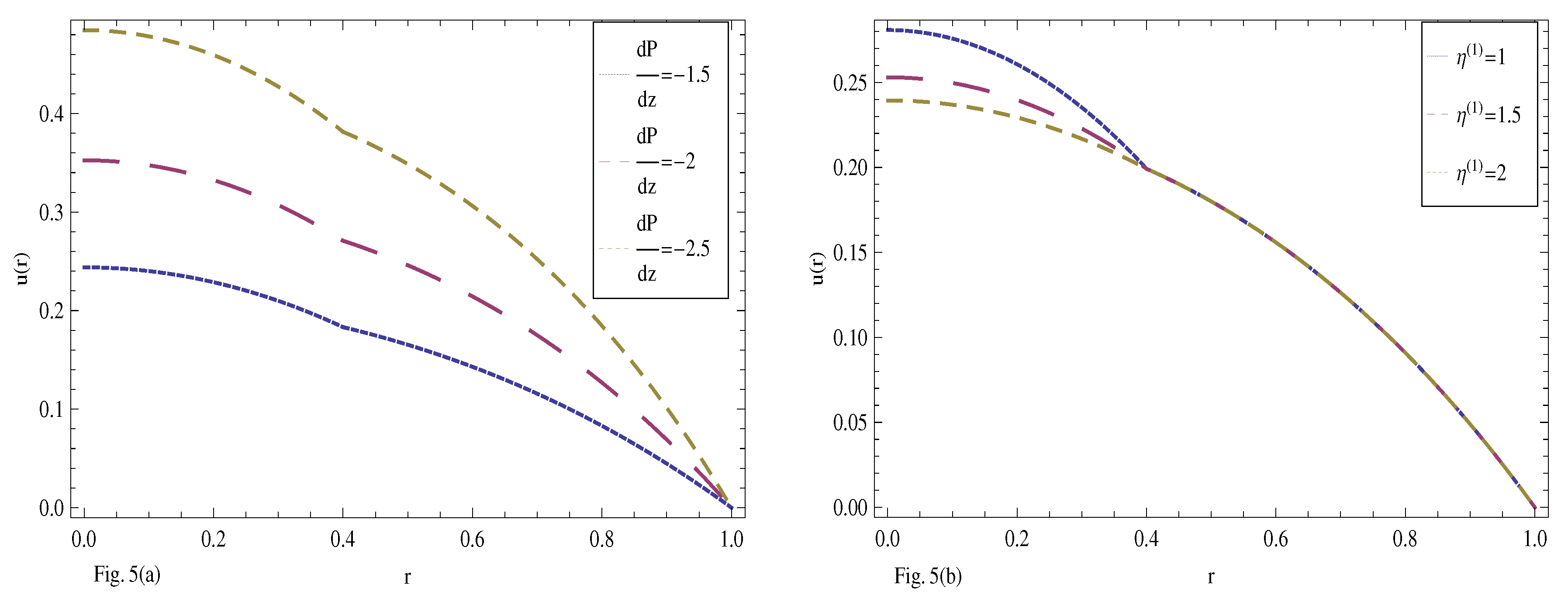
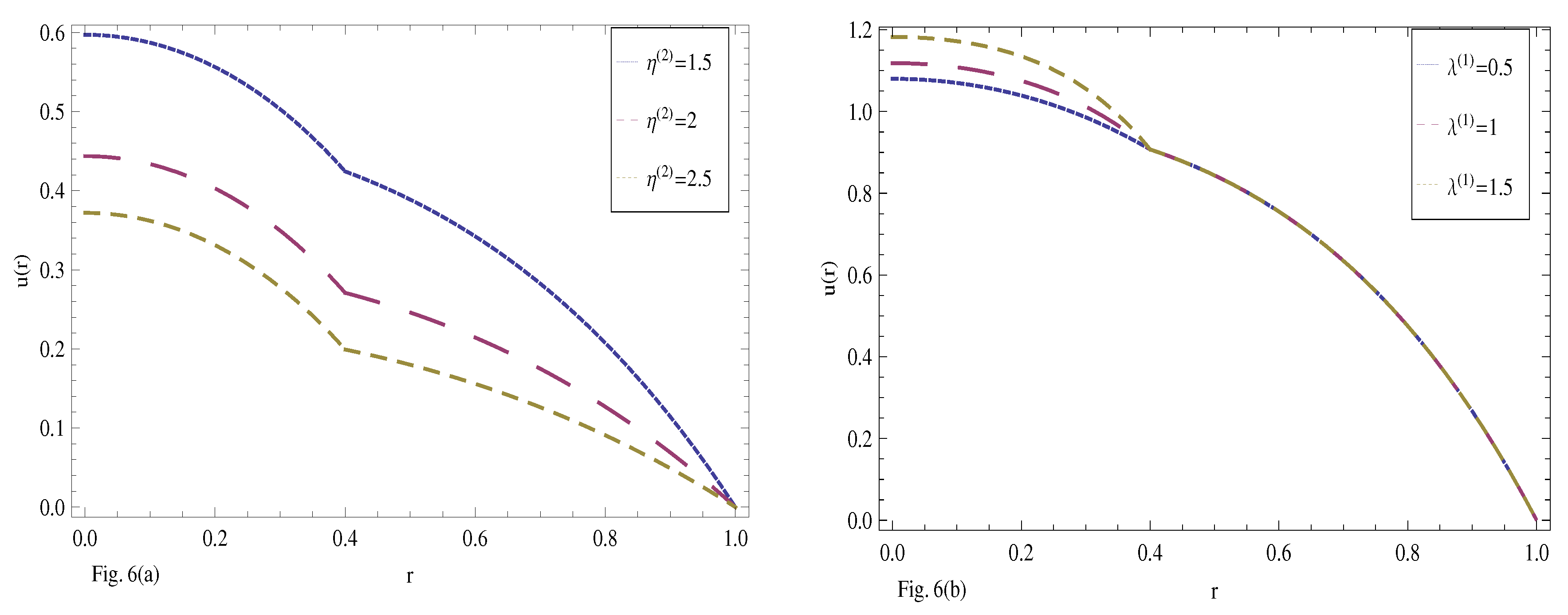
5.2. Velocities
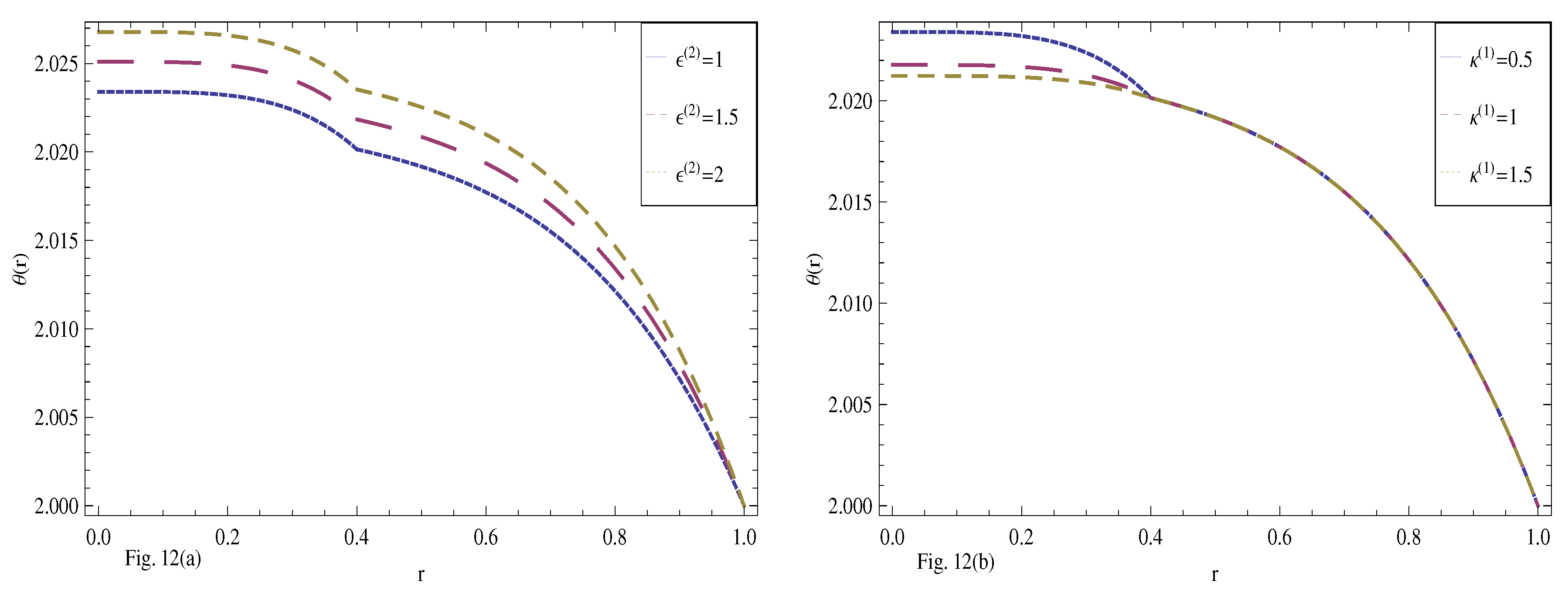
5.3. Temperatures
5.4. Application—Optimal Value of for Maximal Flow Rate
6. Conclusions
- Shear stresses vary linearly and independently of the material constants.
- Normal stresses are not linear in nature and depend on the material constants.
- Continuity of velocities exists at interface.
- The results for Newtonian fluids can be deduced by substituting material parameters .
- The expression for velocities when one of the two fluids is Newtonian in nature can be deduced by substituting the corresponding to that fluid equal to zero.
- Velocities play basic roles in the transportation of the fluids; graphical representations show that fluid velocities can be controlled with the proper choice of the involved parameters.
- The approach for solving the problem is also applicable to the flow of a finite number of layers, in which case, the continuity of velocities at interfaces results in one fluid affecting the flow of its adjacent inner layer.
- The number of fluid layers does not affect the existence of a unique velocity maximum, which is always in the innermost fluid layer, and this observation has great impact on the transportation and pumping of immiscible fluids.
Author Contributions
Funding
Conflicts of Interest
References
- Malashetty, M.S.; Umavathi, J.C. Two-phase magnetohydrodynamic flow and heat transfer in an inclined channel. Int. J. Multiph. Flow 1997, 23, 545–560. [Google Scholar] [CrossRef]
- Elmaboud, Y.A.; Abdelsalam, S.I.; Mekheimer, K.S.; Vafai, K. Electromagnetic flow for two-layer immiscible fluids. Eng. Sci. Technol. Int. J. 2018. [Google Scholar] [CrossRef]
- Packham, B.A.; Shail, R. Stratified laminar flow of two immiscible fluids. Math. Proc. Camb. Philos. Soc. 1971, 69, 443–448. [Google Scholar] [CrossRef]
- Brauner, N. Two-phase liquid-liquid annular flow. Int. J. Multiph. Flow 1991, 17, 59–76. [Google Scholar] [CrossRef]
- Lohrasbi, J.; Sahai, V. Magnetohydrodynamic heat transfer in two-phase flow between parallel plates. Appl. Sci. Res. 1988, 45, 53–66. [Google Scholar] [CrossRef]
- Malashetty, M.S.; Leela, V. Magnetohydrodynamic heat transfer in two phase flow. Int. J. Eng. Sci. 1992, 30, 371–377. [Google Scholar] [CrossRef]
- Shail, R. On laminar two-phase flows in Magnetohydrodynamics. Int. J. Eng. Sci. 1973, 11, 1103–1108. [Google Scholar] [CrossRef]
- Leib, T.M.; Hasson, D. Heat transfer in vertical annular laminar flow of two immiscible liquids. Int. J. Multiph. Flow 1977, 3, 533–549. [Google Scholar] [CrossRef]
- Siddiqui, A.M.; Azim, Q.A.; Rana, M.A. On exact solutions of concentric n-layer flows of viscous fluids in a pipe. Nonlinear Sci. Lett. A 2010, 1, 67–76. [Google Scholar]
- Cruz, D.O.A.; Pinho, F.T. Analysis of isothermal flow of a Phan-Thien-Tanner fluid in a simplified model of a single- screw-extruder. J. Non-Newton. Fluid Mech. 2012, 167–168, 95–105. [Google Scholar] [CrossRef]
- Tanner, R.I. Engineering Rheology; OUP: Oxford, UK, 2000; Volume 52. [Google Scholar]
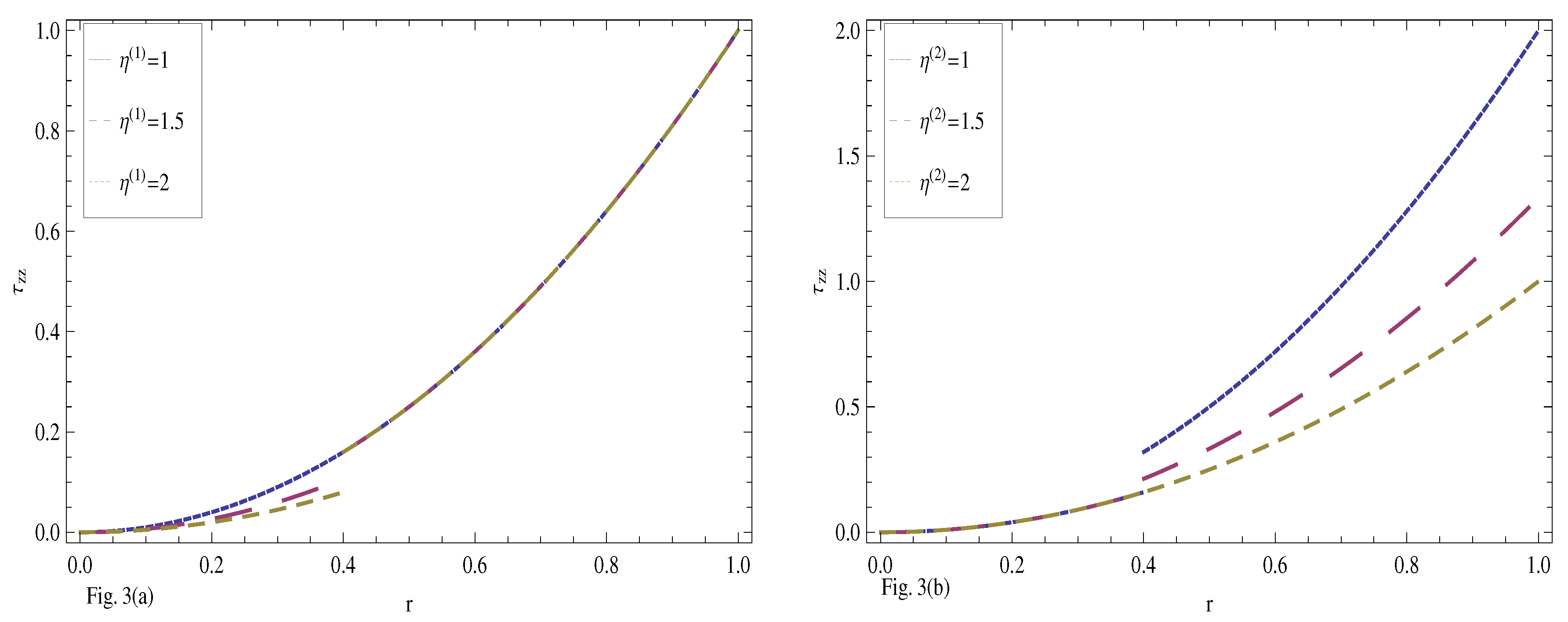
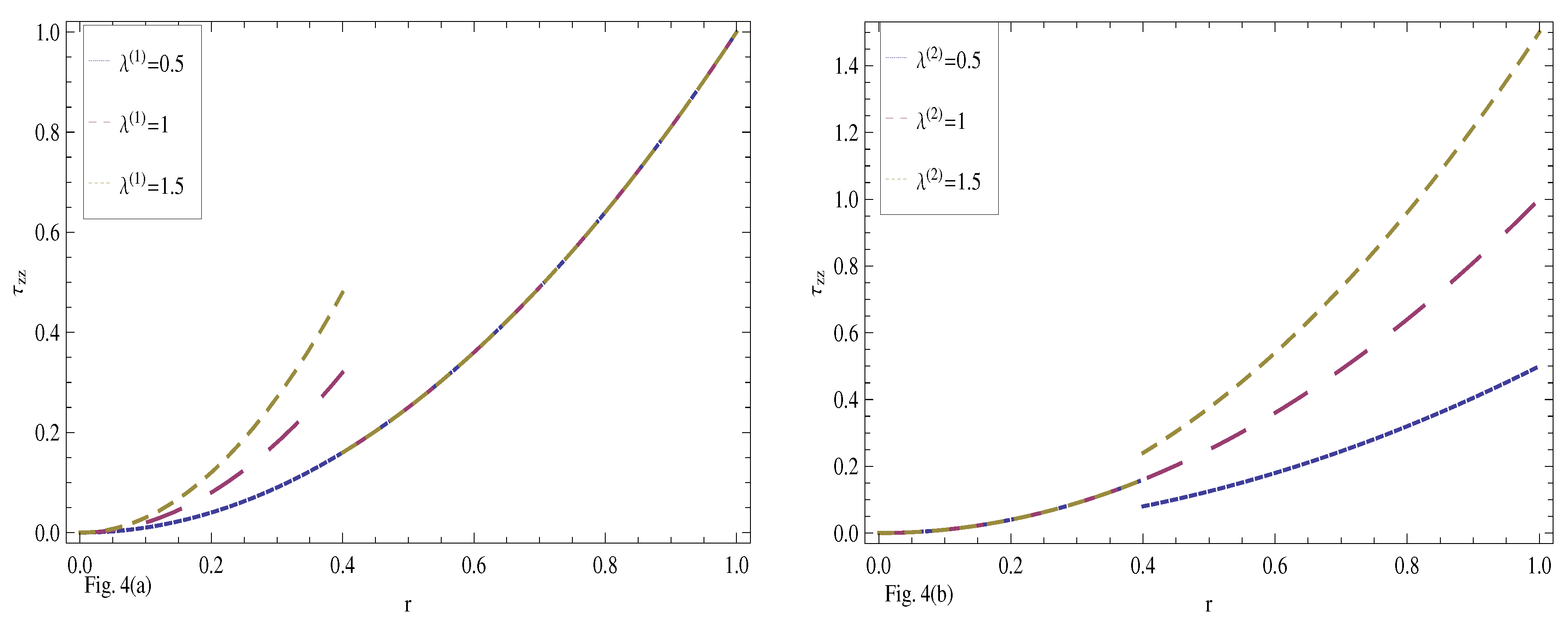

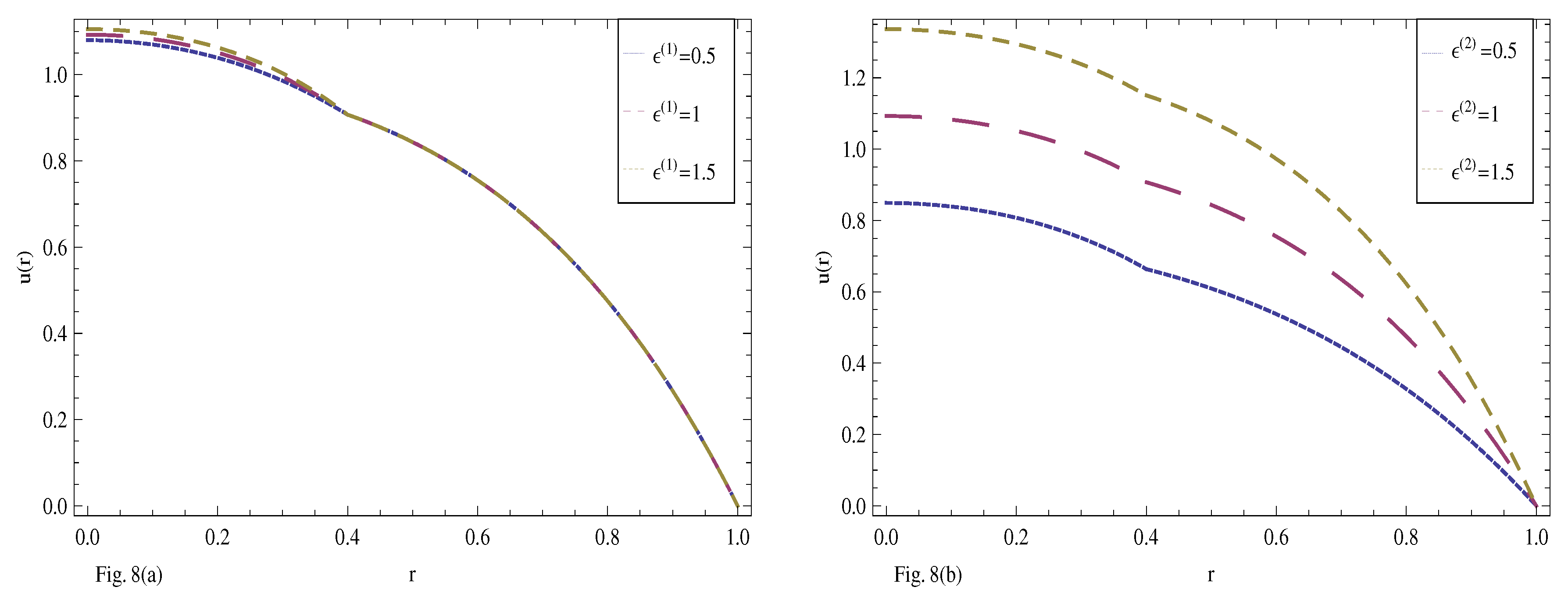
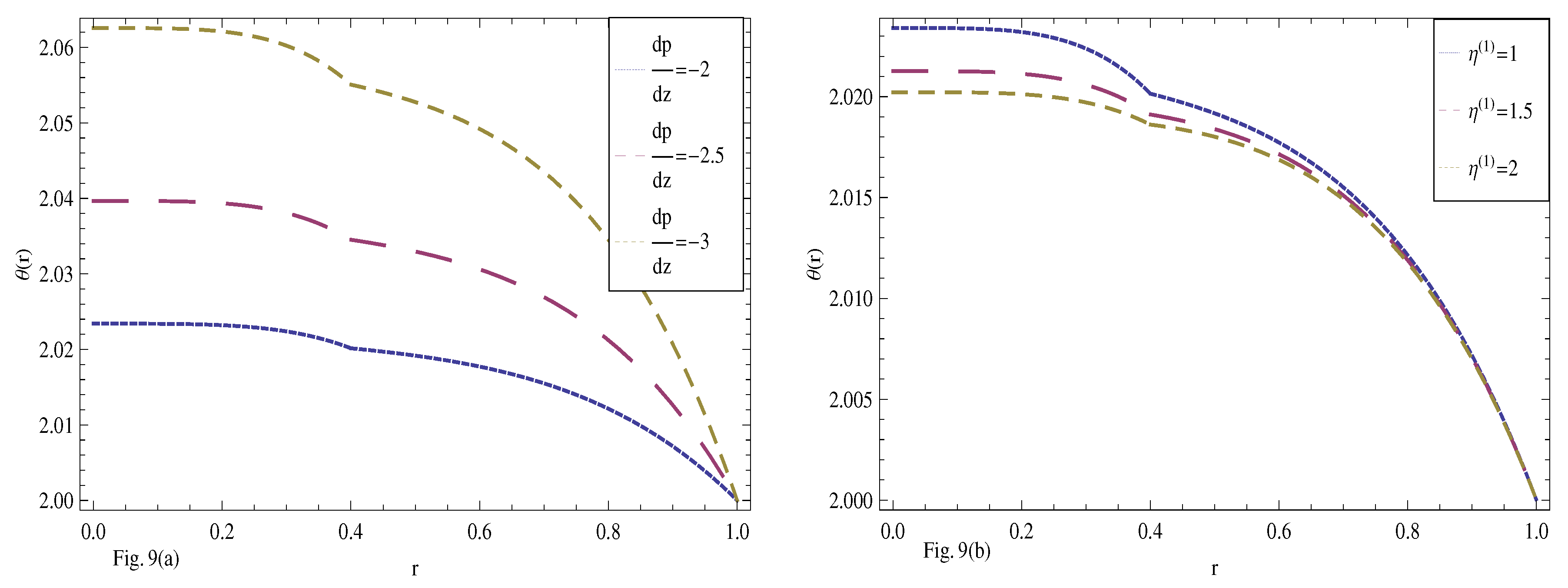

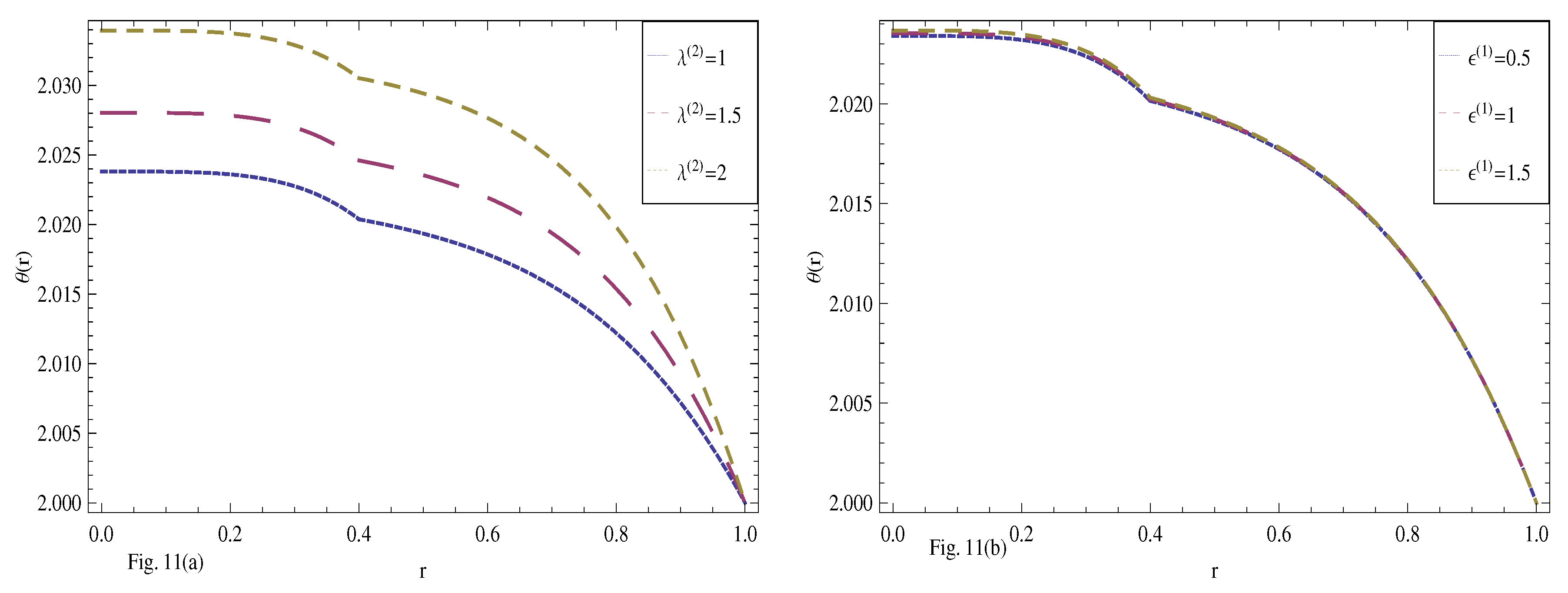
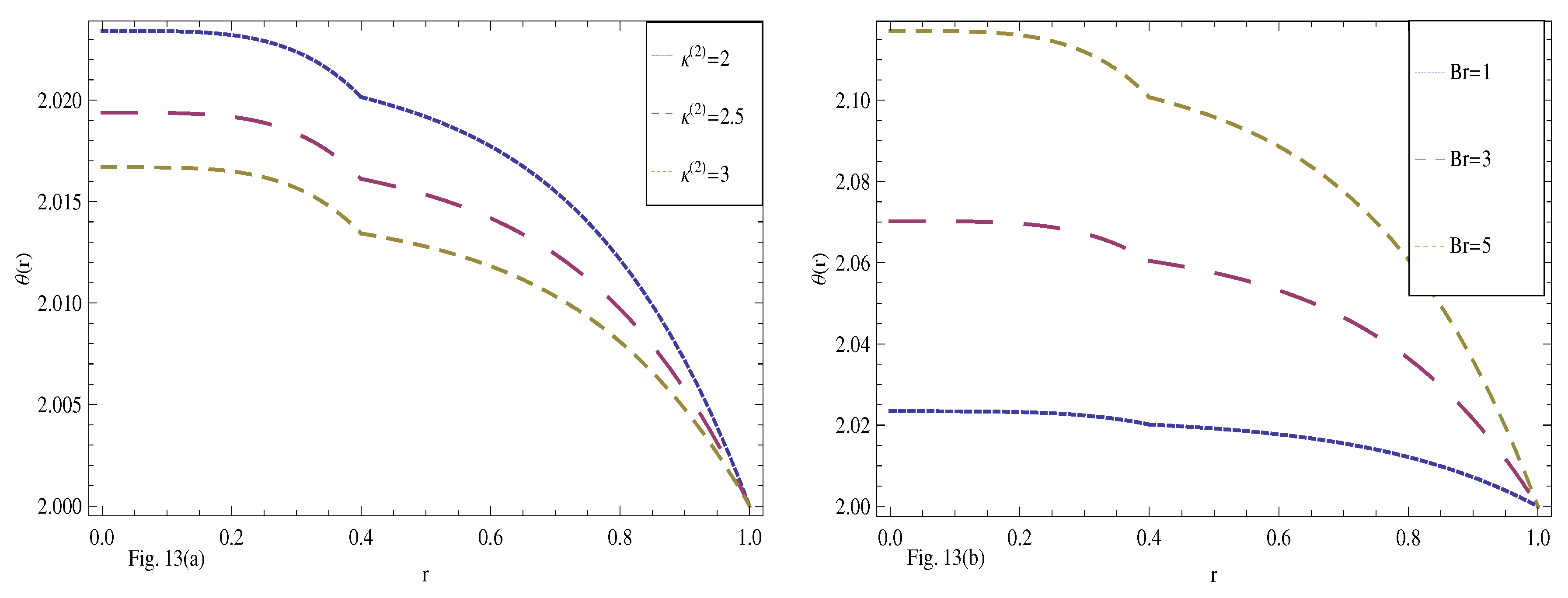
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Siddiqui, A.M.; Zeb, M.; Haroon, T.; Azim, Q.-u.-A. Exact Solution for the Heat Transfer of Two Immiscible PTT Fluids Flowing in Concentric Layers through a Pipe. Mathematics 2019, 7, 81. https://doi.org/10.3390/math7010081
Siddiqui AM, Zeb M, Haroon T, Azim Q-u-A. Exact Solution for the Heat Transfer of Two Immiscible PTT Fluids Flowing in Concentric Layers through a Pipe. Mathematics. 2019; 7(1):81. https://doi.org/10.3390/math7010081
Chicago/Turabian StyleSiddiqui, Abdul Majeed, Muhammad Zeb, Tahira Haroon, and Qurat-ul-Ain Azim. 2019. "Exact Solution for the Heat Transfer of Two Immiscible PTT Fluids Flowing in Concentric Layers through a Pipe" Mathematics 7, no. 1: 81. https://doi.org/10.3390/math7010081
APA StyleSiddiqui, A. M., Zeb, M., Haroon, T., & Azim, Q.-u.-A. (2019). Exact Solution for the Heat Transfer of Two Immiscible PTT Fluids Flowing in Concentric Layers through a Pipe. Mathematics, 7(1), 81. https://doi.org/10.3390/math7010081




