Model Predictive Control of Mineral Column Flotation Process
Abstract
:1. Introduction
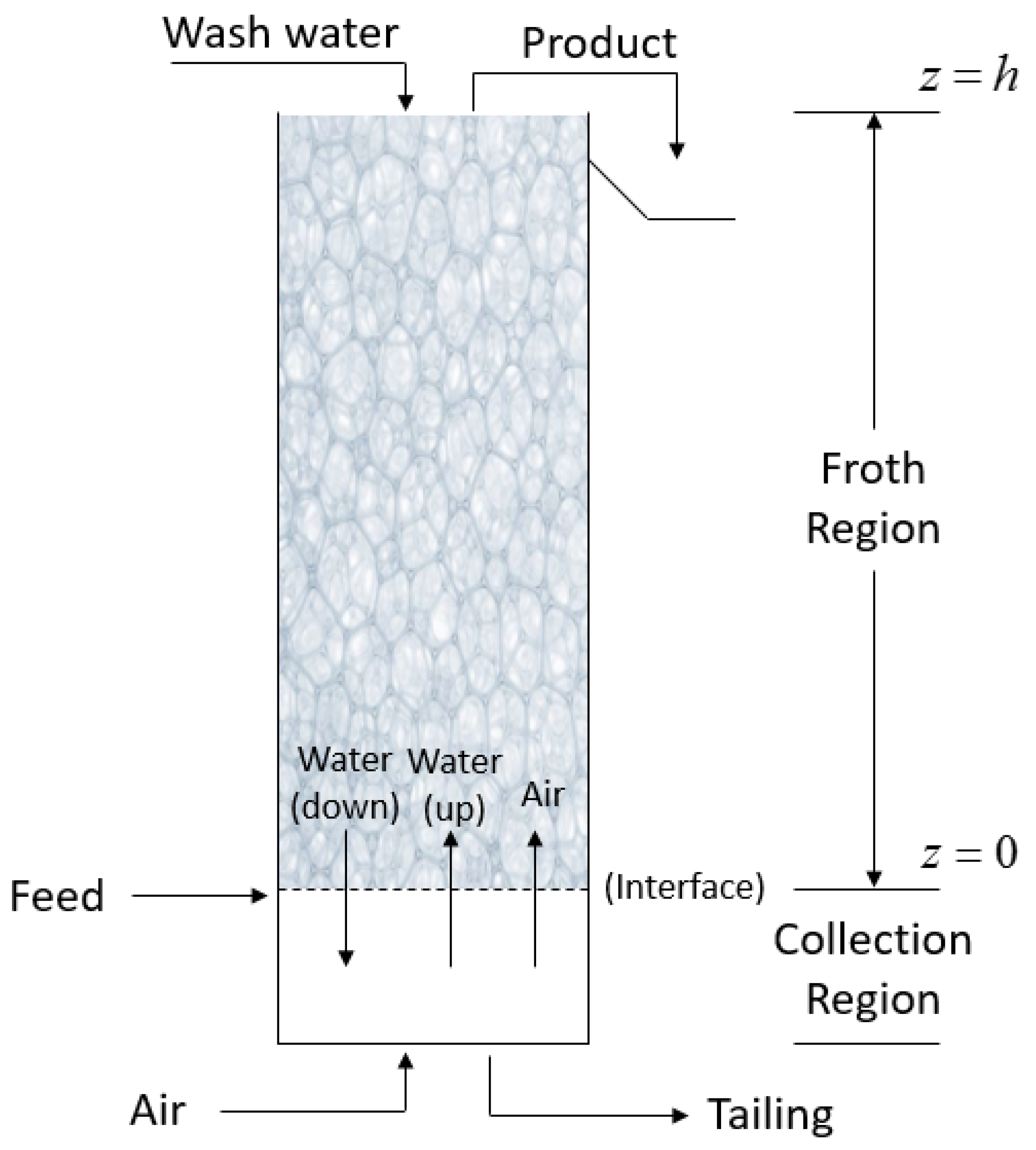
2. Model Formulation of Column Flotation
2.1. Model Description
2.1.1. Model for Collection Region
2.1.2. Model for Froth Region
2.2. Linearized Model
2.3. Discretized Model
2.3.1. Time Discretization for Linear PDE
2.3.2. Time Discretization of Column Flotation System
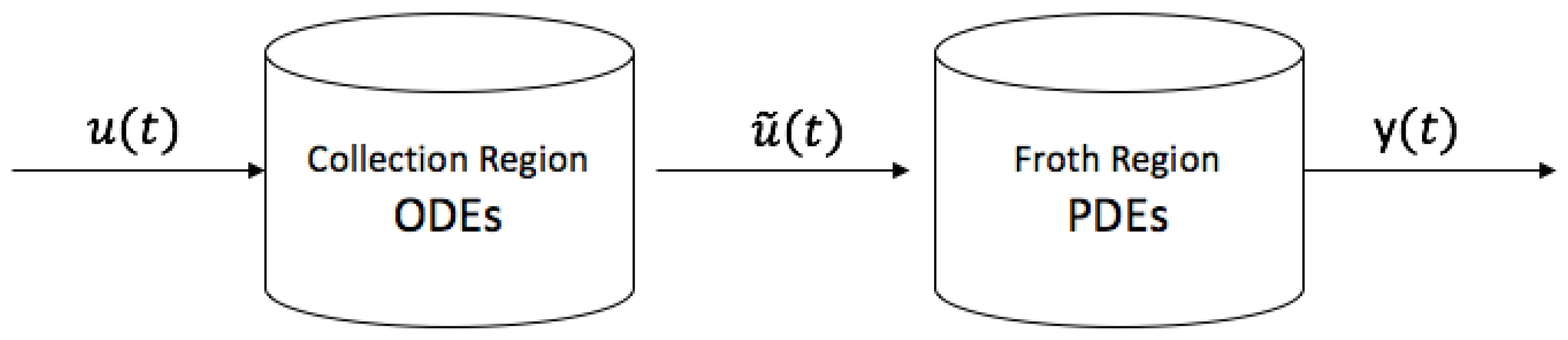
3. Model Predictive Control Design
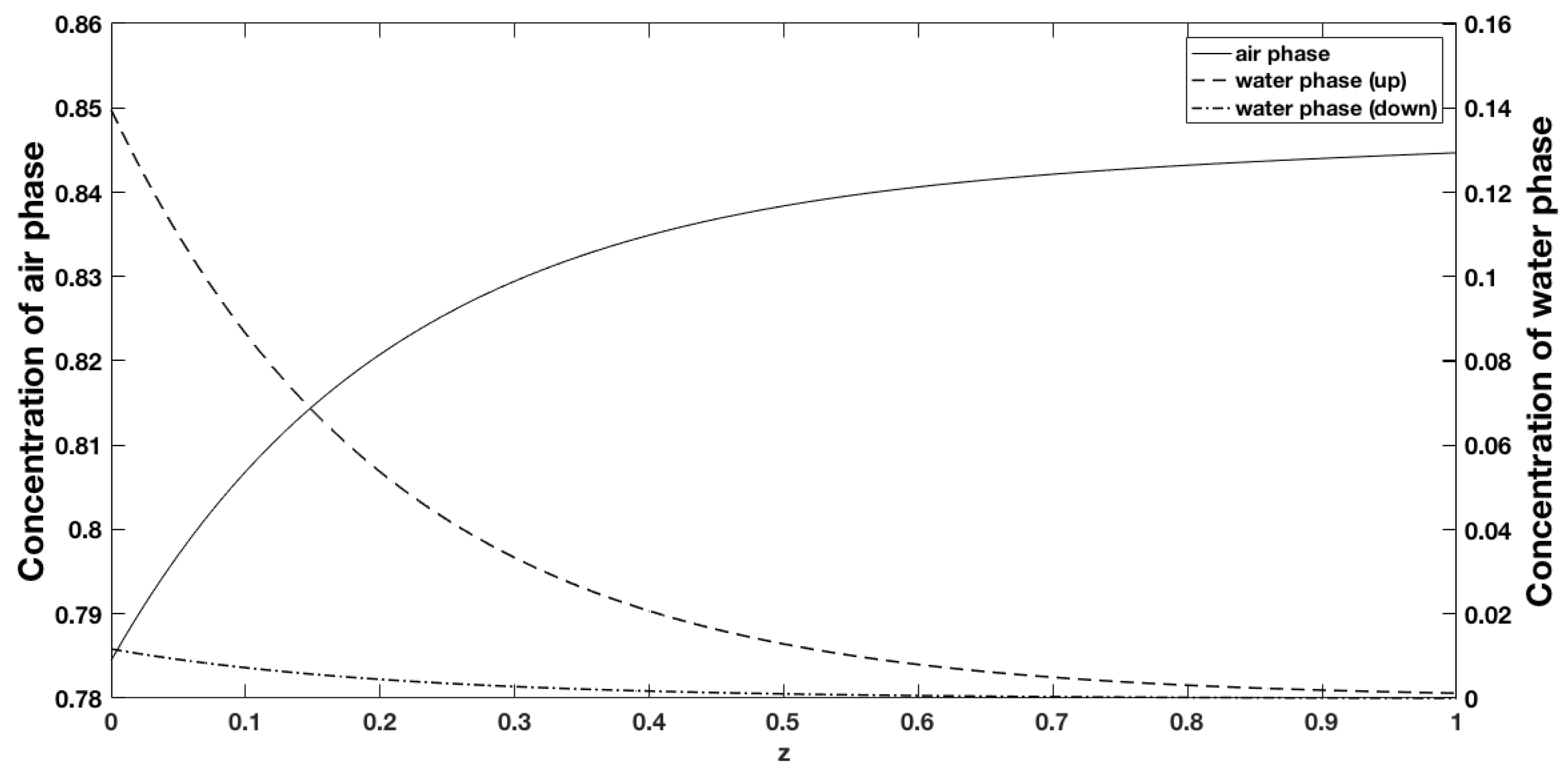
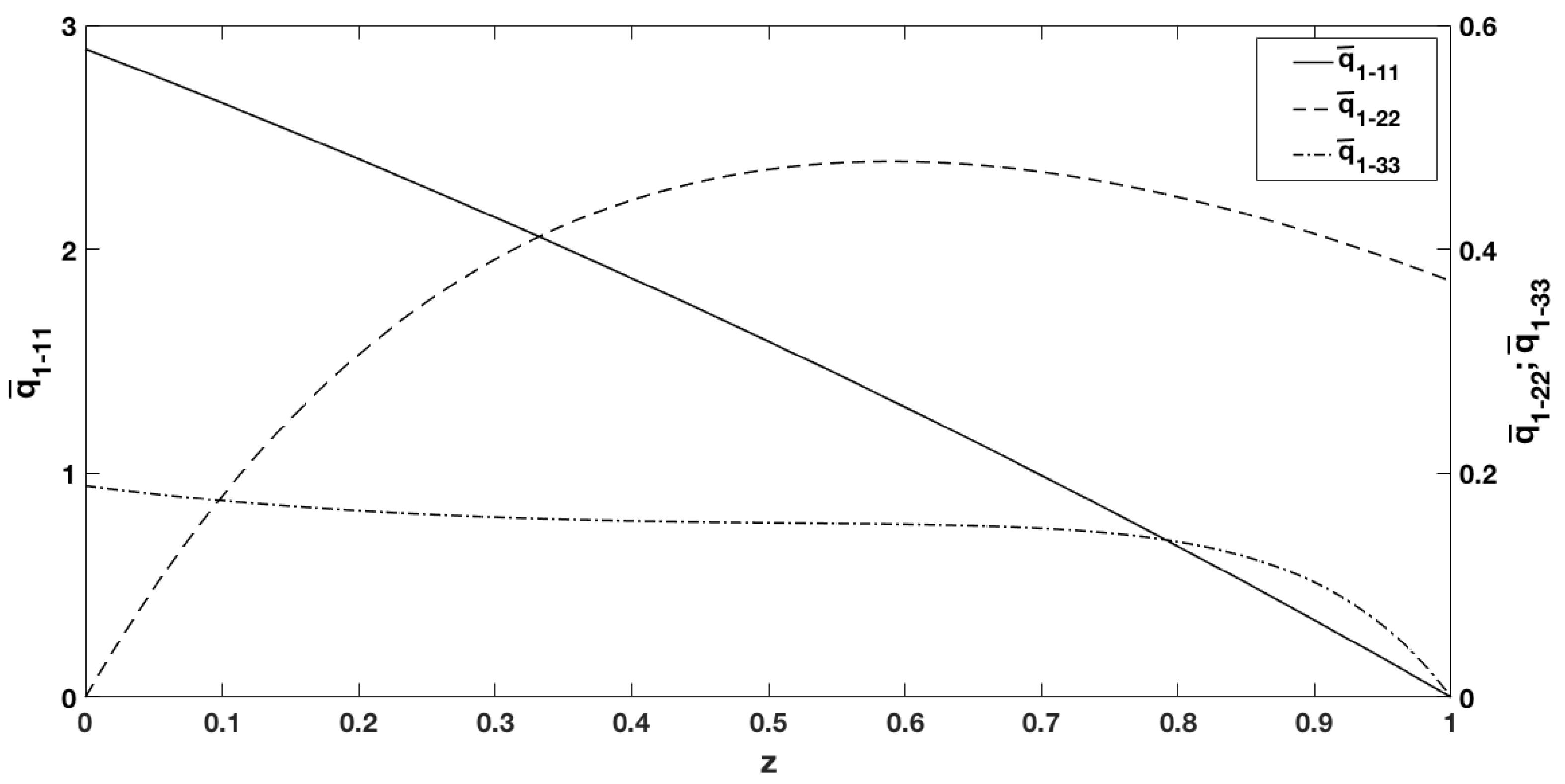
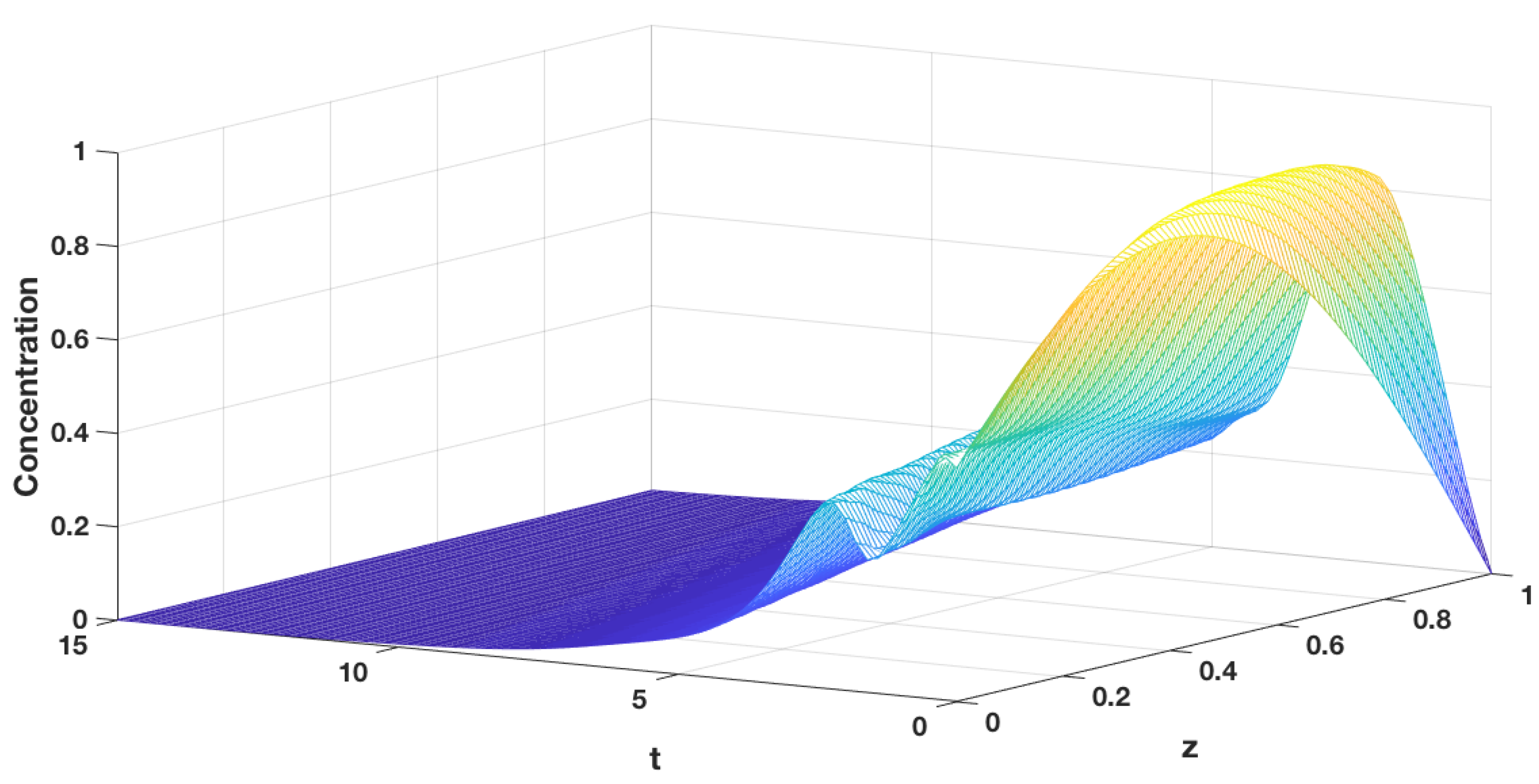
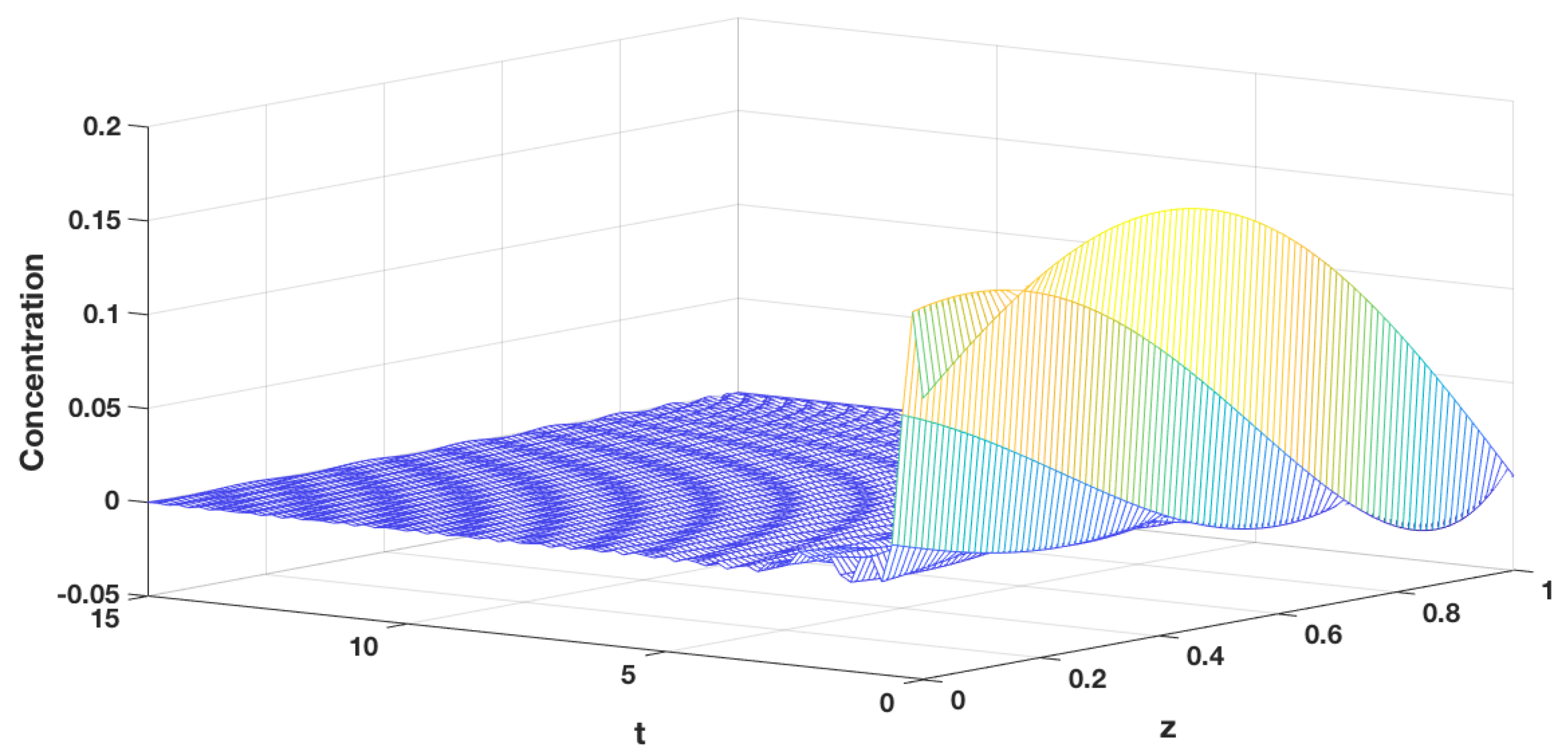
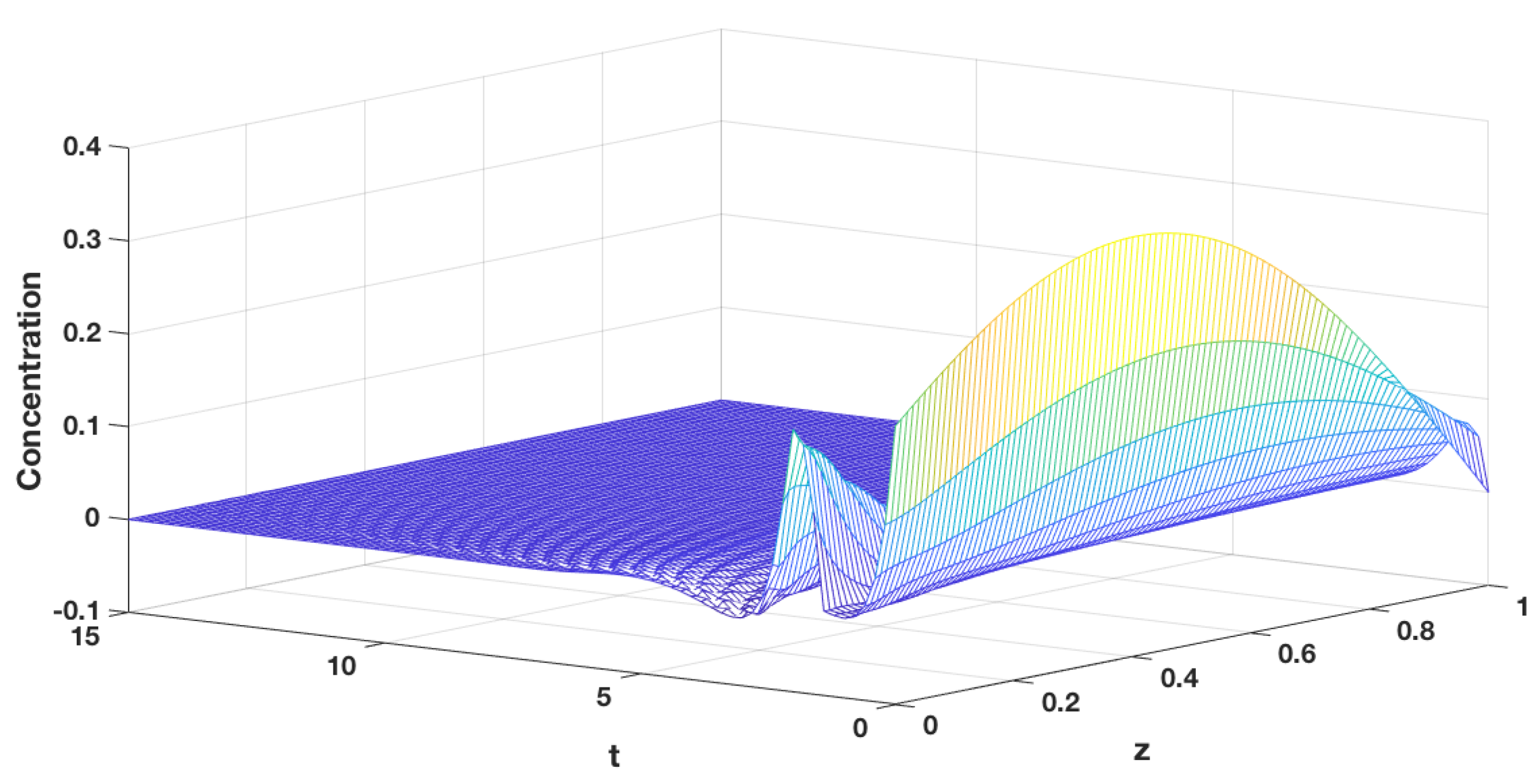
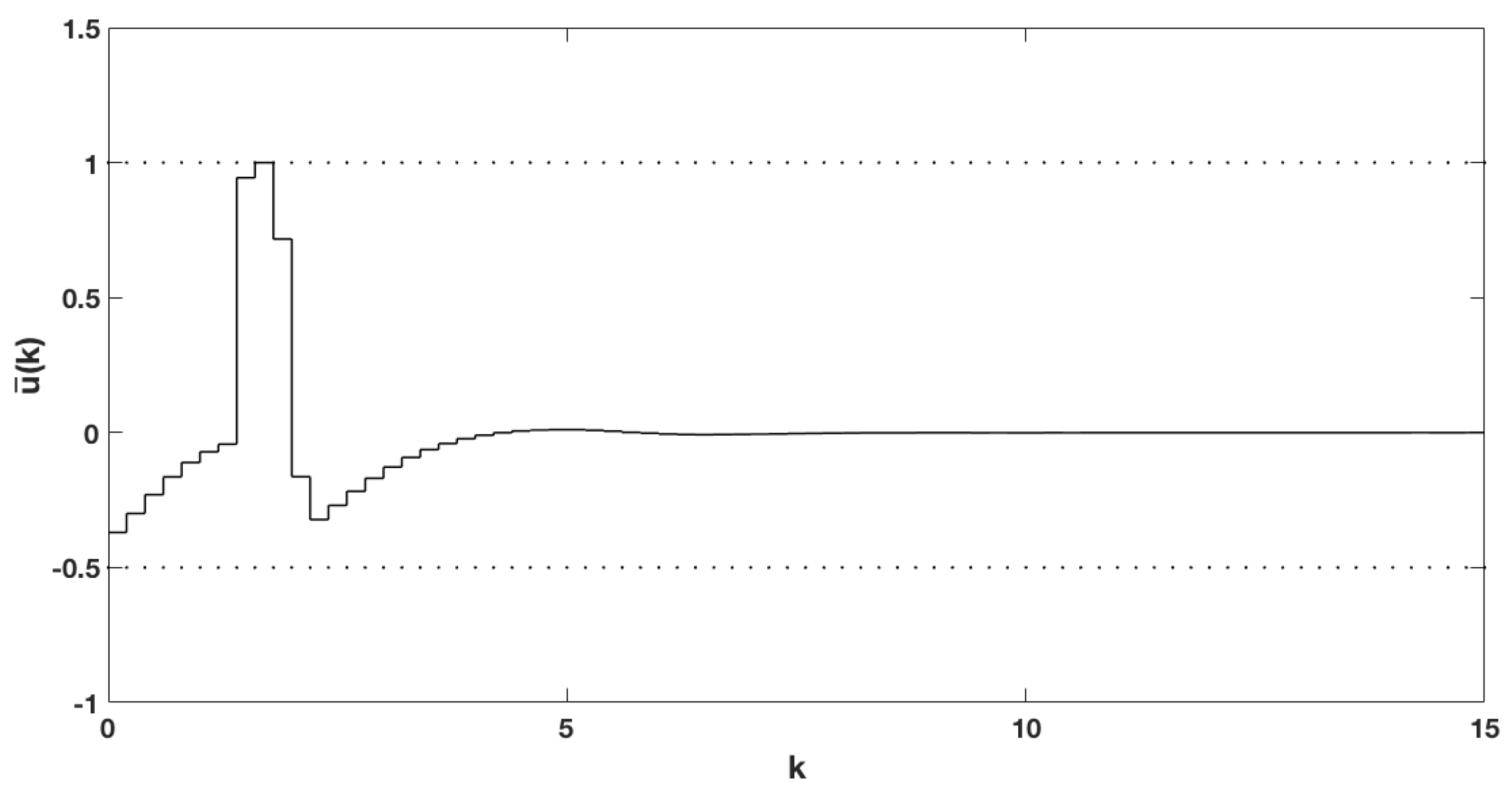
4. Simulation Results
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A. Resolution of Operator A for Discretized Model
References
- Finch, J.; Dobby, G. Column Flotation; Pergamon Press: Oxford, UK, 1990. [Google Scholar]
- Kawatra, S.K. Fundamental principles of froth flotation. In SME Mining Engineering Handbook; Society for Mining, Metallurgy, and Exploration: Englewood, IL, USA, 2009; pp. 1517–1531. [Google Scholar]
- Sbárbaro, D.; Del Villar, R. Advanced Control and Supervision of Mineral Processing Plants; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Mathieu, G. Comparison of flotation column with conventional flotation for concentration of a mo ore. Can. Min. Metall. Bull. 1972, 65, 41–45. [Google Scholar]
- Bergh, L.; Yianatos, J. The long way toward multivariate predictive control of flotation processes. J. Process Control 2011, 21, 226–234. [Google Scholar] [CrossRef]
- McKee, D. Automatic flotation control—A review of 20 years of effort. Miner. Eng. 1991, 4, 653–666. [Google Scholar] [CrossRef]
- Laurila, H.; Karesvuori, J.; Tiili, O. Strategies for instrumentation and control of flotation circuits. Miner. Process. Plant Des. Pract. Control 2002, 2, 2174–2195. [Google Scholar]
- Bouchard, J.; Desbiens, A.; del Villar, R.; Nunez, E. Column flotation simulation and control: An overview. Miner. Eng. 2009, 22, 519–529. [Google Scholar] [CrossRef]
- Bu, X.; Xie, G.; Peng, Y.; Chen, Y. Kinetic modeling and optimization of flotation process in a cyclonic microbubble flotation column using composite central design methodology. Int. J. Miner. Process. 2016, 157, 175–183. [Google Scholar] [CrossRef]
- Mavros, P.; Matis, K.A. Innovations in Flotation Technology; Springer Science & Business Media: Berlin/ Heidelberg, Germany, 2013; Volume 208. [Google Scholar]
- Del Villar, R.; Grégoire, M.; Pomerleau, A. Automatic control of a laboratory flotation column. Miner. Eng. 1999, 12, 291–308. [Google Scholar] [CrossRef]
- Persechini, M.A.M.; Peres, A.E.C.; Jota, F.G. Control strategy for a column flotation process. Control Eng. Pract. 2004, 12, 963–976. [Google Scholar] [CrossRef]
- Bergh, L.; Yianatos, J.; Leiva, C. Fuzzy supervisory control of flotation columns. Miner. Eng. 1998, 11, 739–748. [Google Scholar] [CrossRef]
- Bergh, L.; Yianatos, J.; Acuna, C.; Perez, H.; Lopez, F. Supervisory control at Salvador flotation columns. Miner. Eng. 1999, 12, 733–744. [Google Scholar] [CrossRef]
- Mohanty, S. Artificial neural network based system identification and model predictive control of a flotation column. J. Process Control 2009, 19, 991–999. [Google Scholar] [CrossRef]
- Maldonado, M.; Desbiens, A.; Del Villar, R. Potential use of model predictive control for optimizing the column flotation process. Int. J. Miner. Process. 2009, 93, 26–33. [Google Scholar] [CrossRef]
- Mayne, D.Q.; Rawlings, J.B.; Rao, C.V.; Scokaert, P.O. Constrained model predictive control: Stability and optimality. Automatica 2000, 36, 789–814. [Google Scholar] [CrossRef]
- Malinen, J. Tustin’s method for final state approximation of conservative dynamical systems. IFAC Proc. Vol. 2011, 44, 4564–4569. [Google Scholar] [CrossRef]
- Havu, V.; Malinen, J. Laplace and Cayley transforms—An approximation point of view. In Proceedings of the 44th IEEE Conference on Decision and Control, 2005 and 2005 European Control Conference ( CDC-ECC’05), Seville, Spain, 15 December 2005; pp. 5971–5976. [Google Scholar]
- Havu, V.; Malinen, J. The Cayley transform as a time discretization scheme. Numer. Funct. Anal. Opt. 2007, 28, 825–851. [Google Scholar] [CrossRef]
- Xu, Q.; Dubljevic, S. Linear model predictive control for transport-reaction processes. AIChE J. 2017, 63, 2644–2659. [Google Scholar] [CrossRef]
- Dobby, G.; Finch, J. Mixing characteristics of industrial flotation columns. Chem. Eng. Sci. 1985, 40, 1061–1068. [Google Scholar] [CrossRef]
- Yianatos, J.; Finch, J.; Laplante, A. Cleaning action in column flotation froths. Trans. Inst. Min. Metall. Sect. C-Miner. Process. Extract. Metall. 1987, 96, C199–C205. [Google Scholar]
- Franklin, G.F.; Powell, J.D.; Workman, M.L. Digital Control of Dynamic Systems; Addison-Wesley: Menlo Park, CA, USA, 1998; Volume 3. [Google Scholar]
- Xu, Q.; Dubljevic, S. Modelling and control of solar thermal system with borehole seasonal storage. Renew. Energy 2017, 100, 114–128. [Google Scholar] [CrossRef]
- Muske, K.R.; Rawlings, J.B. Model predictive control with linear models. AIChE J. 1993, 39, 262–287. [Google Scholar] [CrossRef]

| Symbol | Description | Value |
|---|---|---|
| h | Height of froth region | 1.0 |
| l | Height of collection region | 1.0 |
| Bubble saturation parameter | 5 | |
| Air–water interfacial area | C: 1.0; F: 0.3 | |
| Water velocity of collection region | 0.8 | |
| Settling/slip velocity | 1 | |
| Air holdup | C: 0.3; F: 0.7 | |
| Downward water holdup | 0.1 | |
| Upward water holdup | C: 0.7; F: 0.2 | |
| Air velocity | C: 0.1; F: 0.2 | |
| Downward water velocity | 0.1 | |
| Upward water velocity | C: 0.08; F: 0.1 | |
| Attachment-rate parameter for downward water | C: 1.2; F: 1.0 | |
| Attachment-rate parameter for upward water | 1.5 | |
| Detachment rate parameter | C: 0.1; F: 0 | |
| Transfer rate from upward water to downward water | 0.1 | |
| k | Transfer rate from air to downward water | 0.01 |
| , , | Initial-condition coefficients | , , |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, Y.; Luan, X.; Liu, F.; Dubljevic, S. Model Predictive Control of Mineral Column Flotation Process. Mathematics 2018, 6, 100. https://doi.org/10.3390/math6060100
Tian Y, Luan X, Liu F, Dubljevic S. Model Predictive Control of Mineral Column Flotation Process. Mathematics. 2018; 6(6):100. https://doi.org/10.3390/math6060100
Chicago/Turabian StyleTian, Yahui, Xiaoli Luan, Fei Liu, and Stevan Dubljevic. 2018. "Model Predictive Control of Mineral Column Flotation Process" Mathematics 6, no. 6: 100. https://doi.org/10.3390/math6060100
APA StyleTian, Y., Luan, X., Liu, F., & Dubljevic, S. (2018). Model Predictive Control of Mineral Column Flotation Process. Mathematics, 6(6), 100. https://doi.org/10.3390/math6060100







