1. Introduction
One of the main applications of homological algebra is the classical cohomology of associative algebras invented by Hochschild [
1] in 1945. It is a particular case of general machinery developed by Cartan and Eilenberg. Let
A be an associative
k-algebra and let
M be an
A-
A-bimodule. The low dimensional groups (
n2) have well known interpretations of classical algebraic structures such as derivations and extensions [
2,
3,
4].
Let
G be a group and
be a group homomorphism. An
oriented algebra is an associative algebra
A equipped with a
G-module structure
, satisfying the condition
Hence, oriented algebras are more general than G-algebras as well as algebras with involutions. The aim of this work is to prove that classifies the singular extensions of oriented algebras. The construction is based on the possibility of mixing the standard chain complexes computing group with associative algebra cohomologies. We obtain a new bicomplex by deleting the first column and the first row and reindexing.
2. Preliminaries
In this section we fix some notations for the standard chain complexes associated to groups and associative algebras. We also introduce a bicomplex which we will use throughout the paper.
In what follows,
k denotes a ground commutative ring with the unit. All modules and algebras are considered over
k. Moreover, we write ⊗ and
instead of
and
. For a group
G and
G-module
C, we let
denote the standard complex computing the group cohomology. Recall that
and the coboundary map
is given by
Therefore, by the definition
we will say that a cochain complex
is a
G-
if each module
is endowed with a structure of
G-module and each boundary is a
G-homomorphism. If this is the case, we let
be the total complex of the following bicomplex:
![Mathematics 06 00237 i001]()
The cohomology of is denoted by and is called the - of G with coefficients in .
Let
A be an associative
k-algebra. Recall that the Hochschild cohomology of
A with coefficients in a
A-bimodule
M is the cohomology of the following cochain complex:
where the coboundary map
is given by
Hence, , where .
3. Oriented Algebras
In this section, we define oriented algebras and provide some examples.
Definition 1. An orientation is a pair [5], where G is a group, and ε is a group homomorphismIf such orientation is fixed, then we say that G is an oriented group. Example 1. - (1)
Any group G can be equipped with a trivial orientation: for all .
- (2)
For more interesting examples, we can consider the following:
- (a)
and ;
- (b)
more generally, we can consider and ;
- (c)
and , , , ;
Definition 2. Let G be an oriented group and A be an associative algebra [5]. An oriented action of on A is given by a mapsuch that under this action A is a G-module and An oriented algebra over is an associative algebra equipped with an oriented action of on A.
Example 2. - (1)
Observe that if G is equipped with a trivial orientation, then G acts on A via algebra automorphisms; therefore, in this case oriented algebra is nothing but a G-algebra in the classical sense.
- (2)
Another interesting example is obtained when and . In this case A is nothing but involutive algebra. Recall that an involutive algebra is an associative algebra A together with a k-linear mapsuch that - (3)
Let M be a G-module. Consider the tensor algebra Define an action of G on by One checks that this action on the tensor algebra defines an oriented algebra structure.
Definition 3. Let A and B be oriented algebras over an oriented group [5]. A homomorphism of G-modules is called a homomorphism of oriented algebras provided f is a homomorphism of algebras. 4. Oriented Bimodules and Cohomology
Definition 4. Let A be an oriented algebra over an oriented group [5]. An oriented bimodule over A is a usual bimodule X together with a G-module structure on X such that If X and Y are oriented bimodules over an oriented algebra A, then a linear map is a homomorphisms of oriented bimodules iffor all , , and . Let A be an oriented algebra over an oriented group
and let
X be an oriented bimodule. For any
one defines an action of
G on
by
In particular, for the action is independent on the parity of .
Lemma 1. With the action of Equation (2) [5], the Hochschild complexis a G-complex. Thus, one can form the following bicomplex
:
![Mathematics 06 00237 i002]()
where the coboundary maps are given as follows:
The coboundary of every horizontal maps
is given by
The coboundary of the first vertical maps is given by
The coboundary of the second vertical maps when
is given by
The coboundary of the third vertical maps when
is given by
The coboundary of the third vertical maps when
is given by
Definition 5. The homologies of the total complex is denoted by where [5]. Example 3. - (1)
Let A be separable k-algebra. Since for , then we obtain .
- (2)
Let G be a cyclic group of order 2, , and d be an integer which is square-free:so thatNow, let . We define an action of G on A bywhereHence, we obtain the following bicomplex:![Mathematics 06 00237 i003]() where as an abelian group is X, but to make difference between X and for an element , we write , the corresponding element in . The action of G on is given byThe coboundary maps of and are given as follows:
where as an abelian group is X, but to make difference between X and for an element , we write , the corresponding element in . The action of G on is given byThe coboundary maps of and are given as follows: For one has
Now, let and by computing explicitly the cohomology of the total complex when we obtain the following:Similarly, by computing explicitly the cohomology of the total complex when we obtain the following:
Now, we will form a new double complex , which is obtained by deleting the first column and the first row and reindexing.![Mathematics 06 00237 i004]()
Definition 6. The homologies of the total complex of is denoted by where [5]. 5. Classification of Singular Extensions of Oriented Algebras
In [
5], we proved that
classifies the singular extensions of oriented algebras. Here we obtain a similar result.
Definition 7. Let A be an oriented algebra over an oriented group [5]. Moreover, let X be an oriented bimodule over A. A singular extension of A by X is a k-split short exact sequence of G-moduleswhere B is also an oriented algebra over . Furthermore, p is a homomorphism of oriented algebras and i is homomorphism of G-modules such thatfor all and . Theorem 1. Let A be an oriented algebra over an oriented group . Moreover, let X be an oriented bimodule over A. Then there is one-to-one correspondence between equivalence classes of extensions of A by X and .
Before giving the proof, observe that
, where
is the collection of pairs
. Here
and
, satisfying the following conditions:
Observe that the last equality simply states that
is a
G-Hochschild 2-cocycle. Moreover,
if and only if there exists
such that
and
Proof. Let us start with a singular extension as above. To simplify the notation we will assume that
X is a submodule of
B and
. Choose a linear map
such that
. One defines
and
by
and
We claim that
. By the classical argument,
is a
G-Hochschild 2-cocycle. Next, we have
To obtain the remaining equations, we have to consider two cases. If
we have, from Equation (
3),
and from Equation (
4) we have
Comparing these expressions, we see that
By replacing
and
in Equation (
6), we have
Similarly, if
, from Equation (
3), we have
and from Equation (
4) we have
Comparing these expressions, we see that
By replacing
and
in Equation (
8), we have
Hence, we show that in fact .
Conversely, starting with
, one can define
where the multiplication is given by
and
We claim that
B satisfies all properties of oriented algebra and defines an extension. Since
is a
G-Hochschild 2-cocycle, then
B is clearly an associative algebra. Therefore, we only check Equation (
1). There are two cases to consider:
and
. Firstly, we deal with the first case, when
. We have
and
Therefore, from Equation (
7), it follows that
Next, we deal with the second case, when
. We have
and
Therefore, from Equation (
9), it follows that
Thus one obtains an inverse map from the cohomology to extensions. ☐
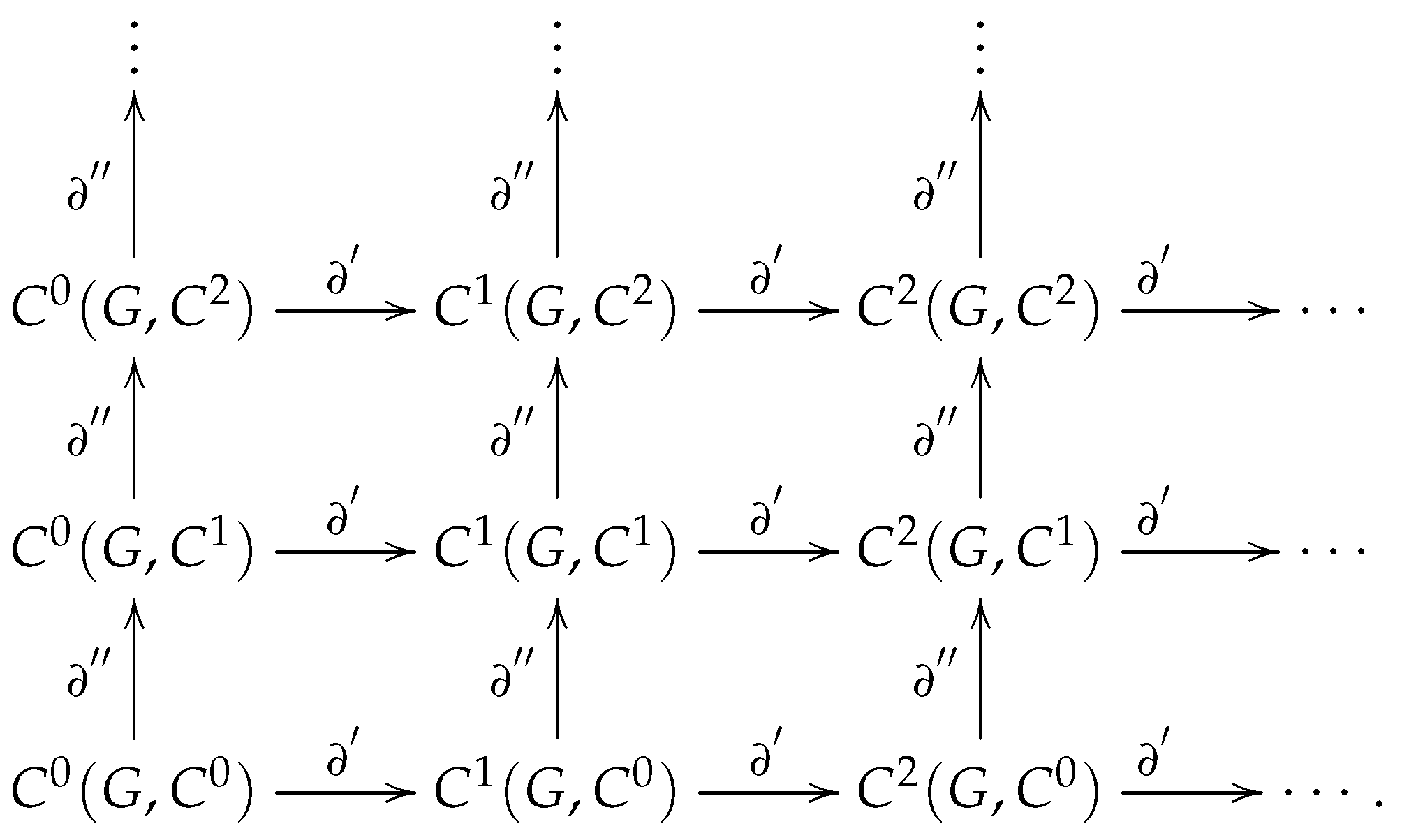
 where the coboundary maps are given as follows:
where the coboundary maps are given as follows:where as an abelian group is X, but to make difference between X and for an element , we write , the corresponding element in . The action of G on is given by
The coboundary maps of and are given as follows:




