Nonequilibrium Molecular Velocity Distribution Functions Predicted by Macroscopic Gas Dynamic Models †
Abstract
1. Introduction
2. Molecular Velocity Distribution Function Approximations
3. Results
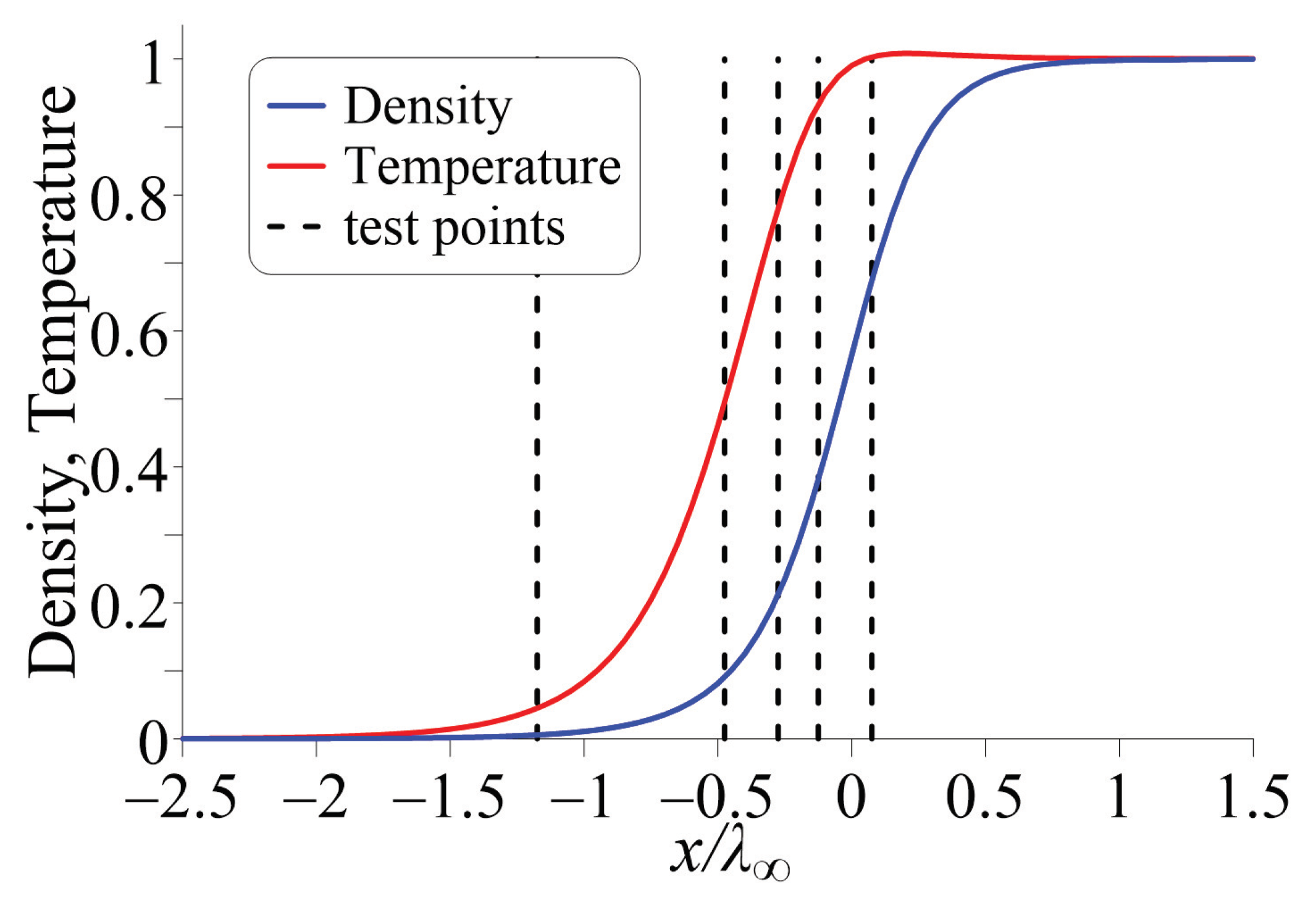
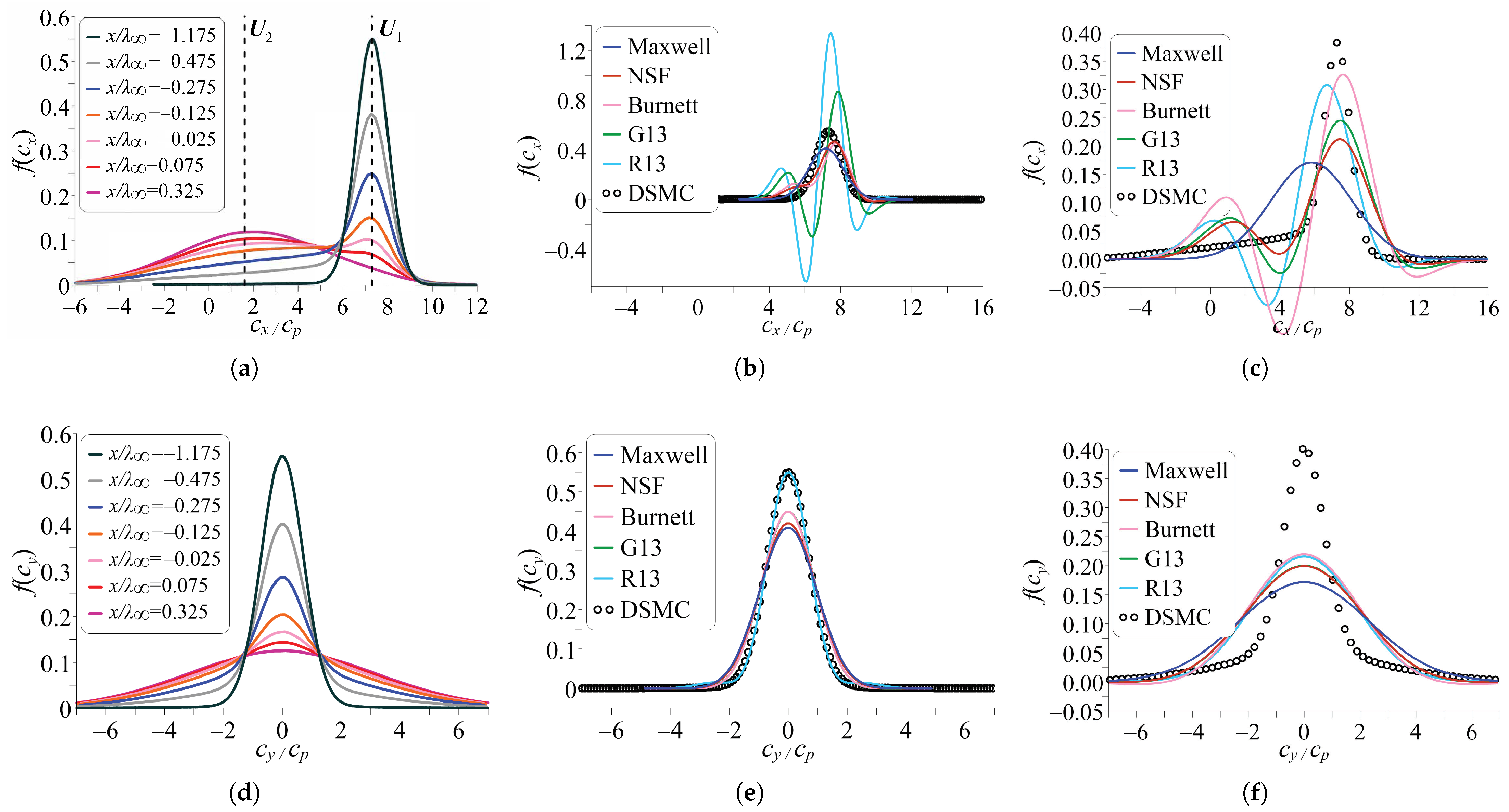
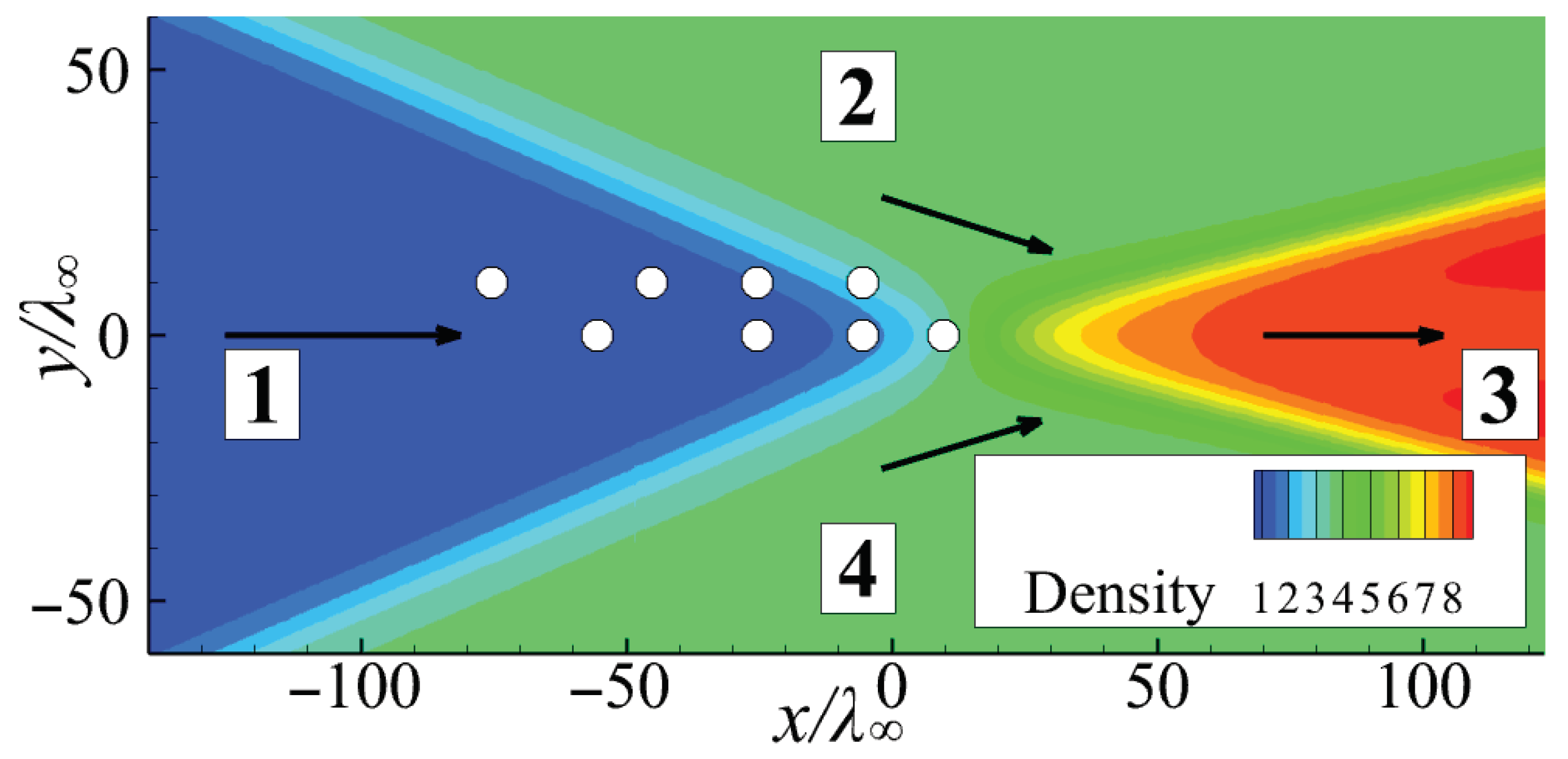
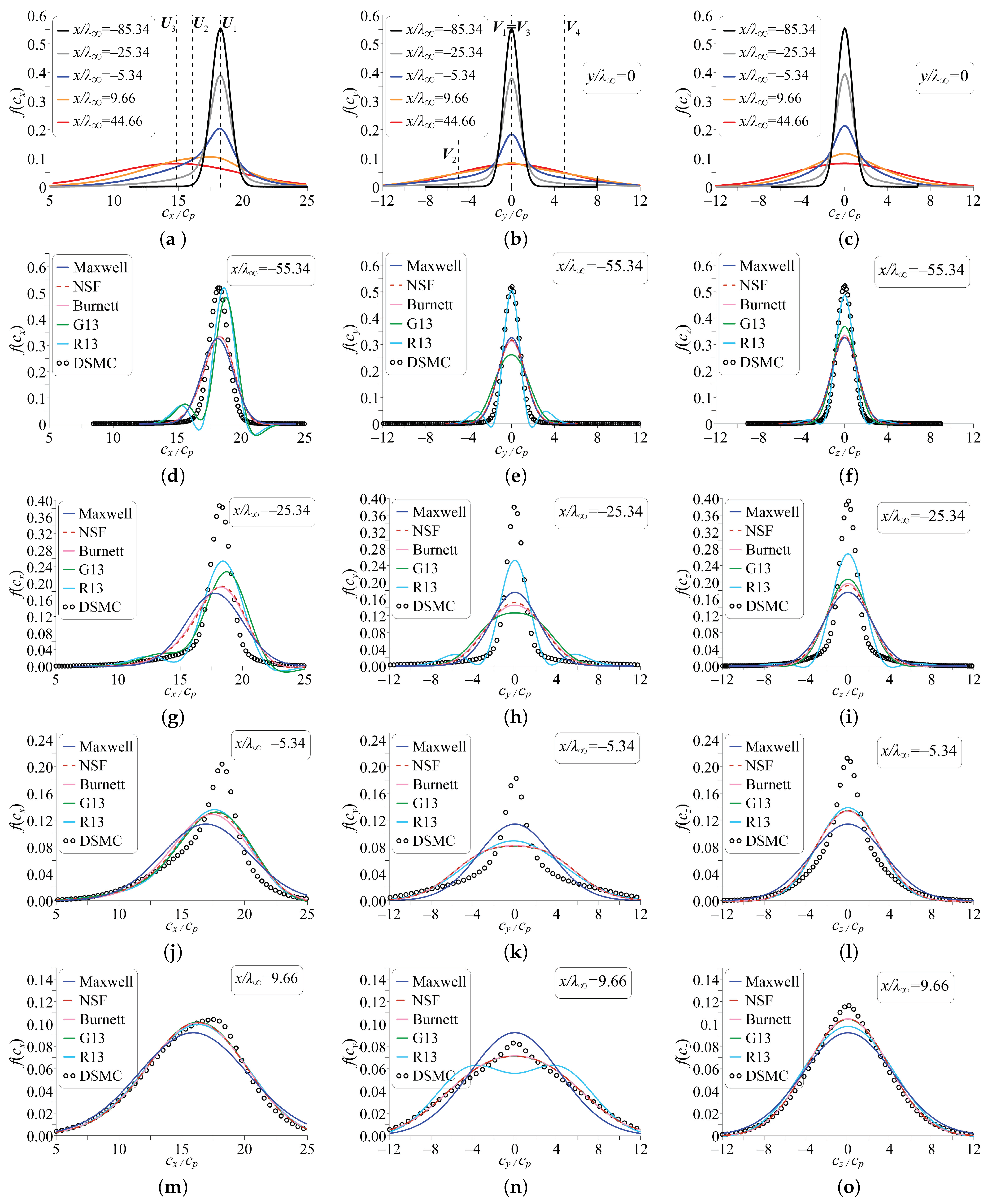
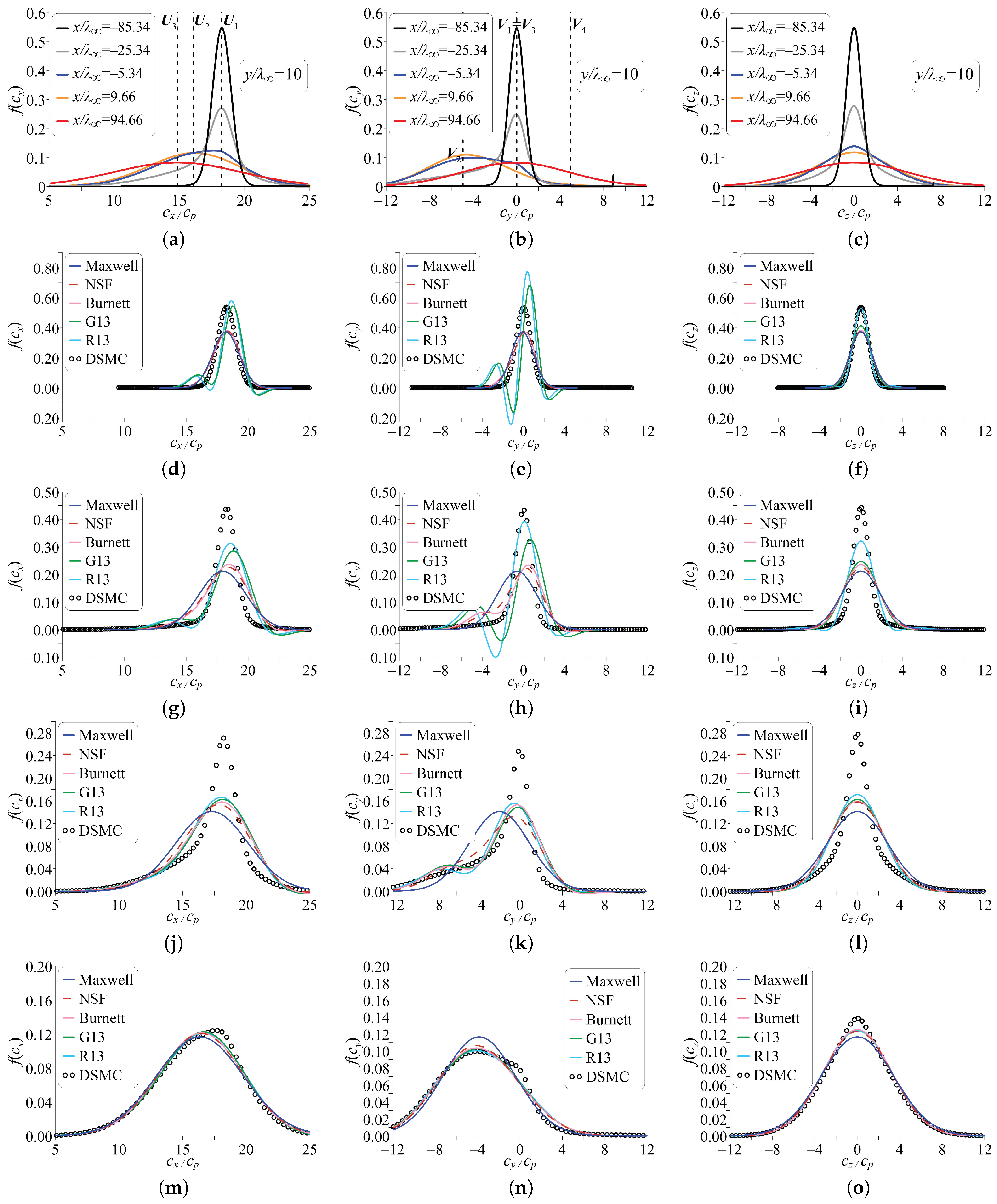
3.1. Shock Wave Structure
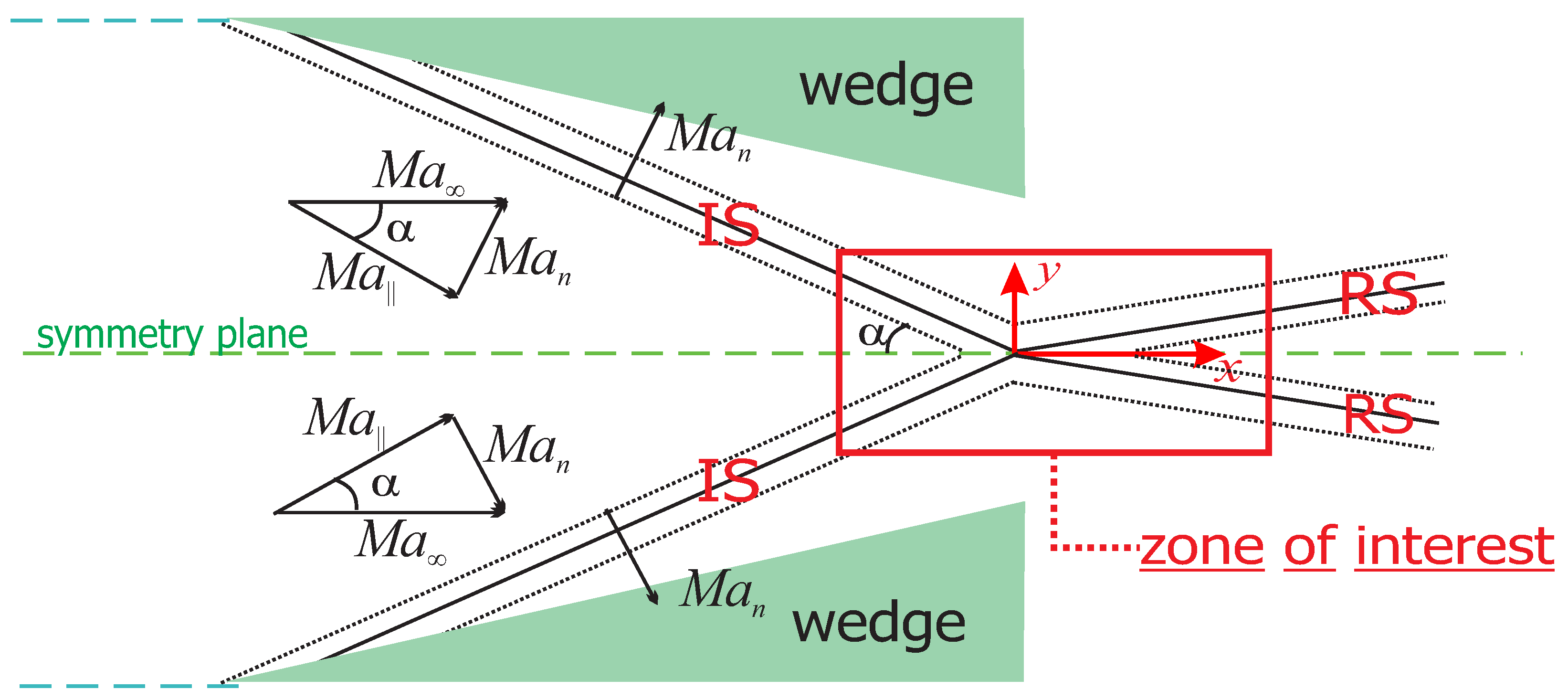
3.2. Regular Reflection of Shock Waves
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cercignani, C. The Boltzmann Equation and Its Applications; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]
- Sitnikov, S.; Tcheremissine, F. A method for numerical simulation of shock waves in rarefied gas mixtures based on direct solution of the Boltzmann kinetic equation. J. Comput. Phys. 2025, 520, 113463. [Google Scholar] [CrossRef]
- Wu, L.; Zhang, J.; Reese, J.M.; Zhang, Y. A fast spectral method for the Boltzmann equation for monatomic gas mixtures. J. Comput. Phys. 2015, 298, 602–621. [Google Scholar] [CrossRef]
- Bird, G.A. Molecular Gas Dynamics and the Direct Simulation of Gas Flows; Clarendon Press: Oxford, UK, 1994. [Google Scholar]
- Boyd, I.D.; Schwartzentruber, T.E. Nonequilibrium Gas Dynamics and Molecular Simulation; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Ivanov, M.S.; Gimelshein, S.F. Computational Hypersonic Raredied Flows. Annu. Rev. Fluid Mech. 1998, 30, 469–505. [Google Scholar] [CrossRef]
- Struchtrup, H. Macroscopic Transport Equations for Rarefied Gas Flows; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Torrilhon, M. Modeling Nonequilibrium Gas Flow Based on Moment Equations. Annu. Rev. Fluid Mech. 2016, 48, 429–458. [Google Scholar] [CrossRef]
- Kogan, M.N. Rarefied Gas Dynamics; Plenum: New York, NY, USA, 1969. [Google Scholar]
- Chapman, S.; Cowling, T.G. The Mathematical Theory of Non-Uniform Gases: An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases; Cambridge Mathematical Library: Cambridge, UK, 1991. [Google Scholar]
- Li, Q.; Zeng, J.; Wu, L. Kinetic modelling of rarefied gas mixtures with disparate mass in strong non-equilibrium flows. J. Fluid Mech. 2024, 1001, A5. [Google Scholar] [CrossRef]
- Grad, H. On the kinetic theory of rarefied gases. Commun. Pure Appl. Math. 1949, 2, 331–407. [Google Scholar] [CrossRef]
- Pham-Van-Diep, G.C.; Erwin, D.A.; Muntz, E.P. Testing continuum descriptions of low-Mach-number shock structures. J. Fluid Mech. 1991, 232, 403–413. [Google Scholar] [CrossRef]
- Erofeev, A.I.; Friedlander, O.G. Macroscopic Models for Nonequilibrium Flows of Monatomic Gas and Normal Solutions. In Proceedings of the 25th International Symposium on Rarefied Gas Dynamics, Saint-Petersburg, Russia, 18–21 July 2007; pp. 117–124. [Google Scholar]
- Ivanov, I.E.; Timokhin, M.Y.; Kryukov, I.A.; Bondar, Y.A.; Kokhanchik, A.A.; Ivanov, M.S. Study of the Shock Wave Structure by Regularized Grad’s Set of Equations. AIP Conf. Proc. 2012, 1501, 215–222. [Google Scholar] [CrossRef]
- Westerkamp, A.; Torrilhon, M. Finite element methods for the linear regularized 13-moment equations describing slow rarefied gas flows. J. Comput. Phys. 2019, 389, 1–21. [Google Scholar] [CrossRef]
- Matsubara, H.; Kikugawa, G.; Ohara, T. All- and one-particle distribution functions at nonequilibrium steady state under thermal gradient. Phys. Rev. E 2019, 99, 052110. [Google Scholar] [CrossRef]
- Han, Q.; Xia, H.; Chen, W.; Wu, G.; Li, J.; Wei, Z.; Zheng, F.; Ma, C. Non-equilibrium molecular motion and interface heat transfer in supersonic rarefied flows. Phys. Fluids 2025, 37, 037132. [Google Scholar] [CrossRef]
- Zarei, A.; Karimipour, A.; Meghdadi Isfahani, A.H.; Tian, Z. Improve the performance of lattice Boltzmann method for a porous nanoscale transient flow by provide a new modified relaxation time equation. Phys. A Stat. Mech. Its Appl. 2019, 535, 122453. [Google Scholar] [CrossRef]
- Bondar, Y.A.; Shoev, G.V.; Timokhin, M.Y. Regular reflection of shock waves in steady flows: Viscous and non-equilibrium effects. J. Fluid Mech. 2024, 984, A10. [Google Scholar] [CrossRef]
- Timokhin, M.Y.; Struchtrup, H.; Kokhanchik, A.A.; Bondar, Y.A. The analysis of different variants of R13 equations applied to the shock-wave structure. AIP Conf. Proc. 2016, 1786, 140006. [Google Scholar] [CrossRef]
- Zheng, Y.; Struchtrup, H. Burnett equations for the ellipsoidal statistical BGK model. Contin. Mech. Thermodyn. 2004, 16, 97–108. [Google Scholar] [CrossRef]
- Gu, X.J.; Emerson, D.R. A high-order moment approach for capturing non-equilibrium phenomena in the transition regime. J. Fluid Mech. 2009, 636, 177–216. [Google Scholar] [CrossRef]
- Shevyrin, A.; Bondar, Y.; Ivanov, M. Analysis of Repeated Collisions in the DSMC Method. AIP Conf. Proc. 2005, 762, 565–570. [Google Scholar] [CrossRef]
- Ivanov, M.; Markelov, G.; Gimelshein, S. Statistical simulation of reactive rarefied flows—Numerical approach and applications. In Proceedings of the 7th AIAA/ASME Joint Thermophysics and Heat Transfer Conference (AIAA 1998–2669), Albuquerque, NM, USA, 15–18 June 1998; p. 2669. [Google Scholar] [CrossRef]
- Ivanov, M.S.; Kashkovsky, A.V.; Vashchenkov, P.V.; Bondar, Y.A. Parallel Object-Oriented Software System for DSMC Modeling of High-Altitude Aerothermodynamic Problems. AIP Conf. Proc. 2011, 1333, 211–218. [Google Scholar] [CrossRef]
- Becker, R. Stoßwelle und Detonation. Z. Physik. 1922, 8, 321–362. [Google Scholar] [CrossRef]
- Mott-Smith, H.M. The Solution of the Boltzmann Equation for a Shock Wave. Phys. Rev. 1951, 82, 885–892. [Google Scholar] [CrossRef]
- Yen, S.M. Temperature Overshoot in Shock Waves. Phys. Fluids 1966, 9, 1417–1418. [Google Scholar] [CrossRef]
- Xu, K.; Huang, J.C. A unified gas-kinetic scheme for continuum and rarefied flows. J. Comput. Phys. 2010, 229, 7747–7764. [Google Scholar] [CrossRef]
- Ohwada, T. Structure of normal shock waves: Direct numerical analysis of the Boltzmann equation for hard-sphere molecules. Phys. Fluids A 1993, 5, 217–234. [Google Scholar] [CrossRef]
- Zhang, Y.D.; Xu, A.G.; Zhang, G.C.; Chen, Z.H.; Wang, P. Discrete ellipsoidal statistical BGK model and Burnett equations. Front. Phys. 2018, 13, 135101. [Google Scholar] [CrossRef]
- Zhu, Q.; Wu, Y.; Zhou, W.; Yang, Q.; Xu, X. A comprehensive study on the roles of viscosity and heat conduction in shock waves. J. Fluid Mech. 2024, 984, A74. [Google Scholar] [CrossRef]
- Hansen, K.; Hornig, D.F. Thickness of Shock Fronts in Argon. J. Chem. Phys. 1960, 33, 913–916. [Google Scholar] [CrossRef]
- Alsmeyer, H. Density Profiles in Argon and Nitrogen Shock Waves Measured by the Absorption of an Electron Beam. J. Fluid Mech. 1976, 74, 497–513. [Google Scholar] [CrossRef]
- Pham-Van-Diep, G.; Erwin, D.; Muntz, E.P. Nonequilibrium Molecular Motion in a Hypersonic Shock Wave. Science 1989, 245, 624–626. [Google Scholar] [CrossRef]
- Rankine, W.J.M. On the thermodynamic theory of waves of finite longitudinal disturbance. Philos. Trans. R. Soc. Lond. 1870, 160, 277–288. [Google Scholar] [CrossRef]
- Lockerby, D.A.; Reese, J.M.; Struchtrup, H. Switching criteria for hybrid rarefied gas flow solvers. Proc. R. Soc. Math. Phys. Eng. Sci. 2009, 465, 1581–1598. [Google Scholar] [CrossRef]
- Su, X.; Lin, C. Nonequilibrium effects of reactive flow based on gas kinetic theory. Commun. Theor. Phys. 2022, 74, 035604. [Google Scholar] [CrossRef]
- Lin, C.; Su, X.; Zhang, Y. Hydrodynamic and Thermodynamic Nonequilibrium Effects around Shock Waves: Based on a Discrete Boltzmann Method. Entropy 2020, 22, 1397. [Google Scholar] [CrossRef] [PubMed]
- Timokhin, M.; Rukhmakov, D. Local non-equilibrium phase density reconstruction with Grad and Chapman-Enskog methods. J. Phys. Conf. Ser. 2021, 1959, 012049. [Google Scholar] [CrossRef]
- Cai, Z.; Torrilhon, M. On the Holway-Weiss debate: Convergence of the Grad-moment-expansion in kinetic gas theory. Phys. Fluids 2019, 31, 126105. [Google Scholar] [CrossRef]
- Cover, T.M.; Thomas, J.A. Elements of Information Theory, 2nd ed.; Wiley-Interscience: Hoboken, NJ, USA, 2006. [Google Scholar]
- Villani, C. Optimal Transport: Old and New; Grundlehren der mathematischen Wissenschaften; Springer: Berlin/Heidelberg, Germany, 2008; Volume 338. [Google Scholar]
- Khotyanovsky, D.; Bondar, Y.; Kudryavtsev, A.; Shoev, G.; Ivanov, M. Viscous effects in steady reflection of strong shock waves. AIAA J. 2009, 47, 1263–1269. [Google Scholar] [CrossRef]
- Gan, Y.; Zhuang, Z.; Yang, B.; Xu, A.; Zhang, D.; Chen, F.; Song, J.; Wu, Y. Supersonic flow kinetics: Mesoscale structures, thermodynamic nonequilibrium effects and entropy production mechanisms. arXiv 2025, arXiv:2502.10832. [Google Scholar]
- Bondar, Y.; Shoev, G.; Kokhanchik, A.; Timokhin, M. Nonequilibrium velocity distribution in steady regular shock-wave reflection. AIP Conf. Proc. 2019, 2132, 120005. [Google Scholar] [CrossRef]
- Timokhin, M.Y.; Kudryavtsev, A.N.; Bondar, Y.A. The Mott-Smith solution to the regular shock reflection problem. J. Fluid Mech. 2022, 950, A14. [Google Scholar] [CrossRef]
- Müller, I.; Ruggeri, T. Rational Extended Thermodynamics; Springer: New York, NY, USA, 1998. [Google Scholar] [CrossRef]
- Bobylev, A.V. Instabilities in the Chapman-Enskog Expansion and Hyperbolic Burnett Equations. J. Stat. Phys. 2006, 124, 371. [Google Scholar] [CrossRef]
- Shakhov, E. The Method of Investigation of Motion of a Rarefied Gas; Nauka: Moscow, Russia, 1974. (In Russian) [Google Scholar]

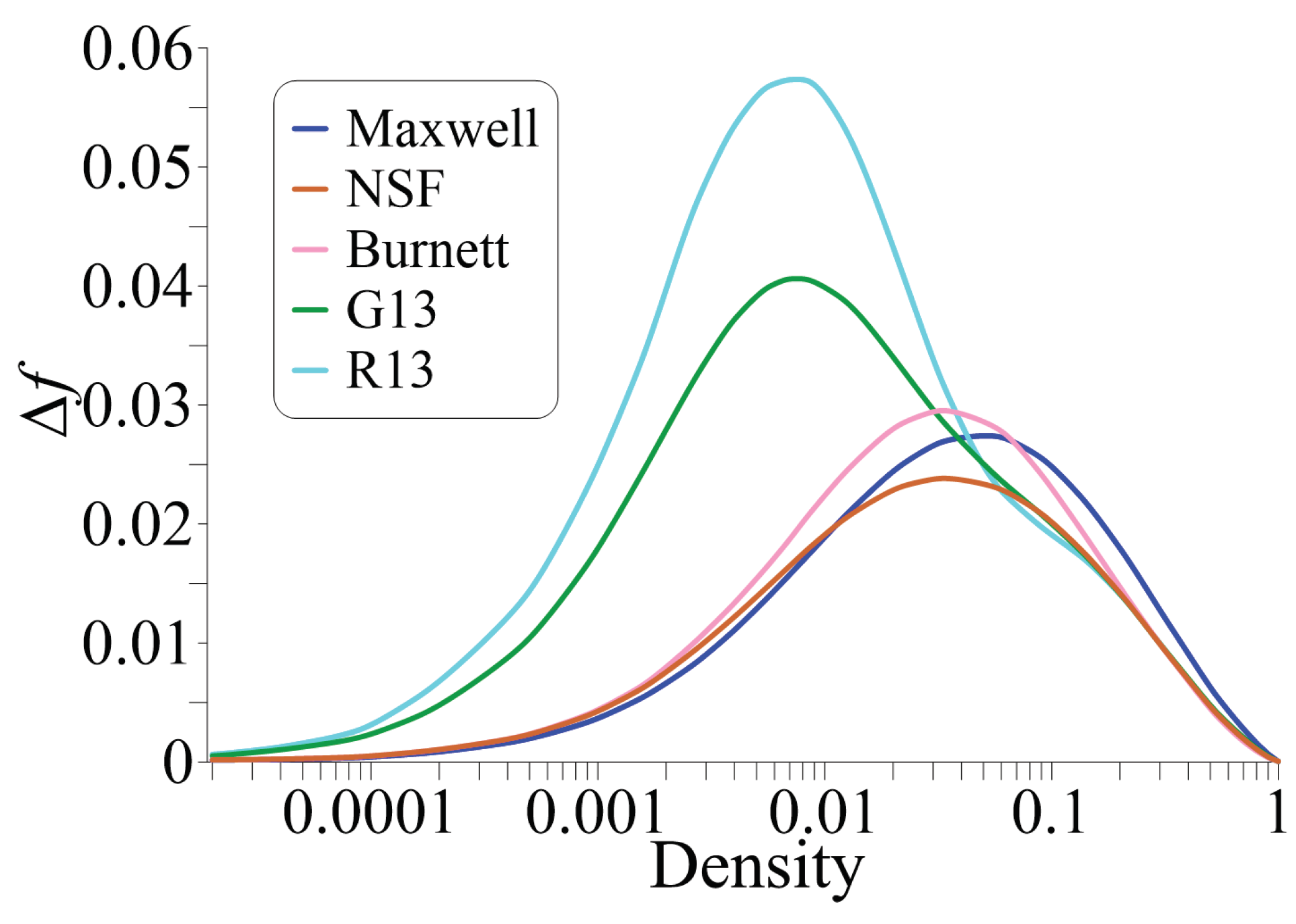
| Maxwell | NSF | Burnett | G13 | R13 | |
|---|---|---|---|---|---|
| 0.0001 | 0.00043 | 0.00051 | 0.00051 | 0.00239 | 0.00302 |
| 0.001 | 0.00370 | 0.00429 | 0.00440 | 0.01803 | 0.02419 |
| 0.01 | 0.01898 | 0.01919 | 0.02252 | 0.03986 | 0.05394 |
| 0.1 | 0.02488 | 0.02018 | 0.02303 | 0.01999 | 0.01911 |
| 1 | 0.00000 | 0.00000 | 0.00000 | 0.00000 | 0.00000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Timokhin, M.; Bondar, Y. Nonequilibrium Molecular Velocity Distribution Functions Predicted by Macroscopic Gas Dynamic Models. Mathematics 2025, 13, 1328. https://doi.org/10.3390/math13081328
Timokhin M, Bondar Y. Nonequilibrium Molecular Velocity Distribution Functions Predicted by Macroscopic Gas Dynamic Models. Mathematics. 2025; 13(8):1328. https://doi.org/10.3390/math13081328
Chicago/Turabian StyleTimokhin, Maksim, and Yevgeniy Bondar. 2025. "Nonequilibrium Molecular Velocity Distribution Functions Predicted by Macroscopic Gas Dynamic Models" Mathematics 13, no. 8: 1328. https://doi.org/10.3390/math13081328
APA StyleTimokhin, M., & Bondar, Y. (2025). Nonequilibrium Molecular Velocity Distribution Functions Predicted by Macroscopic Gas Dynamic Models. Mathematics, 13(8), 1328. https://doi.org/10.3390/math13081328






