Stability of the Additive Splitting Methods for the Generalized Nonlinear Schrödinger Equation
Abstract
1. Split-Step Methods
1.1. Multiplicative Methods
1.2. Additive Methods
1.3. The Root Condition
2. Model Equation
2.1. GNLSE Splitting
2.2. Modulation Instability
2.3. Split-Step Framework for Modulation Instability
3. Applications
3.1. Lie–Trotter Splitting
3.2. The Second Strang Splitting
3.3. Burstein and Mirin Splitting
3.4. ARBBC Splitting
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ARBBC | the additive splitting given by Equation (11) |
| FWM | four wave mixing |
| GNLSE | generalized nonlinear Schrödinger equation |
| MI | modulation instability |
| NLSE | nonlinear Schrödinger equation |
| SVEA | slowly varying envelope approximation |
Appendix A

References
- McLachlan, R.; Quispel, R. Splitting methods. Acta Numer. 2002, 11, 341–434. [Google Scholar] [CrossRef]
- Hundsdorfer, W.; Verwer, J. Numerical Solution of Time-Dependent Advection-Diffusion-Reaction Equations; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Hairer, E.; Lubich, C.; Wanner, G. Geometric Numerical Integration. Structure-Preserving Algorithms for Ordinary Differential Equations, 2nd ed.; Springer Series in Computational Mathematics; Springer: Berlin/Heidelberg, Germany, 2006; Volume 31. [Google Scholar]
- Holden, H.; Karlsen, K.; Lie, K.A.; Risebro, H. Splitting Methods for Partial Differential Equations with Rough Solutions. Analysis and Matlab Programs; European Mathematical Society: Zürich, Switzerland, 2010. [Google Scholar]
- Glowinski, R.; Osher, S.J.; Yin, W. (Eds.) Splitting Methods in Communication, Imaging, Science, and Engineering; Scientific Computation; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Buchholz, S.; Gauckler, L.; Grimm, V.; Hochbruck, M.; Jahnke, T. Closing the gap between trigonometric integrators and splitting methods for highly oscillatory differential equations. IMA J. Numer. Anal. 2018, 38, 57–74. [Google Scholar] [CrossRef]
- Musetti, S.; Serena, P.; Bononi, A. On the Accuracy of Split-Step Fourier Simulations for Wideband Nonlinear Optical Communications. J. Light. Technol. 2018, 36, 5669–5677. [Google Scholar] [CrossRef]
- de Negreiros Júnior, J.; do Nascimento e Sá Cavalcante, D.; de Moraes, J.; Marcelino, L.R.; de Carvalho Belchior Magalhães, F.; Barboza, R.R.; de Carvalho, M.; de Freitas Guimarães, G. Ultrashort pulses propagation through different approaches of the Split-Step Fourier method. J. Mechatronics Eng. 2018, 1, 2–11. [Google Scholar] [CrossRef]
- Zia, H. Simulation of white light generation and near light bullets using a novel numerical technique. Commun. Nonlinear Sci. Numer. Simul. 2018, 54, 356–376. [Google Scholar] [CrossRef]
- Rahman, A.; Dutta, N. Mid-Infrared Supercontinuum Generation in Highly Nonlinear Chalcogenide Fibers. Int. J. High Speed Electron. Syst. 2024, 33, 2440060. [Google Scholar] [CrossRef]
- Lakoba, T.I. Study of instability of the Fourier split-step method for the massive Gross–Neveu model. J. Comput. Phys. 2020, 402, 109100. [Google Scholar] [CrossRef]
- Xiang, L.; Jin, S.; Shao, W.; Gao, M. Evaluation of Crosstalk in Nonlinear Regime for Weakly Coupled Multi-Core Fiber With Random Perturbations Using a Split-Step Numerical Model. J. Light. Technol. 2023, 41, 5729–5736. [Google Scholar] [CrossRef]
- Cardoso, W.B. Alternative split-step method for solving linearly coupled nonlinear Schrödinger equations. Comput. Phys. Commun. 2025, 307, 109414. [Google Scholar] [CrossRef]
- Lu, W.; Yang, J.; Tian, X. Fourth-order split-step pseudo-spectral method for the modified nonlinear Schrödinger equation. Ships Offshore Struct. 2017, 12, 424–432. [Google Scholar] [CrossRef]
- Kormann, K.; Reuter, K.; Rampp, M. A massively parallel semi-Lagrangian solver for the six-dimensional Vlasov–Poisson equation. Int. J. High Perform. Comput. Appl. 2019, 33, 924–947. [Google Scholar]
- Zlotnik, A.; Ĉiegis, R. On Construction and Properties of Compact 4th Order Finite-Difference Schemes for the Variable Coefficient Wave Equation. J. Sci. Comput. 2023, 95, 3. [Google Scholar] [CrossRef]
- Zhu, X.; Wan, H.; Zhang, Y. A split-step finite element method for the space-fractional Schrödinger equation in two dimensions. Sci. Rep. 2024, 14, 24257. [Google Scholar] [CrossRef]
- Strang, G. Accurate Partial Difference Methods I: Linear Cauchy Problems. Arch. Rat. Mech. Anal. 1963, 12, 392–402. [Google Scholar] [CrossRef]
- Strang, G. On the Construction and Comparison of Difference Schemes. SIAM J. Numer. Anal. 1968, 5, 506–517. [Google Scholar] [CrossRef]
- Suzuki, M. Fractal decomposition of exponential operators with applications to many-body theories and Monte Carlo simulations. Phys. Lett. A 1990, 146, 319–323. [Google Scholar] [CrossRef]
- Yoshida, H. Construction of higher order symplectic integrators. Phys. Lett. A 1990, 150, 262–268. [Google Scholar] [CrossRef]
- Blanes, S.; Moan, P.C. Practical symplectic partitioned Runge-Kutta and Runge-Kutta-Nyström methods. J. Comput. Appl. Math. 2002, 142, 313–330. [Google Scholar] [CrossRef]
- Burstein, S.Z.; Mirin, A.A. Third order difference methods for hyperbolic equations. J. Comput. Phys. 1970, 5, 547–571. [Google Scholar] [CrossRef]
- Amiranashvili, S.; Radziunas, M.; Bandelow, U.; Busch, K.; Čiegis, R. Additive splitting methods for parallel solutions of evolution problems. J. Comput. Phys. 2021, 436, 110320. [Google Scholar] [CrossRef]
- Taha, T.; Ablowitz, M. Analytical and numerical aspects of certain nonlinear evolution equations. II. Numerical, nonlinear Schrödinger equation. J. Comput. Phys. 1984, 55, 231–253. [Google Scholar] [CrossRef]
- Lovisetto, M.; Clamond, D.; Marcos, B. Integrating factor techniques applied to the Schrödinger-like equations. Comparison with Split-Step methods. Appl. Numer. Math. 2024, 197, 258–271. [Google Scholar] [CrossRef]
- Štikonas, A. The root condition for polynomial of the second order and a spectral stability of finite-difference schemes for Kuramoto-Tsuzuki equation. Math. Model. Anal. 1998, 3, 214–226. [Google Scholar] [CrossRef]
- Whitham, G.B. Linear and Nonlinear Waves; John Wiley & Sons: New York, NY, USA, 1974. [Google Scholar]
- Boyd, R.W. Nonlinear Optics, 3rd ed.; Academic: New York, NY, USA, 2008. [Google Scholar]
- Dudley, J.M.; Genty, G.; Coen, S. Supercontinuum generation in photonic crystal fiber. Rev. Mod. Phys. 2006, 78, 1135–1184. [Google Scholar] [CrossRef]
- Oughstun, K.E.; Xiao, H. Failure of the Quasimonochromatic Approximation for Ultrashort Pulse Propagation in a Dispersive, Attenuative Medium. Phys. Rev. Lett. 1997, 78, 642–645. [Google Scholar] [CrossRef]
- Nayfeh, A.H. Perturbation Methods; Wiley: Hoboken, NJ, USA, 1973. [Google Scholar]
- Agrawal, G.P. Nonlinear Fiber Optics, 4th ed.; Academic: New York, NY, USA, 2007. [Google Scholar]
- Brabec, T.; Krausz, F. Nonlinear Optical Pulse Propagation in the Single-Cycle Regime. Phys. Rev. Lett. 1997, 78, 3282–3285. [Google Scholar] [CrossRef]
- Kinsler, P. Optical pulse propagation with minimal approximations. Phys. Rev. A 2010, 81, 013819. [Google Scholar] [CrossRef]
- Kolesik, M.; Jakobsen, P.; Moloney, J.V. Quantifying the limits of unidirectional ultrashort optical pulse propagation. Phys. Rev. A 2012, 86, 035801. [Google Scholar] [CrossRef]
- Lakoba, T.I. Long-Time Simulations of Nonlinear Schrödinger-Type Equations using Step Size Exceeding Threshold of Numerical Instability. J. Sci. Comput. 2017, 72, 14–48. [Google Scholar] [CrossRef]
- Weideman, J.A.C.; Herbst, B.M. Split-Step Methods for the Solution of the Nonlinear Schrödinger Equation. SIAM J. Numer. Anal. 1986, 23, 485–507. [Google Scholar] [CrossRef]
- Lakoba, T.I. Instability analysis of the split-step Fourier method on the background of a soliton of the nonlinear Schrödinger equation. Numer. Methods Partial. Differ. Equations 2012, 28, 641–669. [Google Scholar] [CrossRef]
- Lakoba, T.I. Instability of the finite-difference split-step method applied to the nonlinear Schrödinger equation. I. standing soliton. Numer. Methods Partial. Differ. Equ. 2016, 32, 1002–1023. [Google Scholar] [CrossRef]
- Lakoba, T.I. Instability of the finite-difference split-step method applied to the nonlinear Schrödinger equation. II. moving soliton. Numer. Methods Partial. Differ. Equ. 2016, 32, 1024–1040. [Google Scholar] [CrossRef]
- Lakoba, T.I. Instability of the split-step method for a signal with nonzero central frequency. J. Opt. Soc. Am. B 2013, 30, 3260–3271. [Google Scholar] [CrossRef]
- Severing, F.; Bandelow, U.; Amiranashvili, S. Spurious Four-Wave Mixing Processes in Generalized Nonlinear Schrödinger Equations. J. Light. Technol. 2023, 41, 5359–5365. [Google Scholar] [CrossRef]
- Yang, J. Nonlinear Waves in Integrable and Nonintegrable Systems; SIAM: Philadelphia, PA, USA, 2010. [Google Scholar]
- Amiranashvili, S.; Čiegis, R. Stability of the higher-order splitting methods for the nonlinear Schrödinger equation with an arbitrary dispersion operator. Math. Model. Anal. 2024, 29, 560–574. [Google Scholar] [CrossRef]
- Kraych, A.E.; Agafontsev, D.; Randoux, S.; Suret, P. Statistical Properties of the Nonlinear Stage of Modulation Instability in Fiber Optics. Phys. Rev. Lett. 2019, 123, 093902. [Google Scholar] [CrossRef]
- Dudley, J.M.; Genty, G.; Mussot, A.; Chabchoub, A.; Dias, F. Rogue waves and analogies in optics and oceanography. Nat. Rev. Phys. 2019, 1, 675–689. [Google Scholar] [CrossRef]
- Sylvestre, T.; Genier, E.; Ghosh, A.N.; Bowen, P.; Genty, G.; Troles, J.; Mussot, A.; Peacock, A.C.; Klimczak, M.; Heidt, A.M.; et al. Recent advances in supercontinuum generation in specialty optical fibers. J. Opt. Soc. Am. B 2021, 38, F90–F103. [Google Scholar] [CrossRef]
- Zakharov, V.E.; Ostrovsky, L.A. Modulation instability: The beginning. Phys. D Nonlinear Phenom. 2009, 238, 540–548. [Google Scholar] [CrossRef]
- Hasegawa, A.; Matsumoto, M. Optical Solitons in Fibers; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Erkintalo, M.; Hammani, K.; Kibler, B.; Finot, C.; Akhmediev, N.; Dudley, J.M.; Genty, G. Higher-Order Modulation Instability in Nonlinear Fiber Optics. Phys. Rev. Lett. 2011, 107, 253901. [Google Scholar] [CrossRef] [PubMed]
- Yu, M.; McKinstrie, C.J.; Agrawal, G.P. Modulational instabilities in dispersion-flattened fibers. Phys. Rev. E 1995, 52, 1072–1080. [Google Scholar] [CrossRef] [PubMed]
- Blanco-Redondo, A.; de Sterke, C.M.; Sipe, J.E.; Krauss, T.F.; Eggleton, B.J.; Husko, C. Pure-quartic solitons. Nat. Commun. 2016, 7, 10427. [Google Scholar] [CrossRef]
- Kitayama, K.; Okamoto, K.; Yoshinaga, H. Extended four-photon mixing approach to modulational instability. J. Appl. Phys. 1988, 64, 6586–6587. [Google Scholar] [CrossRef]
- Cavalcanti, S.B.; Cressoni, J.C.; da Cruz, H.R.; Gouveia-Neto, A.S. Modulation instability in the region of minimum group-velocity dispersion of single-mode optical fibers via an extended nonlinear Schrödinger equation. Phys. Rev. A 1991, 43, 6162–6165. [Google Scholar] [CrossRef]
- Pitois, S.; Millot, G. Experimental observation of a new modulational instability spectral window induced by fourth-order dispersion in a normally dispersive single-mode optical fiber. Opt. Commun. 2003, 226, 415–422. [Google Scholar] [CrossRef]
- Harvey, J.D.; Leonhardt, R.; Coen, S.; Wong, G.K.L.; Knight, J.C.; Wadsworth, W.J.; Russell, P.S.J. Scalar modulation instability in the normal dispersion regime by use of a photonic crystal fiber. Opt. Lett. 2003, 28, 2225–2227. [Google Scholar] [CrossRef]
- Chua, C.; Liu, H. Existence of positive solutions for a quasilinear Schrödinger equation. Nonlinear Anal. Real World Appl. 2018, 44, 118–127. [Google Scholar] [CrossRef]
- Ankiewicz, A.; Kedziora, D.J.; Chowdury, A.; Bandelow, U.; Akhmediev, N. Infinite hierarchy of nonlinear Schrödinger equations and their solutions. Phys. Rev. E 2016, 93, 012206. [Google Scholar] [CrossRef]
- Bandelow, U.; Ankiewicz, A.; Amiranashvili, S.; Akhmediev, N. Sasa-Satsuma hierarchy of integrable evolution equations. Chaos 2018, 28, 053108. [Google Scholar] [CrossRef]
- Akhmediev, N.; Karlsson, M. Cherenkov radiation emitted by solitons in optical fibers. Phys. Rev. A 1995, 51, 2602–2607. [Google Scholar] [CrossRef] [PubMed]
- Potasek, M.J. Modulation instability in an extended nonlinear Schrödinger equation. Opt. Lett. 1987, 12, 921–923. [Google Scholar] [CrossRef] [PubMed]
- Dudley, J.M.; Taylor, J.R. (Eds.) Supercontinuum Generation in Optical Fibers; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
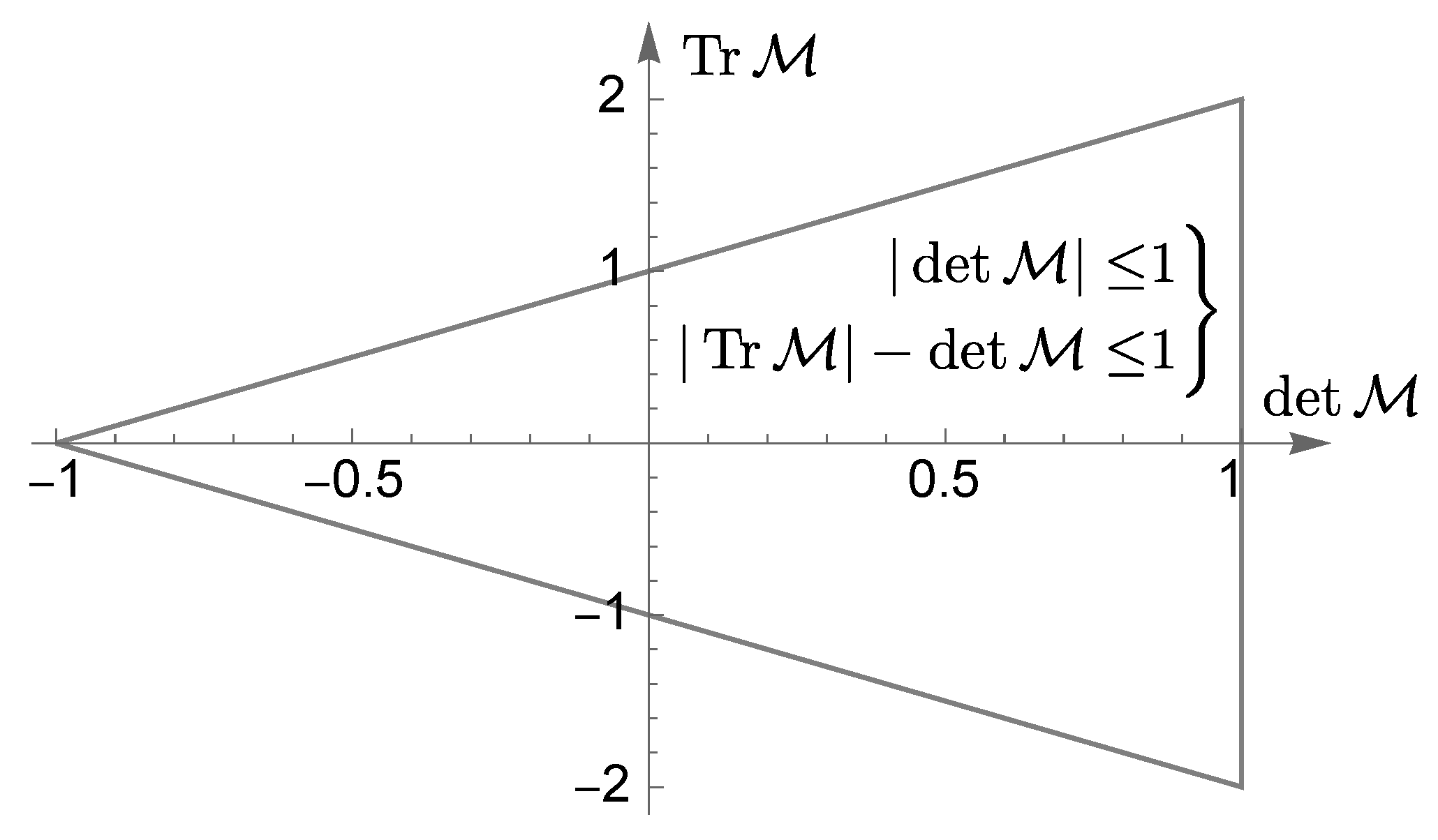

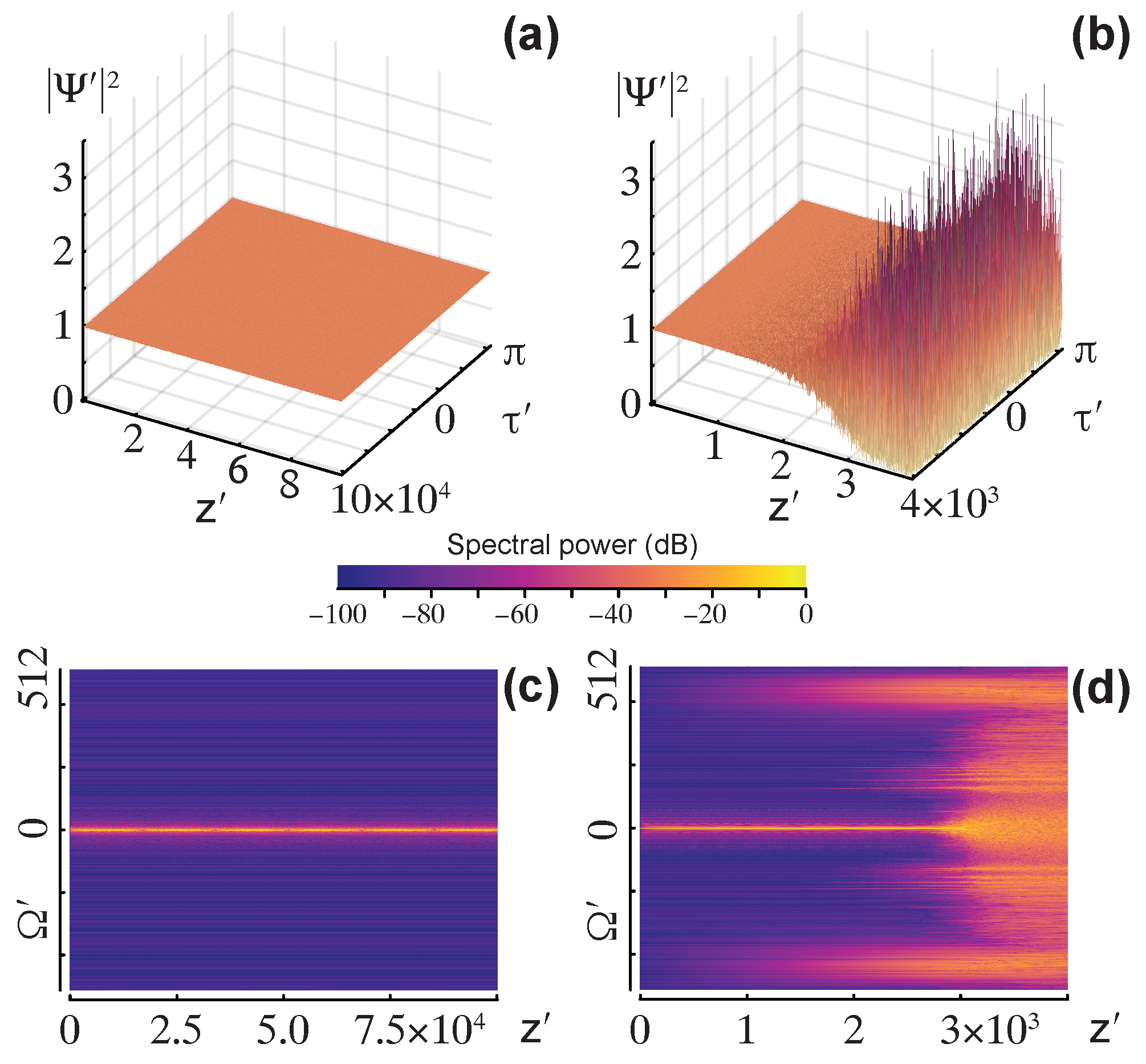

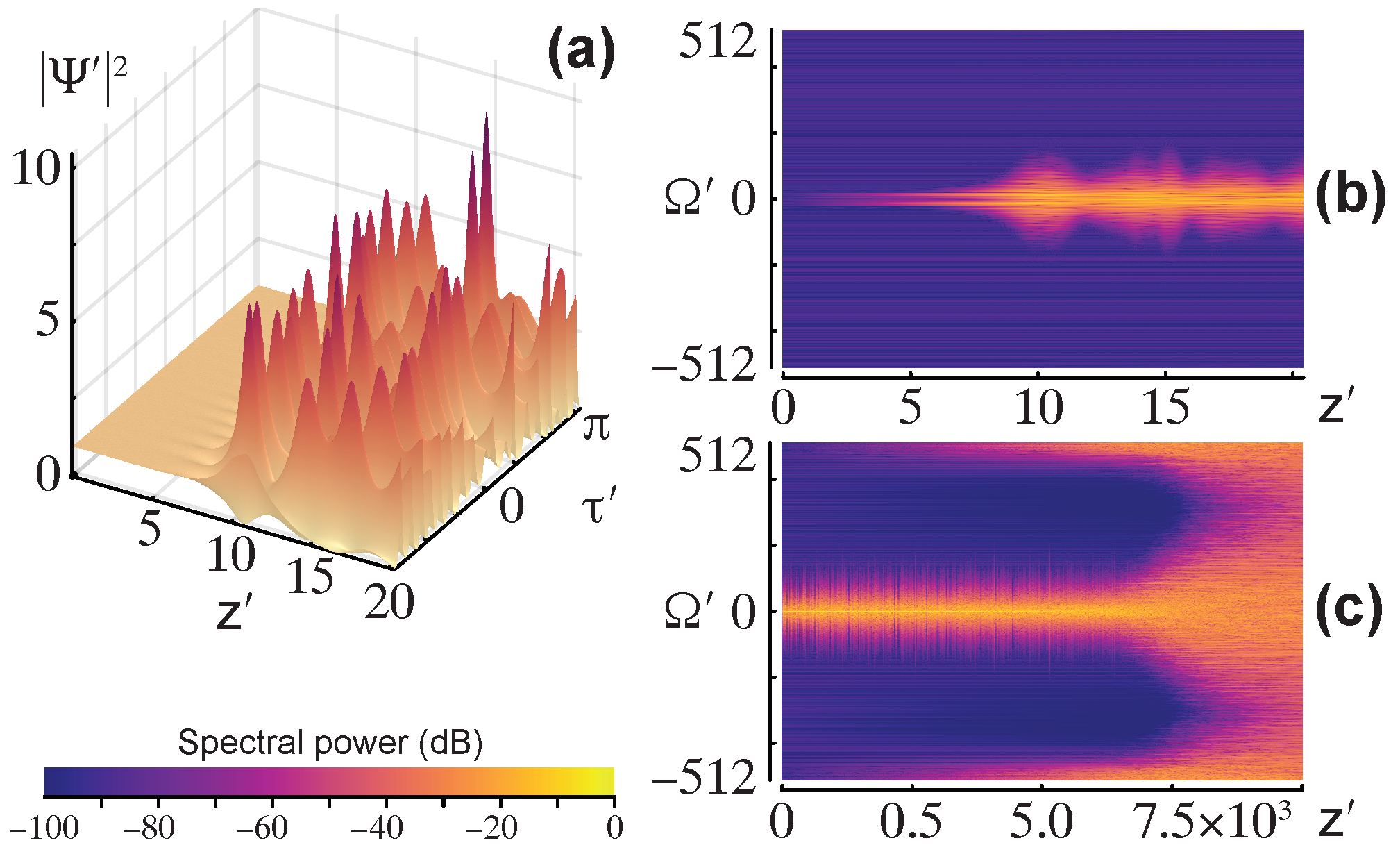
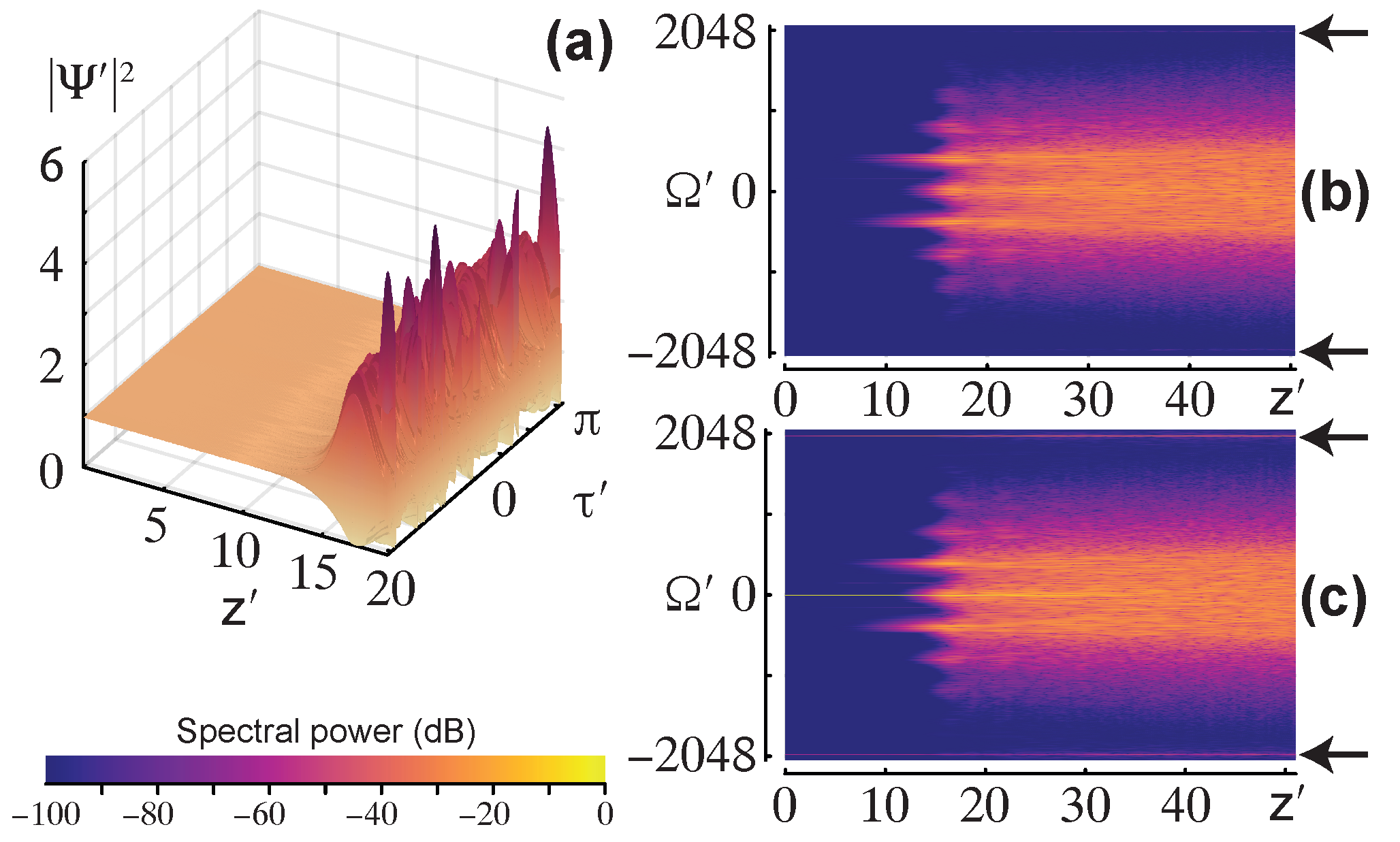
| Equation | Condition | |
|---|---|---|
| (2) | ||
| (9) | ||
| (10) | ||
| (11) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amiranashvili, S.; Bandelow, U.; Čiegis, R. Stability of the Additive Splitting Methods for the Generalized Nonlinear Schrödinger Equation. Mathematics 2025, 13, 1301. https://doi.org/10.3390/math13081301
Amiranashvili S, Bandelow U, Čiegis R. Stability of the Additive Splitting Methods for the Generalized Nonlinear Schrödinger Equation. Mathematics. 2025; 13(8):1301. https://doi.org/10.3390/math13081301
Chicago/Turabian StyleAmiranashvili, Shalva, Uwe Bandelow, and Raimondas Čiegis. 2025. "Stability of the Additive Splitting Methods for the Generalized Nonlinear Schrödinger Equation" Mathematics 13, no. 8: 1301. https://doi.org/10.3390/math13081301
APA StyleAmiranashvili, S., Bandelow, U., & Čiegis, R. (2025). Stability of the Additive Splitting Methods for the Generalized Nonlinear Schrödinger Equation. Mathematics, 13(8), 1301. https://doi.org/10.3390/math13081301






