Demand Information Asymmetry and Supply Chain Financing: A Signaling Perspective
Abstract
1. Introduction
2. Literature Review
2.1. Supply Chain Financing
2.2. Signaling Model Under Demand Information Asymmetry
3. Model and Assumptions
3.1. Model
3.2. Assumptions
4. Benchmark: Contract Decisions Under Symmetric Information
4.1. Wholesale Price Contract Decisions in Bank Financing
4.2. Trade Credit Contract Decisions in Seller Financing
4.3. The Optimal Contract Decisions Under Symmetric Information
5. Contract Design Under Asymmetric Information
5.1. Wholesale Price Contract Design in Bank Financing
5.2. Trade Credit Contract Design in Seller Financing
5.3. The Optimal Contract Decisions Under Asymmetric Information
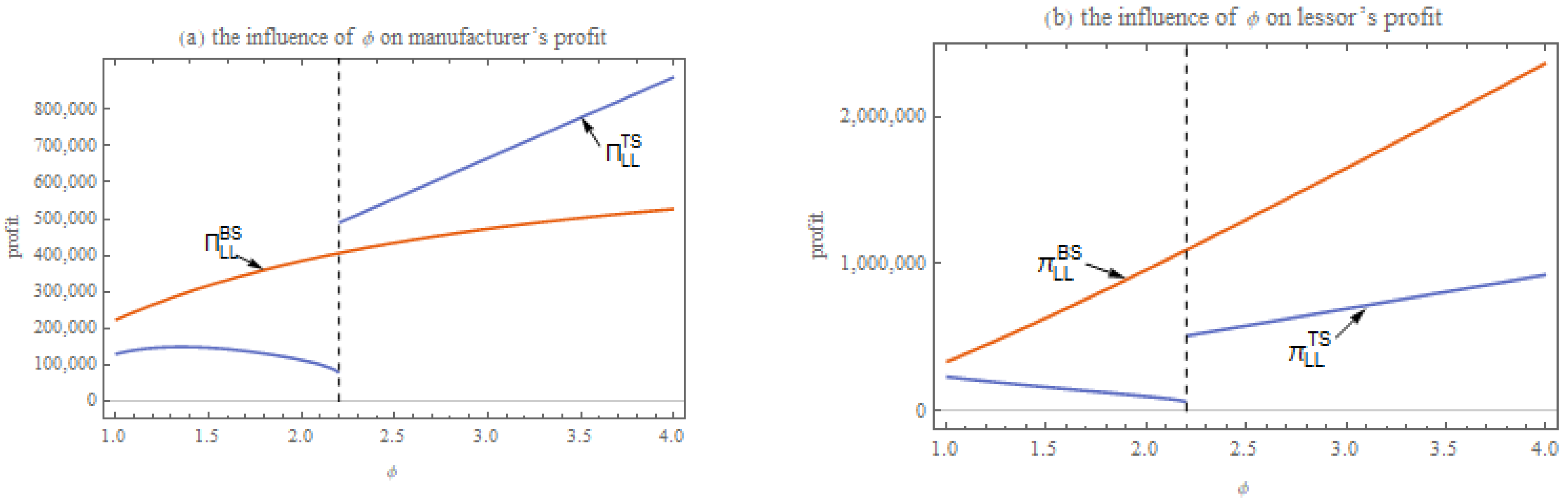
6. The Impact of Information Asymmetry on Optimal Contract Decisions
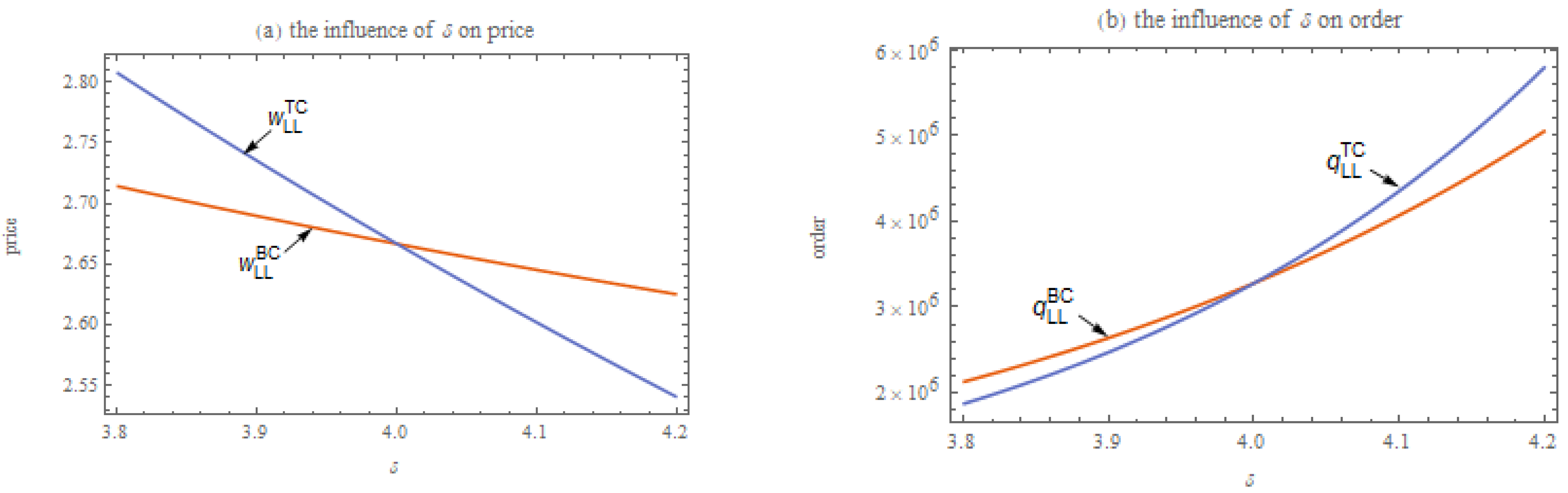
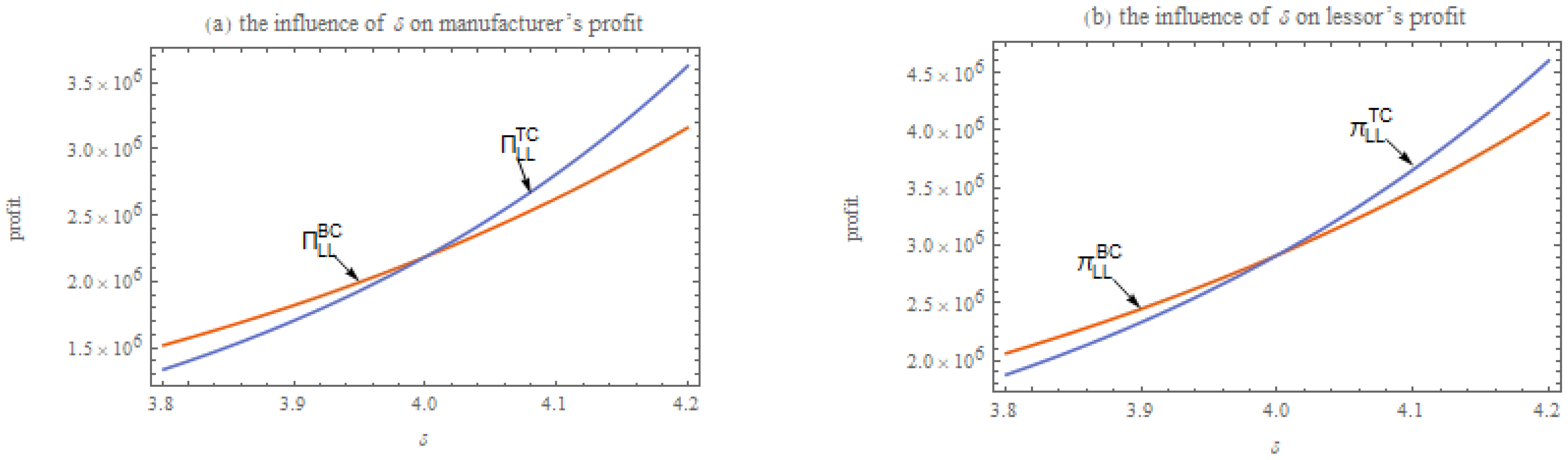
7. An Illustrating Example
7.1. Equilibrium Decisions of Supply Chain Members
7.2. Equilibrium Profits of Supply Chain Members
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Institute, CIRN. Annual Research and Consultation Report of Panorama Survey and Investment Strategy on China Industry 2023. 2024. Available online: https://www.chinairn.com/report/20240219/110838267.html?id=1902501&name=WuAoYan (accessed on 1 March 2025).
- Yoshizawa, D.; Nakamoto, Y.; Kagawa, S. Reduction of life-cycle CO2 emissions by expanding car-sharing services: A case study on Japan. J. Environ. Manag. 2023, 344, 118637. [Google Scholar] [CrossRef] [PubMed]
- Wang, N.; Duan, Y.; Jia, S. An optimization Model Combining Operator-Based Relocation With User-Based Relocation for Electric Carsharing Systems. Transp. Res. Rec. 2022, 2676, 827–846. [Google Scholar] [CrossRef]
- Khotanlou, M.; Kazemiolum, M.; Ziyaei, F. The Impact of Corporate Reputation on Financial Leverage and Trade Credit. Financ. Manag. Strategy 2025, 13, 99–116. [Google Scholar]
- Wu, D.; Zhang, B.; Baron, O. A trade credit model with asymmetric competing retailers. Prod. Oper. Manag. 2019, 28, 206–231. [Google Scholar] [CrossRef]
- Chod, J. Inventory, risk shifting, and trade credit. Manag. Sci. 2017, 63, 3207–3225. [Google Scholar] [CrossRef]
- Deng, S.; Gu, C.; Cai, G.G.; Li, Y. Financing Multiple Heterogeneous Suppliers in Assembly Systems: Buyer Finance vs. Bank Finance. Manuf. Serv. Oper. Manag. 2018, 20, 53–69. [Google Scholar] [CrossRef]
- Fabbri, D.; Klapper, L.F. Bargaining power and trade credit. J. Corp. Financ. 2016, 41, 66–80. [Google Scholar] [CrossRef]
- Jing, B.; Chen, X.; Cai, G. Equilibrium financing in a distribution channel with capital constraint. Prod. Oper. Manag. 2012, 21, 1090–1101. [Google Scholar] [CrossRef]
- Bao, W.; Ni, J.; Singh, S. Informal lending in emerging markets. Mark. Sci. 2018, 37, 123–137. [Google Scholar] [CrossRef]
- Guan, X.; Chen, Y.J. The interplay between information acquisition and quality disclosure. Prod. Oper. Manag. 2017, 26, 389–408. [Google Scholar] [CrossRef]
- Guo, L.; Iyer, G. Information acquisition and sharing in a vertical relationship. Mark. Sci. 2010, 29, 483–506. [Google Scholar] [CrossRef]
- Caldieraro, F.; Zhang, J.Z.; Cunha Jr, M.; Shulman, J.D. Strategic information transmission in peer-to-peer lending markets. J. Mark. 2018, 82, 42–63. [Google Scholar] [CrossRef]
- Yao, Z.; Gendreau, M.; Li, M.; Ran, L.; Wang, Z. Service operations of electric vehicle carsharing systems from the perspectives of supply and demand: A literature review. Transp. Res. Part C Emerg. Technol. 2022, 140, 103702. [Google Scholar] [CrossRef]
- Ersahin, N.; Giannetti, M.; Huang, R. Trade credit and the stability of supply chains. J. Financ. Econ. 2024, 155, 103830. [Google Scholar] [CrossRef]
- Wei, L.; Zhang, J.; Zhu, G. Incentive of retailer information sharing on manufacturer volume flexibility choice. Omega 2021, 100, 102210. [Google Scholar] [CrossRef]
- Jiang, B.; Tian, L.; Xu, Y.; Zhang, F. To share or not to share: Demand forecast sharing in a distribution channel. Mark. Sci. 2016, 35, 800–809. [Google Scholar] [CrossRef]
- Arca, P.; Atzeni, G.; Deidda, L. The signaling role of trade credit: Evidence from a counterfactual analysis. J. Corp. Financ. 2023, 80, 102414. [Google Scholar] [CrossRef]
- Biais, B.; Gollier, C. Trade credit and credit rationing. Rev. Financ. Stud. 1997, 10, 903–937. [Google Scholar] [CrossRef]
- Burkart, M.; Ellingsen, T. In-kind finance: A theory of trade credit. Am. Econ. Rev. 2004, 94, 569–590. [Google Scholar] [CrossRef]
- Lee, Y.W.; Stowe, J.D. Product risk, asymmetric information, and trade credit. J. Financ. Quant. Anal. 1993, 28, 285–300. [Google Scholar] [CrossRef]
- Kouvelis, P.; Zhao, W. Financing the newsvendor: Supplier vs. bank, and the structure of optimal trade credit contracts. Oper. Res. 2012, 60, 566–580. [Google Scholar] [CrossRef]
- Cao, E.; Chen, G. Information sharing motivated by production cost reduction in a supply chain with downstream competition. Nav. Res. Logist. 2021, 68, 898–907. [Google Scholar] [CrossRef]
- Gal-Or, E.; Geylani, T.; Dukes, A.J. Information sharing in a channel with partially informed retailers. Mark. Sci. 2008, 27, 642–658. [Google Scholar] [CrossRef]
- Bansal, A.; Panwar, A.; Unhelkar, B.; Mittal, M. Optimizing Inventory for Imperfect and Gradually Deteriorating Items Under Multi-Level Trade Credit in a Sustainable Supply Chain. Mathematics 2025, 13, 752. [Google Scholar] [CrossRef]
- Barrot, J.N. Trade credit and industry dynamics: Evidence from trucking firms. J. Financ. 2016, 71, 1975–2016. [Google Scholar] [CrossRef]
- Goyal, S.K. Economic order quantity under conditions of permissible delay in payments. J. Oper. Res. Soc. 1985, 36, 335–338. [Google Scholar] [CrossRef]
- Ho, C.-H.; Ouyang, L.-Y.; Su, C.-H. Optimal pricing, shipment and payment policy for an integrated supplier–buyer inventory model with two-part trade credit. Eur. J. Oper. Res. 2008, 187, 496–510. [Google Scholar] [CrossRef]
- Jacobson, T.; Von Schedvin, E. Trade credit and the propagation of corporate failure: An empirical analysis. Econometrica 2015, 83, 1315–1371. [Google Scholar] [CrossRef]
- Kong, G.; Rajagopalan, S.; Zhang, H. Revenue sharing and information leakage in a supply chain. Manag. Sci. 2013, 59, 556–572. [Google Scholar] [CrossRef]
- Kouvelis, P.; Zhao, W. Supply chain contract design under financial constraints and bankruptcy costs. Manag. Sci. 2016, 62, 2341–2357. [Google Scholar] [CrossRef]
- Moorthy, S.; Srinivasan, K. Signaling quality with a money-back guarantee: The role of transaction costs. Mark. Sci. 1995, 14, 442–466. [Google Scholar] [CrossRef]
- Protopappa-Sieke, M.; Seifert, R.W. Interrelating operational and financial performance measurements in inventory control. Eur. J. Oper. Res. 2010, 204, 439–448. [Google Scholar] [CrossRef]
- Yang, S.A.; Birge, J.R. Trade credit, risk sharing, and inventory financing portfolios. Manag. Sci. 2018, 64, 3667–3689. [Google Scholar] [CrossRef]
- Shenoy, J.; Williams, R. Trade credit and the joint effects of supplier and customer financial characteristics. J. Financ. Intermediation 2017, 29, 68–80. [Google Scholar] [CrossRef]
- de Véricourt, F.; Gromb, D. Financing capacity with stealing and shirking. Manag. Sci. 2019, 65, 5128–5141. [Google Scholar] [CrossRef]
- Silaghi, F.; Moraux, F. Trade credit contracts: Design and regulation. Eur. J. Oper. Res. 2022, 296, 980–992. [Google Scholar] [CrossRef]
- Avinadav, T.; Shamir, N. Partial vertical ownership, capacity investment, and information exchange in a supply chain. Manag. Sci. 2023, 69, 6038–6056. [Google Scholar] [CrossRef]
- Zha, Y.; Chen, K.; Dong, L.; Yu, Y. Financing Supplier Through Retailer’s Credit. Prod. Oper. Manag. 2024, 33, 721–736. [Google Scholar] [CrossRef]
- Smith, J.K. Trade credit and informational asymmetry. J. Financ. 1987, 42, 863–872. [Google Scholar] [CrossRef]
- Wang, Z.-H.; Qi, L.; Zhang, Y.; Liu, Z. A trade-credit-based incentive mechanism for a risk-averse retailer with private information. Comput. Ind. Eng. 2021, 154, 107101. [Google Scholar] [CrossRef]
- Cachon, G.P.; Fisher, M. Supply chain inventory management and the value of shared information. Manag. Sci. 2000, 46, 1032–1048. [Google Scholar] [CrossRef]
- Özer, Ö.; Raz, G. Supply chain sourcing under asymmetric information. Prod. Oper. Manag. 2011, 20, 92–115. [Google Scholar] [CrossRef]
- Li, Z.; Gilbert, S.M.; Lai, G. Supplier encroachment under asymmetric information. Manag. Sci. 2014, 60, 449–462. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, J.; Zaccour, G. Market targeting and information sharing with social influences in a luxury supply chain. Transp. Res. Part E Logist. Transp. Rev. 2020, 133, 101822. [Google Scholar] [CrossRef]
- Cao, Y.; Wang, Q. The informational role of guarantee contracts. Eur. J. Oper. Res. 2022, 301, 191–202. [Google Scholar] [CrossRef]
- Liang, L.; Tian, L.; Xie, J.; Xu, J.; Zhang, W. Optimal pricing model of car-sharing: Market pricing or platform pricing. Ind. Manag. Data Syst. 2021, 121, 594–612. [Google Scholar] [CrossRef]
- Lagadic, M.; Verloes, A.; Louvet, N. Can carsharing services be profitable? A critical review of established and developing business models. Transp. Policy 2019, 77, 68–78. [Google Scholar] [CrossRef]
- Cho, I.-K.; Kreps, D.M. Signaling games and stable equilibria. Q. J. Econ. 1987, 102, 179–221. [Google Scholar] [CrossRef]
- Research, G.V. Car Rental Market Size, Share & Trends Analysis Report by Vehicle (Luxury Cars, Executive Cars), By Application (Local Usage, Airport Transport), By Booking Mode, By Region, and Segment Forecasts, 2025–2030. 2024. Available online: https://www.grandviewresearch.com/industry-analysis/car-rental-market (accessed on 1 March 2025).
- He, L.; Hu, Z.; Zhang, M. Robust repositioning for vehicle sharing. Manuf. Serv. Oper. Manag. 2020, 22, 241–256. [Google Scholar] [CrossRef]

| Literature | Supply Chain Financing | Signaling Model Under Demand Information Asymmetry | |
|---|---|---|---|
| Bank Financing | Seller Financing | ||
| Kouvelis and Zhao [22] | √ | √ | |
| Jing et al. [9] | √ | √ | |
| Shenoy and Williams [35] | √ | √ | |
| Smith [40] | √ | ||
| Wang et al. [41] | √ | ||
| Acra et al. [18] | √ | ||
| Zhang et al. [45] | √ | ||
| Jiang et al. [17] | √ | ||
| Cao and Wang [46] | √ | ||
| This paper | √ | √ | √ |
| Notation | Explanation |
|---|---|
| The wholesale price in wholesale price contracts | |
| Trade credit price in trade credit contracts | |
| Unit production cost | |
| Rental period | |
| Leasing price | |
| Random demand | |
| The market saturation demand | |
| The price sensitivity | |
| Probability that the demand signal is high (low) | |
| The market demand type obtained by the manufacturer | |
| The lessor’s belief about the market type | |
| The order quantity | |
| The lessor’s inventory factor | |
| The manufacturer’s separating equilibrium profit with a high market demand in wholesale price contracts (the rest are similar) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, S.; Xie, J. Demand Information Asymmetry and Supply Chain Financing: A Signaling Perspective. Mathematics 2025, 13, 1288. https://doi.org/10.3390/math13081288
Xie S, Xie J. Demand Information Asymmetry and Supply Chain Financing: A Signaling Perspective. Mathematics. 2025; 13(8):1288. https://doi.org/10.3390/math13081288
Chicago/Turabian StyleXie, Shanshan, and Jiamuyan Xie. 2025. "Demand Information Asymmetry and Supply Chain Financing: A Signaling Perspective" Mathematics 13, no. 8: 1288. https://doi.org/10.3390/math13081288
APA StyleXie, S., & Xie, J. (2025). Demand Information Asymmetry and Supply Chain Financing: A Signaling Perspective. Mathematics, 13(8), 1288. https://doi.org/10.3390/math13081288




