Dynamical Analysis of an Economic-Environment Model with Unilateral and Bilateral Control
Abstract
1. Introduction
2. Model Formulation
3. Main Results
3.1. Dynamics of System (1)
3.2. Existence and Stability of the Periodic Solution
- (1)
- If point overlaps point , then forms an order-1 periodic solution.
- (2)
- If point is located on the right side of , this means that , then the successor function . Now it is necessary to find a point such that the corresponding successor function is less than 0.
- (3)
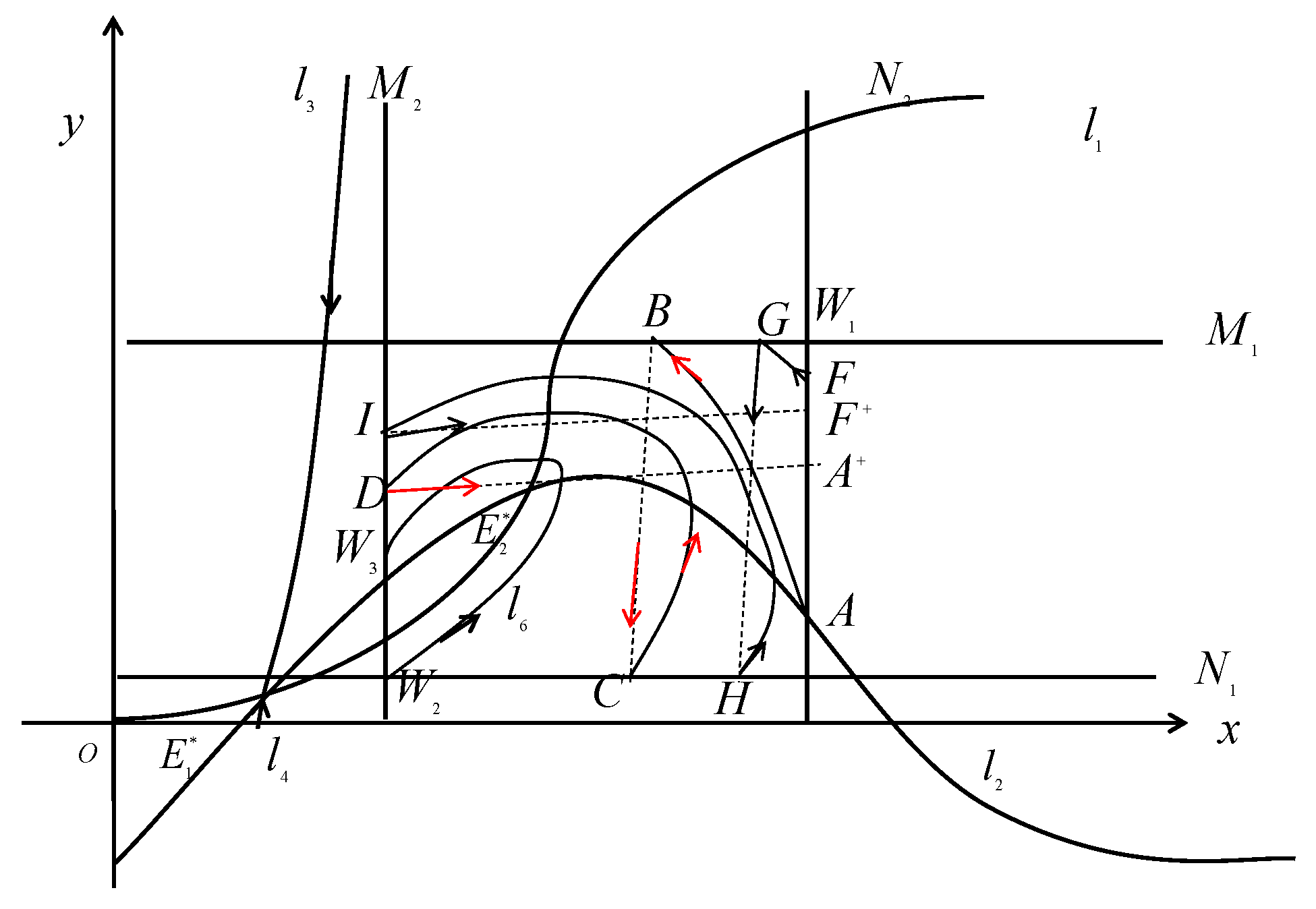
- Let W be the intersection of and phase set . If point is located on the left side of , this means that , then the successor function . In addition, we need to assume that (see Figure 2b). If not, the trajectory will tend to be the origin point.
- (1)
- If , then forms an order-1 periodic solution.
- (2)
- If , then the trajectory will tend to , system (3) does not exist periodic solution. If , i.e., , then it will tend to the origin point.
- (3)
- If , then the successor function . Now it is necessary to choose a point such that the corresponding successor function is less than 0. We further assume that is the intersection point of the curve and phase set , then there exists a trajectory passing through the point , by the geometric theory of differential equation, it will intersect the impulsive set at point , then it is mapped to the point , where is the successor point of . Then we have the successor function . By the continuity of successor function, there is a point such that , this means that there exists an order-1 periodic solution, as is shown in Figure 4.
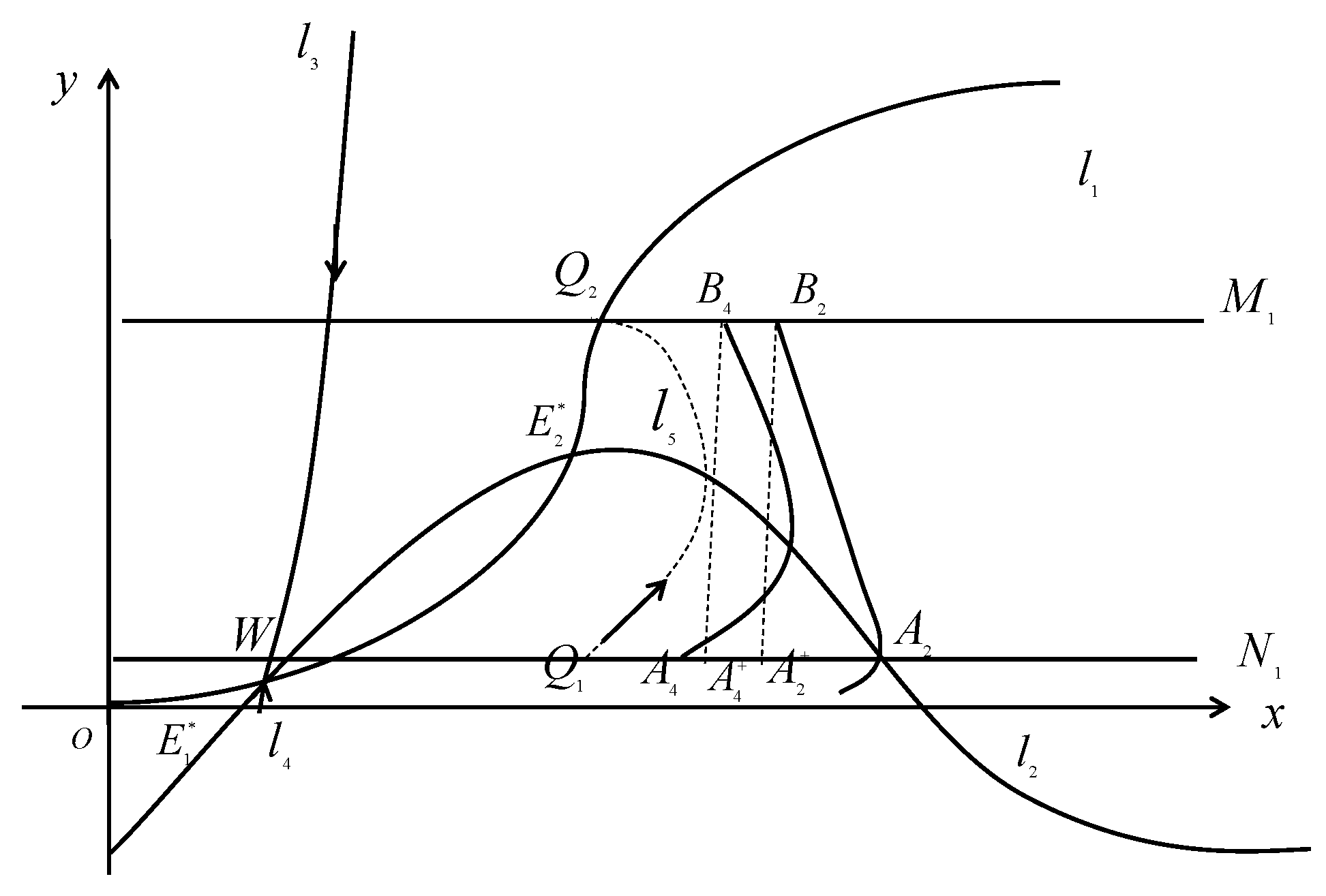
- (1)
- If the successor point of P is below point P, i.e., , then after a finite number of pulses, the trajectory will tend to be the positive equilibrium .
- (2)
- If the successor point of P coincides with P, then forms an order-1 periodic solution.
- (3)
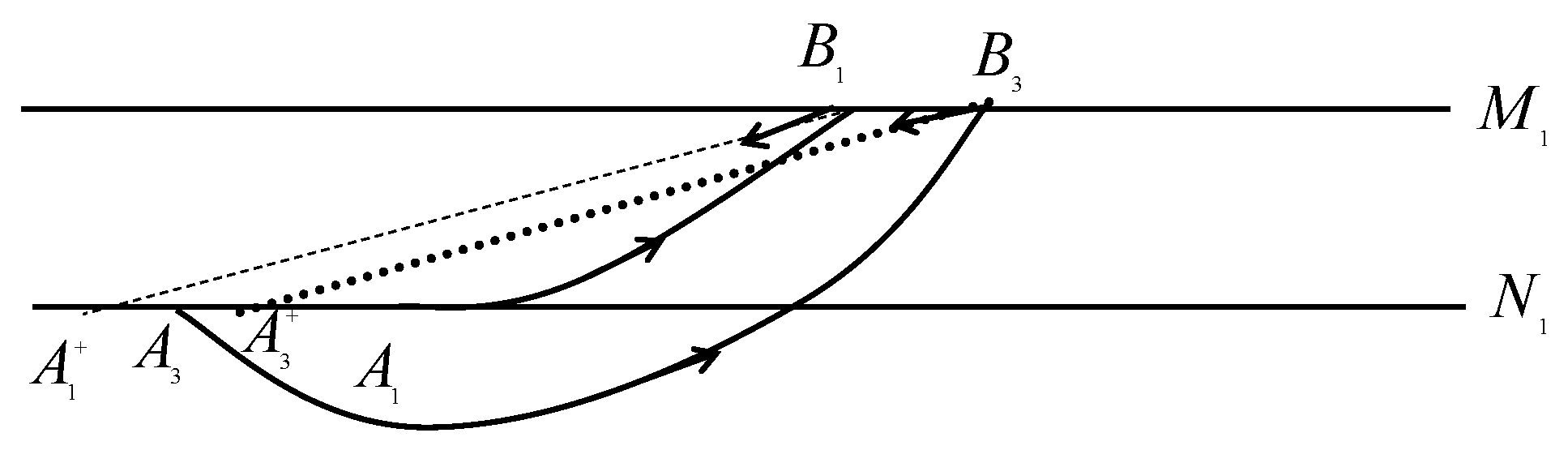
- If the successor point of P is above point P, then the successor function , then it is necessary to find a point such that the corresponding successor function is less than 0. Choose a point away from the x-axis, then the trajectory through point intersects the impulsive set , and it is mapped to point , then the successor function . Then the existence of order-1 periodic solution is proved.

3.2.1. Stability of Order-1 Periodic Solution of System (3)
3.2.2. Stability of the Order-2 Periodic Solution
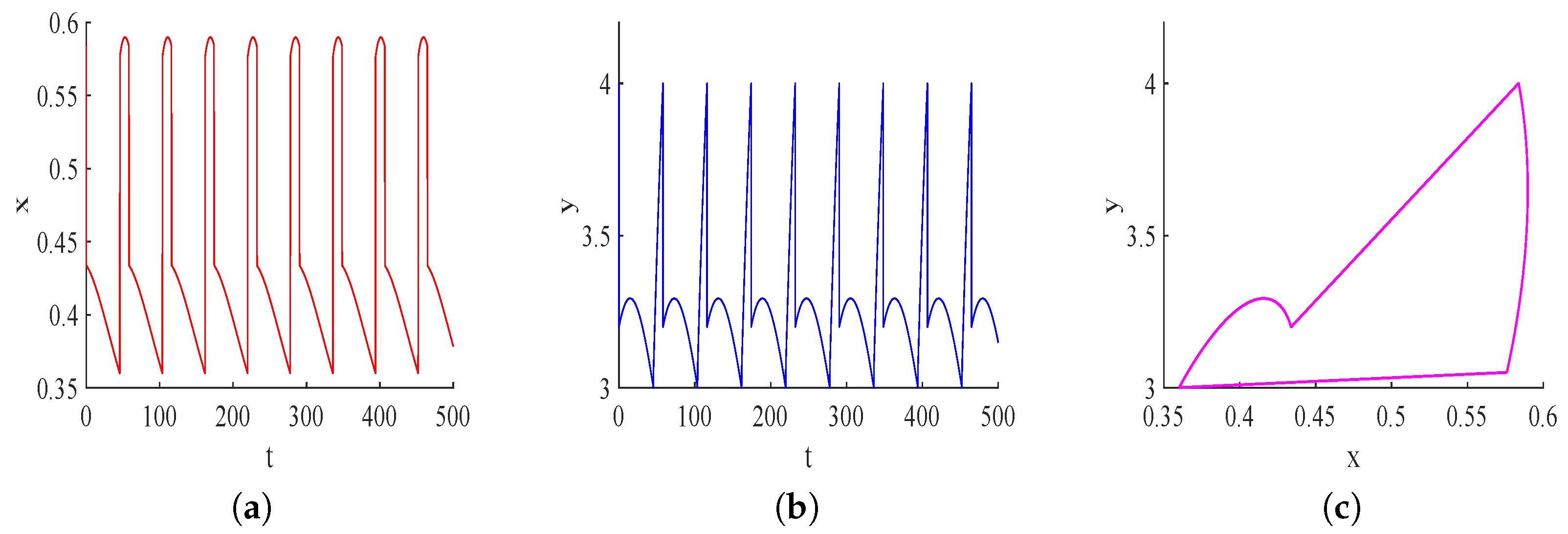
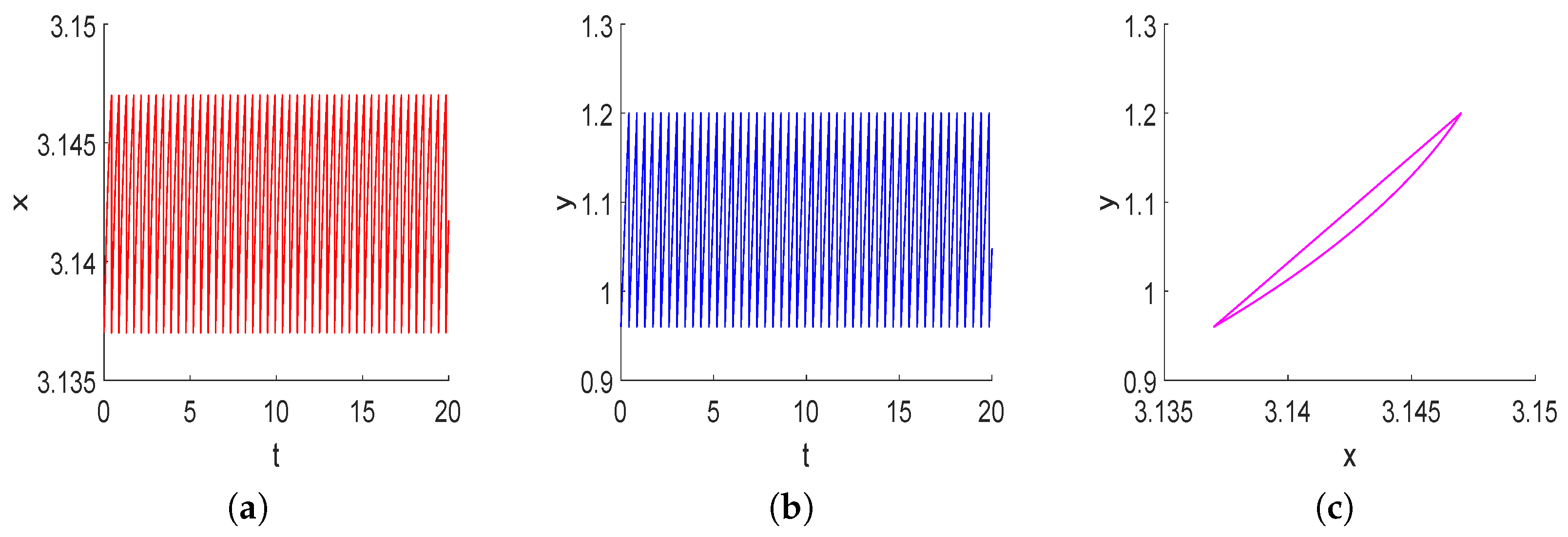
4. Numerical Simulations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- McGlade, C.; Ekins, P. The geographical distribution of fossil fuels unused when limiting global warming to 2 °C. Nature 2015, 554, 229–233. [Google Scholar] [CrossRef] [PubMed]
- Sadr, N.; Bahrdo, T.; Taghizadeh, R. Impacts of Paris agreement, fossil fuel consumption, and net energy imports on CO2 emissions: A panel data approach for three West European countries. Clean Technol. Environ. Policy 2022, 24, 1521–1534. [Google Scholar] [CrossRef]
- Li, Y.; Wu, H.; Shen, K.; Hao, Y.; Zhang, P. Is environmental pressure distributed equally in China? Empirical evidence from provincial and industrial panel data analysis. Sci. Total Environ. 2020, 718, 137363. [Google Scholar] [CrossRef]
- Jia, J.; Xin, L.; Lu, C.; Wu, B.; Zhong, Y. China’s CO2 emissions: A systematical decomposition concurrently from multi-sectors and multi-stages since 1980 by an extended logarithmic mean divisia index. Energy Strateg. Rev. 2023, 49, 101141. [Google Scholar] [CrossRef]
- Zhao, B.; Wang, S.; Hao, J. Challenges and perspectives of air pollution control in China. Front. Env. Sci. Eng. 2024, 18, 68. [Google Scholar] [CrossRef]
- Lei, Y.; Yin, Z.; Lu, X.; Zhang, Q.; Gong, J.; Cai, B.; Cai, C.; Chai, Q.; Chen, H.; Chen, R.; et al. The 2022 report of synergetic roadmap on carbon neutrality and clean air for China: Accelerating transition in key sectors. Environ. Sci. Ecotechnol. 2024, 19, 100335. [Google Scholar] [CrossRef]
- Weichenthal, S.; Pinault, L.; Christidis, T.; Burnett, R.T.; Brook, J.R.; Chu, Y.; Crouse, D.L.; Erickson, A.C.; Hystad, P.; Li, C.; et al. How low can you go? Air pollution affects mortality atvery low levels. Sci. Adv. 2022, 8, eabo3381. [Google Scholar] [CrossRef]
- Li, R.; Li, L.; Wang, Q. The impact of energy efficiency on carbon emissions: Evidence from the transportation sector in Chinese 30 provinces. Sustain. Cities Soc. 2022, 82, 103880. [Google Scholar] [CrossRef]
- Wang, M.; Feng, C. The win-win ability of environmental protection and economic development during China’s transition. Technol. Forecast. Social Change 2021, 166, 120617. [Google Scholar] [CrossRef]
- Brock, W.A. Economics of Natural and Environmental Resources; Gordon & Breach: New York, NY, USA, 1973. [Google Scholar]
- Mäler, K.G. Environmental Economics: A Theoretical Inquiry; Johns Hopkins University Press: Baltimore, MD, USA, 1974. [Google Scholar]
- Nordhaus, W.D. An optimal transition path for controlling greenhouse gases. Science 1992, 258, 1315–1319. [Google Scholar] [CrossRef]
- Nordhaus, W.D. A Question of Balance: Economic Modeling of Global Warming; Yale University Press: New Haven, CT, USA, 2008. [Google Scholar]
- La Torre, D.; Liuzzi, D.; Marsiglio, S. Pollution diffusion and abatement activities across space and over time. Math. Soc. Sci. 2015, 78, 48–63. [Google Scholar] [CrossRef]
- Capasso, V.; Engbers, R.; Torre, D.L. On a spatial Solow model with technological diffusion and nonconcave production function. Nonlinear Anal. Real World Appl. 2010, 11, 3858–3876. [Google Scholar] [CrossRef]
- Skiba, A.K. Optimal growth with a convex-concave production function. Econometrica 1978, 46, 527–539. [Google Scholar] [CrossRef]
- Liuzzi, D.; Venturi, B. Pollution-induced poverty traps via Hopf bifurcation in a minimal integrated economic-environment model. Commun. Nonlinear. Sci. 2021, 93, 105523. [Google Scholar] [CrossRef]
- Sun, D.; Zeng, S.; Lin, H.; Meng, X.; Yu, B. Can transport infrastructure pave a green way? A city-level examination in China. J. Clean. Prod. 2019, 226, 669–678. [Google Scholar] [CrossRef]
- Enyoh, C.E.; Verla, A.W.; Wang, Q.; Ohiagu, F.O.; Chowdhury, A.H.; Enyoh, E.C.; Chowdhury, T.; Verla, E.N.; Chinwendu, U.P. An overview of emerging pollutants in air: Method of analysis and potential public health concern from human environmental exposure. Trends Environ. Anal. Chem. 2020, 28, e00107. [Google Scholar] [CrossRef]
- Liu, S.; Tian, H.; Zhu, C. Reduced but still noteworthy atmospheric pollution of trace elements in China. One Earth 2023, 6, 536–547. [Google Scholar] [CrossRef]
- Sun, K.; Zhang, T.; Tian, Y. Theoretical study and control optimization of an integrated pest management predator–prey model with power growth rate. Math. Biosci. 2016, 279, 13–26. [Google Scholar] [CrossRef]
- Sun, K.; Zhang, T.; Tian, Y. Dynamics analysis and control optimization of a pest management predator–prey model with an integrated control strategy. Appl. Math. Comput. 2017, 292, 253–271. [Google Scholar] [CrossRef]
- Song, X.; Huang, M.; Li, J. Modeling impulsive insulin delivery in insulin pump with time delays. SIAM J. Appl. Math. 2014, 74, 1763–1785. [Google Scholar] [CrossRef]
- Guo, H.; Tian, Y.; Sun, K. Study on dynamic behavior of two fishery harvesting models: Effects of variable prey refuge and imprecise biological parameters. J. Appl. Math. Comput. 2023, 69, 4243–4268. [Google Scholar]
- Tian, Y.; Guo, H.; Sun, K. Complex dynamics of two prey-predator harvesting models with prey refuge and interval-valued imprecise parameters. Math. Methods Appl. Sci. 2023, 46, 14278–14298. [Google Scholar] [CrossRef]
- Xu, J.; Huang, M.; Song, X. Dynamics of a guanaco-sheep competitive system with unilateral and bilateral control. Nonlinear Dyn. 2022, 107, 3111–3126. [Google Scholar] [CrossRef]
- Zhang, M.; Zhao, Y.; Song, X. Dynamics of bilateral control system with state feedback for price adjustment strategy. Int. J. Biomath. 2021, 14, 2150031. [Google Scholar] [CrossRef]
- Yu, H.; Lin, X. Study of environmental pollution control investment efficiency and its influence in Beijing-Tianjin-Hebei region. Ind. Technol. Econ. 2018, 5, 138–146. [Google Scholar]
- Zhao, X.; Long, L.; Sun, Q. How to Evaluate Investment Efficiency of Environmental Pollution Control: Evidence from China. Int. J. Environ. Res. Public Health 2022, 19, 7252. [Google Scholar] [CrossRef]
- Feng, Q.; Sun, T. Comprehensive evaluation of benefits from environmental investment: Take China as an example. Environ. Sci. Pollut. Res. 2020, 27, 15292–15304. [Google Scholar] [CrossRef] [PubMed]
- Xiang, L.; Zhang, H.; Lyu, Y. A Study on the Impact of Reform and Opening-up on Sustainable Development: Evidence from China. Probl. Ekorozw. 2023, 18, 204–215. [Google Scholar] [CrossRef]
- Bainov, D.; Simeonov, P.S. Impulsive Differential Equations: Periodic Solutions and Applications; Routledge: New York, NY, USA, 1993; Volume 66. [Google Scholar]
- Simeonov, P.S.; Bainov, D.D. Orbital stability of periodic solutions of autonomous systems with impulse effect. Int. J. Syst. Sci. 1989, 19, 2561–2585. [Google Scholar] [CrossRef]

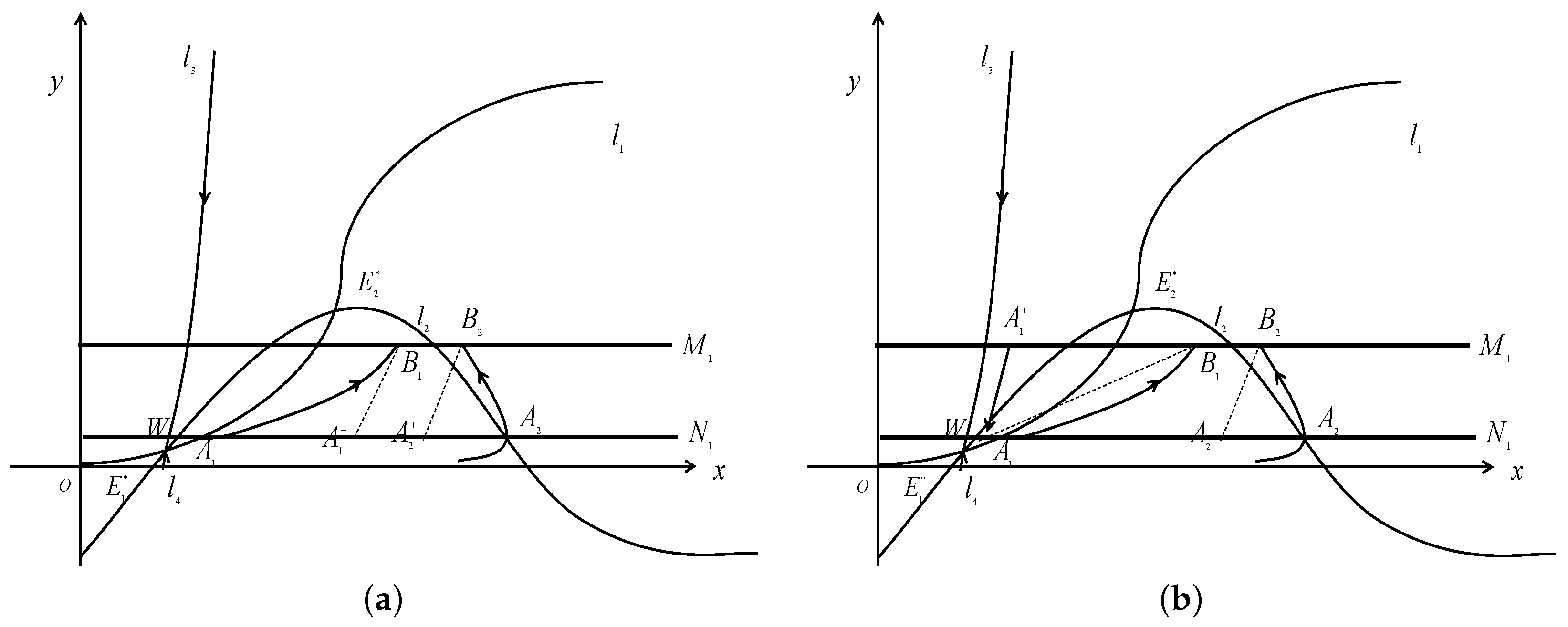



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, J.; Huang, M.; Yang, J. Dynamical Analysis of an Economic-Environment Model with Unilateral and Bilateral Control. Mathematics 2025, 13, 1268. https://doi.org/10.3390/math13081268
Xu J, Huang M, Yang J. Dynamical Analysis of an Economic-Environment Model with Unilateral and Bilateral Control. Mathematics. 2025; 13(8):1268. https://doi.org/10.3390/math13081268
Chicago/Turabian StyleXu, Jing, Mingzhan Huang, and Jingen Yang. 2025. "Dynamical Analysis of an Economic-Environment Model with Unilateral and Bilateral Control" Mathematics 13, no. 8: 1268. https://doi.org/10.3390/math13081268
APA StyleXu, J., Huang, M., & Yang, J. (2025). Dynamical Analysis of an Economic-Environment Model with Unilateral and Bilateral Control. Mathematics, 13(8), 1268. https://doi.org/10.3390/math13081268