Analyzing the Impact of Process Noise for a Flexible Structure During the Minimum-Time Rest-to-Rest Slew Maneuver
Abstract
1. Introduction
- (a)
- First, it discusses the minimum-time optimal control problem in the presence of process noise, analyzes the trajectory equations and their relationships at various switching intervals.
- (b)
- Second, it further analyzes the trajectory equations and discusses the contribution of each variance to the overall variance of the rigid and flexible mode elements.
2. Equations of Motion
3. Analyzing the Minimum-Time Control Problem in the Presence of Process Noise
3.1. Trajectory Equations Analysis
3.2. Further Discussion on the Angular Positions of Rigid and Flexible Modes at the Central Switch
- (a)
- Note on the .Equation (13) contains no additional terms except , and .
- (b)
- Note on the .can be derived as follows.The term is made of various angular terms and constants and is mentioned in Appendix B (see Equation (A9)).
4. Analyzing the Impact of the Variance
- (a)
- , all four elements of the first row of the Matrix in Equation (24) are directly related to the final time or (), so for a rest-to-rest control problem, the variance of is minimal when the final time (i.e., ) is the minimum time. If and are both 0, then the spacecraft position will also have some uncertainty due to the noise associated with the location and velocity of the flexible mode (e.g., solar panel), as the system considered in this article is a coupled system (). Furthermore, if is not too small, then the majority of the variance in should be contributed by the variance in or Var().
- (b)
- , since = 0, therefore, the variance of does not depend on . Also, note that , i.e., is a direct function of . Again, if the variance in is not too small, then Var() should account for most of the variance in .
- (c)
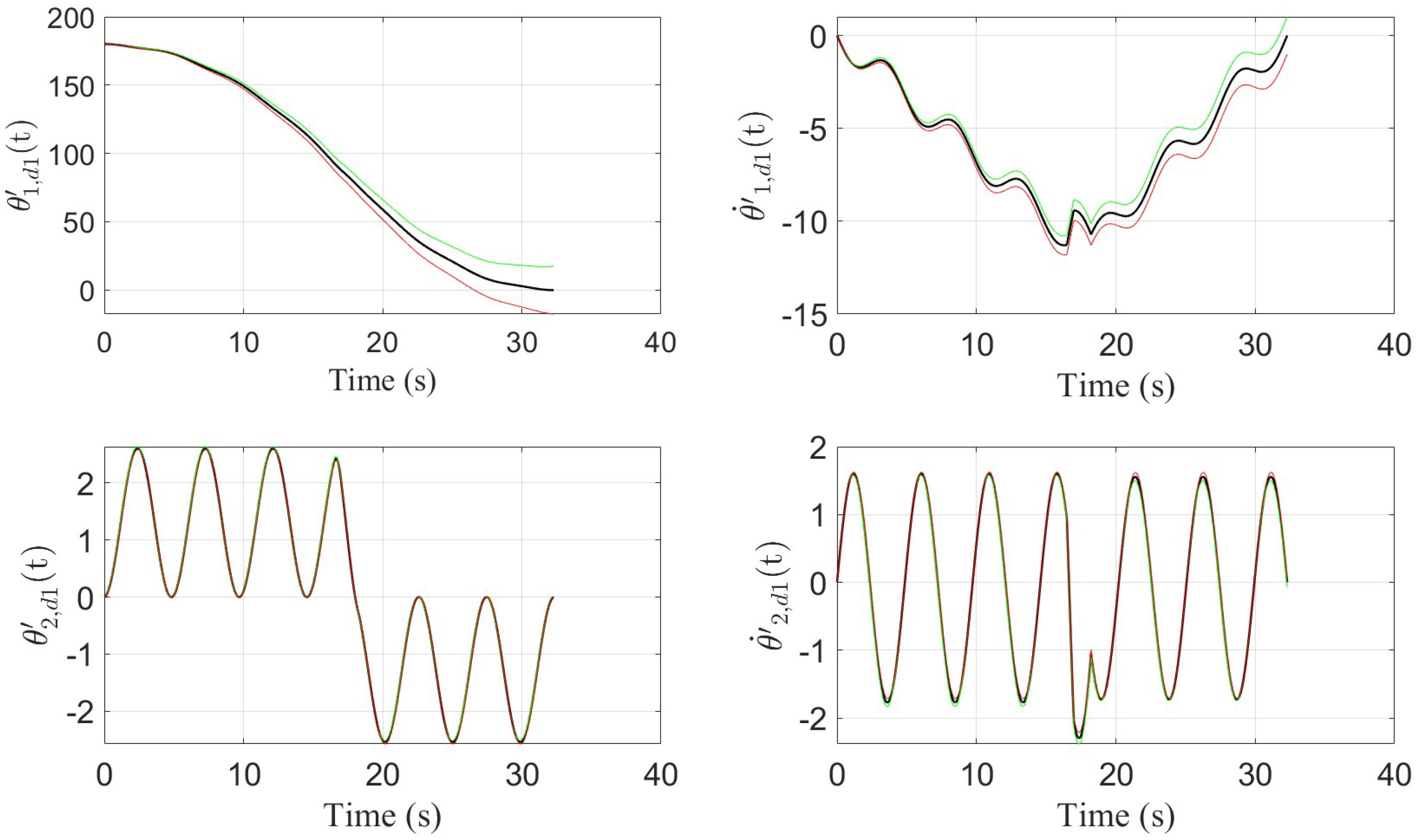
- and , these two terms do not depend on the variances in and . Furthermore, . In addition, and are related to the and terms, which explains the cyclical/periodic pattern seen in Figure 2 and Figure 3 for and . For the variance of , the contributions of Var() and Var() depend on both and , the same for .
5. Examples
5.1. Minimum-Time Control Example
5.2. Considering the Process Noise Variance for Minimum-Time Control
6. Variance-Based Sensitivity Analysis
7. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
- The following nomenclature are used in this manuscript:
| Rigid body position (ideal dynamics) | |
| Rigid body velocity (ideal dynamics) | |
| Flexible mode position (ideal dynamics) | |
| Flexible mode velocity (ideal dynamics) | |
| Coupling parameter (constant) | |
| Flexible mode natural frequency (constant) | |
| Torque magnitude (constant) | |
| Minimum torque magnitude limit (constant) | |
| Maximum torque magnitude limit (constant) | |
| t | Time |
| Slewing angle (constant) | |
| Input parameter (constant) | |
| Input parameter (constant) | |
| Process noise mean | |
| i | Number of flexible modes |
| Total time or the final time (ideal dynamics) | |
| Total time or the final time (uncertain dynamics) | |
| Switching time intervals (ideal dynamics) | |
| Switching time intervals (uncertain dynamics) | |
| w | Weighting factor |
| Switching time interval representation (uncertain dynamics) | |
| Switching time interval representation (ideal dynamics) | |
| Flexible mode positions at V3, V2 and V1, respectively, (uncertain dynamics) | |
| Flexible mode velocities at V3, V2 and V1, respectively, (uncertain dynamics) | |
| Rigid body positions at V3, V2 and V1, respectively, (uncertain dynamics) | |
| Rigid body velocities at V3, V2 and V1, respectively, (uncertain dynamics) | |
| Process noise standard deviation | |
| Process noise variance | |
| Represent the differences between process noise affected and unaffected time intervals | |
| Initial time |
Appendix A. Sobol’s First-Order Variance-Based Sensitivity Analysis Results
| 0.01237 | 0.79016 | 0.00356 | 0.19250 | |
| 0 | 0.79330 | 0.00182 | 0.20215 | |
| 0 | 0 | 0.58823 | 0.40943 | |
| 0 | 0 | 0.96914 | 0.03140 |
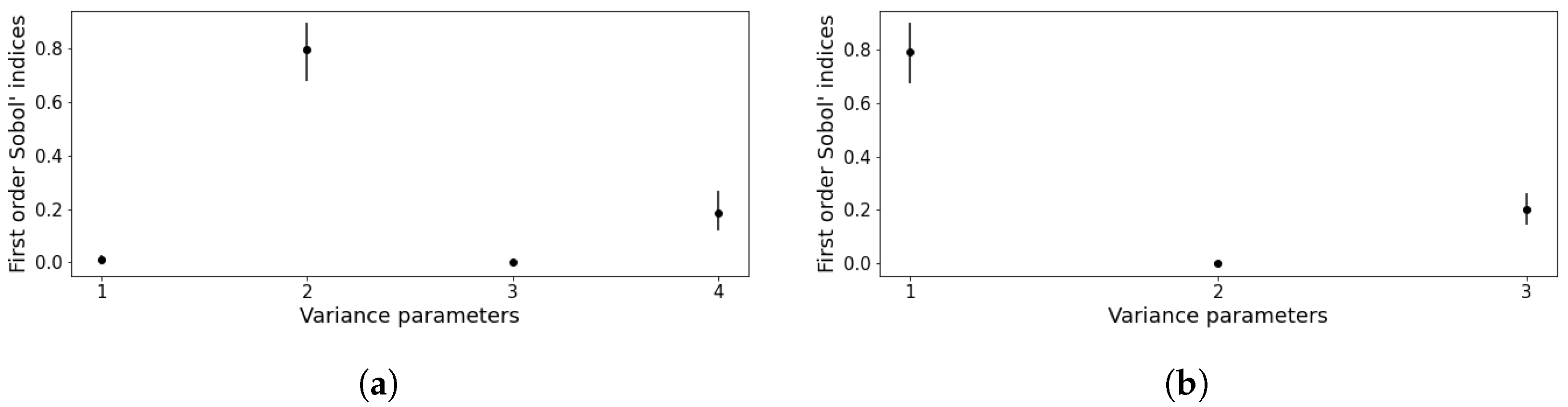
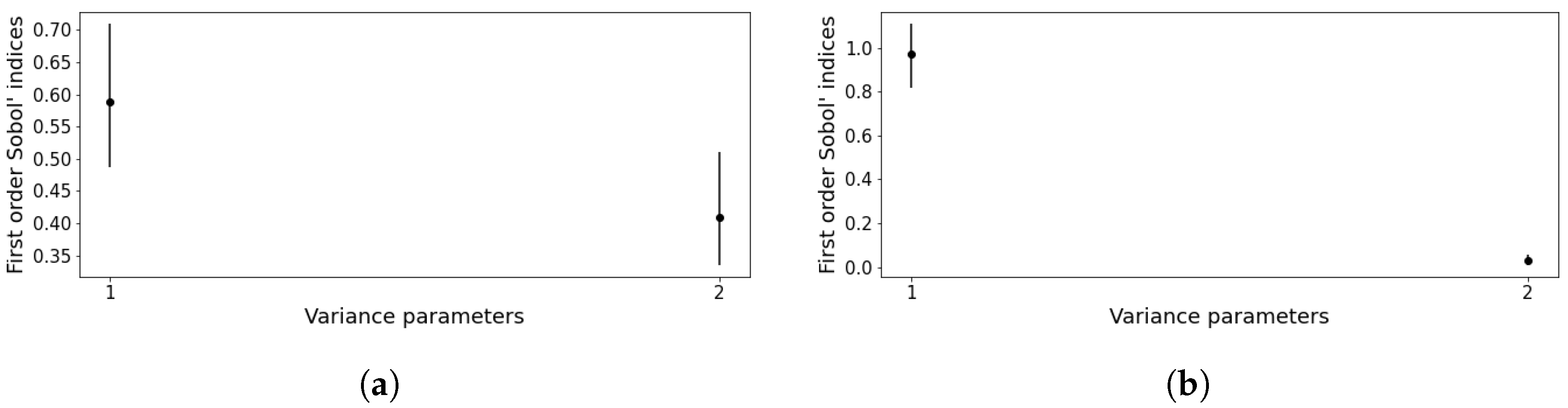
Appendix B. Phase-Plane Equations
- (1)
- Rigid body phase-plane equation.
- (2)
- Flexible mode phase-plane equation.Using Equations (8)–(10), the flexible mode phase-plane equation can be obtained and is written as follows.Using the flexible mode phase-plane equation, the following two relationships can be obtained.
- -
- takes the following form.Additionally, can also be written as follows.
- (3)
- Representing the switching interval time values.
- (4)
- takes the following form.
Appendix C. Linking Process Noise Affected and Unaffected Dynamics
- (a)
- = = = = 0 (or ≈ 0) and
- (b)
- = = = = = = 0 (or ≈ 0) or = = = 0 (or ≈ 0)
Appendix D. A Brief Description of the Model Used
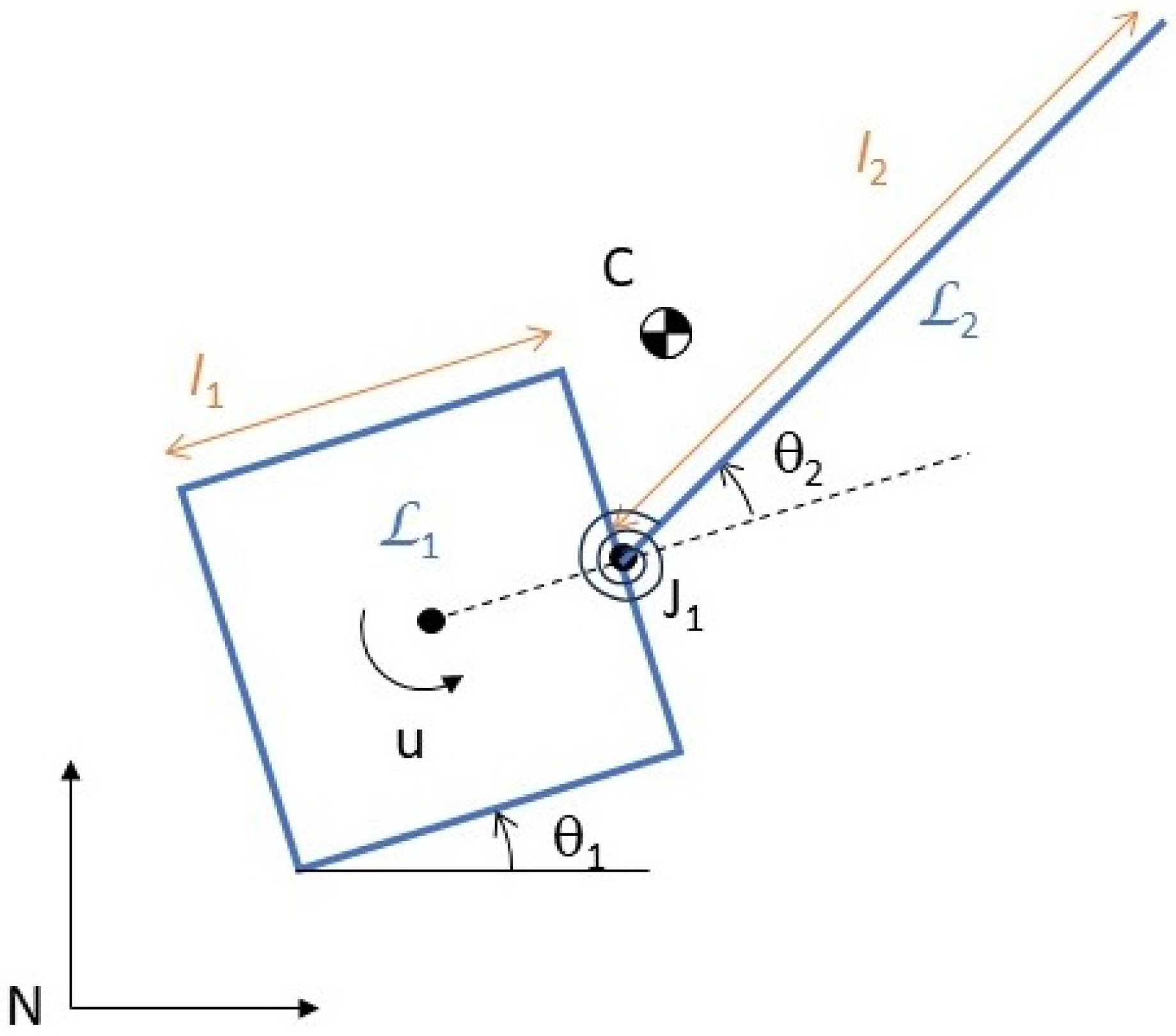
Appendix E. Further Analysis of the Matrix Terms
Appendix F. Further Analysis of the Variance-Based Sensitivity Analysis Terms
References
- Yang, H.; Krishnan, H.; Ang, M. A simple rest-to-rest control command for a flexible link robot. In Proceedings of the International Conference on Robotics and Automation, Albuquerque, NM, USA, 20–25 April 1997; Volume 4, pp. 3312–3317. [Google Scholar] [CrossRef]
- Kim, J.J.; Agrawal, B.N. Rest-to-rest slew maneuver of three-axis rotational flexible spacecraft. IFAC Proc. Vol. 2008, 41, 12054–12060. [Google Scholar] [CrossRef]
- Singh, G.; Kabamba, P.T.; Mcclamroch, N.H. Planar, time-optimal, rest-to-rest slewing maneuvers of flexible spacecraft. J. Guid. Control Dyn. 1989, 12, 71–81. [Google Scholar] [CrossRef]
- Barbieri, E.; Ozguner, U. A new minimum-time control law for a one-mode model of a flexible slewing structure. IEEE Trans. Autom. Control 1993, 38, 142–146. [Google Scholar] [CrossRef]
- Pao, L.Y. Minimum-time control characteristics of flexible structures. J. Guid. Control Dyn. 1996, 19, 123–129. [Google Scholar] [CrossRef]
- Albassam, B.A. Optimal Near-Minimum-Time Control Design for Flexible Structures. J. Guid. Control Dyn. 2002, 25, 618–625. [Google Scholar] [CrossRef][Green Version]
- Hartmann, R.; Singh, T. Fuel/Time Optimal Control of Flexible Space Structures: A Frequency Domain Approach. J. Vib. Control 1999, 5, 795–817. [Google Scholar] [CrossRef]
- Pao, L.Y.; Singhose, W.E. Robust minimum time control of flexible structures. Automatica 1998, 34, 229–236. [Google Scholar] [CrossRef]
- Naidu, S.A.; Leifsson, L.T. Multidisciplinary Spacecraft and Trajectory Uncertainty Quantification for an Interplanetary Mission. In Proceedings of the AIAA SCITECH 2024 Forum, Orlando, FL, USA, 8–12 January 2024. [Google Scholar] [CrossRef]
- Zhang, A.; Wang, Y.; Zhang, Z.; Karimi, H.R.; Sun, W. Robust Control Allocation for Spacecraft Attitude Stabilization under Actuator Faults and Uncertainty. Math. Probl. Eng. 2014, 2014, 789327. [Google Scholar] [CrossRef]
- Federici, L.; Zavoli, A. Robust interplanetary trajectory design under multiple uncertainties via meta-reinforcement learning. Acta Astronaut. 2024, 214, 147–158. [Google Scholar] [CrossRef]
- Yuan, H.; Li, D.; He, G.; Wang, J. Uncertainty-resilient constrained rendezvous trajectory optimization via stochastic feedback control and unscented transformation. Acta Astronaut. 2024, 219, 264–277. [Google Scholar] [CrossRef]
- Cognetti, M.; Salaris, P.; Robuffo Giordano, P. Optimal Active Sensing with Process and Measurement Noise. In Proceedings of the 2018 IEEE International Conference on Robotics and Automation (ICRA), Brisbane, Australia, 21–25 May 2018; pp. 2118–2125. [Google Scholar] [CrossRef]
- Stacey, N.; D’Amico, S. Analytical process noise covariance modeling for absolute and relative orbits. Acta Astronaut. 2022, 194, 34–47. [Google Scholar] [CrossRef]
- Psiaki, M.L.; Klatt, E.M.; Kintner, P.M.; Powell, S.P. Attitude Estimation for a Flexible Spacecraft in an Unstable Spin. J. Guid. Control Dyn. 2002, 25, 88–95. [Google Scholar] [CrossRef][Green Version]
- Vuillod, B.; Montemurro, M.; Panettieri, E.; Hallo, L. A comparison between Sobol’s indices and Shapley’s effect for global sensitivity analysis of systems with independent input variables. Reliab. Eng. Syst. Saf. 2023, 234, 109177. [Google Scholar] [CrossRef]
- Sobol’, I. Global sensitivity indices for nonlinear mathematical models and their Monte Carlo estimates. Math. Comput. Simul. 2001, 55, 271–280. [Google Scholar] [CrossRef]
- Owen, A.B. Variance Components and Generalized Sobol’ Indices. SIAM/ASA J. Uncertain. Quantif. 2013, 1, 19–41. [Google Scholar] [CrossRef]
- Lamboni, M. Uncertainty quantification: A minimum variance unbiased (joint) estimator of the non-normalized Sobol’ indices. Stat. Pap. 2020, 61, 1939–1970. [Google Scholar] [CrossRef]
- Heredia, M.B.; Prieur, C.; Eckert, N. Nonparametric estimation of aggregated Sobol’ indices: Application to a depth averaged snow avalanche model. Int. J. Reliab. Qual. Saf. Eng. 2021, 212, 107422. [Google Scholar] [CrossRef]
- das Neves Carneiro, G.; Antonio, C.C. Sobol’ indices as dimension reduction technique in evolutionary-based reliability assessment. Eng. Comput. 2020, 37, 368–398. [Google Scholar] [CrossRef]
- Iooss, B.; Prieur, C. Shapley effects for sensitivity analysis with correlated inputs: Comparisons with Sobol’ indices, numerical estimation and applications. arXiv 2019. [Google Scholar] [CrossRef]
- Herman, J.; Usher, W. SALib: An open-source Python library for Sensitivity Analysis. J. Open Source Softw. 2017, 2, 97. [Google Scholar] [CrossRef]
- Puy, A.; Piano, S.L.; Saltelli, A.; Levin, S.A. sensobol: An R Package to Compute Variance-Based Sensitivity Indices. J. Stat. Softw. 2022, 102, 1–37. [Google Scholar] [CrossRef]
- Fujii, H.A.; Kojima, H.; Nakajima, N. Slew Maneuver of a Flexible Space Structure with Constraint on Bending Moment. J. Guid. Control Dyn. 2003, 26, 259–266. [Google Scholar] [CrossRef]
- Baum, R.F.; Cesari, L. On a Recent Proof of Pontryagin’s Necessary Conditions. SIAM J. Control 1972, 10, 56–75. [Google Scholar] [CrossRef]
- Lovison, A.; Cardin, F. A Pareto—Pontryagin Maximum Principle for Optimal Control. Symmetry 2022, 14, 1169. [Google Scholar] [CrossRef]
- van Ackooij, W.; Henrion, R.; Zidani, H. Pontryagin’s Principle for Some Probabilistic Control Problems. Appl. Math. Optim. 2024, 90, 5. [Google Scholar] [CrossRef]
- Pao, L.; Franklin, G. Time-optimal control of flexible structures. In Proceedings of the 29th IEEE Conference on Decision and Control, Honolulu, HI, USA, 5–7 December 1990; Volume 5, pp. 2580–2581. [Google Scholar] [CrossRef]
- Ben-Asher, J.; Burns, J.A.; Cliff, E.M. Time optimal slewing of flexible spacecraft. In Proceedings of the 26th IEEE Conference on Decision and Control, Los Angeles, CA, USA, 9 December 1987; Volume 26, pp. 524–528. [Google Scholar] [CrossRef]
- Lasdon, L.; Plummer, J.C. Multistart algorithms for seeking feasibility. Comput. Oper. Res. 2008, 35, 1379–1393. [Google Scholar] [CrossRef]
- Gornov, A.Y.; Zarodnyuk, T.S.; Anikin, A.S.; Finkelstein, E.A. Extension technology and extrema selections in a stochastic multistart algorithm for optimal control problems. J. Glob. Optim. 2020, 76, 533–543. [Google Scholar] [CrossRef]
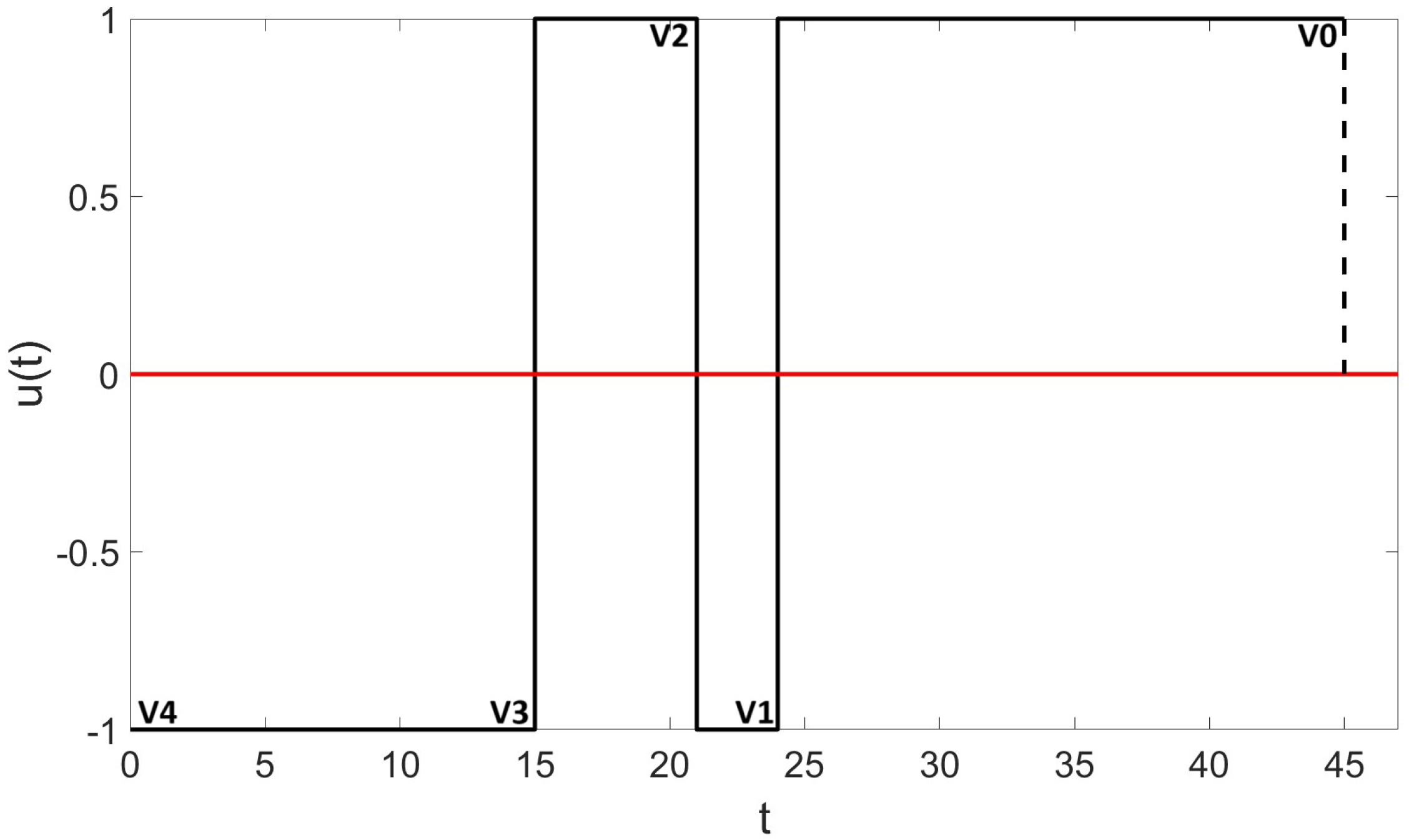


| v | |||||
|---|---|---|---|---|---|
| 4 | 180 | 0 | 0 | 0 | 16.4859 |
| 3 | 93.2246 | 2.3465 | −11.2758 | 0.9291 | 0.4921 |
| 2 | 88.2180 | 2.1068 | −9.4138 | −2.0421 | 1.2450 |
| 1 | 76.1590 | −0.2394 | −10.7210 | −1.0493 | 14.0675 |
| 0 | NA |
| w | ||||
|---|---|---|---|---|
| −3.5 | −17.5570 | 0.0044 | −1.0060 | 0.0779 |
| 0 | ||||
| +3.5 | 17.5570 | −0.0034 | 1.0059 | −0.0778 |
| i | |||||
|---|---|---|---|---|---|
| 1 | 0.01188 | 0.79393 | 0.00300 | 0.19119 | |
| 2 | 0 | 0.79450 | 0.00203 | 0.20347 | |
| 3 | 0 | 0 | 0.59834 | 0.40166 | |
| 4 | 0 | 0 | 0.96893 | 0.03107 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhattacharjee, S. Analyzing the Impact of Process Noise for a Flexible Structure During the Minimum-Time Rest-to-Rest Slew Maneuver. Mathematics 2025, 13, 1144. https://doi.org/10.3390/math13071144
Bhattacharjee S. Analyzing the Impact of Process Noise for a Flexible Structure During the Minimum-Time Rest-to-Rest Slew Maneuver. Mathematics. 2025; 13(7):1144. https://doi.org/10.3390/math13071144
Chicago/Turabian StyleBhattacharjee, Shambo. 2025. "Analyzing the Impact of Process Noise for a Flexible Structure During the Minimum-Time Rest-to-Rest Slew Maneuver" Mathematics 13, no. 7: 1144. https://doi.org/10.3390/math13071144
APA StyleBhattacharjee, S. (2025). Analyzing the Impact of Process Noise for a Flexible Structure During the Minimum-Time Rest-to-Rest Slew Maneuver. Mathematics, 13(7), 1144. https://doi.org/10.3390/math13071144





