A Mathematical Model of Breast Cancer Growth and Drug Resistance Evolution Under Chemotherapy
Abstract
1. Introduction
2. Materials and Methods
2.1. Experimental Setup
2.2. Mathematical Model
- U represents the compartment where the drug is administered;
- Y represents the delay compartment of administered drug;
- Z represents the drug resistance effect;
- X describes the growth of tumor cells;
- V is the tumor volume over time.
2.3. Parameter Estimation
- is the starting time for numerical integration.
- is the final time for numerical integration.
- is the tumor mass volume at time .
2.4. Stability Analysis
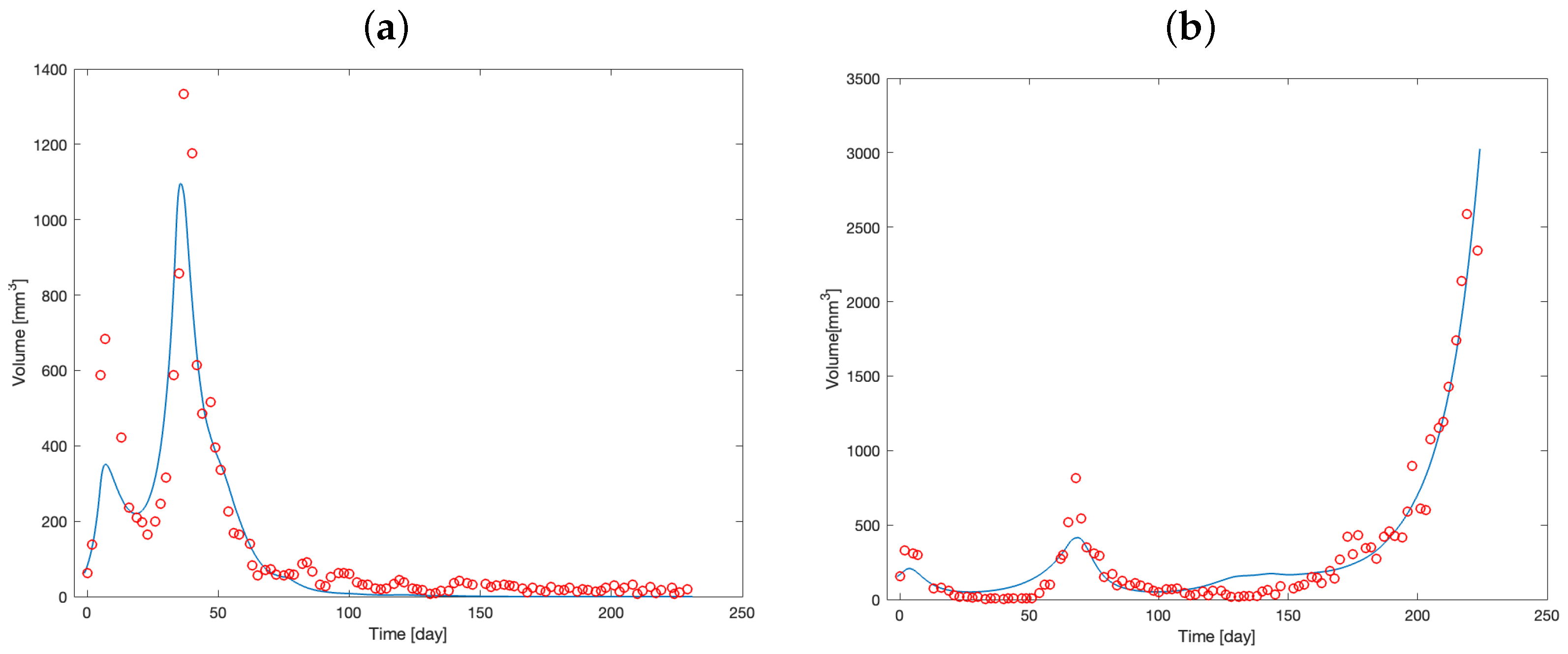
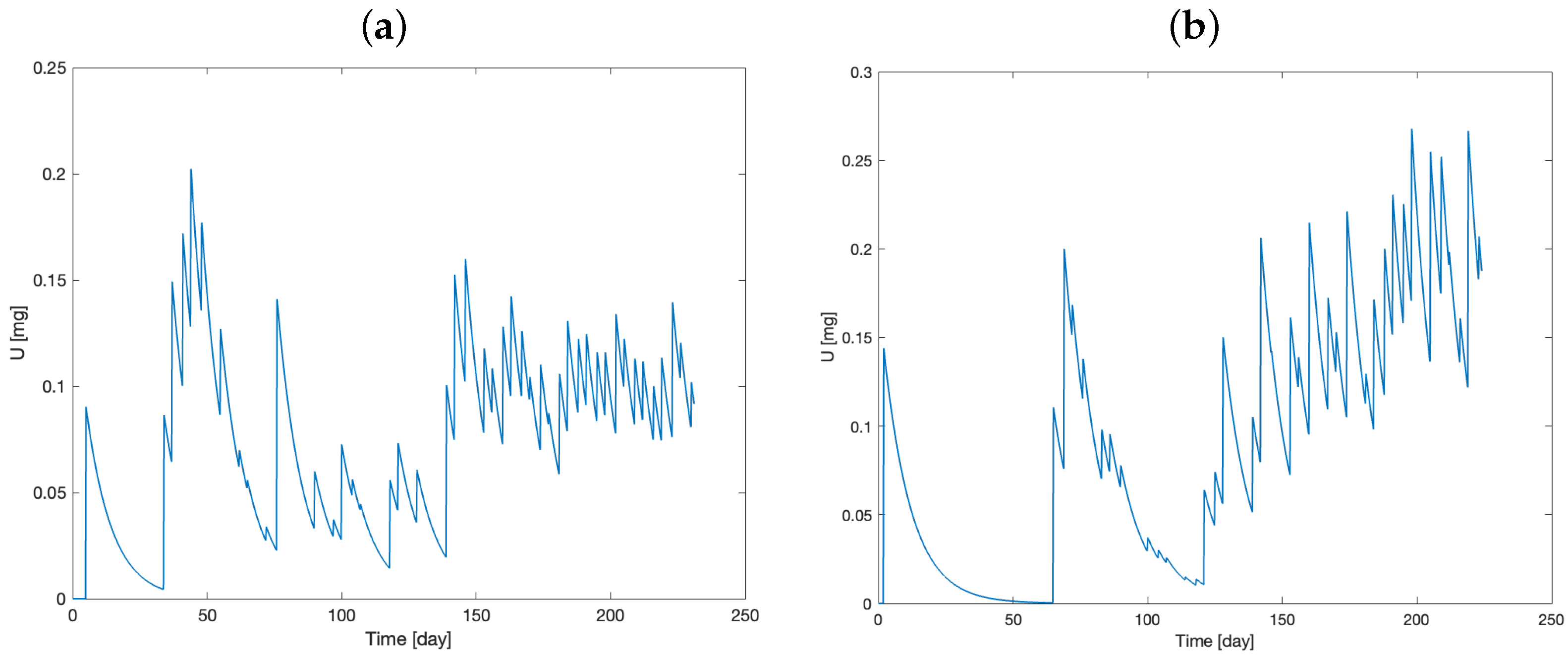
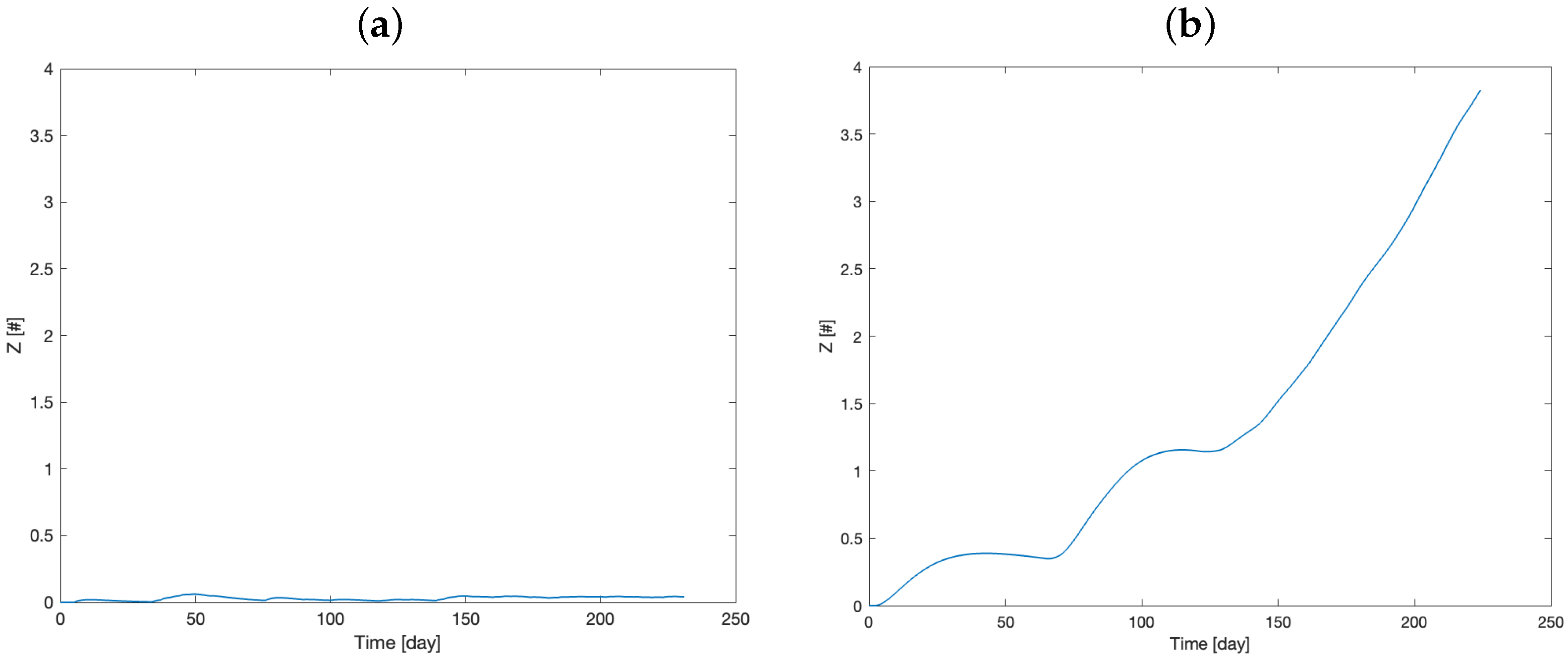
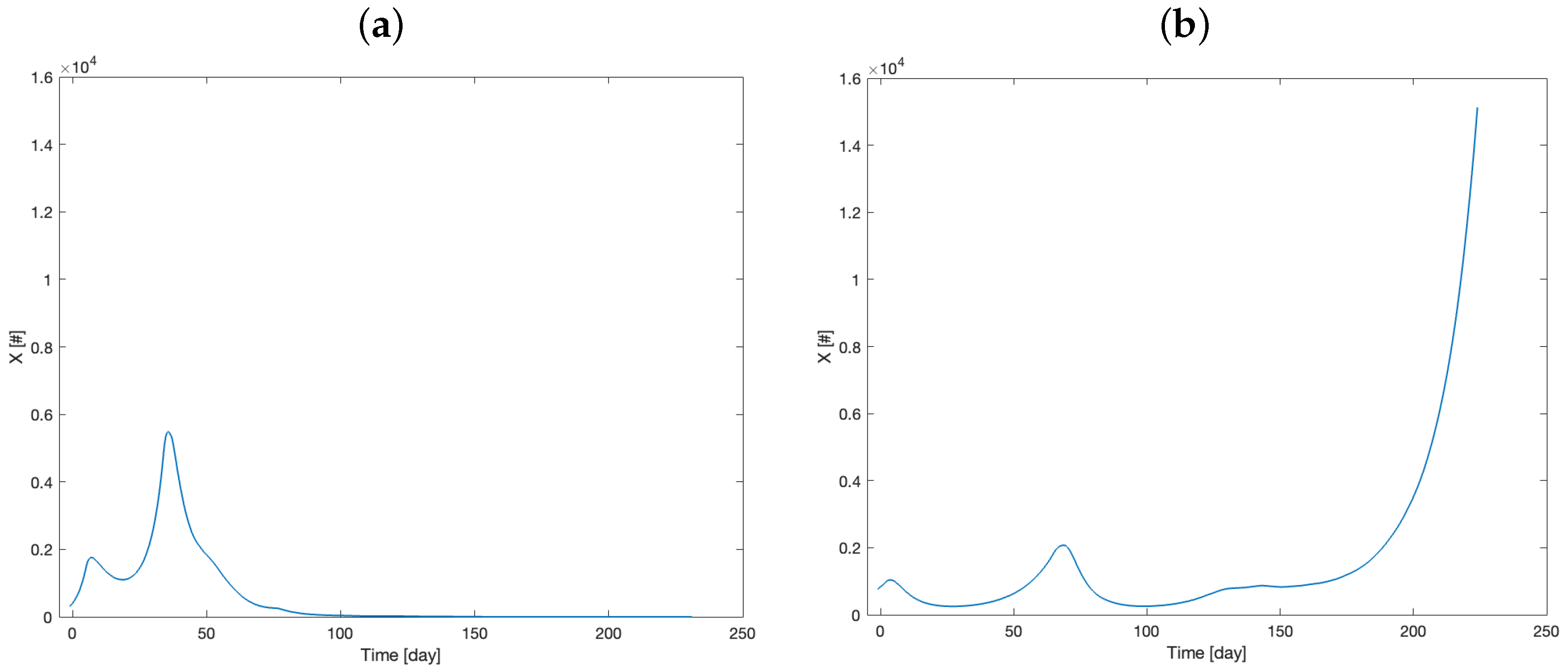
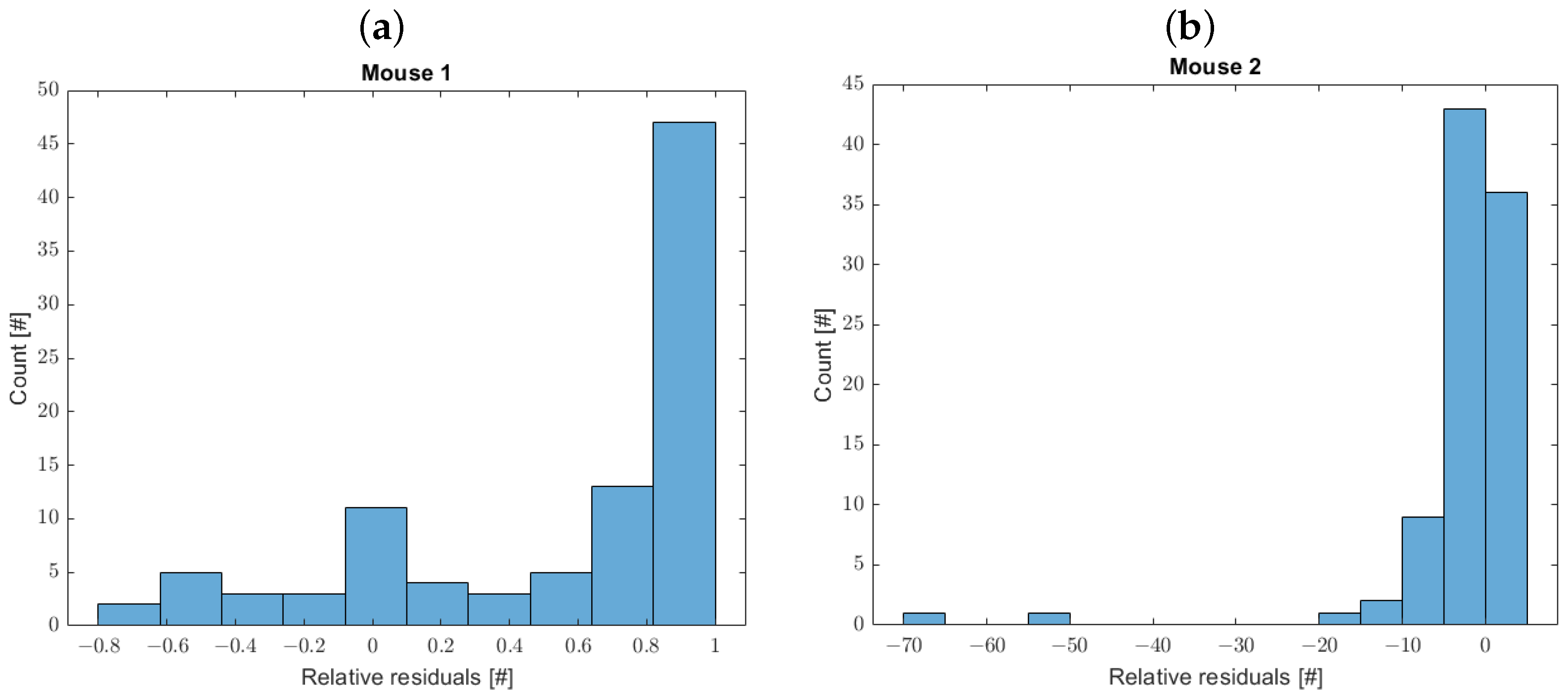
3. Results

4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bartoli, G. Il Tumore al Seno e la sua Prevenzione: Una Survey sulla Consapevolezza della Popolazione. Bachelor’s Thesis, Università Politecnica delle Marche, Rome, Italy, 2021. [Google Scholar]
- Agur, Z.; Elishmereni, M.; Kheifetz, Y. Personalizing oncology treatments by predicting drug efficacy, side-effects, and improved therapy: Mathematics, statistics, and their integration. Wiley Interdiscip. Rev. Syst. Biol. Med. 2014, 6, 239–253. [Google Scholar] [CrossRef] [PubMed]
- Taylor, C.A.; Draney, M.T.; Ku, J.P.; Parker, D.; Steele, B.N.; Wang, K.; Zarins, C.K. Predictive medicine: Computational techniques in therapeutic decision-making. Comput. Aided Surg. Off. J. Int. Soc. Comput. Aided Surg. (ISCAS) 1999, 4, 231–247. [Google Scholar] [CrossRef]
- Young, R.A. Biomedical discovery with DNA arrays. Cell 2000, 102, 9–15. [Google Scholar] [CrossRef] [PubMed]
- Singh, H.; Srivastava, H.M. Mathematical Methods in Medical and Biological Sciences; Elsevier Science & Technology: Amsterdam, The Netherlands, 2024. [Google Scholar]
- Boguski, M.S.; Arnaout, R.; Hill, C. Customized care 2020: How medical sequencing and network biology will enable personalized medicine. F1000 Biol. Rep. 2009, 1, 73. [Google Scholar] [CrossRef]
- Butner, J.D.; Dogra, P.; Chung, C.; Pasqualini, R.; Arap, W.; Lowengrub, J.; Cristini, V.; Wang, Z. Mathematical modeling of cancer immunotherapy for personalized clinical translation. Nat. Comput. Sci. 2022, 2, 785–796. [Google Scholar] [CrossRef]
- Kozłowska, E.; Haltia, U.M.; Puszynski, K.; Färkkilä, A. Mathematical modeling framework enhances clinical trial design for maintenance treatment in oncology. Sci. Rep. 2024, 14, 29721. [Google Scholar] [CrossRef]
- Kosorok, M.R.; Laber, E.B. Precision medicine. Annu. Rev. Stat. Its Appl. 2019, 6, 263–286. [Google Scholar] [CrossRef]
- König, I.R.; Fuchs, O.; Hansen, G.; von Mutius, E.; Kopp, M.V. What is precision medicine? Eur. Respir. J. 2017, 50, 1700391. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, N.; Wang, J.; Wang, Z.; Wu, R. Delivering systems pharmacogenomics towards precision medicine through mathematics. Adv. Drug Deliv. Rev. 2013, 65, 905–911. [Google Scholar] [CrossRef][Green Version]
- Barbolosi, D.; Ciccolini, J.; Lacarelle, B.; Barlési, F.; André, N. Computational oncology—Mathematical modelling of drug regimens for precision medicine. Nat. Rev. Clin. Oncol. 2016, 13, 242–254. [Google Scholar] [CrossRef]
- Ochieng, F.O. Mathematical Modeling of Cancerous Tumor Evolution Incorporating Drug Resistance. Eng. Rep. 2025, 7, e70021. [Google Scholar] [CrossRef]
- Kovács, L.; Ferenci, T.; Gombos, B.; Füredi, A.; Rudas, I.; Szakács, G.; Drexler, D.A. Positive Impulsive Control of Tumor Therapy—A Cyber-Medical Approach. IEEE Trans. Syst. Man Cybern. Syst. 2024, 54, 597–608. [Google Scholar] [CrossRef]
- Scolaris, L. Analisi e Modellizzazione Tramite Equazioni Differenziali della Crescita e della Risposta All’immunoterapia del Glioblastoma Multiforme [Analysis and Modellization Using Differential Equations of the Growth and Response to Immunotherapy of Glioblastoma Multiforme]. Ph.D. Thesis, Politecnico di Torino, Torino, Italy, 2024. [Google Scholar]
- Enderling, H.; Chaplain, M.A.; Anderson, A.R.; Vaidya, J.S. A mathematical model of breast cancer development, local treatment and recurrence. J. Theor. Biol. 2007, 246, 245–259. [Google Scholar] [CrossRef] [PubMed]
- Raguz, S.; Yagüe, E. Resistance to chemotherapy: New treatments and novel insights into an old problem. Br. J. Cancer 2008, 99, 387–391. [Google Scholar] [CrossRef]
- Coley, H.M. Mechanisms and strategies to overcome chemotherapy resistance in metastatic breast cancer. Cancer Treat. Rev. 2008, 34, 378–390. [Google Scholar] [CrossRef]
- Pompa, M.; Drexler, D.A.; Panunzi, S.; Gombos, B.; Füredi, A.; Szakács, G.; Kovács, L.; De Gaetano, A. Modeling the development of drug resistance during chemotherapy treatment. In Proceedings of the 2024 IEEE 63rd Conference on Decision and Control (CDC), Milan, Italy, 16–19 December 2024; IEEE: Piscataway, NJ, USA, 2024; pp. 4804–4810. [Google Scholar] [CrossRef]
- Liu, X.; Holstege, H.; van der Gulden, H.; Treur-Mulder, M.; Zevenhoven, J.; Velds, A.; Kerkhoven, R.M.; van Vliet, M.H.; Wessels, L.F.; Peterse, J.L.; et al. Somatic loss of BRCA1 and p53 in mice induces mammary tumors with features of human BRCA1-mutated basal-like breast cancer. Proc. Natl. Acad. Sci. USA 2007, 104, 12111–12116. [Google Scholar] [CrossRef]
- Rottenberg, S.; Nygren, A.O.; Pajic, M.; van Leeuwen, F.W.; van der Heijden, I.; van de Wetering, K.; Liu, X.; de Visser, K.E.; Gilhuijs, K.G.; Van Tellingen, O.; et al. Selective induction of chemotherapy resistance of mammary tumors in a conditional mouse model for hereditary breast cancer. Proc. Natl. Acad. Sci. USA 2007, 104, 12117–12122. [Google Scholar] [CrossRef]
- Füredi, A.; Szebényi, K.; Tóth, S.; Cserepes, M.; Hámori, L.; Nagy, V.; Karai, E.; Vajdovich, P.; Imre, T.; Szabó, P.; et al. Pegylated liposomal formulation of doxorubicin overcomes drug resistance in a genetically engineered mouse model of breast cancer. J. Control. Release 2017, 261, 287–296. [Google Scholar] [CrossRef]
- McNally, A. Using ODEs to Model Drug Concentrations Within the Field of Pharmacokinetics; Augustana College: Rock Island, IL, USA, 2016. [Google Scholar]
- Singer, S.; Singer, S. Efficient implementation of the Nelder–Mead search algorithm. Appl. Numer. Anal. Comput. Math. 2004, 1, 524–534. [Google Scholar] [CrossRef]
- Fernández, M.S.B. MATLAB implementation for evaluation of measurements by the generalized method of least squares. Measurement 2018, 114, 218–225. [Google Scholar] [CrossRef]
- Eckerling, A.; Ricon-Becker, I.; Sorski, L.; Sandbank, E.; Ben-Eliyahu, S. Stress and cancer: Mechanisms, significance and future directions. Nat. Rev. Cancer 2021, 21, 767–785. [Google Scholar] [CrossRef]
- Eng, J.W.L.; Kokolus, K.M.; Reed, C.B.; Hylander, B.L.; Ma, W.W.; Repasky, E.A. A nervous tumor microenvironment: The impact of adrenergic stress on cancer cells, immunosuppression, and immunotherapeutic response. Cancer Immunol. Immunother. 2014, 63, 1115–1128. [Google Scholar] [CrossRef]
- Ho, V.W.; Leung, K.; Hsu, A.; Luk, B.; Lai, J.; Shen, S.Y.; Minchinton, A.I.; Waterhouse, D.; Bally, M.B.; Lin, W.; et al. A low carbohydrate, high protein diet slows tumor growth and prevents cancer initiation. Cancer Res. 2011, 71, 4484–4493. [Google Scholar] [CrossRef] [PubMed]


| Variables | Units | Meaning |
|---|---|---|
| t | day | Time in days |
| U | mg | Admistration compartment |
| Y | mg | Delay admistration compartment |
| Z | # | Therapy resistance |
| X | # | Number of tumor cells |
| V | mm3 | Tumor Volume |
| Parameter | Units | Meaning |
|---|---|---|
| day | Starting time for numerical integration | |
| day | Final time for numerical integration | |
| day | Time integration step | |
| 1/day | Drug transport rate from U to Y | |
| mg | Starting value for the compartment U (at ) | |
| 1/day | Elimination rate of Y | |
| mg | Starting value for Y (at ) | |
| 1/day | Growth rate of drug resistance | |
| 1/day | Drug resistance clearance | |
| # | Starting value for the compartment Z (at ) | |
| 1/day | Growth rate of X | |
| 1/day | Spontaneous elimination rate of X | |
| 1/day per mg | Drug effect on X elimination | |
| # | Sensitivity of X to therapy resistance | |
| mm3 per million of cells | Conversion factor of cell number to volume | |
| mm3 | Starting value for V (at ) | |
| # | Starting value for X (at ) | |
| mg per kg | Amount of administered drug at time per kg of mouse | |
| kg | Mass of mouse at time |
| Parameter | Mouse 1 | Mouse 2 | Type |
|---|---|---|---|
| 231 | 224 | Fixed | |
| 0.2 | 0.2 | Fixed | |
| 0.1052 | 0.0992 | Free | |
| 0 | 0 | Fixed | |
| 0.2411 | 0.1011 | Free | |
| 0 | 0 | Fixed | |
| 12.6499 | 0.3706 | Free | |
| 13.8894 | 0.0093 | Free | |
| 0 | 0 | Fixed | |
| 0.3036 | 0.1264 | Free | |
| 0.047 | 0.047 | Calibrated | |
| 26.7097 | 3.9527 | Free | |
| 29.5415 | 1.0811 | Free | |
| 0.2 | 0.2 | Fixed | |
| 61.9962 | 153.827 | Fixed | |
| 309.9810 | 769.1350 | Determined |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pompa, M.; Urso, G.; Panunzi, S.; Drexler, D.A.; Gombos, B.; De Gaetano, A. A Mathematical Model of Breast Cancer Growth and Drug Resistance Evolution Under Chemotherapy. Mathematics 2025, 13, 1115. https://doi.org/10.3390/math13071115
Pompa M, Urso G, Panunzi S, Drexler DA, Gombos B, De Gaetano A. A Mathematical Model of Breast Cancer Growth and Drug Resistance Evolution Under Chemotherapy. Mathematics. 2025; 13(7):1115. https://doi.org/10.3390/math13071115
Chicago/Turabian StylePompa, Marcello, Giulia Urso, Simona Panunzi, Dániel András Drexler, Balázs Gombos, and Andrea De Gaetano. 2025. "A Mathematical Model of Breast Cancer Growth and Drug Resistance Evolution Under Chemotherapy" Mathematics 13, no. 7: 1115. https://doi.org/10.3390/math13071115
APA StylePompa, M., Urso, G., Panunzi, S., Drexler, D. A., Gombos, B., & De Gaetano, A. (2025). A Mathematical Model of Breast Cancer Growth and Drug Resistance Evolution Under Chemotherapy. Mathematics, 13(7), 1115. https://doi.org/10.3390/math13071115







